Enhancing the Permeate Flux of Direct Contact Membrane Distillation Modules with Inserting 3D Printing Turbulence Promoters
Abstract
1. Introduction
2. Experimental Setup and Materials
3. Theoretical Formulations
3.1. Mass and Heat Transfer
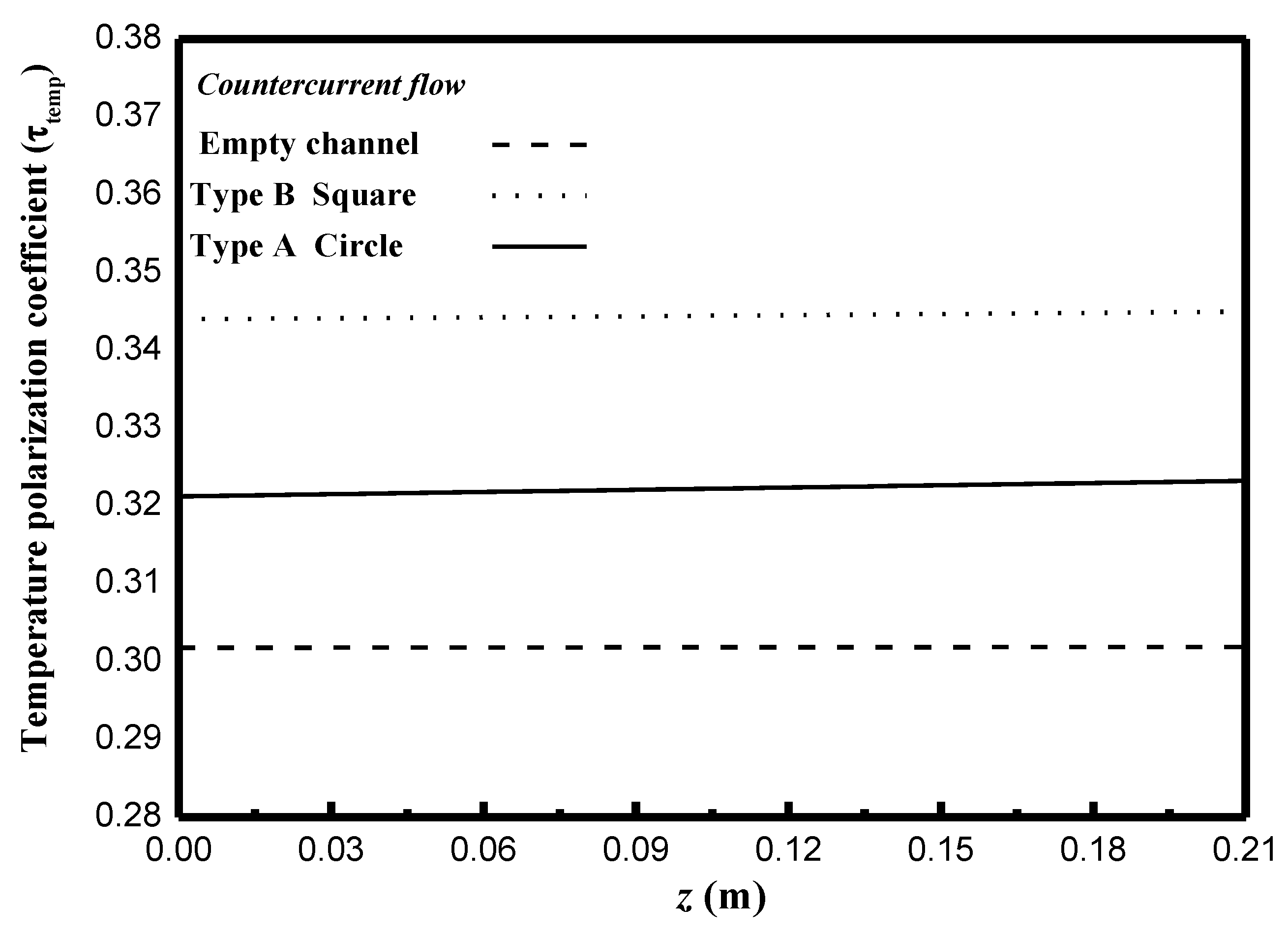
3.2. Temperature Polarization
3.3. Enhancement Factor
3.4. Power Consumption Increment
3.5. The Design of 3D Printed Turbulence Promoters
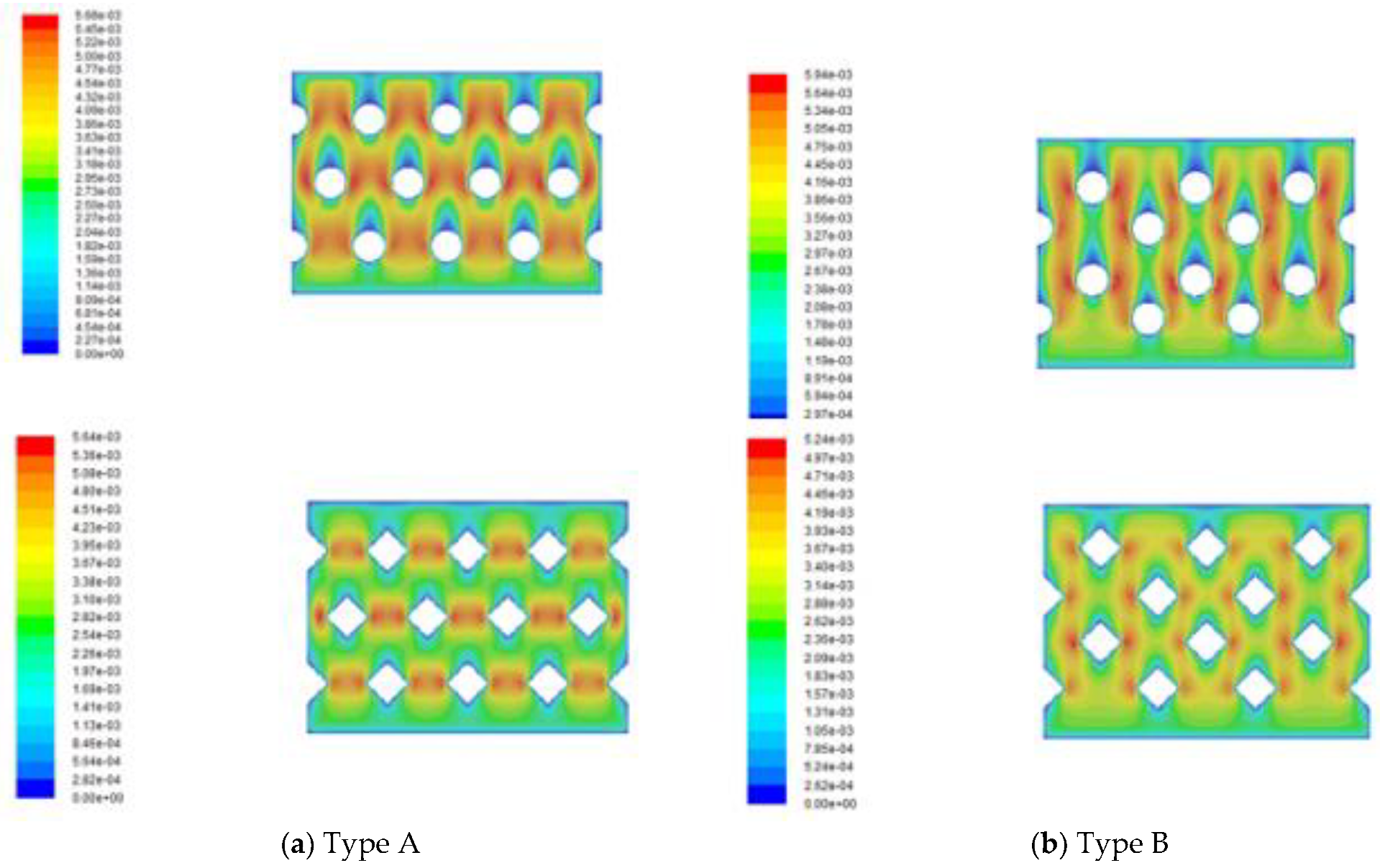
3.6. CFD Simulation
3.7. Numerical Scheme
4. Results and Discussion
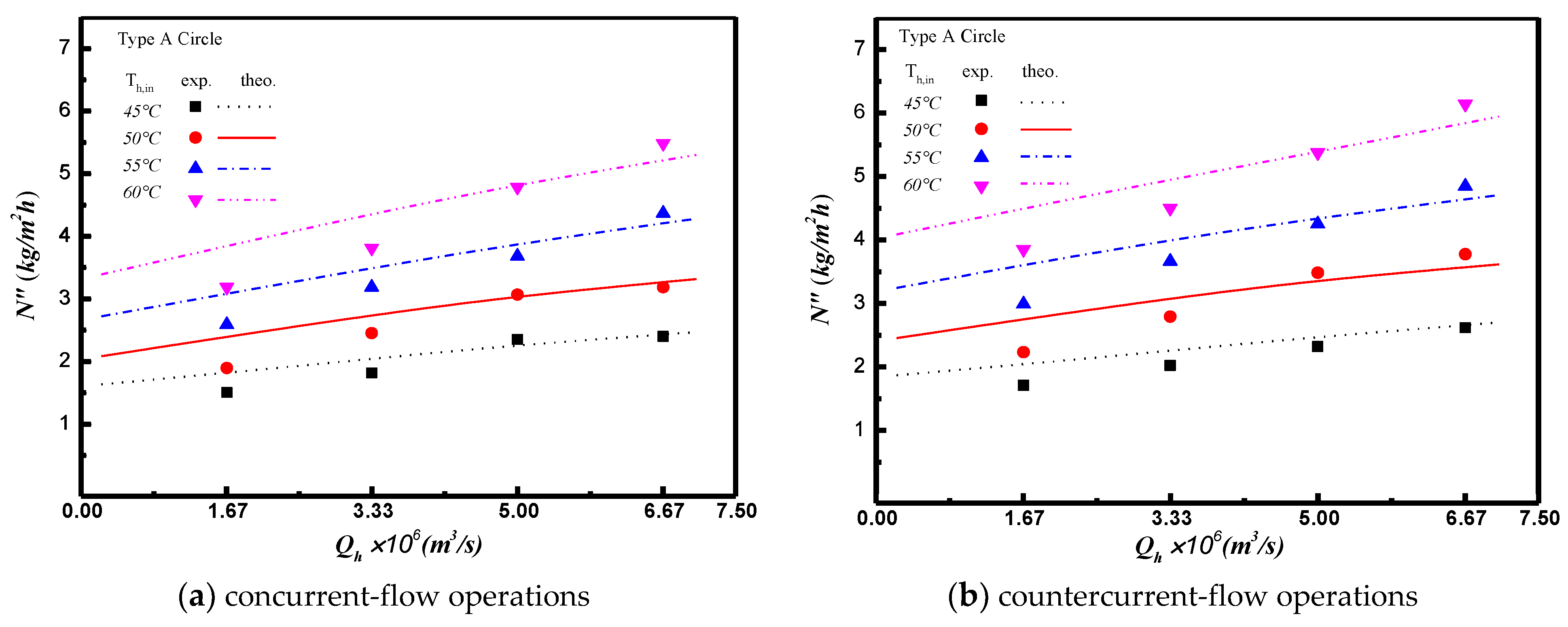
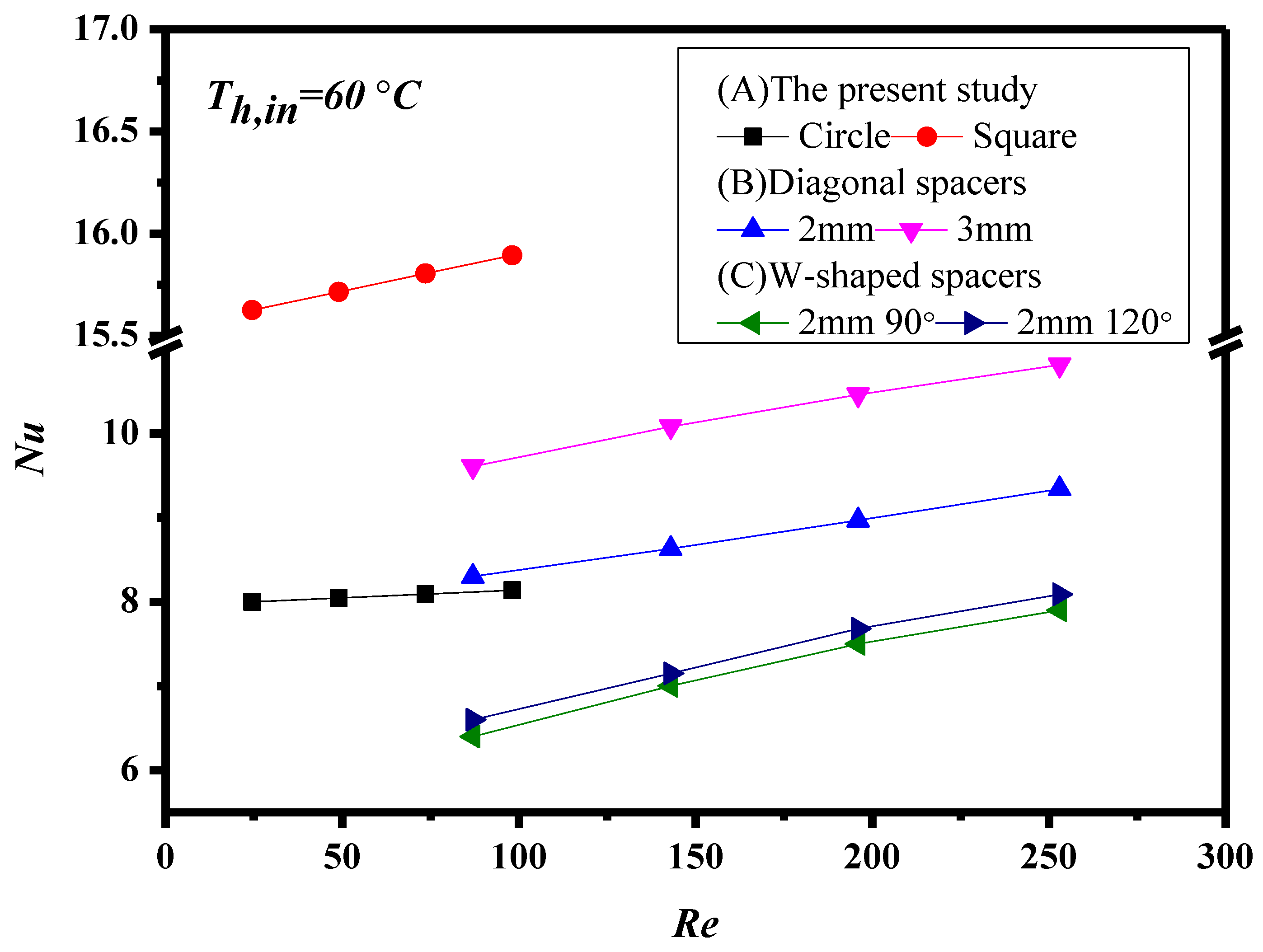
4.1. Flux Enhancement by Inserting Turbulence Promoter in DCMD Module
4.2. Analysis of Cake Properties
4.3. Energy Consumption
5. Conclusions
- The higher inlet hot feed temperature with the array pattern of Type B result in a higher permeate flux for both concurrent- and countercurrent-flow operations. A maximum of 61.7% permeate flux enhancement was found in the device with inserting turbulence promoters compared to that in the empty channel device.
- The permeate flux is principally driven by the temperature gradient between both membrane surfaces along the flowing direction. A higher permeate flux is achieved in countercurrent-flow operations than that in concurrent-flow operations due to the larger temperature gradient for countercurrent-flow operations.
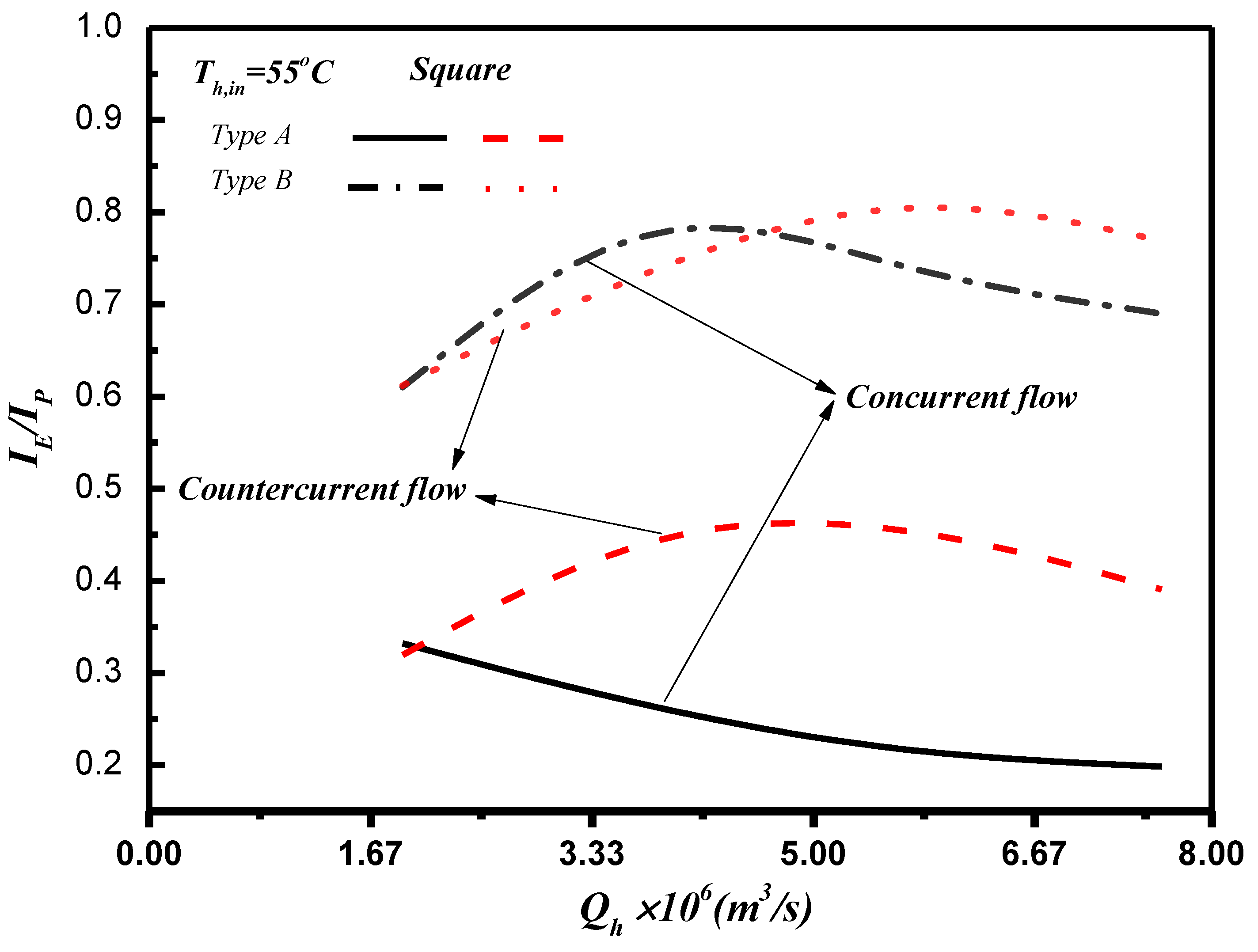
- The ratio of permeate flux enhancement to power consumption increment was used to examine the economic viewpoint to increase pure water productivity in the present implementation. The economic consideration concluded that the power utilization is more effective for the channel with inserting turbulence promoters, and the optimal value of was obtained at some hot feed flow rate between and .
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Abbreviations
| Water activity in NaCl solution | |
| Heat capacity of cold fluid (J kg−1K−1) | |
| Heat capacity of hot fluid (J kg−1K−1) | |
| Mass transfer coefficient of membrane (kg m−2 Pa−1 s−1) | |
| Equivalent hydraulic diameter of empty channel (m) | |
| Equivalent hydraulic diameter of hot side (m) | |
| Equivalent hydraulic diameter of cold side (m) | |
| Height of flow channel (m) | |
| Height of 3D printed turbulence promoter (m) | |
| Deviation of experimental results from the theoretical predictions | |
| Fanning friction factor | |
| Convection coefficient of cold fluid (W m−2 K−1) | |
| Convection coefficient of hot fluid (W m−2 K−1) | |
| Hydraulic dissipate energy (J kg−1), | |
| Thermal convection coefficient of membrane (W m−2 K−1) | |
| Raised percentage of permeate flux | |
| Raised percentage of hydraulic loss | |
| Axial distance (m) | |
| Thermal conductivity coefficient of hot saline feed (W m−1 K−1) | |
| Thermal conductivity coefficient of the vapor in the membrane pore (W m−1 K−1) | |
| Thermal conductivity coefficient of the solid membrane material (W m−1 K−1) | |
| Friction loss of conduits (J kg−1) | |
| Molecular weight of water (kg mol−1) | |
| Mass flow rate (kg s−1) | |
| Permeate flux (kg m−2 h−1) | |
| Nusselt number | |
| Nusselt number of the turbulence promoter | |
| Dimensionless Nusselt number for laminar flow | |
| np | Number of promoters per row |
| Pressure (Pa) | |
| Saturation vapor pressure in the cold feed flow side (Pa) | |
| Saturation vapor pressure in the hot feed flow side (Pa) | |
| Saturated vapor pressure of pure water (Pa) | |
| Prandtl number | |
| Heat transfer rate (W/m2) | |
| Heat transfer rate between cooling plate and cold fluid (W/m2) | |
| Heat transfer rate between hot fluid and membrane surface (W/m2) | |
| Heat transfer rate between membrane surface of hot fluid and air gap (W/m2) | |
| Volumetric flow rate (m3 s−1) | |
| Gas constant (8.314 J mol−1 K−1) | |
| Re | Reynolds number |
| Dimensionless Schmidt number for laminar flow | |
| The precision index of an experimental measurements of permeate flux (kg m−2 h−1) | |
| The mean value of (kg m−2 h−1) | |
| Temperature (°C) | |
| Mean temperature in membrane (°C) | |
| Average velocity (m s−1) | |
| Average equivalent width of 3D printed turbulence promoters | |
| Natural log mean mole fraction of air | |
| Liquid mole fraction of NaCl | |
| Liquid mole fraction of water | |
| Axial coordinate along flow direction (m) | |
| Greek letters | |
| Heat-transfer enhancement factor | |
| Aspect ratio of the channel | |
| Vapor pressure difference of membrane (Pa) | |
| Thickness of membrane (µm) | |
| Membrane porosity | |
| Latent heat of water (J/kg) | |
| Fluid viscosity (kg s−1 m−1) | |
| Density (kg m−3) | |
| Temperature polarization coefficients | |
| Subscripts | |
| 1 | Membrane surface on cold feed side |
| 2 | Membrane surface on hot feed side |
| h | In the hot feed flow channel |
| c | In the cold feed flow channel |
| promoter | Inserting turbulence promoters |
| empty | Inserting nylon fiber as supporters |
| exp | Experimental results |
| in | Inlet |
| lam | Empty channel |
| out | Outlet |
| theo | Theoretical predictions |
| Superscripts | |
| p | The channel with inserting turbulence promoters |
References
- Lawson, K.W.; Lloyd, D.R. Membrane distillation. II. Direct contact MD. J. Membr. Sci. 1996, 120, 123–133. [Google Scholar] [CrossRef]
- Khayet, M. Membranes and theoretical modeling of membrane distillation: A review. Adv. Colloid Interface Sci. 2011, 164, 56–88. [Google Scholar] [CrossRef]
- Al-Obaidani, S.; Curcio, E.; Macedonio, F.; Di Profio, G.; Al-Hinai, H.; Drioli, E. Potential of membrane distillation in sea-water desalination: Thermal efficiency, sensitivity study and cost estimation. J. Membr. Sci. 2008, 323, 85–98. [Google Scholar] [CrossRef]
- Schofield, R.W.; Fane, A.G.; Fell, C.J.D. Heat and mass transfer in membrane distillation. J. Membr. Sci. 1987, 33, 299–313. [Google Scholar] [CrossRef]
- Martinez-Diez, L.; Vázquez-González, M.I. Effects of Polarization on Mass Transport through Hydrophobic Porous Membranes. Ind. Eng. Chem. Res. 1998, 37, 4128–4135. [Google Scholar] [CrossRef]
- Alkhudhiri, A.; Darwish, N.; Hilal, N. Membrane distillation: A comprehensive review. Desalination 2012, 287, 2–18. [Google Scholar] [CrossRef]
- Drioli, E.; Ali, A.; Macedonio, F. Membrane distillation: Recent developments and perspectives. Desalination 2015, 356, 56–84. [Google Scholar] [CrossRef]
- Iversen, S.B.; Bhatia, V.K.; Dam-Jphasen, K.; Jonsson, G. Characterization of microporous membranes for use in membrane contactors. J. Membr. Sci. 1997, 130, 205–217. [Google Scholar] [CrossRef]
- Lee, J.G.; Kim, Y.D.; Kim, W.S.; Francis, L.; Amy, G.; Ghaffour, N. Performance modeling of direct contact membrane distil-lation (DCMD) seawater desalination process using a commercial composite membrane. J. Membr. Sci. 2015, 478, 85–95. [Google Scholar] [CrossRef]
- Chen, L.H.; Huang, A.; Chen, Y.R.; Chen, C.H.; Hsu, C.C.; Tsai, F.Y.; Tung, K.L. Omniphobic membranes for direct contact membrane distillation: Effective deposition of zinc oxide nanoparticles. Desalination 2018, 428, 255–263. [Google Scholar] [CrossRef]
- Ali, A.; Macedonio, F.; Drioli, E.; Aljlil, S.; Alharbi, O.A. Experimental and theoretical evaluation of temperature polariza-tion phenomenon in direct contact membrane distillation. Chem. Eng. Res. Des. 2013, 91, 1966–1977. [Google Scholar] [CrossRef]
- Ho, C.D.; Chang, H.; Chang, C.L.; Huang, C.H. Theoretical and experimental studies of flux enhancement with roughened surface in direct contact membrane distillation desalination. J. Membr. Sci. 2013, 433, 160–166. [Google Scholar] [CrossRef]
- Martínez-Díez, L.; Vázquez-González, M.I.; Florido-Díaz, F.J. Study of membrane distillation using channel spacers. J. Membr. Sci. 1998, 144, 45–56. [Google Scholar] [CrossRef]
- Phattaranawik, J.; Jiraratananon, R.; Fane, A.G. Effects of net-type spacers on heat and mass transfer in direct contact mem-brane distillation and comparison with ultrafiltration studies. J. Membr. Sci. 2003, 217, 193–206. [Google Scholar] [CrossRef]
- Santos JL, C.; Geraldes, V.; Velizarov, S.; Crespo, J.G. Investigation of flow patterns and mass transfer in membrane mod-ule channels filled with flow-aligned spacers using computational fluid dynamics (CFD). J. Membr. Sci. 2007, 305, 103–117. [Google Scholar] [CrossRef]
- Ho, C.D.; Chang, H.; Tsai, C.H.; Lin, P.H. Theoretical and experimental studies of a compact multi-unit direct contact membrane distillation module. I & EC Res. 2016, 55, 5385–5394. [Google Scholar]
- Yang, X.; Yu, H.; Wang, R.; Fane, A.G. Analysis of the effect of turbulence promoters in hollow fiber membrane distillation modules by computational fluid dynamic (CFD) simulations. J. Membr. Sci. 2012, 415–416, 758–769. [Google Scholar] [CrossRef]
- Armbruster, S.; Cheong, O.; Lölsberg, J.; Popovic, S.; Yüce, S.; Wessling, M. Fouling mitigation in tubular membranes by 3D-printed turbulence promoters. J. Membr. Sci. 2018, 554, 156–163. [Google Scholar] [CrossRef]
- Luelf, T.; Rall, D.; Wypysek, D.; Wiese, M.; Femmer, T.; Bremer, C.; Michaelis, J.U.; Wessling, M. 3D-printed rotating spin-nerets create membranes with a twist. J. Membr. Sci. 2018, 555, 7–19. [Google Scholar] [CrossRef]
- Lee, J.Y.; Tan, W.S.; An, J.; Chua, C.K.; Tang, C.Y.; Fane, A.G.; Chong, T.H. The potential to enhance membrane module de-sign with 3D printing technology. J. Membr. Sci. 2016, 499, 480–490. [Google Scholar] [CrossRef]
- Tsai, H.Y.; Huang, A.; Soesanto, J.F.; Luo, Y.L.; Hsu, T.Y.; Chen, C.H.; Hwang, K.J.; Ho, C.D.; Tung, K.L. 3D printing design of turbulence promoters in a cross-flow microfiltration system for fine particles removal. J. Membr. Sci. 2019, 573, 647–656. [Google Scholar] [CrossRef]
- Lawson, K.W.; Lloyd, D.R. Membrane distillation. J. Membr. Sci. 1997, 124, 1–25. [Google Scholar] [CrossRef]
- Schofield, R.W.; Fane, A.G.; Fell, C.J.D. Gas and vapor transport through microporous membranes. I. Knudsen-Poiseuille transition. J. Membr. Sci. 1990, 53, 159–171. [Google Scholar] [CrossRef]
- Warner, S.B. Fiber Science; Princeton-Hall: Englewood Cliffs, NJ, USA, 1995. [Google Scholar]
- Phattaranawik, J.; Jiraratananon, R.; Fane, A.G. Heat transport and membrane distillation coefficients in direct contact membrane distillation. J. Membr. Sci. 2003, 212, 177–193. [Google Scholar] [CrossRef]
- Welty, J.R.; Wicks, C.E.; Wilson, R.E. Fundamentals of Momentum, Heat, and Mass Transfer, 3rd ed.; John Wiley & Sons: New York, NY, USA, 1984. [Google Scholar]
- Kandlikar, S.G.; Schmitt, D.; Carrano, A.L.; Taylor, J.B. Characterization of surface roughness effects on pressure drop in single-phase flow in mini channels. Phys. Fluids 2005, 17, 100606–100616. [Google Scholar] [CrossRef]
- Dimitrov, D.; Schreve, K.; De Beer, N. Advances in three dimensional printing—State of the art and future perspectives. Rapid Prototyp. J. 2006, 12, 136–147. [Google Scholar] [CrossRef]
- Shakaib, M.; Hasani, S.M.F.; Ahmed, I.; Yunus, R.M. A CFD study on the effect of spacer orientation on temperature polari-zation in membrane distillation modules. Desalination 2012, 284, 332–340. [Google Scholar] [CrossRef]
- Moffat, R.J. Describing the uncertainties in experimental results. Exp. Therm. Fluid Sci. 1988, 1, 3–17. [Google Scholar] [CrossRef]
- Ho, C.-D.; Chen, L.; Lai, J.-Y.; Ng, C.A. Theoretical and experimental studies of direct contact membrane distillation modules with inserting W-shaped carbon-fiber spacers. DESALINATION Water Treat. 2017, 71, 32–44. [Google Scholar] [CrossRef]



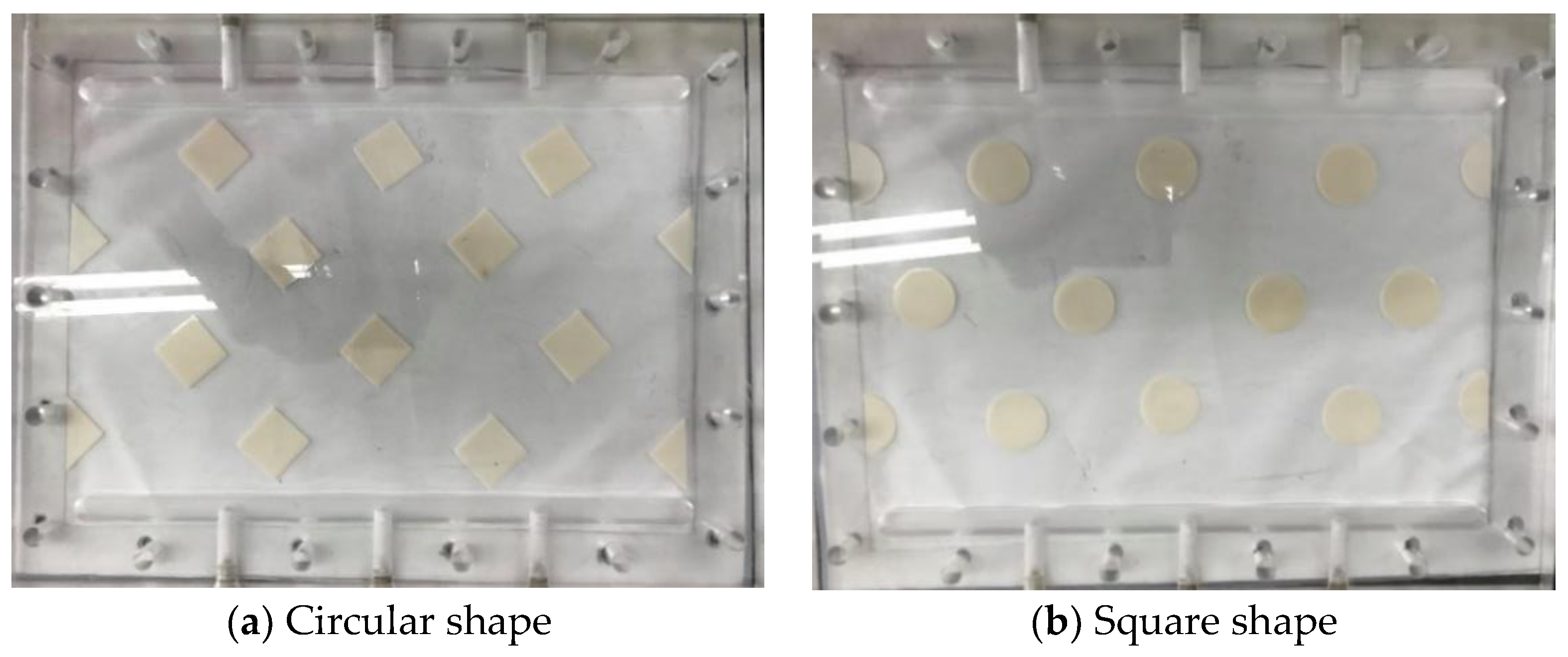


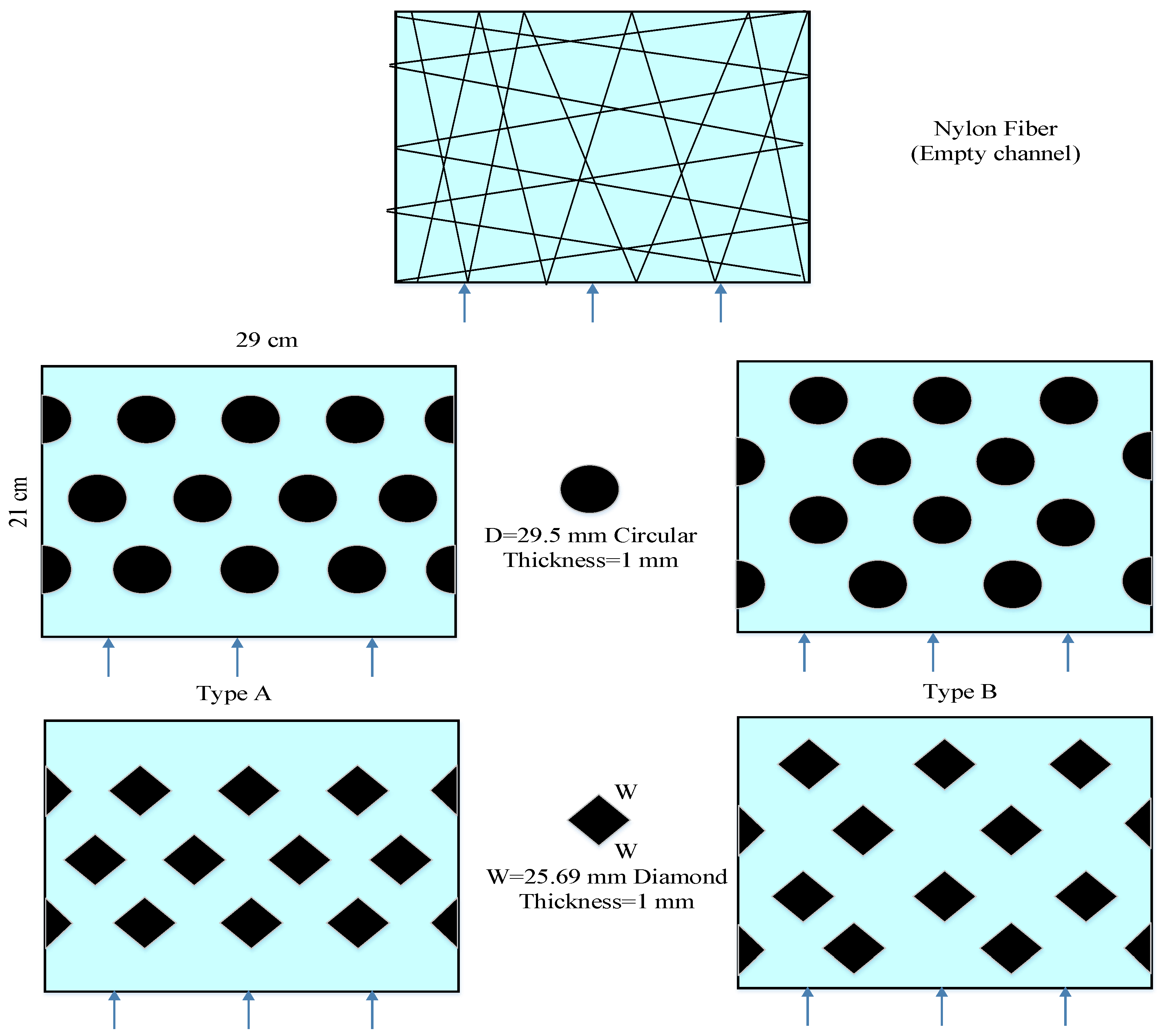




(°C) | (m3 s−1) | Empty Channel | Circle | Square | ||||||
|---|---|---|---|---|---|---|---|---|---|---|
| Type A | Type B | Type A | Type B | |||||||
(kg m−2 h−1) | (kg m−2 h−1) | (%) | (kg m−2 h−1) | (%) | (kg m−2 h−1) | (%) | (kg m−2 h−1) | (%) | ||
| 55 | 1.67 | 1.92 | 2.59 | 34.8 | 2.68 | 39.5 | 2.23 | 16.1 | 2.79 | 45.3 |
| 3.33 | 2.35 | 3.19 | 35.7 | 3.38 | 43.8 | 2.65 | 12.7 | 3.80 | 61.7 | |
| 5.0 | 2.79 | 3.68 | 31.8 | 4.18 | 35.8 | 3.09 | 10.7 | 4.30 | 54.3 | |
| 6.67 | 3.25 | 4.37 | 34.4 | 4.72 | 45.2 | 3.58 | 10.1 | 4.91 | 51.2 | |
| 60 | 1.67 | 2.41 | 3.19 | 32.0 | 3.44 | 42.7 | 2.72 | 12.8 | 3.49 | 44.8 |
| 3.33 | 2.97 | 3.81 | 28.2 | 4.06 | 36.7 | 3.33 | 12.1 | 4.38 | 47.4 | |
| 5.0 | 3.50 | 4.79 | 36.8 | 5.22 | 49.1 | 4.21 | 20.2 | 5.50 | 57.2 | |
| 6.67 | 4.02 | 5.48 | 36.3 | 5.88 | 46.3 | 4.79 | 19.2 | 5.59 | 49.5 | |
(°C) | (m3 s−1) | Empty Channel | Circle | Square | ||||||
|---|---|---|---|---|---|---|---|---|---|---|
| Type A | Type B | Type A | Type B | |||||||
(kg m−2 h−1) | (kg m−2 h−1) | (%) | (kg m−2 h−1) | (%) | (kg m−2 h−1) | (%) | (kg m−2 h−1) | (%) | ||
| 55 | 1.67 | 2.26 | 2.99 | 32.3 | 3.18 | 40.0 | 2.61 | 15.4 | 3.28 | 45.1 |
| 3.33 | 2.64 | 3.66 | 38.6 | 3.92 | 48.8 | 3.22 | 21.9 | 4.10 | 55.0 | |
| 5.0 | 3.06 | 4.25 | 38.8 | 4.70 | 52.5 | 3.73 | 21.9 | 4.94 | 61.0 | |
| 6.67 | 3.56 | 4.85 | 36.2 | 5.21 | 46.3 | 4.22 | 18.5 | 5.58 | 56.7 | |
| 60 | 1.67 | 2.86 | 3.85 | 34.6 | 4.17 | 45.8 | 3.33 | 16.4 | 4.28 | 49.6 |
| 3.33 | 3.28 | 4.50 | 37.1 | 4.90 | 49.3 | 3.91 | 19.2 | 5.07 | 54.5 | |
| 5.0 | 3.87 | 5.37 | 38.7 | 5.84 | 50.9 | 4.61 | 19.1 | 5.85 | 51.1 | |
| 6.67 | 4.41 | 6.14 | 39.2 | 6.55 | 48.5 | 5.36 | 21.5 | 6.87 | 55.7 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chang, H.; Ho, C.-D.; Chen, Y.-H.; Chen, L.; Hsu, T.-H.; Lim, J.-W.; Chiou, C.-P.; Lin, P.-H. Enhancing the Permeate Flux of Direct Contact Membrane Distillation Modules with Inserting 3D Printing Turbulence Promoters. Membranes 2021, 11, 266. https://doi.org/10.3390/membranes11040266
Chang H, Ho C-D, Chen Y-H, Chen L, Hsu T-H, Lim J-W, Chiou C-P, Lin P-H. Enhancing the Permeate Flux of Direct Contact Membrane Distillation Modules with Inserting 3D Printing Turbulence Promoters. Membranes. 2021; 11(4):266. https://doi.org/10.3390/membranes11040266
Chicago/Turabian StyleChang, Hsuan, Chii-Dong Ho, Yih-Hang Chen, Luke Chen, Tze-Hao Hsu, Jun-Wei Lim, Chung-Pao Chiou, and Po-Hung Lin. 2021. "Enhancing the Permeate Flux of Direct Contact Membrane Distillation Modules with Inserting 3D Printing Turbulence Promoters" Membranes 11, no. 4: 266. https://doi.org/10.3390/membranes11040266
APA StyleChang, H., Ho, C.-D., Chen, Y.-H., Chen, L., Hsu, T.-H., Lim, J.-W., Chiou, C.-P., & Lin, P.-H. (2021). Enhancing the Permeate Flux of Direct Contact Membrane Distillation Modules with Inserting 3D Printing Turbulence Promoters. Membranes, 11(4), 266. https://doi.org/10.3390/membranes11040266









