Economic Design of Solar-Driven Membrane Distillation Systems for Desalination
Abstract
1. Introduction
2. Process Description and Modeling
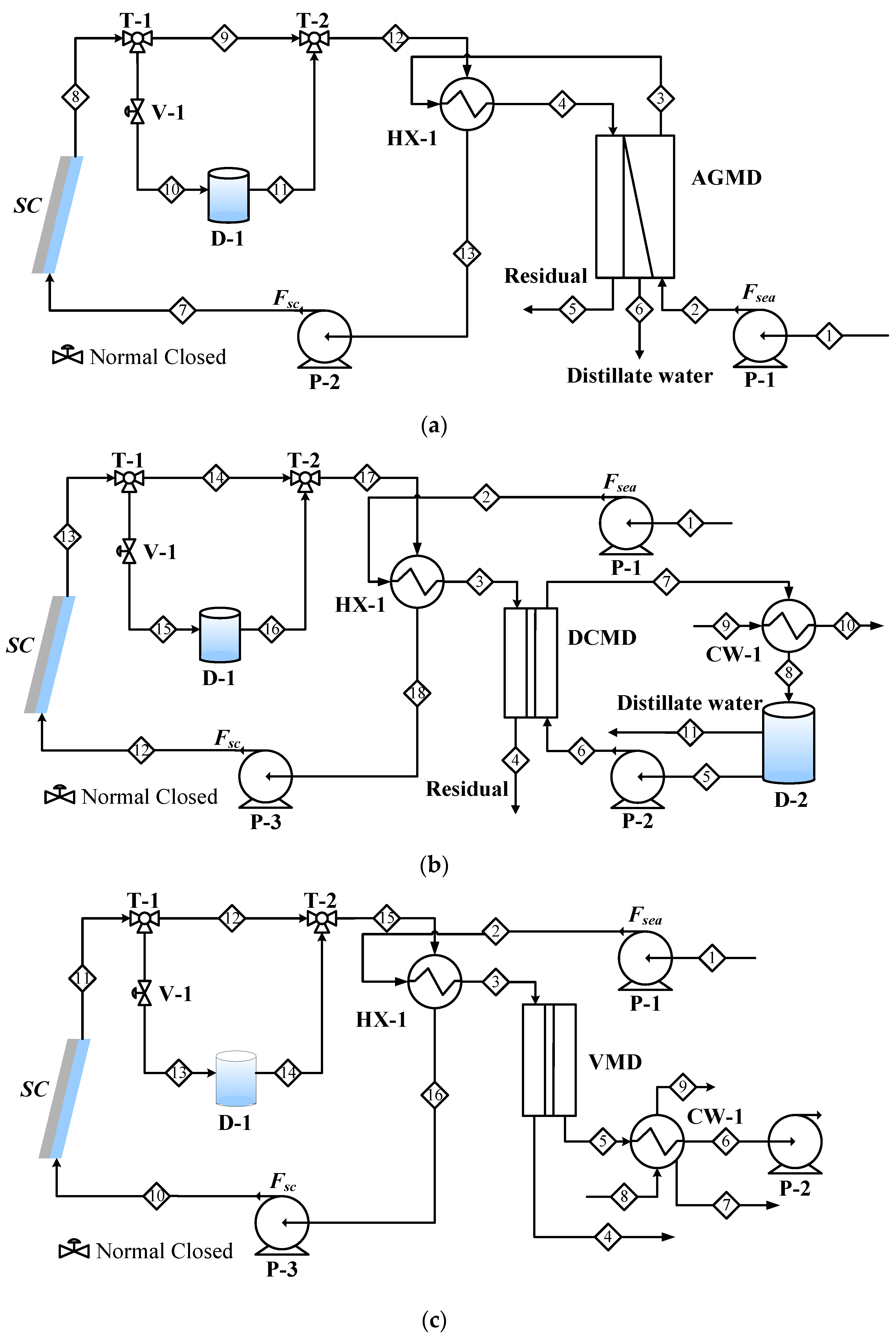
2.1. Process Description
2.1.1. Air Gap Membrane Distillation System for Desalination (AGMD)
2.1.2. Direct Contact Membrane Distillation System for Desalination (DCMD)
2.1.3. Vacuum Membrane Distillation System for Desalination (VMD)
2.2. Modeling
2.2.1. Solar Collector
2.2.2. Heat Exchanger
2.2.3. Thermal Storage Tank
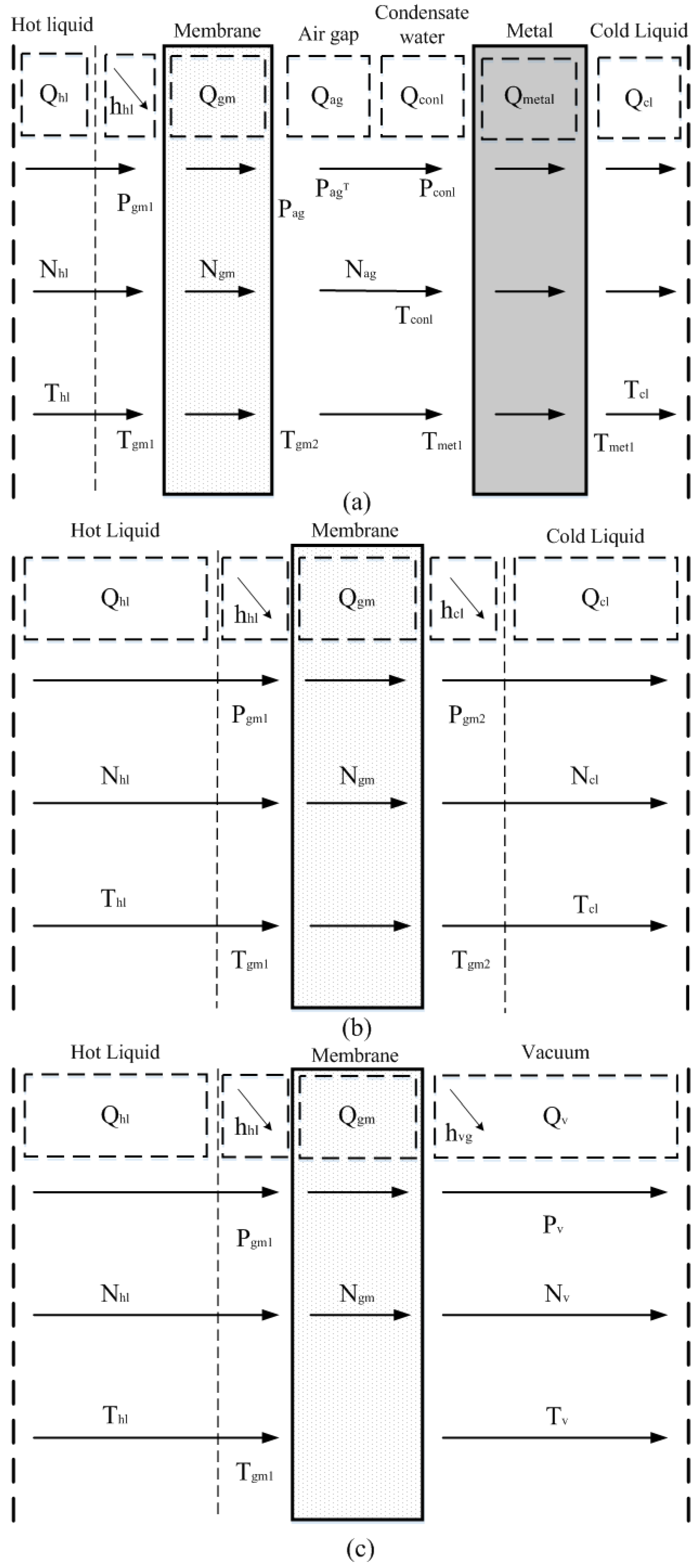
2.2.4. Membrane Distillation Modules
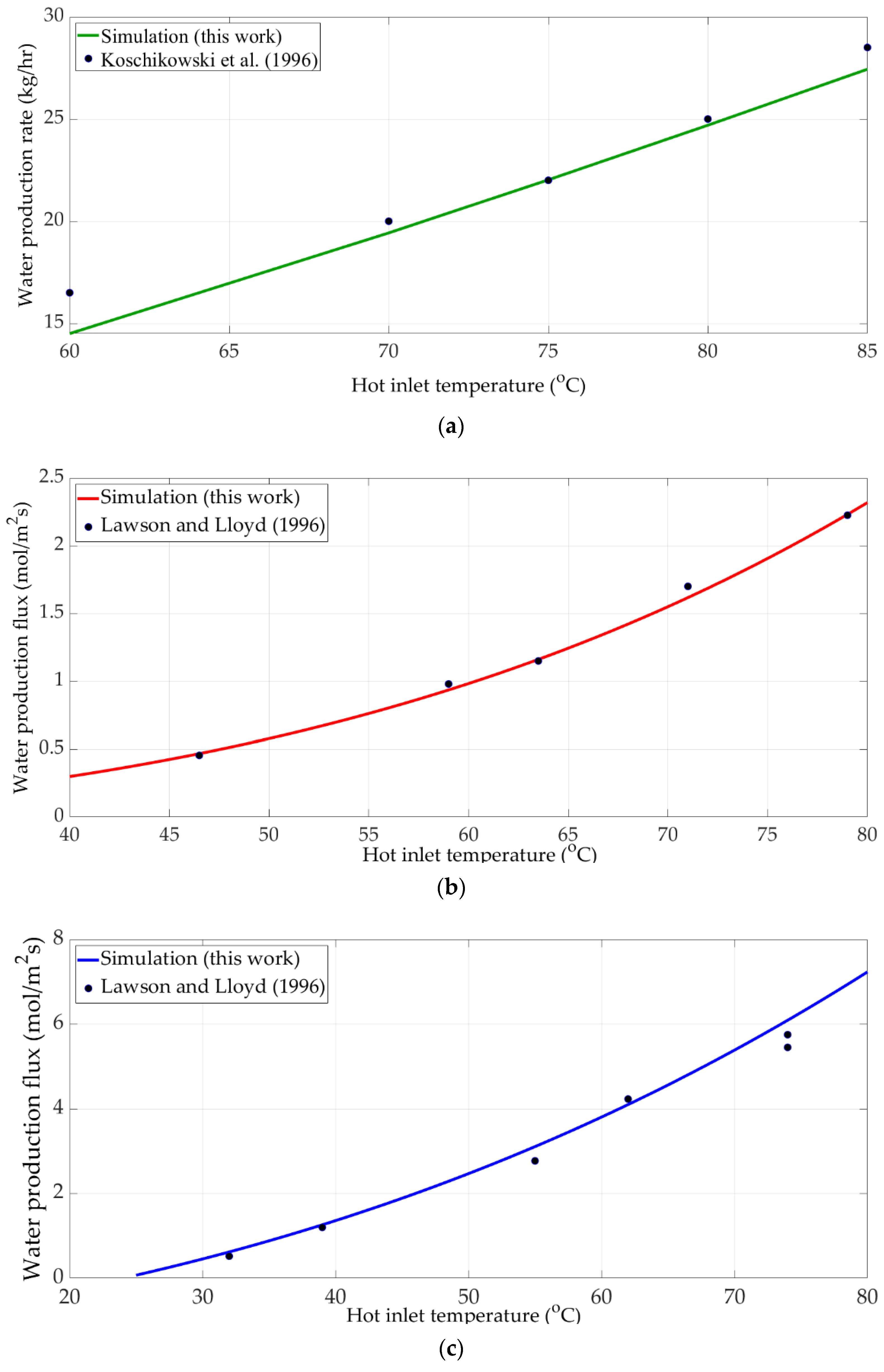
2.3. Membrane Distillation Model Validation of Membrane Distillation Modules
2.4. Overall System Simulation
3. Optimization
3.1. First Design Stage
3.1.1. Design Variables
3.1.2. Cost Functions
3.1.3. Objective Function
- (1)
- The distillate water production rate during day-time operation is 2000 kg/h.
- (2)
- The maximum temperature of the hot water from the solar collector is 95 °C.
- (3)
- The minimum approach temperature of the heat exchanger (ΔTmin) is 10 °C.
- (4)
- The vacuum side pressure of the VMD system is larger than 3 kPa.
3.2. Second Design Stage
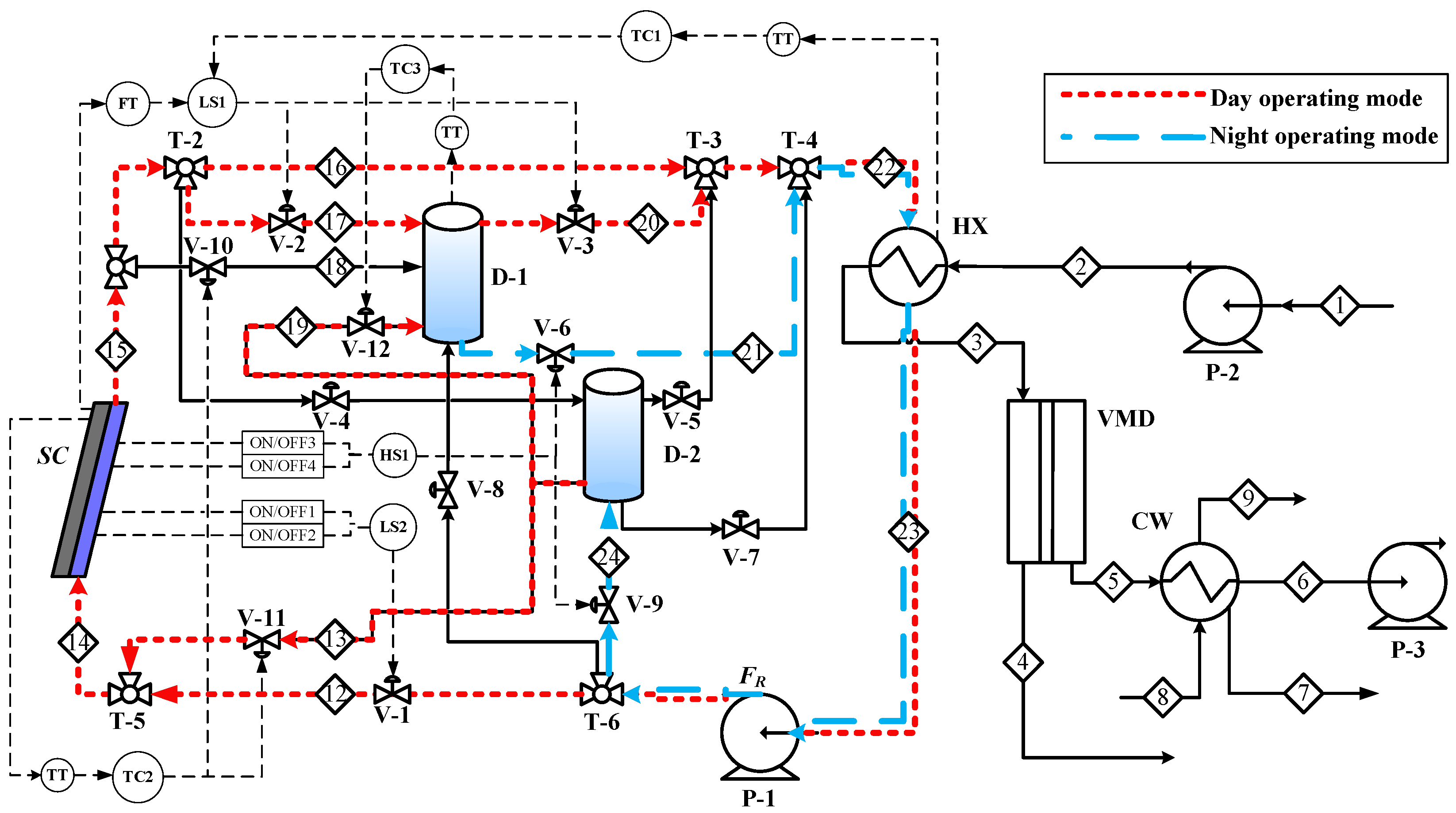
3.2.1. Control System Design
- (1)
- The temperature (T22) of the hot water entering the heat exchanger (HX-1) is controlled by manipulating the inlet flowrate (F17) of D-1. The purpose of this control loop is to maintain constant water production rate.
- (2)
- The outlet stream temperature (T15) of the solar collector is controlled at 95 °C by manipulating the make-up water flowrate (F13) from D-2. The control loop was used to avoid the working fluid boiling problem.
- (3)
- The make-up water flow (F19) from D-2 to the thermal storage tank is used to maintain the temperature of the thermal storage tank (T20) below T22. As the temperature control loop of T22 cannot work when T20 approaches T22.
3.2.2. Objective Function
4. Results and Discussion
4.1. Optimal Solutions from the First Design Stage
4.2. Optimal Solutions from the Second Design Stage
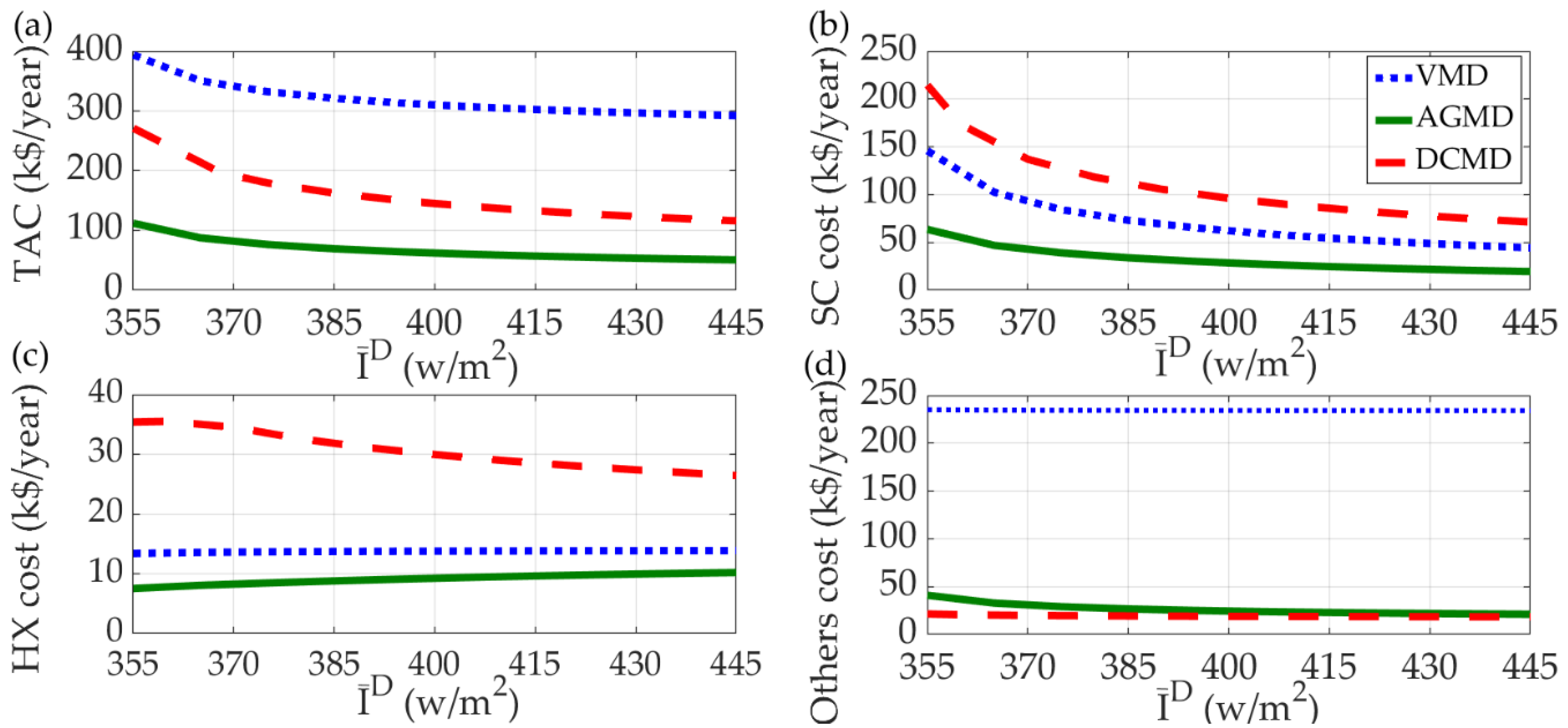
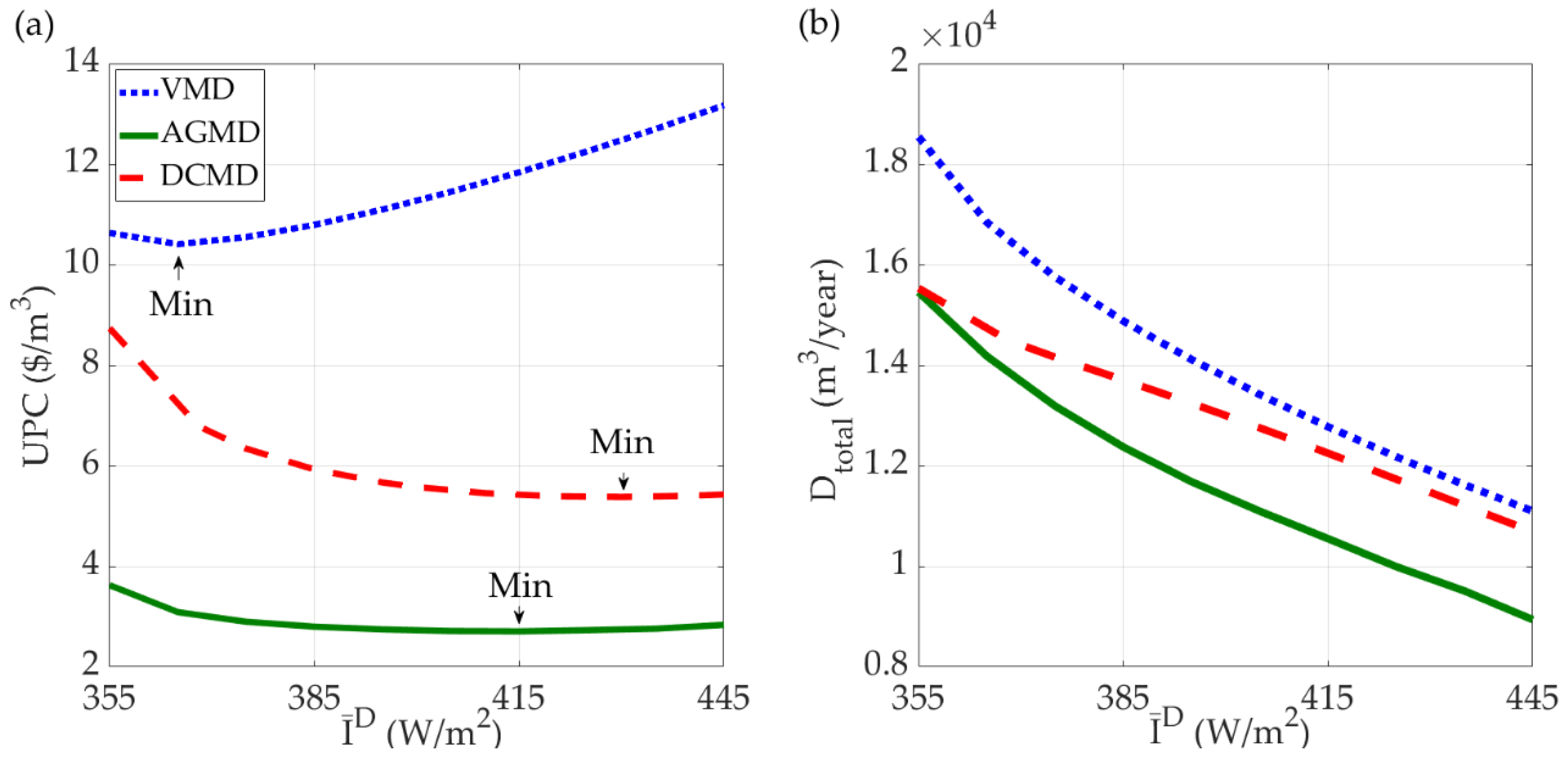
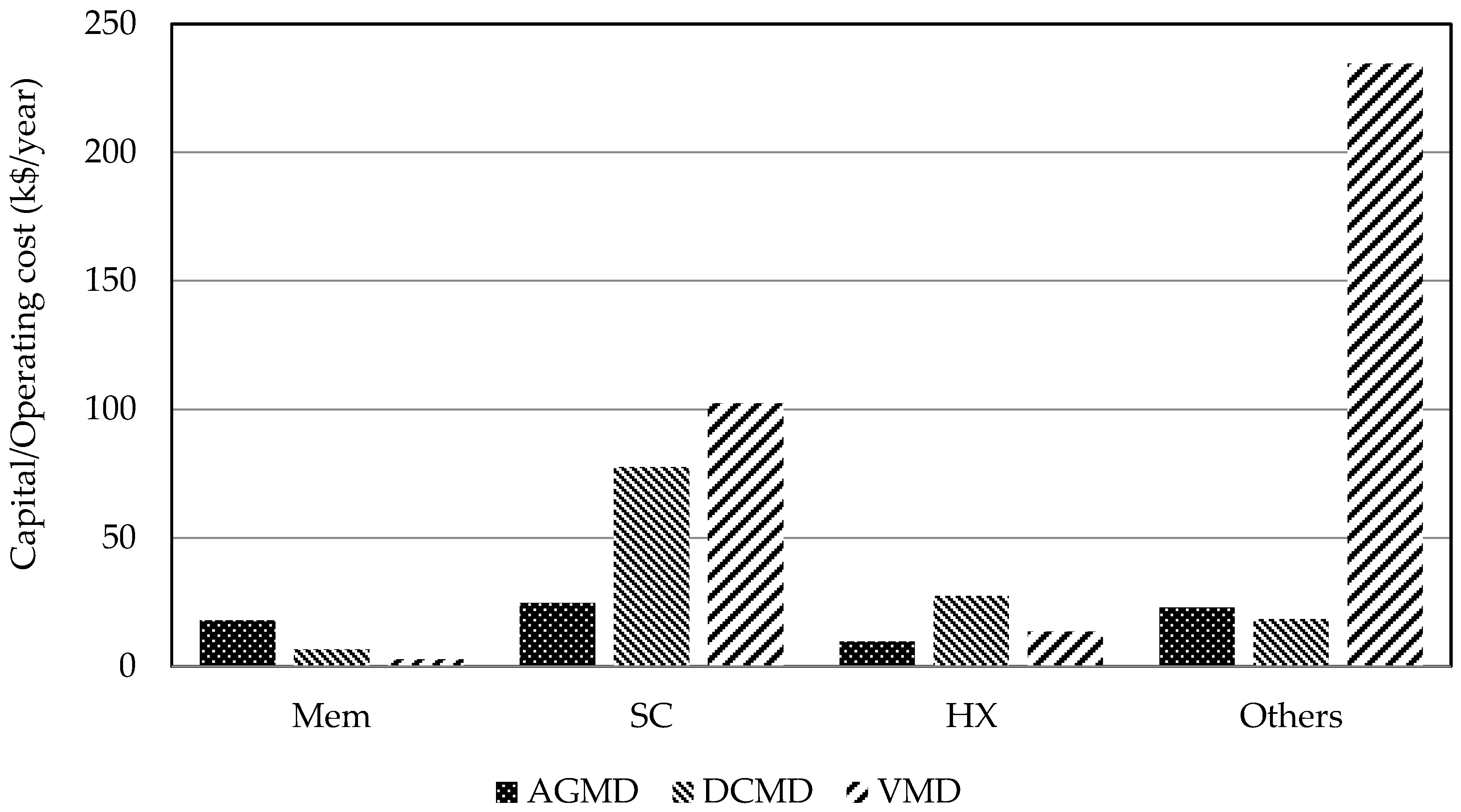
4.3. Comparison of Costs of SDMD Systems
4.4. Effect of Membrane Cost
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| A | Area (m2) |
| a | Amortization factor |
| B | Absorption coefficient (-) |
| bf | Channel height of the membrane distillation module (m) |
| bsc | Channel height of the solar collector (m) |
| CB | Bare module cost ($) |
| Cp | Purchase cost ($) |
| Cpm | Heat capacity (J/kg K) |
| Cpc | Heat capacity of the solar collector plate (J/kg K) |
| Cpf | Heat capacity of the working fluid (J/kg K) |
| D | Water production rate (kg/h) |
| Dh | Hydraulic diameter (m) |
| Dtotal | Annual water production rate (m3/year) |
| FL | Piping length factor |
| FM | Material of construction factor |
| FMD | MD circulation stream flowrate (kg/h) |
| FP | Pressure factor |
| Fsea | Seawater flowrate (kg/h) |
| FSC | Solar collector circulation flowrate (kg/h) |
| FT | Pump type factor |
| FT | Flow transmitter |
| H | Head (m) |
| HS | High signal selector |
| HX | Heat exchanger |
| h | Heat convention coefficient (W/m2 K) |
| hvap | Heat of vaporization (J/kmol) |
| D | Constant-value solar radiation intensities (W/m2) |
| i | Tax rate (%) |
| KC | Controller gain (%/%) |
| k | Heat conduction coefficient (J/s m2 K) |
| L | Length (m) |
| LS | Low signal selector |
| L/W | Aspect ratio (-) |
| M | Weight (kg) |
| mw | Molecular weight (kg/kmol) |
| m | Mass flowrate (kg/h) |
| N | Molar flux (kmol/m2 s) |
| n | Tax amortization period of the asset in years |
| P | Pressure (Pa) |
| Q | Heat flux (kJ/m2s) |
| S | Equipment size coefficient (-) |
| T | Temperature (°C) |
| TAC | Total annual cost ($/yr) |
| TC | Temperature control |
| TT | Temperature transmitter |
| U | Overall heat transfer coefficient (W/m2 K) |
| UPC | Unit production cost($/m3) |
| V | Velocity (m/s) |
| W | Width (m) |
| Symbol | |
| ΔTmin | Minimum approach temperature of the heat exchanger (°C) |
| γ | Pore size (μm) |
| δ | Thickness (μm) |
| ε | Porosity of the membrane (-) |
| τI | Integral time |
| Subscript | |
| a | Ambient |
| ag | Air gap layer |
| cl | Cold fluid |
| conl | Condensate fluid |
| f | Working fluid |
| gm | Internal layer of the membrane |
| gm1 | Interfacial between the hot fluid and the membrane |
| gm2 | Interfacial between the membrane and the other fluid |
| HX | Heat exchanger |
| hl | Hot fluid |
| in | Inlet |
| MD | Membrane |
| m | Metal layer |
| met1 | Interfacial between the metal and the air gap |
| met2 | Interfacial between the metal and the cold fluid |
| No. | Stream no. |
| o | Outlet |
| SC | Solar collector |
| sea | Seawater flowrate |
| v | Vacuum |
| w | Storage tank |
References
- Pangarkar, B.L.; Sane, M.G.; Parjane, S.B.; Guddad, M. Reverse Osmosis and Membrane Distillation for Desalination of Groundwater: A Review. SRN Mater. Sci. 2011, 2011, 1–9. [Google Scholar] [CrossRef]
- Shannon, M.A.; Bohn, P.W.; Elimelech, M.; Georgiadis, J.G.; Marĩas, B.J.; Mayes, A.M. Science and technology for water purification in the coming decades. Nature 2008, 452, 301–310. [Google Scholar] [CrossRef] [PubMed]
- Elimelech, M.; Phillip, W.A. The future of seawater desalination: Energy, technology, and the environment. Science 2011, 333, 712–717. [Google Scholar] [CrossRef] [PubMed]
- Greenlee, L.F.; Lawler, D.F.; Freeman, B.D.; Marrot, B.; Moulin, P. Reverse osmosis desalination: Water sources, technology, and today’s challenges. Water Res. 2009, 43, 2317–2348. [Google Scholar] [CrossRef]
- Fiorenza, G.; Sharma, V.K.; Braccio, G. Techno-economic evaluation of a solar powered water desalination plant. Energy Convers. Manag. 2003, 44, 2217–2240. [Google Scholar] [CrossRef]
- Kalogirou, S. Thermal performance, economic and environmental life cycle analysis of thermosiphon solar water heaters. Solar Energy 2009, 83, 39–48. [Google Scholar] [CrossRef]
- Pangarkar, B.L.; Sane, M.G.; Parjane, S.B.; Guddad, M. Vacuum Membrane Distillation for Desalination of Ground Water by using Flat Sheet Membrane. World Acad. Sci. Eng. Technol. 2011, 51, 797–802. [Google Scholar]
- Macedonio, F.; Drioli, E. Pressure-driven membrane operations and membrane distillation technology integration for water purification. Desalination 2008, 223, 396–409. [Google Scholar] [CrossRef]
- Qtaishat, M.R.; Banat, F. Desalination by solar powered membrane distillation systems. Desalination 2013, 308, 186–197. [Google Scholar] [CrossRef]
- Meindersma, G.W.; Guijt, C.M.; de Haan, A.B. Desalination and water recycling by air gap membrane distillation. Desalination 2006, 187, 291–301. [Google Scholar] [CrossRef]
- El-Bourawi, M.S.; Ding, Z.; Ma, R.; Khayet, M. A framework for better understanding membrane distillation separation process. J. Membr Sci. 2006, 285, 4–29. [Google Scholar] [CrossRef]
- Mericq, J.-P.; Laborie, S.; Cabassud, C. Evaluation of systems coupling vacuum membrane distillation and solar energy for seawater desalination. Chem. Eng. J. 2011, 166, 596–606. [Google Scholar] [CrossRef]
- Chang, H.; Liau, J.S.; Ho, C.D.; Wang, W.H. Simulation of membrane distillation modules for desalination by developing user’s model on Aspen Plus platform. Desalination 2009, 249, 380–387. [Google Scholar] [CrossRef]
- Hogan, P.A.; Fane, A.G.; Morrison, G.L. Desalination by solar heated membrane distillation. Desalination 1991, 81, 81–90. [Google Scholar] [CrossRef]
- Winter, D.; Koschikowski, J.; Gross, F.; Maucher, D.; Duver, D.; Jositz, M.; Mann, T.; Hagedorn, A. Comparative analysis of full-scale membrane distillation contactors—Methods and modules. J. Membr. Sci. 2017, 524, 758–771. [Google Scholar] [CrossRef]
- Banat, F.; Jwaied, N. Economic evaluation of desalination by small-scale autonomous solar-powered membrane distillation units. Desalination 2008, 220, 566–573. [Google Scholar] [CrossRef]
- Moore, S.E.; Mirchandani, S.D.; Karanikola, V.; Nenoff, T.M.; Arnolda, R.G.; Eduardo Sáez, A. Process modeling for economic optimization of a solar-driven sweeping gas membrane distillation desalination system. Desalination 2018, 437, 108–120. [Google Scholar] [CrossRef]
- Miladi, R.; Frikha, N.; Kheiri, A.; Gabsi, S. Energetic performance analysis of seawater desalination with a solar membrane distillation. Energy Convers. Manag. 2019, 185, 143–154. [Google Scholar] [CrossRef]
- Chang, H.; Wang, G.B.; Chen, Y.H.; Li, C.C.; Chang, C.L. Modeling and optimization of a solar-driven membrane distillation desalination system. Renew. Energy 2010, 35, 2714–2722. [Google Scholar] [CrossRef]
- Chen, Y.H.; Li, Y.W.; Chang, H. Optimal design and control of solar-driven air gap membrane distillation desalination systems. Appl. Energy 2012, 100, 193–204. [Google Scholar] [CrossRef]
- Chang, H.; Lyu, S.G.; Tsai, C.M.; Chen, Y.H.; Cheng, T.W.; Chou, Y.H. Experimental and simulation study of a solar thermal driven membrane distillation desalination process. Desalination 2012, 286, 400–411. [Google Scholar] [CrossRef]
- Gil, J.D.; Roca, L.; Zaragoza, G.; Berenguel, M. A feedback control system with reference governor for a solar membrane distillation pilot facility. Renew. Energy 2018, 120, 536–549. [Google Scholar] [CrossRef]
- Bendevis, P.; Karam, A.; Laleg-Kirati, T.M. Optimal model-free control of solar thermal membrane distillation system. Comput. Chem. Eng. 2020, 133, 1066222. [Google Scholar] [CrossRef]
- Fuller, E.N.; Schettler, P.D.; Giddings, J.C. New method for prediction of binary gas-phase diffusion coefficients. Ind. Eng. Chem. 1966, 58, 18–27. [Google Scholar] [CrossRef]
- Reid, R.C.; Prsusnitz, J.M.; Poling, B.E. The Properties of Gases & Liquids, 4th ed.; McGraw-Hill International Edition: New York, NY, USA, 1986. [Google Scholar]
- Dewitt, D.P.; Incropera, F.P.; Bergman, T.L.; Lavine, A.S. Fundamentals of Heat and Mass Transfer, 6th ed.; John Willey & Sons: Hoboken, NJ, USA, 2007. [Google Scholar]
- Posey, M.L.; Rochelle, G.T. A thermodynamic model of methyldiethanolamine-CO2-H2S-Water. Ind. Eng. Chem. Res. 1997, 36, 3944–3953. [Google Scholar] [CrossRef]
- Alkhudhiri, A.; Darwish, N.; Hilal, N. Membrane distillation: A comprehensive review. Desalination 2012, 287, 2–18. [Google Scholar] [CrossRef]
- Turton, R.; Bailie, R.C.; Whiting, W.B.; Shaeiwitz, J.A. Analysis, Synthesis, and Design of Chemical Process; Prentice Hall Inc.: Upper Saddle River, NJ, USA, 1998. [Google Scholar]
- Koschikowski, J.; Wieghaus, M.; Rommel, M. Solar thermal-driven desalination plants based on membrane distillation. Desalination 2003, 156, 295–304. [Google Scholar] [CrossRef]
- Lawson, K.W.; Lloyd, D.R. Membrane distillation. I. Module design and performance evaluation using vacuum membrane distillation. J. Membr. Sci. 1996, 120, 111–121. [Google Scholar] [CrossRef]
- Lawson, K.W.; Lloyd, D.R. Membrane distillation. II. Direct contact MD. J. Membr. Sci. 1996, 120, 123–133. [Google Scholar] [CrossRef]
- Luyben, W.L. Design and control degrees of freedom. Ind. Eng. Chem. Res. 1996, 35, 2204–2214. [Google Scholar] [CrossRef]
- AL-NIMR, M.A.; Kiawn, S.; Al-Alwah, A. Size optimization of convention solar collectors. Energy 1998, 23, 373–378. [Google Scholar] [CrossRef]
- Hollands, K.G.T.; Shewen, E.C. Optimization of flow passage geometry for air-heating, plate-type solar collectors. J. Sol. Energy Eng. 1981, 103, 323–330. [Google Scholar] [CrossRef]
- Avlonitis, S.; Hanbury, W.T.; Boundinar, M.B. Spiral Wound Modules Performance an analytical solution: Part II. Desalination 1993, 89, 227–246. [Google Scholar] [CrossRef]
- Al-Obaidani, S. Potential of membrane distillation in seawater desalination: Thermal efficiency, sensitivity study and cost estimation. J. Membr. Sci. 2008, 323, 85–98. [Google Scholar] [CrossRef]
- Seider, W.D.; Seader, J.D.; Lewin, D.R.; Widagdo, S. Production and Process Design Principles Synthesis, Analysis, and Evaluation, 3rd ed.; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2010. [Google Scholar]
- Tyreus, B.D.; Luyben, W.L. Tuning PI Controllers for Integrator/Dead Time Processes. Ind. Eng. Chem. Res. 1992, 31, 2625–2628. [Google Scholar] [CrossRef]
- Thomas, J.; Yu, C.C.; Luyben, W.L. Improved Multiloop Single—Input/Single—Out put (SISO) Controllers for Multivariable Processes. Ind. Eng. Chem. Res. 1988, 27, 969–973. [Google Scholar]



| 1. Solar subsystem |
| (1) Solar collector |
| (2) Heat exchanger |
| (3) Storage tank |
| 2. AGMD subsystem |
| 3. DCMD subsystem |
| 4. VMD subsystem |
| Property | AGMD | DCMD | VMD |
|---|---|---|---|
| Material | PTFE | PP(3ME) | PP(3MA) |
| Pore size(γ) (μm) | 0.200 | 0.730 | 0.290 |
| Porosity(ε) | 0.770 | 0.850 | 0.660 |
| Thickness(δ) (mm) | 0.140 | 0.079 | 0.091 |
| Area(AMD) (m2) | 7.000 | 9.7 × 10−4 | 9.7 × 10−4 |
| Aspect ratio (L/W) | 0.070 | 24.440 | 24.440 |
| Specifications and Results | AGMD | DCMD | VMD |
|---|---|---|---|
| Solar collector area (ASC) (m2) | 1615.00 | 18,350.00 | 10,297.00 |
| Membrane area (AMD) (m2) | 2040.00 | 500.00 | 58.00 |
| Heat transfer area (AHX) (m2) | 100.00 | 4000.00 | 380.00 |
| Constant-value solar radiation intensity (D) (W/m2) | 458.75 | 458.75 | 458.75 |
| Solar collector circulation flowrate (Fsc) (kg/h) | 24,940 | 45,350.00 | 24,680.00 |
| Solar collector outlet temperature (Tsc,out) (°C) | 84.38 | 93.55 | 94.82 |
| Seawater flowrate (Fsea) (kg/h) | 30,434.00 | 40,100.00 | 32,545.00 |
| MD circulation stream flowrate (FMD) (kg/h) | - | 50,000.00 | - |
| MD inlet stream temperature (TMD,in) (°C) | 75.57 | 92.99 | 70.36 |
| Vacuum pressure (Pv) (Pa) | - | - | 5000.00 |
| Water production rate (D) (kg/h) | 2000.00 | 2000.00 | 2000.00 |
| Amortization factor, a |
| Capital cost of centrifugal pumps ($) |
| S = Q(H)0.5 |
| CB = exp{9.7171 − 0.6019[ln(S)] + 0.0519[ln(S)]2} |
| CP = FTFMCB |
| Capital cost of vacuum pumps ($) |
| S = 50-350 (ft3/min) |
| Cp = 8250S0.35 |
| Capital cost of shell and tube heat exchangers ($) |
| CB = exp{11.0545 − 0.9228[ln(A)] + 0.09861[ln(A)]2} |
| FM = a + (A/100)b |
| FP = 0.09803 + 0.018(P/100) + 0.0017(P/100)2 |
| FL = 1 |
| CP = FP FM FLCB |
| Capital cost of porous membranes ($) |
| Cp = 90 Am |
| Replacement cost of porous membranes ($/yr) |
| Cp, replacement = Cp, i * 20% i = AGMD, DCMD or VMD |
| Capital cost of solar collector ($) |
| Cp = 50 ASC |
| Utility unit cost |
| Electricity cost: $0.06/kWh |
| Variables | AGMD | DCMD | VMD |
|---|---|---|---|
| Constant-value solar radiation intensities (D) (W/m2) | 415.00 | 430.00 | 360.00 |
| Solar collector area (ASC) (m2) | 5111.00 | 16,104.00 | 26,066.00 |
| Membrane area (AMD) (m2) | 673.00 | 245.00 | 80.00 |
| Heat transfer area (AHX) (m2) | 116.00 | 546.00 | 274.00 |
| Solar collector circulation flowrate (Fsc) (kg/h) | 22,854.00 | 34,984.00 | 21,446.00 |
| Solar collector outlet temperature (Tsc,out) (°C) | 95.00 | 95.00 | 95.00 |
| Seawater flowrate (Fsea) (kg/h) | 28,657.00 | 30,142.00 | 35,302.00 |
| MD circulation stream flowrate (FMD) (kg/h) | - | 48,183.00 | - |
| Vacuum pressure (Pv) (Pa) | - | - | 3554.00 |
| Annual water production rate (Dtotal) (m3/year) | 10,550.00 | 11,470.00 | 16,854.00 |
| Total annual cost (TAC) ($/year) | 57,092.00 | 123,411.00 | 350,565.00 |
| Unit production cost (UPC) ($/m3) | 2.71 | 5.38 | 10.41 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, Y.-H.; Hung, H.-G.; Ho, C.-D.; Chang, H. Economic Design of Solar-Driven Membrane Distillation Systems for Desalination. Membranes 2021, 11, 15. https://doi.org/10.3390/membranes11010015
Chen Y-H, Hung H-G, Ho C-D, Chang H. Economic Design of Solar-Driven Membrane Distillation Systems for Desalination. Membranes. 2021; 11(1):15. https://doi.org/10.3390/membranes11010015
Chicago/Turabian StyleChen, Yih-Hang, Hwo-Gan Hung, Chii-Dong Ho, and Hsuan Chang. 2021. "Economic Design of Solar-Driven Membrane Distillation Systems for Desalination" Membranes 11, no. 1: 15. https://doi.org/10.3390/membranes11010015
APA StyleChen, Y.-H., Hung, H.-G., Ho, C.-D., & Chang, H. (2021). Economic Design of Solar-Driven Membrane Distillation Systems for Desalination. Membranes, 11(1), 15. https://doi.org/10.3390/membranes11010015








