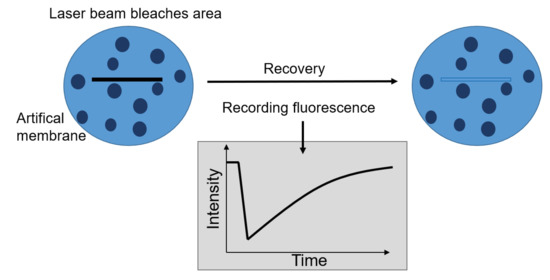
Defining the Diffusion in Model Membranes Using Line Fluorescence Recovery after Photobleaching
Abstract
1. Introduction
2. Materials and Methods
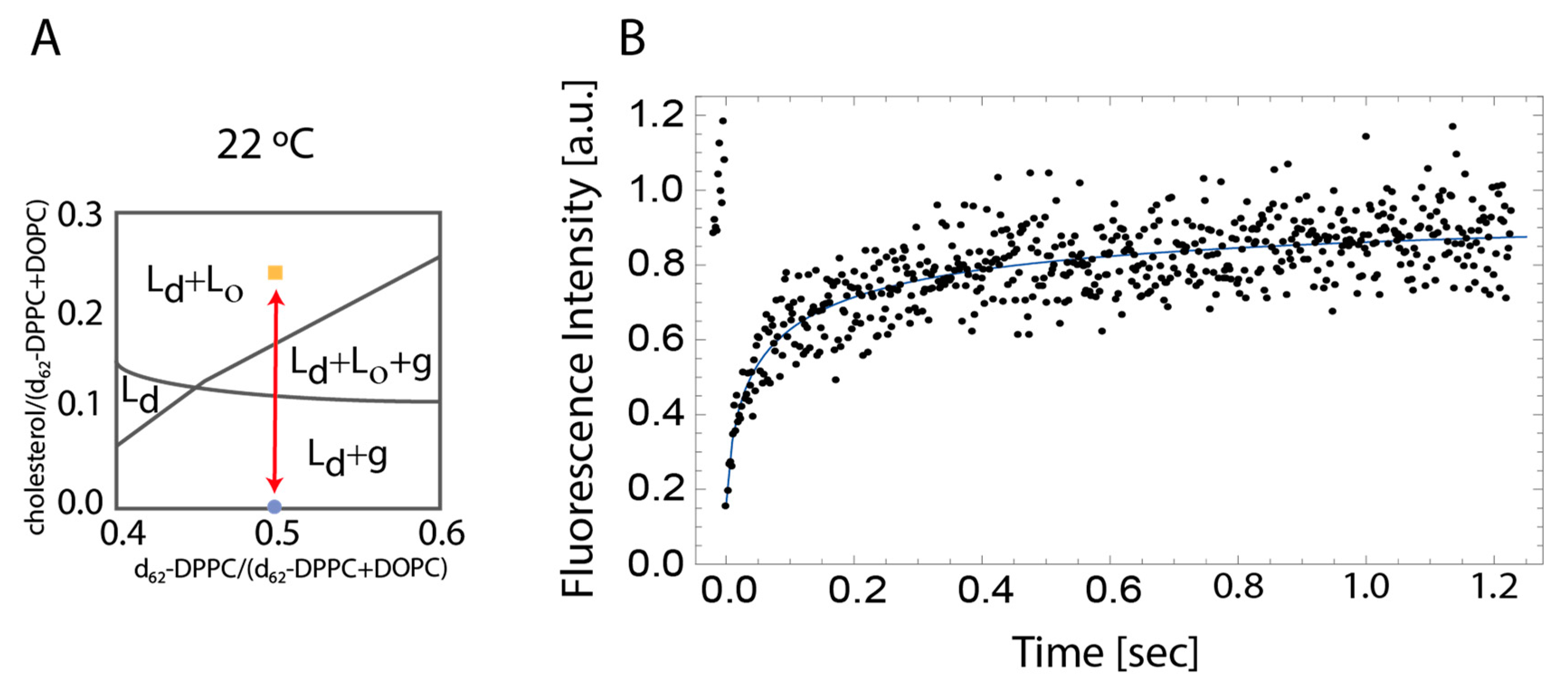
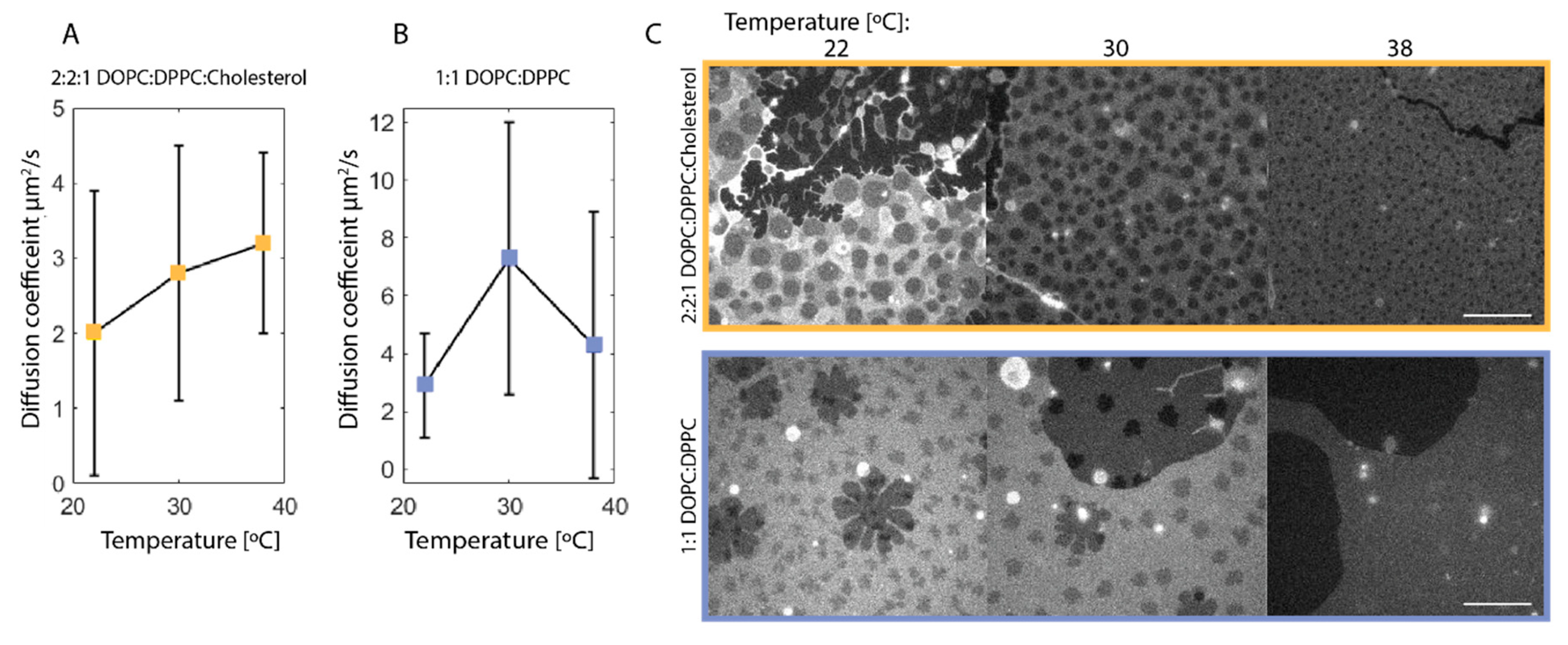
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Singer, S.J.; Nicolson, G.L. The Fluid Mosaic Model of the Structure of Cell Membranes. Science 1972, 175, 720–731. [Google Scholar] [CrossRef] [PubMed]
- Simons, K.; Ikonen, E. Functional Rafts in Cell Membranes. Nature 1997, 387, 569–572. [Google Scholar] [CrossRef] [PubMed]
- Donaldson, S.H.; De Aguiar, H.B. Label-Free Imaging of Cholesterol and Lipid Distributions in Model Membranes. J. Phys. Chem. Lett. 2018, 9, 1528–1533. [Google Scholar] [CrossRef] [PubMed]
- Juhasz, J.; Sharom, F.J.; Davis, J.H. Quantitative Characterization of Coexisting Phases in DOPC/DPPC/Cholesterol Mixtures: Comparing Confocal Fluorescence Microscopy and Deuterium Nuclear Magnetic Resonance. Biochim. Biophys. Acta Biomembr. 2009, 1788, 2541–2552. [Google Scholar] [CrossRef]
- Semrau, S.; Idema, T.; Schmidt, T.; Storm, C. Membrane-Mediated Interactions Measured Using Membrane Domains. Biophys. J. 2009, 96, 4906–4915. [Google Scholar] [CrossRef]
- Sackmann, E. Supported Membranes: Scientific and Practical Applications. Science 1996, 271, 43–48. [Google Scholar] [CrossRef]
- Boxer, S. Molecular Transport and Organization in Supported Lipid Membranes. Curr. Opin. Chem. Biol. 2000, 4, 704–709. [Google Scholar] [CrossRef]
- Davis, J.H.; Clair, J.J.; Juhasz, J. Phase Equilibria in DOPC/DPPC-D62/Cholesterol Mixtures. Biophys. J. 2009, 96, 521–539. [Google Scholar] [CrossRef]
- Marsh, D. Cholesterol-Induced Fluid Membrane Domains: A Compendium of Lipid-Raft Ternary Phase Diagrams. Biochim. Biophys. Acta Biomembr. 2009, 1788, 2114–2123. [Google Scholar] [CrossRef]
- Chan, Y.-H.M.; Boxer, S.G. Model Membrane Systems and Their Applications. Curr. Opin. Chem. Biol. 2007, 11, 581–587. [Google Scholar] [CrossRef]
- Marchetti, L.; Bonsignore, F.; Gobbo, F.; Amodeo, R.; Calvello, M.; Jacob, A.; Signore, G.; Schirripa Spagnolo, C.; Porciani, D.; Mainardi, M.; et al. Fast-Diffusing P75NTR Monomers Support Apoptosis and Growth Cone Collapse by Neurotrophin Ligands. Proc. Natl. Acad. Sci. USA 2019, 116, 21563–21572. [Google Scholar] [CrossRef]
- Axelrod, D.; Koppel, D.E.; Schlessinger, J.; Elson, E.; Webb, W.W. Mobility Measurement by Analysis of Fluorescence Photobleaching Recovery Kinetics. Biophys. J. 1976, 16, 1055–1069. [Google Scholar] [CrossRef]
- Peters, R.; Peters, J.; Tews, K.H.; Bähr, W. A Microfluorimetric Study of Translational Diffusion in Erythrocyte Membranes. Biochim. Biophys. Acta Biomembr. 1974, 367, 282–294. [Google Scholar] [CrossRef]
- Pincet, F.; Adrien, V.; Yang, R.; Delacotte, J.; Rothman, J.E.; Urbach, W.; Tareste, D. FRAP to Characterize Molecular Diffusion and Interaction in Various Membrane Environments. PLoS ONE 2016, 11, e0158457. [Google Scholar] [CrossRef]
- Hell, S.W.; Wichmann, J. Breaking the Diffraction Resolution Limit by Stimulated Emission: Stimulated-Emission-Depletion Fluorescence Microscopy. Opt. Lett. 1994, 19, 780. [Google Scholar] [CrossRef]
- Betzig, E.; Patterson, G.H.; Sougrat, R.; Lindwasser, O.W.; Olenych, S.; Bonifacino, J.S.; Davidson, M.W.; Lippincott-Schwartz, J.; Hess, H.F. Imaging Intracellular Fluorescent Proteins at Nanometer Resolution. Science 2006, 313, 1642–1645. [Google Scholar] [CrossRef] [PubMed]
- Lorén, N.; Hagman, J.; Jonasson, J.K.; Deschout, H.; Bernin, D.; Cella-zanacchi, F.; Diaspro, A.; Mcnally, G.; Ameloot, M.; Smisdom, N.; et al. Fluorescence Recovery after Photobleaching in Material and Life Sciences: Putting Theory into Practice. Q. Rev. Biophys. 2015, 48, 323–387. [Google Scholar] [CrossRef]
- Braeckmans, K.; Peeters, L.; Sanders, N.N.; De Smedt, S.C.; Demeester, J. Three-Dimensional Fluorescence Recovery after Photobleaching with the Confocal Scanning Laser Microscope. Biophys. J. 2003, 85, 2240–2252. [Google Scholar] [CrossRef]
- Braeckmans, K.; Remaut, K.; Vandenbroucke, R.E.; Lucas, B.; De Smedt, S.C.; Demeester, J. Line FRAP with the Confocal Laser Scanning Microscope for Diffusion Measurements in Small Regions of 3-D Samples. Biophys. J. 2007, 92, 2172–2183. [Google Scholar] [CrossRef] [PubMed]
- Deschout, H.; Hagman, J.; Fransson, S.; Jonasson, J.; Rudemo, M.; Lorén, N.; Braeckmans, K. Straightforward FRAP for Quantitative Diffusion Measurements with a Laser Scanning Microscope. Opt. Express 2010, 18, 22886. [Google Scholar] [CrossRef] [PubMed]
- Tsay, T.T.; Jacobson, K.A. Spatial Fourier Analysis of Video Photobleaching Measurements. Principles and Optimization. Biophys. J. 1991, 60, 360–368. [Google Scholar] [CrossRef]
- Jönsson, P.; Jonsson, M.P.; Tegenfeldt, J.O.; Höök, F. A Method Improving the Accuracy of Fluorescence Recovery after Photobleaching Analysis. Biophys. J. 2008, 95, 5334–5348. [Google Scholar] [CrossRef] [PubMed]
- Macháň, R.; Hof, M. Lipid Diffusion in Planar Membranes Investigated by Fluorescence Correlation Spectroscopy. Biochim. Biophys. Acta Biomembr. 2010, 1798, 1377–1391. [Google Scholar] [CrossRef] [PubMed]
- Bernchou, U.; Ipsen, J.H.; Simonsen, A.C. Growth of Solid Domains in Model Membranes: Quantitative Image Analysis Reveals a Strong Correlation between Domain Shape and Spatial Position. J. Phys. Chem. B 2009, 113, 7170–7177. [Google Scholar] [CrossRef]
- Jeppesen, J.C.; Solovyeva, V.; Brewer, J.R.; Johannes, L.; Hansen, P.L.; Simonsen, A.C. Slow Relaxation of Shape and Orientational Texture in Membrane Gel Domains. Langmuir 2015, 31, 12699–12707. [Google Scholar] [CrossRef]
- Casella, S.; Huang, F.; Mason, D.; Zhao, G.Y.; Johnson, G.N.; Mullineaux, C.W.; Liu, L.N. Dissecting the Native Architecture and Dynamics of Cyanobacterial Photosynthetic Machinery. Mol. Plant 2017, 10, 1434–1448. [Google Scholar] [CrossRef]
- Mullineaux, C.W. FRAP Analysis of Photosynthetic Membranes. J. Exp. Bot. 2004, 55, 1207–1211. [Google Scholar] [CrossRef]
- Sezgin, E.; Schneider, F.; Galiani, S.; Urbančič, I.; Waithe, D.; Lagerholm, B.C.; Eggeling, C. Measuring Nanoscale Diffusion Dynamics in Cellular Membranes with Super-Resolution STED–FCS. Nat. Protoc. 2019, 14, 1054–1083. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kure, J.L.; Andersen, C.B.; Rasmussen, T.E.; Lagerholm, B.C.; Arnspang, E.C. Defining the Diffusion in Model Membranes Using Line Fluorescence Recovery after Photobleaching. Membranes 2020, 10, 434. https://doi.org/10.3390/membranes10120434
Kure JL, Andersen CB, Rasmussen TE, Lagerholm BC, Arnspang EC. Defining the Diffusion in Model Membranes Using Line Fluorescence Recovery after Photobleaching. Membranes. 2020; 10(12):434. https://doi.org/10.3390/membranes10120434
Chicago/Turabian StyleKure, Jakob L., Camilla B. Andersen, Thomas E. Rasmussen, B. Christoffer Lagerholm, and Eva C. Arnspang. 2020. "Defining the Diffusion in Model Membranes Using Line Fluorescence Recovery after Photobleaching" Membranes 10, no. 12: 434. https://doi.org/10.3390/membranes10120434
APA StyleKure, J. L., Andersen, C. B., Rasmussen, T. E., Lagerholm, B. C., & Arnspang, E. C. (2020). Defining the Diffusion in Model Membranes Using Line Fluorescence Recovery after Photobleaching. Membranes, 10(12), 434. https://doi.org/10.3390/membranes10120434