Determination of Fracture Properties of Concrete Using Size and Boundary Effect Models
Abstract
:1. Introduction
2. Size and Boundary Effect Models
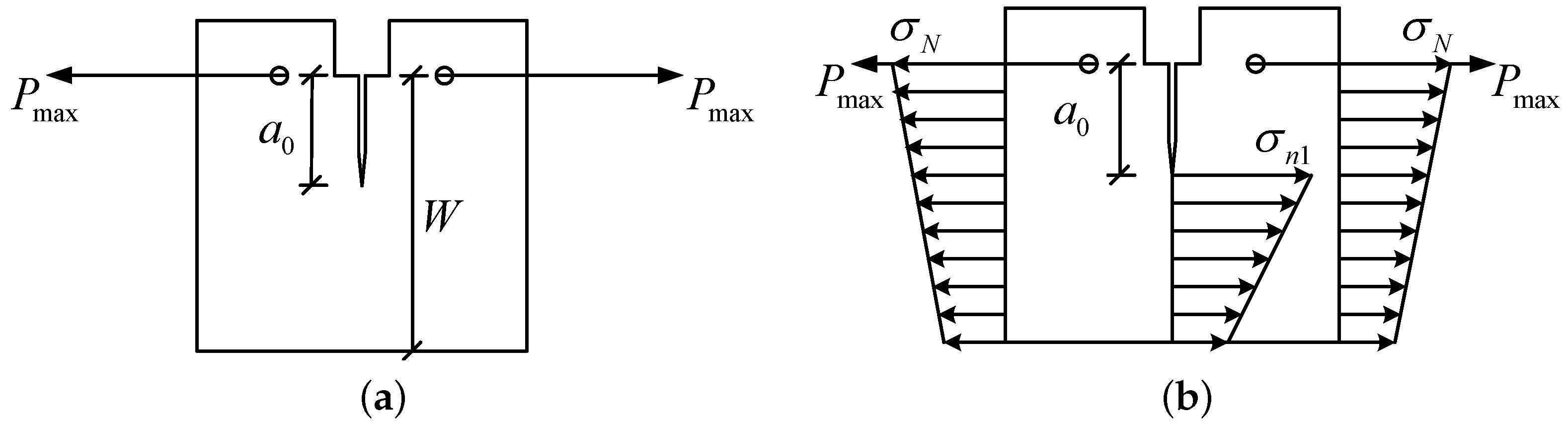
2.1. Hu et al. Boundary Effect Models
2.1.1. Hu-Duan’s Boundary Effect Model
2.1.2. Hu-Guan’s (Hu-Wang’s) Boundary Effect Model
2.1.3. Hu-Zhang’s Boundary Effect Model
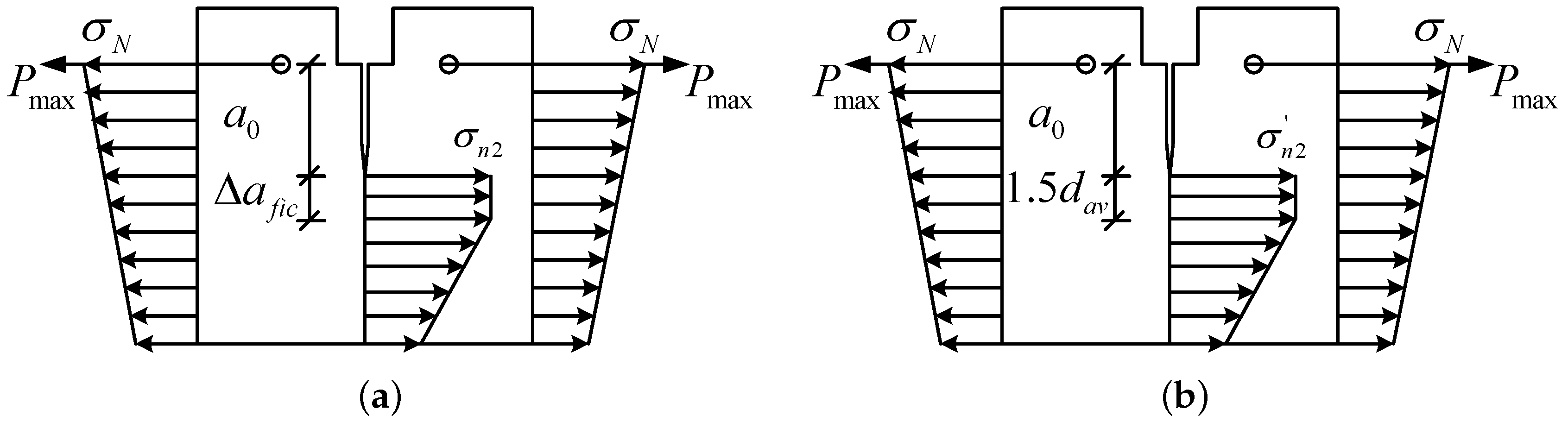
2.1.4. Proposed Modified Hu-Guan’s Boundary Effect Model
2.2. Koval-Gao’s Size and Boundary Effect Model
2.3. Bažant’s Type 2 Size Effect Law
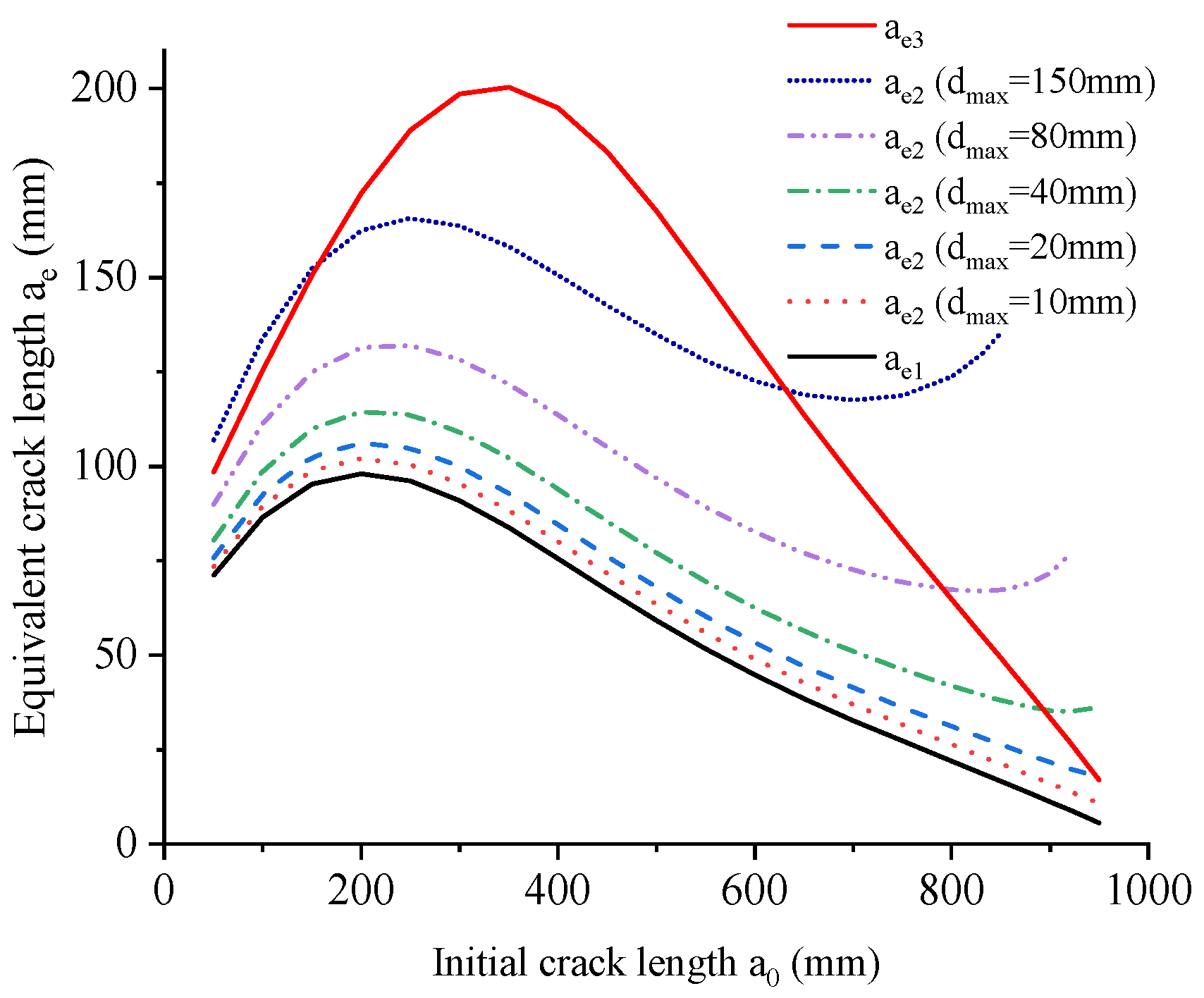
2.4. Comparison of Different Equivalent Crack Lengths
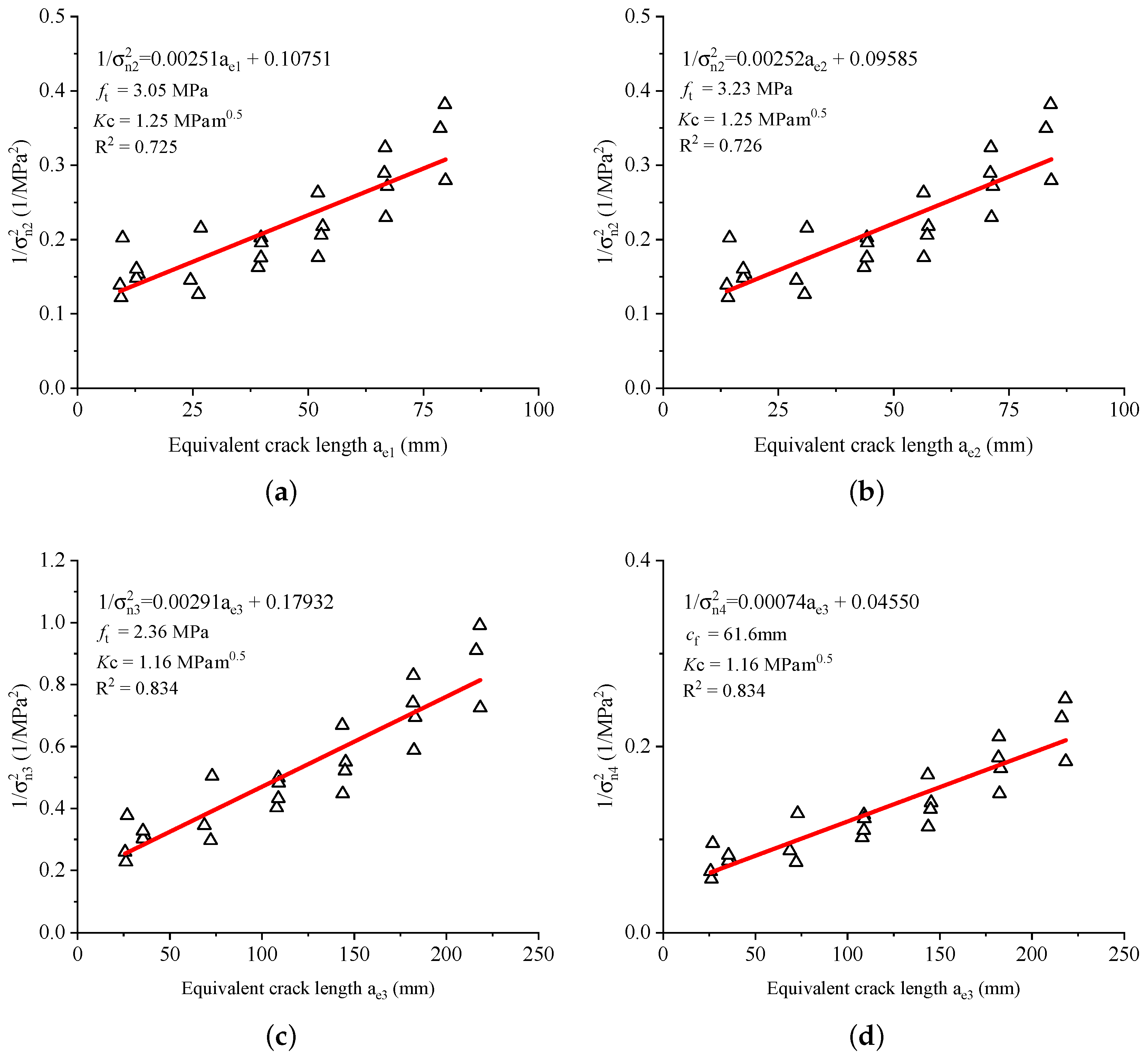
2.5. Method for the Determination of Fracture Parameters
3. Model Verification and Comparison
3.1. Tests of Geometrically Similar Specimens with Constant Crack-Length-to-Height Ratios
3.1.1. Zhang’s Experiments
3.1.2. Wittmann’s Experiments
3.2. Tests of Specimens with Different Initial Crack-Length-to-Height Ratios
3.2.1. Xu’s Experiments
3.2.2. Wu’s Experiments
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
Abbreviations
| BEM | Boundary effect model |
| SEL | Size effect law |
| LEFM | Linear elastic fracture mechanics |
References
- Li, Q.; Guan, J.; Wu, Z.; Dong, W.; Zhou, S. Fracture Behavior of Site-Casting Dam Concrete. ACI Mater. J. 2015, 112, 11–20. [Google Scholar] [CrossRef]
- Hu, Y.; Liang, G.; Li, Q.; Zuo, Z. A monitoring-mining-modeling system and its application to the temperature status of the Xiluodu arch dam. Adv. Struct. Eng. 2017, 20, 235–244. [Google Scholar] [CrossRef]
- Lin, P.; Wei, P.; Wang, W.; Huang, H. Cracking Risk and Overall Stability Analysis of Xulong High Arch Dam: A Case Study. Appl. Sci. 2018, 8, 2555. [Google Scholar] [CrossRef]
- Safiuddin, M.; Kaish, A.; Woon, C.; Raman, S. Early-Age Cracking in Concrete: Causes, Consequences, Remedial Measures, and Recommendations. Appl. Sci. 2018, 8, 1730. [Google Scholar] [CrossRef]
- Mi, Z.; Hu, Y.; Li, Q.; An, Z. Effect of curing humidity on the fracture properties of concrete. Constr. Build. Mater. 2018, 169, 403–413. [Google Scholar] [CrossRef]
- Mi, Z.; Hu, Y.; Li, Q.; Zhu, H. Elevated temperature inversion phenomenon in fracture properties of concrete and its application to maturity model. Eng. Fract. Mech. 2018, 199, 294–307. [Google Scholar] [CrossRef]
- Liu, L.; Ouyang, J.; Li, F.; Xin, J.; Huang, D.; Gao, S. Research on the Crack Risk of Early-Age Concrete under the Temperature Stress Test Machine. Materials 2018, 11, 1822. [Google Scholar] [CrossRef] [PubMed]
- Mi, Z.; Hu, Y.; Li, Q.; Gao, X.; Yin, T. Maturity model for fracture properties of concrete considering coupling effect of curing temperature and humidity. Constr. Build. Mater. 2019, 196, 1–13. [Google Scholar] [CrossRef]
- Li, J.; Gao, X.; Fu, X.; Wu, C.; Lin, G. A Nonlinear Crack Model for Concrete Structure Based on an Extended Scaled Boundary Finite Element Method. Appl. Sci. 2018, 8, 1067. [Google Scholar] [CrossRef]
- Guan, J.; Li, Q.; Wu, Z.; Zhao, S.; Dong, W.; Zhou, S. Minimum specimen size for fracture parameters of site-casting dam concrete. Constr. Build. Mater. 2015, 93, 973–982. [Google Scholar] [CrossRef]
- Raphael, J. Tensile Strength of Concrete. J. Am. Concrete Inst. 1984, 81, 158–165. [Google Scholar]
- Wu, M.; Zang, C.; Chen, Z. Study on the direct tensile, splitting and flexure strengths of concrete. J. Hydraul. Eng. 2015, 46, 981–988. [Google Scholar]
- Bažant, Z. Size effect in blunt fracture: Concrete, rock, metal. J. Eng. Mech. 1984, 110, 518–535. [Google Scholar] [CrossRef]
- Bažant, Z. Scaling theory for quasibrittle structural failure. Proc. Natl. Acad. Sci. USA 2004, 101, 13400–13407. [Google Scholar] [CrossRef]
- Bažant, Z. Scaling of Structural Strength; Butterworth-Heinemann: Oxford, UK, 2005. [Google Scholar]
- Bažant, Z.; Kazemi, M. Size dependence of concrete fracture energy determined by RILEM work-of-fracture method. Int. J. Fract. 1991, 51, 121–138. [Google Scholar]
- Maimí, P.; González, E.; Gascons, N.; Ripoll, L. Size effect law and critical distance theories to predict the nominal strength of quasibrittle structures. Appl. Mech. Rev. 2013, 65, 020803. [Google Scholar] [CrossRef]
- Hu, X. An asymptotic approach to size effect on fracture toughness and fracture energy of composites. Eng. Fract. Mech. 2002, 69, 555–564. [Google Scholar] [CrossRef]
- Duan, K.; Hu, X.; Wittmann, F. Scaling of quasi-brittle fracture: Boundary and size effect. Mech. Mater. 2006, 38, 128–141. [Google Scholar] [CrossRef]
- Hoover, C.; Bažant, Z. Comparison of the Hu-Duan boundary effect model with the size-shape effect law for quasi-brittle fracture based on new comprehensive fracture tests. J. Eng. Mech. 2014, 140, 480–486. [Google Scholar] [CrossRef]
- Wang, Y.; Hu, X. Determination of tensile strength and fracture toughness of granite using notched three-point-bend samples. Rock Mech. Rock Eng. 2017, 50, 17–28. [Google Scholar] [CrossRef]
- Hu, X.; Guan, J.; Wang, Y.; Keating, A.; Yang, S. Comparison of boundary and size effect models based on new developments. Eng. Fract. Mech. 2017, 175, 146–167. [Google Scholar] [CrossRef]
- Guan, J.; Hu, X.; Xie, C.; Li, Q.; Wu, Z. Wedge-splitting tests for tensile strength and fracture toughness of concrete. Theor. Appl. Fract. Mech. 2018, 93, 263–275. [Google Scholar] [CrossRef]
- Zhang, C.; Hu, X.; Wu, Z.; Li, Q. Influence of grain size on granite strength and toughness with reliability specified by normal distribution. Theor. Appl. Fract. Mech. 2018, 96, 534–544. [Google Scholar] [CrossRef]
- Zhang, C.; Hu, X.; Sercombe, T.; Li, Q.; Wu, Z.; Lu, P. Prediction of ceramic fracture with normal distribution pertinent to grain size. Acta Mater. 2018, 145, 41–48. [Google Scholar] [CrossRef]
- Gao, X.; Koval, G.; Chazallon, C. A Size and Boundary Effects Model for Quasi-Brittle Fracture. Materials 2016, 9, 1030. [Google Scholar] [CrossRef]
- Gao, X.; Koval, G.; Chazallon, C. Energetical formulation of size effect law for quasi-brittle fracture. Eng. Fract. Mech. 2017, 175, 279–292. [Google Scholar] [CrossRef]
- Zhang, X.; Xu, S.; Gao, H. Superposition calculation of double-K fracture parameters of concrete using wedge splitting geometry and boundary effect. J. Dalian Univ. Technol. 2006, 46, 868–874. [Google Scholar]
- Zhang, X.; Xu, S.; Zheng, S. Experimental measurement of double-K fracture parameters of concrete with small-size aggregates. Front. Archit. Civ. Eng. China 2007, 1, 448–457. [Google Scholar] [CrossRef]
- Wittmann, F.; Rokugo, K.; Brühwiler, E.; Mihashi, H.; Simonin, P. Fracture energy and strain softening of concrete as determined by means of compact tension specimens. Mater. Struct. 1988, 21, 21–32. [Google Scholar] [CrossRef]
- Xu, S.; Zhao, Y.; Wu, Z. Study on the average fracture energy for crack propagation in concrete. J. Mater. Civ. Eng. 2006, 18, 817–824. [Google Scholar] [CrossRef]
- Wu, Z.; Zhao, G. Influences of relative crack length a0/h of concrete specimens on crack propagation process and fracture toughness. J. Basic Sci. Eng. 1995, 3, 126–130. [Google Scholar]
- Wu, Z.; Xu, S.; Lu, J.; Liu, Y. Influence of specimen initial crack length on double-K fracture parameter of concrete. J. Hydraul. Eng. 2000, 31, 35–39. [Google Scholar]
- Wang, Y.; Hu, X.; Liang, L.; Zhu, W. Determination of tensile strength and fracture toughness of concrete using notched 3-p-b specimens. Eng. Fract. Mech. 2016, 160, 67–77. [Google Scholar] [CrossRef]
- Tada, H.; Paris, P.; Irwin, G. The Stress Analysis of Cracks Handbook; Wiley: Hoboken, NJ, USA, 2000. [Google Scholar]
- Xu, S.; Reinhardt, H. Determination of double-K criterion for crack propagation in quasi-brittle fracture, Part III: Compact tension specimens and wedge splitting specimens. Int. J. Fract. 1999, 98, 179–193. [Google Scholar] [CrossRef]
- Irwin, G. Analysis of stresses and strains near the end of a crack traversing a plate. J. Appl. Mech. 1957, 24, 361–364. [Google Scholar]
- Gao, X. Modelling of Nominal Strength Prediction for Quasi-Brittle Materials. Application to Discrete Element Modelling of Damage and Fracture of Asphalt Concrete Under Fatigue Loading. Ph.D. Thesis, Université de Strasbourg, Strasbourg, France, 2017. [Google Scholar]
- Cusatis, G.; Schauffert, E. Cohesive crack analysis of size effect. Eng. Fract. Mech. 2009, 76, 2163–2173. [Google Scholar] [CrossRef]
- Yu, Q.; Le, J.; Hoover, C.; Bažant, Z. Problems with Hu-Duan boundary effect model and its comparison to size-shape effect law for quasi-brittle fracture. J. Eng. Mech. 2009, 136, 40–50. [Google Scholar] [CrossRef]

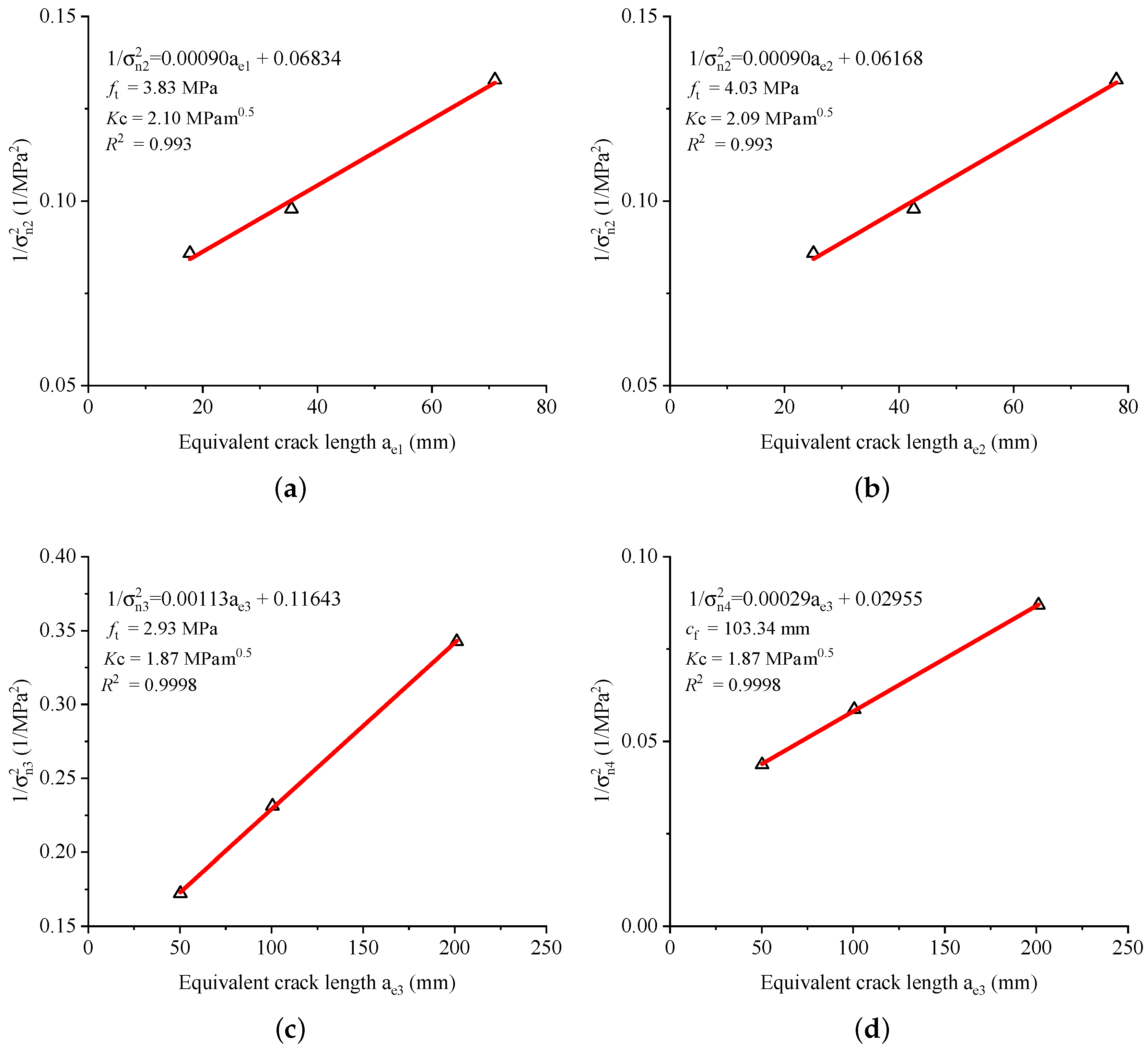
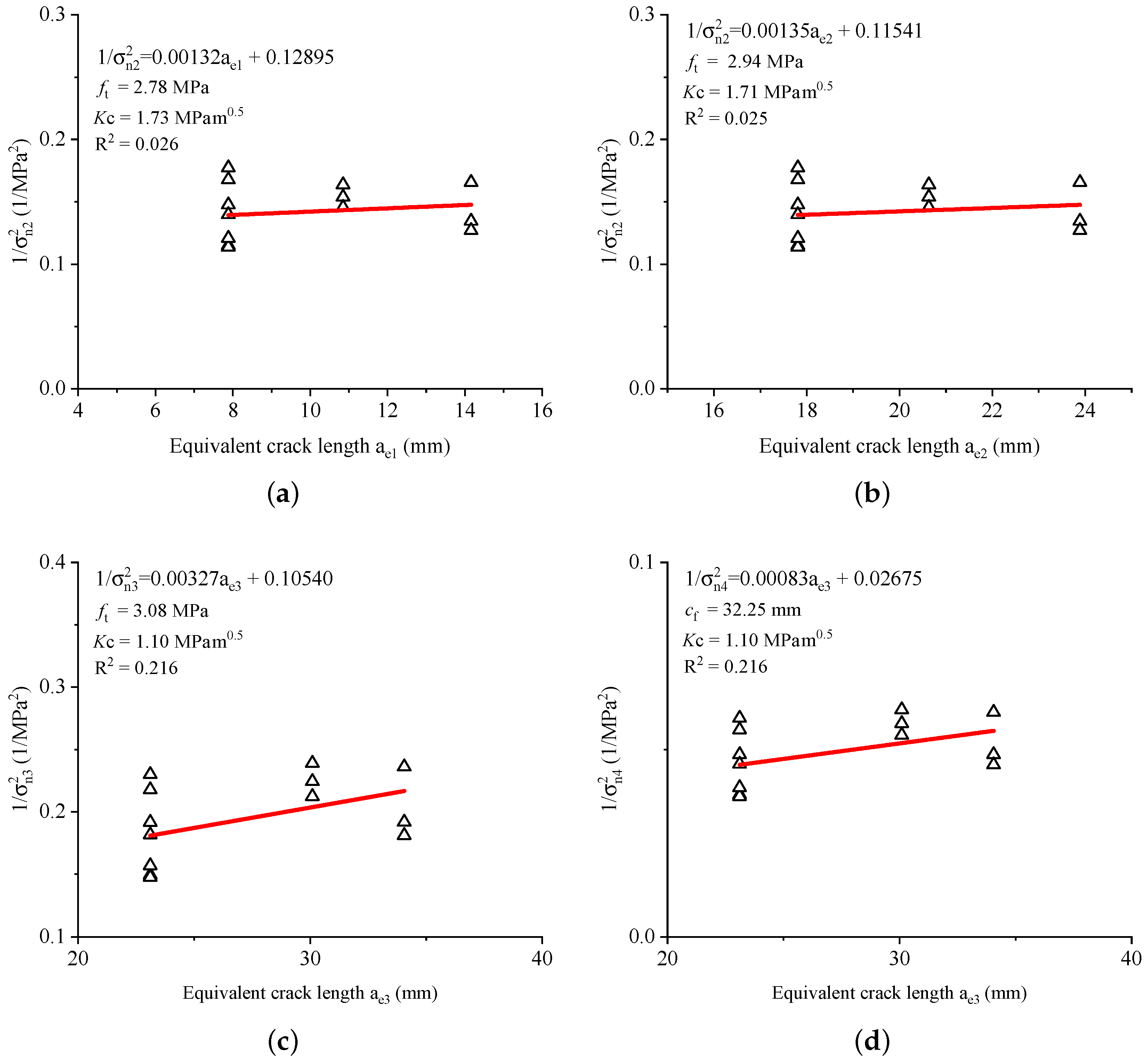
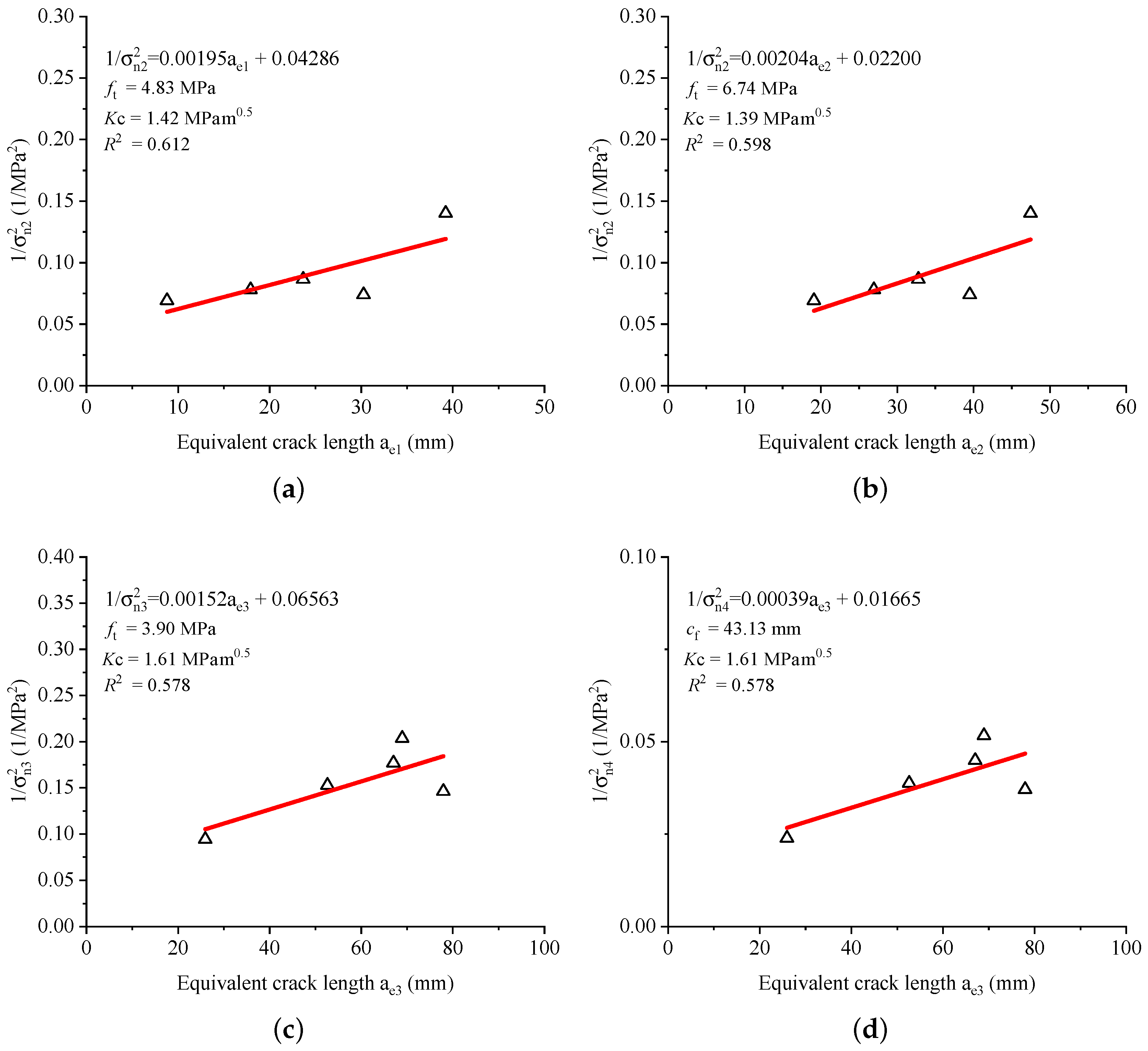
| Label | W (mm) | t (mm) | (kN) | (mm) | (mm) | (mm) | (Mpa) | (Mpa) | |
|---|---|---|---|---|---|---|---|---|---|
| WS150-1 | 150 | 0.4638 | 200 | 4.73405 | 9.75 | 14.41 | 26.89 | 0.631 | 2.225 |
| WS150-2 | 150 | 0.477 | 193 | 5.59249 | 9.43 | 14.08 | 26.27 | 0.773 | 2.863 |
| WS150-3 | 150 | 0.4868 | 200 | 5.22936 | 9.19 | 13.84 | 25.8 | 0.697 | 2.683 |
| WS200-1s | 200 | 0.4579 | 200 | 7.08092 | 13.19 | 17.78 | 36.2 | 0.708 | 2.551 |
| WS200-2s | 200 | 0.4703 | 200 | 6.885 | 12.79 | 17.36 | 35.45 | 0.689 | 2.601 |
| WS200-3d | 200 | 0.4703 | 200 | 6.61111 | 12.79 | 17.36 | 35.45 | 0.661 | 2.498 |
| WS400-1 | 400 | 0.4527 | 200 | 11.37149 | 26.73 | 31.19 | 72.99 | 0.569 | 2.155 |
| WS400-2 | 400 | 0.4602 | 198 | 14.27358 | 26.23 | 30.69 | 72.12 | 0.721 | 2.814 |
| WS400-3 | 400 | 0.4869 | 199 | 12.00834 | 24.5 | 28.93 | 68.78 | 0.603 | 2.623 |
| WS600-1 | 600 | 0.4618 | 193 | 17.82212 | 39.19 | 43.6 | 107.9 | 0.616 | 2.48 |
| WS600-2 | 599 | 0.4551 | 200 | 16.97275 | 39.78 | 44.21 | 108.9 | 0.567 | 2.223 |
| WS600-3 | 600 | 0.4551 | 193 | 16.68785 | 39.85 | 44.27 | 109.08 | 0.576 | 2.261 |
| WS600-5 | 599 | 0.4551 | 200 | 18.22867 | 39.78 | 44.21 | 108.9 | 0.609 | 2.387 |
| WS800-1 | 799 | 0.4624 | 196 | 18.65977 | 52.11 | 56.5 | 143.54 | 0.477 | 1.95 |
| WS800-2 | 800 | 0.4624 | 194 | 22.60376 | 52.17 | 56.56 | 143.72 | 0.583 | 2.384 |
| WS800-4 | 798 | 0.4539 | 200 | 21.62546 | 53.16 | 57.56 | 145.35 | 0.542 | 2.143 |
| WS800-5 | 801 | 0.4577 | 200 | 21.98917 | 52.86 | 57.25 | 145.02 | 0.549 | 2.204 |
| WS1000-1 | 997 | 0.4521 | 200 | 22.14598 | 66.71 | 71.1 | 182.11 | 0.444 | 1.757 |
| WS1000-3 | 997 | 0.4531 | 200 | 23.33836 | 66.55 | 70.94 | 181.82 | 0.468 | 1.86 |
| WS1000-4 | 999 | 0.4522 | 196 | 25.81247 | 66.83 | 71.22 | 182.44 | 0.527 | 2.087 |
| WS1000-5 | 1000 | 0.4506 | 200 | 24.4032 | 67.16 | 71.56 | 183.08 | 0.488 | 1.919 |
| WS1200-0 | 1198 | 0.4597 | 200 | 24.7045 | 78.66 | 83.03 | 216.19 | 0.412 | 1.691 |
| WS1200-1 | 1200 | 0.4554 | 201 | 24.2143 | 79.64 | 84.02 | 218.05 | 0.402 | 1.618 |
| WS1200-2 | 1200 | 0.4545 | 200 | 28.2619 | 79.82 | 84.2 | 218.36 | 0.471 | 1.891 |
| Series | W (mm) | t (mm) | (kN) | (mm) | (mm) | (mm) | (MPa) | (MPa) | |
|---|---|---|---|---|---|---|---|---|---|
| Small | 300 | 0.5 | 120 | 7.30 | 17.76 | 42.18 | 50.27 | 0.811 | 2.631 |
| Medium | 600 | 0.5 | 120 | 12.60 | 35.51 | 58.10 | 100.54 | 0.700 | 2.736 |
| Large | 1200 | 0.5 | 120 | 20.70 | 71.02 | 92.58 | 201.09 | 0.575 | 2.518 |
| Label | W (mm) | t (mm) | (kN) | (mm) | (mm) | (mm) | (MPa) | (MPa) | |
|---|---|---|---|---|---|---|---|---|---|
| WS70-1 | 170 | 0.353 | 200 | 10.71 | 14.16 | 23.89 | 34.05 | 1.260 | 2.727 |
| WS70-2 | 170 | 0.353 | 200 | 9.65 | 14.16 | 23.89 | 34.05 | 1.135 | 2.457 |
| WS70-3 | 170 | 0.353 | 200 | 11.02 | 14.16 | 23.89 | 34.05 | 1.296 | 2.806 |
| WS90-1 | 170 | 0.471 | 200 | 6.56 | 10.85 | 20.63 | 30.1 | 0.772 | 2.471 |
| WS90-2 | 170 | 0.471 | 200 | 6.77 | 10.85 | 20.63 | 30.1 | 0.796 | 2.550 |
| WS90-3 | 170 | 0.471 | 200 | 6.96 | 10.85 | 20.63 | 30.1 | 0.819 | 2.621 |
| WS110-1 | 170 | 0.588 | 200 | 4.09 | 7.88 | 17.81 | 23.11 | 0.481 | 2.441 |
| WS110-2 | 170 | 0.588 | 198 | 4.36 | 7.88 | 17.81 | 23.11 | 0.513 | 2.602 |
| WS110-3 | 170 | 0.588 | 199 | 4.94 | 7.88 | 17.81 | 23.11 | 0.581 | 2.948 |
| WS110-4 | 170 | 0.588 | 193 | 3.98 | 7.88 | 17.81 | 23.11 | 0.468 | 2.375 |
| WS110-5 | 170 | 0.588 | 200 | 4.48 | 7.88 | 17.81 | 23.11 | 0.527 | 2.674 |
| WS110-6 | 170 | 0.588 | 193 | 4.94 | 7.88 | 17.81 | 23.11 | 0.581 | 2.948 |
| WS110-7 | 170 | 0.588 | 200 | 4.97 | 7.88 | 17.81 | 23.11 | 0.585 | 2.966 |
| WS110-8 | 170 | 0.588 | 196 | 4.82 | 7.88 | 17.81 | 23.11 | 0.567 | 2.877 |
| Label | W (mm) | t (mm) | (kN) | (mm) | (mm) | (mm) | (MPa) | (MPa) | |
|---|---|---|---|---|---|---|---|---|---|
| WS1 | 400 | 0.2 | 200 | 34.20 | 39.22 | 47.46 | 68.95 | 1.710 | 2.672 |
| WS2 | 400 | 0.4 | 200 | 25.20 | 30.25 | 39.51 | 77.94 | 1.260 | 3.675 |
| WS3 | 400 | 0.5 | 200 | 16.00 | 23.67 | 32.77 | 67.03 | 0.800 | 3.400 |
| WS4 | 400 | 0.6 | 200 | 10.80 | 17.92 | 26.95 | 52.63 | 0.540 | 3.578 |
| WS5 | 400 | 0.8 | 200 | 3.20 | 8.79 | 19.09 | 25.94 | 0.160 | 3.800 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gao, X.; Liu, C.; Tan, Y.; Yang, N.; Qiao, Y.; Hu, Y.; Li, Q.; Koval, G.; Chazallon, C. Determination of Fracture Properties of Concrete Using Size and Boundary Effect Models. Appl. Sci. 2019, 9, 1337. https://doi.org/10.3390/app9071337
Gao X, Liu C, Tan Y, Yang N, Qiao Y, Hu Y, Li Q, Koval G, Chazallon C. Determination of Fracture Properties of Concrete Using Size and Boundary Effect Models. Applied Sciences. 2019; 9(7):1337. https://doi.org/10.3390/app9071337
Chicago/Turabian StyleGao, Xiaofeng, Chunfeng Liu, Yaosheng Tan, Ning Yang, Yu Qiao, Yu Hu, Qingbin Li, Georg Koval, and Cyrille Chazallon. 2019. "Determination of Fracture Properties of Concrete Using Size and Boundary Effect Models" Applied Sciences 9, no. 7: 1337. https://doi.org/10.3390/app9071337
APA StyleGao, X., Liu, C., Tan, Y., Yang, N., Qiao, Y., Hu, Y., Li, Q., Koval, G., & Chazallon, C. (2019). Determination of Fracture Properties of Concrete Using Size and Boundary Effect Models. Applied Sciences, 9(7), 1337. https://doi.org/10.3390/app9071337





