Two-Dimensional Spatial Coherence for Ultrasonic DMAS Beamforming in Multi-Angle Plane-Wave Imaging
Abstract
1. Introduction
2. Theory
2.1. BB-DMAS Beamforming for Single-Angle PW Imaging
2.2. 2D-DMAS Processing for Multi-Angle PW Imaging
2.3. Relationship between 2D-DMAS and 2D-DAS
3. Research Method
4. Results
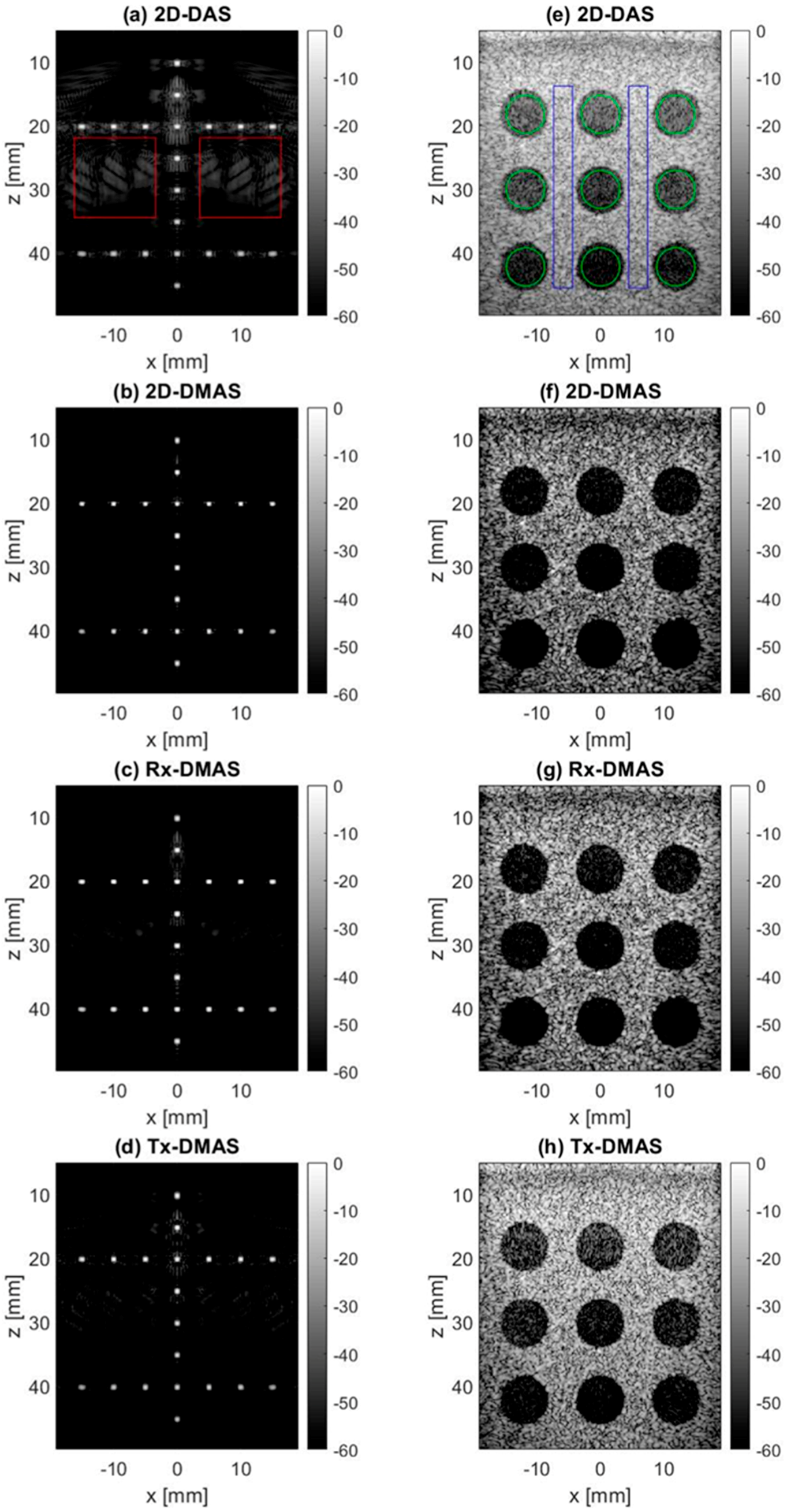
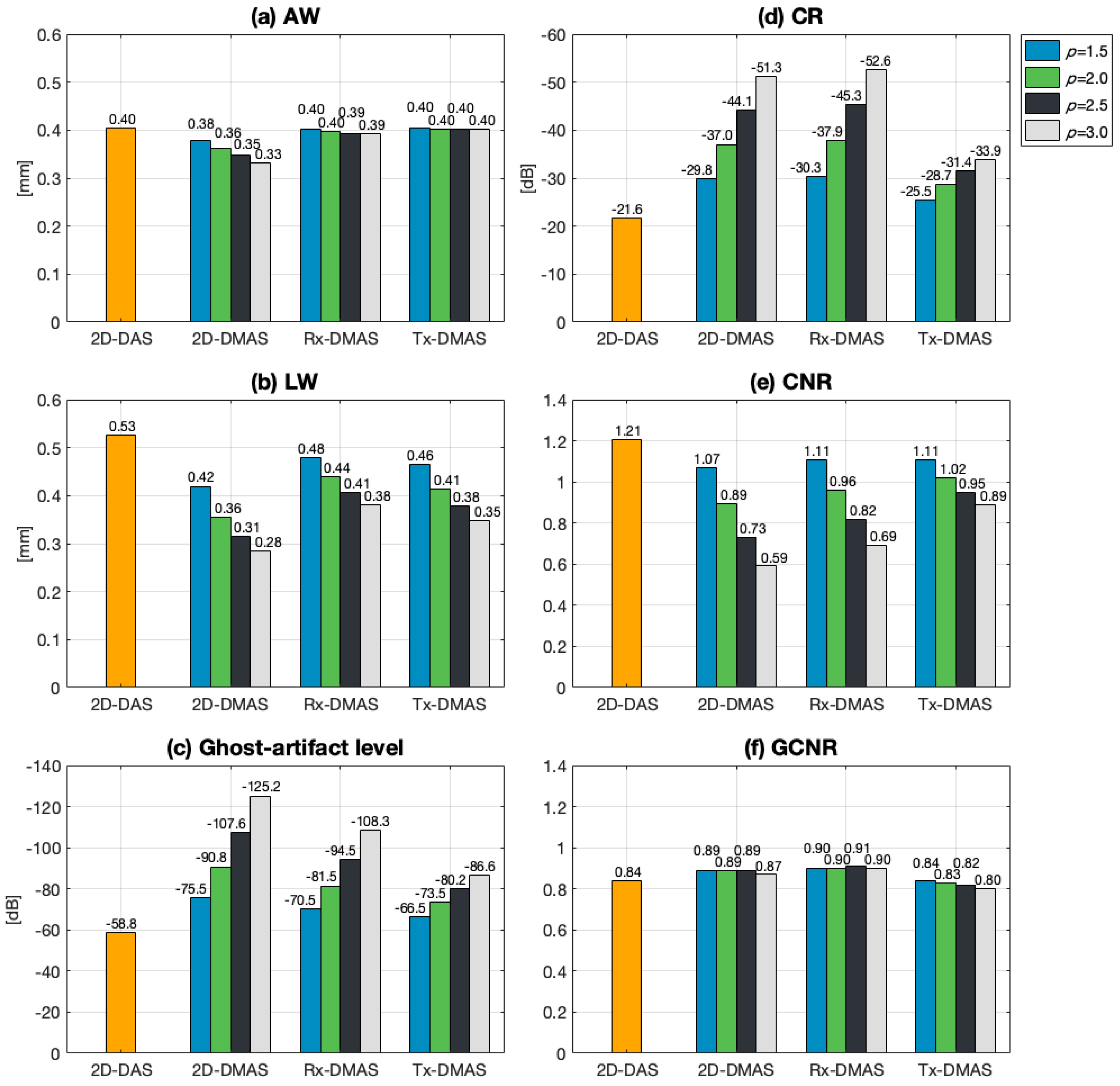
4.1. Phantom Simulations
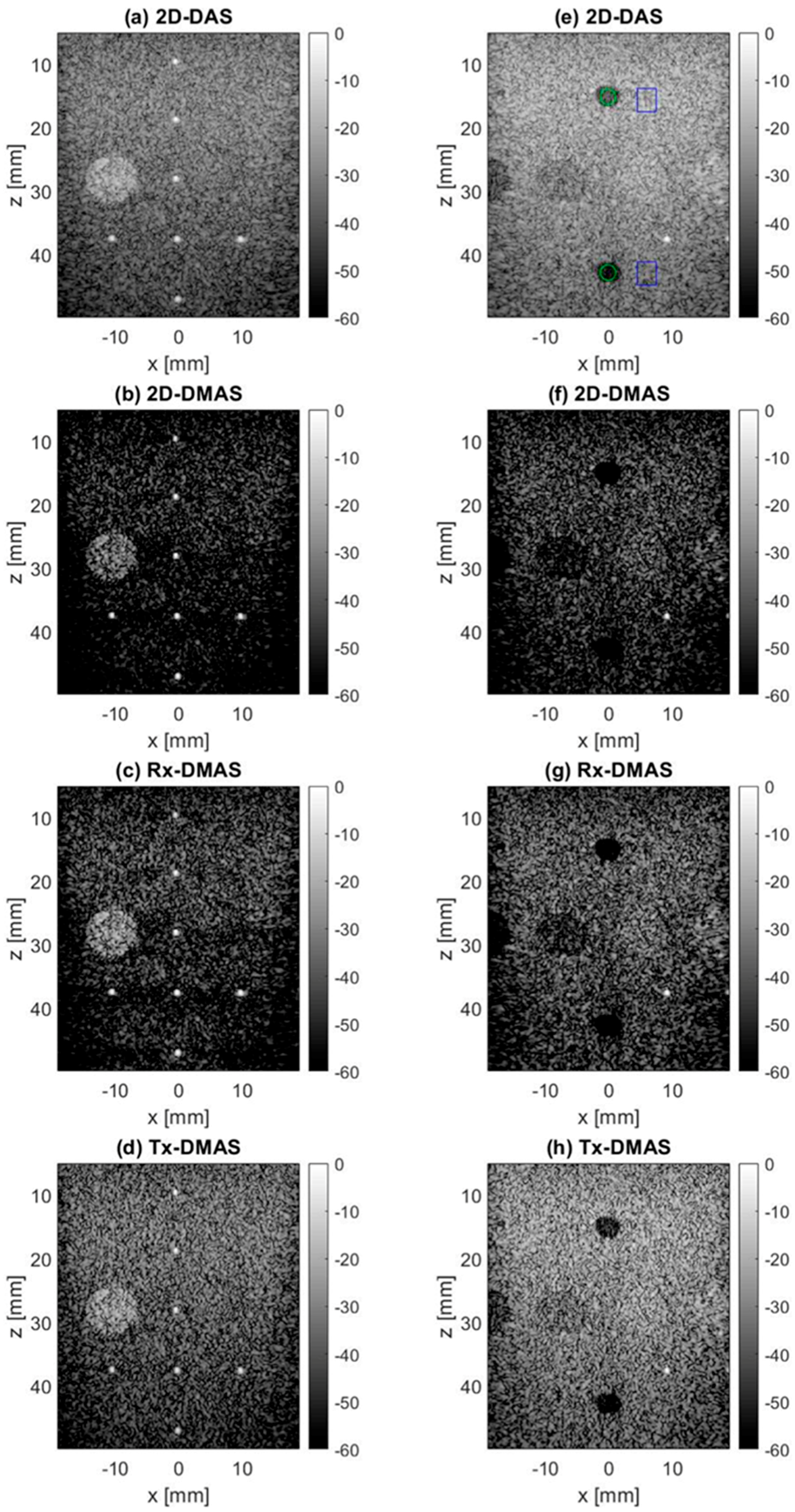
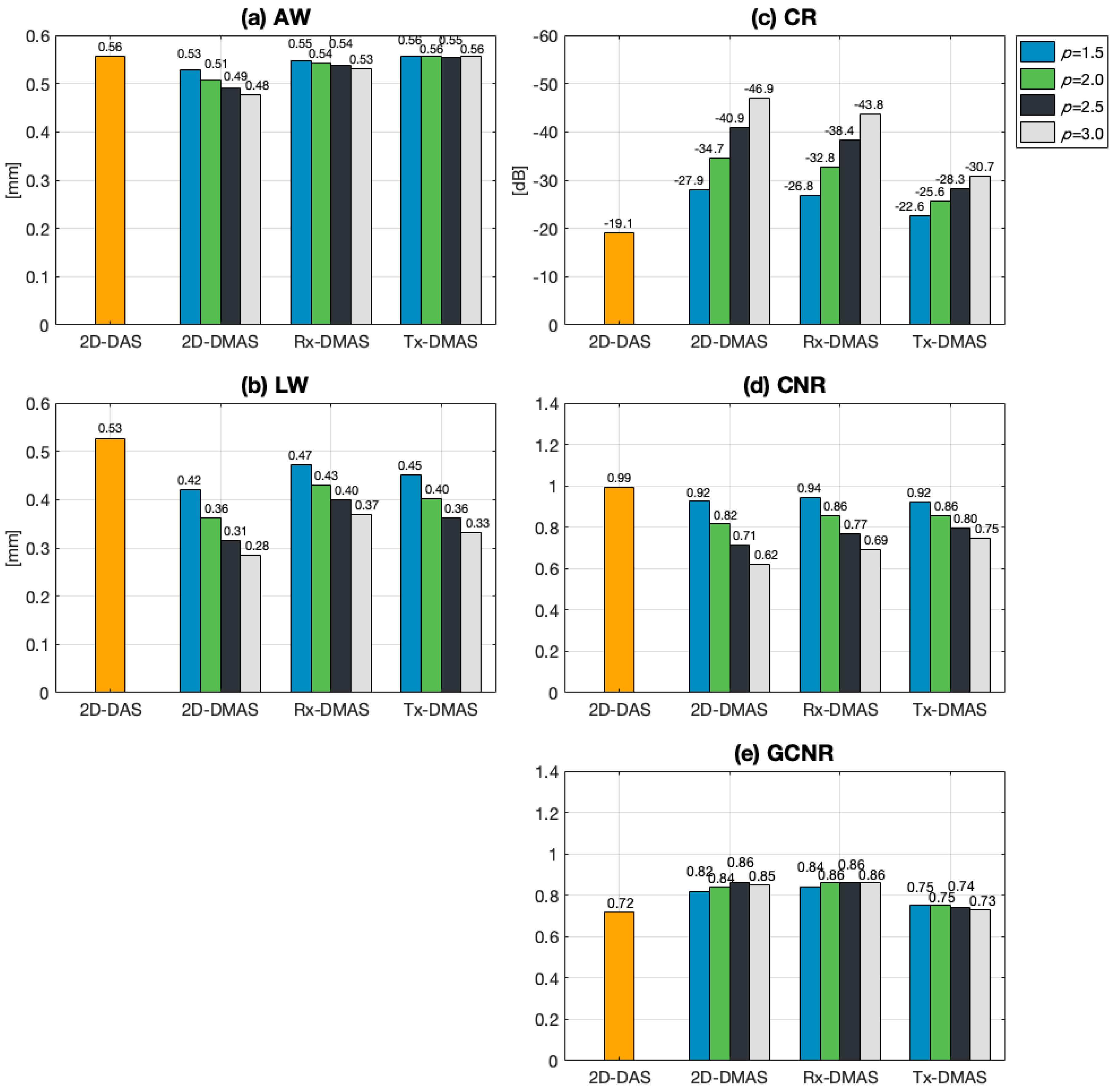
4.2. Phantom Experiments
4.3. In Vivo Experiments
5. Discussions and Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Sandrin, L.; Catheline, S.; Tanter, M.; Hennequin, X.; Fink, M. Time-resolved pulsed elastography with ultrafast ultrasonic imaging. Ultrason. Imaging 1999, 21, 259–272. [Google Scholar] [CrossRef]
- Tanter, M.; Fink, M. Ultrafast imaging in biomedical ultrasound. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2014, 61, 102–119. [Google Scholar] [CrossRef] [PubMed]
- Montaldo, G.; Tanter, M.; Bercoff, J.; Benech, N.; Fink, M. Coherent plane-wave compounding for very high frame rate ultrasonography and transient elastography. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2009, 56, 489–506. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Y.; Guo, Y.; Lee, W.N. Ultrafast ultrasound imaging using combined transmissions with cross-coherence-based reconstruction. IEEE Trans. Med. Imaging 2018, 37, 337–348. [Google Scholar] [CrossRef] [PubMed]
- Zhao, J.; Wang, Y.; Zeng, X.; Yu, J.; Yiu, B.Y.S.; Yu, A.C.H. Plane wave compounding based on a joint transmitting-receiving adaptive beamformer. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2015, 62, 1440–1452. [Google Scholar] [CrossRef] [PubMed]
- Rindal, O.M.H.; Austeng, A. Double adaptive plane-wave imaging. In Proceedings of the 2016 IEEE International Ultrasonics Symposium (IUS), Tours, France, 18–21 September 2016. [Google Scholar]
- Nguyen, N.Q.; Prager, R.W. A spatial coherence approach to minimum variance beamforming for plane-wave compounding. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2018, 65, 522–534. [Google Scholar] [CrossRef]
- Hollman, K.W.; Rigby, K.W.; O’Donnell, M. Coherence factor of speckle from a multi-row probe. In Proceedings of the 1999 IEEE International Ultrasonics Symposium (IUS), Lake Tahoe, NV, USA, 17–20 October 1999. [Google Scholar]
- Asl, B.M.; Mahloojifar, A. Minimum variance beamforming combined with adaptive coherence weighting applied to medical ultrasound imaging. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2009, 56, 1923–1931. [Google Scholar] [CrossRef]
- Nilsen, C.-I.C.; Holm, S. Wiener beamforming and the coherence factor in ultrasound imaging. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2010, 57, 1329–1346. [Google Scholar] [CrossRef] [PubMed]
- Qi, Y.; Wang, Y.; Guo, W. Joint subarray coherence and minimum variance beamformer for multitransmission ultrasound Imaging modalities. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2018, 65, 1600–1617. [Google Scholar] [CrossRef]
- Li, P.C.; Li, M.L. Adaptive imaging using the generalized coherence factor. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2003, 50, 128–141. [Google Scholar]
- Lediju, M.A.; Trahey, G.E.; Byram, B.C.; Dahl, J.J. Short-lag spatial coherence of backscattered echoes: Imaging characteristics. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2011, 58, 1377–1388. [Google Scholar] [CrossRef] [PubMed]
- Pinton, G.F.; Trahey, G.E.; Dahl, J.J. Spatial coherence in human tissue: Implications for imaging and measurement. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2014, 61, 1976–1987. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Wang, Y.; Zheng, C.; Peng, H.; Zhang, C. Coherent plane-wave compounding based on normalized autocorrelation factor. IEEE Access 2018, 6, 36927–36938. [Google Scholar] [CrossRef]
- Guo, W.; Wang, Y.; Yu, J. A sibelobe suppressing beamformer for coherent plane wave compounding. Appl. Sci. 2016, 6, 359. [Google Scholar] [CrossRef]
- Matrone, G.; Savoia, A.S.; Caliano, G.; Magenes, G. The delay multiply and sum beamforming algorithm in ultrasound B-mode medical imaging. IEEE Trans. Med. Imaging 2015, 34, 940–949. [Google Scholar] [CrossRef] [PubMed]
- Matrone, G.; Ramalli, A. Spatial coherence of backscattered signals in multi-line transmit ultrasound imaging and its effect on short-lag filtered-delay multiply and sum beamforming. Appl. Sci. 2018, 8, 486. [Google Scholar] [CrossRef]
- Jeon, S.; Park, E.Y.; Choi, W.; Managuli, R.; Lee, K.; Kim, C. Real-time delay-multiply-and-sum beamforming with coherence factor for in vivo clinical photoacoustic imaging of humans. Photoacoustics 2019, 15, 100136. [Google Scholar] [CrossRef] [PubMed]
- Ramalli, A.; Scaringella, M.; Matrone, G.; Dallai, A.; Boni, E.; Savoia, A.S.; Bassi, L.; Hine, G.E.; Tortoli, P. High dynamic range ultrasound imaging with real-time filtered-delay multiply and sum beamforming. In Proceedings of the 2017 IEEE International Ultrasonics Symposium (IUS), Washington, DC, USA, 6–9 September 2017. [Google Scholar]
- Shen, C.C.; Hsieh, P.Y. Ultrasound baseband delay-multiply-and-sum (BB-DMAS) nonlinear beamforming. Ultrasonics 2019, 96, 165–174. [Google Scholar] [CrossRef]
- Polichetti, M.; Varray, F.; Béra, J.C.; Cachard, C.; Nicolas, B. A nonlinear beamformer based on p-th root compression—Application to plane wave ultrasound imaging. Appl. Sci. 2018, 8, 599. [Google Scholar] [CrossRef]
- Prieur, F.; Rindal, O.M.H.; Austeng, A. Signal coherence and image amplitude with the filtered delay multiply and sum beamformer. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2018, 65, 1133–1140. [Google Scholar] [CrossRef]
- Rindal, O.M.H.; Rodriguez-Molares, A.; Austeng, A. The dark region artifact in adaptive ultrasound beamforming. In Proceedings of the 2017 IEEE International Ultrasonics Symposium (IUS), Washington, DC, USA, 6–9 September 2017. [Google Scholar]
- Matrone, G.; Savoia, A.S.; Caliano, G.; Magenes, G. Ultrasound plane-wave imaging with delay multiply and sum beamforming and coherent compounding. In Proceedings of the 2016 IEEE Conference of Engineering in Medicine and Biology Society (EMBC), Orlando, FL, USA, 17–20 August 2016. [Google Scholar]
- Go, D.; Kang, J.; Yoo, Y. A new compounding method for high contrast ultrafast ultrasound imaging based on delay multiply and sum. In Proceedings of the 2018 IEEE International Ultrasonics Symposium (IUS), Kobe, Japan, 22–25 October 2018. [Google Scholar]
- Liebgott, H.; Rodriguez-Molares, A.; Cervenansky, F.; Jensen, J.A.; Bernard, O. Plane-wave imaging challenge in medical ultrasound. In Proceedings of the 2016 IEEE International Ultrasonics Symposium (IUS), Tours, France, 18–21 September 2016. [Google Scholar]
- Rodriguez-Molares, A.; Rindal, O.M.H.; D’Hooge, J.; Måsøy, S.E.; Austeng, A.; Torp, H. The generalized contrast-to-noise ratio. In Proceedings of the 2018 IEEE International Ultrasonics Symposium (IUS), Kobe, Japan, 22–25 October 2018. [Google Scholar]
- Camacho, J.; Parrilla, M.; Fritsch, C. Phase coherence imaging. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2009, 56, 958–974. [Google Scholar] [CrossRef] [PubMed]
- Matrone, G.; Ramalli, A.; Tortoli, P.; Magenes, G. Experimental evaluation of ultrasound higher-order harmonic imaging with Filtered-Delay Multiply and Sum (F-DMAS) non-linear beamforming. Ultrasonics 2018, 86, 59–68. [Google Scholar] [CrossRef] [PubMed]
- Jensen, A.C.; Nasholm, S.P.; Nilsen, C.C.; Austeng, A.; Holm, S. Applying Thomson’s multitaper approach to reduce speckle in medical ultrasound imaging. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2012, 59, 2178–2185. [Google Scholar] [CrossRef] [PubMed]

| Max. PW angle | ±16° | Number of PW angle | 7 |
| Element pitch | 0.3 mm | Element height | 5 mm |
| Element width | 0.27 mm | Elevation focus | 20 mm |
| Number of elements | 128 | Excitation | 2.5 cycle |
| Center frequency | 5.2 MHz | Sampling frequency | 20.8 MHz |
| Attenuation coefficient | 0.5 dB/(MHz·cm) | Sound velocity | 1540 m/s |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shen, C.-C.; Hsieh, P.-Y. Two-Dimensional Spatial Coherence for Ultrasonic DMAS Beamforming in Multi-Angle Plane-Wave Imaging. Appl. Sci. 2019, 9, 3973. https://doi.org/10.3390/app9193973
Shen C-C, Hsieh P-Y. Two-Dimensional Spatial Coherence for Ultrasonic DMAS Beamforming in Multi-Angle Plane-Wave Imaging. Applied Sciences. 2019; 9(19):3973. https://doi.org/10.3390/app9193973
Chicago/Turabian StyleShen, Che-Chou, and Pei-Ying Hsieh. 2019. "Two-Dimensional Spatial Coherence for Ultrasonic DMAS Beamforming in Multi-Angle Plane-Wave Imaging" Applied Sciences 9, no. 19: 3973. https://doi.org/10.3390/app9193973
APA StyleShen, C.-C., & Hsieh, P.-Y. (2019). Two-Dimensional Spatial Coherence for Ultrasonic DMAS Beamforming in Multi-Angle Plane-Wave Imaging. Applied Sciences, 9(19), 3973. https://doi.org/10.3390/app9193973





