Model-Free Method for Damage Localization of Grid Structure
Abstract
1. Introduction
2. Basic Principle of Structural Damage Localization Based on Displacement Difference
3. Damage Identification of Grid Structure
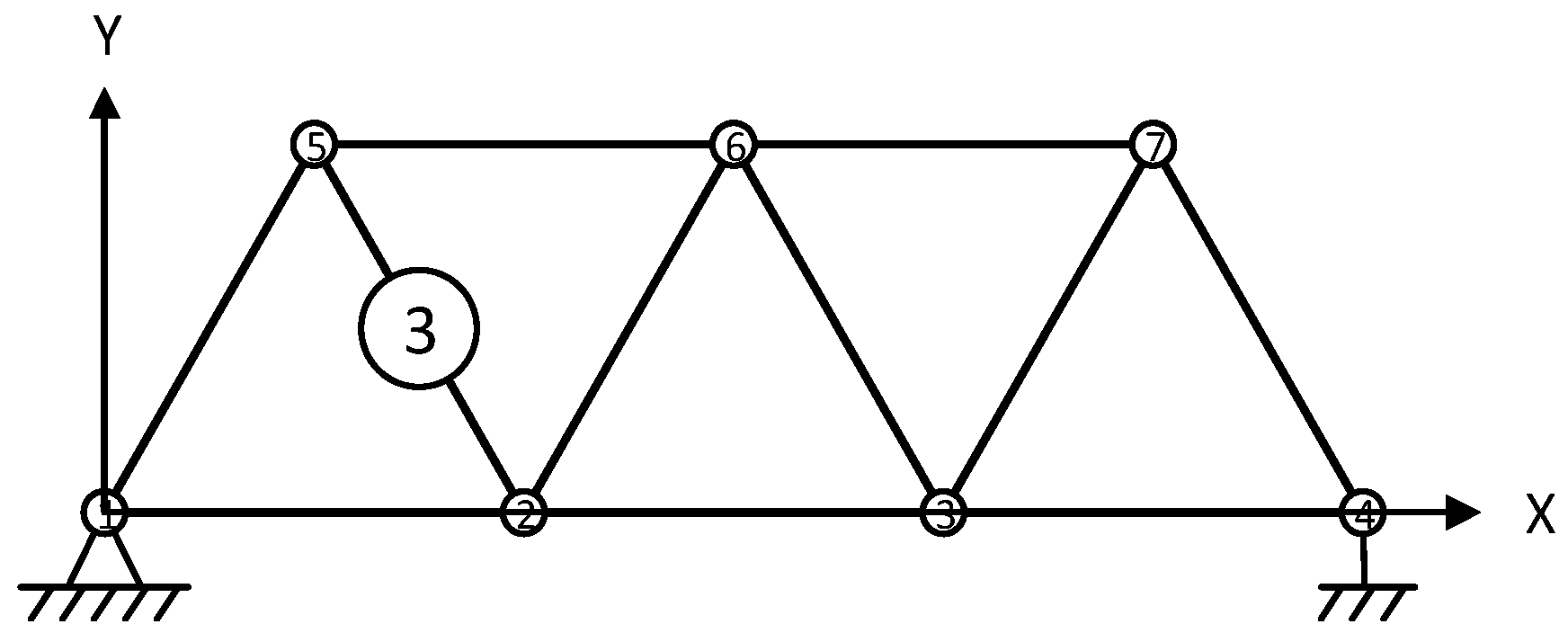
3.1. Characteristic Force and Displacement of Grid Structure
3.2. Damage Localization of Grid Structure Based on Displacement Difference
- (1)
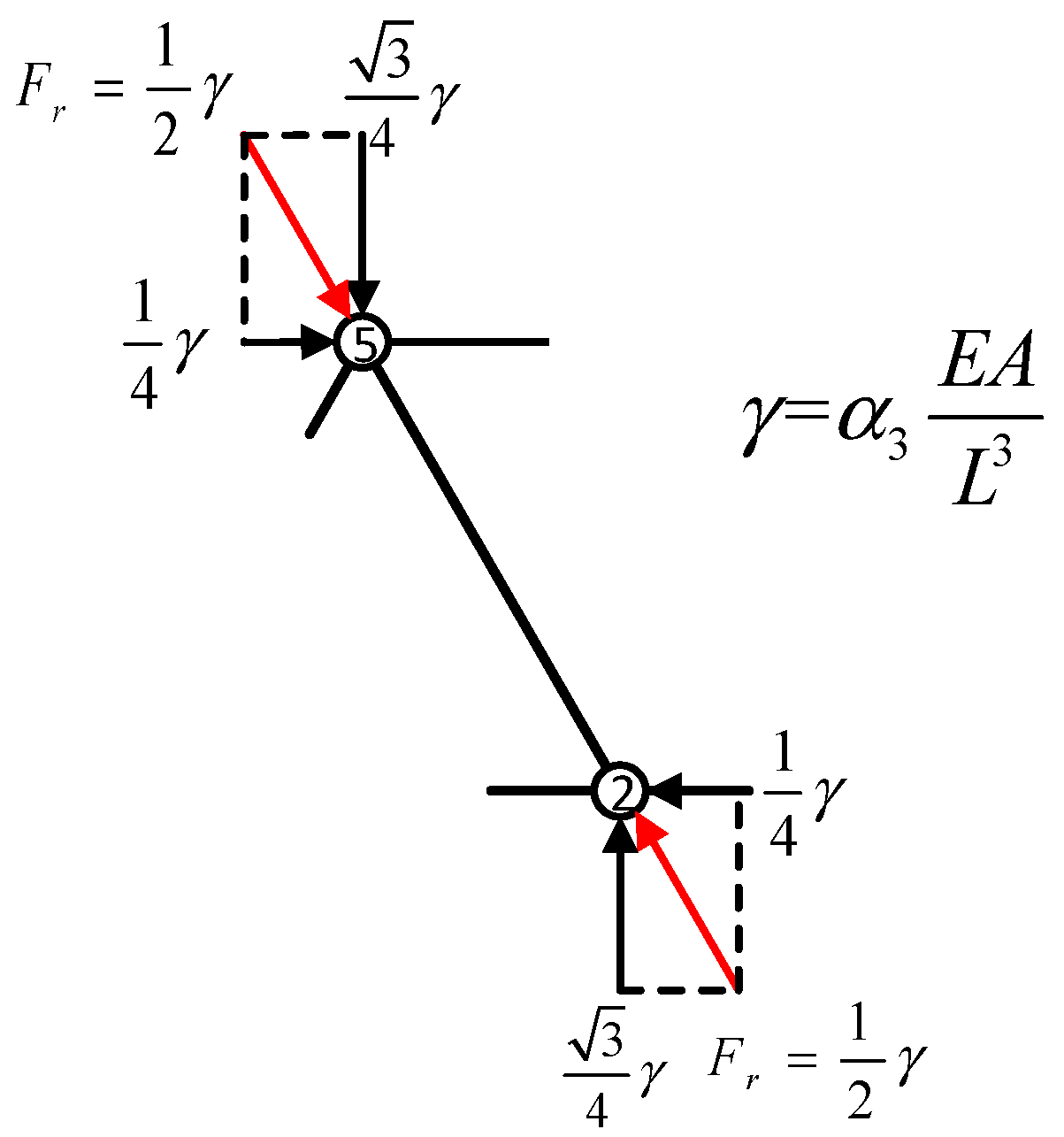
- Obtain the displacement difference ∆u through experiment. It is preferred to use a laser range finder to obtain the displacement difference in the static test. If a static test cannot be performed, we can use the dynamic flexibility method to indirectly obtain the displacement difference (multiply the virtual force vector by the dynamic flexibility difference matrix before and after damage). Similarly, it is not necessary to establish a finite element model when the dynamic flexibility method is used. The approximate flexibility difference matrix can be obtained by the following formula:where F and Fd are the flexibility matrices before and after damage, respectively, m is the measured number of modes, and λj and φj are the jth order eigenvalues and eigenvectors (mode shapes), respectively. The flexibility matrix difference obtained by the dynamic flexibility method was considered in the following equation to obtain the change in displacement:where lv is the virtual force vector. Theoretically, the virtual vector can be selected arbitrarily according to need. For example, lv = [0 --- 1 --- 0]T. The specific steps for the selection of the virtual force vector can be obtained from literature [1];
- (2)
- The length of element after damage was calculated by Equation (16), and the change in length of the element before and after damage was calculated by Equation (18).
- (3)
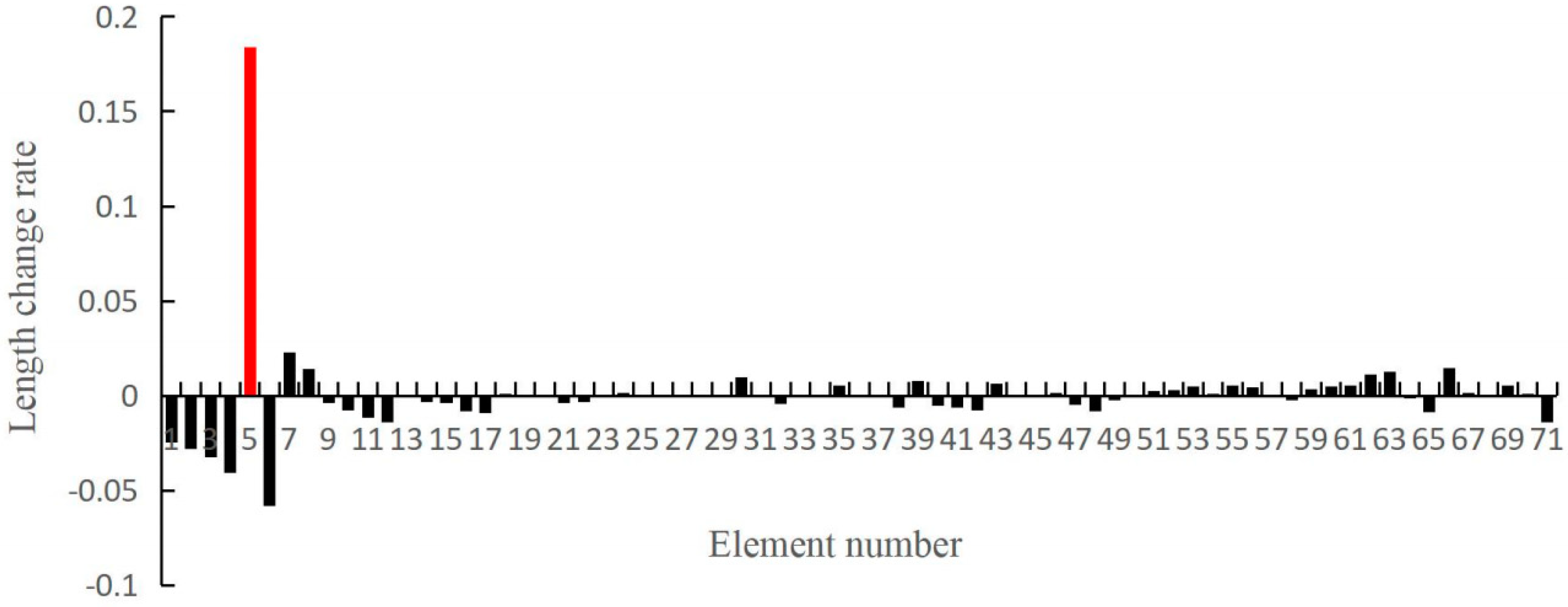
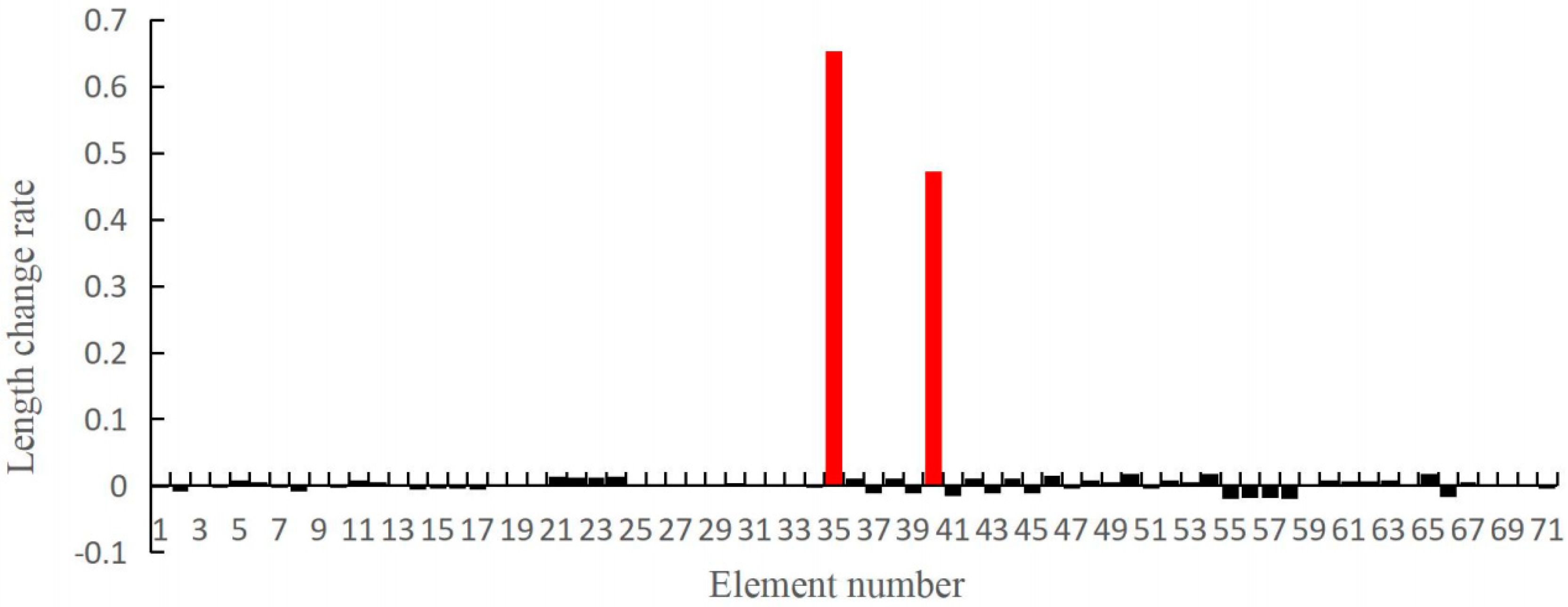
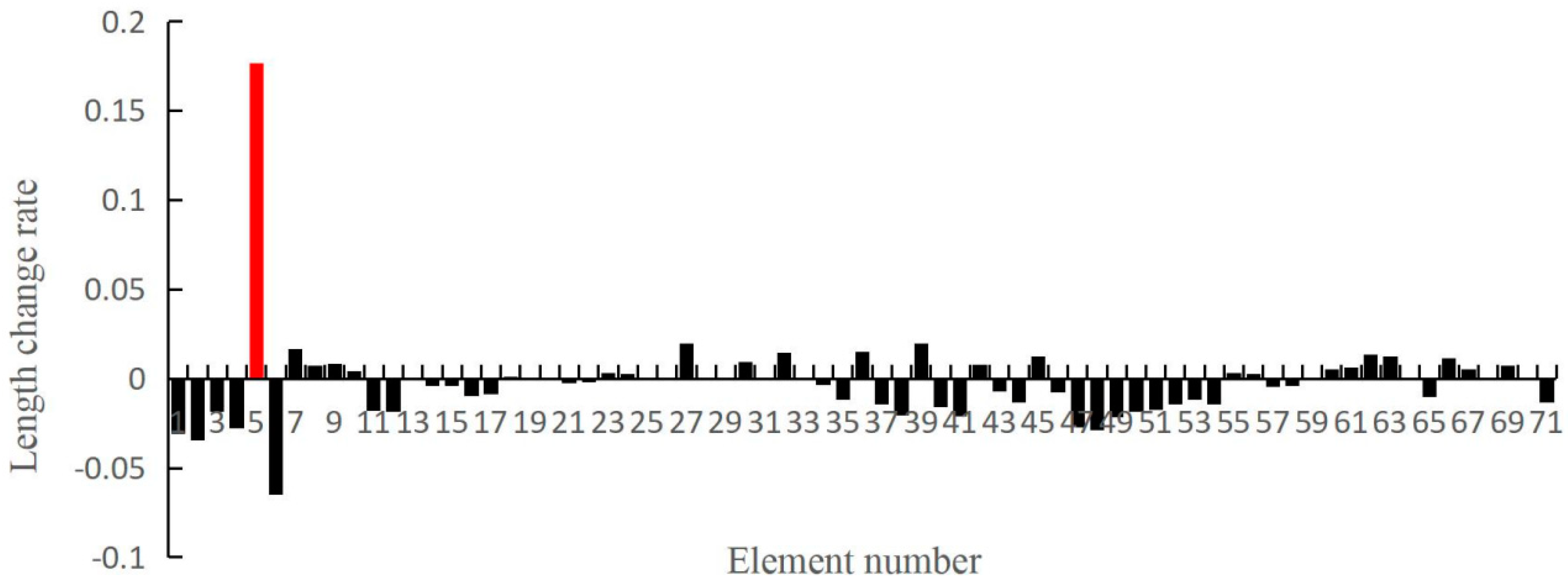
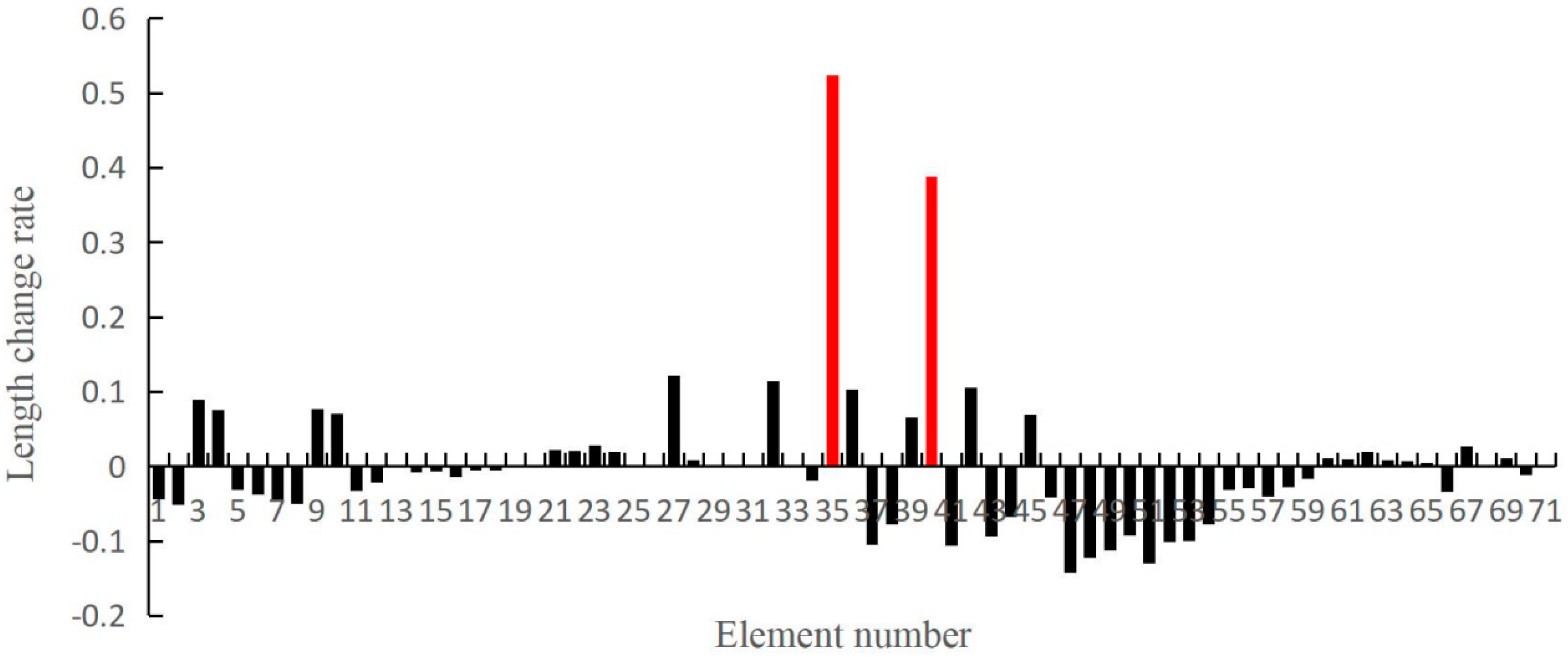
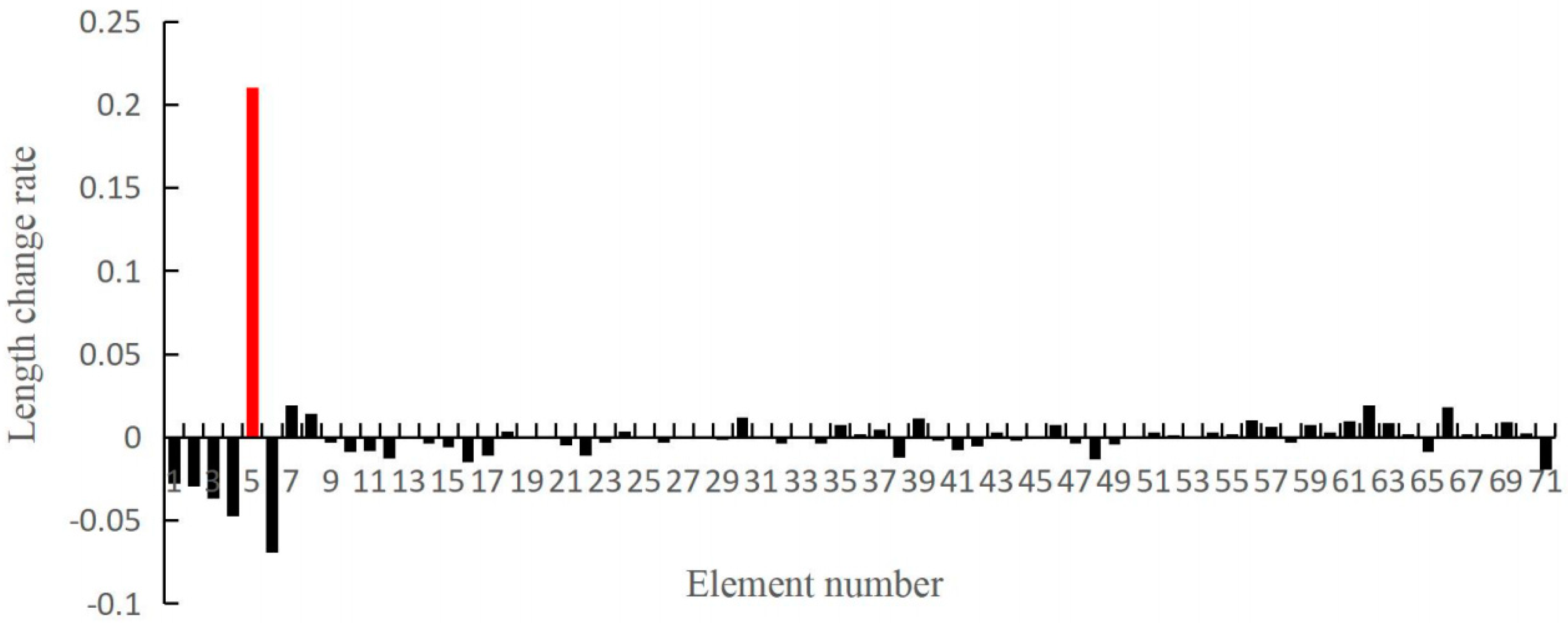
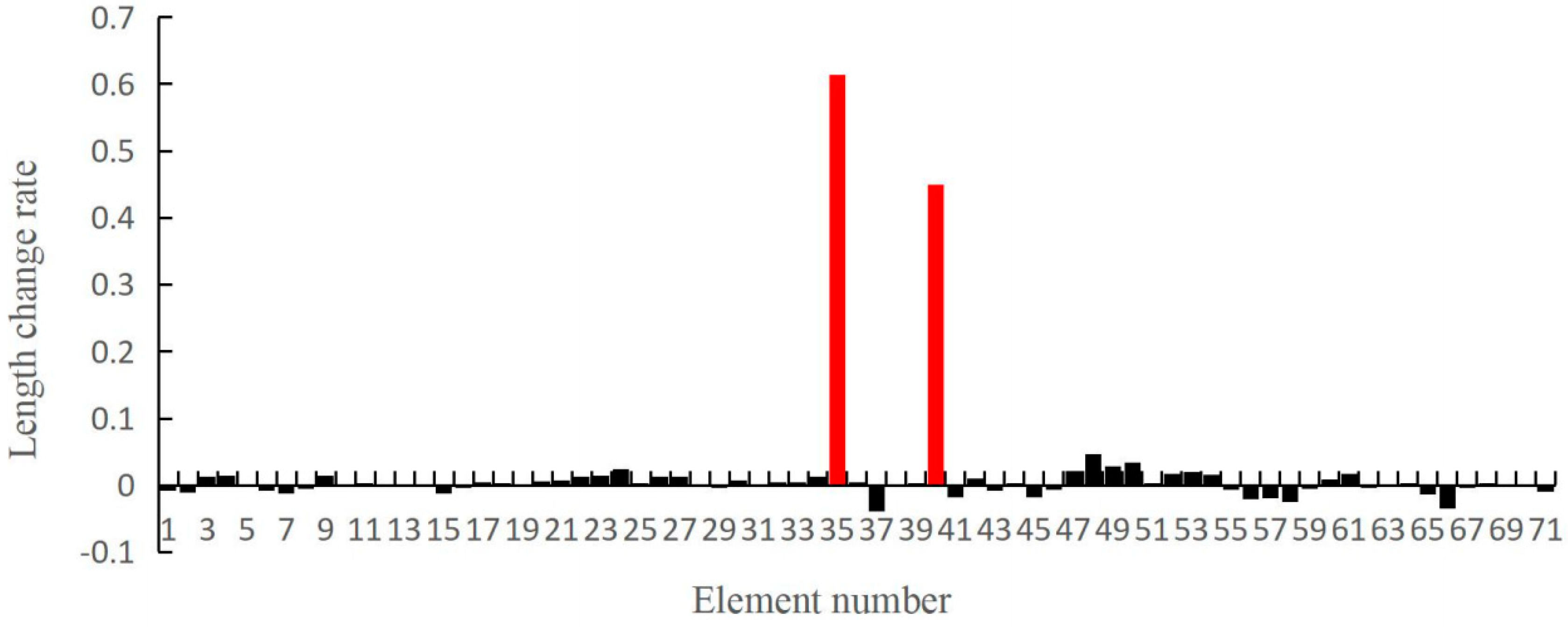
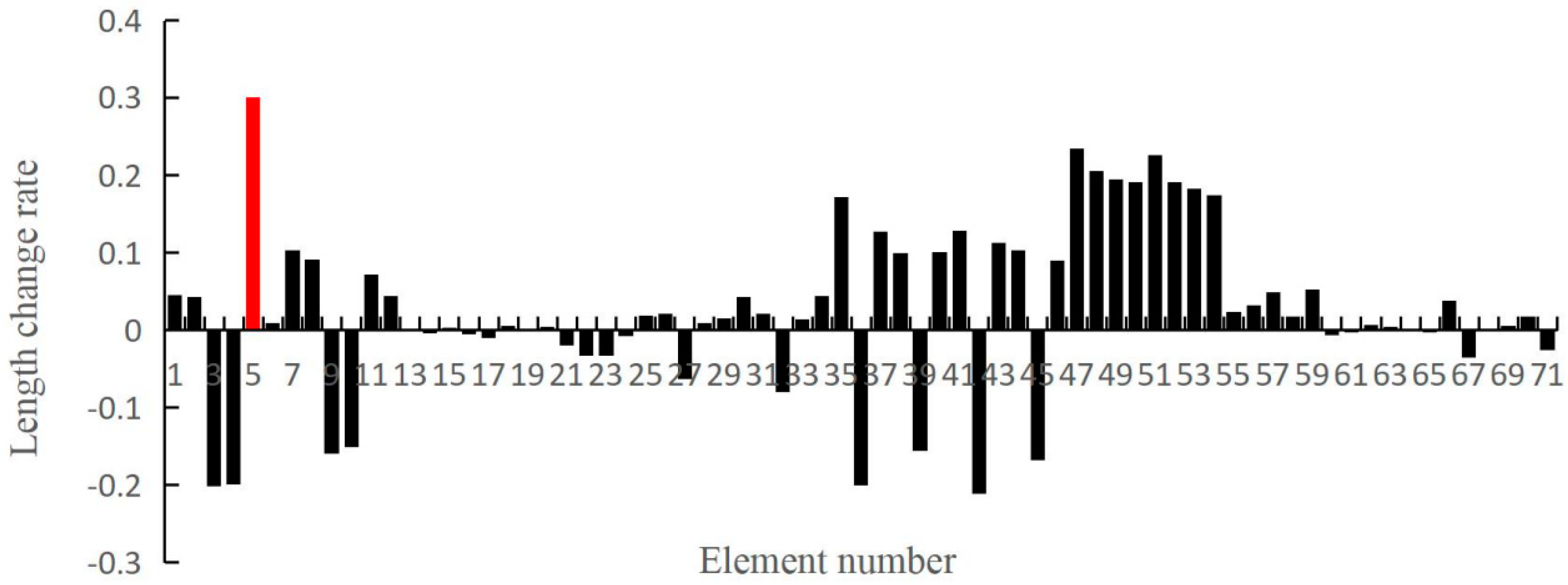
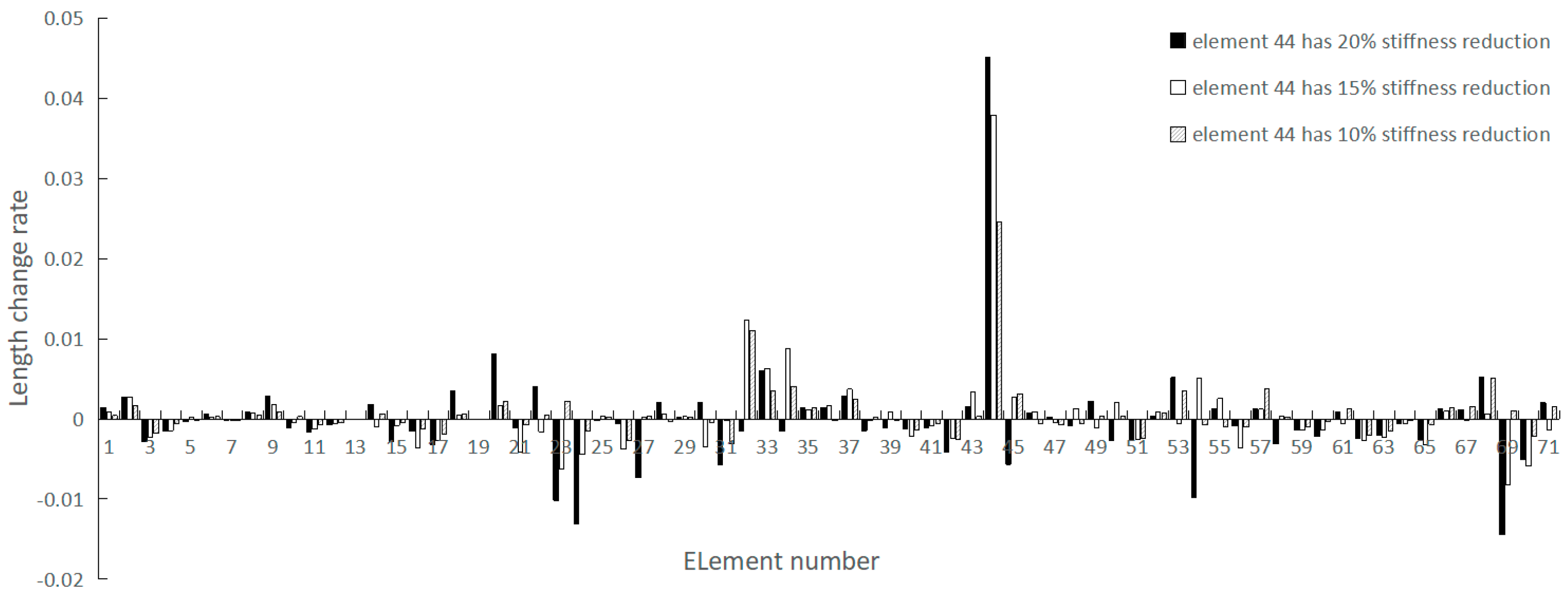
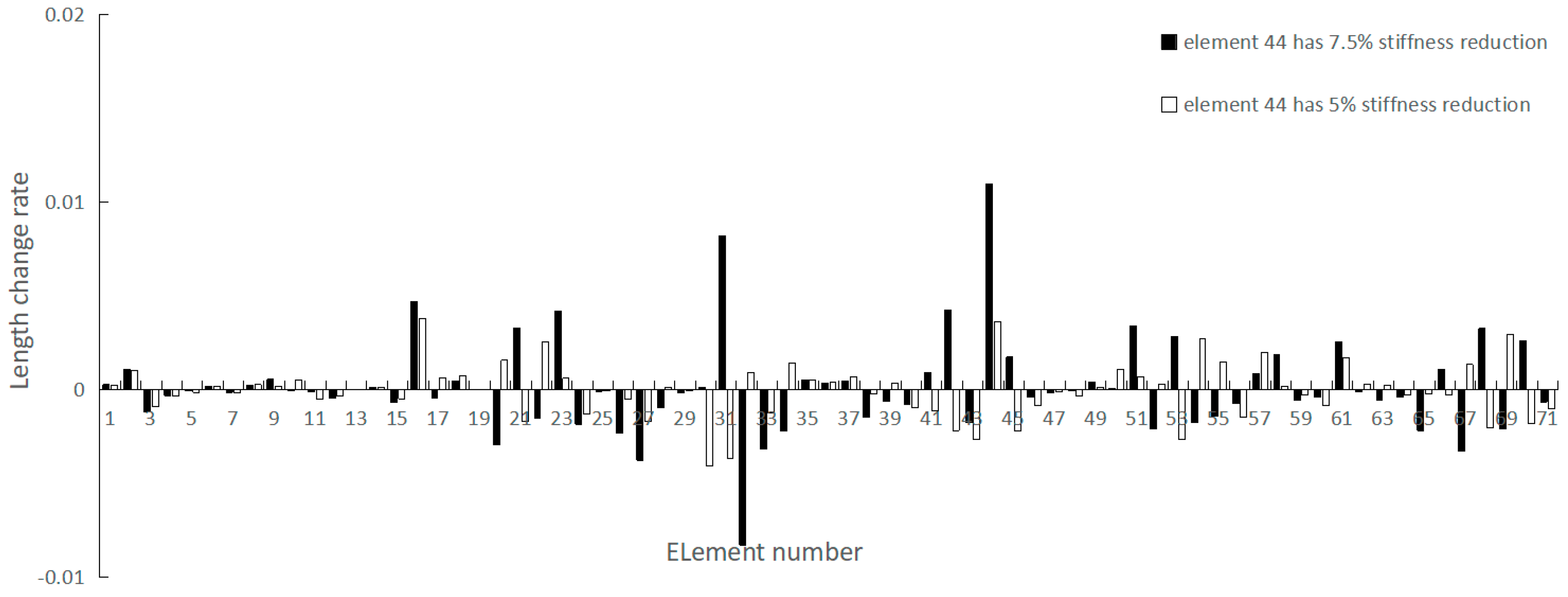
- The rate of change of length ε of the element (strain) is calculated by Equation (19), and the element with the larger length change rate was identified as the position where the damage was most likely to occur.
4. Cases
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Li, C.H.; Yang, Q.W.; Sun, B.X. A Virtual Load Method for Damage Identification of Beam Structures. Recent Pat. Eng. 2018, 12, 117–126. [Google Scholar] [CrossRef]
- Yang, Q.W.; Wang, C.; Li, N.; Wang, W.; Liu, Y. Enhanced Singular Value Truncation Method for Non-Destructive Evaluation of Structural Damage Using Natural Frequencies. Materials 2019, 12, 1021. [Google Scholar] [CrossRef] [PubMed]
- Zhu, H.; Yu, J.; Zhang, J. A summary review and advantages of vibration-based damage identification methods in structural health monitoring. Eng. Mech. 2011, 28, 1–17. [Google Scholar]
- Yin, Z.; Wang, W.; Jiao, D. Experiment and simulation analysis of damage of space truss. J. Civ. Arch. Env. Eng. 2017, 39, 78–84. [Google Scholar]
- Wang, M.; Song, C.; Ji, C. Research on damage detection method for grid structures based on frequency response function and principal component analysis. Build. Struct. 2017, 47, 96–101. [Google Scholar]
- Li, H.; Lin, S.; Yi, T. Study on Structural Damage Identification by Static Virtual Distortion Method. J. Arch. Civ. Eng. 2016, 33, 1–6. [Google Scholar]
- Yu, O.; Chao, X.; Yang, W. A Two-Stage Method for Beam Damage Identification Based on Static Deflection. Chin. Q. Mech. 2017, 38, 458–467. [Google Scholar]
- Huang, B.; Zong, R.; Yang, T. Damage Identification of Random Beam Structure Based on Static Measurement Data. Chin. J. Comput. Mech. 2013, 180–185. [Google Scholar] [CrossRef]
- Huang, B.; Guo, W.; Lu, Y. An Improved Method for Static Damage Identification of Random Beam Structures. Chin. J. Comput. Mech. 2018, 21, 451–456. [Google Scholar]
- Hosseinzadeh, A.; Ghodrati, A.G.; Koo, K.Y. Optimization-based method for structural damage localization and quantification by means of static displacements computed by flexibility matrix. Eng. Optim. 2016, 48, 543–561. [Google Scholar] [CrossRef]
- Mehrisadat, M.; Alamdari, L.; Ge, K.; Zhou, K.Y.; Du, Z. Non-contact structural health monitoring of a cable-stayed bridge: Case study. Struct. Infrastruct. Eng. 2019, 15, 1119–1136. [Google Scholar]
- Chen, Z.H.; Zhu, S.; Xu, Y.; Li, Q.; Cai, Q. Damage Detection in Long Suspension Bridges Using Stress Influence Lines. J. Bridge Eng. 2015, 20, 05014013. [Google Scholar] [CrossRef]
- Kawano, A.; Zine, A. Reliability evaluation of continuous beam structures using data concerning the displacement of points in a small region. Eng. Struct. 2019, 180, 379–387. [Google Scholar] [CrossRef]
- Mao, Q.; Mazzotti, M.; DeVitis, J. Structural condition assessment of a bridge pier: A case study using experimental modal analysis and finite element model updating. Struct. Control Health Monit. 2018, 26, e2273. [Google Scholar] [CrossRef]
- Pu, Q.; Hong, Y.; Chen, L. Model updating-based damage detection of a concrete beam utilizing experimental damped frequency response functions. Adv. Struct. Eng. 2019, 22, 935–947. [Google Scholar] [CrossRef]
- Astroza, R.; Alessandri, A. Effects of model uncertainty in nonlinear structural finite element model updating by numerical simulation of building structures. Struct. Control Health Monit. 2019, 26, e2297. [Google Scholar] [CrossRef]
- Yang, Q.W.; Du, S.G.; Liang, C.F. A Universal Model-Independent Algorithm for Structural Damage Localization. Comput. Modeling Eng. Sci. 2014, 100, 223–248. [Google Scholar]
- Feng, D.; Feng, M.Q. Computer vision for SHM of civil infrastructure: From dynamic response measurement to damage detection A review. Eng. Struct. 2018, 156, 105–117. [Google Scholar] [CrossRef]
- Feng, D.; Feng, M.Q. Model Updating of Railway Bridge Using in Situ Dynamic Displacement Measurement under Trainloads. J. Bridge Eng. 2015, 20, 04015019. [Google Scholar] [CrossRef]
- Qin, J.; Gao, Z.; Wang, X.; Yang, S. Three-Dimensional Continuous Displacement Measurement with Temporal Speckle Pattern Interferometry. Sensors 2016, 16, 2020. [Google Scholar] [CrossRef]
- Hung, Y.Y. Displacement and Strain Measurement; Dekker: New York, NY, USA, 1978; Volume 4, pp. 51–71. [Google Scholar]
- Lee, J.J.; Shinozuka, M. Real-time displacement measurement of a flexible bridge using digital image processing techniques. Exp. Mech. 2006, 46, 105–114. [Google Scholar] [CrossRef]
- Feng, D.; Feng, M.; Ozer, E. A vision-based sensor for noncontact structural displacement measurement. Sensors 2015, 15, 16557–16575. [Google Scholar] [CrossRef] [PubMed]
- Twu, R.C.; Yan, N.Y. Immersion-type KTP sensor for angular displacement measurement. Opt. Laser Technol. 2019, 120, 105690. [Google Scholar] [CrossRef]
- Kondo, T.; Suzuki, Y.; Matoba, K. Displacement measurement device, measurement system, and displacement measurement method. U.S. Patent Application 15/893,702, 28 March 2019. [Google Scholar]


© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, Q.; Wang, C.; Li, N.; Luo, S.; Wang, W. Model-Free Method for Damage Localization of Grid Structure. Appl. Sci. 2019, 9, 3252. https://doi.org/10.3390/app9163252
Yang Q, Wang C, Li N, Luo S, Wang W. Model-Free Method for Damage Localization of Grid Structure. Applied Sciences. 2019; 9(16):3252. https://doi.org/10.3390/app9163252
Chicago/Turabian StyleYang, Qiuwei, Chaojun Wang, Na Li, Shuai Luo, and Wei Wang. 2019. "Model-Free Method for Damage Localization of Grid Structure" Applied Sciences 9, no. 16: 3252. https://doi.org/10.3390/app9163252
APA StyleYang, Q., Wang, C., Li, N., Luo, S., & Wang, W. (2019). Model-Free Method for Damage Localization of Grid Structure. Applied Sciences, 9(16), 3252. https://doi.org/10.3390/app9163252





