Using Allan Variance Based Semi-Parameter Model to Calibrate Pointing Errors of Alt-az Telescopes
Abstract
:1. Introduction
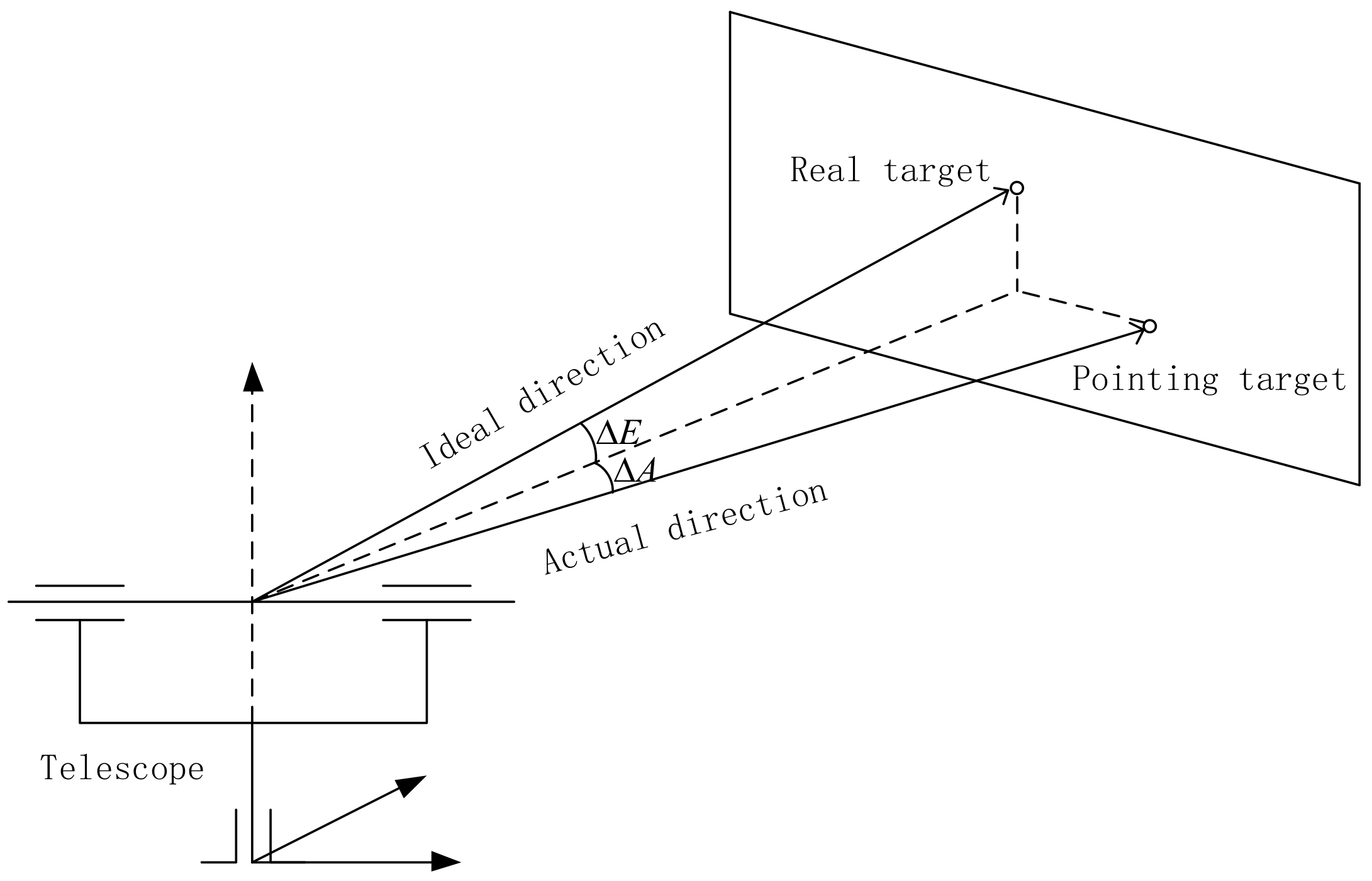
2. Error Analysis and Modeling of Pointing Errors
3. Online Calibration of Pointing Errors
3.1. Calibration of Geometric Errors
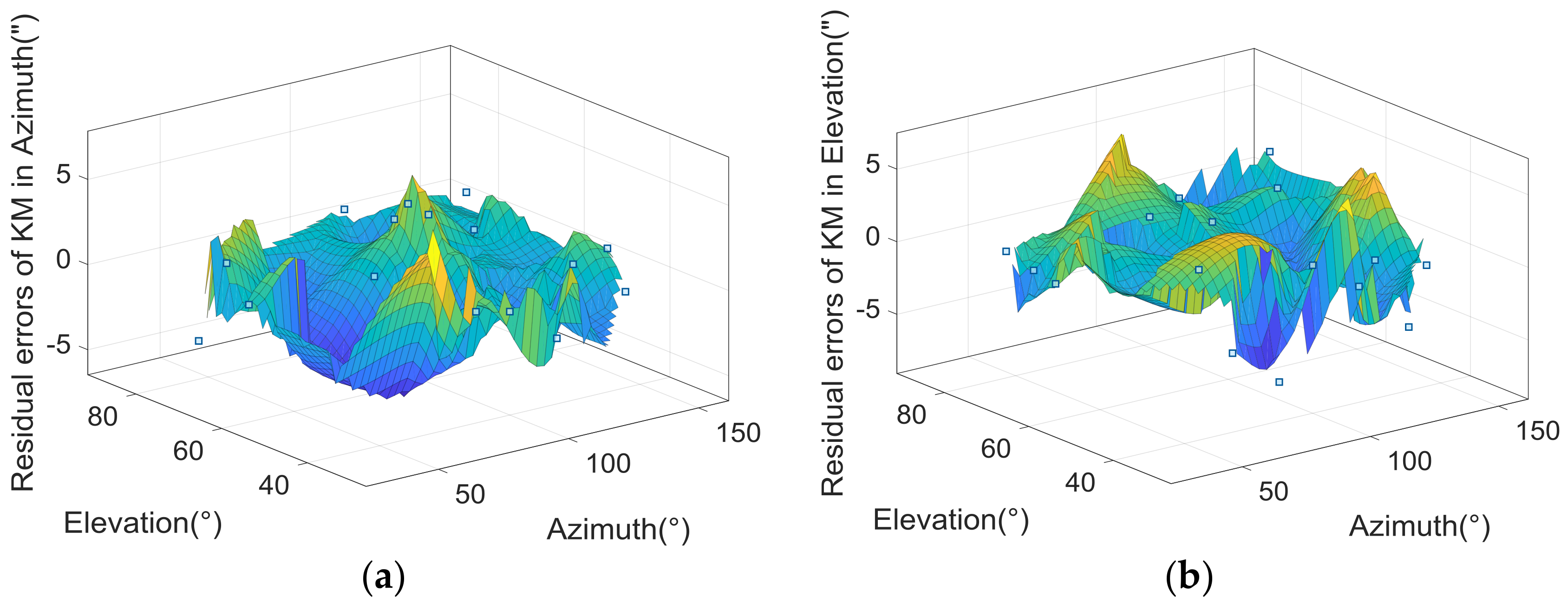
3.2. Estimation of Nonlinear Errors
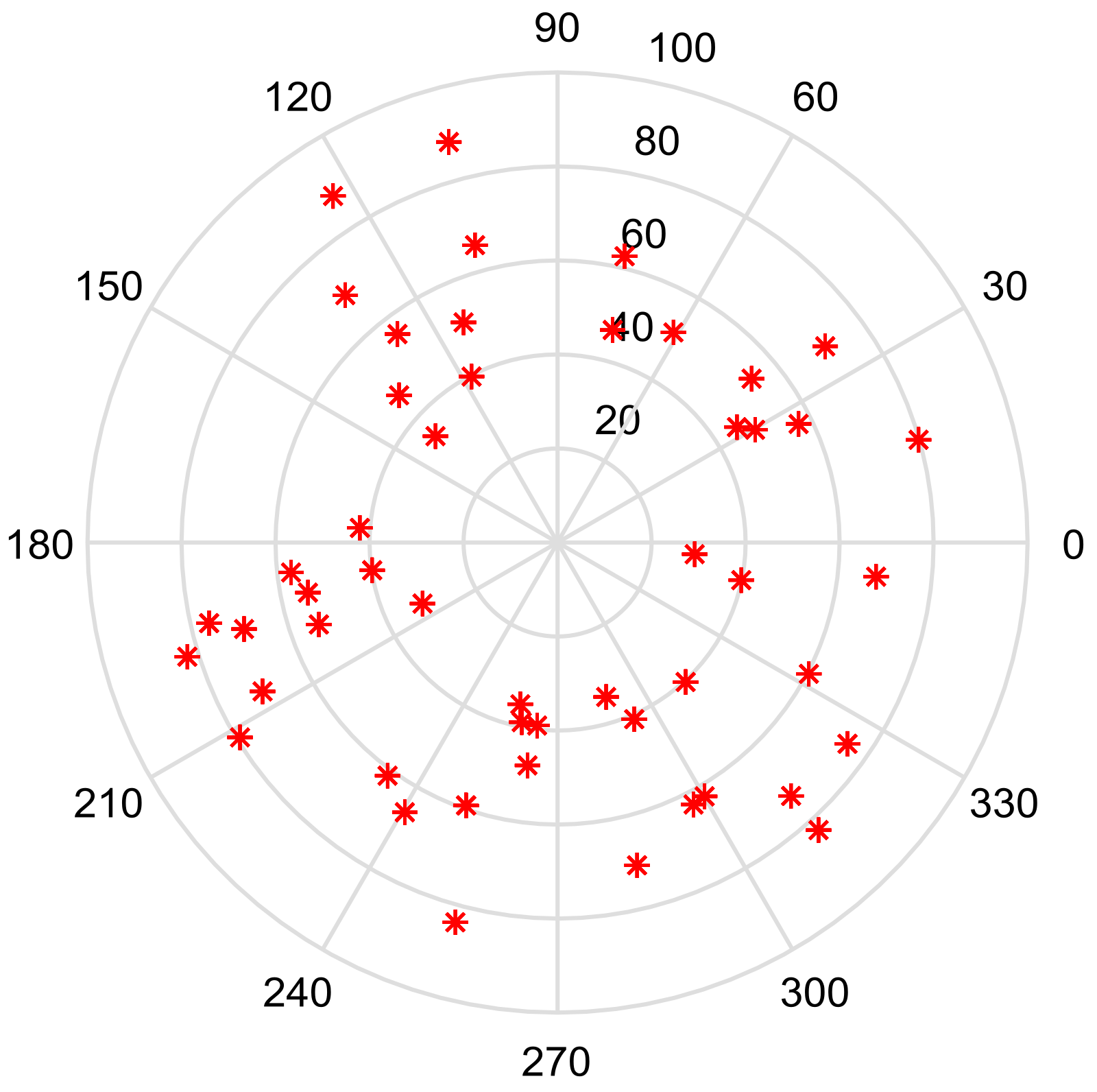
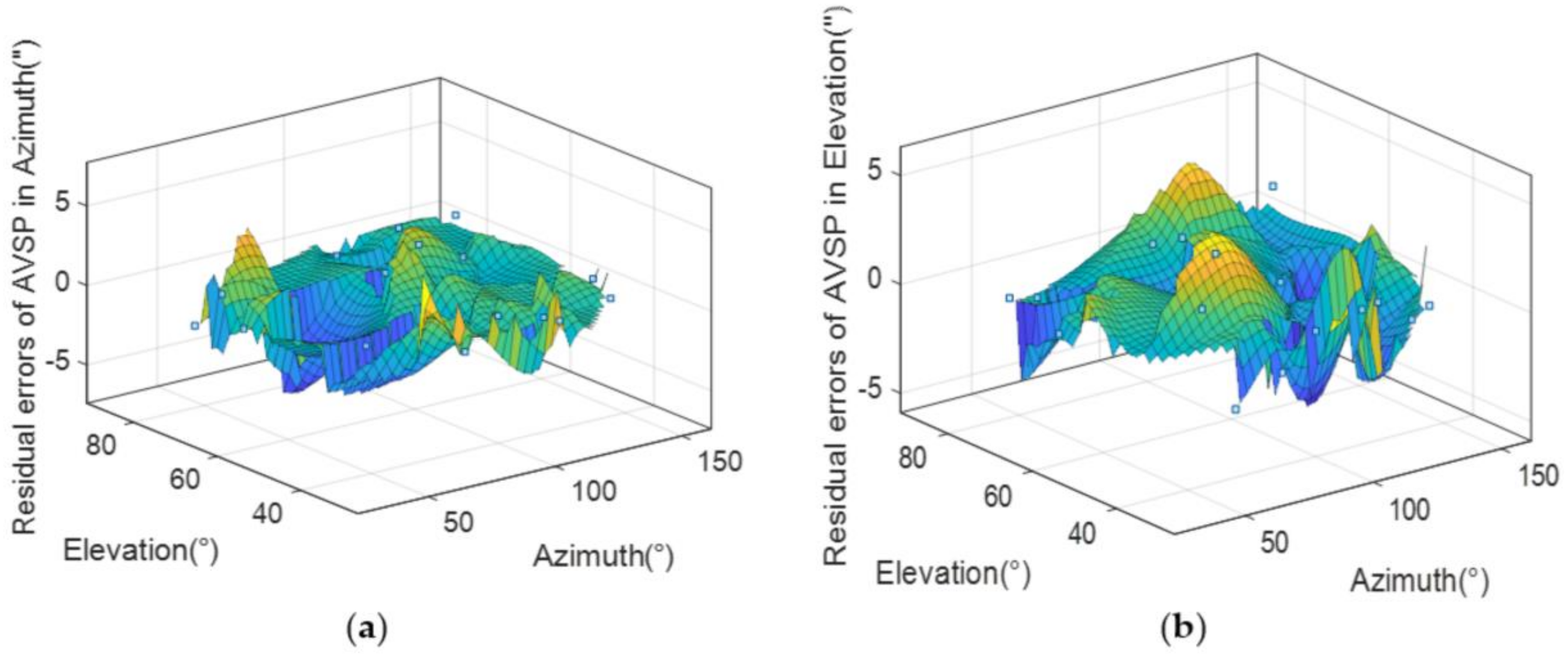
4. Experiment and Results
- (1)
- Preparations for calibration process were made, namely blind-pointing measurements, including measuring LOS pointing errors at positions covering the whole sky and determining parameters of the mount model which most meet with the observed data.
- (2)
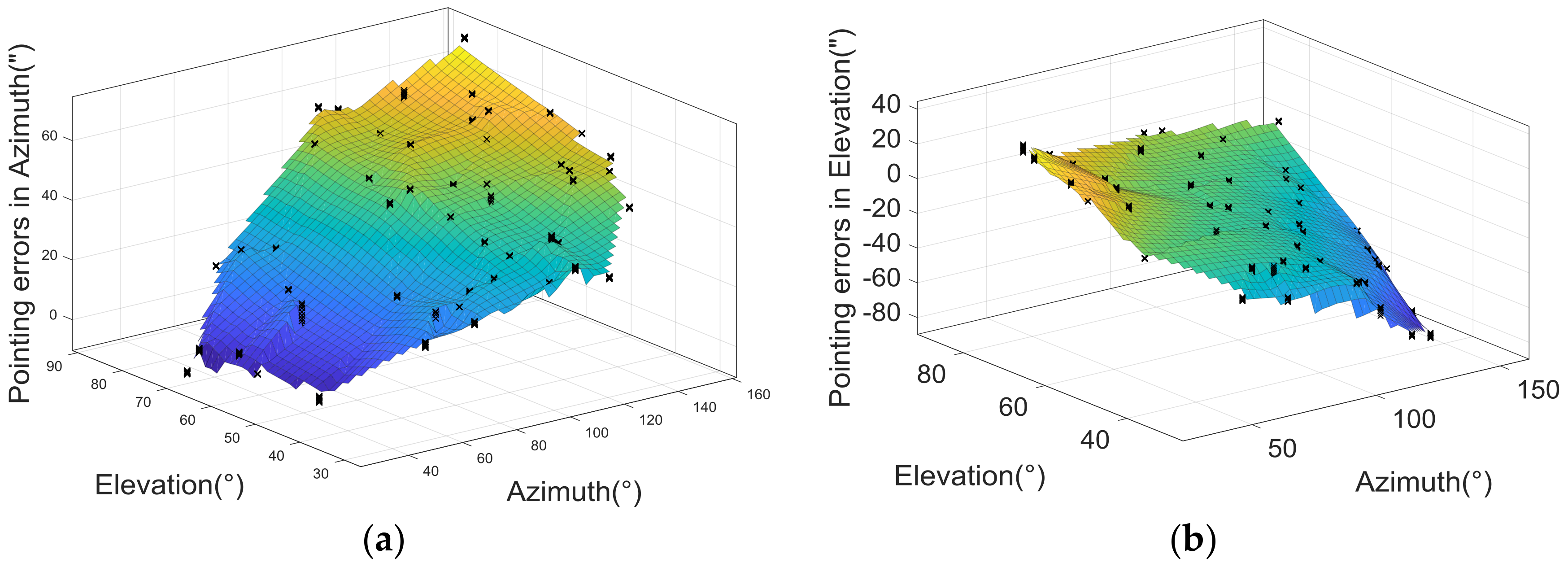
- Active sampling such as blind-pointing measurements was carried out to acquire the correction coefficients of mount model. For the calibration portion, the residual errors after compensation of the mount model is necessary. The proposed AVSPM and conventional mount model were employed to calibrate LOS pointing errors at every pointing angle, making corrections available for the servo system and actuators. A numerical model called Spherical Harmonic Function Model (SHFM) was introduced for contrast. In SHFM, the spherical function is expanded into Fourier series, and then the zonal harmonic terms of series to fourth order are taken to shape the final model.
- (3)
- While the LOS was turned to different directions to cover the whole sky within the movement range, all of the observed data were recorded synchronously, including pointing angles, corresponding errors and time.
5. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Zhang, X.X.; Wu, L.D. The basic parameters of telescope static point model. Acta Astromomica Sin. 2001, 42, 198–205. [Google Scholar]
- Shi, H.L.; Yan, Y.H. Extended interpolation method and its applications in piecewise approximation. Comput. Appl. Math. 1992, 12, 229–236. [Google Scholar]
- Fisk, J.W.; Rue, A.K. Confidence limits for the pointing error of gimbaled sensors. IEEE Trans. Aerosp. Electron. Syst. 2008, AES-2, 648–654. [Google Scholar] [CrossRef]
- Keitzer, S.A.; Kimbrell, J.E.; Greenwald, D. Deterministic errors in pointing and tracking systems I: Identification and correction of static errors. In Proceedings of the International Society for Optics and Photonics Acquisition, Tracking, and Pointing V, Orlando, FL, USA, 1 August 1991; Volume 1482, pp. 415–424. [Google Scholar]
- Denavit, J.; Hartenberg, R.S. A kinematic notation for lower-pair mechanisms based on matrices. Trans. ASME J. Appl. Mech. 1955, 22, 215–221. [Google Scholar]
- Sciavicco, L.; Siciliano, B. Modeling and Control of Robot Manipulators. Ind. Robot Int. J. 1996, 21, 99–100. [Google Scholar]
- Huang, L.; Ma, W.L.; Huang, J.L. Modeling and calibration of pointing errors with alt-az telescope. New Astron. 2016, 47, 105–110. [Google Scholar] [CrossRef]
- Hong, H.J.; Zhou, X.Y.; Zhang, Z.Y.; Fan, D.P. Modeling and calibration of pointing errors using a semi-parametric regression method with applications in inertially stabilized platforms. Eng. Manuf. 2013, 227, 1492–1501. [Google Scholar] [CrossRef]
- Mecozzi, A.; Antonelli, C.; Shtaif, M. Nonlinear propagation in multi-mode fibers in the strong coupling regime. Opt. Express 2012, 20, 11673–11678. [Google Scholar] [CrossRef] [PubMed]
- Allan, D.W. Statistics of atomic frequency standards. Proc. IEEE 1966, 54, 221–230. [Google Scholar] [CrossRef]
- IEEE Aerospace and Electronic Society. IEEE Standard Specification Format Guide and Test Procedure for Single-Axis Interferometric Fiber Optic Gyros; IEEE Press: Piscataway, NJ, USA, 1997. [Google Scholar]
- Scimek, M.G. Smoothing and regression: Approaches, computation and application. J. R. Stat. Soc. 2003, 52, 126–127. [Google Scholar]

| Pointing Model | Pointing Precision (RMSE) of 1 m Alt-az Telescope | ||
|---|---|---|---|
| OE | 18.1477″ | 26.3599″ | 20.6719″ |
| SHFM | 2.3655″ | 2.7226″ | 1.7112″ |
| MM | 2.2376″ | 2.569″ | 1.6923″ |
| AVSPM | 1.1261″ | 1.3054″ | 1.1542″ |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yan, L.; Huang, Y.; Zhang, Y. Using Allan Variance Based Semi-Parameter Model to Calibrate Pointing Errors of Alt-az Telescopes. Appl. Sci. 2018, 8, 614. https://doi.org/10.3390/app8040614
Yan L, Huang Y, Zhang Y. Using Allan Variance Based Semi-Parameter Model to Calibrate Pointing Errors of Alt-az Telescopes. Applied Sciences. 2018; 8(4):614. https://doi.org/10.3390/app8040614
Chicago/Turabian StyleYan, Lingjie, Yongmei Huang, and Yahui Zhang. 2018. "Using Allan Variance Based Semi-Parameter Model to Calibrate Pointing Errors of Alt-az Telescopes" Applied Sciences 8, no. 4: 614. https://doi.org/10.3390/app8040614
APA StyleYan, L., Huang, Y., & Zhang, Y. (2018). Using Allan Variance Based Semi-Parameter Model to Calibrate Pointing Errors of Alt-az Telescopes. Applied Sciences, 8(4), 614. https://doi.org/10.3390/app8040614





