Light Scattering by a Dielectric Sphere: Perspectives on the Mie Resonances
Abstract
1. Introduction
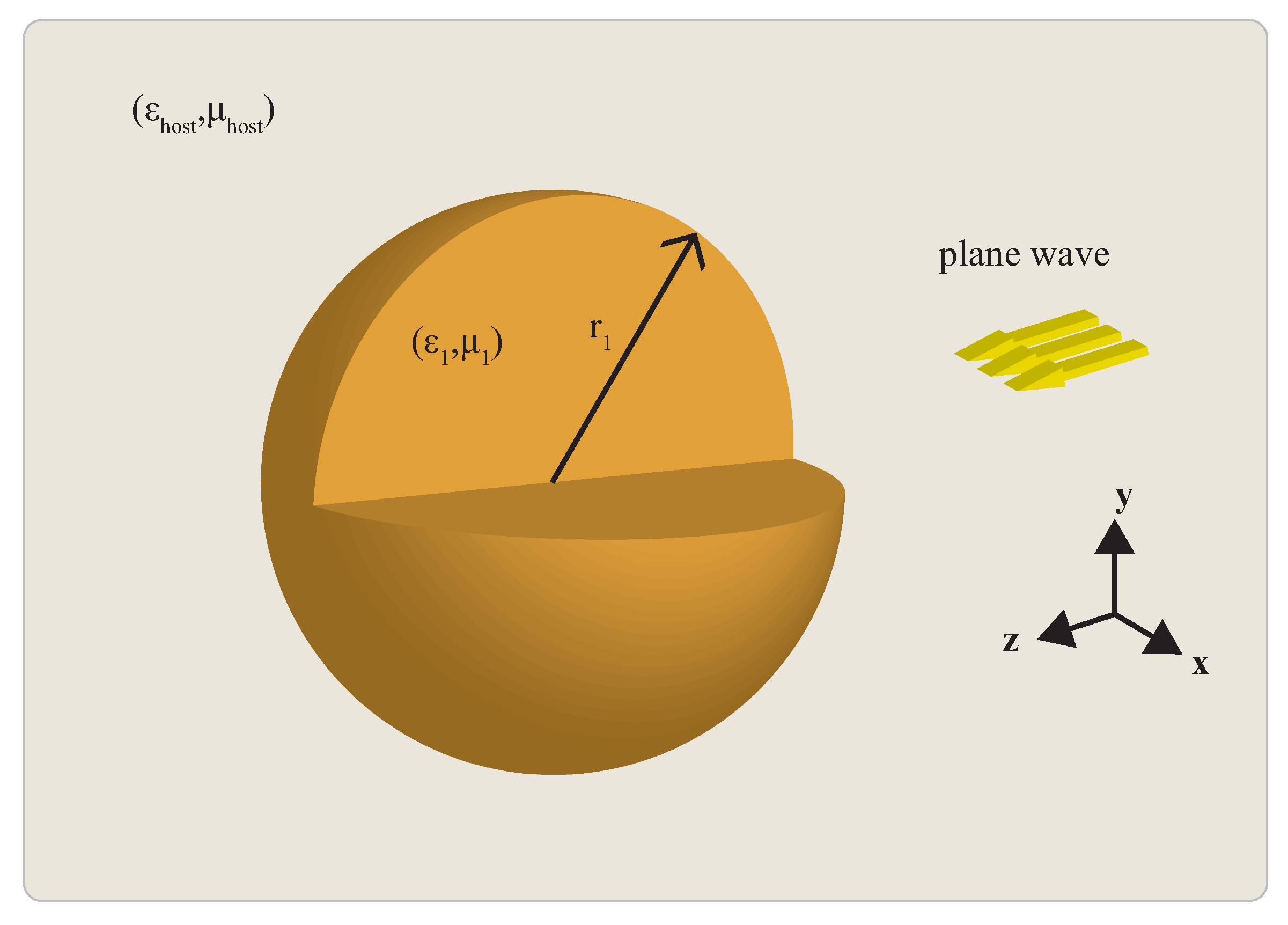
2. Electrodynamic Scattering: Mie Coefficients
3. Electrostatic Scattering by a Sphere
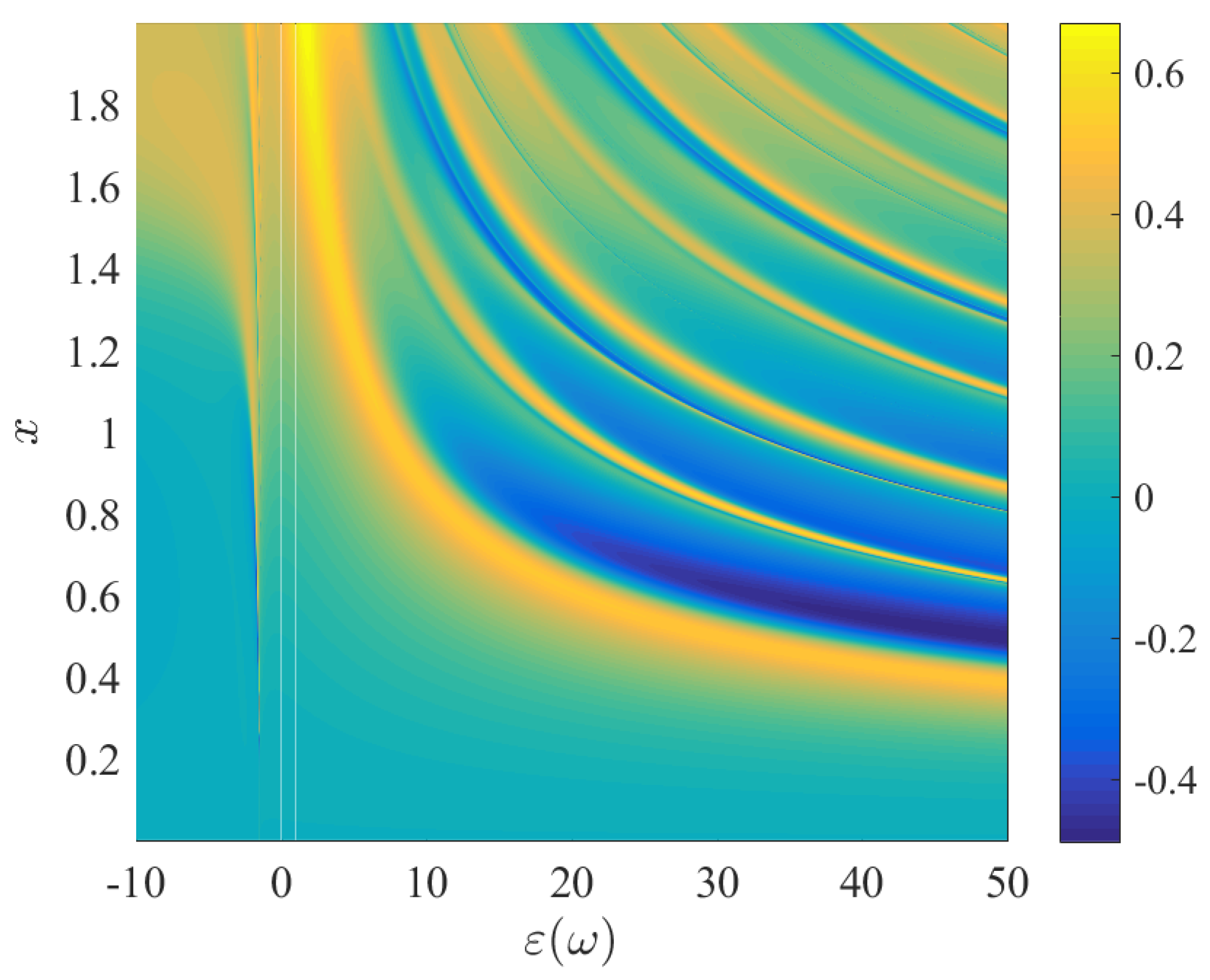
4. Taylor and Padé Expansions of Mie Coefficients
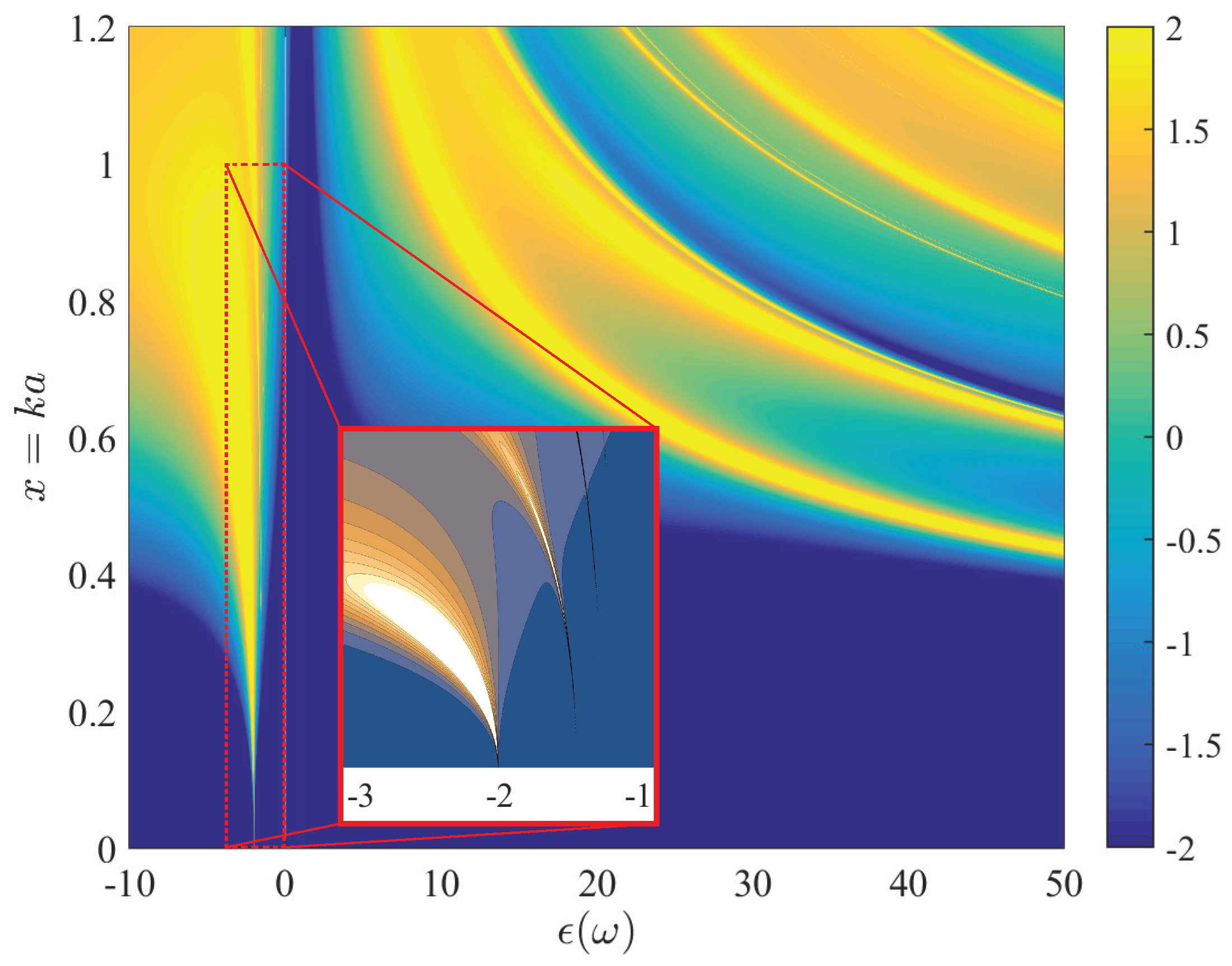
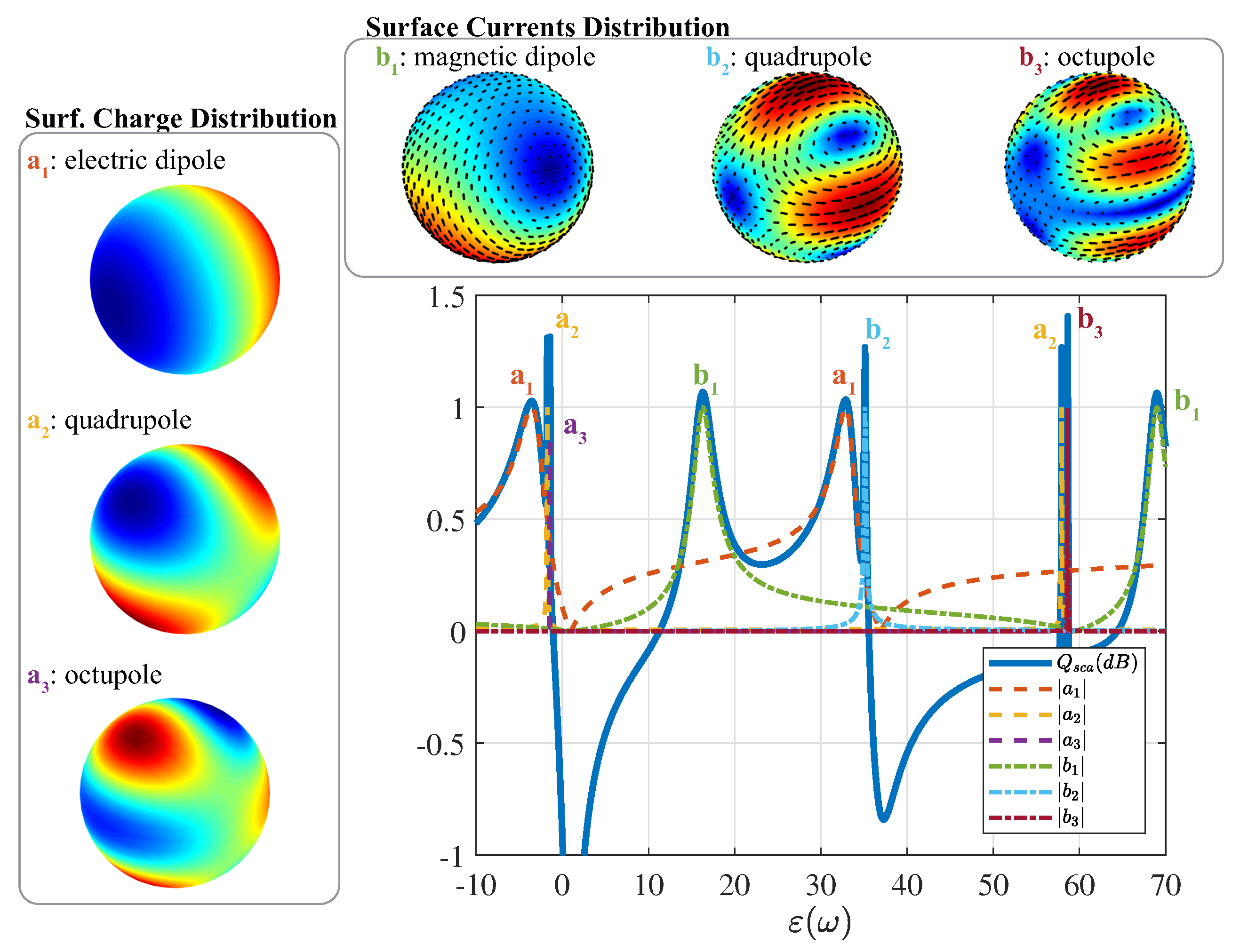
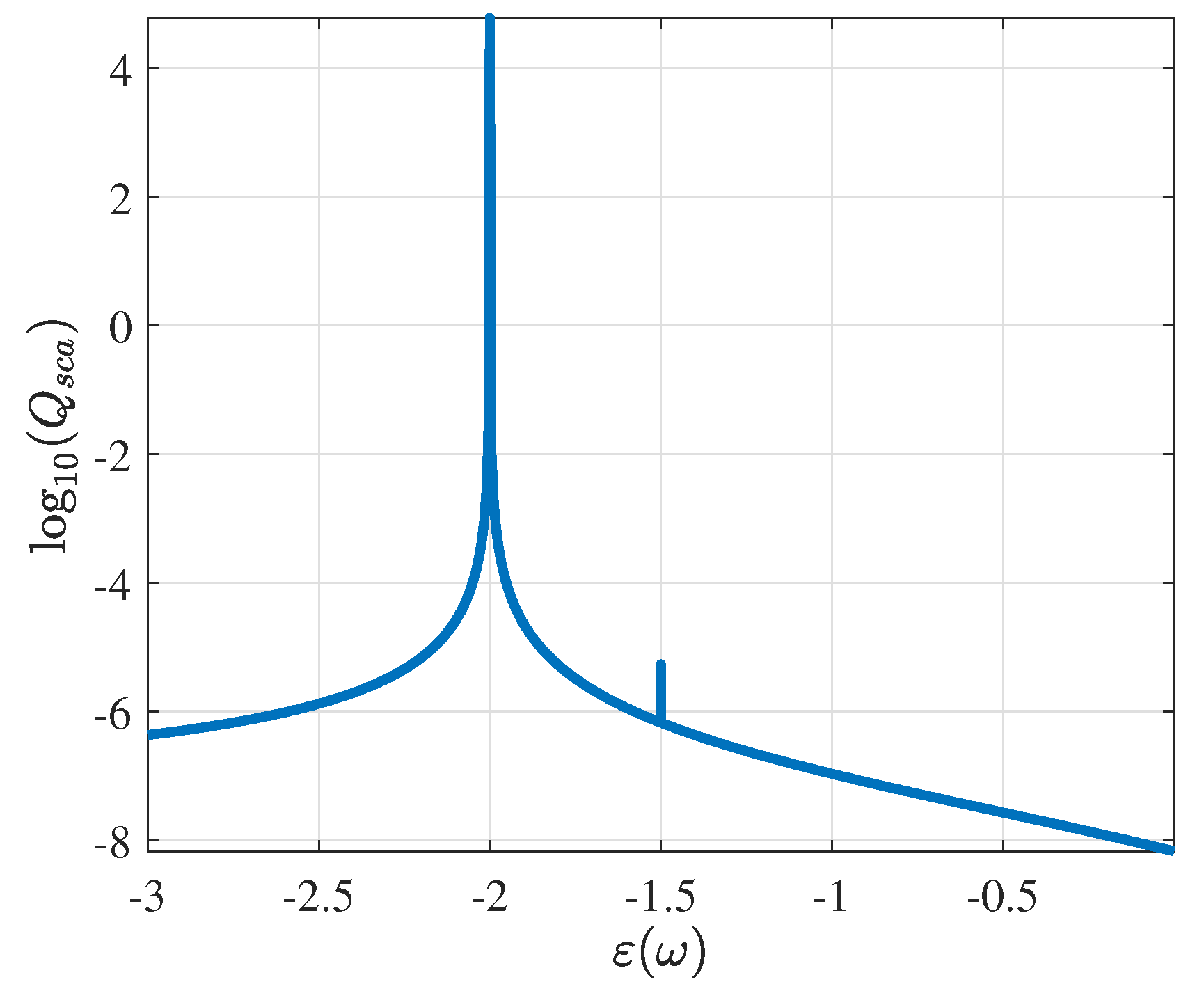
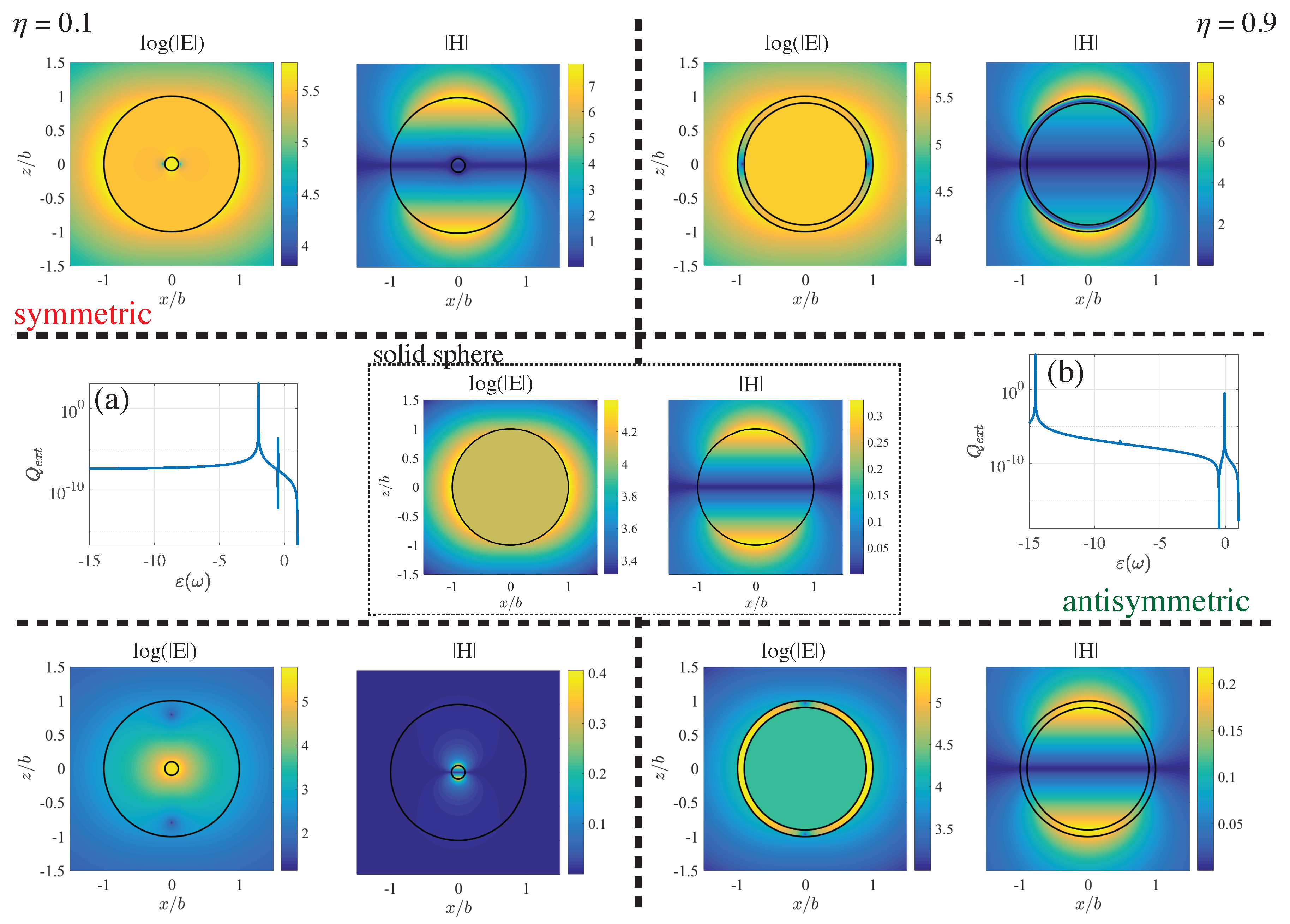
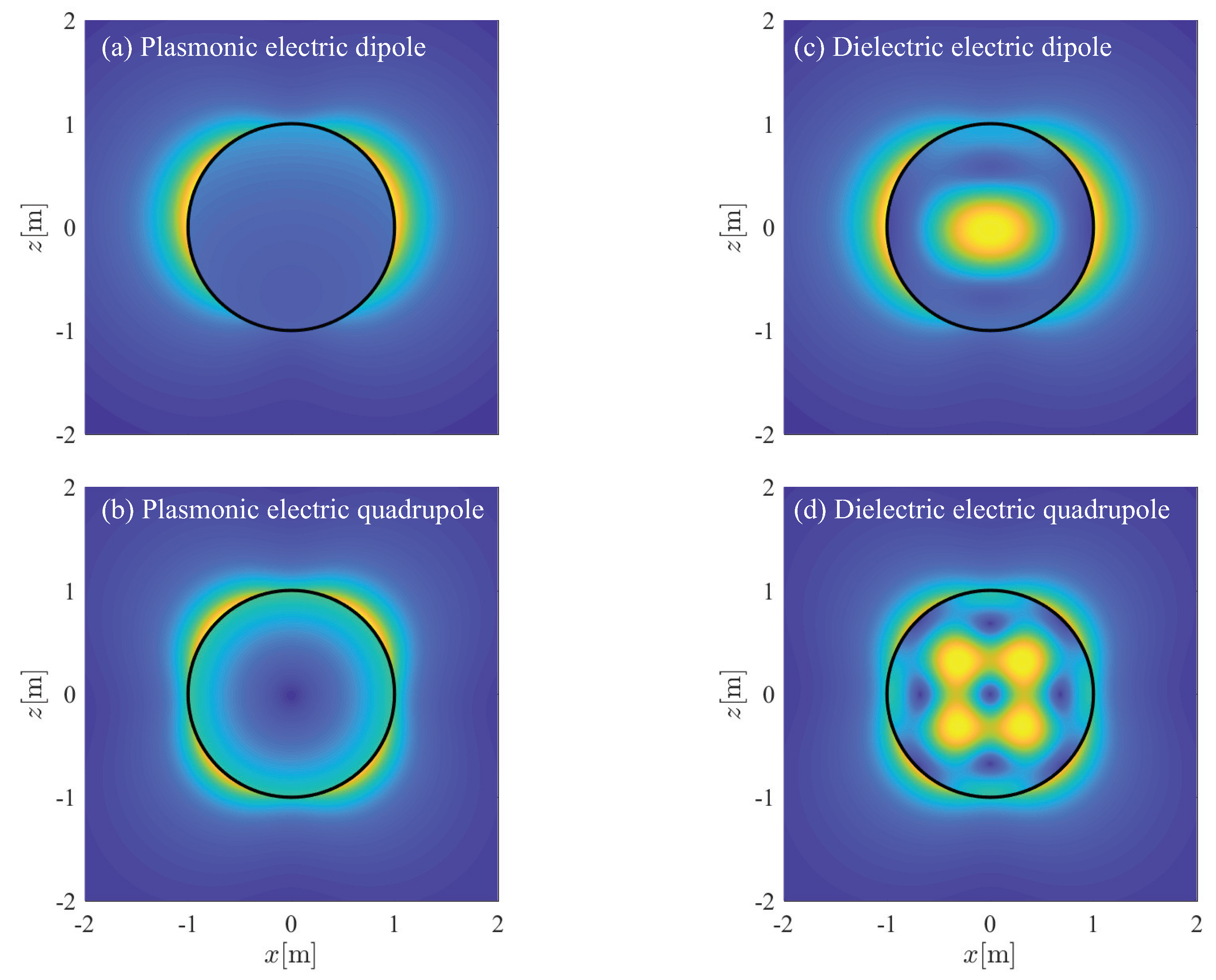
5. Plasmonic and Dielectric Resonances: Summary
6. Directional Scattering Characteristics: Forward and Backscattering Conditions
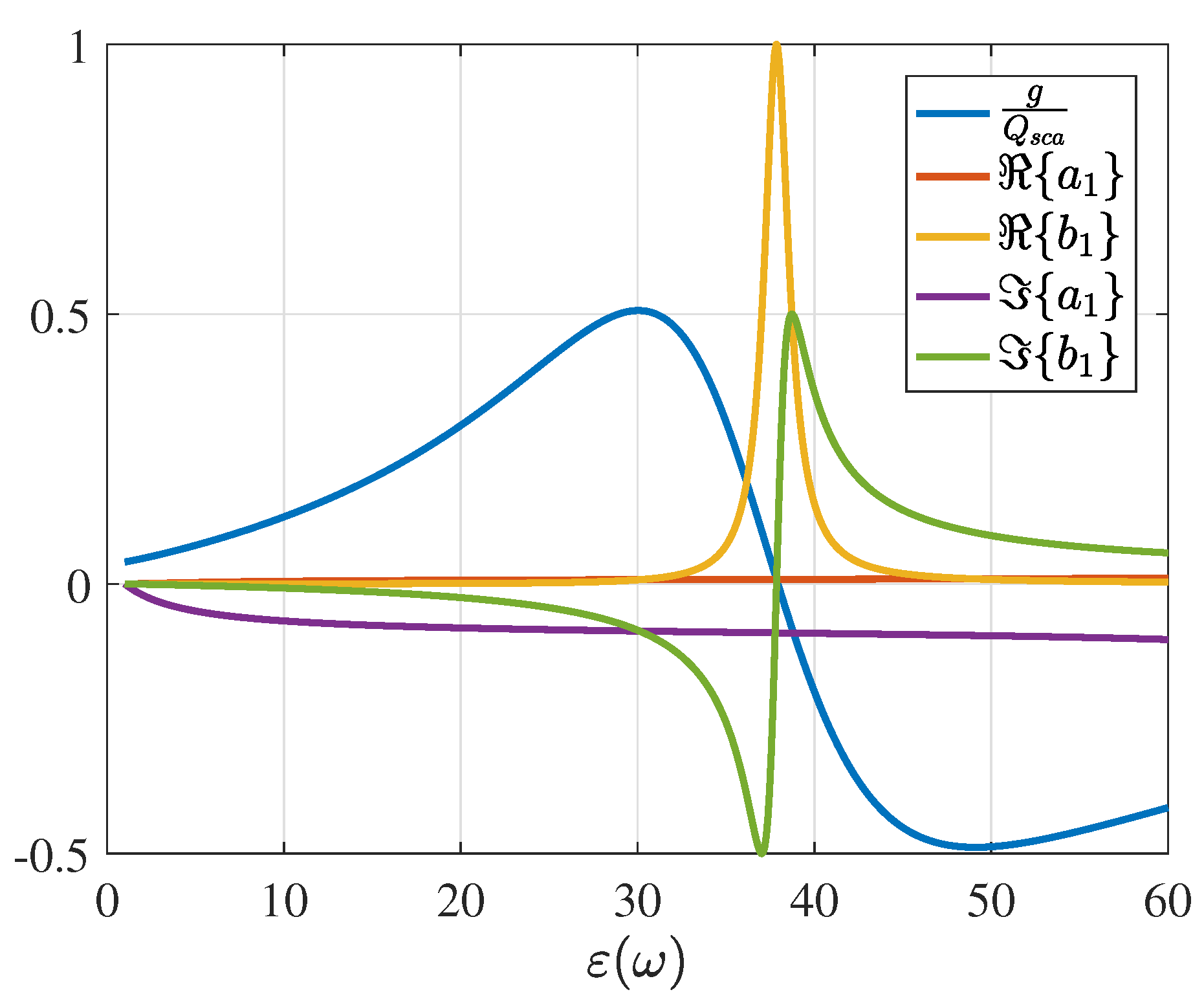
6.1. The Asymmetry Factor
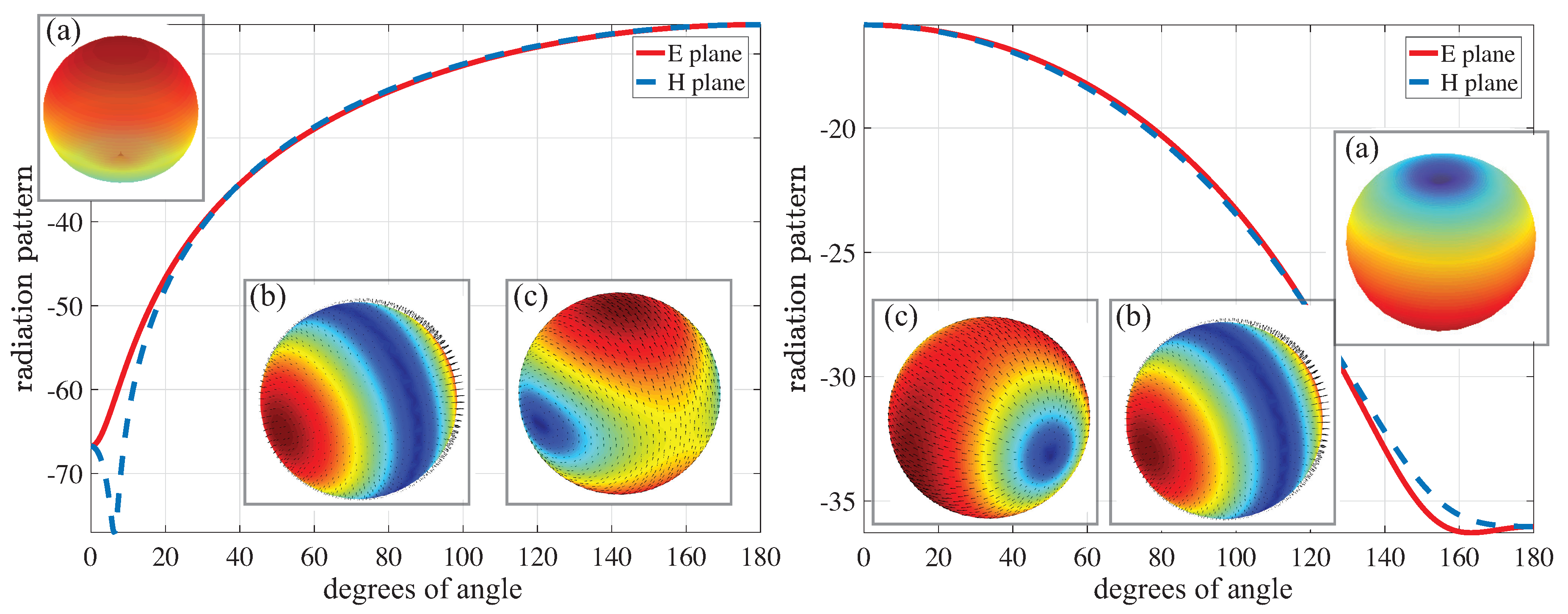
6.2. Directive Scattering Conditions for Dielectric Resonances
7. Summary
Acknowledgments
Author Contributions
Conflicts of Interest
Appendix A. Simple Padé Expansions of Spherical Bessel, Hankel, and Riccati–Bessel/Hankel Functions
References
- Prodan, E.; Radloff, C.; Halas, N.J.; Nordlander, P. A hybridization model for the plasmon response of complex nanostructures. Science 2003, 302, 419–422. [Google Scholar] [CrossRef] [PubMed]
- Atwater, H.A.; Polman, A. Plasmonics for improved photovoltaic devices. Nat. Mater. 2010, 9, 205–213. [Google Scholar] [CrossRef] [PubMed]
- Fan, X.; Zheng, W.; Singh, D.J. Light scattering and surface plasmons on small spherical particles. Light Sci. Appl. 2014, 3, e179. [Google Scholar] [CrossRef]
- Kuznetsov, A.I.; Miroshnichenko, A.E.; Brongersma, M.L.; Kivshar, Y.S.; Luk’yanchuk, B. Optically resonant dielectric nanostructures. Science 2016, 354. [Google Scholar] [CrossRef] [PubMed]
- Liberal, I.; Engheta, N. Near-zero refractive index photonics. Nat. Photonics 2017, 11, 149–158. [Google Scholar] [CrossRef]
- Kelly, K.L.; Coronado, E.; Zhao, L.L.; Schatz, G.C. The optical properties of metal nanoparticles: The influence of size, shape, and dielectric environment. J. Phys. Chem. B 2003, 107, 668–677. [Google Scholar] [CrossRef]
- Halas, N.J.; Lal, S.; Chang, W.S.; Link, S.; Nordlander, P. Plasmons in strongly coupled metallic nanostructures. Chem. Rev. 2011, 111, 3913–3961. [Google Scholar] [CrossRef] [PubMed]
- Jahani, S.; Jacob, Z. All-dielectric metamaterials. Nat. Nanotechnol. 2016, 11, 23–36. [Google Scholar] [CrossRef] [PubMed]
- Shore, R.A. Scattering of an electromagnetic linearly polarized plane wave by a multilayered sphere: Obtaining a computational form of Mie coefficients for the scattered field. IEEE Antennas Propag. Mag. 2015, 57, 69–116. [Google Scholar] [CrossRef]
- Arslanagic, S.; Ziolkowski, R.W. Cylindrical and Spherical Active Coated Nanoparticles as Nanoantennas: Active Nanoparticles as Nanoantennas. IEEE Antennas Propag. Mag. 2017, 59, 14–29. [Google Scholar] [CrossRef]
- Ammari, H.; Millien, P.; Ruiz, M.; Zhang, H. Mathematical analysis of plasmonic nanoparticles: The scalar case. Arch. Ration. Mech. Anal. 2017, 224, 597–658. [Google Scholar]
- Born, M.; Wolf, E. Principles of Optics, 7th ed.; Cambridge University Press: Cambridge, UK, 2010. [Google Scholar]
- Bohren, C.F. How can a particle absorb more than the light incident on it? Am. J. Phys. 1983, 51, 323–327. [Google Scholar] [CrossRef]
- Myroshnychenko, V.; Rodríguez-Fernández, J.; Pastoriza-Santos, I.; Funston, A.M.; Novo, C.; Mulvaney, P.; Liz-Marzán, L.M.; de Abajo, F.J.G. Modelling the optical response of gold nanoparticles. Chem. Soc. Rev. 2008, 37, 1792–1805. [Google Scholar] [CrossRef] [PubMed]
- Mishchenko, M.I.; Travis, L.D.; Lacis, A.A. Scattering, Absorption, and Emission of Light by Small Particles; Cambridge University Press: Cambridge, UK, 2002. [Google Scholar]
- Stratton, J.A. Electromagnetic Theory; John Wiley & Sons: Hoboken, NJ, USA, 2007. [Google Scholar]
- Hulst, H.C.; Van De Hulst, H. Light Scattering by Small Particles; Courier Corporation: North Chelmsford, MA, USA, 1957. [Google Scholar]
- Kerker, M. The Scattering of Light and Other Electromagnetic Radiation: Physical Chemistry: A Series of Monographs; Academic Press: Cambridge, MA, USA, 2013; Volume 16. [Google Scholar]
- Bohren, C.F.; Huffman, D.R. Absorption and Scattering of Light by Small Particles; John Wiley & Sons: Hoboken, NJ, USA, 2007. [Google Scholar]
- Tribelsky, M.I.; Luk’yanchuk, B.S. Anomalous light scattering by small particles. Phys. Rev. Lett. 2006, 97, 263902. [Google Scholar] [CrossRef] [PubMed]
- Luk’yanchuk, B.; Zheludev, N.I.; Maier, S.A.; Halas, N.J.; Nordlander, P.; Giessen, H.; Chong, C.T. The Fano resonance in plasmonic nanostructures and metamaterials. Nat. Mater. 2010, 9, 707–715. [Google Scholar] [CrossRef] [PubMed]
- Miroshnichenko, A.E.; Evlyukhin, A.B.; Yu, Y.F.; Bakker, R.M.; Chipouline, A.; Kuznetsov, A.I.; Luk’yanchuk, B.; Chichkov, B.N.; Kivshar, Y.S.; Luk’yanchuk, B.; et al. Nonradiating anapole modes in dielectric nanoparticles. Nat. Commun. 2015, 6, 8069. [Google Scholar] [CrossRef] [PubMed]
- Stout, B.; Ross, M. Egocentric physics: Just about Mie. Europhys. Lett. 2017, 119, 44002. [Google Scholar] [CrossRef]
- Sihvola, A. Metamaterials in electromagnetics. Metamaterials 2007, 1, 2–11. [Google Scholar] [CrossRef]
- Glybovski, S.B.; Tretyakov, S.A.; Belov, P.A.; Kivshar, Y.S.; Simovski, C.R. Metasurfaces: From microwaves to visible. Phys. Rep. 2016, 634, 1–72. [Google Scholar] [CrossRef]
- Staude, I.; Schilling, J. Metamaterial-inspired silicon nanophotonics. Nat. Photonics 2017, 11, 274–284. [Google Scholar] [CrossRef]
- Maier, S.A.; Atwater, H.A. Plasmonics: Localization and guiding of electromagnetic energy in metal/dielectric structures. J. Appl. Phys. 2005, 98, 011101. [Google Scholar] [CrossRef]
- Schuller, J.A.; Zia, R.; Taubner, T.; Brongersma, M.L. Dielectric metamaterials based on electric and magnetic resonances of silicon carbide particles. Phys. Rev. Lett. 2007, 99, 107401. [Google Scholar] [CrossRef] [PubMed]
- Polman, A. Plasmonics Applied. Science 2008, 322, 868–869. [Google Scholar] [CrossRef] [PubMed]
- Evlyukhin, A.B.; Novikov, S.M.; Zywietz, U.; Eriksen, R.L.; Reinhardt, C.; Bozhevolnyi, S.I.; Chichkov, B.N. Demonstration of magnetic dipole resonances of dielectric nanospheres in the visible region. Nano Lett. 2012, 12, 3749–3755. [Google Scholar] [CrossRef] [PubMed]
- Krasnok, A.E.; Simovski, C.R.; Belov, P.A.; Kivshar, Y.S. Superdirective dielectric nanoantennas. Nanoscale 2014, 6, 7354–7361. [Google Scholar] [CrossRef] [PubMed]
- Monticone, F.; Alù, A. The quest for optical magnetism: from split-ring resonators to plasmonic nanoparticles and nanoclusters. J. Mater. Chem. C 2014, 2, 9059–9072. [Google Scholar] [CrossRef]
- Kruk, S.; Kivshar, Y. Functional meta-optics and nanophotonics Governed by Mie resonances. ACS Photonics 2017, 4, 2638–2649. [Google Scholar]
- Stewart, M.E.; Anderton, C.R.; Thompson, L.B.; Maria, J.; Gray, S.K.; Rogers, J.A.; Nuzzo, R.G. Nanostructured plasmonic sensors. Chem. Rev. 2008, 108, 494–521. [Google Scholar]
- Alù, A.; Engheta, N. Cloaking a sensor. Phys. Rev. Lett. 2009, 102, 233901. [Google Scholar] [CrossRef] [PubMed]
- Kerker, M. Invisible bodies. J. Opt. Soc. Am. 1975, 65, 376–379. [Google Scholar] [CrossRef]
- Alù, A.; Engheta, N. Achieving transparency with plasmonic and metamaterial coatings. Phys. Rev. E 2005, 72, 016623. [Google Scholar] [CrossRef] [PubMed]
- Pendry, J.B.; Schurig, D.; Smith, D.R. Controlling Electromagnetic Fields. Science 2006, 312, 1780–1782. [Google Scholar] [CrossRef] [PubMed]
- Argyropoulos, C.; Chen, P.Y.; Monticone, F.; D’Aguanno, G.; Alù, A. Nonlinear Plasmonic Cloaks to Realize Giant All-Optical Scattering Switching. Phys. Rev. Lett. 2012, 108, 263905. [Google Scholar] [CrossRef] [PubMed]
- Monticone, F.; Alù, A. Invisibility exposed: Physical bounds on passive cloaking. Optica 2016, 3, 718–724. [Google Scholar] [CrossRef]
- Ruan, Z.; Fan, S. Superscattering of light from subwavelength nanostructures. Phys. Rev. Lett. 2010, 105, 013901. [Google Scholar] [CrossRef] [PubMed]
- Gao, D.; Novitsky, A.; Zhang, T.; Cheong, F.C.; Gao, L.; Lim, C.T.; Luk’yanchuk, B.; Qiu, C.W. Unveiling the correlation between non-diffracting tractor beam and its singularity in Poynting vector. Laser Photonics Rev. 2015, 9, 75–82. [Google Scholar] [CrossRef]
- Lannebère, S.; Silveirinha, M.G. Optical meta-atom for localization of light with quantized energy. Nat. Commun. 2015, 6, 8766. [Google Scholar] [CrossRef] [PubMed]
- Ra’di, Y.; Asadchy, V.S.; Kosulnikov, S.U.; Omelyanovich, M.M.; Morits, D.; Osipov, A.V.; Simovski, C.R.; Tretyakov, S.A. Full Light Absorption in Single Arrays of Spherical Nanoparticles. ACS Photonics 2015, 2, 653–660. [Google Scholar] [CrossRef]
- Strutt, J.W. (Baron of Rayleigh) On the scattering of light by small particles. Philos. Mag. 1871, 41, 447–454. [Google Scholar]
- Sipe, J.E.; Kranendonk, J.V. Macroscopic electromagnetic theory of resonant dielectrics. Phys. Rev. A 1974, 9, 1806–1822. [Google Scholar] [CrossRef]
- Ruppin, R. Plasmon frequencies of small metal spheres. J. Phys. Chem. Solids 1978, 39, 233–237. [Google Scholar] [CrossRef]
- Ruppin, R. Optical properties of small metal spheres. Phys. Rev. B 1975, 11, 2871–2876. [Google Scholar] [CrossRef]
- Jackson, J.D. Classical Electrodynamics, 3rd ed.; John Wiley & Sons: Hoboken, NJ, USA, 1999. [Google Scholar]
- Maier, S.A. Plasmonics: Fundamentals and Applications; Springer Science & Business Media: Berlin, Germany, 2007. [Google Scholar]
- Kreibig, U.; Vollmer, M. Optical Properties of Metal Clusters; Springer: Berlin, Germany, 1995. [Google Scholar]
- Khurgin, J.B. How to deal with the loss in plasmonics and metamaterials. Nat. Nanotechnol. 2015, 10, 2–6. [Google Scholar] [CrossRef] [PubMed]
- Johnson, B.R. Theory of morphology-dependent resonances: shape resonances and width formulas. J. Opt. Soc. Am. A 1993, 10, 343–352. [Google Scholar] [CrossRef]
- Aden, A.L.; Kerker, M. Scattering of electromagnetic waves from two concentric spheres. J. Appl. Phys. 1951, 22, 1242–1246. [Google Scholar] [CrossRef]
- Wait, J.R. Electromagnetic scattering from a radially inhomogeneous sphere. Appl. Sci. Res. Sect. B 1962, 10, 441–450. [Google Scholar] [CrossRef]
- Tai, C.T. The electromagnetic theory of the spherical luneberg lens. Appl. Sci. Res. Sect. B 1959, 7, 113–130. [Google Scholar] [CrossRef]
- Wyatt, P.J. Scattering of electromagnetic plane waves from inhomogeneous spherically symmetric objects. Phys. Rev. 1962, 127, 1837–1843. [Google Scholar] [CrossRef]
- Qiu, C.W.; Li, L.W.; Yeo, T.S.; Zouhdi, S. Scattering by rotationally symmetric anisotropic spheres: Potential formulation and parametric studies. Phys. Rev. E 2007, 75, 026609. [Google Scholar] [CrossRef] [PubMed]
- Wallén, H.; Kettunen, H.; Sihvola, A. Anomalous absorption, plasmonic resonances, and invisibility of radially anisotropic spheres. Radio Sci. 2015, 50, 18–28. [Google Scholar] [CrossRef]
- Wiscombe, W.J. Improved Mie scattering algorithms. Appl. Opt. 1980, 19, 1505–1509. [Google Scholar] [CrossRef] [PubMed]
- García-Etxarri, A.; Gómez-Medina, R.; Froufe-Pérez, L.S.; López, C.; Chantada, L.; Scheffold, F.; Aizpurua, J.; Nieto-Vesperinas, M.; Sáenz, J.J. Strong magnetic response of submicron Silicon particles in the infrared. Opt. Express 2011, 19, 4815–4826. [Google Scholar] [CrossRef] [PubMed]
- Kuznetsov, A.I.; Miroshnichenko, A.E.; Fu, Y.H.; Zhang, J.; Luk’yanchuk, B. Magnetic light. Sci. Rep. 2012, 2, 492. [Google Scholar] [CrossRef] [PubMed]
- Tzarouchis, D.C.; Ylä-Oijala, P.; Sihvola, A. Resonant scattering characteristics of homogeneous dielectric sphere. IEEE Trans. Antennas Propag. 2017, 65, 3184–3191. [Google Scholar] [CrossRef]
- Sihvola, A.H. Electromagnetic Mixing Formulas and Applications; Number 47; IET: London, UK, 1999. [Google Scholar]
- Sihvola, A.H. Character of surface plasmons in layered spherical structures. Prog. Electromagn. Res. 2006, 62, 317–331. [Google Scholar] [CrossRef]
- Averitt, R.D.; Westcott, S.L.; Halas, N.J. Linear optical properties of gold nanoshells. J. Opt. Soc. Am. B 1999, 16, 1824–1832. [Google Scholar] [CrossRef]
- Hao, F.; Nordlander, P. Efficient dielectric function for FDTD simulation of the optical properties of silver and gold nanoparticles. Chem. Phys. Lett. 2007, 446, 115–118. [Google Scholar] [CrossRef]
- Mayergoyz, I.D.; Fredkin, D.R.; Zhang, Z. Electrostatic (plasmon) resonances in nanoparticles. Phys. Rev. B 2005, 72, 155412. [Google Scholar] [CrossRef]
- Wang, F.; Shen, Y.R. General properties of local plasmons in metal nanostructures. Phys. Rev. Lett. 2006, 97, 206806. [Google Scholar] [CrossRef] [PubMed]
- Sihvola, A.; Lindell, I.V. Transmission Line Analogy for Calculating the Effective Permittivity of Mixtures with Spherical Multilayer Scatterers. J. Electromagn. Waves Appl. 1988, 2, 741–756. [Google Scholar]
- Alù, A.; Engheta, N. Polarizabilities and effective parameters for collections of spherical nanoparticles formed by pairs of concentric double-negative, single-negative, and/or double-positive metamaterial layers. J. Appl. Phys. 2005, 97, 094310. [Google Scholar] [CrossRef]
- Tzarouchis, D.C.; Sihvola, A. General scattering characteristics of resonant core–shell spheres. IEEE Trans. Antennas Propag. 2018, 66, 323–330. [Google Scholar] [CrossRef]
- Mukhopadhyay, G.; Lundqvist, S. Density oscillations and density response in systems with nonuniform electron density. Nuovo Cimento B 1975, 27, 1–18. [Google Scholar] [CrossRef]
- Román-Velázquez, C.E.; Noguez, C. Designing the plasmonic response of shell nanoparticles: Spectral representation. J. Chem. Phys. 2011, 134, 044116. [Google Scholar] [CrossRef] [PubMed]
- Prodan, E.; Nordlander, P. Exchange and correlations effects in small metallic nanoshells. Chem. Phys. Lett. 2001, 349, 153–160. [Google Scholar] [CrossRef]
- Prodan, E.; Nordlander, P. Electronic structure and polarizability of metallic nanoshells. Chem. Phys. Lett. 2002, 352, 140–146. [Google Scholar] [CrossRef]
- Chýlek, P. Resonance structure of Mie scattering: distance between resonances. J. Opt. Soc. Am. A 1990, 7, 1609–1613. [Google Scholar] [CrossRef]
- Lam, C.C.; Leung, P.T.; Young, K. Explicit asymptotic formulas for the positions, widths, and strengths of resonances in Mie scattering. J. Opt. Soc. Am. B 1992, 9, 1585–1592. [Google Scholar] [CrossRef]
- Kuwata, H.; Tamaru, H.; Esumi, K.; Miyano, K. Resonant light scattering from metal nanoparticles: Practical analysis beyond rayleigh approximation. Appl. Phys. Lett. 2003, 83, 4625–4627. [Google Scholar] [CrossRef]
- Le Ru, E.C.; Somerville, W.R.C.; Auguié, B. Radiative correction in approximate treatments of electromagnetic scattering by point and body scatterers. Phys. Rev. A 2013, 87, 012504. [Google Scholar] [CrossRef]
- Schebarchov, D.; Auguié, B.; Le Ru, E.C. Simple accurate approximations for the optical properties of metallic nanospheres and nanoshells. Phys. Chem. Chem. Phys. 2013, 15, 4233–4242. [Google Scholar] [CrossRef] [PubMed]
- Colom, R.; Devilez, A.; Enoch, S.; Stout, B.; Bonod, N. Polarizability expressions for predicting resonances in plasmonic and Mie scatterers. Phys. Rev. A 2017, 95, 63833. [Google Scholar] [CrossRef]
- Conwell, P.R.; Barber, P.W.; Rushforth, C.K. Resonant spectra of dielectric spheres. J. Opt. Soc. Am. A 1984, 1, 62–67. [Google Scholar] [CrossRef]
- Power, D. Approximate analytic continuation of the Rayleigh series (radar cross-section). IEEE Trans. Antennas Propag. 1988, 36, 1652–1654. [Google Scholar] [CrossRef]
- Tzarouchis, D.C.; Ylä-Oijala, P.; Sihvola, A. Unveiling the scattering behavior of small spheres. Phys. Rev. B 2016, 94, 140301. [Google Scholar] [CrossRef]
- Baker, G.A.; Graves-Morris, P.R. Padé Approximants; Cambridge University Press: Cambridge, UK, 1996; Volume 59. [Google Scholar]
- Bender, C.M.; Orszag, S.A. Advanced Mathematical Methods for Scientists and Engineers I: Asymptotic Methods and Perturbation Theory; Springer Science & Business Media: Berlin, Germany, 2013. [Google Scholar]
- Mei, Z.; Sarkar, T.K.; Salazar-Palma, M. A study of negative permittivity and permeability for small sphere. IEEE Antennas Wirel. Propag. Lett. 2013, 12, 1228–1231. [Google Scholar] [CrossRef]
- Meier, M.; Wokaun, A. Enhanced fields on large metal particles: Dynamic depolarization. Opt. Lett. 1983, 8, 581–583. [Google Scholar] [CrossRef] [PubMed]
- De Vries, P.; van Coevorden, D.V.; Lagendijk, A. Point scatterers for classical waves. Rev. Mod. Phys. 1998, 70, 447–466. [Google Scholar] [CrossRef]
- Doyle, W.T. Optical properties of a suspension of metal spheres. Phys. Rev. B 1989, 39, 9852–9858. [Google Scholar] [CrossRef]
- Carminati, R.; Greffet, J.J.; Henkel, C.; Vigoureux, J.M. Radiative and non-radiative decay of a single molecule close to a metallic nanoparticle. Opt. Commun. 2006, 261, 368–375. [Google Scholar] [CrossRef]
- Moroz, A. Depolarization field of spheroidal particles. J. Opt. Soc. Am. B 2009, 26, 517–527. [Google Scholar] [CrossRef]
- Yu, R.; Liz-Marzán, L.M.; García de Abajo, F.J. Universal analytical modeling of plasmonic nanoparticles. Chem. Soc. Rev. 2017, 46, 6710–6724. [Google Scholar] [CrossRef] [PubMed]
- Liberal, I.; Ziolkowski, R.W. Analytical and equivalent circuit models to elucidate power balance in scattering problems. IEEE Trans. Antennas Propag. 2013, 61, 2714–2726. [Google Scholar] [CrossRef]
- Tretyakov, S. Maximizing absorption and scattering by dipole particles. Plasmonics 2014, 9, 935–944. [Google Scholar] [CrossRef]
- Osipov, A.V.; Tretyakov, S.A. Modern Electromagnetic Scattering Theory with Applications; Wiley: Hoboken, NJ, USA, 2017. [Google Scholar]
- Geffrin, J.M.; García-Cámara, B.; Gómez-Medina, R.; Albella, P.; Froufe-Pérez, L.S.; Eyraud, C.; Litman, A.; Vaillon, R.; González, F.; Nieto-Vesperinas, M.; et al. Magnetic and electric coherence in forward- and back-scattered electromagnetic waves by a single dielectric subwavelength sphere. Nat. Commun. 2012, 3, 1171. [Google Scholar] [CrossRef] [PubMed]
- Person, S.; Jain, M.; Lapin, Z.; Sáenz, J.J.; Wicks, G.; Novotny, L. Demonstration of zero optical backscattering from single nanoparticles. Nano Lett. 2013, 13, 1806–1809. [Google Scholar] [CrossRef] [PubMed]
- Luk’yanchuk, B.S.; Voshchinnikov, N.V.; Paniagua-Domínguez, R.; Kuznetsov, A.I. Optimum forward light scattering by spherical and spheroidal dielectric nanoparticles with high refractive index. ACS Photonics 2015, 2, 993–995. [Google Scholar] [CrossRef]
- Alaee, R.; Filter, R.; Lehr, D.; Lederer, F.; Rockstuhl, C. A generalized Kerker condition for highly directive nanoantennas. Opt. Lett. 2015, 40, 2645–2648. [Google Scholar] [CrossRef] [PubMed]
- Tzarouchis, D.C.; Ylä-Oijala, P.; Sihvola, A. Light scattering characteristics of a small sphere: Resonant and directive scattering conditions. In Proceeding of the 2017 Progress in Electromagnetics Research Symposium, St Petersburg, Russia, 22–25 May 2017; pp. 1503–1510. [Google Scholar]
- Kerker, M.; Wang, D.S.; Giles, C.L. Electromagnetic scattering by magnetic spheres. J. Opt. Soc. Am. 1983, 73, 765–767. [Google Scholar] [CrossRef]
- Liberal, I.; Ederra, I.; Gonzalo, R.; Ziolkowski, R.W. Induction theorem analysis of resonant nanoparticles: Design of a Huygens source nanoparticle laser. Phys. Rev. Appl. 2014, 1, 044002. [Google Scholar] [CrossRef]
- Naraghi, R.R.; Sukhov, S.; Dogariu, A. Directional control of scattering by all-dielectric core-shell spheres. Opt. Lett. 2015, 40, 585–588. [Google Scholar] [CrossRef] [PubMed]
- Fu, Y.H.; Kuznetsov, A.I.; Miroshnichenko, A.E.; Yu, Y.F.; Luk’yanchuk, B. Directional visible light scattering by silicon nanoparticles. Nat. Commun. 2013, 4, 1527. [Google Scholar] [CrossRef] [PubMed]
- Ziolkowski, R.W. Metamaterial-inspired, electrically small Huygens sources. IEEE Antennas Wirel. Propag. Lett. 2010, 9, 501–505. [Google Scholar]
- Ziolkowski, R.W. Using Huygens multipole arrays to realize unidirectional needle-like radiation. Phys. Rev. X 2017, 7, 31017. [Google Scholar] [CrossRef]
- Campione, S.; Basilio, L.I.; Warne, L.K.; Sinclair, M.B. Tailoring dielectric resonator geometries for directional scattering and Huygens’ metasurfaces. Opt. Express 2015, 23, 2293. [Google Scholar] [CrossRef] [PubMed]
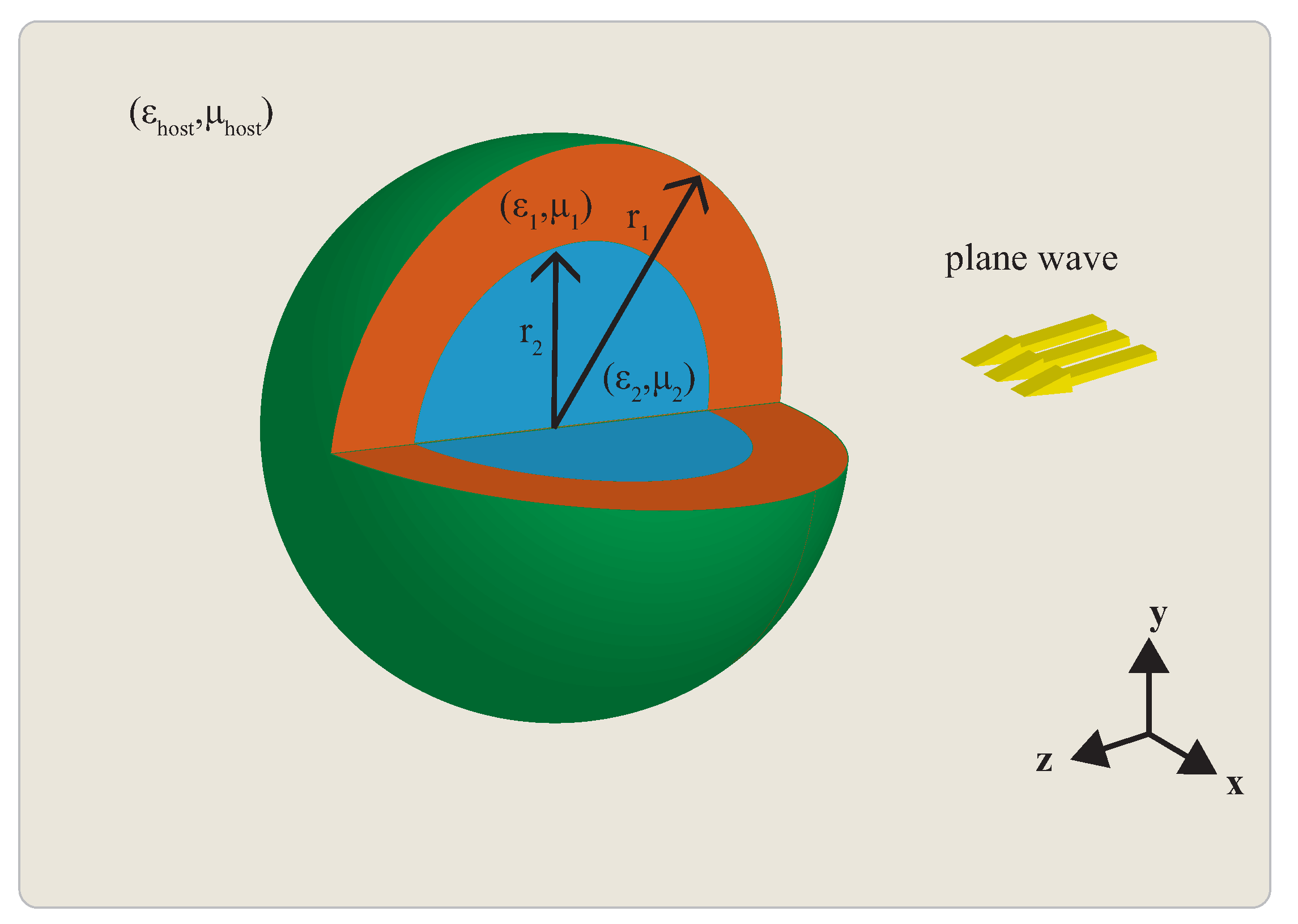
| n | 1 | 2 | 3 | 4 | 5 |
| 4.4934 | 5.7634 | 6.9879 | 8.1428 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tzarouchis, D.; Sihvola, A. Light Scattering by a Dielectric Sphere: Perspectives on the Mie Resonances. Appl. Sci. 2018, 8, 184. https://doi.org/10.3390/app8020184
Tzarouchis D, Sihvola A. Light Scattering by a Dielectric Sphere: Perspectives on the Mie Resonances. Applied Sciences. 2018; 8(2):184. https://doi.org/10.3390/app8020184
Chicago/Turabian StyleTzarouchis, Dimitrios, and Ari Sihvola. 2018. "Light Scattering by a Dielectric Sphere: Perspectives on the Mie Resonances" Applied Sciences 8, no. 2: 184. https://doi.org/10.3390/app8020184
APA StyleTzarouchis, D., & Sihvola, A. (2018). Light Scattering by a Dielectric Sphere: Perspectives on the Mie Resonances. Applied Sciences, 8(2), 184. https://doi.org/10.3390/app8020184





