Waveguided Approach for Difference Frequency Generation of Broadly-Tunable Continuous-Wave Terahertz Radiation
Abstract
Featured Application
Abstract
1. Introduction
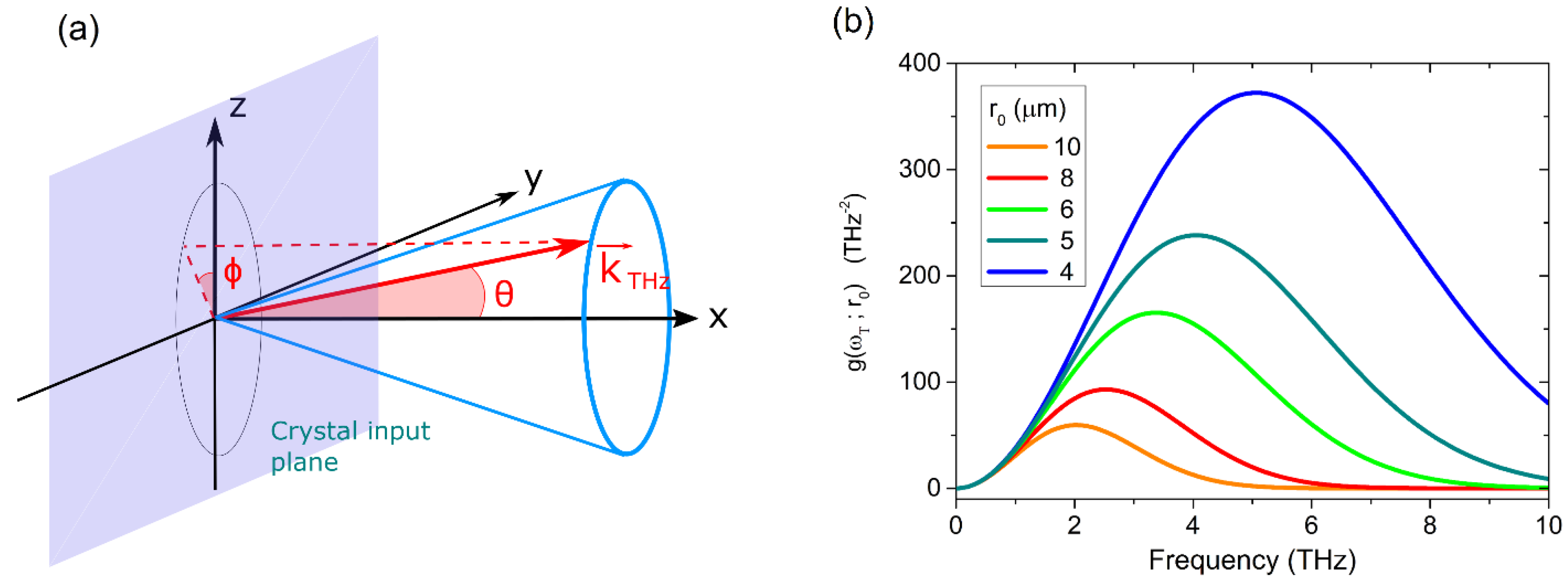
2. Theory
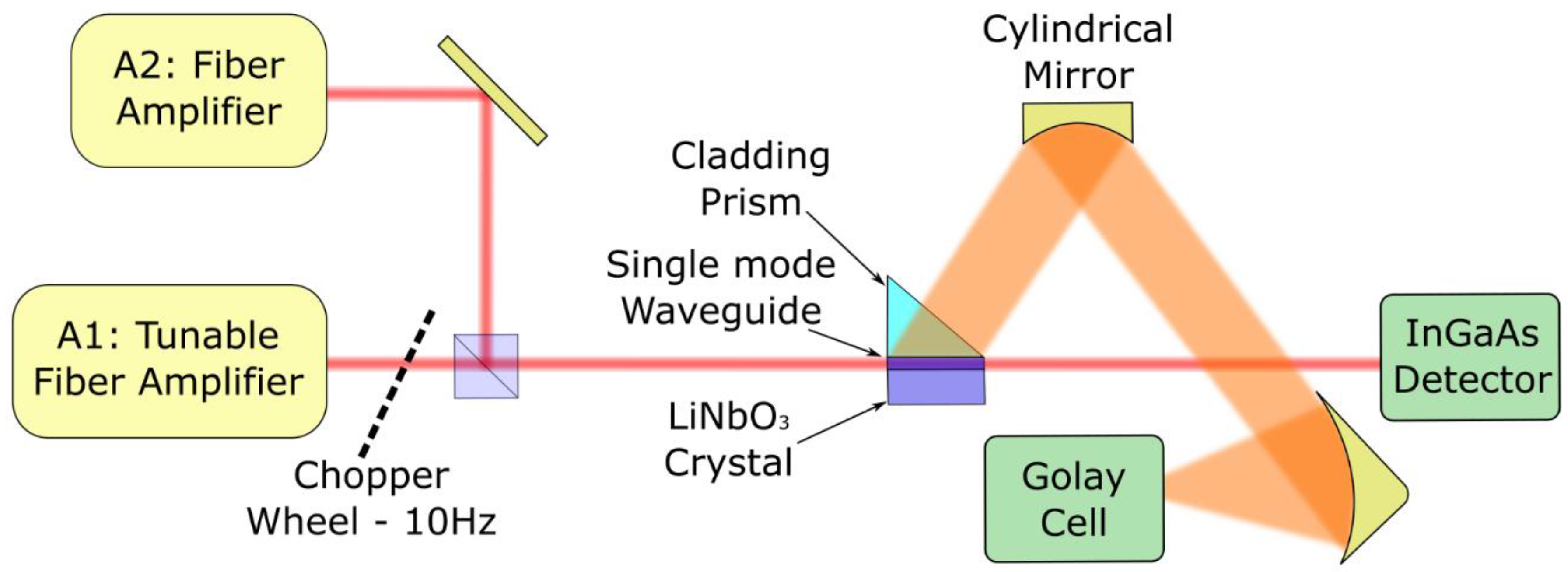
3. Experimental Results and Discussion
3.1. Waveguide Characterization and Power Handling
3.2. THz Generation Efficiency and Spectral Coverage
4. Conclusions
Author Contributions
Acknowledgments
Conflicts of Interest
References
- Federici, J.F. THz imaging and sensing for security applications-explosives, weapons and drugs. Semicond. Sci. Technol. 2005, 20, 266–280. [Google Scholar] [CrossRef]
- Mittleman, D.M.; Jacobsen, R.H.; Nuss, M.C. T-ray imaging. IEEE J. Sel. Top. Quantum Electron. 1996, 2, 679–692. [Google Scholar] [CrossRef]
- Joerdens, C. Detection of foreign bodies in chocolate with pulsed terahertz spectroscopy. Opt. Eng. 2008, 47. [Google Scholar] [CrossRef]
- Locatelli, M.; Ravaro, M.; Bartalini, S.; Consolino, L.; Vitiello, M.S.; Cicchi, R.; de Natale, P. Real-time terahertz digital holography with a quantum-cascade-laser. Sci. Rep. 2015, 5, 13566. [Google Scholar] [CrossRef] [PubMed]
- Consolino, L.; Bartalini, S.; de Natale, P. Terahertz Frequency Metrology for Spectroscopic Applications: A Review. J. Infrared, Millim. Terahertz Waves 2017, 38, 12891315. [Google Scholar] [CrossRef]
- Bellini, M.; Catacchini, E.; de Natale, P.; di Lonardo, G.; Fusina, L.; Inguscio, M. Coherent FIR spectroscopy of molecules of atmospheric interest. Infrared Phys. Technol. 1995, 36, 37–44. [Google Scholar] [CrossRef]
- Pagani, L.; Herpin, F.; Gerin, M.; Encrenaz, P.J. Herschel and the TeraHertz sky. Comptes Rendus Phys. 2012, 13, 5–13. [Google Scholar] [CrossRef]
- Webster, C.R.; Mahaffy, P.R. Determining the local abundance of Martian methane and its13C/12C and D/H isotopic ratios for comparison with related gas and soil analysis on the 2011 Mars Science Laboratory (MSL) mission. Planet. Space Sci. 2011, 59, 2–3. [Google Scholar] [CrossRef]
- de Lucia, F.C. The submillimeter: A spectroscopists view. J. Mol. Spectrosc. 2010, 261, 1–17. [Google Scholar] [CrossRef]
- Consolino, L.; Bartalini, S.; Beere, H.E.; Ritchie, D.A.; Vitiello, M.S.; DeNatale, P. THz QCL based cryogen-free spectrometer for in Situ trace gas sensing. Sensors 2013, 13, 3331–3340. [Google Scholar] [CrossRef] [PubMed]
- Evenson, K.M.; Jennings, D.A.; Petersen, F.R. Tunable far-infrared spectroscopy. Appl. Phys. Lett. 1984, 44, 576–578. [Google Scholar] [CrossRef]
- Nolt, I.G.; Radostitz, J.V.; di Lonardo, G.; Evenson, K.M.; Jennings, D.A.; Leopold, K.R.; Vanek, M.D.; Zink, L.R.; Hinz, A.; Chance, K.V. Accurate rotational constants of CO, HCl, and HF: Spectral standards for the 0.3- to 6-THz (10- to 200 cm−1) region. J. Mol. Spectrosc. 1987, 125, 274–287. [Google Scholar] [CrossRef]
- Zink, L.R.; de Natale, P.; Pavone, F.S.; Prevedelli, M.; Evenson, K.M.; Inguscio, M. Rotational far infrared spectrum of 13CO. J. Mol. Spectrosc. 1990, 143, 304–310. [Google Scholar] [CrossRef]
- Khler, R.; Tredicucci, A.; Beltram, F.; Beere, H.E.; Linfield, E.H.; Davies, A.G.; Ritchie, D.A.; Iotti, R.C.; Rossi, F. Terahertz semiconductor-heterostructure laser. Nature 2002, 411, 156–159. [Google Scholar] [CrossRef] [PubMed]
- Belkin, M.A.; Capasso, F. New frontiers in quantum cascade lasers: High performance room-temperature terahertz sources. Phys. Scr. 2015, 90, 118002. [Google Scholar] [CrossRef]
- Schrottke, L.; Wienold, M.; Sharma, R.; Biermann, K.; Hey, R.; Tahraoui, A.; Richter, H.; Huebers, H.W.; Grahn, H.T. Quantum-cascade lasers as local oscillators for heterodyne spectrometers in the spectral range around 4.745 THz. Semiconduct. Sci. Technol. 2013, 28, 035011. [Google Scholar] [CrossRef]
- Ohtani, K.; Turinkov, D.; Bonzon, C.; Benea-Chelmus, I.C.; Beck, M.; Faist, J.; Justen, M.; Graf, U.U.; Mertens, M.; Stutzki, J. High performance 4.7 THz GaAs quantum cascade lasers based on four quantum wells. New J. Phys. 2016, 18, 035011. [Google Scholar] [CrossRef]
- Odashima, H.; Zink, L.R.; Evenson, K.M. Tunable far-infrared spectroscopy extended to 9.1 THz. Opt. Lett. 1999, 18, 123004. [Google Scholar] [CrossRef]
- di Lonardo, G.; Fusina, L.; de Natale, P.; Inguscio, M.; Prevedelli, M. The pure rotation spectrum of HBr in the submillimeter-wave region. J. Mol. Spectrosc. 1991, 24, 406–407. [Google Scholar] [CrossRef]
- Bellini, M.; de Natale, P.; di Lonardo, G.; Fusina, L.; Inguscio, M.; Prevedelli, M. Tunable far infrared spectroscopy of 16O3 ozone. J. Mol. Spectrosc. 1992, 152, 256–259. [Google Scholar] [CrossRef]
- Modugno, G.; de Natale, P.; Bellini, M.; Inguscio, M.; di Lonardo, G.; Fusina, L.; Auwera, J.V. Precise measurement of molecular dipole moments with a tunable far-infrared Stark spectrometer: Application to HOCl. J. Opt. Soc. Am. B 1996, 13, 1645–1649. [Google Scholar] [CrossRef]
- Odashima, H.; Tachikawa, M.; Zink, L.R.; Evenson, K.M. Extension of tunable far-infrared spectroscopy to 7.9THz. Opt. Lett. 1997, 22, 822–824. [Google Scholar] [CrossRef] [PubMed]
- Viciani, S.; de Natale, P.; Gianfrani, L.; Inguscio, M. Magnetic-field effects on molecular transitions in the far-infrared region: Prospects for more-sensitive spectrometers. J. Opt. Soc. Am. B-Opt. Phys. 1999, 16, 301–307. [Google Scholar] [CrossRef]
- Bartalini, S.; Consolino, L.; Cancio, P.; de Natale, P.; Bartolini, P.; Taschin, A.; de Pas, M.; Beere, H.; Ritchie, D.; Vitiello, M.S.; et al. Frequency-comb-assisted terahertz quantum cascade laser spectroscopy. Phys. Rev. X 2014. [Google Scholar] [CrossRef]
- Consolino, L.; Campa, A.; Ravaro, M.; Mazzotti, D.; Vitiello, M.S.; Bartalini, S.; de Natale, P. Saturated absorption in a rotational molecular transition at 2.5 THz using a quantum cascade laser. Appl. Phys. Lett. 2015, 4, 021006. [Google Scholar] [CrossRef]
- Wienold, M.; Alam, T.; Schrottke, L.; Grahn, H.T.; Huebers, H.W. Doppler-free spectroscopy with a terahertz quantum-cascade-laser. Opt. Exp. 2018, 26, 315862–316870. [Google Scholar] [CrossRef] [PubMed]
- Yu, N.E.; Oh, M.K.; Kang, H.; Jung, C.; Kim, B.H.; Lee, K.S.; Ko, D.K.; Takekawa, S.; Kitamura, K. Continuous tuning of a narrow-band terahertz wave in periodically poled stoichiometric LiTaO3 crystal with a fan-out grating structure. Appl. Phys. Express. 2014, 7. [Google Scholar] [CrossRef]
- Consolino, L.; Taschin, A.; Bartolini, P.; Bartalini, S.; Cancio, P.; Tredicucci, A.; Beere, H.E.; Ritchie, D.A.; Torre, R.; Vitiello, M.S.; et al. Phase-locking to a free-space terahertz comb for metrological-grade terahertz lasers. Nat. Commun. 2012, 3, 1040. [Google Scholar] [CrossRef] [PubMed]
- Huillier, J.A.L.; Torosyan, G.; Theuer, M.; Rau, C.; Avetisyan, Y.; Beigang, R. Generation of THz radiation using bulk, periodically and aperiodically poled lithium niobate-Part 2: Experiments. Appl. Phys. B Lasers Opt. 2007, 86, 197–208. [Google Scholar] [CrossRef]
- Huillier, J.A.L.; Torosyan, G.; Theuer, M.; Rau, C.; Avetisyan, Y.; Beigang, R. Generation of THz radiation using bulk, periodically and aperiodically poled lithium niobate-Part 1: Theory. Appl. Phys. B Lasers Opt. 2007, 86, 185–196. [Google Scholar] [CrossRef]
- Palfalvi, L.; Fulop, J.A.; Almasi, G.; Hebling, J. Novel setups for extremely high power single-cycle terahertz pulse generation by optical rectification. Appl. Phys. Lett. 2008, 92, 171107. [Google Scholar] [CrossRef]
- Theuer, M.; Torosyan, G.; Rau, C.; Beigang, R.; Maki, K.; Otani, C.; Kawase, K. Efficient generation of Cherenkov-type terahertz radiation from a lithium niobite crystal with a silicon prism output coupler. Appl. Phys. Lett. 2006, 88, 071122. [Google Scholar] [CrossRef]
- Kawase, K.; Ichino, S.; Suizu, K.; Shibuya, T. Half cycle terahertz pulse generation by prism coupled cherenkov phase-matching method. J. Infrared Millim. Terahertz Waves 2011, 32, 1168–1177. [Google Scholar] [CrossRef]
- Wang, J.; Zhu, B.; Hao, Z.; Bo, F.; Wang, X.; Gao, F.; Li, Y.; Zhang, G.; Xu, J.J. Thermo-optic effects in on-chip lithium niobate microdisk resonators. Opt. Exp. 2016, 24, 21869–21879. [Google Scholar] [CrossRef] [PubMed]
- Villarroel, J.; Carnicero, J.; Luedtke, F.; Carrascosa, M.; Cabaes, G.; Cabrera, J.M.; Alcazar, A.; Ramiro, B. Analysis of photorefractive optical damage in lithium niobate: Application to planar waveguides. Opt. Express 2010, 18, 20852–20861. [Google Scholar] [CrossRef] [PubMed]
- de Regis, M.; Bartalini, S.; Ravaro, M.; Calonico, D.; de Natale, P.; Consolino, L. Room-Temperature Continuous-Wave Frequency-Referenced Spectrometer up to 7.5 THz. 2018; Submitted for publication. [Google Scholar]
- Cazzoli, G.; Puzzarini, C. Sub-doppler resolution in the THz frequency domain: 1 kHz accuracy at 1 THz by exploiting the lamb-dip technique. J. Phys. Chem. A 2013, 117, 13759–13766. [Google Scholar] [CrossRef] [PubMed]
- Tanabe, T.; Suto, K.; Nishizawa, J.I.; Sasaki, T. Characteristic of terahertz-wave generation from GaSe crystals. J. Phys. D Appl. Phys. 2004, 37, 155–158. [Google Scholar] [CrossRef]
- Roberts, D.A. Simplified Characterization of Uniaxial and Biaxial Nonlinear Optical Crystals: A Plea for Standardization of Nomenclature and Conventions. IEEE J. Quantum Electron. 1992, 28, 2057–2074. [Google Scholar] [CrossRef]
- Harada, A.; Nihei, Y. Bulk periodically poled MgO-LiNbO3 by corona discharge method. Appl. Phys. Lett. 1996, 69, 2629. [Google Scholar] [CrossRef]
- Furuya, H.; Morikawa, A.; Mizuuchi, K.; Yamamoto, K. High-beam-quality continuous wave 3 W green-light generation in bulk periodically poled MgO:LiNbO3. Jpn. J. Appl. Phys. Part 1 2006, 45, 6704–6707. [Google Scholar] [CrossRef]
- Bryan, D.A.; Gerson, R.; Tomaschke, H.E. Increased optical damage resistance in lithium niobate. Appl. Phys. Lett. 1984, 44, 847. [Google Scholar] [CrossRef]
- Schwesyg, J.R.; Phillips, C.R.; Ioakeimidi, K.; Kajiyama, M.C.C.; Falk, M.; Jundt, D.H.; Fejer, M.M. Suppression of mid-infrared light absorption in undoped congruent lithium niobate crystals. Opt. Lett. 2010, 35, 1070–1072. [Google Scholar] [CrossRef] [PubMed]
- Kiessling, J.; Breunig, I.; Schunemann, P.G.; Buse, K.; Vodopyanov, K.L. High power and spectral purity continuous-wave photonic THz source tunable from 1 to 4.5THz for nonlinear molecular spectroscopy. New J. Phys. 2013, 15, 105014. [Google Scholar] [CrossRef]
- Sowade, R.; Breunig, I.; Mayorga, I.C.; Kiessling, J.; Tulea, C.; Dierolf, V.; Buse, K. Continuous-wave optical parametric terahertz source. Opt. Express 2009, 17, 22303–22310. [Google Scholar] [CrossRef] [PubMed]
- Unferdorben, M.; Szaller, Z.; Hajdara, I.; Hebling, J.; Palfalvi, L. Measurement of Refractive Index and Absorption Coefficient of Congruent and Stoichiometric Lithium Niobate in the Terahertz Range. J. Infrared Millim. Terahertz Waves 2015, 36, 1203–1209. [Google Scholar] [CrossRef]
- Gayer, O.; Sacks, Z.; Galun, E.; Arie, A. Temperature and wavelength dependent refractive index equations for MgO-doped congruent and stoichiometric LiNbO3. Appl. Phys. B Lasers Opt. 2008, 91, 343–348. [Google Scholar] [CrossRef]
- Kiessling, J.; Buse, K.; Breunig, I. Temperature-dependent Sellmeier equation for the extraordinary refractive index of 5%MgO-dopedLiNbO3 in the terahertz range. J. Opt. Soc. Am. B. 2013, 30, 950–952. [Google Scholar] [CrossRef]
- Schmidt, R.V.; Kaminow, I.P. Metal-diffused optical waveguide in LiNbO3. Appl. Phys. Lett. 1974, 25, 458. [Google Scholar] [CrossRef]
- Suzaki, Y.; Tachibana, A. Measurement of the µm sized radius of Gaussian laser beam using the scanning knife-edge. App. Opt. 1975, 14, 2809–2810. [Google Scholar] [CrossRef] [PubMed]
- Kaul, A.N.; Thyagarajan, K. Inverse WKB method for refractive index profile estimation of monomode graded index planar optical waveguides. Opt. Commun. 1984, 48, 313316. [Google Scholar] [CrossRef]
- Boyd, R.W. Nonlinear Optics, 3rd ed.; Academic Press: Orlando, FL, USA, 2008; ISBN 0123694701, 9780123694706. [Google Scholar]
- Gallerano, P.; Doria, A.; Giovenale, E.; Spassovsky, I. High power THz sources and applications at ENEA-Frascati. J. Infrared Millim. Terahertz Waves 2014, 35, 1724. [Google Scholar] [CrossRef]
- Bakker, H.J.; Hunsche, S.; Kurz, H. Investigation of anharmonic lattice vibrations with coherent phonon polaritons. Phys. Rev. B 1994, 50, 914–920. [Google Scholar] [CrossRef]
- Born, M.; Wolf, E. Principles of Optics: Electromagnetic Theory of Propagation, Interference and Diffraction of Light; Cambridge University Press: Cambridge, UK, 1999. [Google Scholar] [CrossRef]


© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
De Regis, M.; Consolino, L.; Bartalini, S.; De Natale, P. Waveguided Approach for Difference Frequency Generation of Broadly-Tunable Continuous-Wave Terahertz Radiation. Appl. Sci. 2018, 8, 2374. https://doi.org/10.3390/app8122374
De Regis M, Consolino L, Bartalini S, De Natale P. Waveguided Approach for Difference Frequency Generation of Broadly-Tunable Continuous-Wave Terahertz Radiation. Applied Sciences. 2018; 8(12):2374. https://doi.org/10.3390/app8122374
Chicago/Turabian StyleDe Regis, Michele, Luigi Consolino, Saverio Bartalini, and Paolo De Natale. 2018. "Waveguided Approach for Difference Frequency Generation of Broadly-Tunable Continuous-Wave Terahertz Radiation" Applied Sciences 8, no. 12: 2374. https://doi.org/10.3390/app8122374
APA StyleDe Regis, M., Consolino, L., Bartalini, S., & De Natale, P. (2018). Waveguided Approach for Difference Frequency Generation of Broadly-Tunable Continuous-Wave Terahertz Radiation. Applied Sciences, 8(12), 2374. https://doi.org/10.3390/app8122374





