Analytical Model and Gas Leak Source Localization Based on Acoustic Emission for Cylindrical Storage
Abstract
1. Introduction
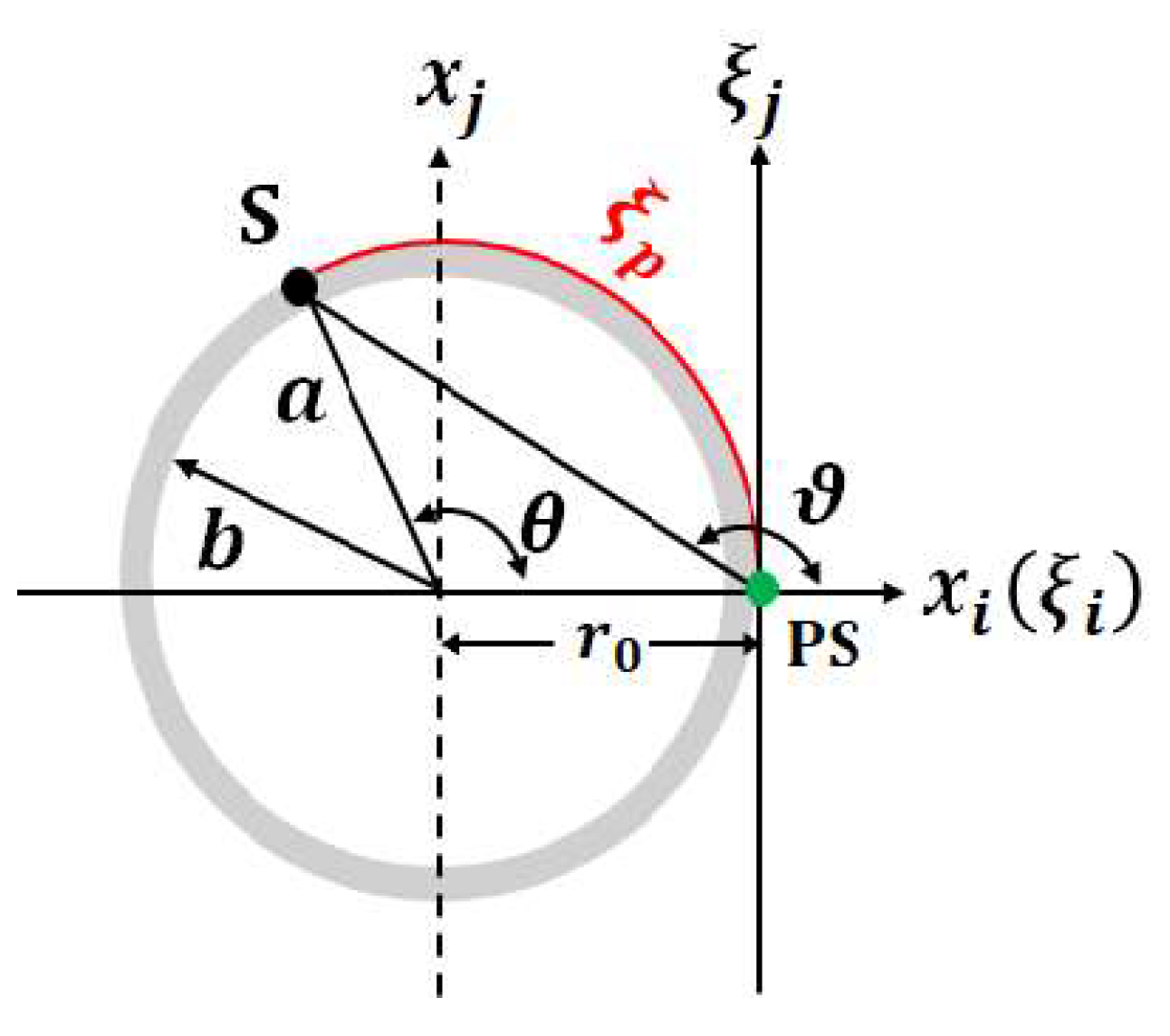
2. Mathematical Solution
2.1. Concentrated Forces
2.2. Displacement Fields
2.3. Analytical Model
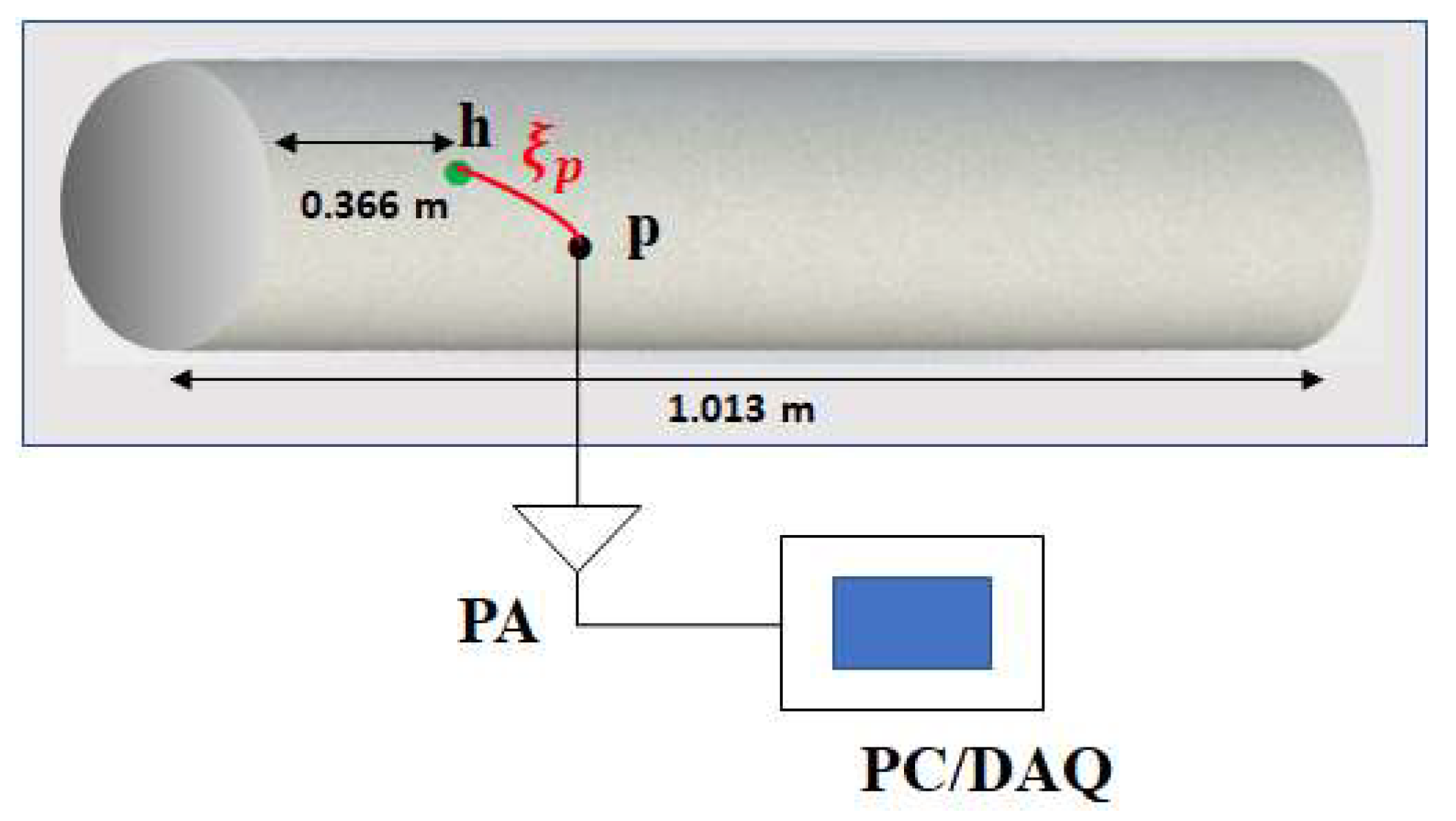
3. Experiment
4. Results and Discussion
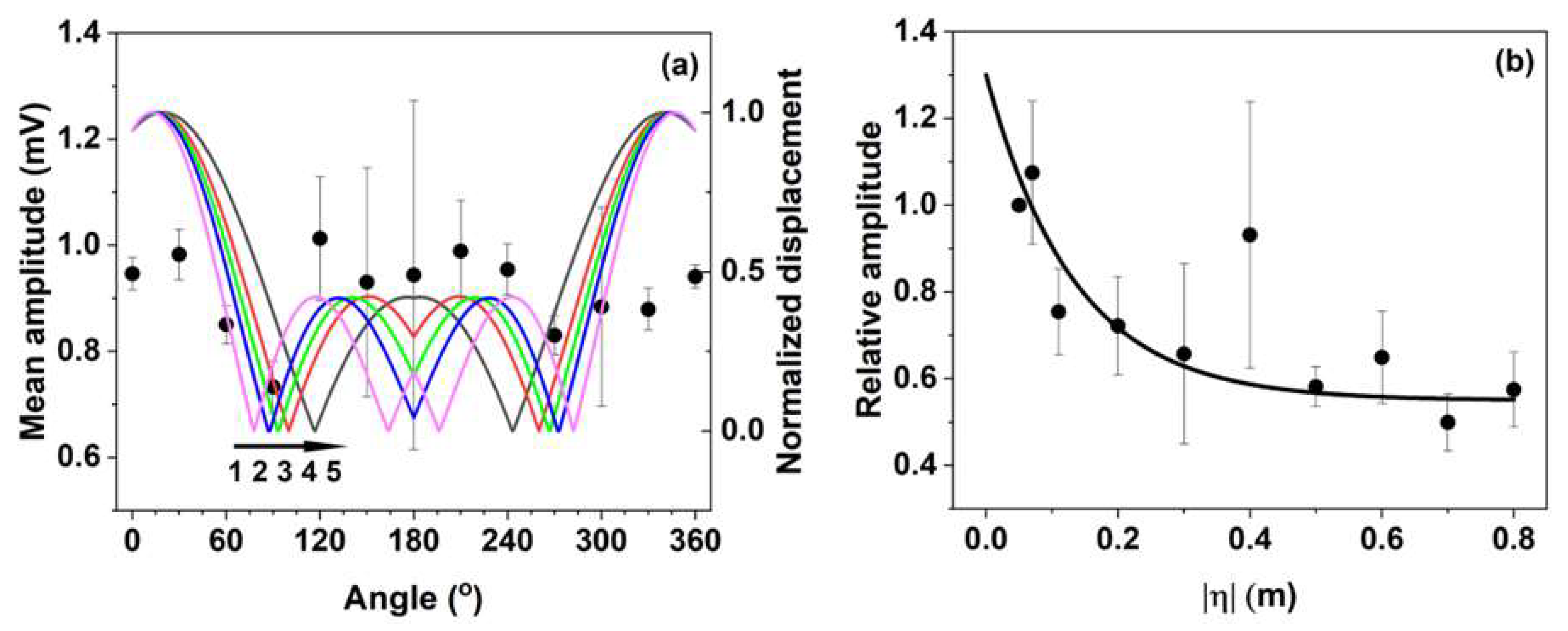
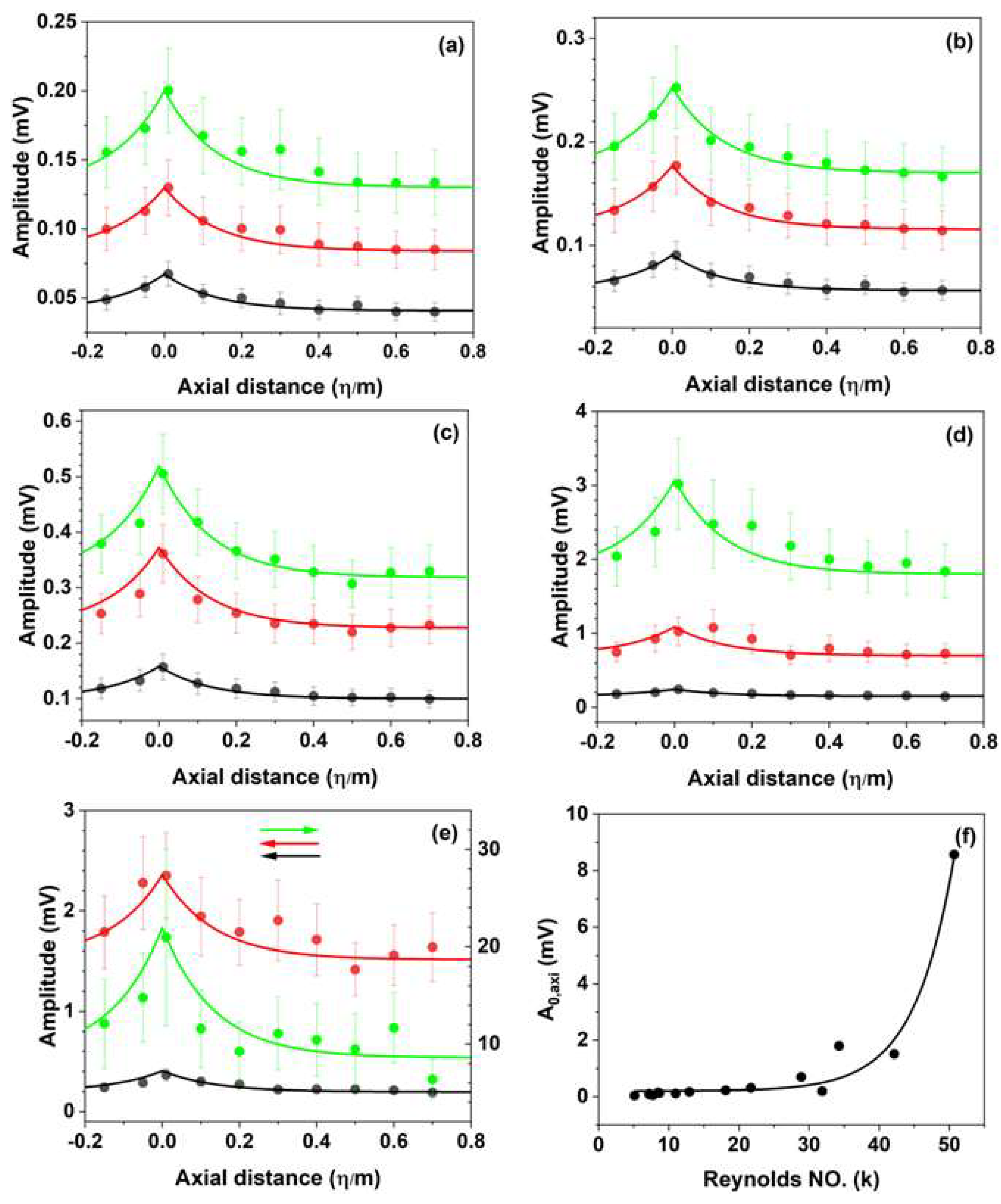
4.1. Determination of and
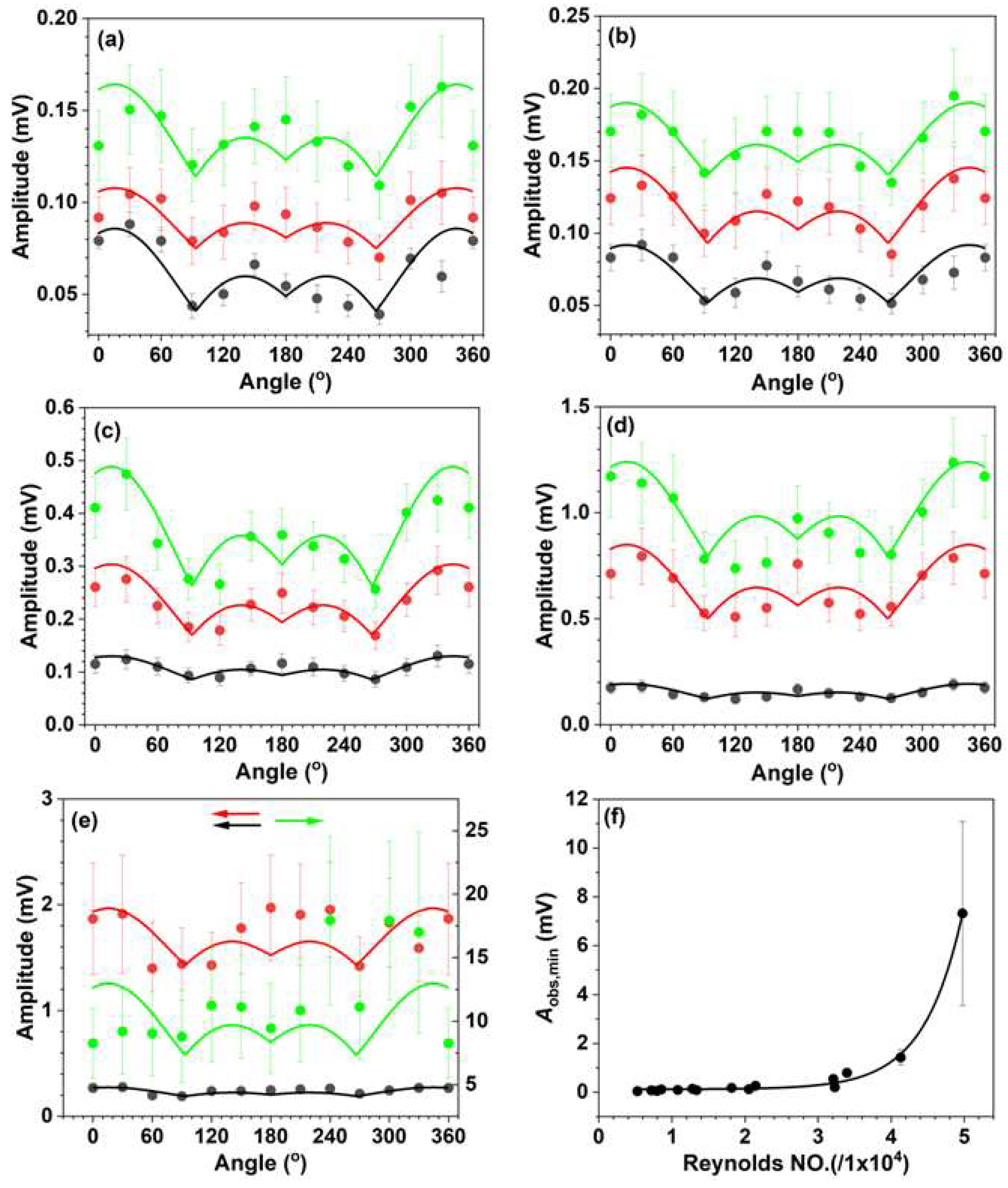
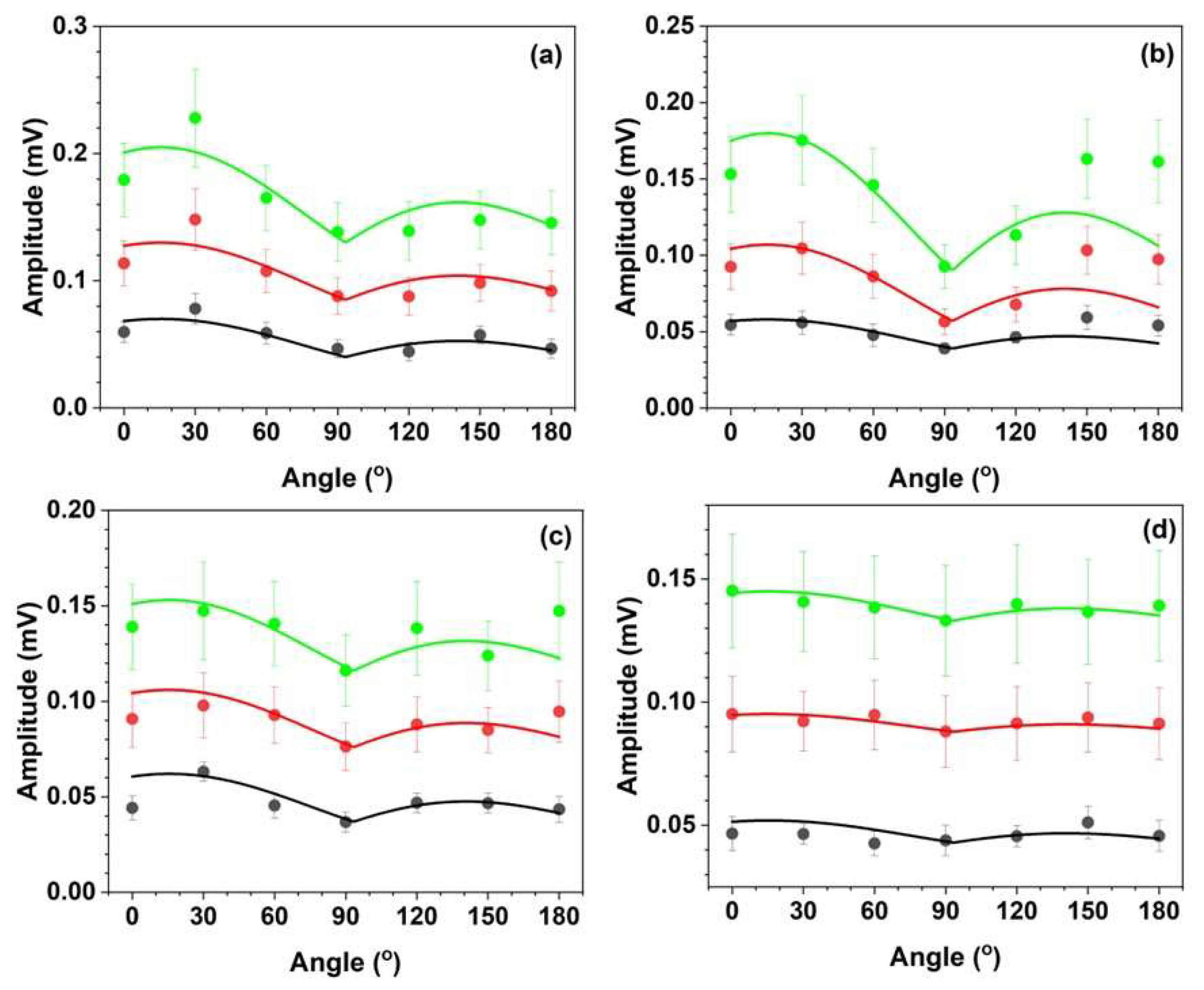
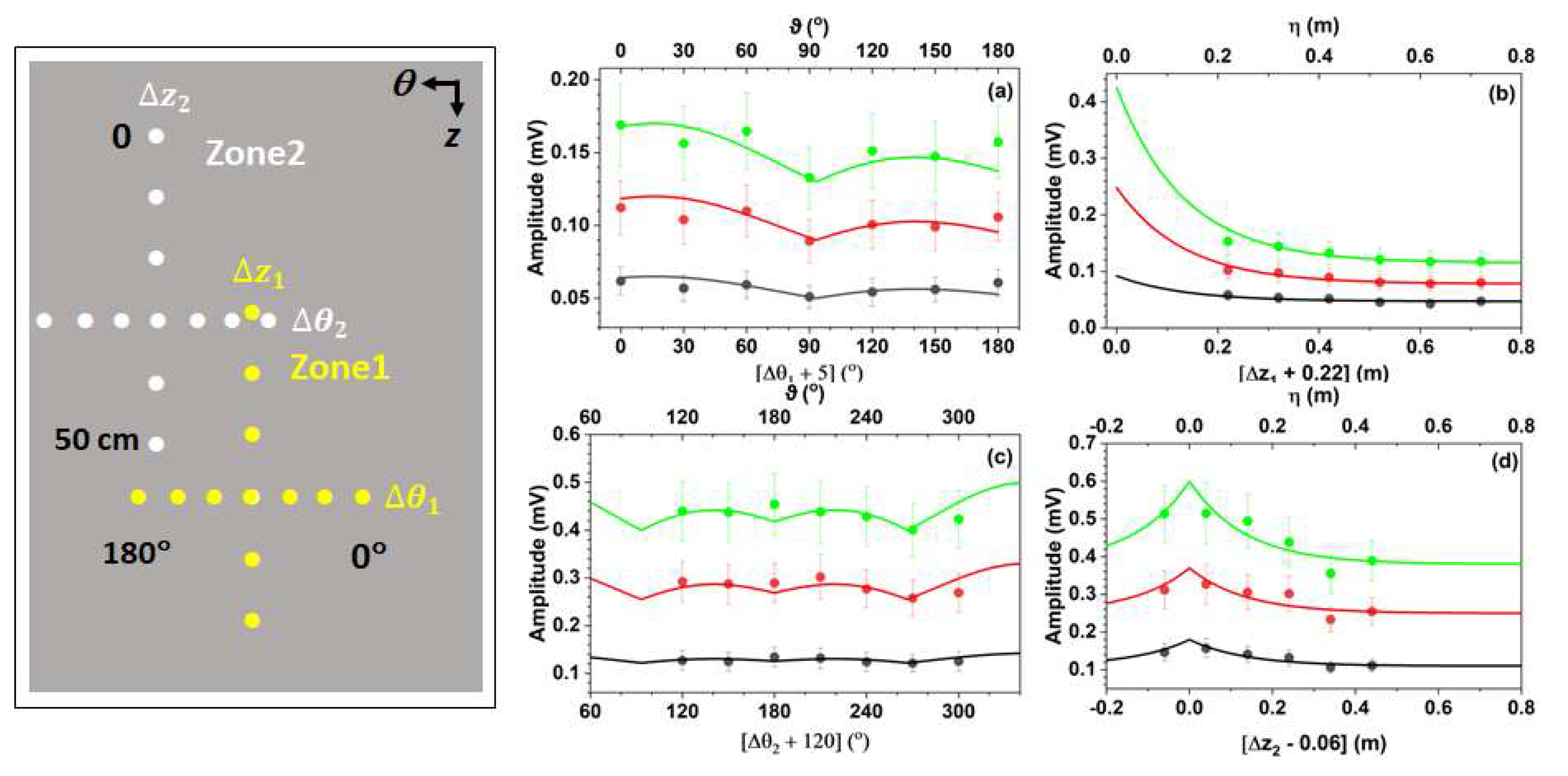
4.2. Verification of Angular and Axial Dependences
4.3. Leak Source Localization
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A. Green’s Function
Appendix B. Leak Characteristics
Appendix C
Appendix C.1. Concentrated Force Incorporated Potential
Appendix C.2. Stress–Strain Displacement Relations
References
- Korlapati, N.V.S.; Khan, F.; Noora, Q.; Mirza, S.; Vaddiraju, S. Review and Analysis of Pipeline Leak Detection Methods. J. Pipeline Sci. Eng. 2022, 2, 100074. [Google Scholar] [CrossRef]
- Meng, L.; Li, Y.; Wang, W.; Fu, J. Experimental Study on Leak Detection and Location for Gas Pipeline Based on Acoustic Method. J. Loss Prev. Process Ind. 2012, 25, 90–102. [Google Scholar] [CrossRef]
- Hao, Y.; Du, Z.; Jiang, J.; Xing, Z.; Yan, X.; Wang, S.; Rao, Y. Research on Multipoint Leak Location of Gas Pipeline Based on Variational Mode Decomposition and Relative Entropy. Shock Vib. 2020, 2020, 8868963. [Google Scholar] [CrossRef]
- Quy, T.B.; Kim, J.-M. Pipeline Leak Detection Using Acoustic Emission and State Estimate in Feature Space. IEEE Trans. Instrum. Meas. 2022, 71, 2518709. [Google Scholar] [CrossRef]
- Nguyen, D.-T.; Nguyen, T.K.; Ahmad, Z.; Kim, J.-M. A Reliable Pipeline Leak Detection Method Using Acoustic Emission with Time Difference of Arrival and Kolmogorov–Smirnov Test. Sensors 2023, 23, 9296. [Google Scholar] [CrossRef]
- Xiao, R.; Joseph, P.; Li, J. The Leak Noise Spectrum in Gas Pipeline Systems: Theoretical and Experimental Investigation. J. Sound Vib. 2020, 488, 115646. [Google Scholar] [CrossRef]
- Tian, X.; Jiao, W.; Liu, T. Intelligent Leak Detection Method for Low-Pressure Gas Pipeline inside Buildings Based on Pressure Fluctuation Identification. J. Civ. Struct Health Moni. 2022, 12, 1191–1208. [Google Scholar] [CrossRef]
- Meribout, M. Gas Leak-detection and Measurement Systems: Prospects and Future trends. IEEE Trans. Inst. Meas. 2021, 70, 4505813. [Google Scholar] [CrossRef]
- Hu, Z.; Tariq, S.; Zayed, T. A Comprehensive Review of Acoustic Based Leak Localization Method in Pressurized Pipelines. Mech. Syst. Signal Process. 2021, 161, 107994. [Google Scholar] [CrossRef]
- Liu, Y.; Habibi, D.; Chai, D.; Wang, X.; Chen, H.; Gao, Y.; Li, S. A Comprehensive Review of Acoustic Methods for Locating Underground Pipelines. Appl. Sci. 2020, 10, 1031. [Google Scholar] [CrossRef]
- Miller, R.K.; Carlos, M.F.; Finlayson, R.D.; Godinez-Azcuaga, V.; Rhodes, M.R.; Shu, F.; Wang, W.D. Acoustic Emission Source Location. In Nondestructive Testing Handbook, 3rd ed.; Miller, R.K., Hill, E.K., Moore, P.O., Eds.; American Society for Nondestructive Testing: Columbus, OH, USA, 2005; pp. 121–130. [Google Scholar]
- Kund, T. Mechanics of Elastic Waves and Ultrasonic Nondestructive Evaluation; CRC Press: Boca Raton, FL, USA, 2019; pp. 317–369. [Google Scholar]
- Gao, Y.; Brennan, M.J.; Joseph, P.F.; Muggleton, J.M.; Hunaidi, O. A Model of the Correlation Function of Leak Noise in Buried Plastic Pipes. J. Sound Vib. 2004, 277, 133–148. [Google Scholar] [CrossRef]
- Kafle, M.D.; Fong, S.; Narasimhan, S. Active Acoustic Leak Detection and Localization in a Plastic Pipe Using Time Delay Estimation. Appl. Acoustics 2022, 187, 108482. [Google Scholar] [CrossRef]
- Xiao, R.; Joseph, P.F.; Muggleton, J.M.; Li, J. Limits for Leak Noise Detection in Gas Pipes Using Cross Correlation. J. Sound Vib. 2022, 520, 116639. [Google Scholar] [CrossRef]
- Banjara, N.K.; Sasmal, S.; Voggu, S. Machine Learning Supported Acoustic Emission Technique for Leakage Detection in Pipelines. Int. J. Press. Vessels Pip. 2020, 188, 104243. [Google Scholar] [CrossRef]
- Zhang, T.; Xu, C.; Ozevin, D. Acoustic Emission Source Localization in Complex Pipe Structure Using Multi-task Deep Learning Models. Adv. Struct. Eng. 2025, 28, 23–37. [Google Scholar] [CrossRef]
- Saleem, F.; Ahmad, Z.; Siddique, M.F.; Umar, M.; Kim, J.-M. Acoustic Emission-Based Pipeline Leak Detection and Size Identification Using a Customized One-Dimensional DenseNet. Sensors 2025, 25, 1112. [Google Scholar] [CrossRef]
- Ullah, N.; Siddique, M.F.; Ullah, S.; Ahmad, Z.; Kim, J.-M. Pipeline Leak Detection System for a Smart City: Leveraging Acoustic Emission Sensing and Sequential Deep Learning. Smart Cities 2024, 7, 2318–2338. [Google Scholar] [CrossRef]
- Kim, K.B.; Kim, B.K.; Kang, J.-G. Modeling Acoustic Emission Due to an Internal Point Source in Circular Cylindrical Structures. Appl. Sci. 2022, 12, 12032. [Google Scholar] [CrossRef]
- Morse, P.M.; Feshbach, H. Methods of Theoretical Physics; McGraw-Hill: New York, NY, USA, 1953; pp. 1764–1767. [Google Scholar]
- Kim, K.B.; Kim, J.-H.; Jin, J.-E.; Kim, H.-J.; Kim, C.-I.; Kim, B.K.; Kang, J.-G. The Characteristics of Acoustic Emissions due to Gas Leaks in Circular Cylinders: A Theoretical and Experimental Investigation. Appl. Sci. 2023, 13, 9814. [Google Scholar] [CrossRef]
- Kim, K.B.; Kim, B.K.; Lee, S.G.; Kang, J.-G. Analytical Modeling of Acoustic Emission due to an Internal Point Source in a Transversely Isotropic Cylinder. Appl. Sci. 2022, 12, 5272. [Google Scholar] [CrossRef]
- Lighthill, M.J. On Sound Generated Aerodynamics. I. General Theory. Proc. R. Soc. A 1952, 211, 564–587. [Google Scholar]
- Brennen, C.E. An Internet Book on Fluid Dynamics. 2006. Available online: http://brennen.caltech.edu/fluidbook/basicfluiddynamics/turbulence.htm (accessed on 20 August 2023).
- Eringen, A.C.; Suhubi, E.S. Fundamentals of Linear Elastodynamics. In Elastodynamics; Academic Press: New York, NY, USA, 1975; Volume II, pp. 343–366. [Google Scholar]
- Honarvar, F.; Enjilel, E.; Sinclair, A.N.; Mirnezami, S.A. Wave Propagation in Transversely Isotropic Cylinders. Int. J. Solids Struct. 2007, 44, 5236–5246. [Google Scholar] [CrossRef]
- Bomelburg, H.J. Estimation of Gas Leak Rates Through Very Small Orifices and Channels; Report for Nuclear Regulatory Commission. BNWL-2223-77; Pacific Northwest Laboratory: Richland, WA, USA, 1977. [Google Scholar]
- Levenspiel, O. Engineering Flow and Heat Exchange, 3rd ed.; Springer Science: New York, NY, USA, 2014; pp. 52–54. [Google Scholar]
- Bariha, N.; Mishra, I.M.; Srivastava, V.C. Hazard Analysis of Failure of Natural Gas and Petroleum Gas Pipelines. J. Loss Prev. Process. Ind. 2016, 40, 217–226. [Google Scholar] [CrossRef]
- Li, Y.; Zhou, P.; Zhuang, Y.; Wu, X.; Liu, Y.; Han, X.; Chen, G. An Improved Gas Leakage Model and Research on the Leakage Field Strength Characteristics of R290 in Limited Space. Appl. Sci. 2022, 12, 5657. [Google Scholar] [CrossRef]
| Steel Cylinder | |||||
|---|---|---|---|---|---|
| m, kg m−3, m s−1, | m m m s−1 | ||||
| Stiffness parameters (kg m−1 ms−2) | |||||
| c11 = 208.6 × 109, c12 = 146.5 × 109, c44 = 126.9 × 109, | c22 = 208.6 × 109, c13 = 146.5 × 109, c55 = 126.9 × 109, | c33 = 208.6 × 109, c23 = 146.5 × 109, c66 = 126.9 × 109 | |||
| Nitrogen gas (25 °C, 1 bar) * | |||||
| Molecular weight, M = 0.028 kg mol−1 Pa s m2 s−1 | kg m−3, , | ||||
| Array Position | P0 (bar) | (error%) | /mm (error%) | Reynolds NO/k Theory/Estimation | |
|---|---|---|---|---|---|
| Zone1 a | 2.07 | −6.1° (1.7) | 5.0 (0.5) | 0.05/0.05 | 5.3/5.5–5.9 |
| 3.06 | −6.1° (1.7) | 5.0 (0.5) | 0.09/0.08 | 7.2/7.0–8.4 | |
| 3.97 | −6.1° (1.7) | 5.0 (0.5) | 0.13/0.12 | 8.5/8.5–11.7 | |
| Zone2 b | 2.01 | 3.6° (1.0) | 7.0 (0.7) | 0.12/0.11 | 13.3/8.0–11.0 |
| 3.01 | 3.6° (1.0) | 7.0 (0.7) | 0.26/0.25 | 18.1/18.1–23.0 | |
| 4.01 | 3.6° (1.0) | 7.0 (0.7) | 0.40/0.38 | 21.5/28.9 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kang, J.-G.; Kim, K.B.; Koh, K.H.; Kim, B.K. Analytical Model and Gas Leak Source Localization Based on Acoustic Emission for Cylindrical Storage. Appl. Sci. 2025, 15, 5072. https://doi.org/10.3390/app15095072
Kang J-G, Kim KB, Koh KH, Kim BK. Analytical Model and Gas Leak Source Localization Based on Acoustic Emission for Cylindrical Storage. Applied Sciences. 2025; 15(9):5072. https://doi.org/10.3390/app15095072
Chicago/Turabian StyleKang, Jun-Gill, Kwang Bok Kim, Kyung Hwan Koh, and Bong Ki Kim. 2025. "Analytical Model and Gas Leak Source Localization Based on Acoustic Emission for Cylindrical Storage" Applied Sciences 15, no. 9: 5072. https://doi.org/10.3390/app15095072
APA StyleKang, J.-G., Kim, K. B., Koh, K. H., & Kim, B. K. (2025). Analytical Model and Gas Leak Source Localization Based on Acoustic Emission for Cylindrical Storage. Applied Sciences, 15(9), 5072. https://doi.org/10.3390/app15095072





