Next Article in Journal
Hybrid Fuzzy–DDPG Approach for Efficient MPPT in Partially Shaded Photovoltaic Panels
Previous Article in Journal
Gesture Classification Using a Smartwatch: Focusing on Unseen Non-Target Gestures
Previous Article in Special Issue
Neurobiomechanical Characterization of Feedforward Phase of Gait Initiation in Chronic Stroke: A Linear and Non-Linear Approach
 
 
Search for Articles:
Title / Keyword
Author / Affiliation / Email
Journal
Article Type
 
 
Advanced Search
 
Section
Special Issue
Volume
Issue
Number
Page
 
Journals
Applied Sciences
Volume 15
Issue 9
10.3390/app15094868
applsci-logo
Submit to this Journal Review for this Journal Propose a Special Issue
► Article Menu

Article Menu

share Share announcement Help format_quote Cite question_answer Discuss in SciProfiles
first_page
settings
Order Article Reprints
Font Type:
Arial Georgia Verdana
Font Size:
Aa Aa Aa
Line Spacing:
Column Width:
Background:
Article

Exploring the Relationship Between Vertical Jump and Short Sprint Performance in Female Basketball Players

by
Raúl Nieto-Acevedo
1,*,
Carlos García-Sánchez
2,*,
Pablo Abián
3,
Javier Abián-Vicén
4,
Alfredo Sánchez-Bravo
4,5 and
Javier Díaz-Lara
4
1
Facultad de Ciencias Biomédicas y de la Salud, Universidad Alfonso X el Sabio (UAX), Avenida de la Universidad, 1, 28691 Villanueva de la Cañada, Comunidad de Madrid, Spain
2
Deporte y Entrenamiento Research Group, Departamento de Deportes, Facultad de Ciencias de la Actividad Física y del Deporte, Universidad Politécnica de Madrid, 28040 Madrid, Comunidad de Madrid, Spain
3
Department of Biomedical Sciences, Faculty of Medicine and Health Sciences, University of Alcala, 28801 Alcalá de Henares, Spain
4
Performance and Sport Rehabilitation Laboratory (DEPORSALUD), Faculty of Sport Sciences, University of Castilla-La Mancha, 45071 Toledo, Spain
5
Faculty of Health Sciences, Universidad Francisco de Vitoria, Ctra. Pozuelo-Majadahonda km 1800, 28223 Pozuelo de Alarcon, Spain
*
Authors to whom correspondence should be addressed.
Appl. Sci. 2025, 15(9), 4868; https://doi.org/10.3390/app15094868
Submission received: 24 February 2025 / Revised: 15 April 2025 / Accepted: 24 April 2025 / Published: 27 April 2025
(This article belongs to the Special Issue Biomechanics and Motor Control on Human Movement Analysis: 2nd Edition)
Browse Figure
Versions Notes

Abstract

:
The aim of this study was to explore the relation between vertical jump and short sprint. A total of 14 female basketball players (n = 14; age: 20.9 ± 6.0 years; height: 177.0 ± 3.0 cm; body mass: 69.0 ± 11.1 kg) were assessed for Abalakov jump (ABKJ), countermovement jump (CMJ), and a 20 m sprint. The relationships between sprint and vertical jumps were analyzed using Pearson’s correlation coefficient and lineal regression, while discriminative parameters between backcourt and frontcourt players were assessed using independent t-tests. Higher associations were found between vertical jumps and sprint parameters, particularly CMJ height, showing a strong correlation with sprint time in 0–10 m (r = 0.805), 10–20 m (r = 0.798), and 0–20 m (r = 0.822). Jump metrics could provide a valid and reliable alternative for estimating sprint times over short distances in female basketball players. This finding could be an efficient alternative to save time in evaluations for team sports.

1. Introduction

The literature on the physical demands in team sports indicates that the most common actions involve maximal intensity development requiring high levels of strength and speed [1]. As a result, the ability to repeatedly perform sprints throughout a game is crucial for achieving high-level performance [2]. Specifically, basketball requires repeating high-intensity movements (e.g., jump, sprint, change in direction, and specific technical skills) [3]. In addition, appropriate anthropometric and body build features, precision, agility, and jumping ability are key elements of success in basketball. Given that the basketball hoop is positioned at a height of 3.05 m, jumping ability plays a crucial role in the game, influencing actions such as shooting, rebounding, and blocking [4]. Consequently, basketball players and coaches are highly focused on testing and enhancing vertical jump ability [5].
Several studies have emphasized the importance of explosive strength and neuromuscular performance in basketball [6], while others have noted how repeated sprint ability is closely tied to fatigue resistance and anaerobic capacity in the sport [7]. Recent advancements in performance analysis have also led to greater interest in using force–time metrics to quantify athlete readiness and explosiveness in jumping tasks [8].
Factors that impact sprinting performance also appear to influence other explosive actions, such as vertical jumping [9,10]. Research has shown positive correlations between jump and sprint capabilities [10]. Limited literature has evidenced that the reactive strength index (calculated using vertical jump or drop-jump metrics) explained 89.6% of average velocities in some sprinting distances [10]. There are studies that have examined the relationships between high-intensity actions and sprint times [10,11,12,13]. However, no studies have found whether there is an association among jump metrics such as peak force or maximum RFD during an Abalakov jump (ABKJ) or countermovement jump (CMJ) in female basketball players. RFD refers to the ability to develop force rapidly, which is crucial in power movements such as vertical jumps, sprints, and quick changes in direction—all of which are common in basketball [14,15,16]. Many studies analyzing the connection between vertical jumps and sprints have found strong correlations across different sprint distances, ranging from 10 to 100 m, typically involving sprinter athletes [10,11,12,13,17]. However, in basketball, sprint distances are significantly shorter due to the court’s dimensions (28 m in length and 15 m in width). Studies have shown that the mean sprint distance and duration in basketball are approximately 7.38 ± 0.86 m and 1.41 ± 0.16 s, respectively [18], highlighting the need to assess short sprints rather than the longer distances relevant to track athletes.
Other studies have highlighted differences among basketball players, showing that intermittent efforts and strength/power characteristics, such as vertical jump height, vary according to playing position [19,20]. For example, research on professional basketball players revealed that guards outperformed centers in the T-test and in 10 and 30 m sprints [21]. Also, centers had significantly lower maximal oxygen uptake (VO2max) than guards [21]. Position-specific differences have also been observed in physical performance, with guards achieving faster times in suicide runs and higher single-leg jump heights compared to centers and forwards [22]. Lastly, in one study, the authors compared different playing positions and performance levels in various modalities of jumping testing in professional male basketball players [4]. Players with high levels of competition exhibited superior jumping performance compared to those at lower levels [4]. However, the literature about the jump variables that could be affected by sprint ability in basketball players and between different players’ positions is scarce.
Based on recent research demonstrating strong associations between vertical jump metrics and sprint performance in athletes [10,11,12,13], we hypothesized that specific force–time variables derived from jump tests—particularly those related to concentric velocity and power—would exhibit significant correlations with 20 m sprint times. Accordingly, the aims of the present study were threefold, as follows: (1) to examine position-specific differences in countermovement jump (CMJ) and abbreviated bilateral jump (ABKJ) force–time characteristics in a cohort of elite female basketball players; (2) to analyze the strength of associations between vertical jump metrics (CMJ and ABKJ) and linear sprint performance over 0–10 m, 10–20 m, and 0–20 m distances; and (3) to evaluate the extent to which vertical jump variables obtained from force plate assessments can predict short sprint performance in this population.

2. Materials and Methods

2.1. Sample

A total of 14 female basketball players (total sample: n = 14; age: 20.9 ± 6.0 years; height: 177.0 ± 3.0 cm; body mass: 69.0 ± 11.1 kg) from the same team voluntarily participated in this study. Recruitment was conducted using a non-probabilistic convenience sampling method. The players were divided into two groups based on their positions, as follows: backcourt players (n = 7; age: 18.4 ± 3.8 years; height: 171.1 ± 8.3 cm; body mass: 61.9 ± 8.4 kg) and frontcourt players (n = 7; age: 23.3 ± 8.1 years; height: 182.9 ± 3.6 cm; body mass: 78.0 ± 13.7 kg). These categorizations were made following the recommendations presented in previous studies [23], with the frontcourt group consisting of forwards and centers and the backcourt group consisting of guards. The younger participants were at an advanced developmental stage. The majority of participants had substantial basketball training experience and had been training for approximately 3 h per day, 4 days per week (including a weekly competition), during the preceding year. The players competed at the third tier of competition (highly trained) according to the Participant Classification Framework described in some studies [24]. Female basketball players between the ages of 18 and 35 years who have at least 2 years of competitive basketball experience and are currently active at a competitive level (e.g., collegiate, semi-professional, or professional leagues) were eligible for participation. The participants were required to provide informed consent to be included in this study. Players with a history of severe or ongoing injuries (e.g., ACL tears, chronic joint problems), serious medical conditions (e.g., cardiovascular diseases, asthma), or pregnancy were excluded from this study to ensure their safety. Before the study began, all players were fully informed of the requirements, risks, and potential benefits associated with the investigation. Each participant provided written informed consent. For players under 18 years of age, consent was also obtained from a parent or legal guardian. All ethical procedures adhered to the Declaration of Helsinki and were approved by the Ethics Committee of Universidad Alfonso X el Sabio (9/298—25 October 2024).

2.2. Procedures

The players performed a standardized, sport-specific warm-up lasting 10 min, led by their strength and conditioning coach. Following the warm-up, the players underwent performance tests, which consisted of two repetitions of each test, as follows: CMJ, ABKJ, and a 20 m sprint (with 2 min of recovery between repetitions).

2.3. CMJ and ABKJ Testing

The players completed two jumps to their preferred depth with 20 s of rest between jumps [25,26]. Verbal instructions were provided, directing them to “jump as fast and as high as possible.” For the CMJ, the players kept their hands on their hips, while, for the ABKJ, they were allowed free arm movement. The players were also instructed to perform the countermovement (downward phase) and propulsion (upward phase) “as quickly as possible while achieving maximum jump height.” These instructions were standardized due to their known influence on the force–time characteristics of the CMJ [27,28]. To record ABKJ and CMJ metrics, a wireless dual force plate system was used, operating at a sample rate of 1000 Hz (ForceDecks FD4000 Dual Force Platforms, Vald Performance, Brisbane, QLD, Australia). The force–time metrics analyzed in this study were chosen based on prior research in basketball [29,30,31]. The variables examined during the concentric phase of the CMJ and ABKJ included concentric maximum RFD (CMRFD), calculated as the maximum force observed over the force–time curve during the jump [32,33]; concentric peak force (CPF); and concentric peak velocity (CPV). Jump height was also calculated using the “Impulse–Momentum equation,” which determines jump height based on specific impulse metrics before takeoff. Contraction time began when the athlete’s system mass was reduced by 20 N (movement onset) and ended at takeoff. Takeoff was defined as the moment when vertical force dropped below 20 N. Detailed descriptions of CMJ force–time metrics are available in the VALD user manual (https://valdperformance.com/forcedecks/; accessed on 1 June 2024) and prior research [29,30,31].

2.4. Twenty-Meter Sprint Testing

Following the jump tests, the players completed two 20 m sprints with 2 min of rest between sprints [20]. Sprint performance was assessed using three pairs of photoelectric cells (Polifemo Light Radio, Microgate, Bolzano, Italy) placed at 0, 10, and 20 m. The players were given technical instructions to ensure proper execution of the sprints. Each sprint began from a stable standing position, with no body movement or rocking allowed prior to takeoff. The players started 0.5 m before the first timing gate and initiated the sprint at their own discretion. Verbal cues were provided to signal the start of each repetition, and technical staff offered oral feedback to encourage maximum effort during each trial. The best performance score was used for analysis [34].

2.5. Statistical Analysis

Descriptive statistics were presented as mean ± standard deviation (SD). Normality of data distribution was confirmed through visual inspection of histograms and the Shapiro–Wilk test. All data followed a normal distribution (p > 0.05). Pearson’s correlation coefficient was used to evaluate the strength of relationships, interpreted using the following scale: <0.10 = trivial; 0.10–0.29 = small; 0.30–0.49 = moderate; 0.50–0.69 = large; 0.70–0.89 = very large; 0.90–0.99 = nearly perfect; and 1.00 = perfect [35]. Stepwise multiple regression analysis was performed to assess the predictive capacity of jump metrics for sprint performance. Independent t-tests were conducted to identify differences between backcourt and frontcourt players. Hedges’ g ES were calculated and interpreted following previous guidelines, as follows: trivial (0.20), small (0.20–0.59), moderate (0.60–1.19), large (1.20–2.00), very large (2.00–4.00), and extremely large (>4.00). A post hoc power analysis was carried out using free software (GPower v3.1). Given this study’s sample size (N = 14) and an alpha error of 0.05, power calculations were conducted for Pearson’s correlation, independent t-tests, and multiple regression using conventional effect size benchmarks. The mean and standard deviation of CMJ height and sprint time assessments demonstrated a Pearson correlation coefficient of 0.75 between measurements. The resulting statistical power was 0.84. Data analysis was conducted using JAMOVI (version 2.3.21.0, 2022) for Macintosh.

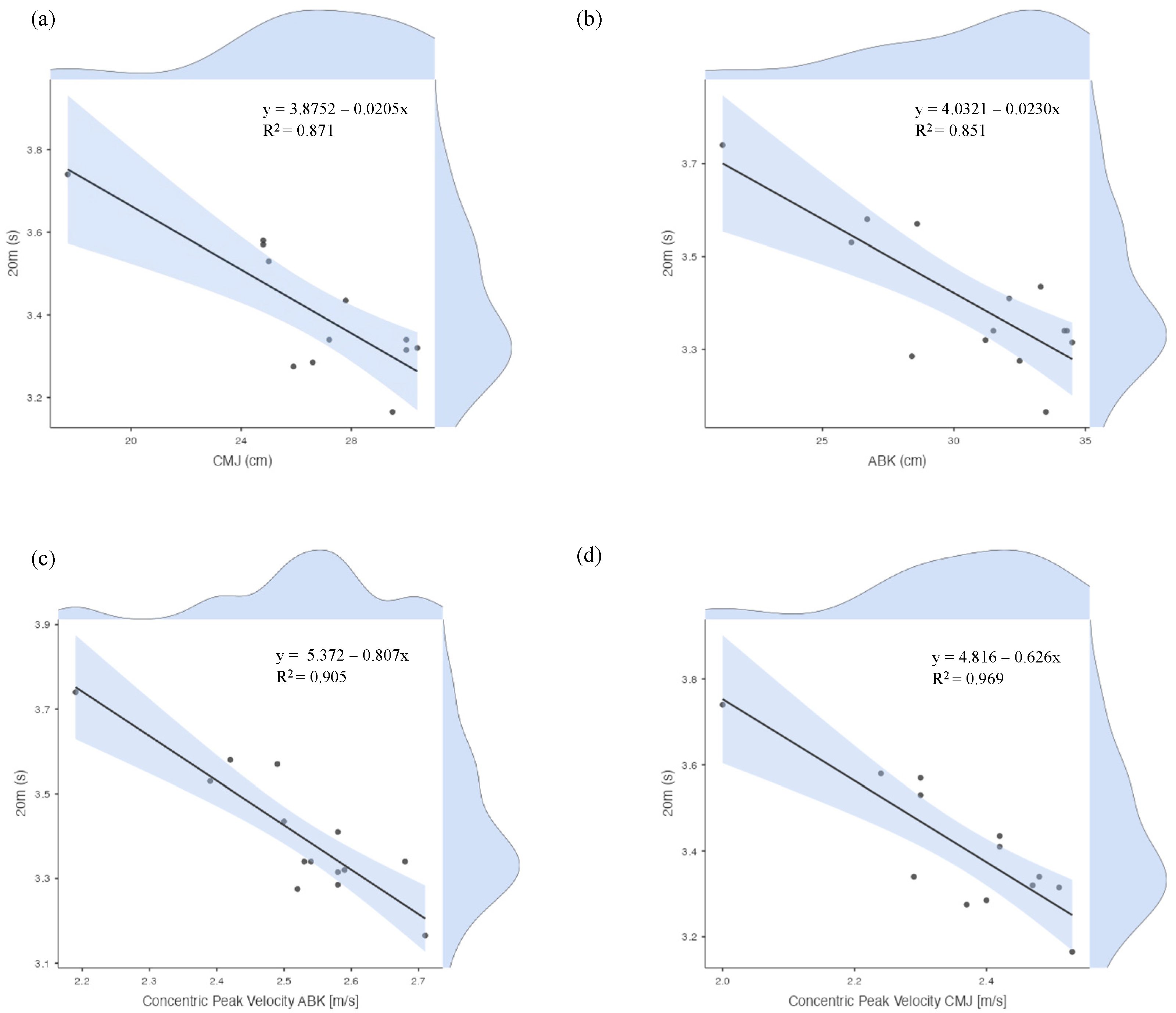
3. Results

Since the correlational analysis demonstrated similar outcomes for backcourt and frontcourt players, they are presented together to facilitate visualization and interpretation (see Table 1, Table 2 and Table 3). The descriptive values for the sprint times and jump biomechanical parameters according to playing positions are presented in Table 1. Frontcourt players show significantly higher body mass than backcourt players (61.9 ± 8.4 vs. 78.0 ± 13.7 kg; p < 0.005). Moreover, significant differences were observed in sprint distances, CMJ height, and concentric peak velocity in CMJ between backcourt and frontcourt players in favor of backcourt players (p < 0.005).
Pearson’s correlation coefficients of the relationship between 20 m sprinting time and vertical jump height in CMJ and ABKJ are presented in Table 2. The correlation between 20 m sprinting time and the vertical jump biomechanical outputs (CMRFD, CPF, and CPV) in the CMJ and ABKJ exercises are shown in Table 3. Significant negative and very large correlations were found between CMJ and ABKJ variables and sprint times in the different distances analyzed (Table 2).
Furthermore, a statistically significant moderate to very large negative correlation (p < 0.01) was detected between CMRFD ABKJ, CPV ABKJ, CPV CMJ, and the time registered in the 0–10 m, 10–20 m, and 20 m sprint test (r range = 0.735–0.853). A significant, very large correlation (p < 0.001) was also detected between CPV CMJ and CPV ABKJ with CMJ height (r = 0.888–0.998) and ABKJ height (r = 0.847–0.895) (Table 3).
The simple linear regressions showed that 20 m sprint time may be predicated by CMJ height (R2 = 0.871; p = 0.022) (Figure 1a), ABKJ height (R2 = 0.851; p < 0.001) (Figure 1b), CPV CMJ (R2 = 0.905; p < 0.001) (Figure 1c), and CPV ABKJ (R2 = 0. 969; p < 0.001) (Figure 1d).

4. Discussion

Our aims in the present study were as follows: (1) to analyze vertical jump force–time metrics according to playing position within a cohort of female basketball players; (2) to identify potential vertical jump metrics associated with a 20 m sprint; and (3) to determine the predictive capacity of vertical jump metrics from force plate system metrics. The main findings of this investigation are as follows: Significant differences were observed between backcourt and frontcourt players in 0–10, 10–20, and 20 m sprints, CMJ height, and concentric peak velocity. Vertical jump test outcomes are largely associated with actual 20 m sprint performance in a sample of female basketball players. Moreover, using jump parameters appears to predict sprint performances with high accuracy.
Higher values were observed in the backcourt for 0–10, 10–20, and 20 m sprint distances, CMJ height, and concentric peak velocity CMJ compared with the frontcourt players (Table 1). Previous research reports that performance on most tests (e.g., VO2max, 10 and 30 m sprint, peak and mean power during the Wingate anaerobic test) tends to favor guards [4,28,29]. While Cabarkapa et al. (2023) [15] found that mean and peak power were significantly higher for guards compared to centers, we observed differences between playing positions, specifically in concentric peak velocity, contrary to their findings. Anthropometric differences—such as greater body mass and limb length in frontcourt players—may partly explain their lower jump and sprint performance. Prior research by Bae (2022) [36] and others [37] has shown that these physical characteristics are less conducive to rapid acceleration and vertical impulse production. This aligns with our findings showing that backcourt players, who typically have lower body mass, exhibit significantly higher CMJ heights and faster sprint times. It appears that the anthropometrical differences between basketball positions are related to playing position. For example, some studies found that centers cover significantly lower total distance compared to guards [38,39]. One potential reason for guards attaining notably higher maximal speeds and covering greater distances at high speed is that they typically traverse more meters during offensive and defensive transitions from basket to basket, thereby allowing them more opportunities to reach elevated velocities [40].
Importantly, this study identified strong correlations between vertical jump metrics (e.g., CMJ and ABKJ height, concentric peak velocity) and sprint performance across all distances (0–10, 10–20, and 20 m), with especially high values for concentric peak velocity (e.g., r = 0.885 for CPV in ABKJ vs. 20 m sprint time). However, we acknowledge that high correlation values do not imply causation, and there was no intervention or longitudinal design to confirm predictive validity. While our regression models (Figure 1) showed high coefficients of determination (e.g., R2 = 0.969), we recognize that such values may reflect overfitting, particularly considering the limited sample size. No cross-validation or adjusted R2 was applied, which limits the generalizability of the models. Future research should include validation datasets or use resampling methods such as k-fold cross-validation to assess model robustness. Our data are similar to those obtained by other authors who found that jump height has a positive correlation with average sprint time (0.51 ≤ r ≤ 0.83), indicating that the capacity to generate an impulse and accelerate the body in a horizontal plate is positively associated with similar capabilities in vertical directions [41]. In addition, [11] detected similar results, finding higher associations between vertical jumps and sprint distances greater than 10 m, especially for squat jump height, relative force peak, and power peak. A hypothetical justification for this is that vertically oriented exercises (e.g., vertical jumps) have greater transferability to top-speed phases, likely due to the enhanced influence of vertical peak forces at higher running velocities [10]. Moreover, concentric maximum RFD in ABKJ has shown large correlations with 10 and 20 m, which could show that RFD is a crucial factor in basketball performance, particularly in movements requiring rapid force production, such as jumping and sprinting. A higher RFD allows athletes to generate explosive power in a shorter time frame, which is essential for actions like rebounding, shot-blocking, and dunking [42]. Studies have shown that elite basketball players tend to exhibit greater RFD values, which contribute to higher jump performance and overall explosiveness on the court [43]. Moreover, RFD is associated with neuromuscular efficiency, indicating that well-trained athletes can rapidly recruit motor units to generate force more effectively [44].
The regression model (Figure 1) shows that some vertical jump metrics (height or concentric peak velocity) could consistently predict sprint performance for all sprint distances. Studies performed with young male elite sprinters showed that linear regression analysis between squat jump height and relative squat strength explained 75% of the variance in the 60 m sprint. Furthermore, previous studies found that jump height during squat jump and CMJ, in addition to the drop-jump height and reactive strength index, explained 63% (r = 0.840) of velocities for different sprint phases [45]. The height achieved during a CMJ or ABKJ is a measure that is able to express a mechanical value already corrected by the subjects’ body mass [46]. Consequently, we found significant differences between backcourts and frontcourts in body mass. Backcourts showed lower values in all sprint distances and higher values in CMJ height. The differences in body mass probably explain these differences between positions. In addition to body composition differences, the physiological factors that may affect RFD during explosive tasks include the intrinsic contractile properties of muscle, the stiffness of the muscle–tendon unit, and the neural drive to the involved musculature. The intrinsic contractile properties can be assessed by measuring RFD in response to evoked contractions and may reflect differences in morphology (e.g., fiber type distribution) [47]. In our sample, concentric peak velocity and rate of force development (RFD) showed particularly strong correlations with sprint times. This is consistent with the findings of Ronda et al. (2020) [48], who emphasized that RFD plays a critical role in basketball-specific actions, such as rebounding, cutting, and sprinting. A higher RFD enables athletes to generate explosive force more quickly, facilitating effective high-velocity movements. Moreover, neuromuscular efficiency—defined by the rapid recruitment of motor units—is likely to underlie both vertical and horizontal acceleration capacities [49].
While we reported differences in body mass between positions, anthropometric variables were not included as covariates in our regression models. Recent studies [50,51,52] propose alternative models that consider the complexity of neuromuscular performance, incorporating contextual variables such as fatigue, hormonal fluctuations (e.g., menstrual cycle), and intra-individual variability, which may affect jump and sprint outputs. These variables, while acknowledged, were not statistically controlled in our analysis and represent a limitation.

5. Practical Applications

This type of descriptive information can be highly valuable for sports scientists and strength and conditioning practitioners engaged in similar physical performance testing with their athletes or teams.
The incorporation of ABKJ and CMJ parameters, such as concentric peak velocity, offers deeper insights into factors that influence sprint performance. It assists coaches and sports scientists in selecting key metrics for testing and monitoring.
Consequently, measuring vertical jumps (CMJ and ABKJ) may be beneficial in training and testing protocols due to their safety and strong correlation with 20 m sprint performance in female basketball players.

Limitations and Directions for Further Research

The current study features both strengths and limitations that must be considered when interpreting the findings. One of the key strengths of this study is that it focuses on basketball players, a specific cohort that can provide normative values to help athletes and coaches to improve sprint performance in a sport where speed and explosive power are critical. These values can be particularly useful for guiding training regimens tailored to the needs of basketball players. However, it is important to note that correlation does not imply causation. While improvements in vertical jump or maximal strength may be associated with enhanced sprint performance, it cannot be assumed that such gains directly lead to improvements in sprinting speed. Further studies are needed to explore the causal relationships between these variables. Although the regression models revealed strong predictive values (e.g., R2 > 0.85), these results may overestimate the true predictive capacity due to potential model overfitting, particularly given the small sample size.
However, there are several limitations to this study. First, the relatively small sample size is a significant constraint. Small sample sizes can lead to the overestimation of effect sizes, making it more difficult to generalize the results for a larger population. This also affects the replicability of the findings, as results based on a small number of participants may not hold true in different settings or with different cohorts. Although the sample represents a high level of competition in female basketball, access to elite players is inherently challenging, which made recruiting a larger sample difficult.
Another limitation is the anthropometric variation within the cohort. Basketball players, even within the same team, exhibit considerable diversity in terms of height, weight, body composition, and other physical attributes. These differences may influence the interpretation of sprinting and explosive strength data, as physical characteristics such as leg length, muscle mass, and overall body composition can affect performance outcomes. Thus, while the findings provide valuable insights, they may not be fully applicable to all basketball players, especially those at different levels of competition or with varying anthropometric profiles.
Finally, the absence of a change-of-direction (COD) test is a notable limitation. In basketball, athletes frequently engage in rapid directional changes, and sprint performance alone does not fully capture the demands of the sport. A COD test would have provided additional insight into the agility and explosive movements critical for basketball performance, offering a more comprehensive understanding of how physical characteristics translate to on-court success. Future studies could incorporate this type of test to better assess the multidimensional aspects of athletic performance in basketball.

6. Conclusions

This study demonstrated the influence of position-specific differences on jump height in a countermovement jump among elite female basketball players, with results favoring backcourt players. Importantly, our findings revealed very strong negative correlations between short sprint distances (0–10 m, 10–20 m, and 0–20 m) and key vertical jump metrics, particularly jump height and concentric peak velocity (CPV), in both CMJ and ABKJ exercises. One of the original contributions of this study lies in identifying CPV—especially from the ABKJ—as a highly accurate predictor of sprint performance (R2 = 0.969), which, to our knowledge, has not been previously reported in elite female basketball players. Furthermore, this is the first study to demonstrate that vertical jump force–time characteristics (such as CPV and RFD) are similarly correlated with sprint performance across both backcourt and frontcourt positions, suggesting that these metrics are robust indicators of explosive performance, regardless of playing role.
Thus, 20 m sprint performance may be reliably predicted by CMJ and ABKJ jump height and CPV. Our results have practical applications in team sports such as basketball, offering a time-efficient and cost-effective approach to assess sprint ability using simple vertical jump tests on a force plate, without the need for track-based sprint measurements.

Author Contributions

Conceptualization, methodology, and validation, R.N.-A., C.G.-S., A.S.-B., J.A.-V., P.A. and J.D.-L.; formal analysis, R.N.-A. and J.D.-L.; investigation, data curation, writing—original draft preparation, writing—review and editing, and supervision, R.N.-A. and J.D.-L. All authors have read and agreed to the published version of the manuscript.

Funding

This research received no external funding.

Institutional Review Board Statement

This study was conducted in accordance with the Declaration of Helsinki and approved by the Institutional Review Board of UNIVERSIDAD ALFONSO X EL SABIO (2024_9/298, 25 October 2024).

Informed Consent Statement

Informed consent was obtained from all subjects involved in this study.

Data Availability Statement

The datasets used and analyzed during the current study are available from the corresponding author upon reasonable request.

Acknowledgments

The authors thank the Club Baloncesto Ciudad de Móstoles (Madrid) for their invaluable contribution to this study.

Conflicts of Interest

The authors declare no conflicts of interest.

References

  1. Harper, D.J.; Carling, C.; Kiely, J. High-Intensity Acceleration and Deceleration Demands in Elite Team Sports Competitive Match Play: A Systematic Review and Meta-Analysis of Observational Studies. Sports Med. 2019, 49, 1923–1947. [Google Scholar] [CrossRef] [PubMed]
  2. Taylor, J.B.; Wright, A.A.; Dischiavi, S.L.; Townsend, M.A.; Marmon, A.R. Activity Demands During Multi-Directional Team Sports: A Systematic Review. Sports Med. 2017, 47, 2533–2551. [Google Scholar] [CrossRef] [PubMed]
  3. Stojanović, E.; Stojiljković, N.; Scanlan, A.T.; Dalbo, V.J.; Berkelmans, D.M.; Milanović, Z. The Activity Demands and Physiological Responses Encountered During Basketball Match-Play: A Systematic Review. Sports Med. 2018, 48, 111–135. [Google Scholar] [CrossRef] [PubMed]
  4. Pehar, M.; Sekulic, D.; Sisic, N.; Spasic, M.; Uljevic, O.; Krolo, A.; Milanovic, Z.; Sattler, T. Evaluation of Different Jumping Tests in Defining Position-Specific and Performance-Level Differences in High Level Basketball Players. Biol. Sport. 2017, 34, 263. [Google Scholar] [CrossRef]
  5. Ziv, G.; Lidor, R. Vertical Jump in Female and Male Basketball Players—A Review of Observational and Experimental Studies. J. Sci. Med. Sport 2010, 13, 332–339. [Google Scholar] [CrossRef]
  6. Akbar, S.; Geok Soh, K.; Jazaily Mohd Nasiruddin, N.; Bashir, M.; Cao, S.; Lam Soh, K.; Cristóbal Andrade, D.; Miguel Henriques-Neto, D.; Hammami, R.; Mohd Nasiruddin, J.N. Effects of Neuromuscular Training on Athletes Physical Fitness in Sports: A Systematic Review. Front. Physiol. 2022, 13, 939042. [Google Scholar] [CrossRef]
  7. Attene, G.; Laffaye, G.; Chaouachi, A.; Pizzolato, F.; Migliaccio, G.M.; Padulo, J. Repeated Sprint Ability in Young Basketball Players: One vs. Two Changes of Direction (Part 2). J. Sports Sci. 2015, 33, 1553–1563. [Google Scholar] [CrossRef]
  8. Sole, C.J.; Mizuguchi, S.; Sato, K.; Moir, G.L.; Stone, M.H. Phase Characteristics of the Countermovement Jump Force-Time Curve: A Comparison of Athletes by Jumping Ability. J. Strength Cond. Res. 2018, 32, 1155–1165. [Google Scholar] [CrossRef]
  9. Comfort, P.; Bullock, N.; Pearson, S.J. A Comparison of Maximal Squat Strength and 5-, 10-, and 20-Meter Sprint Times, in Athletes and Recreationally Trained Men. J. Strength Cond. Res. 2012, 26, 937–940. [Google Scholar] [CrossRef]
  10. Loturco, I.; Kobal, R.; Kitamura, K.; Fernandes, V.; Moura, N.; Siqueira, F.; Cal Abad, C.C.; Pereira, L.A. Predictive Factors of Elite Sprint Performance: Influences of Muscle Mechanical Properties and Functional Parameters. J. Strength Cond. Res. 2019, 33, 974–986. [Google Scholar] [CrossRef]
  11. Washif, J.A.; Kok, L.Y. Relationships Between Vertical Jump Metrics and Sprint Performance, and Qualities That Distinguish Between Faster and Slower Sprinters. J. Sci. Sport. Exerc. 2022, 4, 135–144. [Google Scholar] [CrossRef]
  12. McErlain-Naylor, S.A.; Beato, M. Factors Influencing the Jump Momentum—Sprint Momentum Correlation: A Data Simulation. Eur. J. Sport. Sci. 2022, 22, 1847–1855. [Google Scholar] [CrossRef] [PubMed]
  13. Loturco, I.; Pereira, L.A.; Cal Abad, C.C.; D’Angelo, R.A.; Fernandes, V.; Kitamura, K.; Kobal, R.; Nakamura, F.Y. Vertical and Horizontal Jump Tests Are Strongly Associated With Competitive Performance in 100-m Dash Events. J. Strength Cond. Res. 2015, 29, 1966–1971. [Google Scholar] [CrossRef] [PubMed]
  14. Cabarkapa, D.; Johnson, Q.R.; Cabarkapa, D.V.; Philipp, N.M.; Eserhaut, D.A.; Fry, A.C. Changes in Countermovement Vertical Jump Force-Time Metrics During a Game in Professional Male Basketball Players. J. Strength Cond. Res. 2024, 38, 1326–1329. [Google Scholar] [CrossRef]
  15. Cabarkapa, D.; Philipp, N.M.; Cabarkapa, D.V.; Fry, A.C. Position-Specific Differences in Countermovement Vertical Jump Force-Time Metrics in Professional Male Basketball Players. Front. Sports Act. Living 2023, 5, 1218234. [Google Scholar] [CrossRef]
  16. Rice, P.E.; Goodman, C.L.; Capps, C.R.; Triplett, N.T.; Erickson, T.M.; McBride, J.M. Force– and Power–Time Curve Comparison during Jumping between Strength-Matched Male and Female Basketball Players. Eur. J. Sport. Sci. 2017, 17, 286–293. [Google Scholar] [CrossRef]
  17. Diker, G.; Struzik, A.; Ön, S.; Zileli, R. The Relationship between the Hamstring-to-Quadriceps Ratio and Jumping and Sprinting Abilities of Young Male Soccer Players. Int. J. Environ. Res. Public Health 2022, 19, 7471. [Google Scholar] [CrossRef]
  18. Torres-Ronda, L.; Beanland, E.; Whitehead, S.; Sweeting, A.; Clubb, J. Tracking Systems in Team Sports: A Narrative Review of Applications of the Data and Sport Specific Analysis. Sports Med. Open 2022, 8, 15. [Google Scholar] [CrossRef]
  19. Yáñez-García, J.M.; Rodríguez-Rosell, D.; Mora-Custodio, R.; González-Badillo, J.J. Changes in Muscle Strength, Jump, and Sprint Performance in Young Elite Basketball Players: The Impact of Combined High-Speed Resistance Training and Plyometrics. J. Strength Cond. Res. 2022, 36, 478–485. [Google Scholar] [CrossRef]
  20. Figueira, B.; Goncąlves, B.; Abade, E.; Paulauskas, R.; Masiulis, N.; Kamarauskas, P.; Sampaio, J. Repeated Sprint Ability in Elite Basketball Players: The Effects of 10 × 30 m Vs. 20 × 15 m Exercise Protocols on Physiological Variables and Sprint Performance. J. Hum. Kinet. 2021, 77, 181. [Google Scholar] [CrossRef]
  21. Köklü, Y.; Alemdaroǧlu, U.; Koçak, F.Ü.; Erol, A.E.; Findikoǧlu, G. Comparison of Chosen Physical Fitness Characteristics of Turkish Professional Basketball Players by Division and Playing Position. J. Hum. Kinet. 2011, 30, 99. [Google Scholar] [CrossRef] [PubMed]
  22. Delextrat, A.; Cohen, D. Strength, Power, Speed, and Agility of Women Basketball Players According to Playing Position. J. Strength Cond. Res. 2009, 23, 1974–1981. [Google Scholar] [CrossRef] [PubMed]
  23. Russell, J.L.; McLean, B.D.; Impellizzeri, F.M.; Strack, D.S.; Coutts, A.J. Measuring Physical Demands in Basketball: An Explorative Systematic Review of Practices. Sports Med. 2021, 51, 81–112. [Google Scholar] [CrossRef] [PubMed]
  24. McKay, A.K.A.; Stellingwerff, T.; Smith, E.S.; Martin, D.T.; Mujika, I.; Goosey-Tolfrey, V.L.; Sheppard, J.; Burke, L.M. Defining Training and Performance Caliber: A Participant Classification Framework. Int. J. Sports Physiol. Perform. 2021, 17, 317–331. [Google Scholar] [CrossRef]
  25. Pérez-Castilla, A.; Ramirez-Campillo, R.; Fernandes, J.F.T.; García-Ramos, A. Feasibility of the 2-Point Method to Determine the Load−velocity Relationship Variables during the Countermovement Jump Exercise. J. Sport Health Sci. 2021, 12, 544–552. [Google Scholar] [CrossRef]
  26. Weakley, J.; Morrison, M.; García Ramos, A.; Johnston, R.; James, L.; Cole, M. The Validity and Reliability of Commercially Available Resistance Training Monitoring Devices: A Systematic Review. Sports Med. 2021, 51, 443–502. [Google Scholar] [CrossRef]
  27. Berberet, D.; Petway, A.; Bell, K.; Gillen, Z.; Mundy, P.; Barrera, H.; Kabo, J.; Walker, D.; Medenwald, G.; Welsh, B.; et al. Unlocking Basketball Athletic Performance: Force Plate-Derived Countermovement Jump Normative Reference Values From Seven NCAA Division-I Power Five Men’s College Basketball Teams. Int. J. Strength Cond. 2024, 4. [Google Scholar] [CrossRef]
  28. Pérez-Castilla, A.; García-Ramos, A.; Janicijevic, D.; Delgado-García, G.; de la Cruz, J.C.; Rojas, F.J.; Cepero, M. Between-Session Reliability of Performance and Asymmetry Variables Obtained during Unilateral and Bilateral Countermovement Jumps in Basketball Players. PLoS ONE 2021, 16, e0255458. [Google Scholar] [CrossRef]
  29. Anicic, Z.; Janicijevic, D.; Knezevic, O.M.; Garcia-Ramos, A.; Petrovic, M.R.; Cabarkapa, D.; Mirkov, D.M. Assessment of Countermovement Jump: What Should We Report? Life 2023, 13, 190. [Google Scholar] [CrossRef]
  30. Heishman, A.D.; Daub, B.D.; Miller, R.M.; Freitas, E.D.S.; Frantz, B.A.; Bemben, M.G. Countermovement Jump Reliability Performed With and Without an Arm Swing in NCAA Division 1 Intercollegiate Basketball Players. J. Strength Cond. Res. 2020, 34, 546–558. [Google Scholar] [CrossRef]
  31. Philipp, N.; Cabarkapa, D.; Eserhaut, D.; Cabarkapa, D.; Fry, A. Countermovement Jump Force-Time Metrics and Maximal Horizontal Deceleration Performance. in Professional Male Basketball Players. J. Appl. Sports Sci. 2022, 2, 11–27. [Google Scholar] [CrossRef]
  32. Haff, G.G.; Carlock, J.M.; Hartman, M.J.; Kilgore, J.L.; Kawamori, N.; Jackson, J.R.; Morris, R.T.; Sands, W.A.; Stone, M.H. Force-Time Curve Characteristics of Dynamic and Isometric Muscle Actions of Elite Women Olympic Weightlifters. J. Strength Cond. Res. 2005, 19, 741–748. [Google Scholar] [CrossRef] [PubMed]
  33. Mclellan, C.P.; Lovell, D.I.; Gass, G.C. The Role of Rate of Force Development on Vertical Jump Performance. J. Strength Cond. Res. 2011, 25, 379–385. [Google Scholar] [CrossRef]
  34. Brini, S.; Boullosa, D.; Calleja-González, J.; Delextrat, A. Construct Validity and Reliability of a New Basketball Multidirectional Reactive Repeated Sprint Test. Int. J. Env. Res. Public Health 2021, 18, 10695. [Google Scholar] [CrossRef]
  35. Schober, P.; Schwarte, L.A. Correlation Coefficients: Appropriate Use and Interpretation. Anesth. Analg. 2018, 126, 1763–1768. [Google Scholar] [CrossRef]
  36. Bae, J.Y. Positional Differences in Physique, Physical Strength, and Lower Extremity Stability in Korean Male Elite High School Basketball Athletes. Int. J. Env. Res. Public Health 2022, 19, 3416. [Google Scholar] [CrossRef]
  37. Ishida, A.; Travis, S.K.; Stone, M.H. Associations of Body Composition, Maximum Strength, Power Characteristics with Sprinting, Jumping, and Intermittent Endurance Performance in Male Intercollegiate Soccer Players. J. Funct. Morphol. Kinesiol. 2021, 6, 7. [Google Scholar] [CrossRef]
  38. García, F.; Vázquez-Guerrero, J.; Castellano, J.; Casals, M.M.; Schelling, X.; Garcia, F.; Vazquez-Guerrero, J.; Castellano, J.; Casals, M.M.; Schelling, X. Differences in Physical Demands between Game Quarters and Playing Positions on Professional Basketball Players during Official Competition. J. Sports Sci. Med. 2020, 19, 256–263. [Google Scholar]
  39. Vázquez-Guerrero, J.; Suarez-Arrones, L.; Gómez, D.C.; Rodas, G. Comparing External Total Load, Acceleration and Deceleration Outputs in Elite Basketball Players across Positions during Match Play. Kinesiology 2018, 50, 228–234. [Google Scholar] [CrossRef]
  40. Puente, C.; Abian-Vicen, J.; Salinero, J.J.; Lara, B.; Areces, F.; Del Coso, J. Caffeine Improves Basketball Performance in Experienced Basketball Players. Nutrients 2017, 9, 1033. [Google Scholar] [CrossRef]
  41. Cronin, J.B.; Hansen, K.T. Strength and Power Predictors of Sports Speed. J. Strength Cond. Res. 2005, 19, 349–357. [Google Scholar] [CrossRef] [PubMed]
  42. Suchomel, T.J.; Nimphius, S.; Stone, M.H. The Importance of Muscular Strength in Athletic Performance. Sports Med. 2016, 46, 1419–1449. [Google Scholar] [CrossRef] [PubMed]
  43. Laffaye, G.; Wagner, P.P.; Tombleson, T.I.L. Countermovement Jump Height: Gender and Sport-Specific Differences in the Force-Time Variables. J. Strength Cond. Res. 2014, 28, 1096–1105. [Google Scholar] [CrossRef] [PubMed]
  44. Rodacki, A.L.F.; Fowler, N.E.; Bennett, S.J. Vertical Jump Coordination: Fatigue Effects. Med. Sci. Sports Exerc. 2002, 34, 105–116. [Google Scholar] [CrossRef]
  45. Smirniotou, A.; Katsikas, C.; Paradisis, G.; Argeitaki, P.; Zacharogiannis, E.; Tziortzis, S. Strength-Power Parameters as Predictors of Sprinting Performance. J. Sports Med. Phys. Fit. 2008, 48, 447–454. [Google Scholar]
  46. Lockie, R.G.; Murphy, A.J.; Knight, T.J.; De Jonge, X.A.K.J. Factors That Differentiate Acceleration Ability in Field Sport Athletes. J. Strength Cond. Res. 2011, 25, 2704–2714. [Google Scholar] [CrossRef]
  47. Hannah, R.; Minshull, C.; Buckthorpe, M.W.; Folland, J.P. Explosive Neuromuscular Performance of Males versus Females. Exp. Physiol. 2012, 97, 618–629. [Google Scholar] [CrossRef]
  48. Ronda, L.T.; Cuzzolin, F. Strength Training for Basketball: A Methodological Framework Based on Basketball and Player’s Needs. In Basketball Sports Medicine and Science; Springer: Berlin/Heidelberg, Germany, 2020; pp. 779–789. [Google Scholar] [CrossRef]
  49. Baena-Raya, A.; Jimenez-Reyes, P.; Romea, E.; Soriano-Maldonado, A.; Rodríguez-Pérez, M.A. Gender-Specific Association of the Sprint Mechanical Properties With Change of Direction Performance in Basketball. J. Strength Cond. Res. 2021, 36, 2868–2874. [Google Scholar] [CrossRef]
  50. Spiteri, T.; Newton, R.U.; Binetti, M.; Hart, N.H.; Sheppard, J.M.; Nimphius, S. Mechanical Determinants of Faster Change of Direction and Agility Performance in Female Basketball Athletes. J. Strength Cond. Res. 2015, 29, 2205–2214. [Google Scholar] [CrossRef]
  51. Čaušević, D.; Abazović, E.; Mašić, S.; Hodžić, A.; Ormanović, Š.; Doder, I.; Čović, N.; Lakota, R. Agility, Sprint and Vertical Jump Performance Relationship in Young Basketball Players. Acta Kinesiol. 2021, 1, 133–137. [Google Scholar] [CrossRef]
  52. Čaušević, D.; Čović, N.; Abazović, E.; Rani, B.; Manolache, G.M.; Ciocan, C.V.; Zaharia, G.; Alexe, D.I. Predictors of Speed and Agility in Youth Male Basketball Players. Appl. Sci. 2023, 13, 7796. [Google Scholar] [CrossRef]
Applsci 15 04868 g001
Figure 1. Scatterplots with a linear regression analysis of 20 m sprint time and vertical jump metrics according to playing positions: 20 m and CMJ (a), 20 m and ABKJ (b), 20 m and CPV ABKJ (c), and 20 m and CPV CMJ (d).
Figure 1. Scatterplots with a linear regression analysis of 20 m sprint time and vertical jump metrics according to playing positions: 20 m and CMJ (a), 20 m and ABKJ (b), 20 m and CPV ABKJ (c), and 20 m and CPV CMJ (d).
Applsci 15 04868 g001
Table 1. Definitions of biomechanical parameters examined in the present study. Mean (M) and standard deviation (SD).
Table 1. Definitions of biomechanical parameters examined in the present study. Mean (M) and standard deviation (SD).
Variable (Unit)BackcourtFrontcourtES
(Hedges’ g)		All Players
MSDMSDMSD
0 m–10 m time (s)1.890.04 **2.020.09−1.771.960.09
10 m–20 m time (s)1.400.03 **1.500.06−2.191.450.07
20 m time (s)3.290.06 **3.520.13−2.163.400.15
Abalakov Jump Height (cm)32.262.1228.904.671.6130.583.90
Concentric Maximum RFD (N/s)6809.60346.954946.50156.370.935793.36264.28
Concentric Peak Force (N)1739.43199.733162.29314.510.722450.86226.47
Concentric Peak Velocity (m/s)2.580.062.460.16−0.432.520.13
CMJ Height (cm)28.511.88 *24.023.760.9626.643.52
Concentric Maximum RFD (N/s)3396.50164.322726.33191.870.383109.29164.49
Concentric Peak Force (N)1907.43769.182148.17444.44−0.382018.54627.48
Concentric Peak Velocity (m/s)2.440.09 *2.280.151.272.360.14
Note: CMJ: countermovement jump; ES = effect size, * p < 0.05, ** p < 0.01, significant differences between playing position.
Table 2. Correlation between sprinting time and vertical jump height.
Table 2. Correlation between sprinting time and vertical jump height.
0 m–10 m10 m–20 m20 mCMJ
0 m–10 m
10 m–20 m0.809 ***
20 m0.964 ***0.936 ***
CMJ−0.805 **−0.798 **−0.822 **
Abalakov−0.789 ***−0.743 **−0.808 ***0.898 ***
Note: CMJ: countermovement jump; * p < 0.05, ** p < 0.01, *** p < 0.001.
Table 3. Correlation between sprinting time, vertical jump height, concentric maximum RFD (CMRFD), concentric peak force (CPP), and concentric peak velocity (CPV).
Table 3. Correlation between sprinting time, vertical jump height, concentric maximum RFD (CMRFD), concentric peak force (CPP), and concentric peak velocity (CPV).
0 m–10 m10 m–20 m20 mCMJABKJ
CMRFD ABKJ−0.735 **−0.654 *−0.736 **0.5350.558
CPF ABKJ0.3750.2040.317−0.181−0.149
CPV ABKJ−0.853 ***−0.828 ***−0.885 **0.888 ***0.847 ***
CMRFD CMJ−0.584−0.358−0.4950.3850.403
CPF CMJ−0.237−0.093−0.184−0.0250.050
CPV CMJ−0.830 ***−0.793 **−0.854 ***0.958 ***0.895 ***
Note: CMJ: countermovement jump; ABKJ: Abalakov jump; * p < 0.05, ** p < 0.01, *** p < 0.001.
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.

Share and Cite

MDPI and ACS Style

Nieto-Acevedo, R.; García-Sánchez, C.; Abián, P.; Abián-Vicén, J.; Sánchez-Bravo, A.; Díaz-Lara, J. Exploring the Relationship Between Vertical Jump and Short Sprint Performance in Female Basketball Players. Appl. Sci. 2025, 15, 4868. https://doi.org/10.3390/app15094868

AMA Style

Nieto-Acevedo R, García-Sánchez C, Abián P, Abián-Vicén J, Sánchez-Bravo A, Díaz-Lara J. Exploring the Relationship Between Vertical Jump and Short Sprint Performance in Female Basketball Players. Applied Sciences. 2025; 15(9):4868. https://doi.org/10.3390/app15094868

Chicago/Turabian Style

Nieto-Acevedo, Raúl, Carlos García-Sánchez, Pablo Abián, Javier Abián-Vicén, Alfredo Sánchez-Bravo, and Javier Díaz-Lara. 2025. "Exploring the Relationship Between Vertical Jump and Short Sprint Performance in Female Basketball Players" Applied Sciences 15, no. 9: 4868. https://doi.org/10.3390/app15094868

APA Style

Nieto-Acevedo, R., García-Sánchez, C., Abián, P., Abián-Vicén, J., Sánchez-Bravo, A., & Díaz-Lara, J. (2025). Exploring the Relationship Between Vertical Jump and Short Sprint Performance in Female Basketball Players. Applied Sciences, 15(9), 4868. https://doi.org/10.3390/app15094868

Note that from the first issue of 2016, this journal uses article numbers instead of page numbers. See further details here.

Article Metrics

Back to TopTop