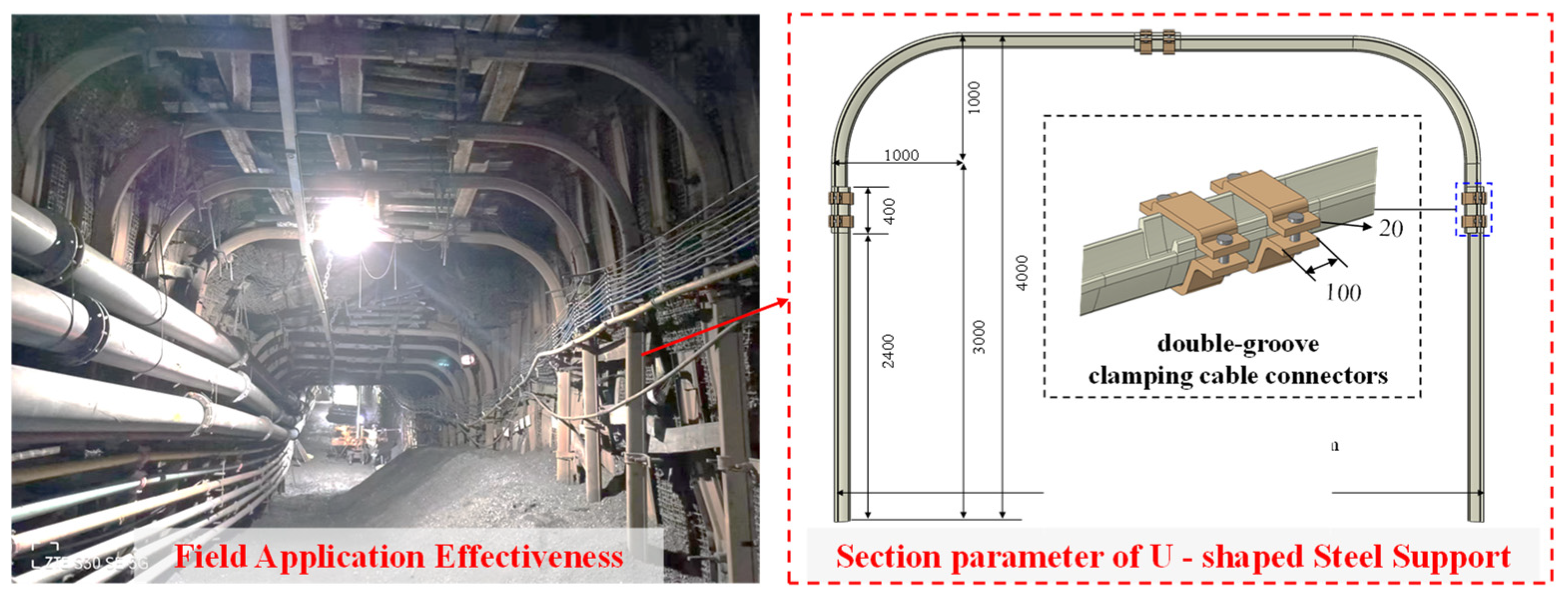
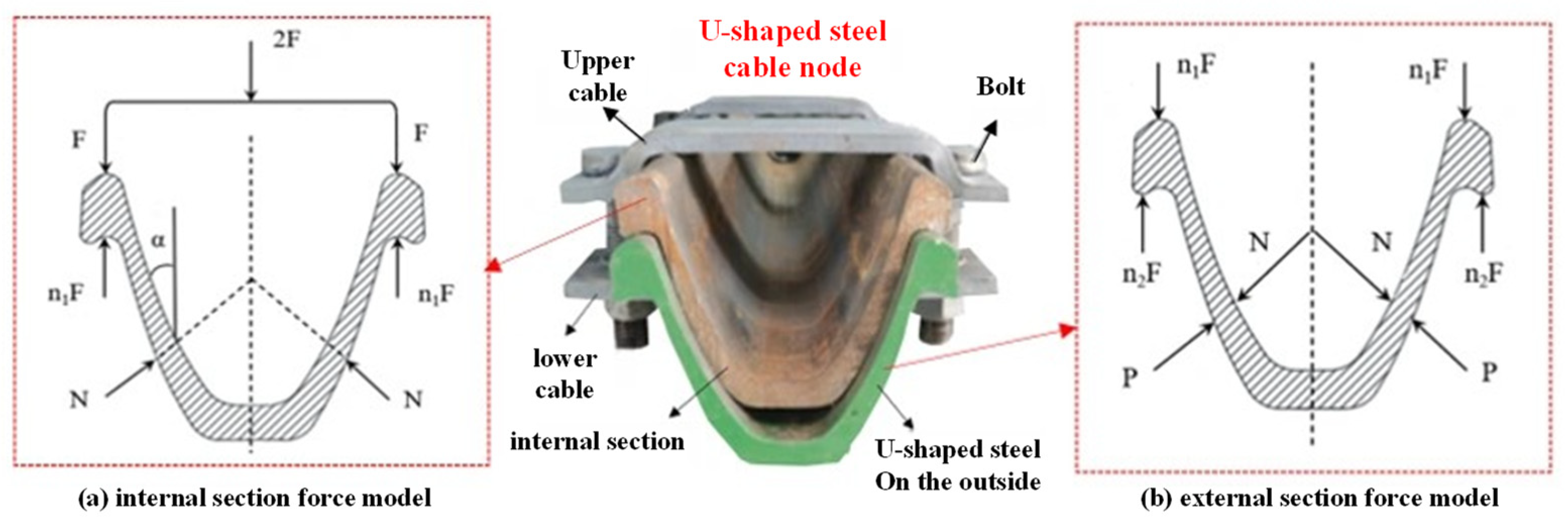
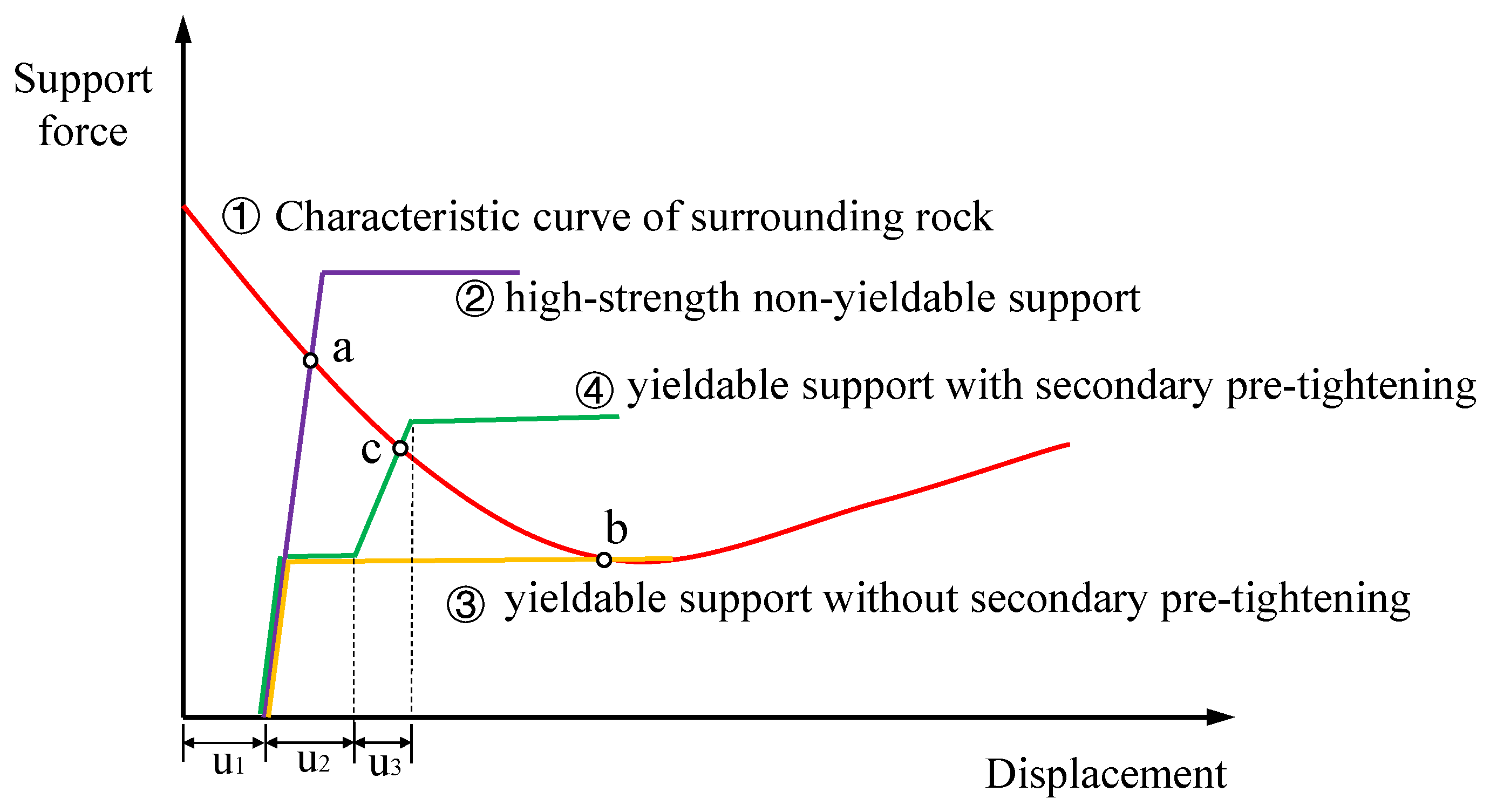
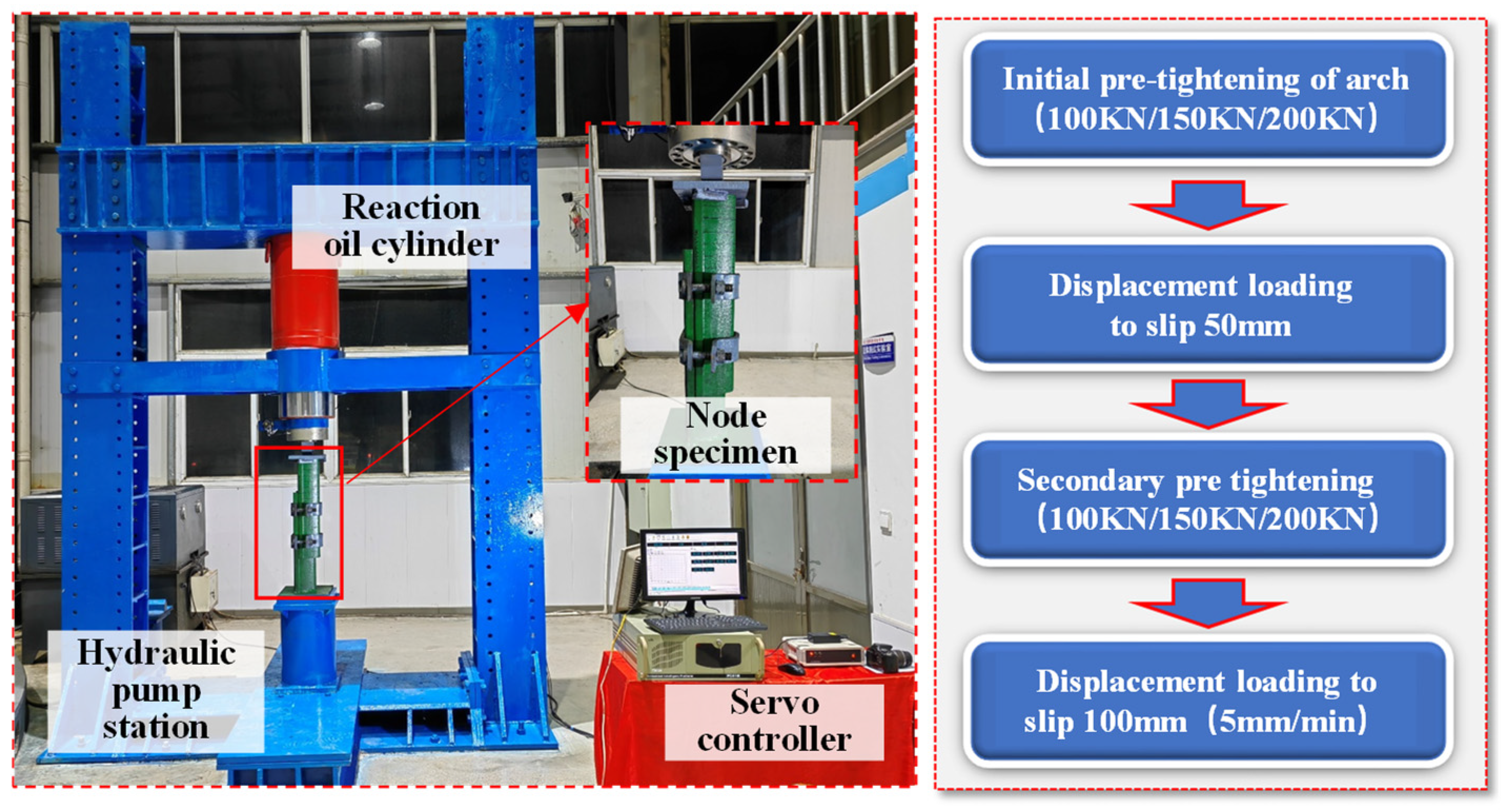
1. Introduction
The progressive exhaustion of shallow coal reserves has necessitated the transition to deep mining operations as imperative for the industry [
1,
2,
3]. Meanwhile, contemporary deep roadway engineering encounters multifaceted geological challenges, including elevated geostress environments, soft-rock strata interactions, and active fault zones [
4,
5,
6]. These compounding factors routinely induce prevalent engineering failures encompassing large-scale peripheral rock deformations and structural roof failures [
7,
8], thereby critically constraining the sustainable exploitation of deep-seated coal deposits.
Yielding support is the core concept for controlling the stability of deep soft rock roadways. That is, it allows a certain amount of deformation of the surrounding rock to release some of the surrounding rock pressure, optimizes the force of the support system, and thus enables the surrounding rock support to reach a new equilibrium state [
9]. Yielding U-steel support is widely used in deep soft rock roadways due to its convenient installation and strong applicability. Many scholars have studied its yieldability and bearing performance. You Chun’an [
10,
11] studied the influence mechanism of the bearing performance of yielding U-steel supports, especially analyzing the stability of the overlapping part of the support. Rodriguez et al. [
12] studied the yielding support characteristics of cable-clip-overlapped U-steel supports in soft rock roadways and generated a typical support characteristic curve for the yielding support. He Manchao et al. [
13] developed an adaptive joint for steel supports in soft rock tunnels, effectively improving the relationship between yieldability and the bearing capacity of the support. Jiao Yuyong et al. [
14] improved traditional U-steel sleeves by backfilling chemical grouting materials behind yieldable steel sleeves to achieve efficient support and stabilize loose thick coal seam roadways, successfully solving problems such as roof collapse, floor heave, large deformations, and support failure in loose thick coal seam roadways. Huang Yubing et al. [
15] studied the bearing performance of a U-shaped steel arch and proposed a segmental design method for U-shaped steel arches. Zhao Yiming et al. [
16] addressed excessive floor deformation by designing closed U-steel supports for comprehensive in situ support, successfully resolving practical site issues via effective control of deformation. Wang Chao et al. [
17] improved the support performance of U-steel supports in roadways by increasing parameters such as the equivalent friction coefficient and foot stiffness to address insufficient stiffness at the connection between invert structures and floor U-steel supports. Heinz et al. [
18] addressed challenges relating to support in high-stress, large-deformation tunnels by using flexible U-steel arches for primary support, achieving effective control of large tunnel deformations. Waclawik P et al. [
19] installed monitoring instruments on U-steel arches supporting roadway surrounding rock to obtain curves of the stress variation between the arches and rock, laying a solid foundation for these instruments’ application.
The above-mentioned research shows that in the bearing process of yield-type U-steel supports, the joint is a key part affecting bearing performance. At present, the overlapping of each section of a U-steel support mainly uses cable-clip joints, and the overlapping of each section is achieved by applying a pre-tightening torque to the cable-clip bolts. Hou Chaojiong et al. [
20] studied the mechanical characteristics of cable-clip bolt connectors and revealed the influence law of pre-tightening torque on improving the working resistance of joints. Xie Wenbing et al. [
21] studied the slip-bearing characteristics and stable-resistance technologies of U-steel joints. The results showed that secondary pre-tightening after slippage is of great significance for maintaining a high support resistance at the joint. Zhang Haowen et al. [
22] carried out indoor tests on the bearing performance of U-steel supports and revealed the influence mechanism of the pre-tightening force of the cable-clip bolts on the overall bearing capacity of the support; Dong Biao et al. [
23] analyzed the influence of bolt pre-tightening force and groove shape on the working performance of yield-type U-steel joints through finite-element simulation and axial load testing of joints. Horyl P et al. [
24] studied numerical simulations of yield-bolt connections in U-steel arches under different torques, summarizing the relationship between clamping force and the load-carrying capacity of the component. Liu Jianzhuang et al. [
25] addressed buckling failure issues in pins in U-steel supports by developing 3D models for U25 and U29 supports, obtaining deformation mechanisms, stress distributions, and details of load variation laws during support deformation, to provide a basis for design improvements. Tan Xianjun et al. [
26] proposed a combined support system consisting of compressible U-steel and foam concrete damping layers to formulate a suitable support scheme for large-deformation soft rock roadways in coal mines, effectively controlling large deformations after application in the field. Su Zhili et al. [
27] developed a “surface-inserted” locking unit to overcome unstable locking between U-steel arch segments, enabling effective management of roadway deformation while preventing steel support failure.
In summary, secondary pre-tightening of cable-clip joints can improve the yield-pressure and bearing performance of the support. However, there is currently a lack of relevant research on the secondary pre-tightening effects of cable-clip joints on yield-type supports, and the design of the pre-tightening torque for cable-clip joints still relies mainly on experience.
Based on this, this paper takes as its research object the U29 steel support, commonly used for support in deep soft rock roadways. This study considered the expression of the slip resistance of a cable-clip joint after secondary pre-tightening, conducted indoor axial compression tests on the components of the cable-clip joint, and analyzed the influence mechanism of secondary pre-tightening on the bearing performance of the joint. On this basis, a numerical analysis model of the yield-type U-steel support was established to analyze the mechanisms through which the initial pre-tightening torque, the secondary pre-tightening torque, and the timing of secondary pre-tightening influenced the bearing performance of the yield-type U-steel support, and engineering suggestions are put forward.
4. Numerical Tests on Mechanical Performance Following Secondary Pre-Tightening of Supports
On the basis of the above-mentioned research, numerical experimental studies were carried out on U29 steel joints and supports using 2022 Abaqus software to clarify the influence mechanism of secondary pre-tightening on the overall bearing performance of the supports.
4.1. Joint Simulation and Analysis
4.1.1. Establishing the Joint Model
Referring to the national standard data of U29 steel for mining, the material of the U-steel was 20 Mnk, with a density of 7800 kg/m3, an elastic modulus of 210 GPa, a Poisson’s ratio of 0.27, a yield strength of 335 MPa, a tensile strength of 490 MPa, and elongation of 16%; the yield strength of the cable-clip splint was 355 MPa, and the tensile strength was 600 MPa.
First, the cross-section of the U29 steel was drawn in 2022 CAD software and imported into the Abaqus software; then, the U-steel component was generated by extrusion. Similarly, other components such as cable-clips were generated and then assembled. Hard surface-to-surface contact was applied between the U-steel sections and between the U-steel and the cable-clip, and the friction coefficient was 0.3 [
29]
4.1.2. Analysis and Verification of Numerical Results
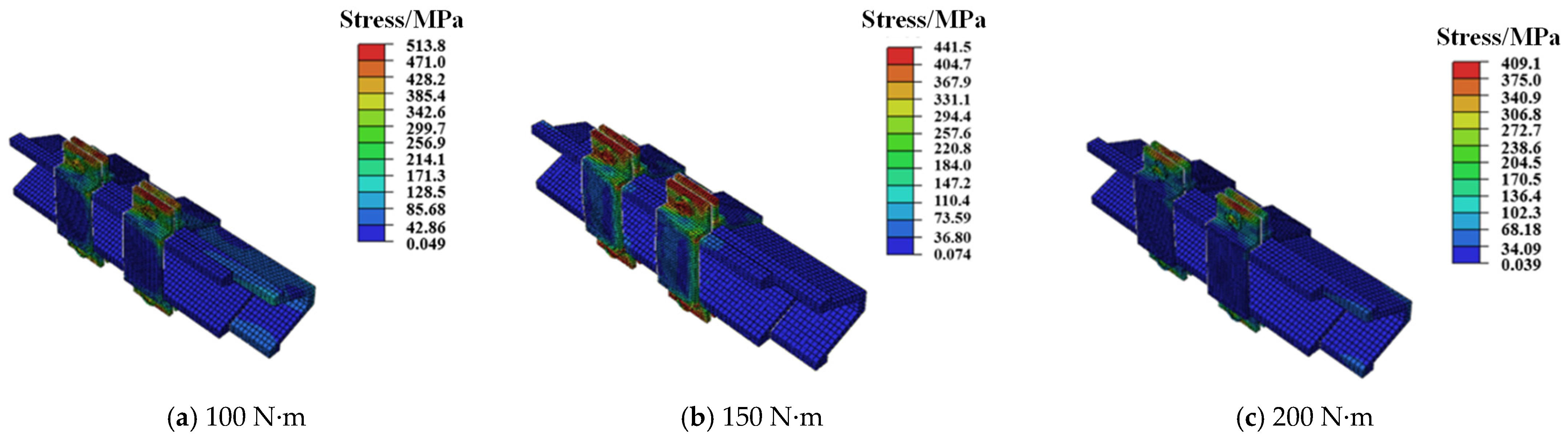
The axial compression simulation process of the joint was consistent with the indoor testing. The stress nephogram of the joint at the end of the test is shown in
Figure 7, and the comparison between the test value and the simulated value of the slip resistance is presented in
Table 4.
It can be seen from the above figures that the stress nephograms of the joints under different pre-tightening torque conditions were similar. The maximum stress was located at the cable-clip bolt, and the stress at the other positions was relatively small. The deformation and slip process measured by the numerical testing was basically the same as in the indoor tests.
Table 4 reveals that the average error between the test value and the simulated value of slip resistance after the initial pre-tightening was 7.1%, which further proves the rationality of the numerical model.
4.2. Simulation and Analysis of U29 Steel Supports
4.2.1. Establishment of the U29 Steel Support Model
On the basis of the numerical model of the support joint, taking the commonly used four-section U29 steel support method employed in deep roadways as the research object, the positions of the support sections were modelled for the numerical calculations. The parameters in this model were the same as those in the above-mentioned joint simulation; the support parameters and section positions are shown in
Figure 8.
4.2.2. Simulation Scheme and Evaluation Index
The test scheme was designed to reflect variables including the absence of secondary pre-tightening torque (Scheme O), the magnitude of the initial pre-tightening torque (Scheme A), the magnitude of the secondary pre-tightening torque (Scheme B), and the timing of the secondary pre-tightening (Scheme C). The detailed scheme is shown in
Table 5.
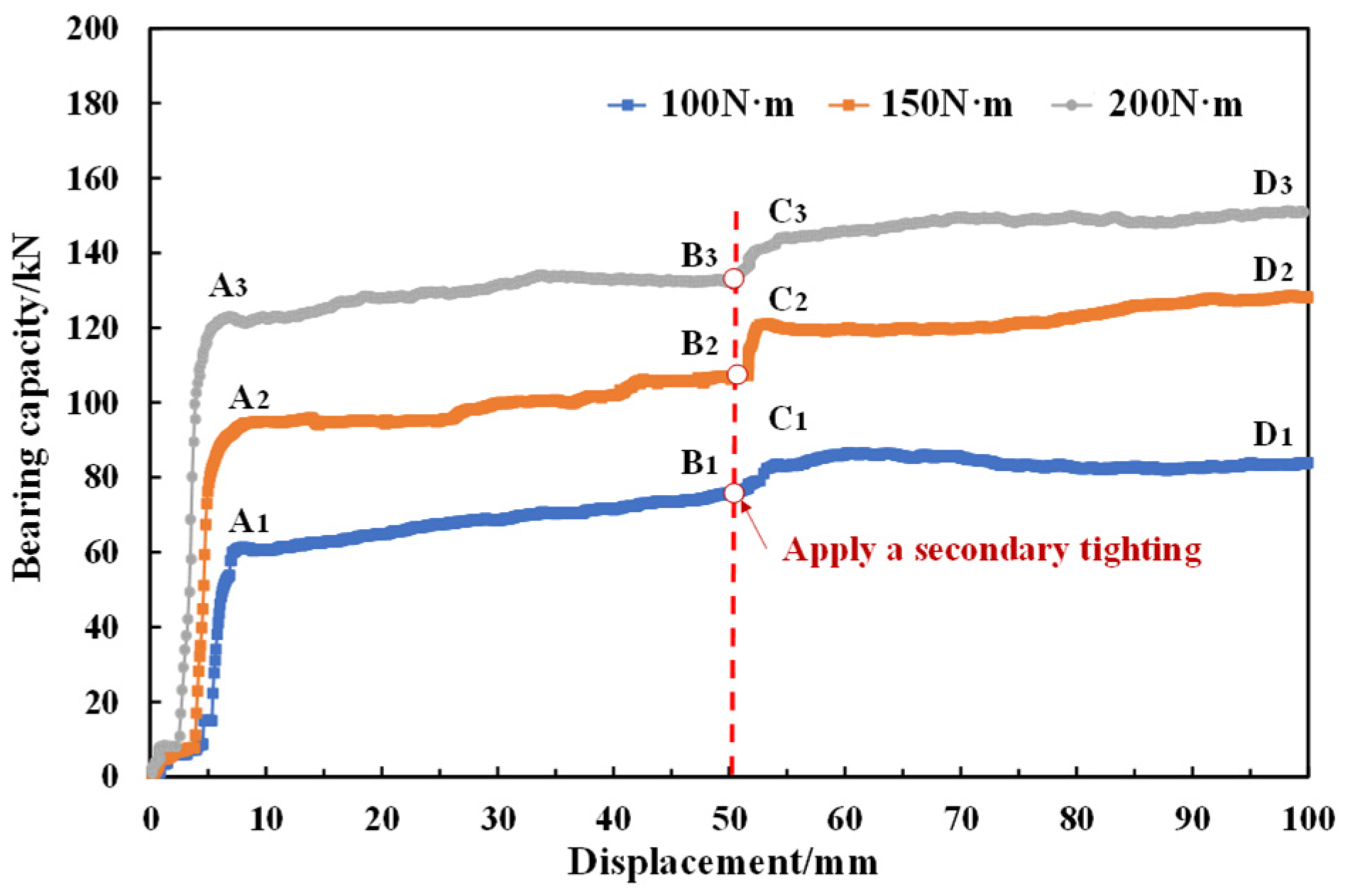
In Scheme O, the initial pre-tightening torque of the cable-clip bolts on the support was 100 N·m, 150 N·m, 200 N·m, 250 N·m, and 300 N·m, respectively. No secondary pre-tightening was carried out during the loading process, and the loading continued until failure. For Scheme A, the initial pre-tightening torque of the cable-clip bolts on the support was 100 N·m, 150 N·m, 200 N·m, 250 N·m, and 300 N·m, respectively. When the yield pressure on the joint reached 50% of the ultimate slippage value, a secondary pre-tightening torque equal to the initial pre-tightening torque was applied, and the loading then continued until failure. In Scheme B, the initial pre-tightening torque of the cable-clip bolts on the support was 200 N·m. When the yield pressure on the joint reached 100 mm, secondary pre-tightening torques of 100 N·m, 150 N·m, 200 N·m, 250 N·m, and 300 N·m, respectively, were applied, and the loading then continued until failure. Scheme C included initial pre-tightening torque of 200 N·m on the cable-clip bolts. When the yield pressure on the joints of the specimens reached 50 mm, 100 mm, 150 mm, 200 mm, and 250 mm, respectively, a secondary pre-tightening torque of 200 N·m was applied, and the loading then continued until failure.
To establish the evaluation indexes, the rate of change in secondary pre-tightening bearing capacity was considered. Under conditions of the same initial pre-tightening magnitude, after applying secondary pre-tightening, the change in the support bearing capacity was compared with that without secondary pre-tightening. Regarding the rate of change in support yield pressure, under conditions of the same initial pre-tightening magnitude, the change in the support yield pressure after applying secondary pre-tightening was compared with that without secondary pre-tightening.
4.2.3. Results Analysis
- (1)
Analysis of the Influence of the Initial Pre-tightening Magnitude
Under different magnitudes of initial pre-tightening torque, after applying a secondary pre-tightening torque of 200 N·m, the support bearing capacities were compared. The results are shown in
Figure 9a, and the comparison of the joint yield pressure is shown in
Figure 9b.
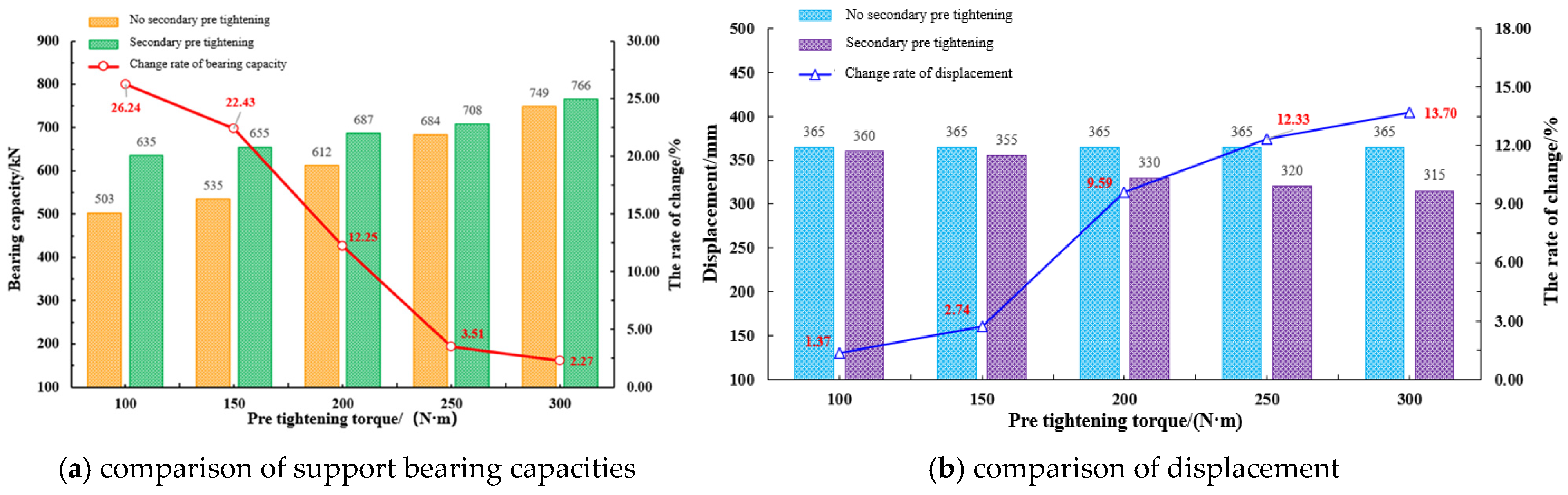
It can be seen from
Figure 9a,b that when the initial pre-tightening torque was 100 N·m, after applying secondary pre-tightening, the support bearing capacity increased from 503 kN to 635 kN, and the rate of change in the secondary pre-tightening bearing capacity reached 27.1%. When the initial torque was increased to 300 N·m, after secondary pre-tightening, the support bearing capacity increased by 131 kN compared with when the initial pre-tightening torque was 100 N·m, and the rate of change in the secondary pre-tightening bearing capacity was 2.5%. Thus, it was observed that applying secondary pre-tightening had a certain enhancing effect on the support bearing capacity. However, when the initial torque exceeded 250 N·m, the degree of influence of the secondary pre-tightening on the bearing capacity gradually decreased.
Regarding the support joint yield pressure, when the initial pre-tightening torque was 100 N·m, after applying secondary pre-tightening, the support joint yield pressure decreased from 476 mm to 351 mm, and the change rate of the yield pressure was 26.3%. When the initial torque was 300 N·m, the support joint yield pressure after secondary pre-tightening decreased by 54 mm compared with when the initial pre-tightening torque was 100 N·m, and the change rate of the yield pressure was 5.7%. It was observed that applying secondary pre-tightening had a certain influence on the yield-pressure performance of the support joint. However, with the increase in the initial torque, the influence of secondary pre-tightening on the yield-pressure performance of the support joint gradually decreased.
- (2)
Analysis of the Influence of Secondary Pre-tightening Magnitude
Comparison of the support bearing capacities under different magnitudes of secondary pre-tightening torque is shown in
Figure 10a, and comparison of the support joint yield pressure is shown in
Figure 10b.
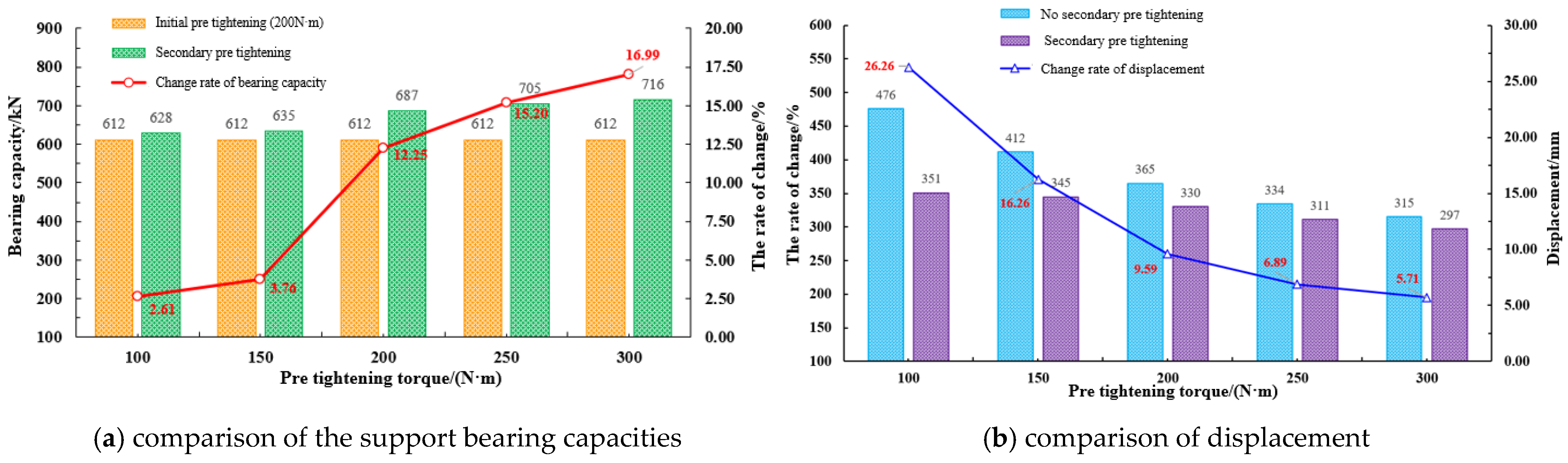
Regarding the support bearing capacity,
Figure 10a,b reveal that when the initial pre-tightening torque was 200 N·m, after applying secondary pre-tightening torques of 100 N·m and 150 N·m, the support bearing capacity increased from 612 kN to 628 kN and 635 kN, and the rates of change in the secondary pre-tightening bearing capacity were only 2.7% and 3.7%, respectively. When the secondary torque increased to 200 N·m and 300 N·m, the support bearing capacity increased from 612 kN to 687 kN and 716 kN, and the rates of change in the secondary pre-tightening bearing capacity were 12% and 17%, respectively. It was observed that when the secondary pre-tightening torque was less than the initial torque, this had no obvious enhancing effect on the support bearing capacity; the higher the secondary pre-tightening torque, the more significant was the enhancement of the support bearing capacity.
In terms of the support joint yield pressure, under conditions of an initial pre-tightening torque of 200 N·m, after applying secondary pre-tightening torques of 100 N·m and 150 N·m, the support joint yield pressure decreased from 365 mm to 360 mm and 355 mm, and the rates of change in the secondary pre-tightening joint yield pressure amounted to only 1.7% and 2.7%, respectively. When the secondary torque was increased to 200 N·m and 300 N·m, the support joint yield pressure decreased from 365 mm to 330 mm and 315 mm, and the rates of change in the secondary pre-tightening bearing capacity were 9.6% and 13.7%, respectively. Thus, it can be seen that when the secondary pre-tightening torque is less than the initial torque, this has no obvious influence on the yield-pressure performance of the support joint; the higher the secondary pre-tightening torque, the more significant is its the influence on the slippage performance of the support joint.
- (3)
Analysis of the Influence of the Timing of Secondary Pre-tightening
Under conditions where both the initial pre-tightening torque and the secondary pre-tightening torque were 200 N·m, comparison of the influence of different timings of secondary pre-tightening on the support bearing capacity is shown in
Figure 11a, and comparison of the influences on the support joint yield pressure is shown in
Figure 11b.
Regarding the support bearing capacity, it can be seen from
Figure 11a,b that when the secondary pre-tightening torque was applied at 30% and 40% of the ultimate yield pressure of the joint, the support bearing capacity increased from 612 kN to 635 kN and 642 kN, and the rates of change in the secondary pre-tightening bearing capacity were only 3.8% and 4.9%, respectively. When the secondary pre-tightening torque was applied at 50% and 70% of the ultimate yield pressure of the joint, the support bearing capacity increased to 687 kN and 706 kN, and the rates of change in the secondary pre-tightening bearing capacity were 12.3% and 15.4%, respectively. Thus, it was observed that when the secondary pre-tightening torque was applied before the joint underwent sufficient slippage, the enhancing effect on the support bearing capacity was limited; meanwhile, when the secondary pre-tightening torque was applied after the joint had slipped by more than 50% of the ultimate yield pressure, the enhancing effect on the support bearing capacity was significant.
Regarding the support joint yield pressure, when the secondary pre-tightening torque was applied at 30% and 40% of the ultimate yield pressure of the joint, the support joint yield pressure decreased from 365 mm to 355 mm and 350 mm, and the rates of change in the secondary pre-tightening joint yield pressure were only 2.7% and 4.1%, respectively. When the secondary pre-tightening torque was applied at 50% and 70% of the joint’s ultimate yield pressure, the yield pressure on the joint decreased from 365 mm to 330 mm and 310 mm, and the rates of change in the secondary pre-tightening joint yield pressure were only 12.3% and 15.1%, respectively. It was observed that when the secondary pre-tightening torque was applied before the joint had slipped sufficiently, the degree of influence degree on the yield-pressure performance of the support was low; when the secondary pre-tightening torque was applied after the joint had slipped by more than 50% of the ultimate yield pressure, the influence on the support’s yield pressure performance was significant.
4.3. Summary and Engineering Suggestions
Applying secondary pre-tightening torque to the cable-clip joint can significantly enhance the bearing capacity of the support. However, in this study, when the initial torque was greater than 250 N·m and the same secondary pre-tightening torque was applied, the degrees of influence on the bearing capacity and the yield-pressure performance of the support were within 5% and 10%, respectively. In practical engineering, it is recommended to perform secondary pre-tightening on the cable-clip joints of yield-type supports. Taking the cable-clip joint of the U29 steel support as an example, the initial pre-tightening torque is preferably 250 N·m.
The larger the secondary pre-tightening torque, the more significant were the influence on the bearing capacity of the support and the yield-pressure performance of the joint. When the secondary pre-tightening torque was less than the initial torque, the increase in the support bearing capacity was less than 5%. For practical engineering applications, taking the cable-clip joint of the U29 steel support as an example, it is recommended that the secondary pre-tightening torque should be greater than the initial pre-tightening torque.
When secondary pre-tightening was applied before the support joint has undergone sufficient slippage, the enhancing effect on the support bearing capacity was limited; when the joint slippage exceeded 50% of the ultimate slippage, applying the secondary pre-tightening torque had a significant enhancing effect on the support bearing capacity. For practical engineering purposes, taking the cable-clip joint of the U29 steel support as an example, it is recommended that secondary pre-tightening should be applied when the amount of joint slippage is not less than 200 mm.
5. Conclusions
Taking the commonly used U29 steel support (specific to underground coal mine applications) as the research object, the expression of the slip resistance of the cable-clip joint after secondary pre-tightening was established and verified through indoor axial compression tests. On this basis, numerical tests were carried out to determine the influence mechanisms of different magnitudes of initial pre-tightening torque and secondary pre-tightening torque as well as the timing of secondary pre-tightening. The findings specific to U29 steel supports and their general implications are summarized as follows:
- (1)
Initial pre-tightening torque effects (U29-specific findings)
When the initial torque exceeded 250 N·m, the influence of secondary pre-tightening on both bearing capacity and joint yield-pressure performance demonstrated a gradually decreasing trend. This threshold appears to characterize U29′s material properties and cross-sectional geometry;
- (2)
Secondary pre-tightening torque (generalizable principles)
The enhanced effect on bearing capacity showed a positive correlation with torque magnitude. Notably, when secondary torque remained below initial torque levels (a relationship potentially applicable to other U-shaped supports), no significant improvement in joint performance was observed;
- (3)
Secondary pre-tightening timing (system-dependent phenomenon)
The timing of the secondary pre-tightening critically affected the U29′s performance. Limited enhancement occurred when tightening was applied before the joint slip reached the critical threshold; significant improvements emerged when tightening was implemented after exceeding 50% of the ultimate slip (this slip-dependent behavior may vary with support stiffness).
Considering the generalizability of the current study, while the fundamental torque–slip relationship provides transferable insights, the specific thresholds (250 N·m torque limit, 50% slip criterion) are intrinsically tied to U29′s dimensional parameters. For alternative U-shaped supports (e.g., U36 or U43 profiles), these parameters require recalibration through adjustment of material properties, modification of gross-sectional geometry, site-specific analysis of load conditions, and so on.
Future research should include comparative studies extending to U36/U43 steel supports with varied rib configurations, in addition to parametric investigations on flange thickness (6–10 mm range) and yield strength (235–355 MPa grades).