An In-Depth Analysis of the Seismic Performance Characteristics of Steel–Concrete Composite Structures

Abstract

This review article provides an in-depth exploration of the recent advancements in the seismic analysis and design of steel–concrete composite structures, as reflected in the literature from the last ten years. It investigates key factors, such as material behavior, connection detailing, analytical modeling techniques, and design methodologies. The article highlights the synergistic benefits derived from the combination of steel and concrete components to improve seismic performance. Various composite systems, including composite beams, beam-columns, frames, shear walls, foundations, and beam–column joints, are analyzed through experimental studies to assess their dynamic response characteristics under extreme earthquake conditions. The article evaluates advanced numerical modeling methods, including finite element analysis and fiber-based models, for their capability to predict the nonlinear behavior of composite buildings and bridges. A comparative analysis of modern seismic isolation and energy dissipation techniques is also included. Furthermore, the optimization of composite structures in seismically active regions is discussed. The article concludes by identifying areas where additional research is necessary to enhance the seismic resilience of steel–concrete composite structures.

1. Introduction

The seismic design and analysis of steel–concrete composite structures encounter numerous significant challenges that necessitate in-depth exploration. Although these structures are commonly utilized, considerable uncertainties persist regarding the intricate interactions between steel and concrete elements when subjected to intense seismic forces. Existing design codes frequently fall short in adequately addressing the nonlinear behavior exhibited by composite systems, particularly at the junctions of the materials. Moreover, the variety of composite configurations complicates the development of standardized design methodologies, while the absence of extensive long-term performance data introduces further uncertainty in durability assessments. Conventional analytical models may overly simplify the dynamic response features of composite structures, which could result in designs that are either excessively conservative or insufficiently robust. Additionally, the construction industry grapples with challenges in evaluating the cost-effectiveness of innovative composite solutions in comparison to traditional building methods, particularly when factoring in life-cycle performance during seismic events. These critical concerns highlight the necessity for a comprehensive review of the existing knowledge and practices related to steel–concrete composite structures.

As urban areas continue to expand and face increasing seismic risks, understanding the behavior of these composite systems under earthquake loading has become crucial for ensuring structural safety and resilience. This review paper seeks to offer a thorough examination of the existing body of research concerning the seismic analysis and design of steel–concrete composite structures. This article examines key research developments, design methodologies, and practical applications that have emerged mainly over the past decade. The paper covers various composite systems, including composite beams, columns, connections, and innovative hybrid structural solutions. This review encompasses the following areas:

• The fundamental principles of steel–concrete composite action under seismic loading
• Experimental studies on the seismic performance of composite elements and systems
• Analytical and numerical modeling techniques for predicting composite behavior
• Current design codes and standards for seismic design of composite structures
• Emerging technologies and novel composite systems for enhanced earthquake resistance
• Case studies of notable composite structures in seismic regions

By synthesizing the latest research findings and practical insights, this paper seeks to identify knowledge gaps, highlight promising research directions, and provide valuable guidance for engineers and researchers working on the seismic design and analysis of steel–concrete composite structures. To provide a comprehensive review, the following 12 topics are examined in depth:

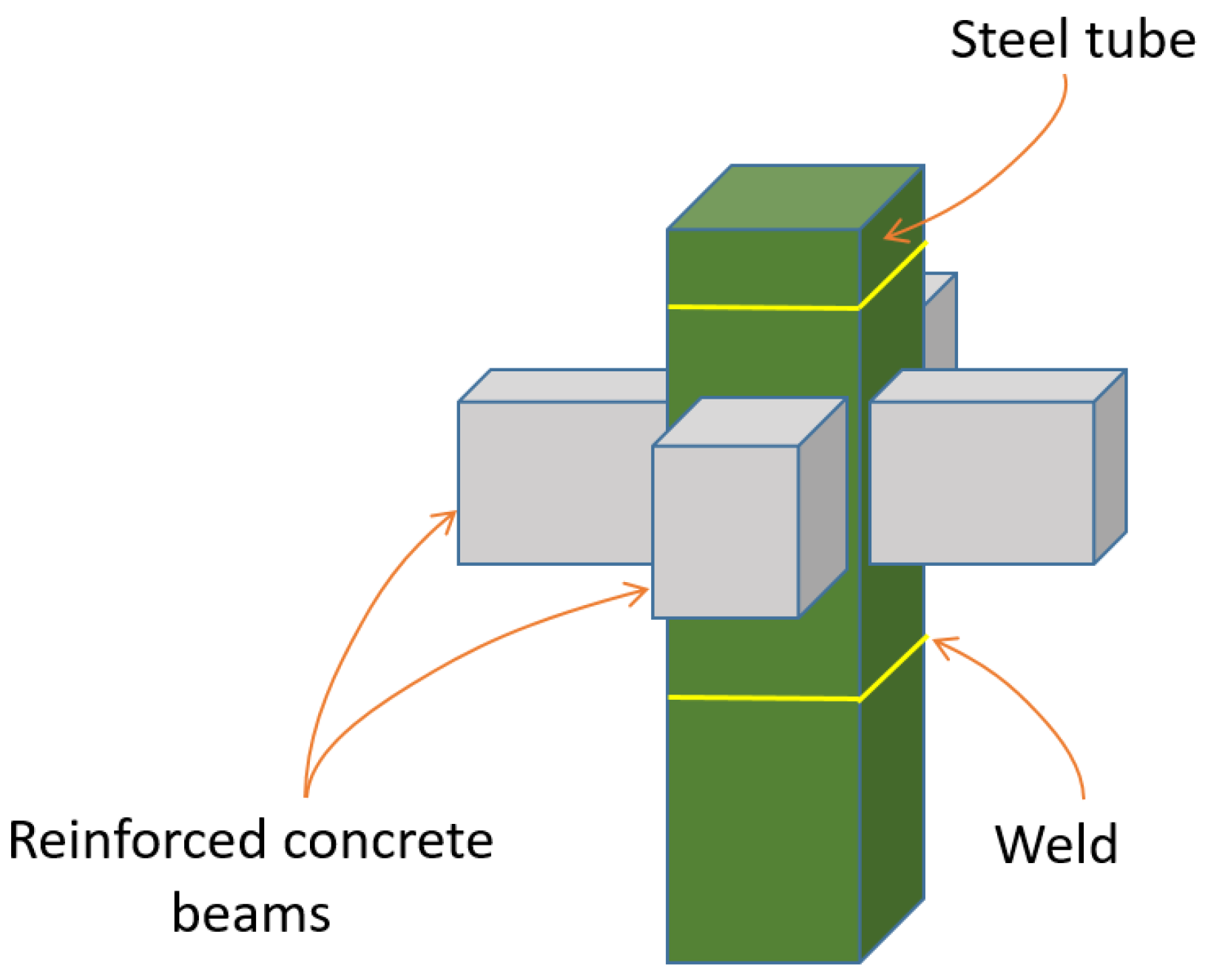
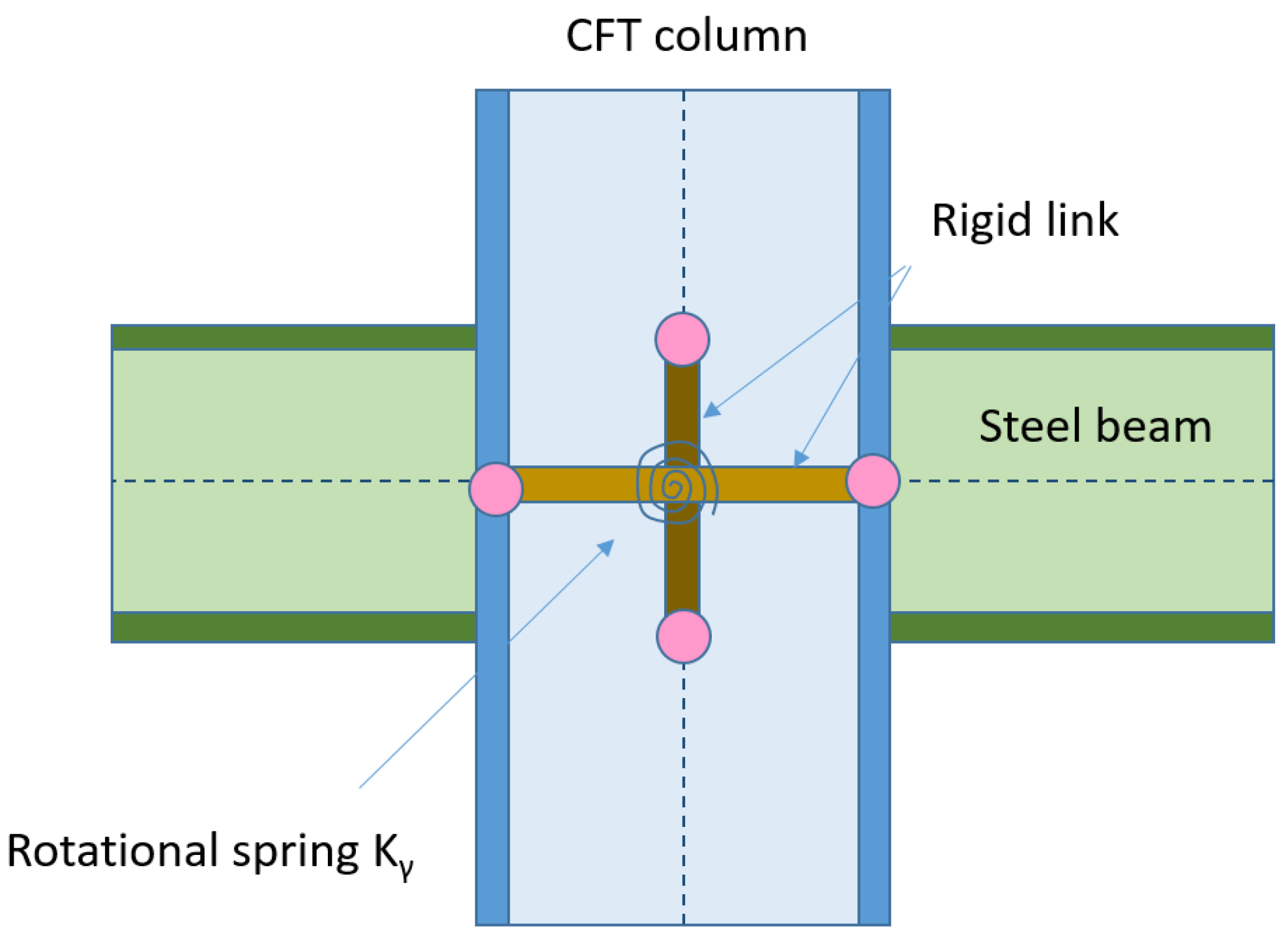
• Beam–column joints of composite frames under seismic loads: the behavior of composite beam–column connections under seismic loading is examined, including moment-resisting frames and various joint configurations. Research on load transfer mechanisms and failure modes is discussed.
• The seismic behavior of steel–concrete composite shear walls: composite concrete/steel shear wall systems, including steel-plate shear walls with concrete infill and composite coupling beams, are reviewed. Their effectiveness in lateral load resistance and energy dissipation is analyzed.
• Modeling techniques for composite members and structures under earthquake excitation: advanced numerical modeling techniques for composite structures, including finite element analysis, multi-scale modeling approaches, and the challenges in accurately representing composite behavior are discussed.
• The behavior of composite beams under cyclic loads: the hysteretic response of composite beams under repeated loading, including local buckling phenomena and the influence of concrete encasement on steel beam performance, is investigated.
• The behavior of composite beam-columns under cyclic loads: the performance of composite columns under cyclic loading, considering various cross-section types (e.g., concrete-filled steel tubes, encased steel sections) and their impact on ductility and energy dissipation is analyzed.
• The seismic performance of composite buildings: the advances in the seismic design of composite building structures are analyzed. The review focuses on the inelastic behavior of composite buildings where the evaluation of inelasticity at various levels of seismic intensity is significantly enhanced by seismic design approaches that allow for the control of structural deterioration at both the elemental scale (for instance, crucial structural parts) and the overall building scale (such as individual floors).
• Composite construction and foundation seismic design: seismic design considerations for foundations supporting composite structures, including pile-to-pile cap connections and soil–structure interaction effects, are discussed.
• Seismic isolation and energy dissipation devices for composite structures: the integration of base isolation systems with composite structures, focusing on their effectiveness in reducing seismic demands and improving overall structural performance, is examined. Additionally, the implementation of various damping devices (e.g., viscous dampers and friction dampers) in composite structures and their contribution to energy dissipation during seismic events is reviewed.
• The progressive collapse resistance of composite structures: the resistance of composite structures to progressive collapse under extreme loading conditions, including the role of composite action in enhancing structural robustness, is evaluated.
• Optimal design strategies for earthquake-resistant composite buildings: optimization strategies for composite structural systems, considering both material usage and seismic performance objectives is explored.
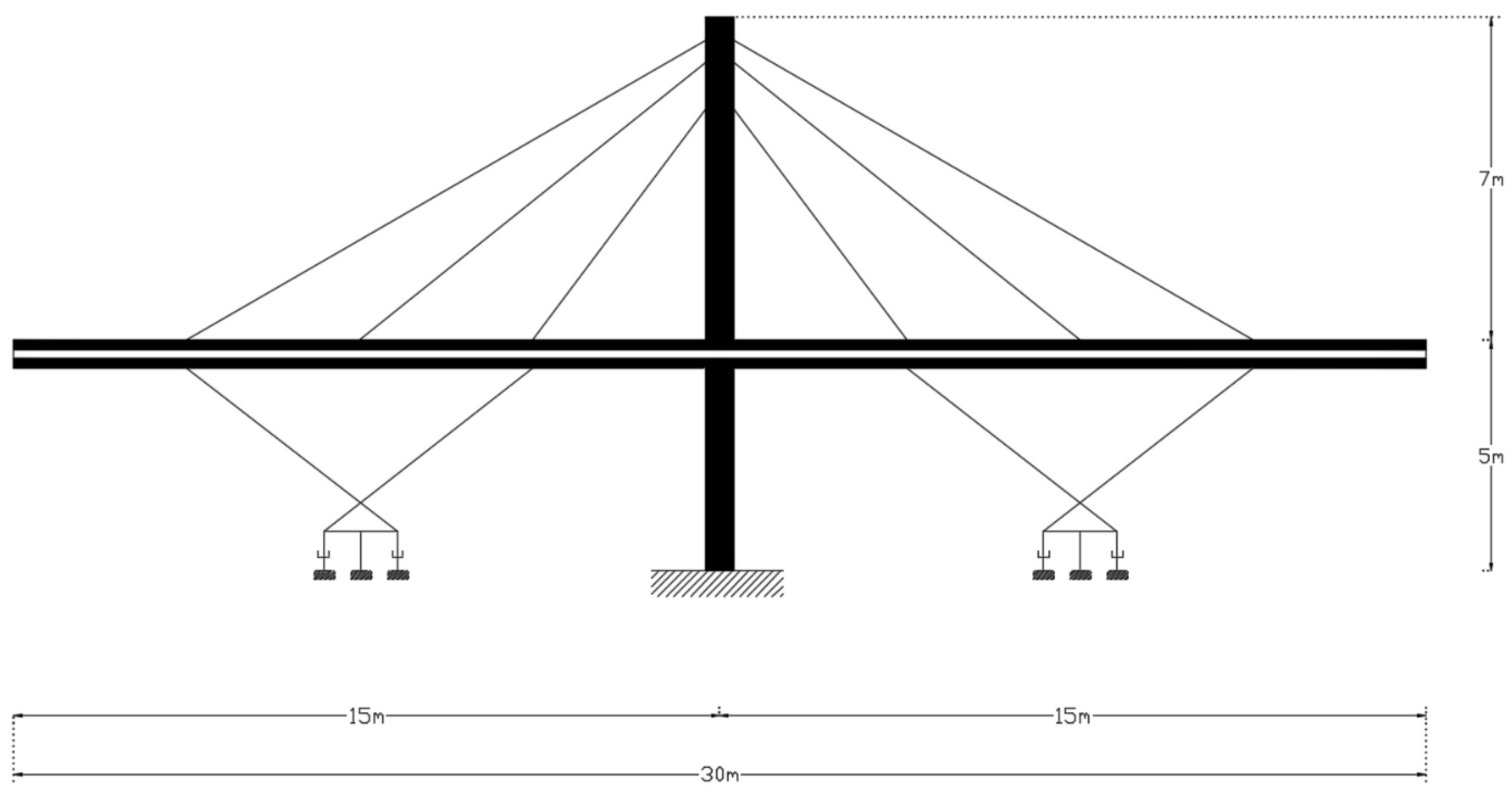
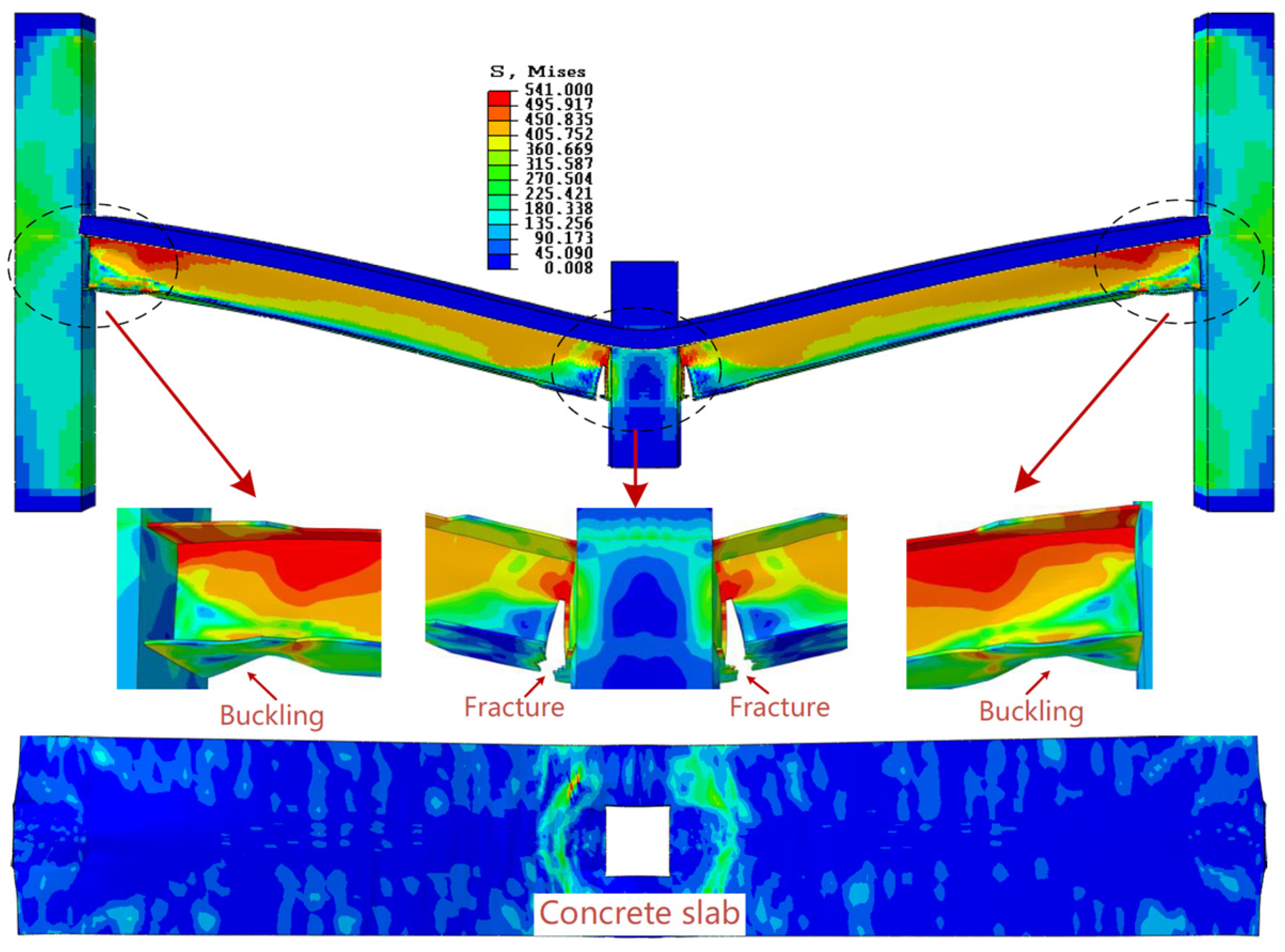
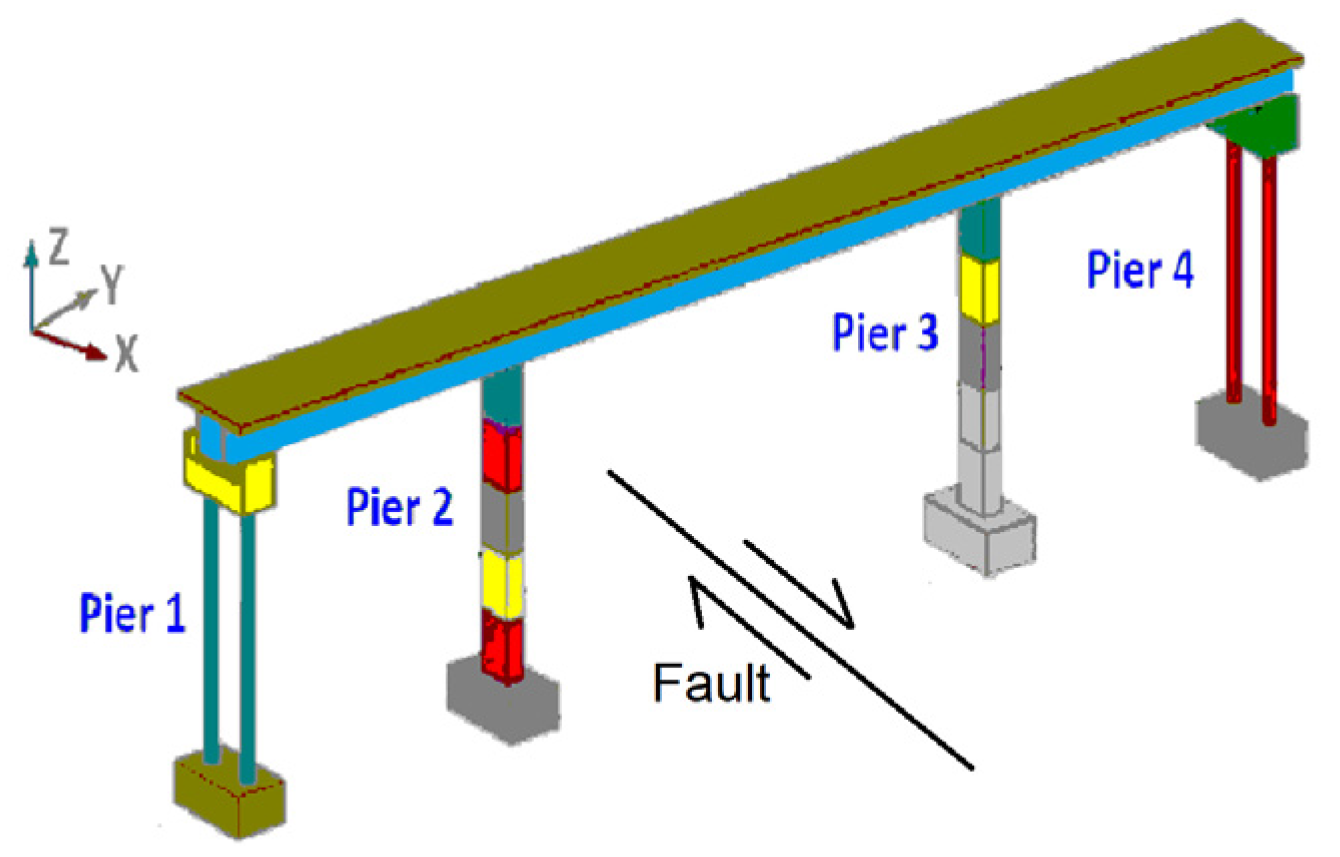
• The seismic performance of steel–concrete composite bridges: the application of composite construction in bridge engineering, focusing on the seismic performance of composite deck systems, piers, and abutments, is explored.
• Smart materials and sensors: new smart materials and sensors enhance the seismic performance of steel–concrete structures, offering self-centering and real-time monitoring capabilities.

The flowchart of the examined topics is shown in Figure 1.

The twelve key areas selected for this comprehensive review were carefully chosen to address the critical challenges in understanding and improving the seismic performance of steel–concrete composite structures. Beginning at the component level, the examination of beam-columns, beams, and beam–column joints under cyclic loads is essential for understanding the fundamental behavior of composite elements during seismic events. The analysis of composite shear walls complements this by addressing lateral load resistance systems. Moving to a broader scale, the review of composite buildings and bridges allows for the evaluation of system-level responses, while the inclusion of foundation design ensures a complete understanding from the ground up. The sections on modeling techniques and optimal design strategies address the crucial need for accurate prediction and efficient design methodologies. The coverage of seismic isolation and energy dissipation devices, along with progressive collapse resistance, tackles the critical aspects of enhancing structural resilience. The smart materials and sensors section reflects the cutting-edge developments in monitoring and adaptive response systems. This carefully structured approach ensures that current practices and emerging technologies are thoroughly examined, providing a comprehensive framework for understanding seismic behavior in composite structures.

The methodological approach for this comprehensive review was carefully designed to ensure both scholarly rigor and comprehensive coverage. Thus, 205 scholarly works published over the past decade are systematically analyzed, employing a multi-stage selection process that prioritized peer-reviewed publications with demonstrable methodological depth. The selection criteria focused on works that provided empirical insights, innovative analytical approaches, or significant advancements in understanding the seismic performance of steel–concrete composite structures.

By intentionally incorporating diverse research perspectives, we aimed to create a narrative that captures the complexity of composite structural systems. This approach prioritizes a holistic representation of current research, acknowledging that scientific understanding emerges from varied methodological traditions and nuanced investigative approaches.

2. Beam–Column Joints of Composite Frames Under Seismic Loads

2.1. Beam–Column Joints of Composite Frames Under Seismic Loads: State-of-the-Art

Beam–column joints play a crucial role in steel–concrete composite structures, particularly during seismic events, as they transfer forces between beams and columns and affect the structure’s earthquake resistance. Composite structures have gained popularity due to their combined strength, stiffness, and ductility. This scholarly paper provides a comprehensive review of the seismic analysis and design of beam–column joints in these structures. It covers recent advancements in experimental studies, analytical methods, and design approaches, focusing on failure modes, influential parameters, experimental investigations, modeling techniques, existing guidelines and limitations, and emerging joint configurations. By consolidating recent research and identifying gaps, this review aims to offer valuable insights to earthquake engineering and structural design professionals.

Thus, Ataei et al. (2017) [1] examined a detailed finite element (FE) model for studying the performance of deconstructable beam-to-column composite joints. Their model was validated through experiments, and a parametric study was conducted to analyze various parameters. Design models were proposed to predict performance parameters. Key findings included the impact of shear connection ratio, reinforcement ratio, bolt size, slab thickness, column flange thickness, and steel grade on joint behavior. It was found that the design models could accurately predict performance parameters, even for joints with high-strength steel components. Furthermore, Van-Long et al. (2015) [2] presented a solution for connecting I-shaped beams to concrete-filled RHS columns using long bolts. The joint configuration improved rigidity and resistance, making it suitable for seismic-resistant moment frames, while experimental tests and analytical models were used to validate the joint design. Moreover, the dynamic response and failure mechanism of a new assembly of blind-bolted CFST composite structures under simulated seismic loading were studied by Wang et al. (2017) [3]. The tests showed excellent seismic performance, including good ductility, energy dissipation capacity, and damage control, while analytical modeling using OpenSees software 3.7.1 (https://opensees.berkeley.edu/ accesed on 10 September 2024) validated the results.

Peng et al. (2018) [4] presented a study on the seismic performance of an innovative end-plate connection between T-shaped CFT columns and reinforced concrete beams. Seven specimens were tested, one under monotonic loading and six under cyclic loading. The proposed end-plate connections exhibited good seismic performance, achieving the desired “strong column and weak beam” and “strong connection” objectives in seismic design. Peng et al. (2018) [5] found that the diameter of the bolts and the length of the (H-shaped) steel corbel influenced the yield strength, ultimate strength, and initial stiffness. A FEM model was also constructed and subsequently verified, and parametric studies revealed the impact of beam reinforcement ratio and concrete strength on seismic performance. Moreover, Peng et al. (2021) [6] examined the shear strength and seismic damage performance of H-section beams with unequal-depth CFT column composite joints. Four specimens with varying height ratios of the beams were subjected to lateral cyclic loading. The findings indicated that shear failure occurred primarily in the joint core area, with the joint core area reaching yield strain earlier as the beam height ratio increased.

A mathematical formula was developed to calculate the shear strength of the CFT columns with a H-shaped beam joint. This equation takes into account the combined effects of the restrained compression struts in the joint central region, concrete compression struts, and steel tube web.

Mou et al. (2021) [7] explored a new type of joint between a reinforced concrete-filled steel tube column and a beam. Four specimens with varying characteristics were tested under cyclic loading. The results showed that slipping between the concrete and steel caused pinch phenomena in the joint’s hysteretic curves. Increasing the size of the middle steel tube improved stiffness and energy dissipation but had little effect on strength. Transfer sleeves played a crucial role in limiting stiffness and strength degradation while enhancing energy dissipation. Yu et al. (2022) [8] focused on evaluating the seismic behaviors of different connections in steel residential buildings, including endplate, side plate, and hot-rolled T-shaped connector (HTC) connections. The experimental findings revealed that both the connections of lateral plate and HTC connections exhibited favorable cyclic response characteristics. These included consistent force-displacement patterns, a gradual reduction in rigidity, adequate ductility, and effective energy absorption capacity. In contrast, the connection of the endplate suffered an untimely rupture at the welded interfaces between the endplate and the side plates. Furthermore, the suggested HTC connections not only demonstrated satisfactory seismic performance but also required no additional stiffeners and had a minimal impact on indoor space, making them easily concealable within partition walls.

Cheng et al. (2022) [9] presented an experimental study on the behavior of connections between T-shaped CFT columns and U-shaped steel–concrete composite beams. Five different connection details were tested, with all showing favorable seismic performance with plastic hinge failure occurring at the beam-ends. The joints demonstrated good ductility and energy dissipation capacity, meeting Eurocode 3 requirements for rigid joints in braced frames. They concluded that their proposed joint design with vertical ribs is seismically feasible and provides recommendations for practical applications. Li et al. (2023) [10] introduced a new manufactured steel beam (H-shaped) to a CFT column joint design. This system aims to improve construction efficiency and structural performance by eliminating welding in column connections. The joint employs embedded threaded steel bars for column segments and outer annular stiffeners for beam connections. They tested four joint specimens under cyclic loading, examining parameters, such as column tube thickness, sleeve distance, and steel strength, and their results showed good flexural capacity, deformability, and energy dissipation.

Chen et al. (2014b) [11] examined the performance of a new through-beam connection between reinforced concrete beams and CFT columns. Tests showed different failure modes and demonstrated good seismic performance, load capacity, and ductility. Finite element modeling validated the results, and this new connection type simplifies construction without sacrificing structural integrity and offers valuable considerations for future CFST column-to-RC beam connections. Moreover, Jeddi et al. (2017) [12] introduced and tested a novel moment-resisting connection called a “through rib stiffener beam connection” for I-beams and concrete-filled circular steel tubular columns. Four cruciform specimens were experimentally examined to evaluate the behavior of the connections under seismic loading. The new connection involved vertical rib stiffeners welded to the beam flanges, passing through pre-slotted holes in the column. It was found that the new connection exhibited rigid moment-resisting behavior, good ductility, and energy dissipation capacity. The connection appears to satisfy seismic design requirements and is practical and cost-effective.

Amadio et al. (2017) [13] introduced a refined finite element model for predicting the behavior of composite steel–concrete welded joints under earthquake loads. The model underwent validation through comparison with experimental data, successfully depicting both global and local behaviors, stress distributions, and failure mechanisms. This was achieved by incorporating the Gurson model for steel, accounting for concrete behavior and damage, and ensuring an accurate representation of connections. The validated model can be used for extensive parametric studies and can lead to suggested improvements to Eurocode 8 design provisions. Similarly, Liu et al. (2017) [14] investigated the performance of special-shaped CFT columns—steel beam connections. Four specimens were tested with different shapes and joint stiffeners. Different failure modes were observed, and it was found that exterior diaphragms performed better than vertical ribs in transferring forces. The study also developed finite element models and proposed design formulas based on internal force transfer mechanisms. The findings suggest that exterior diaphragm connections are suitable for engineering applications, while vertical rib connections require improvement.

Li et al. (2021) [15] investigated concrete-encased CFST columns/steel beam joints using experimental tests. An RC slab was installed for each joint, and an external diaphragm design was used to connect the beam flange and the CFST tube. The joints were subjected to constant axial load and reverse cyclic loading to provide test data for composite joints, evaluate their seismic performance, and analyze the effects of key parameters, specifically the joint type. Additionally, Wu et al. (2020) [16] designed three specimens of joints using an integrated combination scheme and tested them with low-cycle reciprocating loading. The results analyzed the strength degradation, stiffness degradation, failure modes, energy dissipation, and ductility, clarifying the effect of the beam-to-column stiffness ratio on seismic performance of modular composite joints.

Wang et al. (2016) [17] executed an experimental program on two blind-bolted composite joints to CFTST columns in full-scale under short cyclic loading to understand their seismic performance and failure modes. The results were used to assess various aspects, like hysteresis, strength degradation, ductility, and energy dissipation capacity, with implications for enhancing the design of composite joints in practice. Furthermore, Peng et al. (2018) [4] introduced new connections, of a ring-bar-reinforced type, for connecting reinforced concrete slabs, beams, and T-shaped CFST columns. They conducted tests on 34 columns and derived a formula to determine the axial compression bearing capacity. Additionally, an improved composite column was presented, which is more cost-effective and exhibits better seismic behavior. The connection between the T-shaped CFST column and the RC beams is strengthened by the new ring-bar-reinforced connection, enhancing the stiffness and bearing capacity.

The concept and benefits of an innovative prefabricated composite column–beam joint, combining concrete-filled steel tubular (CFST) columns with reinforced concrete (RC) beams, were first presented by Zhang and Li (2021) [18]. To evaluate the earthquake resistance of these connections, which employ bolts and non-adherent post-stressed cables, the researchers conducted alternating load experiments on eight reduced-scale prototypes (one-third of full size). The study examined how various factors, including the vertical load ratio, bolt size, steel tube thickness, and overall structural configuration, influenced the seismic response characteristics of these joints. The resulting data were thoroughly analyzed and interpreted to draw meaningful conclusions about the connections’ performance under simulated earthquake conditions. A novel joining technique for linking a steel–concrete composite column (concrete-encased CFST) with a metallic beam was introduced by Wang et al. (2020) [19]. This method utilizes concealed fasteners. The composite column, which consists of a concrete-filled steel tube further encased in concrete, is manufactured off-site in a controlled environment, with bolt-holes drilled in the steel tube and concrete poured around it. Blind bolts are then installed, and the core concrete is poured on site. Numerical models and experimental results were used to analyze the connection, considering material models and contacts. Component models for stiffness and moment resistance were developed, considering the load transfer mechanism.

The seismic behavior of vertical rib connections for the joint of an H-shaped steel beam to a special-shaped CFT column was tested by Yang et al. (2021) [20]. Frames were tested under vertical and horizontal loads to examine stiffness degradation, failure mode, energy dissipation, strength-bearing capacity, and ductility. Finite element analysis was used to investigate the plastic hinge formation and failure mode. Furthermore, Xu et al. (2021) [21] explored the seismic and shear behavior of joints connecting U-shaped steel–concrete composite beams to T-shaped concrete-filled steel tubular columns. A new joint design with C-shaped slots allowing double-C channels to pass through the joint panel zone was proposed and tested.

The seismic performance of composite steel–concrete frame systems using the URSP (uplift-restricted/slip-permitted) connector was studied by Duan et al. (2022) [22]. A numerical model was used to analyze the dynamic behavior of structures with URSP connectors under seismic loads. The results were compared to traditional structures, revealing that the arrangement of full-span URSP connectors had a significant impact on the system’s behavior, increasing inter-story displacement and causing adverse effects. It was also found that the partial arrangement of URSP connectors had little influence and still maintained good seismic performance. On the other hand, Wang et al. (2022) [23] analyzed the dynamic response of the beam to CFDST column joints for this connection, using established analytical models and pseudo-dynamic tests. The tests explored the effects of end plate type, column hollow ratio, and concrete slab on dynamic responses and failure mechanisms. Seismic behavior, hysteresis curves, viscous damping coefficients, and energy dissipation capacities were also examined, and analytical models of the joints were developed using OpenSees software (https://opensees.berkeley.edu/).

Zhou et al. (2022) [24] conducted experiments on 3D beam to L-shaped steel–concrete column joints subjected to dynamic loading, considering variables, such as encased steel configuration, axial compression ratio, loading angle, and beam type, to provide test data, evaluate seismic behavior, and analyze the effects of key parameters. Additionally, Chen et al. (2023) [25] introduced a new approach to designing PC joints with distinct advantages, including an efficient and eco-friendly assembly method, simple structure, concentrated failure range, and satisfactory seismic performance. The tests were conducted on full-scale joint specimens, considering various factors, like steel tube thickness and internal structure, and analyzed using an ABAQUS (https://www.3ds.com/products/simulia/abaqus) finite element model.

Gan et al. (2019) [26] developed a new joint system for RC beams and square-reinforced concrete-filled steel tube (RCFST) columns. The system utilizes openings on the square hollow section (SHS) for continuous beam reinforcement and interior diaphragms welded to the cold-formed SHS. These diaphragms confine the steel tube and concrete and can be used to position column reinforcement. The system eliminates the need for internal diaphragms to transfer forces from the RC beam, resulting in improved seismic performance. This joint system is shown in Figure 2.

Additionally, Lai et al. (2019) [27] reviewed experimental research on composite special moment frame connections and presented a database of 165 tests conducted on these connections. They summarized connection behavior, evaluated them based on standard requirements, and recommended different connection types for specific frame configurations for seismic applications. Moreover, Fan et al. (2019) [28] examined the mechanical behavior of connections between composite steel–concrete beams and concrete-filled square steel tubular columns. Tests on six specimens under cyclic loading were conducted to evaluate the damage patterns, hysteretic behavior, joint ductility, and energy dissipation capacity. The result of joint geometries, connection details, and loading path on connection behavior were also analyzed.

Wu et al. (2020) [29] analyzed the dynamic behavior of three modular composite joints with various beam–column connections through quasi-static tests. Factors, such as failure mode, strain distribution, hysteresis curve, strength degradation, and energy consumption, were considered. Furthermore, Liu et al. (2020) [30] examined the seismic behavior of steel beams in large-diameter composite column connections. They found that there are no unified design provisions for these connections in high-rise buildings, while the pertinent Chinese code GB 50936–2013 is inadequate for these connections. To investigate the seismic behavior and validate the code-specified calculation method, they tested five connection specimens, examining their energy dissipation stiffness, failure mode, deformation capacity, and ductility.

Amadio et al. (2017) [31] discussed the effects of slab isolation on the structural performance of joints. Properly isolating the slab from the column, even with a small gap, prevents any over-strengthening outcome on the behavior of the joint, improving control of the seismic response of the braced frame. They also found that under lateral loads, the joint acts as a hinge and transfers loads to the bracing system, while under gravitational loads, the continuity of longitudinal rebar leads to a mostly clamped joint performance. Additionally, recent research on composite steel–concrete beam-to-column joints was discussed by Demonceau and Ciutina (2019) [32]. They examined various loading conditions, such as elevated temperatures, combined bending and axial loads, sagging bending moments, and cyclic loading. They also proposed methods to characterize the “concrete slab in compression” component for sagging moments and presented an improved analytical procedure for M-N loading.

2.2. Beam–Column Joints of Composite Frames Under Seismic Loads: Key Findings

The key findings regarding beam–column joints in steel–concrete composite frames subjected to seismic loads are as follows:

• Composite action enhances the strength and stiffness of joints when compared to bare steel joints.
• Proper reinforcement detailing in the joint area is essential for achieving ductile behavior.
• The presence of a concrete slab significantly affects the moment capacity and failure mode of the joint.
• Panel zone deformation has a noteworthy role in the overall rotation of the joint.
• Under cyclic loading, concrete crushing and bond deterioration of reinforcement can occur.
• The shear strength of joints is affected by the depth of the beam and the axial load on the column.
• Incorporating through-beam details can enhance joint performance in comparison to external beam–column connections.
• Composite joints generally demonstrate good energy dissipation capacity when subjected to seismic loading.

3. Seismic Behavior of Steel–Concrete Composite Shear Walls

3.1. Seismic Behavior of Steel–Concrete Composite Shear Walls: State-of-the-Art

Steel–concrete composite shear walls are structural elements that leverage the strengths of both steel and concrete to create efficient lateral force-resisting systems in buildings. These walls are typically composed of steel plates or sections integrated within or attached to reinforced concrete walls. The synergy between the two materials results in a system that offers superior strength, stiffness, and ductility when compared to conventional reinforced concrete or steel-only shear walls. Composite shear walls offer several advantages for seismic loading. They have increased strength and stiffness, improved ductility, better crack control, and can dissipate seismic energy. Additionally, they are lighter and have quicker construction times compared to traditional reinforced concrete walls. The walls also exhibit stable hysteretic behavior, and understanding failure modes is crucial for assessing safety.

Mo et al. (2021) [33] reviewed the behavior of composite shear walls with different connection configurations and loading conditions, highlighting the lack of previous research in the field. They discussed the motivation, structural performance, and development of these important structural elements. Furthermore, Lan et al. (2023) [34] examined a high-rise structure with irregular components that utilizes a steel frame–shear wall system, with the core wall using a double steel plate–concrete composite and the outer frame consisting of steel columns and beams. This composite shear wall was tested under seismic conditions and was proven to meet design requirements, while the structure could withstand progressive collapse caused by removing a corner column.

Todea et al. (2021) [35] investigated the impact of openings, composite connections, and steel fiber-reinforced concrete on the seismic behavior of composite steel–concrete shear walls. They evaluated the performance of these walls, assessed the influence of centrally aligned openings, and explored the use of steel fiber-reinforced concrete as an alternative solution to common concrete. Additionally, innovative composite shear walls with CFST columns and reinforced concrete walls embedded with multiple steel plates were studied by Qiao et al. (2018) [36]. Seven specimens underwent cyclic loading tests to analyze parameters, such as axial force ratio, number of steel plates, and CFT column type. It was found that the embedded steel plates improved seismic behavior and strength. Additionally, recent research by Najm et al. (2022) [37] investigated the seismic performance of steel–concrete composite shear walls compared to traditional RC walls under cyclic loading. Using ANSYS finite element analysis, they examined how reinforcement ratio, concrete strength, shear stud layout, and steel-plate yield stress affect the walls’ behavior, particularly focusing on ductility and energy absorption capabilities. Figure 3 depicts the simulation of local buckling phenomena using the finite element method for the composite steel–concrete shear walls

Rahnavard et al. (2016) [38] presented nonlinear numerical studies on steel–concrete composite shear walls used in high-rise buildings. Five different finite element models were developed to accurately represent the complex behavior of these structures. The findings highlight the importance of parameters, such as concrete failure, hysteresis response, displacement, drift, and energy dissipation. It was observed that a steel frame with concrete on the shear plate on one side exhibited better energy dissipation. Additionally, increasing the concrete thickness reduced shear plate buckling, and decreasing the connector distance increased the energy dissipation. Furthermore, Zhang et al. (2016) [39] proposed an innovative BLC-C composite wall consisting of connected lipped channels welded together. Concrete was poured inside to enhance seismic behavior and reduce steel consumption. The wall system resisted both vertical and lateral loads and was tested for energy dissipation, failure modes, ductility, stiffness, deformation, strength, and hysteresis loops.

Hossain et al. (2016) [40] discussed the behavior of a double-skin composite wall system under cyclic shear loading. They focused on the usage of profiled steel sheets, steel sheet–concrete connections, and high-performance concrete to construct the walls. They have also examined the performance of self-consolidating concrete and engineered cementitious composite as infill materials. The latter was highlighted for its high strain capacity and ability to enhance the structural performance of the walls. They also presented data on stress–strain characteristics, failure modes, ductility, shear strength, and energy absorption, with a focus on strength and stiffness degradation. Moreover, Polat and Bruneau (2017) [41] used the finite element method to model the inelastic cyclic behavior of concrete-filled steel sandwich panel walls. The calibrated model provided insights into the design of these composite walls, addressing the lack of prescriptive guidance. The findings included the distribution of wall-to-footing forces, shear force demands, cumulative plastic strain, steel-plate yielding, and interface friction.

Zhao et al. (2016) [42] first recognized that the research on seismic behavior of steel–concrete composite shear walls for safety-related nuclear facilities lacks reliable hysteretic models for predicting structural performance near collapse. For this reason, they analyzed the experimental results of 32 specimens and developed associated hysteretic rules and a quadrilinear backbone with negative post-peak stiffness. Additionally, to reflect plasticity extension and damage accumulation, they proposed reduction factors for Young’s moduli of concrete and steel. Additionally, Wang et al. (2019) [43] presented a design method for concrete-filled double steel corrugated-plate walls with a T-section. The load-bearing capacities of these walls were upgraded by the combination of infilled concrete and bolt-connected steel corrugated plates. The failure mechanism and design process were analyzed through experiments and numerical simulations, while design formulas for predicting the sectional strength were proposed.

Luo et al. (2021) [44] examined the behavior of corrugated steel-plate composite shear walls. Experimental research was conducted on six shear walls to study their failure process, hysteresis diagrams, energy consumption capacity, and stiffness degradation. It was found that the peak loads of the three shear walls increased significantly compared to conventional shear walls. Moreover, Haghi et al. (2020) [45] developed a fiber-based macro model in the PERFORM-3D program (https://www.csiamerica.com/products/perform3d) to efficiently simulate the global response of steel–concrete composite walls. The model has a simple system and decreases computational time. It was validated using test data for seventeen composite walls with and without boundary elements, considering various design variables and the presence of axial load.

Shafaei et al. (2021) [46] summarized the experimental results and discussed the development of three-dimensional FEM models for large-scale specimens that have to do with composite plate shear walls. Effective stress–strain relationships were proposed to account for various behaviors, including steel yielding, concrete cracking, and composite interaction. Two-dimensional FEM models and fiber-based FEM models were recommended for simulating seismic response, while three-dimensional FEM models were recommended for further parametric studies on structural behavior and design. Additionally, Chen et al. (2015) [47] developed a novel lateral force-resisting system comprising high-strength concrete and a steel plate. Tests were conducted to analyze the response of this composite shear wall to cyclic loading. The axial load ratio and tie bar spacing were investigated. The specimens displayed high strength, deformation capacity, and flexure-dominated behavior. The primary failure mode involved the local buckling of steel plates and compressive crushing of concrete.

An LS-DYNA (https://lsdyna.ansys.com/) finite element model was created by Epackachi et al. (2015) [48] to model the cyclic behavior of flexure-critical steel-plate–concrete composite shear walls. Validation was performed using data from tests on composite wall piers. The damage to the walls included cracking, crushing of infill concrete, and the buckling of steel faceplates. It was found that the model accurately predicted force-displacement responses, damping ratio, and damage distribution. Moreover, Huang and Liew (2016) [49] examined the behavior of composite sandwich walls, composed of two steel plates filled with ultra-lightweight cementitious material. Compression tests on different height composite walls revealed that J-hook connectors provided similar resistance and unloading behavior as overlapped-headed studs. J-hook connectors improved composite action, prevented local buckling, and showed a reasonable correlation with test results when included in predictive methods.

Hu et al. (2014) [50], based on a fiber section analysis approach, developed a program that analyzes the moment–curvature behavior of concrete-filled steel-plate composite shear walls. The program’s accuracy was verified against test results, and a parametric study was conducted on 6379 configurations. Simplified formulas were developed based on the study’s results, which can be used to calculate the drift capacities and ductility of composite shear walls. Furthermore, experimental and numerical analyses were conducted on low shear span ratio dovetailed profiled steel–concrete composite shear walls subjected to axial and cyclic lateral loads by Huang et al. (2022) [51]. The results showed that all specimens failed in a ductile manner with a flexure–shear mixed mode. The lateral stiffness increased with higher thickness ratios, while deformability and energy dissipation capacity decreased. It was found that simplified models accurately estimated the lateral resistance of these composite walls.

Four full-scale experiments were conducted by Wang et al. (2022) [52] to evaluate the seismic performance of double-skin composite walls under earthquakes. The experimental results showed that these specimens had similar damage and flexural failure modes. Additionally, increasing boundary column thickness improved strength but decreased displacement ductility, while increasing the axial compression ratio improved strength but reduced ductility and energy dissipation cycles. Moreover, Shafaei et al. (2021) [46] analyzed the behavior of concrete stiffened steel-plate shear walls with a reinforced concrete panel and a gap between the panel and steel frame. The reinforced concrete panel thickness greatly affects the shear capacity and ultimate strength of these composite walls, prevents elastic buckling, and allows lateral load through shear yield. The results showed that these composite walls and steel-plate shear walls exhibit different behaviors since for the latter type, the steel plate undergoes elastic buckling to resist lateral load. Furthermore, concrete-stiffened steel-plate shear walls offered higher initial elastic stiffness, shear capacity, ultimate strength, ductility ratio, and energy absorption compared to steel-plate shear walls.

Ji et al. (2016) [53] examined the seismic performance of a novel composite wall with encased steel braces. This composite shear wall consists of a steel-braced frame embedded in reinforced concrete, which increases flexural and shear strength. Two wall specimens with different types of encased braces were tested, with both failing similarly with cracked-diagonal cracking and crushing of the concrete. It was found that steel-plate braces could potentially be more efficient and improve construction quality. Formulas for assessing the shear strength of these walls were also proposed. Additionally, Kenarangi et al. (2021) [54] examined the cyclic lateral load behavior of composite plate shear wall/concrete-filled systems for core–wall structures in high-rise construction. Two large-scale walls were tested, investigating their composite behavior and plastic hinge development. The results will aid in the development of design guidelines for high-rise steel buildings with these systems as the primary lateral force-resisting systems.

3.2. Seismic Behavior of Steel–Concrete Composite Shear Walls: Key Findings

The key findings regarding the seismic behavior of steel–concrete composite shear walls are as follows:

• Composite shear walls typically demonstrate enhanced strength and stiffness when compared to conventional reinforced concrete or steel-plate shear walls alone. This is due to the combination of materials, such as steel and concrete, which work together to provide increased structural integrity.
• Furthermore, the use of steel and concrete in composite shear walls often results in improved ductility. This means that the structure is able to endure larger displacements without failure during seismic events. This is crucial for ensuring the safety and resilience of the composite building structures during earthquakes.
• Another advantage of composite shear walls is their ability to dissipate energy. This enhanced energy dissipation capability is essential for seismic resistance, as it helps to absorb and dissolve the energy generated by earthquake forces, plummeting the potential for damage.
• Composite designs also offer the advantage of reduced wall thickness. Compared to traditional reinforced concrete walls, composite designs can often achieve the same level of strength and stiffness with thinner wall sections. This not only saves space but also reduces the amount of material required for construction.
• Additionally, the use of steel elements in composite shear walls can contribute to faster construction. Compared to conventional reinforced concrete systems, the incorporation of steel elements can expedite the construction process, resulting in time and cost savings.
• The presence of steel elements in composite shear walls also improves crack control. Steel helps to control crack formation and propagation in the concrete, enhancing the overall durability and longevity of the structure.
• Composite shear walls generally exhibit improved cyclic performance under cyclic loading conditions, which are representative of seismic actions. This means that they are better able to withstand repeated loading and unloading cycles without significant damage or failure.
• Proper design of composite shear walls can also lead to more predictable and desirable failure modes during extreme seismic events. By carefully considering the design and configuration of the walls, engineers can ensure that the structure behaves in a controlled manner, minimizing the risk of catastrophic failure.
• Furthermore, composite shear walls offer versatility in design. They provide various configuration options, allowing designers to optimize the performance of the walls for specific seismic demands. This flexibility enables engineers to tailor the design to meet the specific requirements of each project.

4. Modeling Techniques for Composite Members and Structures Under Earthquake Excitation

4.1. Modeling Techniques for Composite Members and Structures Under Earthquake Excitation: State-of-the-Art

Accurate simulation of composite members and structures is essential for forecasting performance and guaranteeing safety in seismic analysis. Critical elements encompass material simulation, capturing nonlinear behavior, such as plasticity and cracking, and interface simulation, to depict the interaction between steel and concrete. Member simulation encompasses beams, columns, and floor systems, while joint simulation replicates the behavior of connections. Global structural simulation integrates all elements, while dynamic analysis applies suitable methods. It is also crucial to integrate damping mechanisms and realistic energy dissipation. The range of models varies from simple 2D to complex 3D nonlinear models, depending on design requirements, building complexity, and available computational resources. Thus, a concise and effective analytical simulation for the strength capacity and cyclic behavior of circular CFTs was developed by Serras et al. (2016) [55]. Finite element analysis was used to validate the model and create a database of CFT behavior. Empirical expressions were developed to analyze the Ramberg–Osgood model and to provide a representation of ultimate strength. The proposed comprehensive model reliably captured the cyclic behavior of circular CFT columns.

Sahin et al. (2022) [56] explored the inelastic response of composite steel–concrete beam members, focusing on cyclic degradation phenomena. A continuum simulation was examined to model the hysteretic behavior of concrete slab and composite steel beam assemblages. Parametric assessments were conducted to understand key response characteristics, such as stiffness, capacity, and ductility. The results showed that the deterioration effects depend on various factors, like composite beam depth and steel cross-section slenderness, and that generally, under cyclic loading, composite members show 20% additional deterioration compared to bare steel counterparts. Moreover, Liu et al. (2016) [57] presented a study on high-strength friction-grip bolts as shear connectors for steel–concrete composite beams. A 3D FEM model was constructed to analyze the structural behavior of these beams, including the material nonlinearities and interactions between components. They also explored the differences in mechanical performance compared to traditional headed stud connectors and examined the effects of various factors on beam behavior for design purposes.

Katwal et al. (2018) [58] found that most of the finite element (FE) models simplified complex interactions, limiting their ability to capture failure modes, and for this reason, they developed a detailed FE model for composite beams, considering realistic component interaction and concrete damage. Their model accurately predicted the load–deformation curves and shear force–slip relationships of embedded studs.

Peng et al. (2022) [59] examined the seismic damage assessment process for recycled aggregate concrete-filled square steel tube columns using test results from numerous structures. By considering deformation, energy, and mechanical characteristics, a modified Park–Ang model was proposed, and a performance-based seismic design index as well as damage performance criteria for different failure states were suggested. The damage levels were categorized as intact, slightly damaged, moderately damaged, severely damaged, and collapsed, while by controlling the damage threshold, the assessment basis for different performance levels was established. Moreover, Sahin et al. (2023) [60] modeled and assessed the cyclic behavior of composite members with steel beams and concrete slabs. They proposed nonlinear relationships to simulate their response, showing that degradation is influenced by steel cross-section slenderness and composite beam depth. They also provided important data for seismic design and proposed expressions for computationally efficient frame-level analysis.

El Jisr et al. (2022) [61] presented a macro-model for modeling the response of composite steel beams in completely restrained types of beam-to-column connections. The proposed model accurately captured the asymmetric hysteretic response, cyclic deterioration, and force transfer mechanisms and can be used to assess the seismic collapse risk of composite steel buildings in Europe and to quantify slip demands. The results showed a system over-strength, a low probability of collapse, and that beam–slab connections with partial composite action experienced minimal damage. Additionally, Papavasileiou (2017) [62] introduced a mathematical framework for simulating steel–concrete composite columns using equivalent steel columns. Three simulation methods were presented for circular and rectangular concrete-filled hollow sections and concrete-encased I-shaped sections. The simulation was achieved by satisfying three equations for axial resistance and flexural stiffness and the proposed methods provided the dimensions of equivalent steel sections.

Skalomenos et al. (2015) [63] investigated the seismic performance of planar framed structures made of CFT columns and I steel beams. The impact of modeling details, such as panel zones, beam–column connections, steel I-beams, and composite CFT columns on seismic response, was examined. Their modeling of panel zones of beam–column joints is shown in Figure 4.

Skalomenos et al. (2015) [63] also created fragility curves for three composite frames designed according to European codes, allowing for the selection of the appropriate modeling level for desired seismic behavior. Moreover, Lin and Zhang (2021) [64] highlighted the importance of the slab spatial composite effect in the load-bearing capacity and load resistance of composite frame structures. They modified existing material models in OpenSees (https://opensees.berkeley.edu/) to capture this effect accurately. They found that the conventional fiber model overestimated the probability of the structure being in a specified limit state, while their improved fiber model provided results that are more accurate.

4.2. Modeling Techniques for Composite Members and Structures Under Earthquake Excitation: Key Findings

Some of the key findings regarding the seismic analysis of steel–concrete composite buildings are as follows:

• The seismic response of composite buildings is significantly influenced by the modeling of composite action, which alters the stiffness, strength, and energy dissipation capacity of the structure compared to non-composite systems.
• Accurate modeling of interfaces, such as the steel–concrete interface behavior, is crucial for overall structural performance, including slip and partial interactions.
• Nonlinear behavior, including inelastic deformations in steel and concrete components, as well as their composite action, must be taken into account for realistic seismic analysis.
• The performance of beam–column joints, especially in moment-resisting frames, is greatly influenced by connection details, affecting energy dissipation and overall ductility.
• The modeling of floor diaphragms is important, as composite floor systems affect load distribution and structural dynamics, requiring careful consideration in the modeling process.
• Material degradation due to cyclic loading should be considered, as it can lead to strength and stiffness degradation in both steel and concrete, affecting the seismic response.
• Three-dimensional modeling is often necessary to capture effects, such as torsion and bidirectional loading, which can be significant in composite structures.
• Utilizing sophisticated numerical techniques, like finite element analysis incorporating intricate material models, has the potential to offer precise assessment of the local and global performance of composite structures.
• Future research should focus on creating standardized protocols for assessing the durability of composite structures under different seismic intensities and frequencies.

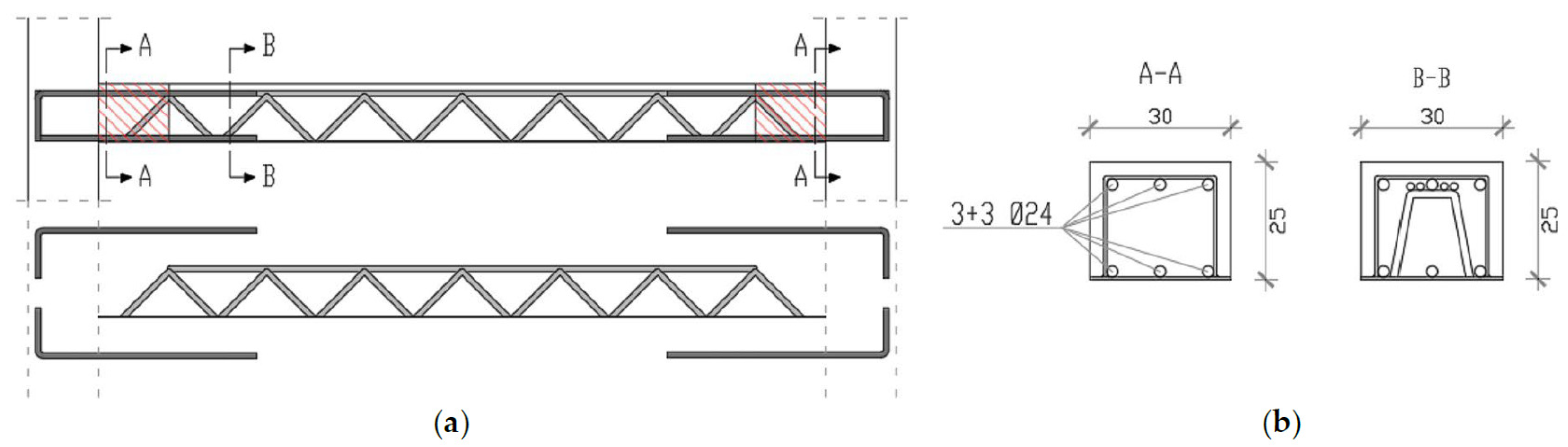
5. Behavior of Composite Beams Under Cyclic Loads

5.1. Behavior of Composite Beams Under Cyclic Loads: State-of-the-Art

Composite beams, which consist of steel beams and concrete slabs, are commonly utilized in the construction industry due to their effectiveness. It is imperative to comprehend how these beams behave when subjected to cyclic loads to ensure safe design practices. Important factors to consider include the transmission of loads via shear connectors, the level of ductility needed for seismic occurrences, the reduction in stiffness over time, the decrease in strength after multiple cycles, the dissipation of energy through inelastic deformation, the role of the concrete slab, the occurrence of local buckling in steel components, and the residual deformations. This knowledge is essential for the development of composite structures that are capable of withstanding seismic forces. Current research is concentrated on enhancing design approaches and connection specifics to improve seismic performance.

Fa-xing et al. (2018) [65] investigated the seismic behavior of steel–concrete composite beams through experiments. Parameters, such as shear connection degree, reinforcement ratio, and section type, were examined. The results showed that composite beams had favorable seismic performance, with a high displacement ductility and damping ratio. Increasing the shear connection, reinforcement, and transverse reinforcement enhanced the bearing and energy dissipation capacity. Transverse reinforcement ratio, shear connection degree, and stud spacing were important considerations. Welding quality and additional reinforcement were advised for practical engineering. Additionally, Han et al. (2015) [66] investigated the bending behavior of large-scale concrete-encased concrete-filled steel tube box members using experimental tests and finite element analysis. Variations in steel tube diameter and sectional height were examined, comparing these composite box members with reinforced concrete box members. A finite element analysis model analyzed the flexural performance and a simplified model for predicting the capacity of composite box members was proposed.

Based on previous tests, Suzuki and Kimura (2019) [67] showed that cyclically loaded stud connections in composite beams degrade faster than in monotonic push-out tests during earthquakes. Then, they used finite element analysis to assess the mechanical performance of the composite connection, considering various factors, such as stud and reinforcement sizes and configurations, material properties, slab widths, and loading protocols. The results were compared with existing equations, and a new mathematical model evaluated stud connection stiffness. Moreover, Zhu et al. (2023) [68] highlighted the application of super-elastic NiTi shape memory alloy (SMA) bolts, and brass-based friction-energy dissipation devices were utilized in self-centering connections. This study involved the cyclic testing of connections between concrete-filled square steel tubular columns and steel beams, showing that the SMA connection exhibited excellent recentering ability and moderate energy dissipation. The pre-strain of the SMA bolt was also modeled, and it was found that its increment improved self-centering capacity, while increasing the pre-tension force in the friction bolts enhanced energy dissipation.

Huang et al. (2014) [69] discussed the behavior of reduced beam section connections with composite beams in steel beam composite frames. A mathematical model was derived, and an amplification factor was proposed to account for the increased potential of the fracture at the beam bottom flange. Parametric analyses were conducted to investigate the effects of beam dimension, reinforcement, and slab dimension. A simplified design formula was proposed based on theoretical data, and the mathematical models were verified with finite element models. Additionally, Di Cesare et al. (2023) [70] conducted experimental and numerical analyses on ductile beam–column connections between composite reinforced concrete truss beams and RC/CFT columns under cyclic loading. This system, entitled the MTR-A beam (see also https://www.metalri.it/en/), is shown in Figure 5. Their study examined two experimental models with different testing schemes, validating the connections’ ductile behavior and demonstrating that the results could be accurately reproduced through simple nonlinear modeling approaches.

Furthermore, Chen et al. (2017) [71] examined partially encased composite beams subjected to monotonic and cyclic loading conditions. More specifically, nine specimens with varying link details, beam lengths, and loading schemes were tested. The results indicated that failure typically occurs through beam flange fracture, local flange buckling, and damage to links and concrete. Moment capacities were higher when specimens experienced combined bending–shear action with an adequate shear span ratio. They also found that Eurocode 4 provides generally reasonable predictions for stiffness but overestimates its initial values. Additionally, El Jisr et al. (2019) [72] presented the construction of a freely available databank on steel beam-to-column connections. They used this databank to offer suggestions for nonlinear performance assessment seismic and design of steel and composite steel–concrete frames, and they concluded that the Eurocode 8-Part 3 overvalues the capacity of plastic rotation for composite beams. Empirical relationships were developed to predict plastic rotation capacity. They also highlighted that the composite steel connections experience higher shear demands in the web panel zone compared to non-composite connections.

Suzuki and Kimura (2021) [73] found that the composite effects of stud shear connectors in composite beams’ design are conventionally measured through push-out tests. However, these tests did not account for the reversed stress on concrete slabs during earthquakes. For this reason, they introduced a component model for composite beams that considers the stress in real structures. Cyclic loading tests on fourteen specimens revealed that the ultimate shear strength was significantly lower under compressive stress. To accurately evaluate performance in composite structures, they presented improved pertinent equations. Furthermore, Suzuki et al. (2023) [74] documented that novel shear connectors, specifically clothoid-shaped connectors, have gained interest in Europe for bridge engineering, although their mechanical performance evaluation has been based on pushout tests, which differ from the stress history experienced during an earthquake. To address this, cyclic loading tests were conducted on 14 specimens, revealing the stress transfer mechanism and confirming the dependence of mechanical capacity on stress orientation. They presented a new formula for evaluating ultimate shear strength and load–displacement relationships, considering the stress history.

An experimental study on concrete-filled tube specimens under cyclic bending loads was conducted by Montuori et al. (2024) [75]. Precise strain measurements using gauges generated load–displacement and moment–curvature curves. A new analytical model incorporating stress–strain relationships for concrete and steel was calibrated to match experimental results. They also provided insights into concrete-filled tube behavior under bending, supporting future modeling and design optimization for engineering applications. Moreover, Kim et al. (2016) [76] introduced a new hybrid composite beam design and assessed its performance through monotonic and cyclic tests. The study fabricated two beam-to-column connection specimens and six bending specimens for testing. The results demonstrated that the beam’s capacity consistently increased with greater beam depth and steel-plate thickness. Additionally, the beam exhibited a satisfactory maximum moment compared to the nominal moment.

5.2. Behavior of Composite Beams Under Cyclic Loads: Key Findings

Some of the key findings regarding the behavior of composite beams under cyclic loads are as follows:

• The beam–column connection performance is essential in composite structures under seismic loads, as these are often critical points for energy dissipation and load transfer.
• Composite beams generally possess excellent energy dissipation capacity under cyclic loading, a crucial factor for seismic performance. This behavior is attributed to the interaction between steel and concrete components.
• Composite beams typically exhibit high ductility when properly designed, allowing for significant deformation without sudden failure. This characteristic is essential for maintaining structural integrity during earthquakes.
• Composite beams may experience stiffness degradation under repeated cyclic loading, often due to concrete cracking, steel yielding, and slip at the steel–concrete interface.
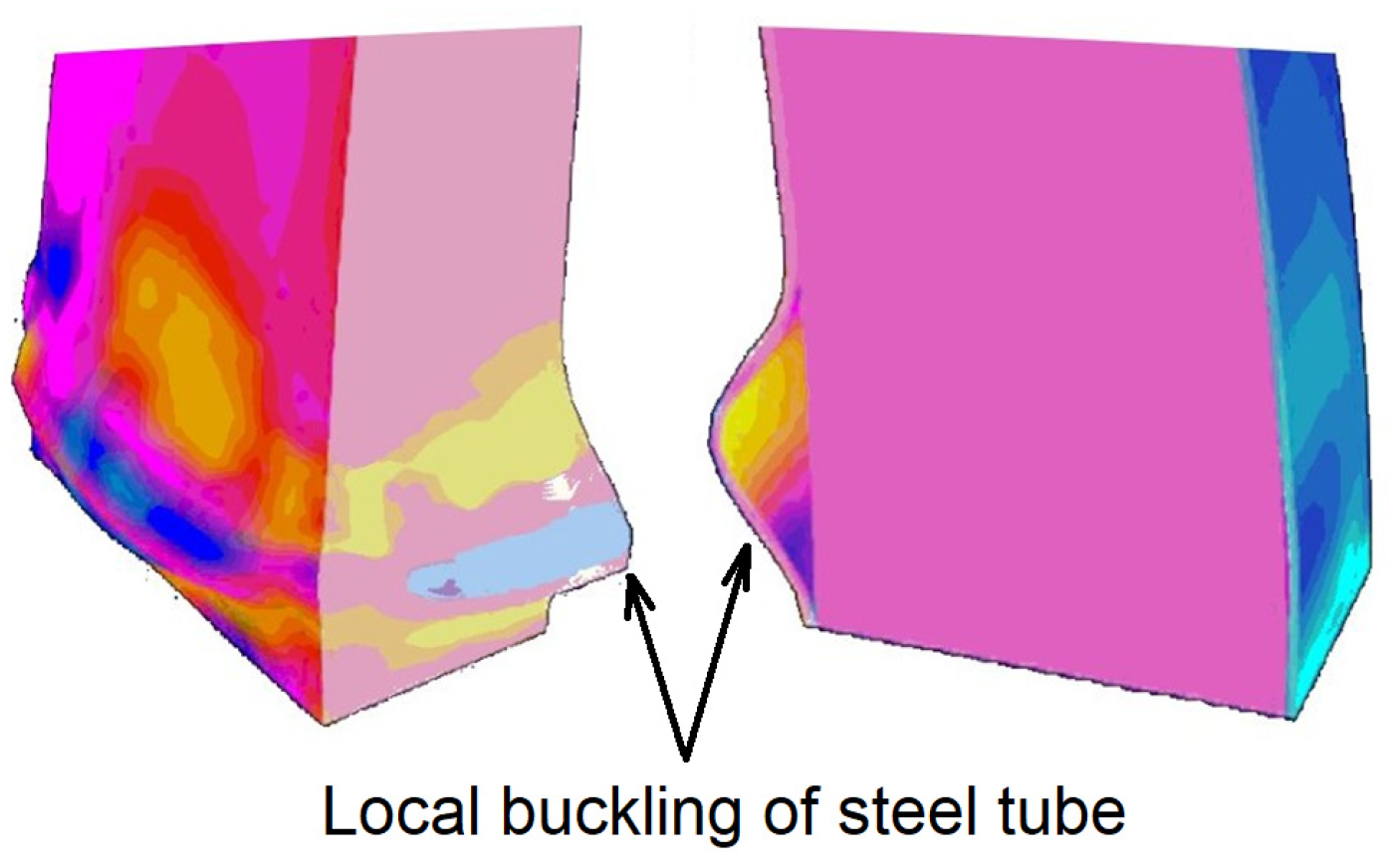
• The strength of composite beams can deteriorate with increasing load cycles, primarily due to local buckling of steel components and concrete crushing.
• The performance of shear connectors, such as headed studs, is critical in composite action. Inadequate shear connection may result in heightened slippage and diminished composite behavior when subjected to cyclic loading.
• Steel components in composite beams, particularly in the beam flanges and web, may experience local buckling under high cyclic loads, affecting the overall performance of the composite beam.
• The concrete slab significantly influences the behavior of composite beams, providing additional stiffness and strength. However, concrete damage and cracking can occur under severe cyclic loading.
• Composite beams may accumulate residual deformations after cyclic loading, which can affect the post-earthquake serviceability of the structure.
• Maintaining composite action throughout cyclic loading is essential for optimal seismic performance. The proper detailing and design of shear connectors are crucial to achieve this.

6. Behavior of Composite Beam-Columns Under Cyclic Loads

6.1. Behavior of Composite Beam-Columns Under Cyclic Loads: State-of-the-Art

Composite beam-columns are widely used in construction due to their strength, stiffness, and ductility. They typically consist of steel sections encased in or filled with concrete. During seismic events, they exhibit complex behavior that is crucial to understand for earthquake-resistant building design. Important features include load transfer mechanisms, ductility, stiffness degradation, strength deterioration, bond–slip behavior, local buckling, and concrete confinement. Understanding these behaviors is crucial for accurately modeling and designing steel–concrete composite buildings to withstand seismic loads. Researchers and engineers use this knowledge to develop design guidelines, analytical models, and performance-based seismic design approaches for composite structures. The current practices and future trends for the behavior of composite beam-columns under cyclic loads are examined in the following section.

Wang et al. (2021) [77] investigated the damage behavior and bearing capacity of circular high-strength CFT columns using quasi-static experimental tests. The moment–axial force relationship was analyzed, and the effects of key parameters were studied to propose a design method. Furthermore, they established a fracture criterion for high-strength steel and a restoring force model. The results showed that steel yield strength, concrete strength, and D/t ratio affected the moment–axial force curves. The proposed model aligned with tested bearing capacity, and the HS steel tube exhibited ductile fracture. Moreover, Qian et al. (2016) [78] discussed the analytical behavior of concrete-encased concrete-filled steel tubular columns during cyclic lateral loading. A finite element analysis model accurately predicted failure modes, load–displacement relationships, and ultimate strength. The study compared the behavior of these composite columns with conventional concrete-filled steel tubular columns and reinforced concrete columns, and a simplified hysteretic model for the Μ–φ relationship of the examined composite columns was proposed.

Tang et al. (2017) [79] conducted low cyclic loading tests on CFT columns filled with normal concrete and recycled aggregate concrete (RAC) to analyze their seismic performance. Findings showed that RAC-filled columns had a similar seismic performance to normal concrete-filled columns. However, RAC-filled columns exhibited better lateral bearing capacity, improved ductility, and slightly lower energy dissipation ability. Similarly, Ma et al. (2022) [80] conducted cyclic loading tests on eleven composite columns filled with RAC to investigate their seismic performance. Factors, such as the diameter-to-thickness ratio of circular steel tube, profile steel ratio, axial compression ratio, replacement percentage of recycled coarse aggregate, and section form of profile steel, were considered. The results showed that increasing the recycled coarse aggregate replacement percentage led to a decrease in ductility and energy dissipation capacity but an initial reduction and subsequent increase in bearing capacity and stiffness. Increasing the wall thickness of the circular steel tube and profile steel ratio improved seismic performance, while increasing the axial compression ratio had a negative effect. Modified formulas for the horizontal bearing capacity were proposed and verified with the test results. In the same way, cyclic load tests were performed on nine steel-reinforced RAC-filled square steel tube composite columns by Ma et al. (2024) [81]. Factors, such as steel ratio, width thickness ratio, recycled coarse aggregate replacement rate, and axial compression ratio were studied. The results showed that columns exhibited a compression-bending failure mode and good energy dissipation capacity. The use of recycled coarse aggregate had minimal impact on seismic performance, and decreasing the width thickness ratio or increasing profile steel ratio improved ductility. The axial compression ratio enhanced the bearing capacity but not the energy dissipation. A modified formula for horizontal bearing capacity was proposed and verified. Similarly, Luo et al. (2022) [82] investigated the behavior of composite columns filled with RAC containing ferronickel slag. Nine specimens were designed and tested, with parameters, such as coarse aggregate replacement ratios, diameter–thickness ratio, and axial load ratios, varied. Damage patterns, hysteresis curves, and other seismic parameters were analyzed. Numerical models were also established and compared with the test results. It was found that the location of damage was in the plastic hinge area, the coarse aggregate replacement ratios had a minimal impact on behavior, the axial load ratio affected ultimate strength and stiffness degradation, and the diameter–thickness ratio improved ultimate strength and ductility. The slenderness ratio, axial load ratio, and steel ratio had a significant effect on strength and ductility, while the RAC strength had a smaller effect. Moreover, Zeng et al. (2020) [83] focused on the behavior of a new composite column that involved a hybrid FRP-recycled aggregate concrete–steel tubular column. This composite column consists of an outer tube made of environmentally friendly basalt fibers and recycled coarse aggregates for the concrete. Quasi-static tests were conducted on five full-scale basalt composite columns to understand their behavior and potential for use in earthquake-prone areas. Various parameters were examined, including RAC replacement percentages, axial load ratios, and steel reinforcement ratios, to determine their influence on the composite columns’ performance. The results showed that RAC replacement percentage has little impact, while axial load ratio significantly affects the seismic performance. These composite columns have better bearing capacity and energy dissipation compared to traditional composite columns with natural aggregate concrete.

The nonlinear response of square concrete-filled steel tubes under axial and flexural loads was studied using computational analysis by Skalomenos et al. (2014) [84]. A reliable finite element model was developed, considering factors, like local buckling, concrete behavior, cyclic softening, and interface interaction, as shown in Figure 6.

Parametric analysis yielded expressions for three hysteretic models. Test results were used to validate these models, which are capable of simulating the response of concrete-filled steel tube columns or composite MRFs to cyclic loading.

Zhang et al. (2019) [85] experimentally examined the behavior of steel tube-reinforced, high-strength concrete columns with high-strength steel bars under cyclic loads. They found that the combination of C90 core concrete with C70 outer concrete provided superior composite effects compared to using C80 concrete for both. Additionally, adding steel fibers to the outer concrete improved ductility and energy dissipation. A calculation model for predicting maximum load-carrying capacity was also proposed and verified. Furthermore, Zheng et al. (2020) [86] investigated the behavior of multi-cell L-shaped concrete-filled steel tubular columns subjected to earthquake loading. Four tests were conducted on columns with different loading angles and axial load levels. The results obtained from experimental tests were compared with finite element analysis. Parametric analysis was also performed to study the effects of different parameters on column performance.

Kim et al. (2022) [87] examined composite columns, featuring a prefabricated steel cage, to enable the rapid construction of large industrial buildings. The cage consists of bolt-connected longitudinal steel angles and transverse steel plates, with integrated steel forms for concrete casting. Through seismic performance tests, it was observed that the steel angles improved flexural strength and stiffness, while the transverse plates reduced spalling and increased deformation capacity. The results aligned with existing models’ predictions. Moreover, a concrete-filled steel tubular column and a metamaterial concrete-filled steel tubular column using rubber, lead, steel, and high load-bearing capacity concrete were designed by Xiong et al. (2024) [88] for vibration mitigation. The flexural bandgap theoretical model based on PWEM and a Timoshenko beam was established and analyzed. Shaking table tests confirmed its effectiveness in reducing vibrations, making it a potential solution for protecting structures from earthquakes and low-frequency lateral vibrations.

Hassan and Farag (2021) [89] recognized that, due to a lack of data, the seismic behavior of steel-reinforced-concrete composite columns having non-seismic details in older buildings is poorly understood. Furthermore, the influence of axial loads on the deformation capacity of steel-reinforced-concrete composite columns is uncertain, and there are no formal guidelines for modeling parameters or acceptance criteria for columns with modern seismic details. For these reasons, they addressed these gaps through experimental and analytical methods, examining these composite columns under cyclic loads. The test results indicate that tension-controlled steel-reinforced-concrete composite columns with non-seismic details meet the seismic assessment criteria, while compression-controlled columns with high axial loads exhibit premature failure. They also proposed new expressions and parameters for assessing steel-reinforced-concrete composite columns with non-seismic details. Mostafa et al. (2019) [90] also discussed the pros and cons for steel-reinforced-concrete composite columns under axial and seismic loads. Additionally, Hassan et al. (2021) [91] developed fragility and resilience functions, and assessed ASCE 41-17, examining the seismic performance of steel-reinforced-concrete composite columns. The methodology used Monte Carlo simulation techniques to establish economic vulnerability functions, and the results showed that ASCE 41-17 criteria underestimate structural capacity and resilience, while overestimate fragility and vulnerability.

Campian et al. (2015) [92] examined the seismic performance of composite steel–concrete columns with steel-encased profiles. It was found that composite columns with high strength concrete experienced an increase in lateral force and maximum lateral loading, while columns with normal strength concrete showed a gradual decline in bearing capacity. HSC columns exhibited a brittle failure mode but have higher energy absorption capacity, making them suitable for seismic areas. Composite columns with class C70/85 concrete demonstrated better structural performance. The fully encased composite column solution is competitive for both seismic and non-seismic zones, offering improved seismic performance and fire protection. Additionally, Chen et al. (2014) [93] examined, under low cyclic reversed loading tests, twenty-six steel–concrete composite columns simulating seismic conditions. An analysis of ductility, energy dissipation, hysteresis loops, and failure patterns highlighted the influence of steel section shape, stirrup ratio, axial compression ratio, and embedded depth ratio on seismic performance.

Chen et al. (2021) [94] conducted an experimental and computational analysis of the seismic performance of fiber-reinforced polymer (FRP)—confined high-strength rectangular CFT columns employing high-strength thin-walled steel tubes and concrete. Aspect ratio, axial compression ratio, number of FRP layers, and fiber direction are among the factors taken into account. Failure modes, hysteretic behaviors, capacity degradation, stiffness degradation, ductility, and stresses were used to assess seismic performance. By raising constraint stress in the effective confinement area, CFRP confinement increased bearing capacity by reducing local buckling and improving the confinement impact of steel tubes on core concrete, according to numerical analysis. Additionally, Gautham and Sahoo (2021) [95] investigated the behavior of structural concrete columns reinforced with steel under combined axial and lateral cyclic loadings. Energy dissipation potential, stiffness degradation, hysteresis response, failure mode, and lateral strength were among the parameters that were assessed in the study. A parametric study was carried out to evaluate the validity of design guidelines from multiple international codes and to predict the flexural capacity of structural steel-reinforced concrete columns under varying axial load levels using the ABAQUS program (https://www.3ds.com/products/simulia/abaqus).

6.2. Behavior of Composite Beam-Columns Under Cyclic Loads: Key Findings

Composite beam-columns have improved ductility, allowing for greater energy dissipation during seismic events. The composite action between steel and concrete results in higher strength and stiffness, improving overall structural performance. Concrete encasement provides confinement, enhancing compressive strength and ductility. The interaction between steel and concrete through shear connectors plays a crucial role in load transfer and overall structural behavior. Under repeated cyclic loading, there may be strength and stiffness degradation, and concrete encasement can help mitigate local buckling of steel sections. The bond between steel and concrete can deteriorate under cyclic loading, affecting overall performance. Composite beam-columns may accumulate residual deformations after severe seismic events, affecting post-earthquake serviceability. Understanding various failure modes (concrete crushing, steel yielding, and shear connector failure) is crucial for proper design. The performance of beam–column connections in composite structures is critical for seismic resistance and energy dissipation.

7. Seismic Performance of Composite Building Structures

7.1. Seismic Performance of Composite Buildings: State-of-the-Art

Considering that steel–concrete composites combine the advantages of both materials and improve seismic resistance, they are common in earthquake-prone areas. These structures consist of steel frames with concrete slabs, composite columns, or composite shear walls. The interaction between steel and concrete components enhances the overall response of the structure. Steel elements provide ductility and energy dissipation capacity, while concrete components enhance stiffness and mass distribution. Composite structures also have higher strength-to-weight ratios. Various factors, like connection design, stiffness and mass distribution, and composite systems, influence seismic performance. Challenges include predicting behavior and considering bonds, concrete cracking, and nonlinear material behavior. Ongoing research aims to optimize seismic performance and create resilient structures.

A displacement/damage-controlled seismic design approach for composite frames—consisting of composite beams and steel tube columns filled with concrete—was created by Serras et al. (2021) [96]. This method directly controls displacement and damage for all seismic performance levels, even near collapse. Empirical expressions were used to estimate the inter-story drift ratio and evaluate the damage index of critical members. By analyzing a large response databank, the necessary expressions were derived. The displacement/damage-controlled method reduced design iterations and eliminated the need for nonlinear time-history analysis. Design examples showed that the method successfully estimated inter-story drift ratio and controlled the damage index, resulting in an improved seismic performance procedure. Thus, Figure 7 shows the response (IDRs, inter-story drift ratios) of a 10-story composite building, using incremental dynamic analysis.

Furthermore, Serras et al. (2017) [97] created a computational process to ascertain how circular steel tube columns filled with concrete would react to constant loading. Experiments were used to validate the creation of precise 3D nonlinear finite element models. A total of 192 CFT specimens with varying diameter-to-thickness ratios, steel tube yield stress, compressive strength of the concrete core, and axial load levels were subjected to a parametric investigation. Empirical expressions were derived from the study to estimate force-displacement behavior. Then, these expressions were applied for the assessment of the seismic performance of composite buildings, examining their behavior through nonlinear time-history analysis. The material models for steel and concrete examined by Serras et al. (2017) [97] are shown in Figure 7.

Bai et al. (2022) [98] examined the impact of strong earthquakes on high-rise steel moment-resisting frames and concrete-filled tubular column frames. Over-design ground motions from large earthquakes can cause severe damage to structural components. The study used synthetic earthquake waves to assess the collapse criteria and deterioration margins of 40-story steel and composite building structures. The results showed that steel building experience collapse mechanisms in lower stories during very rare earthquakes, while the composite frames have a higher margin against overall collapse.

Additionally, the behavior of steel-reinforced concrete structures prestressed by bonding tendons was investigated by Ji et al. (2023) [53]. Fifteen groups of specimens were designed with various parameters. Finite element models were created using ABAQUS software (https://www.3ds.com/products/simulia/abaqus) to analyze the structures under axial forces and horizontal loads. The modeling method was validated by comparing it with the experimental results. The examined composite structures satisfied the strong columns, weak beams principle. The impact of several characteristics, such as ductility, hysteretic curves, skeleton curves, energy dissipation capabilities, and stiffness degradation, on seismic behaviors was investigated.

Wu et al. (2023) [99] found that slip-permitted and uplift-restricted connectors significantly enhanced the anti-cracking capabilities of reinforced concrete slabs. They used numerical analysis and a sophisticated finite element model to analyze the seismic performance of steel–concrete composite frames using these connectors. However, the lack of research has limited their applicability in composite frames. The study emphasized how crucial design elements are for maintaining structural integrity during seismic events, including steel beam height, flange thickness, and connector arrangement length. Furthermore, Zhang et al. (2024) [100] proposed a composite frame with a high-strength steel-plate wall core tube resilient structural system as a new high-performance structural system. It is composed of a core tube with composite frames, interchangeable energy-dissipation coupling beams, and double steel-plate–concrete composite shear walls. They showed how the benefits of this novel system include decreased wall thickness, greater space utilization, enhanced control over the drift ratio, less damage and stiffness degradation, and higher seismic resistance to safeguard people and property.

Taking into account the impact of floor loads, Zhao et al. (2020) [101] examined the seismic behavior of steel–concrete composite frames with large floor slabs. Three load cases were tested on a two-story, two-bay composite frame: pushover, longitudinal cyclic loading, and vertical floor loading. They examined strain distribution, mid-span deflection, and the emergence of cracks in the floor slabs. They also covered force processes of the composite frame, strength and stiffness degradation, load–displacement curves, energy dissipation capacity, and failure events. They also showed that fractures in the welds close to the external joint and the shear lag effect in the wide floor slabs affected the deformation pattern and force mechanism of the composite frame. Additionally, in order to evaluate the seismic risk and calculate the financial damages, Tondini et al. (2018) [102] examined the seismic demand of a steel–concrete composite structure utilizing a high-strength steel moment-resistant frame. Due to the intricate design of the building, a thorough 3D probabilistic seismic demand study that took the angle of the earthquake into account was necessary. Mild steel beams and round, high-strength steel columns filled with concrete made up the construction. There were several incremental dynamic analyses and nonlinear 3D FE models. Taking into account a variety of factors and the randomness of the impact angle, seismic fragility functions were constructed for damage and collapse limit states. The significance of peak ground displacement in the probabilistic model was demonstrated by the results.

Braconi et al. (2015) [103] discussed the results of a performance analysis on various case studies, which are included in a final project report of a research project entitled OPUS. The seismic performance of the structures was assessed using 2D nonlinear models, and the structural behavior and collapse modalities were determined using pushover analysis and incremental dynamic analysis. The study also used the incremental dynamic analysis technique to identify the level of peak ground acceleration that activates collapse, and the Ballio–Setti procedure was modified to account for frequency discrepancies. This allowed for a comparison of the actual q-factor with the design standards. For the same research project, namely OPUS, Badalassi et al. (2017) [104] analyzed the impact of material properties on the seismic performance of steel and steel–concrete structures. Various lateral-resisting systems and steel qualities were considered, with 15 structures designed. An accurate probabilistic procedure was developed to estimate failure probability and a model for the mechanical properties of European structural steel products was calibrated.

The seismic behavior of steel–concrete composite buildings designed with Turkish design codes was investigated by Etli (2022) [105]. Composite moment-resisting frame buildings with different numbers of stories were analyzed at high ductility levels. Nonlinear static and dynamic analyses were conducted to assess the lateral response, over-strength factors, and ductility factors. The examined composite frames exhibited excellent performance, surpassing design expectations. Section deformation capacities were also evaluated during dynamic and static analyses. Similarly, Etli (2023) [106] examined the seismic behavior of steel concrete composite buildings where 5-, 10-, 15-, and 20-story framed structures were designed using concrete-filled steel tube columns. The buildings were designed with high ductility based on Turkish design codes regulations, and nonlinear static pushover and incremental dynamic analyses were applied. The nonlinear analyses showed that the composite framed structures had good performance, with high ductility and the ability to absorb seismic energy through inelastic deformations.

Denavit et al. (2016) [107] examined structural system performance factors, such as strength and ductility for steel–concrete composite moment frames under seismic loads. They used new finite element formulations to analyze the behavior of composite moment frames, where archetype frames were designed according to seismic codes specifications and subjected to nonlinear static pushover and dynamic response history analyses to determine performance factors. Moreover, Zhao et al. (2023) [108] investigated the seismic performance of post-earthquake composite frame structures with varying damage levels. Nonlinear time history analysis was conducted on a ten-story steel–concrete composite frame, using peak ground acceleration (PGA) as a measure of seismic intensity and damage. The structure was subjected to different PGAs in the first stage, resulting in three damage levels. The analysis focused on inter-story drift ratios and plastic hinge ratios, which show a uniform distribution pattern across the damage levels. The structure was deemed safe based on residual inter-story drift ratios. The second stage examined the residual capacity of the structure in strong earthquakes. The results revealed that pre-existing damage could increase lateral deformation and reduce resistance capacity.

The performance of composite frames with circular concrete-filled steel tube columns attached to composite beams made of steel and concrete was studied by Ding et al. (2018) [109] under axial and lateral stresses. The effects of the beam-to-column stiffness ratio, axial compression ratio, and stiffness ratio on seismic performance were examined through the testing of seven frames. Discussions were held regarding the experimental findings, which included ductility, energy dissipation capacity, load–deformation responses, damage development, and stiffness degradation. Additionally, a finite element approach, considering geometrical and material nonlinearity, was developed and validated against the experimental results. Etli and Güneyisi (2021) [110] investigated the performance of steel–concrete composite buildings under earthquake loads with different numbers of stories and different, according to Eurocode 8 regulations, ductility levels. SeismoStruct software (https://seismosoft.com/products/seismostruct/) was used for design and performance assessment, utilizing nonlinear static pushover and incremental dynamic analyses. They analyzed the effects of ductility and the number of stories on the seismic performance of the buildings, finding that all structures had behavior factors higher than the design assumptions, particularly those in the medium ductility class.

Zhao (2016) [111] proposed a simplified approach for analyzing and evaluating the performance of steel and concrete composite frames under extreme loads, such as earthquakes. The study focused on a four-story two-bay underground composite frame. The nonlinear behavior of composite members was characterized to establish a macro-model for the frame. The pushover method was applied to determine the lateral force VS. top horizontal displacement. A method for identifying damage in composite frames was proposed, and the damage evolution of the case study frame was analyzed. The failure mode was attributed to substantial damage in the bottom CFST columns, and the seismic performance of the frame with high-strength steel was compared to that with ordinary strength steel, showing improved lateral resistance and elasticity. Furthermore, the test results of two steel–concrete composite frame specimens with encased CFST columns subjected to cyclic loading were analyzed by Wang et al. (2017) [112] using fiber beam-column elements. Parameters, such as column slenderness ratio, axial compressive ratio, and reinforcement ratio, were studied. Hysteretic models for composite frames were proposed based on parameter analysis, showing favorable energy-dissipating capacity.

Skalomenos et al. (2015) [113] tested a family of 96 regular plane CFT-MRFs using different ground motions to create a response databank. Using regression analysis, they derived simple formulas to estimate the strength reduction factor, ductility demands, drift, and seismic displacements. Several factors, such as the number of stories, stiffness ratio, strength ratio, deformation, and material strengths, were studied. The formulas can be used in seismic design methods and allow for the assessment of existing structures as well as for the design of new ones based on deformation.

7.2. Seismic Performance of Composite Buildings: Key Findings

Examining the seismic performance of steel–concrete composite buildings, these structures appear to have, in comparison with steel or reinforced concrete structures, enhanced ductility, increased stiffness, improved strength, enhanced damping characteristics, effective load transfer, reduced structural weight, enhanced performance of beam–column joints, beneficial composite floor systems, and the importance of proper detailing. Composite buildings exhibit improved energy dissipation, reduced lateral deflections, higher strength capacity, superior damping properties, efficient load transfer, lighter structures, improved behavior under cyclic loading, and effective distribution of lateral loads. Proper detailing, especially in critical areas, is crucial for the seismic performance of these buildings.

The development of comprehensive performance-based design approaches for steel–concrete composite structures in seismic zones represents a critical challenge at the intersection of structural engineering, materials science, and advanced computational modeling. The complexity stems from the multifaceted nature of composite systems, which exhibit intricate material interactions and nonlinear behavioral characteristics under seismic loading conditions. Traditional design methodologies have predominantly relied on prescriptive approaches that often fail to capture the nuanced response of composite structural elements to dynamic loading scenarios [114].

The fundamental limitation of current design frameworks lies in their inability to fully incorporate the inherent variability of material performance and structural response across different seismic intensities and loading configurations. Composite structures present unique challenges due to the differential mechanical properties of steel and concrete, including their distinct elastic moduli, thermal expansion coefficients, and strain compatibility characteristics. These differences generate complex stress transfer mechanisms that are not adequately addressed by conventional linear elastic analysis methods (Duan et al., 2022) [22].

Advanced numerical modeling techniques have emerged as a promising avenue for developing more sophisticated performance-based design approaches. Finite element analysis and multi-scale modeling techniques enable more precise characterization of the nonlinear behavior of composite structural elements. These computational methods allow for more sophisticated predictions of local and global structural responses, including the complex interactions between steel and concrete components under cyclic loading conditions [115]. The integration of machine learning algorithms with advanced numerical modeling has further expanded the potential for developing more adaptive and context-specific design methodologies.

Developing performance-based design guidelines requires a comprehensive understanding of the dynamic response characteristics of composite structures across various scales. This necessitates a holistic approach integrating experimental investigations, advanced numerical modeling, and probabilistic risk assessment techniques. Researchers have increasingly focused on developing hybrid modeling approaches that combine experimental data with sophisticated computational methods to generate robust predictive frameworks (Li and Xu, 2023) [116]. These approaches aim to bridge the existing knowledge gaps in understanding the complex behavior of composite structures under seismic loading.

One of the most significant challenges in developing performance-based design approaches is the characterization of higher mode effects and torsional responses in composite structural systems. Traditional design methodologies often simplify these complex dynamic interactions, potentially leading to significant discrepancies between predicted and actual structural performance. Advanced modal analysis techniques have demonstrated the critical importance of considering multi-modal responses, particularly in tall and irregularly configured composite structures [117,118,119]. These investigations reveal that higher mode effects can significantly influence the overall seismic response, introducing additional complexity beyond fundamental mode considerations.

The implementation of performance-based design approaches requires a fundamental reimagining of design methodologies that move beyond prescriptive code-based requirements. This paradigm shift necessitates the development of more sophisticated performance metrics that can comprehensively evaluate structural response under various seismic loading scenarios [120]. Such metrics must account for not only ultimate limit states but also serviceability requirements, considering various factors, such as residual deformation, energy dissipation capacity, and long-term structural integrity.

Recent advances in sensing and monitoring technologies have opened new avenues for developing more dynamic and adaptive performance-based design approaches. Smart sensor systems and real-time monitoring capabilities enable more precise characterization of structural behavior, providing unprecedented insights into the actual performance of composite structures during seismic events [121,122]. These technologies facilitate a more nuanced understanding of structural response, allowing for more targeted and context-specific design interventions.

The path toward comprehensive performance-based design methodologies for steel–concrete composite structures requires a multidisciplinary approach that integrates advanced computational techniques, experimental investigations, and innovative monitoring technologies. Future research must focus on developing more sophisticated modeling frameworks that can capture the complex material and structural interactions unique to composite systems [123]. This will necessitate continued investment in advanced computational resources, experimental facilities, and interdisciplinary research collaborations that can address the multifaceted challenges inherent in the seismic design of composite structures [124].

7.3. Contextual Variability in the Seismic Performance of Composite Structures

The complexity of seismic performance in steel–concrete composite structures necessitates a critical examination of the inherent variability across different geological, geographical, and structural contexts [108]. While this review synthesizes comprehensive research findings, it is paramount to acknowledge that the behavioral characteristics of composite structures are profoundly influenced by a multitude of site-specific factors that cannot be universally generalized. Geological conditions, including ground motion characteristics, soil properties, and local seismic hazard profiles, play a crucial role in determining structural response that extends beyond standardized design parameters [125]. Regional variations in construction practices, material quality, and local building codes introduce additional layers of complexity that significantly affect the seismic performance of composite systems [126]. For instance, the same structural design may exhibit markedly different responses in regions with varying tectonic environments, such as subduction zones versus transform fault boundaries. Furthermore, micro-level variations in material composition, fabrication techniques, and construction quality can introduce substantial uncertainties that are challenging to predict through generalized analytical models [127]. The interaction between structural geometry, connection detailing, and local seismic loading conditions creates a dynamic system where small variations can lead to significantly divergent performance outcomes [128]. These contextual nuances underscore the importance of site-specific investigations and the limitations of broad, generalized conclusions. Researchers and practitioners must approach composite structural design with a nuanced understanding that recognizes the inherent complexity and site-specific nature of seismic performance. This approach demands a more sophisticated methodology that integrates local geological data, advanced numerical modeling, and comprehensive experimental validation to develop truly robust and context-sensitive design strategies for steel–concrete composite structures in seismic regions [110].

8. Composite Construction and Foundation Seismic Design

8.1. Composite Construction and Foundation Seismic Design: State-of-the-Art

Seismic design of foundations for steel–concrete composite buildings is crucial for ensuring safety during earthquakes. The process involves analyzing the dynamic response of the structure, considering factors, like frequency and resonance. Deep foundations, like piles or caissons, may be used to transfer loads to stable soil layers. Measures, like base isolation and energy dissipation devices, help mitigate ground motion effects. The stiffness and strength of the foundation are important for overall seismic performance. Design codes and standards provide guidelines, emphasizing capacity design principles to ensure plastic deformations occur in predetermined locations. Overall, a comprehensive understanding of soil–structure interaction, material behavior, and dynamic analysis techniques is necessary for creating resilient structures that can withstand seismic events.

Abbas et al. (2021) [129] developed two column-to-foundation connections for square CFT columns. Experimental and numerical assessments were conducted, comparing the connections with existing ones for their inelastic deformation capacity when subjected to cyclic loading. The results demonstrate the efficient and improved behavior of the suggested connections, with the numerical models accurately predicting the experimental response. Similarly, Khateeb et al. (2020) [130] introduced two new and efficient connections for connecting concrete-filled steel tube (CFST) columns to reinforced concrete foundations. The connections were designed to withstand seismic loading and were tested against conventional CFT and reinforced concrete column-to-foundation connections. The results showed that the proposed connections performed better. Finite element models accurately predicted the behavior of these connections, as confirmed by the experimental results.

Serras et al. (2021) [131] examined the use of concrete-filled steel tube piles in deep foundation systems under seismic and cyclic loads. They found that composite piles effectively mitigate damage in hard-to-reach areas, such as the pile heads and deep depths. Controlled loading analyses and seismic-intensity analysis confirmed the capacity margins of the composite pile system. They also discussed the damage patterns, displacement profiles, and residual displacement of composite piles compared to concrete piles. The results showed that composite piles exhibited 40% less damage on average than reinforced concrete piles. Moreover, Zhou et al. (2015) [132] examined the mechanical properties of reinforced concrete and steel–concrete composite members under seismic loads, specifically in the context of elevated pile-group foundations used in bridge and ocean engineering. Four scale-specimens were created and tested, comparing their seismic performance. The results showed that the composite specimens had higher peak strength, higher ultimate displacement, and higher energy dissipation capability than the reinforced concrete specimens.

Wang et al. (2020) [133] focused on steel-plate composite walls and their connections to foundations. Eight large-scale specimens were tested to examine the behavior of composite wall-to-foundation connections under axial compression and cyclic lateral force. The specimens were tested in different loading directions and using different connection construction details and aspect ratios. The results showed that the lap splice connection was stronger, while the embedding connection allowed for more ductility. The current expressions for load-carrying capacities were found to be underestimated. Similarly, Vakili Sadeghi et al. (2022) [134] demonstrated how a baseplate and eccentric anchors could be used to attach steel-plate composite walls to a concrete basemat. Direct force transfer from the wall to the anchors was made possible by the connection of the anchors to the bottom of the faceplates, which eliminated the baseplate from the force transmission chain. LS-Dyna software (https://lsdyna.ansys.com/) was used to verify three test walls. It was discovered that the split-baseplate connection with concentric anchors was more resilient than the other connections. Kurt et al. (2016) [135] examined the direct shear behavior of rebar-coupler anchor systems used in nuclear reactors to secure steel-plate composite walls to the concrete basemat. The anchor specimens were subjected to extensive testing up to failure. The results showed the load–slip displacement responses, direct shear strength, and failure mode. It was found that the American Concrete Institute (ACI) 349 code equation underestimated the shear strength because it assumed that failure occurred in the rebars, while in reality, it happened in the couplers. An updated design equation using the net shear area of the couplers improved the accuracy of calculating shear strength. An empirical model for the shear force vs. slip displacement response of rebar-coupler anchor systems was also proposed with the use of experimental data. Analytical models were developed by Siddiqui et al. (2024) [136] to determine the minimum depths required for connecting circular and square concrete-filled steel tubular columns. The pullout capacity of the reinforced concrete footing, which was reliant on the strength of the footing concrete, reinforcement, and cement grout, was equivalent to the pullout strength of the tube in the models. These models were used to calculate the optimal depths for composite columns using the Monte Carlo simulation technique. The second connecting scheme showed significant improvements over the first scheme, with 37.3% and 45.2% reduced embedment depth for circular and square composite columns, respectively. Additionally, to address concrete-filled steel tube column damage during seismic load, Feng et al. (2024) [137] suggested a rubberized concrete-filled corrugated steel tube composite column–foundation connection. Experimental studies confirmed improved ductility and energy dissipation. The results showed sufficient connection strength under seismic load and proposed seismic design methods using a side shear database to inform future column–foundation connections.

Li et al. (2020) [138] examined the structural behavior of double-pile foundations under cyclic loads. The effects of inclination angles and the embedment depths of unequal-height concrete-filled steel tube piles on failure modes, ductility, stiffness, strength, and energy dissipation capacity were evaluated. Battered piles increase loading capacity and energy dissipation but decrease deformation capacity and ductility. Unequal heights of battered composite piles can lead to premature failure of the shorter pile. Unequal heights also decreased the deformation capacity of the foundation. Won et al. (2020) [139] examined a steel composite hollow reinforced concrete column, characterized by an inner tube within the hollow section, which required innovative connecting techniques for the column–footing joint segment. They introduced three novel connecting methods, which were assessed through experimental and finite element analysis investigations. Subsequently, upon identification of an effective connecting method for column footing joints, it was implemented in large-scale test specimens to validate and assess its performance.

An analysis of composite pile performance was conducted by Thusoo et al. (2021) [140], comprising a comprehensive dataset of 79 bending tests, which facilitated the comparison of observed bending moment capacities against theoretical predictions derived from various design guidelines. However, the assessment of composite pile behavior necessitates consideration of drift capacity predictions for a more thorough evaluation. To address this requirement, researchers developed and validated a computationally optimized fiber-based model suitable for nonlinear static analysis. This analytical framework incorporated the primary failure mechanisms characteristic of composite piles, specifically addressing concrete compressive failure and steel casing local buckling phenomena. Furthermore, Stephens et al. (2016) [141] focused on the seismic behavior of the embedded ring concrete-filled steel tube connections, which allows for accelerated bridge construction and sustains minimal damage during large inelastic deformations caused by earthquakes. Practical design expressions and a design example were provided for implementing the research findings. Furthermore, Wang et al. (2024) [142] focused on the seismic performance of reinforced thin-walled irregular steel tube concrete frame structures after a fire, considering soil–structure interaction. Finite element models were established using ABAQUS software (https://www.3ds.com/products/simulia/abaqus). The analysis evaluated the effects of site conditions and fire duration on various structural parameters. The results showed that soil–structure interaction increased the natural vibration period by 10–30%. The acceleration for structures on softer soil with longer fire durations was higher compared to assuming a rigid foundation. Inter-story shear force and inter-story displacement angle also increased with soil–structure interaction, especially with longer fire duration, larger seismic wave amplitude, and softer soil.

8.2. Composite Construction and Foundation Seismic Design: Key Findings

The seismic design of steel–concrete composite building foundations requires consideration of soil–structure interaction. Adequate stiffness and strength in the foundation system prevent excessive deformation. Proper connection between the superstructure and foundation is crucial for effective load transfer. Foundation type should align with site conditions and building characteristics. Energy dissipation mechanisms reduce seismic demands. Composite action enhances overall performance but requires attention to connection details. Nonlinear behavior of both foundation and superstructure should be considered, and performance-based design approaches can be used to optimize foundations in highly seismic regions.

9. Seismic Isolation and Energy Dissipation Devices for Composite Structures

9.1. Seismic Isolation and Energy Dissipation Devices for Composite Structures: State-of-the-Art

Two common strategies employed to improve the performance of steel–concrete composite buildings and minimize their damage during seismic events are seismic isolation and supplementary dampers. Seismic isolation entails the introduction of a flexible layer between the composite building and its foundation to isolate the structure from ground motion. On the other hand, supplementary dampers work by dissipating energy during seismic events, thereby reducing acceleration and displacement demands on composite structural elements. The combination of these techniques offers numerous benefits for steel–concrete composite buildings, including enhanced strength, ductility, and flexibility. Extensive studies, simulations, and practical applications have demonstrated the effectiveness of seismic isolation and supplementary dampers. By optimizing the integration of these systems, engineers can create resilient and secure composite structures capable of withstanding seismic loads and improving overall performance in earthquake-prone areas.

Darwish and Bhandari (2022) [143] examined the effectiveness of high-rise composite buildings with base isolation in reducing seismic response during earthquakes. Two base-isolated steel–concrete buildings with twelve- and fifteen-story levels were analyzed, using lead rubber-bearing isolators for base isolation. Composite base-isolated buildings were compared to fixed-base composite and reinforced concrete counterparts. Seismic responses were evaluated using the response spectrum method for the highest seismic zone according to the Indian Code. The results showed that base isolation with lead rubber-bearing isolators reduces overall seismic responses of composite buildings by 50–60%. The 15-story building demonstrated higher effectiveness. Base-isolated composite buildings had approximately 50% less story drift and displacements compared to reinforced concrete buildings. Similarly, a comparative analysis of the seismic responses of three different building types, namely reinforced concrete buildings, fixed-base composite buildings, and base-isolated composite buildings using lead rubber-bearing base isolators, was investigated by Darwish and Bhandari (2022) [144]. They indicated that the integrated application of base isolation and composite building in base-isolated composite buildings results in superior overall performance compared to reinforced concrete and fixed-base composite buildings, particularly in three-, six-, and nine-story buildings. Furthermore, the study revealed that fixed-base composite buildings outperform reinforced concrete buildings across all three levels of stories, highlighting the suitability of base-isolated composite buildings for high-rise, mid-rise, and low-rise buildings.

Li and Fa (2023) [145] investigated alternative dissipative systems in composite steel–concrete frame structures. An experiment evaluated the mechanical properties of three plate flanges with different parameters and power factors under various cyclic loadings. The examined systems demonstrated high resistance and the ability to dissipate seismic vibration energy. Similarly, Kanyilmaz et al. (2019) [118] evaluated the effectiveness of a standard multi-story building with a steel–concrete composite frame designed with and without structural fuses using nonlinear transient dynamic analysis. Numerical models were created using a distributed plasticity approach. These models were adjusted based on experimental data from the literature. The study quantified global response parameters including energy dissipation, base shear, and inter-story drifts.

Li et al. (2021) [146] developed a protective system for composite buildings using buckling-restrained braces (BRBs) and viscous dampers (VDs) to address the combined threat of earthquakes and winds. They investigated the effectiveness and design parameters of these devices by employing the fragility function method. The results showed that the hybrid-damped frame (HDF) is effective in withstanding multiple hazards, and that the energy dissipation contributions of VDs and BRBs vary based on the hazard intensities. Zhuang et al. (2022) [147] experimentally studied the seismic behavior of eccentrically braced composite frames with vertical shear links. The use of low-yield-point steel in the shear links was found to enhance seismic performance. The eccentric bracing system increased the stiffness, strength, and energy dissipation capacity of the composite frame without affecting force conditions on primary beams and columns. However, strain hardening of the low-yield-point steel resulted in severe damage to the concrete slab in the middle segment of the primary beam. Similarly, Javaid and Verma (2023) [148] evaluated the impact of buckling-restrained braces and viscous dampers on the seismic performance of asymmetrical composite frames. Both devices effectively reduced seismic response, with viscous dampers being more efficient in reducing period and base shear by 65–73% and 80–90%, respectively. Buckling-restrained braces performed better in reducing maximum overturning moments. Implementing both devices reduced inter-story drift ratio and horizontal displacement, but increased compression force on columns by 20–25% for buckling-restrained braces and 15–22% for viscous dampers. Viscous dampers were found to be more effective.

Guo and Wang (2023) [149] examined a dual system consisting of a double-skin CFT frame and metallic dampers. The energy distribution along the height was analyzed using nonlinear models validated by test results. They found that the metallic damper absorbed significant energy under seismic loads, whereas a semi-rigid connection harmed the column. A method to estimate energy distribution in the dual system was developed, providing valuable insights for future applications.

Zhuang and Zhao (2022) [150] analyzed the seismic performance of an eccentrically braced composite frame with a low-yield-point steel shear link. A theoretical and finite element model is used to understand the structural mechanics and energy dissipation mechanisms. Various design parameters were investigated to determine their impact on the system’s mechanical properties. Additionally, recommended design parameter values were provided.

Furthermore, Thakur and Tiwary (2023) [151] examined the seismic response of composite structures integrated with fluid viscous dampers and base isolation. They evaluated the seismic performance of composite structures using finite element analysis. The results showed that integrating fluid viscous dampers and base isolation reduces the structural response to seismic events. A synergistic combination of fluid viscous dampers and base isolation outperforms their individual use.

A new type of shear panel dampers with bent web panels, installed in composite frames to enhance stiffness and strength was examined by Zhao et al. (2022) [152]. Unlike traditional dampers, BSPDs minimize bending moments on the primary beam, reducing damage to the concrete slab. Experimental and numerical investigations confirmed their ductile behavior and energy dissipation capacities. Design recommendations included using low-yield-point steel and link flanges to increase deformation capacity and efficiency.

Li et al. (2020) [153] presented analytical studies and experiments on blind-bolted end plate CFT composite frames with buckling-restrained braces. Pseudo-dynamic tests on two 2/3-scaled two-story composite frames with buckling-restrained braces showed good hysteretic behavior and high ductility. The moment capacity, rotation capacity, and mathematical model of a composite joint were analyzed and modified. The shear force–deformation relation of a CFT panel zone was checked. A macroscopic finite element model showed that buckling-restrained braces provide lateral stiffness and resistance under small earthquakes and dissipate energy under severe earthquakes. This model is part of the OpenSees program (https://opensees.berkeley.edu/).

Kastimpini et al. (2024) [154] conducted a comprehensive investigation of a cable-stayed bridge featuring concrete-filled steel tube pylons and an innovative seesaw system for seismic protection. The geometry of the problem is shown in Figure 8. Through detailed finite element modeling and extensive parametric studies, they evaluated the system’s effectiveness in mitigating seismic demands under various earthquake scenarios. Their analysis incorporated soil–structure interaction effects across different soil conditions and foundation types, demonstrating the system’s capacity to reduce deck displacements, pylon base shear, and cable forces. Furthermore, Katsimpini (2025) [155] investigated the seismic performance of two-, four-, and six-story composite buildings with viscous wall dampers using concrete-filled steel tubular columns and steel beams. Their work assessed dampers’ effectiveness through nonlinear time history analyses. The results showed reduced inter-story drift ratios and peak floor accelerations, validating the dampers’ integration in mid-rise structures. The behavior and bearing capacity of composite members were based on Ref. [156]. Furthermore, the influence of multiple earthquakes and soil flexibility on the structural response have been examined in Ref. [157]. The results show that multiple ground motions increase demands compared to single events. Incorporating soil–structure interaction reduces drift and accelerations but increases displacements.

Javaid and Verma (2023) [158] examined the effectiveness of buckling-restrained braces and viscous dampers in enhancing the seismic performance of composite buildings during earthquakes. Steel–concrete composite frames were analyzed with and without these devices. The results showed that viscous dampers were more efficient, especially when placed in center bays for regular buildings and corner bays for C- and L-shaped composite buildings, reducing period, maximum story displacement, base shear, and maximum inter-story drift significantly, and making them a great option for mid-rise composite buildings.

9.2. Seismic Isolation and Energy Dissipation Devices for Composite Structures: Key Findings

Seismic isolation and supplementary dampers enhance the performance of steel–concrete composite buildings during earthquakes. They reduce structural damage and improve occupant safety by decoupling the composite building’s movement from ground motion and dissipating seismic energy. Base isolation systems elongate the composite building’s natural period, decreasing inter-story drifts, floor accelerations, and structural demand. Supplementary dampers provide additional energy dissipation capacity. The combination of isolation and dampers offers synergistic benefits, leading to cost savings. Composite buildings with these systems exhibit improved ductility, energy dissipation, and seismic resilience. Design considerations include the interface between isolated and non-isolated portions and the proper integration of dampers. Analysis and design are crucial to maximize the benefits for each project.

10. Progressive Collapse Resistance of Composite Structures

10.1. Progressive Collapse Resistance of Composite Structures: State-of-the-Art

Progressive collapse in composite structures, especially steel–concrete composite buildings under seismic loads, is a complex phenomenon that has received considerable attention in structural engineering. This form of collapse occurs when localized damage triggers a chain reaction of failures, potentially leading to disproportionate and catastrophic structural collapse. The interaction between steel and concrete elements in composite buildings plays a crucial role in their seismic performance. During earthquakes, these structures experience intense lateral forces and dynamic loading, which can cause steel members to yield and the concrete to crack or be crushed. The composite action between steel and concrete has the potential to improve overall structural ductility and energy dissipation capacity, thereby potentially reducing the risk of progressive collapse. However, the behavior of connections between composite elements, load redistribution mechanisms, and the potential for sudden failure of key structural components are critical factors that must be carefully considered in the design and assessment of these buildings to ensure their resilience against progressive collapse under seismic conditions.

Zandonini et al. (2019) [159] focused on steel–concrete composite frames in the event of column loss. Two geometrically distinct three-dimensional composite full-scale substructures were selected from reference buildings and tested to simulate the column collapse scenario. They outlined the preparatory studies, the main features of the specimens, and the results of the initial test. The test provided insight into the need for an improved design of joints and emphasized key aspects of the response of the floor system. Additionally, static tests on six two-story three-span composite frames with CFST columns were conducted by Zheng et al. (2022) [160] under a penultimate column removal scenario. The effects of weld-bolted connection and RC slab on progressive collapse resistance were analyzed. The results showed that the torsion in steel beams at the directly affected region was reduced for frames with composite beams. Differences in failure modes were observed between the penultimate and middle-column removal scenarios. It was also found that frames with weld-bolted connection had lower resistance but multiple protection measures against progressive collapse.

Zheng et al. (2022) [161] initially presented a fiber-based model that incorporates nonlinear beam-column elements and zero-length elements to account for the composite relationship between the slab and beam, as well as the material’s damage evolution for composite members. Then, the suggested modeling method was applied to an anti-collapse analysis of composite frames with various connections. Additionally, design suggestions obtained through parameter analysis were provided to prevent the development of a chain reaction. Furthermore, a multi-scale model was used by Wang et al. (2017) [162] to study the collapse performance of CFT column-to-steel beam connections. Nonlinear static and dynamic analysis methods were used to reveal resistance mechanisms, failure modes, and stress distribution of joints. The results showed that these joints could prevent progressive collapse by forming resistance mechanisms and providing alternate load paths. The adjacent framework also enhanced the anti-collapse ability of the joints.

Papavasileiou and Pnevmatikos (2017) [163] examined the use of steel cables for retrofitting steel–concrete composite buildings to prevent progressive collapse. They also investigated the impact of the building’s characteristics on the overall cost of retrofitting to identify the best cost-effective approach for each scenario. To achieve this, an optimization algorithm known as Evolution Strategies was used to determine the solution that offers the desired performance at the lowest cost. Furthermore, Wang and Li (2023) [164], examining composite structures consisting of CFT columns and H-shaped steel beams, compared six beam–column connections for collapse resistance during a middle-column loss scenario. While the connections showed minimal differences under normal conditions, they vary greatly under extreme loads. A design concept to improve collapse capacity was proposed and validated through finite element analysis. Characteristic results using ABAQUS software (https://www.3ds.com/products/simulia/abaqus) are shown in Figure 9.

Bai et al. (2017) [165] discussed the seismic collapse capacity for the case of high-rise CFT moment-resisting frames subjected to extreme earthquakes beyond design levels. Ground motions with a flat velocity spectral shape were selected to minimize record-to-record uncertainty. A numerical approach using fiber elements with stiffness and strength degradation in stress–strain models was developed. Incremental dynamic analyses were conducted to assess the P-Delta and degradation effects on local and global collapse mechanisms. The results showed drift concentration at lower stories triggering side-sway collapse controlled by post-buckling strength deterioration of CFT columns. Moreover, Wang et al. (2020) [23] concentrated on the ability of composite building structures to withstand accidental loads, such as earthquakes, fires, or explosions, by depending on the beam and catenary mechanisms of steel beams to prevent collapse. They also examined a concrete-filled square steel tubular column attached to a steel beam with a bolted–welded hybrid joint in a scenario where the central column was eliminated. They computed the collapse resistance and mechanism under vertical loads, demonstrating that the steel beam can still provide resistance even after the failure of a short-span beam. They recommended the utilization of a welded haunch joint to enhance collapse resistance, offering valuable insights for engineering design to avert progressive collapse.

A numerical simulation method was proposed by Zheng and Wang (2022) [166] to integrate multi-scale element modeling with simplified beams and columns, reducing modeling elements and increasing computing efficiency. This method allowed for the accurate simulation of material damage and fractures in critical areas. Testing on CFST column composite beam frames showed that the new simplified multi-scale modeling approach was efficient and accurate. Different column-loss scenarios and the number of stories were studied to improve the composite frame’s resistance to progressive collapse. Two improvement alternatives were proposed and analyzed to strengthen the frame’s capacity. Wang et al. (2021) [167] simulated the hysteretic behavior on a single-story, single-span frame with concrete-filled steel tube columns and prestressed concrete-encased steel beams. Parameters for stiffness degradation, strength degradation, and pinching behavior were determined. An analytical model was developed for out-jacketing frames with single-span, multi-story layouts. Seismic response analysis was conducted for different seismic fortification levels, with the results showing that certain frames needed improvements for seismic resistance. The mechanical behavior and collapse potential of out-jacketing frames were determined from the findings of the nonlinear time-history analysis, leading to the development of pertinent seismic design recommendations.

10.2. Progressive Collapse Resistance of Composite Structures: Key Findings

Seismic loads pose a significant risk for progressive collapse in steel–concrete composite structures, making it a critical concern in structural engineering. Research has demonstrated that composite buildings generally outperform traditional steel or concrete structures due to their increased stiffness and strength. However, the interaction between steel and concrete components can result in intricate failure mechanisms. It has been found that proper detailing of connections, particularly beam-to-column joints, is essential for preventing localized failures that could initiate progressive collapse. The utilization of composite action can greatly enhance the overall system ductility and energy dissipation capacity, thereby reducing the likelihood of unbalanced failure. Furthermore, recent studies have emphasized the importance of considering dynamic amplification effects during seismic events, as they can exacerbate the potential for progressive collapse. Moreover, incorporating redundancy and alternate load paths in the design process has been shown to bolster the resilience of composite structures against progressive collapse under seismic loads.

11. Optimal Design Strategies for Earthquake-Resistant Composite Buildings

11.1. Optimal Design Strategies for Earthquake-Resistant Composite Buildings: State-of-the-Art

Achieving the optimal design of earthquake-resistant composite buildings involves a complex interplay of structural engineering principles, material science, and seismic analysis. Strategies for designing cost-effective steel–concrete composite structures aim to strike a balance between safety, performance, and economic efficiency. These strategies typically focus on optimizing the distribution of steel and concrete elements to maximize strength and ductility while minimizing material usage. Advanced analysis techniques, including nonlinear dynamic analysis and performance-based design, are often utilized to evaluate and enhance the seismic response of composite buildings. The ultimate goal is to create structures that can withstand earthquake forces while maintaining occupant safety and minimizing structural damage, all within reasonable cost constraints.

Papavasileiou et al. (2020) [168] compared the cost-effectiveness of three seismic retrofit methods for framed structures with composite columns that did not meet building codes. The first two methods involved strengthening individual composite columns with reinforced concrete jackets or steel cages. The third method upgraded the building frame by adding steel bracings. A specialized optimization procedure was used to minimize retrofit material costs while meeting the design requirements. Thirty cases of under-designed composite buildings were analyzed, revealing the most cost-effective retrofit approach for different conditions. Similarly, Papavasileiou and Charmpis (2020) [169] examined the cost-effectiveness of seismic buildings with pure steel or steel–concrete columns using a structural optimization process for unbiased comparison. An Evolution Strategies algorithm minimized material cost while meeting safety provisions from Eurocodes 4 and 3 and considering seismic behavior. The results from 154 optimization runs suggested the benefits of using concrete to partially replace steel in column designs for earthquake-resistant structures.

Moreover, Papavasileiou and Charmpis (2016) [170] focused on optimizing the design of earthquake-resistant multi-story composite buildings with steel–concrete columns. The composite columns consist of steel members fully encased in concrete, along with steel beams and optional steel bracings. The goal was to minimize materials costs while meeting design code constraints, including Eurocodes. The optimization procedure included constraints on member capacity, inter-story drifts, and top-story displacements, as well as limits on the fundamental period. The Evolution Strategies algorithm was used to solve the optimization problem and was linked with structural analysis software for evaluation purposes.

Lin and Zhang (2023) [171] introduced a practical method for life-cycle cost analysis for composite structures, combining the economic loss rate with probabilistic seismic demand modeling. A framework using a multi-objective cuckoo search algorithm was proposed for seismic design optimization. An eight-story composite frame prototype was used to compare design alternatives. The over-strength factor was identified as critical for construction, seismic damage, and life-cycle costs. Improved fiber models were developed to evaluate spatial composite effect on designs using the OpenSees software (https://opensees.berkeley.edu/). It was found that certain design parameters, like over-strength factor and flexibility, significantly affected costs.

Moreover, Kamaris et al. (2016) [172] developed a seismic optimization framework for composite structures through an extensive parametric study of steel–concrete composite moment-resisting frames with steel I-beams and CFT columns. Based on thousands of nonlinear dynamic analyses under various ground motions, they established empirical expressions for estimating maximum seismic damage using four damage indices. Their optimization process considered multiple parameters, including story count, beam strength ratio, and material properties, to develop efficient damage prediction formulas. The optimization procedure is shown in Figure 10.

Kaveh et al. (2022) [173] introduced a performance-based optimization design for steel–concrete composite MRFs (moment-resisting frames) using a Chebyshev chaotic map-based optimization algorithm. The study focused on 8-story and 20-story frames to minimize total weight and enhance seismic performance. The process included obtaining top designs, nonlinear pushover analysis, fragility curve plotting, and damage-margin ratio calculation to identify the optimal design for each frame, highlighting improved efficiency in weight reduction and seismic behavior assessment.

11.2. Optimal Design Strategies for Earthquake-Resistant Composite Buildings: Key Findings

Recent investigations have indicated that optimized composite systems frequently outperform traditional single-material structures in both cost-effectiveness and seismic resistance. The strategic arrangement of steel and concrete, in conjunction with innovative connection designs, can significantly enhance a building’s ability to dissipate energy during earthquakes. Additionally, research has underscored the importance of considering the entire life cycle of the composite structure, including construction, maintenance, and potential retrofitting costs, in the optimization process. These findings highlight the potential for composite structures to offer superior seismic performance while achieving cost savings, making them an attractive option for earthquake-prone areas.

12. Seismic Performance of Steel–Concrete Composite Bridges

12.1. Seismic Performance of Steel–Concrete Composite Bridges: State-of-the-Art

Composite bridges, which integrate steel and concrete elements, have gained popularity in modern infrastructure due to their effectiveness and adaptability. These bridges capitalize on the advantages of both materials, with steel providing tensile strength and concrete offering compressive resistance. When exposed to seismic loads, composite bridges display intricate behavior that necessitates thorough analysis and design considerations. The interaction between steel and concrete components under dynamic loading can result in distinct stress distributions and energy dissipation mechanisms. Factors, such as shear connectors, deck–girder interactions, and material nonlinearities, significantly influence the overall seismic performance of these bridges. Understanding the behavior of composite structures during earthquake conditions is crucial for developing resilient design approaches and ensuring the safety of bridge infrastructure in seismically active areas. Ongoing research in this field aims to enhance analytical models, refine experimental techniques, and improve design guidelines to optimize the seismic performance of composite bridges.

Lin et al. (2020) [174] introduced a composite rigid-frame bridge that combines a steel–concrete composite box girder and concrete-filled double-skin steel tube piers with rigid connecting joints, showing improved static and dynamic performance over traditional bridges. Shake table tests of a scale model were conducted to assess seismic behavior and damage patterns. The results identified damage mainly at the upper and lower ends of the composite piers, with enhanced responses under near-fault ground motions. Numerical modeling confirmed the bridge’s superior seismic performance, particularly in near-fault earthquakes. Furthermore, Paolacci et al. (2018) [175] examined short-to-medium-span composite I-girder bridges, which are gaining popularity due to their reduced construction time and costs, as well as their suitability for seismic areas. The cyclic behavior of pier-to-deck joints using concrete crossbeams was analyzed and experimental tests showed good seismic performance of the joints, with different typologies tested.

Zhou et al. (2020) [176] examined the behavior of dumbbell steel tube-confined reinforced concrete piers. These composite piers, which are shown in Figure 11, enhance strength and ductility compared to traditional reinforced concrete piers. Five composite piers and one reference dumbbell-reinforced concrete (RC) pier under pseudo-static loading revealed improved performance due to the confinement and enhancement effect of the steel tube. Seismic performance slightly improved with higher axial load ratios and wider webs. A simplified model accurately predicted lateral resistance, showing promising results for improving the performance of RC piers.

Zhou et al. (2022) [177] introduced an enhanced type of concrete-encased column connections for circular CFT piers. They found that the new design eliminated the need for baseplates and anchor bolts. Experimental testing on four concrete-encased column connection specimens showed good seismic performance, with flexural failure and high-energy dissipation capacity. Calculations for strength degradation and load transfer mechanisms matched the test results well. Furthermore, Du et al. (2023) [178] examined the behavior of posttensioned precast segmental bridge piers under three-directional movements. They used shaking table tests to study an innovative posttensioned precast segmental pier under tri-directional ground motions and their results showed that adding more prestressing tendons or energy-dissipating bars reduced displacement responses. Piers with energy-dissipating bars resisted twisting better than those without. They also compared the effects of vertical ground motion on seismic behavior, finding a significant impact on compressive force and shear resistance.

Gu et al. (2024) [179] delved into the seismic performance and potential applications of continuous beam bridges with prefabricated concrete-filled steel tubular piers. Initially, two prototype bridges were compared, one with 10 m double-column reinforced concrete piers and another with 35 m double-column reinforced concrete piers. Then, seven bridges were designed with prefabricated concrete-filled steel tube piers to match the lateral stiffness of the reinforced concrete piers. The hysteretic characteristics of composite piers were verified through numerical models and seismic response analysis. Fragility curves were generated through time-history analyses.

Xiang et al. (2023) [180] proposed a fiber-based nonlinear modeling approach for the seismic analysis of partially concrete-filled steel tubular bridge piers, addressing computation time and convergence issues by considering probable local buckling of the outer steel tube and efficiently captured composite pier behavior. Comparing numerical modeling with experimental data showed the effectiveness of the fiber models in seismic assessments. Thorough cyclic pushover and time-history analyses highlighted the importance of coordinating steel tube and concrete design parameters to improve seismic performance. A new design approach involving layered concrete filling was recommended for enhancing structural seismic performance and was especially useful for retrofitting existing hollow steel piers without increasing foundation forces. Furthermore, a prefabricated steel tube-confined concrete circular pier with a grouted sleeve connection was developed by Fu et al. (2022) [181]. This composite pier could address limitations in typical precast concrete piers, enhancing their use in seismic hazard zones. Scale models of circular piers were tested under cyclic loading, showing tube-confined concrete circular piers had improved flexural strength and energy dissipation due to steel tube confinement. Ductility and stiffness also increased, with a more even distribution of pier body stiffness. Parametric analysis revealed thicker and stronger steel tubes increased flexural strength and stiffness.

Li et al. (2023) [182] examining hollow bridge piers with concrete-filled double-skin tubular sections evaluated their seismic design and cost-effectiveness. The results showed that these composite piers had higher displacement ductility, lateral force-bearing capacity, cost-performance ratio, and flexural stiffness with reduced residual drift ratio. Certain factors, such as the diameter-to-thickness ratio of outer steel tubes and hollow ratio, significantly influenced the seismic performance of composite piers and recommendations include a diameter-to-thickness ratio between 60 and 150 for effective and ductile seismic design of composite bridge piers. Similarly, Li et al. (2023) [183] executed experimental tests comparing posttensioned precast segmental concrete-filled double-skin steel tubular piers with cast-in-place concrete-filled double-skin steel tubular piers, showing superior ductility, energy dissipation, and minimal damage to precast segments. The numerical analysis explored design parameters’ impact on pier behavior, revealing the crucial roles of energy-dissipating bars, gravity loads, and prestressing loads in energy dissipation and self-centering capacities. Initial stress levels in prestressing strands chiefly influenced failure mode and deformation capacity. Lin et al. (2023) [184] investigated the seismic behavior of steel–concrete composite rigid-frame bridges with posttensioned precast segmental concrete-filled double-skin steel tube piers under across-fault ground motions. Figure 12 depicts the positional relationship between the bridge and the seismic faults.

A numerical model was developed using LS-Dyna software (https://lsdyna.ansys.com/), validated by previous tests. Two types of ground motions were considered, with several fling steps. The study compared the damages, joint deformations, energy absorption, and seismic responses of the bridges and found that during strike-slip and dip-slip across-fault ground motions, posttensioned precast segmental piers sustained less damage compared to monolithic piers. Monolithic piers exhibited damage along the entire pier, while posttensioned precast segmental piers experienced localized damage at segment-to-segment joints. Under transverse strike-slip ground motions, posttensioned precast segmental piers showed excellent self-centering performance with minimal residual lateral deformations. Posttensioned precast segmental piers could accommodate displacements through torsion slip at joints and showed better seismic resilience and post-earthquake recovery performance compared to monolithic piers.

A new type of pier has been developed by Yan et al. (2024) [185] using a composite material made of glass fiber-reinforced polymer, steel, and rubber concrete, featuring a double-skin design. Two 1:4-scale specimens were created for an investigation, where the first specimen underwent a mixed loading test involving pseudo-dynamic loading and quasi-static loading. This loading simulated the phenomenon where, after an earthquake, it is common to experience multiple aftershocks, emphasizing the need to assess the seismic performance of newly constructed composite piers. On the other hand, the second specimen was exclusively subjected to a quasi-static test. It is also found that composite piers exhibited elastic behavior in frequent seismic events, with glass fiber fracture and steel tube yielding in infrequent events. Despite seismic damage, specimens still showed energy dissipation, capacity, and ductility. A correction coefficient was proposed for calculating the plastic hinge length due to the underestimation of the plastic deformation capacity in existing specifications.

Zeng et al. (2024) [186] proposed a design method for normal strength and ultra-high performance concrete composite bridge piers, optimizing ultra-high-performance concrete jacket thickness and pier diameter. They suggested that the jacket thickness should be 0.2–0.3 times the pier radius, while the composite pier diameter should be 0.8 times that of an equivalent reinforced concrete pier. The fragility analysis showed that composite piers reduced damage probabilities under small-to-moderate earthquakes across all damage states. For strong earthquakes, they effectively controlled extensive damage but were less effective for slight and moderate damage levels. Similarly, Zeng et al. (2023) [187] investigated ultra-high-performance concrete and normal strength concrete composite bridge piers under cyclic loading, finding that the ultra-high-performance concrete cover effectively prevented compressive crushing and enhanced load-carrying capacity compared to conventional reinforced concrete piers. The composite piers generally failed due to reinforcement fracturing at the bottom. Cracks developed quickly in the ultra-high-performance concrete cover, indicating a need for reinforcement. The ultra-high-performance concrete cover significantly increased the balanced axial load of the composite section, influencing failure modes and load-carrying mechanisms. An optimal axial load ratio of 0.1–0.15 was recommended for composite piers to balance performance and cost-effectiveness.

Wu et al. (2018) [188] examined the shear strength of reinforced concrete bridge piers embedded with steel tubes and braces. By conducting tests on seven specimens, they found that including steel tubes and braces greatly improves the shear resistance and flexibility of the piers compared to conventional reinforced concrete. The combined piers exhibited a higher capacity to bear loads, better distribution of cracks, and more gradual failure patterns. A mathematical model was formulated to estimate the shear strength, and it demonstrated a close correlation with the results obtained from experiments.

12.2. Seismic Performance of Steel–Concrete Composite Bridges: Key Findings

The behavior of composite bridges under seismic loads is complex due to the interaction between steel and concrete elements. Generally, these bridges outperform traditional ones by offering increased strength, ductility, and energy dissipation capabilities. The composite act between concrete and steel enhances the overall stiffness of the structure and reduces deformations, resulting in better seismic resistance. Concrete-filled steel tube piers are known for their excellent ductility and energy absorption, which help to minimize earthquake damage. When designing composite bridges for seismic regions, the focus is on capacity design principles to ensure the formation of plastic hinges in designated areas while maintaining elastic behavior elsewhere. Proper detailing of connections between steel and concrete components is essential to prevent premature failure. Advanced analysis techniques, such as pushover analysis and time-history analysis, are necessary for accurately predicting the seismic response of composite bridges and optimizing their design for enhanced earthquake resilience.

13. Smart Materials and Sensors

Recent advancements in smart materials and sensing technologies offer promising opportunities to enhance the seismic performance of steel–concrete composite structures [189]. Smart materials, such as shape memory alloys and magnetorheological fluids, can provide adaptive responses to seismic loads, while modern sensors enable real-time monitoring of structural behavior during earthquakes [190]. These technologies can potentially revolutionize how composite structures respond to and recover from seismic events. When integrated with traditional composite construction methods, smart materials, and sensors can facilitate more resilient structural systems, offering capabilities, such as self-centering, enhanced energy dissipation, and immediate post-earthquake damage assessment (Shen et al., 2024) [191]. The implementation of these innovations represents a significant step toward developing more intelligent and responsive seismic-resistant structures.

Current research demonstrates the potential of piezoelectric materials, fiber optic sensors, and wireless sensor networks in revolutionizing structural health monitoring for composite structures. Zhang et al. (2018) [192] found that these systems can detect and quantify damage in real-time, enabling prompt post-earthquake assessment and informed decision-making regarding building occupancy and required repairs. Chen et al. (2020) [193] found that embedded sensors could monitor key parameters, such as strain, displacement, and acceleration, providing valuable data for both immediate safety evaluations and long-term performance analysis. The integration of these sensing technologies with machine learning algorithms further enhances their capability to predict structural behavior and identify potential vulnerabilities before they become critical (Hu, 2015) [194].

Zhang et al. (2018) [195] examined the internal concrete damage in L-shaped concrete-filled steel tube (L-CFST) columns using piezo-ceramic smart aggregates under low-frequency cyclic loading. Wavelet packet analysis established the damage index. Experimental results showed the feasibility of using smart aggregates to monitor concrete damage in L-CFST columns.

Zhang et al. (2021) [196] found that the practical implementation of smart materials in composite structures has shown promising results in experimental studies. Chen et al. (2015) [197] reviewed a variety of smart materials’ connection details to concrete-filled rectangular tubular (CFRT) columns that different researchers have developed. Shape memory alloys incorporated into beam–column connections have demonstrated superior energy dissipation and self-centering capabilities compared to conventional solutions. Thus, Hayashi et al. (2018) [198] and Zhong (2023) [199] examined the application of self-centering principles on steel–concrete composite structures, where they found that the self-centering composite frames have reduced permanent deformations while the initial posttensioned forces can control their uplifting force.

Magnetorheological dampers have been successfully employed to provide adaptive damping in response to varying seismic intensities. However, challenges remain in scaling these technologies for widespread application, including cost considerations, long-term durability, and the need for standardized design guidelines [200]. Addressing these obstacles requires collaborative efforts between researchers, practitioners, and regulatory bodies to develop comprehensive frameworks for the effective integration of smart technologies into composite structural systems [201].

14. Towards Next-Generation Composite Structural Systems: Research Perspectives

14.1. Emerging Vibration Protection Strategies

Recent developments in friction dampers and smart controllers offer intriguing insights into advanced structural protection mechanisms. Velychkovych et al. [202] proposed a novel friction damper utilizing an open shell with a helical cut and a deformable filler. This design leverages the bending effects of the shell and frictional interactions to achieve superior damping characteristics. Velychkovych et al. [202] predicted potential applications in earthquake-resistant structures, particularly in the energy and construction industries.

Smart controllers are emerging as powerful tools for vibration assessment and mitigation. A notable example is the Smart 4 controller, which demonstrates remarkable capabilities in monitoring vibration loads [203]. In drilling applications, this technology has shown potential for real-time vibration assessment, enabling operators to adjust drilling modes and prevent potential equipment damage. While initially developed for industrial contexts, such smart monitoring approaches hold promise for structural health monitoring in seismic-sensitive environments.

The development of shell-shock absorbers presents another innovative approach to vibration management. These devices, characterized by parallel spring and friction modules, exhibit exceptional damping properties under high operational loads [204]. The unique design allows for energy dissipation through structural hysteresis, a mechanism that could be particularly relevant in seismic engineering applications.

Furthermore, advanced modeling techniques are enhancing our understanding of vibration protection mechanisms. Dutkiewicz et al. [205] have developed sophisticated mechanical–mathematical models that can predict the behavior of damping systems under various loading conditions. These models, which analyzed contact interactions and energy dissipation, provide valuable insights into the design and optimization of vibration mitigation technologies.

While the aforementioned innovations represent exciting developments in vibration protection, it should be acknowledged that further research is necessary to fully integrate such technologies into steel–concrete composite structural systems. The potential for interdisciplinary collaboration between materials science, mechanical engineering, and structural design remains significant.

14.2. 3D Printing Techniques

Three-dimensional printing techniques represent a revolutionary approach in composite structural engineering, offering unprecedented opportunities for complex geometrical configurations and optimized material distributions. Advanced additive manufacturing methods enable the creation of intricate structural components with precisely controlled material properties, potentially transforming traditional fabrication limitations in steel–concrete composite systems. Current research is exploring multi-material printing strategies that can integrate metallic and cementitious materials with enhanced microstructural characteristics, allowing for localized property modulation and improved load transfer mechanisms [206,207,208]. Computational design optimization coupled with 3D printing technologies permits the development of novel connection geometries that could significantly enhance seismic energy dissipation capabilities [209,210,211,212]. Preliminary experimental studies suggest that 3D-printed composite connections might offer superior ductility and strength characteristics compared to conventional fabrication methods, though extensive validation is required [213,214,215]. The primary research challenges include achieving consistent material interfaces, ensuring long-term structural integrity, and developing standardized manufacturing protocols that meet rigorous engineering performance criteria [216,217,218].

14.3. Self-Healing Material Technologies

Self-healing material technologies present a transformative potential for enhancing the resilience and durability of composite structural systems, particularly in seismically active regions [219,220,221,222]. Innovative approaches involving embedded healing agents, bacterial precipitation, and advanced polymer-based autonomous repair mechanisms offer promising strategies for mitigating progressive damage accumulation during cyclic loading conditions [223,224,225]. Experimental investigations have demonstrated that strategically incorporated healing agents can effectively restore material integrity by autonomously sealing microcracks and preventing potential structural degradation [226,227]. Emerging research focuses on developing sophisticated self-healing concrete formulations that can respond dynamically to mechanical stress, potentially extending the service life of critical structural components [228,229]. The integration of nanoengineered healing capsules, biological agents, and responsive polymeric networks allows for targeted healing interventions at the microstructural level [230,231].

Despite significant technological promise, substantial research challenges remain, including long-term performance validation, the scalability of healing mechanisms, and a comprehensive understanding of healing efficiency under complex loading scenarios, especially for the case of steel–concrete composite structures under seismic loading conditions.

14.4. Machine Learning-Based Models

Recent advancements in machine learning have demonstrated significant potential for predicting the seismic performance of steel–concrete composite structures [1,2,3,4,5,6]. Tang et al. [79] utilized a random forest with firefly algorithm optimization to estimate the seismic performance of recycled aggregate concrete-filled steel tube columns, achieving high correlation coefficients. Zhang et al. [150] developed ensemble machine learning models for bond stress estimation, employing Bayesian optimization and SHAP interpretability techniques to enhance predictive accuracy. Li et al. [15] introduced a multi-indicator percussion method integrated with machine learning, achieving an exceptional 99.6% accuracy in interfacial debonding detection using key damage indices. Guo et al. [149] applied an extremely randomized trees algorithm to analyze damage sensitivity in steel–concrete composite beam bridges, identifying microcracks in steel beams as the most critical performance factor. Li et al. [15] developed a backpropagation neural network for predicting high-strength bolt connector strengths, achieving an over 93% goodness of fit. Yang et al. [6] proposed a novel blending fusion model with generative adversarial network augmentation for identifying failure modes of steel tube-reinforced concrete shear walls, improving prediction accuracy by an average of 13–81% across various metrics.

In conclusion, machine learning-based approaches represent a transformative paradigm in seismic performance prediction, offering unprecedented accuracy, interpretability, and insights into the complex behavior of steel–concrete composite structures under extreme loading conditions.

15. Conclusions and Future Research Directions

This comprehensive review has explored the seismic performance of steel–concrete composite structures through multiple perspectives, from component-level behavior to system-wide response strategies. The synthesis of current research reveals both significant advances and critical research gaps that warrant further investigation.

Properly detailed beam–column joints demonstrate excellent seismic performance, though complex stress distributions at interfaces remain challenging to predict. Future research should develop standardized connection details optimized for varying seismic hazard levels. For composite beams and columns, concrete significantly improves energy dissipation and delays local buckling, with the research needs focused on quantifying long-term performance under repeated seismic events. Composite shear walls offer superior lateral load resistance compared to conventional systems. System-level investigations confirm that properly designed composite structures create redundancy and alternative load paths enhancing structural resilience. Future research should address the optimization of steel–concrete proportions for different building heights and integrate performance-based methodologies with practical construction considerations.

Modeling techniques have advanced significantly, though computational models still struggle to fully represent composite action at interfaces. Future work should develop efficient models that accurately represent material interface deterioration under cyclic loading, potentially integrating machine learning with physics-based approaches.

For foundations and bridges, composite construction enhances seismic resistance with improved load transfer characteristics and ductility. Future research should investigate soil–structure interaction under extreme events and develop optimized connection details.

Progressive collapse studies confirm that composite structures possess inherent robustness against disproportionate collapse. Seismic isolation and energy dissipation devices demonstrate significant reductions in seismic demands when integrated with composite structures. Research should develop adaptive systems responsive to varying earthquake intensities.

Optimization strategies now effectively balance material efficiency with seismic objectives, while emerging smart materials offer promising directions for adaptive structural response. Future work should incorporate life-cycle considerations and explore integration of monitoring systems with traditional composite structures.

In conclusion, while substantial progress has been made, significant challenges remain in developing design methodologies that bridge theoretical advancements with practical implementation to enhance the structural resilience of steel–concrete composite structures in seismic regions.