Size Effect on the Strength Behavior of Cohesionless Soil Under Triaxial Stress State
Abstract
1. Introduction
2. Experimental Schemes
2.1. Experimental Materials
2.2. Specimen Preparation
2.3. Experimental Equipment and Procedure, Experimental Process
3. Results
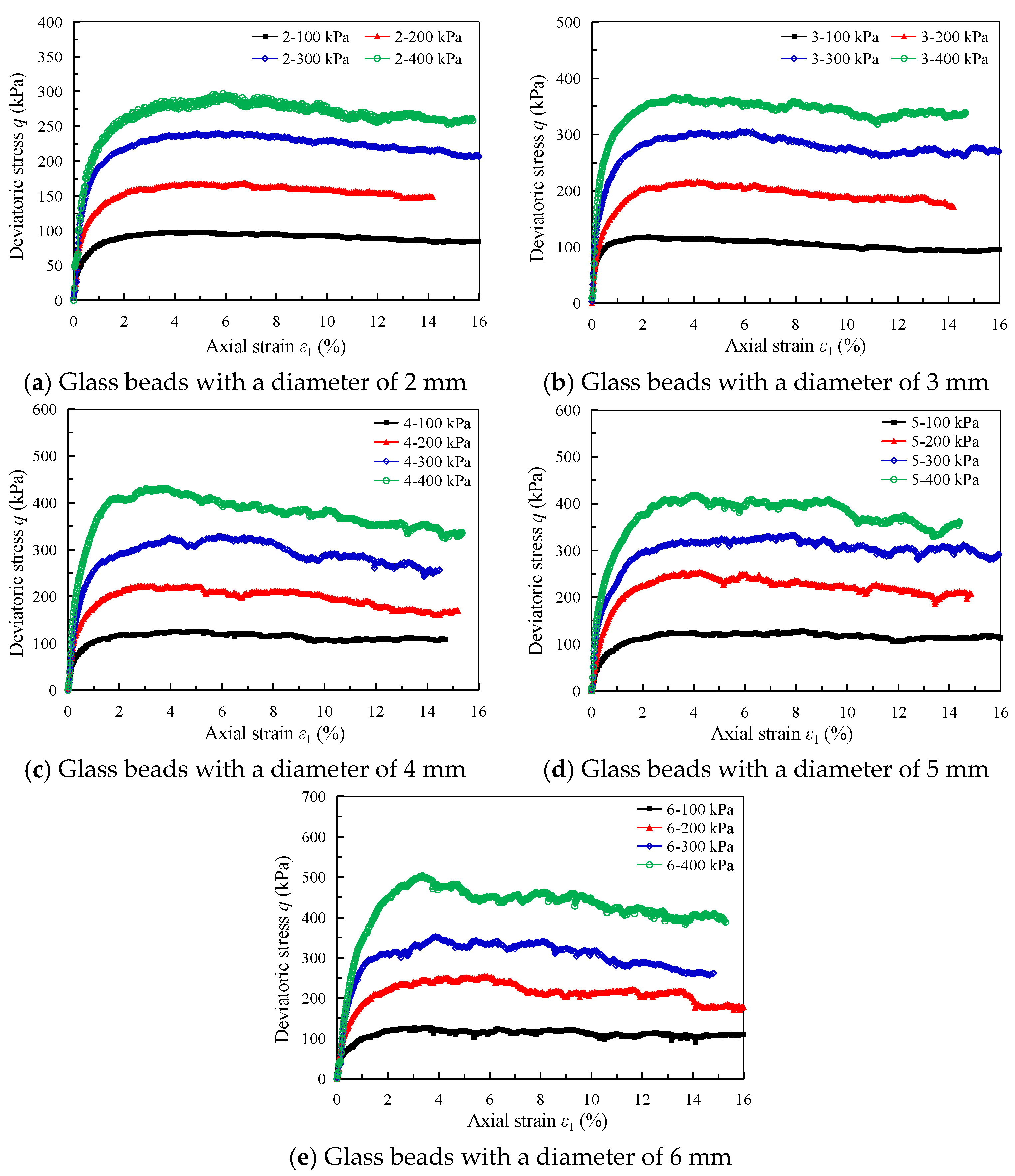
3.1. Test Results and Analyses of Small-Sized Granular Materials
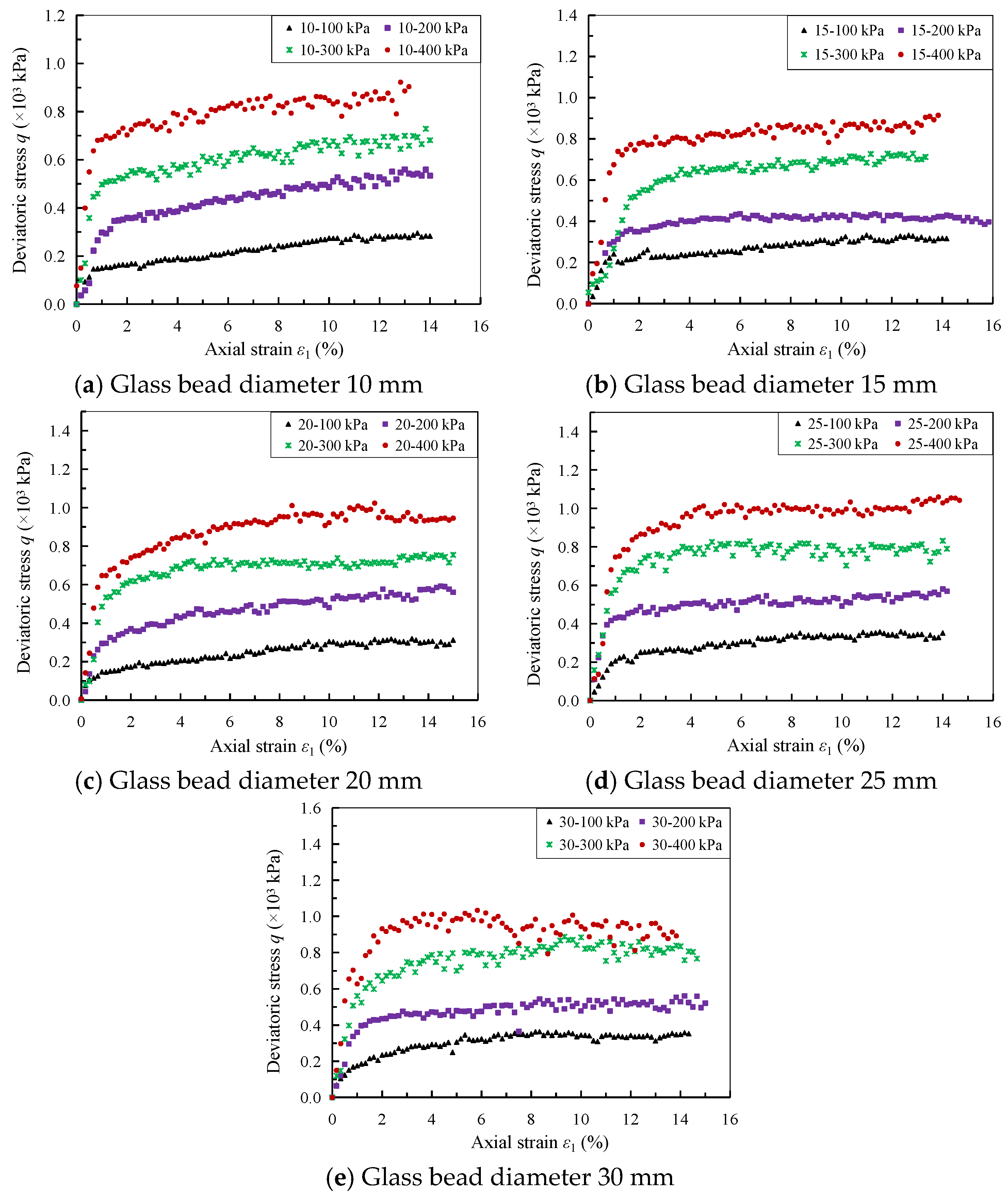
3.1.1. Stress-Strain Characterization
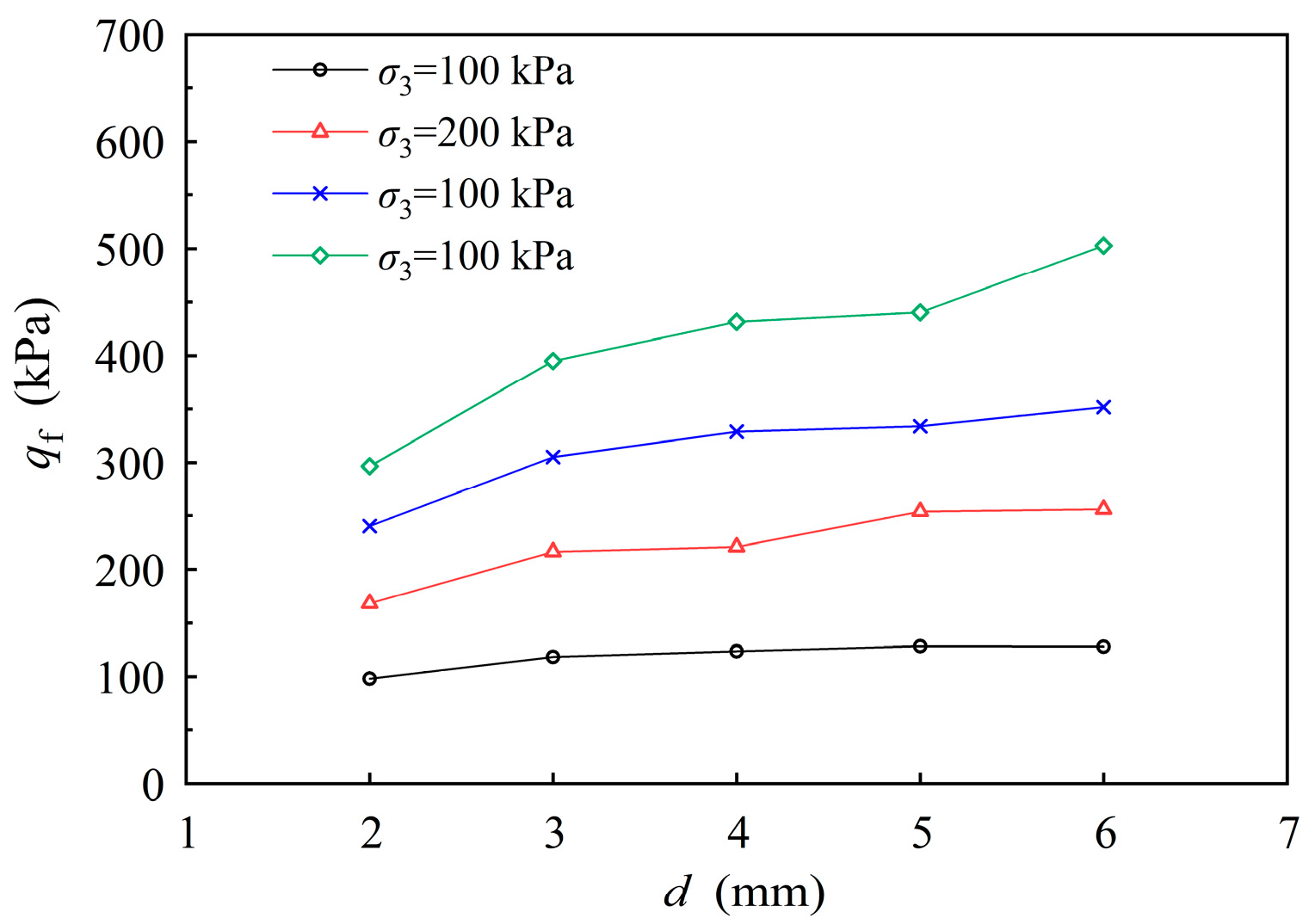
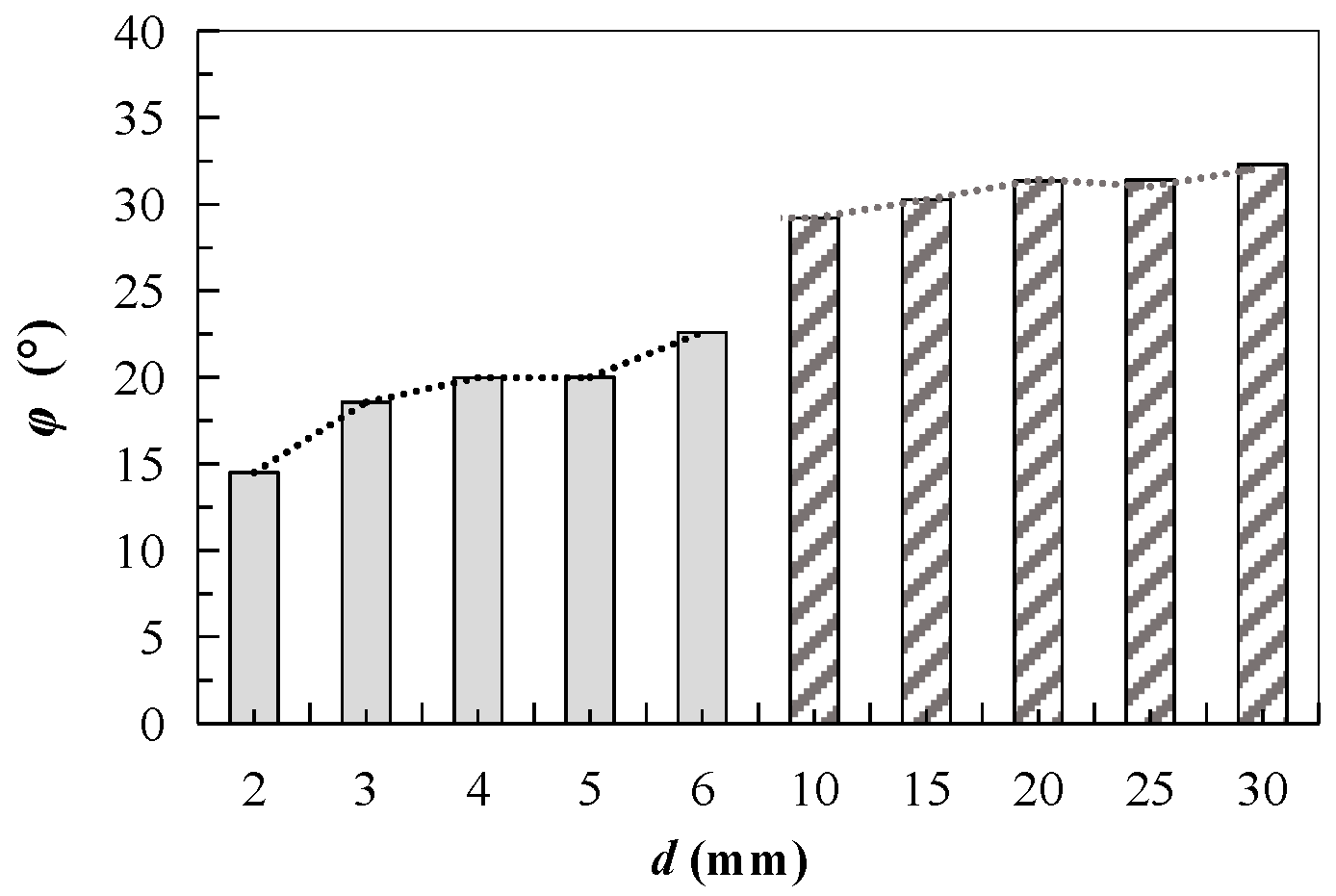
3.1.2. Effect of Particle Diameter on the Shear Strength of Small-Sized Granular Materials
3.2. Test Results and Analyses of Large-Sized Granular Materials
3.2.1. Stress-Strain Characterization
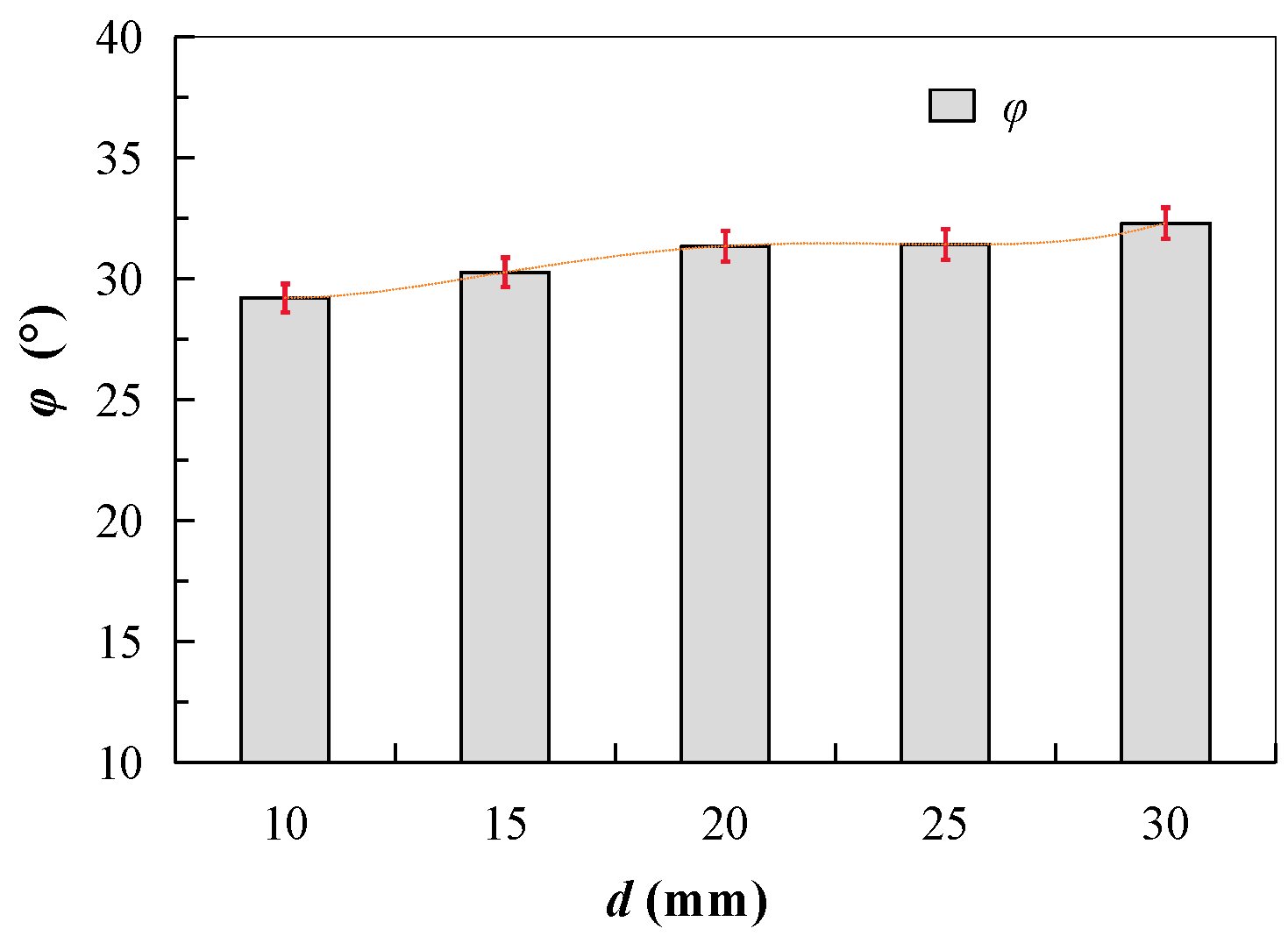
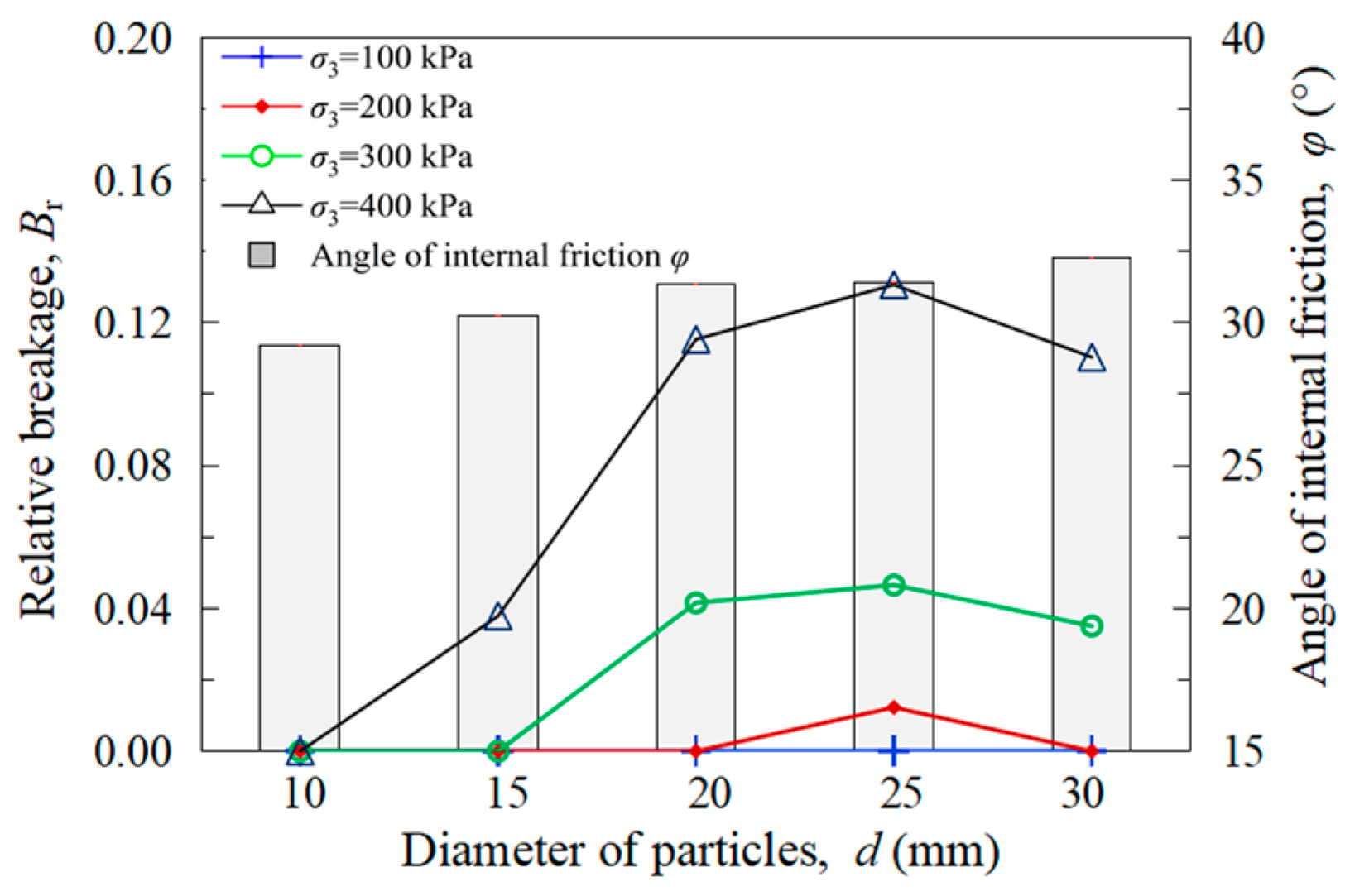
3.2.2. Effect of Particle Diameter on the Shear Strength of Large-Size Granular Materials
3.2.3. Test Results and Analyses of Large-Sized Granular Materials
4. Analysis of the Influence and Mechanisms of Particle Size on the Strength Behavior of Cohesionless Soil
4.1. Regularity of Particle Size Effect on Strength Behavior
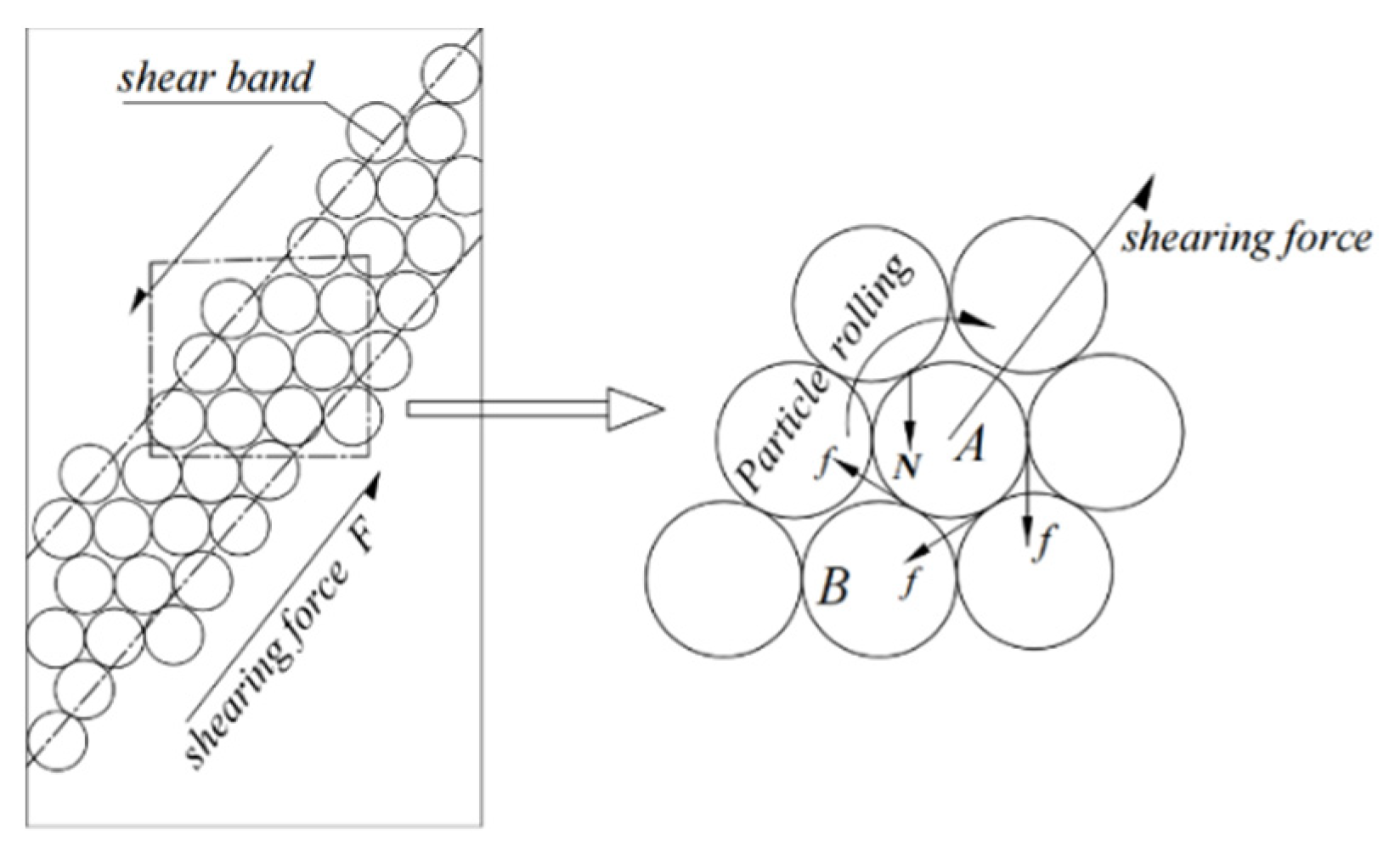
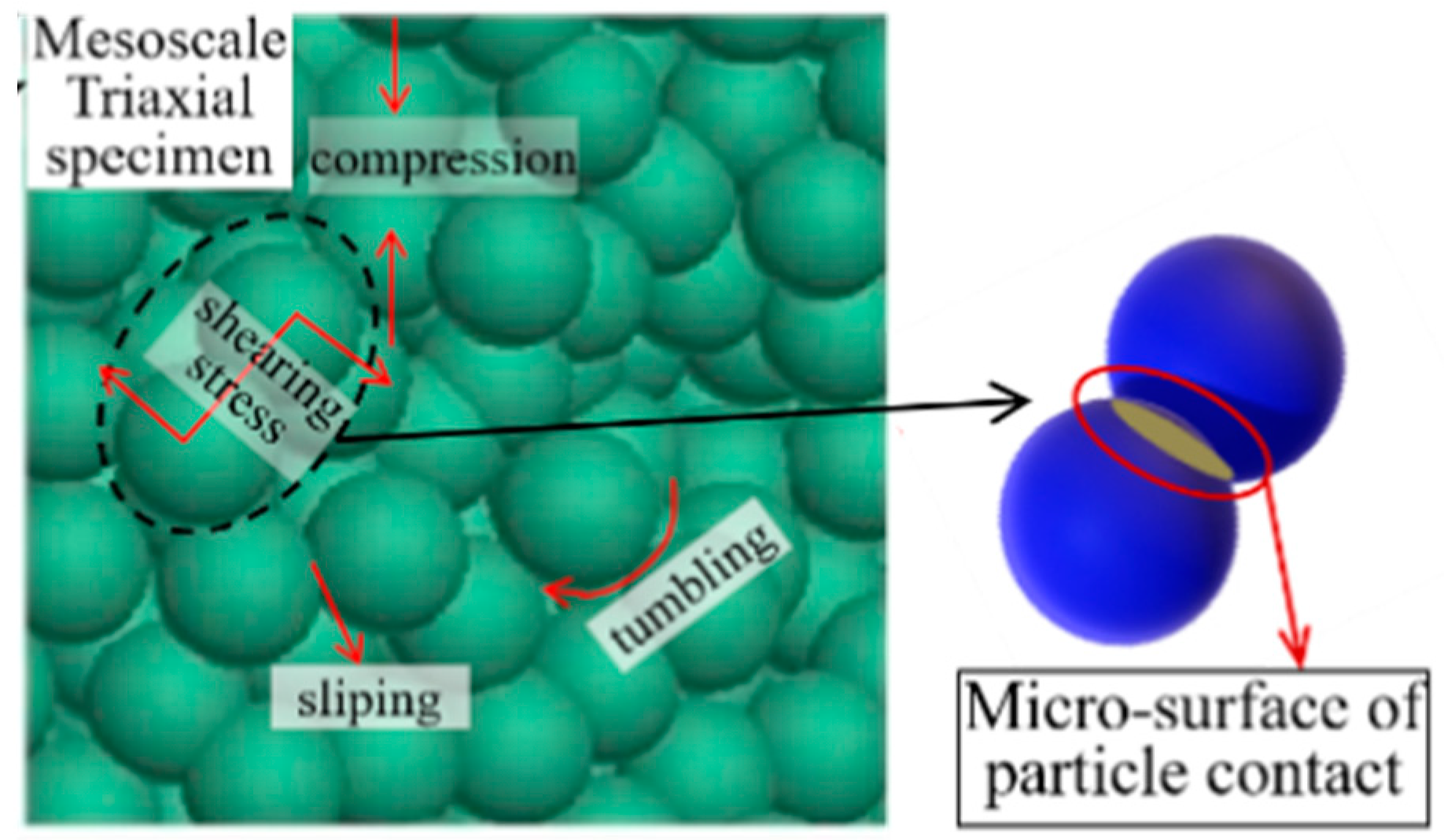
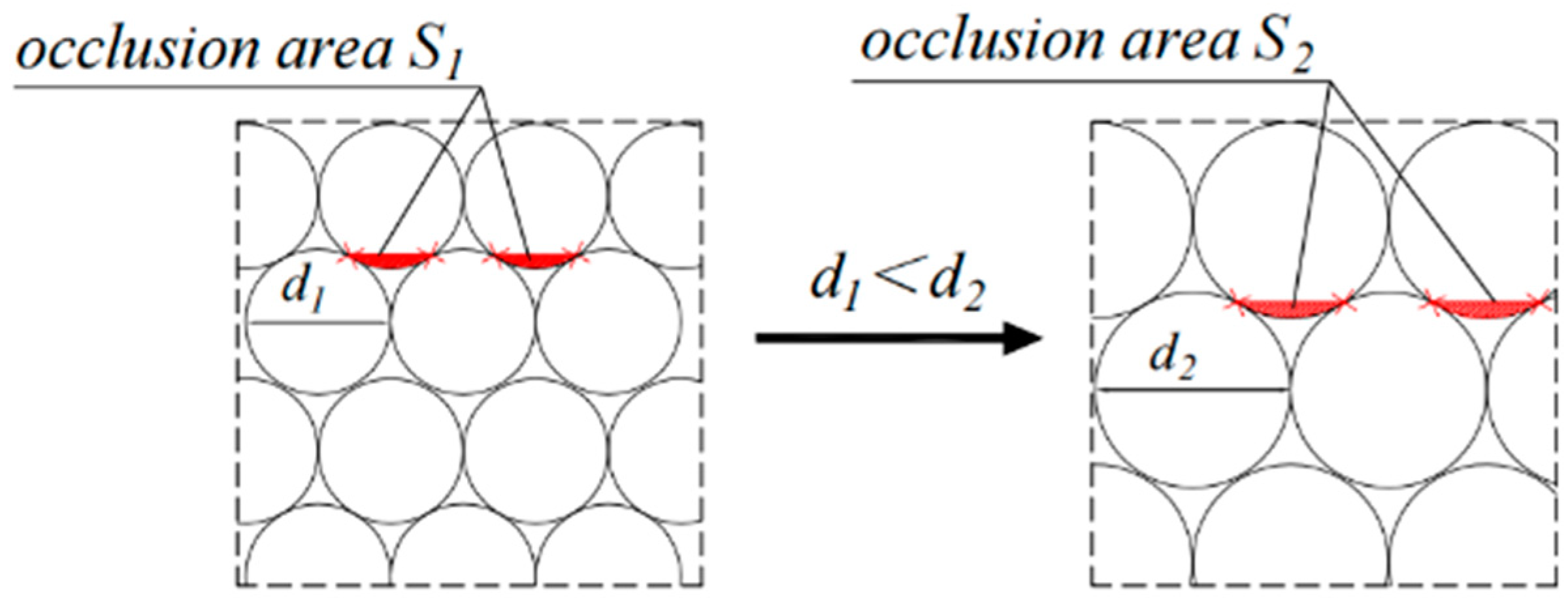
4.2. Mechanistic Analysis of the Effect of Dimensions on Strength Behavior
5. Conclusions
- (1)
- Particle size significantly influences the mechanical properties of cohesionless soil. For a given confining pressure, the peak shear stress (qf) of the specimen increases with particle size. This trend is observed for both small-sized (2 mm–6 mm) and large-sized (10 mm–30 mm) granular particles, with the growth rate of qf being higher under high confining pressures (300 kPa and 400 kPa) compared to low confining pressures (100 kPa and 200 kPa).
- (2)
- The internal friction angle (φ) increases monotonically with particle diameter (d) for both small-sized and large-sized granular materials. However, there exists an insensitive particle size range where the increase in φ is minimal: 4 mm–5 mm for small particles and 20 mm–25 mm for large particles. Additionally, large-sized granular materials exhibit significantly higher strength than small-sized granular materials.
- (3)
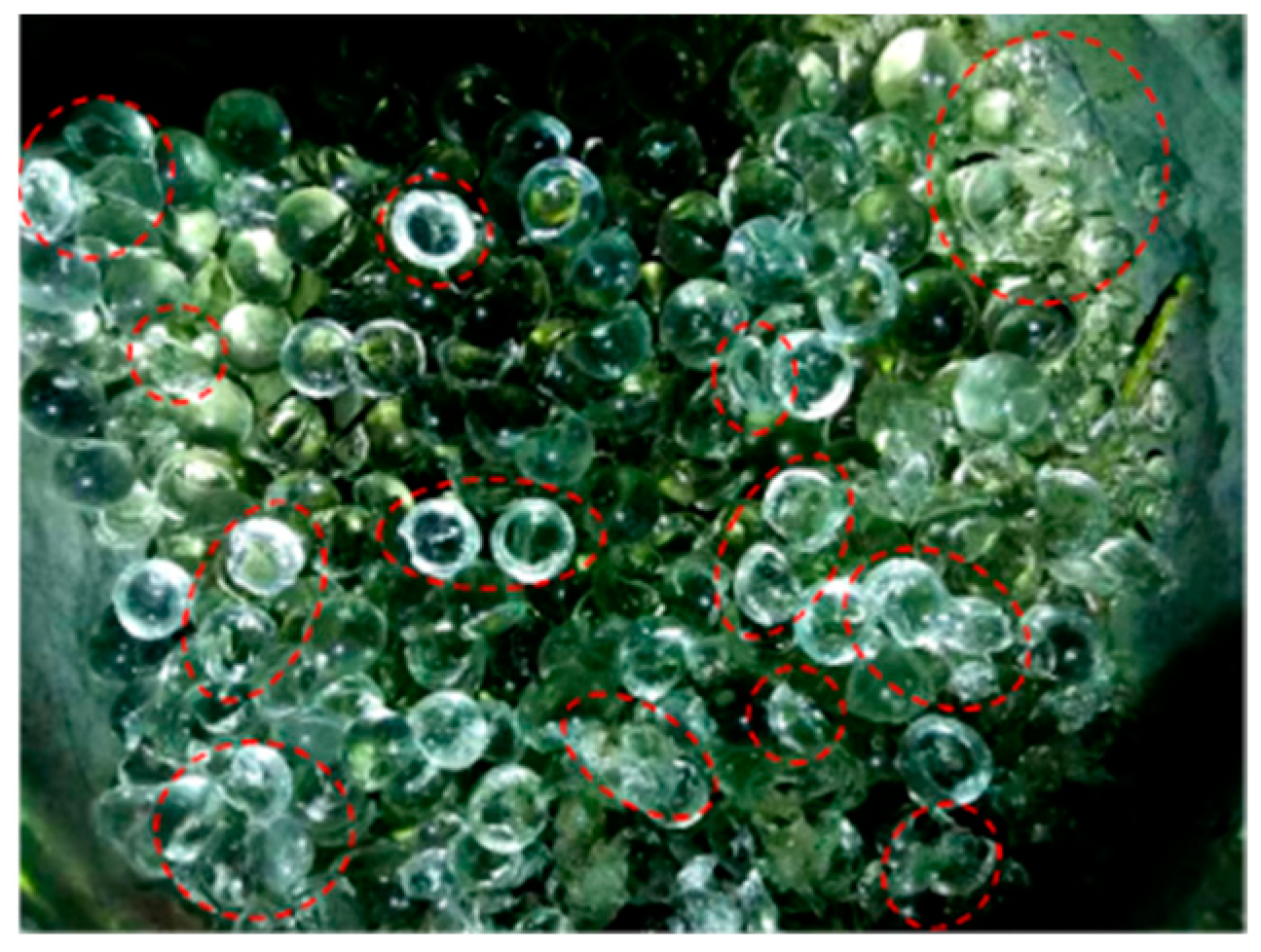
- For large-sized granular particles, the particle breakage rate (Br) increases with confining pressure. Moreover, Br initially increases and then decreases with increasing particle diameter (d).
- (4)
- The insensitive particle size ranges in the increase of internal friction angle (φ) with particle size reflect the macroscopic manifestation of the combined effects of two mesoscopic structural parameters: the number of particle contact points and the micro-contact area between particles. These parameters reach an extremum, resulting in a plateau in the overall strength as particle size increases.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Fang, Q.; Du, J.M.; Wang, G.; Wang, Z.J.; Wang, G.Q. Stratum deformation laws and influence factors analysis of tunnel excavation in sand. Hazard Control Tunneling Undergr. Eng. 2020, 2, 67–76. [Google Scholar]
- Chen, R.P.; Tang, L.J.; Ling, D.S.; Chen, Y.M. Face stability analysis of shallow shield tunnels in dry sandy ground using the discrete element method. Comput. Geotech. 2011, 38, 87–95. [Google Scholar] [CrossRef]
- Alagha, A.S.N.; Chapman, D.N. Numerical modelling of tunnel face stability in homogeneous and layered soft ground. Tunneling Undergr. Space Technol. 2019, 94, 103096. [Google Scholar] [CrossRef]
- Liu, N.F.; Liang, S.H.; Wang, S.J.; Song, Z.P. THM model of rock tunnels in cold regions and numerical simulation. Sci. Rep. 2024, 14, 3465. [Google Scholar] [CrossRef]
- Marshall, A.M.; Farrell, R.; Klar, A.; Mair, R. Tunnels in sands: The effect of size, depth and volume loss on greenfield displacements. Geotechnique 2012, 62, 385–399. [Google Scholar] [CrossRef]
- Shen, X.; Yuan, D.J. Model test of shield tunnel excavation face stability in sand. China Civ. Eng. J. 2015, 48, 261–265. [Google Scholar]
- Miao, L.C.; Wang, Z.X.; Shi, W.B. Theoretical and numerical simulations of face stability around shield tunnels in sand. Chin. J. Geotech. Eng. 2015, 37, 98–104. [Google Scholar]
- Wang, Y.S.; Wang, M.N.; Chen, W.T.; Wei, L.H. Test research on internal friction coefficient of sandy soil tunnel surrounding rock. Rock Soil Mech. 2008, 29, 741–746. [Google Scholar]
- Zhu, J.G.; Guo, W.L.; Xu, J.C.; Chu, F.Y. DEM analysis on impact of gradation and compactness on coarse-grained soil in tri-axial test. J. Chongqing Jiaotong Univ. (Nat. Sci.) 2017, 36, 70–74. [Google Scholar]
- Sun, Z.Z.; Ma, G.; Zhou, W.; Wang, Y.H.; Xiao, H.B. Influence of particle shape on size effect of crushing strength of rockfill particles. Rock Soil Mech. 2021, 42, 430–438. [Google Scholar]
- Hu, S.J.; Guo, N.; Yang, Z.X.; Zhao, J.D. Implicit DEM analyses of size and shape effects on crushing strength of rockfill particles. Chin. J. Geotech. Eng. 2023, 45, 433–440. [Google Scholar]
- Sun, Y.; Shen, Y.; Chen, C. A grading parameter for evaluating the grading-dependence of the shear stiffness of granular aggregates. Particuology 2018, 36, 193–200. [Google Scholar] [CrossRef]
- Li, G.; Liu, Y.J.; Dano, C.; Hicher, P.Y. Grading-dependent behavior of granular materials: From discrete to continuous modeling. J. Eng. Mech. 2014, 141, 04014172. [Google Scholar] [CrossRef]
- Chen, M.L.; Wu, G.J.; Gan, B.R.; Jiang, W.H.; Zhou, J.W. Physical and compaction properties of granular materials with artificial grading behind the particle size distributions. Adv. Mater. Sci. Eng. 2018, 2018, 8093571. [Google Scholar] [CrossRef]
- Li, G.; Liu, Y.J.; Yin, Z.Y.; Dano, C.; Hicher, P.Y. Grading effect on critical state behavior of granular materials. Chin. J. Geotech. Eng. 2014, 36, 452–457. [Google Scholar]
- Liang, C.Y.; Wu, Y.D.; Liu, J.; Wu, H.G. Influences of arrangement and cementation of soil particles on structure of artificial structural soil. Chin. J. Geotech. Eng. 2022, 44, 2135–2142. [Google Scholar]
- Liu, F.Y.; Zhong, L.J.; Zhong, Y. Effects of different particle arrangements on mechanical properties of sand. Chin. J. Geotech. Eng. 2021, 43, 155–160. [Google Scholar]
- Louati, H.; Oulahna, D.; Ryck, A. Effect of the particle size and the liquid content on the shear behavior of wet granular material. Powder Technol. 2017, 315, 398–409. [Google Scholar] [CrossRef]
- Wang, Z.Y.; Wang, P.; Yin, Z.Y.; Wang, R. Micromechanical investigation of the particle size effect on the shear strength of uncrushable granular materials. Acta Geotech. 2022, 17, 4277–4296. [Google Scholar] [CrossRef]
- Dai, B.B.; Yang, J.; Zhou, C.Y. A preliminary investigation on effect of particle size on mechanical behavior of granular materials. Rock Soil Mech. 2014, 35, 1878–1884. [Google Scholar]
- Shao, X.Q.; Chi, S.C. Particle size correlation of deformation parameters for rockfill materials. Chin. J. Geotech. Eng. 2020, 42, 1715–1722. [Google Scholar]
- Varadarajan, A.; Sharma, K.G.; Venkatachalam, K.; Gupta, A.K. Testing and modeling two rockfill materials. J. Geotech. Geoenviron. Eng. 2003, 129, 206–218. [Google Scholar] [CrossRef]
- Wang, B.; Lv, G.; Li, J. Experimental study on micro-macro shear properties of sand considering particle size. Geotech. Eng. Tech. 2023, 37, 618–622. [Google Scholar]
- Li, Z.R. Effect of Particle Size Difference on Shear Properties of Sand and Its Mesomechanical Analysis; Qingdao University of Technology: Qingdao, China, 2019. [Google Scholar]
- Venkatachalam, K. Prediction of Mechanical Behavior of Rockfill Materials; IIT Delhi: New Delhi, India, 1993. [Google Scholar]
- Wu, L.Q.; Ye, F.; Lin, W.Q. Experimental study on scale effect of mechanical properties of rockfill materials. Chin. J. Geotech. Eng. 2020, 42, 141–145. [Google Scholar]
- Ma, G.; Zhou, W.; Chang, X.L.; Zhou, C.B. Mesoscopic mechanism study of scale effects of rockfill. Chin. J. Rock Mech. Eng. 2012, 31, 2473–2482. [Google Scholar]
- Xue, Q.H. Study on Direct Shear Characteristics and Particle Crushing Characteristics of Soil-Rock Mixture; China University of Mining and Technology: Xuzhou, China, 2020. [Google Scholar]
- Marschi, N.D.; Chan, C.K.; Seed, H.B. Evaluation of properties of rockfill materials. ASCE Soil Mech. Found. Div. J. 1972, 98, 95–114. [Google Scholar] [CrossRef]
- Gupta, A.K. Effects of particle size and confining pressure on breakage factor of rockfill materials using medium triaxial test. J. Rock Mech. Geotech. Eng. 2016, 8, 378–388. [Google Scholar] [CrossRef]
- Charles, J.A.; Watts, K.S. The influence of confining pressure on the shear strength of compacted rockfill. Geotechnique 1980, 30, 353–367. [Google Scholar] [CrossRef]
- Marsal, R.J. Large scale testing of rockfill materials. J. Soil Mech. Found. Div. 1900, 94, 1042–1047. [Google Scholar] [CrossRef]
- Kai, W.; Patrick, P.; Frederic, B.; Sébastien, R.; Noredine, A.; Xu, W.Y.; Liu, S.Y. Experimental and numerical study of cylindrical triaxial test on mono-sized glass beads under quasi-static loading condition. Adv. Powder Technol. 2017, 28, 155–166. [Google Scholar]
- Brenda, N.M. Strength Properties of Granular Materials; Louisiana State University and Agricultural and Mechanical College: Baton Rouge, LA, USA, 2003. [Google Scholar]
- Wu, K.; Sébastien, R.; NorEdine, A.; Patrick, P.; Frédéric, B.; Liu, S.Y. Study of the shear behavior of binary granular materials by DEM simulations and experimental triaxial tests. Adv. Powder Technol. 2017, 28, 2198–2210. [Google Scholar] [CrossRef]
- Xu, W.D.; Li, X.F.; Yang, W.W.; Jia, H.J. Triaxial test on glass beads simulating coarse-grained soil. Res. Cold Arid. Reg. 2022, 14, 274–280. [Google Scholar] [CrossRef]
- Ou, F.Y.; Fei, J.B.; Ma, W.B.; Chen, X.S. Influence of Loading Conditions and Particle Size on Stick-Slip Characteristics of Granular Materials. Geotech. Eng. Tech. 2024, 38, 592–597. [Google Scholar]
- GB/T 50123-2019; Standard for Geotechnical Testing Method. China Planning Press: Beijing, China, 2019.
- Liu, F.Y.; Zhong, L.J.; Wang, B.; Yang, Z.; Dong, C. Visual Sealing Specimen Preparation Device, Assembly Method, and Specimen Preparation Method for Bulk Materials. CN Patent 1234567, 22 March 2024. [Google Scholar]
- Qin, H.Y.; Liu, H.L.; Gao, Y.F.; Dai, P.F. Research on strength and deformation behavior of coarse aggregates based on large-scale triaxial tests. Rock Soil Mech. 2004, 25, 1575–1580. [Google Scholar]
- Chu, F.Y.; Zhu, J.G.; Wang, G.Q.; Liu, H.L. Large-scale triaxial test study on deformation and strength characteristic of coarse grained material. J. Shandong Agric. Univ. (Nat. Sci.) 2011, 42, 572–578. [Google Scholar]
- Chu, F.Y.; Zhu, J.G.; Yin, J.H. Study of shear dilatancy behaviors of coarse-grained soil in large-scale triaxial tests under K0-consolidation condition. Rock Soil Mech. 2013, 34, 3431–3436. [Google Scholar]
- Liu, M.C.; Meng, F.; Wang, Y.Y. Evolution of particle crushing of coarse-grained materials in large-scale triaxial tests. Chin. J. Geotech. Eng. 2020, 42, 561–567. [Google Scholar]
- Chen, B.; Li, Y.Q.; Liu, X.F.; Zhou, Y.X.; Xu, Y. Study on Stick-slip Behavior of Granular Materials in Triaxial Test. J. Disaster Prev. Mitig. Eng. 2023, 43, 568–575. [Google Scholar]
- Chen, X.; Shen, J.H.; Wang, X.; Yao, T.; Xu, D.S. Effect of Saturation on Shear Behavior and Particle Breakage of Coral Sand. J. Mar. Sci. Eng. 2022, 10, 1280–1309. [Google Scholar] [CrossRef]

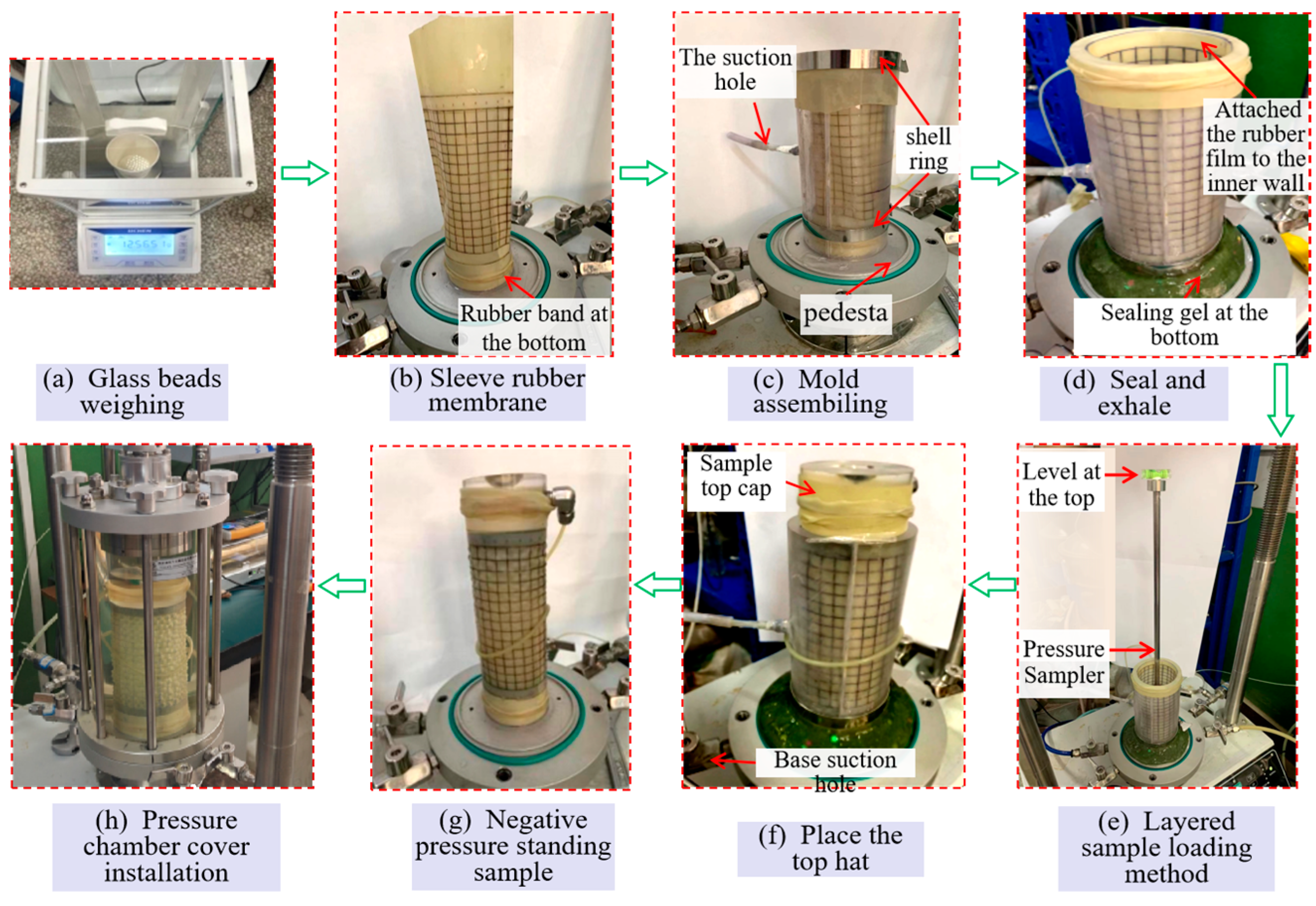
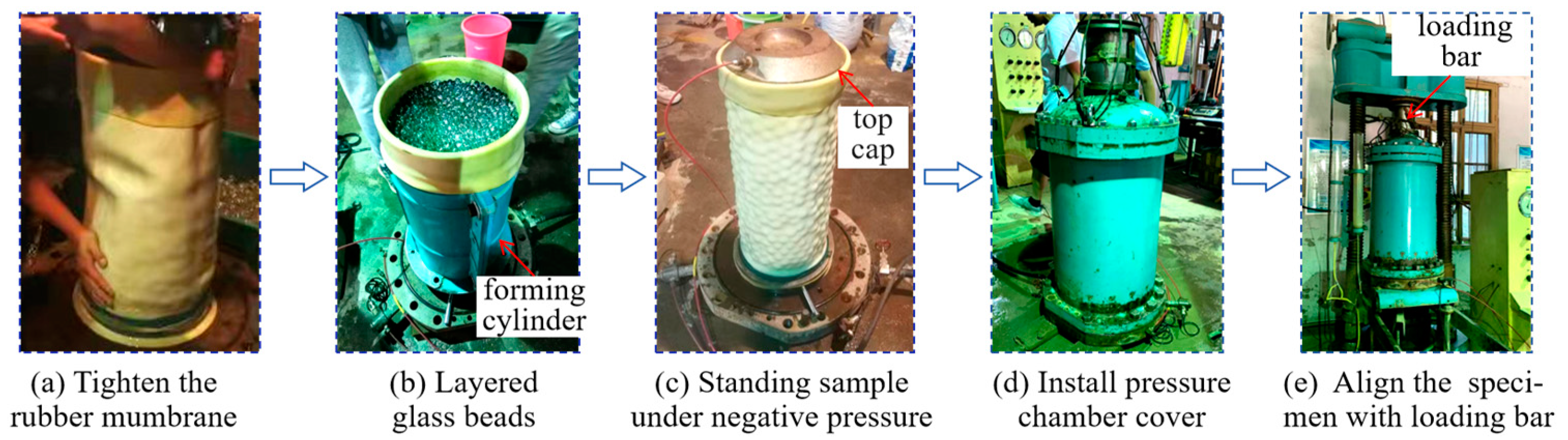
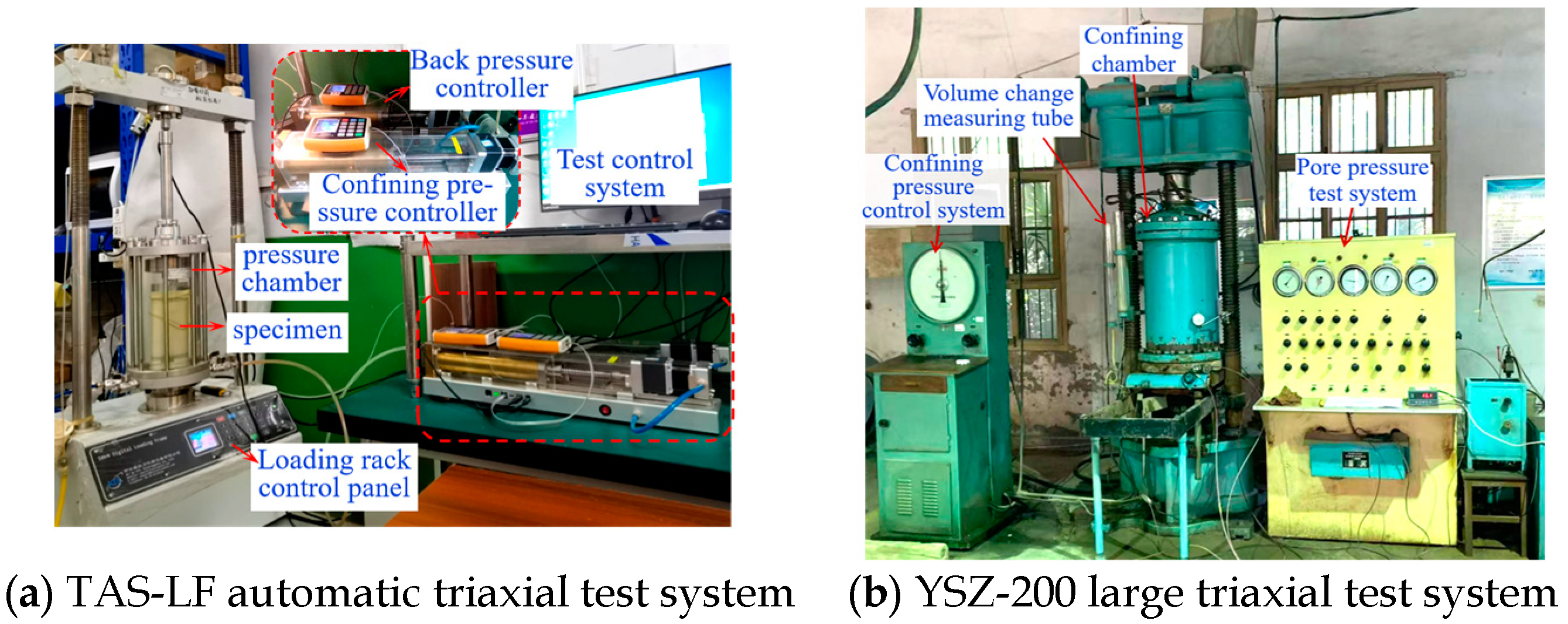



| Glass Bead Diameter (mm) | 2 | 3 | 4 | 5 | 6 | 10 | 15 | 20 | 25 | 30 |
|---|---|---|---|---|---|---|---|---|---|---|
| ρ (g/cm3) | 2.50 (±0.003) | |||||||||
| ρdmax (g/cm3) | 1.63 | 1.62 | 1.60 | 1.59 | 1.58 | 1.55 | 1.50 | 1.49 | 1.48 | 1.47 |
| ρdmin (g/cm3) | 1.43 | 1.43 | 1.41 | 1.40 | 1.40 | 1.53 | 1.47 | 1.45 | 1.43 | 1.41 |
| Specimen size (D × h) | 61.8 mm × 120 mm | 300 mm × 600 mm | ||||||||
| Particle Diameter (mm) | Shear Strength Parameters | |
|---|---|---|
| c (kPa) | φ (°) | |
| 2 | 13.06 | 14.51 |
| 3 | 9.89 | 18.57 |
| 4 | 5.67 | 19.99 |
| 5 | 10.81 | 20.01 |
| 6 | 1.32 | 22.60 |
| Particle Diameter (mm) | Shear Strength Parameters | |
|---|---|---|
| c (kPa) | φ (°) | |
| 10 | 35.33 | 29.21 |
| 15 | 22.54 | 30.27 |
| 20 | 29.73 | 31.45 |
| 25 | 39.63 | 31.35 |
| 30 | 29.95 | 32.10 |
| d (mm) | σ3 (kPa) | Total Mass of Design Specimen Md (kg) | Total Mass of Actual Specimen Mf (kg) | Mass of Unbroken Particles Mu (kg) | Mass Percent of Crushed Particles Pt (%) |
|---|---|---|---|---|---|
| 15 | 100 | 63.278 | 63.266 | 63.266 | 0 |
| 200 | 63.268 | 63.268 | |||
| 300 | 63.271 | 63.271 | |||
| 400 | 63.272 | 61.722 | 2.45% | ||
| 20 | 100 | 62.811 | 62.800 | 62.800 | 0 |
| 200 | 62.791 | 62.791 | |||
| 300 | 62.801 | 61.099 | 2.71% | ||
| 400 | 62.795 | 54.029 | 13.96% | ||
| 25 | 100 | 62.387 | 62.412 | 62.412 | 0 |
| 200 | 62.425 | 62.025 | 0.64% | ||
| 300 | 62.422 | 59.419 | 4.81% | ||
| 400 | 62.420 | 53.313 | 14.59% | ||
| 30 | 100 | 61.920 | 61.922 | 61.922 | 0 |
| 200 | 61.929 | 61.929 | |||
| 300 | 61.915 | 60.367 | 2.50% | ||
| 400 | 61.924 | 56.673 | 8.48% |
| Specimen Diameter | Conventional Triaxial Tests D = 61.8 mm | Large-Scale Triaxial Tests D = 300 | ||
|---|---|---|---|---|
| Glass bead diameter (mm) | 4 | 5 | 20 | 25 |
| D/d | 15.45 | 12.36 | 15 | 12 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhong, L.; Liu, F.; Wu, Z.; Liu, N.; Li, H.; Wang, B. Size Effect on the Strength Behavior of Cohesionless Soil Under Triaxial Stress State. Appl. Sci. 2025, 15, 3310. https://doi.org/10.3390/app15063310
Zhong L, Liu F, Wu Z, Liu N, Li H, Wang B. Size Effect on the Strength Behavior of Cohesionless Soil Under Triaxial Stress State. Applied Sciences. 2025; 15(6):3310. https://doi.org/10.3390/app15063310
Chicago/Turabian StyleZhong, Lijia, Fengyin Liu, Zhonghua Wu, Naifei Liu, Hao Li, and Bo Wang. 2025. "Size Effect on the Strength Behavior of Cohesionless Soil Under Triaxial Stress State" Applied Sciences 15, no. 6: 3310. https://doi.org/10.3390/app15063310
APA StyleZhong, L., Liu, F., Wu, Z., Liu, N., Li, H., & Wang, B. (2025). Size Effect on the Strength Behavior of Cohesionless Soil Under Triaxial Stress State. Applied Sciences, 15(6), 3310. https://doi.org/10.3390/app15063310






