Inversion of Elastic and Fracture Parameters in Tilted Transverse Isotropic Media with Parameter Standardization
Abstract
1. Introduction
2. Theory and Method
2.1. Derivation of the Reflection Coefficient Equation
2.2. Reflection Coefficient Equation Analysis
2.3. Derivation of the Inversion Equation
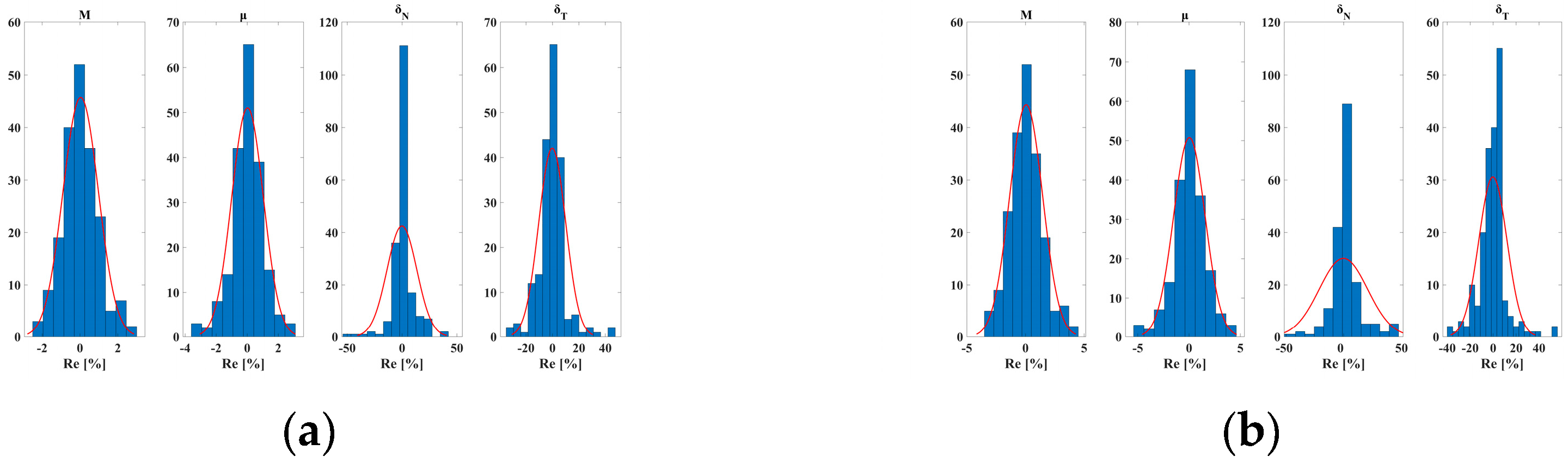
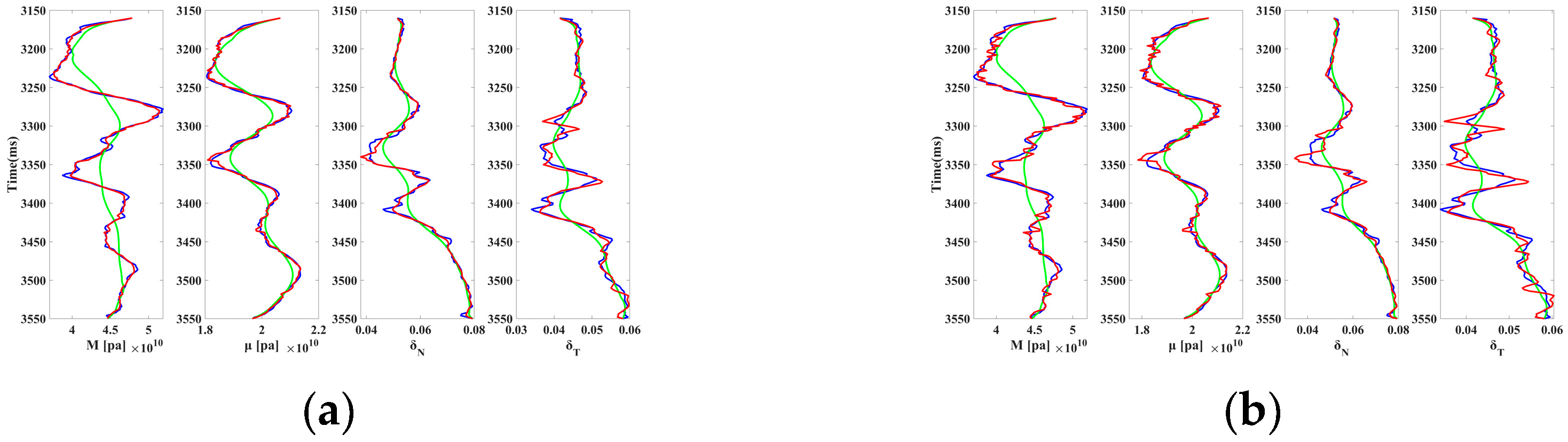
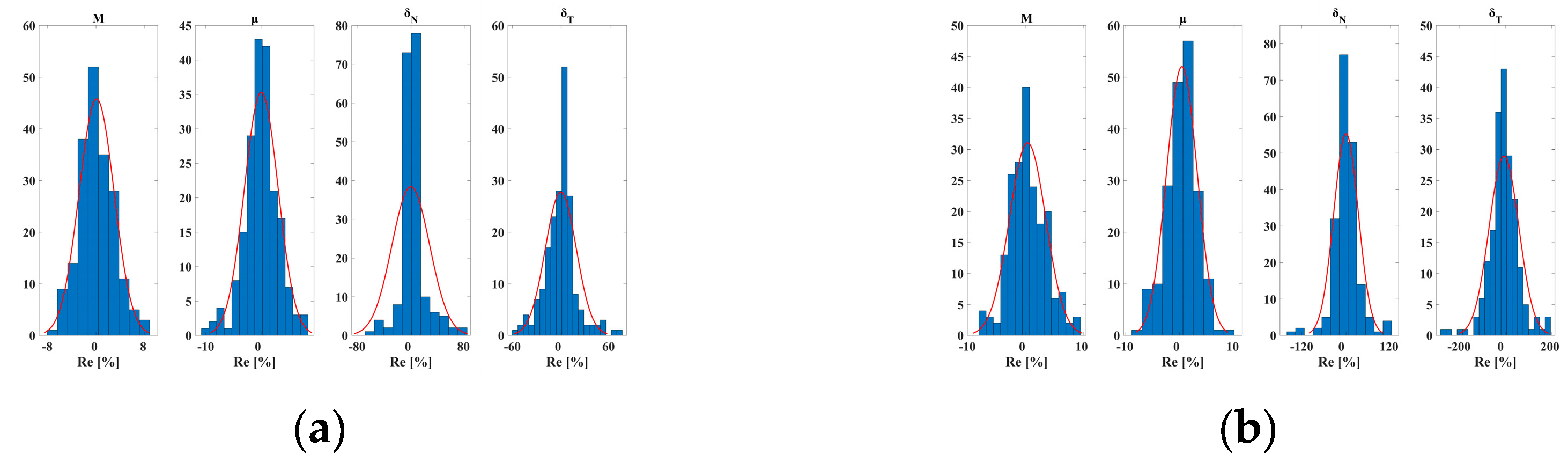
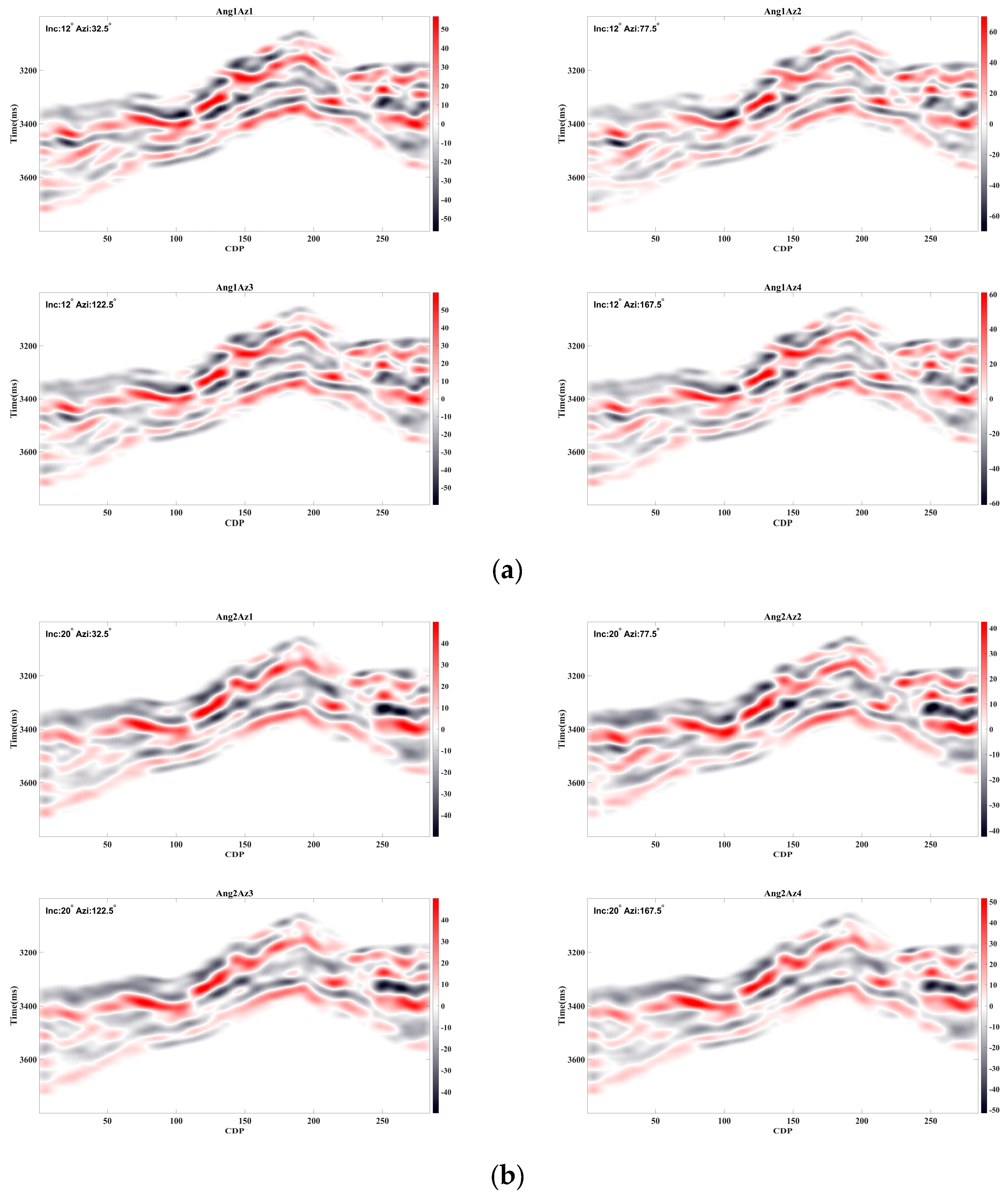
3. Examples
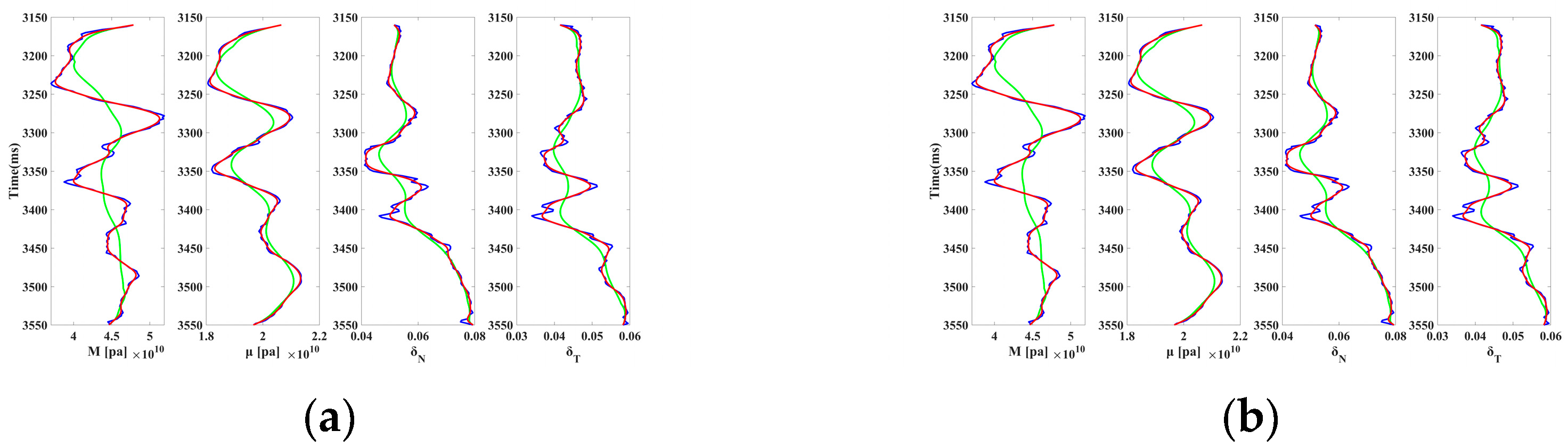
3.1. Synthetic Example
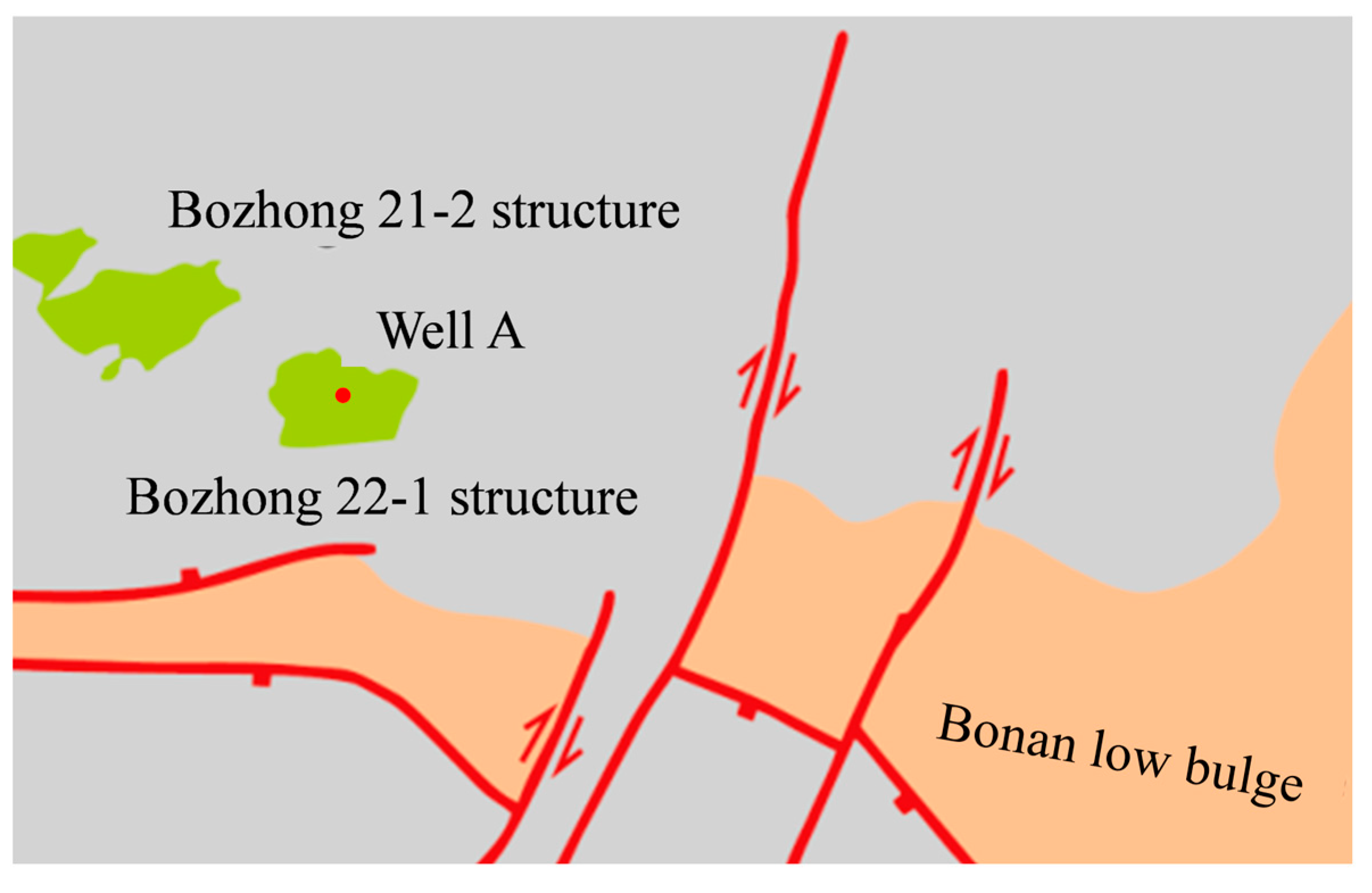
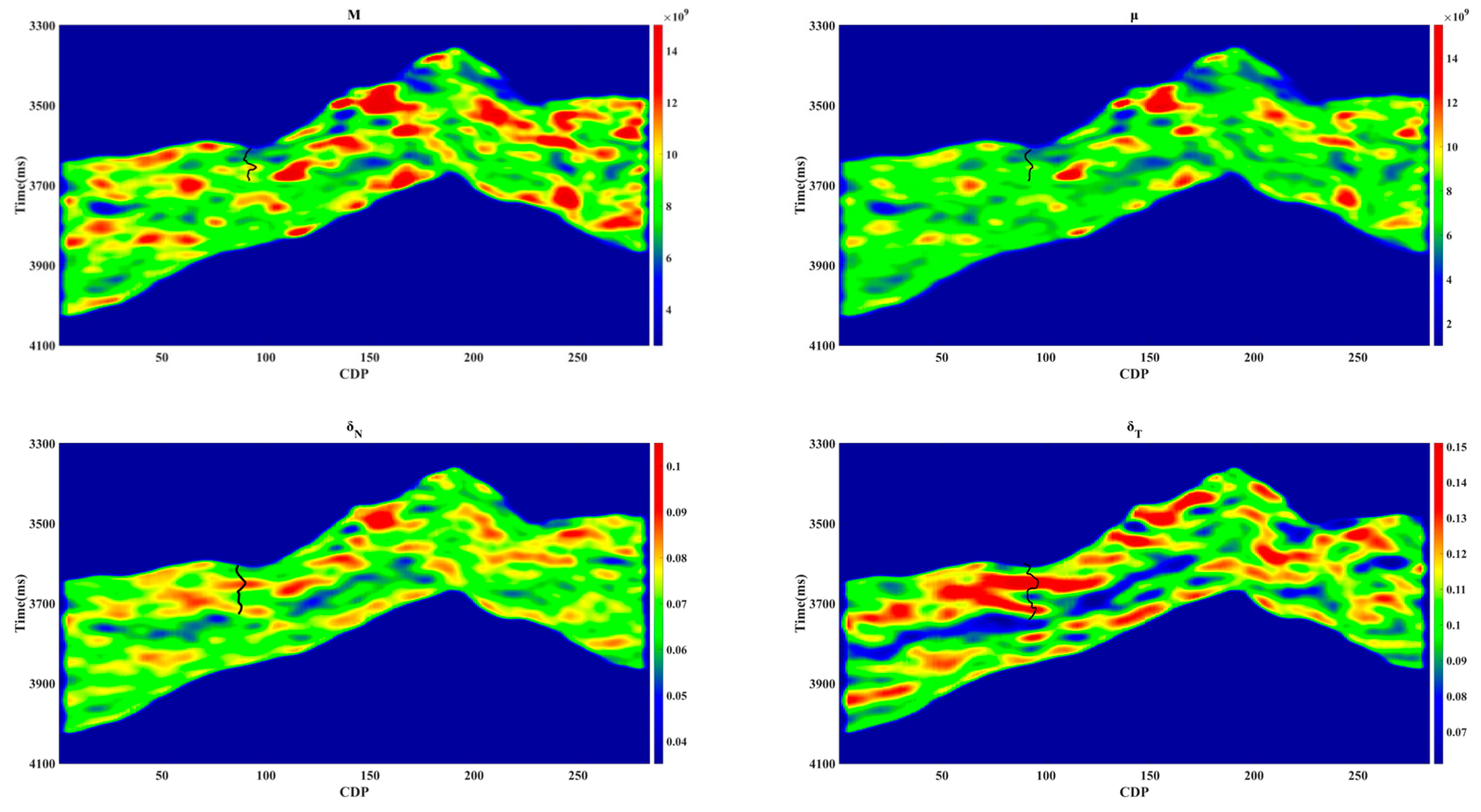
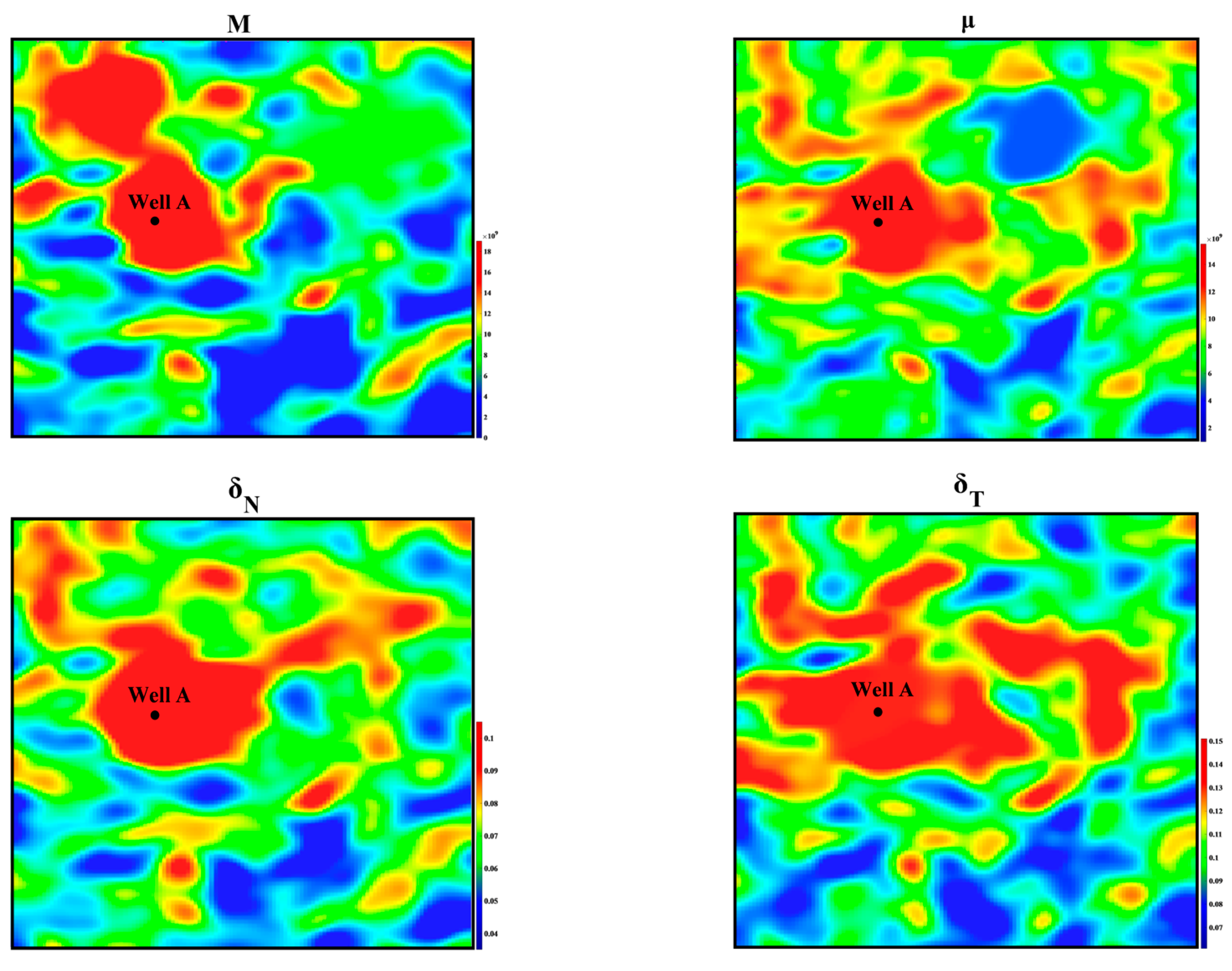
3.2. Field Data Example
4. Discussions
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| M | Longitudinal wave modulus, which is equal to the product of the density and the square of the longitudinal wave velocity |
| μ | The transverse wave modulus, which is equal to the product of the density and the square of the shear wave velocity |
| Normal weakness | |
| Tangential weakness | |
| Re | Relative error |
| AngXazY | Seismic data for the X-th angle of incidence and the Y-th azimuth |
| Inc | The angle of incidence |
| Azi | The azimuth |
References
- Thomsen, L. Weak elastic anisotropy. Geophysics 1986, 51, 1954–1966. [Google Scholar] [CrossRef]
- Rüger, A. Reflection Coefficient and Azimuthal AVO Analysis in Anisotropic Media. Ph.D. Thesis, Colorado School of Mines, Golden, CO, USA, 1996. [Google Scholar]
- Tsvankin, I. Anisotropic parameters and P-wave velocity for orthorhombic media. Geophysics 1997, 62, 1292–1309. [Google Scholar] [CrossRef]
- Hudson, J.A. Overall properties of a cracked solid. Math. Proc. Camb. Philos. Soc. 1980, 88, 371–384. [Google Scholar] [CrossRef]
- Hudson, J.A. Wave speeds and attenuation of elastic waves in material containing cracks. Geophysics 1981, 64, 133–150. [Google Scholar] [CrossRef]
- Schoenberg, M. Elastic wave behavior across linear slip interfaces. J. Acoust. Soc. Am. 1980, 68, 1516–1521. [Google Scholar] [CrossRef]
- Ivančić, F.; Solovchuk, M. Moving mesh strategy for simulating sliding and rolling dynamics of droplets on inclined surfaces with finite element method. Comput. Methods Appl. Mech. Eng. 2022, 400, 115404. [Google Scholar] [CrossRef]
- Bakulin, A.; Grechka, V.; Tsvankin, I. Estimation of fracture parameters from reflection seismic data—Part I: HTI model due to a single fracture set. Geophysics 2000, 65, 1788–1802. [Google Scholar] [CrossRef]
- Chen, H.; Yin, X. Study on Methodology of Pre-Stack Seismic Inversion for Fractured Reservoirs Based on Rock Physics; China University of Petroleum: Qingdao, China, 2015. [Google Scholar]
- Bachrach, R. Uncertainty and nonuniqueness in linearized AVAZ for orthorhombic media. Lead. Edge 2015, 34, 1048–1056. [Google Scholar] [CrossRef]
- Li, L.; Zhang, G.; Pan, X.; Guo, X.; Zhang, J.; Zhou, Y.; Lin, Y. Anisotropic poroelasticity and AVAZ inversion for in situ stress estimate in fractured shale-gas reservoirs. IEEE Trans. Geosci. Remote Sens. 2022, 60, 5911113. [Google Scholar] [CrossRef]
- Chen, F.; Zong, Z.; Lang, K.; Li, J.; Yin, X.; Miao, Z.; Xiao, W. Geofluid discrimination in stress-induced anisotropic porous reservoirs using seismic AVAZ inversion. IEEE Trans. Geosci. Remote Sens. 2024, 62, 5931714. [Google Scholar] [CrossRef]
- Rüger, A. P-wave reflection coefficients for transversely isotropic models with vertical and horizontal axis of symmetry. Geophysics 1997, 62, 713–722. [Google Scholar] [CrossRef]
- Chen, H.; Pan, X.; Ji, Y.; Zhang, G. Bayesian Markov Chain Monte Carlo inversion for weak anisotropy parameters and fracture weaknesses using azimuthal elastic impedance. Geophys. J. Int. 2017, 210, 801–818. [Google Scholar] [CrossRef]
- Shaw, R.K.; Sen, M.K. Born integral, stationary phase and linearized reflection coefficients in weak anisotropic media. Geophys. J. Int. 2004, 158, 225–238. [Google Scholar] [CrossRef]
- Zhang, F.; Zhang, T.; Li, X.Y. Seismic amplitude inversion for the transversely isotropic media with vertical axis of symmetry. Geophys. Prospect. 2019, 67, 2368–2385. [Google Scholar] [CrossRef]
- Pan, X.; Li, L.; Zhou, S.; Zhang, G.; Liu, J. Azimuthal amplitude variation with offset parameterization and inversion for fracture weaknesses in tilted transversely isotropic media. Geophysics 2021, 86, C1–C18. [Google Scholar] [CrossRef]
- Zhao, Y.; Wen, X.T.; Li, C.L.; Liu, Y.; Xie, C.L. Systematic prediction of the gas content, fractures, and brittleness in fractured shale reservoirs with TTI medium. Pet. Sci. 2024, 158, 225–238. [Google Scholar] [CrossRef]
- Hsu, C.J.; Schoenberg, M. Elastic waves through a simulated fractured medium. Geophysics 1993, 58, 964–977. [Google Scholar] [CrossRef]
- Ponthot, J.P.; Belytschko, T. Arbitrary Lagrangian-Eulerian formulation for element-free Galerkin method. Comput. Methods Appl. Mech. Eng. 1998, 152, 19–46. [Google Scholar] [CrossRef]
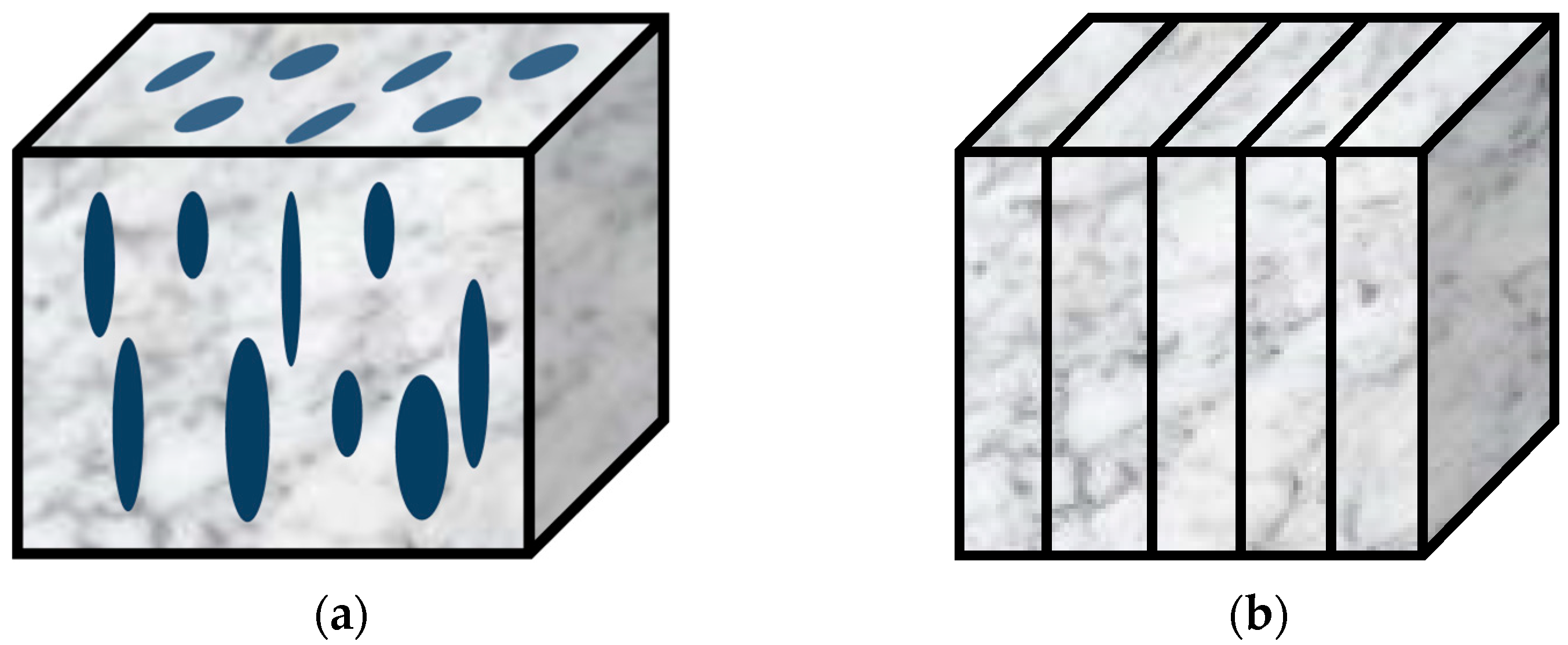

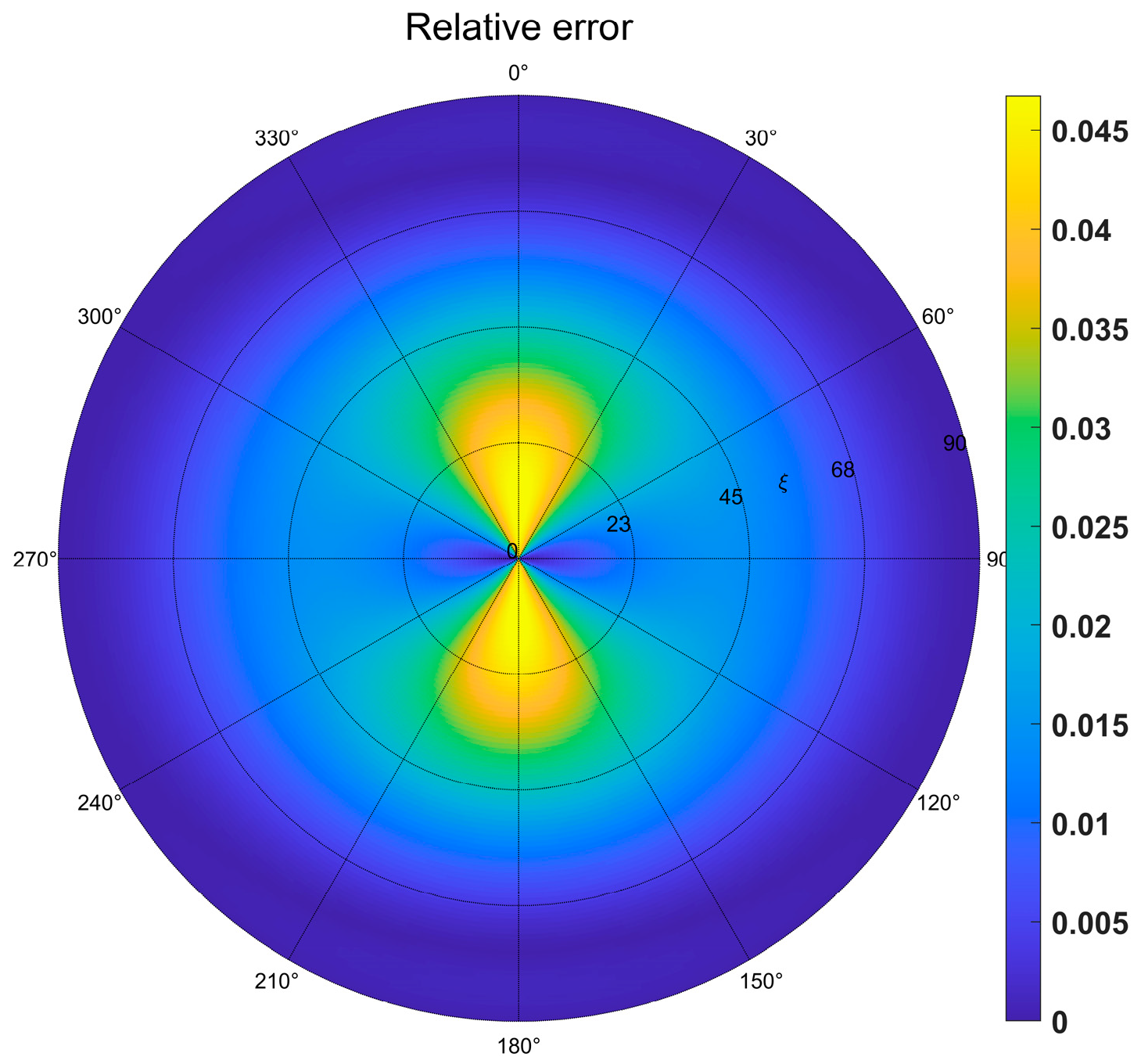

| Layer | M [Gpa] | μ [Gpa] | δN | δT |
|---|---|---|---|---|
| Upeer | 1.85 | 1.15 | 0 | 0 |
| Lower | 2.48 | 1.32 | 0.12 | 0.07 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, G.; Dai, S.; Li, H.; Hao, H.; Chen, T. Inversion of Elastic and Fracture Parameters in Tilted Transverse Isotropic Media with Parameter Standardization. Appl. Sci. 2025, 15, 2792. https://doi.org/10.3390/app15052792
Zhang G, Dai S, Li H, Hao H, Chen T. Inversion of Elastic and Fracture Parameters in Tilted Transverse Isotropic Media with Parameter Standardization. Applied Sciences. 2025; 15(5):2792. https://doi.org/10.3390/app15052792
Chicago/Turabian StyleZhang, Guangzhi, Shengzhao Dai, Han Li, Hongjian Hao, and Tengfei Chen. 2025. "Inversion of Elastic and Fracture Parameters in Tilted Transverse Isotropic Media with Parameter Standardization" Applied Sciences 15, no. 5: 2792. https://doi.org/10.3390/app15052792
APA StyleZhang, G., Dai, S., Li, H., Hao, H., & Chen, T. (2025). Inversion of Elastic and Fracture Parameters in Tilted Transverse Isotropic Media with Parameter Standardization. Applied Sciences, 15(5), 2792. https://doi.org/10.3390/app15052792






