Multiphysics Analysis Process of Front-End Process for Induction Hardening of 3D Structures to Predict Structural Deformation
Abstract
1. Introduction
1.1. Research Background
1.2. Literature Review
1.3. Motivation and Novelty
2. Multiphysics-Coupled Analysis-Based Induction Hardening Process
2.1. Fundamental Theories of Electromagnetic-Thermal Interaction
2.1.1. Joule Heating Theory
2.1.2. Induction Heating Theory
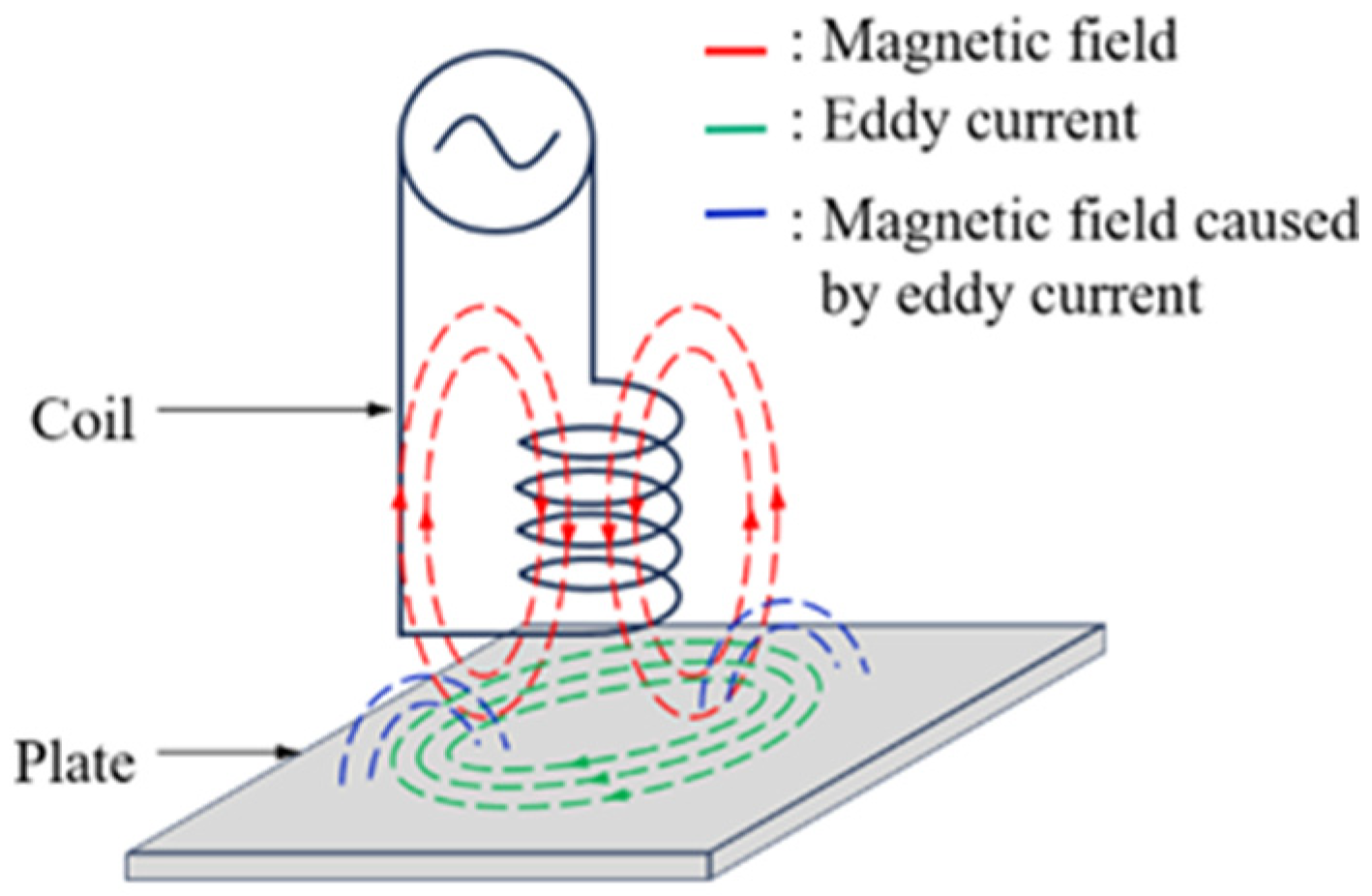
2.1.3. Skin Effect Theory
2.1.4. Current Distribution
2.2. Multiphysics-Coupled Analysis
2.3. Phase Transformation Analysis
2.4. Finite Element Analysis (FEA)
3. Finite Element Analysis Results
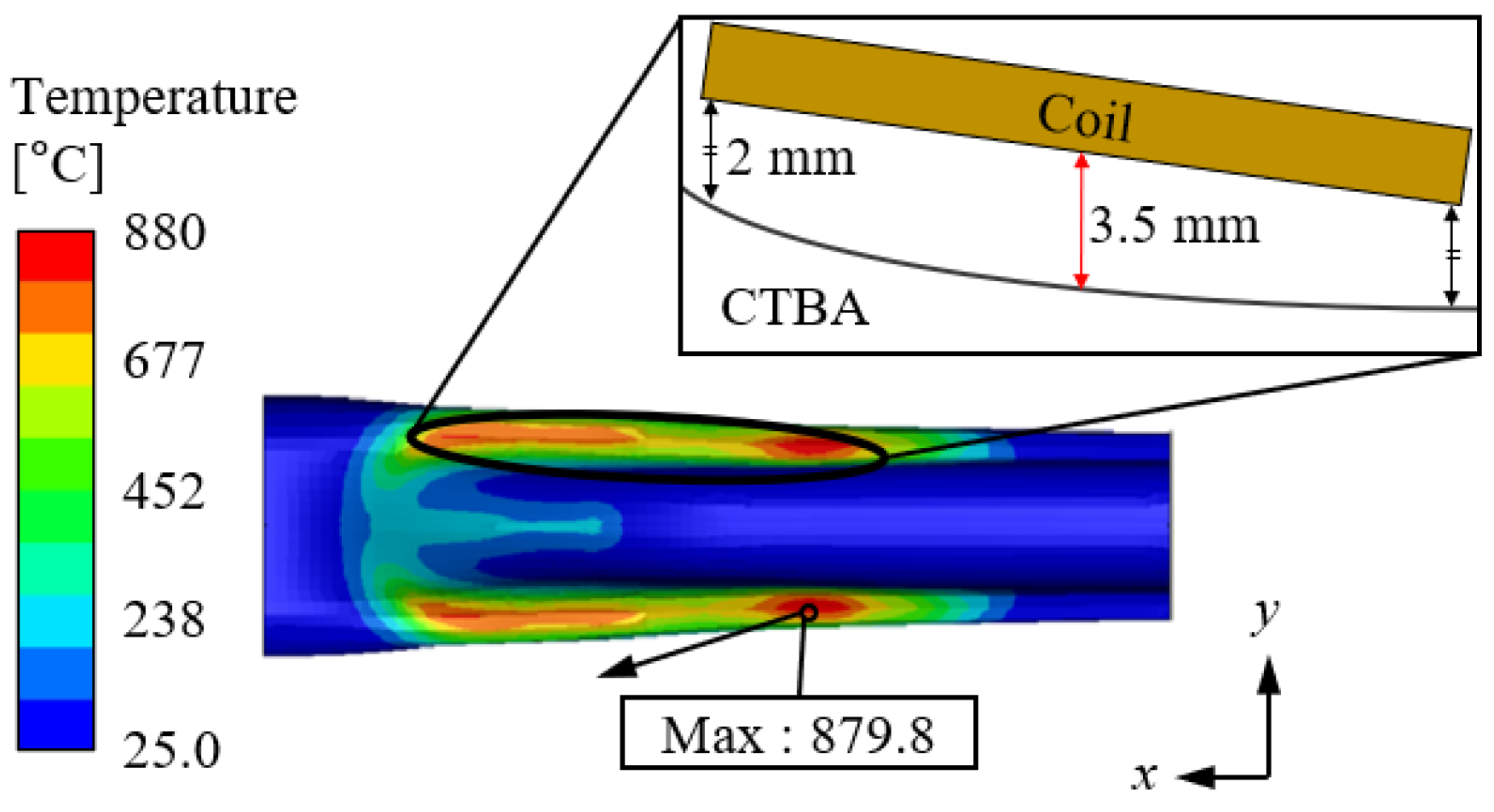
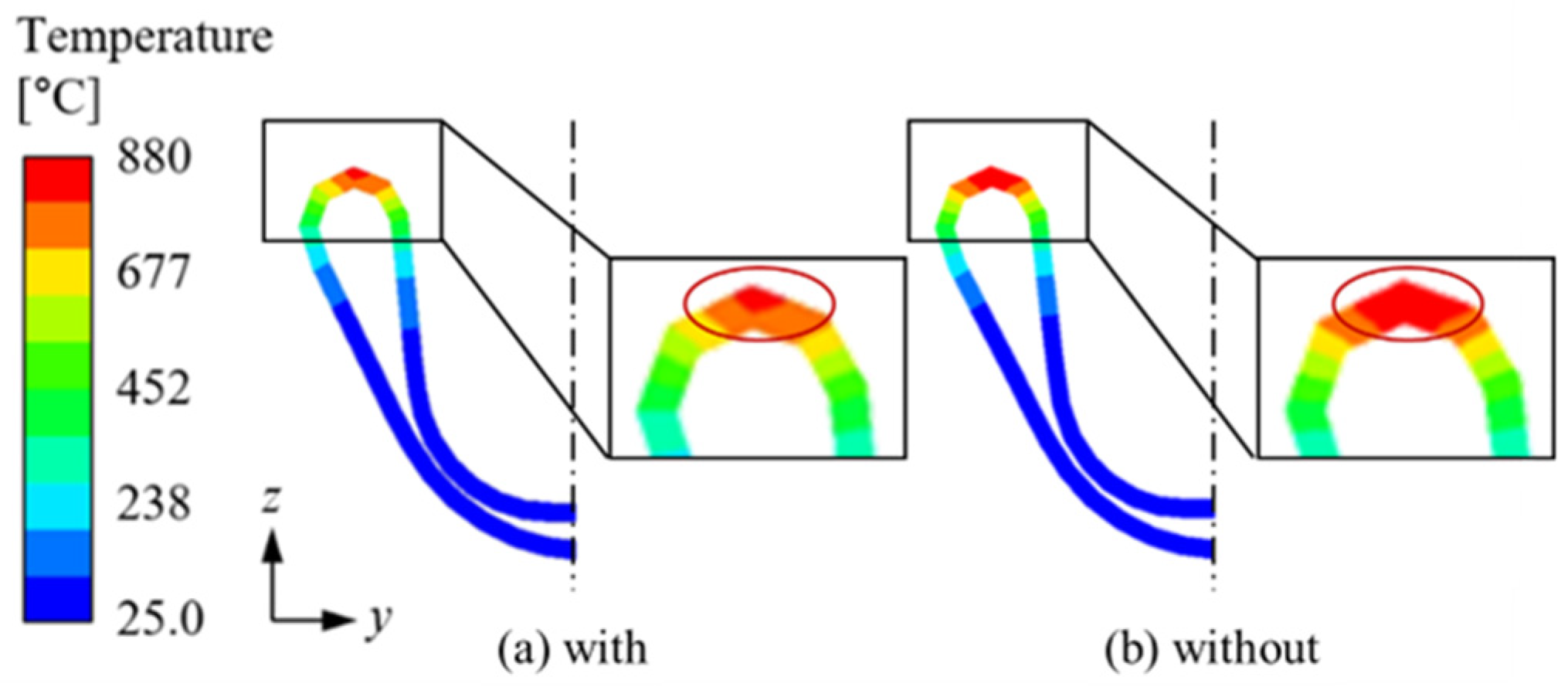
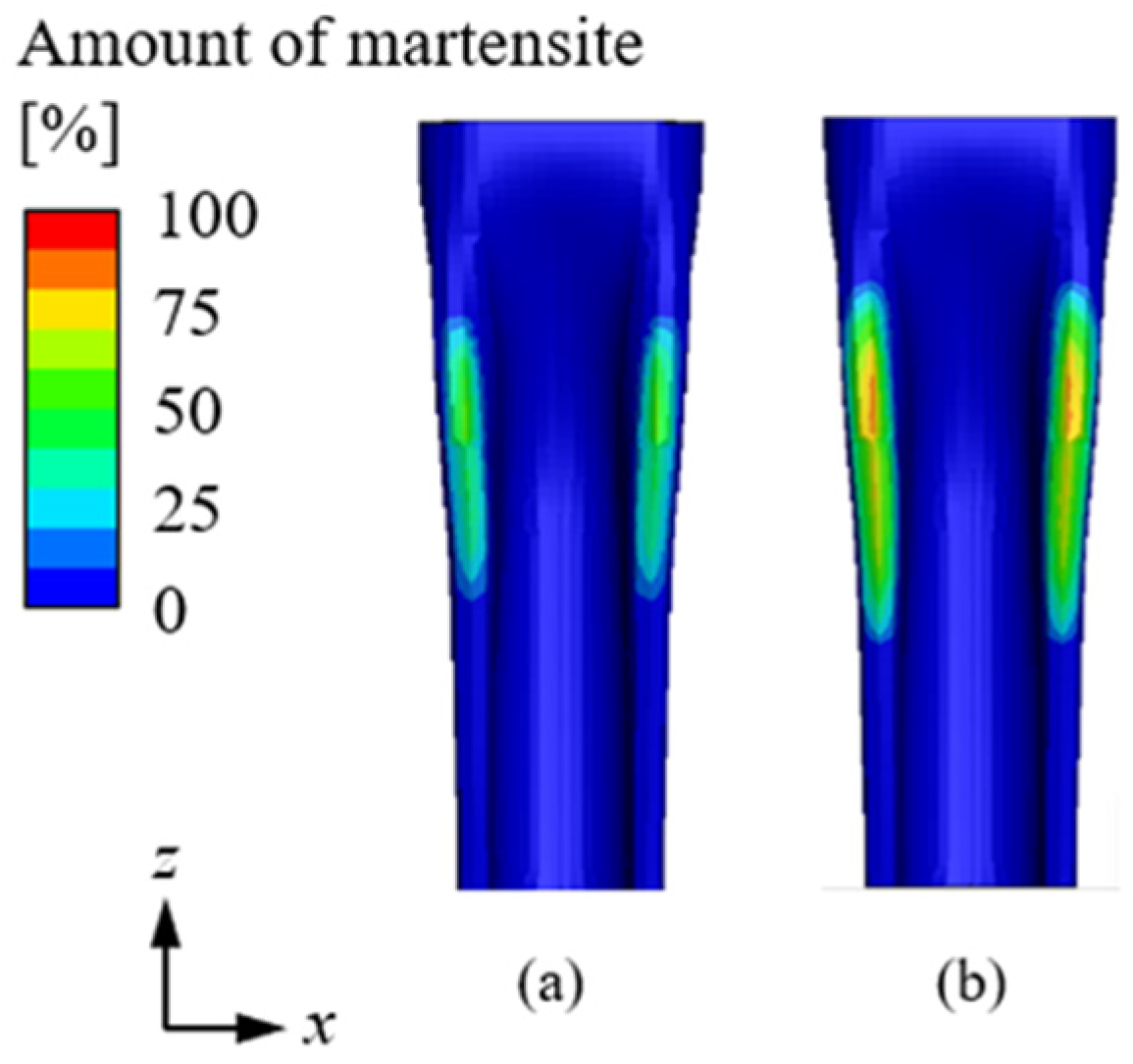
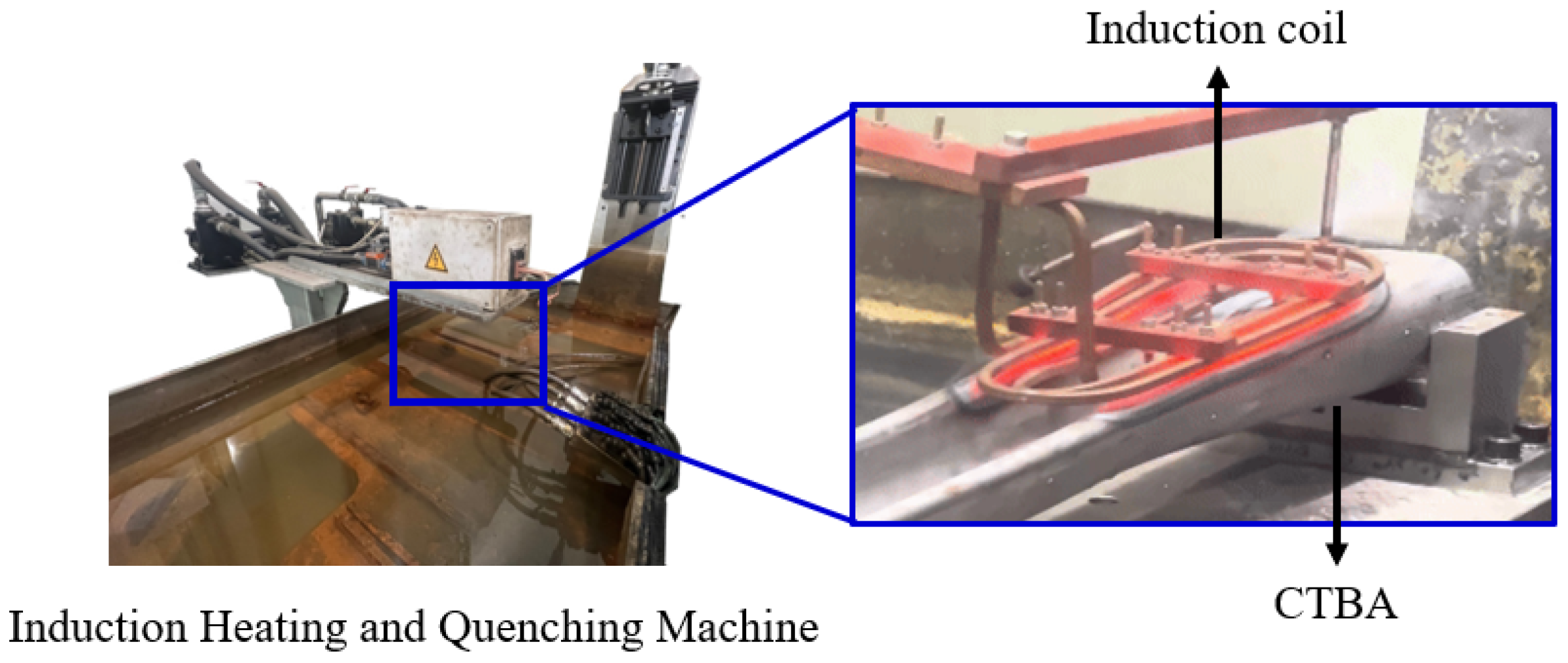
3.1. Heating Analysis
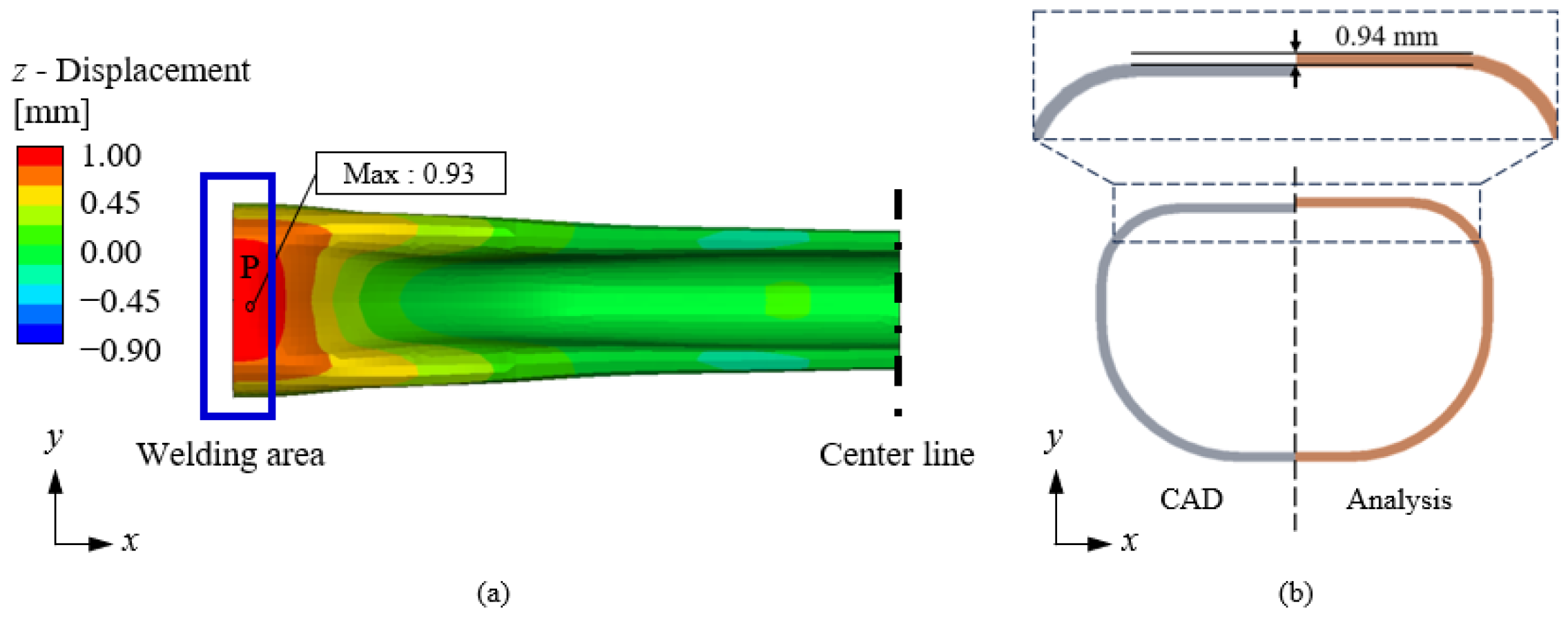
3.2. Quenching Analysis
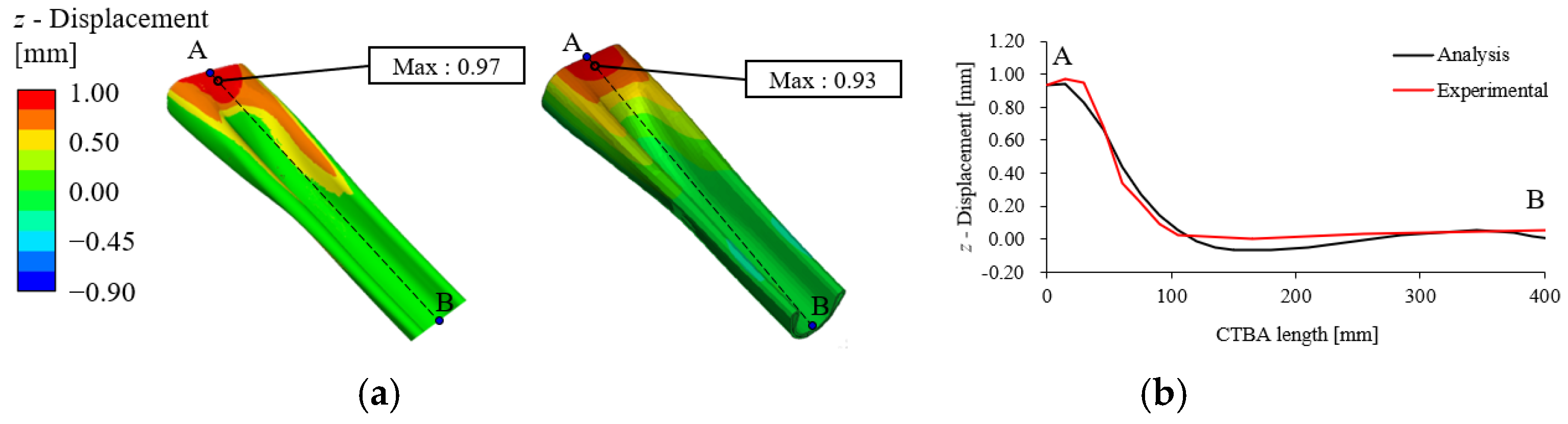
3.3. Validation of the Analysis Process
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- McCrone, D.J.; Margolis, D.; Kim, N. A Bond Graph Approach to Analysis and Simulation of a Coupled Torsion Beam Axle. In Proceedings of the 2019 Spring Simulation Conference (SpringSim), Tucson, AZ, USA, 29 April–2 May 2019; IEEE: Piscataway, NJ, USA, 2019; pp. 1–12. [Google Scholar] [CrossRef]
- Sackl, S.; Leitner, H.; Zuber, M.; Clemens, H.; Primig, S. Induction Hardening vs Conventional Hardening of a Heat Treatable Steel. Metall. Mater. Trans. A 2014, 45, 5657–5666. [Google Scholar] [CrossRef]
- Mühlbauer, A. History of Induction Heating and Melting; Vulkan-Verlag GmbH: Essen, Germany, 2008. [Google Scholar]
- Aswad, M.F.; Mohammed, A.J.; Faraj, S.R. Induction Surface Hardening: A Review. J. Phys. Conf. Ser. 2021, 1973, 012087. [Google Scholar] [CrossRef]
- ScienceDirect. Flux Concentrator. Available online: https://www.sciencedirect.com/topics/engineering/flux-concentrator (accessed on 4 December 2024).
- Hatem, S. Simulation and Implementation of an Induction Heating System for Heating Brittle Thin Metal Sheets by Various Excitation Current Frequencies. Preprint 2023. [Google Scholar] [CrossRef]
- Javaheri, V.; Pohjonen, A.; Asperheim, J.I.; Ivanov, D.; Porter, D. Physically Based Modeling, Characterization and Design of an Induction Hardening Process for a New Slurry Pipeline Steel. Mater. Des. 2019, 182, 108047. [Google Scholar] [CrossRef]
- Tong, D.; Gu, J.; Totten, G.E. Numerical Investigation of Asynchronous Dual-Frequency Induction Hardening of Spur Gear. Int. J. Mech. Sci. 2018, 142–143, 1–9. [Google Scholar] [CrossRef]
- Ferguson, L. Stress and Deformation during Induction Hardening of Tubular Products. In Proceedings of the 6th International Conference on Induction Heating and Heat Treatment of Materials, Chicago, IL, USA, 9–13 September 2012. [Google Scholar]
- Mühl, J.; Pfeifer, T.; Frick, T.; Weiss, D. Simulation of Induction Hardening: Simulative Sensitivity Analysis with Respect to Material Parameters and the Surface Layer State. Comput. Mater. Sci. 2020, 183, 109916. [Google Scholar] [CrossRef]
- Shokouhmand, A.; Sadeghi, M.; Mostafavi, M. Induction Cladding of Alloys and Metal-Matrix Composite Coatings. Mater. Today Proc. 2020, 27, 2034–2042. [Google Scholar] [CrossRef]
- David, W. Permanently Laminated Flux Concentrator Assemblies and Flexible Flux Concentrator Assemblies. KR101671048B1, 25 August 2010. Available online: https://patents.google.com/patent/KR101671048B1/ko (accessed on 4 December 2024).
- Amarulloh, A.; Haikal, H.; Atmoko, N.T.; Utomo, B.R.; Setiadhi, D.; Marchant, D.; Riyadi, T.W.B. Effect of Power and Diameter on Temperature and Frequency in Induction Heating Process of AISI 4140 Steel. Mech. Eng. Soc. Ind. 2022, 2, 26–34. [Google Scholar] [CrossRef]
- Encyclopedia Magnetica™. Skin Depth. Available online: https://www.e-magnetica.pl/doku.php/skin_depth (accessed on 4 December 2024).
- Lee, I.; Tak, S.; Pack, I.; Lee, S. Comparative Study on Numerical Analysis Using Co-Simulation and Experimental Results for High-Frequency Induction Heating on SCM440 Round Bar. J. Aerosp. Syst. Eng. 2017, 11, 1–7. [Google Scholar] [CrossRef]
- Della Posta, G.; Ciri, U.; Leonardi, S.; Bernardini, M. A Novel Two-Way Coupling Method for the Study of the Aeroelasticity of Wind Turbines in a Large Eddy Simulation Framework. In Proceedings of the 14th European Conference on Turbomachinery Fluid Dynamics & Thermodynamics, Gdansk, Poland, 12–16 April 2021. [Google Scholar] [CrossRef]
- Luo, H.; Zhang, X.; Wang, Y.; Jiang, B.; Deng, Z.; Liu, J. Multi-Physics Coupled Numerical Simulation Study to Optimize Process Parameters for Electromagnetic Stirring of Semi-Solid A356 Aluminum Alloy under the Influence of Skin Effect. Int. Commun. Heat Mass Transf. 2024, 157, 107834. [Google Scholar] [CrossRef]
- Ansys. Ansys Granta Materials Data. Available online: https://www.ansys.com/products/materials/materials-data-library#tab1-1 (accessed on 4 December 2024).
- Oh, J.H.; Hong, S.G.; Park, C.K. Influence of Secondary Phase Morphology and Dissolved Carbon on the Plastic Deformation of Transformation-Induced Plasticity Steel. In Proceedings of the Korean Society for Technology of Plasticity Conference, October 1999; pp. 116–119. [Google Scholar]
- Kim, K.W.; Lee, C.H.; Kang, J.Y.; Lee, T.H.; Cho, K.M. Microstructure and Tensile Properties of Commercial TRIP Steel Subjected to Multi-Step Isothermal Transformation Heat Treatment. J. Korean Heat Treat. Soc. 2016, 29, 103–108. [Google Scholar] [CrossRef]
- TRUMPF. TruHeat MF Series 7000 User Manual and Product Specifications. Available online: https://www.trumpf.com (accessed on 7 February 2025).
- GOM ATOS 3D Scanner. Available online: https://www.zeiss.com/metrology/en/systems/optical-3d/3d-scanning/atos/atos-q.html (accessed on 7 February 2025).
- Zeiss Quality Suite. Available online: https://www.handsonmetrology.com/products/software/ (accessed on 7 February 2025).



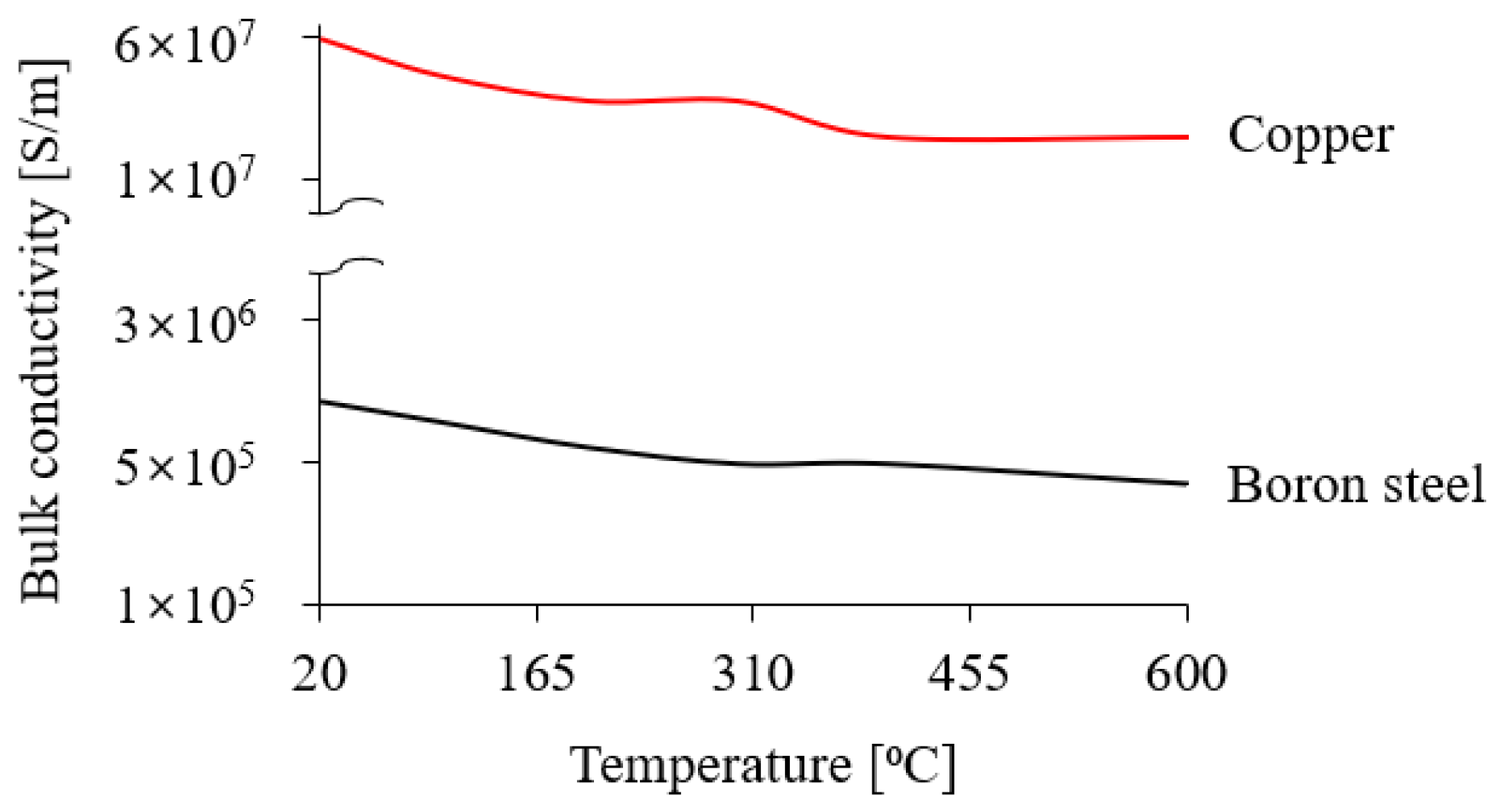


| Properties | Copper | Borron Steel |
|---|---|---|
| Density [g/mm3] | 8.69 | 7.85 |
| Yong’s modulus [GPa] | 110 | 210 |
| Poisson’s ratio [-] | 0.34 | 0.30 |
| Specific heat [J/g °C] | 0.39 | 0.45 |
| Thermal conductivity [W/mm °C] | 0.40 | 0.05 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hwang, S.; Yi, S.; Park, J.; Hong, S. Multiphysics Analysis Process of Front-End Process for Induction Hardening of 3D Structures to Predict Structural Deformation. Appl. Sci. 2025, 15, 2410. https://doi.org/10.3390/app15052410
Hwang S, Yi S, Park J, Hong S. Multiphysics Analysis Process of Front-End Process for Induction Hardening of 3D Structures to Predict Structural Deformation. Applied Sciences. 2025; 15(5):2410. https://doi.org/10.3390/app15052410
Chicago/Turabian StyleHwang, Soonjae, Sarang Yi, Jongkyu Park, and Seokmoo Hong. 2025. "Multiphysics Analysis Process of Front-End Process for Induction Hardening of 3D Structures to Predict Structural Deformation" Applied Sciences 15, no. 5: 2410. https://doi.org/10.3390/app15052410
APA StyleHwang, S., Yi, S., Park, J., & Hong, S. (2025). Multiphysics Analysis Process of Front-End Process for Induction Hardening of 3D Structures to Predict Structural Deformation. Applied Sciences, 15(5), 2410. https://doi.org/10.3390/app15052410





