Abstract
In rock engineering, it is crucial to collect and analyze precursor information of rock failure. This paper has attempted to study the strain rate field of rock-like material to obtain the precursor information of its failure. Based on the available laboratory experiments, the intact BPM (bonded-particle model) and other BPMs with a single open prefabricated flaw were simulated by PFC (Particle Flow Code). The volume strain rate field data before the peak stress have been obtained from two hundred measurement circles across each model. The strain rate field data have been firstly statistically analyzed to explore the failure precursor based on the intact model and 45° flaw model and then compared to find the influence of the pre-existing flaw on the damage evolution and precursor signal. The results indicate that (1) all types of statistical data are positively correlated with the increment of microcracks; (2) corresponding to the fluctuation patterns of statistical data, the damage evolution of BPMs in the pre-peak stage can be divided into three parts; (3) the pre-existing flaw would accelerate the damage evolution; (4) the location and evolution rate of damage could be determined by comprehensively analyzing the average deviation curve, the coefficient of variation, and the contour maps of the strain rate field. These analyses of the particle displacement field can be used to distinguish the impacts of the flaw angle and provide some assistance for the failure forecast.
1. Introduction
The damage evolution and failure precursor of rock-like material are two significant topics in rock mechanics. Under quasi-static compression, the strength, elastic modulus, rock type, defect, and anisotropy of rock and rock-like material can all affect the damage evolution and failure process. Many studies show that the load capacity loss for rocks is a gradual and consequent process under quasi-static compression, and the damage development has three stages (distributed damage, localized damage, and catastrophic failure) [,,,,,]. Micro-cracks, flaws, and pores, existing as defects in rocks and rock-like materials, considerably affect the behaviors of material properties (physical properties, mechanical properties, etc.) and evolve to macro-failure under external loads.
The damage evolution during the failure process of rock-like material is always accompanied by changes in physical properties (electric pulse, electromagnetic radiation, microwave radiation, infrared radiation, acoustic emission, etc.) and surface deformation. Various physical signals, delivered by sophisticated geophysical methods, including magnetometry, gravimetry, geoelectric, electromagnetic, seismic, geothermal, and radiometric, can indirectly characterize the physical and mechanical properties and reflect the damage location []. Different physical signals were used to describe the damage accumulation process of geomaterials among multi-scale analyses and found corresponding information of failure precursors [,,,,,,]. Additionally, the study has shown that the damage localization has been viewed as a precursor to geomaterial failure [].
In related studies, different types of physical information on damage localization have been investigated by laboratory tests, numerical simulation, and theoretical models on recognizing methods, field characteristics, geometric features, development pattern, and other different features in localized damage regions. In studies about the strain field of rock samples, different manifestations of the surface strain field, including linear strain field, shear strain field, volumetric strain field, and apparent equivalent strain, have been adopted to study the strain localization and damage process. Hao et al. [] used the digital speckle correlation method (DSCM) to obtain the spatio-temporal pattern of the first principal strain and the width of the damage localization zone and develop the local-mean-field model to calculate the rupture strain. Cheng et al. [] used the axial and lateral strain field, from digital image correlation (DIC), to describe the rock formation deformation in bedded composite rocks, revealing the failure mechanics of bedded composite rocks with different bedding dip angles. Based on the shear strain field, Ma et al. [] have divided the failure phase into five stages (with the DSCM), and Cheng et al. [] have provided the spatial index and the degree index of the strain field to describe the failure process of pre-existing crack sandstone. Song et al. [] proposed a damage factor, based on the statistic parameter of the apparent equivalent strain, to describe the level of deformation localization. Munoz et al. [] and Sun et al. [] investigated the deformation field evolution characteristics of heterogeneous materials both in pre-peak and post-peak regimes under uniaxial compression.
The existence of discontinuous surfaces in rock material could lead to the deterioration of mechanical properties and accelerate its failure process. Thus, it is significant to study the influence of flaws on various types of physical information during the damage accumulation and at the critical rupture. To investigate the fracture dynamics of SiO2-based materials, Salje et al. [], Nataf et al. [], and Zhao et al. [] have collected and analyzed acoustic emission (AE) signals (the AE activity, amplitude, duration, and energy) in a compression experiment and found the crackling noise distribution of the avalanche criticality. Ma et al. [] and Gao et al. [] have investigated the crack development patterns of rocks under compression stress, including the fracture process zone (FPZ) and the relevant fracture parameters, by measuring the surface strain fields of rock materials. Xianyu et al. [] have investigated the strain feature of single-flaw rock samples with the particle imaging velocimetry (PIV) technique and found that the displacement direction lines significantly changed in the region with flaws. Zhao et al. [] have explained the initiation mechanism of secondary cracks by analyzing the surface strain field, AE event, and distribution during the uniaxial compression tests of rock-like specimens with an internal open-type flaw. Ding et al. [] have observed the AE and charge signal of coal compression tests and found that the richness of the signal increases with the deterioration of coal. Li et al. [] described the strain dispersion of complex fractured rock masses by the standard deviations of the strain field and quantitatively identified the crack initiation stress (CIS). Misra et al. [] have built an elastic–plastic rheological model to calculate the specific crack orientation resulting in different types of crack propagation based on the variability of deformation localization near pre-existing shear cracks. Zhou et al. [] have built a micromechanics-based model, taking the interaction among sliding cracks into account, for analyzing the damage and deformation localization of brittle rock.
Furthermore, the strain field of rock-like material has been statistically analyzed to describe the damage accumulation and some precursor signals of the final collapse. Wang et al. [] explored that the variation coefficients of the vertical linear strain and the maximum shear strain might be the failure precursors of the coal specimens under uniaxial compression. Zhang et al. [] have found that the differential rate of the strain field–axial strain could be used to recognize the precursor before the failure of the fractured rock mass. Through analyzing the absolute standard deviation (ASD) and absolute variation coefficient (AVC) of the strain field obtained from laboratory tests and numerical simulation, Wang et al. [] found that the variation laws of the ASD and AVC of the shear strain field were highly correlated with the rock fracture evolution and obtained the precursory indices of full-field strain information. Some researchers developed a damage variable based on the standard deviation of the apparent equivalent strain fields to evaluate the damage degree of rock samples [,,]. Zhang et al. [,] have scanned the full-field view of axial and circumferential strains on the sandstone specimen surfaces by the distributed fiber optic strain sensing (DFOSS) technology and detected the initialization of microcrack nucleation.
This paper has used two-dimensional (2D) discrete element models to simulate the whole damage process of the intact and fractured rock based on the compression tests of the rock-like material samples. The volumetric strain rate field was obtained by 200 measurement circles covered on each BPM. The statistical data of the strain rate fields, including the mean, variance, simple mean deviation, and correlation coefficient, were analyzed in relation to the characteristics of the damage evolution and failure precursor among the pre-peak stage of compression tests. Moreover, the results of the intact BPM were compared with the results of the BPM with 45° flaw to find the influence of pre-existing flaws on those characteristics. Based on the results of the 2D models, the evolution characteristics of localized deformation have been quantitatively analyzed. Meanwhile, combined with strain rate contour map, the precursory characteristics of the instability of intact rock mass and fractured rock mass are analyzed comparatively.
2. Numerical Simulations
According to the laboratory test results in the paper by Jin et al. [], a set of numerical models were generated by Particle Flow Code (PFC). As a discrete element method, the particles and bonds determine the behavior of the macro material, and it can indicate a similar simulation result in the macro-phenomenon of specimen’s failure []. The particle discrete element model provides support to comprehend the failure process of rock-like material from macroscopic-scale phenomena and statistics. In this paper, the parallel bond was used to generate the bonded-particle models (BPMs).
In the laboratory test, cement, sand, and water were used to make samples with a size of 50 mm (length) × 100 mm (height) × 30 mm (width) []. The ratio of the sample length to height complies with the ISRM standards. Following the block size in the laboratory test, the simulated specimens in the PFC were 100 mm high and 50 mm long. We adopted the two-dimensional models to investigate the surface deformation characteristics. Therefore, the results and conclusions only involve two-dimensional situations and might be slightly different from actual situations or three-dimensional models.
The intact BPM has a uniform particle-size distribution bounded by Rmin (0.25 mm) and Rmax (0.415 mm). The micro-parameters of the parallel bonds were determined by the calibration process of the simulated uniaxial compression test of the intact model. The parameters of the parallel bonds were adopted when the simulated results reproduced the laboratory test values, including ball–ball contact modulus (Ec) of 2.5 GPa, normal strength (σn) of 19 MPa, shear strength (σs) of 27.1 MPa, and ball stiffness ratio (kn/ks) of 2.5. The macro properties of the intact specimens in the physical experiment and numerical simulation are listed in Table 1, which proves the rationality of the numerical model. The uniaxial compressive strength of the cement specimens corresponds to some kinds of homogeneous and isotropic sandstone and argillite shale. After the reproduction of the intact sample, the specimen with 45° flaw was created by deleting some particles in a specific area. The defined flaw in the model had the same geometry as that in the experimental samples.

Table 1.
Material properties of physical experiment and numerical study.
Figure 1 shows the stress–strain curves of intact and flawed samples in the laboratory test and numerical simulation. It is apparent from Figure 1 that the stress–strain curve of the BPM has an obvious similarity with those of the physical test, both in the intact sample and the flawed sample, indicating the models are feasible.
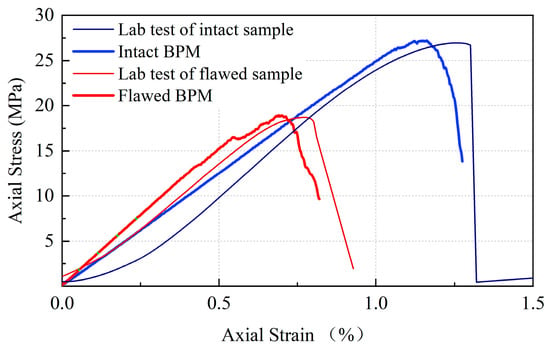
Figure 1.
Stress–strain curves of PFC models and tests.
The numerical models and their failure modes are shown in Figure 2. The regularly arranged small circles on the model surface are measurement circles (MCs), which are used to obtain a further investigation about the process of the deformation localization of the specimen. The final collapses of the intact specimens in Figure 2a show a shear failure with shear cracks (blue dot) and a few tensile cracks (red dot). At 45° tips, small slippages emerged, and tensile cracks interpenetrated each other, while shear cracks quickly developed to lead to failure, as shown in Figure 2b.
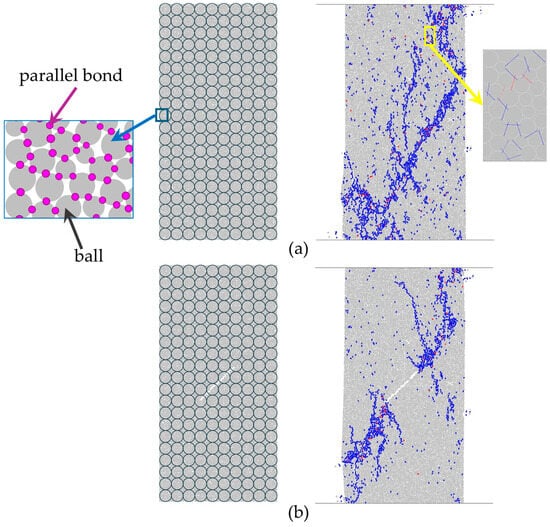
Figure 2.
Measurement circle arrangement (left) and failure modes of numerical simulations (right). (a) Intact BPM; (b) 45° flaw BPM.
3. Results and Discussion
3.1. Case 1: Intact Specimen
During the process of compression, the volume strain rate of each measurement circle has been automatically recorded every 200 steps. Statistical analysis of the volume strain rates were made to get the average, the standard deviation (SD), the range, and the average deviation (AD) at each recorded time. From the statistics, it has been found that the occurrence of floater particles can cause extremely high values of the MC strain rate and significantly impact the statistics at corresponding moments. In subsequent analysis, the measurement circle with floater particles was removed from the data array.
The extremum MC strain rates before the peak stress are plotted in Figure 3. The black curve and blue curve indicate the maximum and minimum value of the strain rate, respectively; the red lines represent the crack increment; and the purple curve represents the average deviation of the strain rate. The bottom axis reflects the normalized strain (from 0% to 100%); and the 100% normalized strain matches the total strain at the peak strength of the intact numerical model.
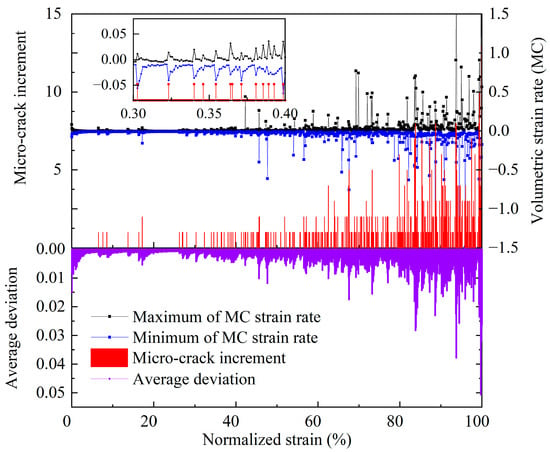
Figure 3.
Extremum values and average deviation of MC strain rate and micro-crack increment (case 1).
In the magnified part of Figure 3, the corresponding gaps between the strain rate extremum curves, i.e., the strain rate ranges, are obviously larger when the micro-cracks increase. This indicates that the variation patterns of the strain rate largely reflect the micro-crack generation and damage evolution of the BPM. Thus, the statistical analysis of the MC strain rates might be used to identify the failure process of specimens and to explore methods for failure predicting.
In Figure 3, before the 30% peak strain, the micro-crack increment emerges occasionally; between 30 and ~60% strain, its value and frequency both change a little; in the last section, its curve has sharp rises. The AD curve is similar to the micro-crack increment. All curves in Figure 3 indicate that the damage accumulation of the intact specimen accelerates after 60% strain and becomes much larger after 80% strain.
Figure 4 shows the average and standard deviation of the MC strain rate at the pre-peak part of the intact specimen simulation. The SD curve (the green one) has larger fluctuations, compared with the average curve. Based on the tendency of the green curve, the SD variation can be split into four phases: phase 1, at 0~40% strain, the values of the SD are close to zero; at 40~60% strain, the green curve has some peaks; at 60~80% strain, fluctuations of the SD curve become denser and larger than before; at 80~100% strain, the larger fluctuations emerge intensively.
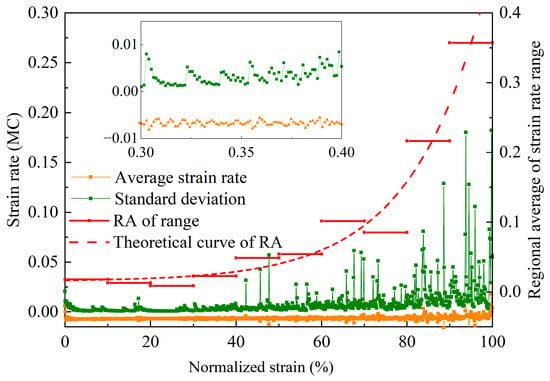
Figure 4.
The standard deviation, average, and range regional averages of MC volume strain rate (case 1).
For a further investigation of the damage stage of the intact BPM, the regional averages for the MC strain rate ranges (RA) have been calculated at each 10% strain, which appear as red segments in Figure 4. Those segments can be characterized as four groups: 0~40% strain is group 1, in which part of the regional average has slightly changed; 40~60% strain is group 2, in which part of the red segments gently increase; 60~80% strain is group 3, in which part of the RA has an obvious integral uplift; and 80~100% strain is group 4, in which part of the RA has a significant growth. By comprehensively analyzing the trends of the RA and SD curves, it can be concluded that the damage evolution of the intact BPM can be seen as three sections: the first section is between 0 and ~40% strain; the second section is between 40 and ~80%; the section is between 80 and ~100% strain.
Through data fitting, it has been found that the relationship of the RA and loading strain fit into the exponential function. This can be described by the following exponential function:
It is a one-phase exponential decay function with a time constant parameter. The theoretical curve is shown as the red dashed line. The fluctuations of the SD curve in Figure 4 indicate that the MC strain rates have a larger dispersion degree as the total strain reaches closer to the peak stress. Therefore, there is a significant correlation between the dispersion of the MC strain rate and the degree of specimen failure.
The coefficient of variation (CV) for each strain rate field was calculated and plotted as a red curve in Figure 5. Then, the averages of 20 contiguous CVs were calculated and plotted as a blue curve in Figure 5. These two curves could be used to analyze the relationship between the dispersion of the strain rate data and the damage evolution of the intact specimen.In this figure, the blue curve has more obvious characteristics that can distinguish the damage stages of pre-peak loading. From 30% to 70% strain, the curve of the strain-contrast RA of the CVs ascends slowly with some slight waves; from 70% to 80% strain, the waves become larger; between 0.8 and ~1.0 strain, the extremely volatile growth of the blue curve correspond with the rapid damage accumulation in the BPM.
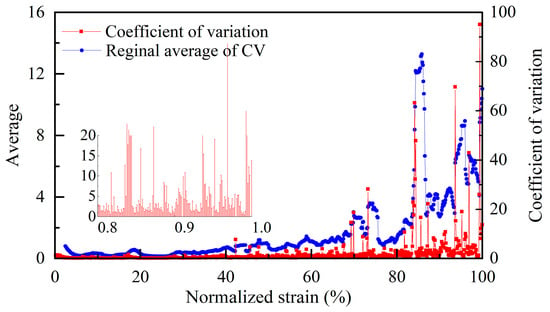
Figure 5.
Coefficient of variation of strain rate field and its regional average (case 1).
To further analyze the evolution of the MC strain rate distribution during the loading process, eight contour maps of the strain rate field at different moments were investigated, as shown in Figure 6. The Z-axes values illustrate the volume strain rate of each measurement circle. A positive strain rate indicates an increase in volume strain, and vice versa. Under uniaxial compression, negative strain rates are the main component of the strain rate field; however, a positive strain rate might occur in situations such as particle ejection at the edge of the specimen, shear dilation, and tensile failure of bonds.
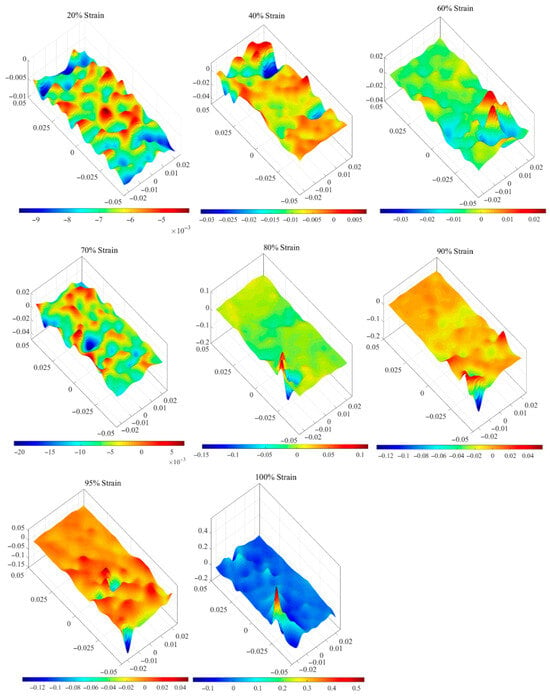
Figure 6.
Strain rate field at different strains (case 1).
In Figure 6, at 20% peak strain, the strain rate field has slight waves uniformly distributed over the whole surface with negative values. At this moment, the intact specimen is in the stage of crack closure. At 40%, 60%, and 70% strain, a few high ratios of MC strain rates randomly emerge in the strain rate field, corresponding to the growth pattern of microcracks in Figure 3. From 40% to 70% strain, the specimen is in the stage of stable crack growth. From 80% to 100% strain, the extreme values of the MC strain rate are larger than ever before and concentrate in the left-bottom side of the sample. This indicates that the damages concentrate in that particular area, and the specimen moves into the stage of unstable crack growth.
Figure 6 represents the failure process of this intact BPM. From scattered to centralized, the damage distribution changed by the load increment and finally collapsed because of damage localization at the left-bottom side of the BPM. The variation of these contour maps confirms the micro-crack increase tendency and other statistical data curves.
As mentioned above, the pre-peak failure process of the intact sample could be divided into three or four sections: the first section is before 30% peak strain; section 2 can be seen as two parts: one is between 30 and 60% peak strain and the other is between 60 and 80% peak strain; the last section is between 80 and 100% strain.
In section 1, the micro-crack increment occasionally and separately emerged; all three statistics (average, SD, and CV) of the MC strain rates are close to zero and have minor up and down patterns; the RA values of the range are decreasing and close to zero; the strain rate field displays regular undulation with negative values. These performances indicate that section 1 corresponds to the crack closure stage and linear elastic deformation stage in which the damage accumulation is essentially close to zero.
In the first stage of section 2 (section 2-1), approximately from 30% strain to 60% strain, the micro-crack increment has shown relatively stable growth; the SD values have some peaks; the RA values of the range and CV both slightly increase; and the strain rate fields show a few randomly independent peaks at 40% and 60% peak strain.
In the second stage of section 2 (section 2-2), approximately from 60% strain to 80% strain, the micro-crack growth basically keeps a constant speed, which is higher than the previous stage of section 2. Meanwhile, the values of the RA of the range and CV both become larger than part one of this section. These phenomena indicate that section 2 corresponds to the stage of stable crack growth. Additionally, in this section, the damages steadily scattered and increased in the specimen.
In section 3, the micro-crack increment is significantly higher than those of section 1 and 2; all statistics and regional average of the CV of the strain rate field are extremely volatile; and the RA of the range increases rapidly. Meanwhile, from 90% to 100% peak strain, the strain rate extremums concentrate near the left-bottom side of the intact model. These phenomena indicate that section 3 corresponds to the stage of unstable crack growth and failure. In this section, the localization deformation section, the damage rapidly grows in a centralized area till the BPM collapses.
3.2. Case 2: Specimen with an Open Flaw
To study the influence of the pre-existing flaw on damage procession, the corresponding data of specimen with 45° flaw have been calculated and statistically analyzed.
Figure 7 has shown the strain rate extremums of the MC and micro-crack increment in the pre-peak stage of the flawed specimen, with the same legends as Figure 3. The micro-crack increment (red bars) appears intermittently before 50% peak strain; then, the red bars become crowded till 80% strain; at last, the red bars become much longer and more intense. Between 80% strain and 100% strain, there are four peaks on the maximum curve, accompanied by the rapid growth of micro-cracks.
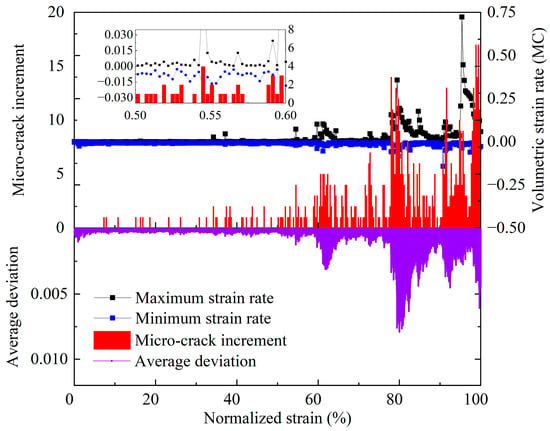
Figure 7.
Extremum values and average deviation of MC strain rate and micro-crack increment (case 2).
The average, standard deviation (SD), and range RA of the MC strain rate at the pre-peak part of the flawed specimen are shown in Figure 8 (the legends are consistent with Figure 3). The SD of the strain rate field is close to zero before 60% strain and has three peaks between 60 and ~100% strain. The average curve shows the same tendency as the SD curve.
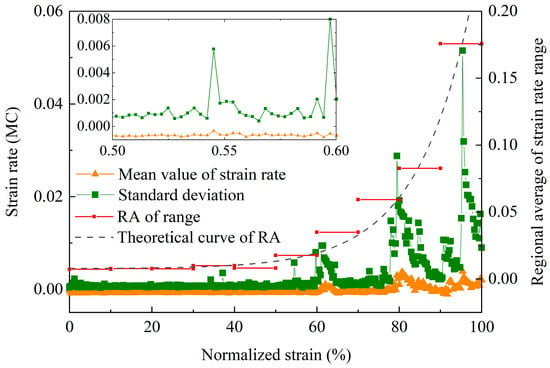
Figure 8.
The standard deviation, average, and range regional averages of MC volume strain rate (case 2).
In Figure 8, the rise height of the range RA segments becomes higher as the loading strain increases, and the skip distance between the last two red segments is obviously larger than before. Compared to the intact BPM, the red segments of the flawed BPM become well regulated and have more uplifts after 50% strain. By fitting the range RA data with an exponential function, we obtain the following exponential function:
The regional average data fits into the exponential function, and the theoretical curve is shown as the black dash curve. Equations (1) and (2) both are a one-phase exponential decay function with a time constant parameter. These two equations have the same time constant 0.17259, but the parameters of the offset and amplitude both reduce to 50% in Equation (2). Compared with the theoretical curve of the single-flaw specimen in Figure 8, the curve of the intact model in Figure 4 is steeper. It indicates that the regional averages of the strain rate range of the intact model are higher than that of the flawed model when the strain proportions were coincided in the two cases.
Figure 9 shows the coefficient of variation in case 2 (the red curve) and the average of 20 contiguous CVs (the blue curve). The blue curve can be loosely divided into two parts: the line part before 60% US strain and the wave part with three waves.
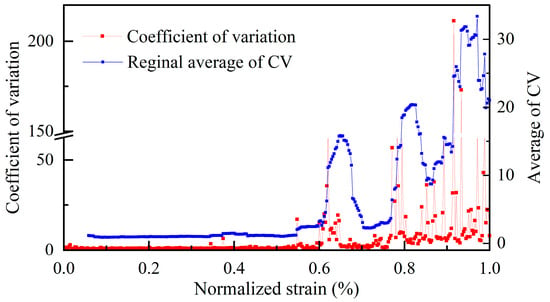
Figure 9.
Coefficient of variation of strain rate field and its region average (case 2).
In Figure 9, between 60% and 90% strain, the blue curve has two waves with clear peaks and troughs; meanwhile, the CV curve has fluctuations that appear at intervals. From approximately 87% strain to 100% strain, the last wave on the blue curve has fluctuated upwards higher than ever before. The blue curve shape in case 2 is significantly different from the corresponding curve in case 1. The existence of the flaw has made the variation pattern of the CV and its regional average more regular and more distinguished.
Eight contour maps of MC strain rates at critical moments are shown in Figure 10, providing help to determine the damage localization of the flawed sample. In contour maps of 20% and 40% strain, the high ratio strain rates surround the pre-existing flaw, and there is basically no change in other areas. This shows that the strain localization appears near the flaw during the initial stage of loading. It is different from the first stage of case 1 that the damage concentration begins from the initial loading period, omitting the scattered damage stage.
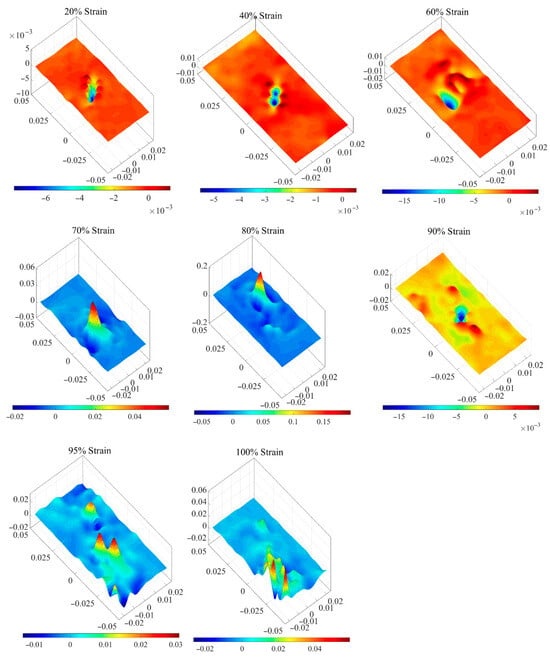
Figure 10.
Strain rate of MC at different loading strains (case 2).
In the contour map of 60% peak strain, the areas with high ratio strain rates move to the flaw tips. When the strain reaches 70% and 80% peak strain, the extreme values of the strain rate field move with crack propagation. This indicates that the damage accumulates with crack propagation, and some tensile micro-cracks form at the crack tips.
The strain rate field at 90% strain shows a relatively smooth surface during the later stage of the pre-peak loading, with peaks dispersed along the wing crack boundaries. At 90% strain, the micro-crack increment and all preliminary statistics are close to zero.
In 95% and 100% strain, the fluctuations of the strain rate field are distributed along the wing cracks and become more congested. From Figure 10, it can be seen that the deformation localization started around flaw and then moved to the edges of cracks with the loading increasing.
By comprehensively analyzing the above results, we can tell that the pre-existing flaw has made the strain localization form early and the variation patterns of the statistical data more regular and distinguished. The pre-peak failure process of the flawed sample could be divided into three sections: section 1 is before 50% peak strain; section 2 is between 50% and 90% peak strain; section 3 is between 90% and 100% peak strain.
In section 1, the micro-crack increment occasionally emerged, as did peaks in the strain rate field around the flaw; meanwhile, all of the statistic curves of the MC strain rates are basically straight lines approaching zero. These phenomena indicate that section 1 corresponds to the stage of the crack closure and the linear elastic deformation stage in which the deformation localization occurs around the pre-existing flaw. The damage evolution has skipped the stage of distributed damage.
In section 2, approximately from 50% strain to 90% strain, the micro-crack growth rate becomes larger; the strain rate peaks follow the tips of the crack propagation; the curves of three statistics and the coefficient of variation all have two waves around 60% and 80% strain, corresponding to the crack initiation and stable propagation; the rise height of the range RA segments gradually become larger as the loading strain increases. These performances indicate that section 2 corresponds to the stage of crack initiation and stable crack growth in which the localized damage accumulation is relatively steady. However, the micro-crack growth presents staged and whitespace between two peaks.
In section 3, approximately from 90% strain to 100% strain, the values of SD and CV both have their largest fluctuations; the RA of the range surges at the last segment; the RA of the CV fluctuates to the highest peak; the strain rate extremums significantly increase and concentrate along the crack propagation path. These phenomena indicate that cracks have unstable growth and coalescence in section 3. They show that section 3 corresponds to the later stage of localized damage and the stage of catastrophic failure in which the damage rapidly grows in a centralized area till the BPM collapses.
3.3. Comparative Analysis
The correlation coefficients between the micro-crack increment and four types of statistics (the range, average, average deviation, and standard deviation) on the MC strain rates have been calculated and plotted in Figure 11. All of the correlation coefficients are positive, which illustrates that the crack increment is positively correlated with the four types of statistics both in case 1 and case 2. Three types of statistics in case 2 are lower than those in case 1, meaning that the existence of a flaw would degrade the correlation between the strain rate field and damage evolution and reduce the dispersion of the strain rate field.
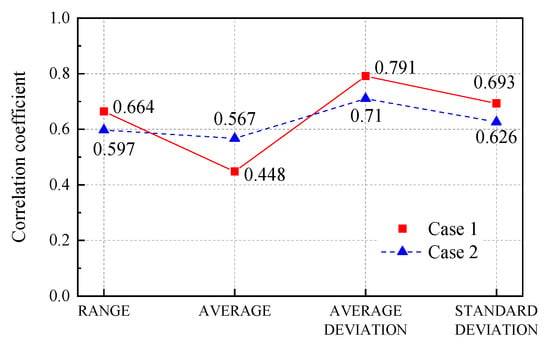
Figure 11.
Correlation coefficient between micro-crack increment and statistical data of MC strain rate.
In Figure 11, these two curves have a similar tendency: the minimum of each case is the correlation coefficient between the strain rate average and crack increment; the maximum of each case is the correlation coefficient between the strain rate average deviation and crack increment. This means that the crack growth is highly correlated with the average deviation. Therefore, the average deviation of the MC strain rate field is a better choice for making discriminating judgments and evaluations of specimen damage evolution.
The volume strain rates of MCs and their statistical data could illustrate the damage evolution during the loading process from varying points and degrees. For integrating preceding analysis, the applications of each parameter have been collected and listed in Table 2. In this table, the plus sign (+) means a parameter could facilitate the corresponding function, and the minus sign (−) has the opposite meaning.

Table 2.
Parameter indication on different applications.
Summarizing the above work, Table 2 shows the following results: the MC strain rate contour maps can tell the damage position; the average deviation can judge the crack increment; the average fluctuation and other statistics can indicate the damage stage of samples. Therefore, comprehensive analysis of the MC strain rates and the corresponding statistics can be used for the collapse prediction of rock-like material.
4. Conclusions
Based on the laboratory uniaxial compression test of the rock-like material, this paper builds the corresponding numerical models for an intact sample and a sample with a 45° flaw. In the numerical simulations, the measurement circles are uniformly distributed on numerical specimens to monitor the variation of the volumetric strain rate field. We analyzed the five types of statistics (average, range, average deviation, standard deviation, and coefficient of variation) and drew the contour maps of the strain rate field. We obtained the following conclusions:
- Several relationships between the damage evolution of the rock-like material and statistical data of the volumetric strain rate field have been built in this paper. The micro-crack increasement, or the damage accumulation, corresponds to large gaps between the strain rate extremums. In the stage of the pre-peak loading, statistical data always have growth and volatility, and the corresponding regional averages rise higher as the strain increases. The results showed that all types of statistical data positively correlate with the microcrack increment, and the regional average of the strain rate range has an exponential raise as the strain increases.
- Based on the comprehensive analysis of the variation characteristics of all MC strain rate statistic data, the damage evolution of the pre-peak loading stage could be separated into three sections. In section 1, the strain rate field is relatively flat, and all the statistical data are flat. In section 2, all types of statistic data of case 1 have different variation patterns from those of case 2. However, in section 3, relatively large - scale local fluctuations occur in the strain rate field,, and all the statistical data rapidly increased. Among all types of statistics, the standard deviation, the regional average of range, and the CV are more distinguished than others.
- Due to the pre-existing flaw, the development of the strain localization of case 1 and case 2, the intact sample, and the flawed sample is different from each other. The pre-existing flaw accelerates the damage evolution. The intact specimen in section 1 has a strain rate field with a regular fluctuation pattern, and, in section 2-1, the strain rate field has peaks randomly distributed. The damage evolution of the intact sample developed to the stage of the distributed damage. Meanwhile, the strain rate field of the flawed sample has the strain localization around the flaw. In section 2 and section 3, the pre-existing flaw has made all curves of the statistics fluctuate with some regular patterns.
- By taking full advantage of the volumetric strain rate field, the status of the damage evolution of the rock-like material sample can be analyzed and evaluated. The field contour maps of the MC strain rate can tell the damage position; the average deviation curve can judge the crack increment; the region average of the variation coefficient can indicate the damage section. This strain rate field can provide assistance on the prediction of catastrophic failure of rock-like material.
Author Contributions
Conceptualization, J.J. and P.C.; methodology, J.J. and J.Z.; software, J.J., P.C., and C.M.; validation, J.L.; resources, J.J., P.C., J.Z., and X.B.; data curation, J.J., Y.W., and J.L.; writing original draft preparation, J.J.; funding acquisition, J.J., Y.W., and C.M.; supervision, X.B. All authors have read and agreed to the published version of the manuscript.
Funding
This research was financially supported by Fundamental Research Program of Shanxi Province (Program Nos. 202303021212078 and 202303021224001); the National Natural Science Foundation of China (NSFC) (No. 52178341); the Research Project of Science and Technology Department of Shanxi Province (No. 202203021222428).
Institutional Review Board Statement
Not applicable.
Informed Consent Statement
Not applicable.
Data Availability Statement
The data used to support the findings of this study are available from the corresponding author upon request.
Acknowledgments
We thank Central South University for their technical support.
Conflicts of Interest
Authors Jin Jin, Jun Zhang, Yanchao Wang and Chenxi Miao were employed by the company Shanxi Province Transportation Technology Research and Development Co., Ltd. The remaining authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.
References
- Cook, N.G.W. The failure of rock. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1965, 2, 389–403. [Google Scholar] [CrossRef]
- Ashby, M.F.; Sammis, C.G. The damage mechanics of brittle solids in compression. Pure Appl. Geophys. 1990, 133, 489–521. [Google Scholar] [CrossRef]
- Martin, C.D.; Chandler, N.A. The progressive fracture of Lac du Bonnet granite. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1994, 31, 643–659. [Google Scholar] [CrossRef]
- Xu, X.; Ma, S.; Xia, M.; Ke, F.; Bai, Y. Damage evaluation and damage localization of rock. Theor. Appl. Fract. Mech. 2004, 42, 131–138. [Google Scholar] [CrossRef]
- Liang, C.; Li, X.; Wang, S.; Li, S.; He, J.; Ma, C. Experimental investigations on rate-dependent stress-strain characteristics and energy mechanism of rock under uniaixal compression. Yanshilixue Yu Gongcheng Xuebao/Chin. J. Rock Mech. Eng. 2012, 31, 1830–1838. [Google Scholar]
- Nikolic, M.; Ibrahimbegovic, A. Rock mechanics model capable of representing initial heterogeneities and full set of 3D failure mechanisms. Comput. Methods Appl. Mech. Eng. 2015, 290, 209–227. [Google Scholar] [CrossRef]
- Schön, J.H. Physical Properties of Rocks—Fundamentals and Principles of Petrophysics (first edition); Pergamon Press, Ltd.: Oxford, UK, 2004; pp. 3–4. [Google Scholar]
- Soloviev, S.P.; Spivak, A.A. Electromagnetic signals generated by the electric polarization during the constrained deformation of rocks. Izv. Phys. Solid Earth 2009, 45, 347–355. [Google Scholar] [CrossRef]
- Larionov, I.; Malkin, E.; Uvarov, V. Deformation-Electromagnetic Relations in Lithospheric Activity Manifestations. E3s Web Conf. 2018, 62, 03002. [Google Scholar] [CrossRef]
- Song, X.; Li, X.; Li, Z.; Cheng, F.; Zhang, Z.; Niu, Y. Experimental research on the electromagnetic radiation (EMR) characteristics of cracked rock. Environ. Sci. Pollut. Res. 2017, 25, 6596–6608. [Google Scholar] [CrossRef]
- Carlà, T.; Intrieri, E.; Di Traglia, F.; Nolesini, T.; Gigli, G.; Casagli, N. Guidelines on the use of inverse velocity method as a tool for setting alarm thresholds and forecasting landslides and structure collapses. Landslides 2016, 14, 517–534. [Google Scholar] [CrossRef]
- Liu, S.; Xu, Z.; Wei, J.; Huang, J.; Wu, L. Experimental Study on Microwave Radiation From Deforming and Fracturing Rock Under Loading Outdoor. IEEE Trans. Geosci. Remote Sens. 2016, 54, 5578–5587. [Google Scholar] [CrossRef]
- Shi, D.; Wang, J.; Xiong, L. Study on Noise Correction Algorithm of Infrared Emissivity of Rock under Uniaxial Compression. Sustainability 2022, 14, 12769. [Google Scholar] [CrossRef]
- Huang, L.-Q.; Wu, X.; Li, X.-B.; Wang, S.-F. Influence of sensor array on MS/AE source location accuracy in rock mass. Trans. Nonferrous Met. Soc. China 2023, 33, 254–274. [Google Scholar] [CrossRef]
- Bai, Y.; Xia, M.; Ke, F.; Li, H. Damage Field Equation and Criterion for Damage Localization. In IUTAM Symposium on Rheology of Bodies with Defects; Solid Mechanics and its Applications; Wang, R., Ed.; Springer: Dordrecht, The Netherlands, 1999; Volume 64, pp. 55–66. [Google Scholar] [CrossRef]
- Hao, S.; Wang, H.; Xia, M.; Ke, F.; Bai, Y. Relationship between strain localization and catastrophic rupture. Theor. Appl. Fract. Mech. 2007, 48, 41–49. [Google Scholar] [CrossRef]
- Cheng, J.; Yang, S.; Chen, K.; Ma, D.; Li, F.; Wang, L.M. Uniaxial experimental study of the acoustic emission and deformation behavior of composite rock based on 3D digital image correlation (DIC). Acta Mech. Sin. 2017, 33, 999–1021. [Google Scholar] [CrossRef]
- Ma, S.; Zhou, H. Surface strain field evolution of rock specimen during failure process. Chin. J. Rock Mech. Eng. 2008, 27, 1667–1673. [Google Scholar]
- Cheng, H.; Yang, X.; Zhang, C.; Ning, Z.; Li, Y. Characteristics of heterogeneous deformation and identification model of unstable damage state in pre-existing crack sandstone. J. Min. Saf. Eng. 2023, 40, 1290–1300. [Google Scholar] [CrossRef]
- Song, H.; Zhang, H.; Fu, D.; Kang, Y.; Huang, G.; Qu, C.; Cai, Z. Experimental study on damage evolution of rock under uniform and concentrated loading conditions using digital image correlation. Fatigue Fract. Eng. Mater. Struct. 2013, 36, 760–768. [Google Scholar] [CrossRef]
- Munoz, H.; Taheri, A.; Chanda, E.K. Pre-Peak and Post-Peak Rock Strain Characteristics During Uniaxial Compression by 3D Digital Image Correlation. Rock Mech. Rock Eng. 2016, 49, 2541–2554. [Google Scholar] [CrossRef]
- Sun, Q.; Cai, C.; Zhang, S.; Tian, S.; Li, B.; Xia, Y.; Sun, Q. Study of localized deformation in geopolymer cemented coal gangue-fly ash backfill based on the digital speckle correlation method. Constr. Build. Mater. 2019, 215, 321–331. [Google Scholar] [CrossRef]
- Salje, E.K.; Soto-Parra, D.E.; Planes, A.; Vives, E.; Reinecker, M.; Schranz, W. Failure mechanism in porous materials under compression: Crackling noise in mesoporous SiO2. Philos. Mag. Lett. 2011, 91, 554–560. [Google Scholar] [CrossRef]
- Nataf, G.F.; Castillo-Villa, P.O.; Baró, J.; Illa, X.; Vives, E.; Planes, A.; Salje, E.K. Avalanches in compressed porous SiO2-based materials. Phys. Review. E Stat. Nonlinear Soft Matter Phys. 2014, 902, 022405. [Google Scholar] [CrossRef] [PubMed]
- Zhao, Y.; Liu, H.; Xie, K.; Salje, E.K.; Jiang, X. Avalanches in Compressed Sandstone: Crackling Noise under Confinement. Crystals 2019, 9, 582. [Google Scholar] [CrossRef]
- Ma, J.; Li, D.; Luo, P.; Zhang, C.; Gao, F. Dynamic Damage and Failure of Layered Sandstone with Pre-cracked Hole Under Combined Cyclic Impact and Static Loads. Rock Mech. Rock Eng. 2022, 56, 2271–2291. [Google Scholar] [CrossRef]
- Gao, G.; Yao, W.; Xia, K.; Li, Z. Investigation of the rate dependence of fracture propagation in rocks using digital image correlation (DIC) method. Eng. Fract. Mech. 2015, 138, 146–155. [Google Scholar] [CrossRef]
- Xianyu, W.; Lu, X.; Zhao, Q.; Han, G. Strength and deformation characteristics of rock with single pre-existing fissure under different loading conditions. China Meas. Test 2017, 43, 124–129. [Google Scholar]
- Zhao, Y.; Gao, Y.; Wu, S.; Chen, L.; Zhang, C. Experimental and numerical study of failure characteristics of brittle rocks with single internal 3D open-type flaw. Acta Geotech. 2021, 16, 3087–3113. [Google Scholar] [CrossRef]
- Ding, X.; Xiao, X.-C.; Lv, X.-F.; Wu, D.; Pan, Y. Analysis of Similarities and Differences between Acoustic Emission and Charge Signal Based on Fractal Characteristics of Coal Fracture. Adv. Civ. Eng. 2020, 2020, 8745039. [Google Scholar] [CrossRef]
- Li, N.; Zhang, K.; Bao, R.; Fan, W. Quantitative description of fracture behavior and mechanical anisotropy of fractured rock mass: Sand 3D printing and DIC explorations. Theor. Appl. Fract. Mech. 2023, 127, 104065. [Google Scholar] [CrossRef]
- Misra, S. Deformation localization at the tips of shear fractures: An analytical approach. Tectonophysics 2011, 503, 182–187. [Google Scholar] [CrossRef]
- Zhou, X.; Zhang, Y.; Ha, Q.; Wang, J. Analysis of the localization of damage and the complete stress-strain relation for mesoscopic heterogeneous brittle rock subjected to compressive loads. Appl. Math. Mech. 2004, 25, 1039–1046. [Google Scholar] [CrossRef]
- Wang, X.; Hou, W.; Dong, W. Assessment of the failure precursors of the strain fields for the coal specimens in the uniaxial compression based on the digital image correlation method. J. Saf. Environ. 2018, 18, 1237–1245. [Google Scholar] [CrossRef]
- Zhang, K.; Li, N. Evolution law of strain field and precursor identification of flawed sandstone based on Digital Image Correlation method. Hydrogeol. Eng. Geol. 2021, 48, 150–156. [Google Scholar] [CrossRef]
- Wang, P.; Liu, Q.; Zhang, Y.; Huang, Z. Identifying rock fracture precursor by multivariate analysis based on the digital image correlation technique. Theor. Appl. Fract. Mech. 2023, 126, 103987. [Google Scholar] [CrossRef]
- Zhu, J.; Zhai, T.; Liao, Z.; Yang, S.; Liu, X.; Zhou, T. Low-Amplitude Wave Propagation and Attenuation Through Damaged Rock and a Classification Scheme for Rock Fracturing Degree. Rock Mech. Rock Eng. 2020, 53, 3983–4000. [Google Scholar] [CrossRef]
- Zhang, L.; Yang, D.; Chen, Z.; Liu, A. Deformation and failure characteristics of sandstone under uniaxial compression using distributed fiber optic strain sensing. J. Rock Mech. Geotech. Eng. 2020, 12, 1046–1055. [Google Scholar] [CrossRef]
- Hegger, S.; Vlachopoulos, N.; Poles, T.; Diederichs, M.S. Measuring the Full-Field Strain Response of Uniaxial Compression Test Specimens Using Distributed Fiber Optic Sensing. Rock Mech. Rock Eng. 2021, 55, 2615–2630. [Google Scholar] [CrossRef]
- Jin, J.; Cao, P.; Chen, Y.; Pu, C.; Mao, D.; Fan, X. Influence of single flaw on the failure process and energy mechanics of rock-like material. Comput. Geotech. 2017, 86, 150–162. [Google Scholar] [CrossRef]
- Sun, Q.; Liu, X.; Zhang, G.; Liu, C.; Jin, F. The mesoscopic structures of dense granular materials. Adv. Mech. 2017, 47, 263–308. [Google Scholar] [CrossRef]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).