Digital Image Correlation and Reliability-Based Methods for the Design of Structural Beams Made from Recycled Concrete Using Aggregates from Precast Rejects
Abstract
1. Introduction
2. Materials and Methods
2.1. Materials and Mix Proportions
2.2. Mechanical Characterisation of the Concrete
2.2.1. Test Set up and Specimens
2.2.2. Digital Image Correlation Fundaments and Prototype
2.3. Structural Beam Prototype
2.3.1. Manufacture of Prototypes and Test
2.3.2. Prediction of Flexural Performance
3. Experimental Results
3.1. Mechanical Properties of the Recycled Concrete Evaluated
3.2. Beam Performance
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- United Nations. Transforming Our World: The 2030 Agenda for Sustainable Development; United Nations: New York, NY, USA, 2015. [Google Scholar]
- Akhtar, A.; Sarmah, A.K. Construction and demolition waste generation and properties of recycled aggregate concrete: A global perspective. J. Clean. Prod. 2018, 186, 262–281. [Google Scholar] [CrossRef]
- Gálvez-Martos, J.-L.; Styles, D.; Schoenberger, H.; Zeschmar-Lahl, B. Construction and demolition waste best management practice in Europe. Resour. Conserv. Recycl. 2018, 136, 166–178. [Google Scholar] [CrossRef]
- Eurostat. Generation of Waste by Waste Category, Hazardousness and NACE Rev. 2 Activity. Available online: https://ec.europa.eu/eurostat/databrowser/view/env_wasgen/default/table?lang=en (accessed on 1 September 2024).
- Xiao, J.; Xie, H.; Zhang, C. Investigation on building waste and reclaim in Wenchuan earthquake disaster area. Resour. Conserv. Recycl. 2012, 61, 109–117. [Google Scholar] [CrossRef]
- de Brito, J.; Kurda, R. The past and future of sustainable concrete: A critical review and new strategies on cement-based materials. J. Clean. Prod. 2021, 281, 123558. [Google Scholar]
- Arezoumandi, M.; Smith, A.; Volz, J.S.; Khayat, K.H. An experimental study on flexural strength of reinforced concrete beams with 100% recycled concrete aggregate. Eng. Struct. 2015, 88, 154–162. [Google Scholar] [CrossRef]
- Regulation, E.U. No 305/2011 of the European Parliament and of the Council of 9 March 2011 Laying down Harmonised Conditions for the Marketing of Construction Products and Repealing Council Directive 89/106; European Parliament and of the Council: Brussels, Belgium, 2011. [Google Scholar]
- Gonçalves, P.; Brito, J.D. Recycled aggregate concrete (RAC)—Comparative analysis of existing specifications. Mag. Concr. Res. 2010, 62, 339–346. [Google Scholar] [CrossRef]
- Juan-Valdés, A.; Rodríguez-Robles, D.; García-González, J.; Guerra-Romero, M.I.; Morán-del Pozo, J.M. Mechanical and microstructural characterization of non-structural precast concrete made with recycled mixed ceramic aggregates from construction and demolition wastes. J. Clean. Prod. 2018, 180, 482–493. [Google Scholar] [CrossRef]
- Guo, Z.; Tu, A.; Chen, C.; Lehman, D.E. Mechanical properties, durability, and life-cycle assessment of concrete building blocks incorporating recycled concrete aggregates. J. Clean. Prod. 2018, 199, 136–149. [Google Scholar] [CrossRef]
- Tamashiro, J.R.; Kinoshita, A.; Silva, L.H.P.; de Paiva, F.F.G.; Antunes, P.A.; Simões, R.D. Compressive resistance of concrete produced with recycled concrete aggregate and sugarcane vinasse waste-water. Clean. Eng. Technol. 2022, 6, 100362. [Google Scholar] [CrossRef]
- Rodríguez, C.; Parra, C.; Casado, G.; Miñano, I.; Albaladejo, F.; Benito, F.; Sánchez, I. The incorporation of construction and demolition wastes as recycled mixed aggregates in non-structural concrete precast pieces. J. Clean. Prod. 2016, 127, 152–161. [Google Scholar] [CrossRef]
- Datta, S.D.; Sobuz, M.H.R.; Akid, A.S.M.; Islam, S. Influence of coarse aggregate size and content on the properties of recycled aggregate concrete using non-destructive testing methods. J. Build. Eng. 2022, 61, 105249. [Google Scholar] [CrossRef]
- Martín-Morales, M.; Zamorano, M.; Ruiz-Moyano, A.; Valverde-Espinosa, I. Characterization of recycled aggregates construction and demolition waste for concrete production following the Spanish Structural Concrete Code EHE-08. Constr. Build. Mater. 2011, 25, 742–748. [Google Scholar] [CrossRef]
- Fiol, F.; Thomas, C.; Muñoz, C.; Ortega-López, V.; Manso, J.M. The influence of recycled aggregates from precast elements on the mechanical properties of structural self-compacting concrete. Constr. Build. Mater. 2018, 182, 309–323. [Google Scholar] [CrossRef]
- Velay-Lizancos, M.; Vazquez-Burgo, P.; Restrepo, D.; Martinez-Lage, I. Effect of fine and coarse recycled concrete aggregate on the mechanical behavior of precast reinforced beams: Comparison of FE simulations, theoretical, and experimental results on real scale beams. Constr. Build. Mater. 2018, 191, 1109–1119. [Google Scholar] [CrossRef]
- Liao, Z. Bond-Slip Behavior and Tensile Stiffness of FRP Reinforcement and Precast Recycled Aggregate Concrete. Master’s Thesis, Guangdong University of Technology, Guangzhou, China, 2023. [Google Scholar]
- Real Decreto 470/2021, de 29 de junio, por el que se aprueba el Código Estructural; Ministerio de Fomento, Gobierno de España: Madrid, Spain, 2021.
- Yu, Y.; Pacheco-Torgal, F.; Zhao, X.-Y.; Wang, X.-L. Cleaner production of the precast concrete industry: Comparative life cycle analysis of concrete using recycled aggregates from crushed precast rejects. Eur. J. Environ. Civ. Eng. 2024, 28, 1014–1038. [Google Scholar] [CrossRef]
- Pérez Benedicto, J.Á.; del Río Merino, M.; Canudo, J.L.P.; La Mata, M.d.l.R. Características mecánicas de hormigones con áridos reciclados procedentes de los rechazos en prefabricación. Mater. Construcción 2012, 62, 25–37. [Google Scholar] [CrossRef]
- Zhao, Z.; Courard, L.; Groslambert, S.; Jehin, T.; Leonard, A.; Xiao, J. Use of recycled concrete aggregates from precast block for the production of new building blocks: An industrial scale study. Resour. Conserv. Recycl. 2020, 157, 104786. [Google Scholar] [CrossRef]
- Soares, D.; De Brito, J.; Ferreira, J.; Pacheco, J. Use of coarse recycled aggregates from precast concrete rejects: Mechanical and durability performance. Constr. Build. Mater. 2014, 71, 263–272. [Google Scholar] [CrossRef]
- Thomas, C.; Setién, J.; Polanco, J.A. Structural recycled aggregate concrete made with precast wastes. Constr. Build. Mater. 2016, 114, 536–546. [Google Scholar] [CrossRef]
- Yu, Y.; Fang, G.-H.; Kurda, R.; Sabuj, A.R.; Zhao, X.-Y. An agile, intelligent and scalable framework for mix design optimization of green concrete incorporating recycled aggregates from precast rejects. Case Stud. Constr. Mater. 2024, 20, e03156. [Google Scholar] [CrossRef]
- Fiol, F.; Revilla-Cuesta, V.; Skaf, M.; Thomas, C.; Manso, J.M. Scaled concrete beams containing maximum levels of coarse recycled aggregate: Structural verifications for precast-concrete building applications. Struct. Concr. 2023, 24, 3476–3497. [Google Scholar] [CrossRef]
- Revilla-Cuesta, V.; Fiol, F.; Perumal, P.; Ortega-López, V.; Manso, J.M. Using recycled aggregate concrete at a precast-concrete plant: A multi-criteria company-oriented feasibility study. J. Clean. Prod. 2022, 373, 133873. [Google Scholar] [CrossRef]
- Pedro, D.; De Brito, J.; Evangelista, L. Influence of the use of recycled concrete aggregates from different sources on structural concrete. Constr. Build. Mater. 2014, 71, 141–151. [Google Scholar] [CrossRef]
- Xiao, J.; Zhang, K.; Akbarnezhad, A. Variability of stress-strain relationship for recycled aggregate concrete under uniaxial compression loading. J. Clean. Prod. 2018, 181, 753–771. [Google Scholar] [CrossRef]
- Sutton, M.A.; Orteu, J.J.; Schreier, H.X. Image Correlation for Shape, Motion and Deformation Measurements: Basic Concepts, Theory and Applications; Springer Science & Business Media: New York, NY, USA, 2009. [Google Scholar]
- Pisonero, J.; López-Rebollo, J.; García-Martín, R.; Rodríguez-Martín, M.; Sánchez-Aparicio, L.J.; Muñoz-Nieto, A.; González-Aguilera, D. A comparative study of 2D and 3D digital image correlation approaches for the characterization and numerical analysis of composite materials. IEEE Access 2021, 9, 160675–160687. [Google Scholar] [CrossRef]
- Villarino, A.; López-Rebollo, J.; Antón, N. Analysis of Mechanical Behavior through Digital Image Correlation and Reliability of Pinus halepensis Mill. Forests 2020, 11, 1232. [Google Scholar] [CrossRef]
- Garcia-Martin, R.; López-Rebollo, J.; Sánchez-Aparicio, L.J.; Fueyo, J.G.; Pisonero, J.; Gonzalez-Aguilera, D. Digital image correlation and reliability-based methods for the design and repair of pressure pipes through composite solutions. Constr. Build. Mater. 2020, 248, 118625. [Google Scholar] [CrossRef]
- María, R.-M.; Paula, V.-L.; Jaime, F.-G.; Jorge, L.R. Improvement of tensile properties of carbon fibre-reinforced cementitious matrix composites with coated textile and enhanced mortars. Constr. Build. Mater. 2023, 369, 130552. [Google Scholar] [CrossRef]
- Le, D.B.; Tran, S.D.; Torero, J.L.; Dao, V.T.N. Application of digital image correlation system for reliable deformation measurement of concrete structures at high temperatures. Eng. Struct. 2019, 192, 181–189. [Google Scholar] [CrossRef]
- Dzaye, E.D.; Tsangouri, E.; Spiessens, K.; De Schutter, G.; Aggelis, D.G. Digital image correlation (DIC) on fresh cement mortar to quantify settlement and shrinkage. Arch. Civ. Mech. Eng. 2019, 19, 205–214. [Google Scholar] [CrossRef]
- Abdulqader, A.; Rizos, D.C. Advantages of using digital image correlation techniques in uniaxial compression tests. Results Eng. 2020, 6, 100109. [Google Scholar] [CrossRef]
- Liu, B.; Guo, J.; Wen, X.; Zhou, J.; Deng, Z. Study on flexural behavior of carbon fibers reinforced coral concrete using digital image correlation. Constr. Build. Mater. 2020, 242, 117968. [Google Scholar] [CrossRef]
- Ni, P.; Li, J.; Hao, H.; Yan, W.; Du, X.; Zhou, H. Reliability analysis and design optimization of nonlinear structures. Reliab. Eng. Syst. Saf. 2020, 198, 106860. [Google Scholar] [CrossRef]
- Zhou, Y.; Zhang, J.; Li, W.; Hu, B.; Huang, X. Reliability-based design analysis of FRP shear strengthened reinforced concrete beams considering different FRP configurations. Compos. Struct. 2020, 237, 111957. [Google Scholar] [CrossRef]
- Huang, X.; Zhou, Y.; Li, W.; Hu, B.; Zhang, J. Reliability-based design of FRP shear strengthened reinforced concrete Beams: Guidelines assessment and calibration. Compos. Struct. 2023, 323, 117421. [Google Scholar] [CrossRef]
- Ramamoorthy, D.; Guo, B.; Kazmi, S.M.S.; Wu, Y. A Reliability-Based Design Approach for the Flexural Resistance of Compression Yielded Fibre-Reinforced Polymer (FRP)-Reinforced Concrete Beams. Buildings 2024, 14, 2415. [Google Scholar] [CrossRef]
- Meng, Z.; Yıldız, B.S.; Li, G.; Zhong, C.; Mirjalili, S.; Yildiz, A.R. Application of state-of-the-art multiobjective metaheuristic algorithms in reliability-based design optimization: A comparative study. Struct. Multidiscip. Optim. 2023, 66, 191. [Google Scholar] [CrossRef]
- Fuller, W.B.; Thompson, S.E. The laws of proportioning concrete. Trans. Am. Soc. Civ. Eng. 1907, 59, 67–143. [Google Scholar] [CrossRef]
- Bolomey, J. Détermination de la Résistance à la Compression des Mortiers et Bétons. Bull. Tech. la Suisse Rom. 1925, 51, 126–132. [Google Scholar]
- UNE-EN 12390-6:2010; Ensayos de Hormigón Endurecido. Parte 6: Resistencia a Tracción Indirecta de Probetas. AENOR: Madrid, Spain, 2010.
- UNE-EN 12390-3:2020; Ensayos de Hormigón Endurecido. Parte 3: Determinación de la Resistencia a Compresión de Probetas. AENOR: Madrid, Spain, 2020.
- UNE-EN 12390-13:2022; Ensayos de Hormigón Endurecido. Parte 13: Determinación del Módulo Secante de Elasticidad en Compresión. AENOR: Madrid, Spain, 2022.
- UNE-EN 12390-2:2020; Ensayos de Hormigón Endurecido. Parte 2: Fabricación y Curado de Probetas para Ensayos de Resistencia. AENOR: Madrid, Spain, 2020.
- Dong, Y.L.; Pan, B. A review of speckle pattern fabrication and assessment for digital image correlation. Exp. Mech. 2017, 57, 1161–1181. [Google Scholar] [CrossRef]
- Pan, B.; Lu, Z.; Xie, H. Mean intensity gradient: An effective global parameter for quality assessment of the speckle patterns used in digital image correlation. Opt. Lasers Eng. 2010, 48, 469–477. [Google Scholar] [CrossRef]
- Lecompte, D.; Sol, H.; Vantomme, J.; Habraken, A. Analysis of speckle patterns for deformation measurements by digital image correlation. In SPECKLE06: Speckles, From Grains to Flowers; SPIE: Bellingham, WA, USA, 2006; pp. 80–85. [Google Scholar]
- Garcia-Martin, R.; Bautista-De Castro, Á.; Sánchez-Aparicio, L.J.; Fueyo, J.G.; Gonzalez-Aguilera, D. Combining digital image correlation and probabilistic approaches for the reliability analysis of composite pressure vessels. Arch. Civ. Mech. Eng. 2019, 19, 224–239. [Google Scholar] [CrossRef]
- Pan, B.; Qian, K.; Xie, H.; Asundi, A. Two-dimensional digital image correlation for in-plane displacement and strain measurement: A review. Meas. Sci. Technol. 2009, 20, 062001. [Google Scholar] [CrossRef]
- Blaber, J.; Adair, B.; Antoniou, A. Ncorr: Open-source 2D digital image correlation matlab software. Exp. Mech. 2015, 55, 1105–1122. [Google Scholar] [CrossRef]
- Solav, D.; Moerman, K.M.; Jaeger, A.M.; Genovese, K.; Herr, H.M. MultiDIC: An open-source toolbox for multi-view 3D digital image correlation. IEEE Access 2018, 6, 30520–30535. [Google Scholar] [CrossRef]
- Visintin, P.; Dadd, L.; Alam, M.U.; Xie, T.; Bennett, B. Flexural performance and life-cycle assessment of multi-generation recycled aggregate concrete beams. J. Clean. Prod. 2022, 360, 132214. [Google Scholar] [CrossRef]
- UNE-EN 1992-1-1:2010; Eurocode 2: Design of Concrete Structures—Part 1-1: General Rules and Rules for Buildings. AENOR: Madrid, Spain, 2010.
- Sobol, I.M. Sensitivity estimates for non-linear mathematical models. Math. Model. Comput. Exp. 1993, 1, 407–414. [Google Scholar]
- UNE-EN 12350-2:2020; Ensayos de Hormigón Fresco. Parte 2: Ensayo de Asentamiento. AENOR: Madrid, Spain, 2020.
- Teijón-López-Zuazo, E.; López-Rebollo, J.; Sánchez-Aparicio, L.J.; Garcia-Martín, R.; Gonzalez-Aguilera, D. Compression and strain predictive models in non-structural recycled concretes made from construction and demolition wastes. Materials 2021, 14, 3177. [Google Scholar] [CrossRef]
- Sasikumar, P.; Suresh, R.; Vijayaghosh, P.K.; Gupta, S. Experimental characterisation of random field models for CFRP composite panels. Compos. Struct. 2015, 120, 451–471. [Google Scholar] [CrossRef]

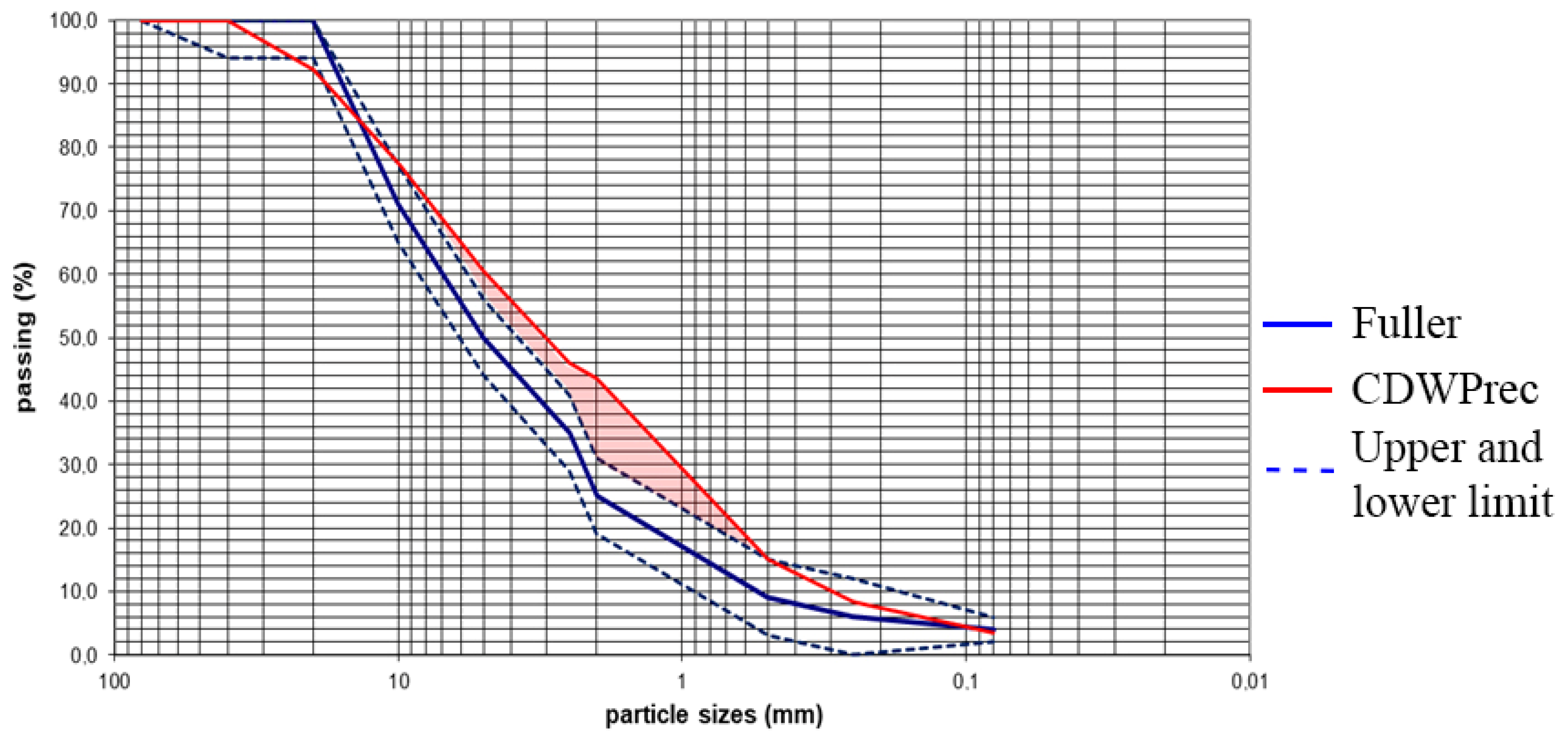
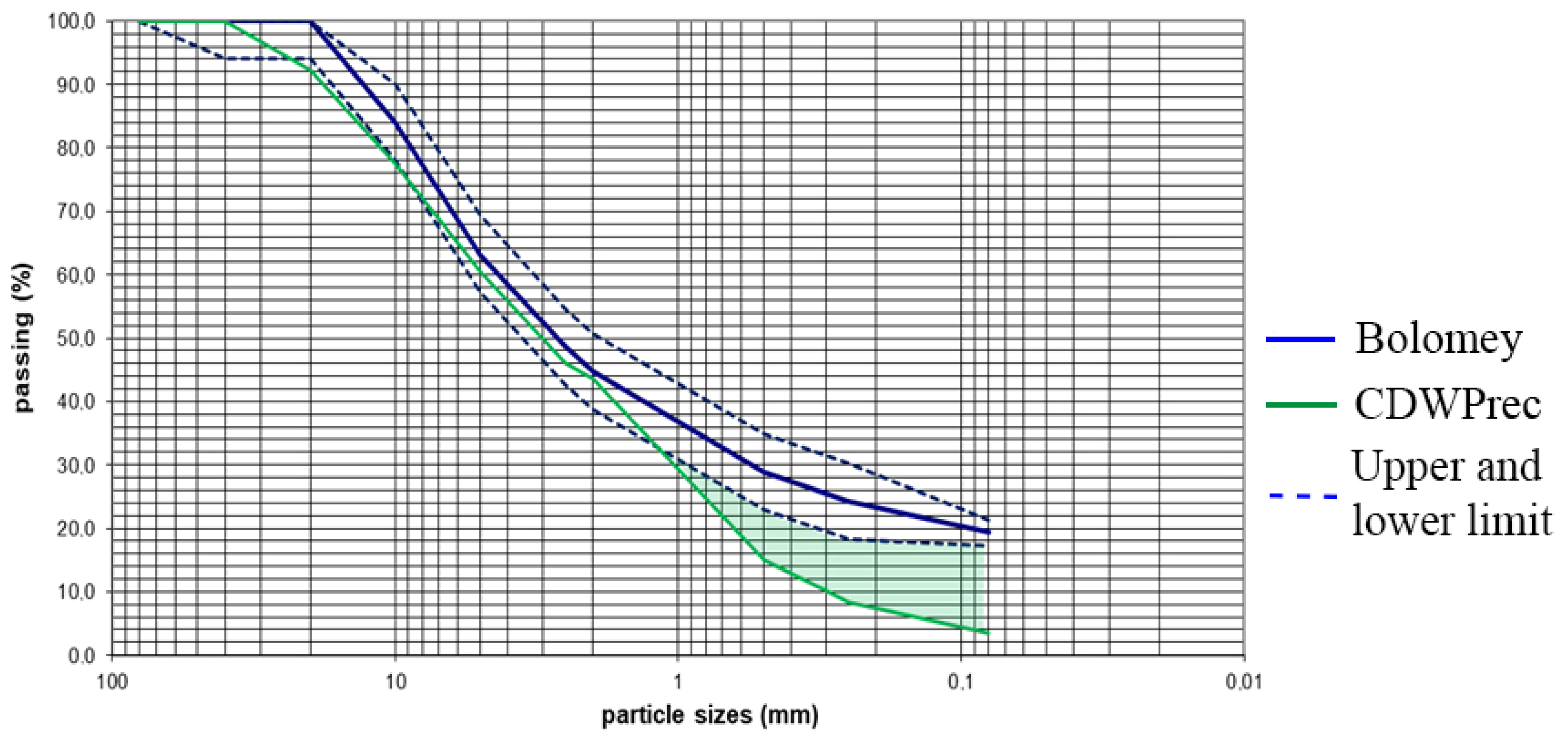





| Material | D10 (mm) | D30 (mm) | D50 (mm) | D60 (mm) | Cu | Cc | % Fines | % Sand Size | % (4.75–9.5) mm | % (9.5–40.0) mm |
|---|---|---|---|---|---|---|---|---|---|---|
| CDWRCer | 0.25 | 1.0 | 3.0 | 5.0 | 20 | 0.8 | 3.4 | 60 | 16 | 24 |
| Property | Mean | STD | CoV (%) | Lower Bound | Upper Bound |
|---|---|---|---|---|---|
| fc-cyl | 36.5 | 4.3 | 11.8 | 29.1 | 39.4 |
| fc-cub | 40.5 | 2.2 | 5.4 | 37.8 | 43.5 |
| fti | 2.8 | 0.3 | 11.2 | 2.5 | 3.1 |
| E | 27.62 | 4.56 | 16.5 | 21.18 | 36.82 |
| εp-cyl | 0.0021 | 0.0002 | 11.6 | 0.0017 | 0.0026 |
| εp-cub | 0.0034 | 0.0006 | 18.3 | 0.0023 | 0.0048 |
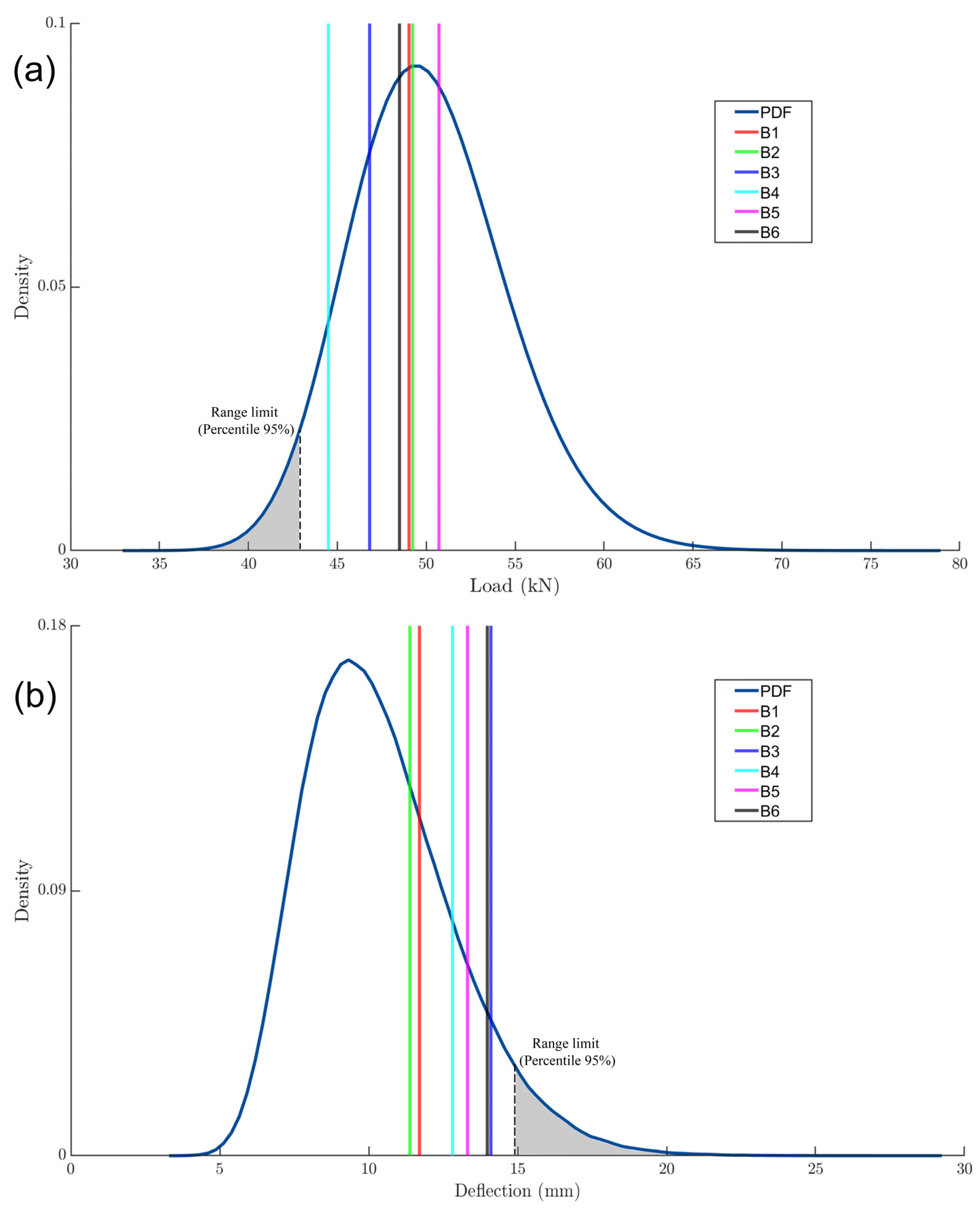
| Beam | Load (kN) | Pload (%) | Deflection (mm) | Pdef (%) |
|---|---|---|---|---|
| B1 | 49.02 | 58.2 | 11.70 | 73.2 |
| B2 | 49.23 | 56.3 | 11.38 | 69.4 |
| B3 | 46.83 | 77.1 | 14.11 | 92.0 |
| B4 | 44.51 | 91.0 | 12.81 | 84.0 |
| B5 | 50.72 | 42.8 | 13.31 | 87.6 |
| B6 | 48.50 | 63.0 | 13.98 | 91.4 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
López-Rebollo, J.; Teijón-López-Zuazo, E.; García-Martin, R.; Sánchez-Aparicio, L.J.; González-Aguilera, D. Digital Image Correlation and Reliability-Based Methods for the Design of Structural Beams Made from Recycled Concrete Using Aggregates from Precast Rejects. Appl. Sci. 2025, 15, 656. https://doi.org/10.3390/app15020656
López-Rebollo J, Teijón-López-Zuazo E, García-Martin R, Sánchez-Aparicio LJ, González-Aguilera D. Digital Image Correlation and Reliability-Based Methods for the Design of Structural Beams Made from Recycled Concrete Using Aggregates from Precast Rejects. Applied Sciences. 2025; 15(2):656. https://doi.org/10.3390/app15020656
Chicago/Turabian StyleLópez-Rebollo, Jorge, Evelio Teijón-López-Zuazo, Roberto García-Martin, Luis Javier Sánchez-Aparicio, and Diego González-Aguilera. 2025. "Digital Image Correlation and Reliability-Based Methods for the Design of Structural Beams Made from Recycled Concrete Using Aggregates from Precast Rejects" Applied Sciences 15, no. 2: 656. https://doi.org/10.3390/app15020656
APA StyleLópez-Rebollo, J., Teijón-López-Zuazo, E., García-Martin, R., Sánchez-Aparicio, L. J., & González-Aguilera, D. (2025). Digital Image Correlation and Reliability-Based Methods for the Design of Structural Beams Made from Recycled Concrete Using Aggregates from Precast Rejects. Applied Sciences, 15(2), 656. https://doi.org/10.3390/app15020656











