Mode Competition Phenomena and Impact of the Initial Conditions in Nonlinear Vibrations Leading to Railway Curve Squeal
Abstract
1. Introduction
2. Materials and Methods
2.1. Description of the Model
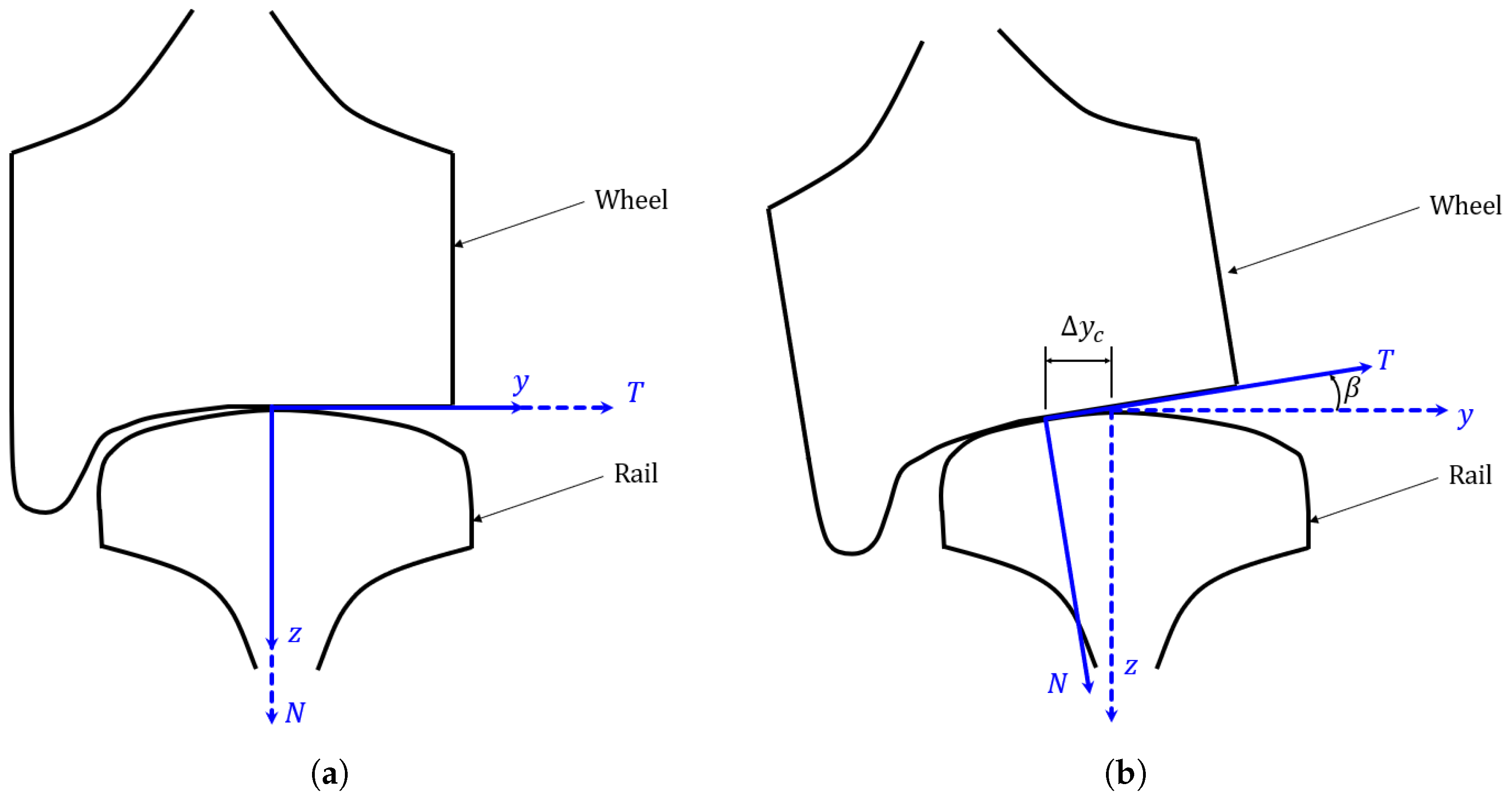
2.1.1. Contact Forces
2.1.2. Equations of Motion
2.2. Stability Analysis of the Self-Excited System
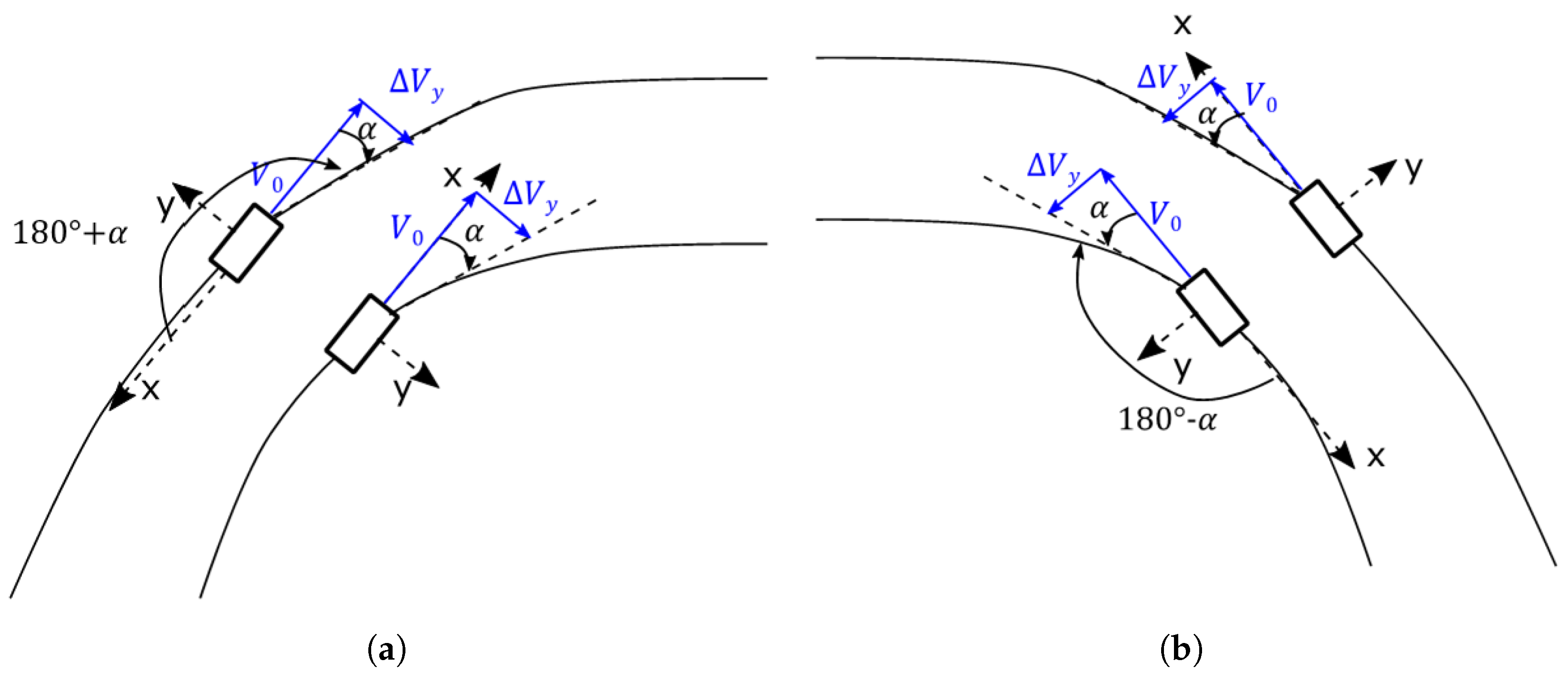
2.3. Derivation of the Initial Conditions
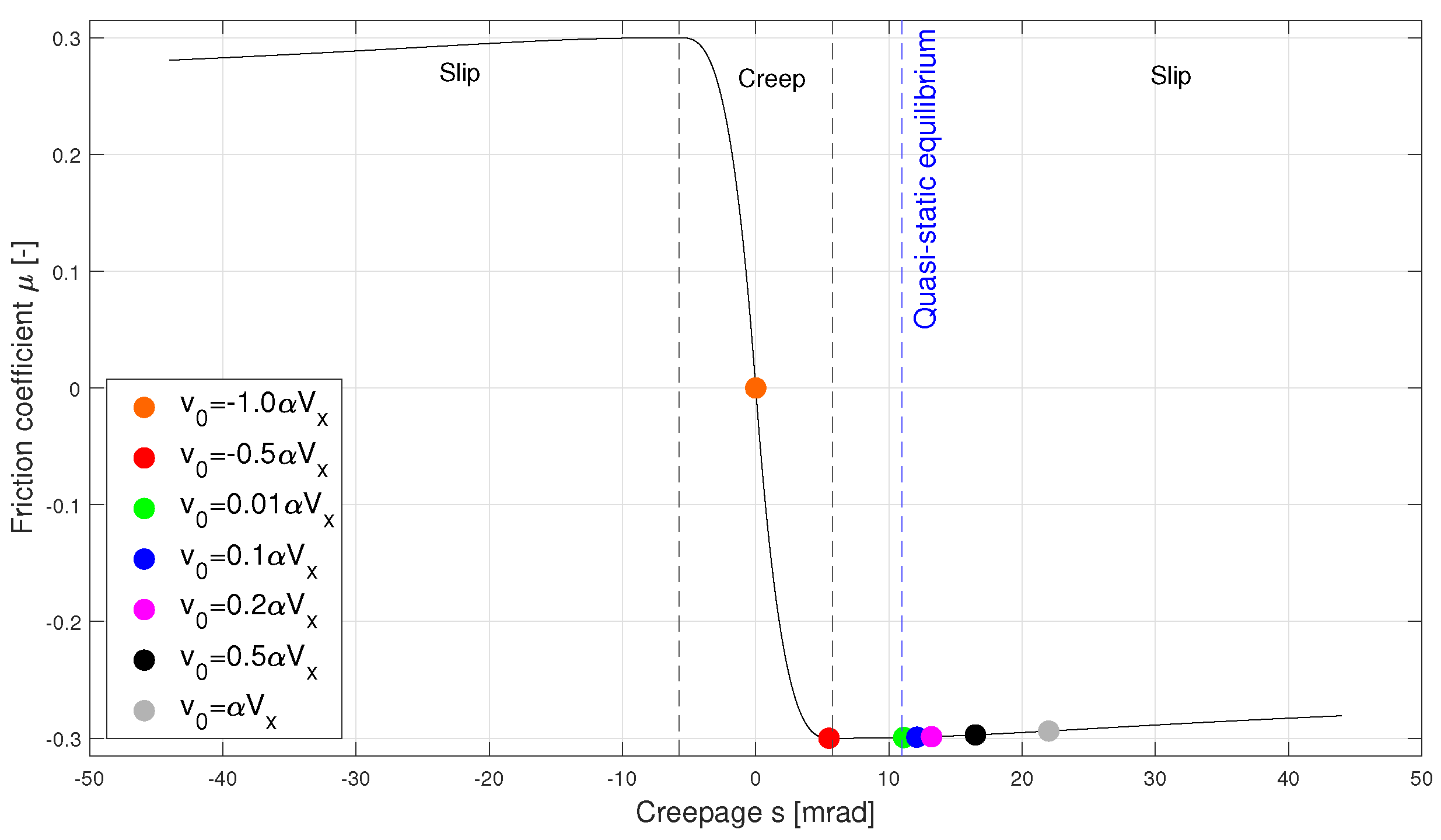
2.3.1. Initial Condition on Velocity
2.3.2. Initial Condition on Displacement
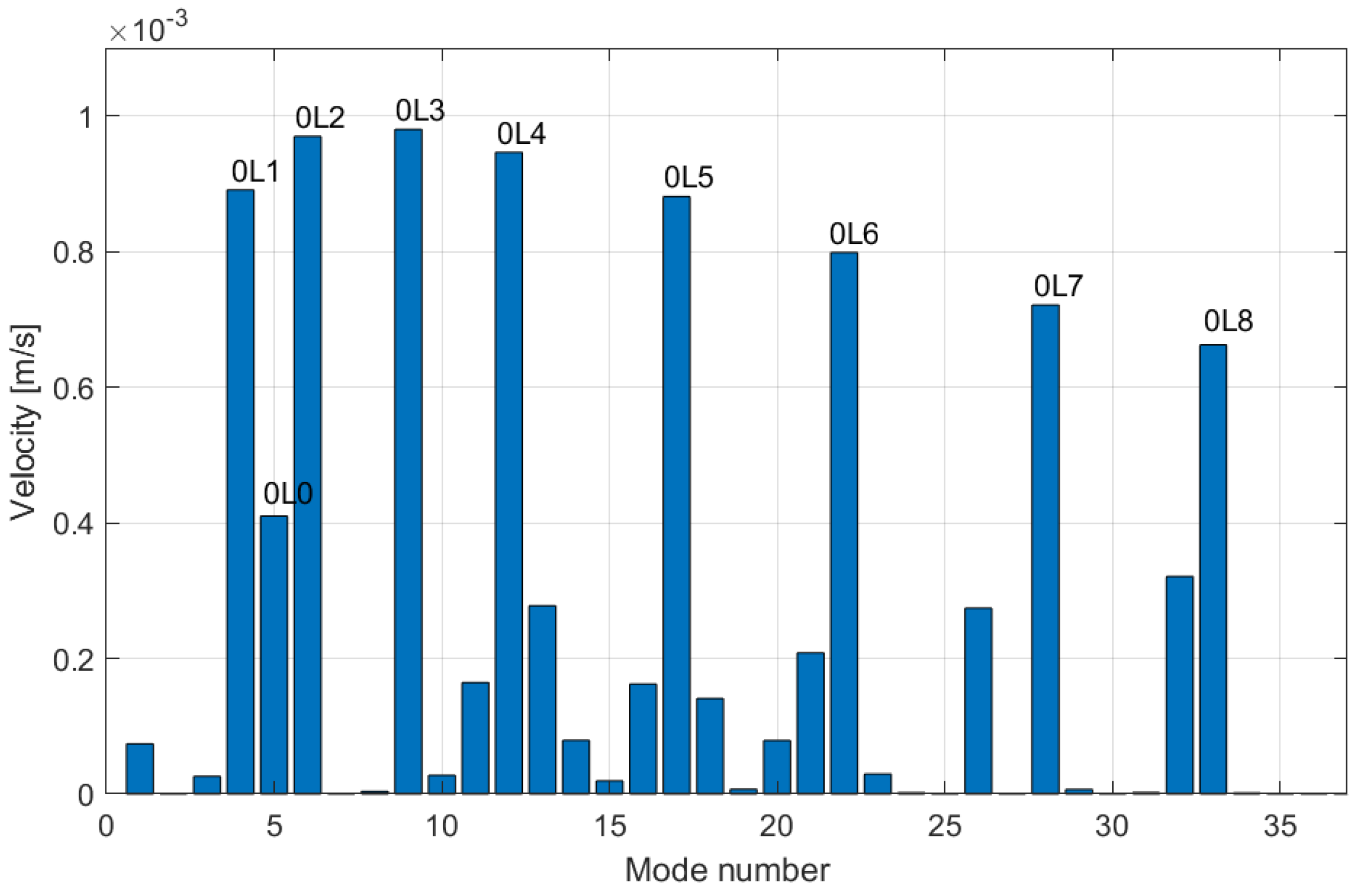
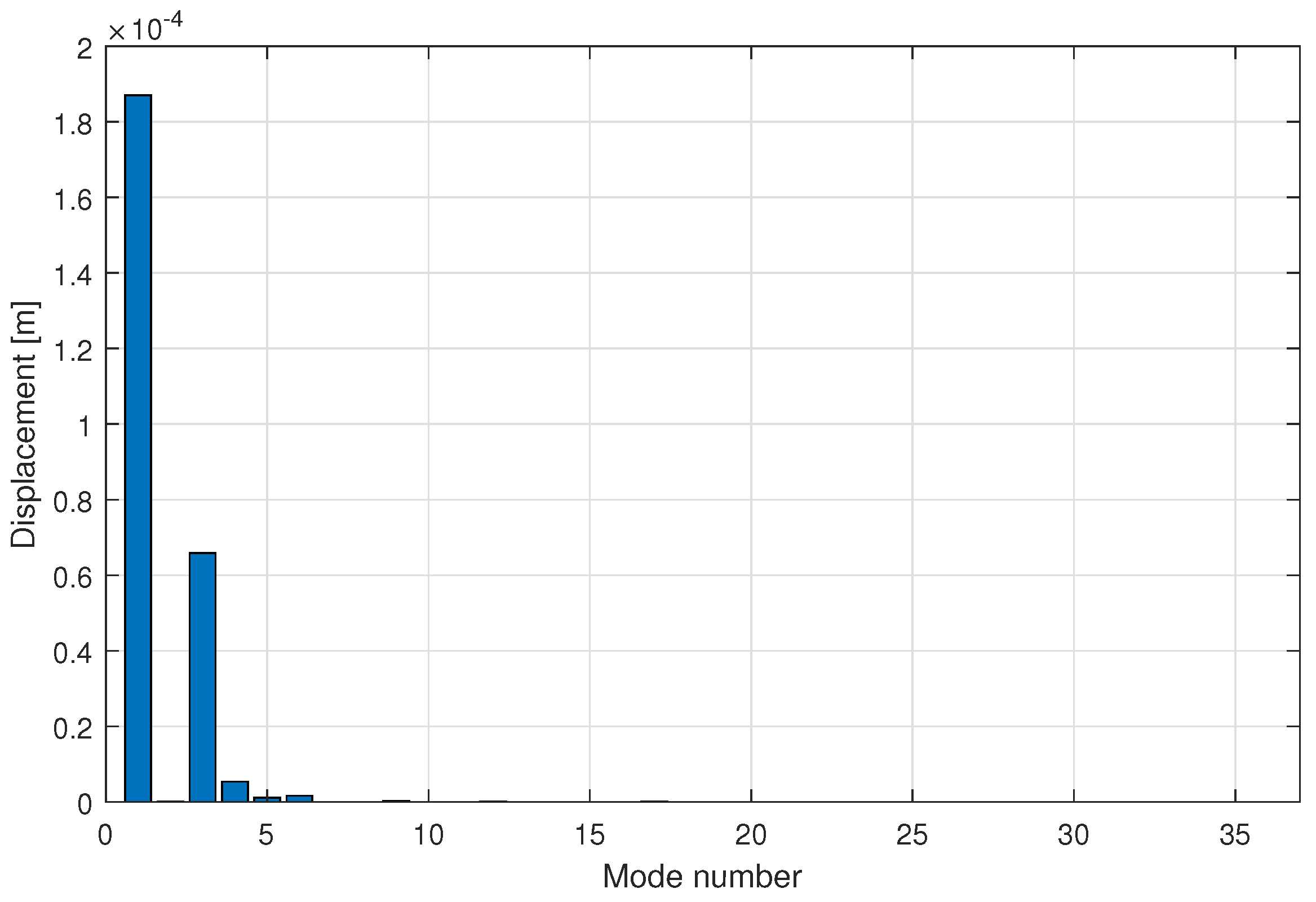
2.3.3. Modal-Derived Initial Conditions
3. Results and Discussion
3.1. System’s Parameters
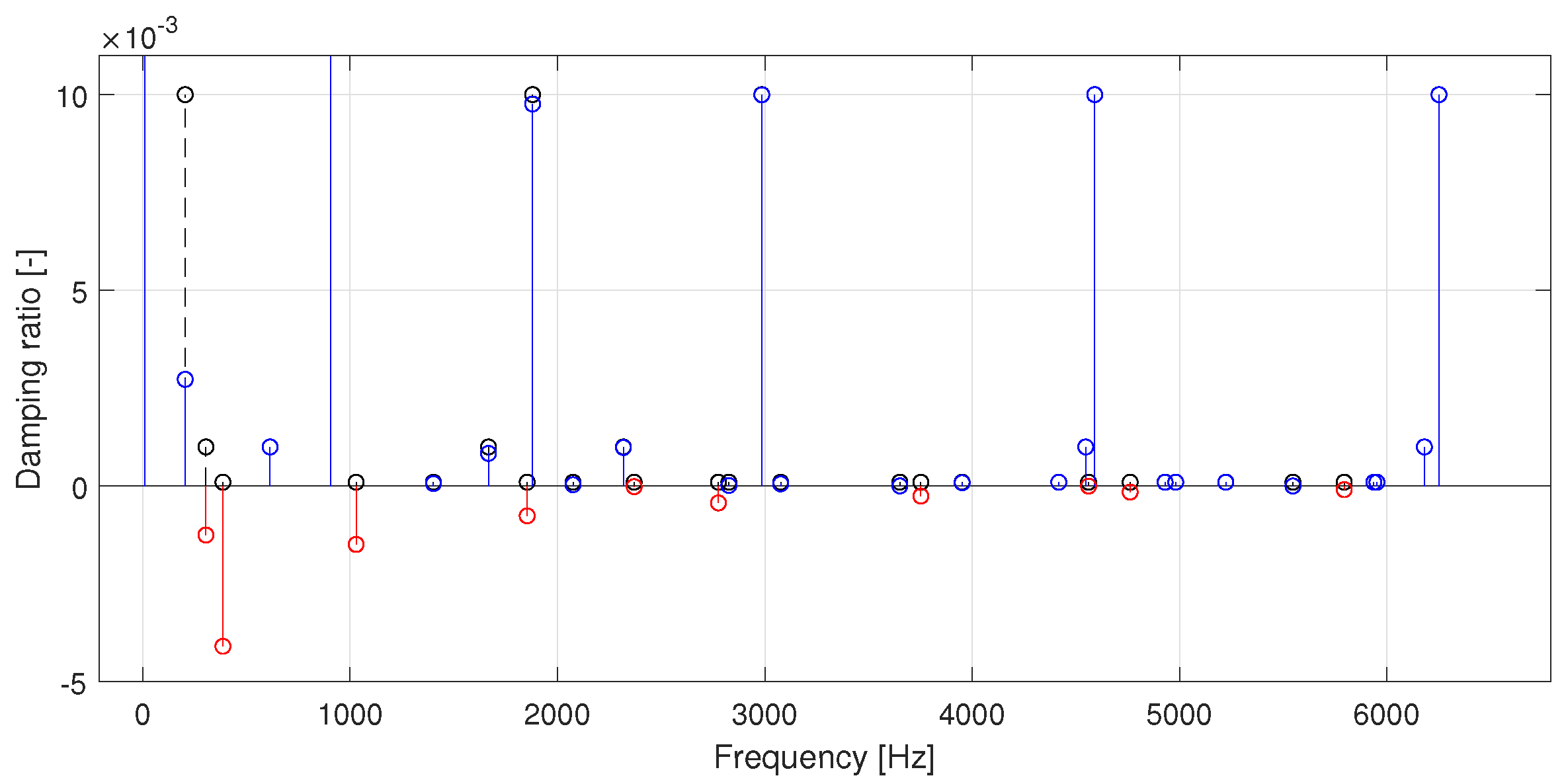
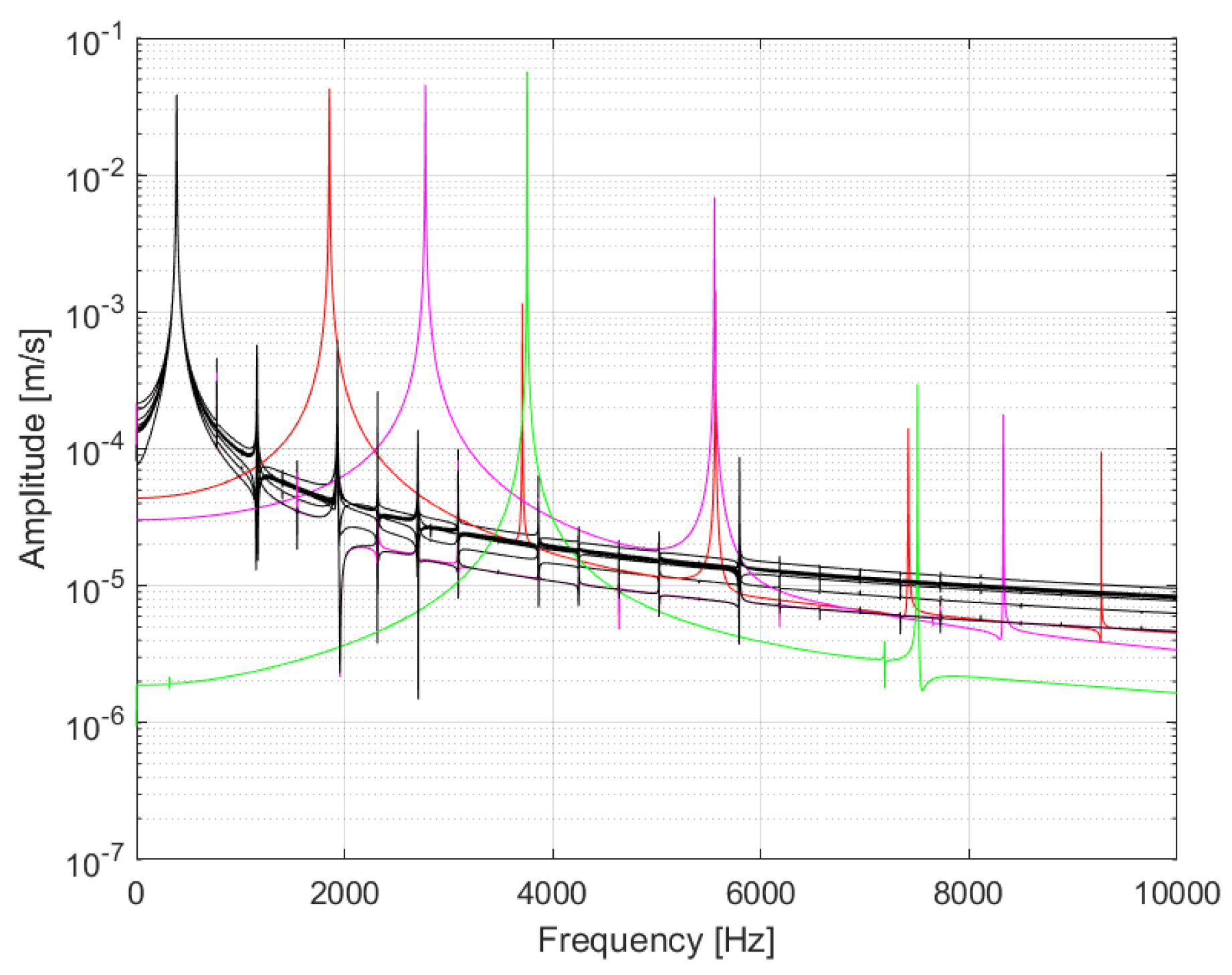
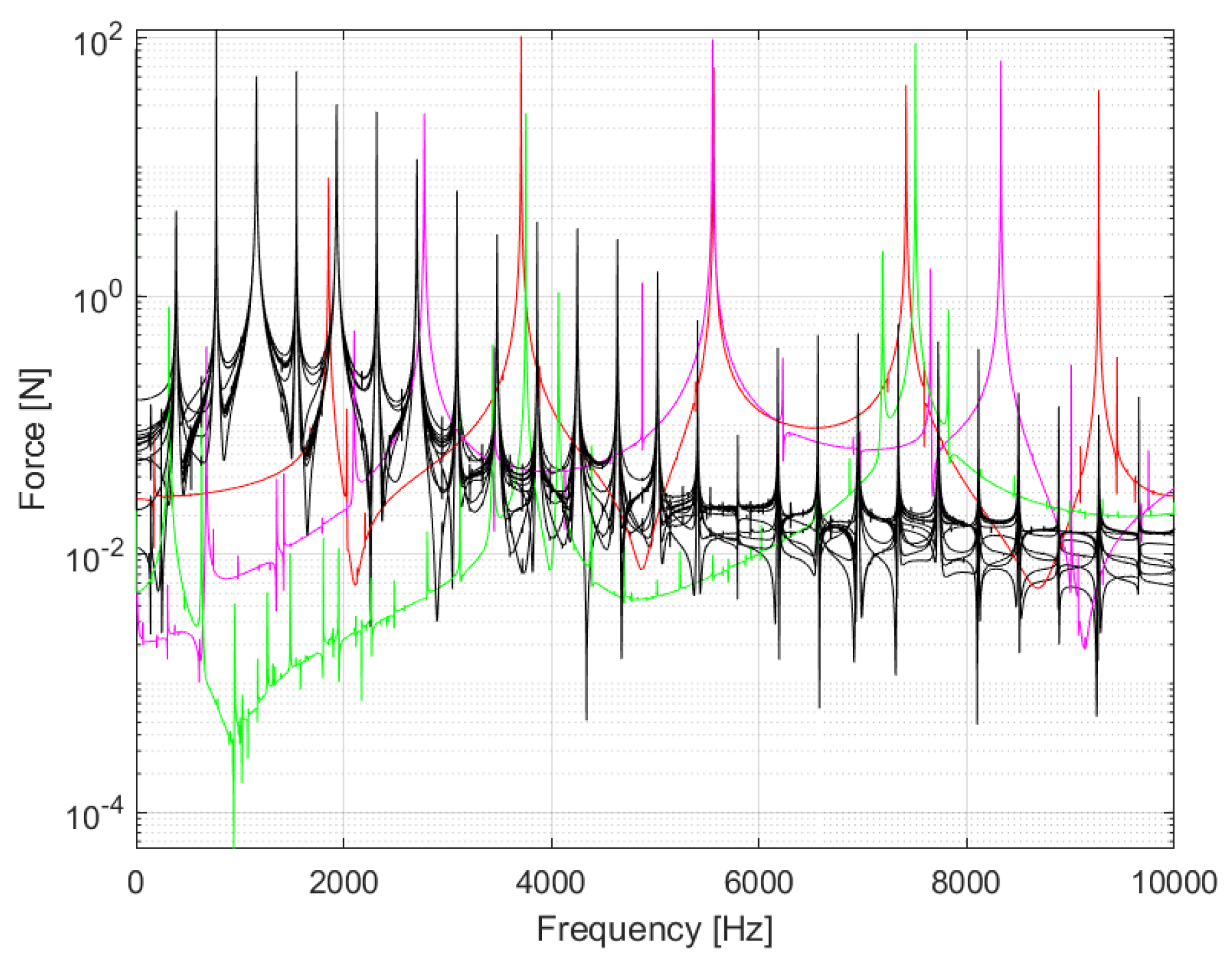
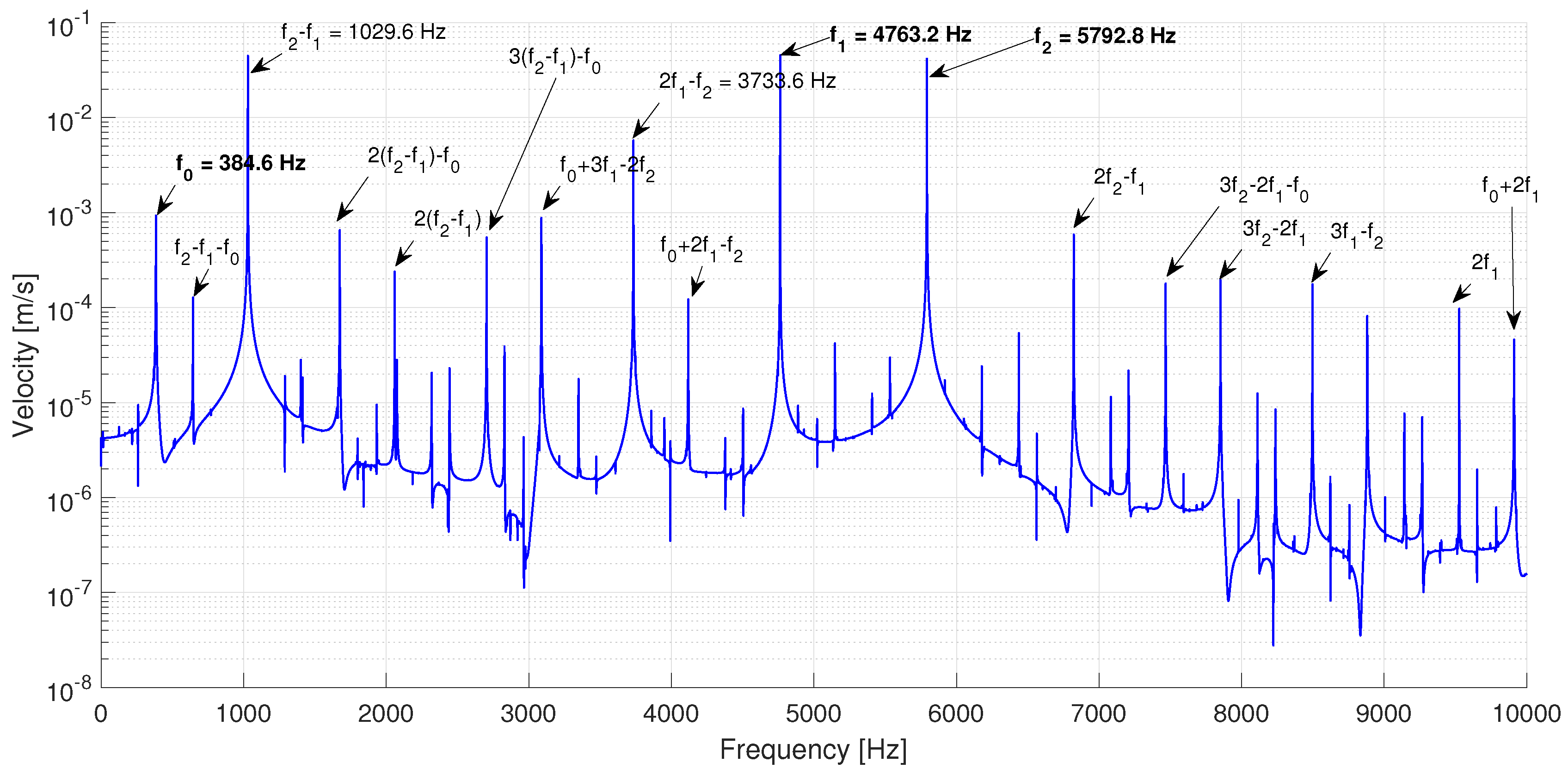
3.2. Stability Results
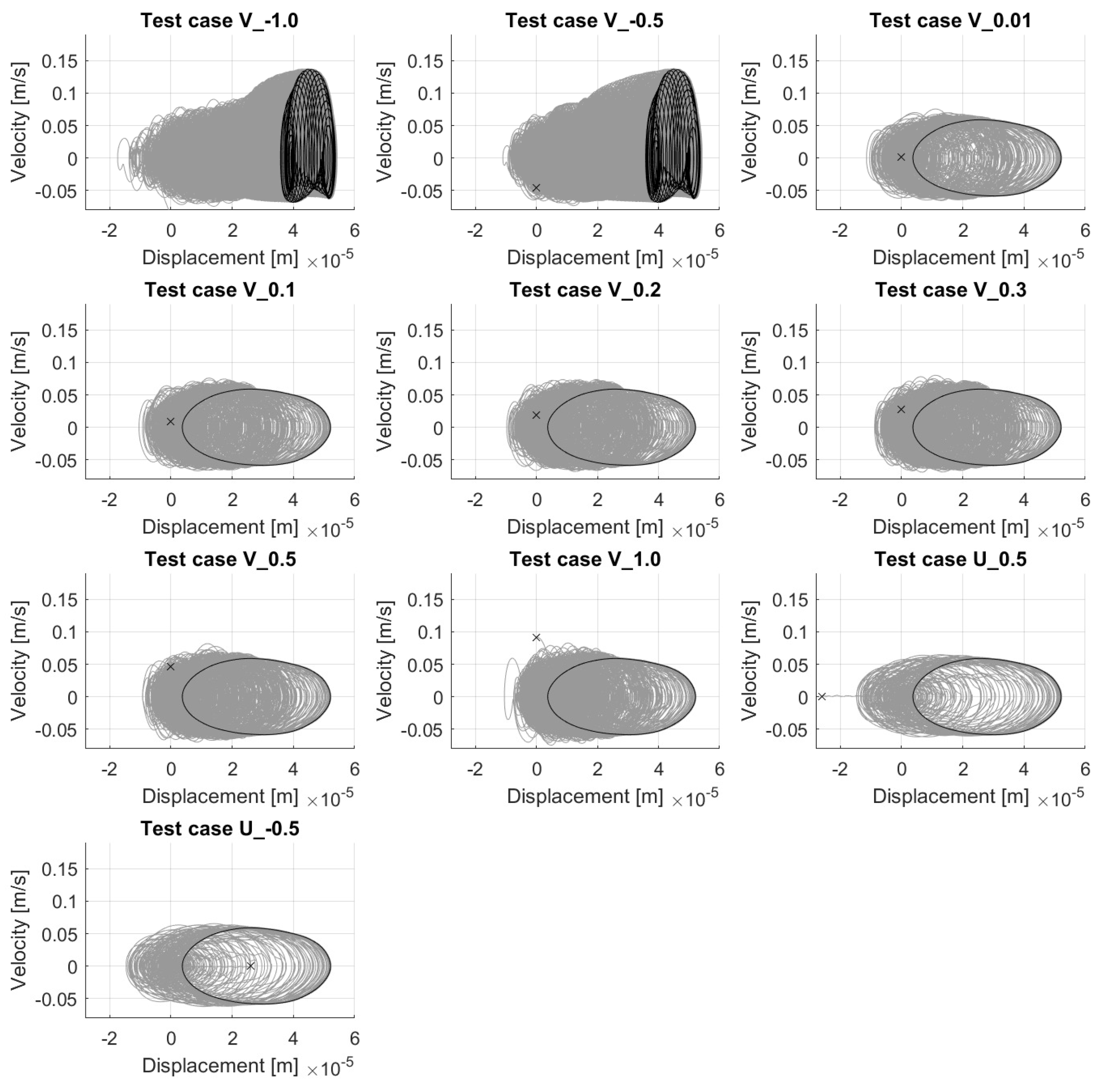
3.3. Initial Conditions
3.3.1. Initial Condition on Velocity
3.3.2. Initial Condition on Displacement
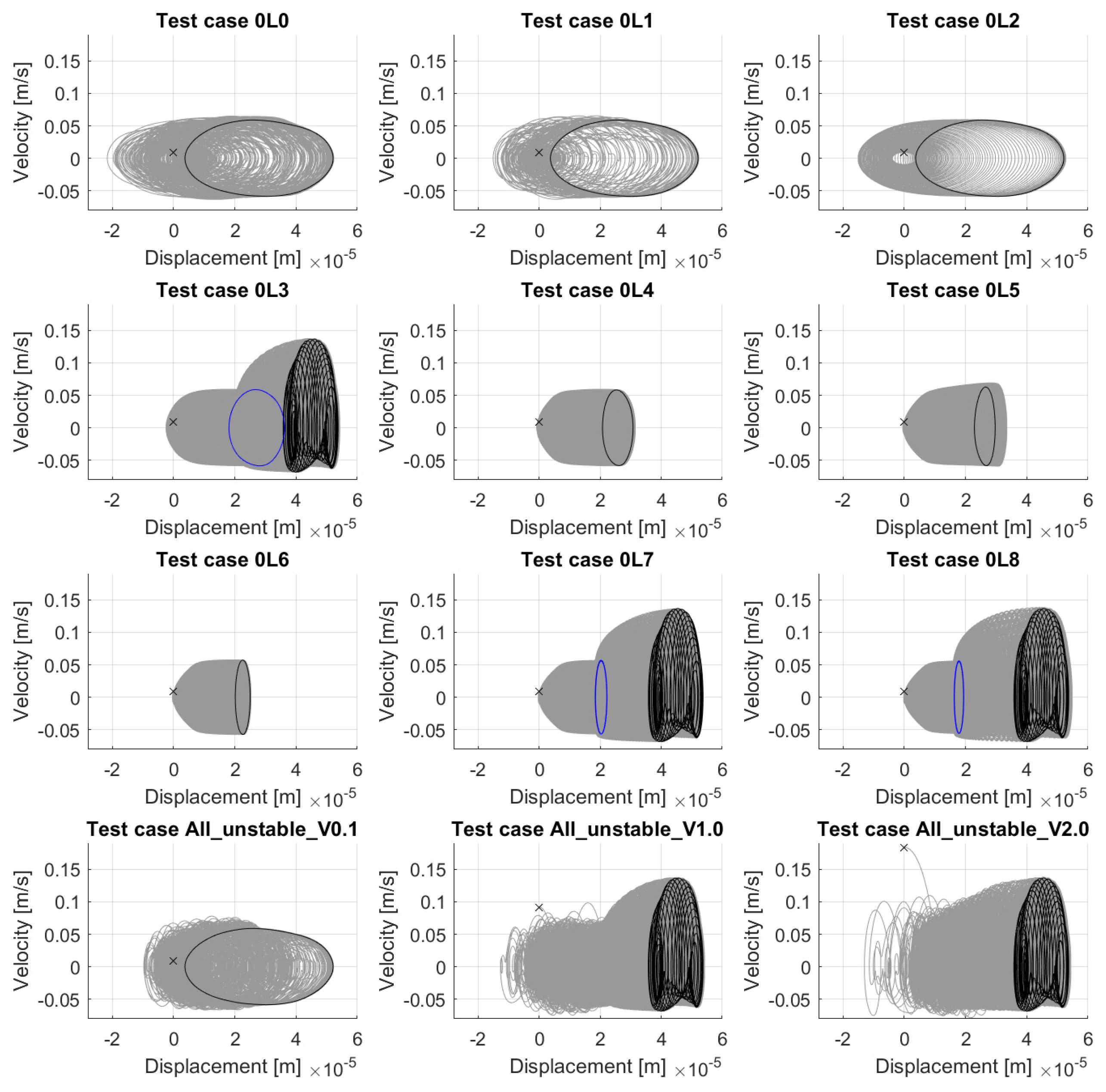
3.3.3. Modal-Derived Initial Conditions and Summary of the Test Cases
- All unstable modes
- Mode 0Ln, with
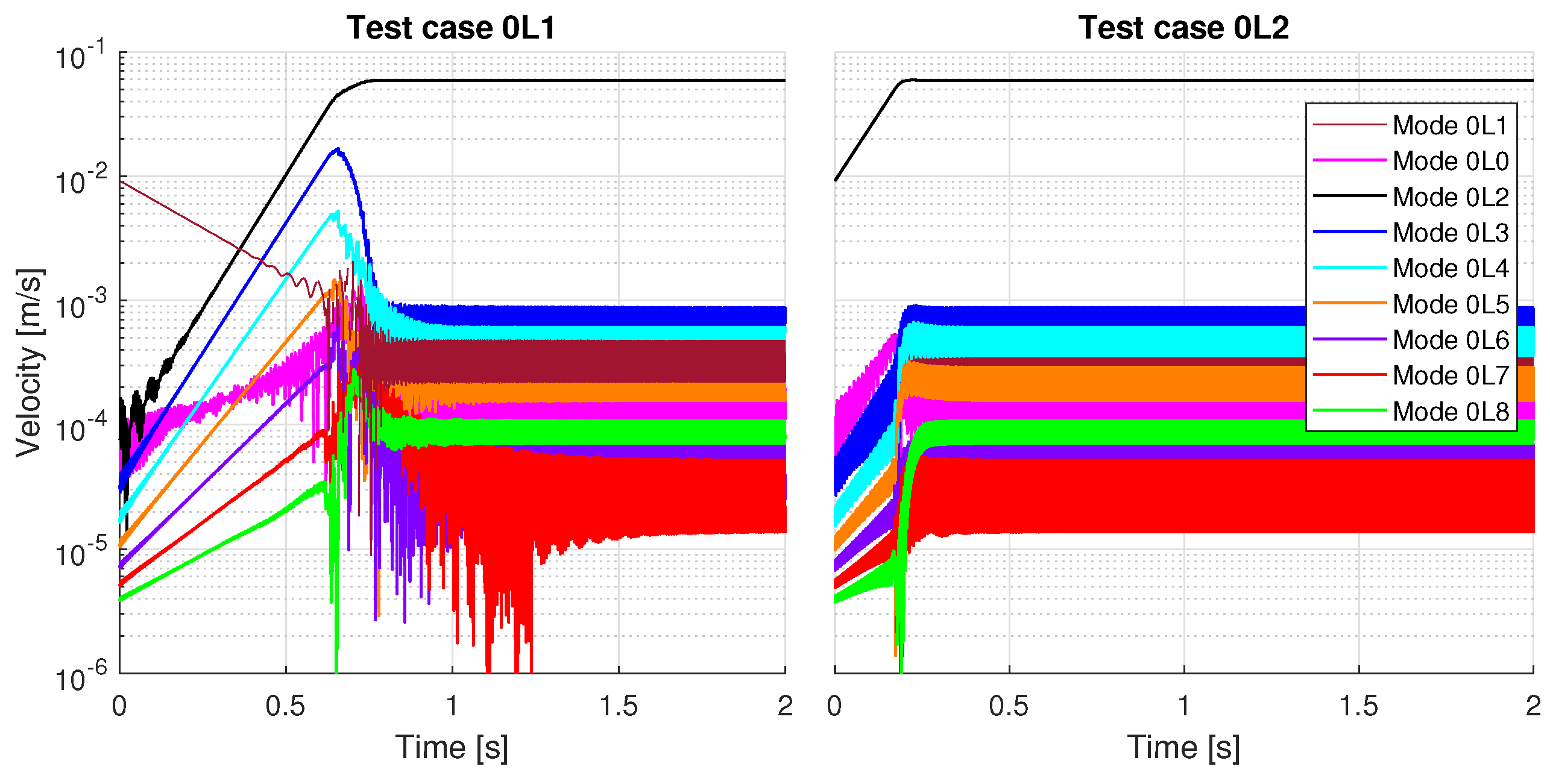
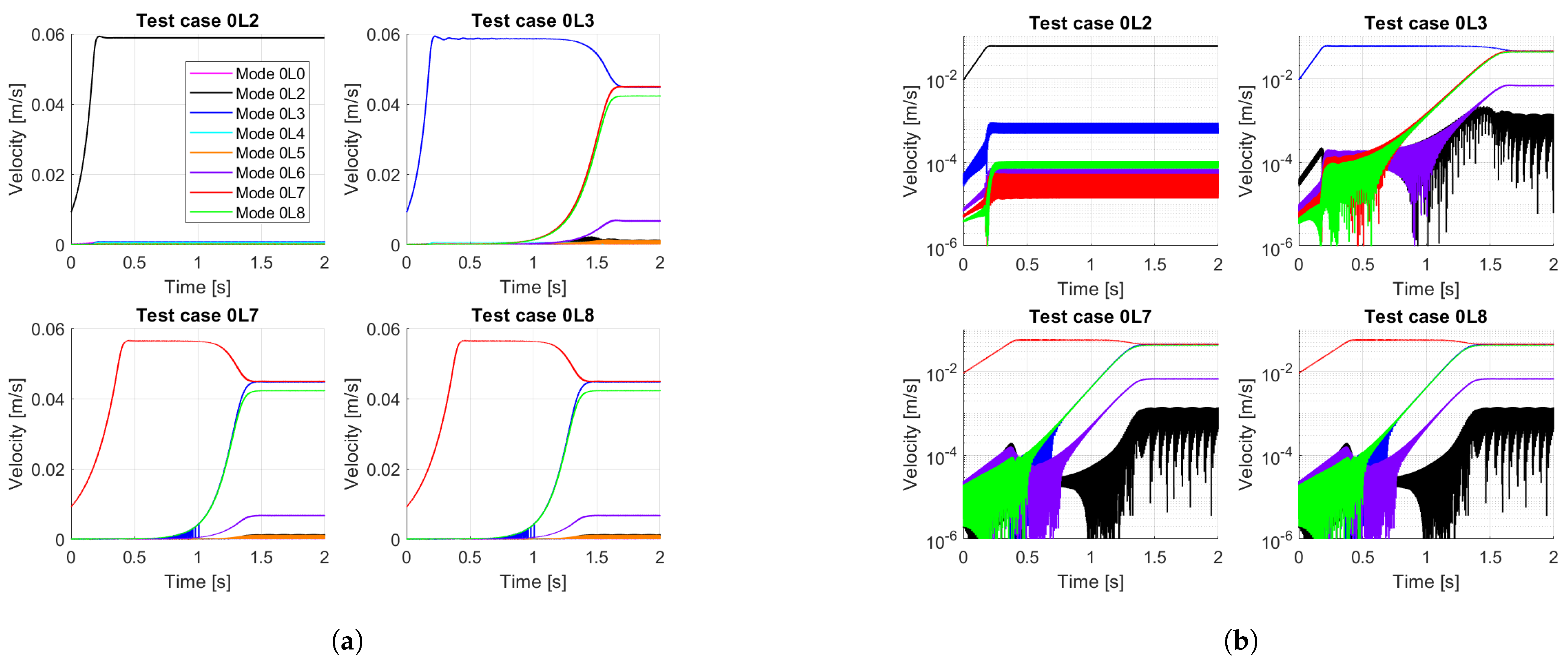
3.4. Time Integration Results
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Thompson, D.J.; Squicciarini, G.; Ding, B.; Baeza, L. A State-of-the-Art Review of Curve Squeal Noise: Phenomena, Mechanisms, Modelling and Mitigation. In Noise and Vibration Mitigation for Rail Transportation Systems; Notes on Numerical Fluid Mechanics and Multidisciplinary Design; Springer: Cham, Switzerland, 2018; pp. 3–41. [Google Scholar] [CrossRef]
- Rudd, M.J. Wheel/rail noise—Part II: Wheel squeal. J. Sound Vib. 1976, 46, 381–394. [Google Scholar] [CrossRef]
- van Ruiten, C.J.M. Mechanism of squeal noise generated by trams. J. Sound Vib. 1988, 120, 245–253. [Google Scholar] [CrossRef]
- Fingberg, U. A model of wheel-rail squealing noise. J. Sound Vib. 1990, 143, 365–377. [Google Scholar] [CrossRef]
- de Beer, F.G.; Janssens, M.H.A.; Kooijman, P.P. Squeal noise of rail-bound vehicles influenced by lateral contact position. J. Sound Vib. 2003, 267, 497–507. [Google Scholar] [CrossRef]
- Chiello, O.; Ayasse, J.B.; Vincent, N.; Koch, J.R. Curve squeal of urban rolling stock—Part 3: Theoretical model. J. Sound Vib. 2006, 293, 710–727. [Google Scholar] [CrossRef]
- Hoffmann, N.; Fischer, M.; Allgaier, R.; Gaul, L. A minimal model for studying properties of the mode-coupling type instability in friction induced oscillations. Mech. Res. Commun. 2002, 29, 197–205. [Google Scholar] [CrossRef]
- Glocker, C.; Cataldi-Spinola, E.; Leine, R.I. Curve squealing of trains: Measurement, modelling and simulation. J. Sound Vib. 2009, 324, 365–386. [Google Scholar] [CrossRef]
- Pieringer, A. A numerical investigation of curve squeal in the case of constant wheel/rail friction. J. Sound Vib. 2014, 333, 4295–4313. [Google Scholar] [CrossRef]
- Ding, B.; Squicciarini, G.; Thompson, D. Effect of rail dynamics on curve squeal under constant friction conditions. J. Sound Vib. 2019, 442, 183–199. [Google Scholar] [CrossRef]
- Lai, V.V.; Chiello, O.; Brunel, J.F.; Dufrénoy, P. The critical effect of rail vertical phase response in railway curve squeal generation. Int. J. Mech. Sci. 2020, 167, 105281. [Google Scholar] [CrossRef]
- Jiang, J.; Anderson, D.C.; Dwight, R. The Mechanisms of Curve Squeal. In Noise and Vibration Mitigation for Rail Transportation Systems; Notes on Numerical Fluid Mechanics and Multidisciplinary Design; Springer: Berlin/Heidelberg, Germany, 2015; pp. 587–594. [Google Scholar] [CrossRef]
- Meehan, P.A. Prediction of wheel squeal noise under mode coupling. J. Sound Vib. 2020, 465, 115025. [Google Scholar] [CrossRef]
- Huang, Z.Y.; Thompson, D.J.; Jones, C.J.C. Squeal Prediction for a Bogied Vehicle in a Curve. Noise and Vibration Mitigation for Rail Transportation Systems; Springer: Berlin/Heidelberg, Germany, 2008; pp. 313–319. [Google Scholar] [CrossRef]
- Ding, B.; Squicciarini, G.; Thompson, D.; Corradi, R. An assessment of mode-coupling and falling-friction mechanisms in railway curve squeal through a simplified approach. J. Sound Vib. 2018, 423, 126–140. [Google Scholar] [CrossRef]
- Charroyer, L.; Chiello, O.; Sinou, J.J. Self-excited vibrations of a non-smooth contact dynamical system with planar friction based on the shooting method. Int. J. Mech. Sci. 2018, 144, 90–101. [Google Scholar] [CrossRef]
- Coudeyras, N.; Nacivet, S.; Sinou, J.J. Periodic and quasi-periodic solutions for multi-instabilities involved in brake squeal. J. Sound Vib. 2009, 328, 520–540. [Google Scholar] [CrossRef]
- Zenzerovic, I. Time-Domain Modelling of Curve Squeal: A Fast Model for One- and Two-Point Wheel/Rail Contact. Ph.D Thesis, Chalmers University of Technology, Gothenburg, Sweden, 2017. ISBN 9789175976471. [Google Scholar]
- Ding, B. The Mechanism of Railway Curve Squeal. Ph.D Thesis, University of Southampton, Southampton, UK, 2018. [Google Scholar]
- Loyer, A.; Sinou, J.J.; Chiello, O.; Lorang, X. Study of nonlinear behaviors and modal reductions for friction destabilized systems. Application to an elastic layer. J. Sound Vib. 2012, 331, 1011–1041. [Google Scholar] [CrossRef]
- Lai, V.V.; Anciant, M.; Chiello, O.; Brunel, J.F.; Dufrénoy, P. A nonlinear FE model for wheel/rail curve squeal in the time-domain including acoustic predictions. Appl. Acoust. 2021, 179, 108031. [Google Scholar] [CrossRef]
- Heckl, M.A.; Abrahams, I.D. Curve squeal of train wheels, part 1: Mathematical model for its generation. J. Sound Vib. 2000, 229, 669–693. [Google Scholar] [CrossRef]
- Giner-Navarro, J.; Martínez-Casas, J.; Denia, F.D.; Baeza, L. Study of railway curve squeal in the time domain using a high-frequency vehicle/track interaction model. J. Sound Vib. 2018, 431, 177–191. [Google Scholar] [CrossRef]
- Schneider, E.; Popp, K.; Irretier, H. Noise generation in railway wheels due to rail-wheel contact forces. J. Sound Vib. 1988, 120, 227–244. [Google Scholar] [CrossRef]
- Thompson, D.J. Railway Noise and Vibration: Mechanisms, Modelling and Means of Control; Elsevier: Amsterdam, The Netherlands, 2009. [Google Scholar]
- Ding, B.; Squicciarini, G.; Thompson, D.J. Effects of rail dynamics and friction characteristics on curve squeal. J. Phys. Conf. Ser. 2016, 744, 012146. [Google Scholar] [CrossRef]
- Kalker, J.J. Wheel-rail rolling contact theory. Wear 1991, 144, 243–261. [Google Scholar] [CrossRef]
- Shen, Z.Y.; Hedrick, J.K.; Elkins, J.A. A Comparison of Alternative Creep Force Models for Rail Vehicle Dynamic Analysis. Vehicle System Dynamics; Taylor & Francis: Abingdon, UK, 1983; Volume 12, pp. 79–83. [Google Scholar] [CrossRef]
- Newmark, N.M.; Asce, F. A method of computation for structural dynamics. J. Eng. Machanics Div. 1959, 85, 67–94. [Google Scholar] [CrossRef]
- Géradin, M.; Rixen, D. Mechanical Vibrations: Theory and Application to Structural Dynamics, 2nd ed.; Wiley: Hoboken, NJ, USA, 1997. [Google Scholar]



| Parameter [Units] | Description | Value |
|---|---|---|
| [km/h] | Rolling speed | 30 |
| [mrad] | Angle of attack | 11 |
| N [kN] | Normal load per wheel | 51 |
| [m] | Wheel diameter | 0.86 |
| [mm] | Lateral offset of the contact point | 15 |
| [rad] | Contact plane angle | −0.0145 |
| [m] | Rail transverse radius | 0.3 |
| E [Gpa] | Young modulus | 210 |
| [-] | Poisson’s ratio | 0.3 |
| [-] | Static friction coefficient | 0.3 |
| [-] | Parameter for friction law | 0.05 |
| [-] | Falling ratio on saturated regime | 0.2 |
| Free Modes | Stability Analysis | ||||
|---|---|---|---|---|---|
| Mode | Denomination | Frequency [Hz] | Damping Ratio [-] | Frequency [Hz] | Equivalent Damping Ratio [-] |
| 5 | 0L0 | 304.43 | 304.43 | ||
| 6 | 0L2 | 386.38 | 386.35 | ||
| 9 | 0L3 | 1028.8 | 1028.79 | ||
| 12 | 0L4 | 1853.9 | 1853.91 | ||
| 16 | - | 2369.8 | 2369.8 | ||
| 17 | 0L5 | 2776.3 | 2776.3 | ||
| 22 | 0L6 | 3752.6 | 3752.59 | ||
| 26 | - | 4561.5 | 4561.5 | ||
| 28 | 0L7 | 4762.2 | 4762.19 | ||
| 33 | 0L8 | 5794.7 | 5794.69 | ||
| Case Name | ||
|---|---|---|
| Modal-derived initial conditions | ||
| All_unstable_V0.1 | With Unstable modes and | |
| All_unstable_V1.0 | ⋯ Unstable modes and | |
| All_unstable_V2.0 | ⋯ Unstable modes and | |
| 0L0 | ⋯ “mode 0L0” | |
| 0L1 | ⋯ “mode 0L1” | |
| 0L2 | ⋯ “mode 0L2” | |
| 0L3 | ⋯ “mode 0L3” | |
| 0L4 | ⋯ “mode 0L4” | |
| 0L5 | ⋯ “mode 0L5” | |
| 0L6 | ⋯ “mode 0L6” | |
| 0L7 | ⋯ “mode 0L7” | |
| 0L8 | ⋯ “mode 0L8” | |
| Physically-derived initial conditions | ||
| V_-1.0 | With | |
| V_-0.5 | ||
| V_0.01 | ||
| V_0.1 | ||
| V_0.2 | ||
| V_0.3 | ||
| V_0.5 | ||
| V_1.0 | ||
| U_0.5 | With | |
| U_-0.5 | ||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Arango Montoya, J.; Chiello, O.; Sinou, J.-J.; Tufano, R. Mode Competition Phenomena and Impact of the Initial Conditions in Nonlinear Vibrations Leading to Railway Curve Squeal. Appl. Sci. 2025, 15, 509. https://doi.org/10.3390/app15020509
Arango Montoya J, Chiello O, Sinou J-J, Tufano R. Mode Competition Phenomena and Impact of the Initial Conditions in Nonlinear Vibrations Leading to Railway Curve Squeal. Applied Sciences. 2025; 15(2):509. https://doi.org/10.3390/app15020509
Chicago/Turabian StyleArango Montoya, Jacobo, Olivier Chiello, Jean-Jacques Sinou, and Rita Tufano. 2025. "Mode Competition Phenomena and Impact of the Initial Conditions in Nonlinear Vibrations Leading to Railway Curve Squeal" Applied Sciences 15, no. 2: 509. https://doi.org/10.3390/app15020509
APA StyleArango Montoya, J., Chiello, O., Sinou, J.-J., & Tufano, R. (2025). Mode Competition Phenomena and Impact of the Initial Conditions in Nonlinear Vibrations Leading to Railway Curve Squeal. Applied Sciences, 15(2), 509. https://doi.org/10.3390/app15020509







