Performance Analysis of Hybrid Steel–Concrete and Timber–Concrete Composite Pile Systems in Variable Density Sandy Soils Using Experimental and Numerical Insights
Abstract
1. Introduction
2. Literature Review
3. Materials, Methods, and Equipment
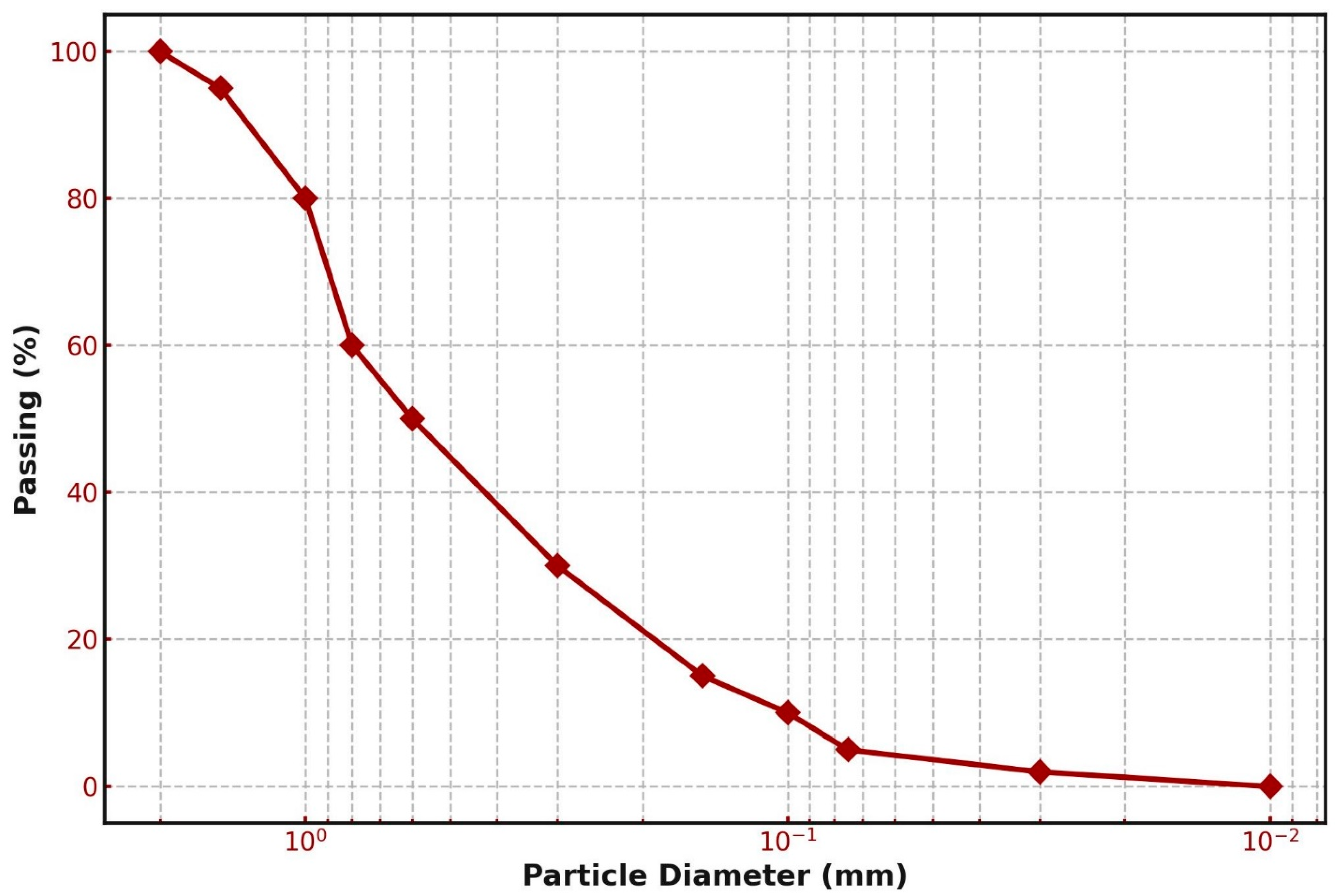
3.1. Granulometric and Index Properties Analysis of Well-Graded Sand
3.2. Pile Material Properties
3.2.1. Case Study I- Hybrid Steel–Concrete Composite Pile
3.2.2. Case Study II- Hybrid Timber–Concrete Composite Pile
3.3. Specimen Preparation and Test Method for Calibration of Relative Densities
3.4. Direct Shear Test (DST)
3.5. Interface Shear Test (IST)
3.6. Classical Bearing Capacity Methods
- = shaft (or skin friction) resistance mobilized along the pile’s lateral surface;
- = end-bearing resistance developed at the pile tip.
3.6.1. Terzaghi (1943) Methods
- K = lateral earth pressure coefficient (often parameterized as as per Bhusan’s (1983) equation to estimate K with (Dr) representing the relative density in percent);
- interface friction (or adhesion) angle between the pile composite materials and the soil.
3.6.2. Berezantsev’s (1961) Method
3.6.3. Brom (1966) Method
3.6.4. Hansen (1970) Method
- A = pile base area ( for circular piles);
- q′ = effective overburden pressure at the pile toe;
- B′ = pile diameter.
3.6.5. Meyerhof (1976) Method
3.6.6. Janbu (1976) Method
- = pile base area;
- q′ = effective overburden pressure at pile tip;
- Nq* = Janbu’s modified bearing capacity factor.
3.6.7. Vesic’s Method (1977)
- = soil elastic modulus;
- Poisson’s ratio.
3.6.8. Coyle and Castello (1981) Method
4. Results and Discussions
4.1. Properties and Behavior of Sand at Various Relative Densities
4.2. Skin Friction Angle Analysis in Pile–Soil Interfaces
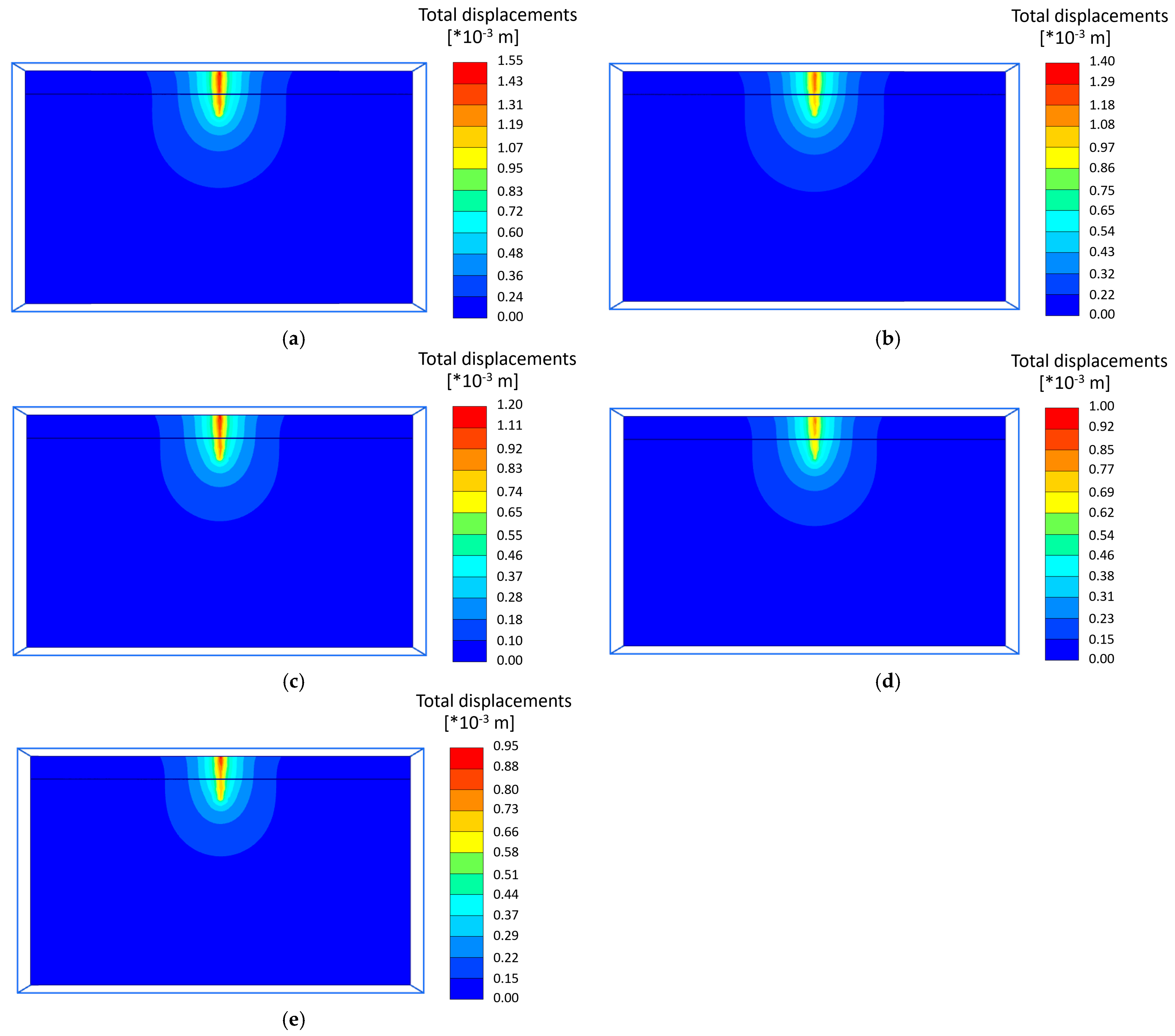
4.3. Load Transfer Mechanisms and Performance Dynamic in Hybrid Composite Pile Foundation Systems
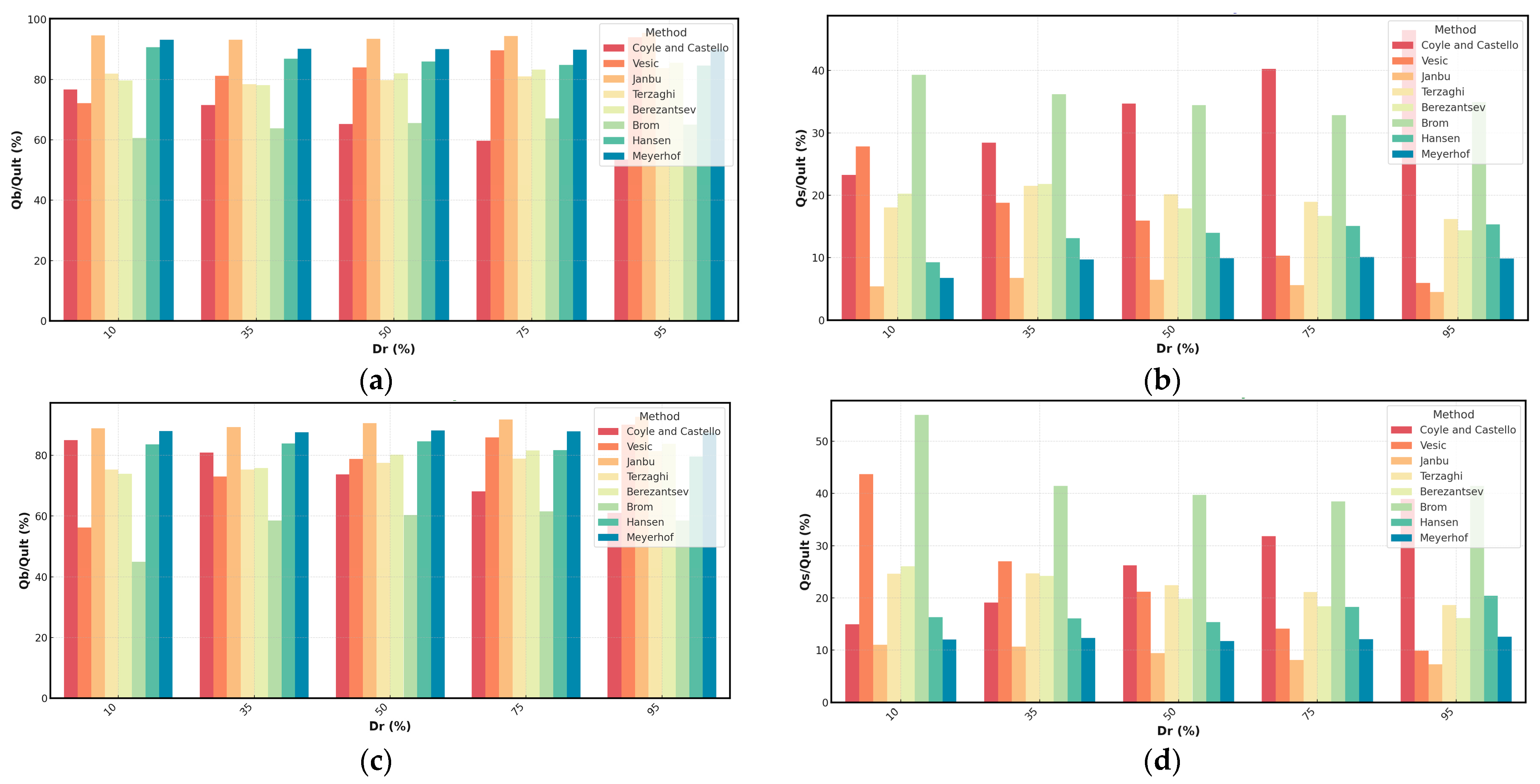
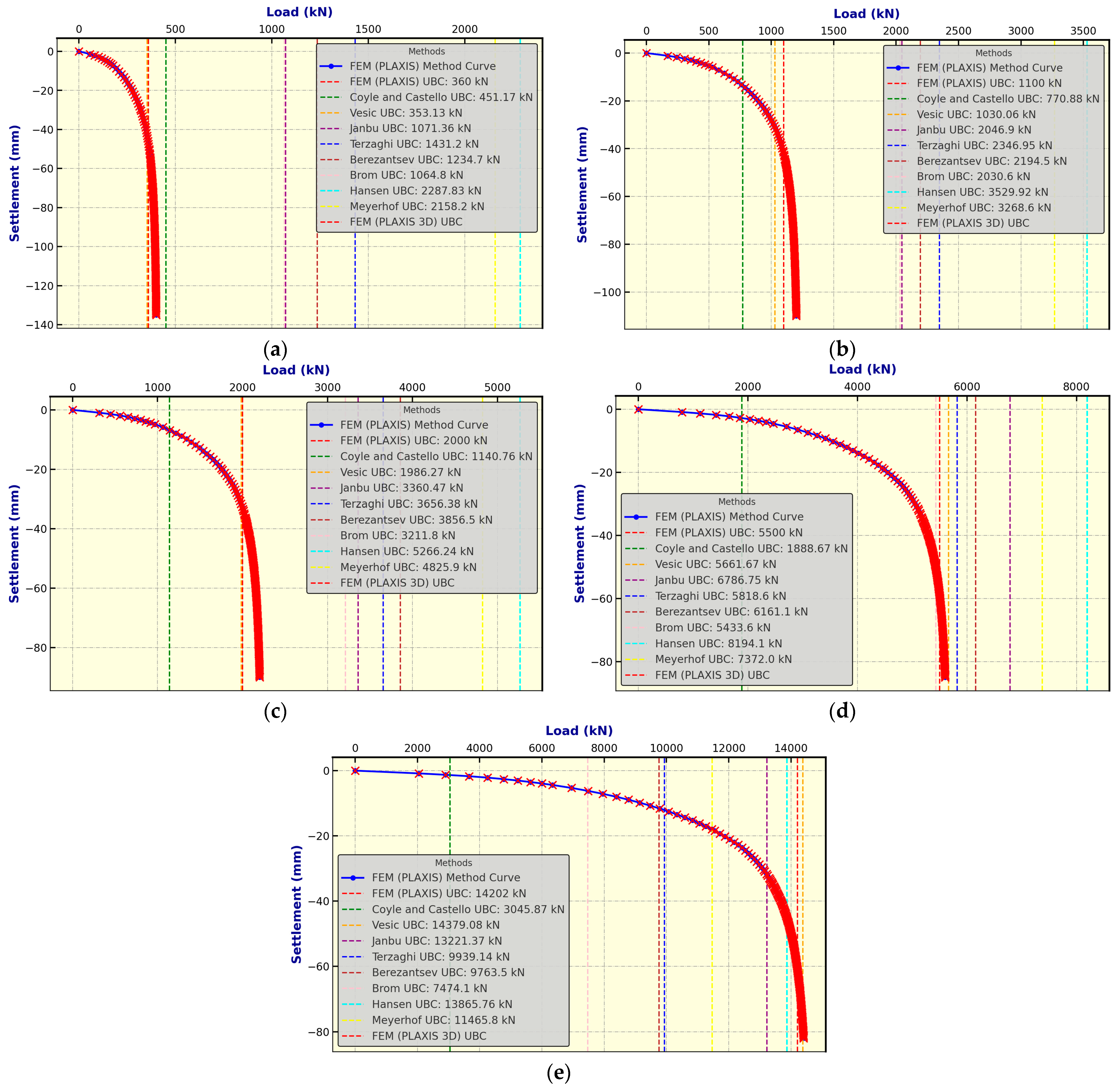
4.4. Ultimate Bearing Capacity (UBC) Variability in Hybrid Composite Pile Foundation Systems
5. Conclusions
- This study demonstrated the systematic relationship between sand relative density (Dr) and geotechnical properties, where increasing Dr from 10% to 95% reduced void ratios from 0.886 to 0.476, increased dry unit weight from 14.1 kN/m3 to 18.0 kN/m3, and elevated internal friction angles from 28° to 41°. Permeability decreased by three orders of magnitude (0.01 cm/s to 0.0001 cm/s), underscoring the critical role of compaction in enhancing soil strength and stability.
- Skin friction angles at pile–soil interfaces exhibited material-dependent behavior: concrete–steel interfaces increased from 12.8° (Dr = 10%) to 32.8° (Dr = 95%), while concrete–timber interfaces showed higher values, rising from 20.2° to 37.4° over the same Dr range. The 7.4° difference in very loose sand (Dr = 10%) highlights timber’s superior interfacial performance in low-density conditions.
- Load transfer mechanisms shifted significantly with soil density. For hybrid steel–concrete piles, skin friction contribution (Qs) increased from 23.3% (Dr = 10%) to 46.5% (Dr = 95%), while base resistance (Qb) decreased from 76.7% to 53.5%. Timber–concrete piles displayed a more variable response, with Qs dropping from 43.7% to 9.90% under Vesic’s method, demonstrating density-dependent interaction dynamics.
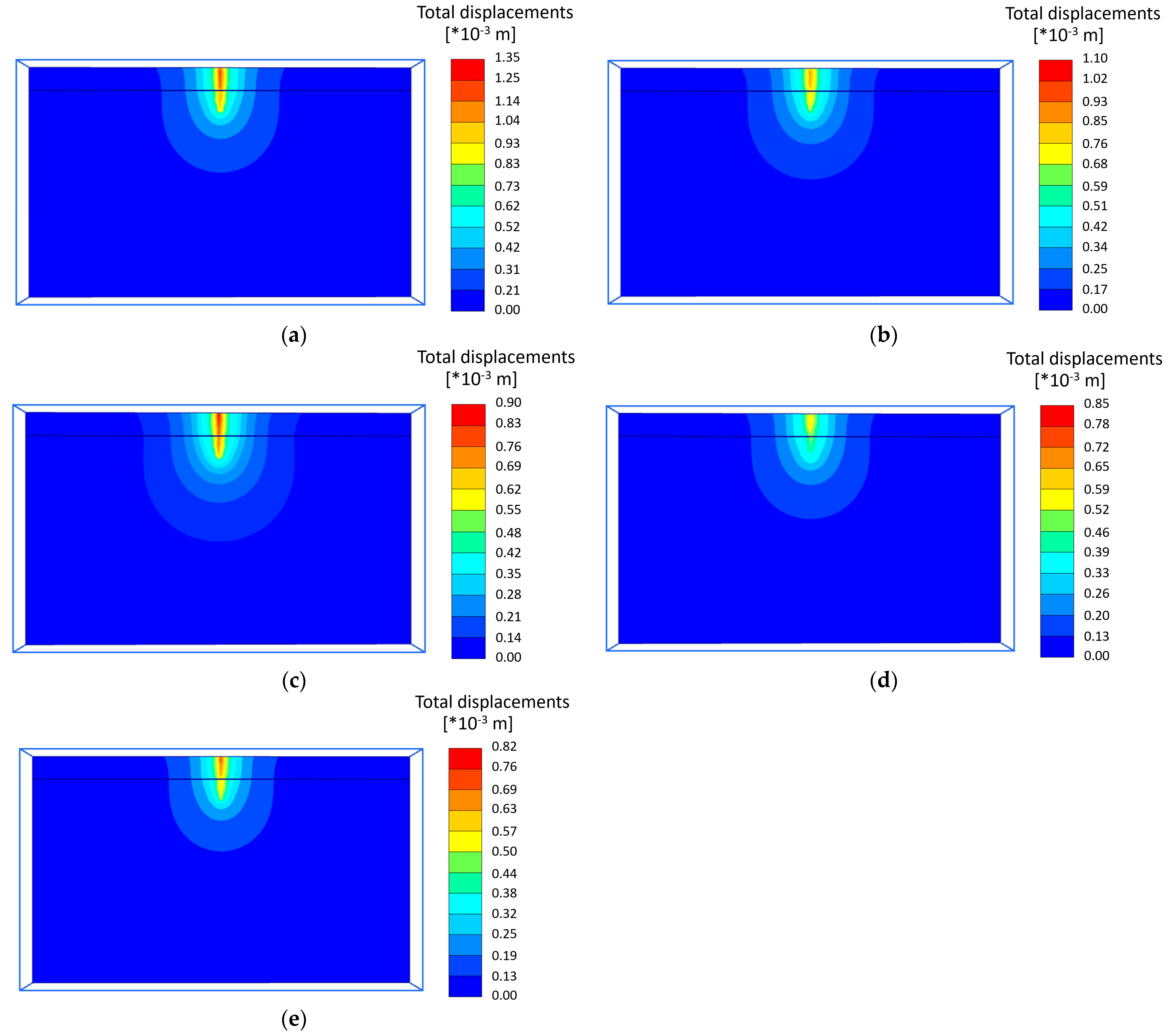
- Ultimate bearing capacity (UBC) predictions varied widely across methods, with steel–concrete piles ranging from 353.1 kN (Vesic, Dr = 10%) to 14,379 kN (Vesic, Dr = 95%), a 40.7-fold increase. Timber–concrete piles showed capacities between 244.1 kN (Vesic, Dr = 10%) and 9537.5 kN (Hansen, Dr = 95%). PLAXIS 3D simulations validated theoretical results, yielding 14,202 kN for steel–concrete at Dr = 95%, closely matching Vesic’s prediction (14,379 kN).
- Field implementations in Kano, Nigeria, confirmed hybrid systems’ efficacy: steel–concrete piles achieved a 22% higher UBC than conventional bored piles, while timber–concrete systems exhibited minimal differential settlement (≤5 mm over 18 months). Volumetric strain values (0.050–0.006) and interface friction angles (δ = 32.8° for steel–concrete at Dr = 95%) aligned with performance metrics, validating the designs’ adaptability to regional soil conditions.
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ibrahim, A.; Idris, A.; Umar, I.; Lin, H.; Shehu, H. Enhanced bearing capacity prediction using hybrid tree-based ensemble learning with advanced meta-heuristic optimization. Eng. Res. Express 2025, 7, 015113. [Google Scholar] [CrossRef]
- Umar, I.H.; Abubakar, A.; Salisu, I.M.; Lin, H.; Hassan, J.I. Geotechnical Stability Analysis of the Tiga Dam, Nigeria on the Assessment of Downstream Soil Properties, Erosion Risk, and Seasonal Expansion. Appl. Sci. 2024, 14, 6422. [Google Scholar] [CrossRef]
- Umar, I.H.; Lin, H.; Hassan, J.I. Transforming Landslide Prediction: A Novel Approach Combining Numerical Methods and Advanced Correlation Analysis in Slope Stability Investigation. Appl. Sci. 2024, 14, 3685. [Google Scholar] [CrossRef]
- Umar, I.H.; Lin, H.; Liu, H.; Cao, R. HELIOS-Stack: A Novel Hybrid Ensemble Learning Approach for Precise Joint Roughness Coefficient Prediction in Rock Discontinuity Analysis. Materials 2025, 18, 1807. [Google Scholar] [CrossRef]
- Umar, I.H.; Muhammad, A.; Lin, H.; Hassan, J.I.; Cao, R. Seasonal Dynamics in Soil Properties Along a Roadway Corridor: A Network Analysis Approach. Materials 2025, 18, 1708. [Google Scholar] [CrossRef]
- Umar, I.H.; Sukairaj, S.M.; Hang, L.; Izge, H.J.; Abdulaziz, A.; Shafiu, I.A.; Jechira, B.K. Performance characterisation of machine learning models for geotechnical axial pile load capacity estimation: An enhanced GPR-based approach. Geomech. Geoengin. 2025, 1–42. [Google Scholar] [CrossRef]
- Umar, I.; Abubakar, S.; Lin, H.; Hassan, J. Metakaolin as a soil stabilizing admixture: A comprehensive analysis of California bearing ratio and consolidation behavior using experimental and machine learning approaches. Earth Sci. Inform. 2025, 18, 200. [Google Scholar] [CrossRef]
- Umar, I.H.; Salisu, I.M.a.; Lin, H.; Hassan, J.I. Soil characterization, CBR modeling, and spatial variability analysis for road subgrade: A case study of Danchuwa—Jajere Road, Yobe State, Nigeria. Eng. Res. Express 2024, 6, 035119. [Google Scholar] [CrossRef]
- Zyka, K.; Mohajerani, A. Composite piles: A review. Constr. Build. Mater. 2016, 107, 394–410. [Google Scholar] [CrossRef]
- Dias, A.; Skinner, J.; Crews, K.; Tannert, T. Timber-concrete-composites increasing the use of timber in construction. Eur. J. Wood Wood Prod. 2016, 74, 443–451. [Google Scholar] [CrossRef]
- El-Salakawy, T.S.; Gamal, A.A. Behavior of strengthened Timber Concrete Composite under axial loads. Case Stud. Constr. Mater. 2021, 14, e00497. [Google Scholar] [CrossRef]
- Trabucco, D.; Perrucci, G. Steel–Timber Hybrid Buildings: A Comparative Life Cycle Assessment Study of Global Warning Potential Impacts. Sustainability 2025, 17, 718. [Google Scholar] [CrossRef]
- Zhang, X.; Xuan, L.; Huang, W.; Yuan, L.; Li, P. Structural Design and Analysis for a Timber-Concrete Hybrid Building. Front. Mater. 2022, 9, 844398. [Google Scholar] [CrossRef]
- Uge, B.U.; Guo, Y.-C. CFG Pile Composite Foundation: Its Engineering Applications and Research Advances. J. Eng. 2020, 19, 5343472. [Google Scholar] [CrossRef]
- He, D.; Cheng, Y.; Liu, H.; Lin, H. Pile–Soil Interaction and Group Pile Effect in Composite Foundation Under Different Pile Length Conditions. Buildings 2025, 15, 1248. [Google Scholar] [CrossRef]
- Zhang, W.; Xiang, J.; Huang, R.; Liu, H. A review of bio-inspired geotechnics-perspectives from geomaterials, geo-components, and drilling & excavation strategies. Biogeotechnics 2023, 1, 100025. [Google Scholar] [CrossRef]
- Jelušič, P. Enhancing Sustainability of Building Foundations with Efficient Open-End Pile Optimization. Sustainability 2024, 16, 6880. [Google Scholar] [CrossRef]
- Najar, I.A.; Ahmadi, R.; Amuda, A.G.; Mourad, R.; Bendary, N.E.; Ismail, I.; Bakar, N.A.; Tang, S. Advancing soil-structure interaction (SSI): A comprehensive review of current practices, challenges, and future directions. J. Infrastruct. Preserv. Resil. 2025, 6, 5. [Google Scholar] [CrossRef]
- Chorafa, E.; Skrapalliou, E.; Katsimpini, P. On the Nonlinear Behavior of Composite Structures under Multiple Earthquakes Considering Soil–Structure Interaction. CivilEng 2024, 5, 673–693. [Google Scholar] [CrossRef]
- Liu, T.-Y.; Ho, S.-J.; Tserng, H.-P.; Tzou, H.-K. Using a Unique Retaining Method for Building Foundation Excavation: A Case Study on Sustainable Construction Methods and Circular Economy. Buildings 2022, 12, 298. [Google Scholar] [CrossRef]
- Bao, Y.; Lu, W.; Yue, K.; Lu, B.; Dai, C.; Qiu, G. Experimental and nonlinear-analytical studies of cross-laminated-timber concrete composite floors with interface shear resistance enhancement of plate-end. Constr. Build. Mater. 2023, 403, 133042. [Google Scholar] [CrossRef]
- Sun, L.; Dong, W.; Lin, J. Analysis of Pull-out of Shear Connectors in Steel-Concrete Composite Structures Considering Discontinuous Deformation. J. Phys. Conf. Ser. 2023, 2519, 012024. [Google Scholar] [CrossRef]
- Kia, L.; Valipour, H. Composite timber-steel encased columns subjected to concentric loading. Eng. Struct. 2021, 232, 111825. [Google Scholar] [CrossRef]
- Zhang, D.; Bao, Y.; Gao, J.; Xiao, L.; Li, X. Research on Load Transfer Mechanism of Steel-Concrete Joint Section of Hybrid Beam Cable-Stayed Bridge. Adv. Mater. Res. 2013, 639, 216–219. [Google Scholar] [CrossRef]
- You, X.; Xing, Z.; Jiang, S.; Zhu, Y.; Lin, Y.; Qiu, H.; Nie, R.; Yang, J.; Hui, D.; Chen, W.; et al. A review of research on aluminum alloy materials in structural engineering. Dev. Built Environ. 2024, 17, 100319. [Google Scholar] [CrossRef]
- Chen, W.; Fang, H.; Zhu, L.; Zhang, X.; Zhu, J. Bending energy absorption performance of composite fender piles with different winding angles. Rev. Adv. Mater. Sci. 2024, 63, 20240004. [Google Scholar] [CrossRef]
- Salga, M.S. Modelling of Axial Capacity of Driven Piles Using Artificial Neural Network (ANN) and Artificial Neurofuzzy Inference System (ANFIS). Master’s Thesis, Bayero University Kano, Kano, Nigeria, 2023. [Google Scholar]
- Spagnoli, G. Some considerations regarding the use of helical piles as foundation for offshore structures. Soil Mech. Found. Eng. 2013, 50, 102–110. [Google Scholar] [CrossRef]
- Leong, W.K.; Yusoff, N.A.; Aziz, A.N.A.; Talib, Z.A. Theoretical and Actual Bearing Capacity of Driven Piles Using Model Piles in Sand. Appl. Mech. Mater. 2015, 773, 1453–1459. [Google Scholar] [CrossRef]
- Dehghanbanadaki, A.; Motamedi, S. Bearing capacity prediction of shallow foundation on sandy soils: A comparative study of analytical, FEM, and machine learning approaches. Multiscale Multidiscip. Model. Exp. Des. 2023, 7, 1293–1310. [Google Scholar] [CrossRef]
- Gavin, K.; O’Kelly, B. Effect of Friction Fatigue on Pile Capacity in Dense Sand. J. Geotech. Geoenvironmental Eng. 2007, 133, 63–71. [Google Scholar] [CrossRef]
- Randolph, M. Science and empiricism in pile foundation design. Geotechnique 2003, 53, 847–875. [Google Scholar] [CrossRef]
- Meyerhof, G. The bearing capacity of rigid piles and pile groups under inclined loads in clay. Can. Geotech. J. 2011, 18, 297–300. [Google Scholar] [CrossRef]
- Huat, C.Y.; Moosavi, S.M.; Mohammed, A.S.; Armaghani, D.J.; Ulrikh, D.V.; Monjezi, M.; Hin Lai, S. Factors Influencing Pile Friction Bearing Capacity: Proposing a Novel Procedure Based on Gradient Boosted Tree Technique. Sustainability 2021, 13, 11862. [Google Scholar] [CrossRef]
- Schanz, T.; Vermeer, P. Angles of friction and dilatancy of sand. Géotechnique 1996, 46, 145–151. [Google Scholar] [CrossRef]
- Reese, L.; Isenhower, W.; Wang, S.T. Analysis and Design of Shallow and Deep Foundations; John Wiley & Sons: Hoboken, NJ, USA, 2007; pp. 1–569. [Google Scholar] [CrossRef]
- Alawneh, A.; Nusier, O.; Husein Malkawi, A.; Alsaleh, M. Axial compressive capacity of driven piles in sand: A method including post-driving residual stresses. Can. Geotech. J. 2001, 38, 364–377. [Google Scholar] [CrossRef]
- Fu, D.; Li, S.; Zhang, H.; Jiang, Y.; Liu, R.; Li, C. The Influence Depth of Pile Base Resistance in Sand-Layered Clay. Sustainability 2023, 15, 7221. [Google Scholar] [CrossRef]
- Loukidis, D.; Salgado, R. Effect of relative density and stress level on the bearing capacity of footings on sand. Géotechnique 2011, 61, 107–119. [Google Scholar] [CrossRef]
- Robertson, P. In situ testing and its application to foundation engineering. Can. Geotech. J. 2011, 23, 573–594. [Google Scholar] [CrossRef]
- Robertson, P.K. Interpretation of cone penetration tests—A unified approach. Can. Geotech. J. 2009, 46, 1337–1355. [Google Scholar] [CrossRef]
- Clausen, C.J.F.; Aas, P.M.; Karlsrud, K. Bearing capacity of driven piles in sand, the NGI approach. In Proceedings of the International Symposium. on Frontiers in Offshore Geotechnics, Perth, Australia, 19–21 September 2005; pp. 677–681. [Google Scholar] [CrossRef]
- Zhang, Y.; Liu, Y.; Luo, H.; Chen, P.; Li, D.; Liu, E.; Yang, B. A method of calculating the bearing capacity of sand pile composite foundations in a mucky soil layer considering consolidation. Sci. Rep. 2021, 11, 16962. [Google Scholar] [CrossRef]
- Chen, Y.-J.; Lin, S.-S.; Chang, H.-W.; Marcos, M. Evaluation of side resistance capacity for drilled shafts. J. Mar. Sci. Technol. 2011, 19, 13. [Google Scholar] [CrossRef]
- O’Neill, M. Side Resistance in Piles and Drilled Shafts. J. Geotech. Geoenviron. Eng. 2001, 127, 11–20. [Google Scholar] [CrossRef]
- Rausche, F.; Nagy, M.; Webster, S.; Liang, L. CAPWAP and Refined Wave Equation Analyses for Driveability Predictions and Capacity Assessment of Offshore Pile Installations. In Proceedings of the ASME 2009 28th International Conference on Ocean, Offshore and Arctic Engineering, Honolulu, Hawaii, USA, 31 May–6 June 2009. [Google Scholar]
- Salgado, R.; Zhang, Y.; Abou-Jaoude, G.; Loukidis, D.; Bisht, V. Pile driving formulas based on pile wave equation analyses. Comput. Geotech. 2017, 81, 307–321. [Google Scholar] [CrossRef]
- Kalauni, H.K.; Masud, N.B.; Ng, K.; Wulff, S.S. Improved Wave Equation Analysis for Piles in Soil-Based Intermediate Geomaterials with LRFD Recommendations and Economic Impact Assessment. Geotechnics 2024, 4, 362–381. [Google Scholar] [CrossRef]
- Khazaei, J.; Eslami, A. Behavior of helical piles—As a geoenvironmental choice—By frustum confining vessel. Adv. Sci. Technol. Res. J. 2016, 10, 8–22. [Google Scholar] [CrossRef]
- Abu-Farsakh, M.; Titi, H. Probabilistic CPT Method for Estimating the Ultimate Capacity of Friction Piles. Geotech. Test. J. 2007, 30, 12–24. [Google Scholar] [CrossRef]
- Ullah, S.N.; O’Loughlin, C.; Hu, Y.; Hou, L.F. Torsional installation and vertical tensile capacity of helical piles in clay. Geotechnique 2024, 74, 1360–1376. [Google Scholar] [CrossRef]
- Venkatesan, V.; Mayakrishnan, M. Behavior of Mono Helical Pile Foundation in Clays under Combined Uplift and Lateral Loading Conditions. Appl. Sci. 2022, 12, 6827. [Google Scholar] [CrossRef]






| Author (Study) | Year | Methods | Results Summary |
|---|---|---|---|
| Performance of hybrid cross-laminated timber (CLT)–concrete composite by Bao, Lu [21] | 2023 | Push-out tests | CLT–concrete composites exhibit efficient force-slip response and slip modulus, with defined failure modes. |
| Steel–concrete composites with anti-pullout connectors by Sun, Dong [22] | 2023 | Sample analysis | Anti-pullout connectors reduce rebar stress by >50% and mitigate the capacity decline. |
| Composite timber columns confined with steel by Kia and Valipour [23] | 2021 | Compression experiments | Confined timber columns show enhanced compressive performance. |
| Timber–concrete composites (TCC) by El-Salakawy and Gamal [11] | 2024 | Case studies, regulatory analysis | TCC systems reduce embodied carbon by 30% and increase timber used in construction. |
| Steel–concrete joints in hybrid bridges by Zhang, Bao [24] | 2025 | Analytical investigation | Load transfer mechanisms in steel–concrete joints depend on connection design and material synergy. |
| Steel, aluminum, and composite structures by You, Xing [25] | 2024 | Review of trends | Covers advancements in composite structural systems and design frameworks. |
| Composite fender piles by Chen, Fang [26] | 2024 | Bending tests | Bending energy absorption varies with pile material and configuration. |
| Steel–timber hybrid buildings by Trabucco and Perrucci [12] | 2025 | LCA analysis | Life cycle assessments (LCAs) reveal that timber–concrete hybrids reduce embodied carbon |
| Current Study | 2025 | Classical bearing capacity methods (e.g., Terzaghi, Vesic) + PLAXIS 3D simulations | Work on composite systems, soil densification, and sustainability, offering optimized design strategies for semi-arid regions with fluctuating groundwater. |
| Sand Geotechnical Properties | Values |
|---|---|
| D10 (particle diameter at 10% finer) | 0.10 mm |
| D30 (particle diameter at 30% finer) | 0.30 mm |
| D50 (particle diameter at 50% finer) | 0.60 mm |
| D60 (particle diameter at 60% finer) | 0.80 mm |
| Coefficient of curvature (Cc) | 1.13 |
| Coefficient of uniformity (Cu) | 8.00 |
| USCS class | SW |
| Maximum grain size (Dmax) | 2.00 mm |
| Minimum grain size (Dmin) | 0.01 mm |
| Difference (Dmax − Dmin) | 1.99 mm |
| Specific gravity (Gs) | 2.68 |
| Minimum void ratio (emin) | 0.453 |
| Maximum void ratio (emax) | 0.920 |
| Minimum dry unit weight (γd, min) | 13.7 kN/m3 |
| Maximum dry unit weight (γd, max) | 18.2 kN/m3 |
| Minimum optimum moisture content (OMCmin) | 7.0% |
| Minimum optimum moisture content (OMCmax) | 10.0% |
| Parameter | Steel Pile | Concrete Pile | Timber Pile |
|---|---|---|---|
| Material Model | Linear Elastic | Linear Elastic | Linear Elastic |
| Poisson’s Ratio, ν | 0.20 ± 0.02 | 0.20 ± 0.02 | 0.20 ± 0.02 |
| Modulus of Elasticity, E (kN/m2) | 2.01 × 108 ± 5% | 2.35 × 107 ± 5% | 1.22 × 107 ± 5% |
| Dry Unit Weight, γd (kN/m3) | 76.5 ± 1.5 | 25.0 ± 0.5 | 5.10 ± 0.10 |
| Relative Density, Dr (%) | 10 | 35 | 50 | 75 | 95 |
|---|---|---|---|---|---|
| Description | Very Loose | Loose | Medium | Dense | Very Dense |
| Void Ratio, e | 0.886 ± 0.015 | 0.771 ± 0.012 | 0.682 ± 0.010 | 0.565 ± 0.008 | 0.476 ± 0.006 |
| Dry Unit Weight, γd (kN/m3) | 14.1 ± 0.3 | 15.2 ± 0.3 | 15.9 ± 0.3 | 17.0 ± 0.3 | 18.0 ± 0.4 |
| Internal Friction Angle, φ (°) | 28 ± 0.5 | 30.9 ± 0.6 | 33.7 ± 0.6 | 36.6 ± 0.7 | 40 ± 0.8 |
| Permeability, k (cm/s) | 1.0 × 10−2 ± 8% | 2.6 × 10−3 ± 8% | 1.1 × 10−3 ± 8% | 3.0 × 10−4 ± 12% | 1.0 × 10−4 ± 12% |
| Relative Density, Dr (%) | 10 | 35 | 50 | 75 | 95 |
|---|---|---|---|---|---|
| Sand Description | Very Loose | Loose | Medium | Dense | Very Dense |
| δ (Concrete–Steel) (°) | 12.8 ± 0.5 | 18.6 ± 0.6 | 22.6 ± 0.7 | 27.4 ± 0.8 | 32.8 ± 0.9 |
| δ (Concrete–Timber) (°) | 20.2 ± 0.6 | 22.3 ± 0.7 | 25.5 ± 0.7 | 30.7 ± 0.8 | 37.4 ± 1.0 |
| Relative Density, Dr (%) | 10 | 35 | 50 | 75 | 95 |
|---|---|---|---|---|---|
| Sand Description | Very Loose | Loose | Medium | Dense | Very Dense |
| Material Model | Mohr–Coulomb | Mohr–Coulomb | Mohr–Coulomb | Mohr–Coulomb | Mohr–Coulomb |
| Drainage Type | Drained | Drained | Drained | Drained | Drained |
| Poisson’s Ratio, ν | 0.3 ± 0.02 | 0.3 ± 0.02 | 0.3 ± 0.02 | 0.3 ± 0.02 | 0.3 ± 0.02 |
| Modulus of Elasticity, E (kN/m2) | 12,000 ± 600 | 26,500 ± 1325 | 32,300 ± 1615 | 58,800 ± 2940 | 70,100 ± 3505 |
| Dry Unit Weight, γd (kN/m3) | 14.1 ± 0.3 | 15.2 ± 0.3 | 15.9 ± 0.3 | 17.0 ± 0.3 | 18.0 ± 0.4 |
| Saturated Unit Weight, γsat (kN/m3) | 22.60 ± 0.45 | 22.60 ± 0.45 | 22.60 ± 0.45 | 22.60 ± 0.45 | 22.60 ± 0.45 |
| Internal Friction Angle, ϕ (°) | 28 ± 0.5 | 30.9 ± 0.6 | 33.7 ± 0.6 | 36.6 ± 0.7 | 40 ± 0.8 |
| Dilatancy Angle, ψ (°) | 0 ± 0.2 | 5.9 ± 0.3 | 9.4 ± 0.4 | 15.3 ± 0.5 | 20 ± 0.6 |
| Cohesion, c (kN/m2) | 0 ± 0.05 kN/m2 | 0.115 ± 0.01 | 0.15 ± 0.01 | 0.445 ± 0.03 | 0.706 ± 0.04 |
| Interaction Factor, Rint | 0.8 ± 0.05 | 0.8 ± 0.05 | 0.8 ± 0.05 | 0.8 ± 0.05 | 0.8 ± 0.05 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Umar, I.H.; Fırat, M.E.; Lin, H.; Shehu, H.T.; Cao, R. Performance Analysis of Hybrid Steel–Concrete and Timber–Concrete Composite Pile Systems in Variable Density Sandy Soils Using Experimental and Numerical Insights. Appl. Sci. 2025, 15, 5868. https://doi.org/10.3390/app15115868
Umar IH, Fırat ME, Lin H, Shehu HT, Cao R. Performance Analysis of Hybrid Steel–Concrete and Timber–Concrete Composite Pile Systems in Variable Density Sandy Soils Using Experimental and Numerical Insights. Applied Sciences. 2025; 15(11):5868. https://doi.org/10.3390/app15115868
Chicago/Turabian StyleUmar, Ibrahim Haruna, Müge Elif Fırat, Hang Lin, Hamza Tijjani Shehu, and Rihong Cao. 2025. "Performance Analysis of Hybrid Steel–Concrete and Timber–Concrete Composite Pile Systems in Variable Density Sandy Soils Using Experimental and Numerical Insights" Applied Sciences 15, no. 11: 5868. https://doi.org/10.3390/app15115868
APA StyleUmar, I. H., Fırat, M. E., Lin, H., Shehu, H. T., & Cao, R. (2025). Performance Analysis of Hybrid Steel–Concrete and Timber–Concrete Composite Pile Systems in Variable Density Sandy Soils Using Experimental and Numerical Insights. Applied Sciences, 15(11), 5868. https://doi.org/10.3390/app15115868









