Characterisation of the Pump-Suction Flow Field of Antarctic Krill and Key Influencing Factors
Abstract
1. Introduction
2. Materials and Methods
2.1. Control Equations
2.2. Turbulence Modelling
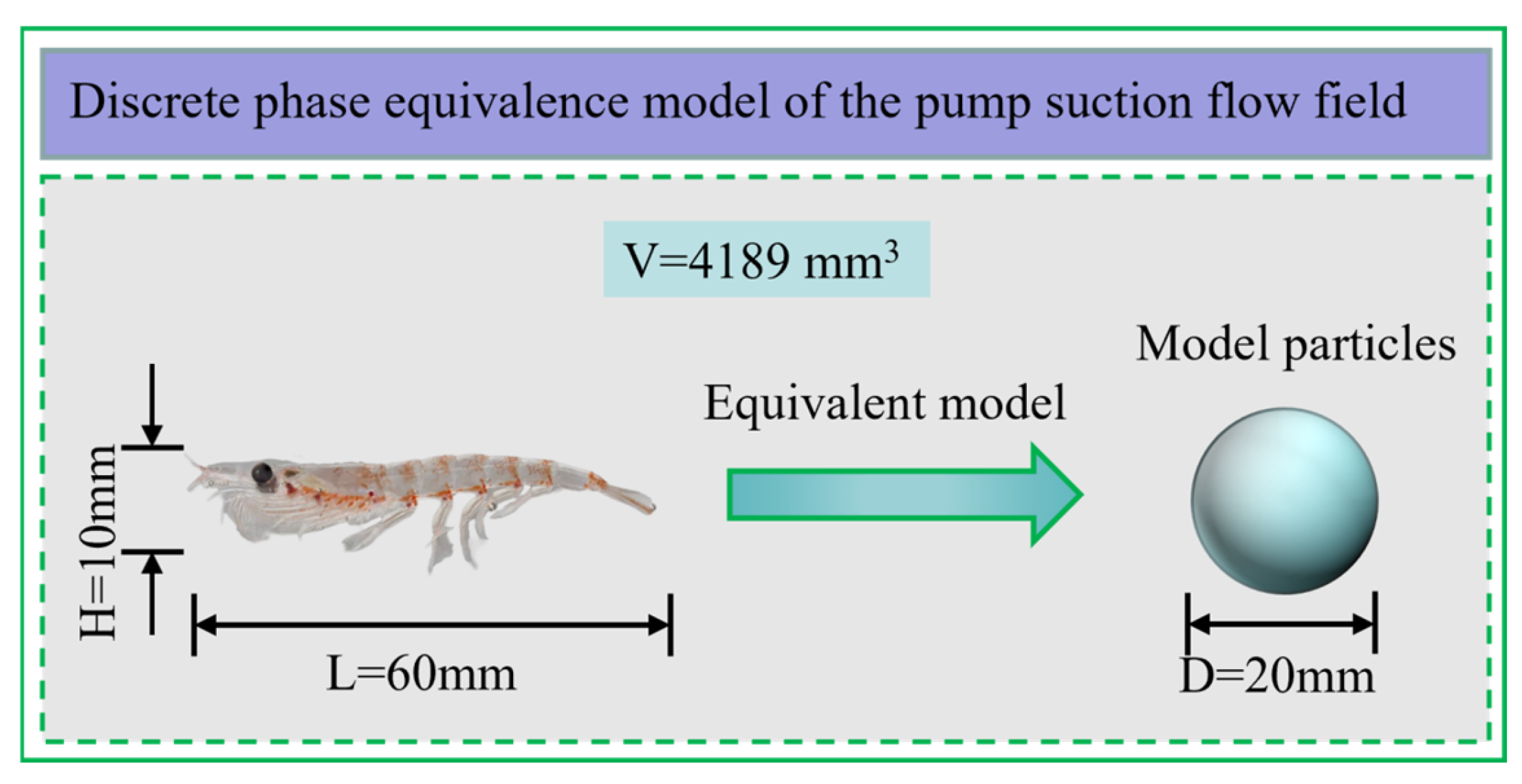
2.3. DPM Model
2.4. Model Parameter
2.5. Verification of Results
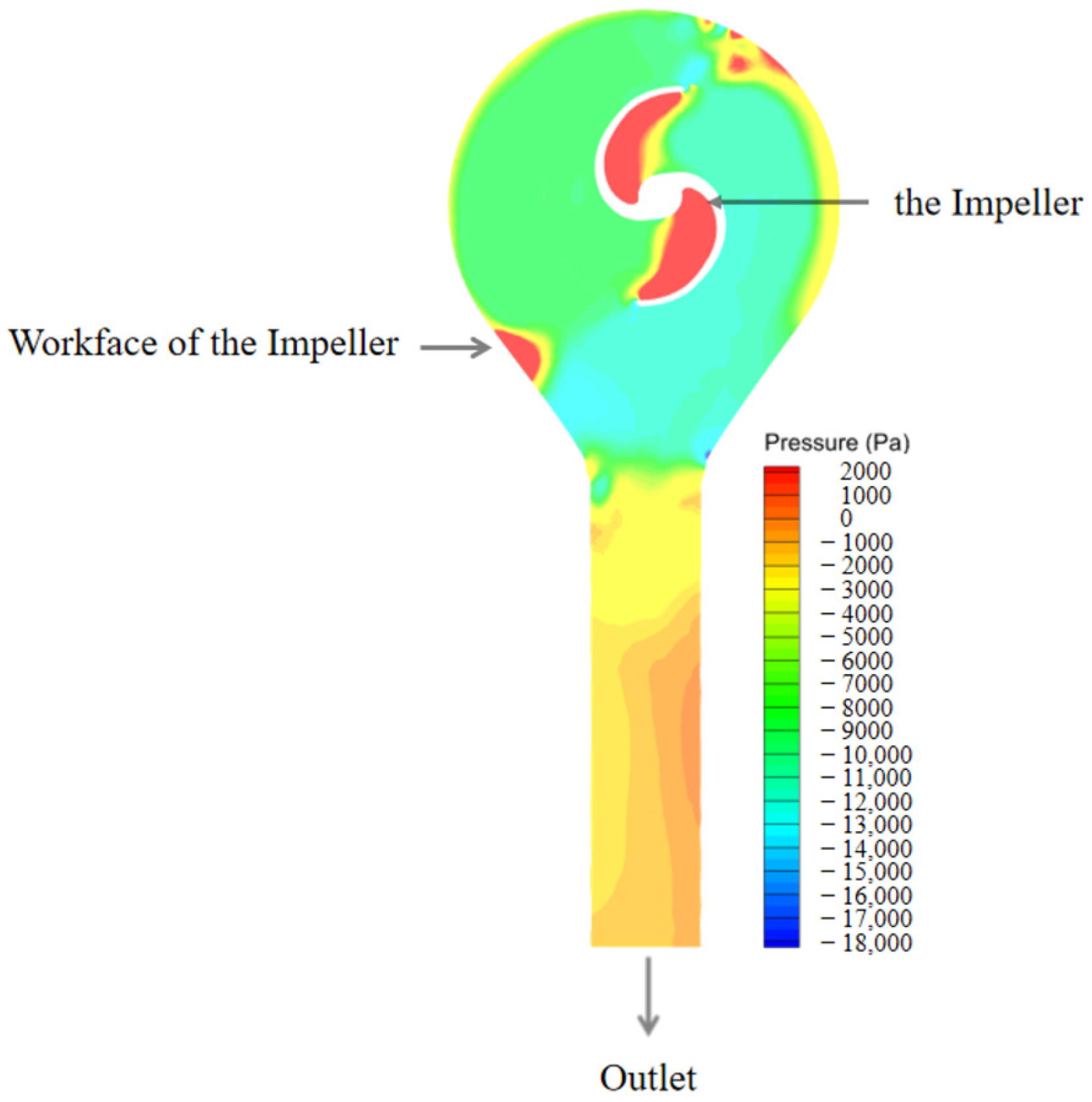
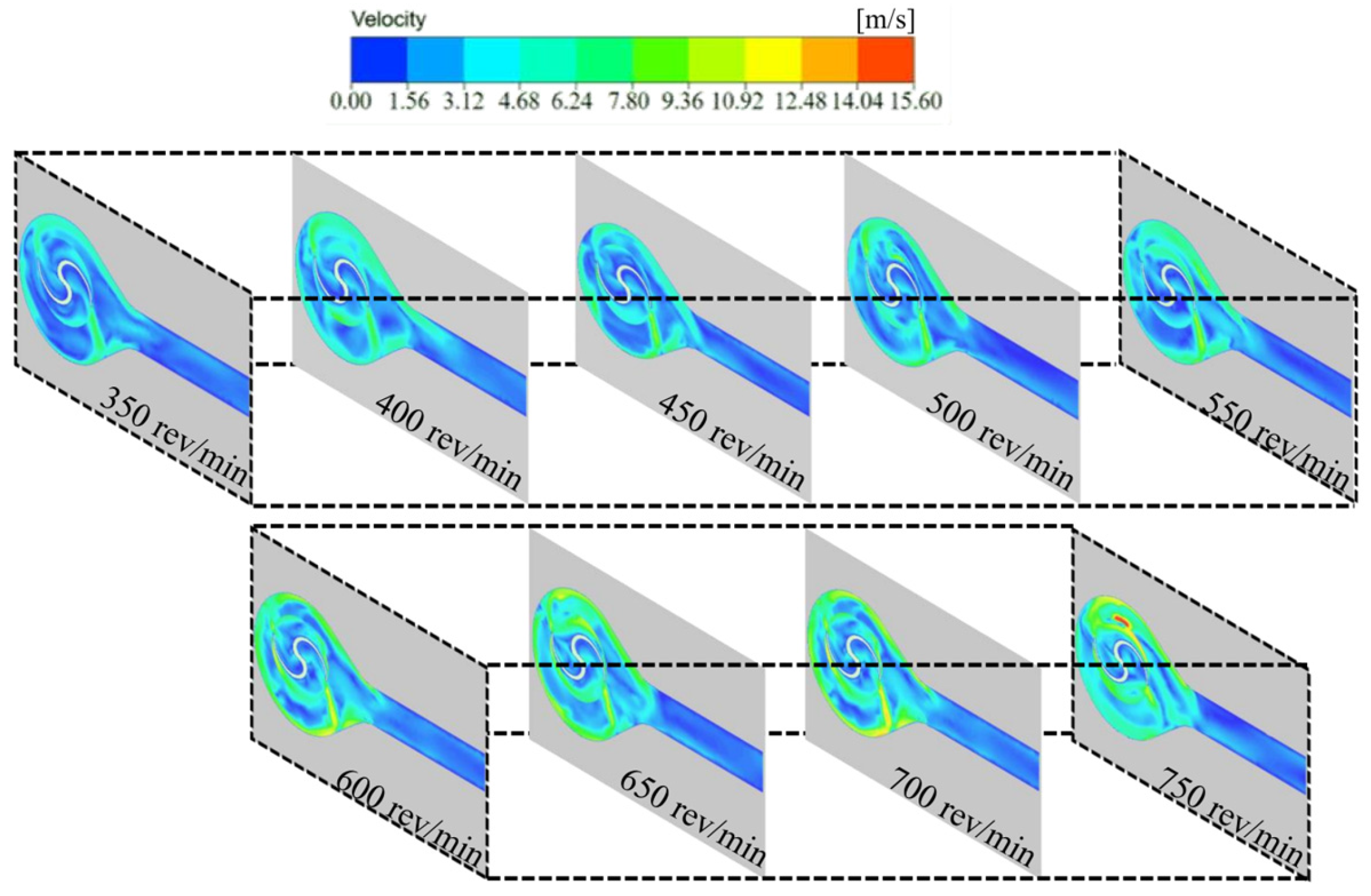
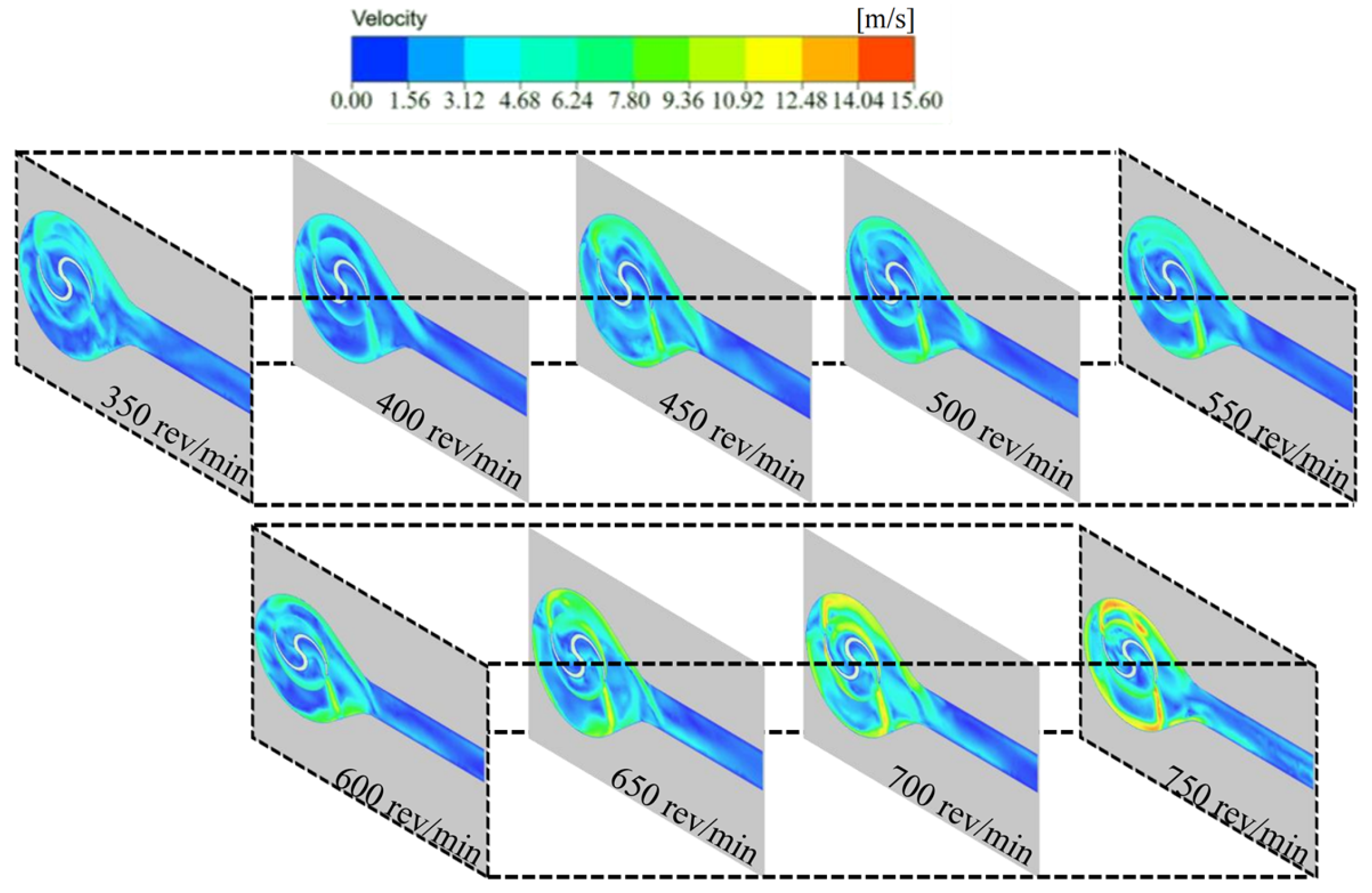
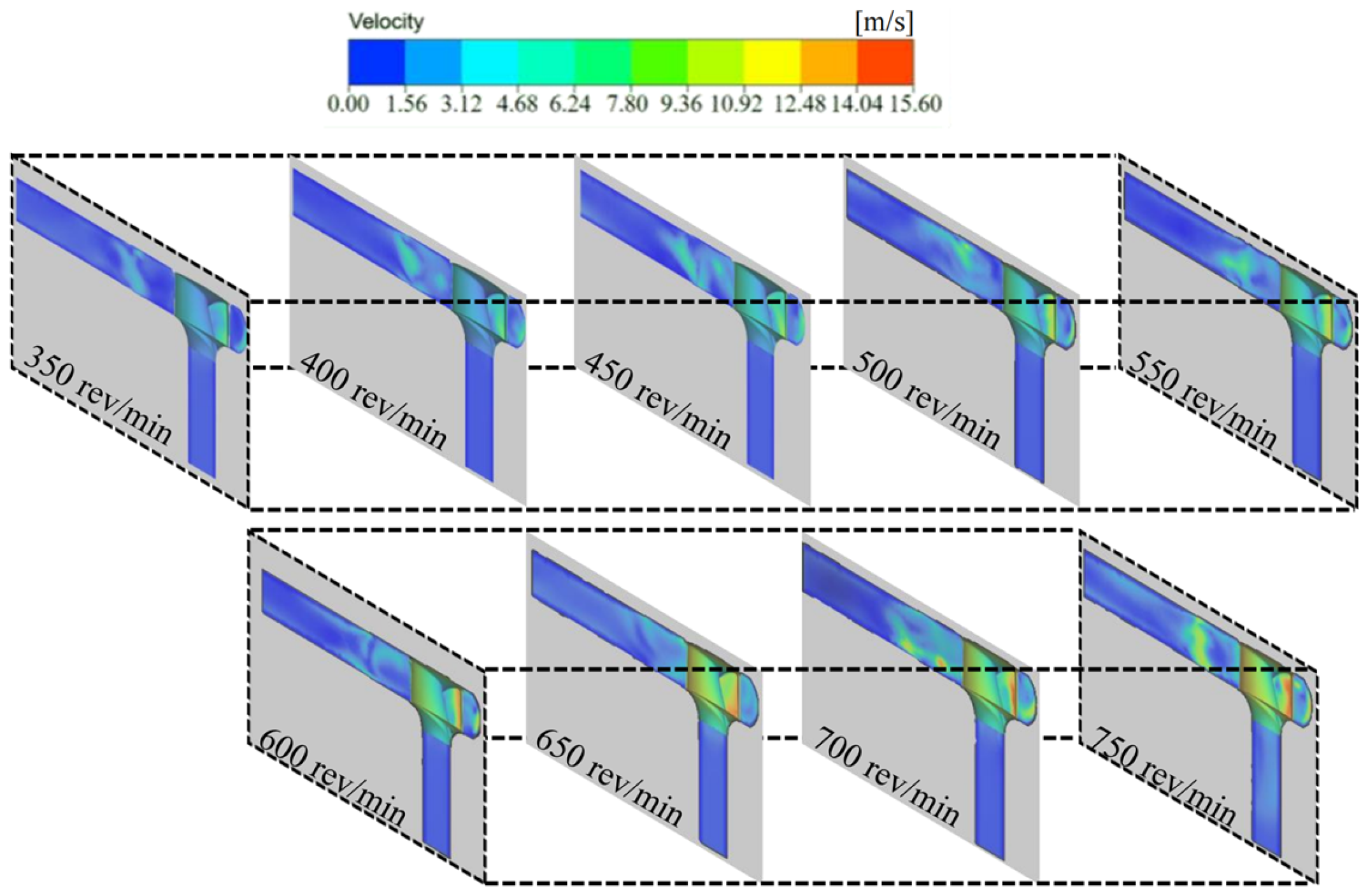
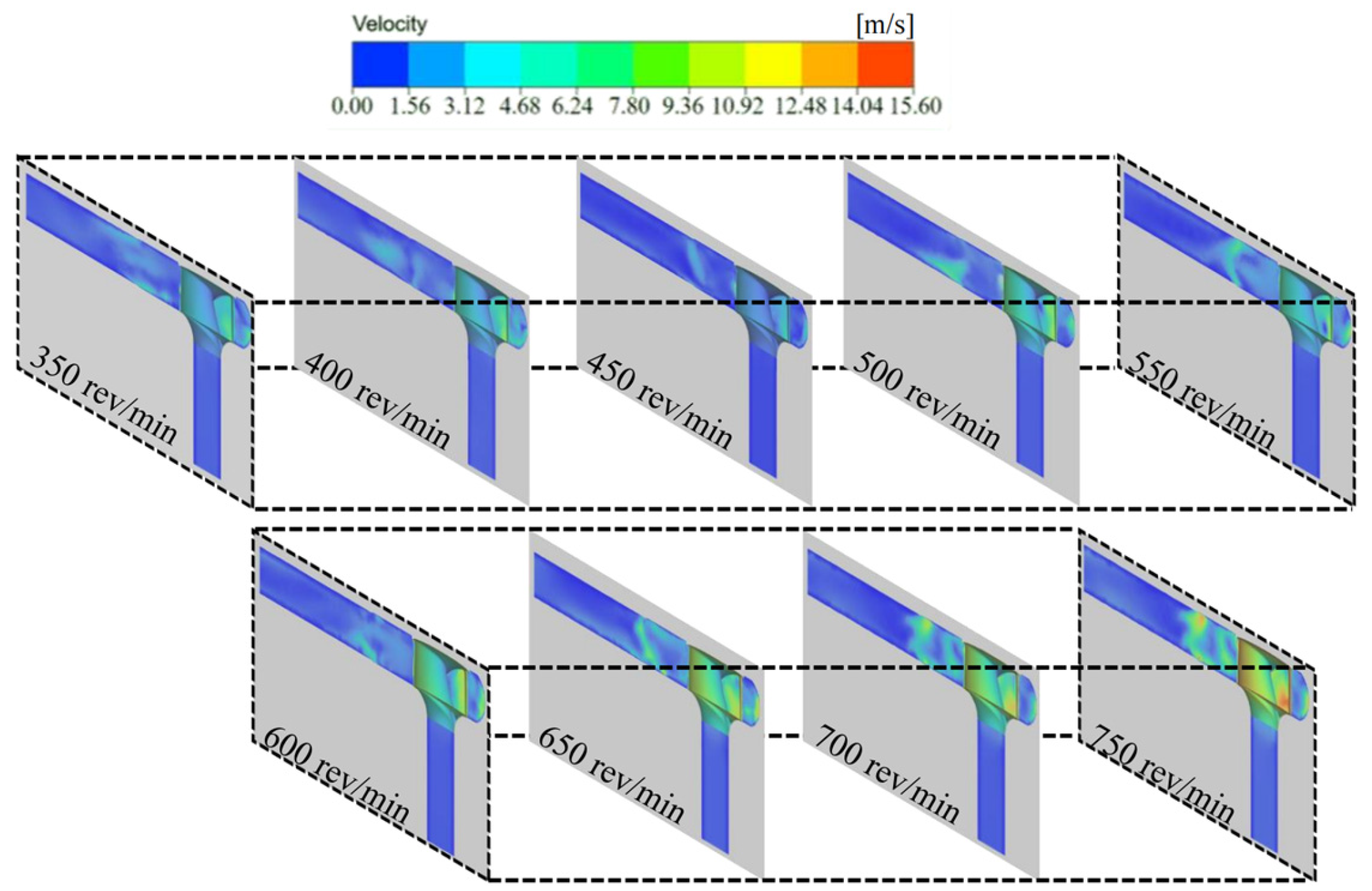
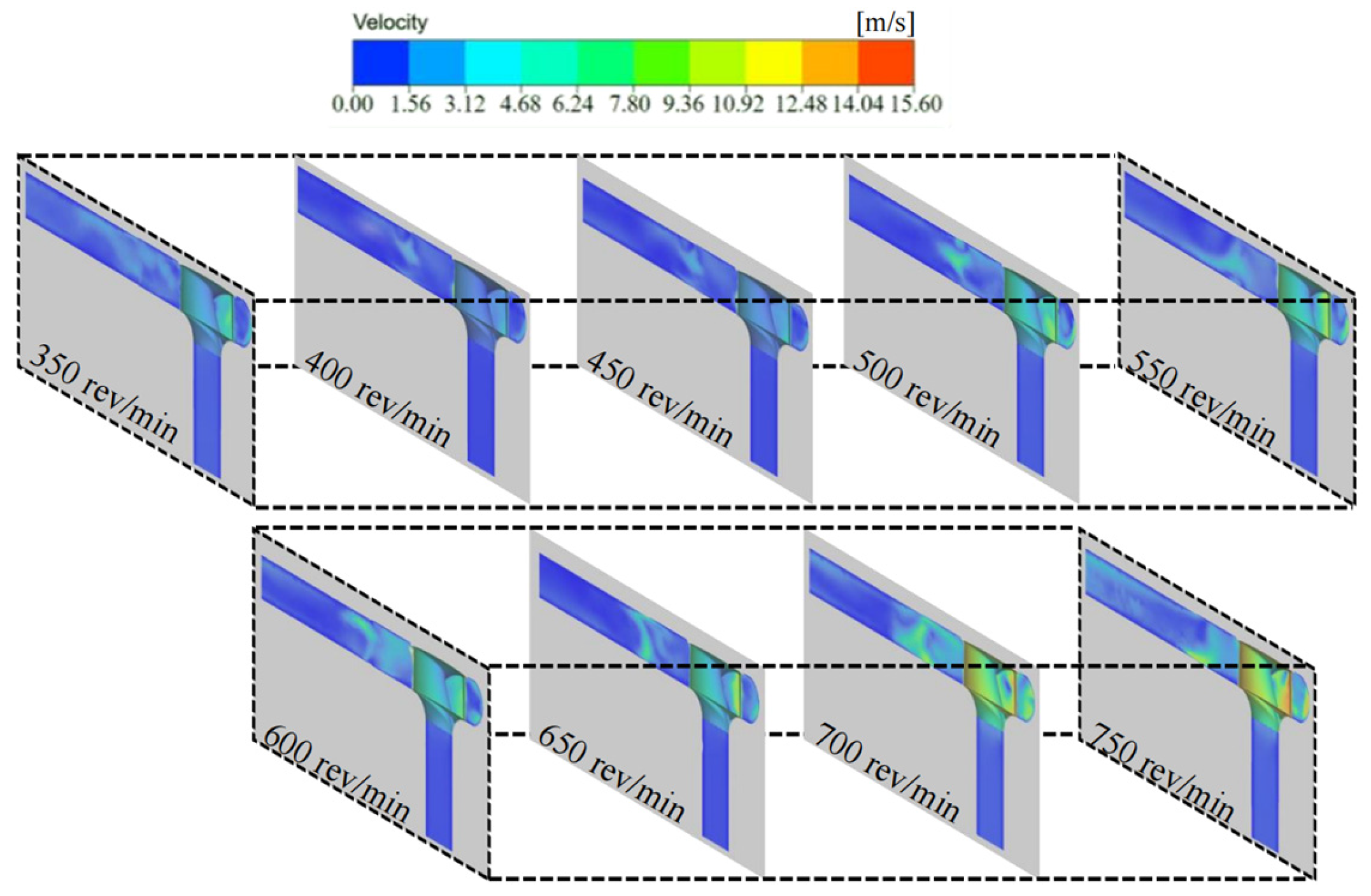
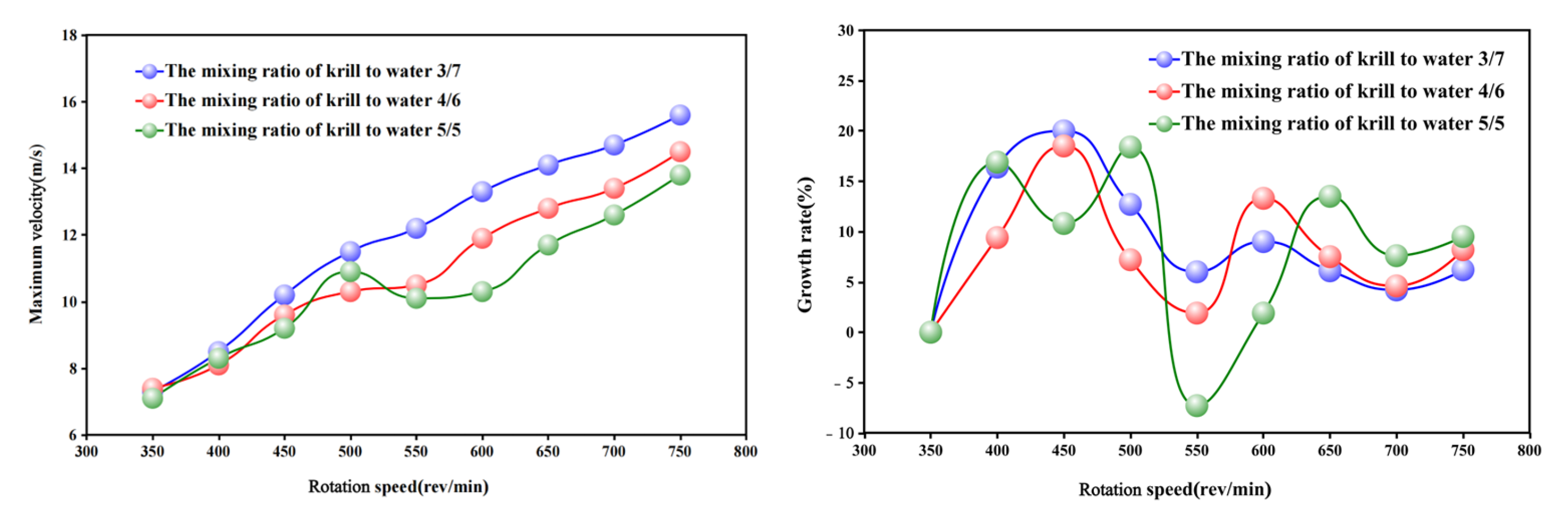
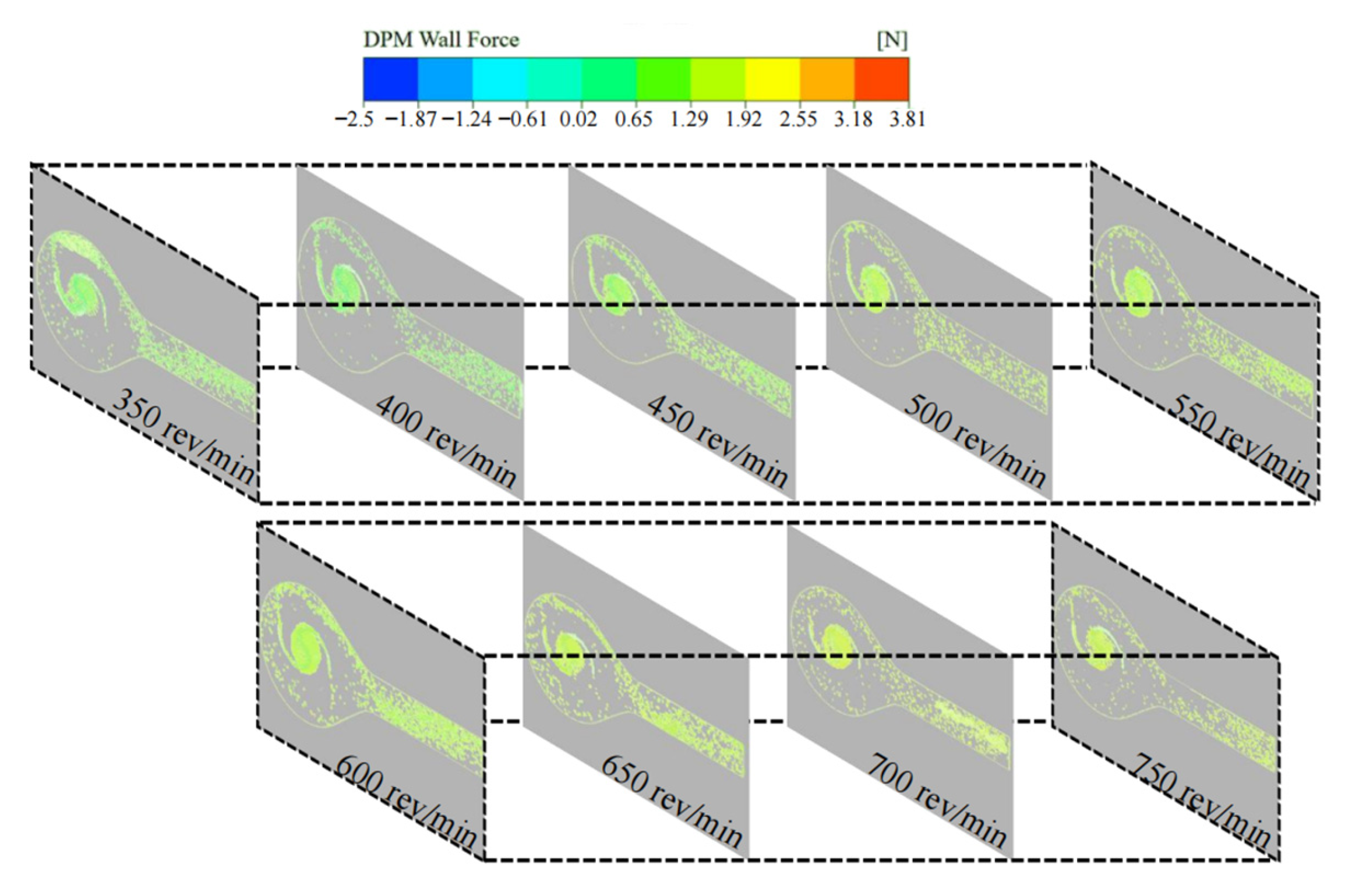
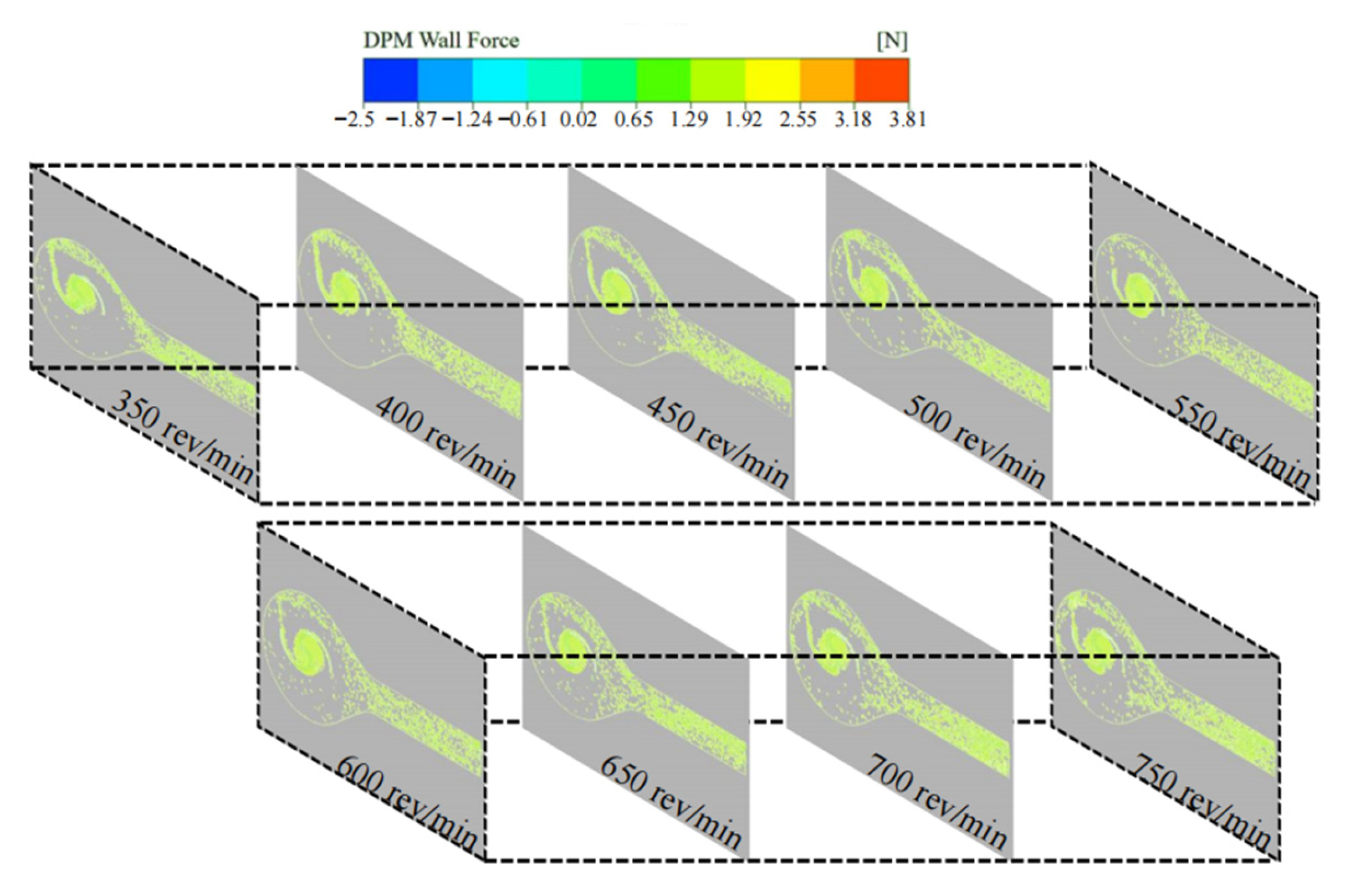
3. Results
4. Conclusions
- (1)
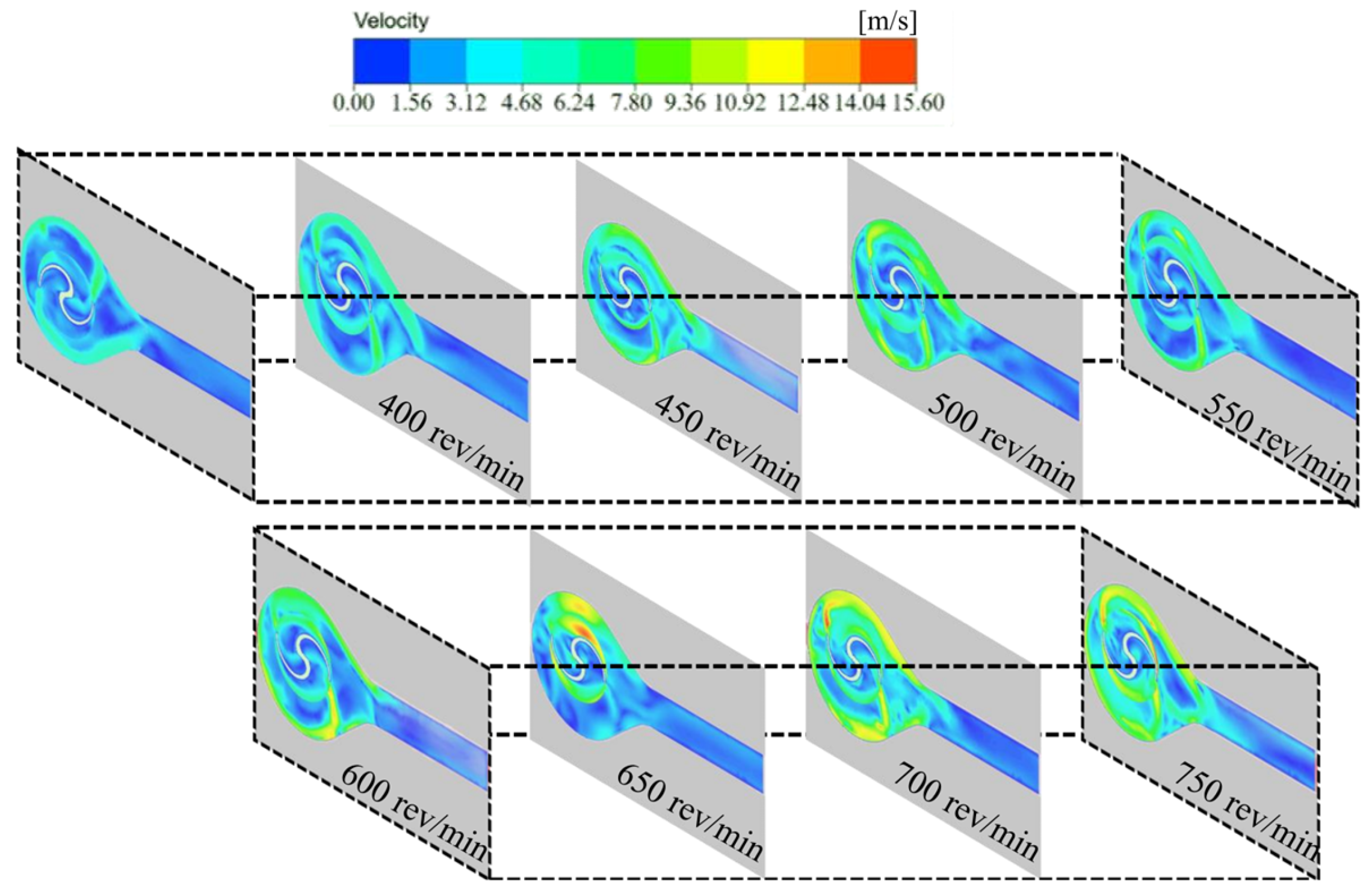
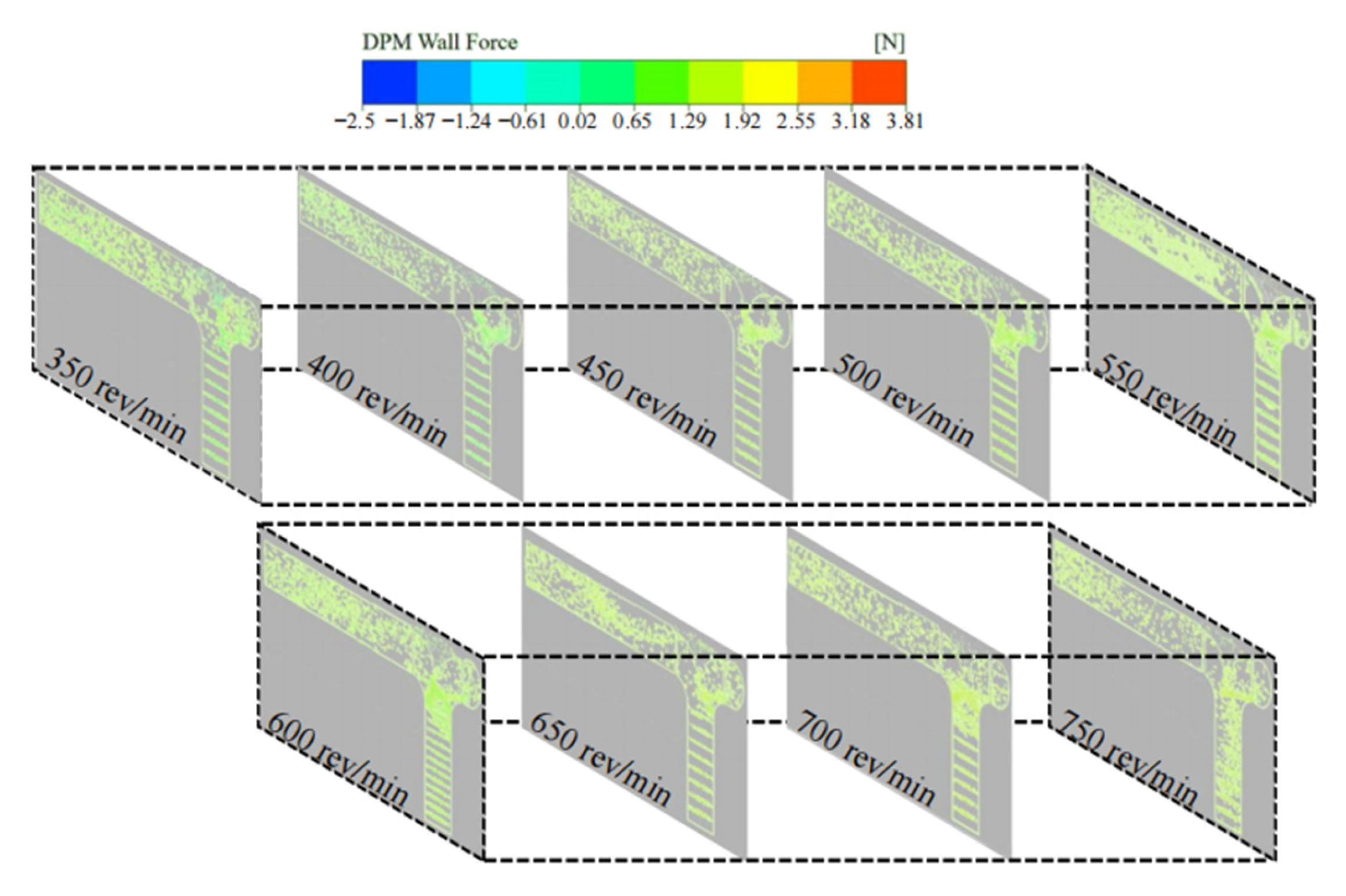
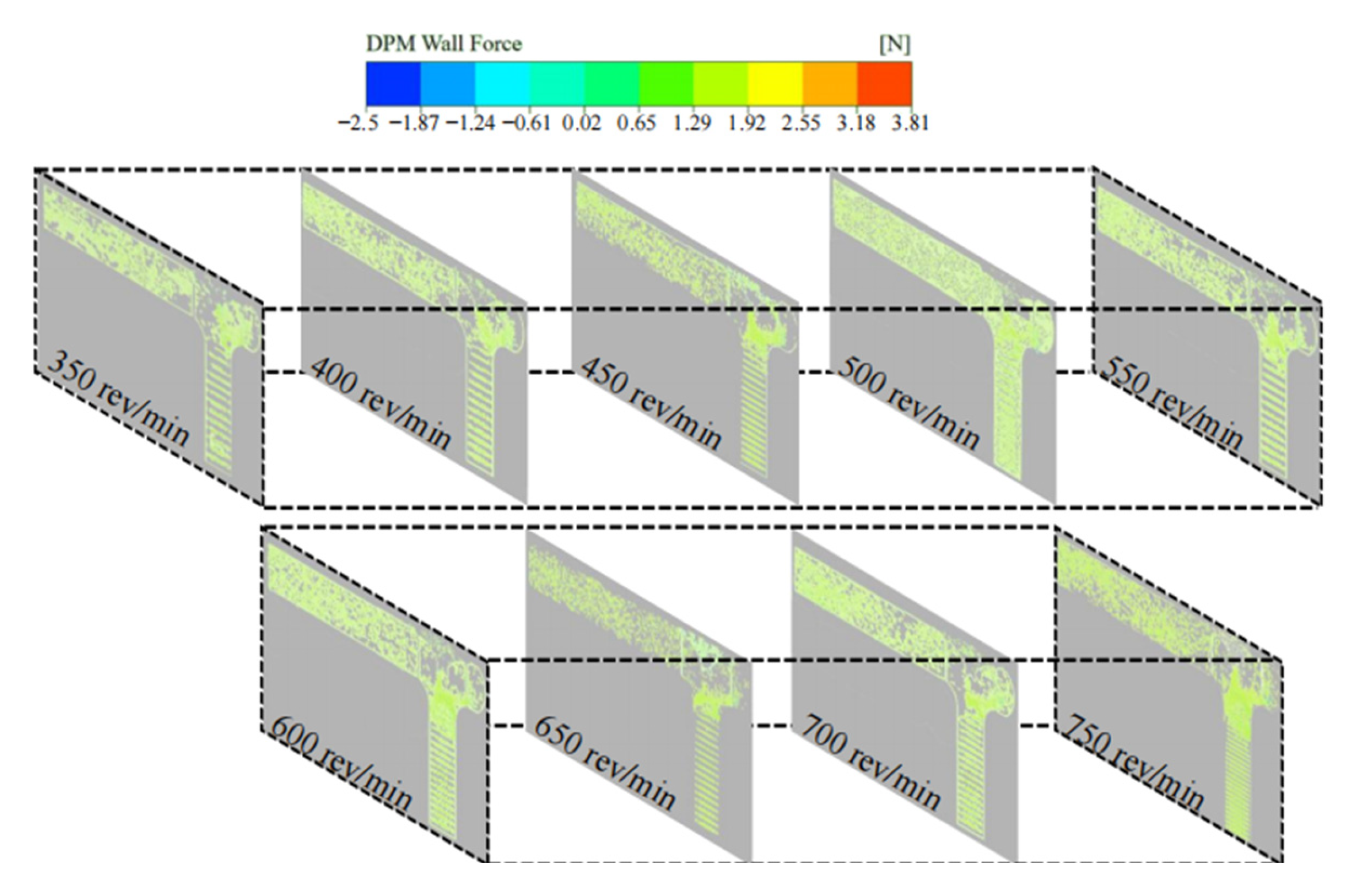
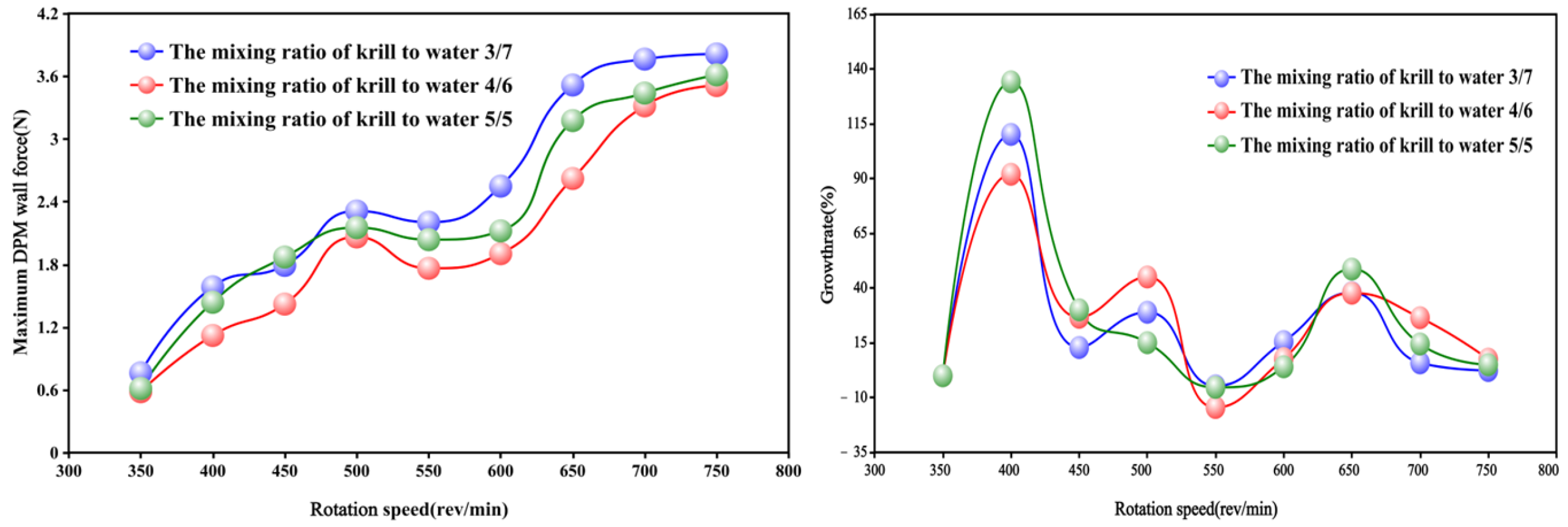
- The simulation study demonstrated that when the centrifuge rotation speed was within the range of 550–600 rev/min, the collision between the krill body and the pumping machine wall, as well as the krill body itself, was significantly reduced. This result indicated that this condition resulted in the lowest damage rate. Within this specific range of rotation speeds, the growth rate of the velocity gradient in the centrifugal region exhibits a minimum at each working condition, particularly at 550 rev/min. Additionally, the increase in the particle collision force on the krill body with an increasing rotation speed is marginal, suggesting that this range can effectively balance centrifugal separation efficiency and mechanical damage control.
- (2)
- The mixing ratio of krill–water is 4:6, and the centrifuge flow characteristics and krill density are selected to reach the optimal match. This process ensures that the krill body is minimised by the particle collision force and internal extrusion pressure. In this scenario, the propulsion exerted by the water flow on the krill body is found to be less significant than in the 3/7 condition. This, in turn, results in a decrease in the frequency of collisions between the krill body and the inner wall surface of the pumping apparatus. Moreover, in contrast to the 5/5 condition, the decline in krill body density serves to reduce extrusion stress between the groups, thereby conferring a dual benefit in the form of damage inhibition.
- (3)
- Through the combined analysis of the interaction effect of rotational speed and mixing ratio, it was determined that a combination of 550–600 rev/min and a 4/6 mixing ratio could optimise the velocity distribution of the flow field and the mechanical response of the krill body concurrently. In this condition, the maximum velocity in the centrifuge and the particle collision force on the krill body were both significantly reduced, and the uniformity of the distribution of the particle collision force was improved. In addition, the damage to the krill body was significantly reduced in comparison with the other 25 groups of conditions. This provides a basis for the selection of krill suction pump parameters.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Murphy, E.J.; Cavanagh, R.D.; Drinkwater, K.F.; Grant, S.M.; Heymans, J.J.; Hofmann, E.E.; Hunt, G.L., Jr.; Johnston, N.M. Understanding the structure and functioning of polar pelagic ecosystems to predict the impacts of change. Proc. R. Soc. B 2016, 283, 20161646. [Google Scholar] [CrossRef] [PubMed]
- Trathan, P.N.; Hill, S.L. The Importance of Krill Predation in the Southern Ocean. In Biology and Ecology of Antarctic Krill; Springer: Cham, Switzerland, 2016; pp. 321–350. [Google Scholar]
- Cavan, E.L.; Belcher, A.; Atkinson, A.; Hill, S.L.; Kawaguchi, S.; McCormack, S.; Meyer, B.; Nicol, S.; Ratnarajah, L.; Schmidt, K.; et al. The importance of Antarctic krill in biogeochemical cycles. Nat. Commun. 2019, 10, 4742. [Google Scholar] [CrossRef] [PubMed]
- Schmidt, K.; Atkinson, A.; Steigenberger, S.; Fielding, S.; Lindsay, M.; Pond, D.W.; Tarling, G.; Klevjer, T.A.; Allen, C.S.; Nicol, S.; et al. Seabed foraging by Antarctic krill: Implications for stock assessment, bentho-pelagic coupling, and the vertical transfer of iron. Limnol. Oceanogr. 2011, 56, 1411–1428. [Google Scholar] [CrossRef]
- Nicol, S.; Foster, J. The Fishery for Antarctic Krill: Its Current Status and Management Regime. In Biology and Ecology of Antarctic Krill; Springer: Cham, Switzerland, 2016; pp. 387–421. [Google Scholar]
- Xie, D.; Gong, M.; Wei, W.; Jin, J.; Wang, X.; Wang, X.; Jin, Q. Antarctic Krill (Euphausia superba) Oil: A Comprehensive Review of Chemical Composition, Extraction Technologies, Health Benefits, and Current Applications. Compr. Rev. Food Sci. Food Saf. 2019, 18, 514–534. [Google Scholar] [CrossRef] [PubMed]
- Yin, L.; Jiang, X.; Fan, Y.; Wang, J.; Xue, C.; Xue, Y. Preparation, Gel electrophoresis analysis, and nutritional evaluation of a functional krill protein concentrate with low fluoride level from Antarctic krill (Euphausia superba). J. Aquat. Food Prod. Technol. 2017, 26, 958–968. [Google Scholar] [CrossRef]
- Krafft, B.A.; Krag, L.A. Assessment of mortality of Antarctic krill (Euphausia superba) escaping from a trawl. Fish. Res. 2015, 170, 102–105. [Google Scholar] [CrossRef]
- Zhang, R.; Chen, X.; Luo, J. Knowledge Mining of Low Specific Speed Centrifugal Pump Impeller Based on Proper Orthogonal Decomposition Method. J. Therm. Sci. 2021, 30, 840–848. [Google Scholar] [CrossRef]
- Qian, H.; Mou, J.; Ren, Y.; Zhu, Z.; Liu, N.; Zheng, S.; Wu, D. Investigation of Self-Priming Process of a Centrifugal Pump with Double Blades. J. Therm. Sci. 2021, 30, 849–858. [Google Scholar] [CrossRef]
- Li, G.; Zhang, J.; Mao, J.; Yuan, S.; Jia, J. Numerical Investigation of the Transient Flow and Frequency Characteristic in a Centrifugal Pump with Splitter Blades. J. Therm. Sci. 2021, 30, 562–573. [Google Scholar] [CrossRef]
- Li, Q.; Li, S.; Wu, P.; Huang, B.; Wu, D. Investigation on Reduction of Pressure Fluctuation for a Double-Suction Centrifugal Pump. Chin. J. Mech. Eng. 2021, 34, 12. [Google Scholar] [CrossRef]
- Ou, J.; Jin, D.; Gui, X. Numerical Investigation of the Load Distribution between the Main Blade and the Splitter Blade in a High-Loading Numerical Investigation of the Load Distribution between the Main Blade and the Splitter Blade in a High-Loading Centrifugal Compressor. J. Therm. Sci. 2022, 31, 1682–1695. [Google Scholar] [CrossRef]
- Huang, M.; Liu, S.; Qiao, X.; Chen, P.; Liu, Z.; Zhang, X. Simulation study on the drag reduction characteristics of bionic microstructured blades for centrifugal pumps. Surf. Technol. 2023, 52, 196–205. [Google Scholar]
- Wu, S.; Zhang, Y.; Zhang, P. Simulation of transient startup process of centrifugal pump. J. Drain. Irrig. Mach. Eng. 2018, 36, 461–465. [Google Scholar]
- Sorguven, E.; Incir, S.; Highgate, J. Understanding loss generation mechanisms in a centrifugal pump using large eddy simulation. Int. J. Heat Fluid Flow 2022, 96, 108994. [Google Scholar] [CrossRef]
- Xue, X.; Dong, X.; Meng, F.; Li, Z. Numerical simulation analysis of internal flow field and external characteristics of electric submersible centrifugal pump. Comput. Simul. 2024, 41, 120–126. [Google Scholar]
- Wei, Y.; Yang, Y.; Zhou, L.; Jiang, L.; Shi, W.; Huang, G. Influence of Impeller Gap Drainage Width on the Performance of Low Specific Speed Centrifugal Pump. J. Mar. Sci. Eng. 2021, 9, 106. [Google Scholar] [CrossRef]
- Zhang, H.; You, H.; Lu, H.; Li, K.; Zhang, Z.; Jiang, L. CFD-Rotordynamics Sequential Coupling Simulation Approach for the Flow-Induced Vibration of Rotor System in Centrifugal Pump. Appl. Sci. 2020, 10, 1186. [Google Scholar] [CrossRef]
- Blais, B.; Bertrand, O.; Fradette, L.; Bertrand, F. CFD-DEM simulations of early turbulent solid-liquid mixing: Prediction of suspension curve and just-suspended speed. Chem. Eng. Res. Des. 2017, 123, 388–406. [Google Scholar] [CrossRef]
- Blais, B.; Bertrand, F. CFD-DEM investigation of viscous solid-liquid mixing: Impact of particle properties and mixer characteristics. Chem. Eng. Res. Des. 2017, 118, 270–285. [Google Scholar] [CrossRef]
- Blais, B.; Lassaigne, M.; Goniva, C.; Fradette, L.; Bertrand, F. Development of an unresolved CFD-DEM model for the flow of viscous suspensions and its application to solid-liquid mixing. J. Comput. Phys. 2016, 318, 201–221. [Google Scholar] [CrossRef]
- Satish, K.; Gandhi, B.K.; Mohapatra, S.K. Performance characteristics of centrifugal slurry pump with multi-sized particulate bottom and fly ash mixtures. Part. Sci. Technol. 2014, 32, 466–476. [Google Scholar]
- Huang, S.; Su, X.H.; Qiu, G.Q. Transient numerical simulation for solid–liquid flow in a centrifugal pump by DEM–CFD Coupling. Eng. Appl. Comput. Fluid Mech. 2015, 9, 411–418. [Google Scholar] [CrossRef]
- Beccati, N.; Ferrari, C.; Parma, M.; Semprini, M. Eulerian multi-phase CFD model for predicting the performance of a centrifugal dredge pump. Int. J. Comput. Methods Exp. Meas. 2019, 7, 316–326. [Google Scholar] [CrossRef]
- Dong, S.L.; Dong, Y.W.; Huang, L.Y.; Zhou, Y.; Cao, L.; Tian, X.L.; Han, L.M.; Li, D.H. Advancements and hurdles of deeper offshore aquaculture in China. Rev. Aquac. 2024, 16, 644–655. [Google Scholar] [CrossRef]
- Huang, Y. History, classification and outlook of fishery equipment in China. China Fish. 2024, 51–55. [Google Scholar]
- Xu, J.; Han, L.M.; Zhang, Y. Industrial characteristics of deep-sea aquaculture in China and its policy support. China Fish Econ 2021, 39, 98–107. [Google Scholar]
- Yu, J.; Wang, Y. Evolution of deep-sea fisheries policies in China: Review, performance and prospects. Fish. Manag. Ecol. 2023, 30, 109–120. [Google Scholar] [CrossRef]
- Guo, X.; Tan, F.C. Analysis of main structure type and application prospect of fish suction pump. Fish. Inf. Strategy 2013, 28, 214–218. [Google Scholar]
- Chu, S.; Chen, Z.; Tan, Y.; Liu, P. Research on three-dimensional solid modeling of double runner fish suction pump based on SolidWorks. Fish. Mod. 2018, 45, 55–60. [Google Scholar]
- Long, X.; Xu, M.; Wang, J.; Zou, J.; Ji, B. An experimental study of cavitation damage on tissue of Carassius auratus in a jet fish pump. Ocean Eng. 2019, 174, 43–50. [Google Scholar] [CrossRef]
- Sui, B.; Feng, Y.; Han, W. A Kind of Net Capsule Fish Suction Technology for Large Pelagic Trawlers. zl201210548872. 2, 10 July 2013. [Google Scholar]
- Fang, X.; Mao, W.; Shen, J.; Xu, Z.; Chen, Z.; Wang, Z. Research design and exploration of an Antarctic krill suction pump. Electromech. Equip. 2019, 36, 63–66. [Google Scholar]
- Liu, P.; Jiao, E.; Lin, L. Performance analysis of fish suction pumps with different forms of worm casings based on fluid analysis. Mar. Eng. 2020, 42, 72–76. [Google Scholar]
- Matsusaka, Japan. Live Fish Transfer Pump [EB/OL]. Available online: http://www.matsusakaltd.co.jp/product.htm (accessed on 10 February 2025).
- Mcnabb, C.D.; Liston, C.R.; Borthwick, S.M. Passage of juvenile chinook salmon and other fish species through archimedes lifts and a hydrostat pump at Red Bluff California. Trans. Am. Fish. Soc. 2003, 132, 326–334. [Google Scholar] [CrossRef]
- Inventive Marine Products Limited of Canada. CanaVac Fish Pumps [EB/OL]. Available online: https://inventivemarine.com/canavac-fish-pumps (accessed on 18 May 2025).
- VAKI. Aquaculture Systems Limited of Iceland: Heathro Fish Pump [EB/OL]. Available online: http://www.seaquest.ie (accessed on 25 January 2025).
- Zhang, F. Fluid Mechanics; China Water & Power Press: Beijing, China, 2013. [Google Scholar]
- Wang, H. My Understanding of Fluid Mechanics; National Defense Industry Press: Beijing, China, 2014. [Google Scholar]
- Schetz, J.A.; Fuhs, A.E. Fundamentals of Fluid Mechanics; John Wiley & Sons: Hoboken, NJ, USA, 1999. [Google Scholar]
- Yang, W.; Jin, X.; Gu, M. Research and application of balanced atmospheric boundary layer in numerical simulation of wind engineering. J. Civ. Eng. 2007, 40, 1–5. [Google Scholar]
- Kawaguchi, S.; Nicol, S. Learning about Antarctic Krill from the Fishery. Antarct. Sci. 2007, 19, 219–230. [Google Scholar] [CrossRef]
- Zhu, W.; Xiong, Z.; He, M. Numerical simulation and optimal design of the flow field in the cargo hold of an Antarctic krill ship. Int. Jt. Conf. Civ. Mar. Eng. 2023, 2023, 334–340. [Google Scholar] [CrossRef]
- Pagalthivarthi, K.V.; Gupta, P.K.; Tyagi, V.; Ravi, M.R. CFD Prediction of Erosion Wear in Centrifugal Slurry Pumps for Dilute Slurry Flows. Comput. Multiph. Flows 2011, 50, 52–56. [Google Scholar] [CrossRef]
- Zhang, D.; Mei, J.; Zhao, R.; Huang, J.; Jin, Y. Optimized design of fluoroplastic two-phase flow pump based on response surface method. J. Irrig. Drain. Mach. Eng. 2020, 38, 898–903+921. [Google Scholar]
- Li, B.; Wang, X.; Li, C.; Liu, H.; Ding, K.; Tan, M. Trajectory and distribution of large particles in a slurry transfer pump. Min. Res. Dev. 2020, 40, 138–144. [Google Scholar]
- Shen, Z.J.; Li, R.N.; Han, W.; Zhao, W.G.; Wang, X.H. The research on particle trajectory of solid-liquid two-phase flow and erosion predicting in screw centrifugal pump. IOP Conf. Ser. Mater. Sci. Eng. 2016, 129, 012052. [Google Scholar] [CrossRef]
- Huang, L.X.; Kumar, K.; Mujumdar, A.S. A comparative study of a spray dryer with rotary disc atomizer and pressure nozzle using computational fluid dynamic simulations. Chem. Eng. Process. Process Intensif. 2006, 45, 461–470. [Google Scholar] [CrossRef]
- Liu, P.; Xu, Z.; Xu, Z. Design of impeller of centrifugal fish suction pump. Fluid Mach. 2016, 50–54. [Google Scholar]
- Liang, J.C. Design and Research of a Fish Suction Pump for an Antarctic Krill Fishing Vessel. Ph.D. Thesis, Dalian Ocean University, Dalian, China, 2022. [Google Scholar]

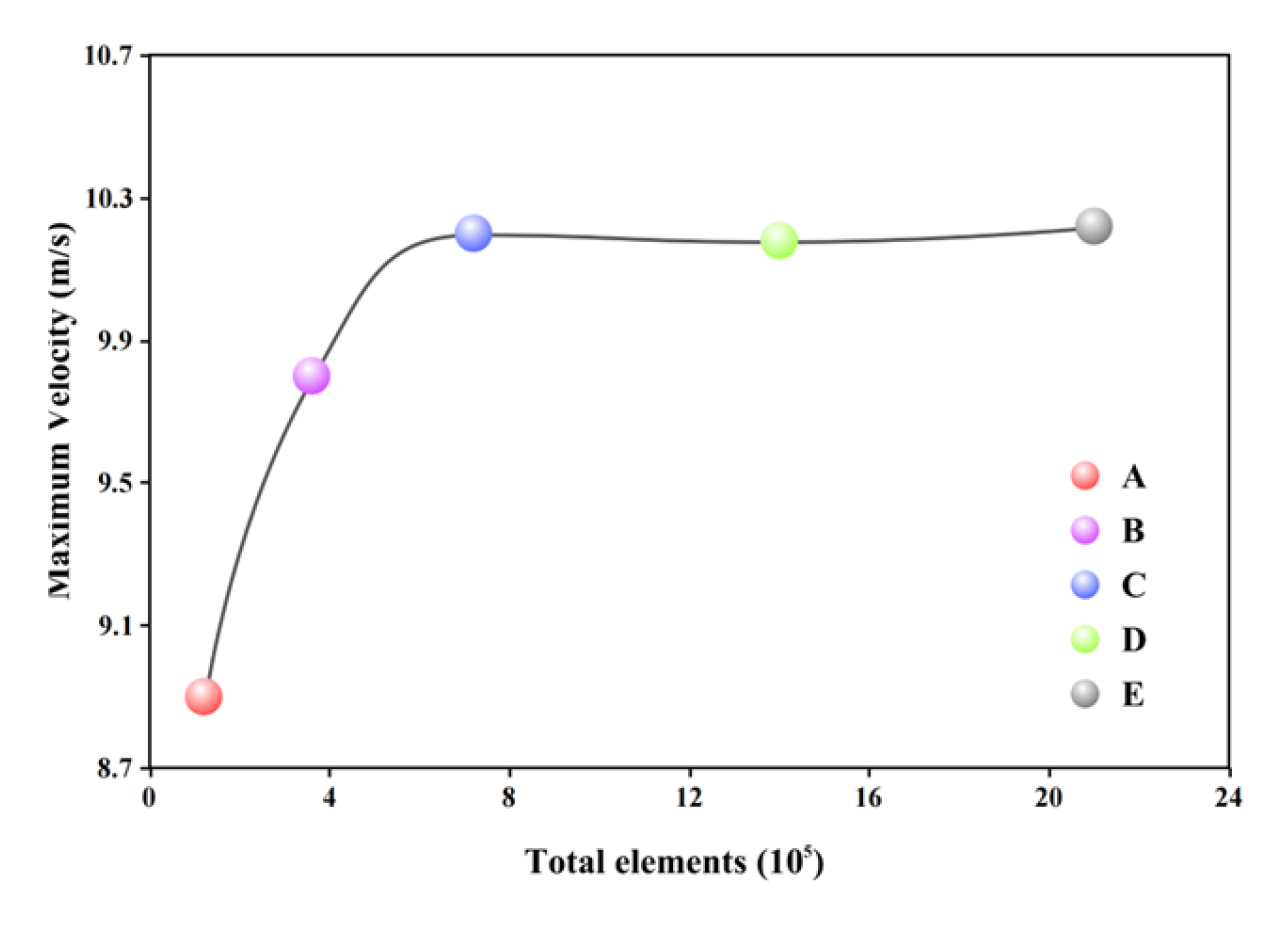


| Mesh Type | Total Elements | Maximum Velocity/m s−1 |
|---|---|---|
| A | 1.2 × 105 | 8.9 |
| B | 3.6 × 105 | 9.8 |
| C | 7.2 × 105 | 10.20 |
| D | 1.4 × 106 | 10.18 |
| E | 2.1 × 106 | 10.22 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, P.; Lin, L.; Xu, Z. Characterisation of the Pump-Suction Flow Field of Antarctic Krill and Key Influencing Factors. Appl. Sci. 2025, 15, 5836. https://doi.org/10.3390/app15115836
Liu P, Lin L, Xu Z. Characterisation of the Pump-Suction Flow Field of Antarctic Krill and Key Influencing Factors. Applied Sciences. 2025; 15(11):5836. https://doi.org/10.3390/app15115836
Chicago/Turabian StyleLiu, Ping, Liqun Lin, and Zhiqiang Xu. 2025. "Characterisation of the Pump-Suction Flow Field of Antarctic Krill and Key Influencing Factors" Applied Sciences 15, no. 11: 5836. https://doi.org/10.3390/app15115836
APA StyleLiu, P., Lin, L., & Xu, Z. (2025). Characterisation of the Pump-Suction Flow Field of Antarctic Krill and Key Influencing Factors. Applied Sciences, 15(11), 5836. https://doi.org/10.3390/app15115836





