Influence of Steel Oxidation on the Behavior of the Wheel–Rail Electrical Contact in Dynamic Conditions
Abstract
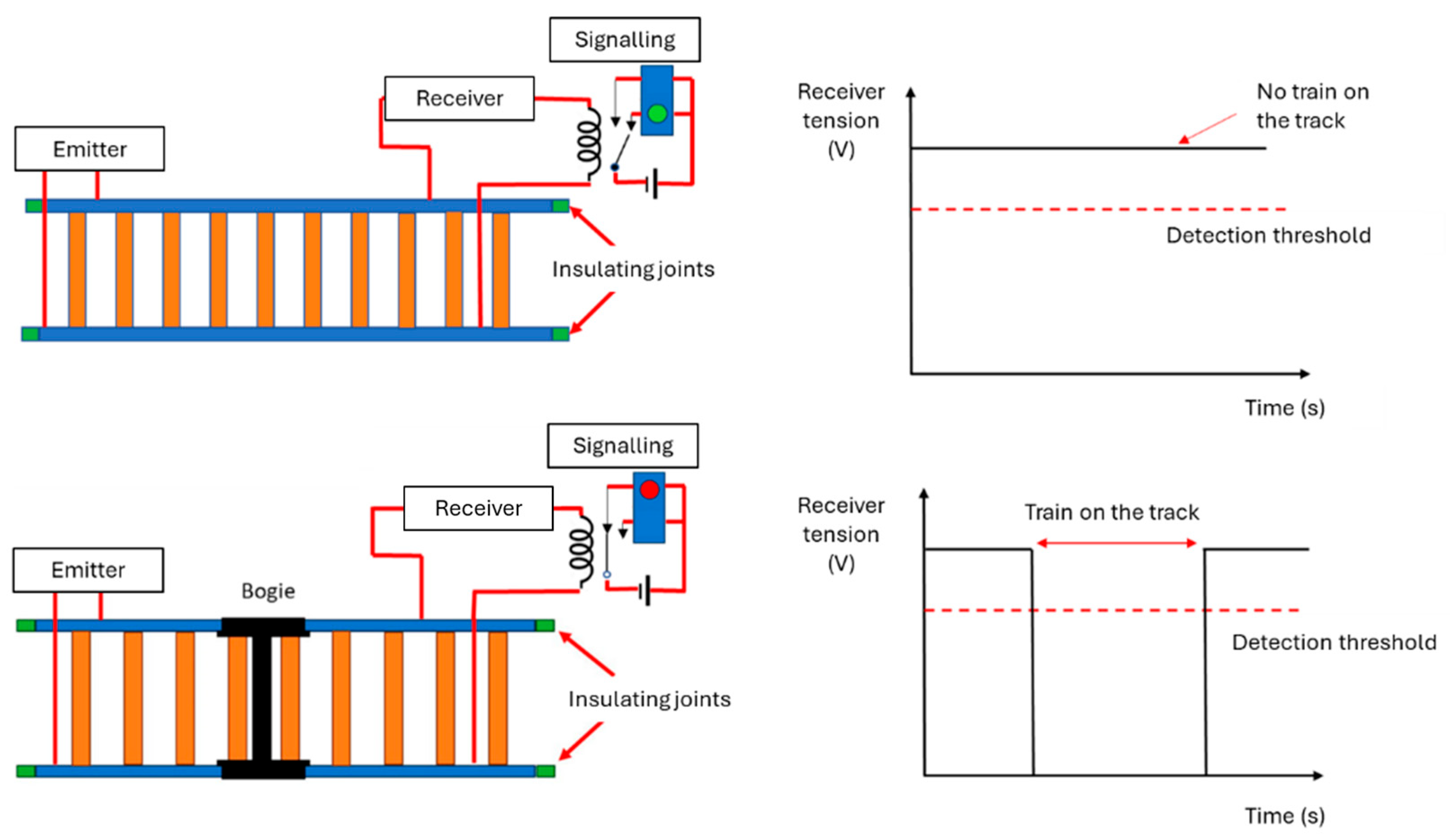
1. Introduction
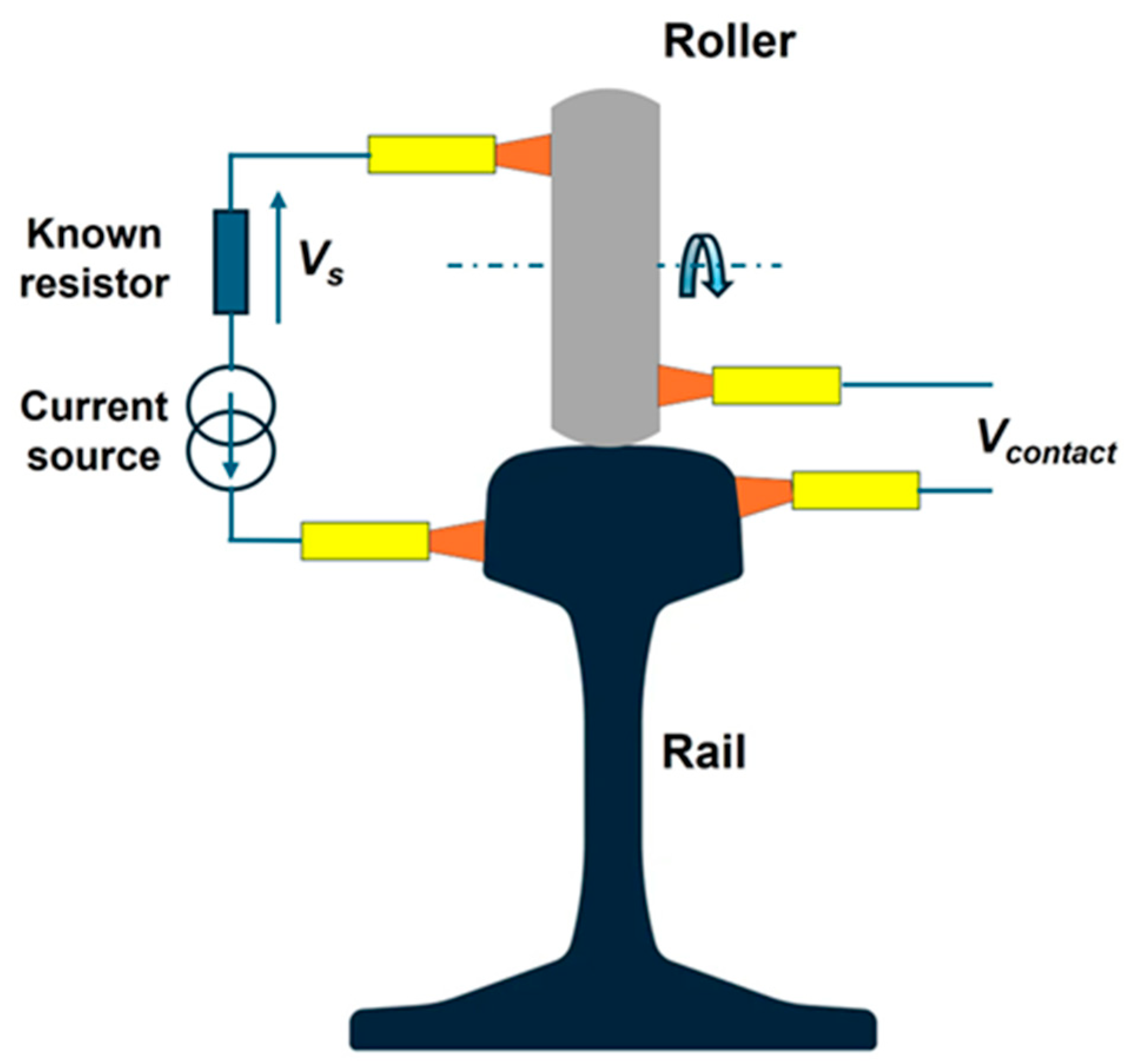
2. Experimental Setup and Tests Conditions
3. Analytical Methods
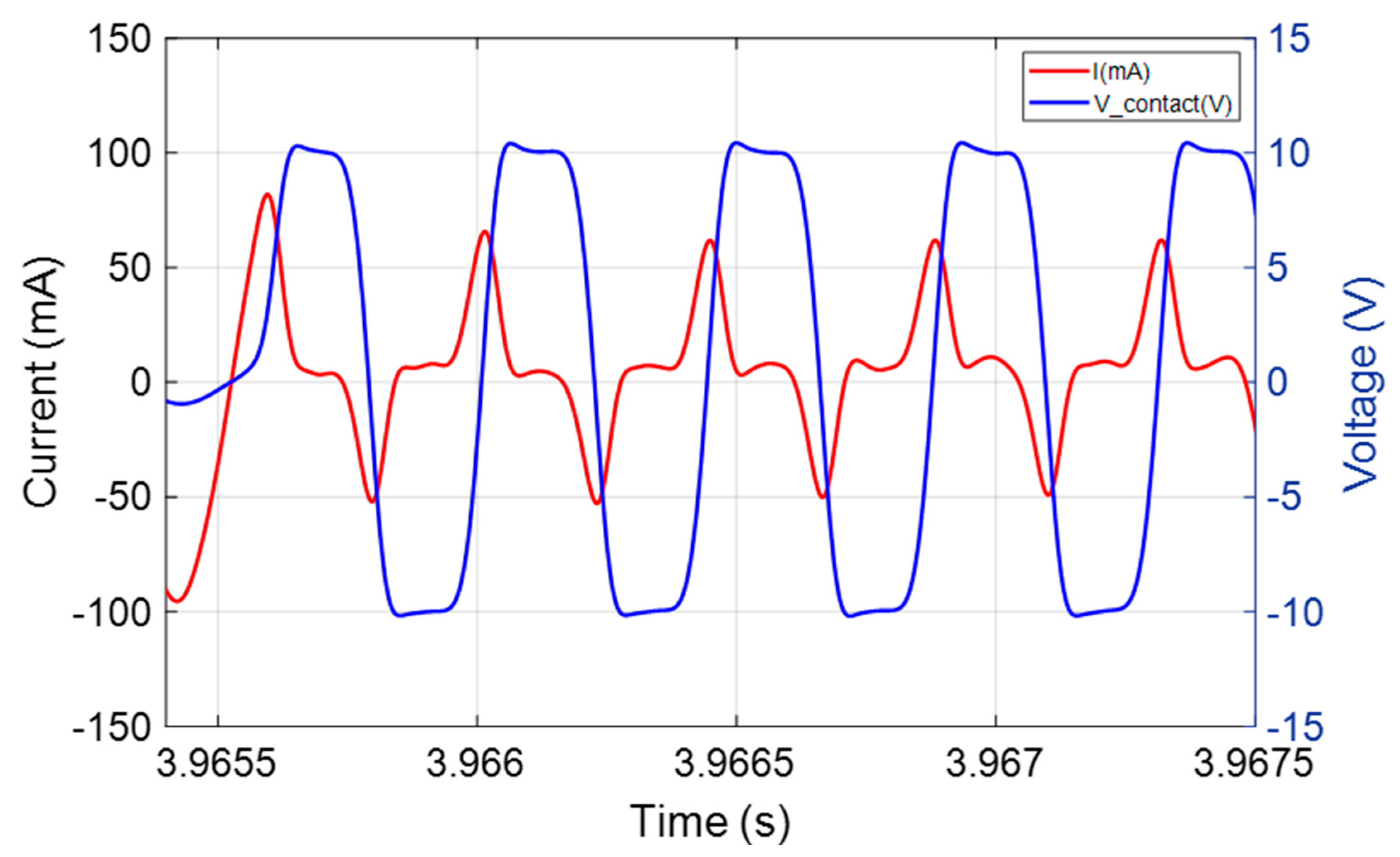
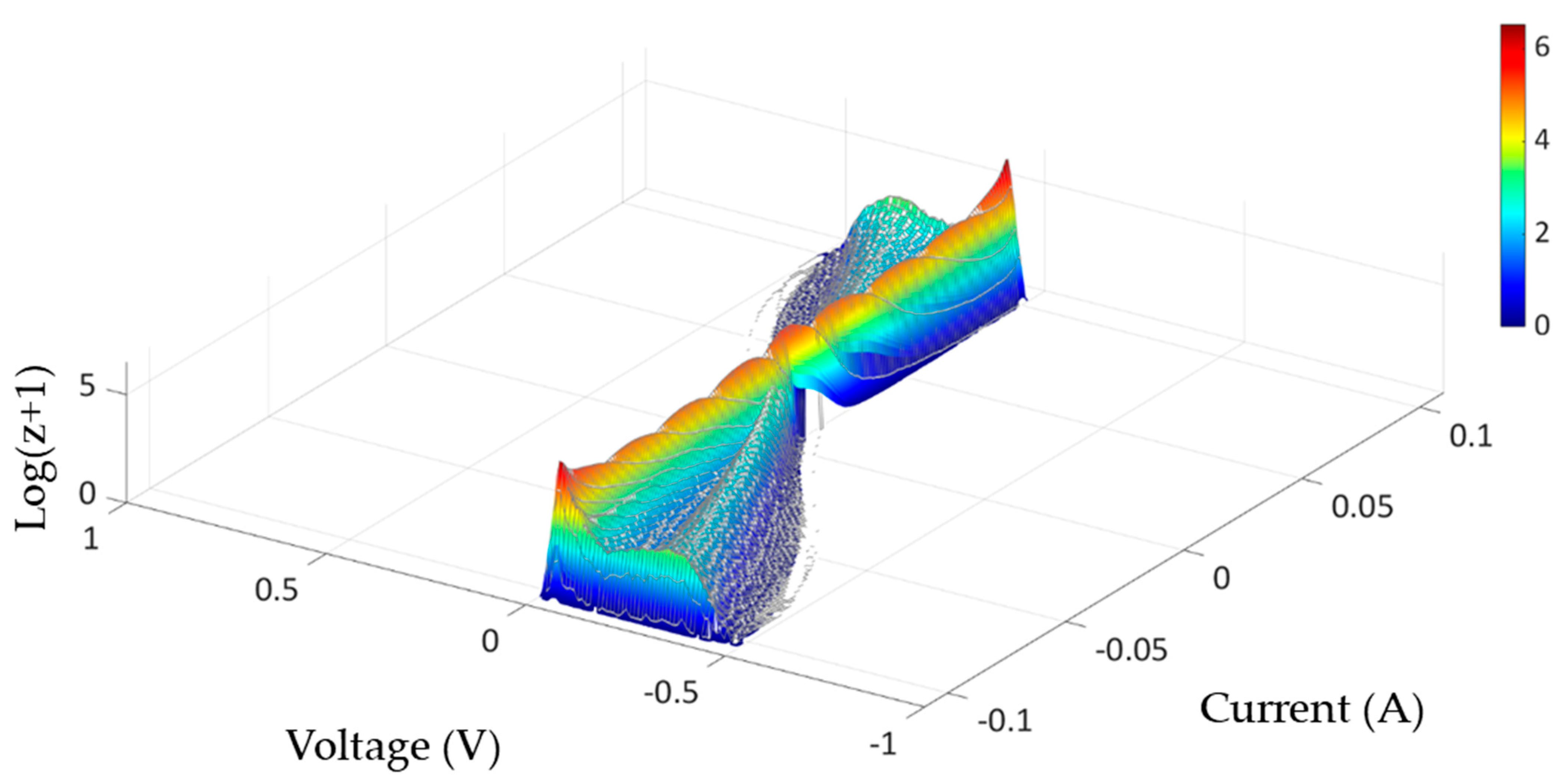
3.1. Statistical Representation of Voltage/Current Characteristics
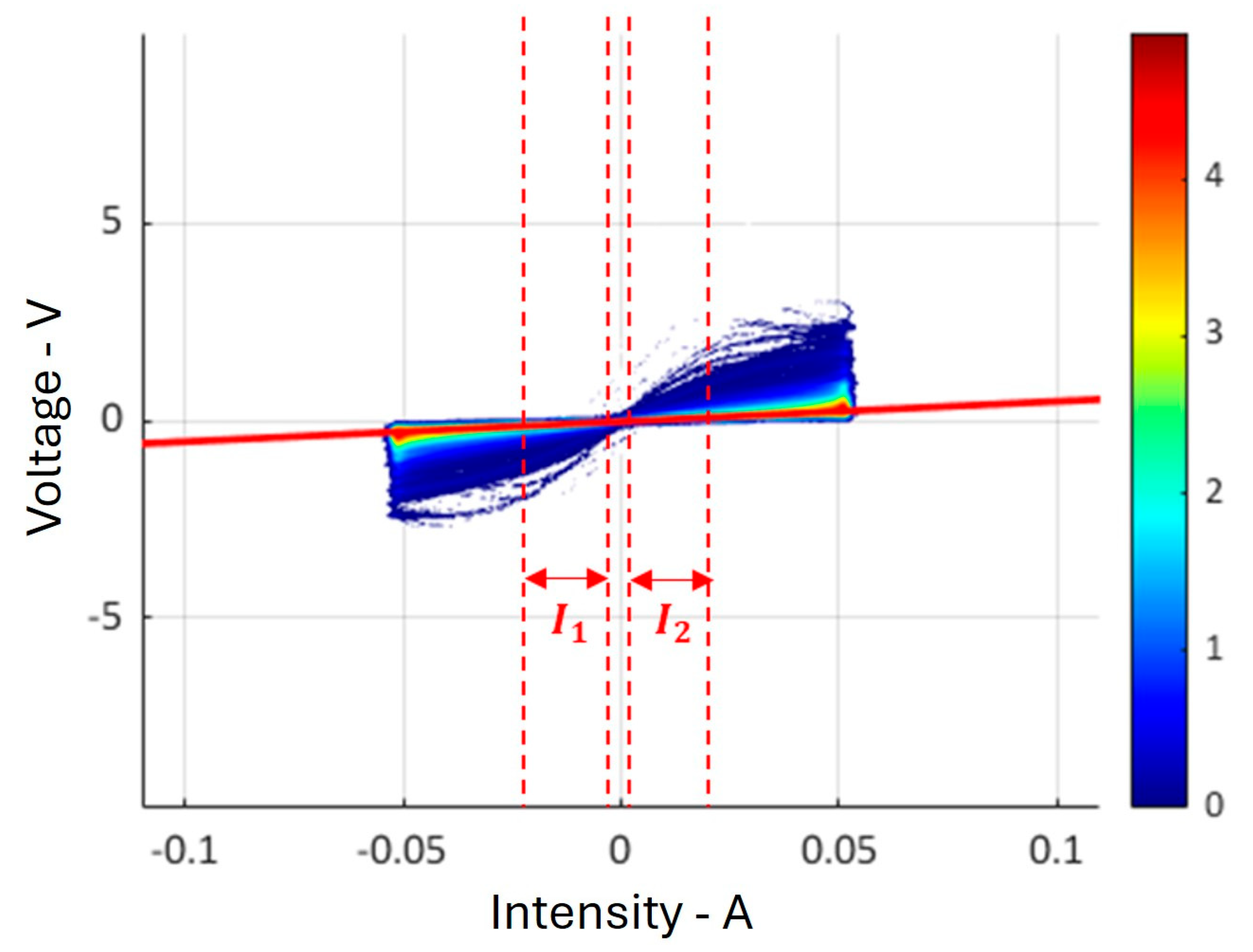
3.2. Estimation of ‘Main Resistance’ from U-I Data
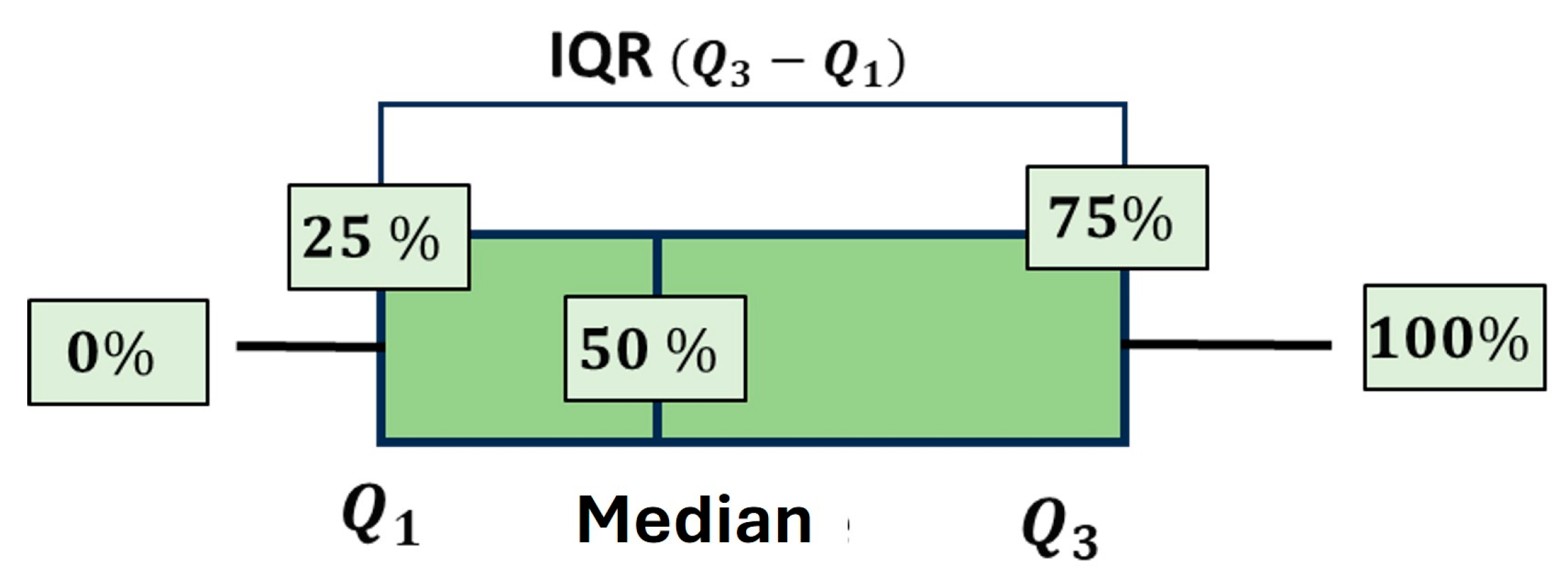
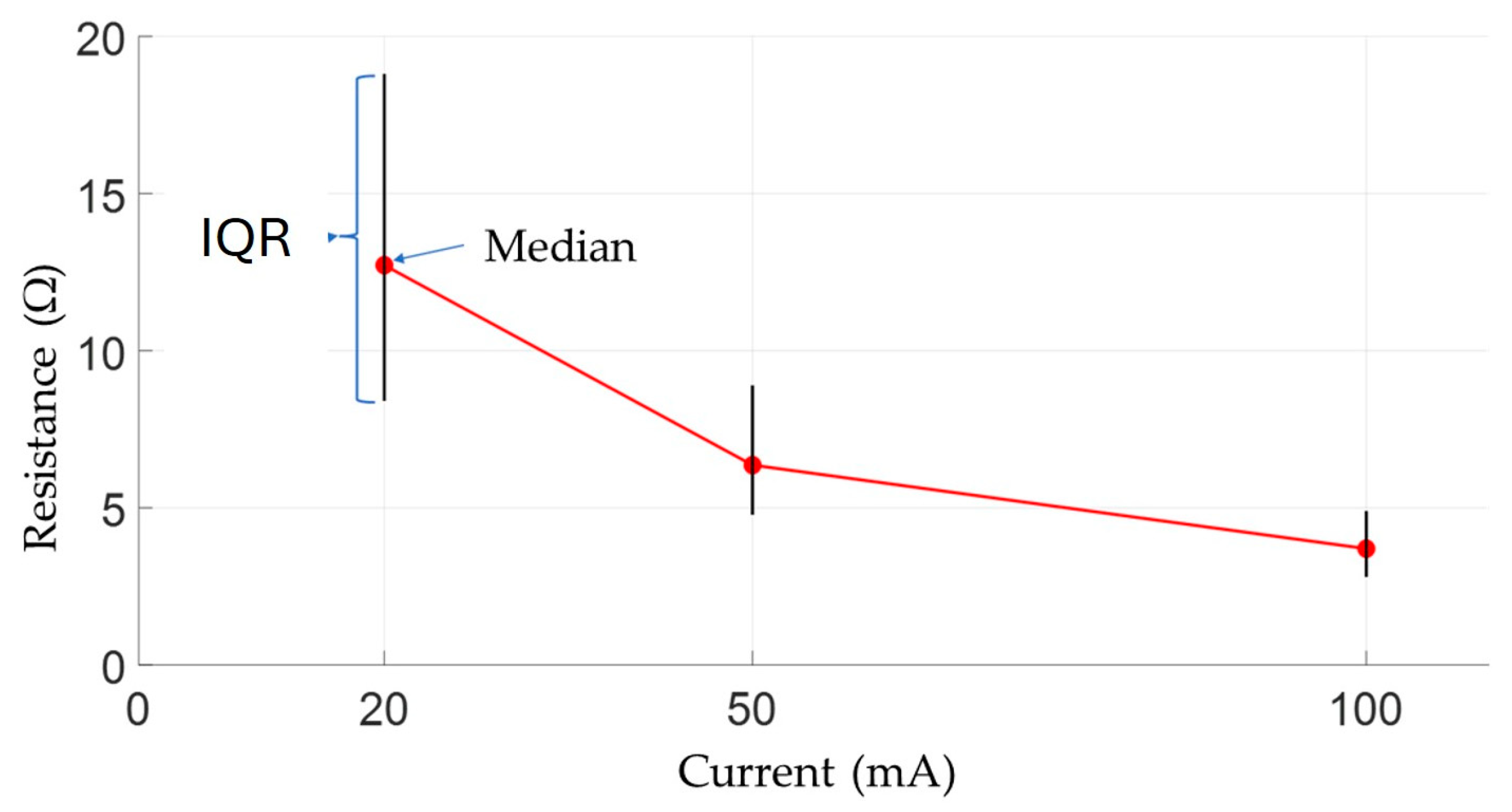
3.3. Statistical Analysis of Instantaneous Resistances
4. Dynamic Testing
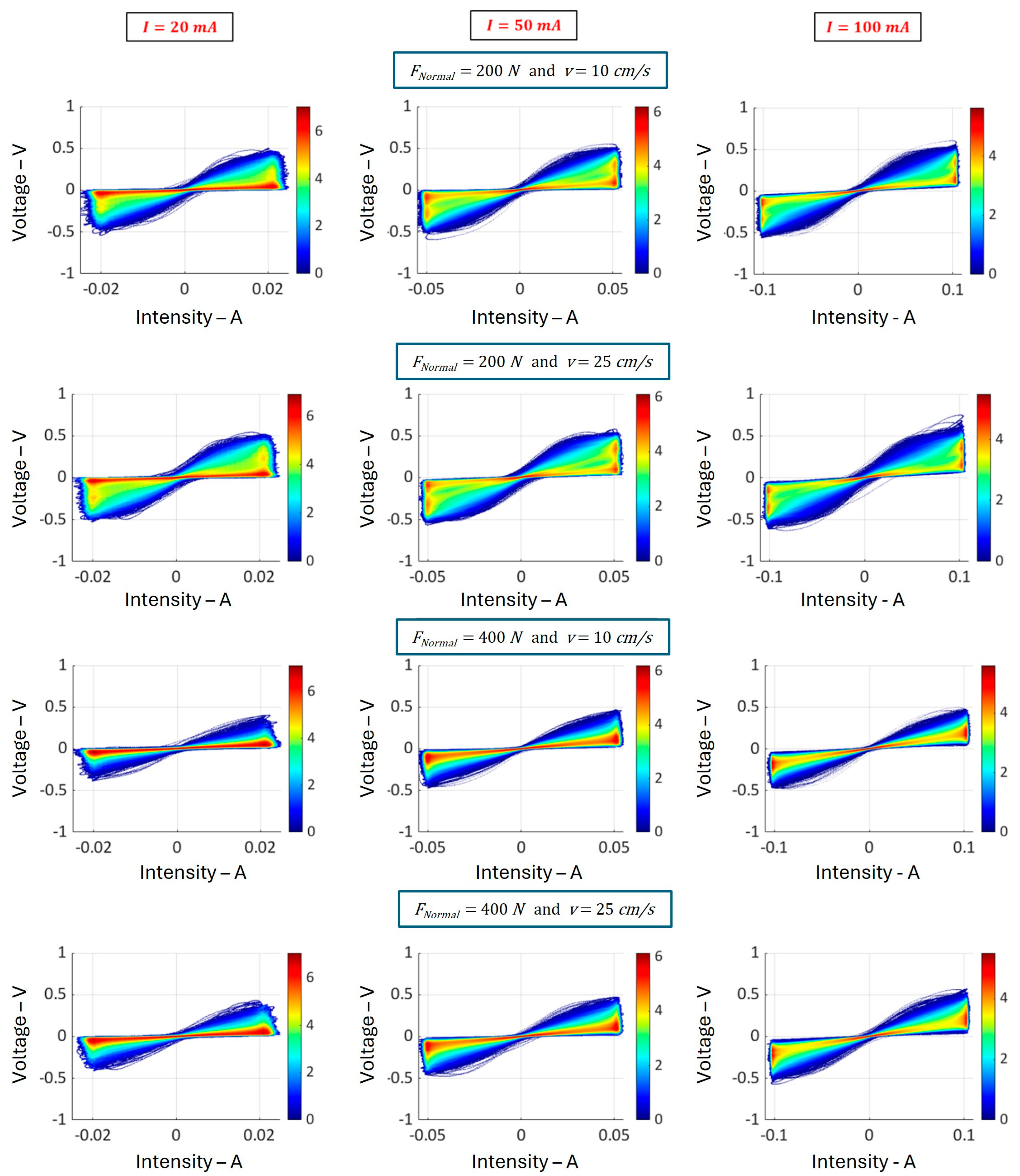
4.1. Clean Rail
4.1.1. Clean Rail: Statistical Mapping of the U-I Characteristics
4.1.2. Clean Rail: Statistical Analysis of Instantaneous Resistances
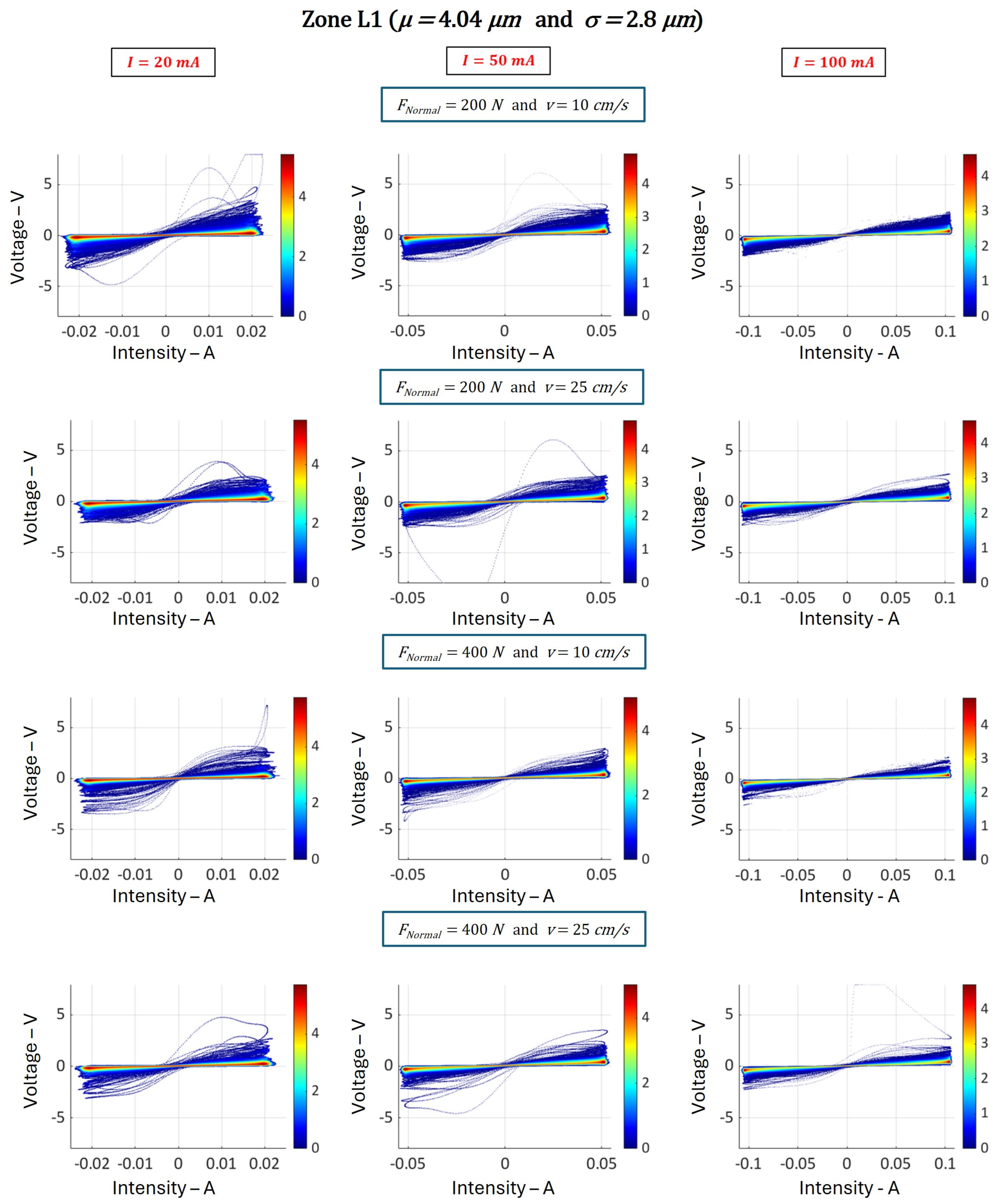
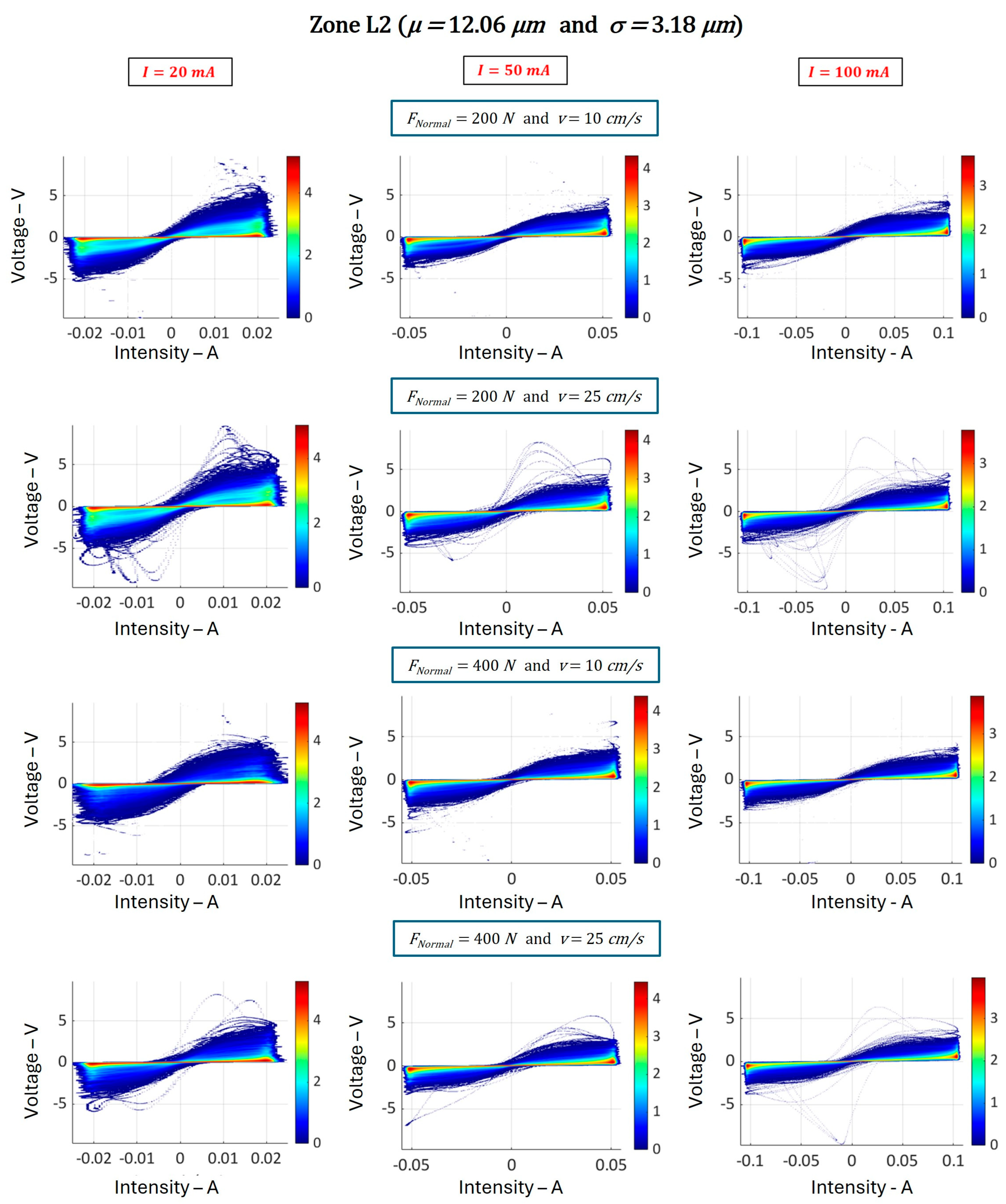
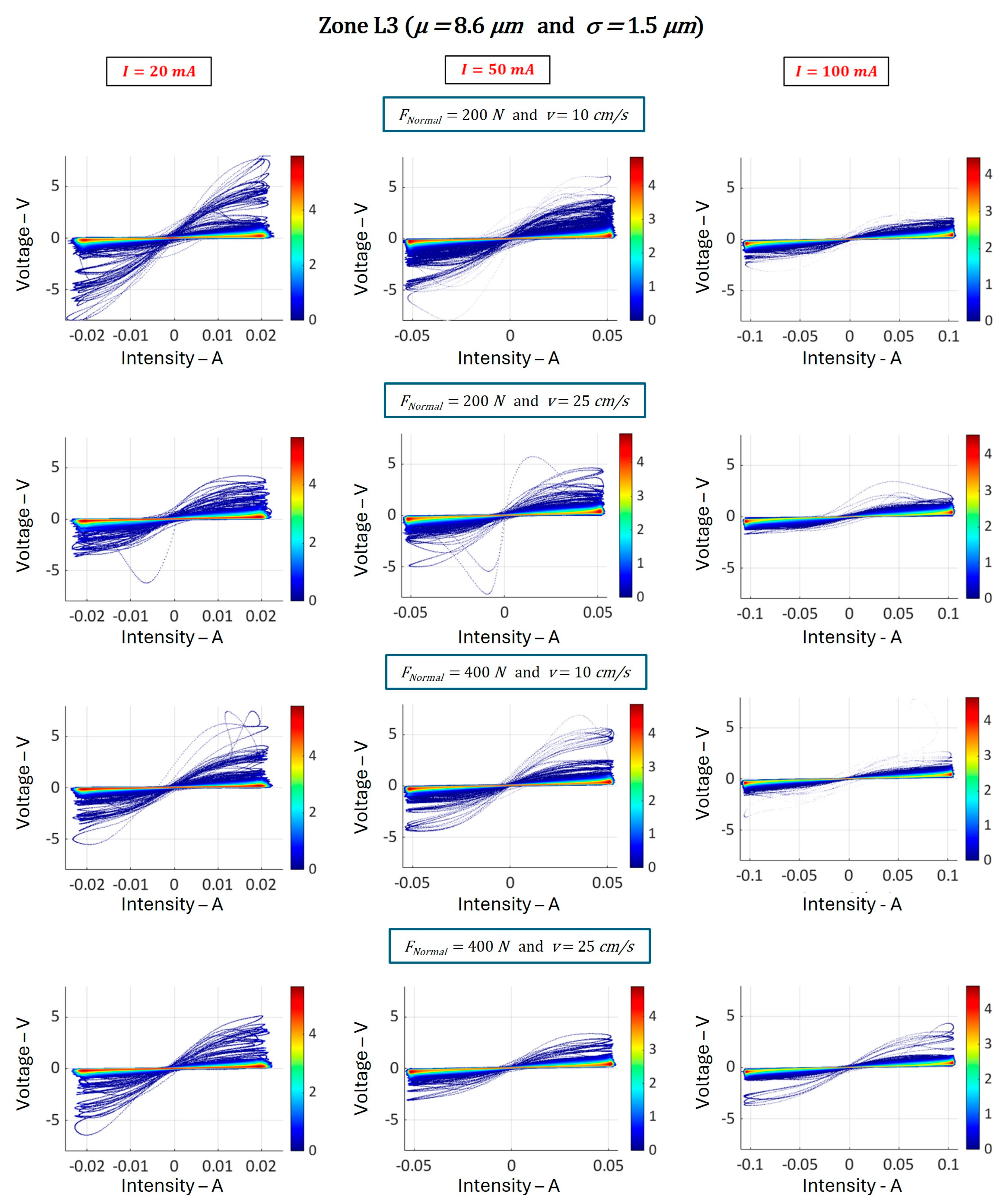
4.2. Light Oxidation
4.2.1. Light Oxidation: Statistical Mapping of the U-I Characteristics
- The effect of current is noticeable:
- (i)
- This trend is reflected in a decrease in the maximum voltages reached as the current increases.
- (ii)
- Increasing current also tends to reduce the dispersion of characteristics.
- However, the effects of force and speed are less obvious to evidence from these data.
4.2.2. Lignt Oxydation: Statistical Analysis of Instantaneous Resistances
- Effect of oxide thickness: Resistance tends to increase with oxide thickness. In particular, zone L2, which has a thicker oxide layer than the other two zones, shows particularly high resistance values, up to around , especially for low currents (). On the other hand, zones L1 and L3, with thinner oxide layers, show resistances relatively close to each other under all test conditions, and well below those recorded for zone L2.
- Current effect:
- (i)
- Resistance tends to decrease with the increasing current intensity, irrespective of oxide thickness and test conditions. The median decreases with the increasing intensity, a trend also observed in statistical maps. In zones L1 and L3, resistance decreases gradually from around at to approximately at . In zone L2, resistance is reduced from at to around at .
- (ii)
- Dispersion of the voltage–current characteristics is reduced with the increasing current. This is also observed in the statistical maps.
- (iii)
- As the current intensity increases, the effect of force and speed on contact resistance becomes less pronounced.
- Effect of force: as force increases, the contact resistance tends to decrease and the voltage–current characteristics are less dispersed. This can be attributed to an increase in the number of asperities coming into contact and a widening of the contact zones, mainly due to the flattening of asperities under the effect of pressure.
- Effect of speed: as speed increases, the contact resistance tends to rise and the voltage–current characteristics become more dispersed. In fact, increasing speed leads to accelerated renewal of the contact surface, thus reducing the number and size of asperities involved.
5. Conclusions
- -
- Force: as force increases, the resistance and dispersion of the voltage–current characteristics tend to decrease.
- -
- Speed: as speed increases, the resistance and dispersion of the voltage–current characteristics tend to increase.
- -
- Current intensity: for lightly oxidized rail sections, as the current intensity increases, contact resistance and dispersion tend to decrease; for ‘clean’ rail sections, the effect of the current is less pronounced.
- -
- Oxidation: contact resistance tends to increase with oxide thickness. This was expected, but it is important to note that it can also be affected by the temperature of the contact point, which can dramatically increase due to the current flowing through small contact spot.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Haydar, L.; Loete, F.; Houzé, F.; Slimani, K.; Guiche, F.; Testé, P. Influence of the oxide layer thickness on the behavior of the electrical wheel-rail contact in static conditions. Appl. Sci. 2025, 15, 471. [Google Scholar] [CrossRef]
- Na, L.; Cai, B.; Zhang, C.; Liu, J.; Li, Z. A heterogeneous transfer learning method for fault prediction of railway track circuit. Eng. Appl. Artif. Intell. 2025, 140, 109740. [Google Scholar] [CrossRef]
- Peng, F.; Liu, T. Method for Fault Diagnosis of Track Circuits Based on a Time–Frequency Intelligent Network. Electronics 2024, 13, 859. [Google Scholar] [CrossRef]
- Mosleh, A.; Montenegro, P.A.; Costa, P.A.; Calçada, R. Railway Vehicle Wheel Flat Detection with Multiple Records Using Spectral Kurtosis Analysis. Appl. Sci. 2021, 11, 4002. [Google Scholar] [CrossRef]
- Mosleh, A.; Montenegro, P.; Alves Costa, P.; Calçada, R. An approach for wheel flat detection of railway train wheels using envelope spectrum analysis. Struct. Infrastruct. Eng. 2021, 17, 1710–1729. [Google Scholar] [CrossRef]
- Wei, Y.; Han, J.; Yang, T.; Wu, Y.; Chen, Z. Research on wheel/rail contact surface temperature and damage characteristics during sliding contact of a wheel. J. Eng. Appl. Sci. 2024, 71, 201. [Google Scholar] [CrossRef]
- Li, J.; Zhang, Y.; Zhao, B.; Zheng, Z. Research and Analysis on Contact Resistance of Wheel and Insulated Rail Joint in High-Speed Railway Stations. Electronics 2023, 12, 1272. [Google Scholar] [CrossRef]
- Ren, W.; Zhang, C.; Sun, X. Electrical Contact Resistance of Contact Bodies with Cambered Surface. IEEE Access 2020, 8, 93857–93867. [Google Scholar] [CrossRef]
- Itagaki, M.; Nozue, R.; Watanabe, K.; Katayama, H.; Noda, K. Electrochemical impedance of thin rust film of low-alloy steels. Corros. Sci. 2004, 46, 1301–1310. [Google Scholar] [CrossRef]
- Al-Juboori, A.; Zhu, H.; Wexler, D.; Li, H.; Lu, C.; Azdiar; Gazder, A.; McLeod, J.; Pannila, S.; Barnes, J. Microstructural changes on railway track surfaces caused by electrical leakage between wheel and rail. Tribol. Int. 2019, 140, 105875. [Google Scholar] [CrossRef]
- Descartes, S.; Desrayaud, C.; Niccolini, E.; Berthier, Y. Presence and role of the third body in a wheel–rail contact. Wear 2005, 258, 1081–1090. [Google Scholar] [CrossRef]
- White, B.; Lewis, R.; Fletcher, D.; Harrison, T.; Hubbard, P.; Ward, C. Rail-wheel friction quantification and its variability under lab and field trial conditions. Proc. Inst. Mech. Eng. Part F J. Rail Rapid Transit 2024, 238, 569–579. [Google Scholar] [CrossRef]
- Broster, M.; Pritchard, C.; Smith, D.A. Wheel/rail adhesion: Its relation to rail contamination on british railways. Wear 1974, 29, 309–321. [Google Scholar] [CrossRef]
- Descartes, S.; Renouf, M.; Fillot, N.; Gautier, B.; Descamps, A.; Berthier, Y.; Demanche, P. A new mechanical-electrical approach to the wheel-rail contact. Wear 2008, 265, 1408–1416. [Google Scholar] [CrossRef]
- Xu, W.; Zhang, B.; Deng, Y.; Wang, Z.; Jiang, Q.; Yang, L.; Zhang, J. Corrosion of rail tracks and their protection. Corros. Rev. 2021, 39, 1–13. [Google Scholar] [CrossRef]
- Suzumura, J.; Sone, Y.; Ishizaki, A.; Yamashita, D.; Nakajima, Y.; Ishida, M. In situ X-ray analytical study on the alteration process of iron oxide layers at the railhead surface while under railway traffic. Wear 2011, 271, 47–53. [Google Scholar] [CrossRef]
- Fukuda, M.; Terada, N.; Ban, T. Study of quantifying and reducing electrical resistance between wheels and rails. Q. Rep. RTRI 2008, 49, 158–162. [Google Scholar] [CrossRef]
- Chollet, H.; Houzé, F.; Testé, P.; Loete, F.; Lorang, X.; Debucquoi, S. Observation of Branly’s effect during shunting experiments on scaled wheel-rail contacts. In Proceedings of the 9th International Conference on Contact Mechanics and Wear of Rail/Wheel Systems (CM2012), Chengdu, China, 27–30 August 2012; pp. 111–114. [Google Scholar]
- Houzé, F.; Chollet, H.; Testé, P.; Lorang, X.; Loëte, F.; Andlauer, R.; Debucquoi, S.; Lerdu, F.; Antoni, M. Electrical behaviour of the wheel-rail contact. In Proceedings of the 26th International Conference on Electrical Contacts (ICEC-ICREPEC2012), Beijing, China, 14–17 May 2012; pp. 67–72. [Google Scholar]
- Haydar, L.; Loete, F.; Houzé, F.; Choupin, T.; Guiche, F.; Testé, P. Enhancing Railway Network Safety by Reproducing Wheel–Rail Electrical Contact on a Laboratory Scale. Appl. Sci. 2023, 13, 10253. [Google Scholar] [CrossRef]
- Kaza, G.-L.; Houzé, F.; Loëte, F.; Testé, P. Experimental Study and Phenomenological Laws of Some Nonlinear Behaviours of the Wheel–Rail Contact Associated with the Deshunting Phenomenon. Appl. Sci. 2023, 13, 11752. [Google Scholar] [CrossRef]
- Holm, R. Electrical Contacts: Theory and Applications, 4th ed.; Springer: Berlin, Germany, 2000. [Google Scholar]
- Duvivier, P.-Y. Étude Expérimentale et Modélisation du Contact Electrique et Mécanique Quasi Statique Entre Surfaces Rugueuses d’or: Application aux Micro-Relais MEMS. Ph.D. Thesis, Ecole Nationale Supérieure des Mines de Saint-Étienne, Saint-Étienne, France, 2010. [Google Scholar]
- Kim, W.; Wang, Q.J. Numerical Computation of Surface Melting at Imperfect Electrical Contact between Rough Surfaces. In Proceedings of the 52nd IEEE Holm Conference on Electrical Contacts, Montréal, QC, Canada, 25–27 September 2006; pp. 81–88. [Google Scholar]
- Puech, L. Élaboration et Caractérisations de Couches Minces de Magnétite pour des Applications Microbolométriques. Ph.D. Thesis, Université Toulouse 3 Paul Sabatier, Toulouse, France, 2009. [Google Scholar]
- Huang, Y.-S.; Chen, L.; Lui, H.-W.; Cai, M.-H.; Yeh, J.-W. Microstructure, Hardness, Resistivity and Thermal Stability of Sputtered Oxide Films of AlCoCrCu0.5NiFe High-Entropy Alloy. Mater. Sci. Eng. A 2007, 457, 77–83. [Google Scholar] [CrossRef]
- Falcon, E.; Castaing, B. Electrical conductivity in granular media and Branly’s coherer: A simple experiment. Am. J. Phys. 2005, 73, 302–307. [Google Scholar] [CrossRef]
- Dorbolo, S.; Ausloos, M.; Vandewalle, N. Reexamination of the Branly effect. Phys. Rev. E 2003, 67, 040302. [Google Scholar] [CrossRef] [PubMed]
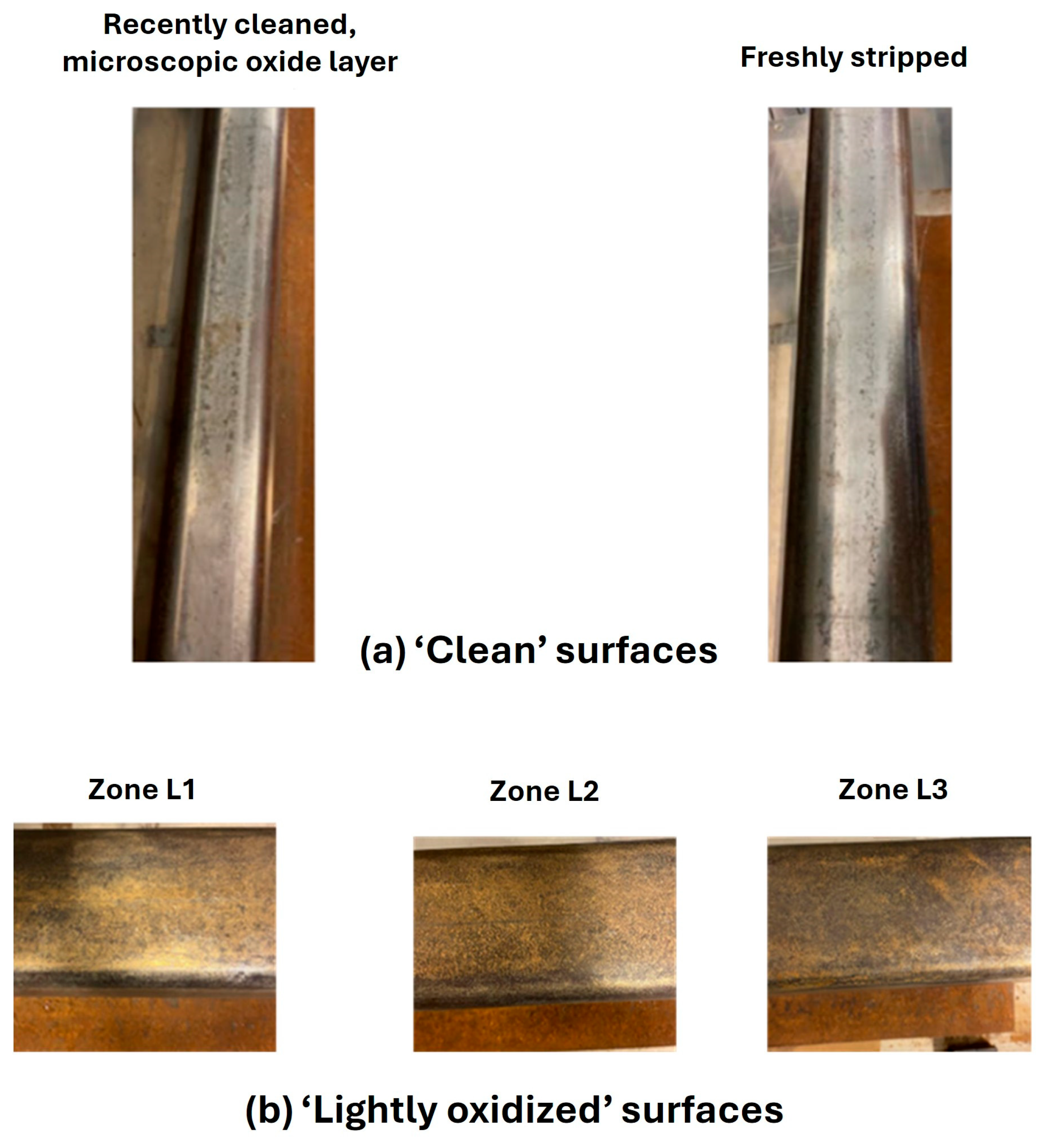


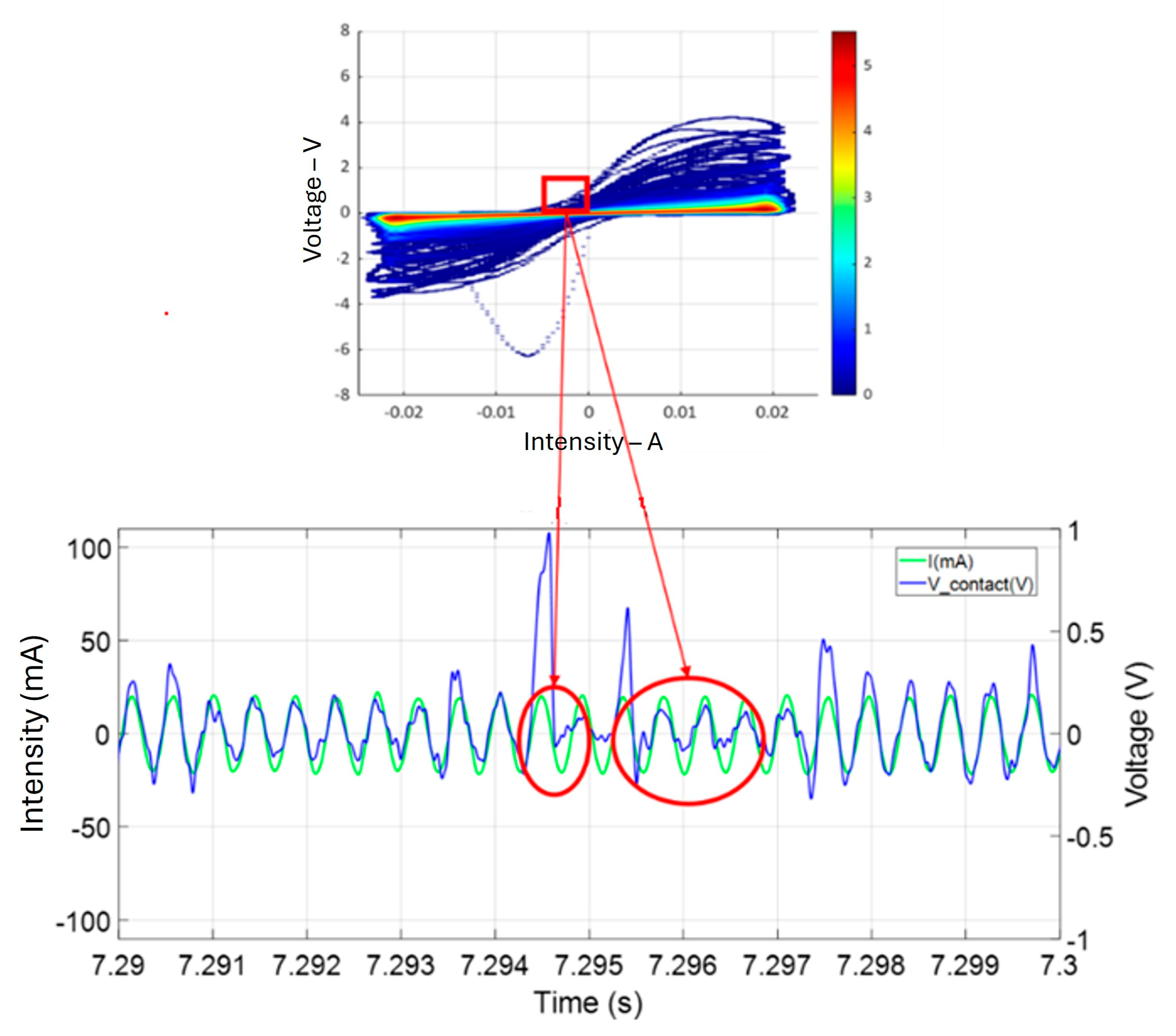
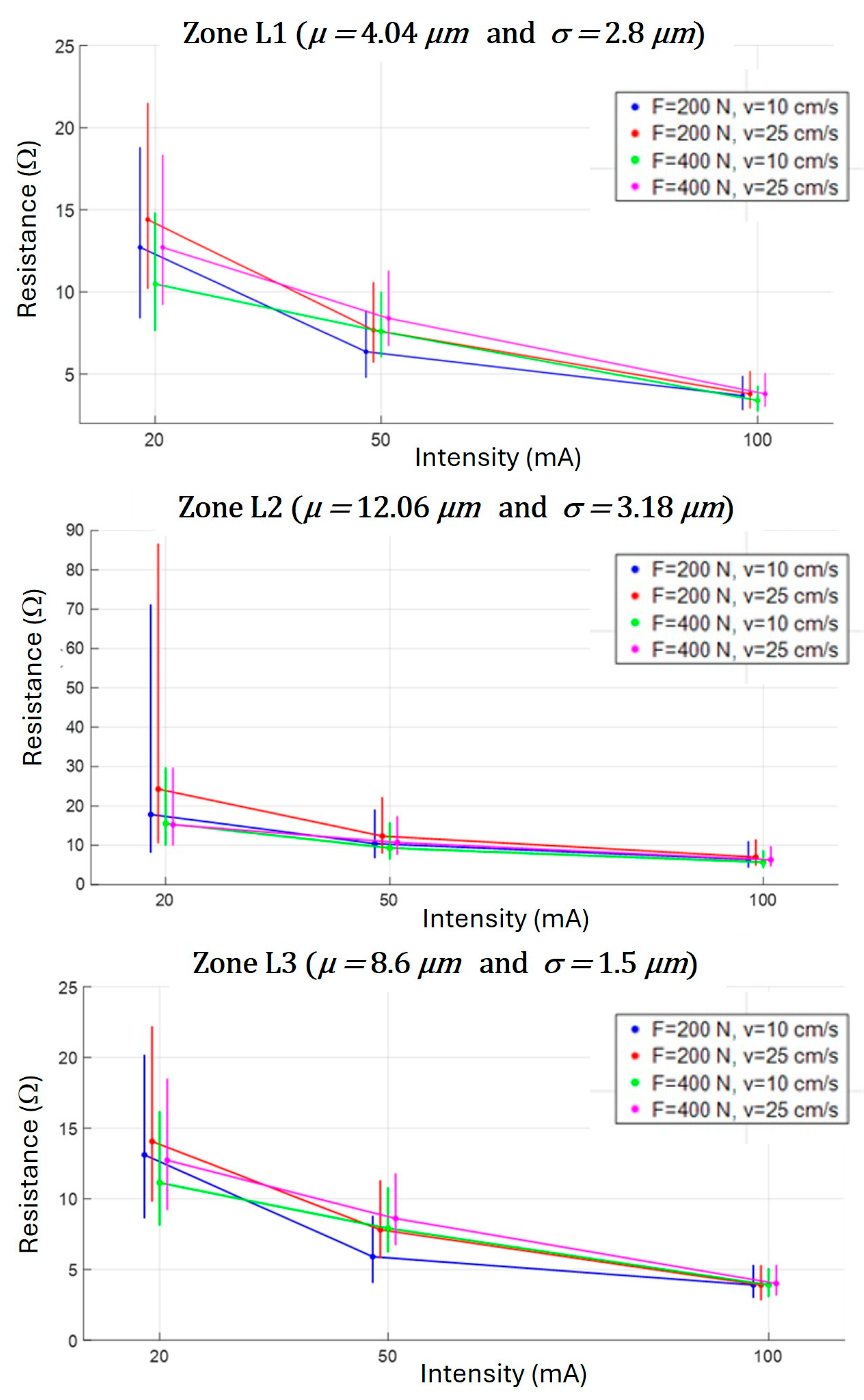
| Zone | Oxide Thickness (µm) | Standard Deviation (µm) |
|---|---|---|
| L1 | 4.04 | 2.8 |
| L2 | 12.06 | 3.18 |
| L3 | 8.6 | 1.5 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Haydar, L.; Loete, F.; Houzé, F.; Slimani, K.; Guiche, F.; Testé, P. Influence of Steel Oxidation on the Behavior of the Wheel–Rail Electrical Contact in Dynamic Conditions. Appl. Sci. 2025, 15, 5760. https://doi.org/10.3390/app15105760
Haydar L, Loete F, Houzé F, Slimani K, Guiche F, Testé P. Influence of Steel Oxidation on the Behavior of the Wheel–Rail Electrical Contact in Dynamic Conditions. Applied Sciences. 2025; 15(10):5760. https://doi.org/10.3390/app15105760
Chicago/Turabian StyleHaydar, Luna, Florent Loete, Frédéric Houzé, Karim Slimani, Fabien Guiche, and Philippe Testé. 2025. "Influence of Steel Oxidation on the Behavior of the Wheel–Rail Electrical Contact in Dynamic Conditions" Applied Sciences 15, no. 10: 5760. https://doi.org/10.3390/app15105760
APA StyleHaydar, L., Loete, F., Houzé, F., Slimani, K., Guiche, F., & Testé, P. (2025). Influence of Steel Oxidation on the Behavior of the Wheel–Rail Electrical Contact in Dynamic Conditions. Applied Sciences, 15(10), 5760. https://doi.org/10.3390/app15105760









