Experimental Behavior and FE Modeling of Buckling Restrained Braced Frame with Slip-Critical Connection

Abstract

This paper examines the hysteretic behavior of the buckling restrained braces (BRBs) in the steel frame. Both experimental and finite element (FE) studies were conducted. The experimental results showed that the well-detailed buckling restrained braced frame (BRBF) withstood significant drift demands, while the BRB exhibited significant yield without severe damage. Although the BRB inside the steel frame was subjected to 2.69% strain of the CP under the axial compression demands, the local and global deformations were not observed. The FE model was developed and validated. The numerical investigations of hysteretic behavior of the BRBF in the literature are generally focused on the friction between the core plate (CP) and the casing member (CM). The results suggest that the behavior of the BRBF is significantly affected not only by the friction between CP and CM but also by the pretension load on the bolts and the friction between the contact surfaces of steel plates of slip-critical connections in the steel frame. The FE analysis showed that pretension loads of 35 kN and 75 kN gave accurate predictions for cyclic responses of BRBF under tension and compression demands, respectively. Moreover, the FE predictions were in good agreement with the test results when the friction coefficient is 0.05 between CP and CM and it is 0.20 between steel plates.

1. Introduction

The lateral drifts and internal forces appear in cases where the structures are subjected to lateral forces instigated by strong ground motions or wind. The structural and non-structural members are damaged if these lateral drifts are excessive. In order to limit lateral drifts, using steel braces is one of the most well-known solutions for structures. One of the most important disadvantages in the design of the steel braces is that the tensile and the compression capacities are not equal or symmetric. Black et al. [1] reported that when the steel braces yielded under the large tension forces, they buckled under the compressive forces, and their axial load-carrying capacity suddenly decreased. This unsymmetrical hysteretic behavior in tension and compression results in a reduction in lateral stiffness and excessive damage to beams and columns in the steel braced frame. If the steel brace members are prevented from buckling, their hysteretic behavior can be shifted from unsymmetrical to symmetrical. For this reason, buckling restrained braces (BRBs) have become an attractive research topic among researchers all over the world. The BRBs consist of a core plate (CP), a casing member (CM), and debonding material (see Figure 1). The compression capacity of CP can be enhanced by using a CM or by preventing it from buckling, although it has negligible compression capacity. Therefore, the CP may yield in tension or compression or can buckle in high buckling modes. As can be seen in Figure 1, a conventional BRB has three parts, namely, an unrestrained elastic zone, a restrained elastic zone, and a restrained plastic zone.

The CMs usually consist of mortar-infilled steel tubes. Ozcelik et al. [2] tested the BRBs under cyclic loading using the CMs with additional end restrainers made of steel hollow sections (SHSs) and steel plates. The debonding material was used to prevent the friction effect and stress transfer between the CP and CM. Iwata et al. [3] conducted tests on BRBs with CMs made of steel tubes filled with mortar and built-up steel members. BRBs with rectangular and cruciform sections were tested by Clark et al. [4] and Black et al. [5]. Chen et al. [6] tested BRBs with low-yield-point steel and a ductile CP. They used silicone grease to prevent friction between the CP and CM, and the compression capacity of BRBs was about 1.5 times higher than their tension capacity due to the inadequate gap between the CP and CM. Higgins and Newell [7] used a steel pipe filled with a confined non-cohesive material. Ju et al. [8] tested BRBs consisting of the I-section CP and the CM with SHS without mortar infill. Takeuchi et al. [9] suggested a way to prevent in-plane local buckling failure of a BRB whose restrainer consisted of a circular or rectangular steel tube with mortar of various thicknesses. They made a proposal that the local buckling failure of BRBs can be changed by mortar thickness and steel tube shape. An experimental program of a BRB with a CM consisting of a built-up steel section was conducted by Eryasar and Topkaya [10]. Tsai et al. [11] experimentally investigated the hysteretic behavior of BRBs that were used for retrofitting an existing structure. Tremblay et al. [12] conducted sub-assemblage test series of the BRBs that were used to improve the lateral stiffness of a four-story steel frame. In order to determine the effects of flexure stress on the BRBs, the influence of the CP length, the axial rigidity, and fatigue life, the BRBs were tested by Tremblay et al. [13]. BRBs with concrete-filled tubes in the steel frame were tested in the USA by Uriz and Mahin [14]. Lopez et al. [15] and Merritt et al. [16] tested BRBs by subjecting them to both end rotation and axial displacement using a shake table facility. Okazaki et al. [17] and Hikino et al. [18] performed shake table tests on BRBs to investigate their out-of-plane stability. Kasai et al. [19] performed sinusoidal deformation tests on full-scale five-story buildings with dampers by using a shaking table. Christopulos [20] conducted five buckling-restrained braced frame (BRBF) tests under cyclic loading. During these tests, local buckling of BRBs occurred at a drift ratio of 1.5%. Tsai et al. [21] and Tsai and Hsiao [22] tested a full-scale three-story three-bay BRBF by using a pseudo-dynamic testing method. Furthermore, Mazzolani [23], Di Sarno and Manfredi [24], and Brown et al. [25] examined the strengthening of deficient reinforced concrete (RC) frames with BRBs. Wu et al. [26] tested 8.5 m and 3 m BRBs under axial compression and tension demands to investigate the effects of their yield length on the energy dissipation capacity. They concluded that BRBs with shorter yield lengths have higher energy dissipation capacity compared to those with longer ones. Alemayehu et al. [27] carried out the brace and subassembly tests of tube-in-tube BRBs consisting of circular and square CPs, tube CM, and infill polymer to investigate their capability of stability and inelastic deformation. A bare frame and a BRBF were tested under cyclic loading by Li et al. [28]. They observed that the BRBs improve the lateral resistance, initial rigidity, and energy dissipation capacity of frames. As can be understood from the recent literature, the behavior of BRBF has yet to be fully understood [29,30,31,32,33,34], although it is an area with great potential when considering energy dissipation.

Many researchers have proposed the finite element model (FEM) to simulate the behavior of the BRBs. AlHamaydeh et al. [35] numerically investigated the effects of significant parameters, such as material strength, the gap between the CP and CM, and the initial imperfection of CP on the behavior of the BRBs. When the cyclic response of BRBs was quite symmetrical in tension and compression demands, half and quarter models, due to the advantages of reduced number of elements and decreasing analysis time, were used [36]. The full and quarter FEM models were used to simulate BRBs [35]. It was observed that the results were comparable [35]. Pandikkadavath et al. [37] numerically investigated the effect of the length of the yielding of CP segments on their hysteretic response, energy dissipation, strength adjustment factor, and axial resistance. Guo et al. [38] conducted component tests on five BRBs under the different loading protocols. Two of them were performed under cyclic pure compression loading, and three of them were subjected to reversed cyclic loads. In their research, a three-dimensional (3D) FEM for BRBs was developed by using Abaqus software [39], and the numerical results were compared to the test results. Hoveidae and Rafezy [40] carried out a numerical analysis series on BRBs with different types of CMs. They investigated the effects of gap size and flexural response of CMs on the global buckling of BRB. The 3D FEM of the BRBs was modeled by using Abaqus software [39] to numerically predict their hysteretic behavior [41]. They compared the predicted hysteretic behavior of the BRBs, the CP fracture, and energy dissipation with those of the test results to verify the FE model. Chou and Chen [42] conducted a parametric study on the different sandwiched BRBs to find the size effect of CM, cross-section area, and length of CP on their buckling behavior. Zub et al. [36] developed the FEM for BRBs by calibrating materials (e.g., steel used for CP and concrete used for mortar) and friction coefficient between the CP and CM. They conducted a parametric study using this calibrated material model to evaluate the influence of the strength of CM, concrete strength, mechanical properties of steel, self-weight, geometrical imperfection, and frame effects of the BRBs. As can be understood from the recent literature related to numerical studies, the numerical investigations of hysteretic behavior of BRBF in the literature are generally focused on the friction between the CP and CM. However, this paper focused on the effects of pre-tension load on the bolts of slip-critical connections in the steel frame and the friction between the contact surfaces of the steel plates on the numerical behavior of the BRBF. Moreover, conducting experimental studies to understand the structural behavior of BRBF can be prohibitive due to their high cost. However, carrying out FE studies with validated physical tests can reduce the cost and time, together with considering different parameters that affect structural behavior. It is therefore required to develop a proper FE model to predict the hysteretic behavior of BRBF. This paper also offers an FE model accurately predicting the behavior of BRBF.

The aim of this paper is to both experimentally and numerically investigate the behavior of BRBFs. The BRBF specimen was tested under the reversed cyclic loading protocol. The BRB specimen used in the steel frame had identical properties to the BRBs tested by Ozcelik et al. [2]. An FE model was developed by using the Abaqus software [39] and validated to conduct further parametric research. A numerical parametric study was first conducted on three BRBs [2] to calibrate material used for the CP and the friction coefficient between the CP and the CM. This was followed by a numerical parametric study carried out on the steel frame with a slip-critical connection to determine the friction coefficient between contact surfaces of steel plates of slip-critical connections. The pretension load for bolts was taken as 110 KN in accordance with the Turkish Steel Code (TSC) [43]. The numerical hysteretic behavior of the BRBF was last parametrically investigated using the calibrated values for the BRB and steel frame obtained from the first and second phases of the FE study.

2. Materials and Methods

There are three sets of experimental studies that were simulated by using Abaqus software Version 6.14 [39] in this paper. Steel frame and BRBF, which were tested in this study, were simulated in Abaqus software [39]. The single-BRB specimens tested by Ozcelik et al. [2] were also simulated in this study.

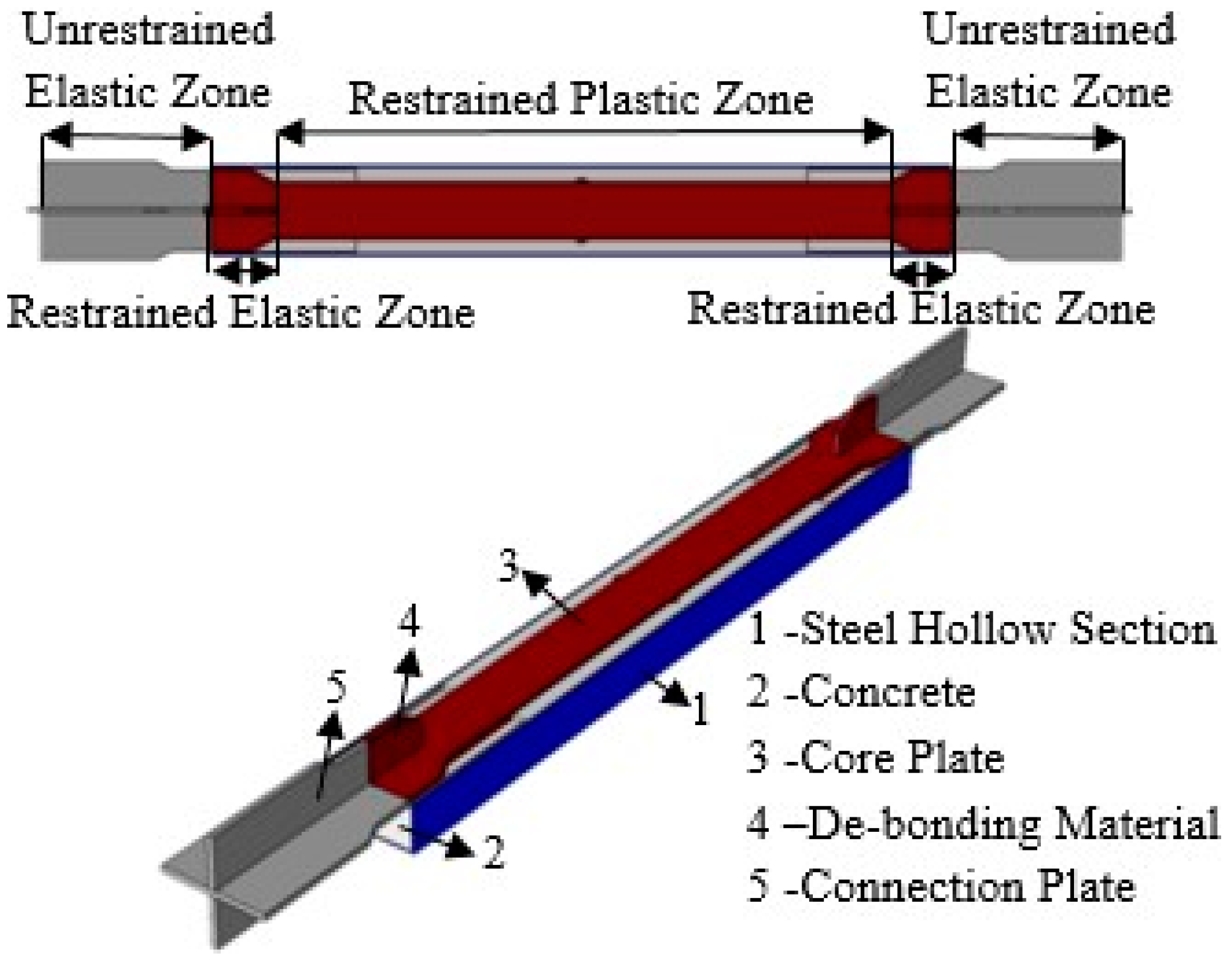
2.1. BRB Test Specimens

Ozcelik et al. [2] conducted an experimental program consisting of ten BRBs to explore the effects of different end restrainers and the CMs on the hysteretic behavior of BRBs. In the FE study of this paper, BRB2, BRB4, and BRB7 were used among the BRB specimens of Ozcelik et al. [2] in order to determine material parameters used for the CP and friction coefficient between the CM and the CP. The geometrical details of these BRBs are given in Figure 2 and

Table 1. Geometrical details of BRBs.

Spec. No.	End Restrainers	Thickness of Rubber	Area (mm2)
			Cruciform Sec.	Cruciform Sec.	CP
			Section A-A	Section B-B	Section C-C
BRB2 [2]	40 cm-SHS 100 × 3	4	7875	6375	2250
BRB4 [2]	40 cm-Steel Plates	4	7875	6375	2250
BRB7 [2]	40 cm-Steel Plates	4	7875	6375	2250
BRB *	40 cm-Steel Plates	4	7875	4875	1500

* Used in the BRBF specimen.

.

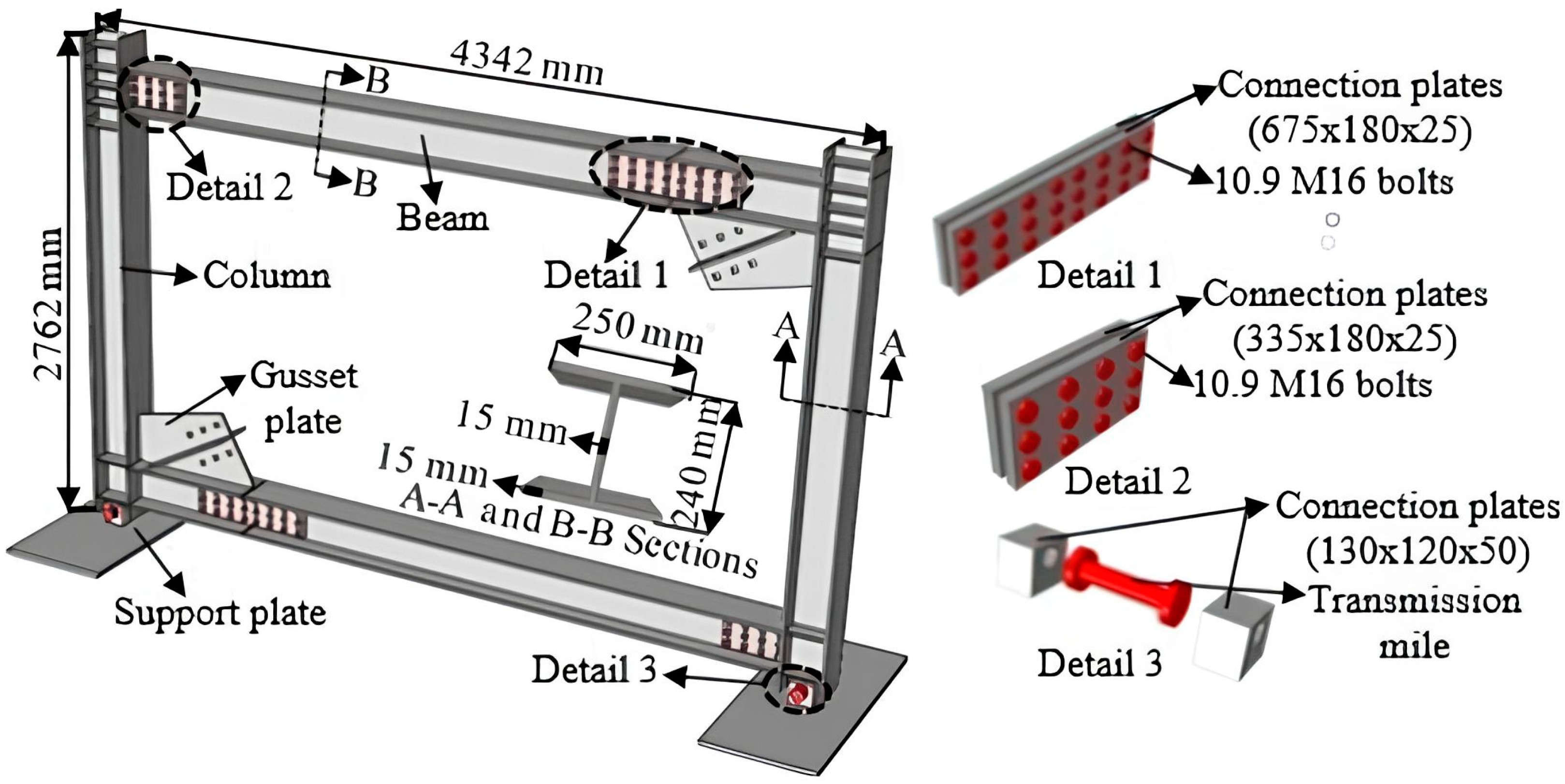
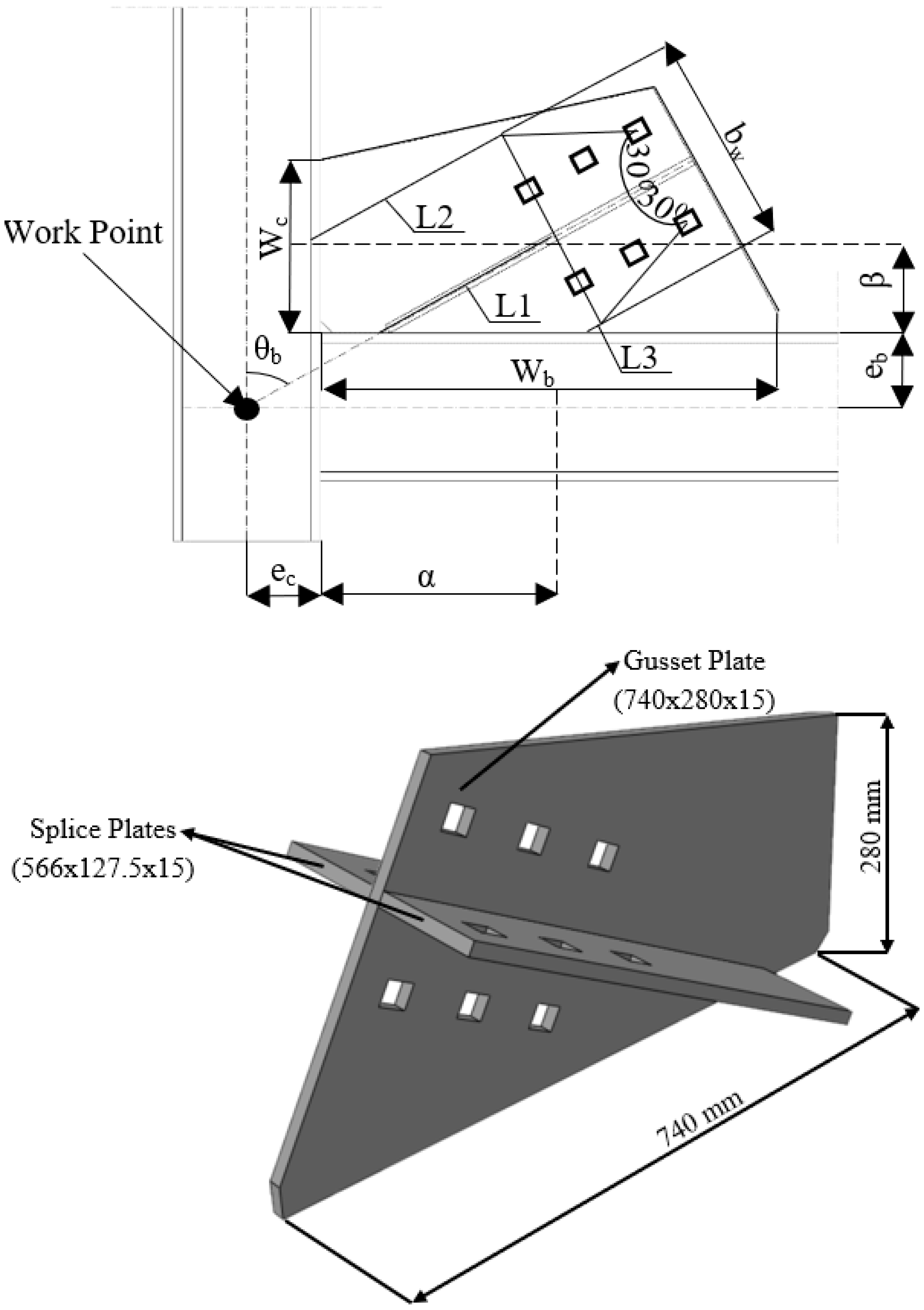
2.2. Steel Frame

The BRB elements must have sufficient cyclic strength to be used as the energy damper in the structures. Their cyclic performance is determined by the brace and subassembly testing procedures found in the AISC. In the brace test, their ability to sustain large cyclic axial tension and compression without buckling and fracture is verified. In the subassembly test, their ability to accommodate axial and rotational demands imposed by the frame is verified. In this study, a subassembly test of BRB whose geometrical properties are similar to those of BRB4 tested by Ozcelik et al. [2] was performed to determine whether its cyclic performance is sufficient or insufficient. It should be noted that the steel frame should remain within the elastic limit while the BRB is yielding, according to the AISC in the subassembly test. Therefore, in the design of all steel frame members, strength-based design provisions were used to ensure that they remained within the elastic limit. In addition, all connections in the steel frame were designed to allow for rotation so that all demands were only withstood by BRB (see Figure 3). For these reasons, the columns, beams, and gusset plates of the steel frame were designed to remain elastic under lateral demands up to 1000 KN. A lateral excursion was applied by using a displacement-controlled hydraulic actuator that has a 1000 kN load capacity.

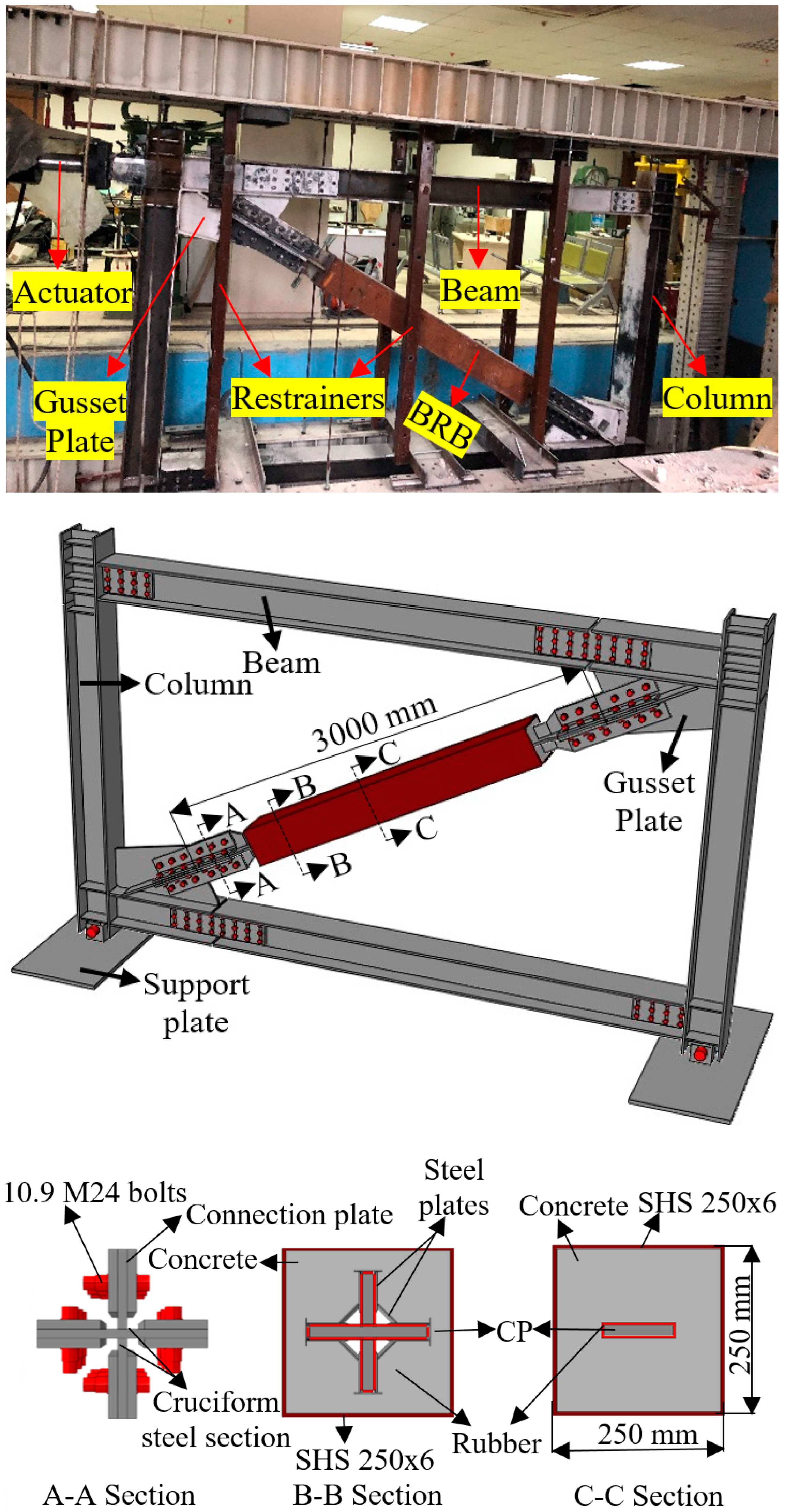
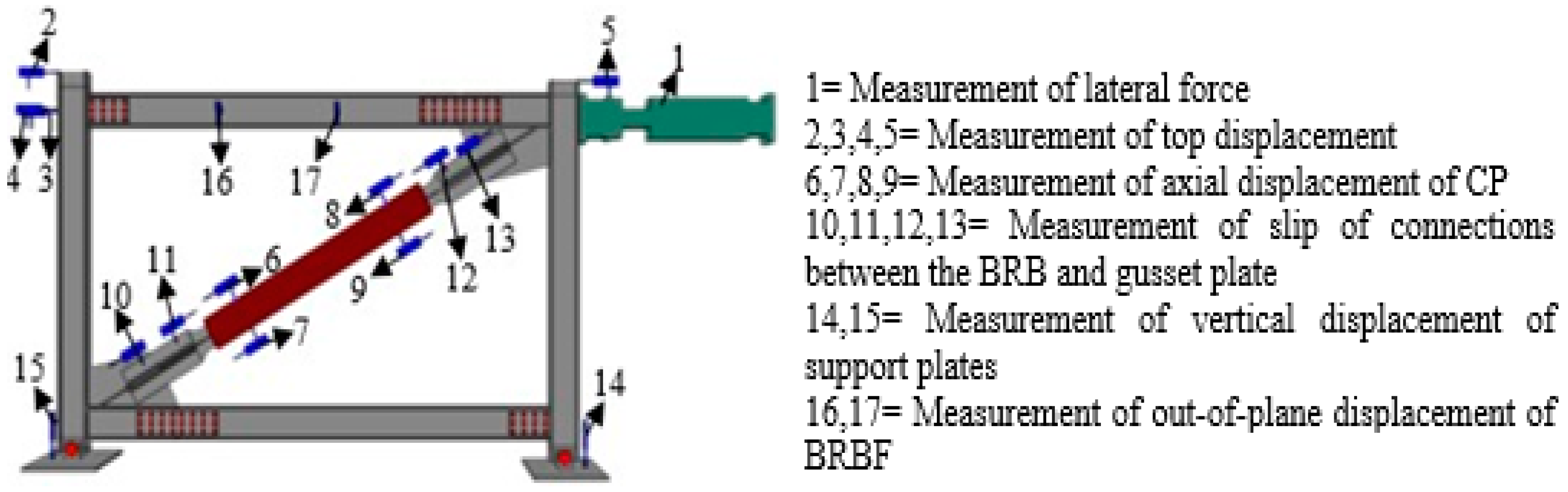
2.3. The BRBF

The geometrical properties of BRB used in the BRBF specimen were identical to those of BRB4, which was tested by Ozcelik et al. [2]. As can be seen in Figure 4, the CM with a concrete compressive cylinder strength of 25 MPa consisted of an SHS 250X6. The CP was made of a 15 mm thick steel plate wrapped with 4 mm thick rubber (see Figure 4). The additional end restrainers were provided with steel plates welded to each other to prevent local deformation at both ends of the BRB (see Figure 4). The yield stress of CP and members of the steel frame were determined to be 270 MPa and 330 MPa, respectively, from the tensile coupon tests. All connections in the BRBF were designed to allow for rotation so that all demands were withstood by the BRB. The boundary conditions were considered to be pinned connections, which allow for rotation. They were provided with high-strength transmission miles. The support plates were installed first. This was followed by the steel columns being assembled with support plates by using high-strength transmission miles. The details of connection with the transmission mile are given in Figure 3. The steel beams and steel columns were then connected to each other with high-strength 10.9 M16 bolts. The details of the connection between the steel column and beam are given in Figure 3. The BRB was lastly attached to the steel frame with high-strength 10.9 M24 bolts (see Figure 4) and the LVDTs with a 200 mm measuring capacity were placed to measure the displacements in the BRBF. The yield and the tensile strengths were 900 MPa and 1000 MPa, respectively, for all high-strength 10.9 bolts with respect to TSC [43].

The bolted connection with slotted holes between the BRB and gusset plate was used to ensure easy installation of the BRB. In order to prevent the slip in these connections, they were designed according to the slip-critical connection design provisions in the TSC [43]. The gusset plate was designed with respect to the Uniform Force Method (UFM) given in the American Institute of Steel Construction (AISC) [44]. The size of the gusset plate was determined by solving equilibrium equations about the working point, which is the intersection point of axes of the beam, column, and brace members (see Figure 5).

The buckling capacity of the gusset plate was predicted with Thornton’s Method [45]. According to this method, the equivalent width (b_w) is determined by using 30-degree extrapolation (see Figure 5). The buckling length (l_t) is determined by Equation (1) [45]. l₁, l₂, and l₃ are given in Figure 5.

$$l_t=min\left(l_2,{\displaystyle \frac{\left(l_1,l_2,l_3\right)}{3}}\right)$$

(1)

It is then used to calculate a buckling coefficient (${\mathsf{\lambda }}_{\mathrm{t}}$). The ${\mathsf{\lambda }}_{\mathrm{t}}$ is calculated by Equation (2) [43], where i_y is the radius of gyration and the effective length factor (k) is taken as 0.5 for square gusset plates and 0.65 for tapered gusset plates [44]. However, k was taken to be 1 in this study.

$${\lambda }_t={\displaystyle \frac{k\times l_t}{i_y}}$$

(2)

The critical buckling capacity of the gusset plate was determined to be 1841 kN with respect to Equations (3)–(5) given in TSC [43], where F_e is the elastic buckling stress, E is the elastic modulus, F_y is yield stress, P_n is the buckling capacity, F_cr is the critical buckling stress, and A is the cross-section area.

$$F_e={\displaystyle \frac{{\pi }^2\times E}{{\left({\lambda }_t\right)}^2}}$$

(3)

$$F_{cr}=\left[{0.658}^{{\displaystyle \frac{F_y}{F_e}}}\right]\times F_y$$

(4)

$$P_n=F_{cr}\times A$$

(5)

In addition, the measurement of lateral forces, top displacement of BRBF, axial displacement of CP, slip of connection between the BRB and gusset plate, vertical displacement of support plates, and out-of-plane displacement of BRBF were carried out by the LVDTs during the test. The positions of the LVDTs are given in Figure 6.

2.4. Loading Protocol

The lateral excursion was based on the strain of CP for the BRB members [2] and the inter-story drift ratio (IDR) for BRBF. The BRBF was loaded up to 2.5% IDR using the displacement-controlled hydraulic actuator. In this study, subassembly test procedures were adopted with respect to AISC [44]. The loading protocol of the BRB specimens [2] was as follows: 1/3δy, 2/3δy, 1.0δy, 1/3δstr, 0.5δstr, 1.0δstr, 1.5δstr, 2.0δstr and 2.5δstr, where δ_y and δ_str were the axial yield displacement of the CP and the displacement value corresponding to 1% strain of the CP, respectively. The loading protocol of BRBF was as follows: 0.3% IDR, 0.5% IDR, 0.75% IDR, 1.0% IDR, 1.5% IDR, 2.0% IDR, and 2.5% IDR. Two cycles were performed for each displacement defined in both loading protocols. These loading protocols are shown in Figure 7.

3. FE Study

The FE study was performed by considering three phases. The 3D FEM of all BRBs [2], the steel frame with slip-critical bolted connection, and the BRBF were constructed by Abaqus software [39] to simulate their hysteretic behavior under cyclic loading.

The 3D hexahedron solid elements with eight integration points are not only suitable for plasticity problems but can also better predict the actual behavior of the test specimens [39]. These elements are therefore usually adopted by researchers [30,35,36,37,38,40,41,42]. There are three types of 3D hexahedron solid elements with eight integration points: 3D solid element with full integration (C3D8), 3D solid element with reduced integration (C3D8R), and 3D solid element with full integration nourished by incompatible modes (C3D8I). The shear locking and hourglass effects determine which type of these elements are used in the FEM. An element bends in reality (see Figure 8a), but it does not bend in the FE analysis. As can be seen in Figure 8b, the angle between the grids decreases to less than 90 degrees. That is, the angle between the grids changes in the FEA, but this is not true, since no shear occurs in the element under bending. The shear locking causes that element to exhibit more rigid behavior in the FE analysis. Due to the computed strain for the C3D8 element being constant, shear locking occurs in these elements. The C3D8R element can overcome the shear locking in the FE analysis because there is only one integration point at its center (see Figure 8c).

The grids at this integration point (see Figure 8c) are constant without being affected by changes in the edges of elements. That is, it is not important whether the edge of the element is bent or not, and the angle between grids at the integration point always remains as 90 degrees [39]. Even if the C3D8R element can overcome the shear locking under the bending, singular modes (hourglass modes) can take place due to its insufficient rank of rigidity matrix. These modes cannot generate stress and strain, but they are zero-energy modes that can affect the accuracy of FE analysis. They can also result in unrealistic strains, stresses, bends, and contacts in FE analysis [39]. The 3D hexahedron solid elements with eight integration points that are available can overcome shear locking as well as hourglass modes. The C3D8I element can eliminate the stresses that cause the shear locking through the additional degrees of freedom that are provided with incompatible modes, and the hourglass modes also cannot occur owing to their full integration. For these reasons, the C3D8I element was used in all FE analyses to avoid shear locking and hourglass modes. Furthermore, Korzekwa and Tremblay [46] suggested that kinetic energy and artificial strain energy should be considered to be lower than 1.0% of internal energy for valid numerical results.

The slip-critical bolted connections in both the BRBF and the steel frame were modeled by using the micro-modeling technique. The contact boundary conditions between main plates and splice plates, bolt screws and bolt holes, and bolt nuts and splice plates were determined so as to reconstruct contact, separation, and fixing conditions in the FEA after the slips occurred in the slip-critical connections. While using the surface-to-surface discretization method to prevent surface penetration, the finite sliding approach was used to enable the slips between the contact surfaces [39]. Hard contact and penalty friction were used to define normal and tangential behaviors of contact pairs, respectively. The friction between the contact surfaces was defined by using Coulomb friction [47,48,49,50]. The interactions described above are shown in Figure 9. The bolts are generally modeled as 3D solid or 2D spring elements in the FEM [47,48,49,50,51,52,53,54,55,56,57,58,59,60,61]. The behavior of the slip-critical connection can be predicted more accurately by the bolts modeled with 3D solid elements when compared to simplified methods [62]. Furthermore, the pretension load was applied to bolts with a bolt load, which is available as a load option in Abaqus [39]. The pretension load on the bolts is given in Figure 9. Moreover, the CP was modeled to interact with the CM by using the hard contact with the allowed-for separation to disallow tensile and compressive stress transfer between the CP and the CM surfaces. In addition, the tangential behavior provided by penalty contact with the friction coefficient was defined between the CP and CM surfaces instead of modeling the rubber.

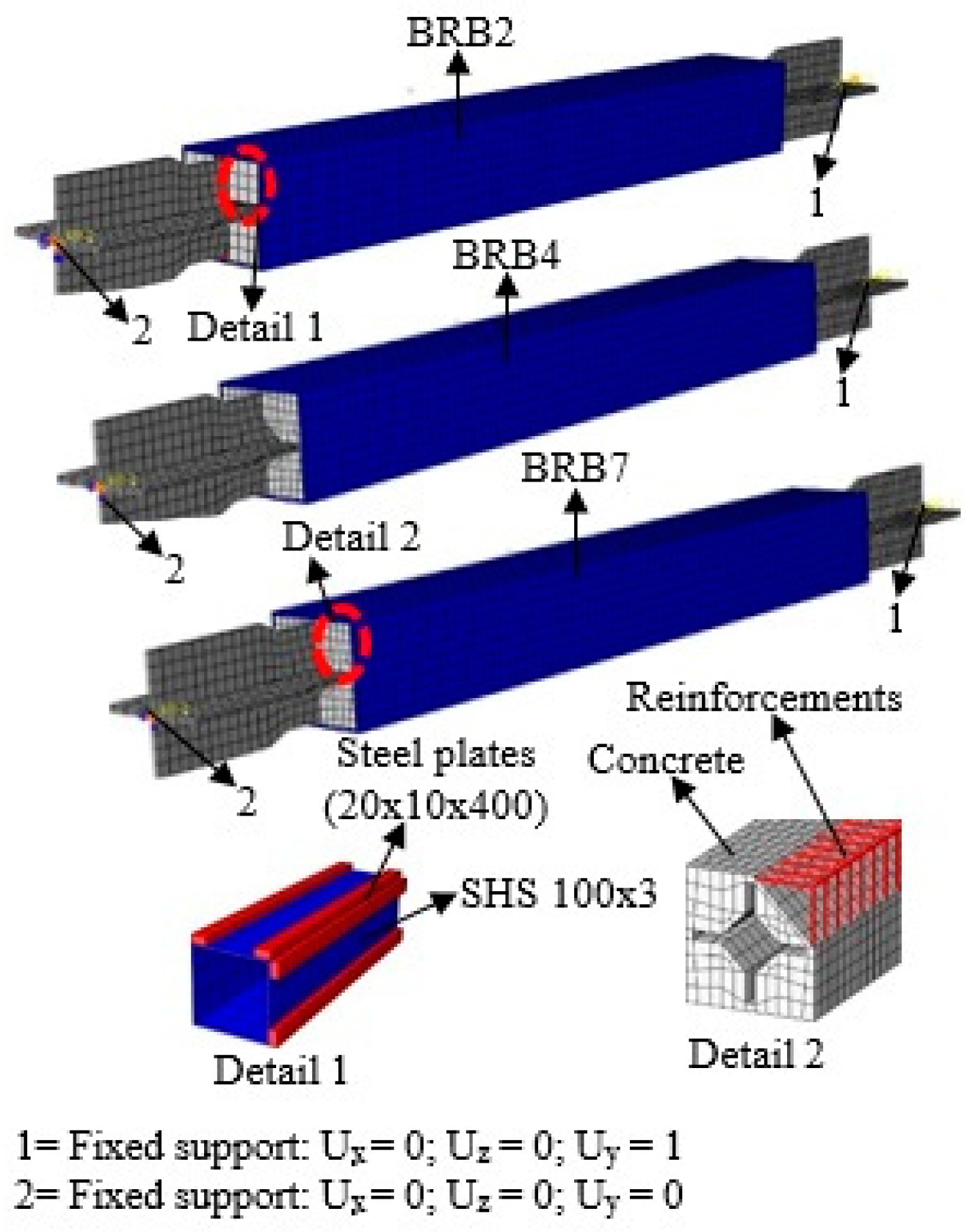
3.1. FEM of BRBs

The BRB2, BRB4, and BRB7 had the same CP, but their CMs were different [2]. The additional end restrainer of BRB2 was formed by welding 20 × 10 steel plates on the alignment of SHS 100 × 3. The tied constraint was used to simulate the welded connection between their surfaces. It was also used between the steel tubes and concrete for FEM of all BRBs because no slip was observed between them during the test. The CM of the BRB7 consisted of reinforced concrete. The reinforcements were modeled using two node truss elements (T3D2). The truss elements were joined to the concrete solid element using embedded constraints. The reference points were connected with the surfaces at both ends of the BRBs using a continuum-distributing coupling constraint. The boundary conditions were defined in these reference points. The FEM of all BRBs is given in Figure 10.

The stress–strain curve of steel material under cyclic loading is different from that under monotonic loading [63]. Therefore, the combined isotropic–kinematic hardening material model was used for the behavior of the CP under cyclic loading. The hardening parameters of steel material should be calibrated from the cyclic coupon test data [39].

In this study, the fatigue tests of coupon specimens for CPs were not available, but tensile coupon tests of material for CPs were conducted. Therefore, to obtain the hardening parameters of steel material under the cyclic loading, a numerical parametric study was carried out on the BRB2 using combined isotropic–kinematic hardening steel material models of many previous studies in the literature [36,46]. In addition, these parameters of material models were suitably calibrated by the trial-and-error method to match the numerical and test results. The calibrated material models were then used in all FE analyses. All parameters for material models are given in

Table 2. Material models.

Material Model	Kinematic Hardening Parameters (Mpa)											Isotropic Hardening Parameters (Mpa)
	σ0	C1	γ1	C2	γ2	C3	γ3	C4	γ4	C5	γ5	Q∞	b
Korzekwa and Tremblay [46]	270	8000	75	-	-	-	-	-	-	-	-	110	4
Zub et al. [36]	270	95,000	1300	40,500	680	5000	120	500	2.5	200	2	-	-
Calibrated Korzekwa and Tremblay [46]	270	8000	75	-	-	-	-	-	-	-	-	110	2
Calibrated Zub et al. [36]	270	87,000	1300	32,500	680	4600	120	275	2.5	160	2	-	-

σ₀: yield stress; C_i: kinematic hardening moduli; γ_i: the corresponding decreasing rate of kinematic hardening moduli with increasing plastic deformations Q_∞: the maximum change in size of yield plateau; b: defines the rate of change in yield plateau as the plastic strain develops.

. The other components (concrete, steel tubes, and steel plates) remained within the elastic limit during the tests [2]; so, they were taken as elastic in the FE analyses. The mechanical properties of these components are given in

Table 3. Mechanical properties of components of all BRBs.

Components	Young’s Modulus (MPa)	Poisson’s Ratio	Fy (MPa)	Fu (MPa)	fc (MPa)
CP	210,000	0.3	270	401	-
SHS 250X6	210,000	0.3	235	-	-
SHS 100X3	210,000	0.3	235	-	-
Concrete	25,000	0.2	-	-	25

. Numerical parametric studies were performed on the BRBs to find the effects of the material models of steel used for CP and the value of the friction coefficient between the CP and CM. The results of these parametric studies are given in Section 4.

3.2. FEM of Steel Frame

The 3D FEM of the steel frame with slip-critical connection was developed by using Abaqus software [39] to simulate its cyclic response (see Figure 11). The pretension load on the bolts and the friction coefficient between the steel plates are the most important parameters for the behavior of slip-critical connections. The 10.9 M16 high-strength bolts were used in the slip-critical connections. A pretension load of 110 kN was applied for each bolt, according to TSC [43]. Except for the slip-critical connections of the steel frame, its other components were welded to each other; hence, the tied constraint was used between them. All slip-critical connections in the steel frame were modeled according to the contact model, as given in Figure 9. The tensile coupon test was not performed on 10.9 M16 bolts; therefore, their mechanical properties were taken with respect to TSC [43]. Hence, their yield and ultimate stress were taken to be 900 MPa and 1000 MPa, respectively. The yield stress of other components of steel frame were 330 MPa according to results of their coupon tests. The calibrated material models given in Table 2 were used for the FEM of steel frame. To calibrate the value of friction coefficient between the contact surfaces of steel plates of the slip-critical connections, a numerical parametric study was performed by using different values of friction coefficient. The analysis procedure consisted of three steps. In the first step, the boundary conditions were defined with continuum-distributing coupling. In the second step, the pretension load was applied on the bolts. In the final step, the cyclic loading protocol mentioned in previous section was horizontally applied to the steel frame. A comparison of the numerical and test results is given in Section 4.

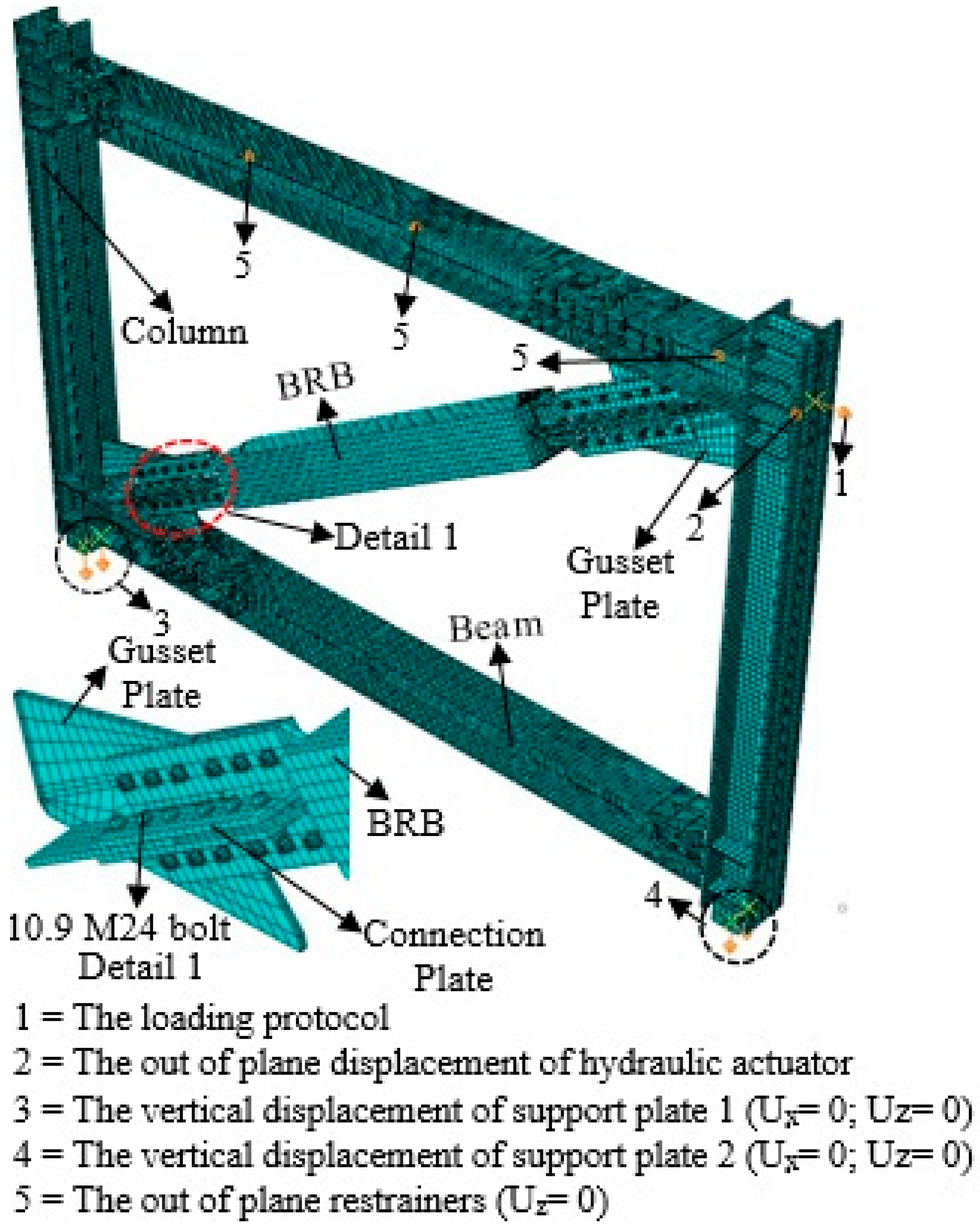
3.3. FEM of BRBF

The 3D FEM of BRBF was developed by using the Abaqus software [39], as shown in Figure 12. The 10.9 M24 bolts were used for connections between the BRB and the gusset plate. Based on the recommendation of TSC [43] for the minimum value of the pretension load, 247 kN, was applied to each 10.9 M24 bolt. No slip was observed in these connections during the test. The out-of-plane displacements from the hydraulic actuator and vertical displacements from the support plates were measured during the test. Therefore, these displacements were applied as the external load to the FEM of the BRBF. These displacements are shown in Figure 13. The slip was not measured in the slip-critical connection of the steel frame during the test. Therefore, different values of pretension load were applied to 10.9 M16 bolts, thereby allowing for a numerical parametric study to investigate the effect of the pretension load on the cyclic behavior of BRBF. In this phase, the value of the friction coefficient between the CP and CM was taken to be 0.05 due to the best numerical results matching the test results. Similarly, the value of the friction coefficient between the contact surfaces of steel plates in the slip-critical connections of steel frame was taken to be 0.2. The yield stress of CP was obtained as 330 MPa from its coupon tests. Therefore, in the FEM of BRBF, the yield stress and young’s modules of CP were taken to be 330 MPa and 210,000 MPa, respectively. A numerical parametric study was conducted using the calibrated material models on BRBF. A comparison between the numerical analysis and the test results is given in the next section.

4. Results and Discussion

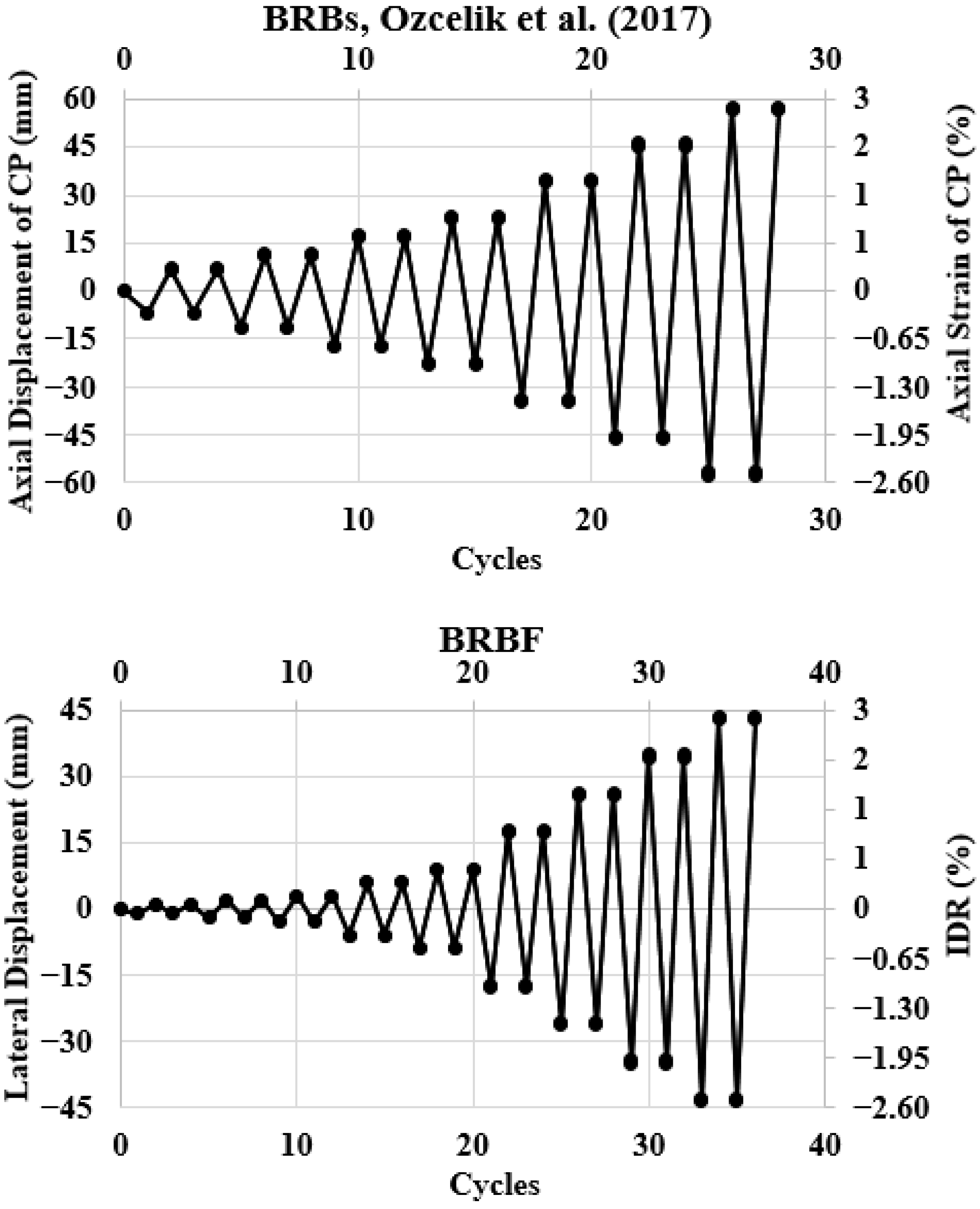
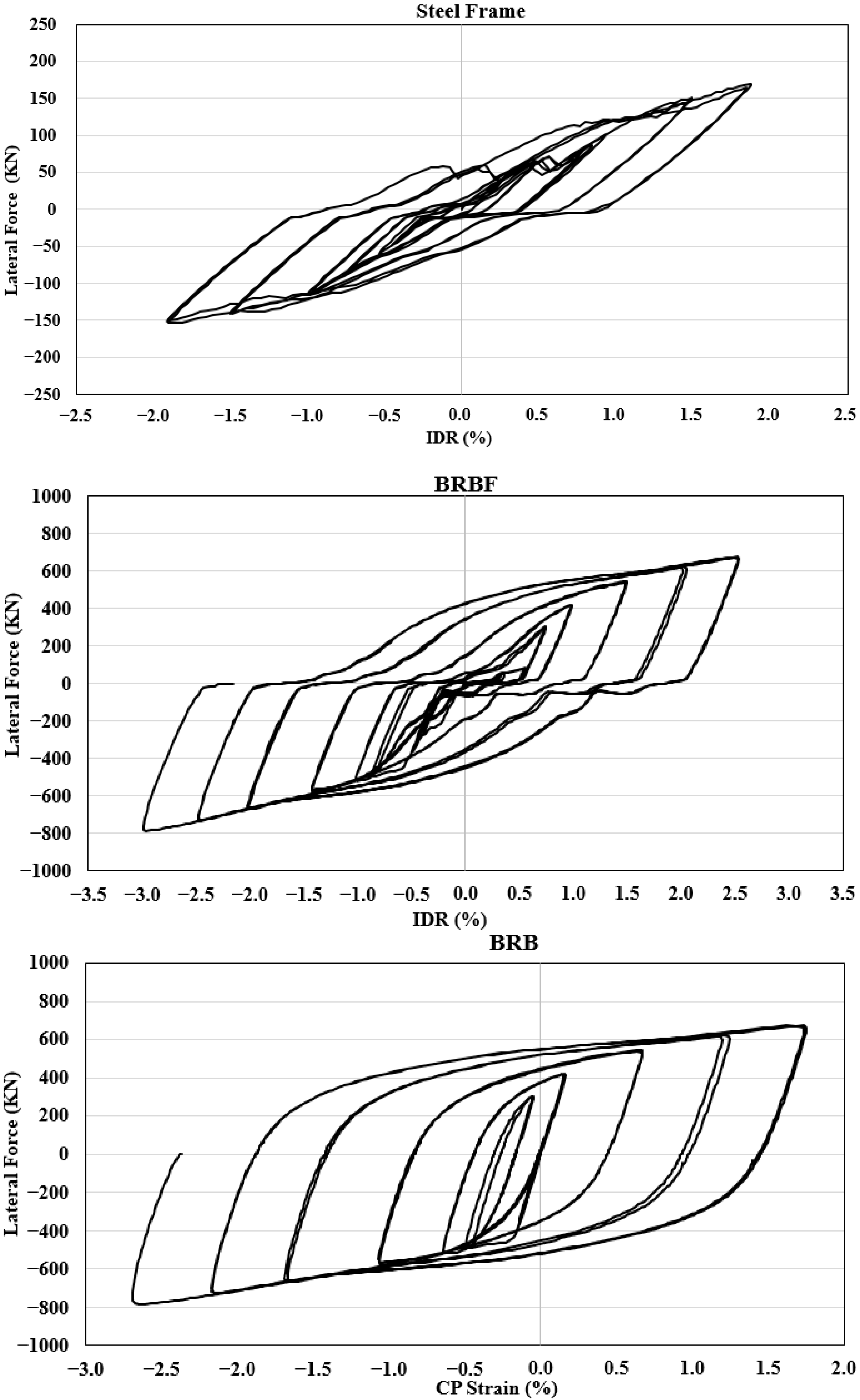
4.1. Test Results

The component test results of the BRBs are available elsewhere [2]; hence, further details are not given in this paper. The steel frame was tested by using the cyclic loading protocol given in Figure 7. This was performed to determine the bare frame capacity and cyclic behavior steel frame. Hence, it allowed for the determination of the contribution of the BRB member to the braced frame. The cyclic response of the steel frame and BRBF is presented in Figure 14. This figure shows the lateral force versus IDR for both the steel frame and the BRBF, and the lateral force versus CP strain for the BRB. The IDR was the ratio between lateral displacement and height of the steel frame (2265 mm). The value of the axial displacement of CP was measured from LVDTs (6–9 in Figure 6). The value of axial strain of CP was the ratio between the average displacement LVDTs (6–9 in Figure 6) and the length of the CP (1702 mm). The steel frame remained within the elastic limit during the test and its maximum tensile and compression lateral forces were measured as 170 kN and 154 kN, respectively. In addition, the maximum tensile and compression lateral forces of BRBF were measured as 677 kN and 788 kN, respectively. The out-of-plane displacement of BRBF and slip between the BRB and gusset plate are summarized in

Table 4. Out-of-plane displacement and slip between the BRB and gusset plate.

Slip (mm)					Out-of-Plane Displacement (mm)
LVDTs (in Figure 6)					LVDTs (in Figure 6)
	10	11	12	13	16	17
Max.	0.065	0.075	0.098	0.08	1.08	1.08
Min.	−0.005	−0.033	−0.048	−0.072	−1.06	−1.06

.

The compression strength adjustment factor (β) and strain hardening factor (ω) for BRB was determined by AISC [44]. The β and ω factors are defined as follows:

$$P_{C, BRB}=\beta \times \omega \times P_{y, BRB}$$

(6)

$$P_{T, BRB}=\omega \times P_{y, BRB}$$

(7)

$$P_{y, BRB}=F_{y, BRB}\times A_{ BRB}$$

(8)

where F_y,BRB is the yield stress of CP, A_BRB is the cross-section area of CP, and P_C,BRB and P_T,BRB are the compression and tension strength of BRB, respectively. As can be seen in Figure 14, the value of β was determined to be 1.16. At the end of the test, the steel frame remained within the elastic limit and high mode buckling was observed on the CP (see Figure 15).

4.2. FEM Results

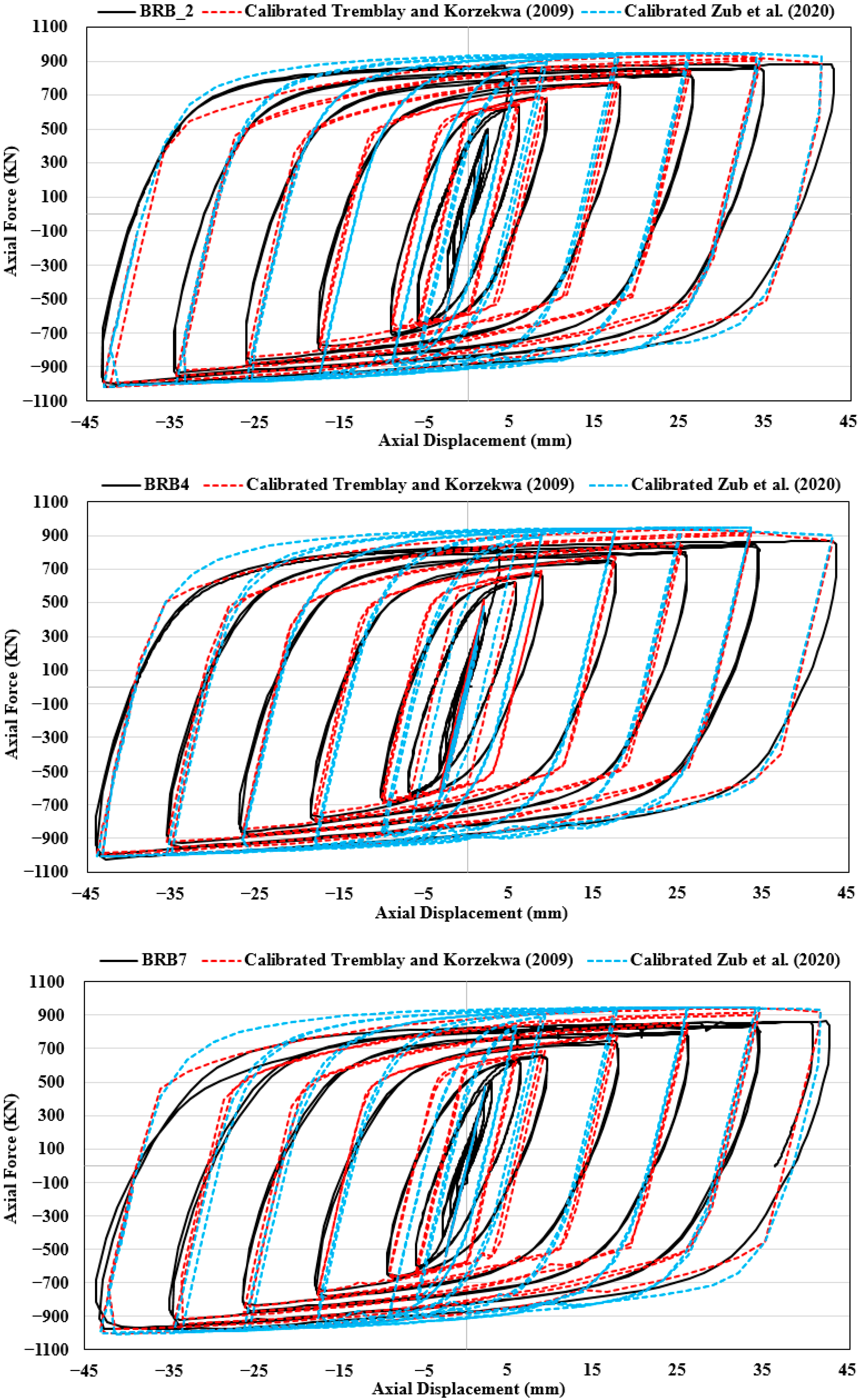
4.2.1. The BRBs

The different values of the friction coefficient (0.05, 0.1, and 0.2) for the tangential behavior in the numerical analysis were used to observe their effects on the numerical cyclic response of BRB2. In addition, the material models [27,41] were calibrated by matching the test result of BRB2. Figure 16 shows the effects of both calibrated material models and friction coefficients on the numerical hysteretic behavior of BRBs. The numerical cyclic behavior of BRB2 exhibited good agreement with the test results when the value of the friction coefficient between the CP and CM was taken to be 0.05. Both calibrated material models gave approximately identical numerical results for all BRBs [2] (see Figure 17).

4.2.2. Steel Frame

The effect of the value of the friction coefficient between the contact surfaces of steel plates of the slip-critical connections on the numerical cyclic behavior of the steel frame with slip-critical connection is presented in Figure 18. As can be seen in Figure 18, when the value of the friction coefficient was set to 0.2, the numerical result was in good agreement with the test result.

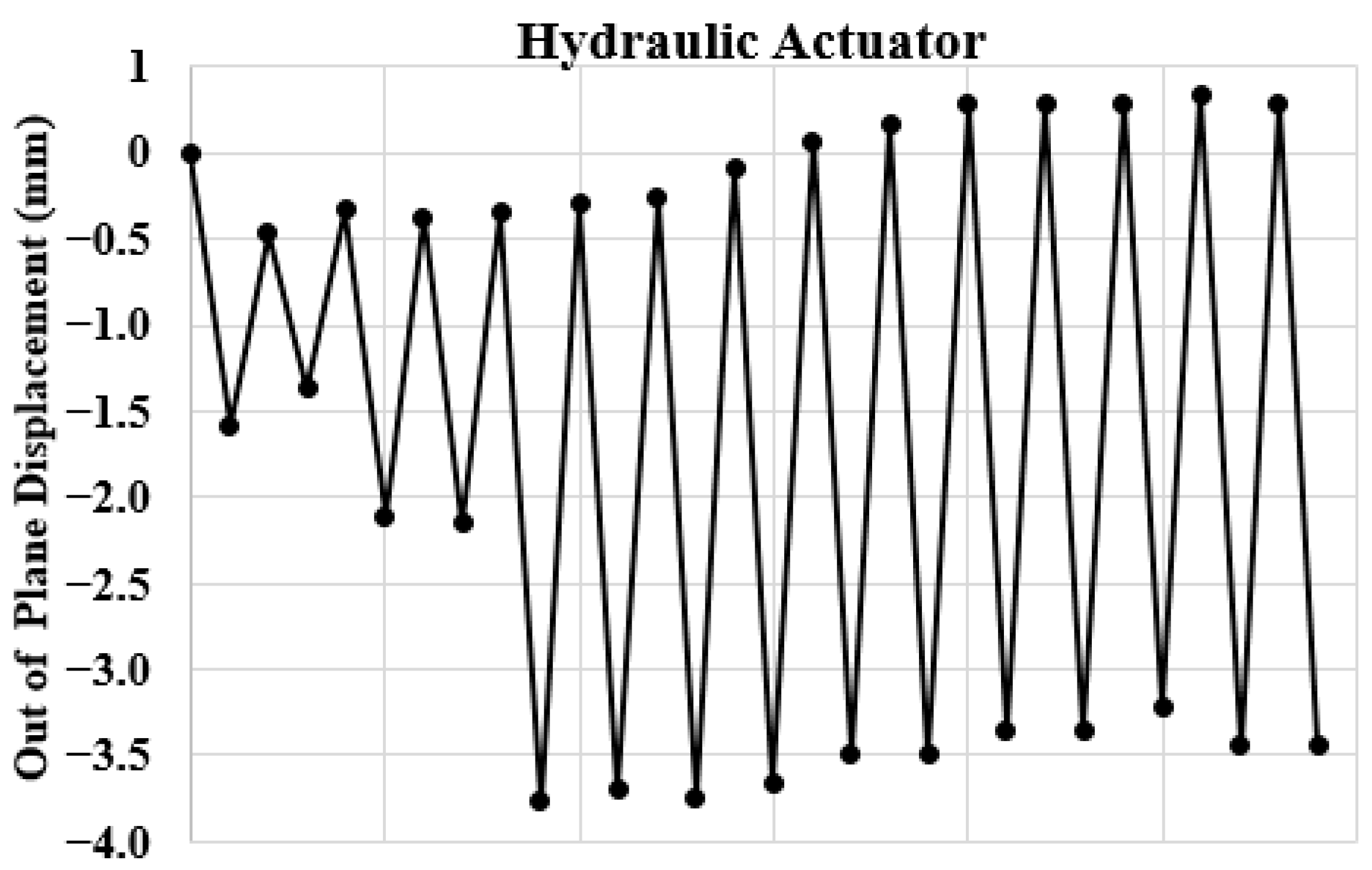
4.2.3. The BRBF

In this phase, the pretension load was applied to 10.9 M16 high-strength bolts, which were used for slip-critical connections of the steel frame, and the slips on the steel plates used for the slip-critical connections of the steel frame were not measured during the test. Therefore, the effect of the pretension load was investigated on the numerical hysteretic behavior of BRBF. In addition, a comparison of the numerical results of calibrated material models with the test result is also given in Figure 19. In addition, the R² scores for both calibrated material models were determined by the quantitative statistical error analysis to clarify their ability to predict the test result. As this score has a high value, the material model has good prediction abilities. The maximum and minimum lateral forces corresponding to each IDR defined in the loading protocol and values of R² scores for both calibrated material models are summarized in

Table 5. The comparison between the numerical analysis and the test results.

Demands	Calibrated Korzekwa and Tremblay [46]					Calibrated Zub et al. [36]
Tension	IDR	Max. Lateral Force (KN)		Error	R2	Drift Ratio	Max. Lateral Force (KN)		Error	R2
		Exp.	Num.				Exp.	Num.
	1.00%	471	529	12.31%	92.21%	1.00%	471	546	15.92%	87.36%
	1.50%	592	638	7.77%		1.50%	592	627	5.91%
	2.00%	617	624	1.13%		2.00%	617	633	2.59%
	2.50%	677	669	1.18%		2.50%	677	668	1.33%
Compression	1.00%	576	585	1.56%		1.00%	576	651	13.02%
	1.50%	612	652	6.54%		1.50%	612	678	10.78%
	2.00%	660	683	3.48%		2.00%	660	697	5.61%
	2.50%	737	718	2.57%		2.50%	737	719	2.44%
	3.00%	788	726	7.87%		3.00%	788	735	6.73%

.

4.3. Discussion

The value of β should not exceed 1.3, according to the AISC [44]. The value of β was determined as 1.16 from Equations (6)–(8). If this value was lower than 1.3, it indicated that the value of the friction coefficient between the CP and CM was reasonable. The maximum tensile and compression strains of the CP were measured as 1.75% and 2.69%, respectively. Although the BRB resisted up to 2.69% axial compression demands, local and global deformation did not occur on it.

The numerical results demonstrated that the kinematic and isotropic hardening parameters had a significant effect on the hysteretic behavior of BRB. When the value of the friction coefficient was increased, it led to compression over-strength and less ductility for the BRBs. While the friction coefficient between the contact surfaces of the steel plates of slip-critical connections in the steel frame had major effects on the numerical results, the calibrated material models had minor effects on it as the steel frame with slip-critical connections was within the elastic limit during the test.

The pretension load had a major effect on the tensile and compression strength of BRBF. While the pretension load of 35 kN predicted the cyclic response of BRBF under the tension demands well, the pretension load of 75 KN predicted its cyclic response under the compression demands well. Both calibrated material models had almost similar hysteretic predictions for the FEM of BRBF. However, the calibrated Tremblay and Korzekwa [46] material model was better matched with the test results than the calibrated Zub et al. [36] material model.

Ostovar and Hejazi [64] performed FE analysis using the isotropic hardening steel material model for CP on the BRBs. However, even if this material model performs an acceptable prediction for the cyclic behavior of steel material, it does not have a better predictive ability than the combined isotropic–kinematic hardening steel material model. The finite element analyses in this paper and the literature [35,36,38,46,65,66] confirmed this phenomenon. Many studies [36,51,52] demonstrated that the finite element analysis adopting the C3D8I element can more accurately predict the experimental results because it can overcome the shear locking effect. Moreover, the actual normal and tangential behaviors of contact pairs in both BRB and slip-critical connection were properly predicted by hard contact and penalty friction, respectively [47,48,49,50]. For these reasons, the numerical results that predicted experimental behaviors well in this paper and the past studies demonstrated the accuracy of the material and contact models used in all FEMs.

5. Conclusions

The aim of this study was to both experimentally and numerically investigate the behavior of BRBFs. The non-linear FE model was developed and validated against the experimental results. The following conclusions can be drawn based on the results of experimental and numerical studies observed.

• Although the BRB inside the steel frame was subjected to 2.69% strain of the CP under the axial compression demands, local and global deformations were not observed. For this reason, it provided symmetrical hysteretic behavior to the steel frame. The construction details of the BRB are hence acceptable. This can be useful for further studies and design provisions.
• The test results indicated that the well-detailed BRBF can resist significant drift demands, while the BRB exhibited significant yielding without severe damage. This is important since BRBFs in critical applications where drift demands play a vital role can offer more resilience.
• The parametric study based on material parameters indicated that the isotropic and kinematic hardening parameters had a significant effect on the hysteretic behavior of the BRB. Therefore, a fatigue test needs to be performed together with coupon tests to determine material parameters of the CP. This approach can greatly enhance the resistance of BRB against dynamic loading as well as provide a safer design.
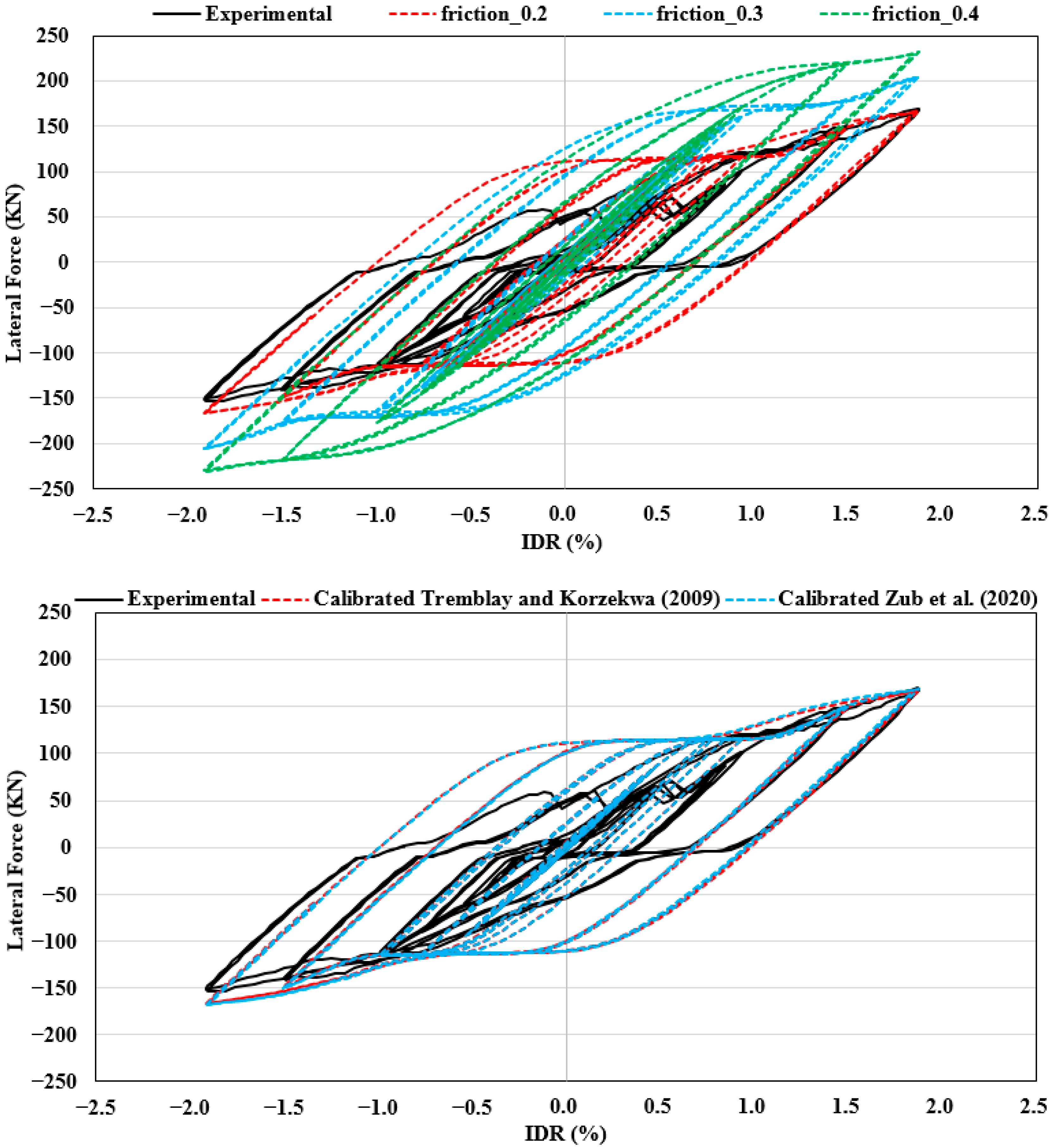
• The sufficient pretension load applied to bolts in the FEM of the steel frame resulted in no slip in the bolted connection. Therefore, the value of the friction coefficient was determined from the ultimate load capacity of the steel frame. The value of the friction coefficient between the contact surfaces of steel plates seems to be determined experimentally for further studies. Furthermore, the pretension load also has significant effects on the hysteretic behavior of the BRBF.
• The value of the friction coefficient between the CP and the CM in the FEMs of BRBs and between the contact surfaces of steel plates in the FEM of the steel frame was taken as 0.05 and 0.2, respectively. The best numerical results were obtained for the BRBs and the steel frame by using these values. In addition, while the pretension load of 75 kN provided the best numerical behavior for the BRBF under the compression demands, its behavior under the tension demands was obtained by the pretension load of 35 kN.
• The steel frame and BRBF were modeled by using a micro-modeling technique. The numerical results indicated that the numerical hysteretic behavior of the steel frame and BRBF was highly affected by the pretension load applied to bolts and the value of the friction coefficient between the contact surfaces of steel plates, which were parameters of micro-modeling techniques. Both calibrated material models by Korzekwa and Tremblay [46] and Zub et al. [36] provided approximately the same numerical hysteretic response for all the FEMs. The developed and validated FE model can be used as a tool to predict the structural behavior of BRBFs.
• The numerical investigations of the behavior of BRBF in the literature are generally focused on the friction between the CP and CM. This paper has demonstrated that the numerical behavior of the BRBF is significantly affected not only by the friction between the CP and CM but also by the friction between contact surfaces of steel plates in the slip-critical connections, the pretension load of bolts, vertical displacements of the support, and the out-of-plane displacements of the frame.
• In this study, the subassembly test of BRB, whose geometrical properties are identical to BRB4 tested by Ozcelik et al. [2], was performed. The test results demonstrated that the friction between CP and CM was acceptable and the BRB had stable hysteretic behavior. For this reason, it is thought that the BRB tested in this paper can constitute a basis for its practice as an energy damper in the structures.