A Confocal Ellipsoidal Densification Model for Estimating Improvement Effects on Soil Under Dynamic Compaction
Abstract
:1. Introduction
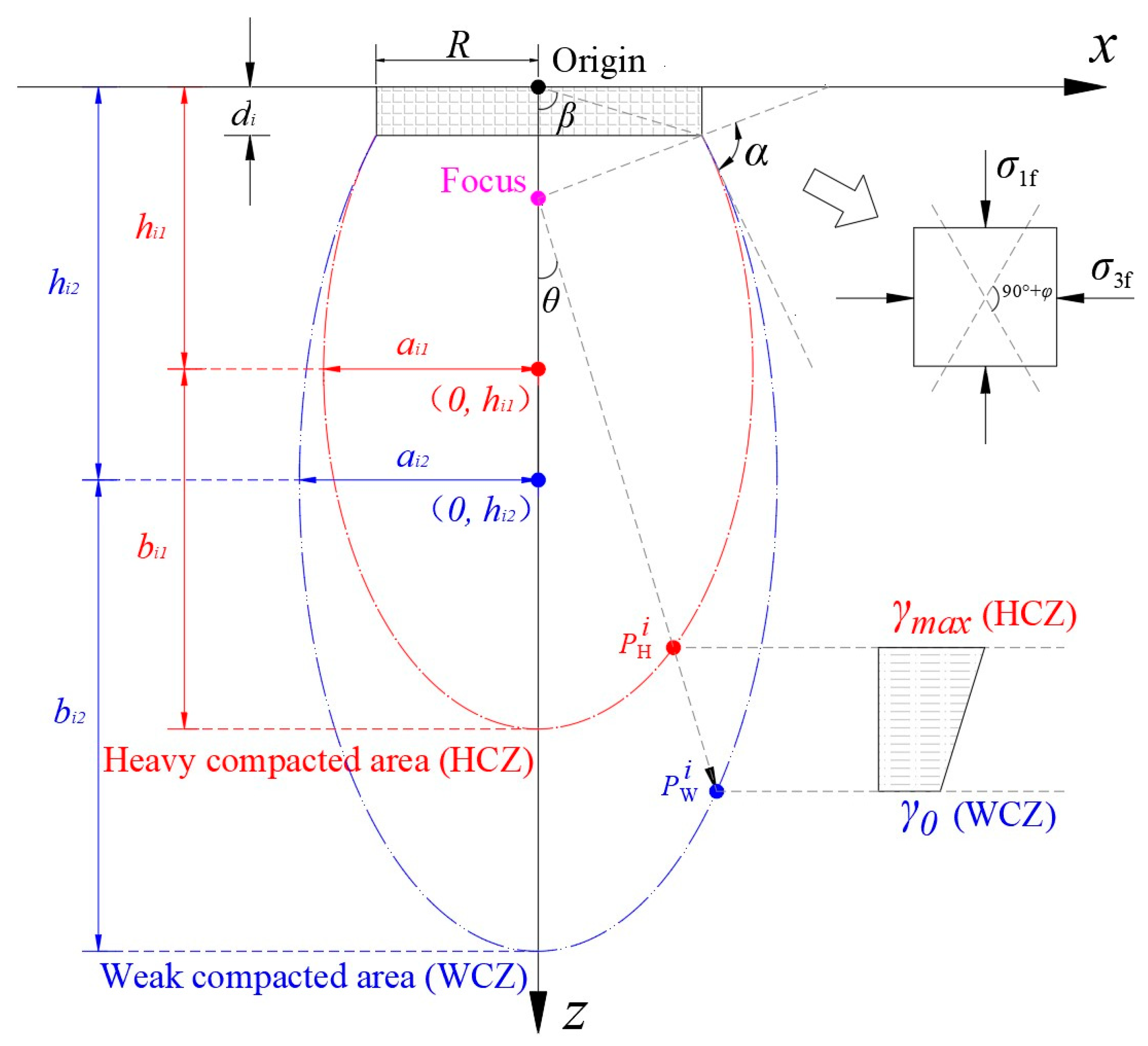
2. Confocal Ellipsoidal Densification Model (CEDM)
2.1. Description of CEDM
2.2. Ellipsoid Equation for CEDM
2.3. Mass Balance Equation for CEDM
2.4. Solution of Ellipsoid Parameters for Different Compacted Zones
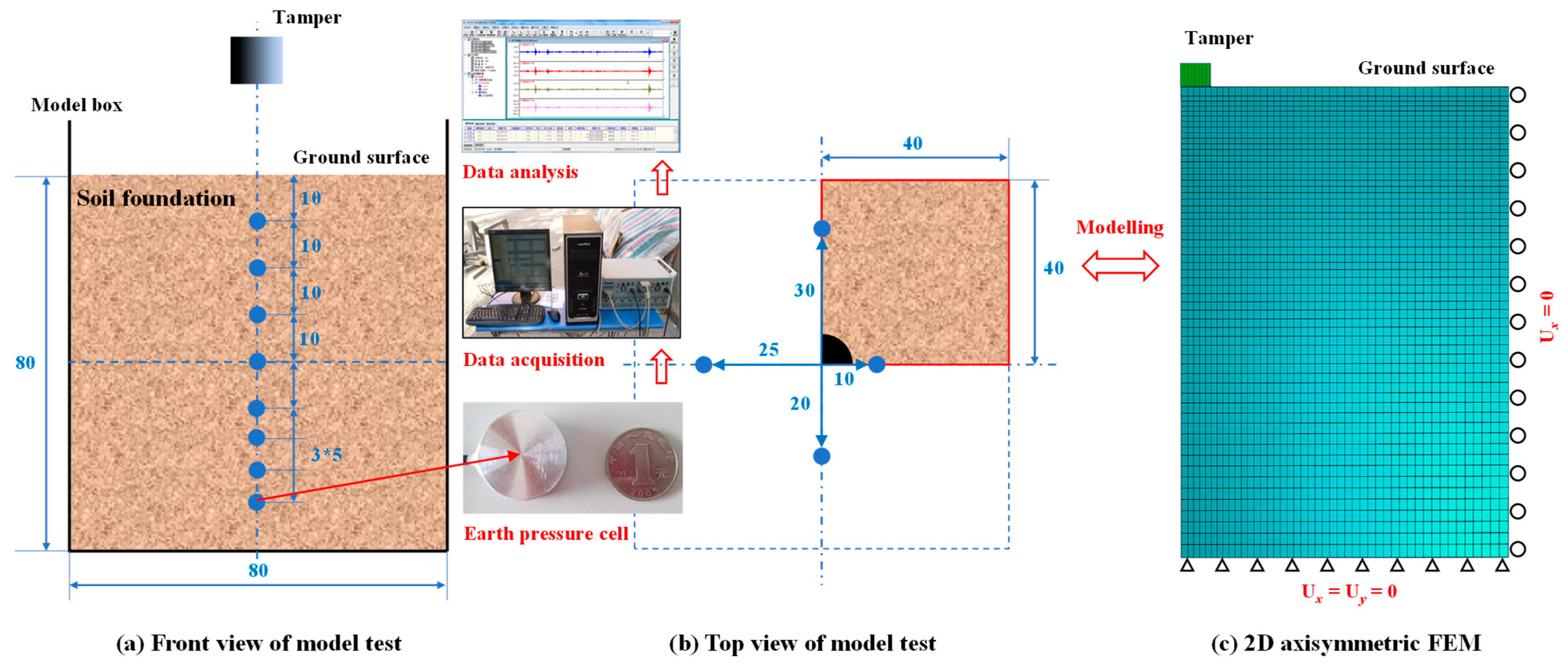
3. Validation of Proposed CEDM
3.1. Model Test and Numerical Simulation
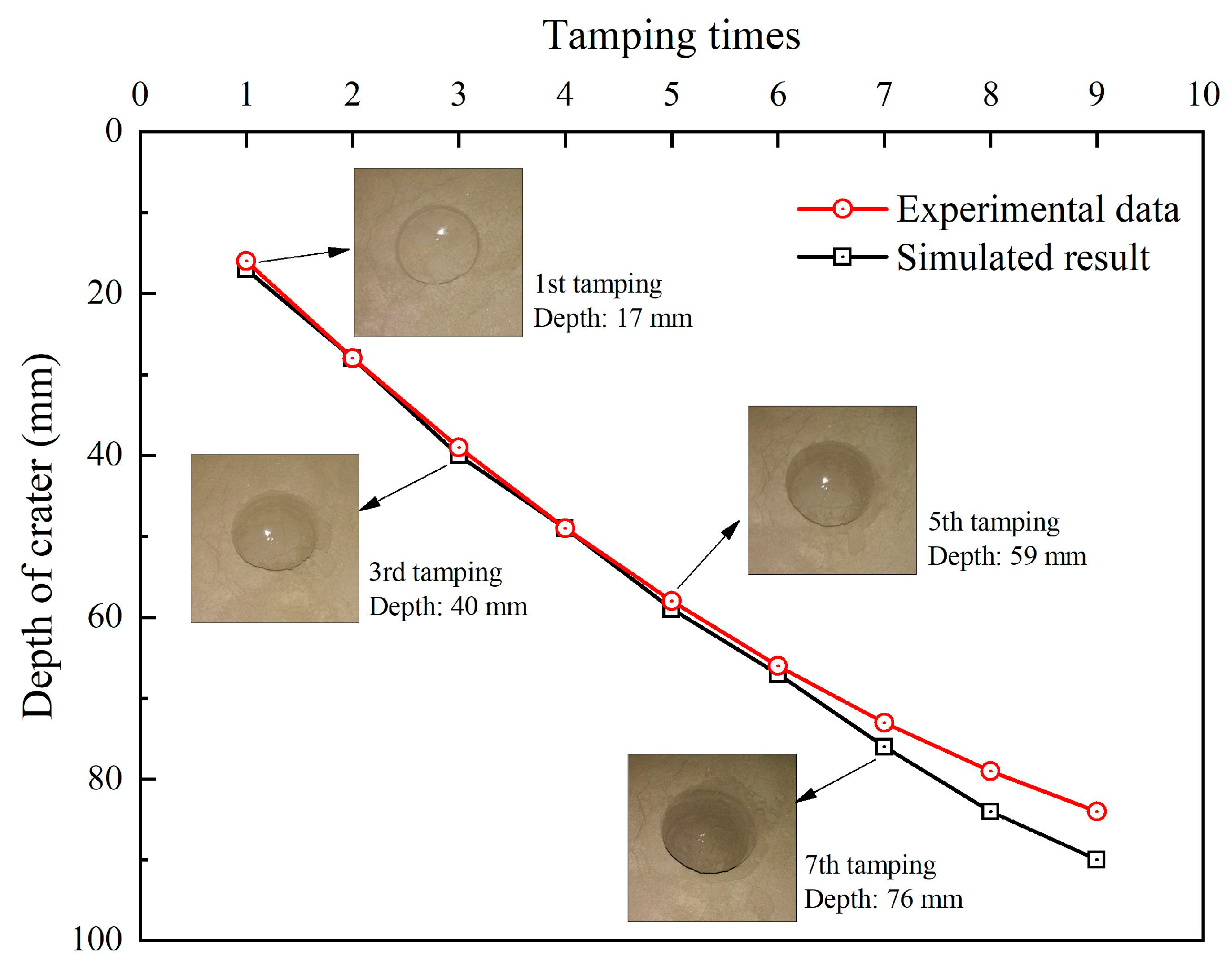
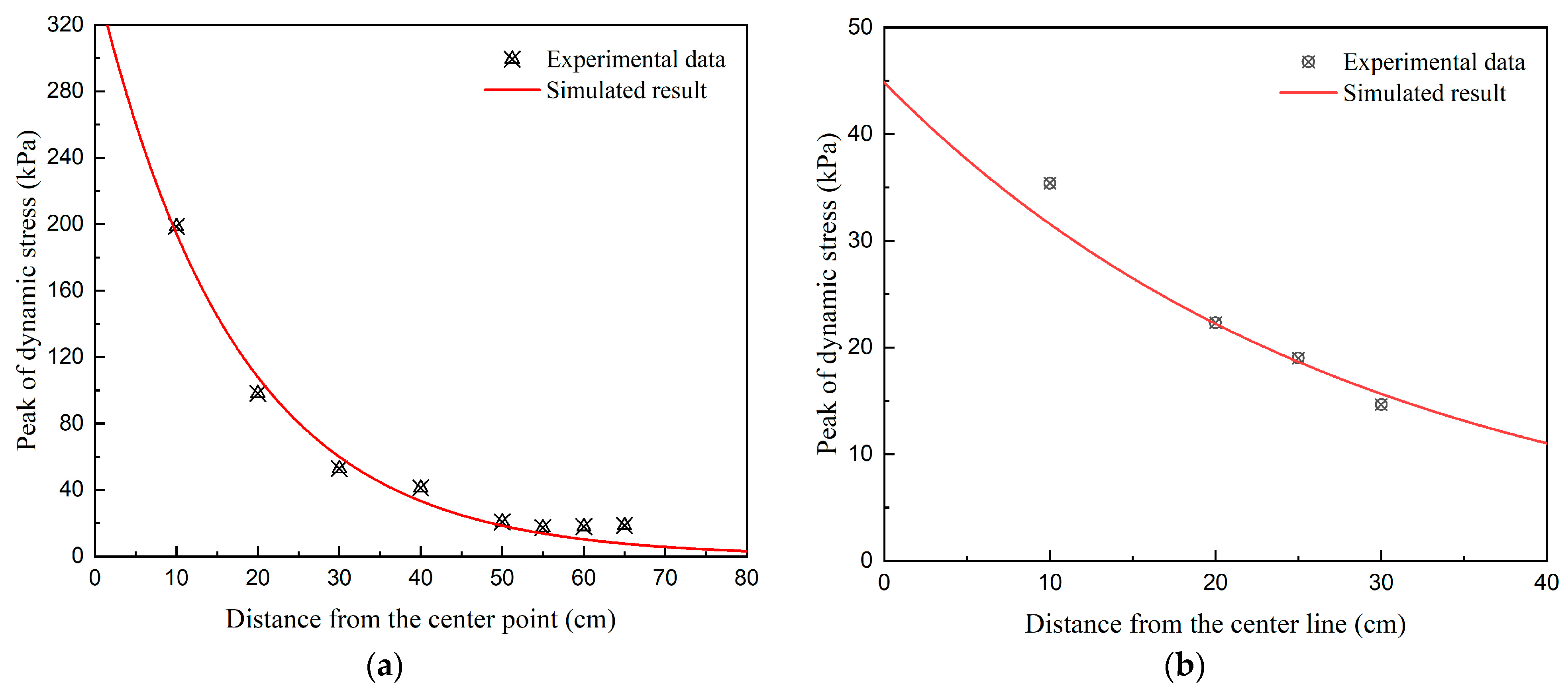
3.2. Results and Analysis
3.3. Proposed CEDM for Estimating Improvement Range
3.4. Discussion of Proposed CEDM for Future Research
4. Conclusions
- A CEDM composed of HCZ and WCZ was proposed for describing the subarea characteristics of improvement ranges of soil foundations under DC. Based on the confocal assumption of HCZ and WCZ ellipses, a mass balance equation considering variations in soil dry density in different compacted zones was established. Six available parameters, including radius of tamper, crater depth, initial dry density and its corresponding friction angle, and maximum dry density and its corresponding friction angle, were selected to solve the HCZ and WCZ ellipsoidal parameters (center point, major and minor axes).
- The development pattern of the crater depth obtained by FEM simulations was consistent with the measured results, and the relative errors between the simulated and measured values under different tamping times were within ±10%. In addition, the same attenuation laws for peak dynamic stresses in the vertical and horizontal directions were observed. The simulated peak dynamic stresses at specific positions were close to the measured values, and their relative errors remained consistently below 15%. These results demonstrated that the established FEM exhibits reliable capability for simulating the DC process and can be further used for verifying the applicability of the proposed CEDM.
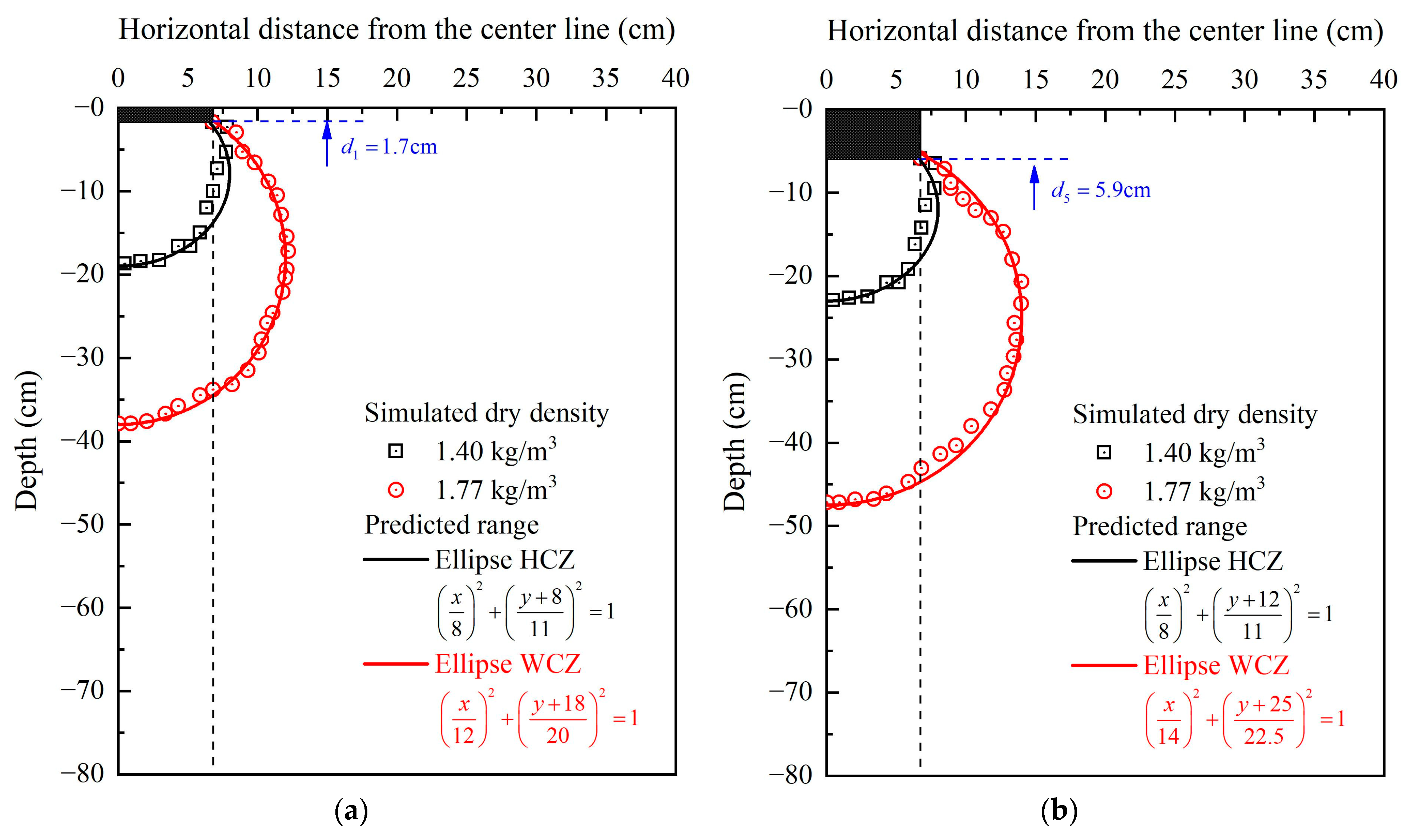
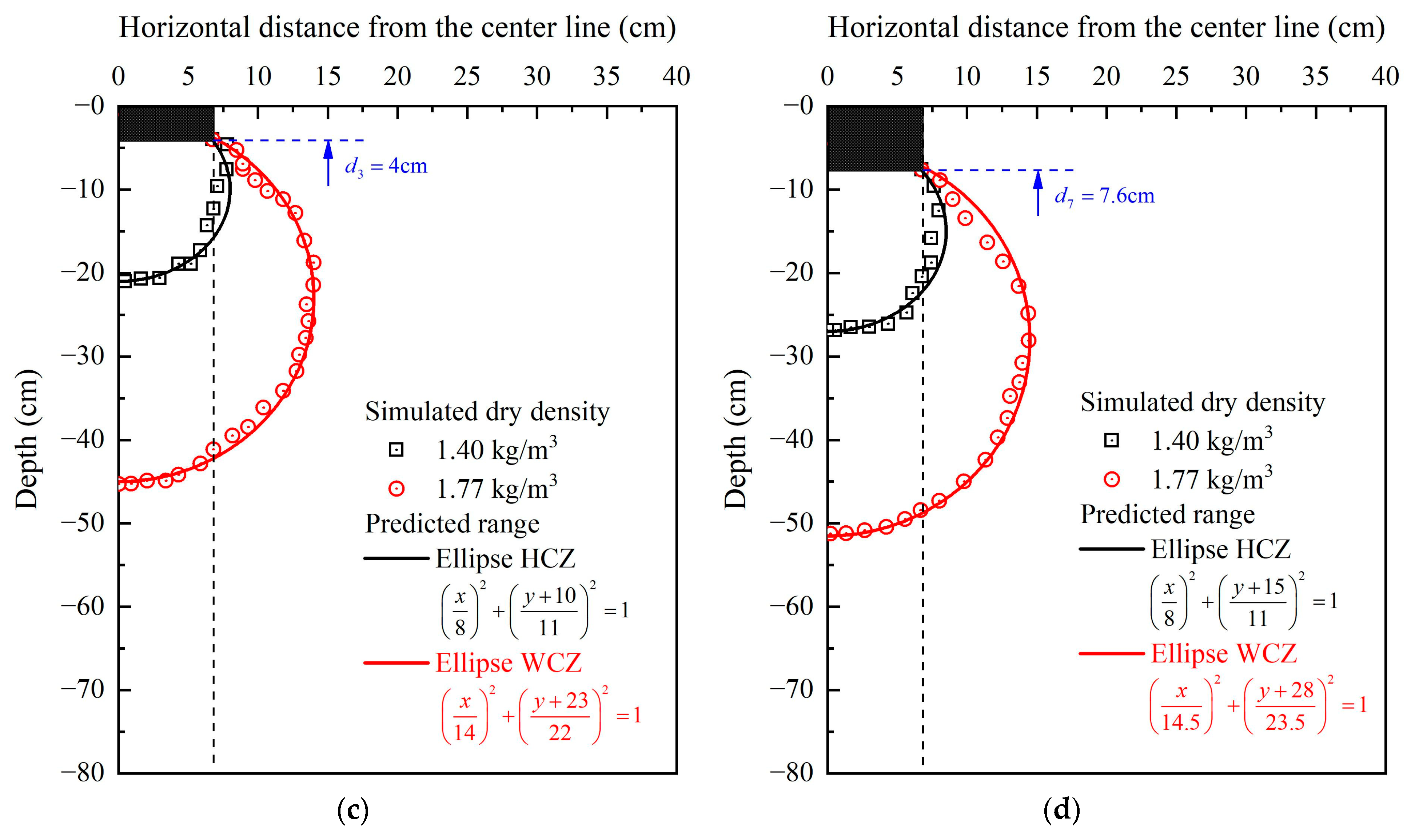
- Because the edge of the densification ellipsoid was assumed to pass through the corner point of the tamper or crater, HCZ and WCZ ellipses tended to underestimate improvement in lateral extent in this area. However, the overall compacted zones corresponding to initial and maximum dry densities after the first, third, fifth, and seventh tampings obtained from simulations were well fitted by the predicted HCZ and WCZ ellipses, with fitting degrees of over 90%. These results indicated the feasibility of the proposed CEDM for estimating improvement ranges of soil foundations caused by DC.
- With increases in tamping times, the HCZ ellipse moved down in the vertical direction without volumetric expansion, while the WCZ ellipse expanded along both depth and lateral directions. Specifically, as the number of tampings increased from one to seven, the HCZ ellipse moved downward by 7 cm, while the major and minor axes remained unchanged. At the same time, the center point of WCZ ellipse moved downward by 10 cm, while the major axes increased from 20 cm to 23.5 cm and the minor axes increased from 12 cm to 14.5 cm. These findings indicated that successive tamping mainly contributed to WCZ expansion, with an average area expansion rate of approximately 7%.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| DC | Dynamic Compaction |
| CEDM | Confocal Ellipsoidal Densification Model |
| HCZ | Heavy Compacted Zone |
| WCZ | Weak Compacted Zone |
| FEM | Finite Element Model |
References
- Menard, L.; Broise, Y. Theoretical and practical aspects of dynamic consolidation. Geotechnique 1975, 25, 3–18. [Google Scholar] [CrossRef]
- Leonards, G.A.; Holtz, R.D.; Cutter, W.A. Dynamic compaction of granular soils. J. Geotech. Eng. 1980, 106, 35–44. [Google Scholar] [CrossRef]
- Mayne, P.W.; Jones, J.S.; Dumas, J.C. Ground response to dynamic compaction. J. Geotech. Eng. 1984, 10, 757–774. [Google Scholar] [CrossRef]
- Lukas, R.G. Dynamic compaction engineering considerations. In Grouting, Soil Improvement and Geosynthetics; ASCE: Reston, VA, USA, 2010; pp. 940–953. [Google Scholar]
- Zhang, P.C.; Wang, R. A study of dynamic consolidation depth in engineering practice. China Rock Soil Mech. 2000, 21, 76–78. [Google Scholar] [CrossRef]
- Feng, S.J.; Shui, W.H.; Gao, L.Y.; He, L.J.; Tan, K. Field studies of the effectiveness of dynamic compaction in coastal reclamation areas. Bull. Eng. Geol. Environ. 2010, 69, 129–136. [Google Scholar] [CrossRef]
- Feng, S.J.; Tan, K.; Shui, W.H.; Zhang, Y. Densification of desert sands by high energy dynamic compaction. Eng. Geol. 2013, 157, 48–54. [Google Scholar] [CrossRef]
- Poran, C.J.; Rodriguez, J.A. Design of dynamic compaction. Can. Geotech. J. 1992, 29, 796–802. [Google Scholar] [CrossRef]
- Poran, C.J.; Rodriguez, J.A. Finite element analysis of impact behavior of sand. Soils Found. 1992, 32, 68–80. [Google Scholar] [CrossRef]
- Oshima, A.; Takada, N. Effect of ram momentum on compaction by heavy tamping. In Proceedings of the 13th International Conference on Soil Mechanics and Foundation Engineering, New Delhi, India, 5–10 January 1994; pp. 1141–1144. [Google Scholar]
- Oshima, A.; Takada, N. Relation between compacted area and ram momentum by heavy tamping. In Proceedings of the 14th International Conference on Soil Mechanics and Foundation Engineering, Hamburg, Germany, 6–12 September 1997; pp. 1641–1644. [Google Scholar]
- Li, X.; Lu, Y.; Cui, Y.; Qian, G.; Zhang, J.; Wang, H. Experimental investigation into the effects of tamper weight and drop distance on dynamic soil compaction. Acta Geotech. 2024, 19, 2563–2578. [Google Scholar] [CrossRef]
- Lee, F.H.; Gu, Q. Method for estimating dynamic compaction effect on sand. J. Geotech. Geoenviron. Eng. 2004, 130, 139–152. [Google Scholar] [CrossRef]
- Yao, Y.P.; Zhang, Z.B. Reinforcement range of dynamic compaction based on volumetric strain. China Rock Soil Mech. 2016, 37, 2663–2671. [Google Scholar] [CrossRef]
- Wang, W.; Chen, J.J.; Wang, J.H. Estimation method for ground deformation of granular soils caused by dynamic compaction. Soil Dyn. Earthq. Eng. 2017, 92, 266–278. [Google Scholar] [CrossRef]
- Miller, G.F.; Pursey, H. On the partition of energy between elastic waves in a semi-infinite solid. In Proceedings of the Royal Society of London; Royal Society: London, UK, 1955; pp. 55–69. [Google Scholar] [CrossRef]
- He, C.M.; Zou, J.F.; Li, L. Field test on measurement of dynamic stress of dynamic compaction. China J. Geotech. Eng. 2007, 29, 628–632. [Google Scholar] [CrossRef]
- Chow, Y.K.; Yong, D.M.; Yong, K.Y.; Lee, S.L. Dynamic compaction analysis. J. Geotech. Eng. 1992, 118, 1141–1157. [Google Scholar] [CrossRef]
- Chow, Y.K.; Yong, D.M.; Yong, K.Y.; Lee, S.L. Dynamic compaction of loose granular soils: Effect of print spacing. J. Geotech. Eng. 1994, 120, 1115–1133. [Google Scholar] [CrossRef]
- Cheshomi, A. Lateral Extent and Environmental Effects of Dynamic Compaction in a Coastal Area of Southern Iran. Environ. Eng. Geosci. 2019, 25, 203–211. [Google Scholar] [CrossRef]
- Ghassemi, A.; Pak, A.; Shahir, H. Numerical study of the coupled hydro-mechanical effects in dynamic compaction of saturated granular soils. Comput. Geotech. 2010, 37, 10–24. [Google Scholar] [CrossRef]
- Wen, S.Q.; Li, Y.P.; Ma, K. Research on inversion of energy efficiency and compacted and affected scope of dynamic compaction. China Rock Soil Mech. 2015, 36, 185–192. [Google Scholar] [CrossRef]
- Dou, J.Z.; Chen, J.J.; Wang, W. Method for estimating the degree of improvement in soil between adjacent tamping locations under dynamic compaction. Int. J. Geomech. 2019, 19, 04019134. [Google Scholar] [CrossRef]
- Yao, Z.; Zhou, C.; Lin, Q.Q.; Yao, K.; Satchithananthan, U.; Lee, F.H.; Tang, A.M.; Jiang, H.; Pan, Y.; Wang, S.T. Effect of dynamic compaction by multi-point tamping on the densification of sandy soil. Comput. Geotech. 2022, 151, 104909. [Google Scholar] [CrossRef]
- Zhou, C.Y.; Yao, K.; Rong, Y.; Lee, F.; Zhang, D.; Jiang, H.; Yang, C.; Yao, Z.; Chen, L. Numerical investigation on zone of improvement for dynamic compaction of sandy ground with high groundwater table. Acta Geotech. 2022, 18, 695–709. [Google Scholar] [CrossRef]
- Ma, Z.Y.; Dang, F.N.; Liao, H.J. Numerical study of the dynamic compaction of gravel soil ground using the discrete element method. Granul. Matter 2014, 16, 881–889. [Google Scholar] [CrossRef]
- Li, Y.; Ma, Z.; Yang, F. Numerical study on micro-reinforcement mechanism and environmental control of gravel soil under dynamic compaction. Arab. J. Geosci. 2021, 14, 2682. [Google Scholar] [CrossRef]
- Li, Y.; Fang, Y.; Yang, Z. Two criteria for effective improvement depth of sand foundation under dynamic compaction using discrete element method. Comput. Part. Mech. 2022, 10, 397–404. [Google Scholar] [CrossRef]
- Li, X.; Lu, Y.B.; Qian, G.P.; Yang, H.; Yu, H.N.; Wang, H.; Zhu, Z.H. A New Index for Estimating the Improved Depth of Dynamic Compaction. Int. J. Geomech. 2024, 24, 06023027. [Google Scholar] [CrossRef]
- Zhang, R.; Sun, Y.; Song, E. Simulation of dynamic compaction and analysis of its efficiency with the material point method. Comput. Geotech. 2019, 116, 103218. [Google Scholar] [CrossRef]
- Helwany, S. Applied Soil Mechanics with ABAQUS Applications; John Wiley and Sons: Hoboken, NJ, USA, 2007. [Google Scholar]


| ω (%) | ρ (kg/m3) | E (kPa) | c (kPa) | φ | χ | A (kPa) | B |
|---|---|---|---|---|---|---|---|
| 32.1 | 1850 | 5000 | 8 | 12.5 | 0.8 | 0.0002 | 0.4 |
| ω (%) | ρ (kg/m3) | E (kPa) | c (kPa) | φ | Dense Degree |
|---|---|---|---|---|---|
| 32.1 | 1850 | 5000 | 8 | 12.5 | Loose |
| 18.4 | 2100 | 10,000 | 8 | 30 | Dense |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shan, H.; Zhao, F.; Liu, X.; Sheng, K.; Xu, F. A Confocal Ellipsoidal Densification Model for Estimating Improvement Effects on Soil Under Dynamic Compaction. Appl. Sci. 2025, 15, 5292. https://doi.org/10.3390/app15105292
Shan H, Zhao F, Liu X, Sheng K, Xu F. A Confocal Ellipsoidal Densification Model for Estimating Improvement Effects on Soil Under Dynamic Compaction. Applied Sciences. 2025; 15(10):5292. https://doi.org/10.3390/app15105292
Chicago/Turabian StyleShan, Hao, Futian Zhao, Xin Liu, Ke Sheng, and Fenqiang Xu. 2025. "A Confocal Ellipsoidal Densification Model for Estimating Improvement Effects on Soil Under Dynamic Compaction" Applied Sciences 15, no. 10: 5292. https://doi.org/10.3390/app15105292
APA StyleShan, H., Zhao, F., Liu, X., Sheng, K., & Xu, F. (2025). A Confocal Ellipsoidal Densification Model for Estimating Improvement Effects on Soil Under Dynamic Compaction. Applied Sciences, 15(10), 5292. https://doi.org/10.3390/app15105292







