Biomechanical and Injury Tolerance Analysis of Tibial Movement Under Different Postures and Impact Loads
Abstract
1. Introduction
2. Methods and Materials
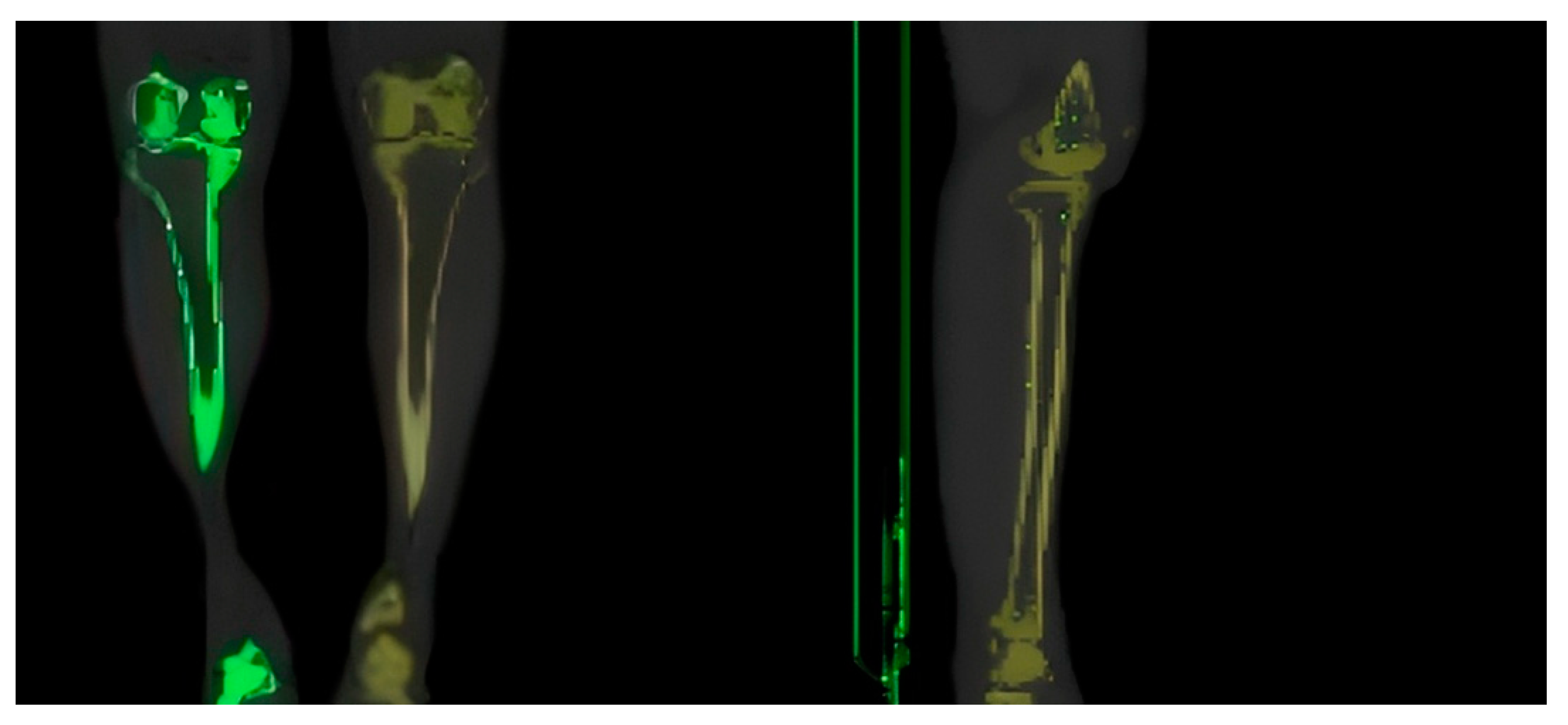
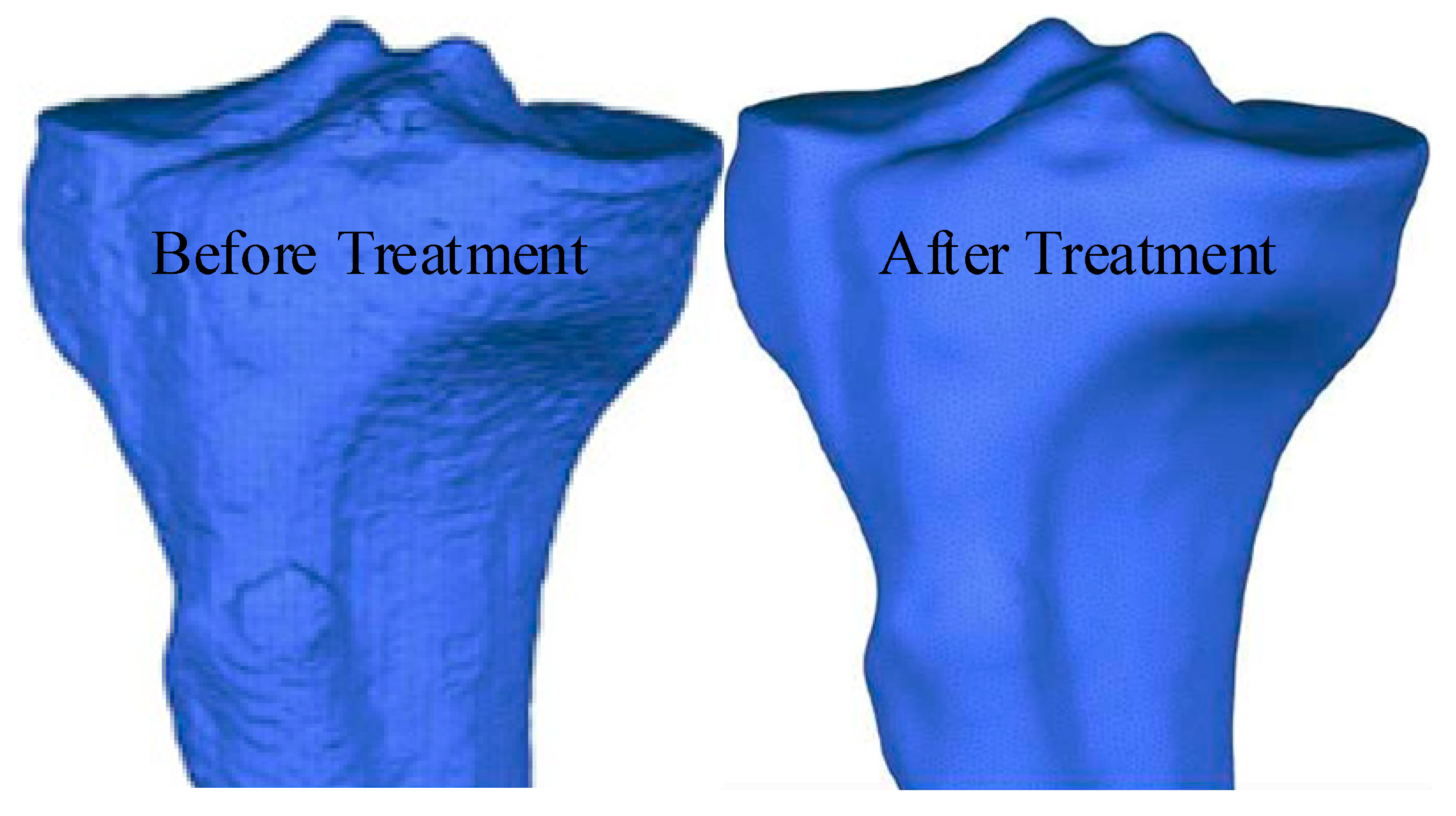
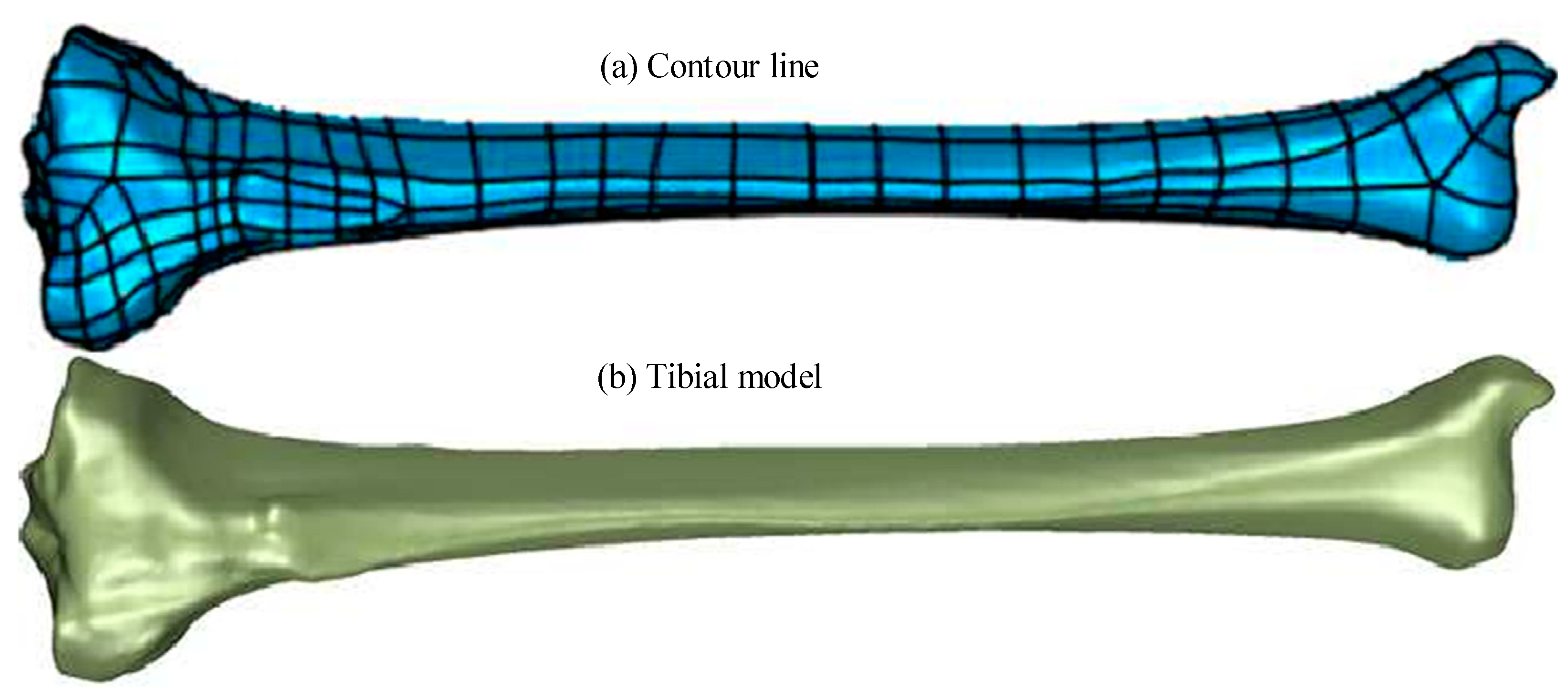
2.1. Construction of 3D Model of Human Tibia
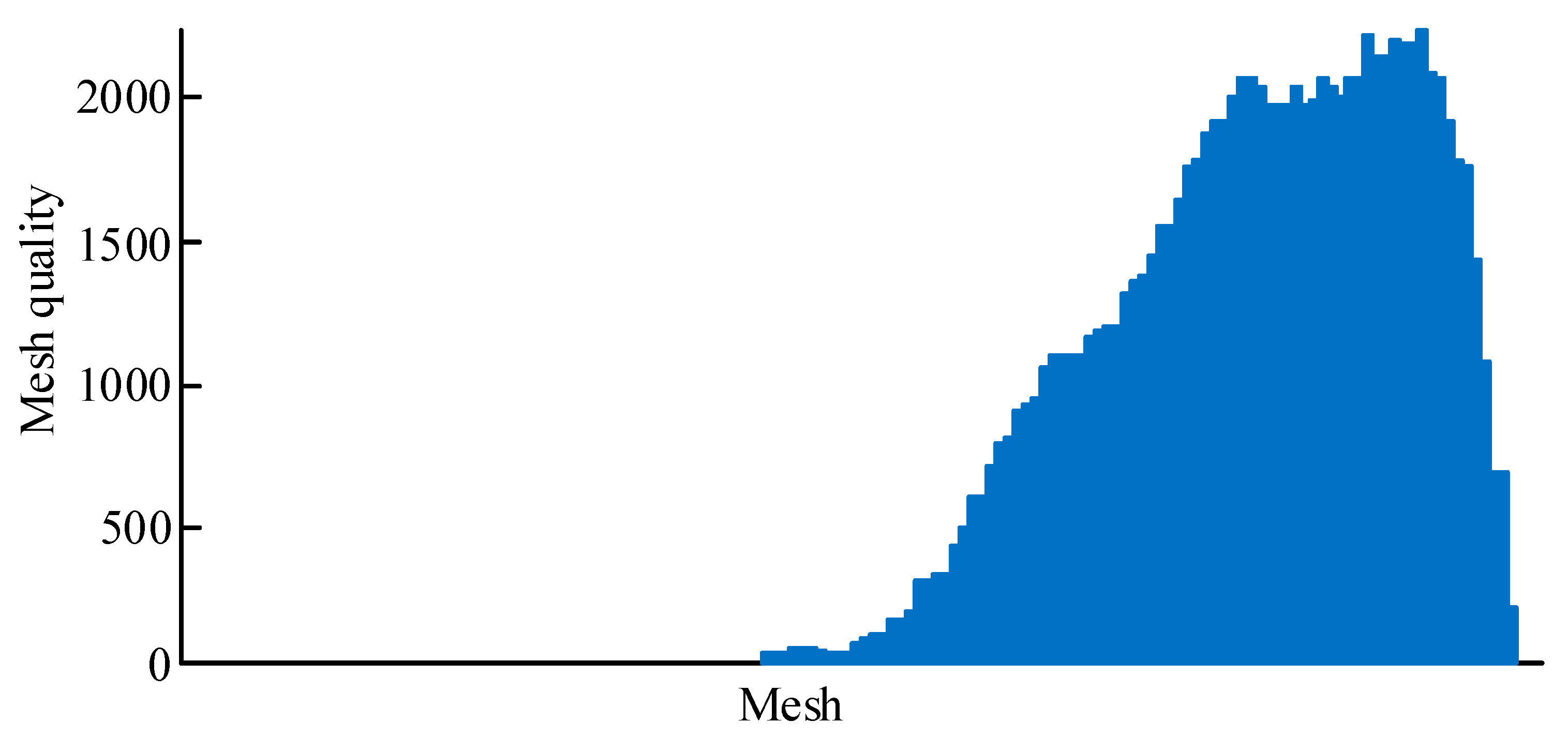
2.2. Construction of Finite Element Model of Human Tibia
3. Results
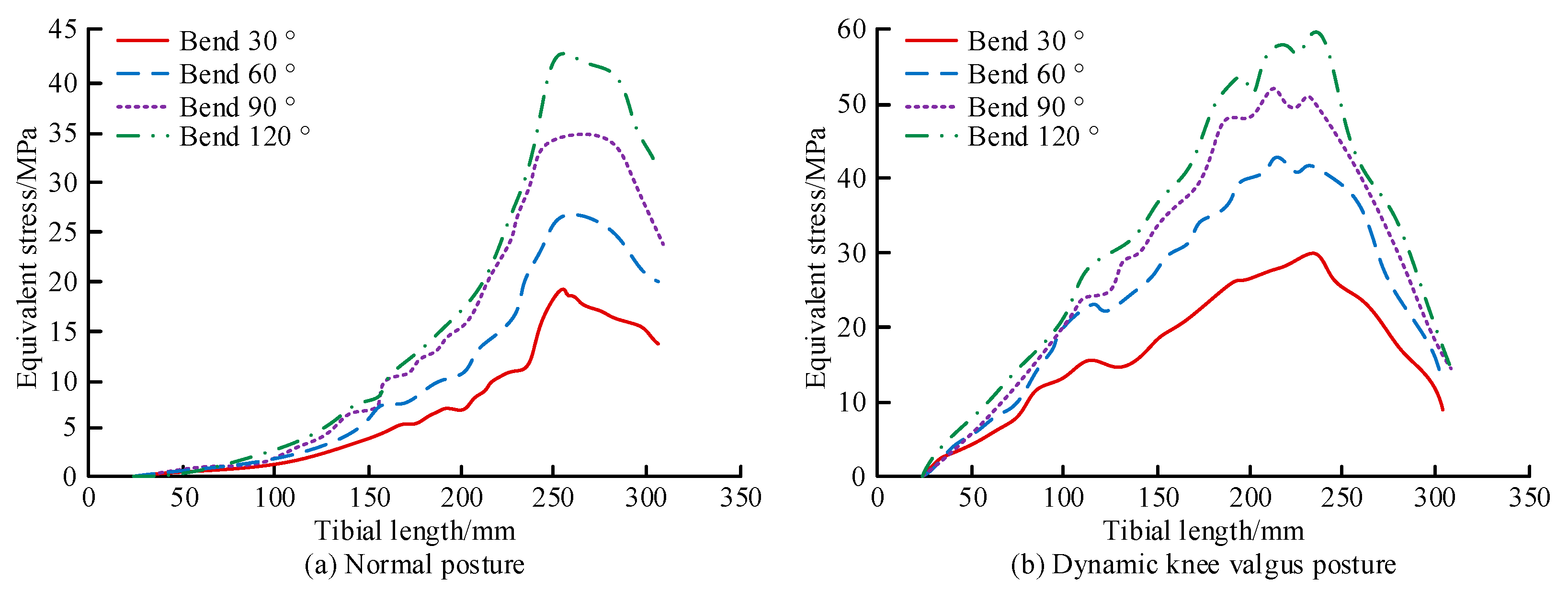
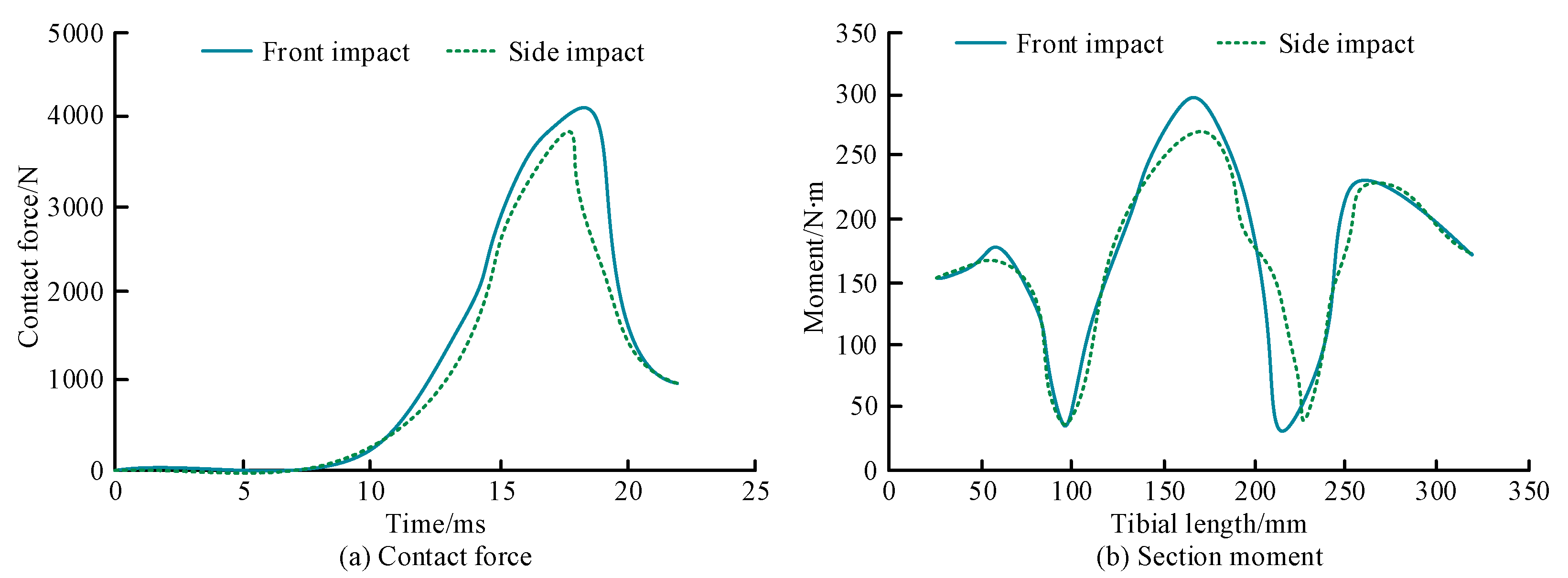
3.1. Biomechanical Analysis of Tibia Under Flexion Movement
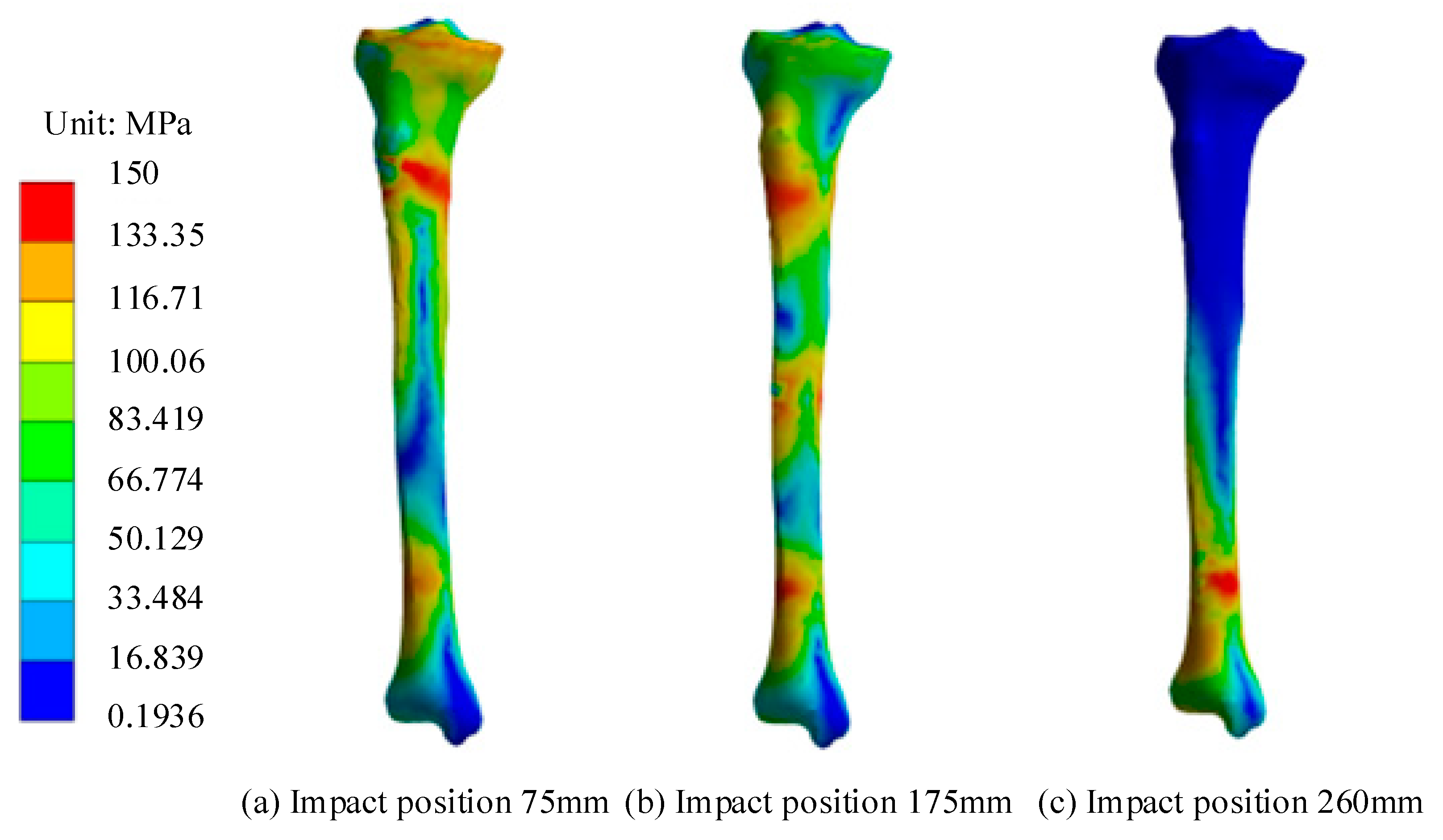
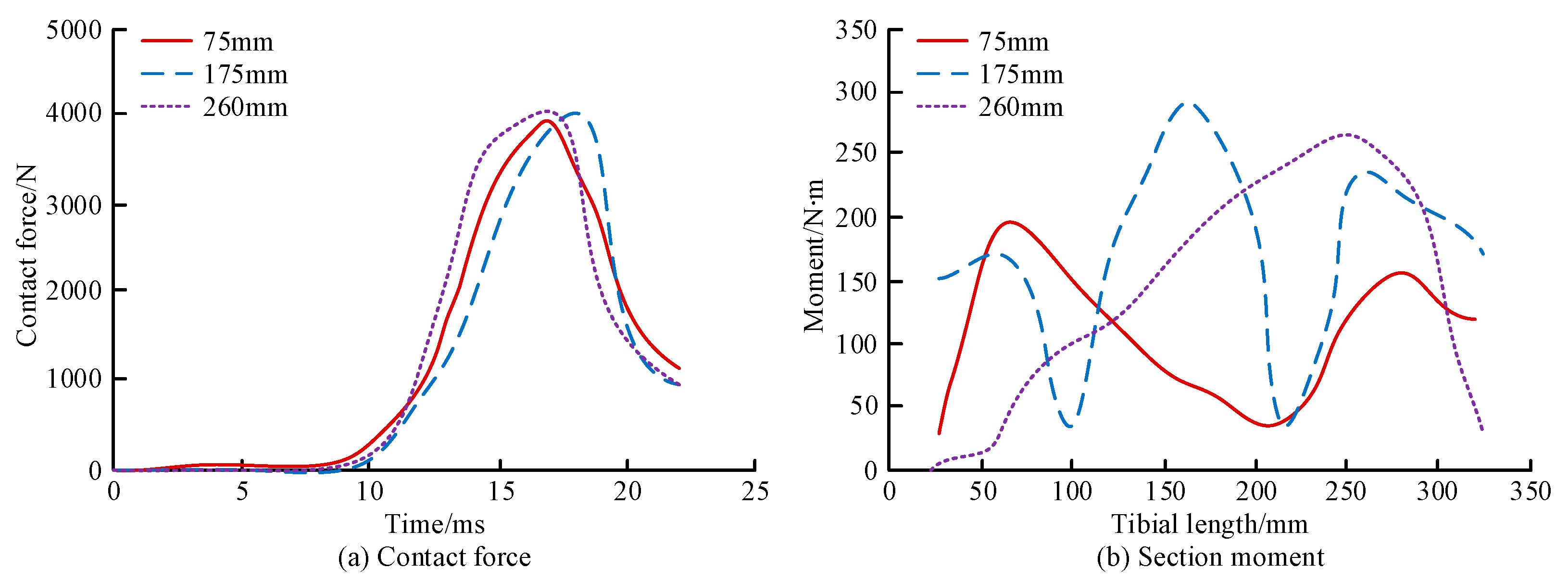
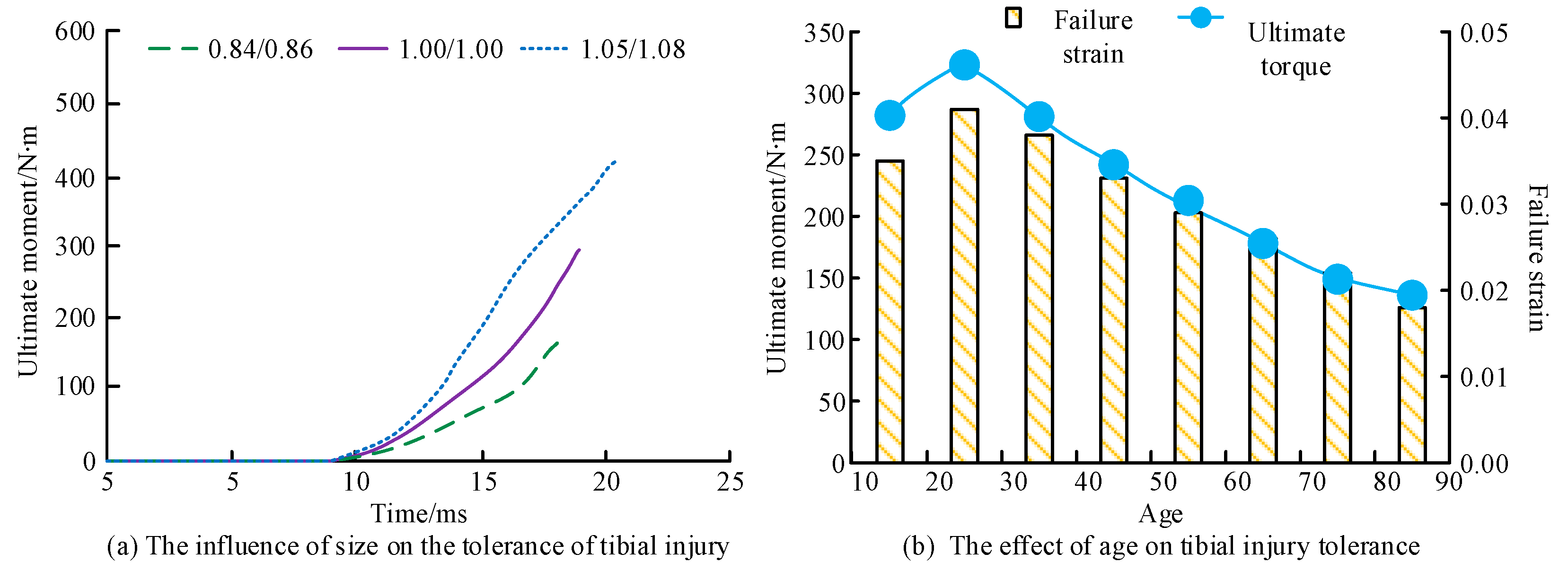
3.2. Analysis of Injury Tolerance of Human Tibia
4. Discussion
5. Conclusions
Author Contributions
Funding
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- He, Y.; Shao, S.; Fekete, G.; Yang, X.; Cen, X.; Song, Y.; Sun, D.; Gu, Y. Lower Limb Muscle Forces in Table Tennis Footwork during Topspin Forehand Stroke Based on the OpenSim Musculoskeletal iModel: A Pilot Study. Mol. Cell. Biomech. 2022, 19, 221–235. [Google Scholar] [CrossRef]
- Xu, P.; Shin, I. Preparation and Performance Analysis of Thin-Film Artificial Intelligence Transistors Based on Integration of Storage and Computing. IEEE Access 2024, 12, 30593–30603. [Google Scholar] [CrossRef]
- Minematsu, A.; Nishii, Y. Effects of Whole Body Vibration on Bone Properties in Growing Rats. Int. Biomech. 2022, 9, 19–26. [Google Scholar] [CrossRef] [PubMed]
- Maghami, E.; Moore, J.P.; Josephson, T.O.; Najafi, A.R. Damage Analysis of Human Cortical Bone under Compressive and Tensile Loadings. Comput. Methods Biomech. Biomed. Eng. 2022, 25, 342–357. [Google Scholar] [CrossRef]
- Hu, Y.; Peng, A.; Wang, S.; Pan, S.; Zhang, X. Flexion tibial plateau fractures: 3-dimensional CT simulation-based subclassification by injury pattern. Orthop. Surg. 2022, 14, 543–554. [Google Scholar] [CrossRef] [PubMed]
- Liu, Z.; Wang, S.; Tian, X.; Peng, A. The relationship between the injury mechanism and the incidence of ACL avulsions in Schatzker type IV tibial plateau fractures: A 3D quantitative analysis based on mimics software. J. Knee Surg. 2023, 36, 644–651. [Google Scholar] [CrossRef]
- Quevedo Gonzalez, F.J.; Steineman, B.D.; Sturnick, D.R.; Deland, J.T.; Demetracopoulos, C.A.; Wright, T.M. Biomechanical Evaluation of Total Ankle Arthroplasty. Part II: Influence of Loading and Fixation Design on Tibial Bone–Implant Interaction. J. Orthop. Res. 2021, 39, 103–111. [Google Scholar] [CrossRef]
- Liu, H.; Zhang, H.; Lee, J.; Xu, P.; Shin, I.; Park, J. Motor Interaction Control Based on Muscle Force Model and Depth Reinforcement Strategy. Biomimetics 2024, 9, 150. [Google Scholar] [CrossRef] [PubMed]
- Salvatore, G.; Berton, A.; Orsi, A.; Egan, J.; Walley, K.C.; Johns, W.L.; Nazarian, A. Lateral Release with Tibial Tuberosity Transfer Alters Patellofemoral Biomechanics Promoting Multidirectional Patellar Instability. Arthrosc. J. Arthrosc. Relat. Surg. 2022, 38, 953–964. [Google Scholar] [CrossRef] [PubMed]
- Xiang, L.; Gu, Y.; Wang, V.F.J. Effect of Foot Pronation During Distance Running on the Lower Limb Impact Acceleration and Dynamic Stability. Acta Bioeng. Biomech. 2022, 24, 21–30. [Google Scholar] [CrossRef] [PubMed]
- Willey, M.C.; Kern, A.M.; Goetz, J.E.; Marsh, J.L.; Anderson, D.D. Biomechanical Guidance Can Improve Accuracy of Reduction for Intra-Articular Tibia Plafond Fractures and Reduce Joint Contact Stress. J. Orthop. Res. 2023, 41, 546–554. [Google Scholar] [CrossRef] [PubMed]
- Hadeed, M.M.; Prakash, H.; Yarboro, S.R.; Weiss, D.B. Intramedullary Nailing of Extra-Articular Distal Tibial Fractures: Biomechanical Evaluation of Stability Immediately After Fixation in a Synthetic Bone Model. Bone Jt. J. 2021, 103, 294–298. [Google Scholar] [CrossRef] [PubMed]
- González, F.J.Q.; Sculco, P.K.; Kahlenberg, C.A.; Mayman, D.J.; Lipman, J.D.; Wright, T.M.; Vigdorchik, J.M. Undersizing the Tibial Baseplate in Cementless Total Knee Arthroplasty Has Only a Small Impact on Bone-Implant Interaction: A Finite Element Biomechanical Study. J. Arthroplast. 2023, 38, 757–762. [Google Scholar] [CrossRef] [PubMed]
- Van den Berghe, P.; Derie, R.; Gerlo, J.; Bauwens, P.; Segers, V.; Leman, M.; De Clercq, D. Influence of Music-Based Feedback Training on Peak Tibial Acceleration During Running Outside of the Biomechanics Laboratory. Footwear Sci. 2021, 13, 39–41. [Google Scholar] [CrossRef]
- Naruse, K.; Takegami, Y.; Tokutake, K.; Shimizu, K.; Sudo, Y.; Shinohara, T.; Imagama, S. What is the Radiographic Factor Associated with Meniscus Injury in Tibial Plateau Fractures? Multicenter Retrospective (TRON) Study. Indian J. Orthop. 2023, 57, 1076–1082. [Google Scholar] [CrossRef] [PubMed]
- Shao, B.; Xing, J.; Zhao, B.; Wang, T.; Mu, W. Role of the Proximal Tibiofibular Joint on the Biomechanics of the Knee Joint: A Three-Dimensional Finite Element Analysis. Injury 2022, 53, 2446–2453. [Google Scholar] [CrossRef] [PubMed]
- Goyal, A.; Prasad, J. An in Silico Model for Woven Bone Adaptation to Heavy Loading Conditions in Murine Tibia. Biomech. Model. Mechanobiol. 2022, 21, 1425–1440. [Google Scholar] [CrossRef] [PubMed]
- Wang, S.; Peng, A.Q.; Pan, S.; Hu, Y.N.; Zhang, X.; Gao, J.G. Analysis of medial tibial plateau fracture injury patterns using quantitative 3D measurements. J. Orthop. Sci. 2021, 26, 831–843. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Y.; Wang, R.; Hu, J.; Qin, X.; Chen, A.; Li, X. Magnetic resonance imaging (MRI) and computed topography (CT) analysis of Schatzker type IV tibial plateau fracture revealed possible mechanisms of injury beyond varus deforming force. Injury 2022, 53, 683–690. [Google Scholar] [CrossRef] [PubMed]
- Johnson, C.D.; Outerleys, J.; Davis, I.S. Agreement Between Sagittal Foot and Tibia Angles During Running Derived from an Open-Source Markerless Motion Capture Platform and Manual Digitization. J. Appl. Biomech. 2022, 38, 111–116. [Google Scholar] [CrossRef] [PubMed]
- Scolaro, J.A.; Wright, D.J.; Lai, W.; Fraipont, G.; Hitchens, H.; Kwak, D.; McGarry, M.; Lee, T.Q. Fixation of Extra-Articular Proximal Tibia Fractures: Biomechanical Comparison of Single and Dual Implant Constructs. J. Am. Acad. Orthop. Surg. 2022, 30, 629–635. [Google Scholar] [CrossRef]
- Yao, J.; Crockett, J.; D’souza, M.; Day, G.A.; Wilcox, R.K.; Jones, A.C.; Mengoni, M. Effect of Meniscus Modelling Assumptions in a Static Tibiofemoral Finite Element Model: Importance of Geometry Over Material. Biomech. Model. Mechanobiol. 2024, 23, 1055–1065. [Google Scholar] [CrossRef] [PubMed]
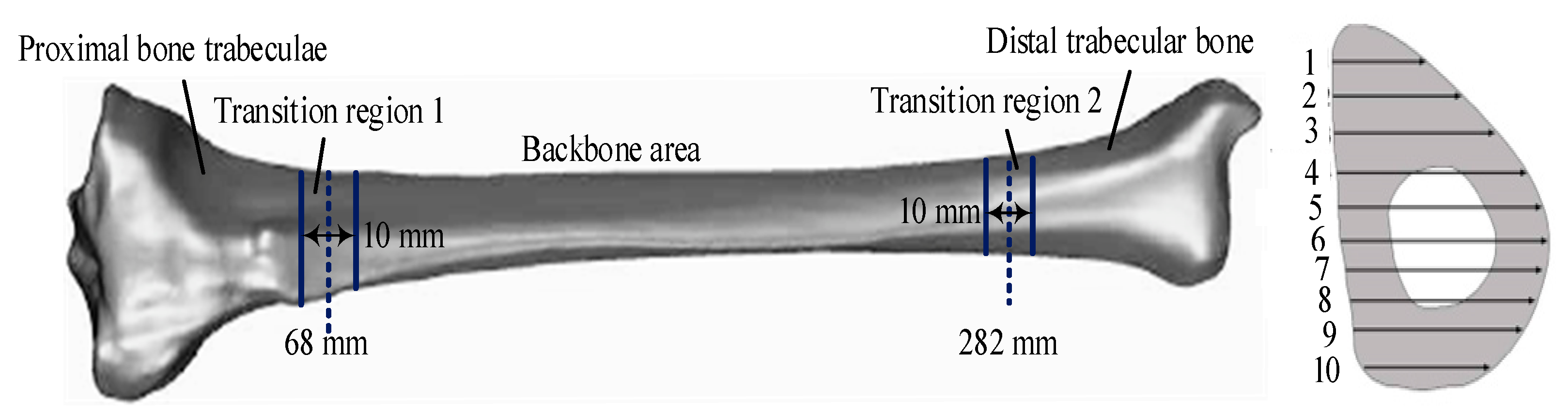


| Parameter | Proximal Trabecular Area (A1) | Transition Region 1 (A4) | Backbone Area (A3) | Transition Region 2 (A5) | Distal Trabecular Area (A2) |
|---|---|---|---|---|---|
| Range (mm) | 0–63 | 63–73 | 73–277 | 277–287 | 287–342 |
| Number of detection points | 1,824,215 | / | 1,610,736 | / | 1,765,913 |
| Mean CT number | 361.7 | / | 1840.4 | / | 411.9 |
| Apparent density (kg/m3) | 1409.3 | / | 2913.1 | / | 1458.2 |
| Young modulus (GPa) | 8.37 | Equation (8) | 17.37 | Equation (8) | 8.62 |
| Poisson ratio | 0.35 | Equation (9) | 0.14 | Equation (9) | 0.35 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, L.; Qian, Q.; Liu, H.; Liu, H.; Park, J.-C. Biomechanical and Injury Tolerance Analysis of Tibial Movement Under Different Postures and Impact Loads. Appl. Sci. 2024, 14, 11760. https://doi.org/10.3390/app142411760
Liu L, Qian Q, Liu H, Liu H, Park J-C. Biomechanical and Injury Tolerance Analysis of Tibial Movement Under Different Postures and Impact Loads. Applied Sciences. 2024; 14(24):11760. https://doi.org/10.3390/app142411760
Chicago/Turabian StyleLiu, Lei, Qian Qian, Hengjia Liu, Hongyan Liu, and Jong-Chul Park. 2024. "Biomechanical and Injury Tolerance Analysis of Tibial Movement Under Different Postures and Impact Loads" Applied Sciences 14, no. 24: 11760. https://doi.org/10.3390/app142411760
APA StyleLiu, L., Qian, Q., Liu, H., Liu, H., & Park, J.-C. (2024). Biomechanical and Injury Tolerance Analysis of Tibial Movement Under Different Postures and Impact Loads. Applied Sciences, 14(24), 11760. https://doi.org/10.3390/app142411760










