Brake Torque Sensor Calibration Device
Abstract
1. Introduction
2. Brake System
- Service brakes, the main feature of which is foot control. During operation, they must act on all vehicle wheels and vehicle sets and their effect must be variable. They must ensure a reduction in speed or a complete stop of the vehicle while maintaining the desired direction.
- Emergency brakes, which, unlike service brakes, do not have to act on every single wheel of the vehicle or set of vehicles. In case of failure, they replace the service brakes, and it is important that they act on at least one wheel on each side. Emergency brakes often work with less effect than service brakes.
- Parking brakes, the main task of which is to prevent a stationary vehicle from moving off a slope even if there is no driver in the vehicle.
- Auxiliary brakes, which are characterized by maintaining a constant speed of the vehicle, but also by reducing it. In this way, they increase the effect of the service brake while lightening it, thereby extending its service life [35].
2.1. Types of Brake Systems
- Drum brakes—use drum-shaped housing where the brake shoes are pressed against the inner surface to create friction. They are durable and cost-effective for rear wheels.
- Disc brakes—use a disc-shaped rotor that rotates with the wheel and the brake pads press against it to brake. Due to better heat dissipation and stopping power, they are usually used in front-wheel and high-performance vehicles.
2.2. Residual Torque
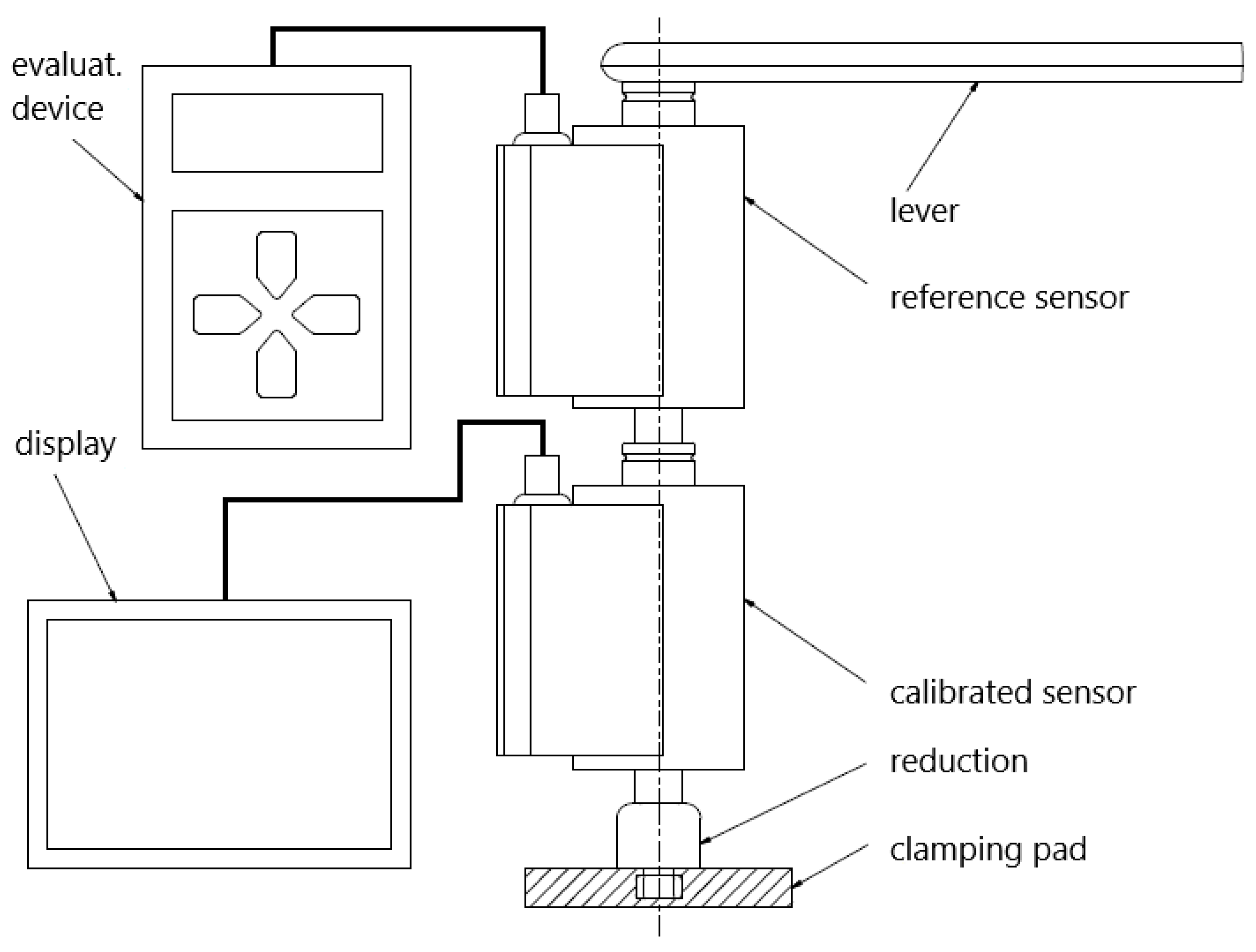
3. Description of the Measuring Device
- Bases 1 and 2 serve to fix the universal plate and the brake disc. Base 2 is equipped at one end with a simple mechanism for clamping the torque sensor.
- A universal plate serves to fasten the measured brake caliper.
- A brake disc is used for measurements. It is fixed through the flange on base 2.
- Gearbox system with an el. motor, which serves to rotate the brake disc.
- Torque sensor, which is used to measure the residual torque on the brake disc.
- Type: T4A.
- Accuracy class: 0.1.
- Nominal (measuring) range: 10 Nm; 20 Nm.
- Nominal (measurement) sensitivity: 2 mV/V < ±0.2%.
- Amount of rotation at the nominal (measuring) torque: 0.9° for a 10 Nm sensor and 1.1° for a 20 Nm sensor.
4. Calibration Equipment Models
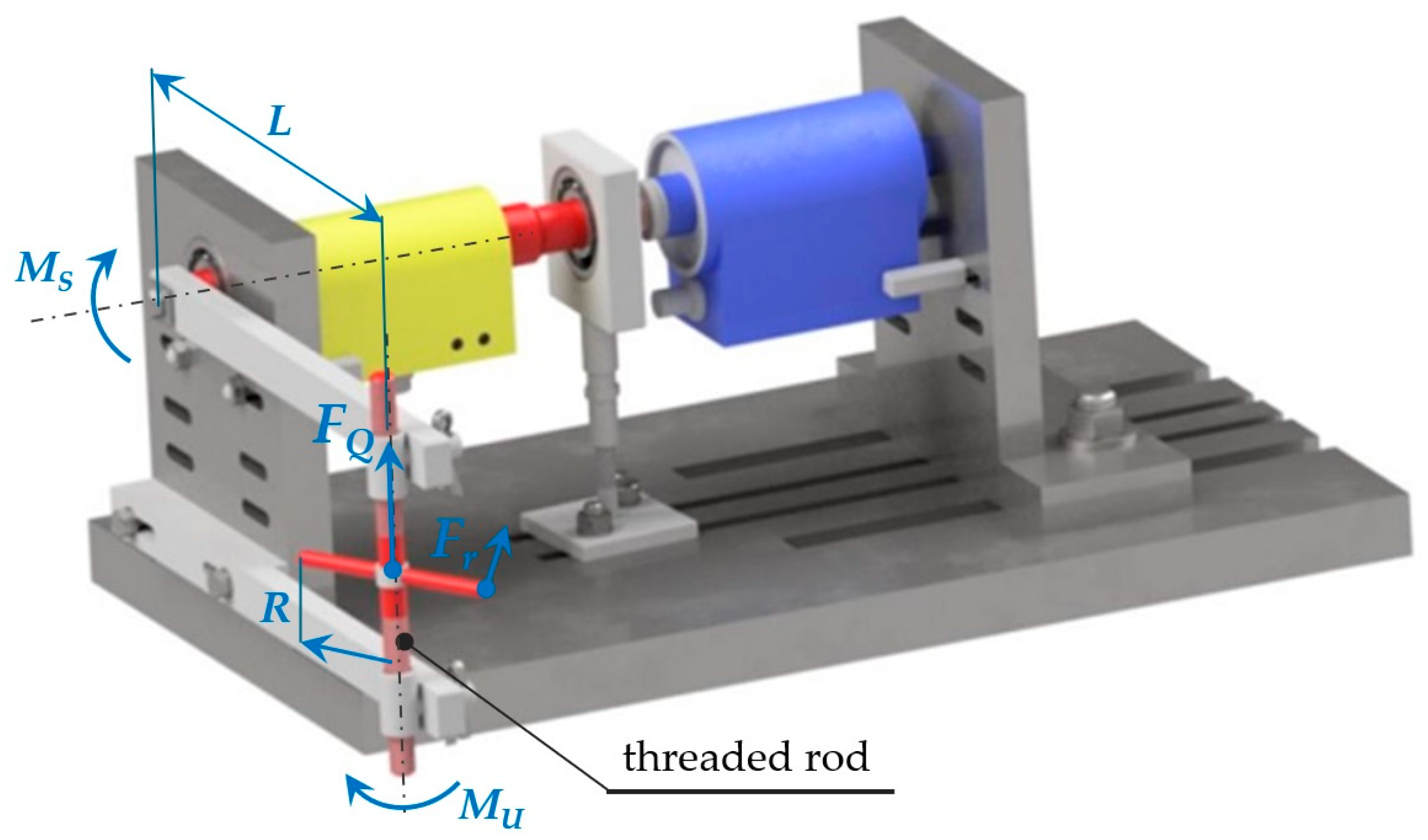
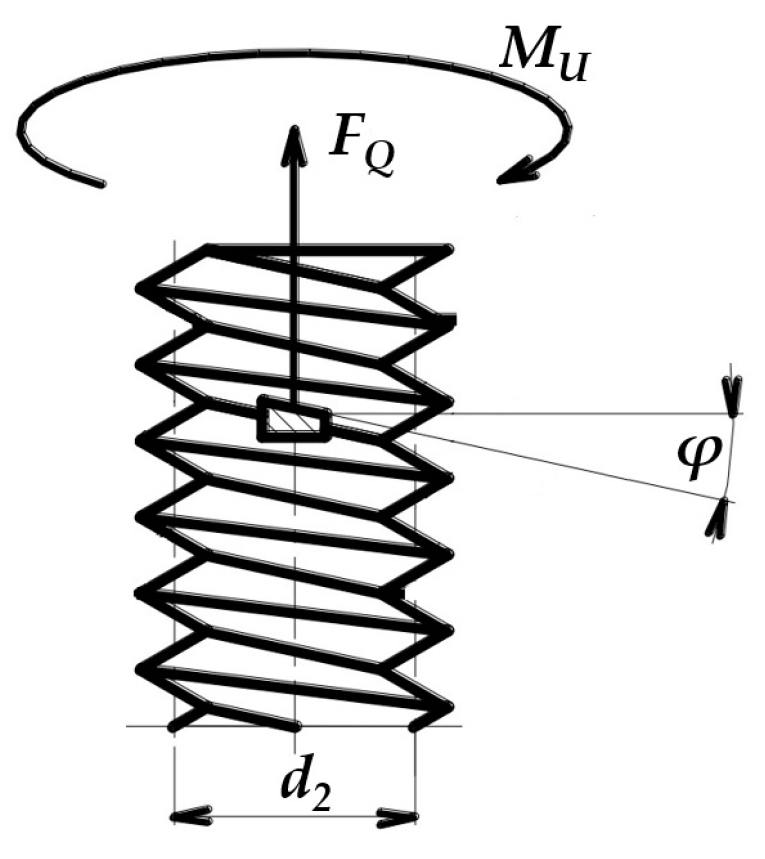
4.1. Design of a Calibration Device with a Threaded Rod
- denotes the force in the treaded rod [N].
- denotes the distance between the shaft axis and the treaded rod axis (Figure 4).
- denotes the mean screw diameter [m].
- denotes the pitch angle of the thread [rad].
- denotes the friction angle [rad].
- denotes the friction coefficient in a thread.
- denotes the mean diameter of the contact surface [m].
- denotes the force on a handle of a threaded screw deduced by an operator [N].
- denotes the diameter of a threaded screw handle [m].
4.2. Design with the Angular Gearbox
- denotes the torque on the gearbox input [Nm].
- denotes the force induced by an operator [N].
- denotes the wheel radius [m].
- denotes the mechanical efficiency of the gearbox.
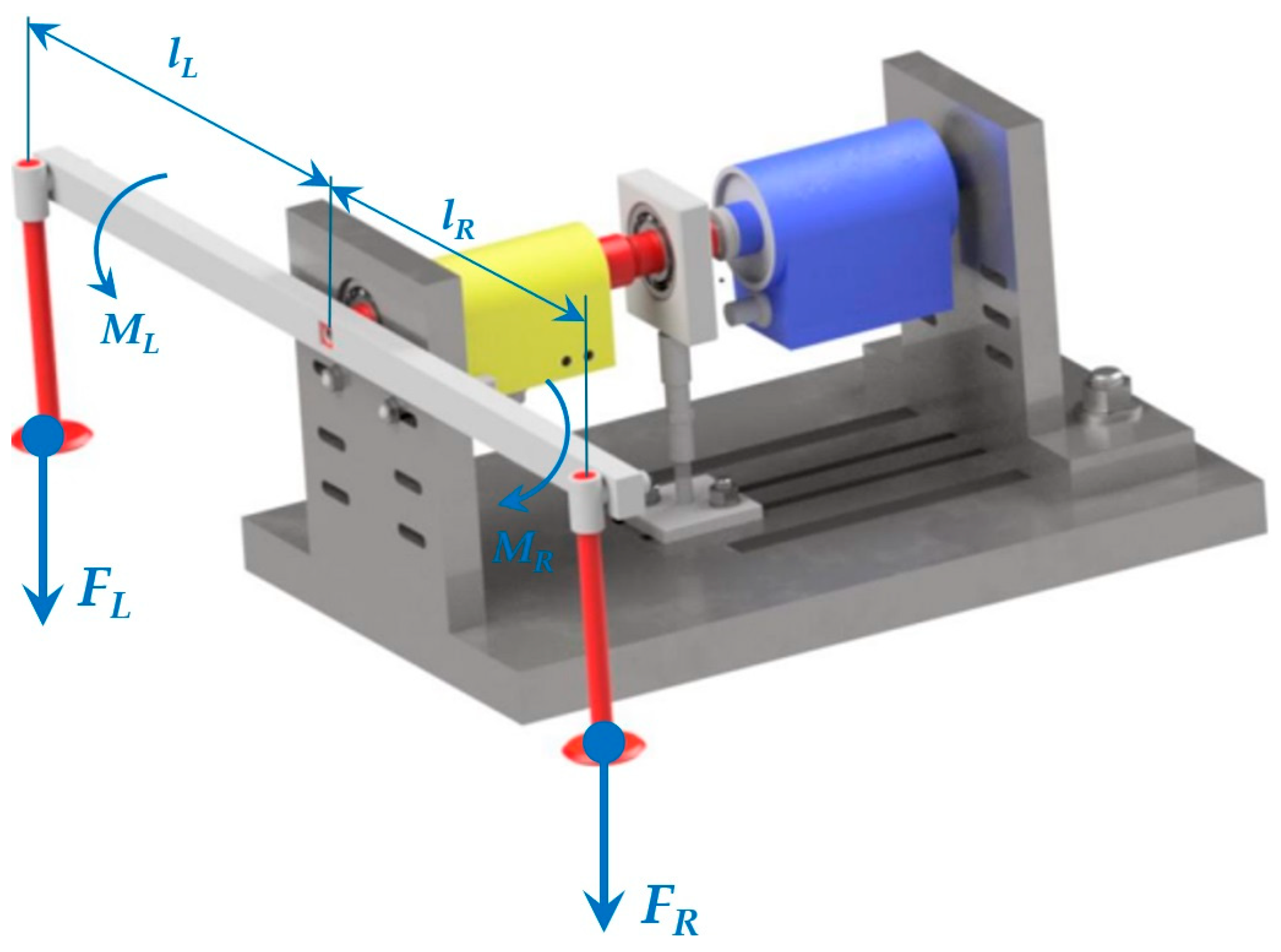
4.3. Design with Weight Carriers
- , denote the gravitational forces due to the weights [N].
- , denote the lengths of the arms that the weights act on [m].
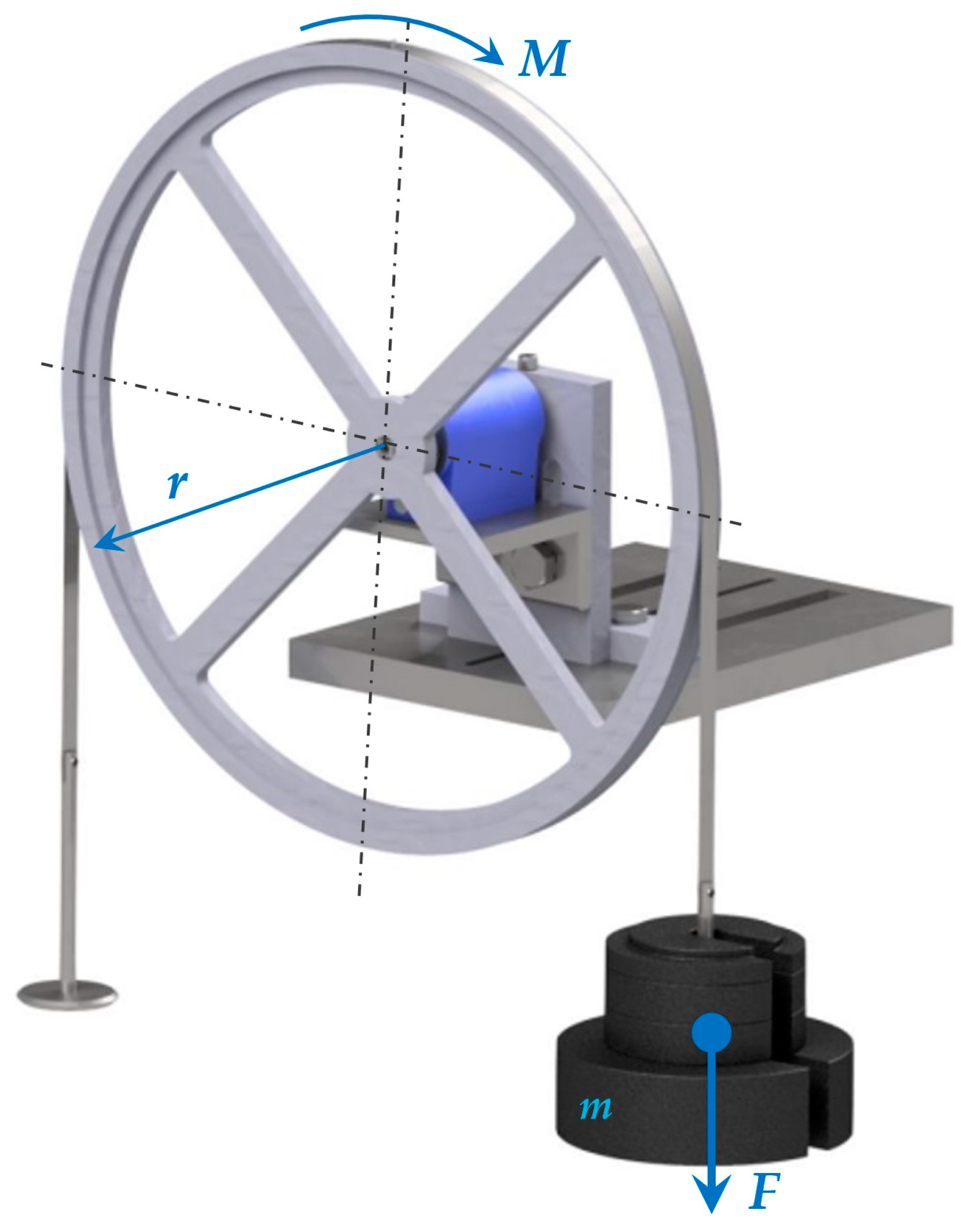
4.4. Design with a Calibration Wheel
- denotes the force moment [Nm].
- denotes the force induced by the calibration weight [N].
- denotes the length of the arm on which the force F acts [m].
- denotes the weight of the weight [kg].
- denotes the local gravity acceleration [m/s2].
5. Calculation of the Calibration Device Error
- is the mass of the given weight [kg].
- is the value of the given calibration weight [N].
- is the local gravitational acceleration [m/s2].
- is the mass of the weight increased or reduced by the tolerance value [kg].
- is the value of the mass of the given calibration weight [kg].
- is the tolerance value for the given weight [kg].
- is the actual diameter of the calibration wheel enlarged or reduced by the uncertainty of the 3D scanner [m].
- is the actual diameter of the calibration wheel [m].
- is the uncertainty of the 3D scanner [m].
- is the maximum or minimum radius of the calibration wheel [m].
- is the actual diameter of the calibration wheel enlarged or reduced by the uncertainty of the 3D scanner [m].
- is half the thickness of the steel strip [m].
- is the maximum or minimum value of the torque induced by the given weight [Nm].
- is the maximum or minimum value of the calibration weight [N].
- is the maximum or minimum value of the radius of the calibration wheel [m].
- is the sum of the maximum or minimum torque value induced for a given combination of calibration weights [Nm].
- is the maximum or minimum torque value induced by the given weight [Nm].
- is the range of deviations from the nominal value [Nm].
- is the sum of maximum torque values for a given combination of calibration weights [Nm].
- is the sum of the minimum torque values for the given combination of calibration weights [Nm].
- is the standard uncertainty of the considered source [Nm].
- is the range of deviations from the nominal value of torque [Nm].
- k is the value of the chosen approximation of the probability distribution, while for this type of approximation of the distribution, the uniform distribution was chosen.
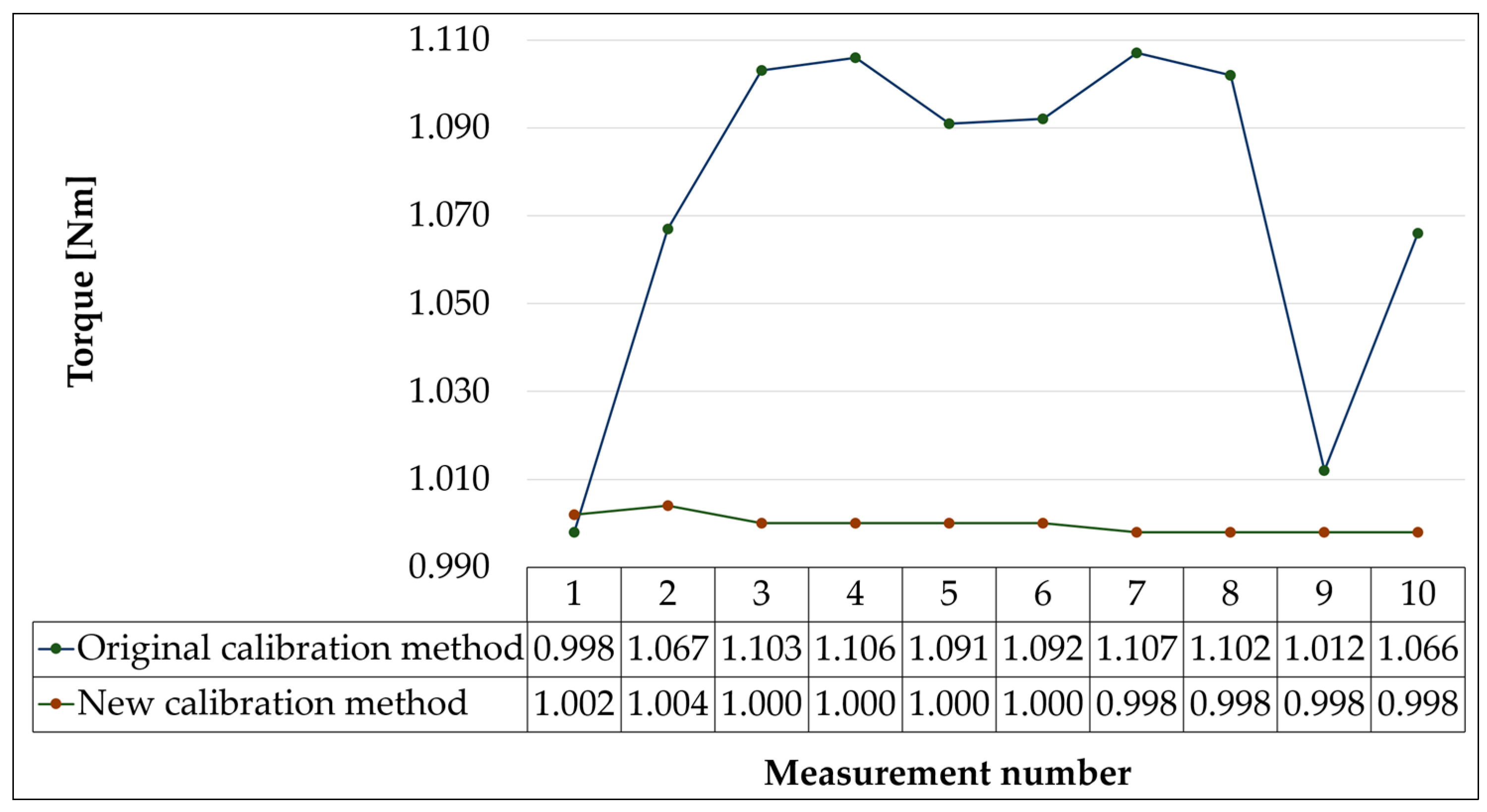
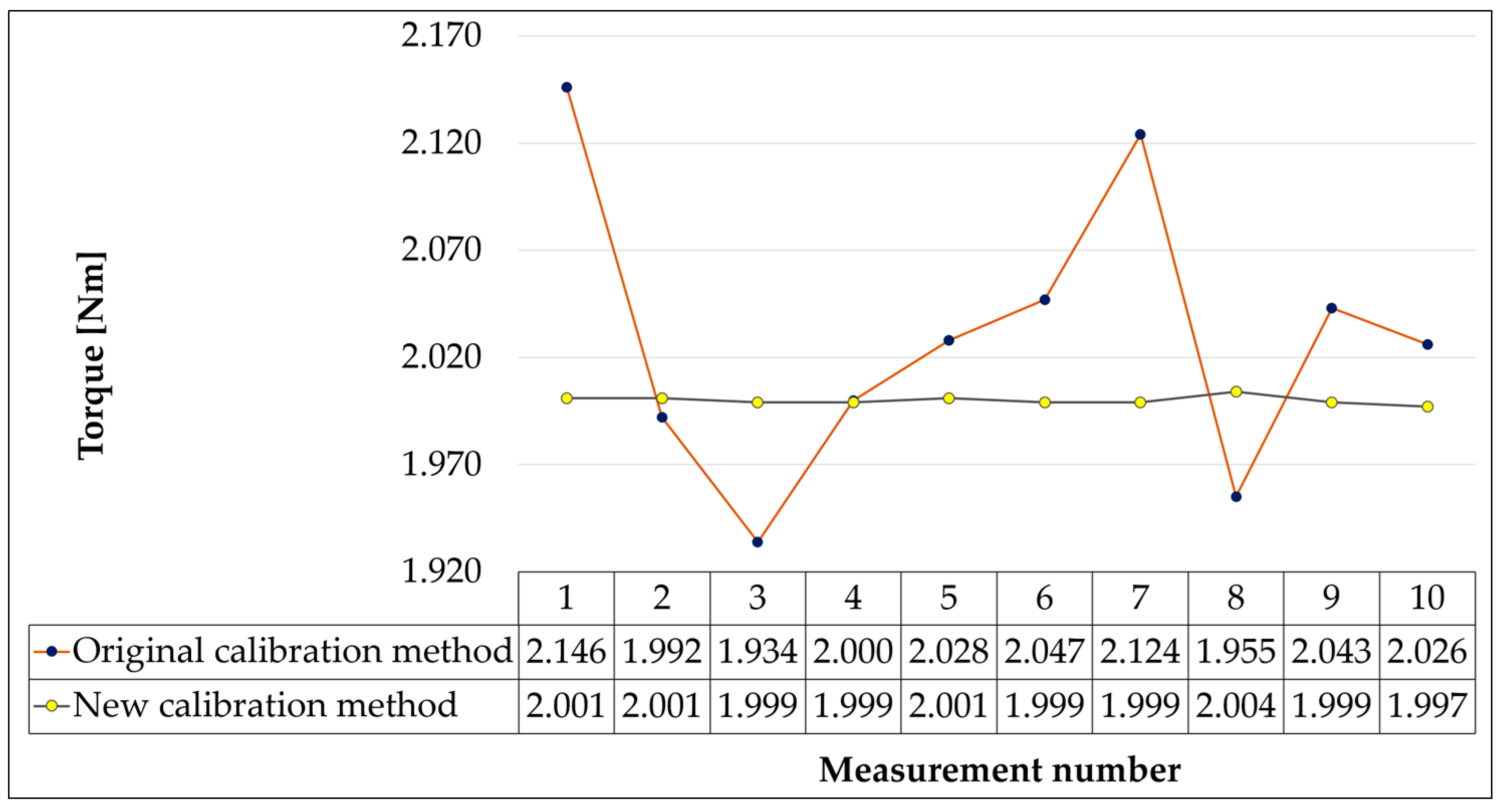
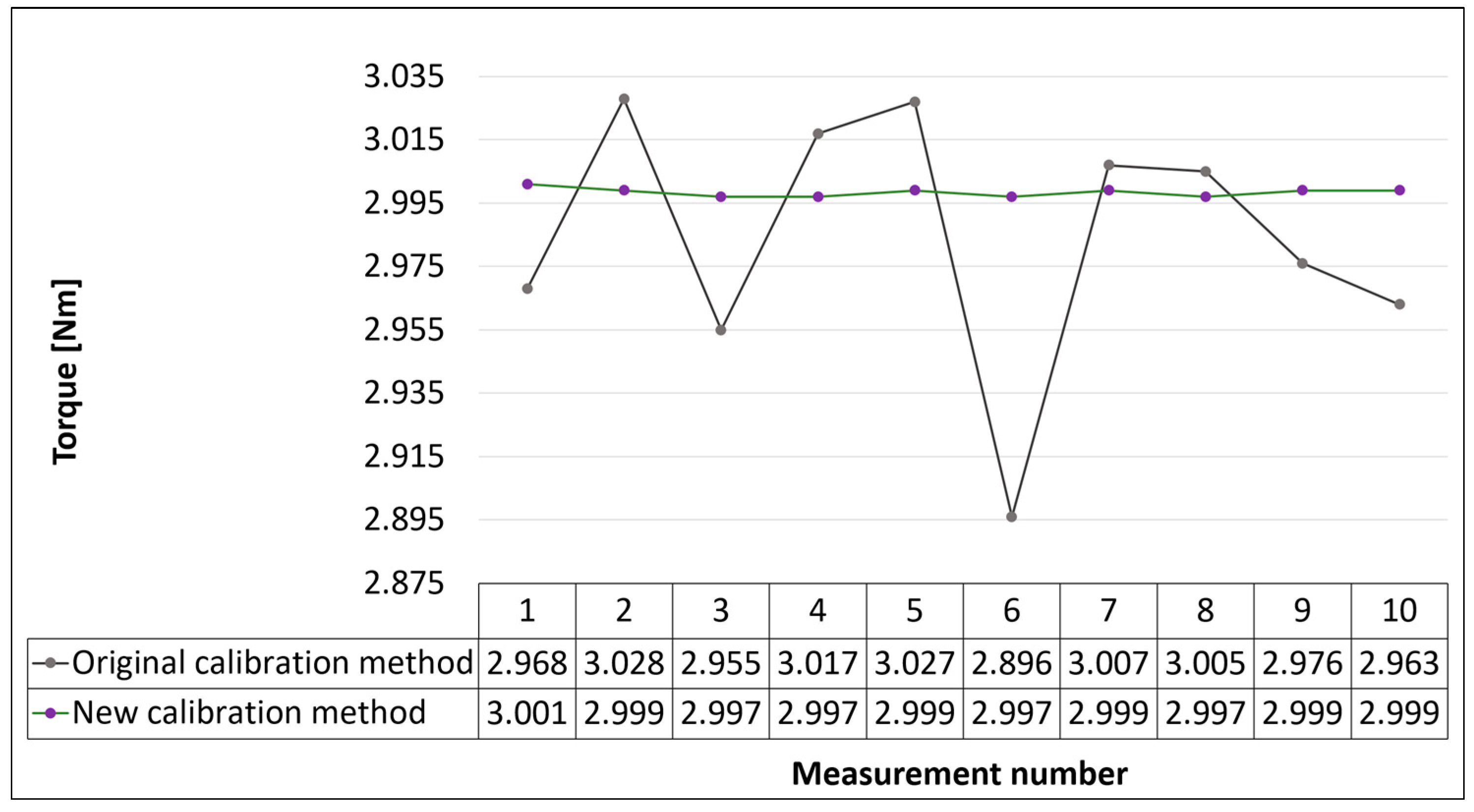
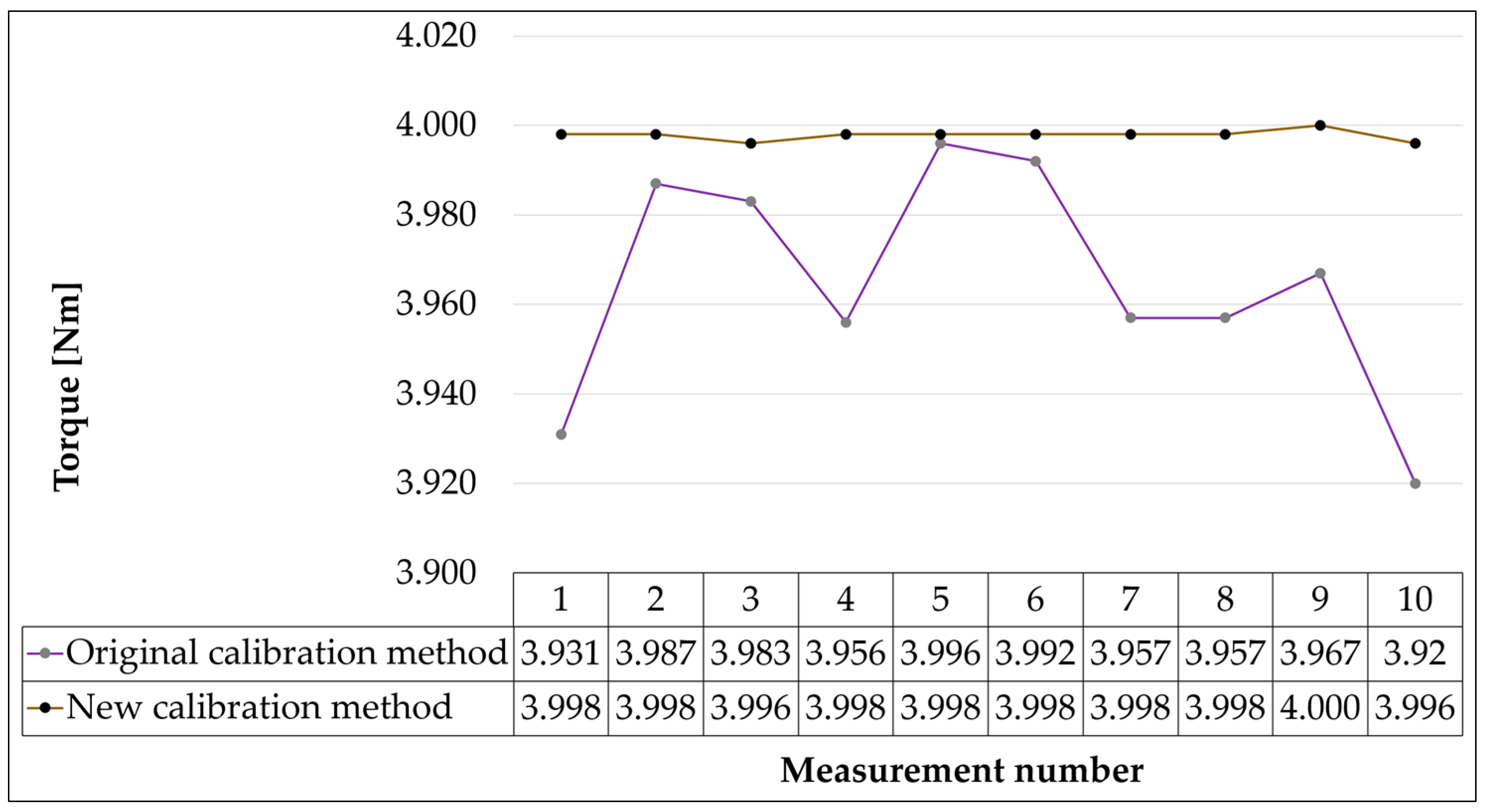
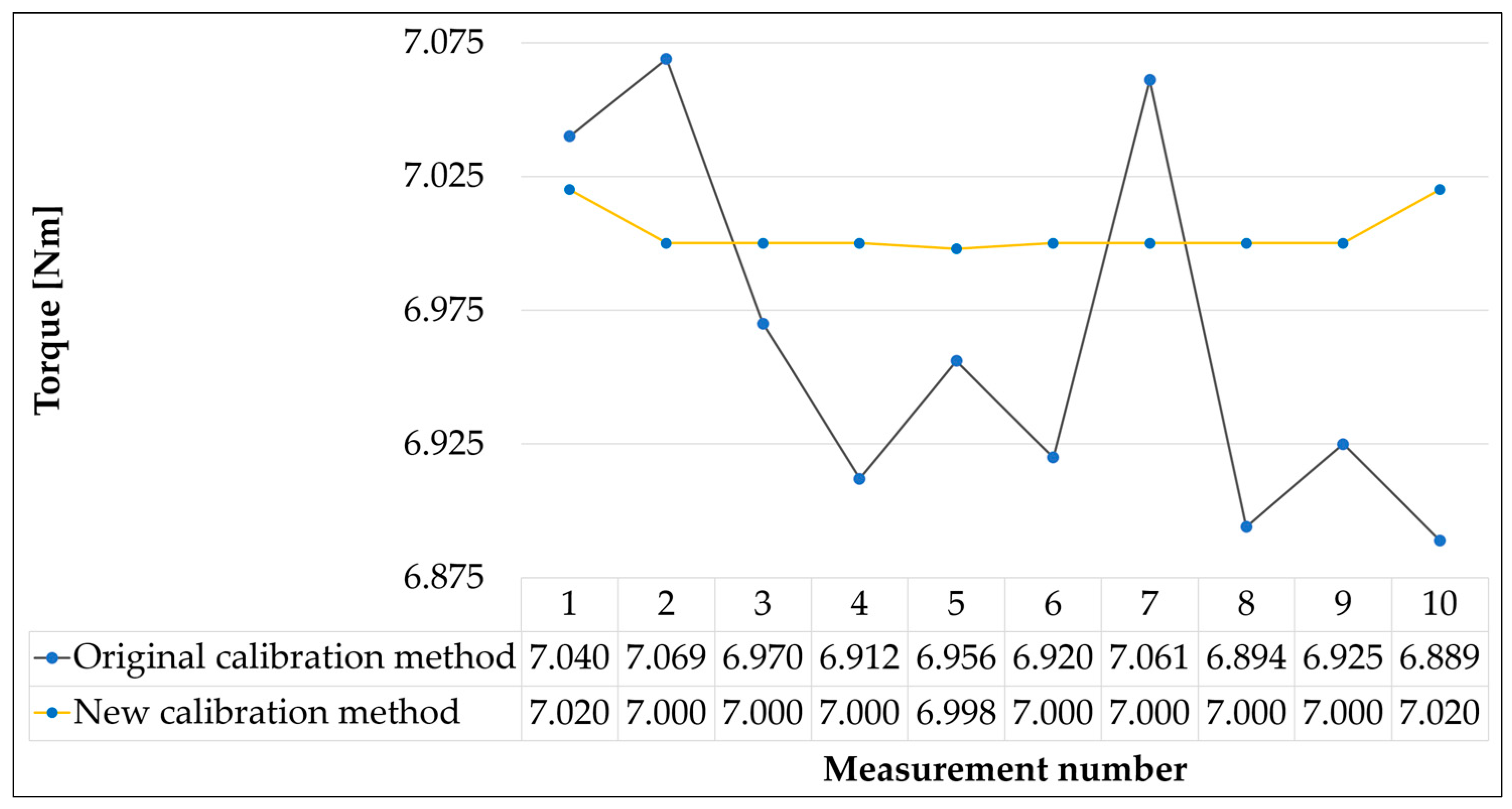
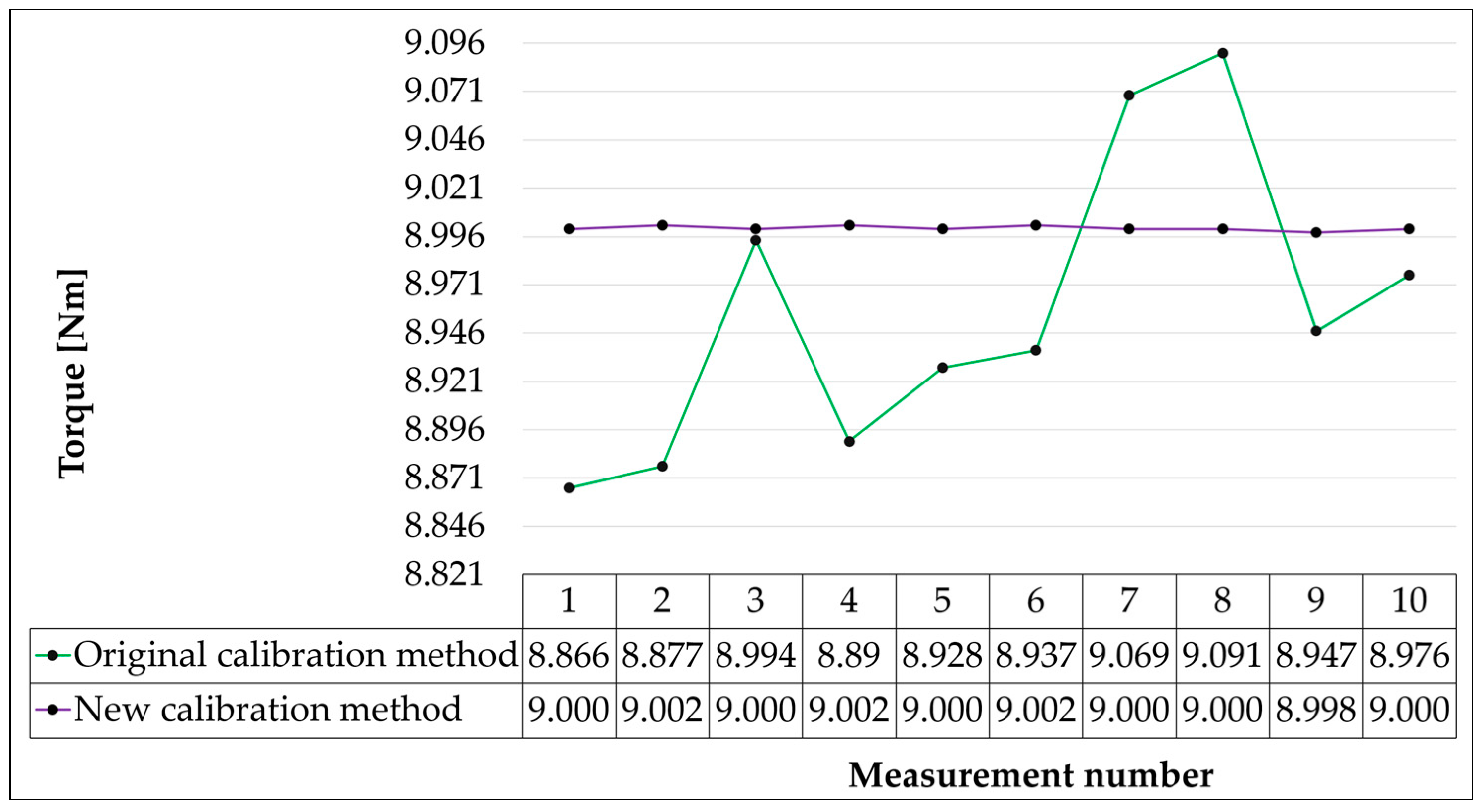
6. Calibration Measurements That Were Performed
7. Discussion
8. Conclusions
- The necessity of exactly setting the torque sensor and the standard on “one axis” and maintaining the stability of this position.
- Ensuring the temperature stability of the measuring device.
- Selecting a suitable accuracy class for the reference sensor.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Songkitti, W.; Wirojsakunchai, E.; Aroonsrisopon, T. Identifying Factors that Affect Brake Wear PM Emissions during Real-World Test Conditions; SAE Technical Paper; SAE International: Pittsburgh, PA, USA, 2022; p. 2022-01-0570. [Google Scholar] [CrossRef]
- Amato, F. Non-Exhaust Emissions: An Urban Air Quality Problem for Public Health; Impact and Mitigation Measures, 1st ed.; Academic Press: Cambridge, MA, USA, 2018. [Google Scholar]
- Grigoratos, T.; Martini, G. Brake wear particle emissions: A review. Environ. Sci. Pollut. Res. Int. 2015, 22, 2491–2504. [Google Scholar] [CrossRef] [PubMed]
- Harrison, R.M.; Jones, A.M.; Gietl, J.; Yin, J.; Green, D.C. Estimation of the contributions of brake dust, tire wear, and resuspension to nonexhaust traffic particles derived from atmospheric measurement. Environ. Sci. Technol. 2012, 46, 6523–6529. [Google Scholar] [CrossRef] [PubMed]
- Thorpe, A.; Harrison, R.M. Sources and properties of non-exhaust particulate matter from road traffic: A review. Sci. Total Environ. 2008, 400, 270–282. [Google Scholar] [CrossRef]
- Wahid, S.M.S. Automotive brake wear: A review. Environ. Sci. Pollut. Res. Int. 2018, 25, 174–180. [Google Scholar] [CrossRef]
- Timmers, V.R.J.H.; Achten, P.A.J. Non-exhaust PM emissions from electric vehicles. Atmos. Environ. 2016, 134, 10–17. [Google Scholar] [CrossRef]
- Beddows, D.C.S.; Harrison, R.M. PM10 and PM2.5 emission factors for non-exhaust particles from road vehicles: Dependence upon vehicle mass and implications for battery electric vehicles. Atmos. Environ. 2021, 244, 117886. [Google Scholar] [CrossRef]
- Jiang, R.; Liu, Y.; Hu, D.; Zhu, L. Exhaust and non-exhaust airborne particles from diesel and electric buses in Xi’an: A comparative analysis. Chemosphere 2022, 306, 135523. [Google Scholar] [CrossRef]
- Woo, S.-H.; Jang, H.; Lee, S.-B.; Lee, S. Comparison of total PM emissions emitted from electric and internal combustion engine vehicles: An experimental analysis. Sci. Total Environ. 2022, 842, 156961. [Google Scholar] [CrossRef]
- Gramstat, S.; Mertens, T.; Waninger, R.; Lugovyy, D. Impacts on brake particle emission testing. Atmosphere 2020, 11, 1132. [Google Scholar] [CrossRef]
- Kozuch, P.; Hujo, L.; Kaszkowiak, J.; Markiewicz-Patalon, M. Evaluation of service brake braking of selected group of vehicles depending on wear of brake system’s parts. Acta Technol. Agric. 2024, 27, 101–107. [Google Scholar] [CrossRef]
- Zhong, C.; Sun, J.; Liu, Z.; Niu, H.; Zhang, J.; Liang, X.; Yin, J.; Wu, L.; Peng, J.; Zhang, Q.; et al. Size distribution of brake wear particulate matter based on a brake dynamometer investigation. Front. Future Transp. 2024, 5, 1407660. [Google Scholar] [CrossRef]
- Mathissen, M.; Grochowicz, J.; Schmidt, C.; Vogt, R.; Hagen, F.H.F.; Grabiec, T.; Steven, H.; Grigoratos, T. A novel real-world braking cycle for studying brake wear particle emissions. Wear 2018, 414–415, 219–226. [Google Scholar] [CrossRef]
- Kukutschová, J.; Moravec, P.; Tomásek, V.; Matejka, V.; Smolík, J.; Schwarz, J.; Seidlerová, J.; Safárová, K.; Filip, P. On airborne nano/micro-sized wear particles released from low-metallic automotive brakes. Environ. Pollut. 2011, 159, 998–1006. [Google Scholar] [CrossRef] [PubMed]
- Straffelini, G.; Gialanella, S. Airborne particulate matter from brake systems: An assessment of the relevant tribological formation mechanisms. Wear 2021, 478–479, 203883. [Google Scholar] [CrossRef]
- Kozuch, P.; Hujo, L.; Muślewski, L.; Markiewicz-Patalon, M. Dynamic and stationary testing of vehicle braking systems. Acta Technol. Agric. 2023, 26, 238–243. [Google Scholar] [CrossRef]
- Otto, J.; Ostermeyer, G.P. High-frequency vibrations in the contact of brake systems. Facta Univ.-Ser. Mech. Eng. 2019, 17, 103. [Google Scholar] [CrossRef]
- Koch, S.; Gödecker, H.; von Wagner, U. On the interrelation of equilibrium positions and work of friction forces on brake squeal. Arch. Appl. Mech. 2022, 92, 771–784. [Google Scholar] [CrossRef]
- Koch, S.; Köppen, E.; Gräbner, N.; von Wagner, U. On the influence of multiple equilibrium positions on brake noise. Facta Univ.-Ser. Mech. Eng. 2021, 19, 613–632. [Google Scholar] [CrossRef]
- Kim, Y.; Kwon, T.; Lee, S. Analysis of the effect of the wedged type brake caliper piston on brake drag. In Proceedings of the 39th Annual Brake Colloquium and Exhibition, BRAKE 2021, Virtual Event, 17–20 October 2021. [Google Scholar] [CrossRef]
- Mohan, S.; Dake, P.; Mullapudi, D.; Ingole, S. A method to capture and analyze brake dynamic drag. In Proceedings of the 17th Symposium on International Automotive Technology, SIAT 2021, Virtual Event, 29 September–1 October 2021. [Google Scholar] [CrossRef]
- Synák, F.; Rievaj, V.; Kucera, M.; Sebök, M.; Skrúcany, T. Effect of repeated vehicle braking on the warming of selected parts of the vehicle. Sci. J. Silesian Univ. Technol.-Ser. Transp. 2020, 107, 183–196. [Google Scholar] [CrossRef]
- Vecchiato, L.; Vecchiato, L.; Negri, M.; Picci, G.; Viale, L.; Zaltron, G.; Giacometti, S.; Meneghetti, G. Design and development of a brake test bench for formula SAE Race Cars. Machines 2024, 12, 135. [Google Scholar] [CrossRef]
- Dixit, C.; Gaurkar, P.; Ramakrishnan, R.; Shankar Ram, C.S.; Vivekanandan, G.; Sivaram, S. Calibration of an inertial measurement unit and its impact on antilock braking system performance. In Proceedings of the 18th Symposium on International Automotive Technology, SIAT 2024, Pune, India, 23–25 January 2024. [Google Scholar] [CrossRef]
- Tawadros, P.; Awadallah, M.; Walker, P.; Zhang, N. Using a low-cost bluetooth torque sensor for vehicle jerk and transient torque measurement. Proc. Inst. Mech. Eng. Part D-J. Automob. Eng. 2020, 234, 095440701986161. [Google Scholar] [CrossRef]
- Zhang, C.; Li, Z.P.; Chen, J.; Qiu, F.; Na, S.D. Design and research of a novel non-contact vertical inductive torque sensor. Measurements 2021, 177, 109252. [Google Scholar] [CrossRef]
- De Carvalho Pinheiro, H.; Sisca, L.; Carello, M.; Ferraris, A.; Airale, A.G.; Falossi, M.; Carlevaris, A. methodology and application on LOAD monitoring using strain-gauged bolts in brake calipers. In Proceedings of the SAE 2022 Annual World Congress Experience, WCX 2022, Virtual Event, 5–7 April 2022. [Google Scholar] [CrossRef]
- Wheatley, G.; Rubel, R.I. An autonomous braking control system for a 2017 Yamaha Grizzly 700. Sci. J. Silesian Univ. Technol.-Ser. Transp. 2022, 115, 211–226. [Google Scholar] [CrossRef]
- Jneid, M.S.; Harth, P. Integrated torque vectoring control using vehicle yaw rate and sideslip angle for improving steering and stability of all off wheel-motor drive electric vehicles. Acta Polytech. Hung. 2024, 21, 87–106. [Google Scholar] [CrossRef]
- Ishak, M.R.; Abu Bakar, A.R.; Belhocine, A.; Taib, J.M.; Omar, W.Z.W. Brake torque analysis of fully mechanical parking brake system: Theoretical and experimental approach. Measurements 2016, 64, 487–497. [Google Scholar] [CrossRef]
- Panchenko, S.; Gerlici, J.; Vatulia, G.; Lovska, A.; Ravlyuk, V.; Harusinec, J. Studying the load of composite brake pads under high-temperature impact from the rolling surface of wheels. EUREKA Phys. Eng. 2023, 2023, 155–167. [Google Scholar] [CrossRef]
- Panchenko, S.; Gerlici, J.; Lovska, A.; Vatulia, G.; Ravlyuk, V.; Rybin, A. Method for Determining the Factor of Dual Wedge-Shaped Wear of Composite Brake Pads for Freight Wagons. Commun.-Sci. Lett. Univ. Zilina 2024, 26, B31–B40. [Google Scholar] [CrossRef]
- Panchenko, S.; Gerlici, J.; Lovska, A.; Ravlyuk, V. The Service Life Prediction for Brake Pads of Freight Wagons. Commun.-Sci. Lett. Univ. Zilina 2024, 26, B80–B89. [Google Scholar] [CrossRef]
- Hu, C.; Pan, G.; Kong, L.; Yu, J. Research of brake by wire system. J. Phys.-Conf. Ser. 2023, 2479, 012051. [Google Scholar] [CrossRef]
- Testbook. 2023. Available online: https://testbook.com/mechanical-engineering/drum-brakes-vs-disc-brakes (accessed on 21 August 2024).
- Zhou, X.; Wu, G.; Wang, C.; Zhang, R.; Shi, S.; Zhao, W. Cooperative optimization of energy recovery and braking feel based on vehicle speed prediction under downshifting conditions. Energy 2024, 301, 131699. [Google Scholar] [CrossRef]
- Li, W.; Zheng, H.Y.; Zong, C.F. A brake pad wear control algorithm for electronic brake system. Adv. Mater. Res. 2013, 694–697, 2099–2105. [Google Scholar] [CrossRef]
- Gerlici, J.; Kravchenko, K.; Fomina, Y. Development of an innovative technical solution to improve the efficiency of rolling stock friction brake elements operation. In Transbaltica XII: Transportation Science and Technology; Springer: Berlin/Heidelberg, Germany, 2022; pp. 28–38. [Google Scholar]
- Ledbestov. 2020. Available online: https://ledbestov.live/product_details/9746208.html (accessed on 5 May 2024).
- SAE. 2024. Available online: https://www.sae.org/news/2023/05/brakes-sustainability-dust-regulations (accessed on 21 August 2024).
- ACEA. 2023. Available online: https://www.acea.auto/fact/euro-standards/ (accessed on 21 August 2024).
- Dieselnet. 2024. Available online: https://dieselnet.com/standards/eu/ld.php (accessed on 21 August 2024).
- NOTA, S. Design of a Device for Calibration Torque Sensor for Measuring Residual Brake Moment. Master’s Thesis, University of Žilina, Žilina, Slovensko, 2019. [Google Scholar]
- HBM. 2019. Available online: https://www.sensor-hbm.com/upload/product-file/b0591.pdf (accessed on 18 May 2024).






| Aspect | Drum Brakes | Disc Brakes |
|---|---|---|
| Design | Enclosed design with brake shoes inside a drum | Open design with brake pads gripping a rotor |
| Heat dissipation | Less efficient heat dissipation | More efficient heat dissipation |
| Cooling | Limited cooling due to enclosed design | Better cooling due to open design |
| Brake fade | More susceptible to brake fade | Less susceptible to brake fade |
| Stopping distances | Generally longer stopping distances | Shorter stopping distances |
| Maintenance | Require periodic adjustments for optimal performance | Require less maintenance and adjustments |
| Noise | Produce less noise during braking | Can produce more noise during braking |
| Corrosion | Generally less susceptible to corrosion | More susceptible to corrosion in rotor and caliper |
| Brake dust | Generate less brake dust | Generate more brake dust |
| Complex components | Simpler design with fewer components | More complex with calipers, pistons, and rotors |
| Typically more affordable | Generally more expensive | |
| Parking brake | Integrated parking brake mechanism | Parking brake functionality may vary |
| Weight | Heavier compared to disc brakes | Lighter compared to drum brakes |
| Type of Brake | Advantages | Disadvantages |
|---|---|---|
| Disc brake with a floating caliper | Better heat dissipation, smaller built-in dimensions, lower weight, | Uneven-wear brake pads; option to use (at most) two brake pistons |
| Disc brake with a fixed caliper | lower residual torque Possibility of using several brake pistons; stronger construction | Higher weight, larger dimensions |
| Standard | EURO 1 | EURO 2 | EURO 3 | EURO 4 | EURO 5 | EURO 6 | EURO 7 |
|---|---|---|---|---|---|---|---|
| Valid from | July 1992 | January 1996 | January 2000 | January 2005 | September 2009 | September 2014 | November 2026 |
| CO [g/km] Petrol diesel | 2.72 2.72 | 1.0 2.2 | 0.64 2.3 | 0.5 1.0 | 0.5 1.0 | 0.5 1.0 | 0.5 1.0 |
| PM [g/km] Diesel eng. | 0.14 | 0.08–0.1 | 0.05 | 0.025 | 0.005 | 0.0045 | 0.0045 |
| PM [g/km] Petrol eng. | - | - | - | - | 0.005 * | 0.0045 * | 0.0045 |
| PM [g/km] Brakes, tires | - | - | - | - | - | - | 0.003 EL 0.007 CE, 0.007 HY |
| Variant of Calibration Device | Advantages | Disadvantages |
|---|---|---|
| 1. Threaded rod | The possibility of simple clamping on the measuring table. | More time-consuming setting of the torque value using a threaded rod and it was problematic to set the torque sensor with the standard on one axis. Bearings that would cause resistance during calibration. |
| 2. Angular gearbox | Easier and faster setting of the torque value, which could be set in both directions of rotation. | Problematic axial adjustment of the torque sensor to the standard and the introduction of errors due to the effect of rolling bearings. Higher mass and size due to the gearbox. |
| 3. Weight carriers | Simpler and lighter construction. | There is an imperfect alignment of the system caused by friction between the arm and the weight carriers. There remains a problem with the axial adjustment of the torque sensor to the standard and with errors caused by the rolling of bearings. |
| 4. Calibration wheel | Eliminated errors due to friction from rolling bearings, problematic adjustment of the measuring assembly, and relatively massive construction. Easy handling and good positioning of the calibration device on the measuring device. The universality of using the calibration device for various measuring devices of the laboratory. The system can stabilize itself into an equilibrium position. | A need to adapt the thickness of the steel strip to the load. |
| Weight Value [N] | Max. Mass Value [kg] | Max. Weight Value [N] | Min. Mass Value [kg] | Min. Weight Value [N] |
|---|---|---|---|---|
| 2.5 | 0.25488519 | 2.50004903 | 0.25487519 | 2.49995095 |
| 5 | 0.50976538 | 5.00004902 | 0.50975538 | 4.99995094 |
| 10 | 1.01953076 | 10.00009805 | 1.01951076 | 9.99990187 |
| 50 | 5.09765382 | 50.00049042 | 5.09755382 | 49.99950957 |
| Calibrated Weight Value [N] | Value of Torque [Nm] | Min. Value of Torque [Nm] | Max. Value of Torque [Nm] |
|---|---|---|---|
| 2.5 | 0.5 | 0.50024294 | 0.50026556 |
| 5 | 1 | 1.00049568 | 1.00052131 |
| 10 | 2 | 2.00099136 | 2.00104262 |
| 50 | 10 | 10.00495686 | 10.00521313 |
| Required Torque Value [Nm] | Calibrated Weight Value [N] | Combination of Weights | Maximum Torque Tolerance σMmax [Nm] | Minimum Torque Tolerance σMmin [Nm] |
|---|---|---|---|---|
| 0.5 | 2.5 | 1 × 2.5N | 0.50026556 | 0.50024294 |
| 1 | 5 | 1 × 5N | 1.00052131 | 1.00049568 |
| 2 | 10 | 1 × 10N | 2.00104262 | 2.00099136 |
| 3 | 15 | 1 × 10N + 1 × 5N | 2.23723366 | 2.23717635 |
| 4 | 20 | 2 × 10N | 2.82990161 | 2.82982912 |
| 5 | 25 | 2 × 10N + 1 × 5N | 3.00156393 | 3.00148704 |
| 7 | 35 | 3 × 10N + 1 × 5N | 3.60743089 | 3.60733847 |
| 9 | 45 | 4 × 10N + 1 × 5N | 4.12525504 | 4.12514937 |
| 10 | 50 | 1 × 50N | 10.00521313 | 10.00495686 |
| 15 | 75 | 1 × 50N + 2 × 10N + 1 × 5N | 10.44574917 | 10.44548162 |
| Required Torque Value [Nm] | Calibrated Weight Value [N] | The Range of Deviation Changes ± [Nm] | [Nm] |
|---|---|---|---|
| 0.5 | 2.5 | 0.00002262 | 0.000011310 |
| 1 | 5 | 0.00002563 | 0.000012815 |
| 2 | 10 | 0.00005126 | 0.000025630 |
| 3 | 15 | 0.00005731 | 0.000028655 |
| 4 | 20 | 0.00007249 | 0.000036245 |
| 5 | 25 | 0.00007689 | 0.000038445 |
| 7 | 35 | 0.00009242 | 0.000046210 |
| 9 | 45 | 0.00010567 | 0.000052835 |
| 10 | 50 | 0.00025627 | 0.000128135 |
| 15 | 75 | 0.00026755 | 0.000133775 |
| Required Torque Value [Nm] | Calibrated Weight Value [N] | Standard Uncertainty of the Considered Source [Nm] |
|---|---|---|
| 0.5 | 2.5 | 0.0000065298 |
| 1 | 5 | 0.0000073987 |
| 2 | 10 | 0.0000147975 |
| 3 | 15 | 0.0000165439 |
| 4 | 20 | 0.0000209261 |
| 5 | 25 | 0.0000221962 |
| 7 | 35 | 0.0000266793 |
| 9 | 45 | 0.0000305043 |
| 10 | 50 | 0.0000739788 |
| 15 | 75 | 0.000077235 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kowalski, S.; Barta, D.; Dižo, J.; Dittrich, A. Brake Torque Sensor Calibration Device. Appl. Sci. 2024, 14, 7927. https://doi.org/10.3390/app14177927
Kowalski S, Barta D, Dižo J, Dittrich A. Brake Torque Sensor Calibration Device. Applied Sciences. 2024; 14(17):7927. https://doi.org/10.3390/app14177927
Chicago/Turabian StyleKowalski, Sławomir, Dalibor Barta, Ján Dižo, and Aleš Dittrich. 2024. "Brake Torque Sensor Calibration Device" Applied Sciences 14, no. 17: 7927. https://doi.org/10.3390/app14177927
APA StyleKowalski, S., Barta, D., Dižo, J., & Dittrich, A. (2024). Brake Torque Sensor Calibration Device. Applied Sciences, 14(17), 7927. https://doi.org/10.3390/app14177927








