Revisiting the Numerical Evaluation and Visualization of the Gravity Fields of Asteroid 4769 Castalia Using Polyhedron and Harmonic Expansions Models
Abstract
1. Introduction
2. Gravity Calculation Method
2.1. Polyhedral Method
2.2. Gravitational Acceleration and Coordinate Transformation
3. Asteroid Castalia Shape and Some Parameters
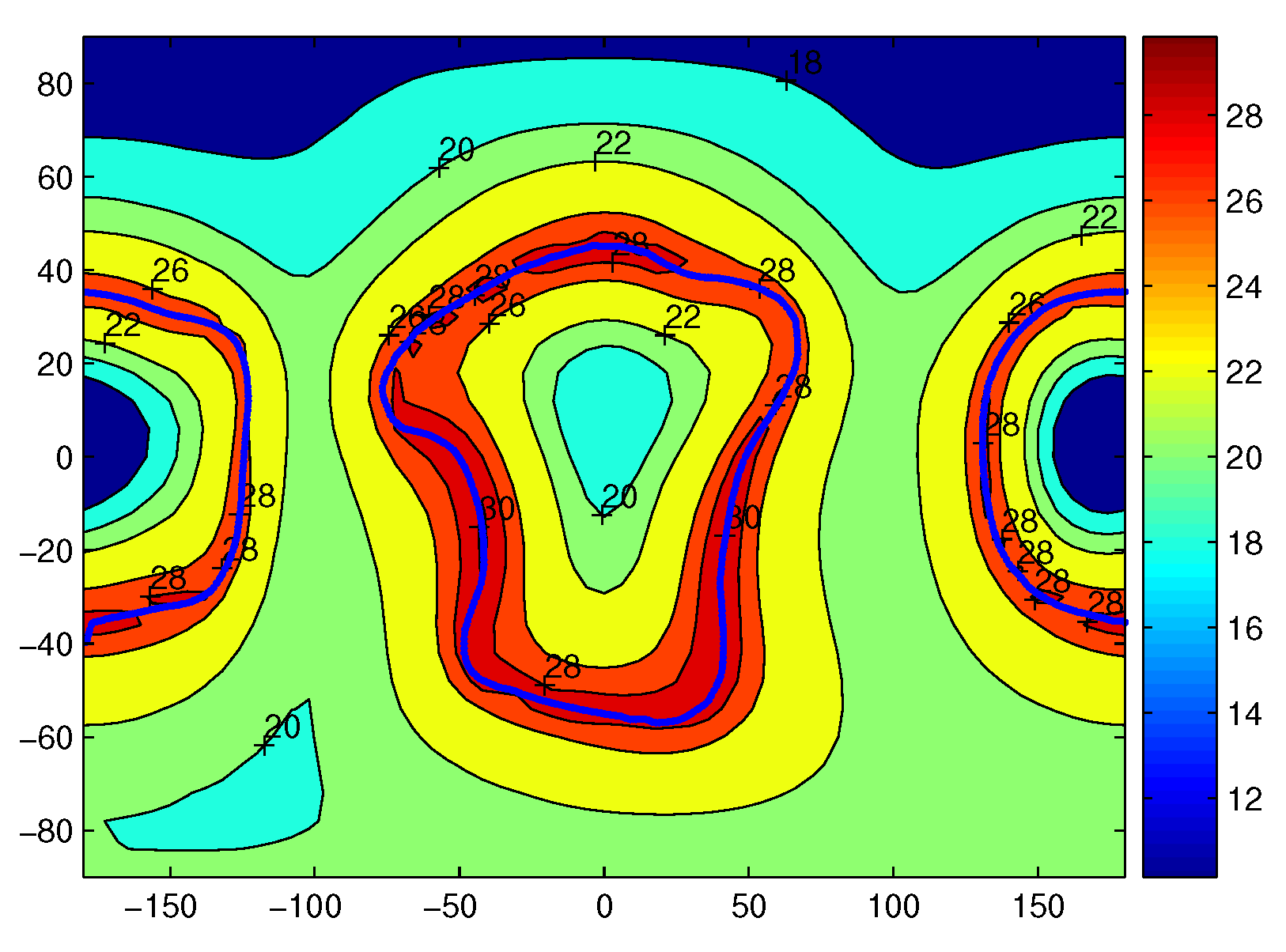
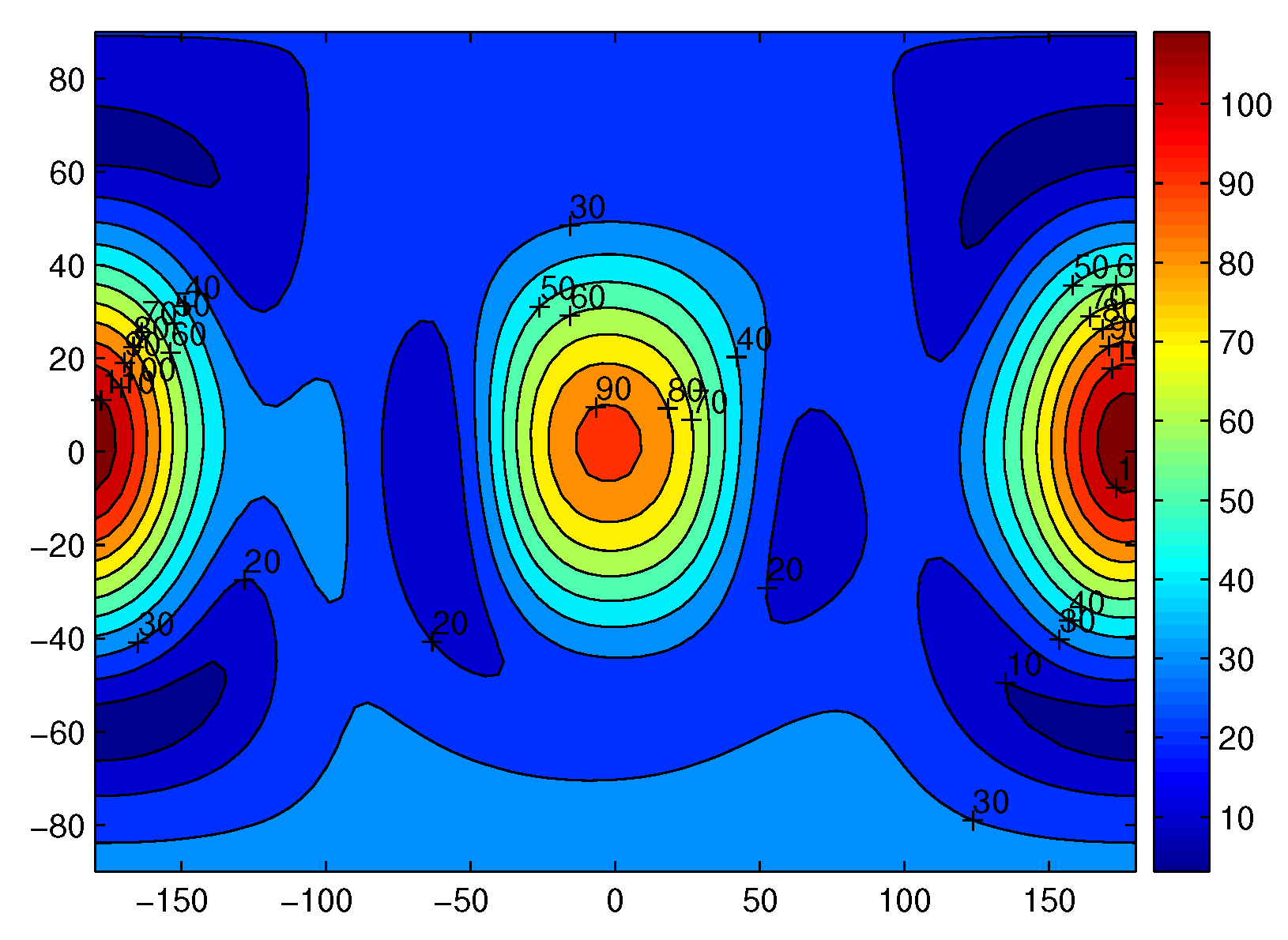
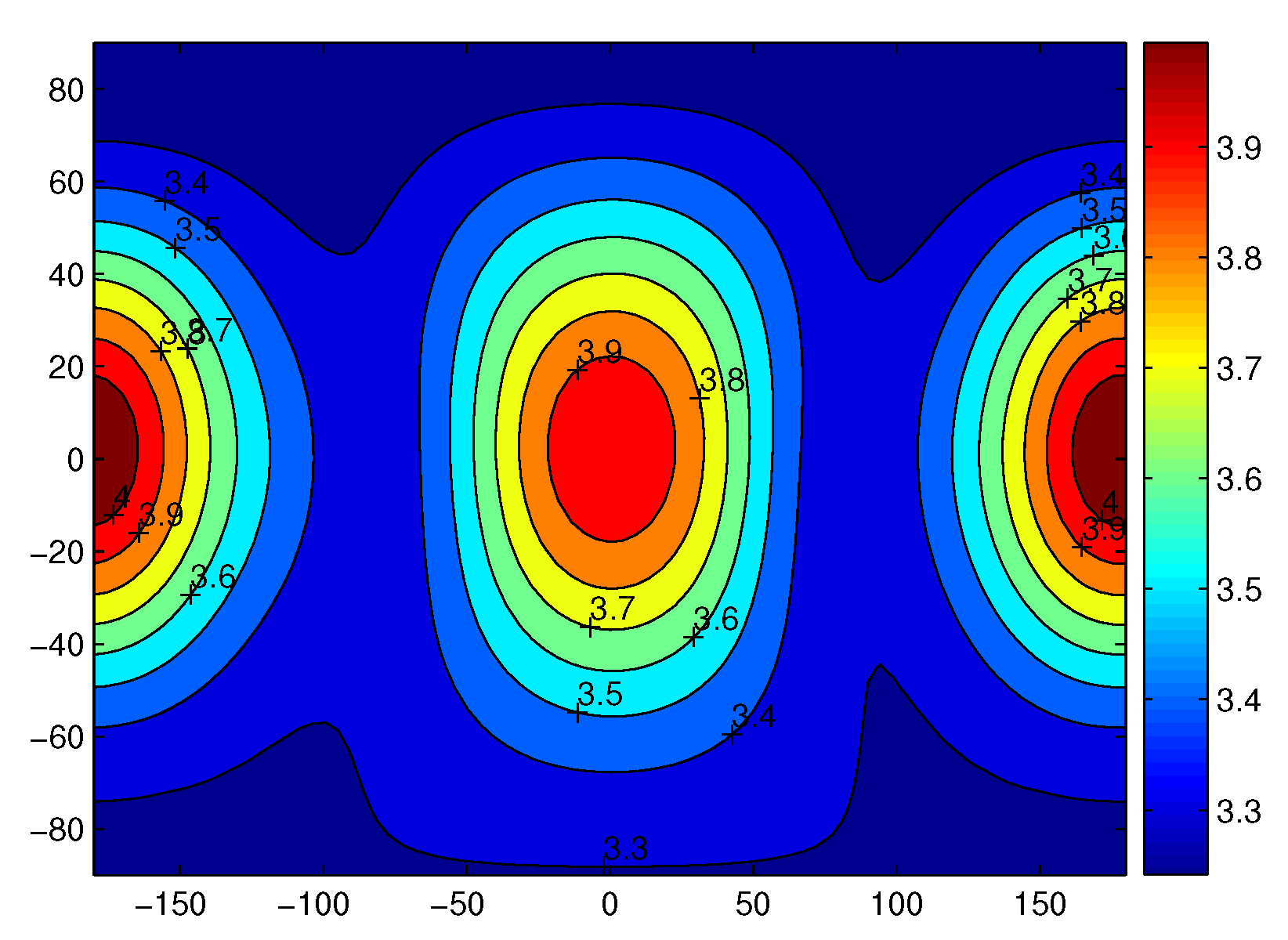
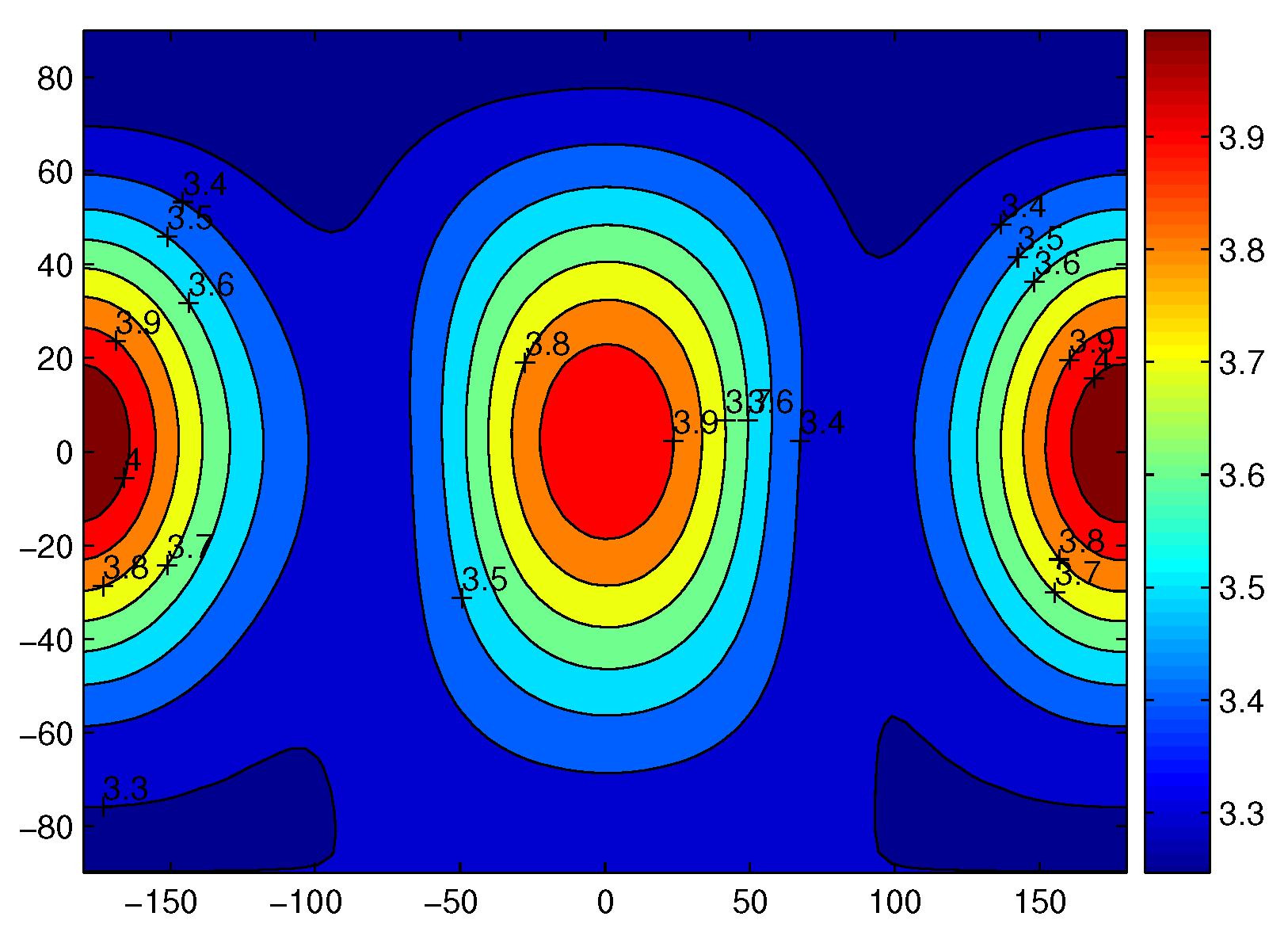
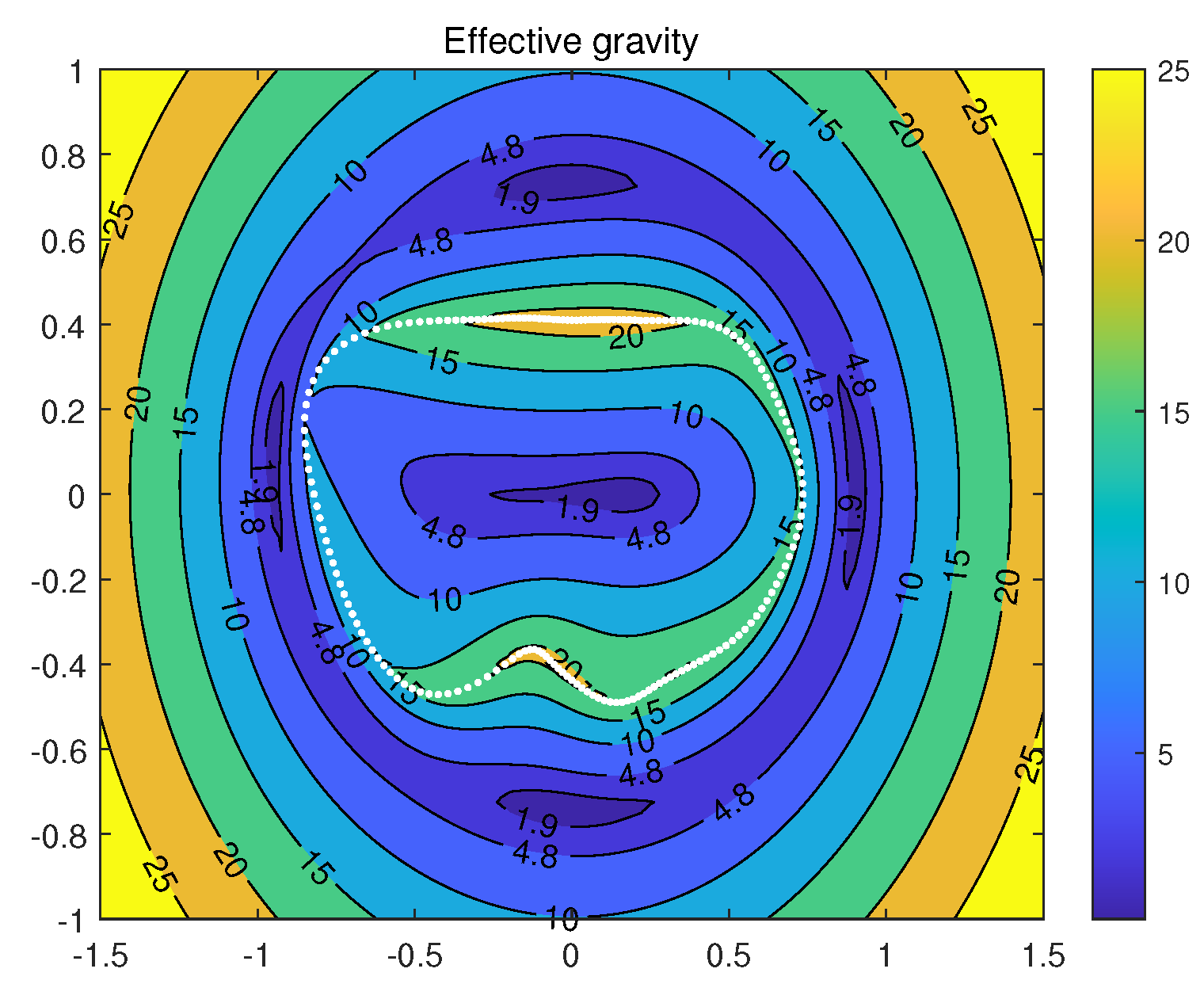
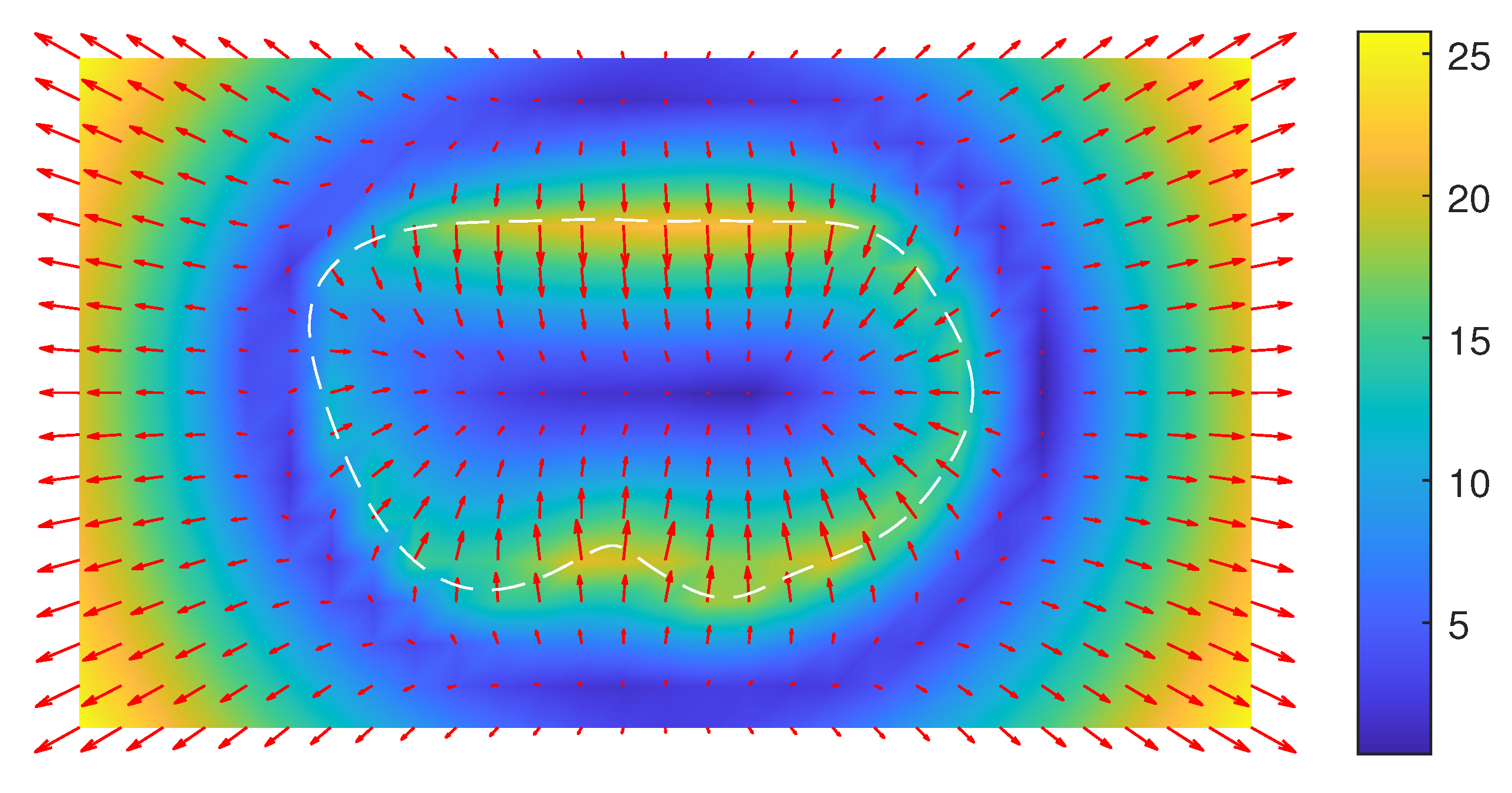
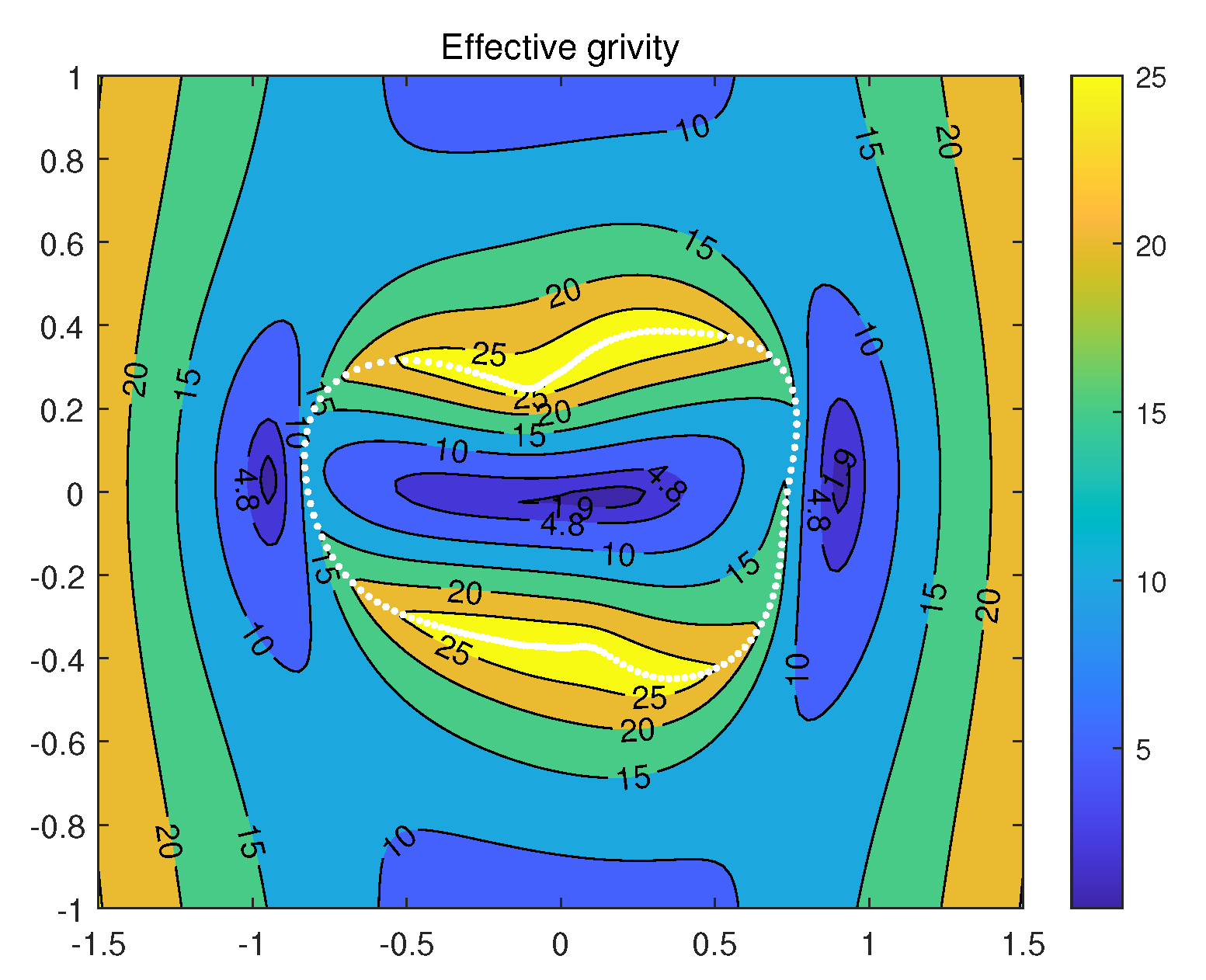
4. Calculation Results and Analysis
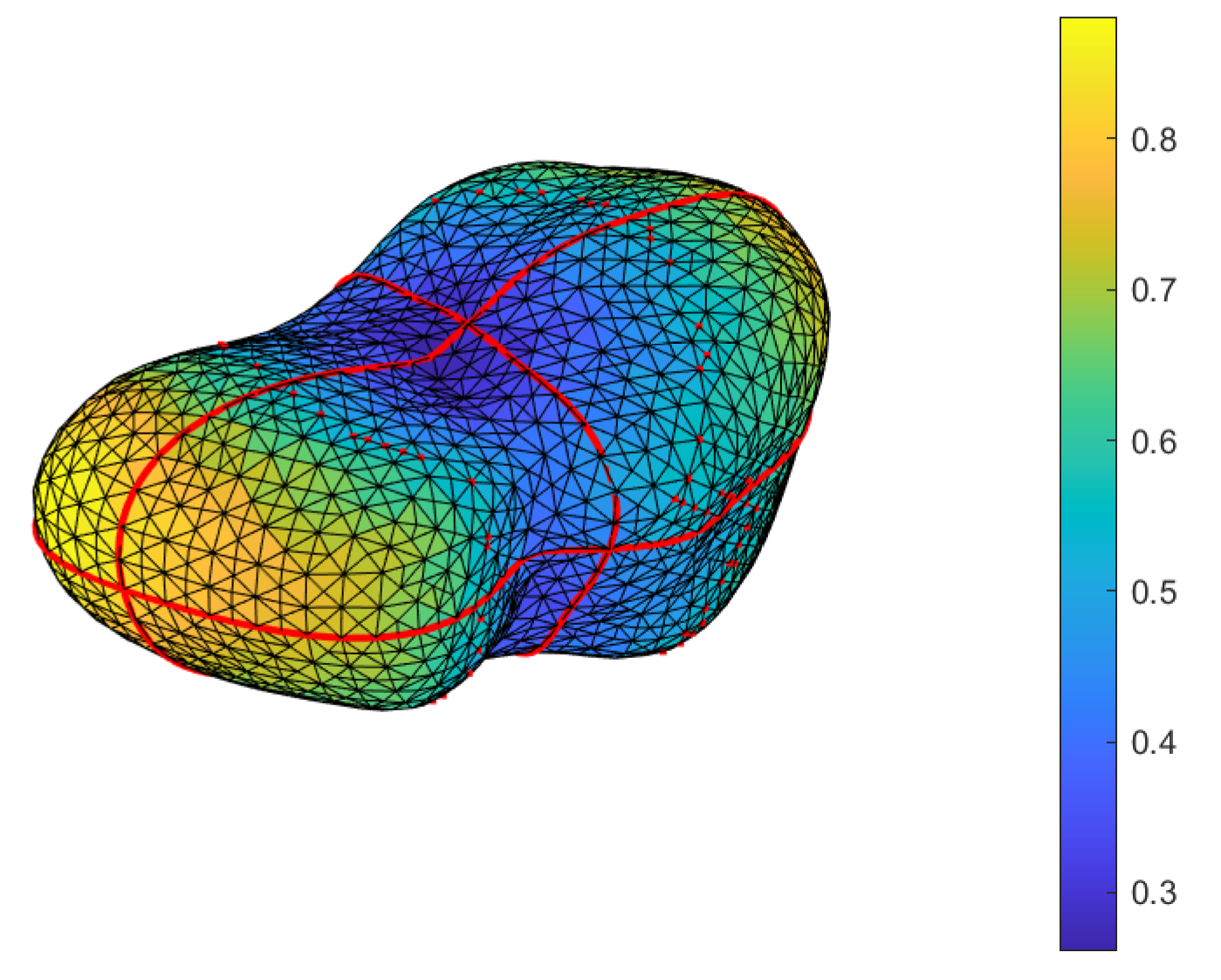
4.1. On the Asteroid Surface
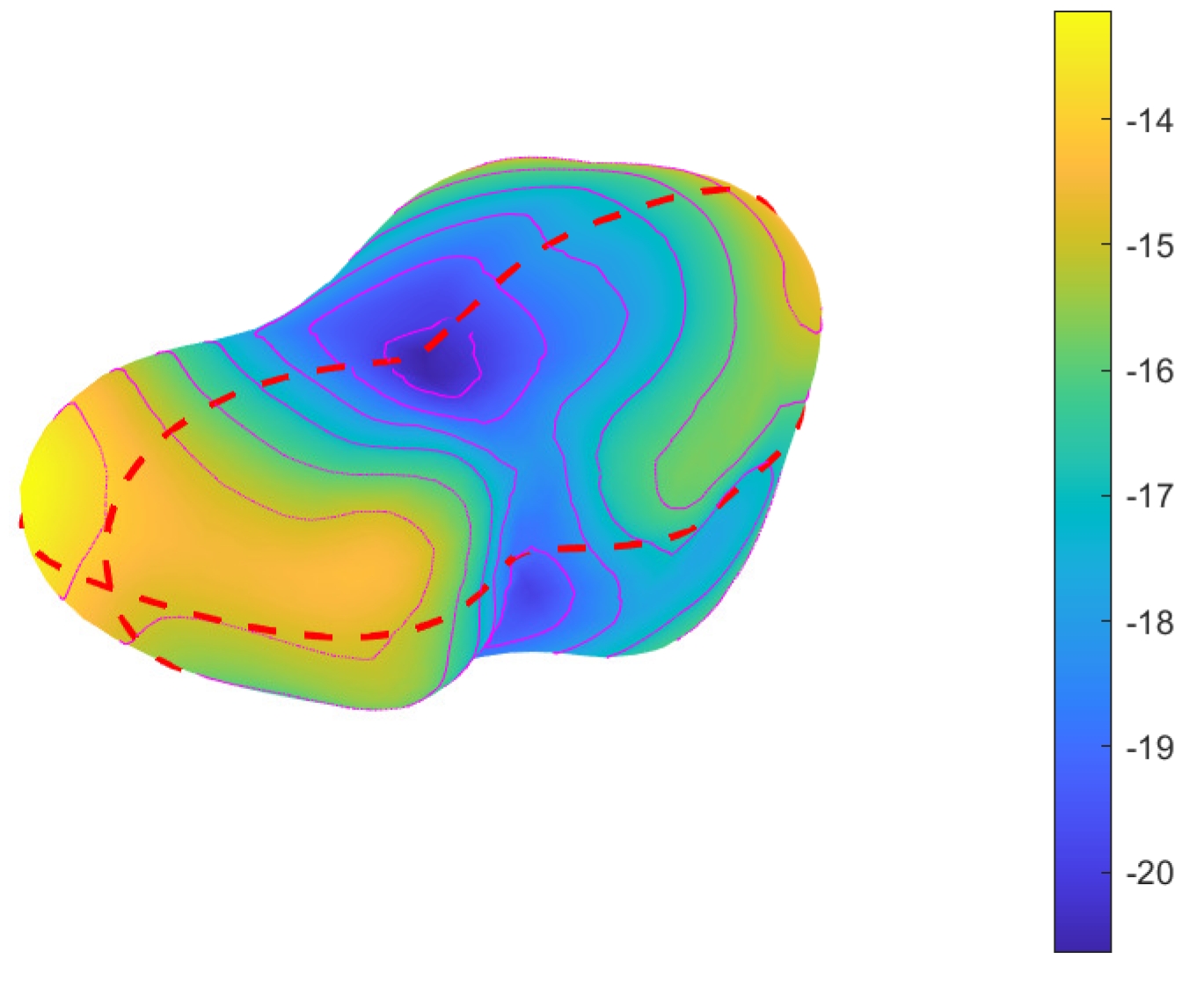
4.1.1. Gravity Potential and Acceleration by Polyhedral Model
4.1.2. Harmonic-Expansion Method
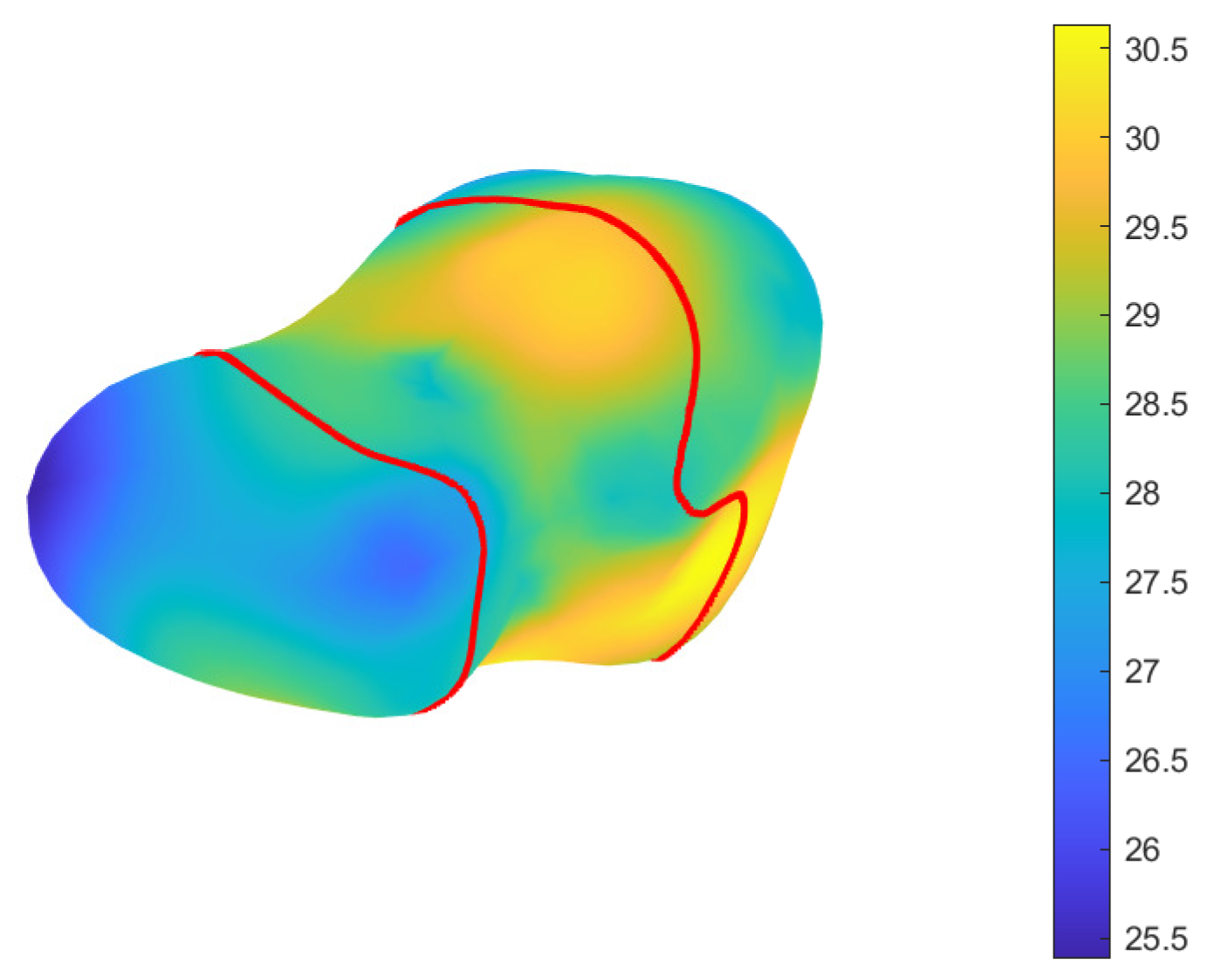
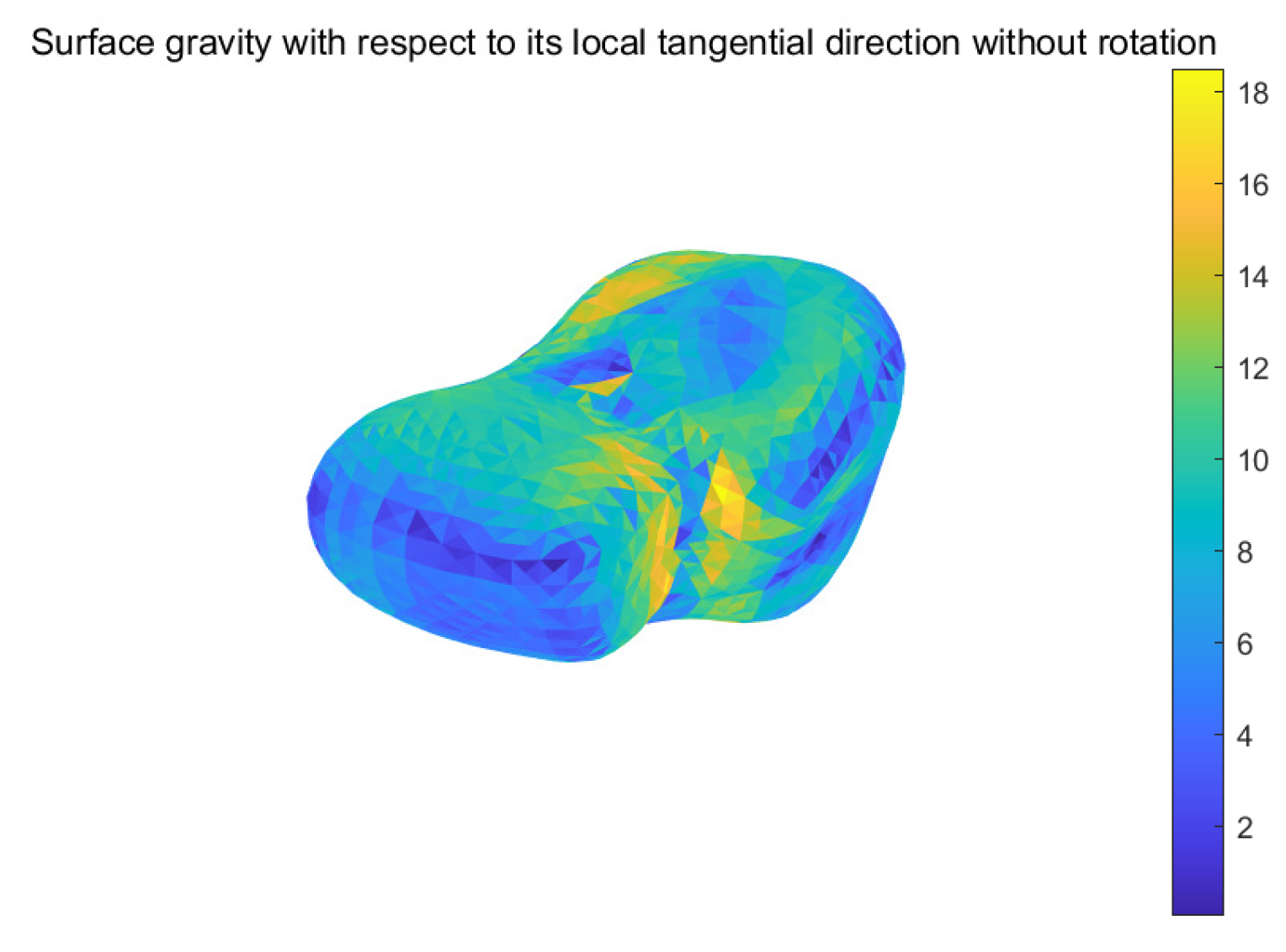
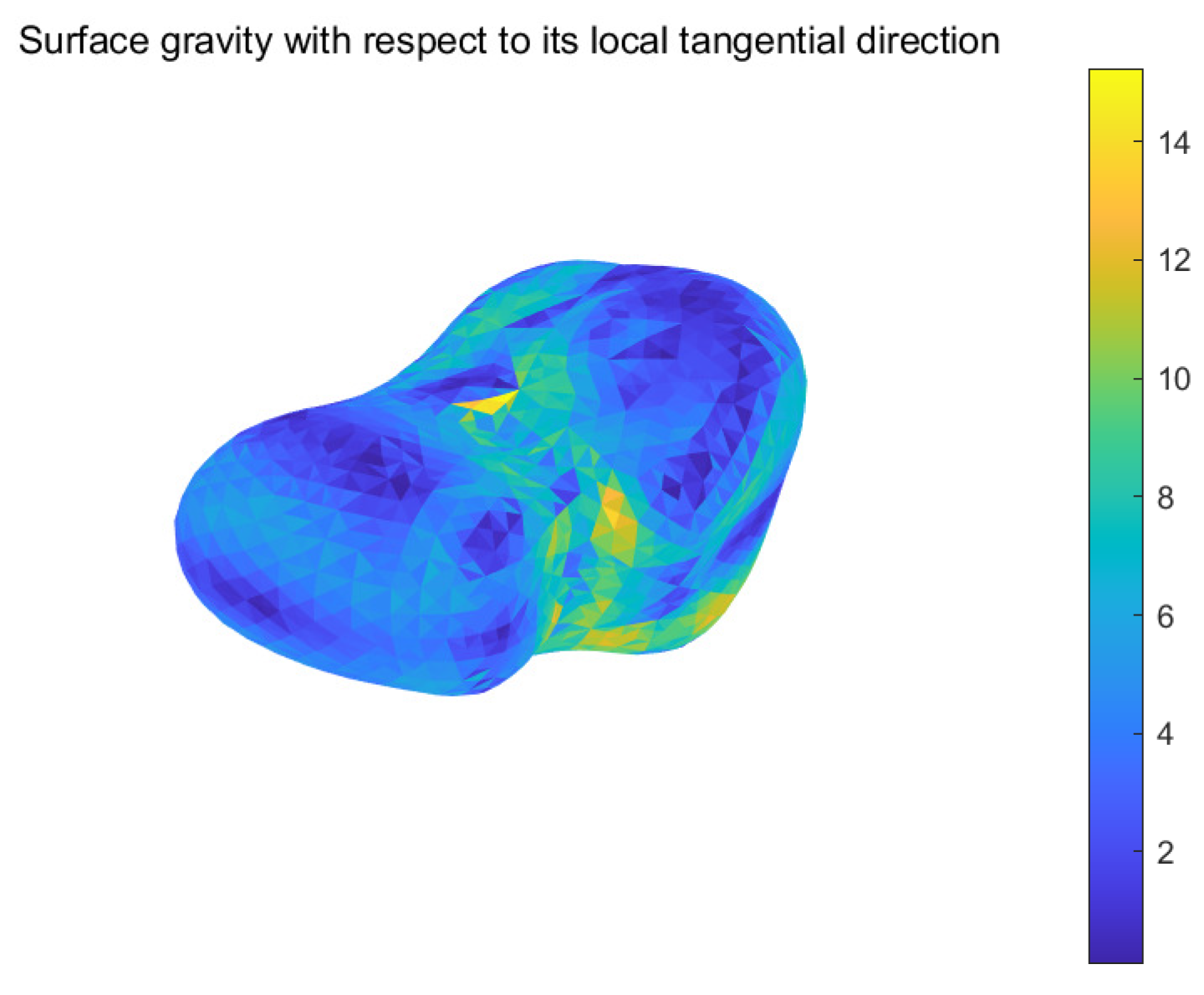
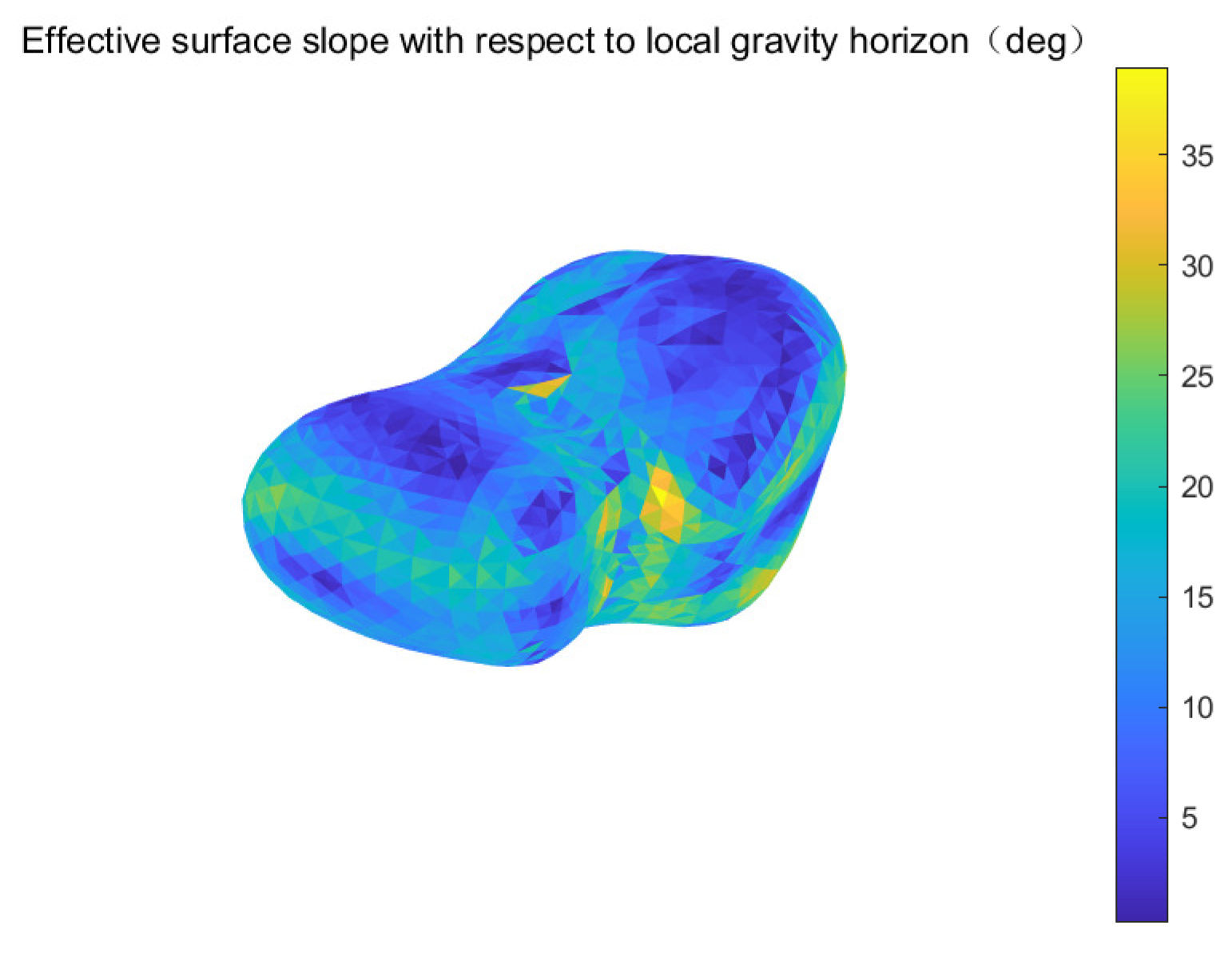
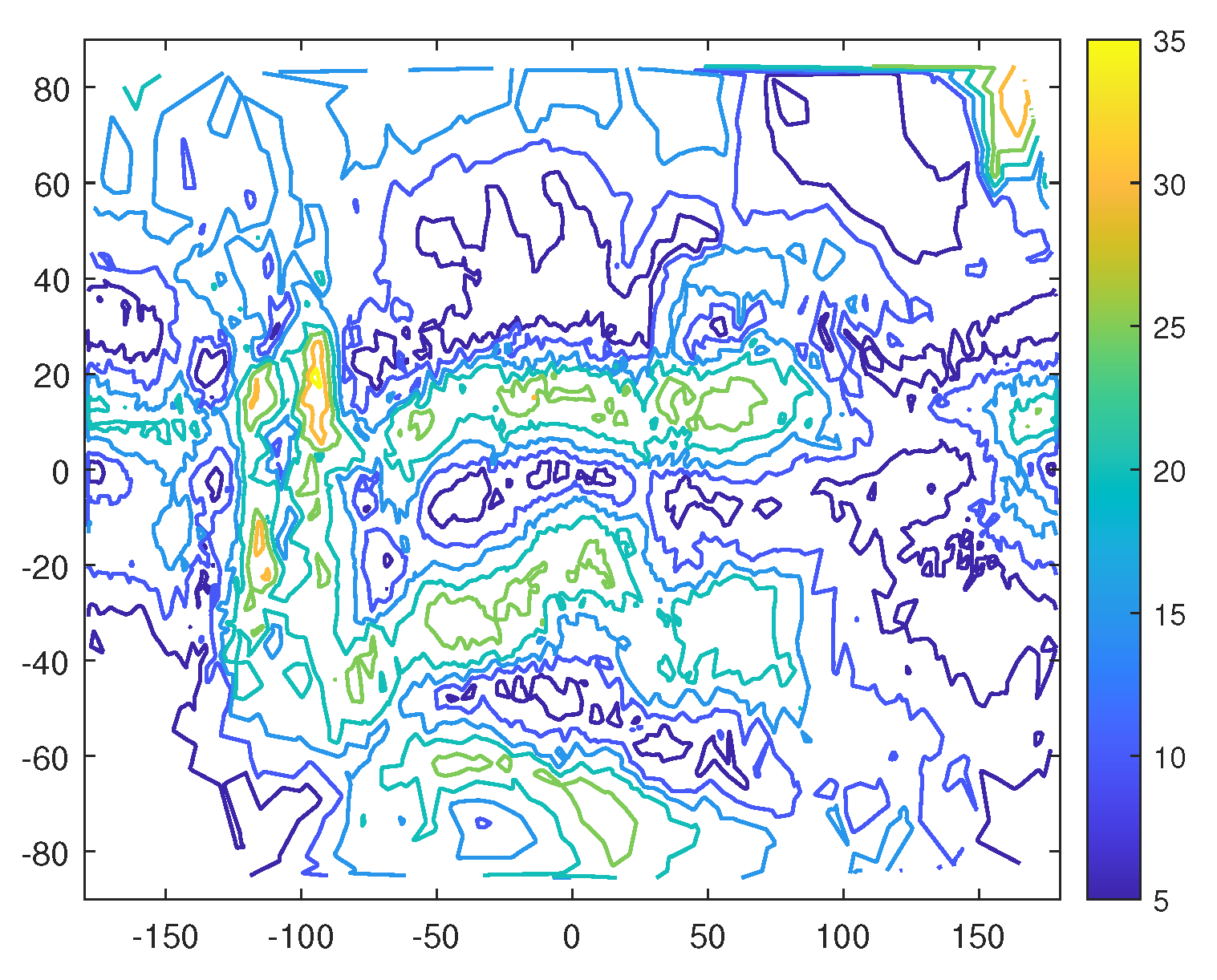
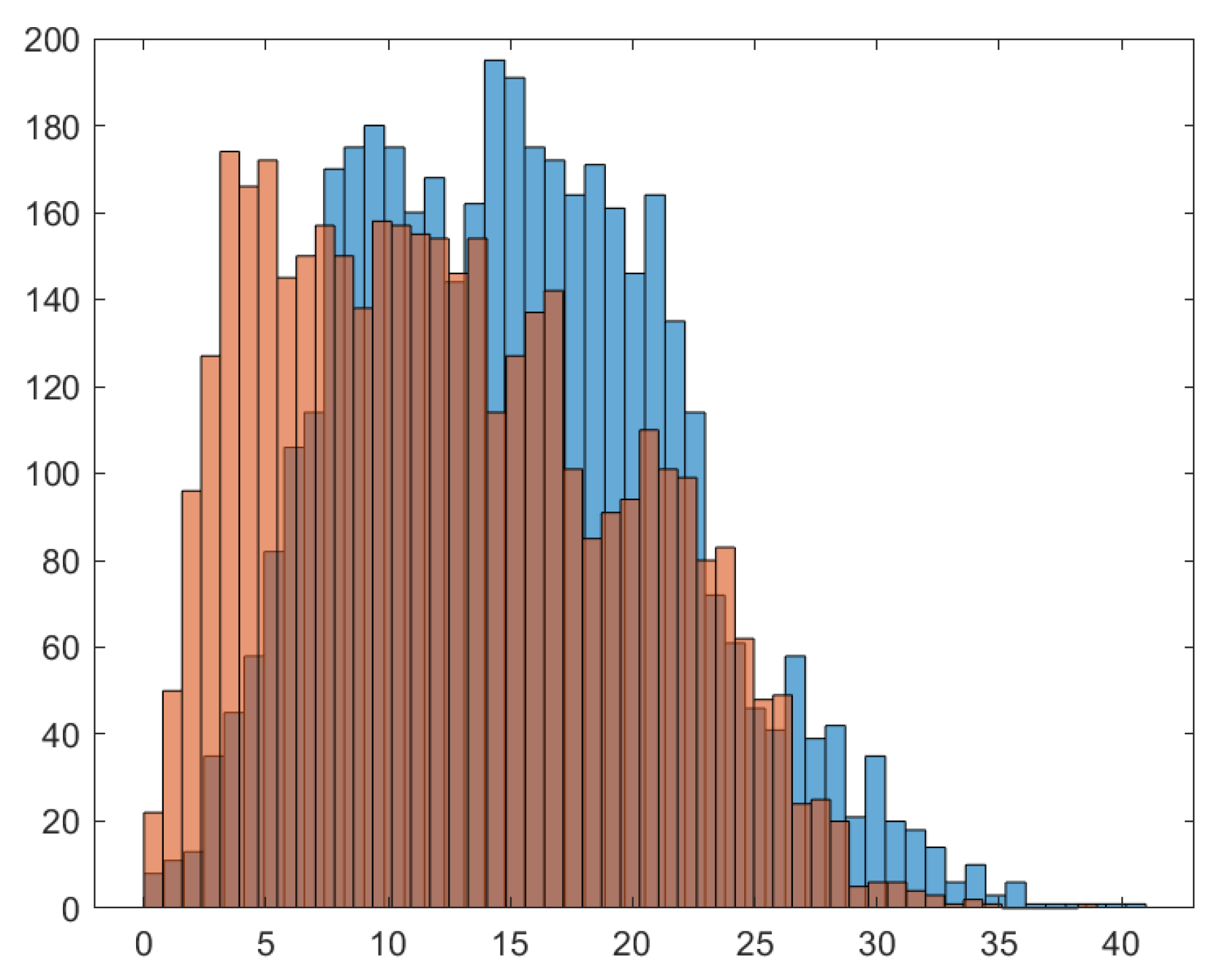
4.1.3. Gravity Tangential Acceleration and Slopes
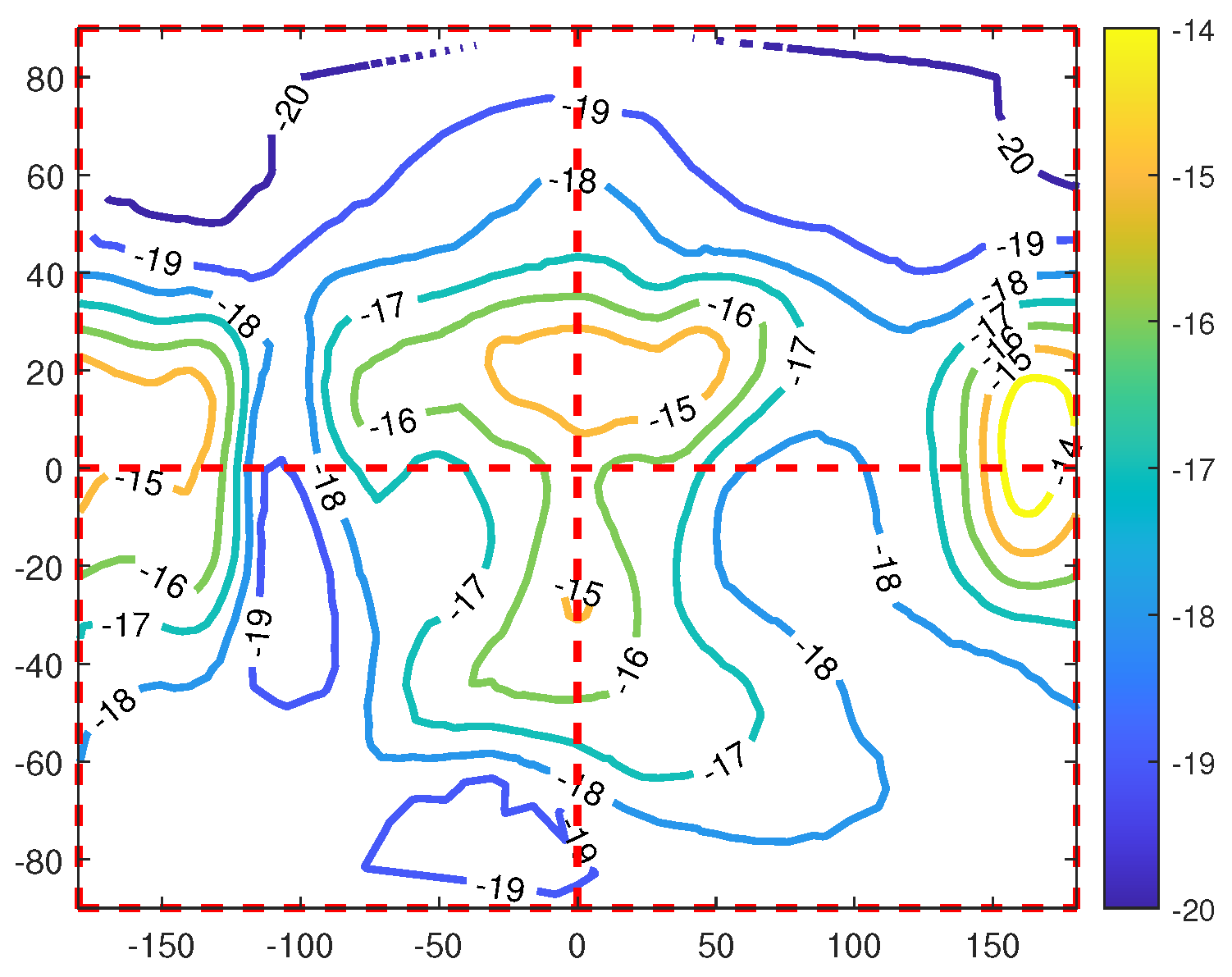
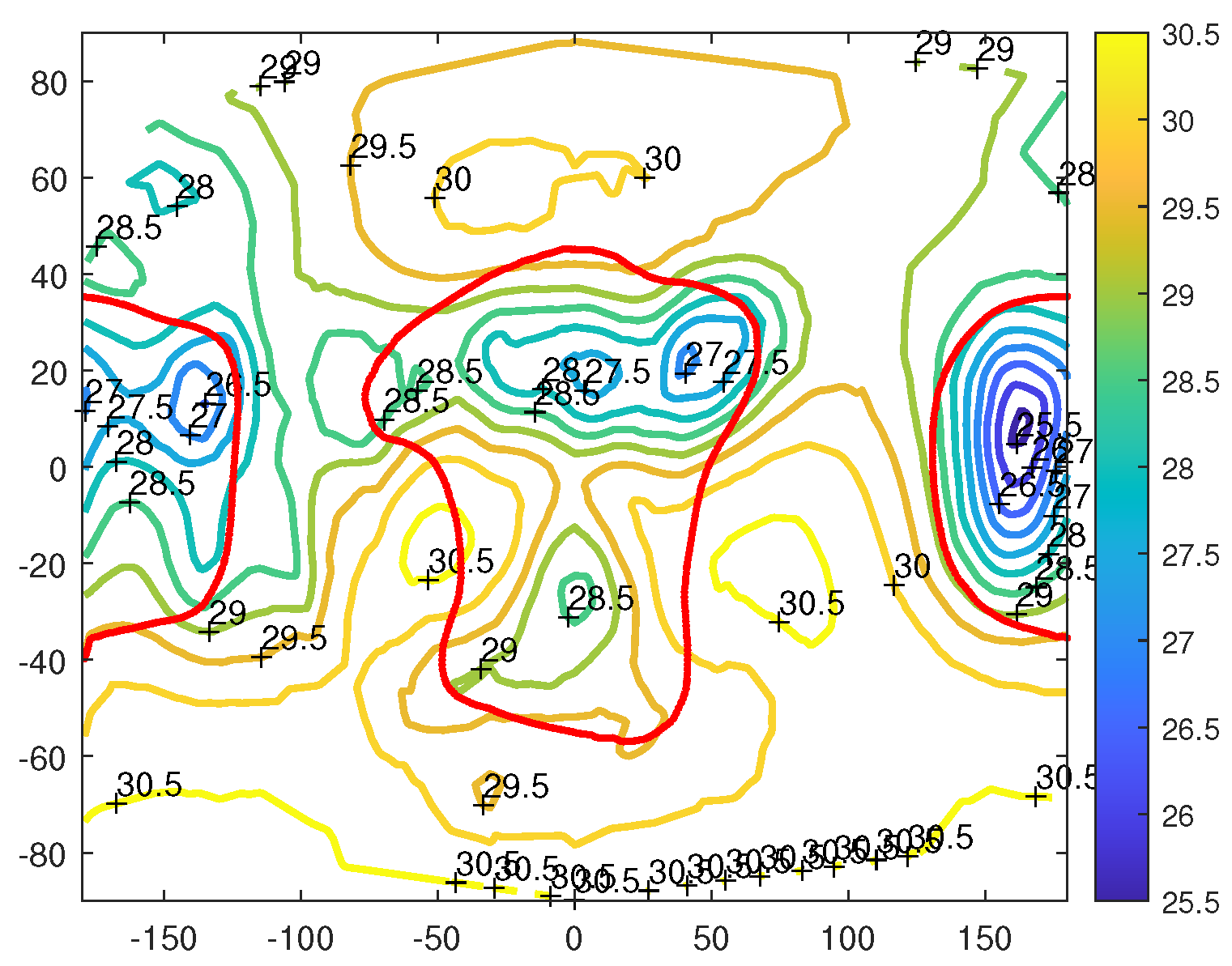
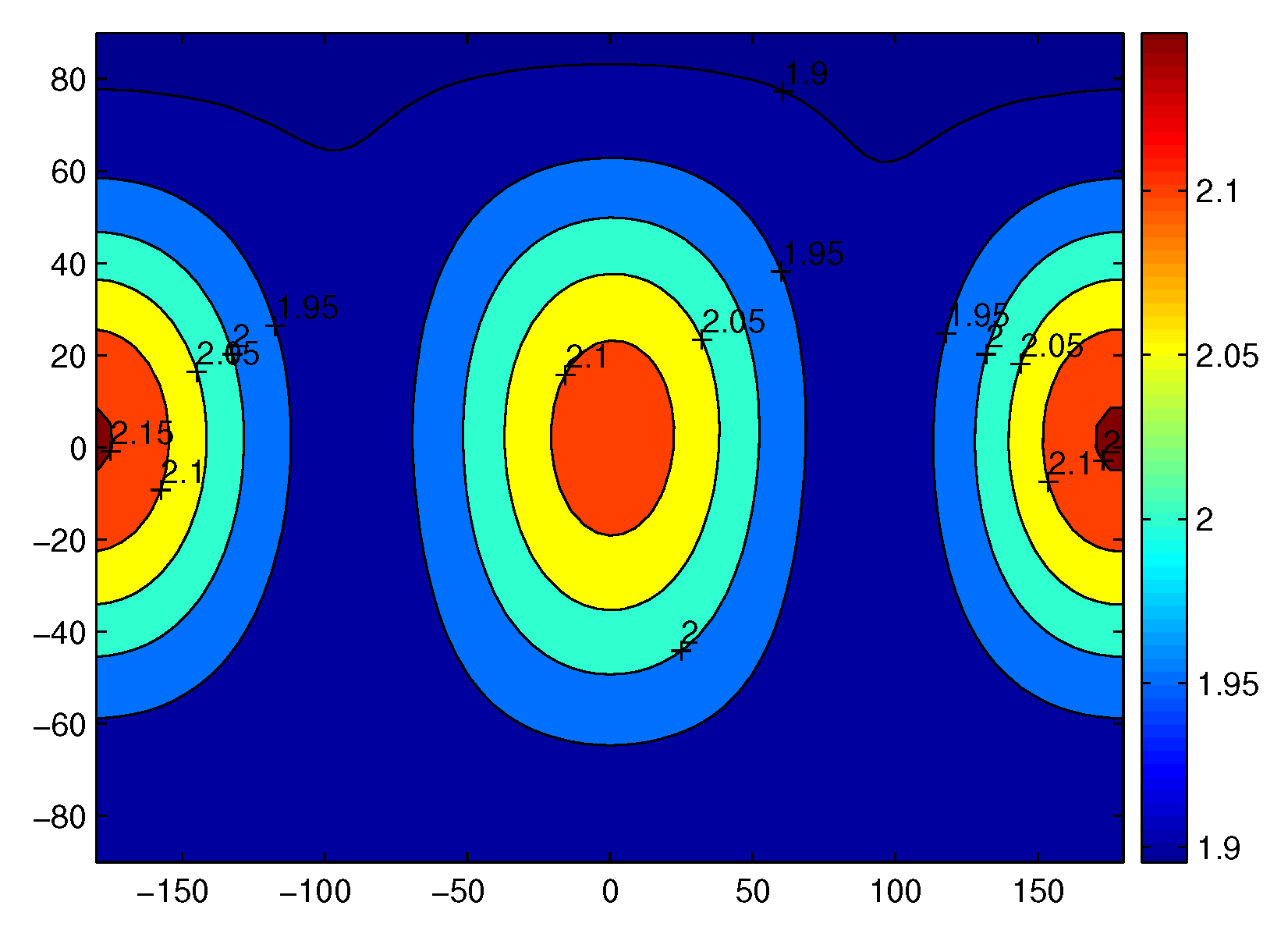
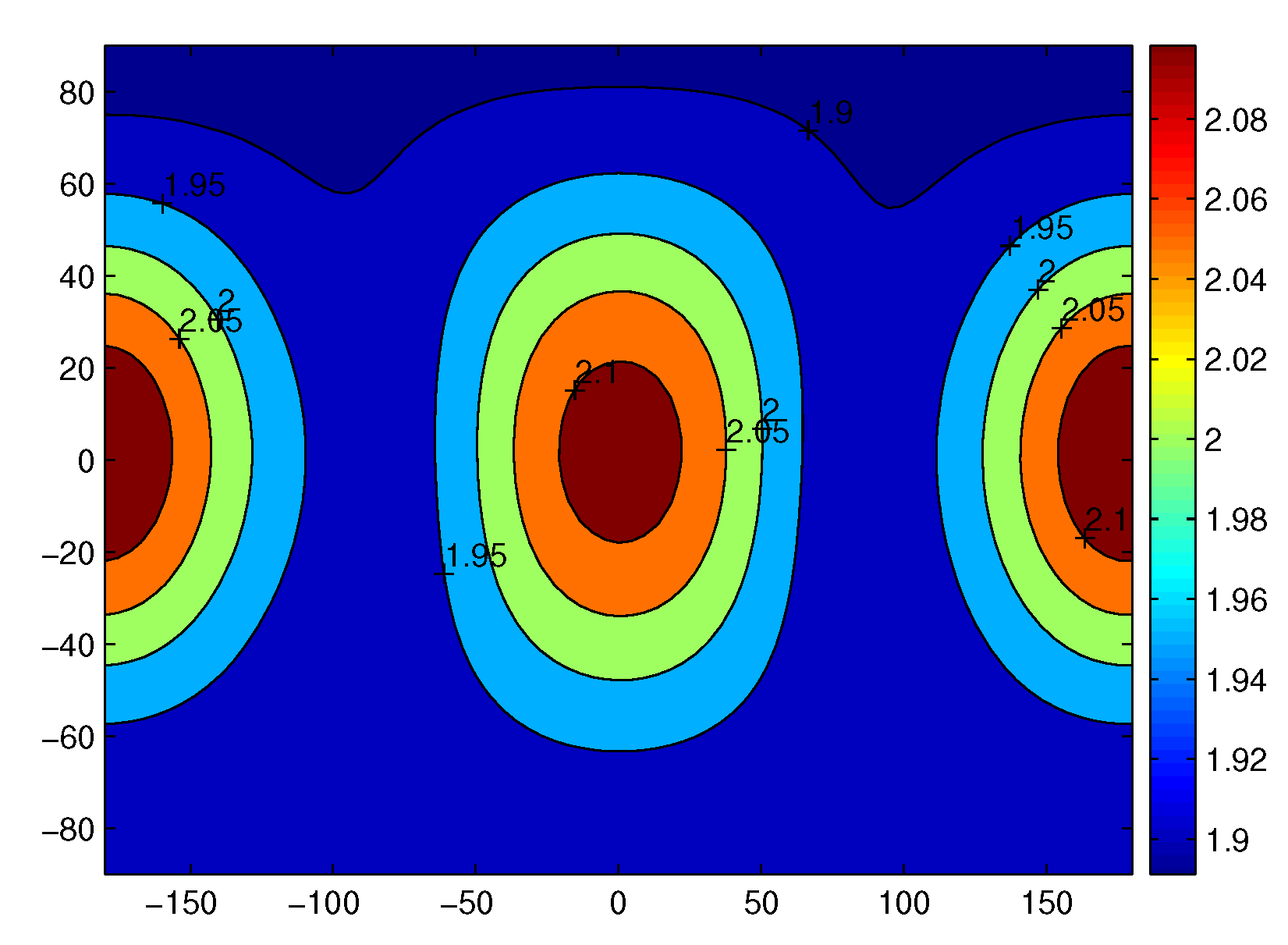
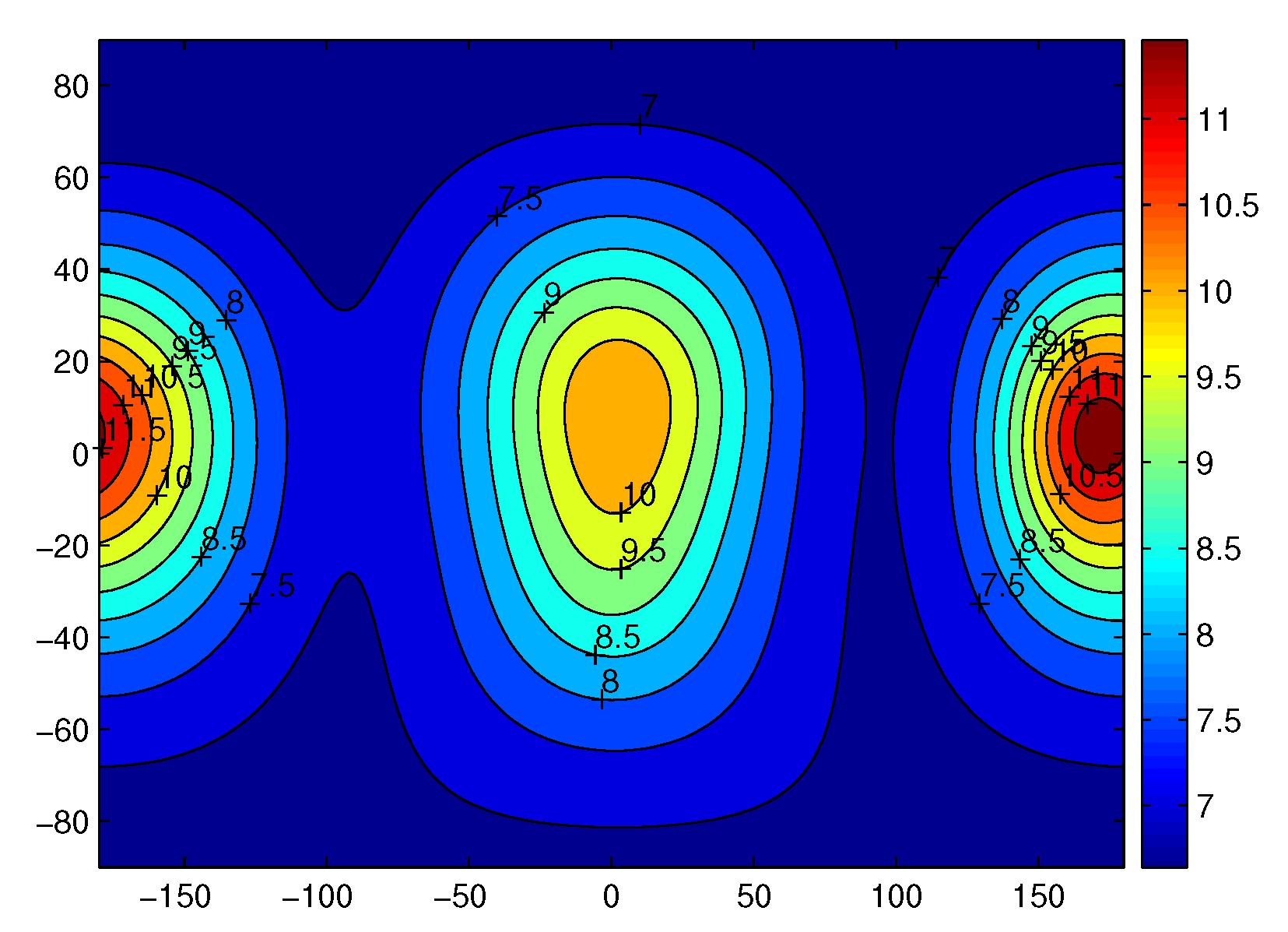
4.2. Gravity on Spheres Intersecting or Outside the Asteroid
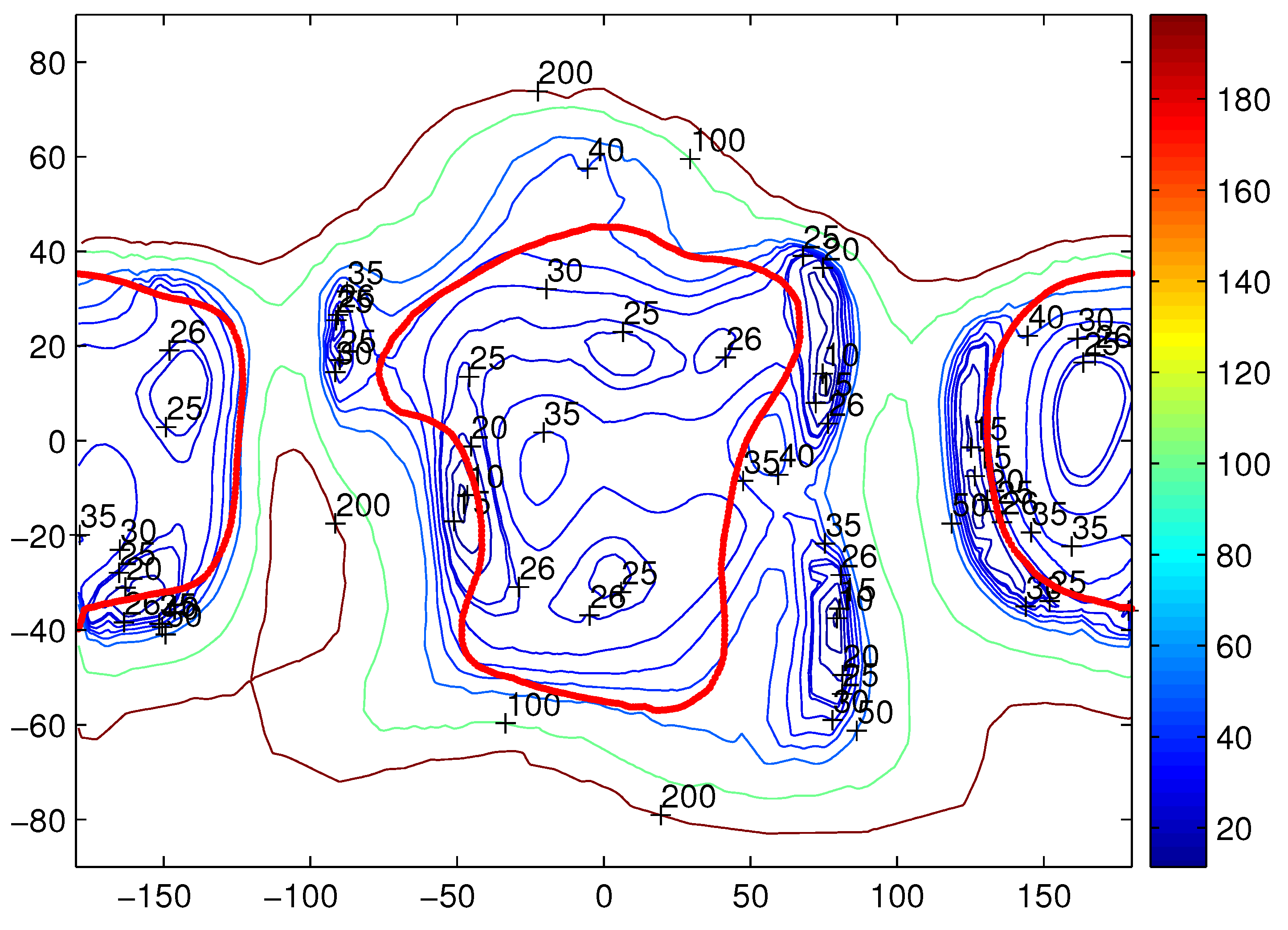
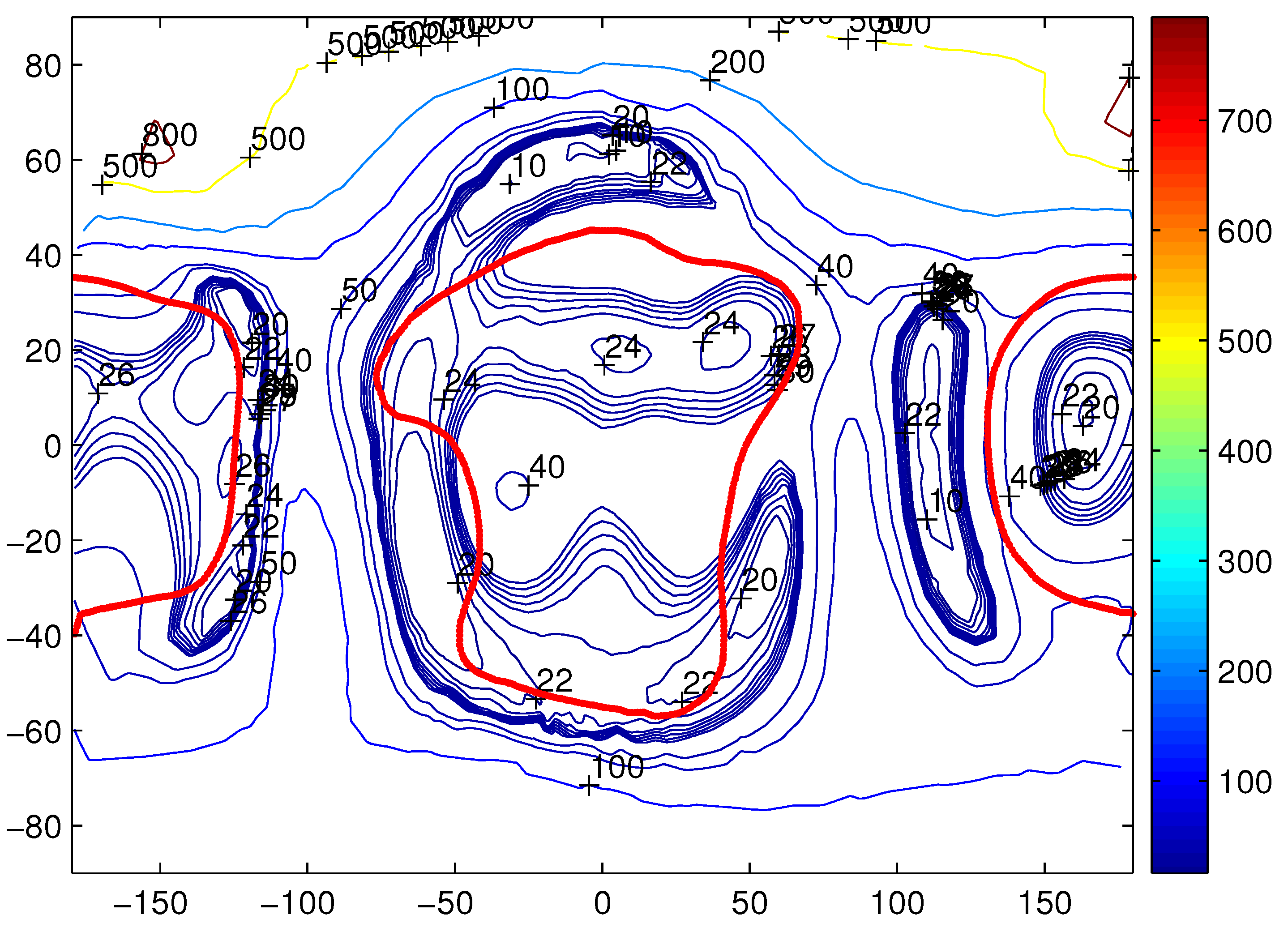
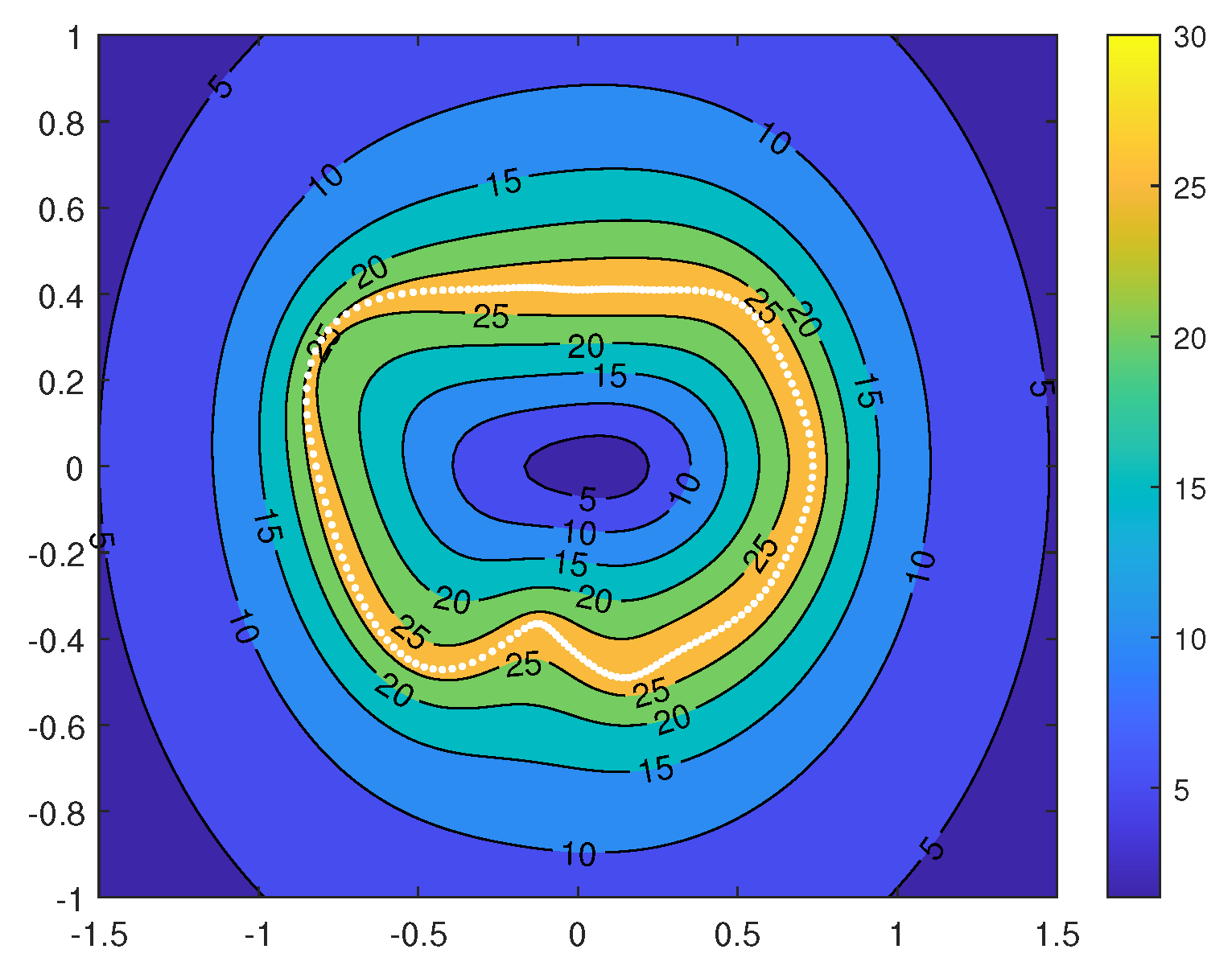
4.3. Gravitational Accelerations on Intersecting Planes
5. Conclusions and Discussion
- (1)
- If the calculated sphere’s radius is larger than the maximum radius of the asteroid, for example, if the radius is greater than 2 = 1.0862 km here, the gravity harmonics work as well as polyhedron method; the farther the better, and the higher the degree the better. The sphere with the maximum radius of the asteroid is the so-called Brillouin sphere [8]. The maximum radius for the asteroid Castalia is 0.851 km. See Equation (1), for all the calculated spheres with the radius , , for both the polyhedral and spherical harmonic models.
- (2)
- For preliminary analysis, the 4th-order and degree gravity model is good enough, especially for spheres with , and not much of an obvious difference can be obtained by using the 8th- or 16th-order model or the polyhedron method with less than 1% error. The farther from the asteroid, the lower the order of the coefficient dominating the gravity. See Figure 20, Figure 25 and Figure 26, etc.
- (3)
- On surface points where the radius is less than the reference radius and the points inside the asteroid, only polyhedron method can obtain reasonable results.
- (4)
- For the points outside the asteroid or on the surface of the asteroid, if its radius is greater than reference or less than its maximum radius, the harmonic expansion model still works with relatively large errors but still with acceptable accuracy; i.e., it still converges with 5% error, and the higher order of the model of harmonic functions, the closer to the polyhedra model’s results. See Equation (1), . As all the coefficients and are much less than 1, and for the normalized Legendre polynomial , it is easy to understand that all the points with converge.A rough proof for understanding the above comments is as follows.
- (5)
- Again, more clearly, if the radius is inside the Brillouin sphere, say km for Castalia, but larger than its mean reference radius, km, the spherical harmonic-expansion method still works, and the higher the degree and order that are chosen, the higher accuracy that can be obtained. This is an important conclusion reached in this paper, which is more specific than the previous discussions [8].
- (6)
- For the gravity inside the reference radius, the polyhedral method without much modification can still apply both on and inside the surface of Castalia. This is another important conclusion obtained in this paper.
- (7)
- Based on the calculation of the minimum overall mean effective slope, we found that the density of Castalia could be more likely 2.9 g/cm than the commonly assumed 2.1. This is the third special contribution of this paper.
- (8)
- In a word, the spherical harmonic expansion model only works at a position . When it is near , a higher-order model presents a better estimation of gravity.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Scheeres, D.; Ostro, S. Orbits close to asteroid 4769 Castalia. Icarus 1996, 121, 67–87. [Google Scholar] [CrossRef]
- Scheeres, D. Orbital Motion in Strongly Perturbed Environments; Springer Praxis Books: Berlin/Heidelberg, Germany, 2014. [Google Scholar]
- Hu, W.; Scheeres, D. Numerical determination of stability regions for orbital motion in uniformly rotating second degree and order gravity fields. Planet. Space Sci. 2004, 52, 685–692. [Google Scholar] [CrossRef]
- Hu, W.; Scheeres, D. Averaging analyses for spacecraft orbital motions around asteroids. Acta Mech. Sinica 2014, 30, 294–300. [Google Scholar] [CrossRef]
- Yada, T.; Abe, M.; Okada, T.; Nakato, A.; Yogata, K.; Miyazaki, A.; Hatakeda, K.; Kumagai, K.; Nishimura, M.; Hitomi, Y.; et al. Preliminary analysis of the Hayabusa2 samples returned from C-type asteroid Ryugu. Nat. Astron. 2022, 6, 214–220. [Google Scholar] [CrossRef]
- Liu, C.; Guo, W.; Hu, W.; Chen, R.; Liu, J. Real-time crater-based monocular 3-D pose tracking for planetary landing and navigation. IEEE Trans. Aero. Elec. Syst. 2023, 59, 311–335. [Google Scholar] [CrossRef]
- Werner, R.; Scheeres, D. Exterior gravitation of a polyhedron derived and compared with harmonic and mascon gravitation representations of asteroid 4769 Castalia. Celest. Mech. Dyn. Astron. 1997, 65, 313–344. [Google Scholar] [CrossRef]
- Takahashi, Y.; Scheeres, D. Surface gravity fields for asteroids and comets. AIAA J. Guid. Control. Dyn. 2013, 36, 362–374. [Google Scholar] [CrossRef]
- Sebera, J.; Bezdek, A.; Pešek, I.; Henych, T. Spheroidal models of the exterior gravitational field of asteroids Bennu and Castalia. Icarus 2016, 272, 70–79. [Google Scholar] [CrossRef]
- Chauvineau, B.; Farinella, P.; Mignard, F. Planar orbits about a triaxial body: Application to asteroidal satellite. Icarus 1993, 105, 370–384. [Google Scholar] [CrossRef]
- Dobrovolskis, A. Classifacation of ellipsoids by shape and surface gravity. Icarus 2019, 321, 891–928. [Google Scholar] [CrossRef]
- Pearl, J.; Hitt, D. Mascon distribution techniques for asteroids and comets. Cele. Mech. Dyn. Astro. 2022, 134, 58. [Google Scholar] [CrossRef]
- Sanchez, J.C.; Schaub, H. Simutaneous navigation and mascon gravity estimation around small bodies. ACTA Astronaut. 2023, 213, 725–740. [Google Scholar] [CrossRef]
- Elkins-Tanton, L.T.; Asphaug, E.; Bell, J.F., III; Bercovici, H.; Bills, B.; Binzel, R.; Bottke, W.F.; Dibb, S.; Lawrence, D.J.; Marchi, S.; et al. Observations, meterorites, and models: A preflight assessment of the composition and formation of (16) Psyche. JGR Planets 2020, 125, e2019JE006296. [Google Scholar] [CrossRef] [PubMed]
- Della, G.; Ballouz, R.; Walsh, K.; Marusiak, A.G.; Bray, V.J.; Bailey, S.H. Seismology of rubble-pile asteroids in binary systems. Mon. Not. R. Astron. Soc. 2024, 10348–10357. [Google Scholar]
- Yin, Z.; Sneeuw, N. Modeling the gravityational field by using CFD techniques. J. Geod. 2021, 95, 68–78. [Google Scholar] [CrossRef]
- Duan, Y.; Yin, Z.; Zhang, K.; Zhang, S.; Wu, S.; Li, H.; Zheng, N.; Bian, C. Modeling the gravityational field of the ore-bearing asteroid by using the CFD-based method. Acta Astronaut. 2024, 215, 664–673. [Google Scholar] [CrossRef]
- Kaula, W. Theory of Satellite Geodesy; Blaisdel: Waltham, MA, USA, 1966; Chapter 1; pp. 1–11. [Google Scholar]
- Hu, W. Fundamental Spacecraft Dynamics and Control; John Wiley and Sons: Singapore, 2015; pp. 123–127, 165–167, 173–184. [Google Scholar]
- Hudson, R.; Ostro, S. Shape of asteroid 4769 Castalia (1989 PB) from inversion of radar images. Science 1994, 263, 940–943. [Google Scholar] [CrossRef]
- MacMillan, W.D. The Theory of the Potential; McGraw-Hill: New York, NY, USA; Dover, NY, USA, 1958. [Google Scholar]
- Thomas, P.C.; Veverka, J.; Smonelli, D.; Helfenstein, P.; Carcich, B.; Belton, M.J.S.; Davies, M.E.; Chapman, C. The shape of Gaspra. Icarus 1994, 107, 23–36. [Google Scholar] [CrossRef]
- Scheeres, D.J.; McMahon, J.W.; French, A.S.; Brack, D.N.; Chesley, S.R.; Farnocchia, D.; Takahashi, Y.; Leonard, J.M.; Geeraert, J.; Page, B.; et al. The dynamic geophysical evironment of (101955) Bennu based on OSIRIS-REx measurements. Nat. Astron. 2019, 3, 352–361. [Google Scholar] [CrossRef]
- Pearl, J.; Louisons, W.; Hitt, D. Hybrid Gravity Model from Asteroid Surface Topology. In Proceedings of the AIAA Space Flight Mechanics Meeting, Kissimmee, FL, USA, 8–12 January 2018. [Google Scholar]
- Arora, N.; Russell, R. Efficient interpolation of high-fidelity geopotentials. AIAA J. GCD 2016, 39, 128–143. [Google Scholar] [CrossRef]
- Fukushima, T. Precise and fast computation of the gravitational field of a general finite body and its application to the gravitational study of asteroid Eros. Astron. J. 2017, 154, 145. [Google Scholar] [CrossRef]








| Castalia | Rectangle (1.7 × 0.9 × 0.8) | ||||
|---|---|---|---|---|---|
| n | m | C | S | C | S |
| 0 | 0 | 1.00000000000000 | 0 | 1.000000000000000 | 0 |
| 1 | 0 | −0.00014168122220 | 0 | 0.000000000000000 | 0 |
| 1 | 1 | −0.00004075795821 | −0.00002282770478 | 0.000000000000000 | 0.000000000000000 |
| 2 | 0 | −0.10473883593850 | 0 | −0.102404625451483 | 0 |
| 2 | 1 | 0.00005077855278 | −0.00001641325376 | 0.000000000000000 | 0.000000000000000 |
| 2 | 2 | 0.14774171385537 | −0.00007350942982 | 0.152450260149168 | 0.000000000000000 |
| 3 | 0 | −0.01992884455238 | 0 | 0.000000000000000 | 0 |
| 3 | 1 | −0.03410426627931 | 0.00074227942951 | 0.000000000000000 | 0.000000000000000 |
| 3 | 2 | 0.01165825781331 | 0.00224122654733 | 0.000000000000000 | 0.000000000000000 |
| 3 | 3 | 0.01771996117460 | −0.01186045941413 | 0.000000000000000 | 0.000000000000000 |
| 4 | 0 | 0.03510656662646 | 0 | 0.017542426440102 | 0 |
| 4 | 1 | 0.00260220108679 | 0.00163584922657 | 0.000000000000000 | 0.000000000000000 |
| 4 | 2 | −0.04830939903696 | 0.00311423803618 | −0.039143149995087 | 0.000000000000000 |
| 4 | 3 | 0.00690976107455 | −0.00383406677217 | 0.000000000000000 | 0.000000000000000 |
| 4 | 4 | 0.04473930002048 | −0.01216108958396 | 0.019151577641493 | 0.000000000000000 |
| 5 | 0 | 0.02398903330765 | 0 | 0.000000000000000 | 0.000000000000000 |
| 5 | 1 | 0.03681372375277 | −0.00231269356380 | 0.000000000000000 | 0.000000000000000 |
| 5 | 2 | −0.02285422699738 | −0.00181873143701 | 0.000000000000000 | 0.000000000000000 |
| 5 | 3 | −0.02349704830265 | 0.00997187133947 | 0.000000000000000 | 0.000000000000000 |
| 5 | 4 | 0.00652991532599 | −0.00084721036230 | 0.000000000000000 | 0.000000000000000 |
| 5 | 5 | 0.00353895156596 | −0.02436464043876 | 0.000000000000000 | 0.000000000000000 |
| 6 | 0 | −0.01499101017996 | 0 | 0.021480381809402 | 0 |
| 6 | 1 | 0.00183803644113 | −0.00116294838910 | 0.000000000000000 | 0.000000000000000 |
| 6 | 2 | 0.02197171243552 | −0.00529808274697 | −0.014894515446723 | 0.000000000000000 |
| 6 | 3 | −0.00577570989586 | 0.00446161579351 | 0.000000000000000 | 0.000000000000000 |
| 6 | 4 | −0.01904232642471 | 0.01127642233858 | −0.003093652246108 | 0.000000000000000 |
| 6 | 5 | 0.00690519883075 | −0.00499919673362 | 0.000000000000000 | 0.000000000000000 |
| 6 | 6 | 0.01326276839505 | −0.02937007076311 | −0.051021169584544 | 0.000000000000000 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hu, W.; Fu, T.; Liu, C. Revisiting the Numerical Evaluation and Visualization of the Gravity Fields of Asteroid 4769 Castalia Using Polyhedron and Harmonic Expansions Models. Appl. Sci. 2024, 14, 4058. https://doi.org/10.3390/app14104058
Hu W, Fu T, Liu C. Revisiting the Numerical Evaluation and Visualization of the Gravity Fields of Asteroid 4769 Castalia Using Polyhedron and Harmonic Expansions Models. Applied Sciences. 2024; 14(10):4058. https://doi.org/10.3390/app14104058
Chicago/Turabian StyleHu, Weiduo, Tao Fu, and Chang Liu. 2024. "Revisiting the Numerical Evaluation and Visualization of the Gravity Fields of Asteroid 4769 Castalia Using Polyhedron and Harmonic Expansions Models" Applied Sciences 14, no. 10: 4058. https://doi.org/10.3390/app14104058
APA StyleHu, W., Fu, T., & Liu, C. (2024). Revisiting the Numerical Evaluation and Visualization of the Gravity Fields of Asteroid 4769 Castalia Using Polyhedron and Harmonic Expansions Models. Applied Sciences, 14(10), 4058. https://doi.org/10.3390/app14104058







