Bioelectrical Impedance Vector Analysis (BIVA) and Somatotype in Female Rugby Players
Abstract
Featured Application
Abstract
1. Introduction
2. Materials and Methods
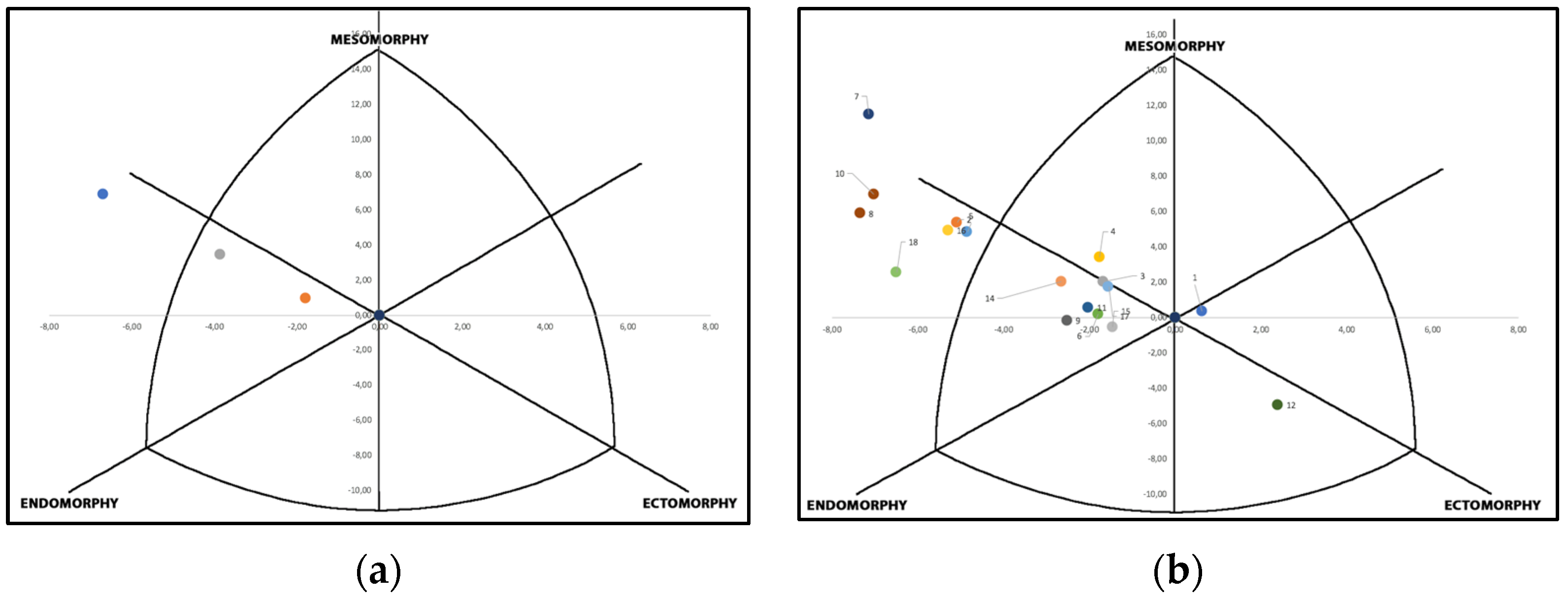
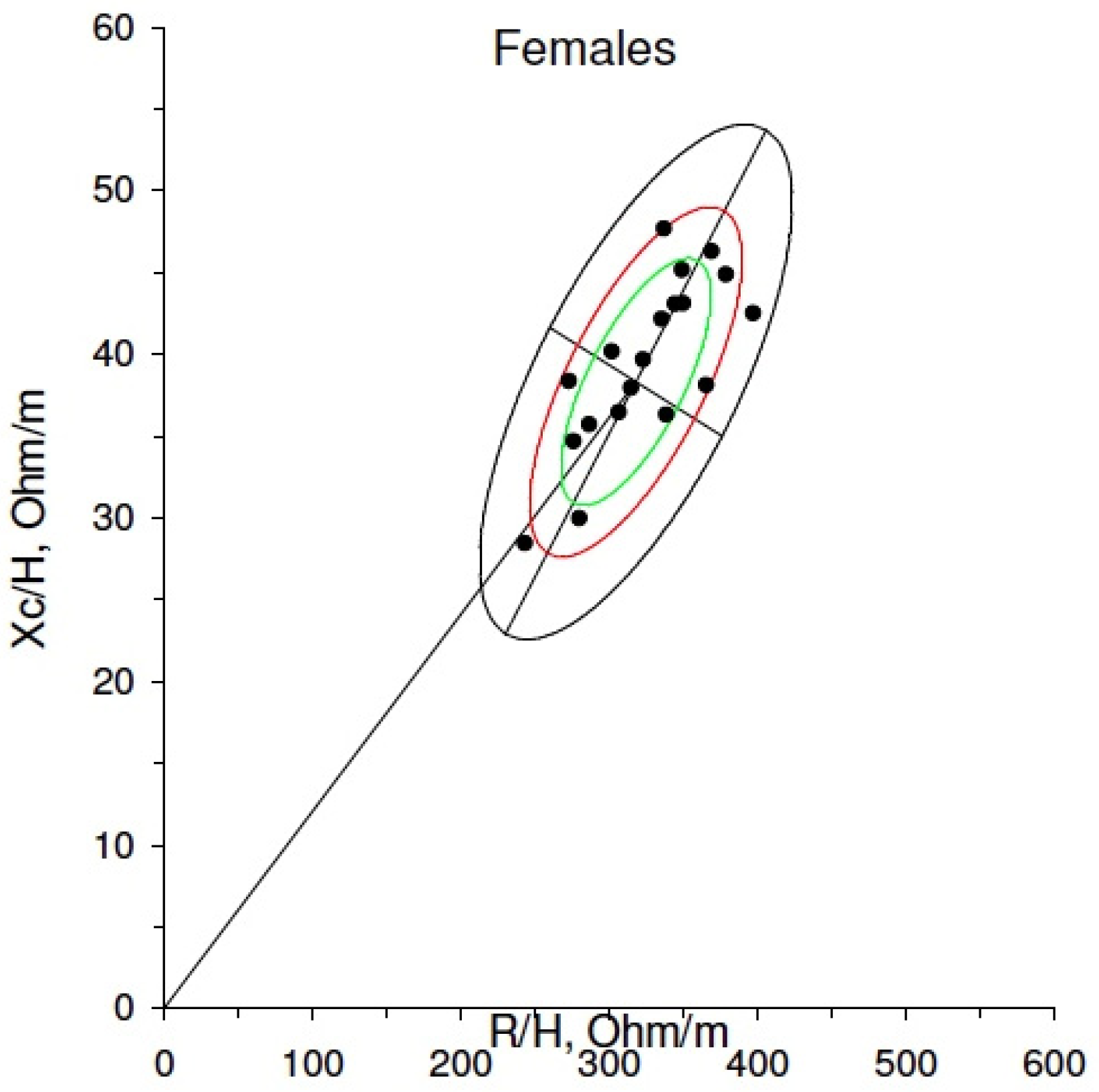
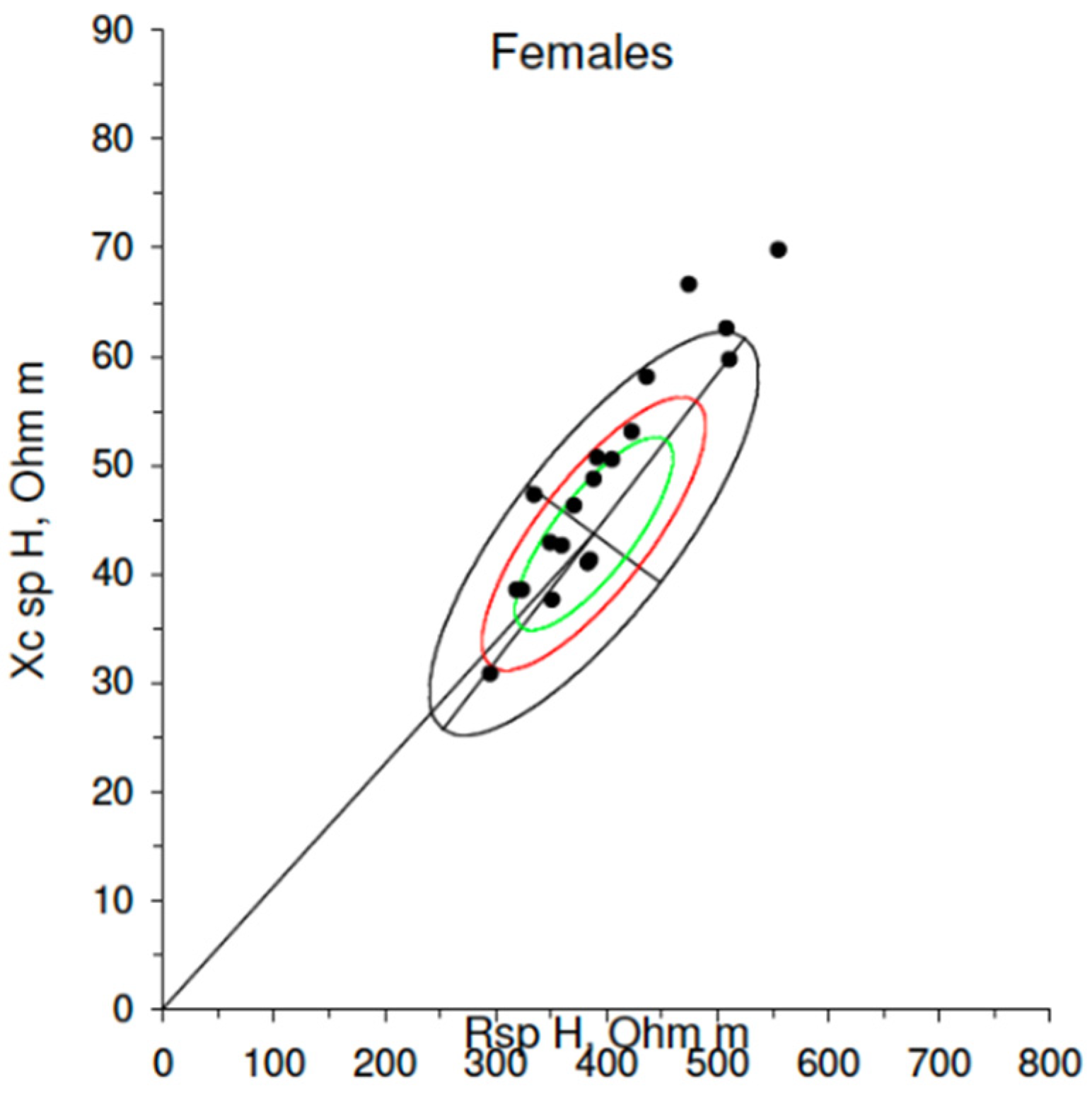
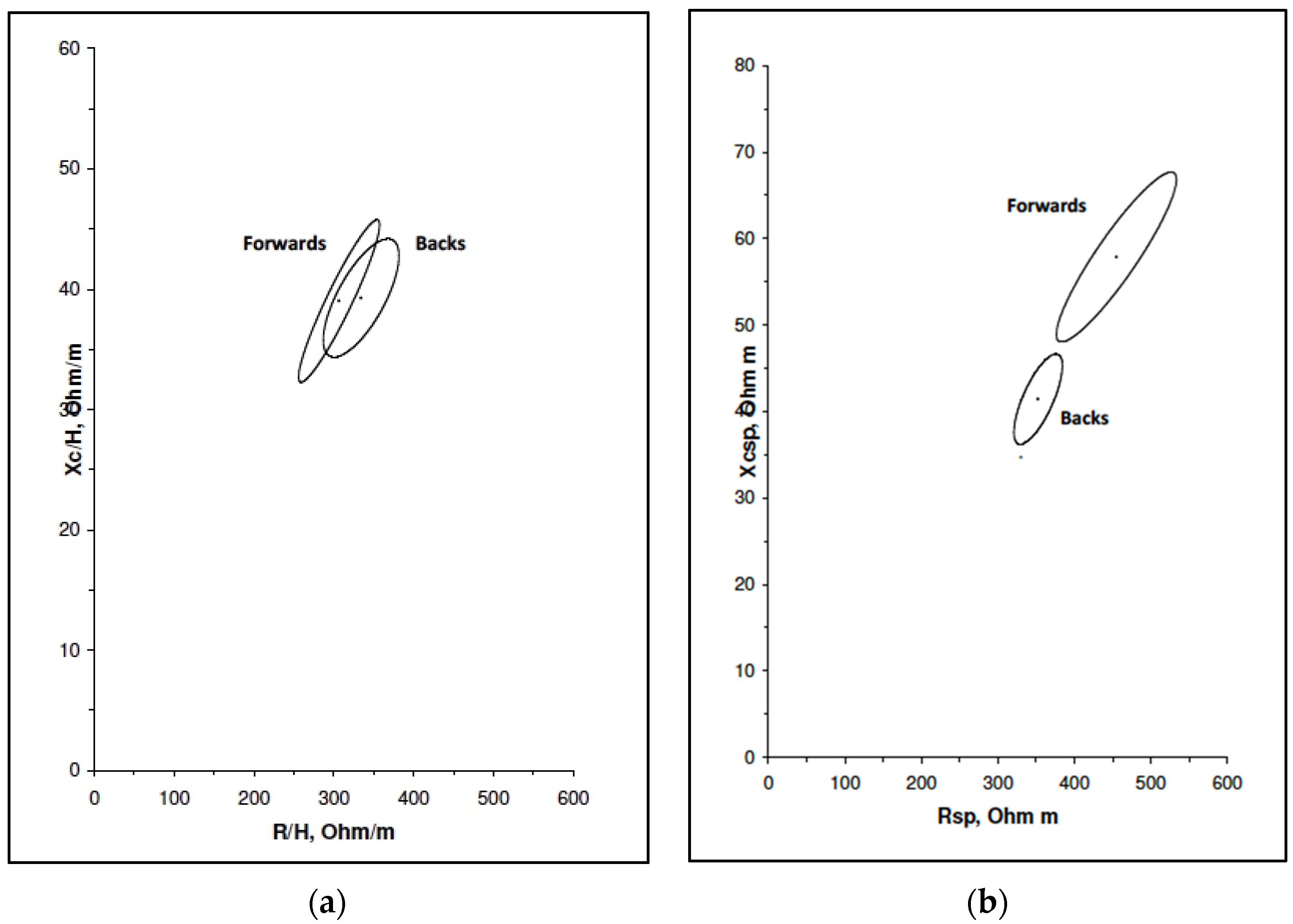
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Brazier, J.; Antrobus, M.; Stebbings, G.K.; Day, S.H.; Callus, P.; Erskine, R.M.; Mark, A. Anthropometric and physiological characteristics of elite male rugby athletes. J. Strength Cond. Res. 2020, 34, 1790–1801. [Google Scholar] [CrossRef] [PubMed]
- Duthie, G.; Pyne, D.; Hooper, S. Applied Physiology and Game Analysis of Rugby Union. Sports Med. 2003, 33, 973–991. [Google Scholar] [CrossRef] [PubMed]
- Quarrie, K.L.; Wilson, B.D. Force production in the rugby union scrum. J. Sports Sci. 2000, 18, 237–246. [Google Scholar] [CrossRef] [PubMed]
- Berral-Aguilar, A.J.; Schröder-Vilar, S.; Rojano-Ortega, D.; la Rosa, F.J.B.-D. Body Composition, Somatotype and Raw Bioelectrical Impedance Parameters of Adolescent Elite Tennis Players: Age and Sex Differences. Int. J. Environ. Res. Public Health 2022, 19, 17045. [Google Scholar] [CrossRef]
- Campa, F.; Gobbo, L.A.; Stagi, S.; Cyrino, L.T.; Toselli, S.; Marini, E.; Coratella, G. Bioelectrical impedance analysis versus reference methods in the assessment of body composition in athletes. Eur. J. Appl. Physiol. 2022, 122, 561–589. [Google Scholar] [CrossRef]
- Wang, Z.M.; Pierson, R.N.; Heymsfield, S.B. The five-level model: A new approach to organizing body-composition research. Am. J. Clin. Nutr. 1992, 56, 19–28. [Google Scholar] [CrossRef]
- Müller, M.J.; Braun, W.; Pourhassan, M.; Geisler, C.; Bosy-Westphal, A. Application of standards and models in body composition analysis. Proc. Nutr. Soc. 2016, 75, 181–187. [Google Scholar] [CrossRef][Green Version]
- Heymsfield, S.B.; Ebbeling, C.B.; Zheng, J.; Pietrobelli, A.; Strauss, B.J.; Silva, A.M.; Ludwig, D.S. Multi-component molecular-level body composition reference methods: Evolving concepts and future directions. Obes. Rev. 2015, 16, 282–294. [Google Scholar] [CrossRef][Green Version]
- Alvero Cruz, J.R.; Cabañas Armesilla, M.D.; Herrero de Lucas, A.; Martínez Riaza, L.; Moreno Pascual, C.; Porta Manzañido, J.; Sillero Quintana, M.; Sirvent Belando, J.E. Protocolo de valoración de la composición corporal para el reconocimiento médico-deportivo. Documento de consenso del Grupo Español de Cineantropometría de la Federación Española de Medicina del De-porte. Arch. Med. Deporte 2009, 26, 166–179. [Google Scholar]
- Ackland, T.; Lohman, T.G.; Sundgot-Borgen, J.; Maughan, R.J.; Meyer, N.L.; Stewart, A.; Müller, W. Current Status of Body Composition Assessment in Sport: Review and Position Statement on Behalf of the Ad Hoc Research Working Group on Body Composition Health and Performance, under the Auspices of the I.O.C. Medical Commission. Sports Med. 2012, 42, 227–249. [Google Scholar] [CrossRef]
- Campa, F.; Matias, C.N.; Moro, T.; Cerullo, G.; Casolo, A.; Teixeira, F.J.; Paoli, A. Methods over Materials: The Need for Sport-Specific Equations to Accurately Predict Fat Mass Using Bioimpedance Analysis or Anthropometry. Nutrients 2023, 15, 278. [Google Scholar] [CrossRef] [PubMed]
- Carter, J.E.L.; Heath, B. Somatotyping Development and Applications; Cambridge University Press: Cambridge, UK; New York, NY, USA, 1990. [Google Scholar]
- Kyle, U.G.; Bosaeus, I.; De Lorenzo, A.D.; Deurenberg, P.; Elia, M.; Gómez, J.M.; Heitmann, B.L.; Kent-Smith, L.; Melchior, J.C.; Pirlich, M.; et al. Bioelectrical impedance analysis, part I: Review of principles and methods. Clin. Nutr. 2004, 23, 1226–1243. [Google Scholar] [CrossRef] [PubMed]
- Lukaski, H.C. Evolution of bioimpedance: A circuitous journey from estimation of physiological function to assessment of body composition and a return to clinical research. Eur. J. Clin. Nutr. 2013, 67 (Suppl. S1), S2–S9. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Kyle, U.G.; Bosaeus, I.; De Lorenzo, A.D.; Deurenberg, P.; Elia, M.; Gómez, J.M.; Heitmann, B.L.; Kent-Smith, L.; Melchior, J.-C.; Pirlich, M.; et al. Bioelectrical impedance analysis—Part II: Utilization in clinical practice. Clin. Nutr. 2004, 23, 1430–1453. [Google Scholar] [CrossRef] [PubMed]
- Norman, K.; Smoliner, C.; Valentini, L.; Lochs, K.; Pirlich, M. Is bioelectrical vector analysis of value in the elderly with malnutrition and impaired functionality? Nutrition 2007, 23, 564–569. [Google Scholar] [CrossRef] [PubMed]
- Piccoli, A.; Rossi, B.; Pillon, L.; Bucciante, G. A new method for monitoring body fluid variation by bioimpedance analysis: The RXc graph. Kidney Int. 1994, 46, 534–539. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Norman, K.; Stobäus, N.; Pirlich, M.; Bosy-Westphal, A. Bioelectrical phase angle and impedance vector analysis—Clinical relevance and applicability of impedance parameters. Clin. Nutr. 2012, 31, 854–861. [Google Scholar] [CrossRef]
- Piccoli, A.; Nescolarde, L.D.; Rosell, J. Análisis convencional y vectorial de impedancia en la práctica clínica. Nefrologia 2002, 23, 228–236. [Google Scholar]
- Buffa, R.; Saragat, B.; Cabras, S.; Rinaldi, A.C.; Marini, E. Accuracy of specific BIVA for the assessment of body composition in the United States population. PLoS ONE 2013, 8, e58533. [Google Scholar] [CrossRef]
- Mereu, E.; Buffa, R.; Lussu, P.; Marini, E. Phase angle, vector length, and body composition. Am. J. Clin. Nutr. 2016, 104, 845–847. [Google Scholar] [CrossRef][Green Version]
- Marini, E.; Sergi, G.; Succa, V.; Saragat, B.; Sarti, S.; Coin, A.; Manzato, E.; Buffa, R. Efficacy of specific bioelectrical impedance vector analysis (BIVA) for assessing body composition in the elderly. J. Nutr. Health Aging 2013, 17, 515–521. [Google Scholar] [CrossRef] [PubMed]
- Castizo-Olier, J.; Irurtia, A.; Jemni, M.; Carrasco-Marginet, M.; Fernández-García, R.; Rodríguez, F.A. Bioelectrical impedance vector analysis (BIVA) in sport and exercise: Systematic review and future perspectives. PLoS ONE 2018, 13, e0197957. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Campa, F.; Toselli, S.; Mazzilli, M.; Gobbo, L.A.; Coratella, G. Assessment of Body Composition in Athletes: A Narrative Review of Available Methods with Special Reference to Quantitative and Qualitative Bioimpedance Analysis. Nutrients 2021, 13, 1620. [Google Scholar] [CrossRef]
- Di Vincenzo, O.; Marra, M.; Scalfo, L. Bioelectrical impedance phase angle in sport: A systematic review. J. Intern. Soc. Sports Nutr. 2019, 16, 49. [Google Scholar] [CrossRef][Green Version]
- Campa, F.; Silva, A.M.; Talluri, J.; Matias, C.N.; Badicu, G.; Toselli, S. Somatotype and Bioimpedance Vector Analysis: A New Target Zone for Male Athletes. Sustainability 2020, 12, 4365. [Google Scholar] [CrossRef]
- Stewart, A.; Marfell-Jones, M.; Olds, T.; Riidder, H. International Standards for Anthropometric Assessment; ISAK: Lower Hutt, New Zealand, 2011. [Google Scholar]
- WHO. Clasificación Internacional Para Adultos de Bajo Peso, Sobrepeso y Obesidad en Relación al IMC: Criterios de la OMS. 2006. Available online: https://www.who.int/es/news-room/fact-sheets/detail/obesity-and-overweight (accessed on 1 March 2023).
- Carter, J.E.L. (Ed.) Body composition of Montreal Olympic athletes. In Physical Structure of the Olympic Athletes. Part I: The Montreal Olympic Games Anthropological Project; Karger: Basel, Switzerland, 1982; pp. 107–116. [Google Scholar]
- Rocha, M.L. Peso ósseo do brasileiro de ambos os sexos de 17 a 25 anos. Aeq. Anat. Antrop. 1975, 1, 445. [Google Scholar]
- Würch, A. La femme et le sport. Médecine Sport. Fr. 1974, 4, 441–445. [Google Scholar]
- Lee, R.C.; Wang, Z.; Heo, M.; Ross, R.; Janssen, I.; Heymsfield, S.B. Total-body skeletal muscle mass: Development and cross-validation of anthropometric prediction models. Am. J. Clin. Nutr. 2000, 72, 796–803. [Google Scholar] [CrossRef][Green Version]
- Canda, A.S. Variables Antropométricas de la Población Deportista Española; Consejo Superior de Deportes de España: Madrid, Spain, 2012. [Google Scholar]
- Lukaski, H.C. Assessment of body composition using tetrapolar impedance analysis. In New Techniques in Nutritional Research; Whitehead, R.G., Prentice, A., Eds.; Academic Press: San Diego, CA, USA, 1991; pp. 303–315. [Google Scholar]
- Matias, C.N.; Campa, F.; Santos, D.A.; Lukaski, H.; Sardinha, L.B.; Silva, A.M. Fat-free Mass Bioelectrical Impedance Analysis Predictive Equation for Athletes using a 4-Compartment Model. Int. J. Sports Med. 2021, 42, 27–32. [Google Scholar] [CrossRef] [PubMed]
- Janssen, I.; Heymsfield, S.B.; Baumgartner, R.N.; Ross, R. Estimation of skeletal muscle mass by bioelectrical impedance analysis. J. Appl. Physiol. 2000, 89, 465–471. [Google Scholar] [CrossRef][Green Version]
- Campa, F.; Matias, C.; Gatterer, H.; Toselli, S.; Koury, J.C.; Andreoli, A.; Melchiorri, G.; Sardinha, L.B.; Silva, A.M. Classic Bioelectrical Impedance Vector Reference Values for Assessing Body Composition in Male and Female Athletes. Int. J. Environ. Res. Public Health 2019, 16, 5066. [Google Scholar] [CrossRef][Green Version]
- Ibáñez, M.E.; Mereu, E.; Buffa, R.; Gualdi-Russo, E.; Zaccagni, L.; Cossu, S.; Rebato, E.; Marini, E. New specific bioelectrical impedance vector reference values for assessing body composition in the Italian-Spanish young adult population. Am. J. Hum. Biol. 2015, 27, 871–876. [Google Scholar] [CrossRef] [PubMed]
- Piccoli, A.; Pastori, G. BIVA Software; Department of Medical and Surgical Sciences, University of Padova: Padova, Italy, 2002. [Google Scholar]
- Dubois, R.; Paillard, T.; Lyons, M.; McGrath, D.; Maurelli, O.; Prioux, J. Running and Metabolic Demands of Elite Rugby Union Assessed Using Traditional, Metabolic Power, and Heart Rate Monitoring Methods. J. Sports Sci. Med. 2017, 16, 84–92. [Google Scholar]
- Rodríguez Rodríguez, F.J.; Berral de la Rosa, F.J.; Almagià Flores, A.A.; Iturriaga Zuleta, M.F.; Rodríguez Briceño, F. Comparación de la composición corporal y de la masa muscular por segmentos corporales, en estudiantes de educación física y deportistas de distintas disciplinas. Int. J. Morphol. 2012, 30, 7–14. [Google Scholar] [CrossRef][Green Version]
- Harty, P.S.; Zabriskie, H.A.; Stecker, R.A.; Currier, B.S.; Moon, J.M.; Richmond, S.R.; Jagim, A.R.; Kerksick, C.M. Position-Specific Body Composition Values in Female Collegiate Rugby Union Athletes. J. Strength Cond. Res. 2021, 35, 3158–3163. [Google Scholar] [CrossRef] [PubMed]
- Suárez-Moreno Arrones, L.J.; Núñez, F.J. Physicological and antropometric characteristics of elite rugby players in Spain and relative power out as predictor of performance in sprint and RSA. J. Sport Health Res. 2011, 3, 191–202. [Google Scholar]
- Gabbett, T.J. Physiological and anthropometric characteristics of elite women rugby league players. J. Strength Cond. Res. 2007, 21, 875–881. [Google Scholar] [CrossRef] [PubMed]
- Ramos-Álvarez, J.J.; Montoya, J.J.; Solís-Mencia, C.; Miguel-Tobal, F.; López-Tapia, P.; Sánchez-Oliver, A.J.; Domínguez, R.; Martínez-Sanz, J.M. Anthropometric Profile Assessed by Bioimpedance and Anthropometry Measures of Male and Female Rugby Players Competing in the Spanish National League. Appl. Sci. 2021, 11, 11759. [Google Scholar] [CrossRef]
- Jones, B.; Emmonds, S.; Hind, K.; Nicholson, G.; Rutherford, Z.; Till, K. Physical Qualities of International Female Rugby League Players by Playing Position. J. Strength Cond. Res. 2016, 30, 1333–1340. [Google Scholar] [CrossRef]
- Hene, N.M.; Bassett, S.H.; Andrews, B.S. Physical fitness profiles of elite women’s rugby union players. Afr. J. Phys. Health Educ. Recreat. Dance 2011, 17 (Suppl. S1), 1–8. [Google Scholar]
- Scantlebury, S.; McCormack, S.; Sawczuk, T.; Emmonds, S.; Collins, N.; Beech, J.; Ramirez, C.; Owen, C.; Jones, B. The anthropometric and physical qualities of women’s rugby league Super League and international players; identifying differences in playing position and level. PLoS ONE 2022, 17, e0249803. [Google Scholar] [CrossRef] [PubMed]
- Escrivá, D.; Caplliure-Llopis, J.; Benet, I.; Mariscal, G.; Mampel, J.V.; Barrios, C. Differences in Adiposity Profile and Body Fat Distribution between Forwards and Backs in Sub-Elite Spanish Female Rugby Union Players. J. Clin. Med. 2021, 10, 5713. [Google Scholar] [CrossRef] [PubMed]
- Lukaski, H.C.; Raymond-Pope, J.C. New frontiers of body composition in sport. Int. J. Sports Med. 2021, 42, 588–601. [Google Scholar] [CrossRef]
- Coratella, G.; Campa, F.; Matias, C.N.; Toselli, S.; Koury, J.C.; Andreoli, A.; Sardinha, L.B.; Silva, A.M. Generalized bioelectric impedance-based equations underestimate body fluids in athletes. Scand. J. Med. Sci. Sports 2021, 31, 2123–2132. [Google Scholar] [CrossRef]
- Campa, F.; Thomas, D.M.; Watts, K.; Clark, N.; Baller, D.; Morin, T.; Toselli, S.; Koury, J.C.; Melchiorri, G.; Andreoli, A.; et al. Reference Percentiles for Bioelectrical Phase Angle in Athletes. Biology 2022, 11, 264. [Google Scholar] [CrossRef]
- Campa, F.; Silva, A.M.; Matias, C.N.; Monteiro, C.P.; Paoli, A.; Nunes, J.P.; Talluri, J.; Lukaski, H.; Toselli, S. Body Water Content and Morphological Characteristics Modify Bioimpedance Vector Patterns in Volleyball, Soccer, and Rugby Players. Int. J. Environ. Res. Public Health 2020, 17, 6604. [Google Scholar] [CrossRef] [PubMed]
- Marini, E.; Campa, F.; Buffa, R.; Stagi, S.; Matias, C.N.; Toselli, S.; Sardinha, L.B.; Silva, A.M. Phase angle and bioelectrical impedance vector analysis in the evaluation of body composition in athletes. Clin. Nutr. 2020, 39, 447–454. [Google Scholar] [CrossRef] [PubMed]
- Reis, J.F.; Matias, C.N.; Campa, F.; Morgado, J.P.; Franco, P.; Quaresma, P.; Almeida, N.; Curto, D.; Toselli, S.; Monteiro, C.P. Bioimpedance Vector Patterns Changes in Response to Swimming Training: An Ecological Approach. Int. J. Environ. Res. Public Health 2020, 17, 4851. [Google Scholar] [CrossRef]
- Campa, F.; Matias, C.N.; Nunes, C.L.; Monteiro, C.P.; Francisco, R.; Jesus, F.; Marini, E.; Sardinha, L.B.; Martins, P.; Minderico, C.; et al. Specific Bioelectrical Impedance Vector Analysis Identifies Body Fat Reduction after a Lifestyle Intervention in Former Elite Athletes. Biology 2021, 10, 524. [Google Scholar] [CrossRef]
- Buffa, R.; Mereu, E.; Succa, V.; Latini, V.; Marini, E. Specific BIVA recognizes variation of body mass and body composition: Two related but different facets of nutritional status. Nutrition 2017, 35, 1–5. [Google Scholar] [CrossRef]
- Kim, C.H.; Park, J.H.; Kim, H.; Chung, S.; Park, S.H. Modeling the human body shape in bioimpedance vector measurements. Annu. Int. Conf. IEEE Eng. Med. Biol. Soc. 2010, 2010, 3872–3874. [Google Scholar] [PubMed]
- Campa, F.; Bongiovanni, T.; Matias, C.N.; Genovesi, F.; Trecroci, A.; Rossi, A.; Iaia, F.M.; Alberti, G.; Pasta, G.; Toselli, S. A New Strategy to Integrate Heath–Carter Somatotype Assessment with Bioelectrical Impedance Analysis in Elite Soccer Players. Sports 2020, 8, 142. [Google Scholar] [CrossRef] [PubMed]
- de la Cruz Marcos, S.; Redondo del Río, M.P.; de Mateo Silleras, B. Applications of bioelectrical impedance vector analysis (BIVA) in the study of body composition in athletes. Appl. Sci. 2021, 11, 9781. [Google Scholar] [CrossRef]
| Variables | Total Sample (n = 19) | Player’s Position | |
|---|---|---|---|
| Forwards (n = 8) | Backs (n = 11) | ||
| Height (cm) | 165.5 (7.8) | 161.9 (9.1) | 168.2 (5.7) |
| Body mass (kg) | 71.1 (17.0) | 82.2 (20.6) | 63.0 (7.3) * |
| FM% | 24.2 (8.3) | 30.7 (7.5) | 19.4 (5.1) * |
| FM (kg) | 18.2 (10.3) | 26.1 (11.0) | 12.5 (4.7) * |
| BM (kg) | 9.5 (1.4) | 9.9 (1.8) | 9.2 (0.9) |
| RM (kg) | 14.9 (3.6) | 17.2 (4.3) | 13.2 (1.5) * |
| SMM Lee (kg) | 25.2 (4.0) | 27.3 (5.1) | 23.7 (2.1) |
| SMM% Lee | 36.0 (3.5) | 33.8 (3.6) | 37.7 (2.3) * |
| Somatotype Components | Total Sample (n = 19) | Player’s Position | |
|---|---|---|---|
| Forwards (n = 8) | Backs (n = 11) | ||
| Endomorphy | 5.48 (1.89) | 7.10 (1.35) | 4.30 (1.24) * |
| Mesomorphy | 5.29 (2.27) | 7.21 (1.87) | 3.89 (1.30) * |
| Ectomorphy | 1.62 (1.39) | 0.41 (0.36) | 2.50 (1.17) * |
| Electrical Values | Total Sample (n = 19) | Players’ Position | |
|---|---|---|---|
| Forwards (n = 8) | Backs (n = 11) | ||
| R (Ohm) | 536.7 (64.5) | 498.4 (53.4) | 564.6 (58.7) * |
| Xc (Ohm) | 65.2 (7.7) | 63.5 (7.4) | 66.4 (8.1) |
| Z (Ohm) | 540.7 (64.7) | 502.4 (53.8) | 568.5 (59.0) * |
| PA (°) | 6.95 (0.59) | 7.26 (0.40) | 6.73 (0.61) * |
| R/H (Ohm/m) | 324.9 (40.8) | 309.2 (41.4) | 336.2 (38.2) |
| Xc/H (Ohm/m) | 39.5 (5.2) | 39.4 (5.5) | 39.6 (5.3) |
| Z/H (Ohm/m) | 327.3 (41.0) | 311.7 (41.7) | 338.6 (38.4) |
| Rsp (Ohm m) | 398.2 (71.2) | 458.9 (64.1) | 354.2 (34.1) * |
| Xcsp (Ohm m) | 48.8 (10.6) | 58.4 (8.0) | 41.8 (5.6) * |
| Zsp (Ohm m) | 401.2 (71.9) | 462.6 (64.5) | 356.6 (34.4) * |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Silleras, B.d.M.; Ares, G.C.; Marcos, S.d.l.C.; Enciso, L.C.; Fernández, E.Q.; Río, P.R.d. Bioelectrical Impedance Vector Analysis (BIVA) and Somatotype in Female Rugby Players. Appl. Sci. 2023, 13, 5242. https://doi.org/10.3390/app13095242
Silleras BdM, Ares GC, Marcos SdlC, Enciso LC, Fernández EQ, Río PRd. Bioelectrical Impedance Vector Analysis (BIVA) and Somatotype in Female Rugby Players. Applied Sciences. 2023; 13(9):5242. https://doi.org/10.3390/app13095242
Chicago/Turabian StyleSilleras, Beatriz de Mateo, Guillermo Casas Ares, Sandra de la Cruz Marcos, Laura Carreño Enciso, Emiliano Quinto Fernández, and Paz Redondo del Río. 2023. "Bioelectrical Impedance Vector Analysis (BIVA) and Somatotype in Female Rugby Players" Applied Sciences 13, no. 9: 5242. https://doi.org/10.3390/app13095242
APA StyleSilleras, B. d. M., Ares, G. C., Marcos, S. d. l. C., Enciso, L. C., Fernández, E. Q., & Río, P. R. d. (2023). Bioelectrical Impedance Vector Analysis (BIVA) and Somatotype in Female Rugby Players. Applied Sciences, 13(9), 5242. https://doi.org/10.3390/app13095242









