Truss-like Discrete Element Method Applied to Damage Process Simulation in Quasi-Brittle Materials
Abstract
1. Introduction
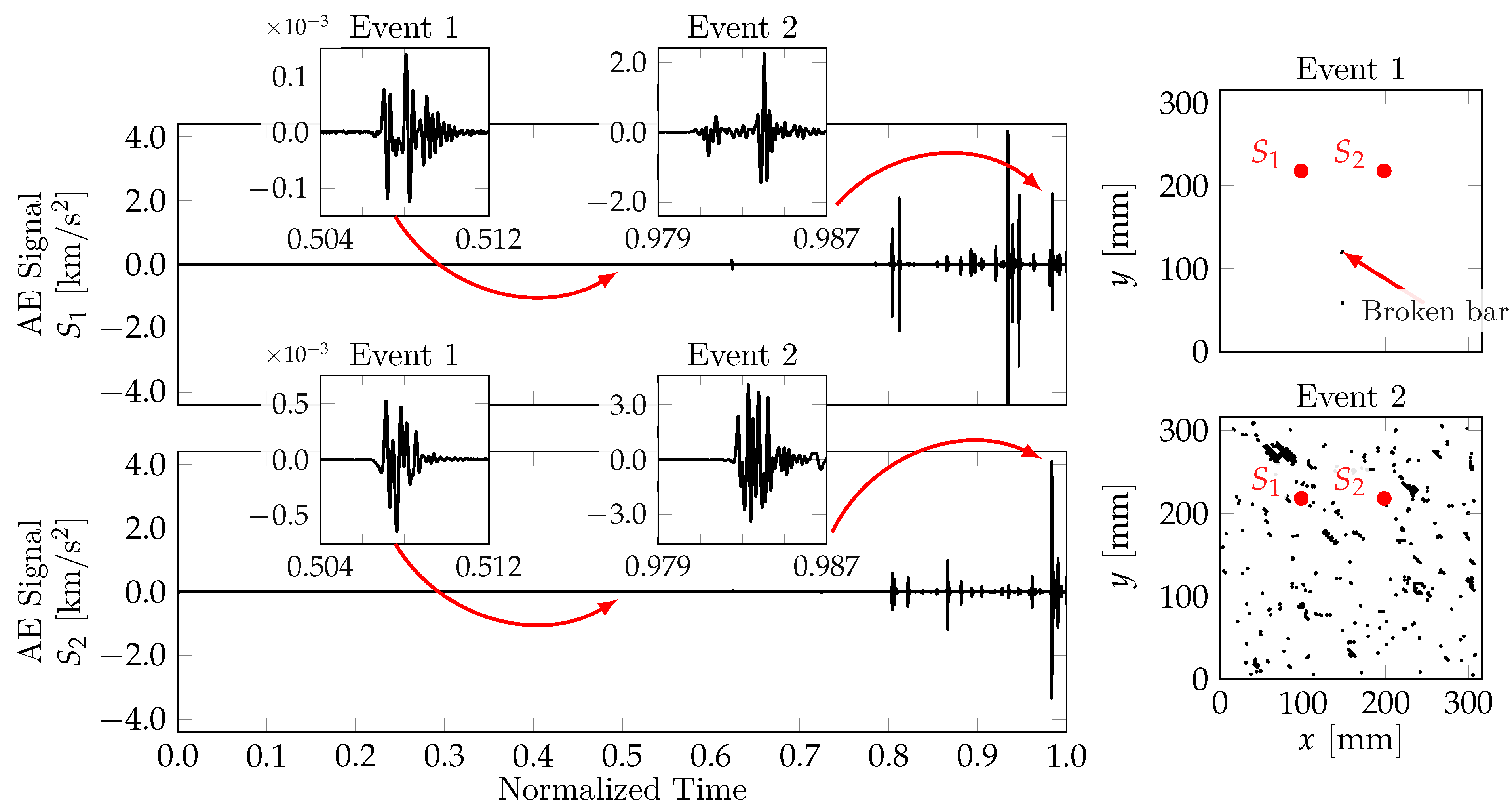
- Simulating a concrete slab under pure-shear stress and observing how the internal damage process translated into the signals recorded by an AE sensor.
- Subjecting a pre-fissured sandstone beam to a three-point-bending test, carried out experimentally by [41], as well as through simulations performed in this study. The simulated dataset was then used to deepen the understanding of the damage process through comparisons with the corresponding experimental data.
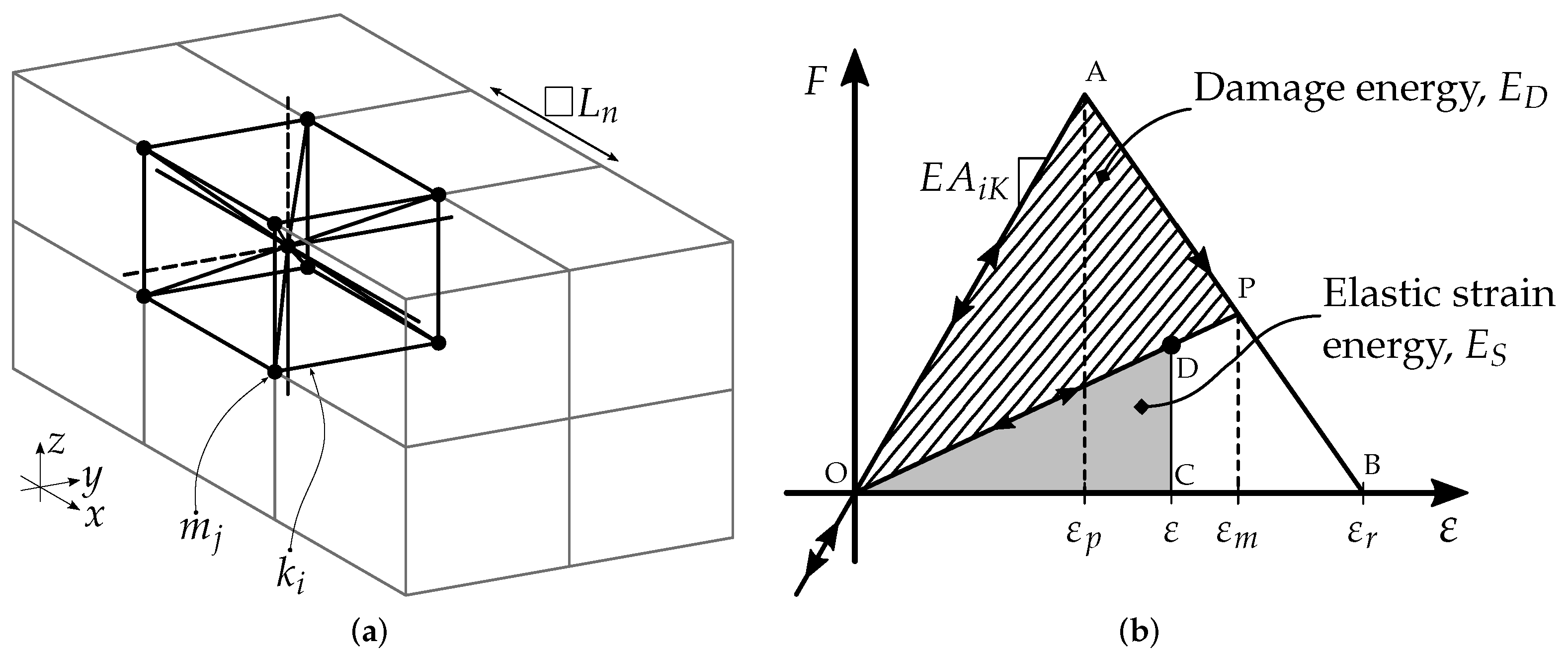
2. Overview of the LDEM Approach
- Introducing small disturbances throughout the mesh with the node coordinates () defined by:where , , and are the node coordinates for a perfect cubic array, whereas , , and are normally distributed random numbers with zero mean and variation coefficient .
- Defining the material’s specific fracture energy as a random 3D-field, according to a Type-III (Weibull) distribution, where the mean and the variation coefficient would appear as input parameters. This option also considered a spatial correlation () for when .
3. First Application: AE Events in a Simulated Fracture Process
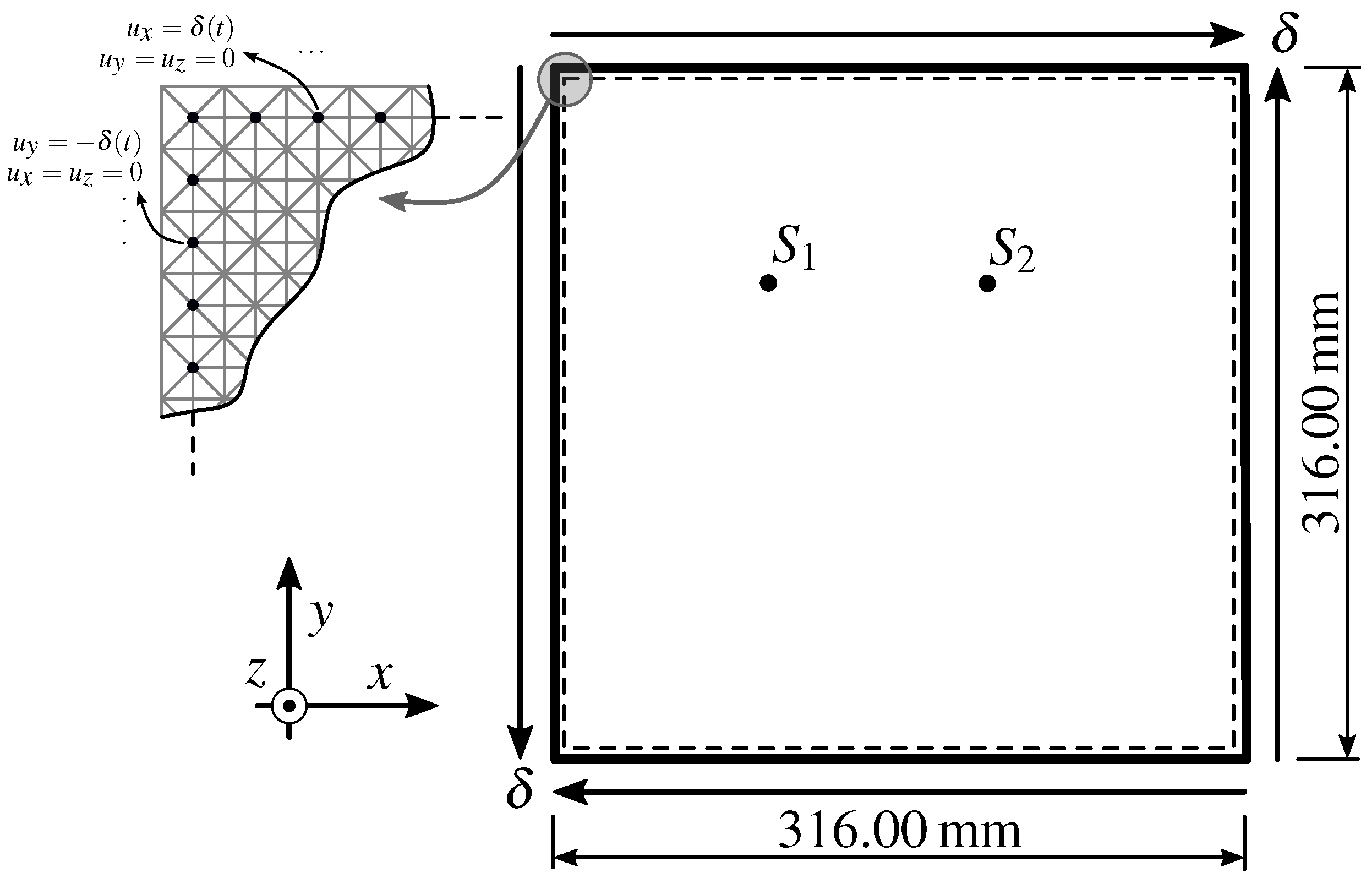
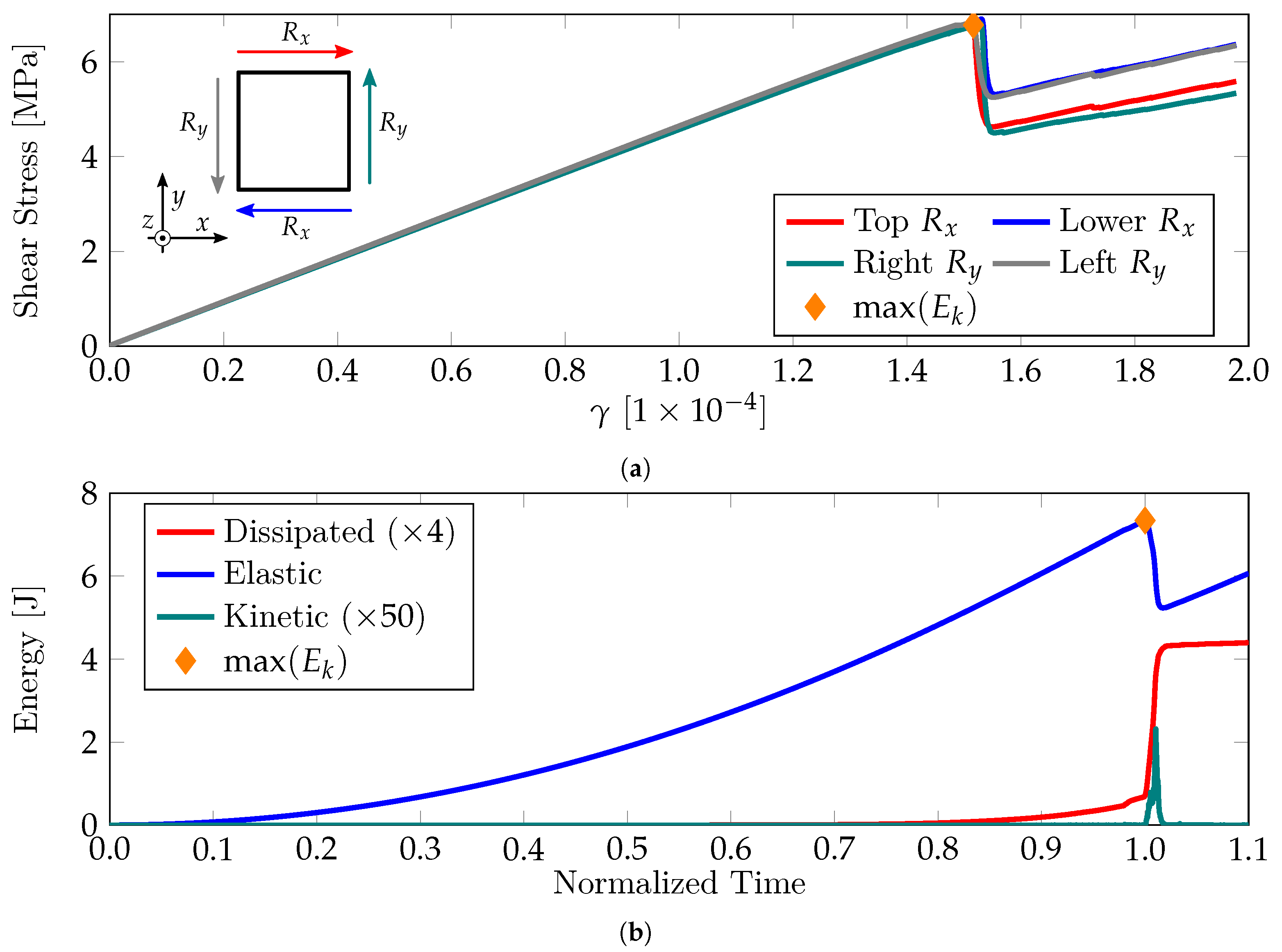
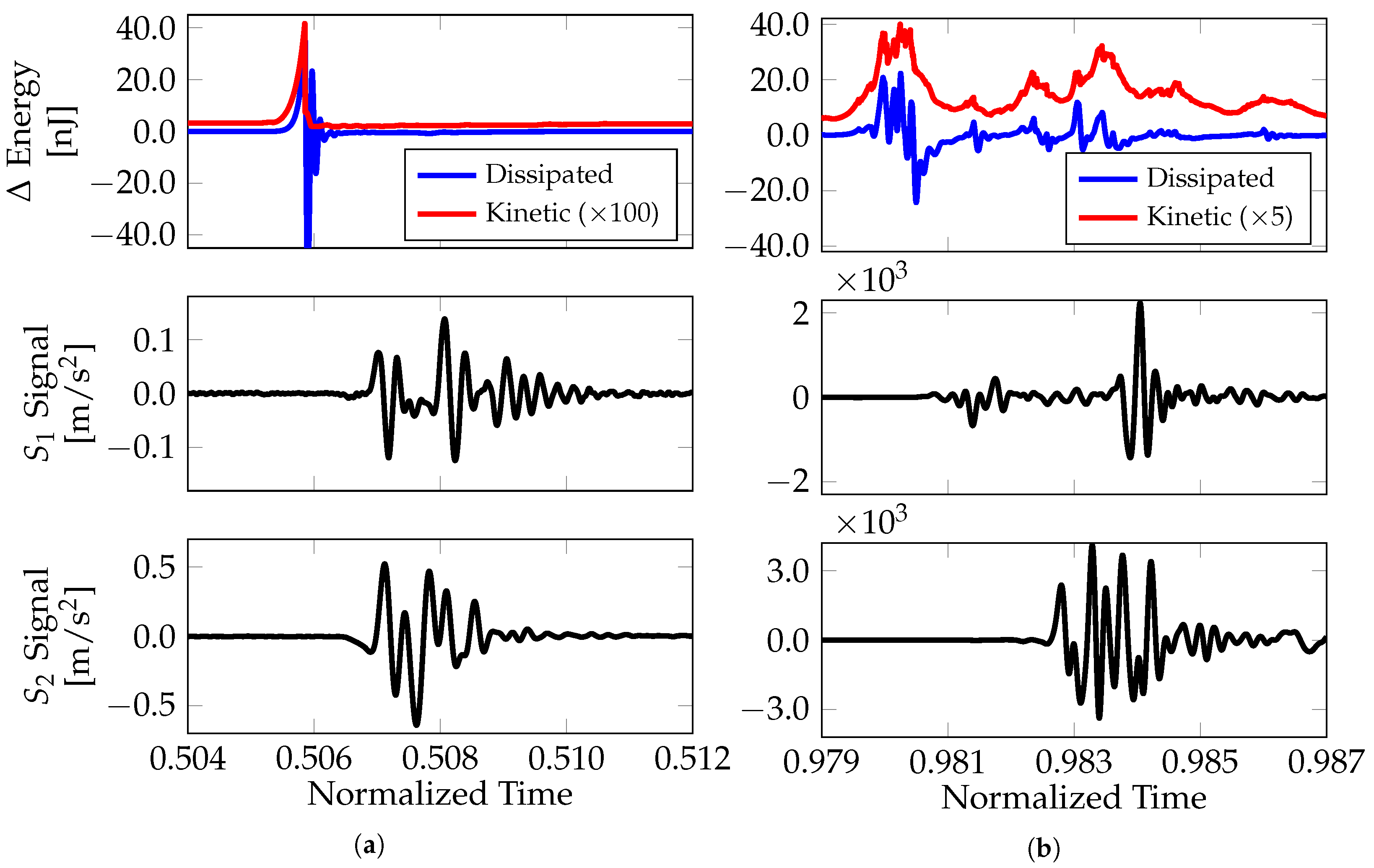
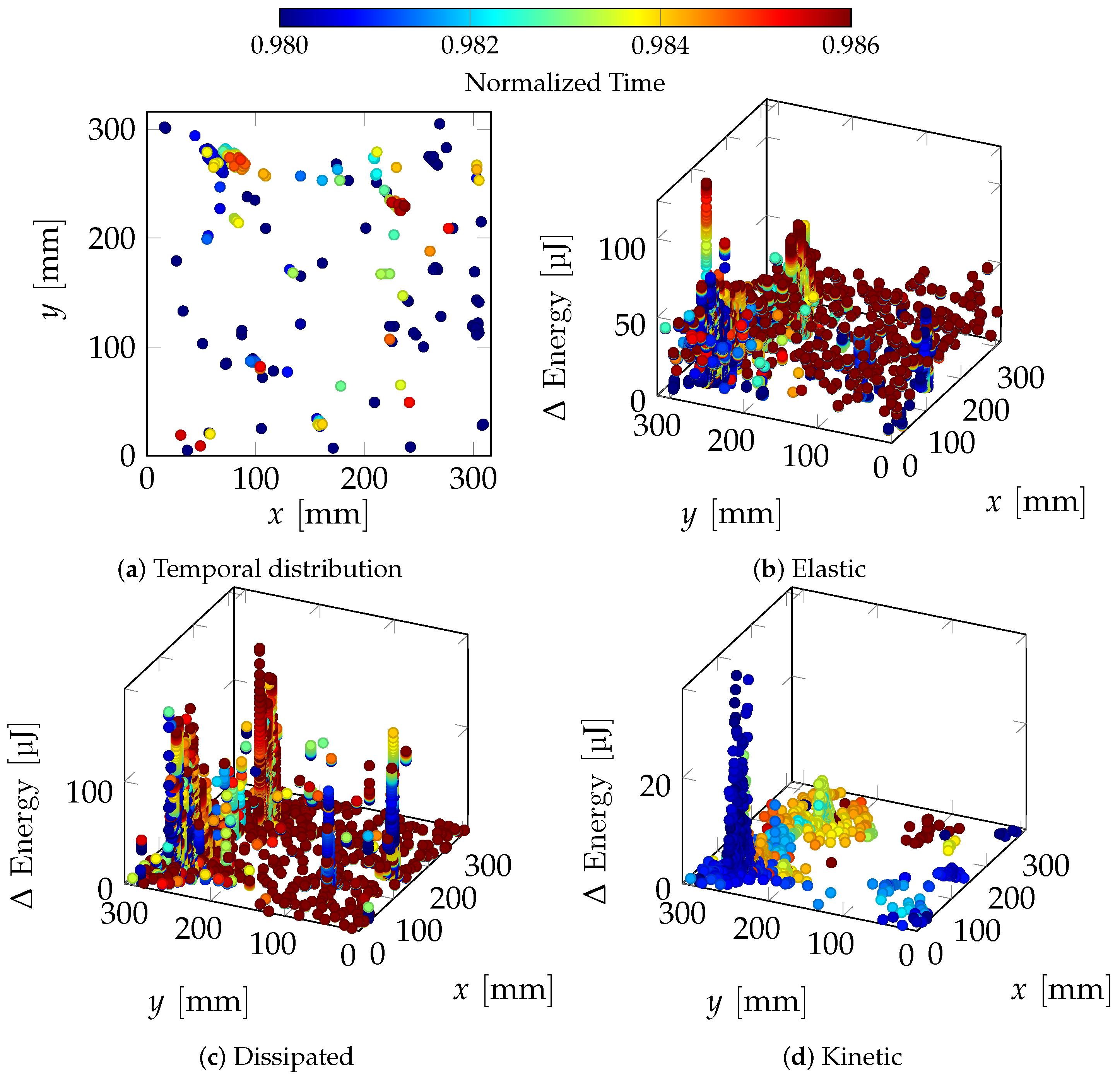
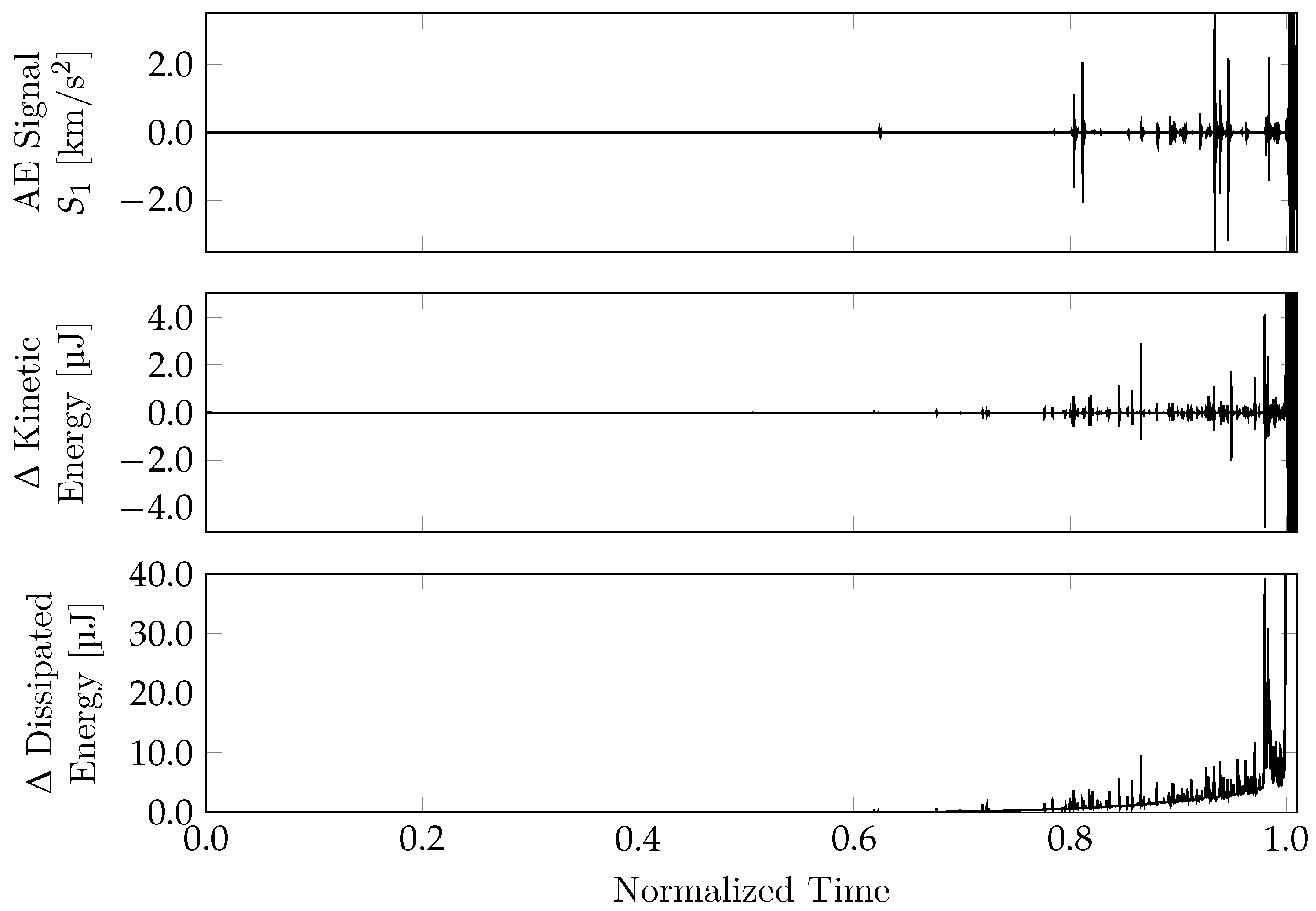
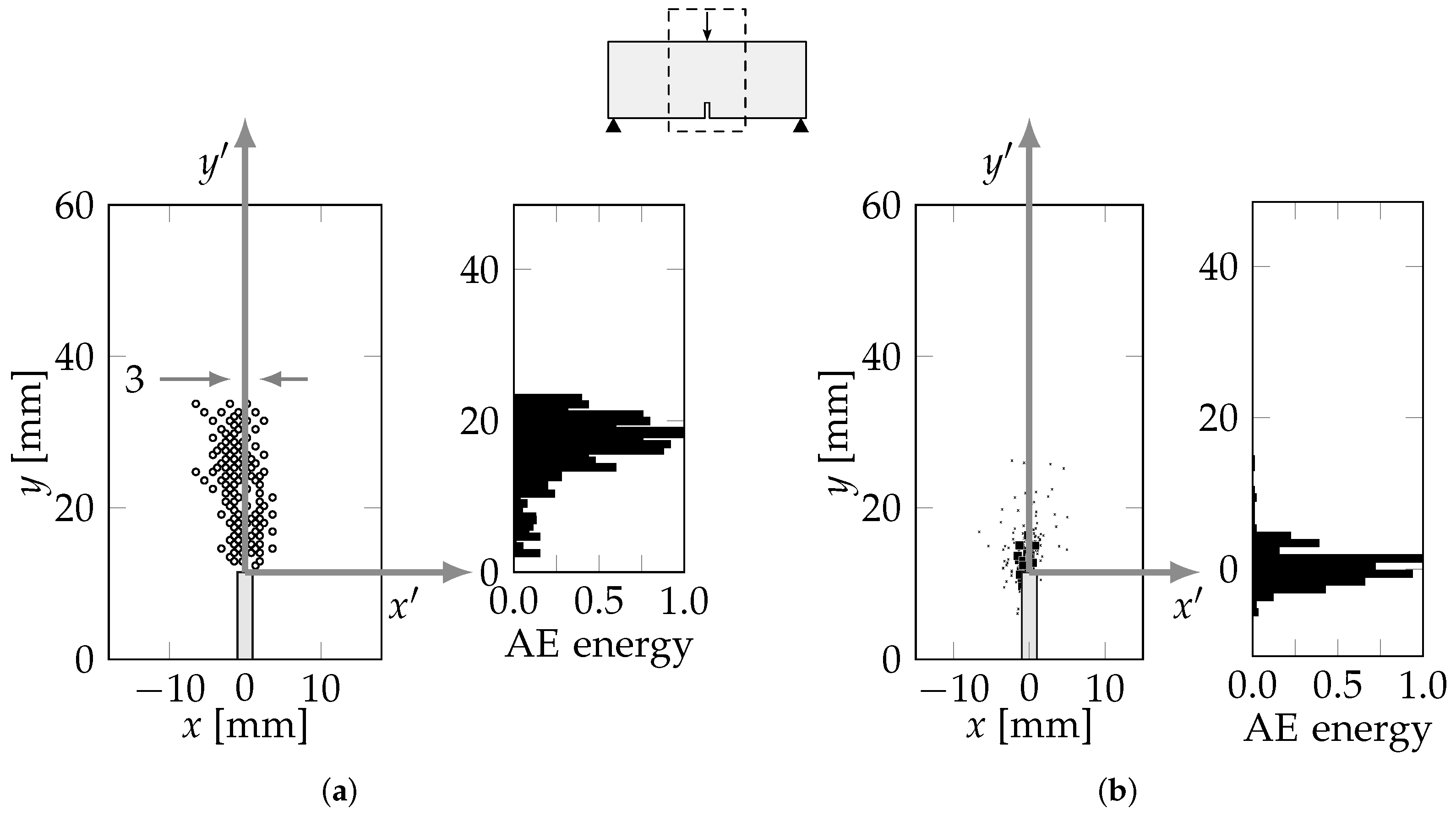
3.1. Model Description
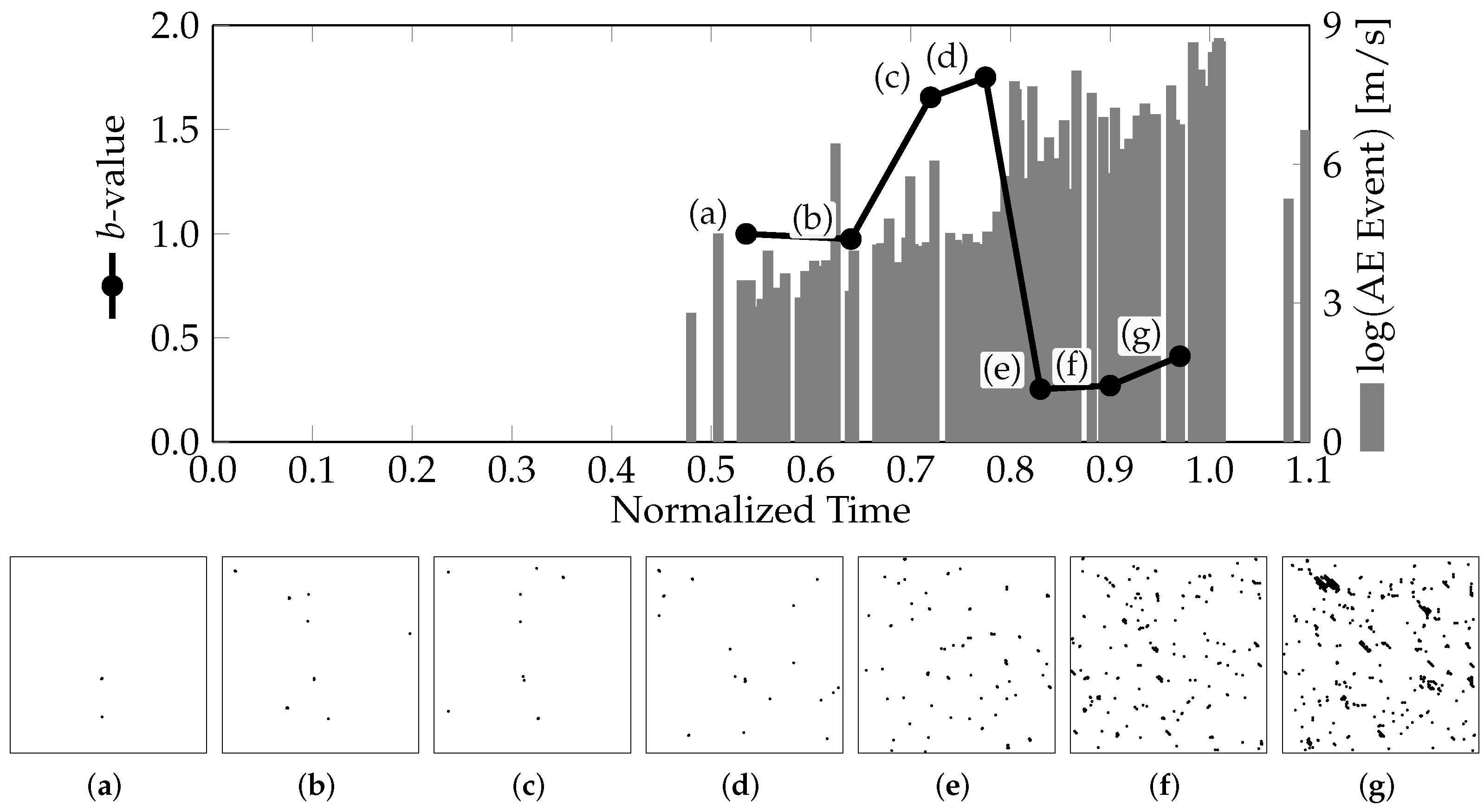
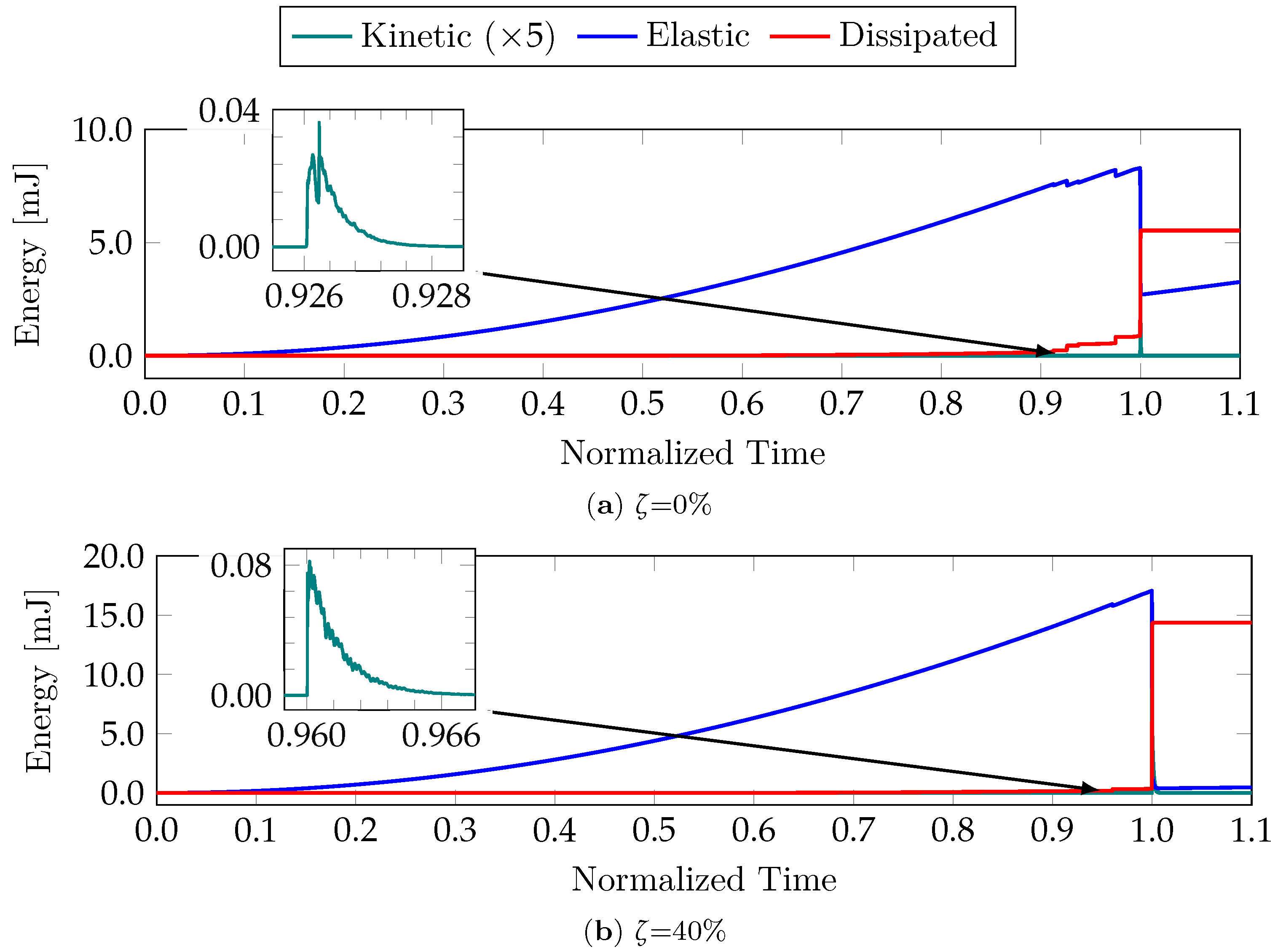
3.2. Results
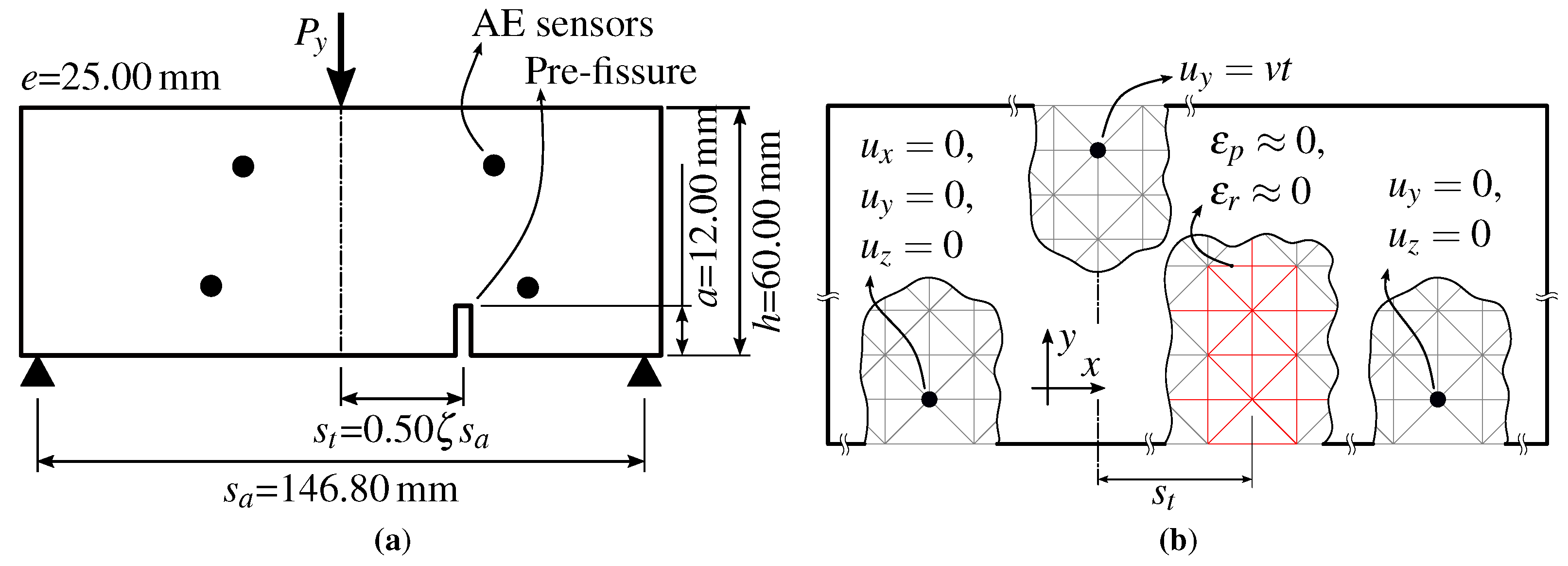
4. Second Application: Three-Point Bending Test and Comparison to Experimental Data
4.1. Model Description
- was considered a random field with a Weibull distribution, with a mean value computed by considering = , as shown in Figure 11, and using the classical fracture-intensity factor expression for the three-point-bending test, as provided in Equations (13) and (14) [61], yielding = 345,860 Nm, and = = .where
- The material porosity used by [41] was in the [0.1 mm–0.8 mm] interval, which was lower than the discretization level adopted in this study. For this reason, we assumed = , meaning that the random generation of each bar would be statistically independent. However, in the same reference, the variations in the tensile stress test were about 6% ( = [3.4 MPa–3.6 MPa]), whereas it was around 10% in [62] for sandstone specimens with the same dimensions. Previous studies using LDEM on tensile specimens with similar sizes showed that to obtain a close to 10%, the bars’ had to be about 65%. The links between the toughness random field properties and the global parameter variations were discussed in more detail in [50,63].
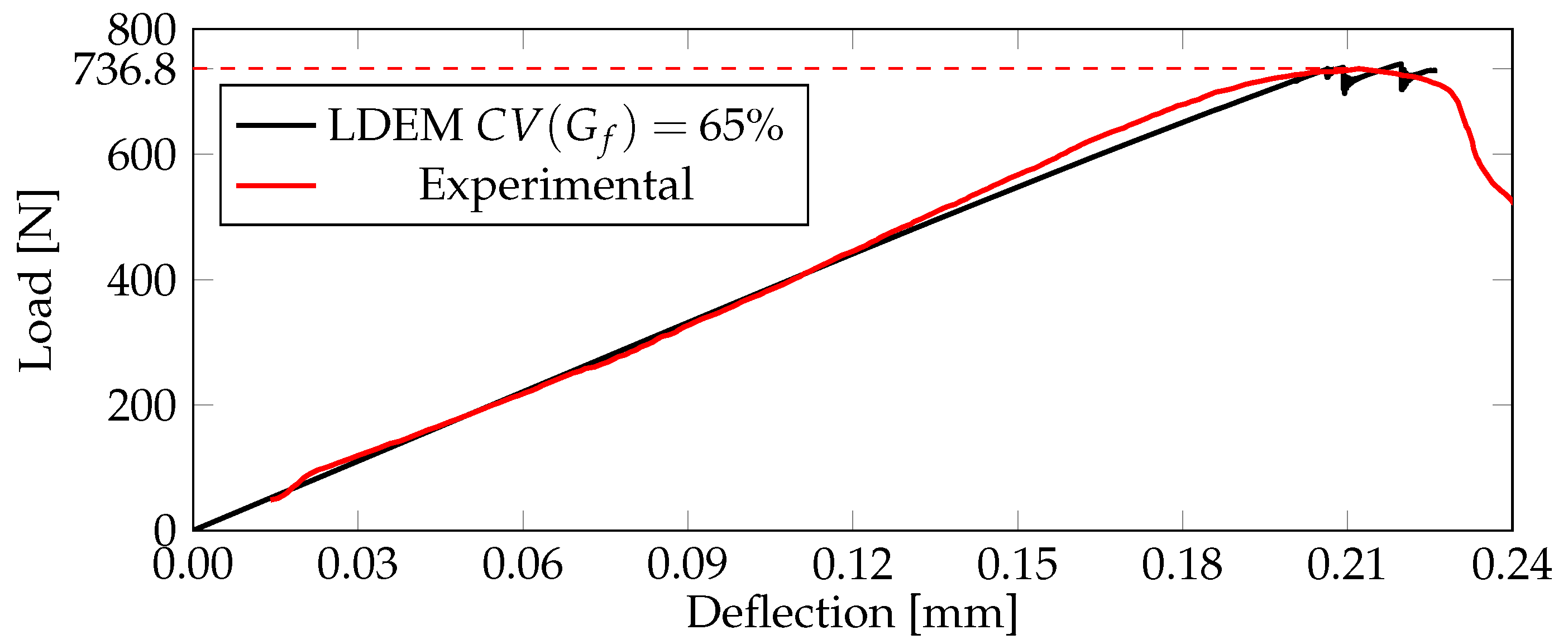
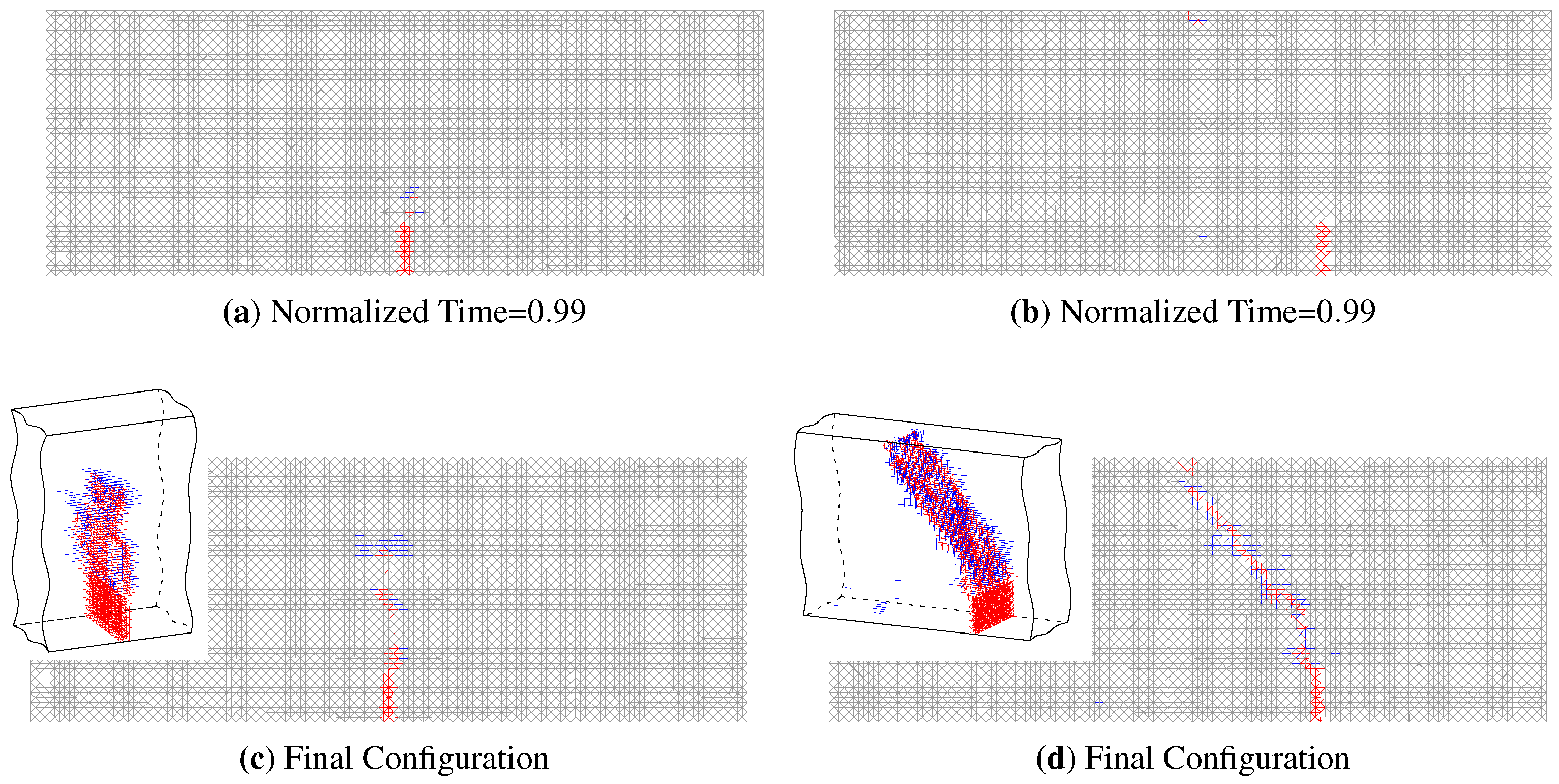
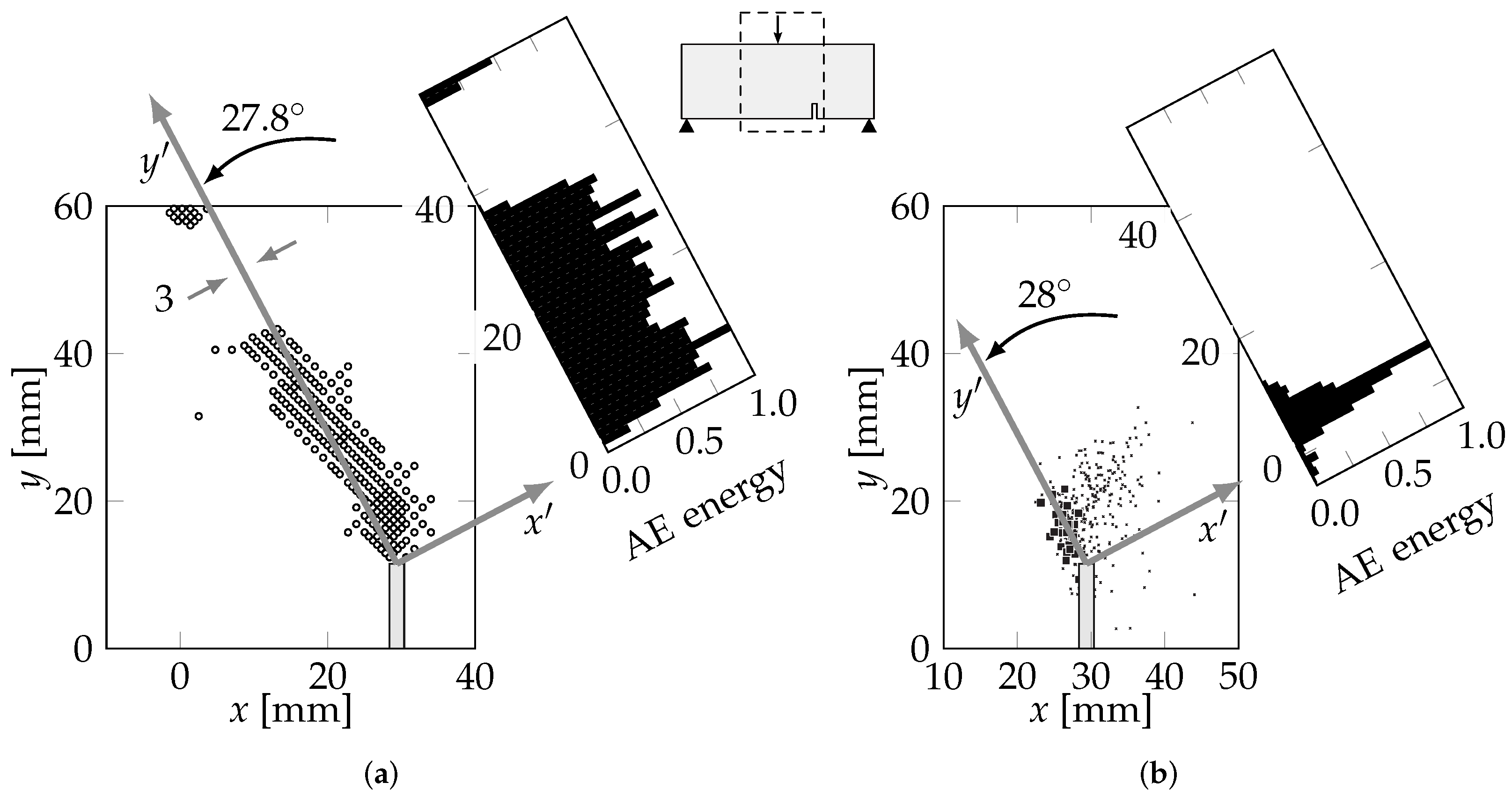
4.2. Results
- A significant part of the experimental AE events occurred below the pre-fissure’s head, whereas almost none appeared in the LDEM simulation. That difference was probably due to unintended damage in the pre-fissure region during the specimen preparation.
- The simulations indicated noticeable AE activities in regions other than along the main crack, such as in the vicinity of the load application (both tests) and in the horizontal direction crossing the top of the main fissure’s head (non-centered case). No such activity occurred in the corresponding regions during the experiments. These discrepancies were probably derived from the determination methods for identifying AE activity: The events in the numerical data were calculated from the kinetic energy produced inside the model, free from the attenuation that affected the signals captured by the sensors.
5. Conclusions
- The first study used simulations to illustrate the LDEM’s ability to emulate the typical results of AE tests, such as the spatial and temporal distributions of signals captured by AE sensors. It also yielded the calculations of the global parameters usually employed in AE methods for predicting local and global damage-induced instabilities.Those parameters were complemented by capturing the temporal and spatial distributions of the simulated elastic, dissipated, and kinetic energies involved. These were then used in an inverse analysis, linking the signals from the virtual AE sensors with the element-breaking events that caused their emission. These signal patterns were consistent with the system’s kinetic energy progression, i.e., every large-amplitude AE signal could be traced back to a correspondingly significant variation in the energy.By avoiding the numerous hard-to-track variables that characterize any experimental work, this approach showed a clear cause–effect link between the damage processes and the conclusions reached in our AE-based analysis, thus confirming AE coefficients as reliable failure predictors. The extension of this concept to real-world systems is subject to the effects of many extraneous factors, reducing the effectiveness accordingly. Nevertheless, AE analysis remains a valuable method for identifying global tendencies in structures undergoing damage, as indicated by Wilson in their re-normalization group procedure [64], and in other works addressing quasi-brittle materials [1,29,30,60].
- The second application used numerical simulations combined with the AE analysis of the experimental data from an actual pre-fissured sandstone beam undergoing damage. Here, the simulations were used not to mimic the experiment and corroborate the calculations of AE coefficients but to investigate the time–space distributions of the events, so the AE results could be linked to their probable causes in the structure’s interior as the damage progressed. The main points observed in this study are the following:
- –
- The simulation results of the LDEM model were qualitatively similar to the damage patterns observed experimentally, especially regarding the orientation of the major cracks.
- –
- The wave attenuation as it traveled throughout the structure was a primary limitation in identifying damage patterns through AE coefficients because it masked the corresponding signals from the data-acquisition apparatus.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| AE | Acoustic Emission |
| CMOD | Crack Mouth-Opening Displacement |
| LDEM | Lattice Discrete Element Method |
References
- Rundle, J.B.; Turcotte, D.L.; Shcherbakov, R.; Klein, W.; Sammis, C. Statistical physics approach to understanding the multiscale dynamics of earthquake fault systems: Statistical Physics of Earthquakes. Rev. Geophys. 2003, 41. [Google Scholar] [CrossRef]
- Needleman, A. A Continuum Model for Void Nucleation by Inclusion Debonding. J. Appl. Mech. 1987, 54, 525–531. [Google Scholar] [CrossRef]
- Belytschko, T.; Chen, H.; Xu, J.; Zi, G. Dynamic crack propagation based on loss of hyperbolicity and a new discontinuous enrichment. Int. J. Numer. Methods Eng. 2003, 58, 1873–1905. [Google Scholar] [CrossRef]
- Park, T.; Ahmed, B.; Voyiadjis, G.Z. A review of continuum damage and plasticity in concrete: Part I —Theoretical framework. Int. J. Damage Mech. 2022, 31, 901–954. [Google Scholar] [CrossRef]
- Voyiadjis, G.Z.; Ahmed, B.; Park, T. A review of continuum damage and plasticity in concrete: Part II —Numerical framework. Int. J. Damage Mech. 2022, 31, 762–794. [Google Scholar] [CrossRef]
- Jivkov, A.P.; Yates, J.R. Elastic behaviour of a regular lattice for meso-scale modelling of solids. Int. J. Solids Struct. 2012, 49, 3089–3099. [Google Scholar] [CrossRef]
- Mastilovic, S.; Rinaldi, A. Two-Dimensional Discrete Damage Models: Discrete Element Methods, Particle Models, and Fractal Theories. In Handbook of Damage Mechanics; Voyiadjis, G.Z., Ed.; Springer: New York, NY, USA, 2013; pp. 1–27. [Google Scholar] [CrossRef]
- Jenabidehkordi, A. Computational methods for fracture in rock: A review and recent advances. Front. Struct. Civ. Eng. 2019, 13, 273–287. [Google Scholar] [CrossRef]
- Atilgan, A.; Durell, S.; Jernigan, R.; Demirel, M.; Keskin, O.; Bahar, I. Anisotropy of Fluctuation Dynamics of Proteins with an Elastic Network Model. Biophys. J. 2001, 80, 505–515. [Google Scholar] [CrossRef]
- Rosenberg, E. Fractal Dimensions of Networks; Springer International Publishing: Cham, Switzerland, 2020. [Google Scholar] [CrossRef]
- Silling, S.; Askari, E. A Meshfree Method Based on the Peridynamic Model of Solid Mechanics. Comput. Struct. 2005, 83, 1526–1535. [Google Scholar] [CrossRef]
- Madenci, E.; Oterkus, E. Peridynamic Theory and Its Applications; Springer: New York, NY, USA, 2014. [Google Scholar] [CrossRef]
- Sheikhbahaei, P.; Mossaiby, F.; Shojaei, A. An efficient peridynamic framework based on the arc-length method for fracture modeling of brittle and quasi-brittle problems with snapping instabilities. Comput. Math. Appl. 2023, 136, 165–190. [Google Scholar] [CrossRef]
- Shojaei, A.; Hermann, A.; Seleson, P.; Silling, S.A.; Rabczuk, T.; Cyron, C.J. Peridynamic elastic waves in two-dimensional unbounded domains: Construction of nonlocal Dirichlet-type absorbing boundary conditions. Comput. Methods Appl. Mech. Eng. 2023, 407, 115948. [Google Scholar] [CrossRef]
- Ongaro, G.; Bertani, R.; Galvanetto, U.; Pontefisso, A.; Zaccariotto, M. A multiscale peridynamic framework for modelling mechanical properties of polymer-based nanocomposites. Eng. Fract. Mech. 2022, 274, 108751. [Google Scholar] [CrossRef]
- Shojaei, A.; Hermann, A.; Cyron, C.J.; Seleson, P.; Silling, S.A. A hybrid meshfree discretization to improve the numerical performance of peridynamic models. Comput. Methods Appl. Mech. Eng. 2022, 391, 114544. [Google Scholar] [CrossRef]
- Pierce, F.T. Tensile Tests for Cotton Yarns: “The Weakest Link” Theorems on the Strength of Long and of Composite Specimens. J. Text. Inst. Trans. 1926, 17, 355–368. [Google Scholar] [CrossRef]
- Daniels, H.E. The Statistical Theory of the Strength of Bundles of Threads. I. Proc. R. Soc. London. Ser. A. Math. Phys. Sci. 1945, 183, 405–435. [Google Scholar] [CrossRef]
- Hansen, A.; Hemmer, P.C.; Pradhan, S. The Fiber Bundle Model: Modeling Failure in Materials; Statistical Physics of Fracture and Breakdown; Wiley-VCH Verlag GmbH & Co. KGaA: Weinheim, Germany, 2015. [Google Scholar]
- de Arcangelis, L.; Redner, S.; Herrmann, H. A random fuse model for breaking processes. J. Phys. Lett. 1985, 46, 585–590. [Google Scholar] [CrossRef]
- Biswas, S.; Ray, P.; Chakrabarti, B.K. Statistical Physics of Fracture, Breakdown, and Earthquake: Effects of Disorder and Heterogeneity; John Wiley & Sons: Weinheim, Germany, 2015. [Google Scholar]
- Alava, M.J.; Nukala, P.K.V.V.; Zapperi, S. Statistical Models of Fracture. Adv. Phys. 2006, 55, 349–476. [Google Scholar] [CrossRef]
- Rabczuk, T.; Bordas, S.; Zi, G. A three-dimensional meshfree method for continuous multiple-crack initiation, propagation and junction in statics and dynamics. Comput. Mech. 2007, 40, 473–495. [Google Scholar] [CrossRef]
- Rossi Cabral, N.; Invaldi, M.A.; Barrios D’Ambra, R.; Iturrioz, I. An alternative bilinear peridynamic model to simulate the damage process in quasi-brittle materials. Eng. Fract. Mech. 2019, 216, 106494. [Google Scholar] [CrossRef]
- Friedrich, L.F.; Colpo, A.B.; Kosteski, L.E.; Vantadori, S.; Iturrioz, I. A novel peridynamic approach for fracture analysis of quasi-brittle materials. Int. J. Mech. Sci. 2022, 227, 107445. [Google Scholar] [CrossRef]
- Nayfeh, A.H.; Hefzy, M.S. Continuum Modeling of Three-Dimensional Truss-Like Space Structures. AIAA J. 1978, 16, 779–787. [Google Scholar] [CrossRef]
- Colpo, A.; Vantadori, S.; Friedrich, L.; Zanichelli, A.; Ronchei, C.; Scorza, D.; Iturrioz, I. A novel LDEM formulation with crack frictional sliding to estimate fracture and flexural behaviour of the shot-earth 772. Compos. Struct. 2023, 305, 116514. [Google Scholar] [CrossRef]
- Richter, C.F. Elementary Seismology; W. H. Freeman and Company: San Francisco, CA, USA; Bailey Bros. & Swinfen Ltd.: London, UK, 1958; Volume 2. [Google Scholar]
- Carpinteri, A.; Lacidogna, G.; Puzzi, S. From Criticality to Final Collapse: Evolution of the “b-Value” from 1.5 to 1.0. Chaos Solitons Fractals 2009, 41, 843–853. [Google Scholar] [CrossRef]
- Varotsos, P.A.; Sarlis, N.V.; Skordas, E.S. Natural Time Analysis: The New View of Time; Springer: Berlin/Heidelberg, Germany, 2011. [Google Scholar] [CrossRef]
- Grosse, C.; Ohtsu, M. (Eds.) Acoustic Emission Testing; Springer: Berlin/Heidelberg, Germany, 2008. [Google Scholar] [CrossRef]
- Shiotani, T.; Fujii, K.; Aoki, T.; Amou, K. Evaluation of Progressive Failure Using Ae Sources and Improved b-value on Slope Model Tests. In Progress in Acoustic Emission VII, Proceedings of the 12th International Acoustic Emission Symposium, Sapporo, Japan, 17–20 October 1994; Kishi, T., Ed.; Japanese Society for Non-Destructive Inspection: Tokyo, Japan, 1994; Volume 7, pp. 529–534. [Google Scholar]
- Colombo, I.S.; Main, I.G.; Forde, M.C. Assessing Damage of Reinforced Concrete Beam Using b-value Analysis of Acoustic Emission Signals. J. Mater. Civ. Eng. 2003, 15, 280–286. [Google Scholar] [CrossRef]
- Turcotte, D.L.; Newman, W.I.; Shcherbakov, R. Micro and Macroscopic Models of Rock Fracture. Geophys. J. Int. 2003, 152, 718–728. [Google Scholar] [CrossRef]
- Potirakis, S.; Mastrogiannis, D. Critical features revealed in acoustic and electromagnetic emissions during fracture experiments on LiF. Phys. A Stat. Mech. Its Appl. 2017, 485, 11–22. [Google Scholar] [CrossRef]
- Niccolini, G.; Potirakis, S.M.; Lacidogna, G.; Borla, O. Criticality Hidden in Acoustic Emissions and in Changing Electrical Resistance during Fracture of Rocks and Cement-Based Materials. Materials 2020, 13, 5608. [Google Scholar] [CrossRef]
- Lacidogna, G.; Piana, G.; Accornero, F.; Carpinteri, A. Multi-technique damage monitoring of concrete beams: Acoustic Emission, Digital Image Correlation, Dynamic Identification. Constr. Build. Mater. 2020, 242, 118114. [Google Scholar] [CrossRef]
- Rojo Tanzi, B.N.; Sobczyk, M.; Becker, T.; Segovia González, L.A.; Vantadori, S.; Iturrioz, I.; Lacidogna, G. Damage Evolution Analysis in a “Spaghetti” Bridge Model Using the Acoustic Emission Technique. Appl. Sci. 2021, 11, 2718. [Google Scholar] [CrossRef]
- Friedrich, L.; Colpo, A.; Maggi, A.; Becker, T.; Lacidogna, G.; Iturrioz, I. Damage process in glass fiber reinforced polymer specimens using acoustic emission technique with low frequency acquisition. Compos. Struct. 2021, 256, 113105. [Google Scholar] [CrossRef]
- Friedrich, L.F.; Rojo Tanzi, B.N.; Colpo, A.B.; Sobczyk, M.; Lacidogna, G.; Niccolini, G.; Iturrioz, I. Analysis of Acoustic Emission Activity during Progressive Failure in Heterogeneous Materials: Experimental and Numerical Investigation. Appl. Sci. 2022, 12, 3918. [Google Scholar] [CrossRef]
- Lin, Q.; Mao, D.; Wang, S.; Li, S. The Influences of Mode II Loading on Fracture Process in Rock Using Acoustic Emission Energy. Eng. Fract. Mech. 2018, 194, 136–144. [Google Scholar] [CrossRef]
- Kosteski, L.; Barrios D’Ambra, R.; Iturrioz, I. Crack propagation in elastic solids using the truss-like discrete element method. Int. J. Fract. 2012, 174, 139–161. [Google Scholar] [CrossRef]
- Hillerborg, A. A Model for Fracture Analysis; Report TVBM; Division of Building Materials, LTH, Lund University: Lund, Sweden, 1978; Volume 3005. [Google Scholar]
- Dimarogonas, A.D. Vibration for Engineers, 2nd ed.; Prentice-Hall International Prentice Hall: London, UK, 1996. [Google Scholar]
- Dassault Systèmes Americas Corp®. SIMULIA Academic Research, Release 2016; Abaqus: Waltham, MA, USA, 2016. [Google Scholar]
- Iturrioz, I.; Riera, J.D. Assessment of the Lattice Discrete Element Method in the simulation of wave propagation in inhomogeneous linearly elastic geologic materials. Soil Dyn. Earthq. Eng. 2021, 151, 106952. [Google Scholar] [CrossRef]
- Iturrioz, I.; Riera, J.D.; Miguel, L.F.F. Introduction of Imperfections in the Cubic Mesh of the Truss-Like Discrete Element Method. Fatigue Fract. Eng. Mater. Struct. 2014, 37, 539–552. [Google Scholar] [CrossRef]
- Kosteski, L.E. Aplicação Do Método Dos Elementos Discretos Formado Por Barras No Estudo Do Colapso De Estruturas. Ph.D. Thesis, Universidade Federal Do Rio Grande Do Sul, Porto Alegre, Brazil, 2012. [Google Scholar]
- Birck, G.; Rinaldi, A.; Iturrioz, I. The fracture process in quasi-brittle materials simulated using a lattice dynamical model. Fatigue Fract. Eng. Mater. Struct. 2019, 42, 2709–2724. [Google Scholar] [CrossRef]
- Puglia, V.B.; Kosteski, L.E.; Riera, J.D.; Iturrioz, I. Random Field Generation of the Material Properties in the Lattice Discrete Element Method. J. Strain Anal. Eng. Des. 2019, 54, 236–246. [Google Scholar] [CrossRef]
- Carpinteri, A. Application of Fracture Mechanics to Concrete Structures. J. Struct. Div. 1982, 108, 833–848. [Google Scholar] [CrossRef]
- Taylor, D. The Theory of Critical Distances: A New Perspective in Fracture Mechanics; Elsevier: Amsterdam, The Netherlands; Boston, MA, USA, 2007. [Google Scholar]
- Kosteski, L.E.; Iturrioz, I.; Lacidogna, G.; Carpinteri, A. Size effect in heterogeneous materials analyzed through a lattice discrete element method approach. Eng. Fract. Mech. 2020, 232, 107041. [Google Scholar] [CrossRef]
- Rojo Tanzi, B.N. Análise do Processo de Dano com a Técnica de Emissão Acústica e Métodos Discretos. Master’s Thesis, Universidade Federal Do Rio Grande Do Sul, Porto Alegre, Brazil, 2020. [Google Scholar]
- Birck, G.; Riera, J.D.; Iturrioz, I. Numerical DEM simulation of AE in plate fracture and analogy with the frequency of seismic events in SCRs. Eng. Fail. Anal. 2018, 93, 214–223. [Google Scholar] [CrossRef]
- Riera, J.D.; Miguel, L.F.F.; Iturrioz, I. Study of imperfections in the cubic mesh of the truss-like discrete element method. Int. J. Damage Mech. 2014, 23, 819–838. [Google Scholar] [CrossRef]
- Gutenberg, B.; Richter, C.F. Magnitude and Energy of Earthquakes. Nature 1955, 176, 795. [Google Scholar] [CrossRef]
- Cutugno, P. Space-Time Correlation of Earthquakes and Acoustic Emission Monitoring of Historical Constructions. Ph.D Thesis, Politecnico di Torino, Torino, Italy, 2017. [Google Scholar]
- Carpinteri, A.; Lacidogna, G.; Corrado, M.; Di Battista, E. Cracking and Crackling in Concrete-Like Materials: A Dynamic Energy Balance. Eng. Fract. Mech. 2016, 155, 130–144. [Google Scholar] [CrossRef]
- Iturrioz, I.; Lacidogna, G.; Carpinteri, A. Experimental Analysis and Truss-Like Discrete Element Model Simulation of Concrete Specimens Under Uniaxial Compression. Eng. Fract. Mech. 2013, 110, 81–98. [Google Scholar] [CrossRef]
- Anderson, T. Fracture Mechanics: Fundamentals and Applications, 3rd ed.; CRC Press: Boca Raton, FL, USA, 2017. [Google Scholar] [CrossRef]
- van Vliet, M.R.; van Mier, J.G. Size effect of concrete and sandstone. HERON 2000, 45, 91–108. [Google Scholar]
- Kosteski, L.E.; Iturrioz, I.; Friedrich, L.F.; Lacidogna, G. A study by the lattice discrete element method for exploring the fractal nature of scale effects. Sci. Rep. 2022, 12, 16744. [Google Scholar] [CrossRef]
- Wilson, K.G. Problems in Physics with many Scales of Length. Sci. Am. 1979, 241, 158–179. [Google Scholar] [CrossRef]


| E | ||||||||
|---|---|---|---|---|---|---|---|---|
| 70 −1 | 100% | 2.50% | 32 | 2400 −3 | 0.25 | 4 | 4 |
| E | |||||||
|---|---|---|---|---|---|---|---|
| −1 | 65% | 15 | 2800 −3 | 0.25 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tanzi, B.N.R.; Birck, G.; Sobczyk, M.; Iturrioz, I.; Lacidogna, G. Truss-like Discrete Element Method Applied to Damage Process Simulation in Quasi-Brittle Materials. Appl. Sci. 2023, 13, 5119. https://doi.org/10.3390/app13085119
Tanzi BNR, Birck G, Sobczyk M, Iturrioz I, Lacidogna G. Truss-like Discrete Element Method Applied to Damage Process Simulation in Quasi-Brittle Materials. Applied Sciences. 2023; 13(8):5119. https://doi.org/10.3390/app13085119
Chicago/Turabian StyleTanzi, Boris Nahuel Rojo, Gabriel Birck, Mario Sobczyk, Ignacio Iturrioz, and Giuseppe Lacidogna. 2023. "Truss-like Discrete Element Method Applied to Damage Process Simulation in Quasi-Brittle Materials" Applied Sciences 13, no. 8: 5119. https://doi.org/10.3390/app13085119
APA StyleTanzi, B. N. R., Birck, G., Sobczyk, M., Iturrioz, I., & Lacidogna, G. (2023). Truss-like Discrete Element Method Applied to Damage Process Simulation in Quasi-Brittle Materials. Applied Sciences, 13(8), 5119. https://doi.org/10.3390/app13085119









