An Improvement of DV-Hop Localization Algorithm Based on Cyclotomic Method in Wireless Sensor Networks
Abstract
1. Introduction
2. Related Works
3. DV-Hop and Error Analysis
3.1. DV-Hop Localization Algorithm
3.2. Error Analysis
4. Proposed Algorithm: CMWN-DV-HOP
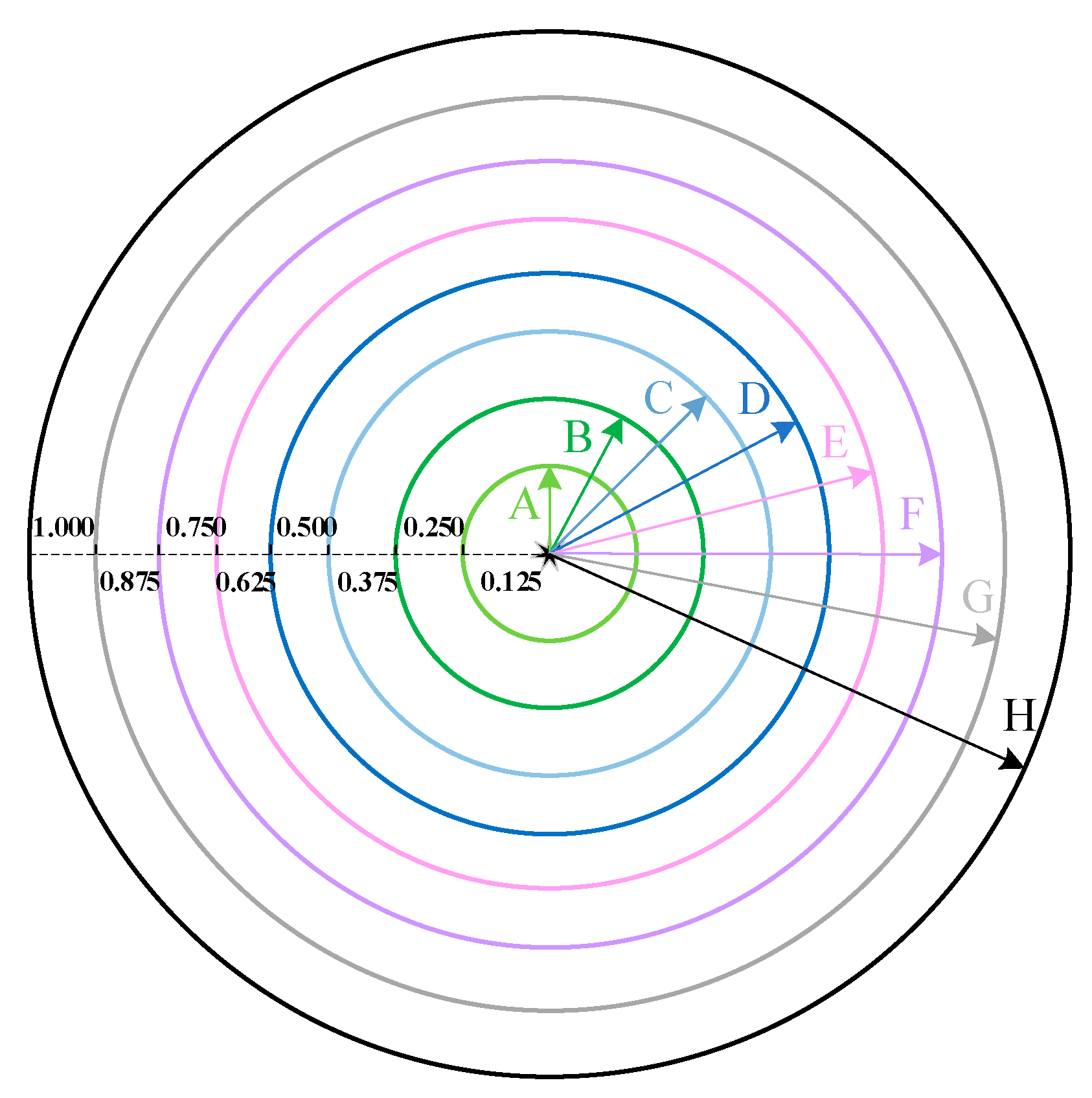
4.1. Cyclotomic Method
4.2. Weighted Recursive Least-Squares Algorithm (WRLS)
4.3. CMWN-DV-Hop
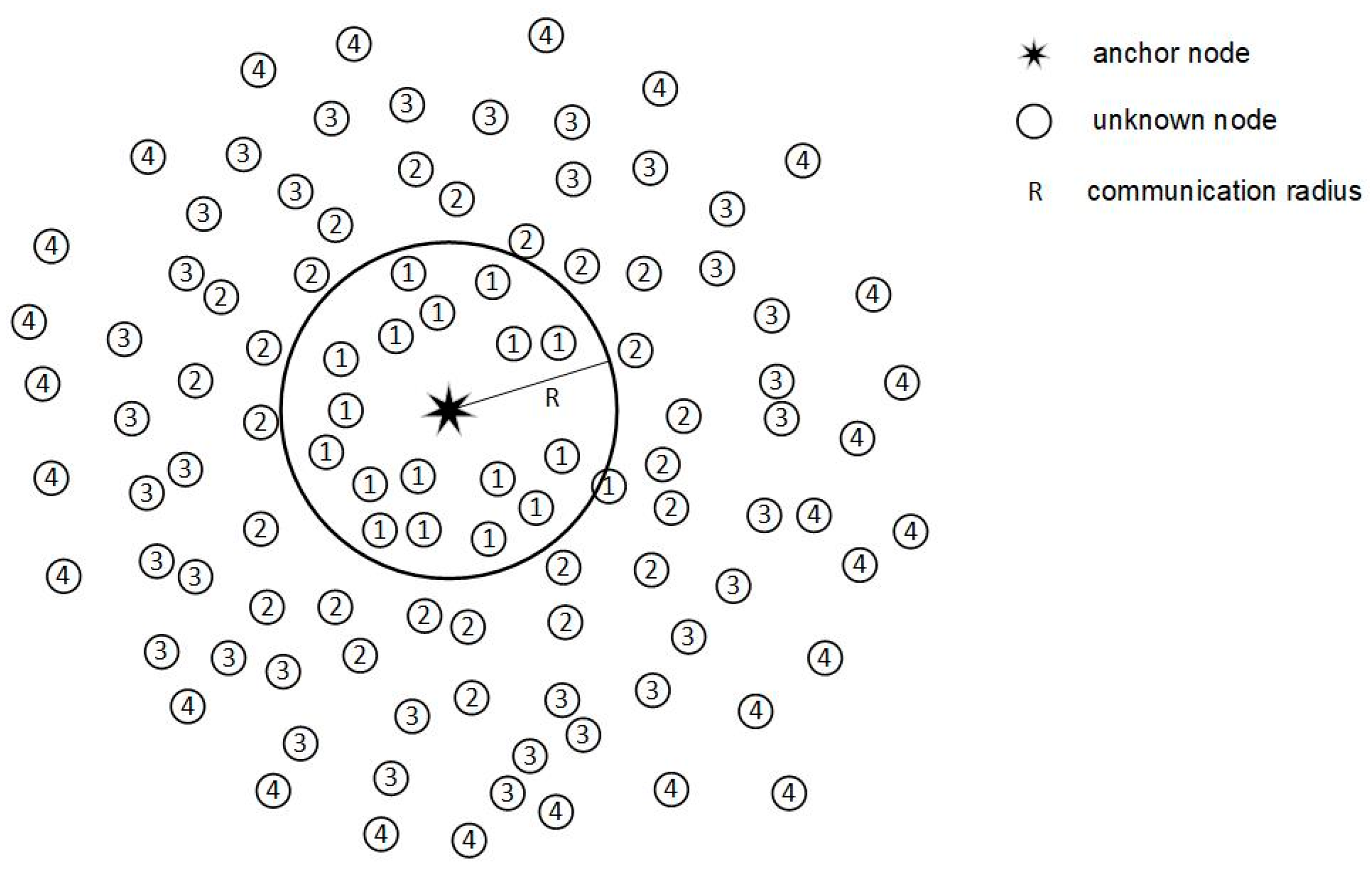
4.3.1. Calculation of the Hop Value Based on the Circle Cutting Technique
4.3.2. Introduce the Weighting Factor
4.3.3. WRLS Is Applied to Calculate the Unknown Node Coordinates
5. Simulation Outcomes and Analysis
5.1. Virtual Setting and Appraisal Criteria
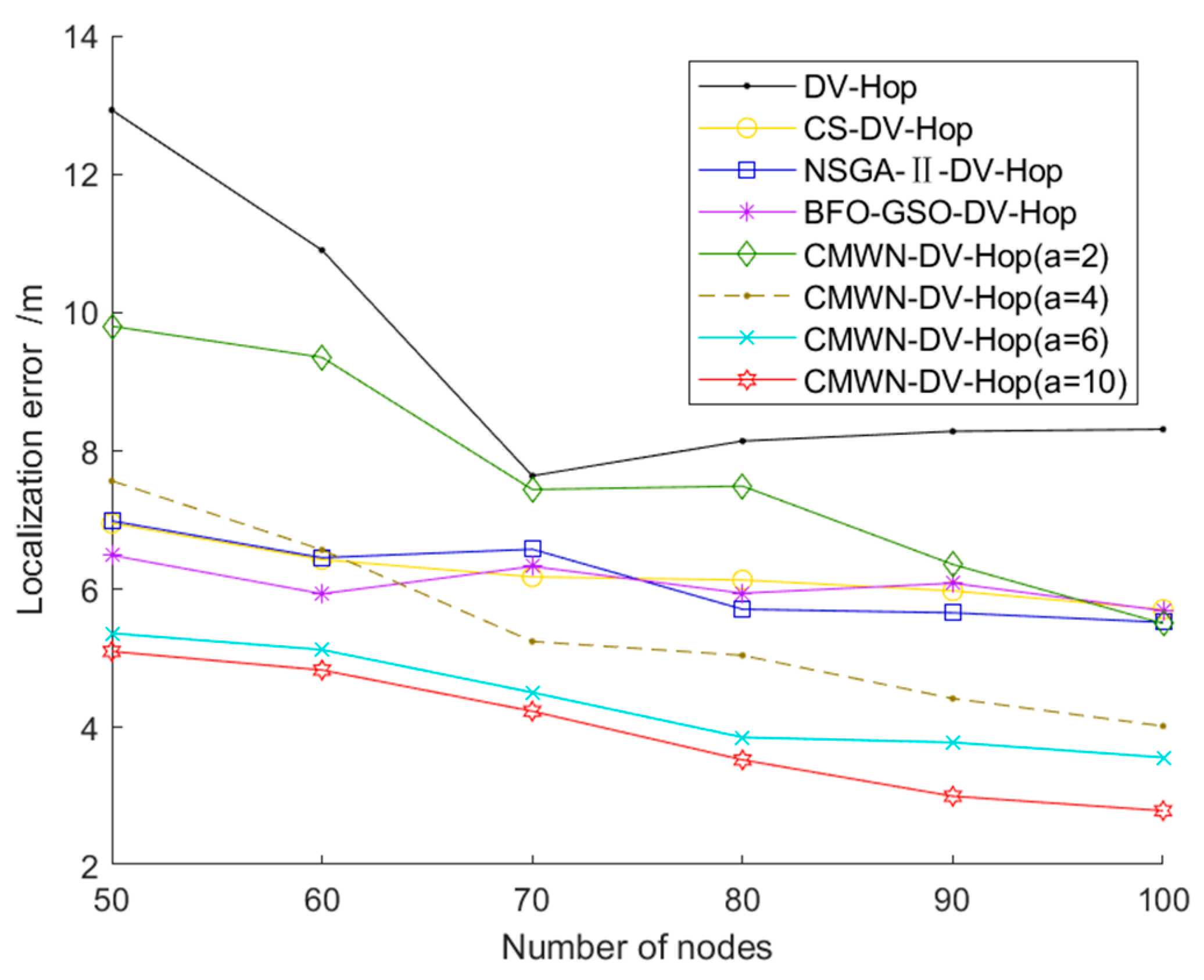
5.2. Influence of the Number of Nodes
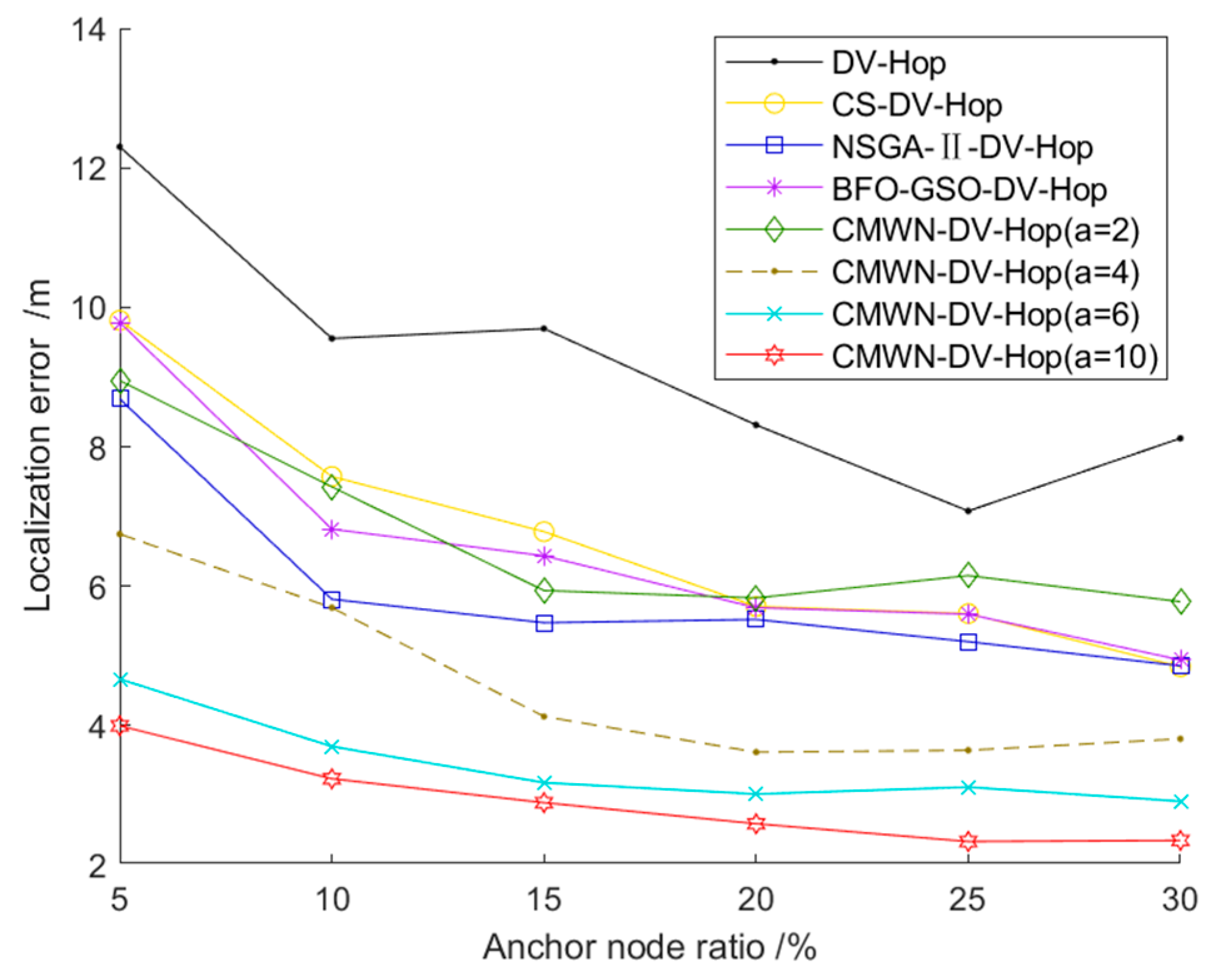
5.3. Influence of Anchor Node Ratio
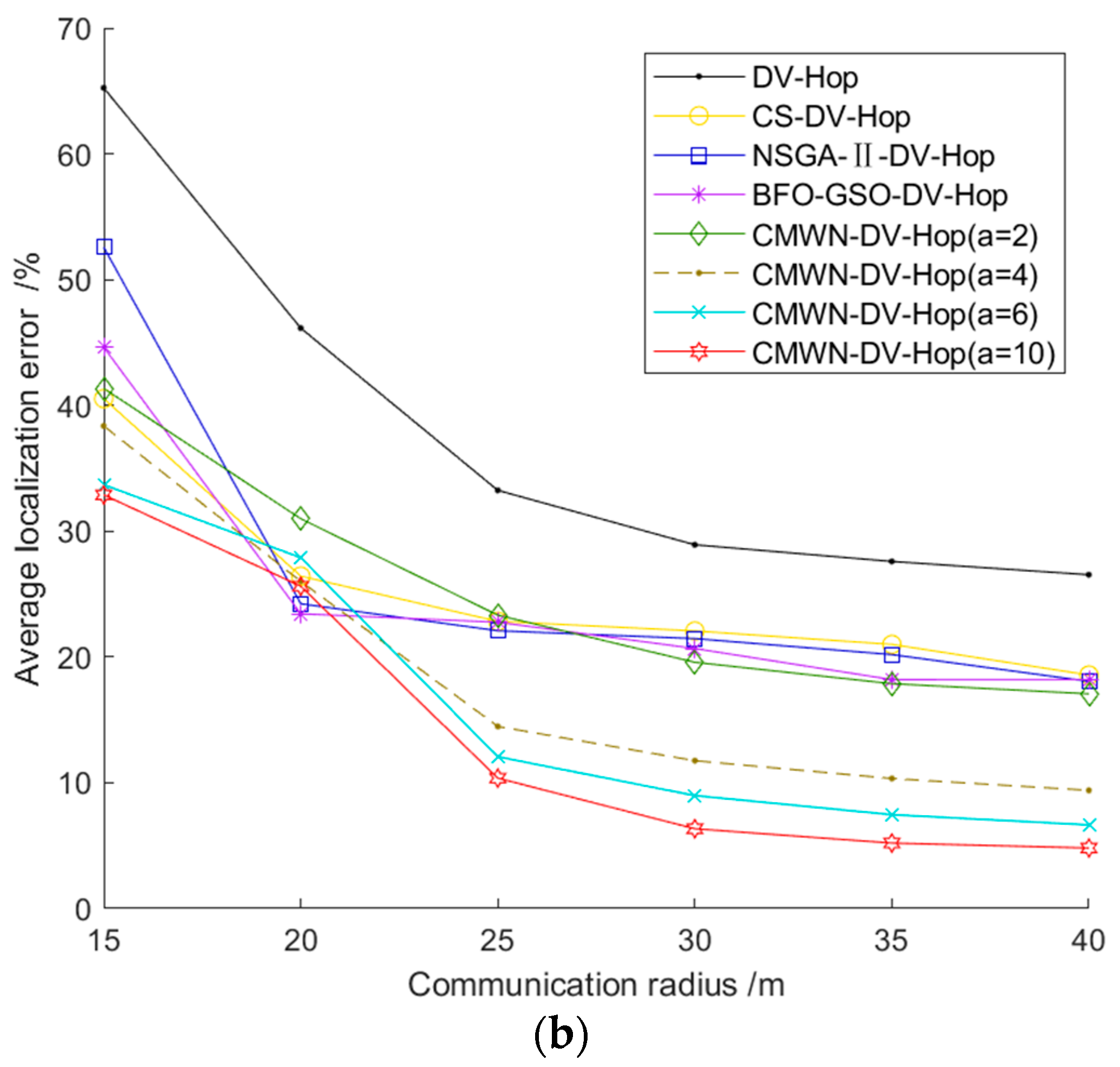
5.4. Influence of Communication Radius
5.5. Energy Consumption Analysis
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Gui, L.; Xiao, F.; Zhou, Y.; Shu, F.; Val, T. Connectivity Based DV-Hop Localization for Internet of Things. IEEE Trans. Veh. Technol. 2020, 8, 8949–8958. [Google Scholar] [CrossRef]
- Qichen, W. Research progress on wireless sensor network (WSN) security technology. J. Phys. Conf. Ser. 2022, 2256, 012043. [Google Scholar] [CrossRef]
- Lu, W.; Xu, X.; Huang, G.; Li, B.; Wu, Y.; Zhao, N.; Yu, F.R. Energy efficiency optimization in SWIPT enabled WSNs for smart agriculture. IEEE Trans. Ind. Inform. 2020, 6, 4335–4344. [Google Scholar] [CrossRef]
- Oğur, N.B.; Al-Hubaishi, M.; Çeken, C. IoT data analytics architecture for smart healthcare using RFID and WSN. ETRI J. 2022, 1, 135–146. [Google Scholar] [CrossRef]
- Strumberger, I.; Minovic, M.; Tuba, M.; Bacanin, N. Performance of Elephant Herding Optimization and Tree Growth Algorithm Adapted for Node Localization in Wireless Sensor Networks. Sensors 2019, 11, 2515. [Google Scholar] [CrossRef]
- Han, D.; Yu, Y.; Li, K.-C.; de Mello, R.F. Enhancing the sensor node localization algorithm based on improved DV-hop and DE algorithms in wireless sensor Networks. Sensors 2020, 2, 343. [Google Scholar] [CrossRef]
- Zhong, Z.; He, T. MSP: Multi-sequence positioning of wireless sensor nodes. In Proceedings of the 5th International Conference on Embedded Networked Sensor Systems, Sydney, Australia, 6–9 November 2007; pp. 15–28. [Google Scholar] [CrossRef]
- He, T.; Huang, C.; Blum, B.M.; Stankovic, J.A.; Abdelzaher, T. Range-free localization schemes for large scale sensor networks. In Proceedings of the 9th Annual International Conference on Mobile Computing and Networking, San Diego, CA, USA, 14–19 September 2003; pp. 81–95. [Google Scholar] [CrossRef]
- Niculescu, D.; Nath, B. DV based positioning in ad hoc networks. Telecommun. Syst. 2003, 1, 267–280. [Google Scholar] [CrossRef]
- Xiong, Y.; Wu, N.; Shen, Y.; Win, M.Z. Cooperative Network Synchronization: Asymptotic Analysis. IEEE Trans. Signal Process. 2017, 3, 757–772. [Google Scholar] [CrossRef]
- Xiong, Y.; Wu, N.; Shen, Y.; Win, M.Z. Cooperative Localization in Massive Networks. IEEE Trans. Inf. Theory 2021, 1237–1258. [Google Scholar] [CrossRef]
- Tang, Q.; Wang, J. An Improved DV-Hop Localization Algorithm for Wireless Sensor Network Based on TDOA Quantization. In Proceedings of the 2017 International Conference on Network and Information Systems for Computers (ICNISC), Shanghai, China, 14–16 April 2017; pp. 19–24. [Google Scholar] [CrossRef]
- Cai, X.; Wang, H.; Cui, Z.; Cai, J.; Xue, Y.; Wang, L. Bat algorithm with triangle-flipping strategy for numerical optimization. Int. J. Mach. Learn. Cybern. 2018, 2, 199–215. [Google Scholar] [CrossRef]
- Li, M.; Liu, Y. Rendered path: Range-free localization in anisotropic sensor networks with holes. In Proceedings of the 13th Annual ACM International Conference on Mobile Computing and Networking, Montréal, QC, Canada, 9–14 September 2007; pp. 51–62. [Google Scholar] [CrossRef]
- Shahzad, F.; Sheltami, T.R.; Shakshuki, E.M. DV-maxHop: A fast and accurate range-free localization algorithm for anisotropic wireless networks. IEEE Trans. Mob. Comput. 2016, 16, 2494–2505. [Google Scholar] [CrossRef]
- Li, X.; Wang, K.; Liu, B.; Xiao, J.; Han, S. An improved range-free location algorithm for industrial wireless sensor networks. EURASIP J. Wirel. Commun. Netw. 2020, 1, 1–13. [Google Scholar] [CrossRef]
- Yu, W.; Li, H. An improved DV-Hop localization method in wireless sensor networks. In Proceedings of the 2012 IEEE International Conference on Computer Science and Automation Engineering (CSAE), Zhangjiajie, China, 25–27 May 2012; pp. 199–202. [Google Scholar] [CrossRef]
- Prashar, D.; Jyoti, K.; Kumar, D. Design and analysis of distance error correction–based localization algorithm for wireless sensor networks. Trans. Emerg. Telecommun. Technol. 2018, 12, e3547. [Google Scholar] [CrossRef]
- Yi, L.; Chen, M. An Enhanced Hybrid 3D Localization Algorithm Based on APIT and DV-Hop. Int. J. Online Eng. 2017, 9, 69–86. [Google Scholar] [CrossRef]
- Li, T.; Wang, C.; Na, Q. Research on DV-Hop improved algorithm based on dual communication radius. EURASIP J. Wirel. Commun. Netw. 2020, 1, 1–10. [Google Scholar] [CrossRef]
- Gui, L.; Huang, X.; Xiao, F.; Zhang, Y.; Shu, F.; Wei, J.; Val, T. DV-hop localization with protocol sequence based access. IEEE Trans. Veh. Technol. 2018, 10, 9972–9982. [Google Scholar] [CrossRef]
- Kaur, A.; Kumar, P.; Gupta, G.P. A weighted centroid localization algorithm for randomly deployed wireless sensor networks. J. King Saud Univ.-Comput. Inf. Sci. 2019, 1, 82–91. [Google Scholar] [CrossRef]
- Cui, Z.; Li, F.; Zhang, W. Bat algorithm with principal component analysis. Int. J. Mach. Learn. Cybern. 2019, 3, 603–622. [Google Scholar] [CrossRef]
- Zhou, C.; Yang, Y.; Wang, Y. DV-Hop localization algorithm based on bacterial foraging optimization for wireless multimedia sensor networks. Multimed. Tools Appl. 2019, 4, 4299–4309. [Google Scholar] [CrossRef]
- Kaur, A.; Kumar, P.; Gupta, G. Nature Inspired Algorithm-Based Improved Variants of DV-Hop Algorithm for Randomly Deployed 2D and 3D Wireless Sensor Networks. Wirel. Pers. Commun. 2018, 101, 567–582. [Google Scholar] [CrossRef]
- Song, L.; Zhao, L.; Ye, J. DV-hop node location algorithm based on GSO in wireless sensor networks. J. Sens. 2019, 9, 298695. [Google Scholar] [CrossRef]
- Kumar, S.; Lobiyal, D. Novel DV-Hop localization algorithm for wireless sensor networks. Telecommun. Syst. 2017, 3, 509–524. [Google Scholar] [CrossRef]
- Yang, X.-S.; Deb, S. Cuckoo search via Lévy flights. In Proceedings of the 2009 World congress on Nature & Biologically Inspired Computing (NaBIC), Coimbatore, India, 9–11 December 2009; pp. 210–214. [Google Scholar] [CrossRef]
- Cui, Z.; Sun, B.; Wang, G.; Xue, Y.; Chen, J. A novel oriented cuckoo search algorithm to improve DV-Hop performance for cyber–physical systems. J. Parallel Distrib. Comput. 2017, 103, 42–52. [Google Scholar] [CrossRef]
- Wang, Y.; Wang, P.; Zhang, J.; Cai, X.; Li, W.; Ma, Y. A novel DV-Hop method based on coupling algorithm used for wireless sensor network localization. Int. J. Wirel. Mob. Comput. 2019, 2, 128–137. [Google Scholar] [CrossRef]
- Farjow, W.; Raahemifar, K.; Fernando, X. Novel wireless channels characterization model for underground mines. Appl. Math. Model. 2015, 19, 5997–6007. [Google Scholar] [CrossRef]
- Chen, W.; Jiang, X.; Li, X.; Gao, J.; Xu, X.; Ding, S. Wireless Sensor Network nodes correlation method in coal mine tunnel based on Bayesian decision. Measurement 2013, 8, 2335–2340. [Google Scholar] [CrossRef]
- Albaidhani, A.; Morell, A.; Vicario, J.L. Anchor selection for UWB indoor positioning. Trans. Emerg. Telecommun. Technol. 2019, 6, e3598. [Google Scholar] [CrossRef]
- Yusoff, Y.; Ngadiman, M.S.; Zain, A.M. Overview of NSGA-II for optimizing machining process parameters. Procedia Eng. 2011, 15, 3978–3983. [Google Scholar] [CrossRef]
- Wang, P.; Xue, F.; Li, H.; Cui, Z.; Xie, L.; Chen, J. A multi-objective DV-Hop localization algorithm based on NSGA-II in internet of things. Mathematics 2019, 2, 184. [Google Scholar] [CrossRef]
- Huang, Y.; Zhang, L. Weighted DV-Hop Localization Algorithm for Wireless Sensor Network based on Differential Evolution Algorithm. In Proceedings of the 2019 IEEE 2nd International Conference on Information and Computer Technologies (ICICT), Kahului, HI, USA, 14–17 March 2019; pp. 14–18. [Google Scholar] [CrossRef]
- Liu, D.; Peng, S.; Lin, K.; Zhang, J. A Weight-based DV-HOP Improved Localization Algorithm for Wireless Sensor Networks. Int. J. Online Eng. 2014, 10, 22–27. [Google Scholar] [CrossRef]
- Qiang, L.; Xia, H.; Yuhang, X.; Dan, Z. Improved DV-Hop Based on Dynamic Parameters Differential Evolution Localization Algorithm. In Proceedings of the 2020 IEEE 8th International Conference on Information, Communication and Networks (ICICN), Xi’an, China, 22–25 August 2020; pp. 129–134. [Google Scholar] [CrossRef]
- Cao, Y.; Wang, Z. Improved DV-hop localization algorithm based on dynamic anchor node set for wireless sensor networks. IEEE Access 2019, 7, 124876–124890. [Google Scholar] [CrossRef]
- Huang, X.; Han, D.; Cui, M.; Lin, G.; Yin, X. Three-Dimensional Localization Algorithm Based on Improved A* and DV-Hop Algorithms in Wireless Sensor Network. Sensors 2021, 2, 448. [Google Scholar] [CrossRef]
- Hu, Y.; Li, X. An improvement of DV-Hop localization algorithm for wireless sensor networks. Telecommun. Syst. 2013, 1, 13–18. [Google Scholar] [CrossRef]
- Mehrabi, M.; Taghdiri, P.; Latzko, V.; Salah, H.; Fitzek, F.H. Accurate Energy-Efficient Localization Algorithm for IoT Sensors. In Proceedings of the ICC 2020—2020 IEEE International Conference on Communications (ICC), Dublin, Ireland, 7–11 June 2020; pp. 1–6. [Google Scholar] [CrossRef]
- Tong, H.; Ng, M. Analysis of regularized least squares for functional linear regression model. J. Complex. 2018, 49, 85–94. [Google Scholar] [CrossRef]
- Xiaohui, Z.; Xuanze, Z.; Gang, W.; Cui, H. Some Examples of Materialist Dialectics in the Concept of Higher Mathematics. Educ. Res. Int. 2022, 2022, 5200153. [Google Scholar] [CrossRef]
- Xihai, Z.; Junlong, F.; Fanfeng, M. An efficient node localization approach with RSSI for randomly deployed wireless sensor networks. J. Electr. Comput. Eng. 2016, 2016, 2080854. [Google Scholar] [CrossRef]



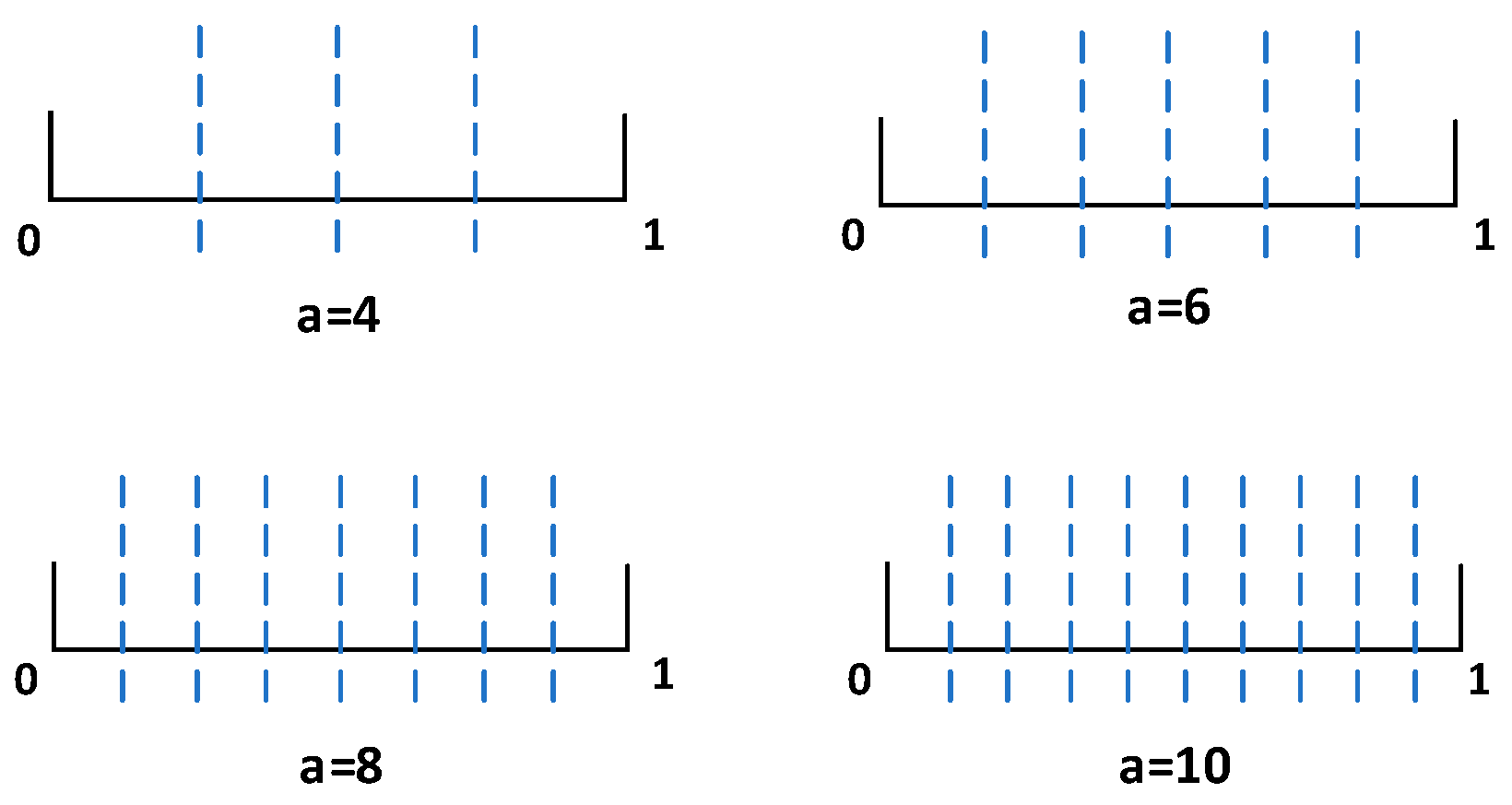



| Parameter | Value |
|---|---|
| Node distribution | Random |
| Anchor node ratio | 20% (5–30%) |
| Communication radius (R) | 25 (15–40) |
| Number of nodes | 100 (50–100) |
| Segmentation factor (a) | 2, 4, 6, 10 |
| Deployed environment | 100 m × 100 m |
| Transmission signal power Ps | 20 dBm |
| Frequency of signal f | 5 GHz |
| Path loss exponent η | 4 |
| Reference distance d0 | 1 m |
| Transmitting energy consumption | 1.50 mJ |
| Computational energy consumption | 0.20 mJ |
| Receiving energy consumption | 1.15 mJ |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhao, Q.; Xu, Z.; Yang, L. An Improvement of DV-Hop Localization Algorithm Based on Cyclotomic Method in Wireless Sensor Networks. Appl. Sci. 2023, 13, 3597. https://doi.org/10.3390/app13063597
Zhao Q, Xu Z, Yang L. An Improvement of DV-Hop Localization Algorithm Based on Cyclotomic Method in Wireless Sensor Networks. Applied Sciences. 2023; 13(6):3597. https://doi.org/10.3390/app13063597
Chicago/Turabian StyleZhao, Qing, Zhen Xu, and Lei Yang. 2023. "An Improvement of DV-Hop Localization Algorithm Based on Cyclotomic Method in Wireless Sensor Networks" Applied Sciences 13, no. 6: 3597. https://doi.org/10.3390/app13063597
APA StyleZhao, Q., Xu, Z., & Yang, L. (2023). An Improvement of DV-Hop Localization Algorithm Based on Cyclotomic Method in Wireless Sensor Networks. Applied Sciences, 13(6), 3597. https://doi.org/10.3390/app13063597







