Analysis of the Strength of the Supporting Structure of a TwoSection Hopper Wagon under Operating Loading Conditions
Abstract
1. Introduction
2. Analysis of Recent Research and Publications
3. The Purpose and Main Tasks of the Article
- -
- To calculate the strength of the two-section hopper-wagon-supporting structure under the main operational load modes;
- -
- To determine the vertical load of a two-section hopper wagon;
- -
- To investigate the bearing-structure load of a two-section hopper wagon on a railway ferry.
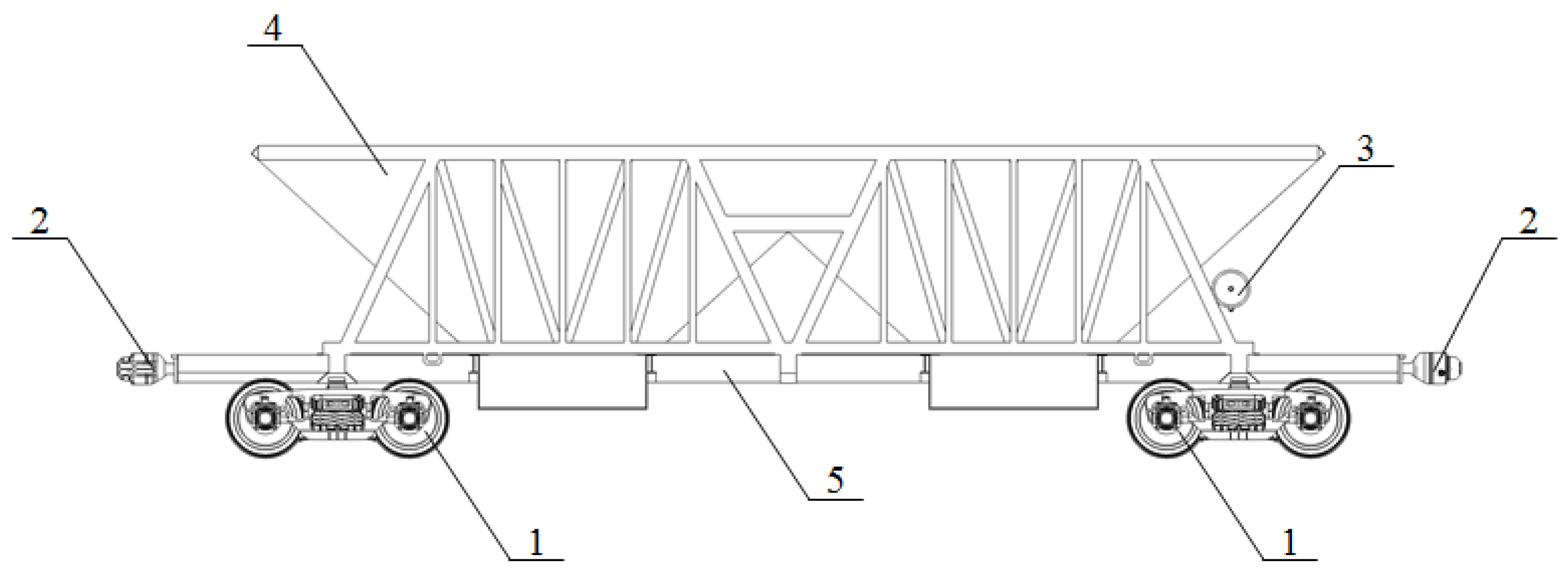
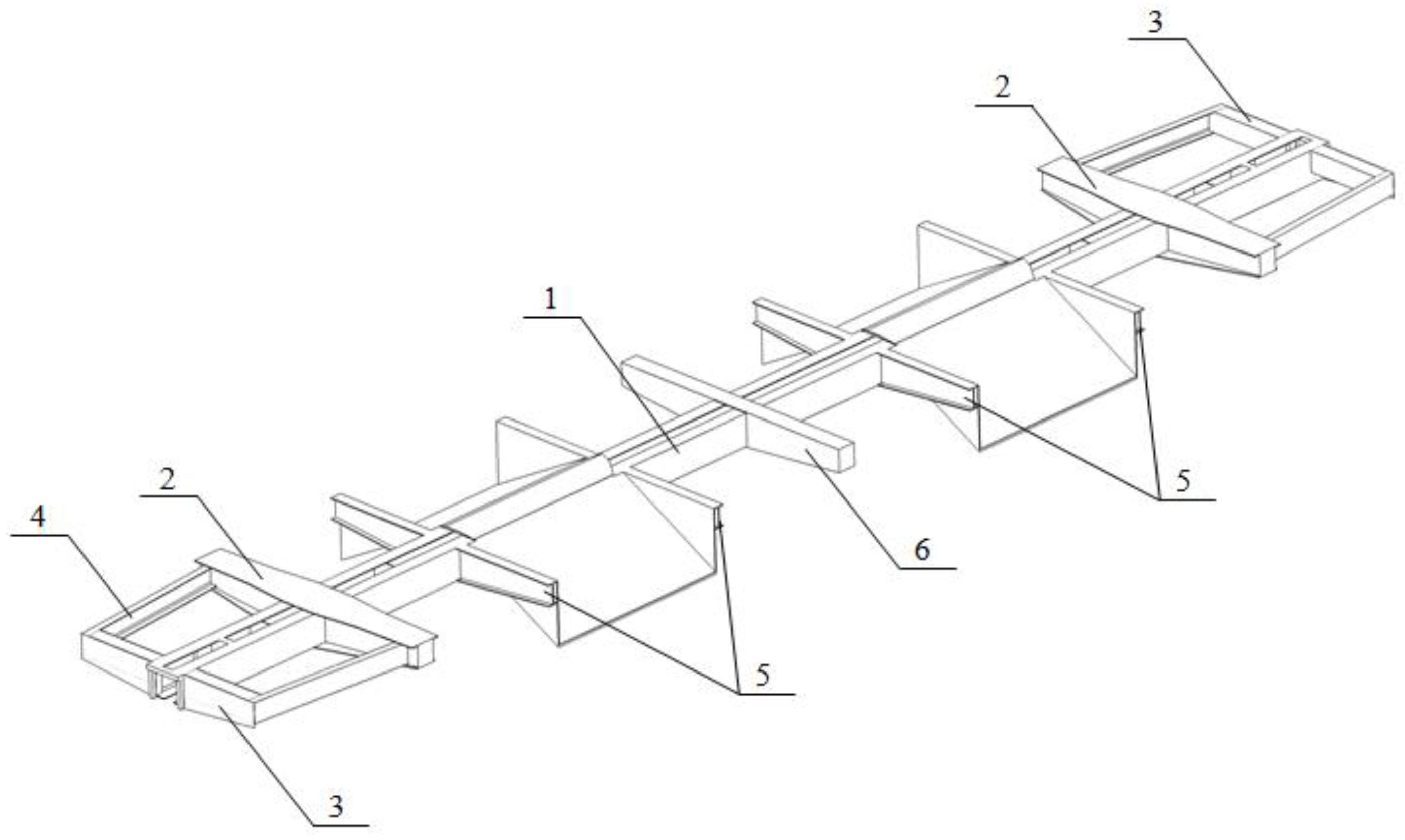
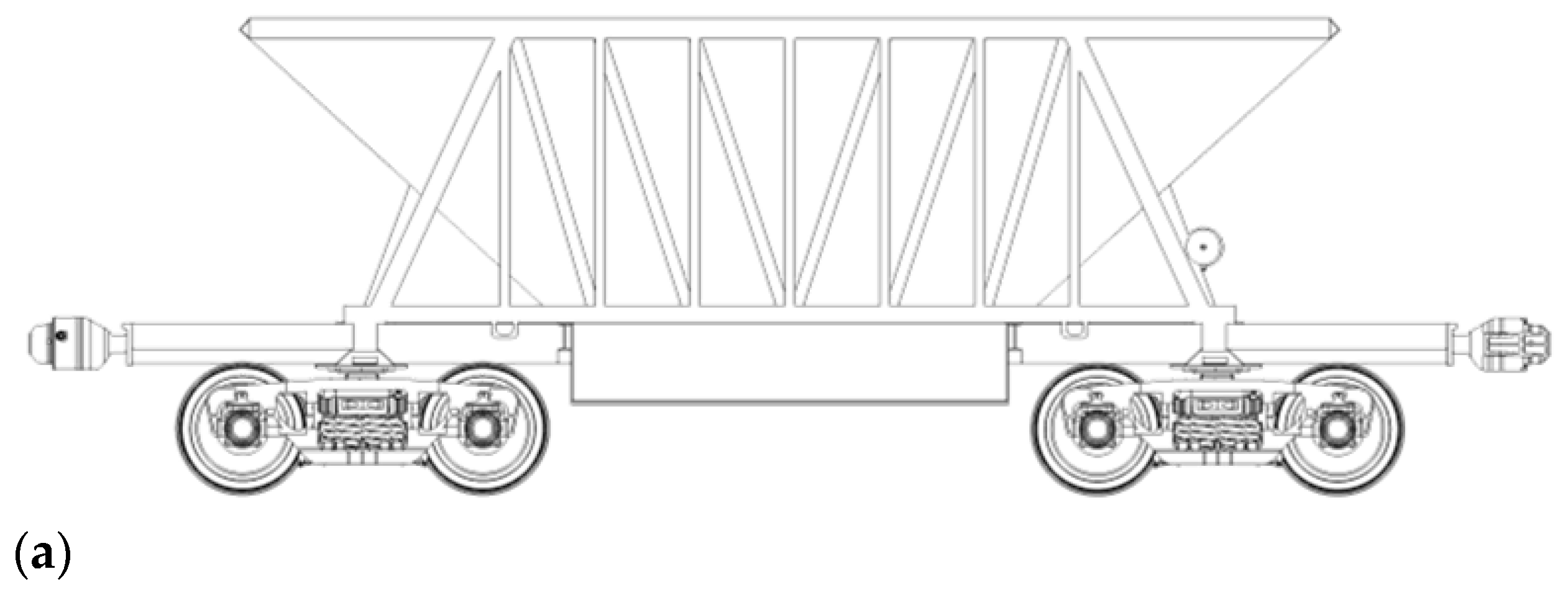
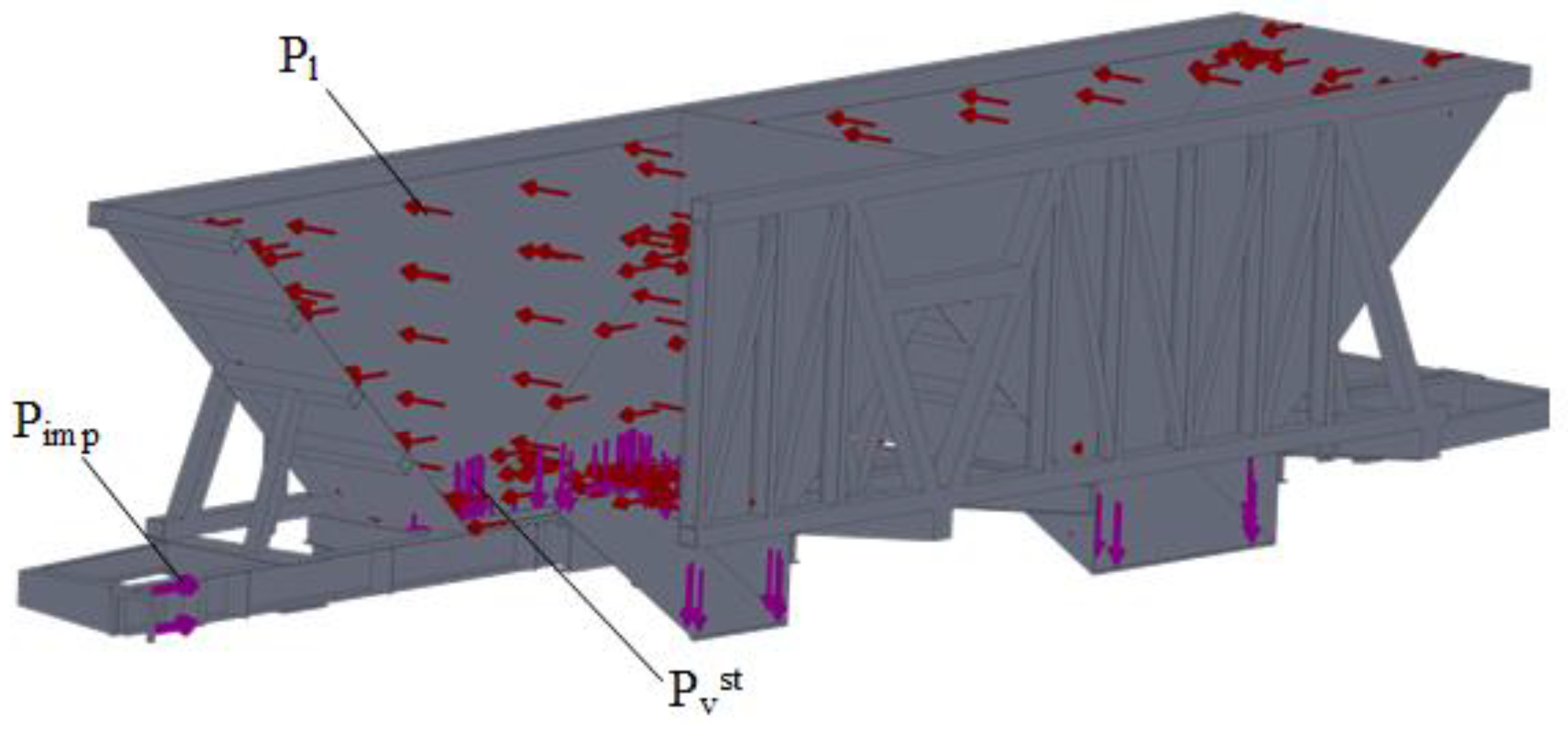
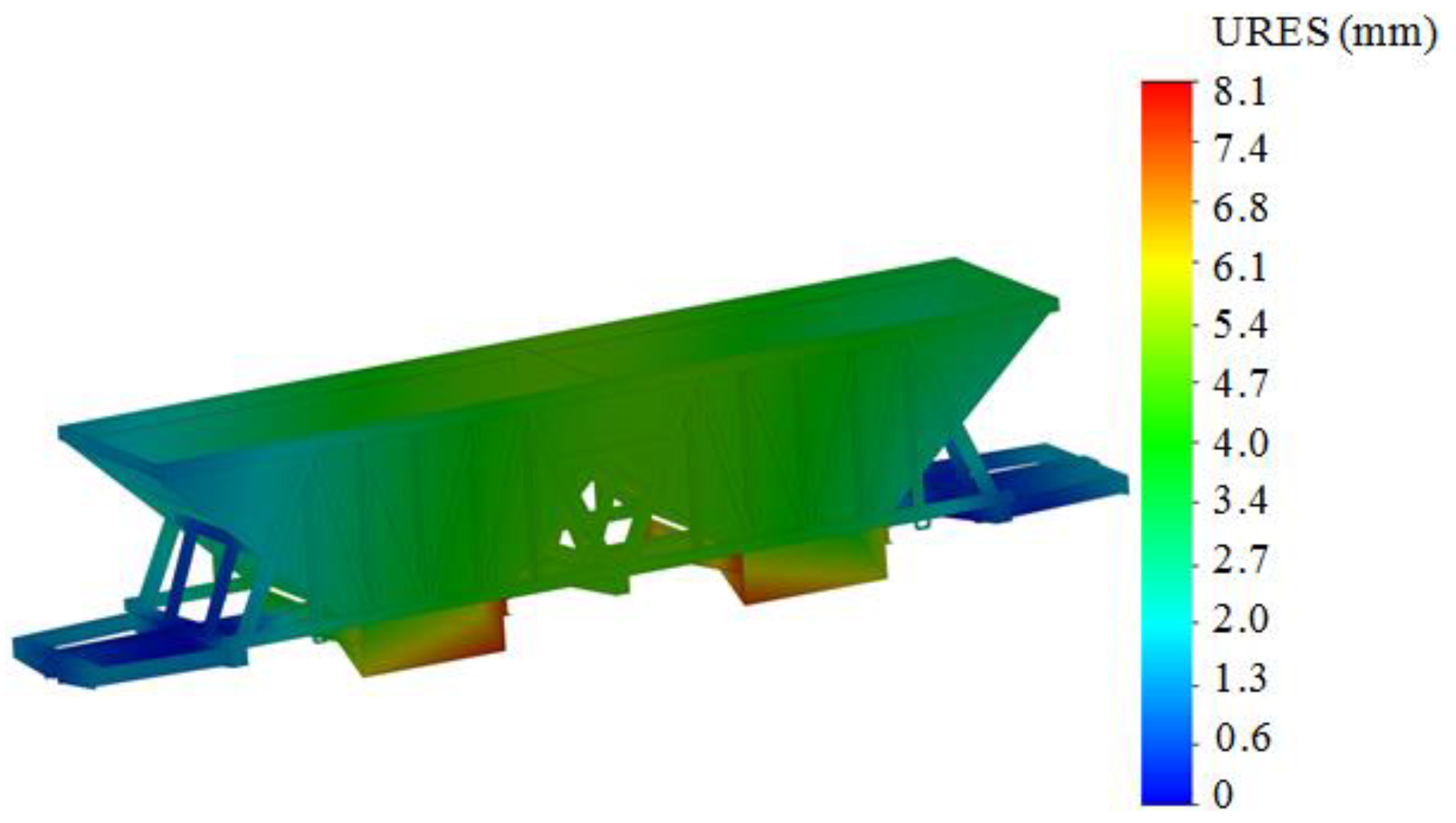
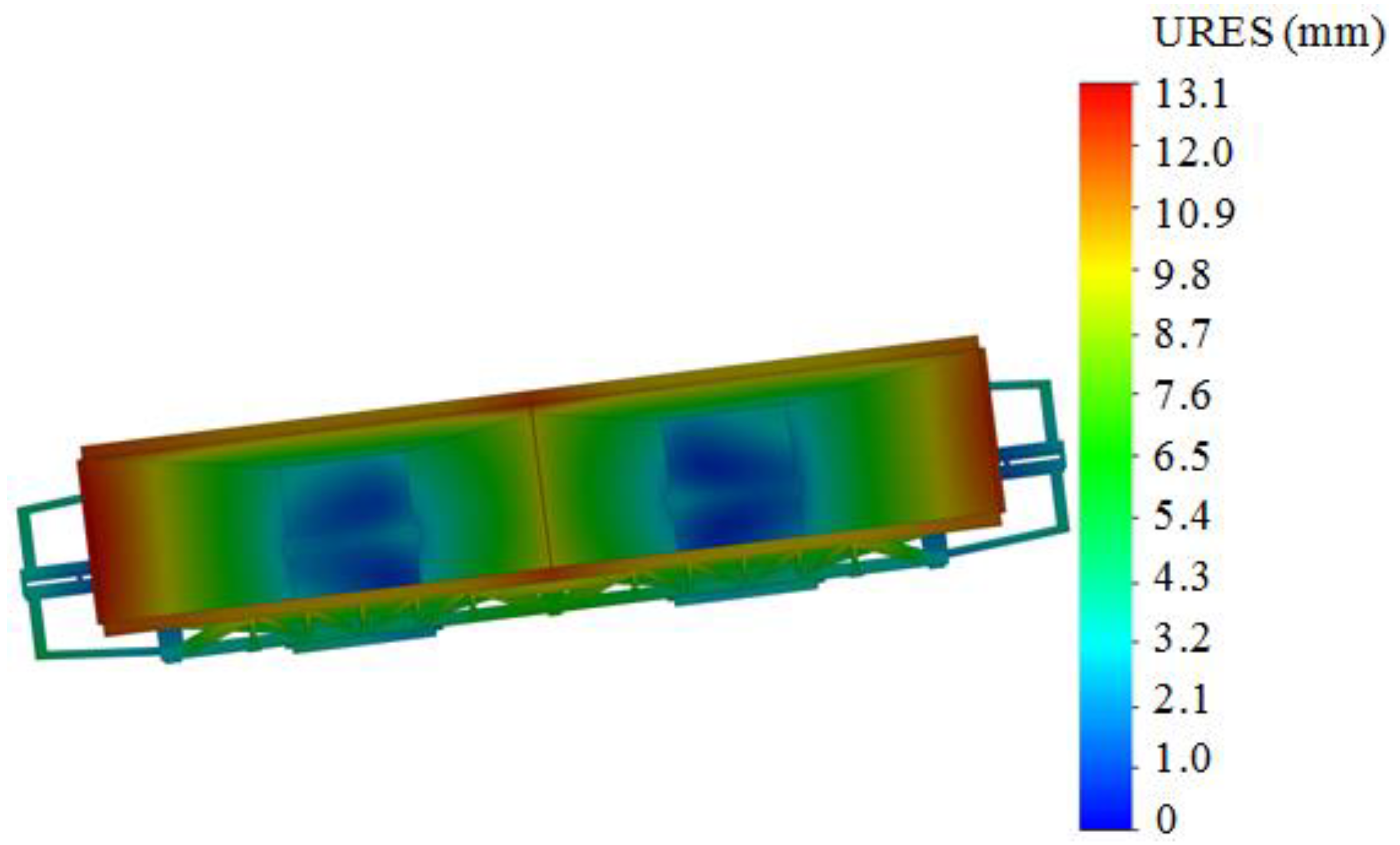
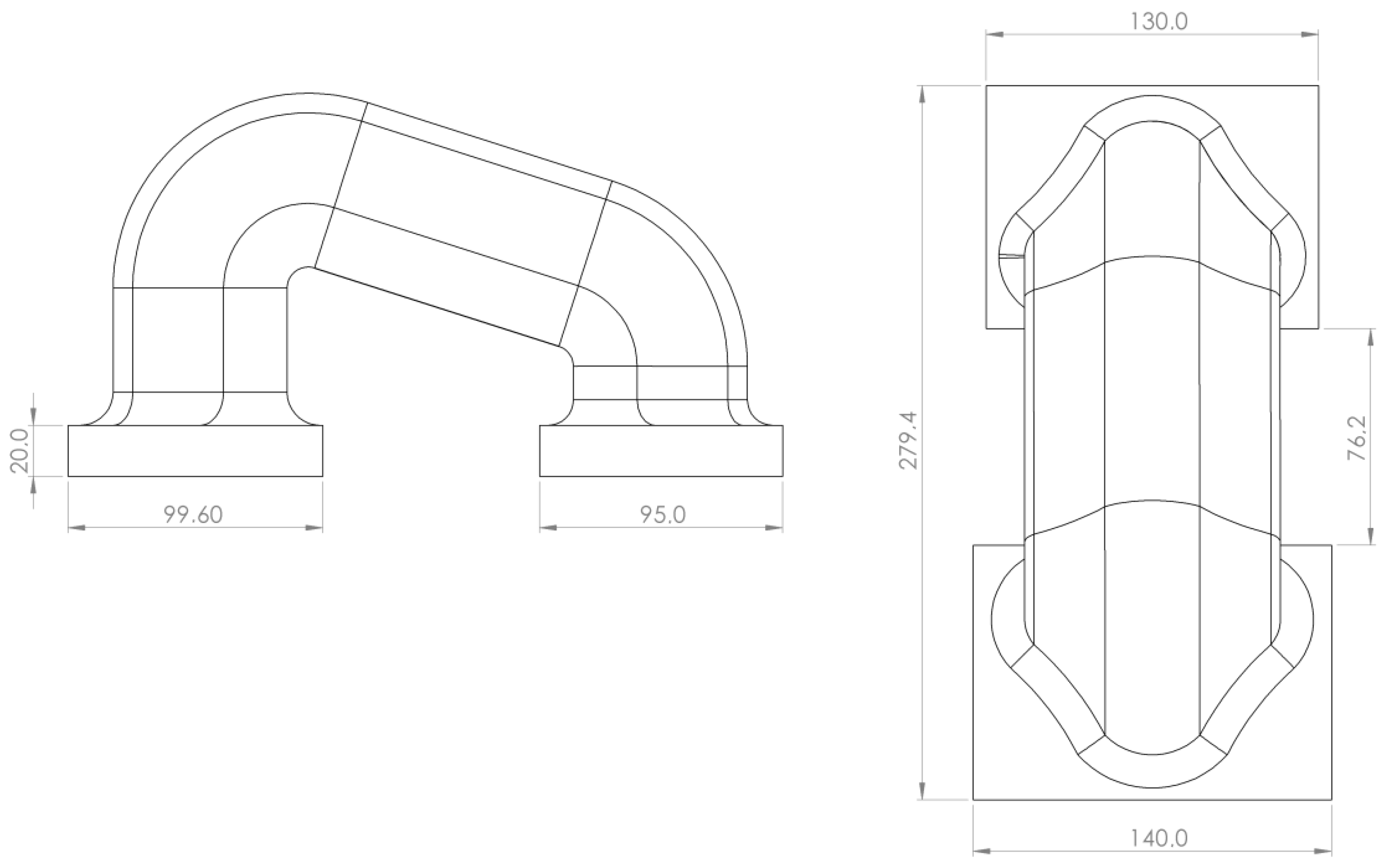
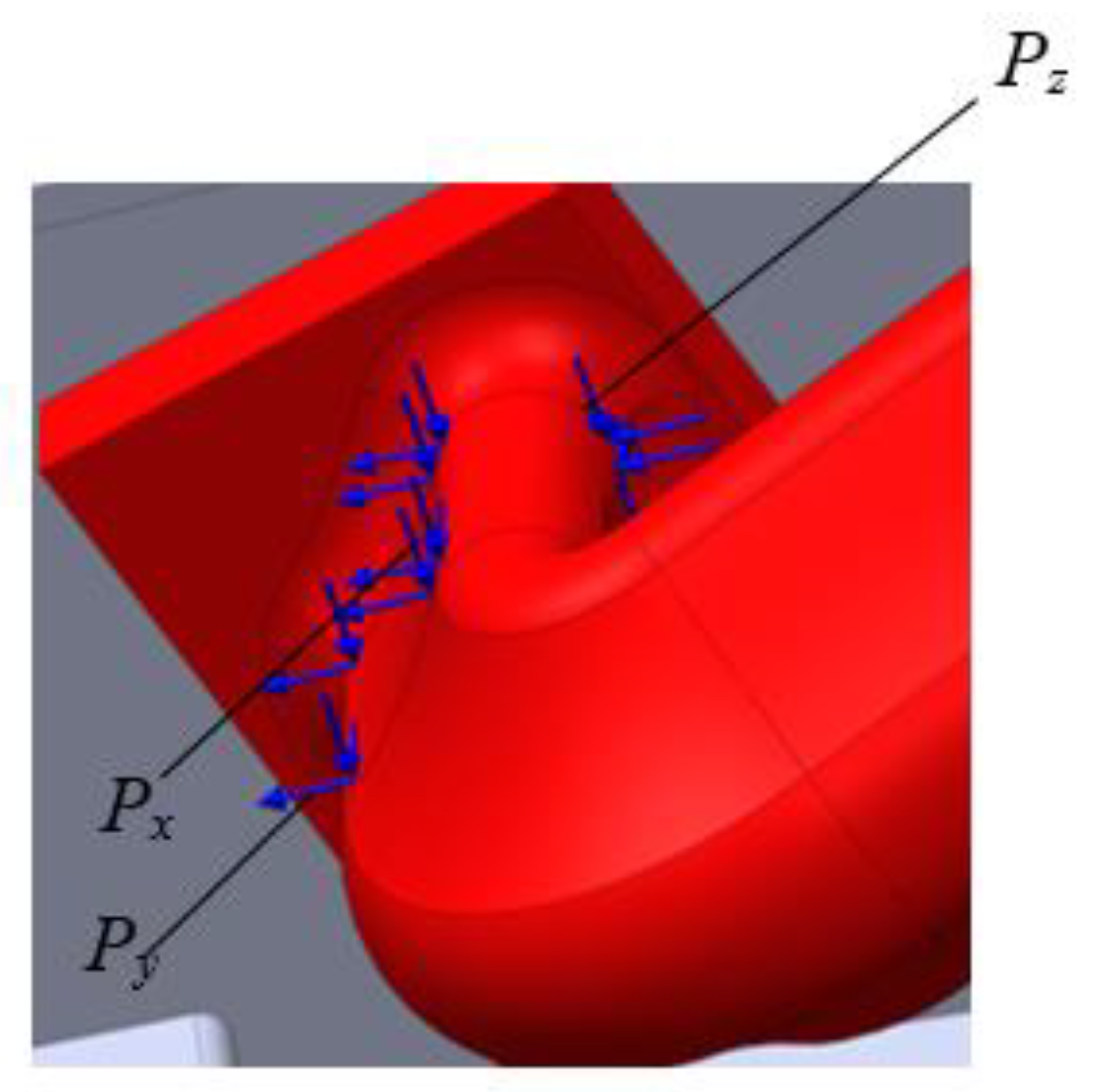
4. The Strength Analysis of the Two-Section Hopper-Wagon-Bearing Structure under the Main Operational Load Modes
5. Determination of the Vertical Load of a Two-Section Hopper Wagon
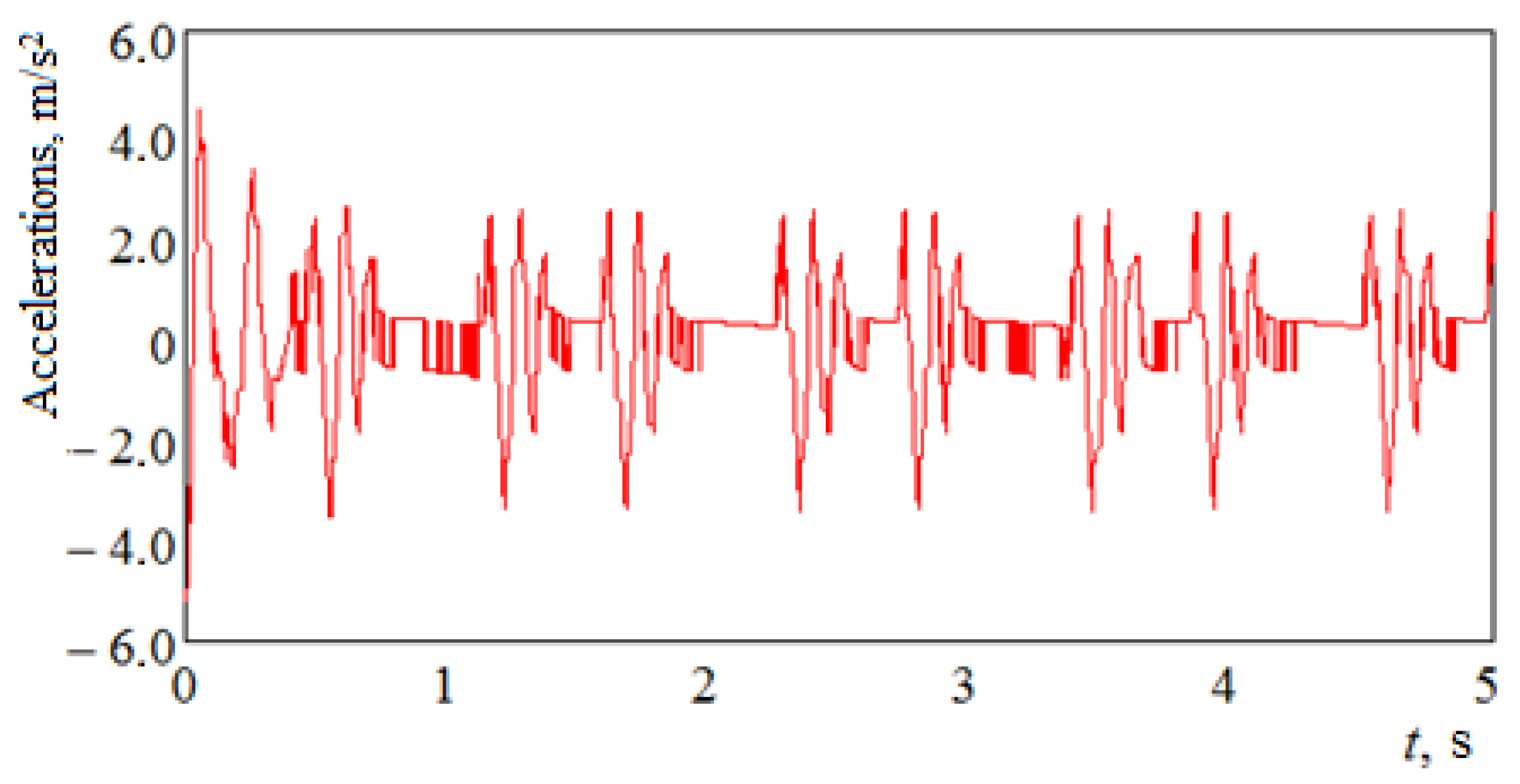
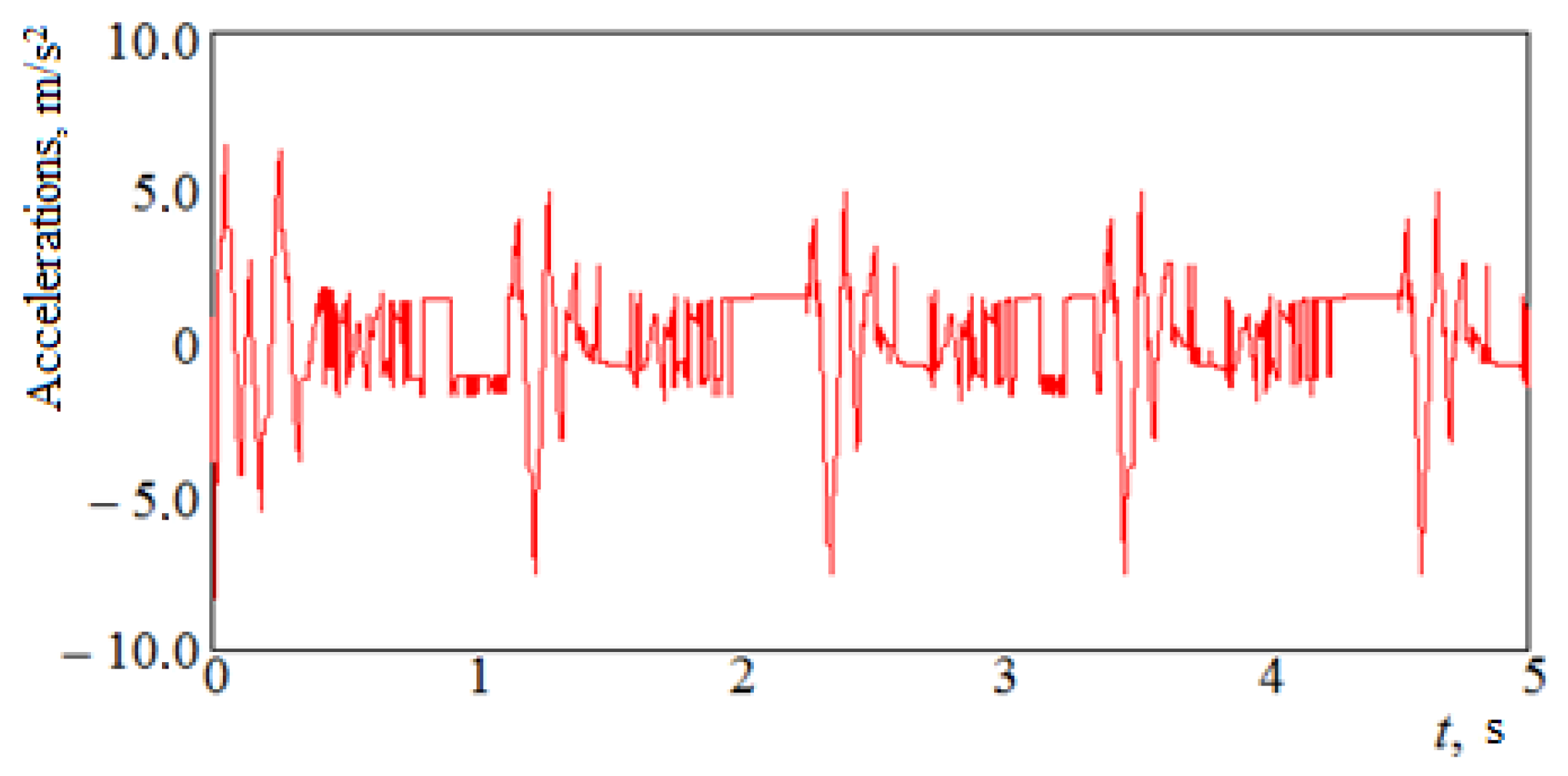
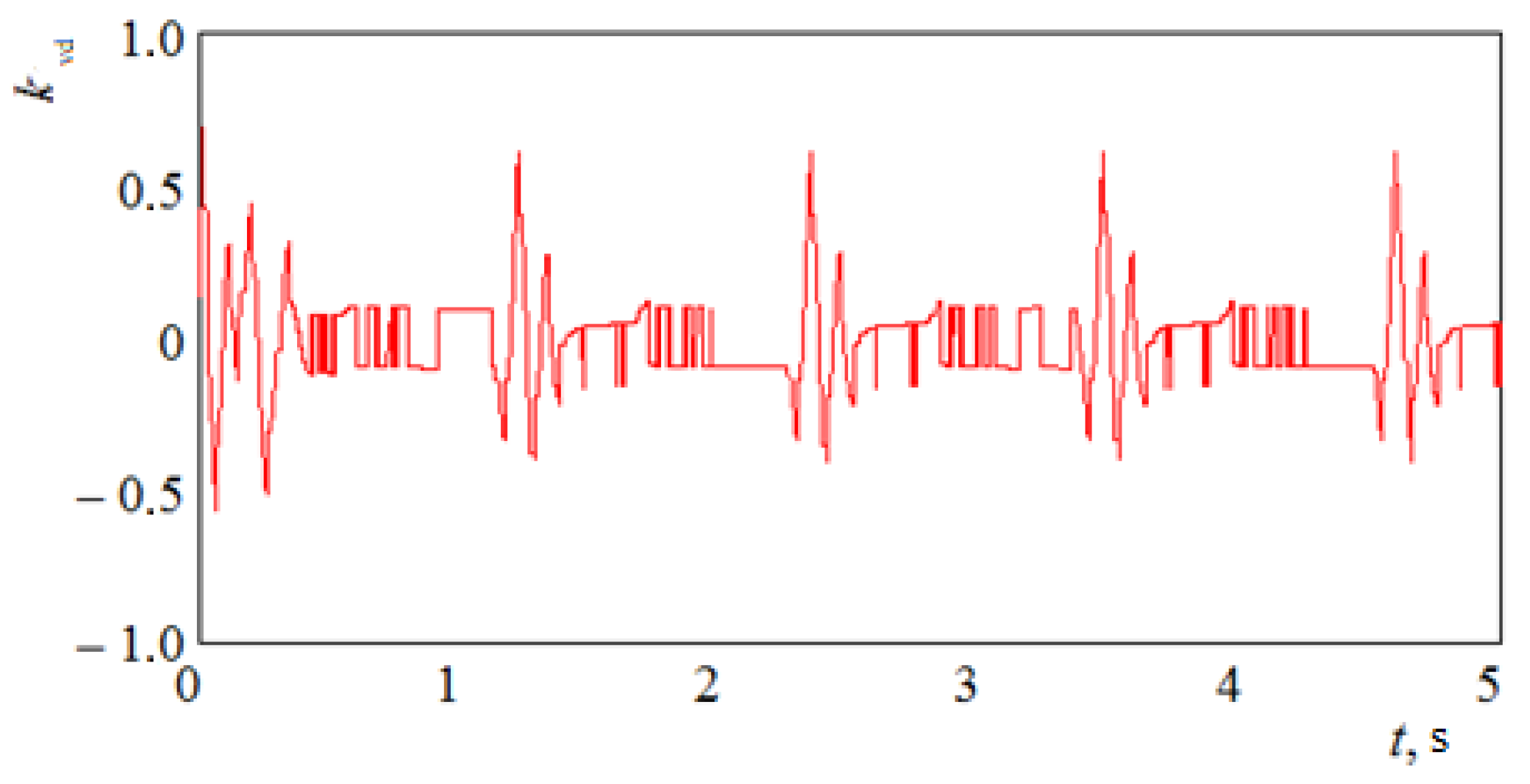
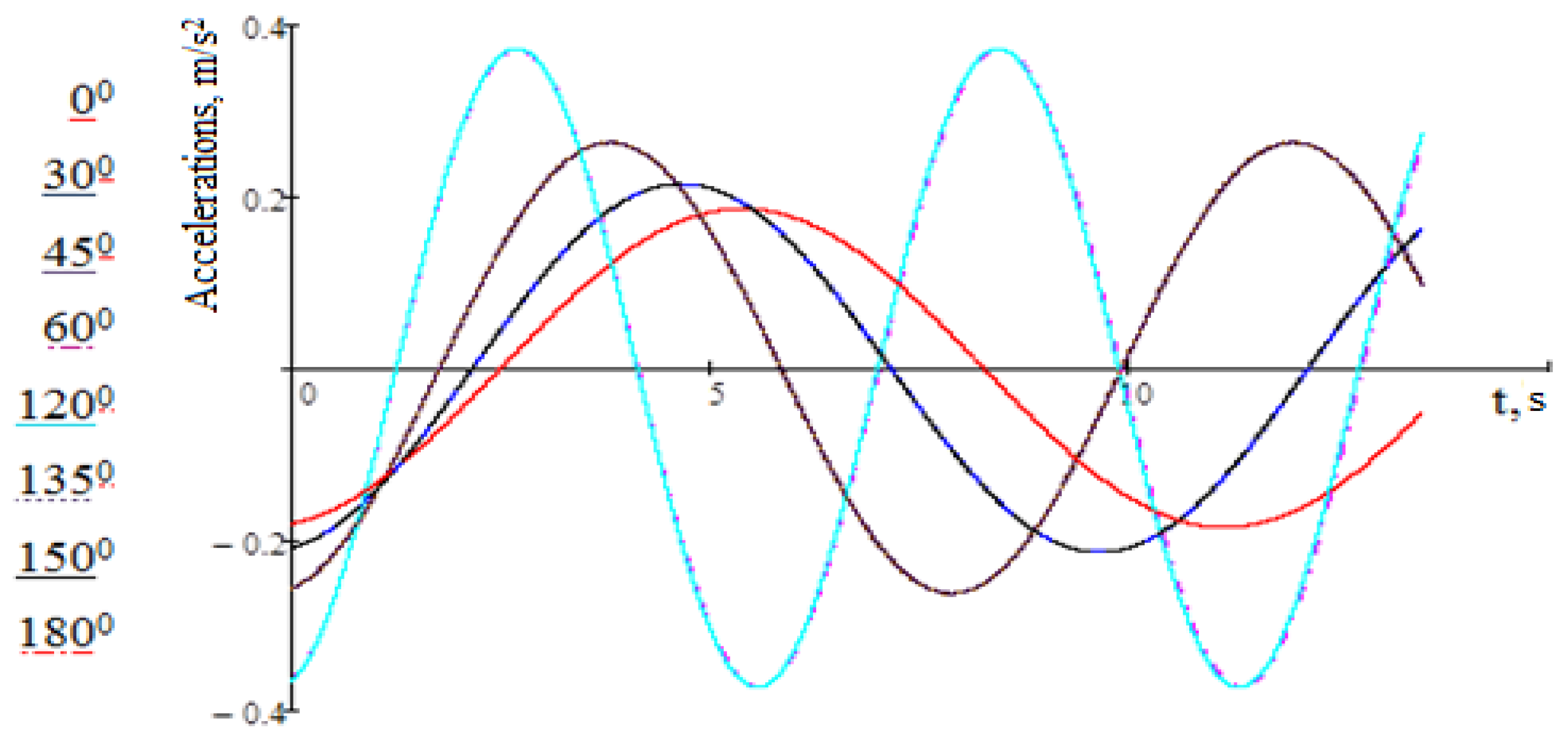
6. Study of the Two-Section Hopper Wagon Load during Transportation by Railway Ferry
7. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Gubarevych, O.; Goolak, S.; Daki, O.; Tryshyn, V. Investigation of Turn-to-Turn Closures of Stator Windings to Improve the Diagnostics System for Induction Motors. Probl. Energeticii Reg. 2021, 2, 10–24. [Google Scholar] [CrossRef]
- Goolak, S.; Tkachenko, V.; Sapronova, S.; Lukoševičius, V.; Keršys, R.; Makaras, R.; Liubarskyi, B. Synthesis of the Current Controller of the Vector Control System for Asynchronous Traction Drive of Electric Locomotives. Energies 2022, 15, 2374. [Google Scholar] [CrossRef]
- Goolak, S.; Tkachenko, V.; Šťastniak, P.; Sapronova, S.; Liubarskyi, B. Analysis of Control Methods for the Traction Drive of an Alternating Current Electric Locomotive. Symmetry 2022, 14, 150. [Google Scholar] [CrossRef]
- Senko, V.I.; Pigunov, A.V.; Afanasiyev, P.M.; Shestakov, C.B. Improving the body structure of the hopper car for the transportation of cement. J. Bull. P.O. Sukhoi Gomel State Tech. Univ. 2017, 2, 3–10. [Google Scholar]
- Afanasiyev, E.V.; Bityutskiy, N.A.; Tsyganskaya, L.V.; Ispolova, E.A.; Filippova, I.O. Fleet of hopper cars renewal designed to transport carbon. Sci. Peer-Rev. J. Proc. Petersburg Transp. Univ. 2019, 1, 37–50. [Google Scholar]
- Tsunami Gate Wagon to Make a Splash at Railway Interchange. Railway Gazette, 11 September 2019.
- Yuan, Y.Q.; Li, Q.; Ran, K. Analysis of C80B Wagons Load-Stress Transfer Relation. Appl. Mech. Mater. 2012, 148–149, 331–335. [Google Scholar] [CrossRef]
- Yoon, S.C.; Kim, G.K.; Jeon, C.S.; Choe, K.Y. Evaluation of Structural Strength in Body Structure of Freight Car. Key Eng. Mater. 2010, 417–418, 181–184. [Google Scholar] [CrossRef]
- Pɫaczek, M.; Wróbel, A.; Buchacz, A. A concept of technology for freight wagons modernization. IOP Conf. Ser. Mater. Sci. Eng. 2016, 161, 012107. [Google Scholar] [CrossRef]
- Fomin, O.; Lovska, A.; Skliarenko, I.; Klochkov, Y. Substantiating the optimization of the loadbearing structure of a hopper car for transporting pellets and hot agglomerate. East.-Eur. J. Enterp. Technol. 2020, 1, 65–74. [Google Scholar]
- Fomin, O.; Vatulia, G.; Lovska, A. Formation of flash-concept for a resource-saving articulated hopper car to transport hot pellets and agglomerate. E3S Web Conf. 2020, 166, 07002. [Google Scholar] [CrossRef]
- Ushakov, A.E.; Safonov, A.A.; Sergeichev, I.V.; Fedulov, B.N.; Kornienko, E.I.; Timofeev, M.A.; Izotov, A.V.; Klenin, Y.G.; Rozin, N.V. Design and optimization of a vacuum infusion technological process for hopper car fabrication using polymeric composite materials. J. Mach. Manuf. Reliab. 2015, 44, 276–282. [Google Scholar] [CrossRef]
- Al-Sukhon, A.; ElSayed, M.S.A. Design optimization of hopper cars employing functionally graded honeycomb sandwich panels. Proc. Inst. Mech. Eng. Part F J. Rail Rapid Transit 2021, 236, 920–935. [Google Scholar] [CrossRef]
- Lee, W.G.; Kim, J.S.; Sun, S.J.; Lim, J.-Y. The next generation material for lightweight railway car body structures: Magnesium alloys. Proc. Inst. Mech. Eng. Part F J. Rail Rapid Transit 2016, 232, 25–42. [Google Scholar] [CrossRef]
- Lee, H.A.; Jung, S.B.; Jang, H.H.; Shin, D.-H.; Lee, J.U.; Kim, K.W.; Park, G.-J. Structural-optimization-based design process for the body of a railway vehicle made from extruded aluminum panels. Proc. Inst. Mech. Eng. Part F J. Rail Rapid Transit 2015, 230, 1283–1296. [Google Scholar] [CrossRef]
- Wennberg, D.; Stichel, S.; Wennhage, P. Substitution of corrugated sheets in a railway vehicle’s body structure by a multiple-requirement based selection process. Proc. Inst. Mech. Eng. Part F J. Rail Rapid Transit 2012, 228, 143–157. [Google Scholar] [CrossRef]
- Molavitabrizi, D.; Laliberte, J. Methodology for multiscale design and optimization of lattice core sandwich structures for lightweight hopper railcars. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2020, 234, 4224–4238. [Google Scholar] [CrossRef]
- Fomin, O.; Lovska, A. Determination of dynamic loading of bearing structures of freight wagons with actual dimensions. East.-Eur. J. Enterp. Technol. 2021, 2, 6–15. [Google Scholar] [CrossRef]
- Vatulia, G.; Lovska, A.; Pavliuchenkov, M.; Nerubatskyi, V.; Okorokov, A.; Hordiienko, D.; Vernigora, R.; Zhuravel, I. Determining patterns of vertical load on the prototype of a removable module for long-size cargoes. East.-Eur. J. Enterp. Technol. 2022, 6, 21–29. [Google Scholar] [CrossRef]
- Antipin, D.Y.; Racin, D.Y.; Shorokhov, S.G. Justification of a Rational Design of the Pivot Center of the Open-top Wagon Frame by means of Computer Simulation. Procedia Eng. 2016, 150, 150–154. [Google Scholar] [CrossRef]
- Prakash, S.C.; Bharti, P.K. Study and Analysis of Doors of BCNHL Wagons. Int. J. Eng. Res. Technol. IJERT 2015, 4, 1195–1200. [Google Scholar]
- Patrascu, A.I.; Hadar, A.; Pastrama, S.D. Structural Analysis of a Freight Wagon with Composite Walls. Mater. Plast. 2019, 57, 140–151. [Google Scholar] [CrossRef]
- Street, G.E.; Mistry, P.J. Michael Sylvester Johnson. Impact Resistance of Fibre Reinforced Composite Railway Freight Tank Wagons. J. Compos. Sci. 2021, 5, 152. [Google Scholar] [CrossRef]
- Kosobudzki, M.; Jamroziak, K.; Bocian, M.; Kotowski, P.; Zając, P. The analysis of structure of the repaired freight wagon. AIP Conf. Proc. 2018, 2029, 020030. [Google Scholar] [CrossRef]
- Płaczek, M.; Wróbel, A.; Olesiejuk, M. Modelling and arrangement of composite panels in modernized freight cars. MATEC Web Conf. 2017, 112, 06022. [Google Scholar] [CrossRef]
- Fomin, O.; Lovska, A.; Kulbovskyi, I.; Holub, H.; Kozarchuk, I.; Kharuta, V. Determining the dynamic loading on a semi-wagon when fixing it with a viscous coupling to a ferry deck. East.-Eur. J. Enterp. Technol. 2019, 2, 6–12. [Google Scholar] [CrossRef]
- Fomin, O.; Gorbunov, M.; Gerlici, J.; Lovska, A.; Kravchenko, K. Dynamics and strength of circular tube open wagons with aluminum foam filled center sills. Materials 2021, 14, 1915. [Google Scholar] [CrossRef] [PubMed]
- Alyamovskij, A.A. SolidWorks/COSMOSWorks 2006–2007. Engineering Finite Element Analysis; Series “Design”; DMK: Moscow, Russia, 2007; 784p. [Google Scholar]
- Alyamovskij, A.A. COSMOSWorks. Fundamentals of Structural Analysis in SolidWorks; Series “Design”; DMK: Moscow, Russia, 2010; 784p. [Google Scholar]
- Vatulia, G.L.; Petrenko, D.H.; Novikova, M.A. Experimental estimation of load-carrying capacity of circular, square and rectangular CFTS columns. J. Nauk. Visnyk Natsionalnoho Hirnychoho Univ. 2017, 6, 97–102. [Google Scholar]
- DSTU 7598:2014; Freight Wagons. General Requirements for Calculations and Design of New and Modernized Wagons of 1520 mm Track (Non-Self-Propelled). UkrNDNTS: Kiev, Ukraine, 2015; 162p.
- Lukin, V.V.; Shadur, L.A.; Koturanov, V.I.; Hohlov, A.A.; Anisimov, P.S. Design and Calculation of Wagons; UMK MPS: Moskov, Russia, 2000; 731p. [Google Scholar]
- Domin, Y.V.; Chernyak, G.Y. Basics of Wagon Dynamics; Kyiv University of Economics and Transport Technology: Kyiv, Ukraine, 2003; 269p. [Google Scholar]
- Fomin, O.; Gerlici, J.; Gorbunov, M.; Vatulia, G.; Lovska, A.; Kravchenko, K. Research into the Strength of an OpenWagon with Double Sidewalls Filled with Aluminium Foam. Materials 2021, 14, 3420. [Google Scholar] [CrossRef]
- Krol, O.; Porkuian, O.; Sokolov, V.; Tsankov, P. Vibration stability of spindle nodes in the zone of tool equipment optimal parameters. Comptes Rendus De L’acade’mie Bulg. Des Sci. 2019, 72, 1546–1556. [Google Scholar] [CrossRef]
- Nalapko, O.; Shyshatskyi, A.; Ostapchuk, V.; Mahdi, Q.A.; Zhyvotovskyi, R.; Petruk, S.; Lebed, Y.; Diachenko, S.; Velychko, V.; Poliak, I. Development of a method of adaptive control of military radio network parameters. East.-Eur. J. Enterp. Technol. 2021, 1, 18–32. [Google Scholar] [CrossRef]
- Panchenko, S.; Vatulia, G.; Lovska, A.; Ravlyuk, V.; Elyazov, I.; Huseynov, I. Influence of structural solutions of an improved brake cylinder of a freight car of railway transport on its load in operation. EUREKA Phys. Eng. 2022, 6, 45–55. [Google Scholar] [CrossRef]
- Lovskaya, A. Assessment of dynamic efforts to bodies of wagons at transportation with railway ferries. East.-Eur. J. Enterp. Technol. 2014, 3, 36–41. [Google Scholar] [CrossRef]
- Lovska, A.; Fomin, O.; Pistek, V.; Kucera, P. Dynamic load and strength determination of carrying structure of wagons transported by ferries. J. Mar. Sci. Eng. 2020, 8, 902. [Google Scholar] [CrossRef]
- Kiryanov, D.V. Mathcad 13; BHV-Petersburg: St. Petersburg, Russia, 2006; 608p. [Google Scholar]
- Dyakonov, V. MATHCAD 8/2000: A Special Guide; Piter: St. Petersburg, Russia, 2000; 592p. [Google Scholar]
- Fomin, O.; Gerlici, J.; Vatulia, G.; Lovska, A.; Kravchenko, K. Determination of the Loading of a Flat Rack Container during Operating Modes. Appl. Sci. 2021, 11, 7623. [Google Scholar] [CrossRef]
- Krol, O.; Sokolov, V. Modeling of Spindle Node Dynamics Using the Spectral Analysis Method. Lect. Notes Mech. Eng. 2020, 1, 35–44. [Google Scholar] [CrossRef]
- Panchenko, S.; Gerlici, J.; Vatulia, G.; Lovska, A.; Pavliuchenkov, M.; Kravchenko, K. The Analysis of the Loading and the Strength of the FLAT RACK Removable Module with Viscoelastic Bonds in the Fittings. Appl. Sci. 2023, 13, 79. [Google Scholar] [CrossRef]
- Reference Data on the Regime of Wind and Waves of the Baltic, Northern, Black, Azov and Mediterranean Seas. Russian Maritime Register of Shipping. Available online: https://files.stroyinf.ru/Data2/1/4293747/4293747775.pdf (accessed on 28 July 2021).












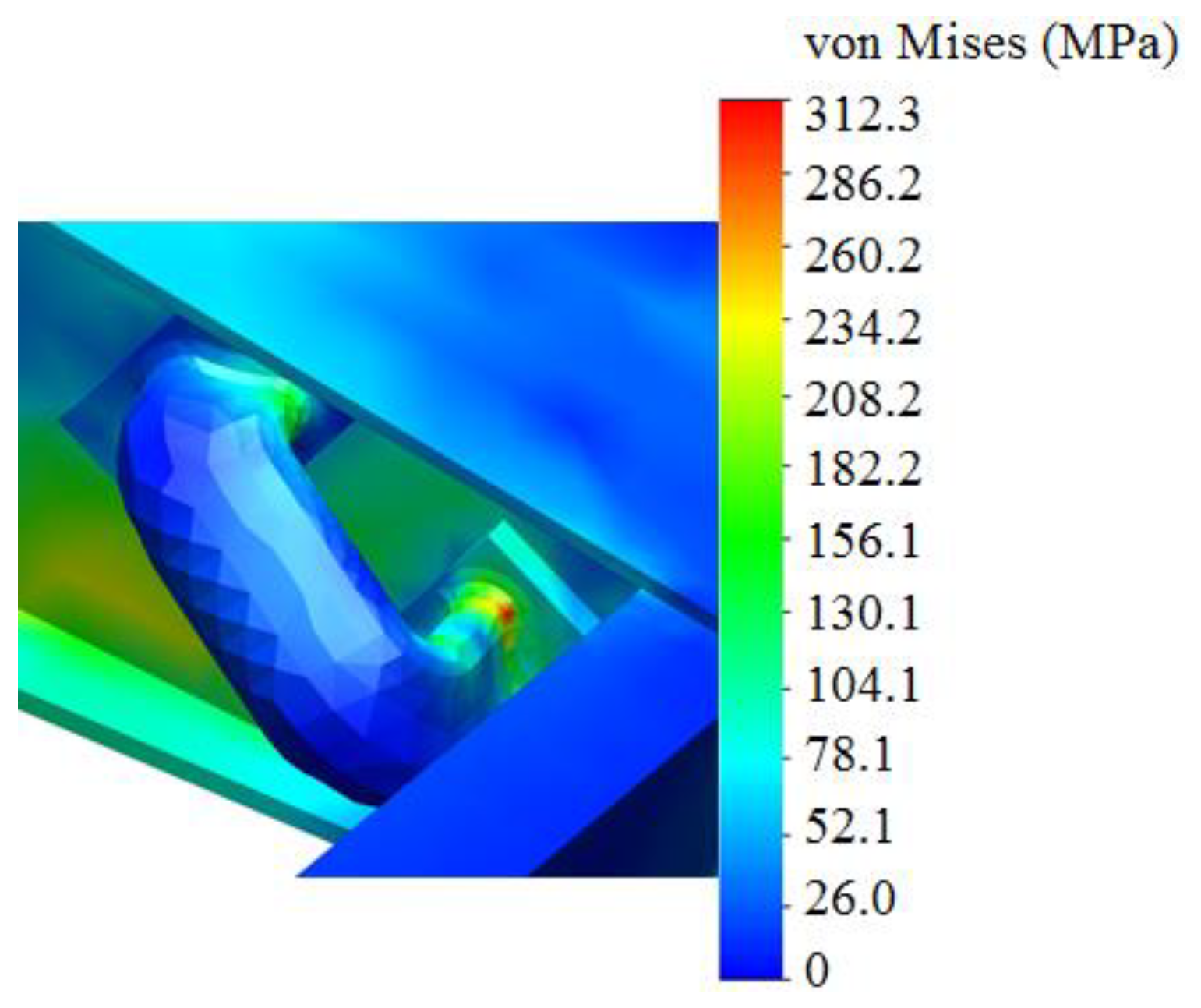
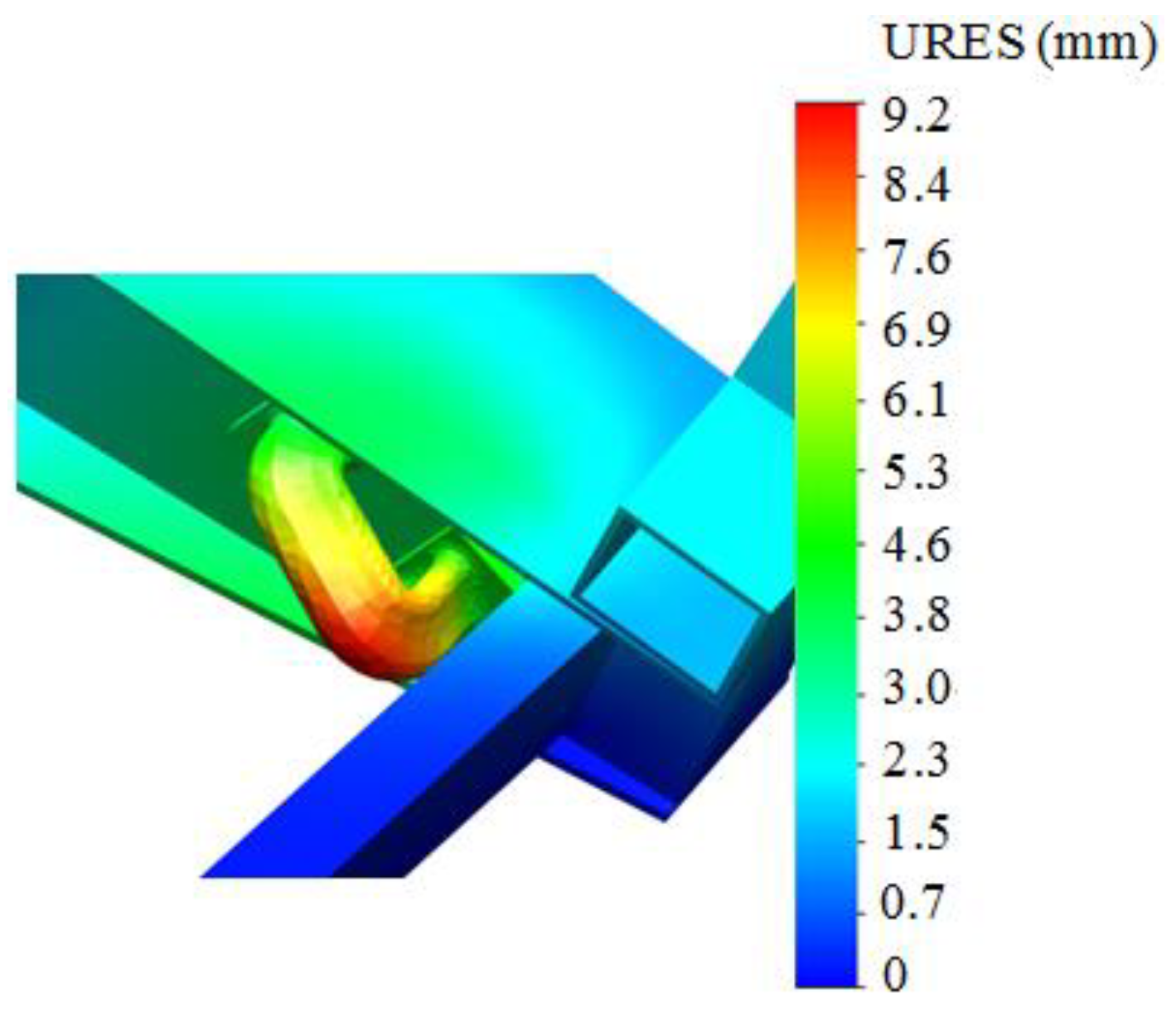
| Strength Index | Load Mode | ||||
|---|---|---|---|---|---|
| I | III | ||||
| Impact | Squash | Jerk–Stretch | Impact–Squash | Jerk–Stretch | |
| Stress, MPa | 240.7 | 193.1 | 186.1 | 204.5 | 176.1 |
| Displacement in the nodes, mm | 2.4 | 2.3 | 2.3 | 2.4 | 2.3 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Vatulia, G.; Gerlici, J.; Fomin, O.; Lovska, A.; Fomina, Y.; Kravchenko, K. Analysis of the Strength of the Supporting Structure of a TwoSection Hopper Wagon under Operating Loading Conditions. Appl. Sci. 2023, 13, 859. https://doi.org/10.3390/app13020859
Vatulia G, Gerlici J, Fomin O, Lovska A, Fomina Y, Kravchenko K. Analysis of the Strength of the Supporting Structure of a TwoSection Hopper Wagon under Operating Loading Conditions. Applied Sciences. 2023; 13(2):859. https://doi.org/10.3390/app13020859
Chicago/Turabian StyleVatulia, Glib, Juraj Gerlici, Oleksij Fomin, Alyona Lovska, Yuliia Fomina, and Kateryna Kravchenko. 2023. "Analysis of the Strength of the Supporting Structure of a TwoSection Hopper Wagon under Operating Loading Conditions" Applied Sciences 13, no. 2: 859. https://doi.org/10.3390/app13020859
APA StyleVatulia, G., Gerlici, J., Fomin, O., Lovska, A., Fomina, Y., & Kravchenko, K. (2023). Analysis of the Strength of the Supporting Structure of a TwoSection Hopper Wagon under Operating Loading Conditions. Applied Sciences, 13(2), 859. https://doi.org/10.3390/app13020859








