Modelling of Cantilever-Based Flow Energy Harvesters Featuring C-Shaped Vibration Inducers: The Role of the Fluid/Beam Interaction
Abstract
1. Introduction
2. Methods
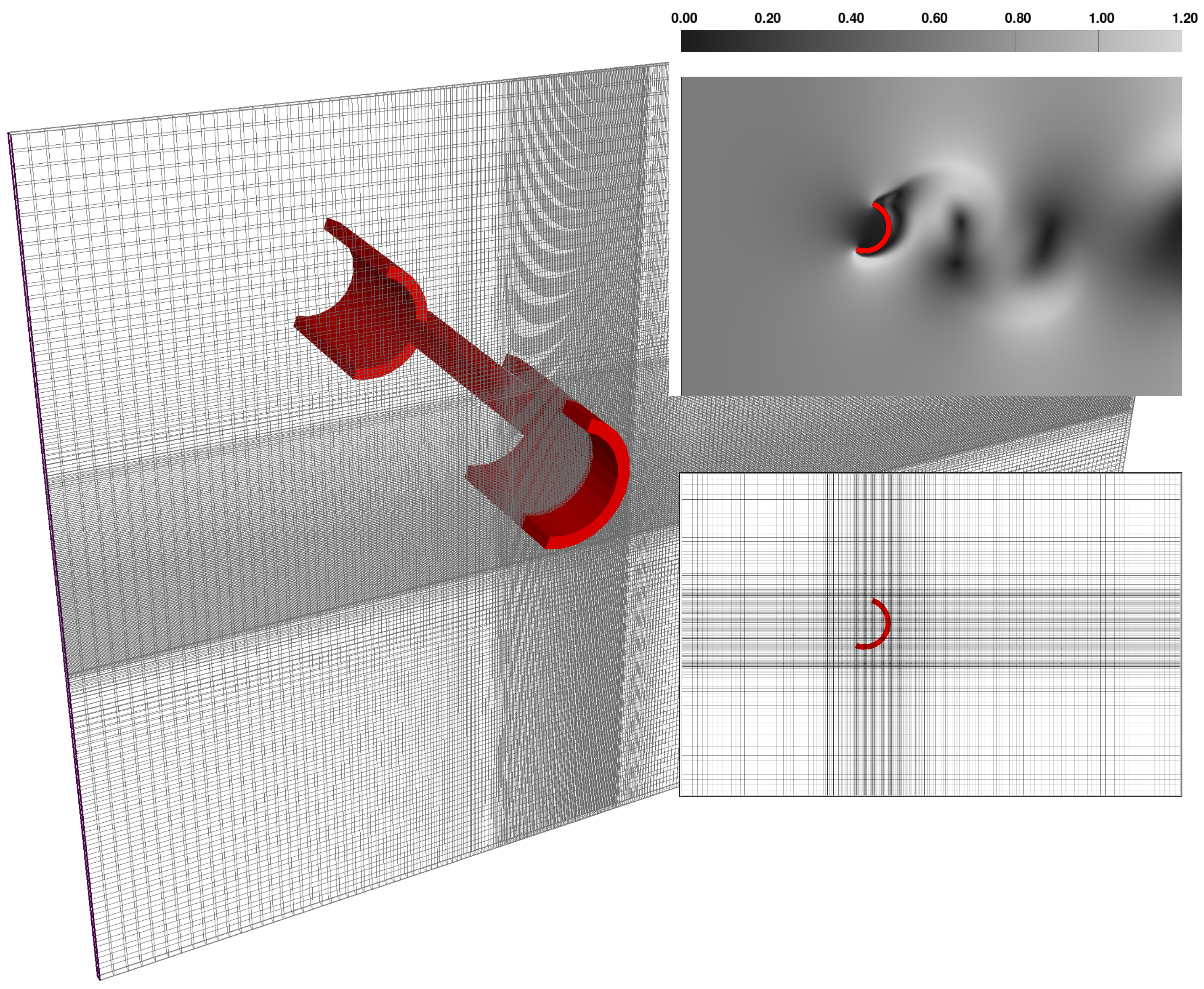
2.1. CFD Modelling of the FSI for the VI
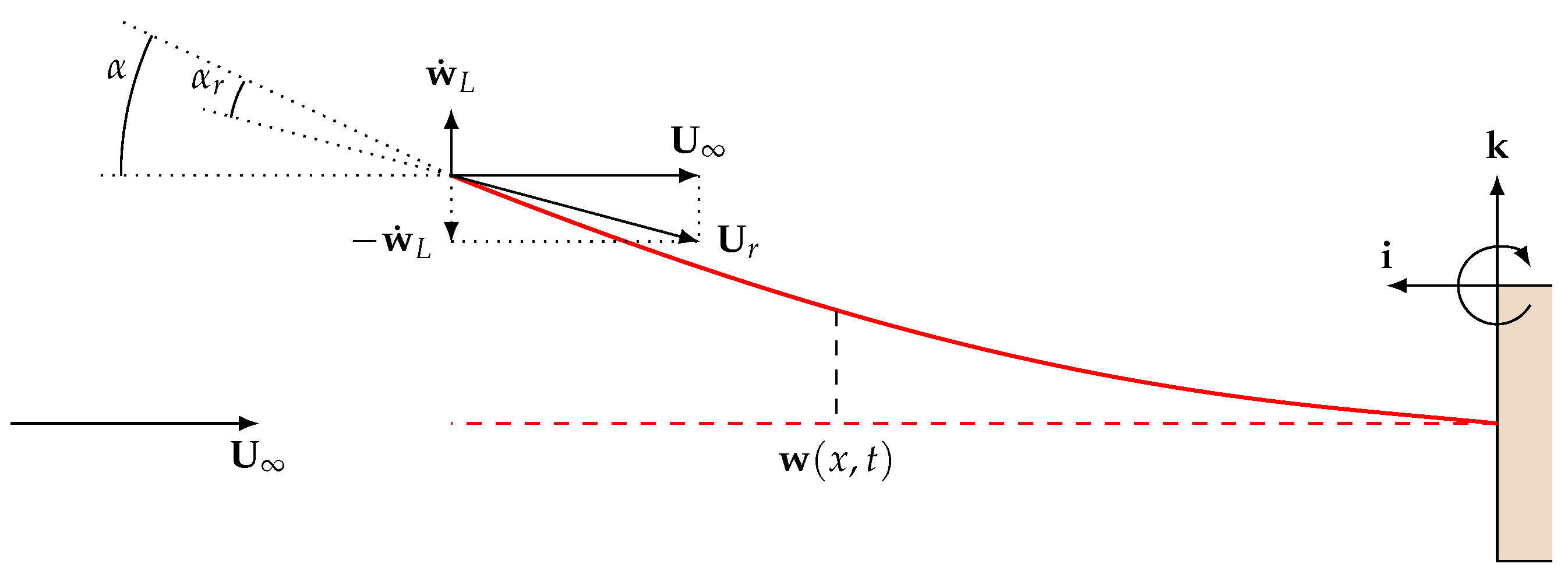
2.2. The Beam Model: Modified Euler-Bernoulli Equation
2.3. The Quasi Steady Forcing
2.4. The Experimental Setup
2.5. Finite Dimensional Approach and Galerkin Expansion
3. Results
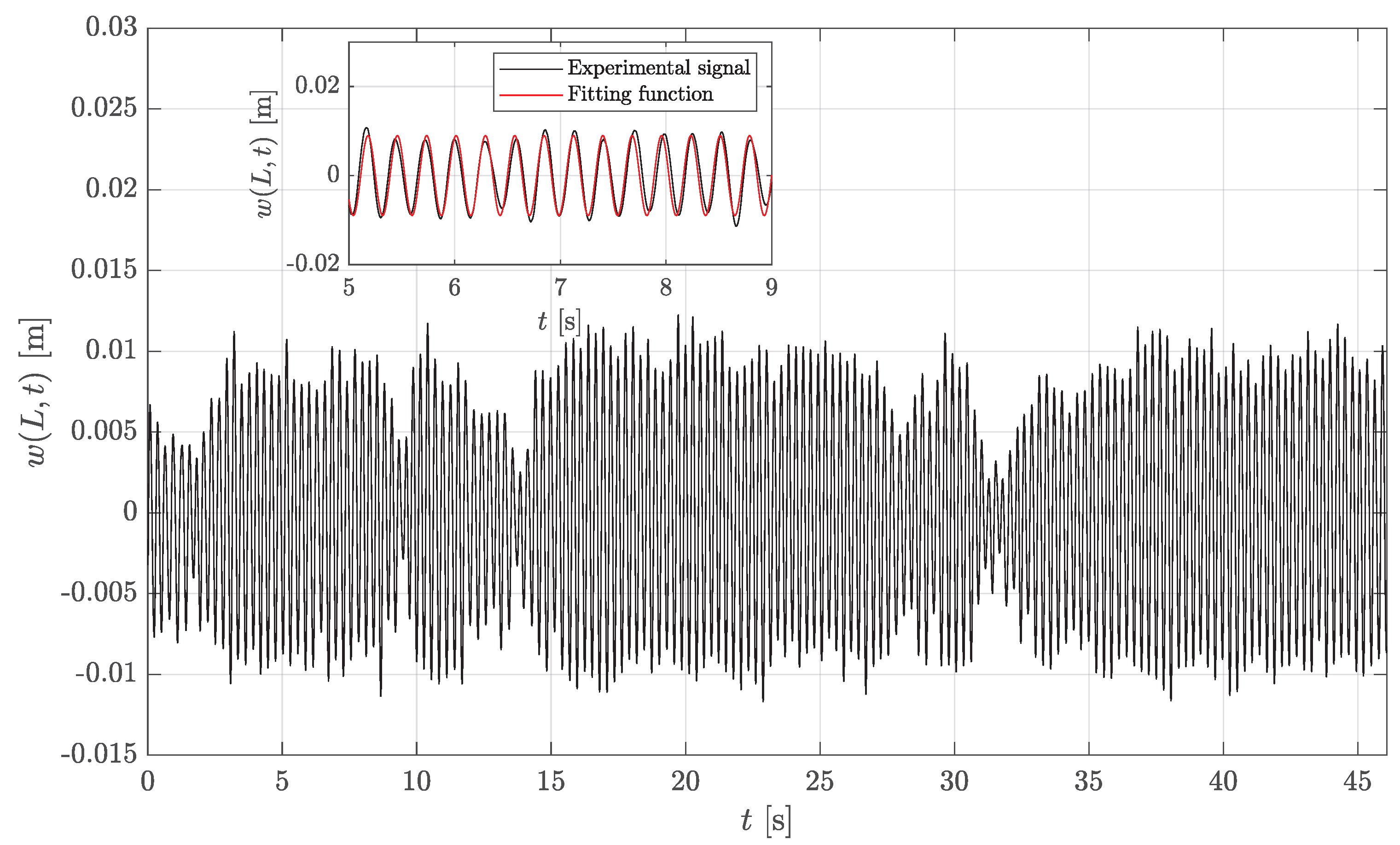
3.1. Model Calibration against Experimental Results
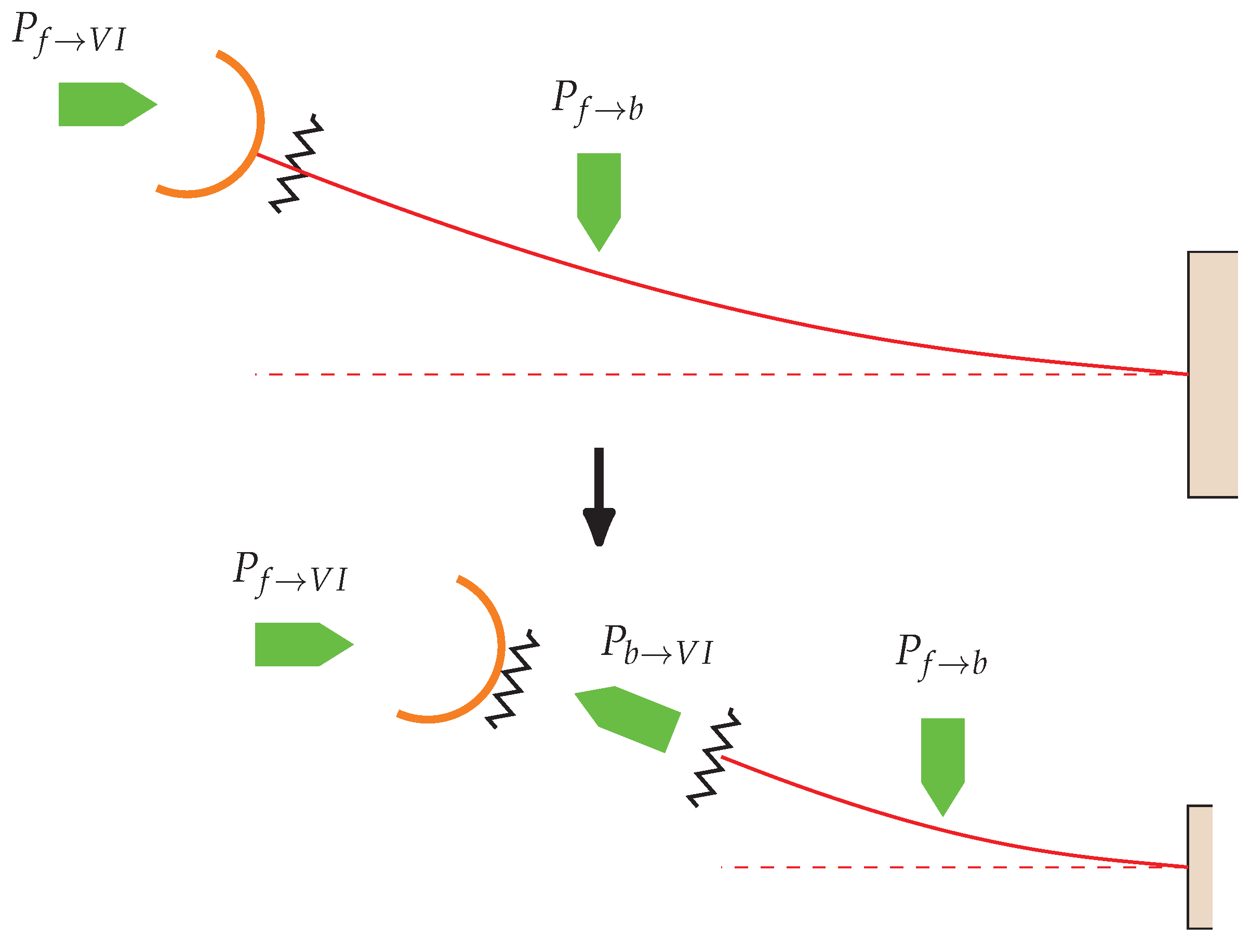
3.2. Evaluation of the Work Done by Fluid Forces on the VI
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| FEH | Flow Energy Harvester |
| VI | Vibration Inducer |
| AoA | Angle of Attack |
| VIV | Vortex-Induced Vibration |
| CFD | Computational Fluid Dynamics |
| EB | Euler-Bernoulli |
Appendix A. Equivalent Beam Model
- The first natural angular frequency of the equivalent beam must match , namely:where and b the width of the beam’s section;
- The tip deflection must match the measured one, namely:
| Steel | Equivalent | Units |
|---|---|---|
| kg/m3 | ||
| GPa | ||
| mm |
References
- Lu, F.; Lee, H.P.; Lim, S.P. Modeling and analysis of micro piezoelectric power generators for micro-electromechanical-systems applications. Smart Mater. Struct. 2003, 13, 57. [Google Scholar] [CrossRef]
- Cook-Chennault, K.A.; Thambi, N.; Sastry, A.M. Powering MEMS portable devices—A review of non-regenerative and regenerative power supply systems with special emphasis on piezoelectric energy harvesting systems. Smart Mater. Struct. 2008, 17, 043001. [Google Scholar] [CrossRef]
- St Clair, D.; Bibo, A.; Sennakesavababu, V.R.; Daqaq, M.F.; Li, G. A scalable concept for micropower generation using flow-induced self-excited oscillations. Appl. Phys. Lett. 2010, 96, 144103. [Google Scholar] [CrossRef]
- Huang, G.; Xia, Y.; Dai, Y.; Yang, C.; Wu, Y. Fluid–structure interaction in piezoelectric energy harvesting of a membrane wing. Phys. Fluids 2021, 33, 063610. [Google Scholar] [CrossRef]
- Alam, M.M.; Chao, L.-M.; Rehamn, S.; Ji, C.; Wang, H. Energy harvesting from passive oscillation of inverted foil. Phys. Fluids 2021, 33, 075111. [Google Scholar] [CrossRef]
- Pobering, S.; Schwesinger, N. A novel hydropower harvesting device. In Proceedings of the International Conference on MEMS, NANO and Smart Systems (ICMENS 04), Banff, AB, Canada, 25–27 August 2004; pp. 480–485. [Google Scholar]
- Kouritem, S.A.; Bani-Hani, M.A.; Beshir, M.; Elshabasy, M.M.Y.B.; Altabey, W.A. Automatic Resonance Tuning Technique for an Ultra-Broadband Piezoelectric Energy Harvester. Energies 2022, 15, 7271. [Google Scholar] [CrossRef]
- Renno, J.M.; Daqaq, M.F.; Inman, D.J. On the optimal energy harvesting from a vibration source. J. Sound Vib. 2009, 320, 386–405. [Google Scholar] [CrossRef]
- Ma, X.; Zhou, S. A review of flow-induced vibration energy harvesters. Energy Convers. Manag. 2022, 254, 115223. [Google Scholar] [CrossRef]
- Akaydin, H.D.; Elvin, N.; Andreopoulos, Y. Energy harvesting from highly unsteady fluid flows using piezoelectric materials. J. Intell. Mater. Syst. Struct. 2010, 21, 1263–1278. [Google Scholar] [CrossRef]
- Tang, D.M.; Yamamoto, H.; Dowell, E.H. Flutter and limit cycle oscillations of two-dimensional panels in three-dimensional axial flow. J. Fluids Struct. 2003, 17, 225–242. [Google Scholar] [CrossRef]
- Hassan, M.M.; Hossain, M.Y.; Mazumder, R.; Rahman, R.; Rahman, M.A. Vibration energy harvesting in a small channel fluid flow using piezoelectric transducer. AIP Conf. Proc. 2016, 1754, 050041. [Google Scholar]
- Akaydin, H.D.; Elvin, N.; Andreopoulos, Y. The performance of a self-excited fluidic energy harvester. J. Intell. Mater. Syst. Struct. 2012, 21, 025007. [Google Scholar] [CrossRef]
- Seyed-Aghazadeh, B.; Samandari, H.; Dulac, S. Flow-induced vibration of inherently nonlinear structures with applications in energy harvesting. Phys. Fluids 2020, 32, 071701. [Google Scholar] [CrossRef]
- Hamlehdar, M.; Kasaeian, A.; Safaei, M.R. Energy harvesting from fluid flow using piezoelectrics: A critical review. Renew. Energy 2019, 143, 1826–1838. [Google Scholar] [CrossRef]
- Naqvi, A.; Ali, A.; Altabey, W.A.; Kouritem, S.A. Energy Harvesting from Fluid Flow Using Piezoelectric Materials: A Review. Energies 2022, 15, 7424. [Google Scholar] [CrossRef]
- Forouzi Feshalami, B.; He, S.; Scarano, F.; Gan, L.; Morton, C. A review of experiments on stationary bluff body wakes. Phys. Fluids 2022, 34, 011301. [Google Scholar] [CrossRef]
- Jo, S.; Sun, W.; Son, C.; Seok, J. Galloping-based energy harvester considering enclosure effect. AIP Adv. 2018, 8, 095309. [Google Scholar] [CrossRef]
- Bibo, A.; Abdelkefi, A.; Daqaq, M.F. Modeling and characterization of a piezoelectric energy harvester under combined aerodynamic and base excitations. J. Vib. Acoust. 2015, 137, 031017. [Google Scholar] [CrossRef]
- Yang, Y.; Zhao, L.; Tang, L. Comparative study of tip cross-sections for efficient galloping energy harvesting. Appl. Phys. Lett. 2013, 102, 064105. [Google Scholar] [CrossRef]
- Elvin, N.; Erturk, A. Advances in Energy Harvesting Methods; Springer: New York, NY, USA, 2013. [Google Scholar]
- Wang, J.; Zhou, S.; Zhang, Z.; Yurchenko, D. High-performance piezoelectric wind energy harvester with Y-shaped attachments. Energy Convers. Manag. 2019, 181, 645–652. [Google Scholar] [CrossRef]
- Parkinson, G.V.; Brooks, N.P.H. On the aeroelastic instability of bluff cylinders. J. Appl. Mech. 1961, 28, 252–258. [Google Scholar] [CrossRef]
- Parkinson, G.V.; Smith, J.D. The square prism as an aeroelastic non-linear oscillator. Q. J. Mech. Appl. Math. 1964, 17, 225–239. [Google Scholar] [CrossRef]
- Song, J.; Hu, G.; Tse, K.T.; Li, S.W.; Kwok, K.C.S. Performance of a circular cylinder piezoelectric wind energy harvester fitted with a splitter plate. Appl. Phys. Lett. 2017, 111, 223903. [Google Scholar] [CrossRef]
- Curatolo, M.; La Rosa, M.; Prestininzi, P. Energy harvesting in a fluid flow using piezoelectric material. In Proceedings of the 2018 COMSOL Conference, Lausanne, Switzerland, 22–24 October 2018. [Google Scholar]
- Curatolo, M.; Lombardi, V.; Prestininzi, P. Enhancing flow induced vibrations of a thin piezoelectric cantilever: Experimental analysis. In Proceedings of the International Conference on Fluvial Hydraulics River Flow 2020, Delft, The Netherlands, 6–17 July 2020. [Google Scholar]
- Lombardi, V.; La Rocca, M.; Prestininzi, P. A new dynamic masking technique for time resolved PIV analysis. J. Vis. 2021, 24, 979–990. [Google Scholar] [CrossRef]
- Tucker Harvey, S.; Khovanov, I.A.; Denissenko, P. A galloping energy harvester with flow attachment. Appl. Phys. Lett. 2019, 114, 104103. [Google Scholar] [CrossRef]
- Tucker Harvey, S.; Khovanov, I.A.; Murai, Y.; Denissenko, P. Characterisation of aeroelastic harvester efficiency by measuring transient growth of oscillations. Appl. Energy 2020, 268, 115014. [Google Scholar] [CrossRef]
- Shi, T.; Gang, H.; Lianghao, Z. Aerodynamic Shape Optimization of an Arc-Plate-Shaped Bluff Body via Surrogate Modeling for Wind Energy Harvesting. Appl. Sci. 2022, 12, 3965. [Google Scholar] [CrossRef]
- FLOW-3D, Version 12.0; Flow Science, Inc.: Santa Fe, NM, USA, 2019; Available online: https://www.flow3d.com/ (accessed on 1 January 2020).
- Lindsey, W.F. Drag of cylinders of simple shapes. NACA Tech. Rep. 1938, 619, 169–176. [Google Scholar]
- Hoerner, S.F. Fluid Dynamic Drag; Hoerner Fluid Dynamics: Bakersfield, CA, USA, 1965. [Google Scholar]
- Wilcox, D.C. Turbulence Modeling for CFD; 3DCW Industries: La Canada, CA, USA, 1998. [Google Scholar]
- Javed, U.; Abdelkefi, A. Impacts of the aerodynamic force representation on the stability and performance of a galloping-based energy harvester. J. Sound Vib. 2017, 400, 213–226. [Google Scholar] [CrossRef]
- Blevins, R. Flow-Induced Vibration; Van Nostrand Reinhold: New York, NY, USA, 1990. [Google Scholar]
- Barrero-Gil, A.; Alonso, G.; Sanz-Andres, A. Energy harvesting from transverse galloping. J. Sound Vib. 2010, 329, 2873–2883. [Google Scholar] [CrossRef]
- Bibo, A.; Daqaq, M.F. An analytical framework for the design and comparative analysis of galloping energy harvesters under quasi-steady aerodynamics. Smart Mater. Struct. 2015, 24, 094006. [Google Scholar] [CrossRef]
- Curatolo, M.; La Rosa, M.; Prestininzi, P. On the validity of plane state assumptions in the bending of bimorph piezoelectric cantilevers. J. Intell. Mater. Syst. Struct. 2019, 30, 1508–1517. [Google Scholar] [CrossRef]
- Physik Instrumente. Available online: https://www.physikinstrumente.com/en/ (accessed on 14 November 2022).
- Blevins, R. Wave Motion in Elastic Solids; VDover Publications Inc.: New York, NY, USA, 2003. [Google Scholar]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sciortino, G.; Lombardi, V.; Prestininzi, P. Modelling of Cantilever-Based Flow Energy Harvesters Featuring C-Shaped Vibration Inducers: The Role of the Fluid/Beam Interaction. Appl. Sci. 2023, 13, 416. https://doi.org/10.3390/app13010416
Sciortino G, Lombardi V, Prestininzi P. Modelling of Cantilever-Based Flow Energy Harvesters Featuring C-Shaped Vibration Inducers: The Role of the Fluid/Beam Interaction. Applied Sciences. 2023; 13(1):416. https://doi.org/10.3390/app13010416
Chicago/Turabian StyleSciortino, Giampiero, Valentina Lombardi, and Pietro Prestininzi. 2023. "Modelling of Cantilever-Based Flow Energy Harvesters Featuring C-Shaped Vibration Inducers: The Role of the Fluid/Beam Interaction" Applied Sciences 13, no. 1: 416. https://doi.org/10.3390/app13010416
APA StyleSciortino, G., Lombardi, V., & Prestininzi, P. (2023). Modelling of Cantilever-Based Flow Energy Harvesters Featuring C-Shaped Vibration Inducers: The Role of the Fluid/Beam Interaction. Applied Sciences, 13(1), 416. https://doi.org/10.3390/app13010416







