Semi-Active Cable Damping to Compensate for Damping Losses Due to Reduced Cable Motion Close to Cable Anchor
Abstract
:1. Introduction
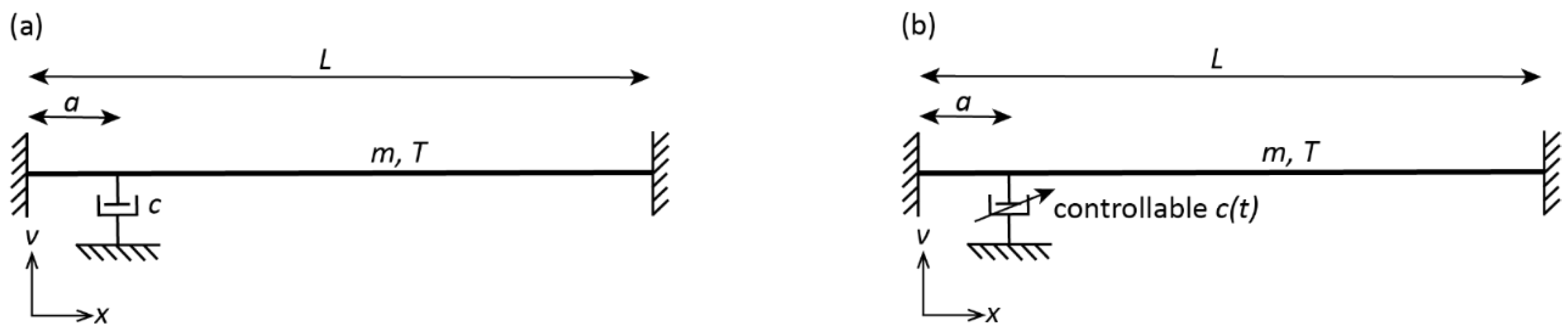
2. Cable with Passive Transverse Damper
2.1. Cable without Bending Rigidity and Simply Supported Ends
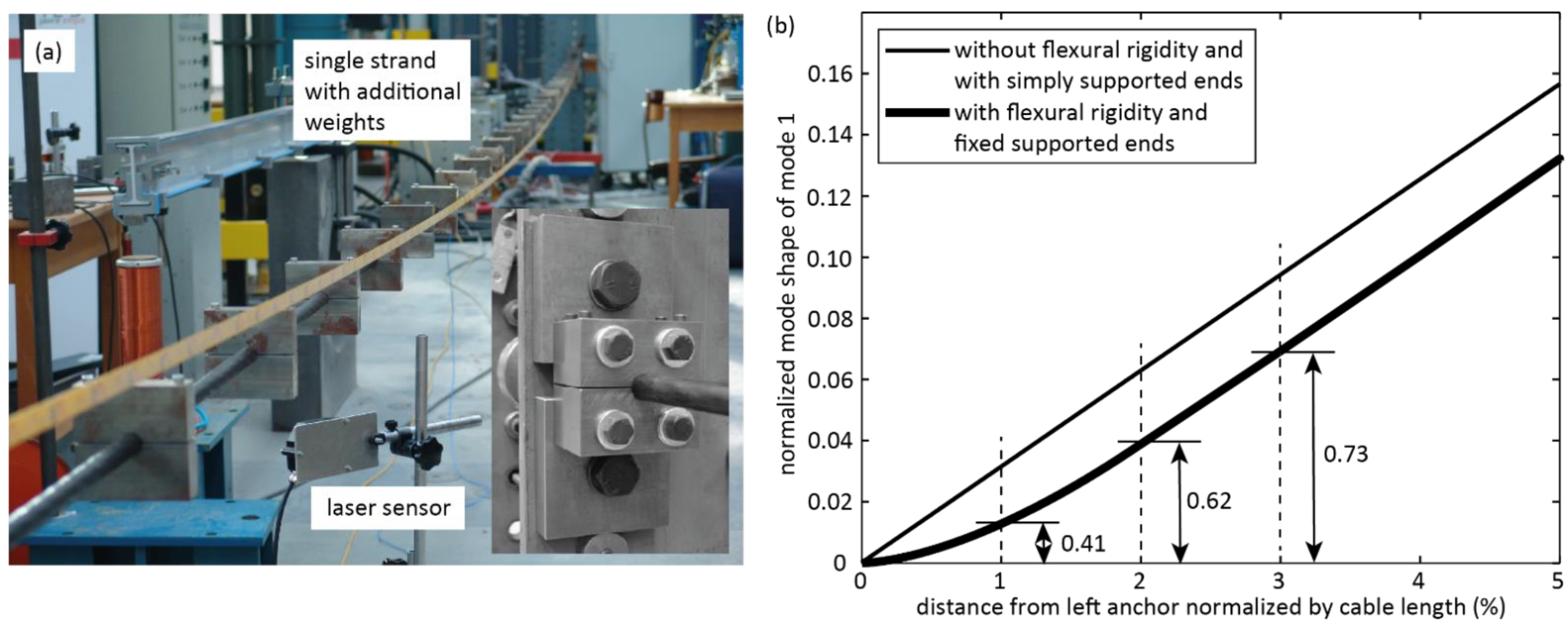
2.2. Cable with Flexural Rigidity and Fixed Supported Ends
2.3. Cable Model for Simulation
2.4. Optimum Viscous Damper for One Targeted Mode and Taut String Behaviour
2.5. Optimum Viscous Damper for Several Targeted Modes and Taut String Behaviour
3. Semi-Active Cable Damper
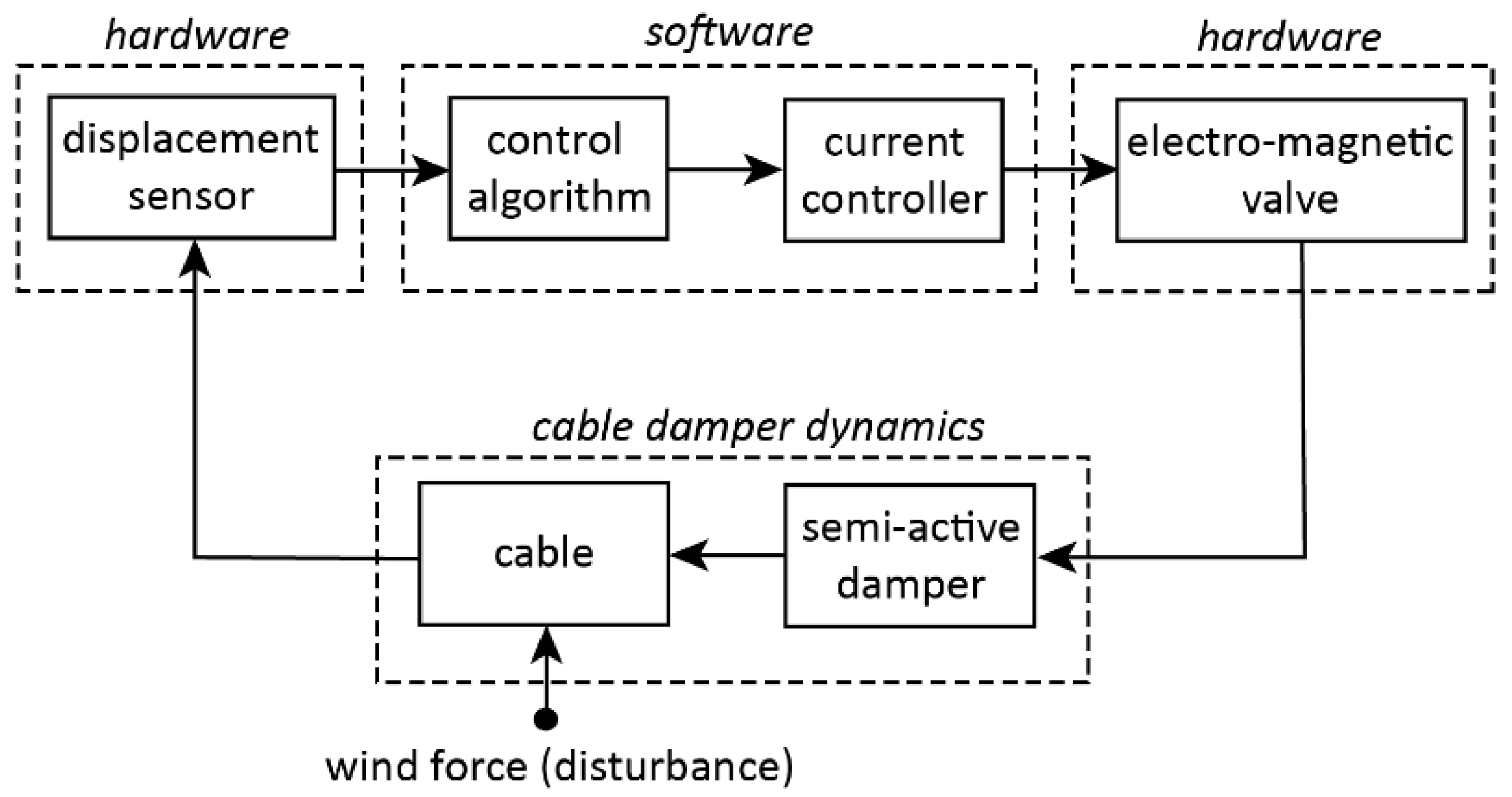
3.1. System Description
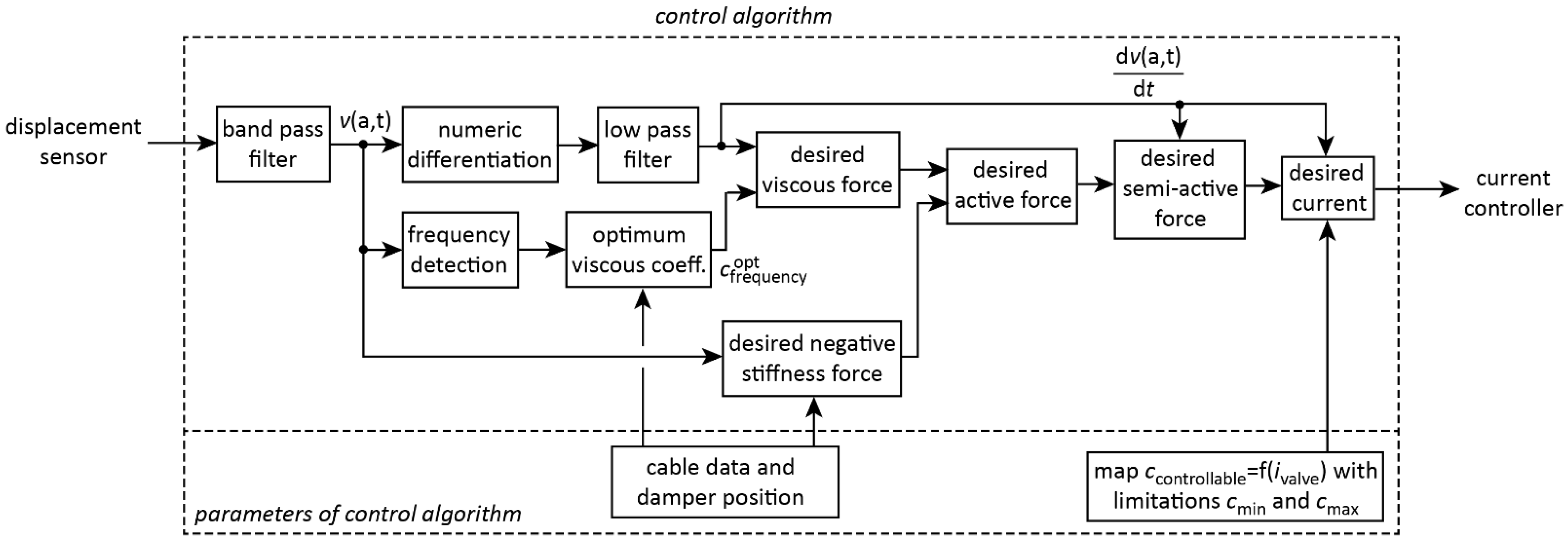
3.2. Control Law
- Bandpass filtering of damper relative motion (displacement sensor signal, Figure 3a) to remove offset and noise in the displacement signal.
- Derivation of damper relative velocity by numeric differentiation with subsequent low pass filtering to attenuate noise due to the numeric differentiation.
- Frequency detection from the peaks of the displacement signal resulting in a maximum time delay of half a period. This time delay is more than acceptable considering that resonant vibrations are long standing vibrations, see cable vibration measurements presented in [30].
- Computing the desired active control force (17) and the desired semi-active control force (18).
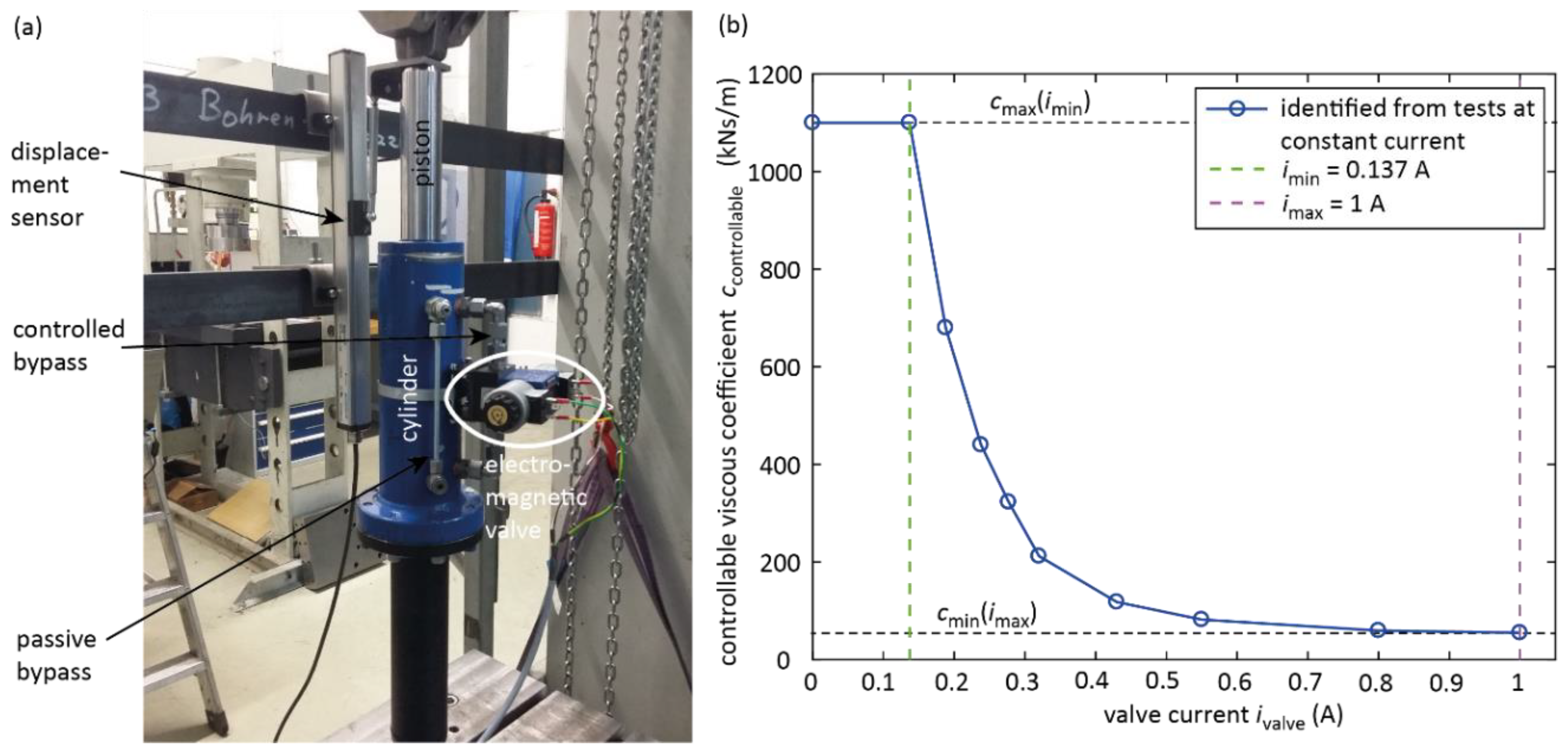
- The control force tracking task is solved in two steps. First, the desired viscous coefficient cdes(t) of the controllable damper is computed based on the desired semi-active control force (19) and the actual damper relative velocity, then the desired current of the electromagnetic valve is computed based on the steady state relation between valve current and viscous damper coefficient (Figure 3a, (20)). Equation (19) shows that the controllable damper exerts quadratic viscous damping at constant bypass valve position, i.e., at constant valve current, because both the controlled bypass valve and the passive bypass act as throttles resulting in quadratic viscous damping.
- The PID-based current controller including an anti-reset windup (ARW) due to the current limitations ensures that the actual valve current precisely tracks the desired current .
- The state variables, which are given as function of time t in (15)–(20), are computed in real time at the controller frequency of 1000 Hz.
- The closed-loop structure consisting of hardware components, software components and cable damper dynamics is depicted in Figure 5.
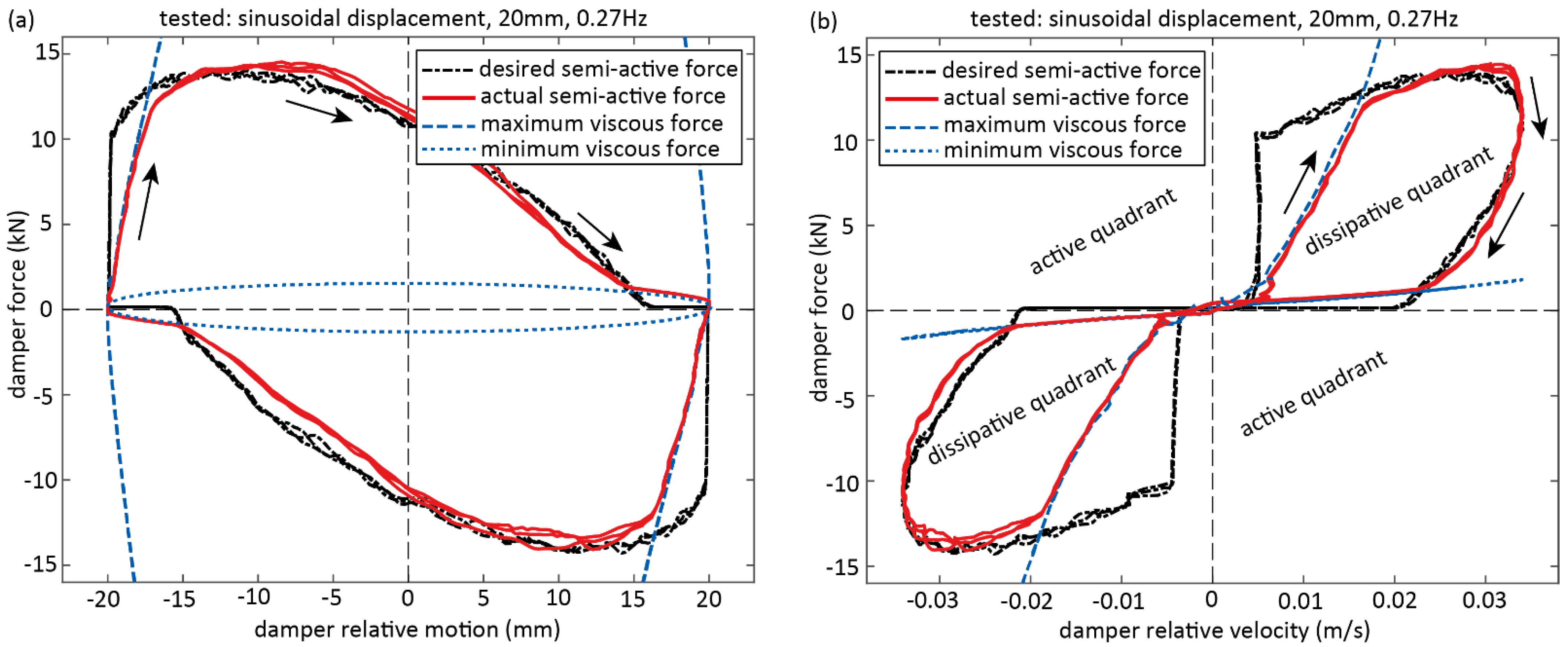
3.3. Experimental Validation
3.4. Modell of Semi-Active Damper Force including Force Limitations
4. Simulation results
4.1. Assumptions, Cable Properties
- Damper types:
- -
- Passive linear viscous damper being optimized to one targeted mode, i.e., the excited mode, which represents a theoretical benchmark as passive dampers always need to mitigate several targeted modes.
- -
- Passive linear viscous damper being optimized to the targeted modes 1 to 4 describing the realistic benchmark.
- -
- Semi-active damper controlled by clipped VDNS including the control force constraints due to the minimum and maximum controllable viscous coefficients.
- The vibration mitigation of the considered transverse dampers are computed for the first four cable modes.
- Single mode vibrations are considered as resonant vibrations result in greatest cable displacement amplitudes.
- The excitation force due to vortex-shedding is modelled as harmonic excitation.
- The simulations are performed assuming typical cable properties and typical damper positions:
- -
- Cable length L = 300 m.
- -
- Cable tension force T = 6700 kN.
- -
- Mass per unit length m = 100 kg/m.
- -
- First eigenfrequency f1 = 0.431 Hz (determined by L, T, m).
- -
- Inherent cable damping ratio ζcable= 0.4%.
- -
- Relative damper positions a/L = 1%, 1.67%, 2%, 2.5% and 3%.
4.2. Excitation Force Amplitude
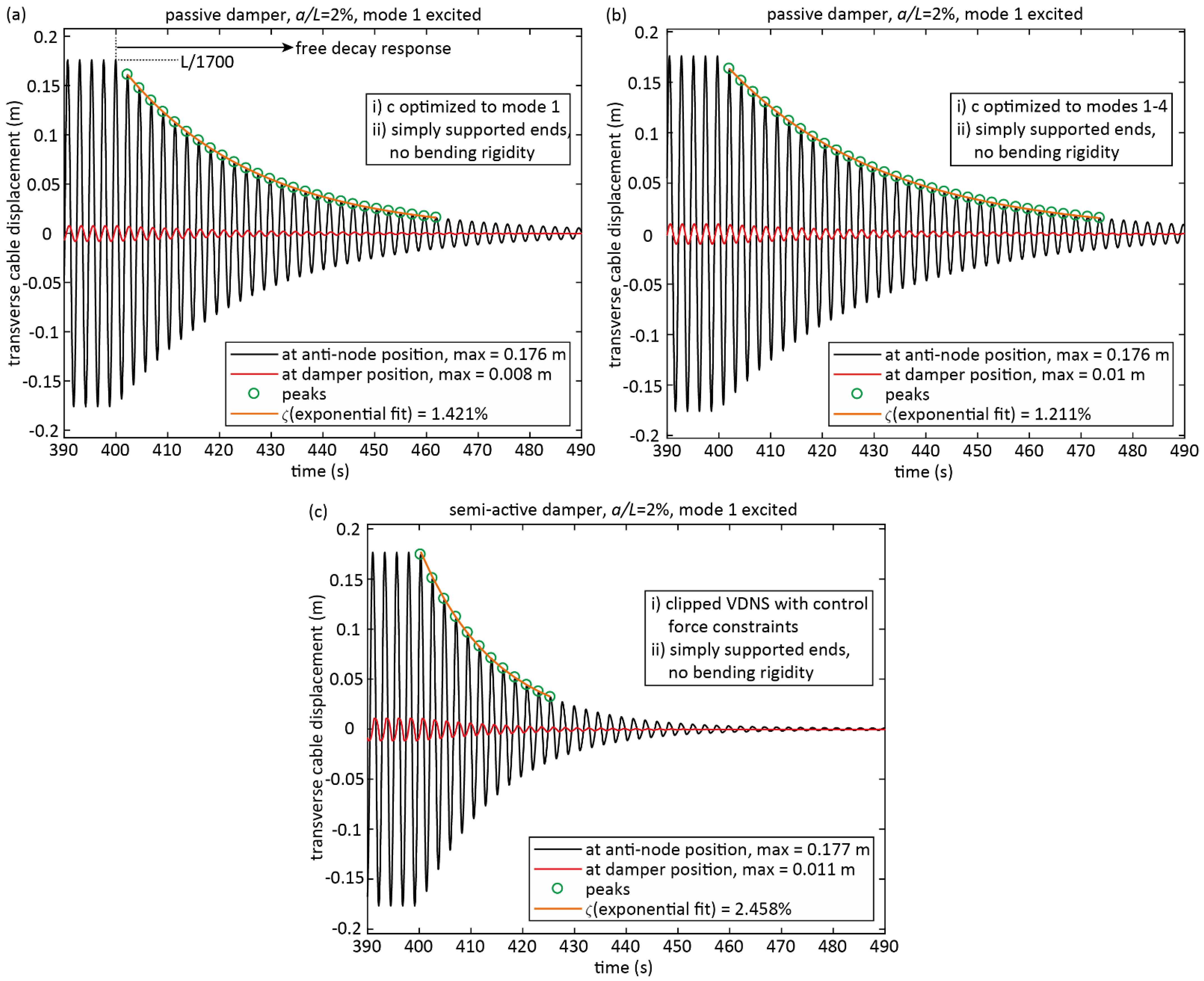
4.3. Damper Performance Assessment from Free Decay Response
4.4. Damper Efficiency
4.5. Results
- Damper types:
- -
- Passive, tuned to mode X (1, 2, 3, 4): linear viscous damper being optimized to the excited mode (19); this is a theoretical benchmark because transverse cable dampers always need to provide the specified damping in several targeted modes; this computation is required to determine the numerical damping.
- -
- Passive, m1-m4: linear viscous damper being optimized to the targeted modes 1–4 (12).
- -
- Semi-active: clipped VDNS including control force constraints due to cmin and cmax (21).
- Cable model:
- -
- Taut string: cable without flexural rigidity and simply supported ends.
- -
- FR and FSC: cable with flexural rigidity and fixed support end conditions.
- Relative damper positions a/L = 1%, 1.67%, 2%, 2.5% and 3%.
- The reduced cable motion at damper position due to cable flexural rigidity and fixed supported ends has strong impact on the cable damping ratio due to passive dampers located at a/L ≤ 2%. This fact is explained by the associated reduced transverse damper motion, see Figure 2b, whereby less energy per cycle can be dissipated.
- The damper efficiencies obtained from the simulation case of a cable with flexural rigidity and fixed supported ends, mode 1 excited, typical damper positions around 2% to 2.5% and passive damper being optimized to modes 1–4 match well with the reported damper efficiencies [23,24,25,26,27,28,29,30,31]. The according results for mode 2 are greater because a damper being optimized to modes 1–4 generates optimum damping in mode 2 (14), see [19]. In this perspective the according simulation results for modes 3 and 4 of the cable model with bending stiffness and fixed support conditions (FR and FSC) seem to be unrealistically high. This irrational result is interpreted by the fact that the cable model FR and FSC is validated for the modeshape of mode 1 of the test steel wire strand depicted in Figure 2 but not for higher modes.
- For some simulation cases, the damping of the cable model with flexural rigidity and fixed supported ends is greater than for the taut string model. This unexpected result is observed for some simulations of modes 3 and 4 and mainly for the passive damper being optimized to modes 1 to 4. An explanation can be that the reduced cable motion at damper position reduces the damper velocity as well whereby the viscous coefficient being optimized for modes 1 to 4 (12) almost generates the optimum viscous force for modes 3 and 4.
- The damping results due to the semi-active damper with the consideration of the minimum and maximum force limitations due to fully open and fully closed bypass valve show the following picture:
- -
- Mode 1: The damping is approximately 2.5…2.6 (taut string) and approximately 2.8…3.2 (FR and FSC) times greater than the damping due to the passive damper being optimized to modes 1 to 4.
- -
- Mode 2: The damping is approximately 2.0…2.6 (taut string) and approximately 1.6…2.1 (FR and FSC) times greater than the damping due to the passive damper being optimized to modes 1 to 4.
- -
- Mode 3: The damping is approximately 2.0…2.1 (taut string) and approximately 1.3…1.9 (FR and FSC) times greater than the damping due to the passive damper being optimized to modes 1 to 4.
- -
- Mode 4: The damping is approximately 2.3…2.5 (taut string) and approximately 1.2…2.1 (FR and FSC) times greater than the damping due to the passive damper being optimized to modes 1 to 4.
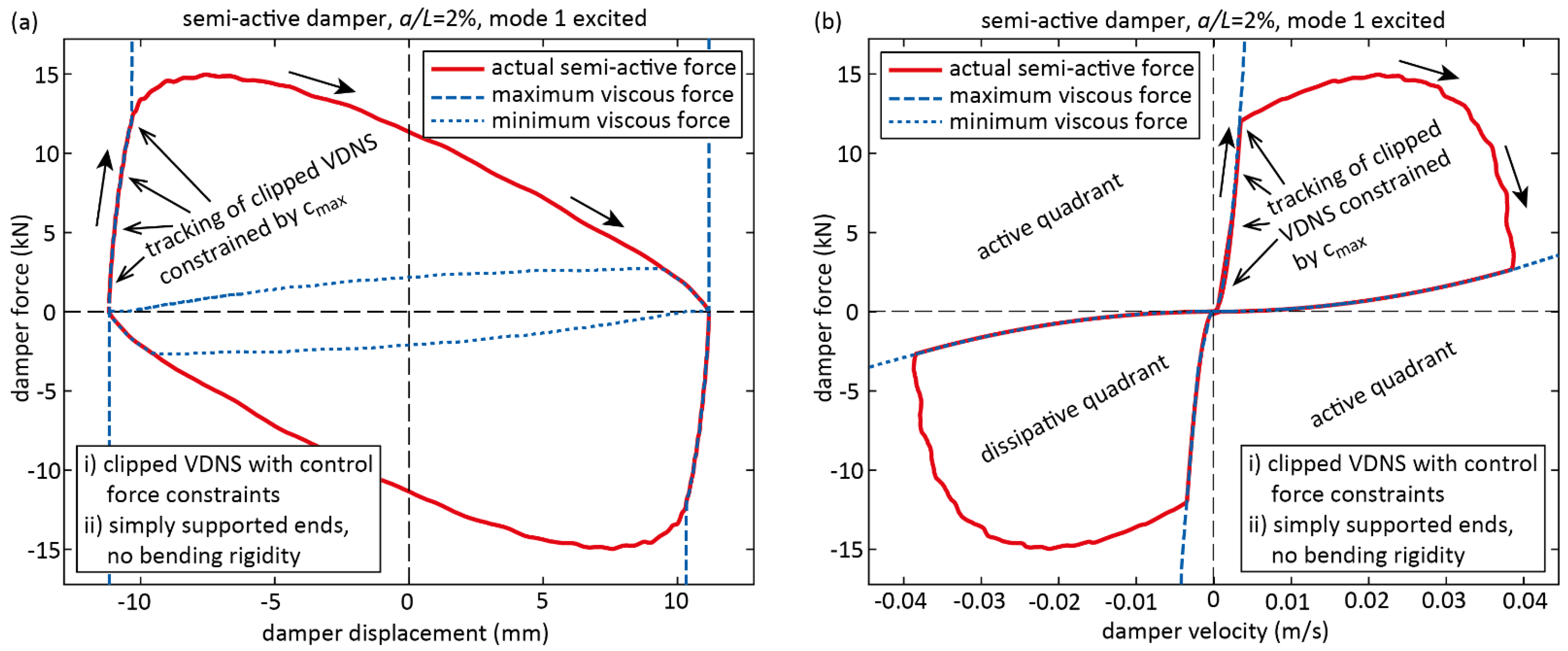
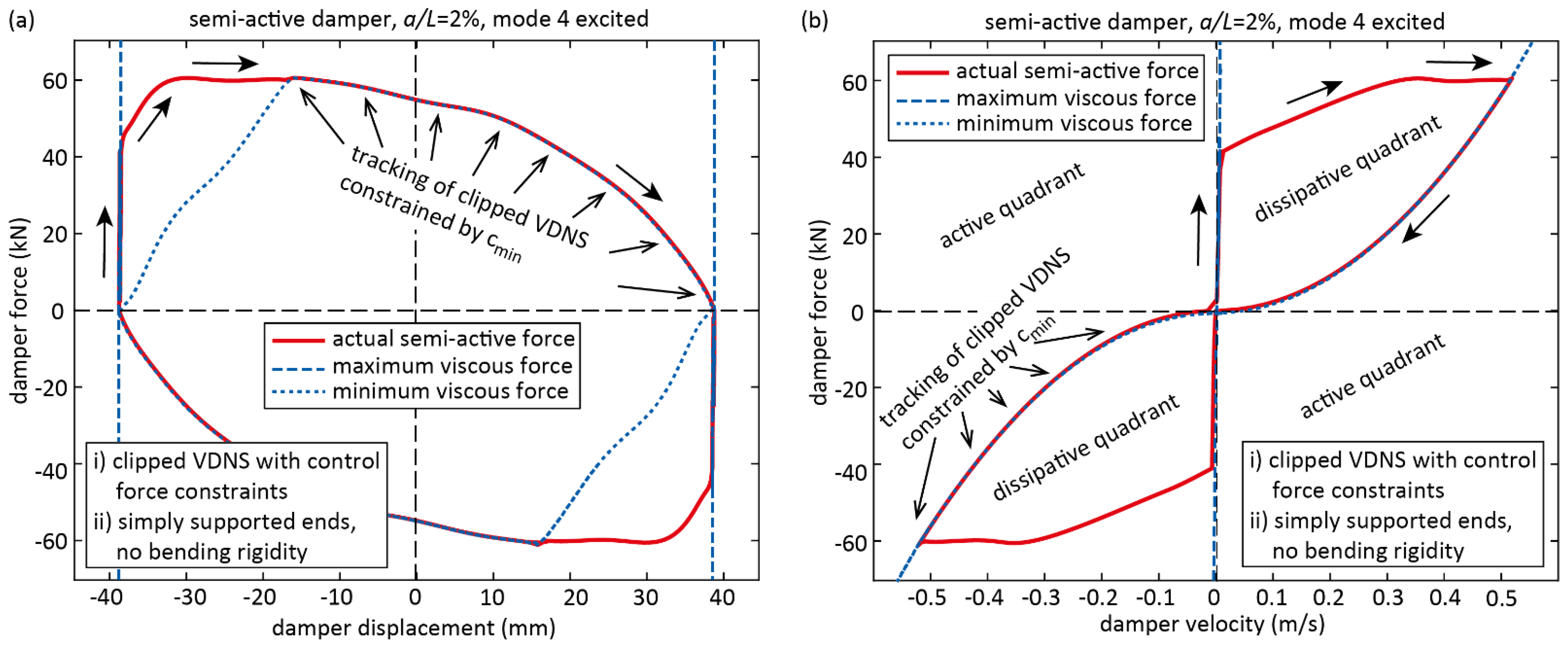
- The tracking of the desired semi-active force is limited by the minimum and maximum viscous coefficients of the controllable damper. The according force displacement curves due to the minimum and maximum quadratic viscous forces are depicted in Figure 8 and Figure 9 for the simulations of mode 1 and 4. It is observed that cmax mainly constrains the force tracking accuracy for lower frequency modes while cmin mainly limits the force tracking accuracy for higher frequency modes. This is explained by the fact that the according limiting control forces are in proportion to the square of the damper relative velocity, see (21). Hence, how precisely the desired semi-active force can be tracked by the semi-active damper with control force limitations due to cmin and cmax depends on mode number, which makes the interpretation of the obtained cable damping results for modes 3 and 4 difficult.
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Fib Bulletin 30. Acceptance of stay cable systems using prestressing steels. In Bulletin 30; International Federation for Structural Concrete Fib: Lausanne, Switzerland, 2005; ISBN 2-88394-070-3. [Google Scholar]
- Jain, A.; Simsir, C.; Sarkar, P.P. Analysis, Measurement, and Mitigation of Stay Cable Vibrations. Eighth Congr. Forensic Eng. 2018, 791–799. [Google Scholar] [CrossRef]
- Matsumoto, M.; Saitoh, T.; Kitazawa, M.; Shirato, H.; Nishizaki, T. Response characteristics of rain-wind induced vibration of stay-cables of cable-stayed bridges. J. Wind Eng. Ind. Aerodyn. 1995, 57, 323–333. [Google Scholar] [CrossRef]
- Zuo, D.; Jones, N.P. Interpretation of field observations of wind- and rain-wind-induced stay cable vibrations. J. Wind Eng. Ind. Aerodyn. 2010, 98, 73–87. [Google Scholar] [CrossRef]
- Li, S.; Chen, Z.; Wu, T.; Kareem, A. Rain-wind-induced in-plane and out-of-plane vibrations of stay cables. J. Eng. Mech. 2013, 139, 1688–1698. [Google Scholar] [CrossRef]
- Wang, H.; Li, A.; Niu, J.; Zong, Z.; Li, J. Long-term monitoring of wind characteristics at Sutong Bridge site. J. Wind Eng. Ind. Aerodyn. 2013, 115, 39–47. [Google Scholar] [CrossRef]
- Guo, J.; Zhu, X. Field Monitoring and Analysis of the Vibration of Stay Cables under Typhoon Conditions. Sensors 2020, 20, 4520. [Google Scholar] [CrossRef] [PubMed]
- Vo-Duy, H.; Nguyen, C.H. Vibration Control Techniques for Dynamic Response Mitigation of Civil Structures under Multiple Hazards. Shock Vib. 2020, 2020, 5845712. [Google Scholar] [CrossRef]
- Daniottia, N.; Jakobsen, J.B.; Snæbjörnsson, J.; Cheynet, E.; Wang, J. Observations of bridge stay cable vibrations in dry and wet conditions: A case study. J. Sound Vib. 2021, 503, 116106. [Google Scholar] [CrossRef]
- An, M.; Li, S.; Liu, Z.; Yan, B.; Li, L.; Chen, Z. Galloping vibration of stay cable installed with a rectangular lamp: Field observations and wind tunnel tests. J. Wind Eng. Ind. Aerodyn. 2021, 215, 104685. [Google Scholar]
- Ni, Y.Q.; Wang, X.Y.; Chen, Z.Q.; Ko, J.M. Field observations of rain-wind-induced cable vibration in cable-stayed Dongting Lake Bridge. J. Wind Eng. Ind. Aerodyn. 2007, 95, 303–328. [Google Scholar] [CrossRef]
- Savor, Z.; Radic, J.; Hrelja, G. Cable vibrations at Dubrovnik bridge. Bridge Struct. 2006, 2, 97–106. [Google Scholar] [CrossRef]
- Casas, J.R.; Aparicio, A.C. Rain-wind-induced cable vibrations in the Alamillo cable-stayed bridge (Sevilla, Spain). Assessment and remedial action. Struct. Infrastruct. Eng. 2010, 6, 549–556. [Google Scholar] [CrossRef] [Green Version]
- Pacheco, B.M.; Fujino, Y.; Sulekh, A. Estimation curve for modal damping in stay cables with viscous damper. J. Struct. Eng. 1993, 119, 1961–1979. [Google Scholar] [CrossRef]
- Krenk, S. Vibrations of a taut cable with an external damper. J. Appl. Mech. 2000, 67, 772–776. [Google Scholar] [CrossRef]
- Main, J.A.; Jones, N.P. Free vibrations of taut cable with attached damper. I: Linear viscous damper. J. Eng. Mech. 2002, 128, 1062–1071. [Google Scholar] [CrossRef] [Green Version]
- Krenk, S.; Høgsberg, J.R. Damping of cables by a transverse force. J. Eng. Mech. 2005, 131, 340–348. [Google Scholar] [CrossRef]
- Fujino, Y.; Siringoringo, D. Vibration Mechanisms and Controls of Long-Span Bridges: A Review. Struct. Eng. Int. 2013, 23, 248–268. [Google Scholar] [CrossRef]
- Weber, F.; Feltrin, G.; Maślanka, M.; Fobo, W.; Distl, H. Design of viscous dampers targeting multiple cable modes. Eng. Struct. 2009, 31, 2797–2800. [Google Scholar] [CrossRef]
- Hoang, N.; Fujino, Y. Analytical study on bending effects in a stay cable with a damper. J. Eng. Mech. 2007, 133, 1241–1246. [Google Scholar] [CrossRef]
- Hammoudi, Z.S.; Abbas, A.L.; Mahmood, H.A. Free Vibration Analysis of Cable Stayed-Bridge by Finite Element Method. Diyala J. Eng. Sci. 2019, 12, 67–72. [Google Scholar]
- Liu, M.; Zhang, G. Damping of Stay Cable-Passive Damper System with Effects of Cable Bending Stiffness and Damper Stiffness. Appl. Mech. Mater. 2021, 204–208, 4513–4517. [Google Scholar] [CrossRef]
- Boston, C.; Weber, F.; Guzzella, L. Optimal semi-active damping of cables with bending stiffness. Smart Mater. Struct. 2011, 20, 055005. [Google Scholar] [CrossRef]
- Weber, F.; Boston, C. Clipped viscous damping with negative stiffness for semi-active cable damping. Smart Mater. Struct. 2011, 20, 045007. [Google Scholar] [CrossRef]
- Duan, Y.F.; Ni, Y.Q.; Ko, J.M. State-derivative feedback control of cable vibration using semiactive magnetorheological dampers. Comput.-Aided Civ. Infrastruct. Eng. 2005, 20, 431–449. [Google Scholar] [CrossRef]
- Christenson, R.E.; Spencer, B.F., Jr.; Johnson, E.A. Experimental verification of smart cable damping. J. Eng. Mech. 2006, 132, 268–278. [Google Scholar] [CrossRef]
- Duan, Y.F.; Ni, Y.Q.; Ko, J.M. Cable vibration control using magnetorheological dampers. J. Intell. Mater. Syst. Struct. 2006, 17, 321–325. [Google Scholar] [CrossRef]
- Zhou, H.; Sun, L.; Xing, F. Damping of Full-Scale Stay Cable with Viscous Damper: Experiment and Analysis. Adv. Struct. Eng. 2014, 17, 265–274. [Google Scholar] [CrossRef]
- Weber, F.; Distl, H. Amplitude and frequency independent cable damping of Sutong Bridge and Russky Bridge by MR dampers. Struct. Control Health Monit. 2015, 22, 237–254. [Google Scholar] [CrossRef]
- Weber, F.; Distl, H. Damping estimation from free decay responses of cables with MR dampers. Sci. World J. Spec. Issue Cable Struct. Dyn. Control Monit. 2015, 2015, 861954. [Google Scholar] [CrossRef] [Green Version]
- Zhou, H.; Xiang, N.; Huang, X.; Sun, L.; Xing, F.; Zhou, R. Full-scale test of dampers for stay cable vibration mitigation and improvement measures. Struct. Monit. Maint. 2018, 5, 489–506. [Google Scholar]
- Lee, J.-J.; Kim, J.-M.; Ahn, S.-S.; Choi, J.-S. Development of a cable exciter to evaluate damping ratios of a stay cable. KSCE J. Civ. Eng. 2010, 14, 363–370. [Google Scholar] [CrossRef]
- Bournand, Y.; Crigler, J. The VSL friction damper for cable-stayed bridges. Some results from maintenance and testing on long cables. In Proceedings of the 6th International Conference on Cable Dynamics 2005, Charleston, SC, USA, 19–22 September 2005; pp. 199–204. [Google Scholar]
- Weber, F.; Høgsberg, J.; Krenk, S. Optimal tuning of amplitude proportional Coulomb friction damper for maximum cable damping. J. Struct. Eng. 2010, 136, 123–134. [Google Scholar] [CrossRef]
- Weber, F.; Boston, C. Energy Based Optimization of Viscous-Friction Dampers on Cables. Smart Mater. Struct. 2010, 19, 045025. [Google Scholar] [CrossRef]
- Maślanka, M.; Sapinski, B.; Snamina, J. Experimental study of vibration control of a cable with an attached MR damper. J. Theor. Appl. Mech. 2007, 45, 893–917. [Google Scholar]
- Li, H.; Liu, M.; Ou, J. Negative stiffness characteristics of active and semi-active control systems for stay cables. Struct. Control Health Monit. 2008, 15, 120–142. [Google Scholar] [CrossRef]
- Weber, F. Bouc-Wen model-based real-time force tracking scheme for MR dampers. Smart Mater. Struct. 2013, 22, 045012. [Google Scholar] [CrossRef]
- Weber, F. Robust force tracking control scheme for MR dampers. Struct. Control Health Monit. 2015, 22, 1373–1395. [Google Scholar] [CrossRef]
- Zhou, P.; Li, H. Modeling and control performance of a negative stiffness damper for suppressing stay cable vibrations. Struct. Control Health Monit. 2016, 23, 764–782. [Google Scholar] [CrossRef]
- Wang, Z.H.; Xu, Y.W.; Gao, H.; Chen, Z.Q.; Xu, K.; Zhao, S.B. Vibration control of a stay cable with a rotary electromagnetic inertial mass damper. Smart Struct. Syst 2019, 23, 627–639. [Google Scholar]
- Li, Y.; Shen, W.; Zhu, H. Vibration mitigation of stay cables using electromagnetic inertial mass dampers: Full-scale experiment and analysis. Eng. Struct. 2019, 200, 109693. [Google Scholar] [CrossRef]
- Di, F.; Sun, L.; Chen, L. Cable vibration control with internal and external dampers: Theoretical analysis and field test validation. Smart Struct. Syst. 2020, 26, 575–589. [Google Scholar]
- Jeong, S.; Lee, Y.-J.; Sim, S.-H. Serviceability Assessment Method of Stay Cables with Vibration Control Using First-Passage Probability. Math. Probl. Eng. 2019, 2019, 4138279. [Google Scholar] [CrossRef]
- Bathe, K.-J. Finite Element Procedures in Engineering Analysis; Prentice-Hall: Englewood Cliffs, NJ, USA, 1982. [Google Scholar]
- Weber, F.; Distl, H. Semi-active damping with negative stiffness for multi-mode cable vibration mitigation: Approximate collocated control solution. Smart Mater. Struct. 2015, 24, 115015. [Google Scholar] [CrossRef]
- Weber, F.; Maślanka, M. Precise Stiffness and Damping Emulation with MR Dampers and its Application to Semi-active Tuned Mass Dampers of Wolgograd Bridge. Smart Mater. Struct. 2014, 23, 015019. [Google Scholar] [CrossRef]
| Damper Type | Cable Model | a/L (%) | ½ a/L | η (%) | ||
|---|---|---|---|---|---|---|
| passive, tuned to mode 1 | taut string | 1% | 0.505% | 0.005% (*) | 0.5% | 100% (*) |
| FR & FSC | 0.354% | 69.8% | ||||
| passive, tuned to modes 1–4 | taut string | 0.403% | 79.6% | |||
| FR & FSC | 0.198% | 38.6% | ||||
| semi-active | taut string | 1.036% | 206.2% | |||
| FR & FSC | 0.571% | 113.2% | ||||
| passive, tuned to mode 1 | taut string | 1.67% | 0.848% | 0.013% (*) | 0.84% | 100% (*) |
| FR & FSC | 0.716% | 84.2% | ||||
| passive, tuned to modes 1–4 | taut string | 0.675% | 79.3% | |||
| FR & FSC | 0.431% | 50.1% | ||||
| semi-active | taut string | 1.723% | 204.8% | |||
| FR & FSC | 1.310% | 155.3% | ||||
| passive, tuned to mode 1 | taut string | 2% | 1.021% | 0.021% (*) | 1% | 100% (*) |
| FR & FSC | 0.912% | 89.1% | ||||
| passive, tuned to modes 1–4 | taut string | 0.811% | 79.0% | |||
| FR & FSC | 0.570% | 54.9% | ||||
| semi-active | taut string | 2.058% | 203.7% | |||
| FR & FSC | 1.790% | 176.9% | ||||
| passive, tuned to mode 1 | taut string | 2.5% | 1.284% | 0.034% (*) | 1.25% | 100% (*) |
| FR & FSC | 1.194% | 92.8% | ||||
| passive, tuned to modes 1–4 | taut string | 1.017% | 78.6% | |||
| FR & FSC | 0.775% | 59.3% | ||||
| semi-active | taut string | 2.636% | 208.2% | |||
| FR & FSC | 2.395% | 188.9% | ||||
| passive, tuned to mode 1 | taut string | 3% | 1.549% | 0.049% (*) | 1.5% | 100% (*) |
| FR & FSC | 1.471% | 94.8% | ||||
| passive, tuned to modes 1–4 | taut string | 1.224% | 78.3% | |||
| FR & FSC | 0.978% | 61.9% | ||||
| semi-active | taut string | 3.169% | 208.0% | |||
| FR & FSC | 3.014% | 197.7% |
| Damper Type | Cable Model | a/L (%) | ½ a/L | η (%) | ||
|---|---|---|---|---|---|---|
| passive, tuned to mode 2 | taut string | 1% | 0.506% | 0.006% (*) | 0.5% | 100% (*) |
| FR & FSC | 0.354% | 69.6% | ||||
| passive, tuned to modes 1–4 | taut string | 0.506% | 100.0% | |||
| FR & FSC | 0.354% | 69.6% | ||||
| semi-active | taut string | 1.025% | 203.8% | |||
| FR & FSC | 0.571% | 113.0% | ||||
| passive, tuned to mode 2 | taut string | 1.67% | 0.849% | 0.014% (*) | 0.84% | 100% (*) |
| FR & FSC | 0.716% | 84.1% | ||||
| passive, tuned to modes 1–4 | taut string | 0.849% | 100.0% | |||
| FR & FSC | 0.716% | 84.1% | ||||
| semi-active | taut string | 1.732% | 205.7% | |||
| FR & FSC | 1.342% | 159.0% | ||||
| passive, tuned to mode 2 | taut string | 2% | 1.023% | 0.023% (*) | 1% | 100% (*) |
| FR & FSC | 0.913% | 89.0% | ||||
| passive, tuned to modes 1–4 | taut string | 1.023% | 100.0% | |||
| FR & FSC | 0.913% | 89.0% | ||||
| semi-active | taut string | 2.083% | 206.0% | |||
| FR & FSC | 1.807% | 178.4% | ||||
| passive, tuned to mode 2 | taut string | 2.5% | 1.288% | 0.038% (*) | 1.25% | 100% (*) |
| FR & FSC | 1.196% | 92.6% | ||||
| passive, tuned to modes 1–4 | taut string | 1.288% | 100.0% | |||
| FR & FSC | 1.196% | 92.6% | ||||
| semi-active | taut string | 2.574% | 202.9% | |||
| FR & FSC | 2.461% | 193.8% | ||||
| passive, tuned to mode 2 | taut string | 3% | 1.557% | 0.057% (*) | 1.5% | 100% (*) |
| FR & FSC | 1.474% | 94.5% | ||||
| passive, tuned to modes 1–4 | taut string | 1.557% | 100.0% | |||
| FR & FSC | 1.474% | 94.5% | ||||
| semi-active | taut string | 3.083% | 201.7% | |||
| FR & FSC | 3.081% | 201.6% |
| Damper Type | Cable Model | a/L (%) | ½ a/L | η (%) | ||
|---|---|---|---|---|---|---|
| passive, tuned to mode 3 | taut string | 1% | 0.506% | 0.006% (*) | 0.5% | 100% (*) |
| FR & FSC | 0.354% | 69.6% | ||||
| passive, tuned to modes 1–4 | taut string | 0.465% | 91.8% | |||
| FR & FSC | 0.452% | 89.2% | ||||
| semi-active | taut string | 0.975% | 193.8% | |||
| FR & FSC | 0.567% | 112.2% | ||||
| passive, tuned to mode 3 | taut string | 1.67% | 0.851% | 0.016% (*) | 0.84% | 100% (*) |
| FR & FSC | 0.716% | 83.8% | ||||
| passive, tuned to modes 1–4 | taut string | 0.782% | 91.7% | |||
| FR & FSC | 0.836% | 98.2% | ||||
| semi-active | taut string | 1.655% | 196.3% | |||
| FR & FSC | 1.318% | 155.9% | ||||
| passive, tuned to mode 3 | taut string | 2% | 1.028% | 0.028% (*) | 1% | 100% (*) |
| FR & FSC | 0.913% | 88.5% | ||||
| passive, tuned to modes 1–4 | taut string | 0.944% | 91.6% | |||
| FR & FSC | 1.025% | 99.7% | ||||
| semi-active | taut string | 1.980% | 195.2% | |||
| FR & FSC | 1.761% | 173.3% | ||||
| passive, tuned to mode 3 | taut string | 2.5% | 1.296% | 0.046% (*) | 1.25% | 100% (*) |
| FR & FSC | 1.199% | 92.2% | ||||
| passive, tuned to modes 1–4 | taut string | 1.190% | 91.5% | |||
| FR & FSC | 1.295% | 99.9% | ||||
| semi-active | taut string | 2.403% | 188.6% | |||
| FR & FSC | 2.319% | 181.8% | ||||
| passive, tuned to mode 3 | taut string | 3% | 1.569% | 0.069% (*) | 1.5% | 100% (*) |
| FR & FSC | 1.481% | 94.1% | ||||
| passive, tuned to modes 1–4 | taut string | 1.442% | 91.5% | |||
| FR & FSC | 1.563% | 99.6% | ||||
| semi-active | taut string | 2.833% | 184.3% | |||
| FR & FSC | 2.924% | 190.3% |
| Damper Type | Cable Model | a/L (%) | ½ a/L | η (%) | ||
|---|---|---|---|---|---|---|
| passive, tuned to mode 4 | taut string | 1% | 0.507% | 0.007% (*) | 0.5% | 100% (*) |
| FR & FSC | 0.354% | 69.4% | ||||
| passive, tuned to modes 1–4 | taut string | 0.402% | 79.0% | |||
| FR & FSC | 0.497% | 98.0% | ||||
| semi-active | taut string | 0.949% | 188.4% | |||
| FR & FSC | 0.557% | 110.0% | ||||
| passive, tuned to mode 4 | taut string | 1.67% | 0.855% | 0.020% (*) | 0.84% | 100% (*) |
| FR & FSC | 0.715% | 83.2% | ||||
| passive, tuned to modes 1–4 | taut string | 0.675% | 78.4% | |||
| FR & FSC | 0.850% | 99.4% | ||||
| semi-active | taut string | 1.647% | 194.9% | |||
| FR & FSC | 1.290% | 152.1% | ||||
| passive, tuned to mode 4 | taut string | 2% | 1.033% | 0.033% (*) | 1% | 100% (*) |
| FR & FSC | 0.914% | 88.1% | ||||
| passive, tuned to modes 1–4 | taut string | 0.814% | 78.1% | |||
| FR & FSC | 1.009% | 97.6% | ||||
| semi-active | taut string | 1.954% | 192.1% | |||
| FR & FSC | 1.761% | 172.8% | ||||
| passive, tuned to mode 4 | taut string | 2.5% | 1.308% | 0.058% (*) | 1.25% | 100% (*) |
| FR & FSC | 1.204% | 91.7% | ||||
| passive, tuned to modes 1–4 | taut string | 1.029% | 77.7% | |||
| FR & FSC | 1.242% | 94.7% | ||||
| semi-active | taut string | 2.419% | 188.9% | |||
| FR & FSC | 2.346% | 183.0% | ||||
| passive, tuned to mode 4 | taut string | 3% | 1.590% | 0.090% (*) | 1.5% | 100% (*) |
| FR & FSC | 1.488% | 93.2% | ||||
| passive, tuned to modes 1–4 | taut string | 1.245% | 77.0% | |||
| FR & FSC | 1.475% | 92.3% | ||||
| semi-active | taut string | 2.842% | 183.5% | |||
| FR & FSC | 2.954% | 190.9% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Weber, F.; Spensberger, S.; Obholzer, F.; Distl, J.; Braun, C. Semi-Active Cable Damping to Compensate for Damping Losses Due to Reduced Cable Motion Close to Cable Anchor. Appl. Sci. 2022, 12, 1909. https://doi.org/10.3390/app12041909
Weber F, Spensberger S, Obholzer F, Distl J, Braun C. Semi-Active Cable Damping to Compensate for Damping Losses Due to Reduced Cable Motion Close to Cable Anchor. Applied Sciences. 2022; 12(4):1909. https://doi.org/10.3390/app12041909
Chicago/Turabian StyleWeber, Felix, Simon Spensberger, Florian Obholzer, Johann Distl, and Christian Braun. 2022. "Semi-Active Cable Damping to Compensate for Damping Losses Due to Reduced Cable Motion Close to Cable Anchor" Applied Sciences 12, no. 4: 1909. https://doi.org/10.3390/app12041909
APA StyleWeber, F., Spensberger, S., Obholzer, F., Distl, J., & Braun, C. (2022). Semi-Active Cable Damping to Compensate for Damping Losses Due to Reduced Cable Motion Close to Cable Anchor. Applied Sciences, 12(4), 1909. https://doi.org/10.3390/app12041909







