A Multi-Scale Approach for Modelling Airborne Transport of Mucosalivary Fluid
Abstract
1. Introduction
2. Governing Equations
2.1. Eulerian Phase
2.2. Lagrangian Phase
2.3. PSI–PBE Coupling
3. Numerical Approximation
Initial and Boundary Conditions
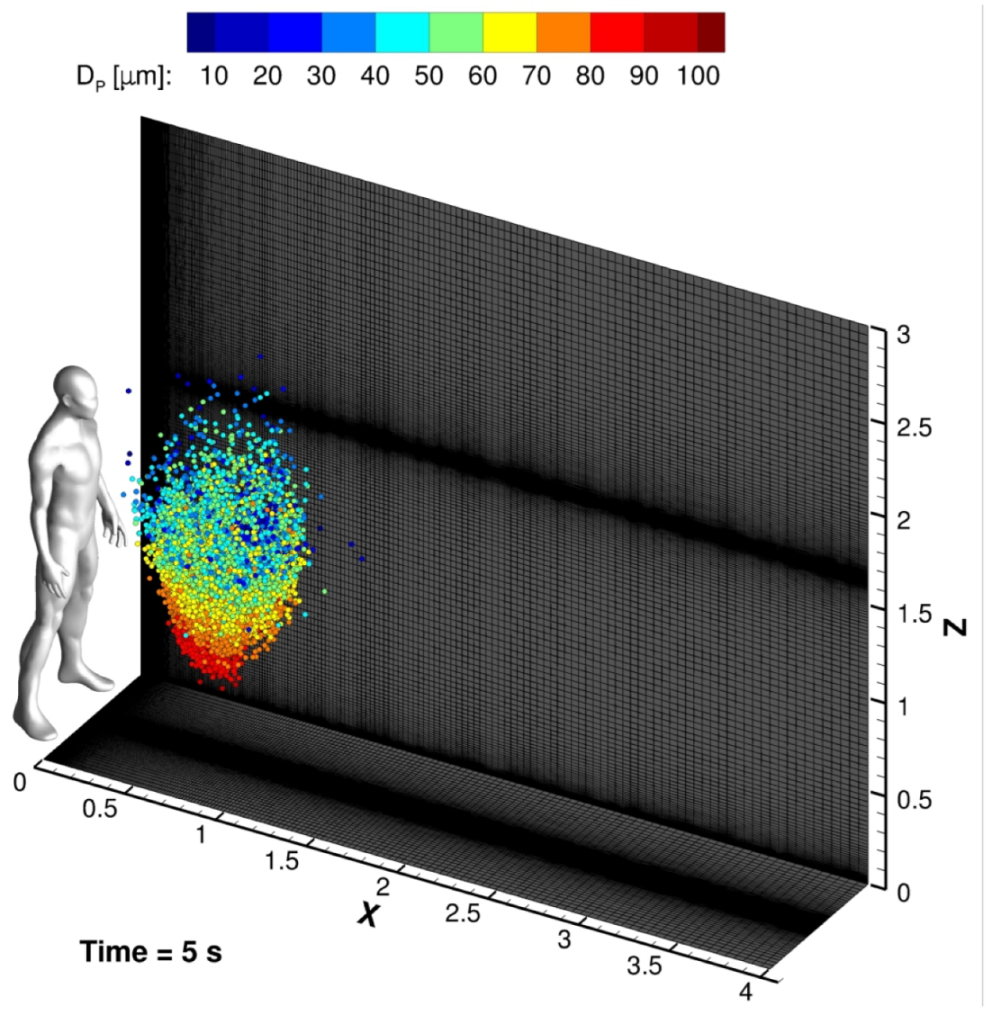
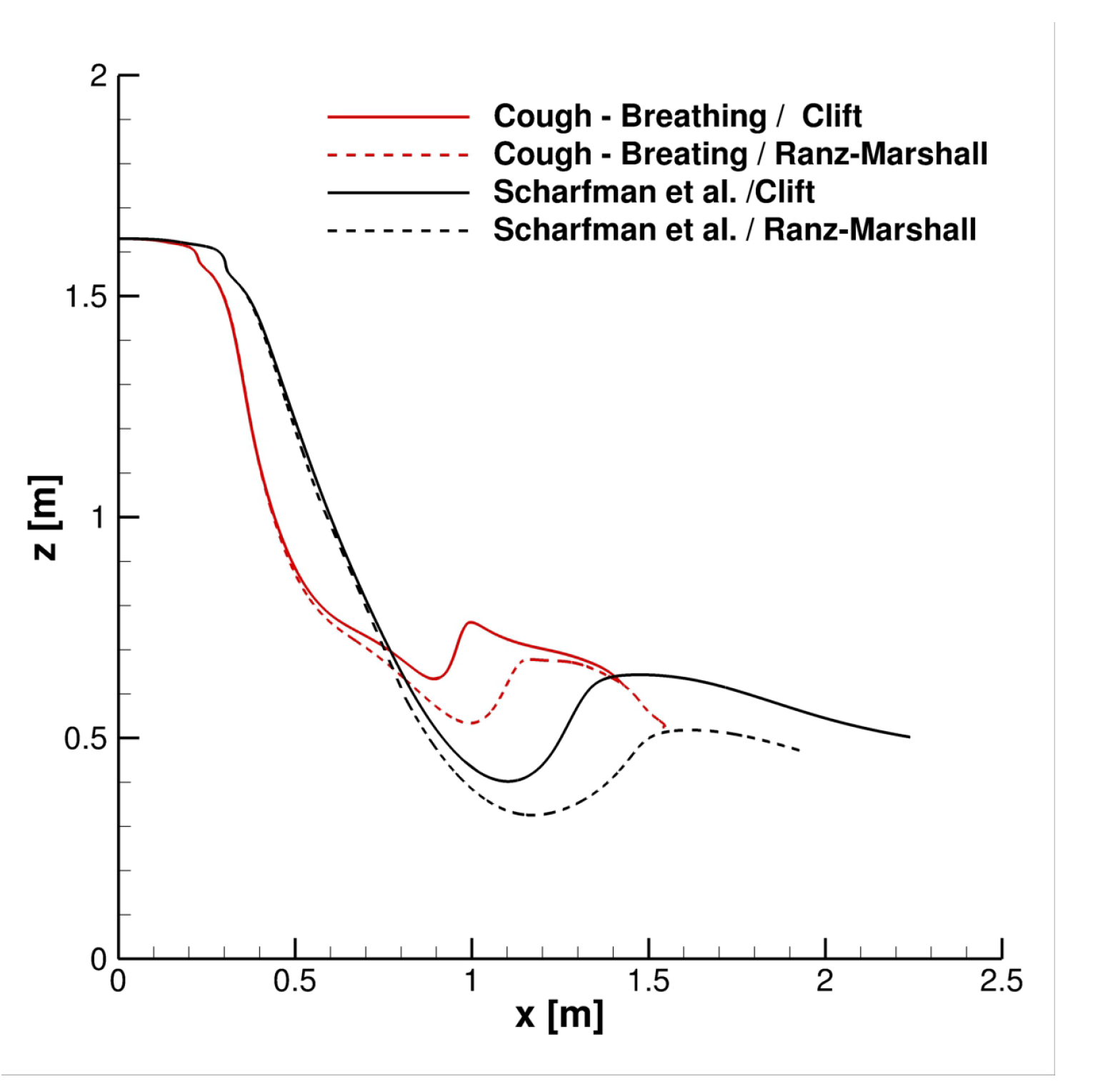
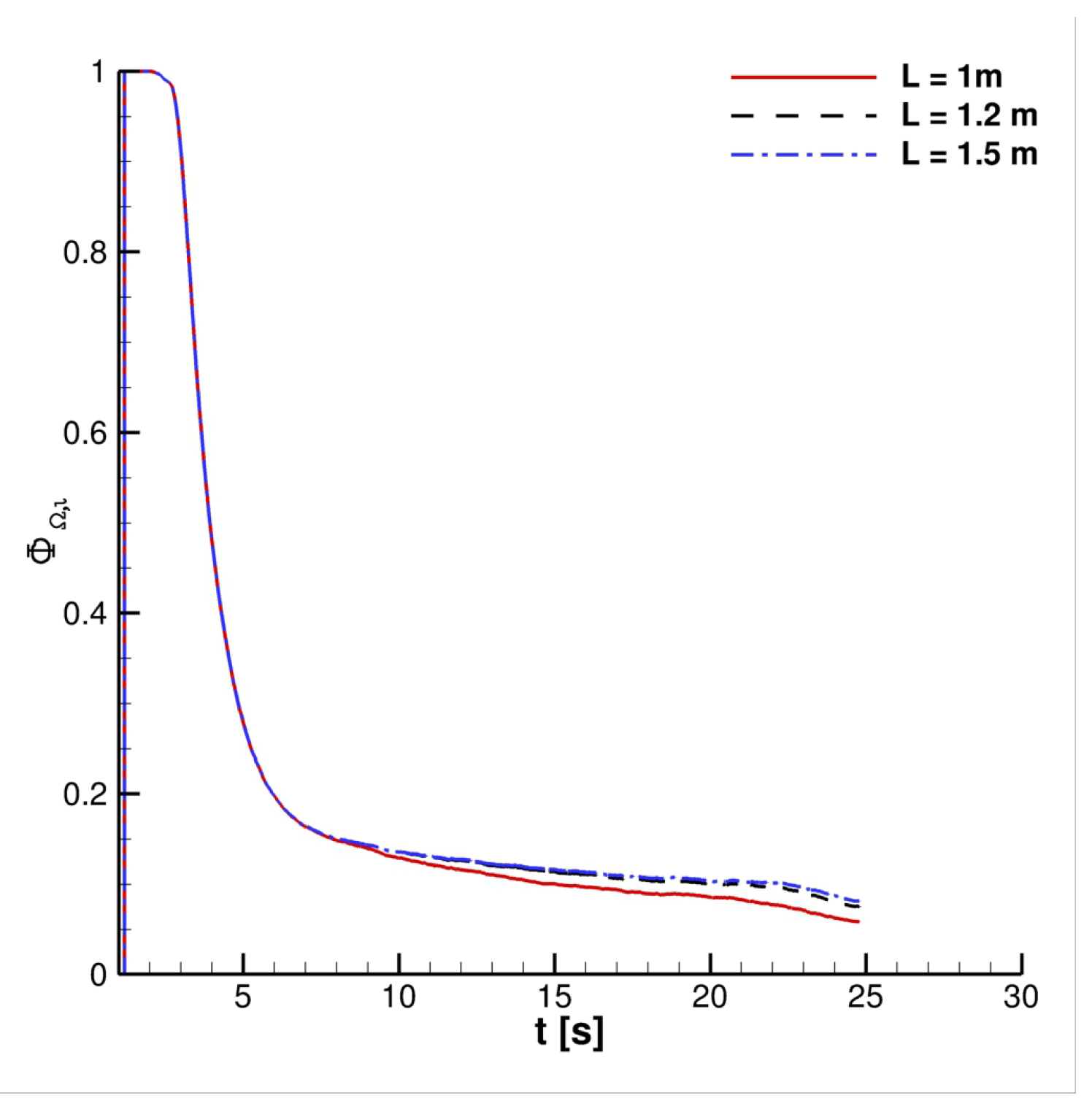
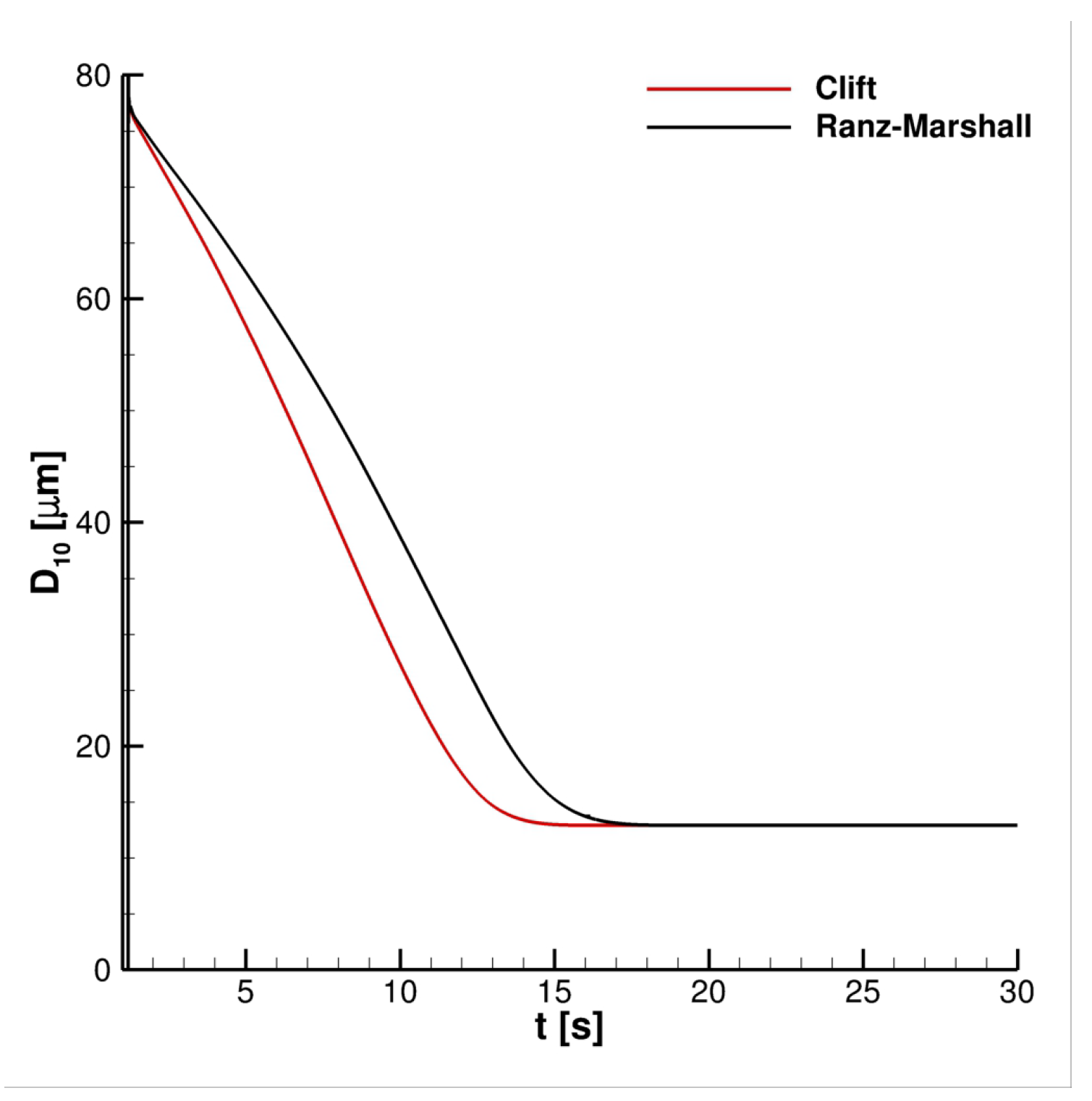
4. Results
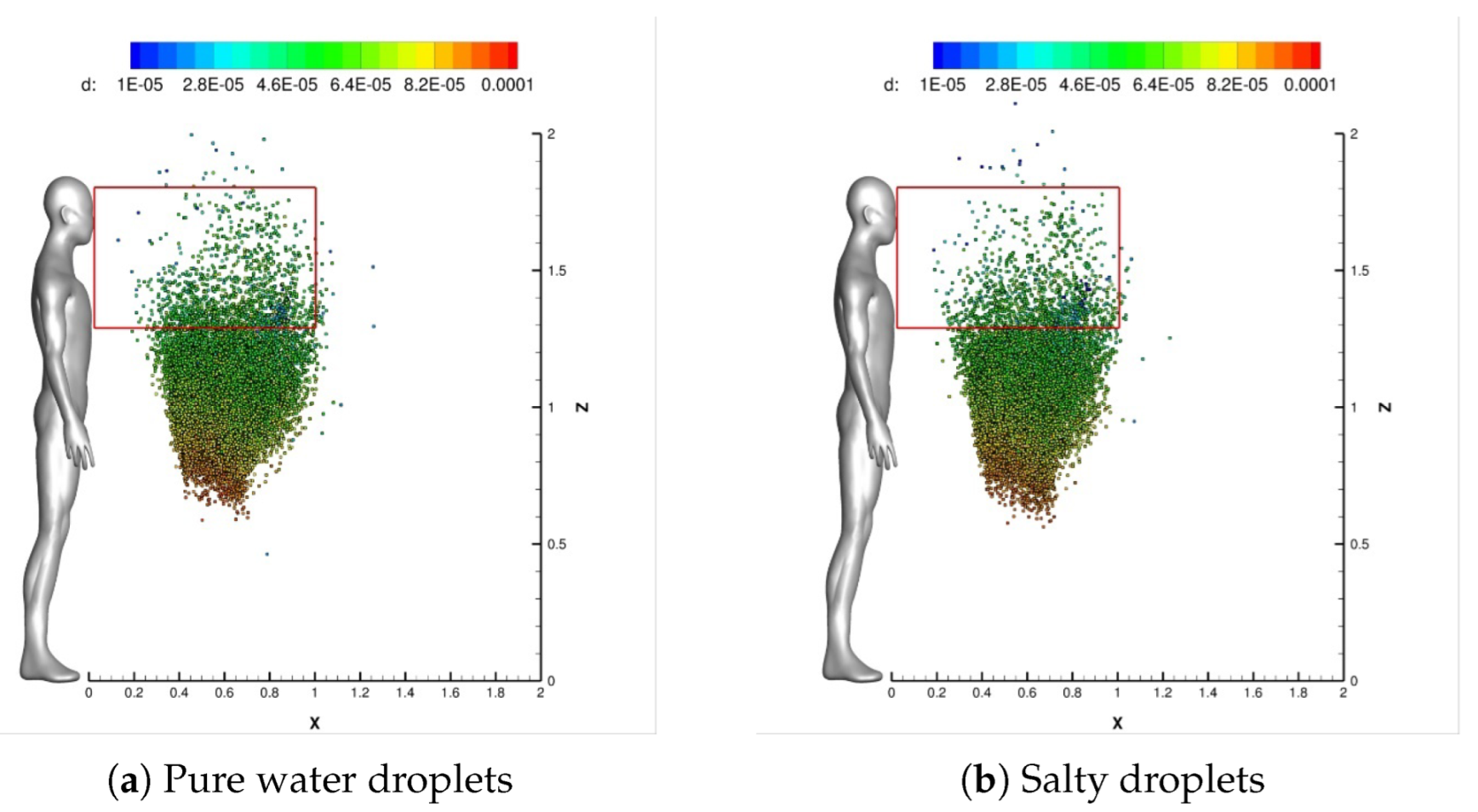
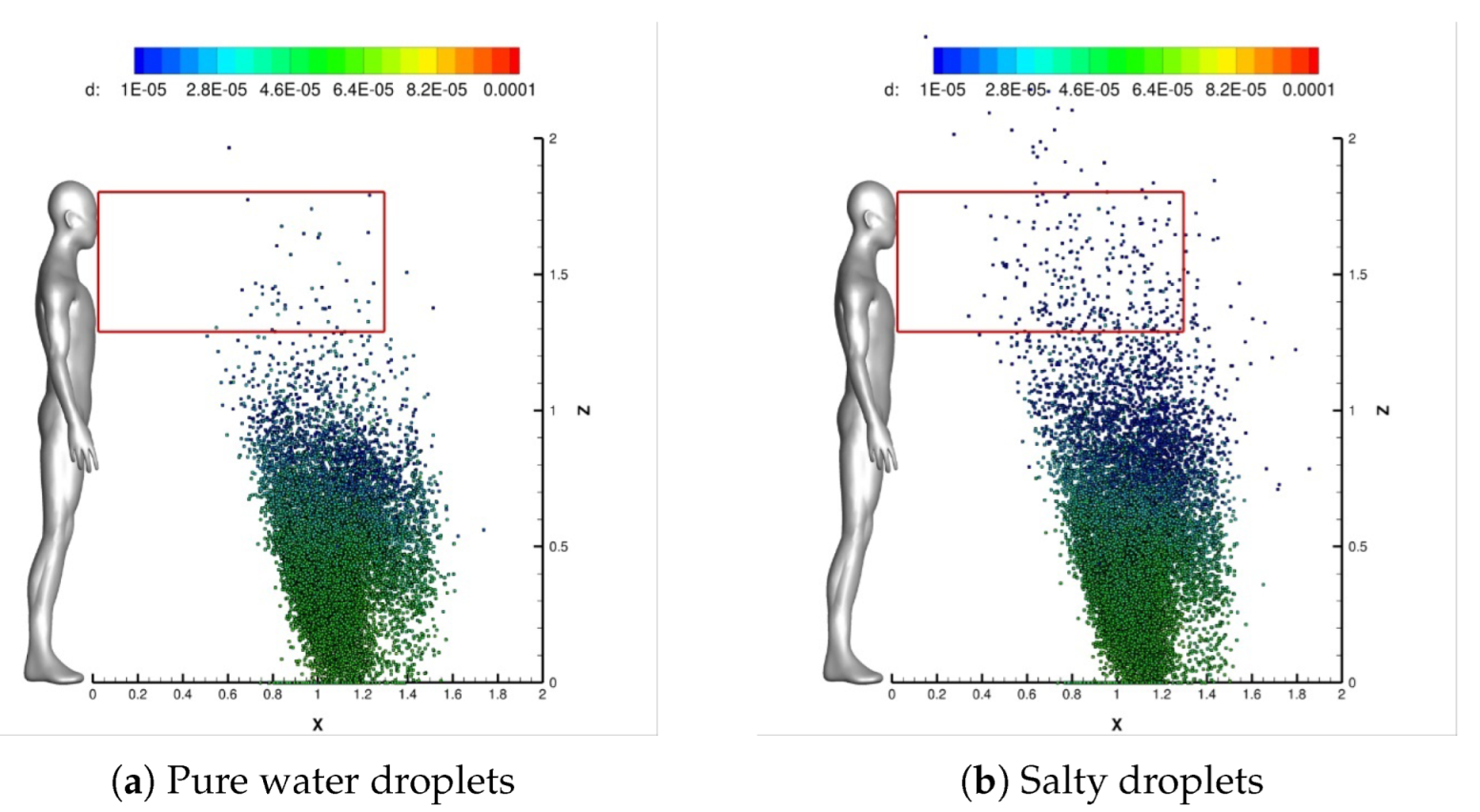
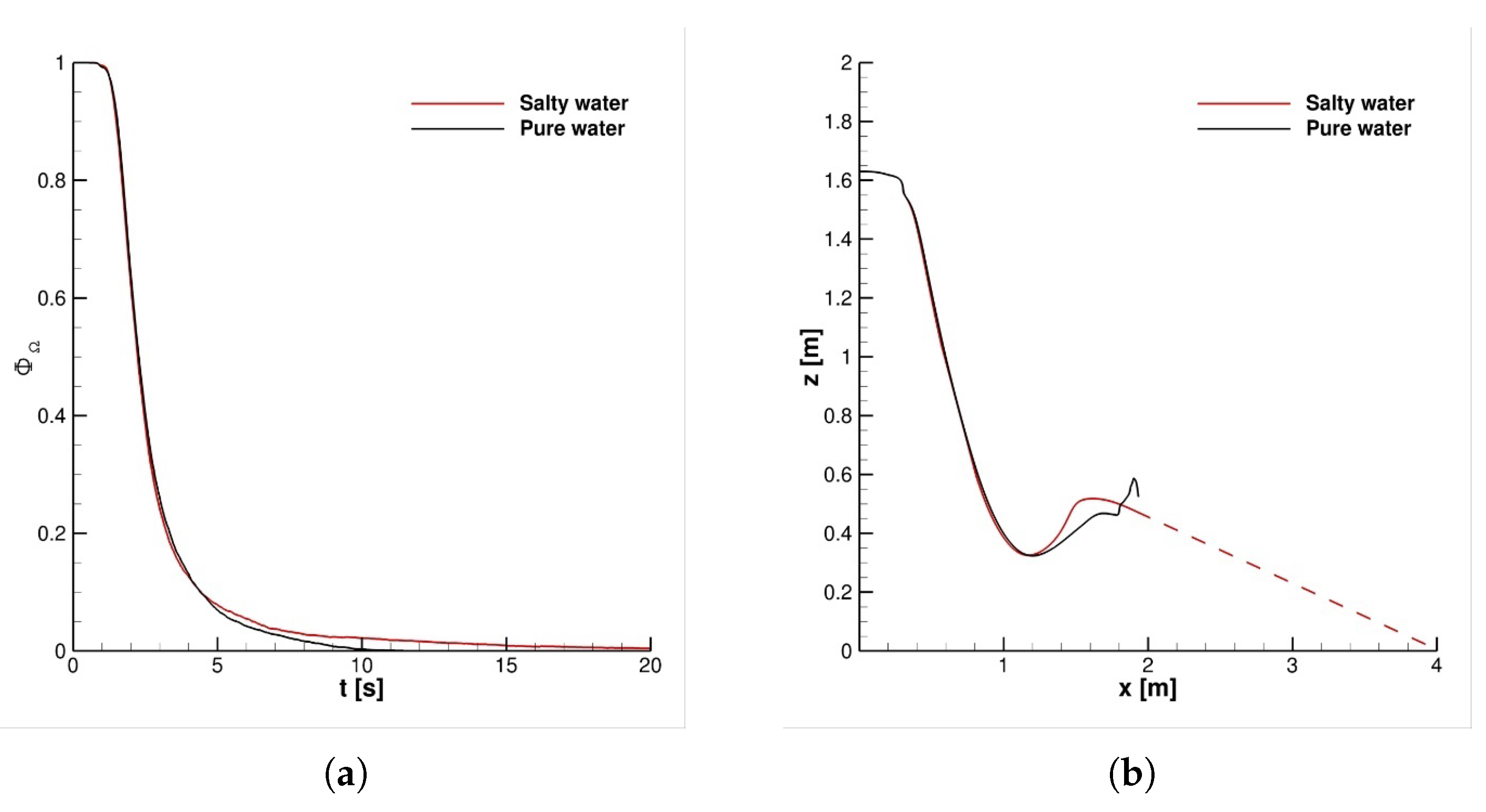
4.1. Impact of Saliva Chemical Composition
4.2. Effect of Velocity Inlet Time-Histories
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| COVID-19 | Coronavirus Diseases-2019 |
| SARS-CoV-2 | Severe acute respiratory syndrome coronavirus 2 |
| PSI | Particle-Source-in-cell method |
| CFD | Computational Fluid–Dynamics |
| RANS | Reynolds Averaged Navier–Stokes equations |
| PBE | Population Balance Equation |
| PISO | Pressure–Implicit with Splitting Operators procedure |
| FVM | Finite–Volume Method |
| PCG | Preconditioned Coniugate Gradient |
| PBiCG | Preconditioned Bi–Coniugate Gradient |
| DILU | Diagonal incomplete–Lower Upper |
| NaCl | Sodium Chloride |
References
- WHO Coronavirus (COVID-19) Dashboard. Available online: https://covid19.who.int (accessed on 1 October 2022).
- Zhang, R.; Li, Y.; Zhang, A.L.; Wang, Y.; Molina, M.J. Identifying airborne transmission as the dominant route for the spread of COVID-19. Proc. Natl. Acad. Sci. USA 2020, 117, 14857–14863. [Google Scholar] [CrossRef] [PubMed]
- Mittal, R.; Ni, R.; Seo, J.H. The flow physics of COVID-19. J. Fluid Mech. 2020, 894, F2. [Google Scholar] [CrossRef]
- Xie, X.; Li, Y.; Chwang, A.T.Y.; Ho, P.L.; Seto, W.H. How far droplets can move in indoor environments—Revisiting the Wells evaporation-falling curve. Indoor Air 2007, 17, 211–225. [Google Scholar] [CrossRef]
- Liu, L.; Wei, J.; Li, Y.; Ooi, A. Evaporation and dispersion of respiratory droplets from coughing. Indoor Air 2017, 27, 179–190. [Google Scholar] [CrossRef] [PubMed]
- van Doremalen, N.; Bushmaker, T.; Morris, D.H.; Holbrook, M.G.; Gamble, A.; Williamson, B.N.; Tamin, A.; Harcourt, J.L.; Thornburg, N.J.; Gerber, S.I.; et al. Aerosol and Surface Stability of SARS-CoV-2 as Compared with SARS-CoV-1. N. Engl. J. Med. 2020, 382, 1564–1567. [Google Scholar] [CrossRef] [PubMed]
- Rosti, M.E.; Olivieri, S.; Cavaiola, M.; Seminara, A.; Mazzino, A. Fluid dynamics of COVID-19 airborne infection suggests urgent data for a scientific design of social distancing. Sci. Rep. 2020, 10, 22426. [Google Scholar] [CrossRef] [PubMed]
- Bourouiba, L.; Dehandschoewercker, E.; Bush, J.W.M. Violent expiratory events: On coughing and sneezing. J. Fluid Mech. 2014, 745, 537–563. [Google Scholar] [CrossRef]
- Bourouiba, L. Turbulent Gas Clouds and Respiratory Pathogen Emissions: Potential Implications for Reducing Transmission of COVID-19. JAMA J. Am. Med. Assoc. 2020, 323, 1837–1838. [Google Scholar] [CrossRef]
- Faleiros, D.; van den Bos, W.; Botto, L.; Scarano, F. TU Delft COVID-app: A tool to democratize CFD simulations for SARS-CoV-2 infection risk analysis. Sci. Total. Environ. 2022, 826, 154143. [Google Scholar] [CrossRef] [PubMed]
- Pourfattah, F.; Wang, L.P.; Deng, W.; Ma, Y.F.; Hu, L.; Yang, B. Challenges in simulating and modeling the airborne virus transmission: A state-of-the-art review. Phys. Fluids 2021, 33, 101302. [Google Scholar] [CrossRef] [PubMed]
- Mohamadi, F.; Fazeli, A. A Review on Applications of CFD Modeling in COVID-19 Pandemic. Arch. Comput. Methods Eng. 2022, 29, 3567–3586. [Google Scholar] [CrossRef] [PubMed]
- Li, H.; Leong, F.Y.; Xu, G.; Ge, Z.; Kang, C.W.; Lim, K.H. Dispersion of evaporating cough droplets in tropical outdoor environment. Phys. Fluids 2020, 32, 113301. [Google Scholar] [CrossRef] [PubMed]
- Stiti, M.; Castanet, G.; Corber, A.; Alden, M.; Berrocal, E. Transition from saliva droplets to solid aerosols in the context of COVID-19 spreading. Environ. Res. 2022, 204, 112072. [Google Scholar] [CrossRef] [PubMed]
- Luo, Q.; Ou, C.; Hang, J.; Luo, Z.; Yang, H.; Yang, X.; Zhang, X.; Li, Y.; Fan, X. Role of pathogen-laden expiratory droplet dispersion and natural ventilation explaining a COVID-19 outbreak in a coach bus. Build. Environ. 2022, 220, 109160. [Google Scholar] [CrossRef] [PubMed]
- D’Alessandro, V.; Falone, M.; Giammichele, L.; Ricci, R. A multiscale approach for modelling saliva droplets airborne transport in relation to SARS-CoV-2 transmission. In Proceedings of the 6th AIGE/IIETA International Conference and XV AIGE Conference, Ancona, Italy, 8–9 July 2021. [Google Scholar]
- Weller, H.; Tabor, G.; Jasak, H.; Fureby, C. A tensorial approach to computational continuum mechanics using object-oriented techniques. Comput. Phys. 1998, 12, 620–631. [Google Scholar] [CrossRef]
- Crowe, C.T.; Sharma, M.P.; Stock, D.E. The Particle-Source-In Cell (PSI-CELL) Model for Gas-Droplet Flows. J. Fluid Eng. 1977, 99, 325–332. [Google Scholar] [CrossRef]
- Menter, F. Two-Equation Eddy Viscosity Turbulence Models for Engineering Applications. AIAA J. 1994, 32, 1598–1695. [Google Scholar] [CrossRef]
- Busco, G.; Yang, S.; Seo, J.; Hassan, Y.A. Sneezing and asymptomatic virus transmission. Phys. Fluids 2020, 32, 073309. [Google Scholar] [CrossRef] [PubMed]
- Abuhegazy, M.; Talaat, K.; Anderoglu, O.; Poroseva, S.V. Numerical investigation of aerosol transport in a classroom with relevance to COVID-19. Phys. Fluids 2020, 32, 103311. [Google Scholar] [CrossRef]
- Putnam, A. Integratable form of droplet drag coefficient. ARS J. 1961, 31, 1467–1468. [Google Scholar]
- Ranz, W.E.; Marshall, W.R. Evaporation from drops. Chem. Eng. Prog. 1952, 48, 141–146. [Google Scholar]
- Clift, R.; Grace, J.R.; Weber, M.E. Bubbles, Drops, and Particles; Dover Publications, Inc.: New York, NY, USA, 2005. [Google Scholar]
- Mugele, R.A.; Evans, H.D. Droplet Size Distribution in Sprays. Ind. Eng. Chem. 1951, 43, 1317–1324. [Google Scholar] [CrossRef]
- Xie, X.; Li, Y.; Sun, H.; Liu, L. Exhaled droplets due to talking and coughing. J. R. Soc. Interface 2012, 6, S703–S714. [Google Scholar] [CrossRef] [PubMed]
- Dbouk, T.; Drikakis, D. On coughing and airborne droplet transmission to humans. Phys. Fluids 2020, 32, 053310. [Google Scholar] [CrossRef] [PubMed]
- Woo, X.Y.; Tan, R.B.H.; Chow, P.S.; Braatz, R.D. Simulation of Mixing Effects in Antisolvent Crystallization Using a Coupled CFD-PDF-PBE Approach. Cryst. Growth Des. 2006, 6, 1291–1303. [Google Scholar] [CrossRef]
- Desarnaud, J.; Derluyn, H.; Carmeliet, J.; Bonn, D.; Shahidzadeh, N. Metastability Limit for the Nucleation of NaCl Crystals in Confinement. J. Phys. Chem. Lett. 2014, 5, 890–895. [Google Scholar] [CrossRef]
- Naillon, A.; Joseph, P.; Prat, M. Sodium chloride precipitation reaction coefficient from crystallization experiment in a microfluidic device. J. Crys. Growth 2017, 463, 201–210. [Google Scholar] [CrossRef]
- Issa, R.I. Solution of the implicitly discretised fluid flow equations by operator-splitting. J. Comput. Phys. 1986, 62, 40–65. [Google Scholar] [CrossRef]
- Ruuth, S.J.; Spiteri, R.J. High-Order Strong-Stability-Preserving Runge-Kutta Methods with Downwind-Biased Spatial Discretizations. SIAM J. Numer. Anal. 2005, 42, 974–996. [Google Scholar] [CrossRef]
- D’Alessandro, V.; Falone, M.; Giammichele, L.; Ricci, R. Eulerian–Lagrangian modeling of cough droplets irradiated by ultraviolet–C light in relation to SARS-CoV-2 transmission. Phys. Fluids 2021, 33, 031905. [Google Scholar] [CrossRef]
- Scharfman, B.E.; Techet, A.H.; Bush, J.W.M.; Bourouiba, L. Visualization of sneeze ejecta: Steps of fluid fragmentation leading to respiratory droplets. Exp. Fluids 2016, 57, 24. [Google Scholar] [CrossRef] [PubMed]
- Cortellessa, G.; Stabile, L.; Arpino, F.; Faleiros, D.E.; Van Den Bos, W.; Morawska, L.; Buonanno, G. Close proximity risk assessment for SARS-CoV-2 infection. Sci. Total. Environ. 2021, 794, 148749. [Google Scholar] [CrossRef] [PubMed]
- Abkarian, M.; Mendez, S.; Xue, N.; Yang, F.; Stone, H.A. Speech can produce jet-like transport relevant to asymptomatic spreading of virus. Proc. Natl. Acad. Sci. USA 2020, 117, 25237–25245. [Google Scholar] [CrossRef] [PubMed]
- Lieber, C.; Melekidis, S.; Koch, R.; Bauer, H.J. Insights into the evaporation characteristics of saliva droplets and aerosols: Levitation experiments and numerical modeling. J. Aerosol Sci. 2021, 154, 105760. [Google Scholar] [CrossRef]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
D’Alessandro, V.; Falone, M.; Giammichele, L.; Ricci, R. A Multi-Scale Approach for Modelling Airborne Transport of Mucosalivary Fluid. Appl. Sci. 2022, 12, 12381. https://doi.org/10.3390/app122312381
D’Alessandro V, Falone M, Giammichele L, Ricci R. A Multi-Scale Approach for Modelling Airborne Transport of Mucosalivary Fluid. Applied Sciences. 2022; 12(23):12381. https://doi.org/10.3390/app122312381
Chicago/Turabian StyleD’Alessandro, Valerio, Matteo Falone, Luca Giammichele, and Renato Ricci. 2022. "A Multi-Scale Approach for Modelling Airborne Transport of Mucosalivary Fluid" Applied Sciences 12, no. 23: 12381. https://doi.org/10.3390/app122312381
APA StyleD’Alessandro, V., Falone, M., Giammichele, L., & Ricci, R. (2022). A Multi-Scale Approach for Modelling Airborne Transport of Mucosalivary Fluid. Applied Sciences, 12(23), 12381. https://doi.org/10.3390/app122312381








