Dynamic Modeling and Nonlinear Analysis of a Spur Gear System Considering a Nonuniformly Distributed Meshing Force
Abstract
1. Introduction
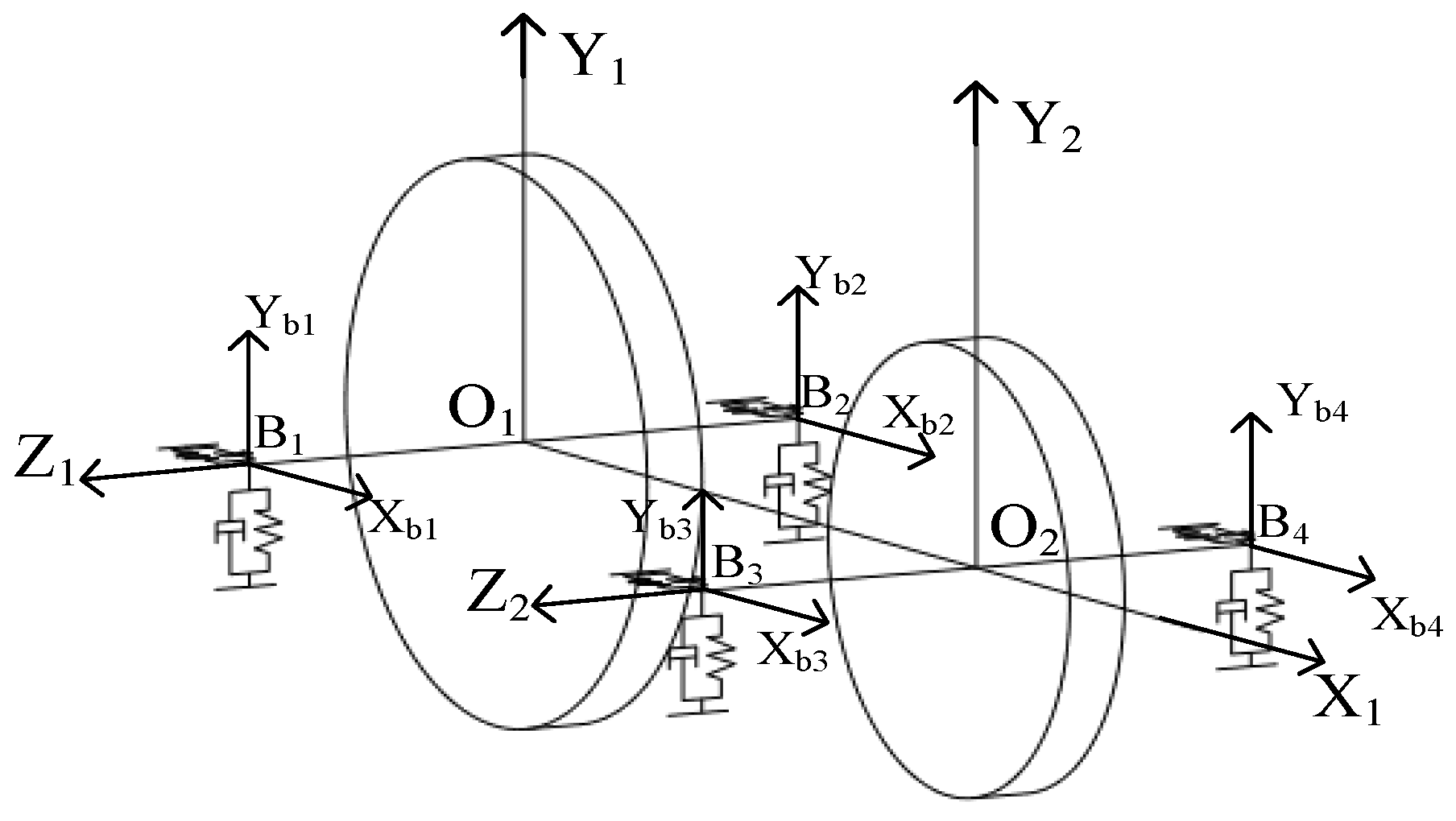
2. Nonlinear Dynamic Modeling of Spur Gear Systems
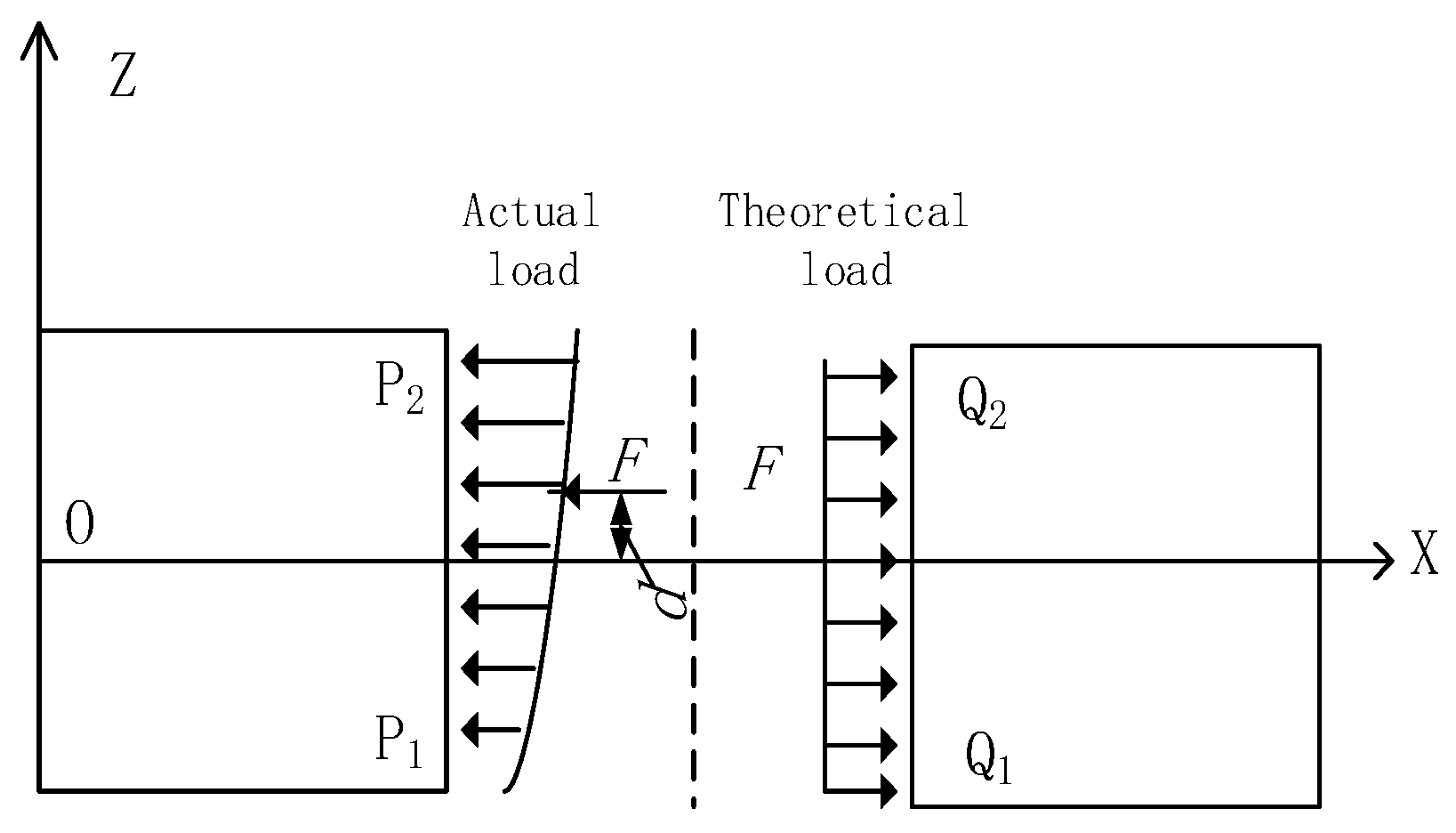
2.1. Nonuniformly Distributed Meshing Force
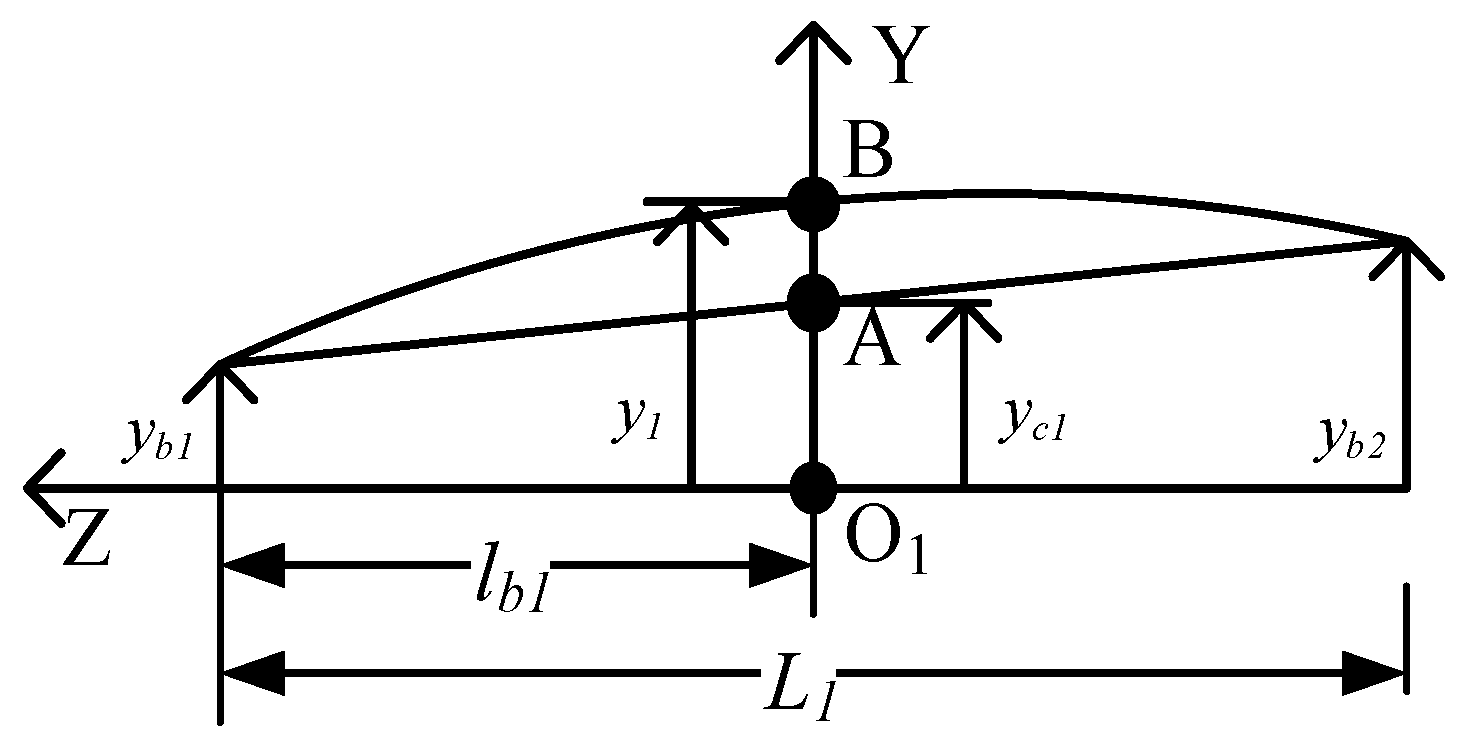
2.2. Bending Deformation of Driveshafts
2.3. Dynamic Center Distance
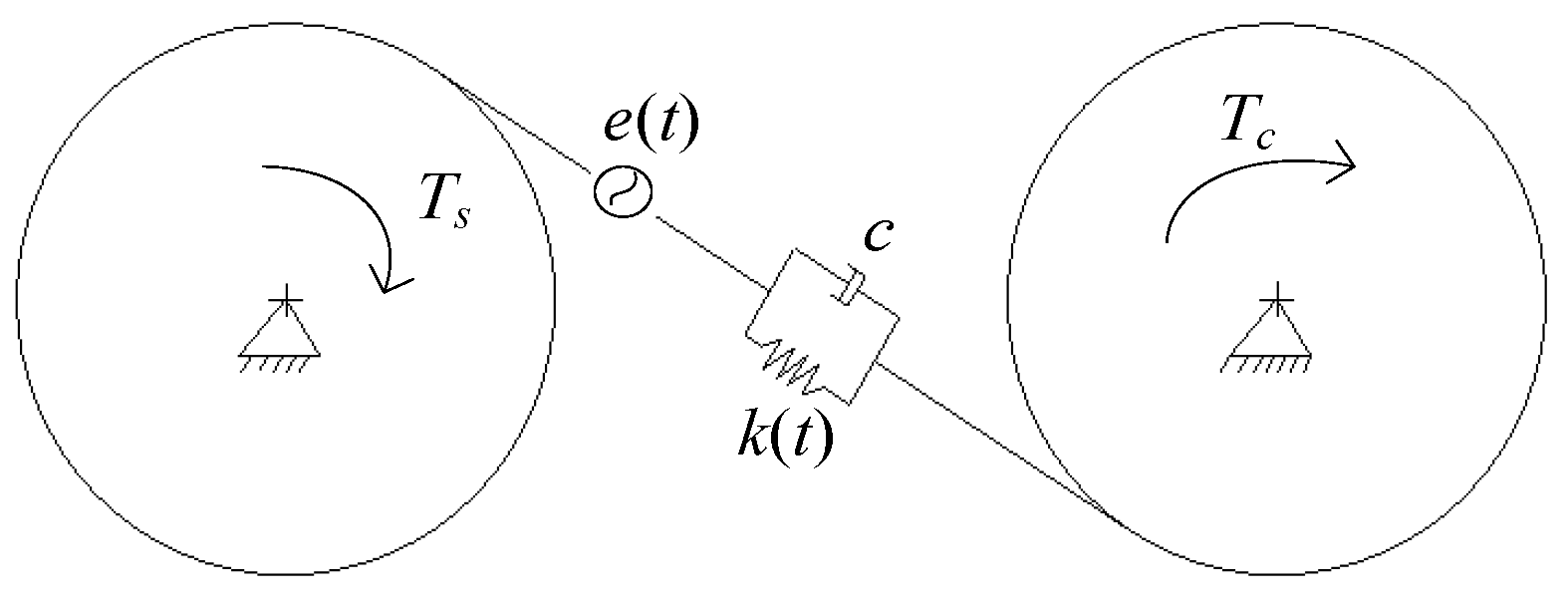
2.4. Time-Varying Meshing Stiffness
2.5. Derivation of the Dynamic Model
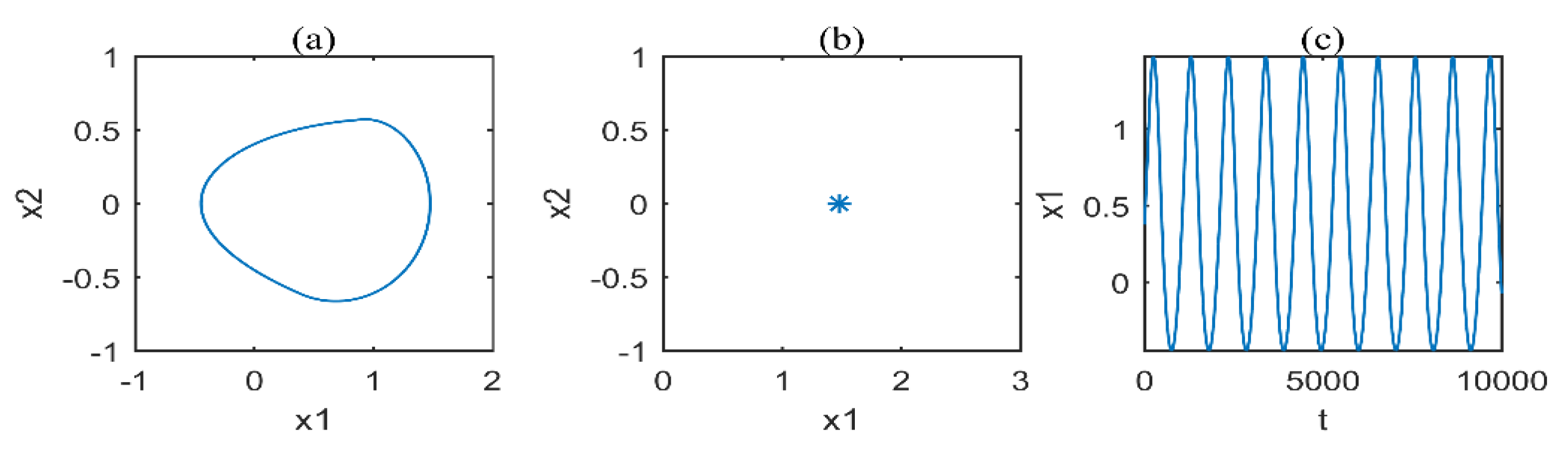
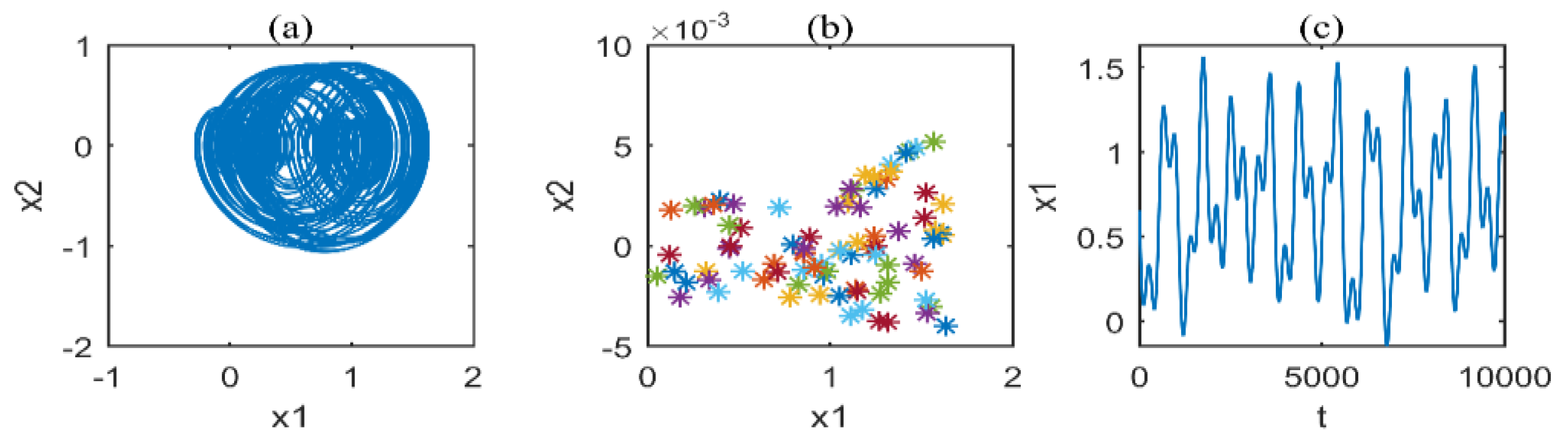
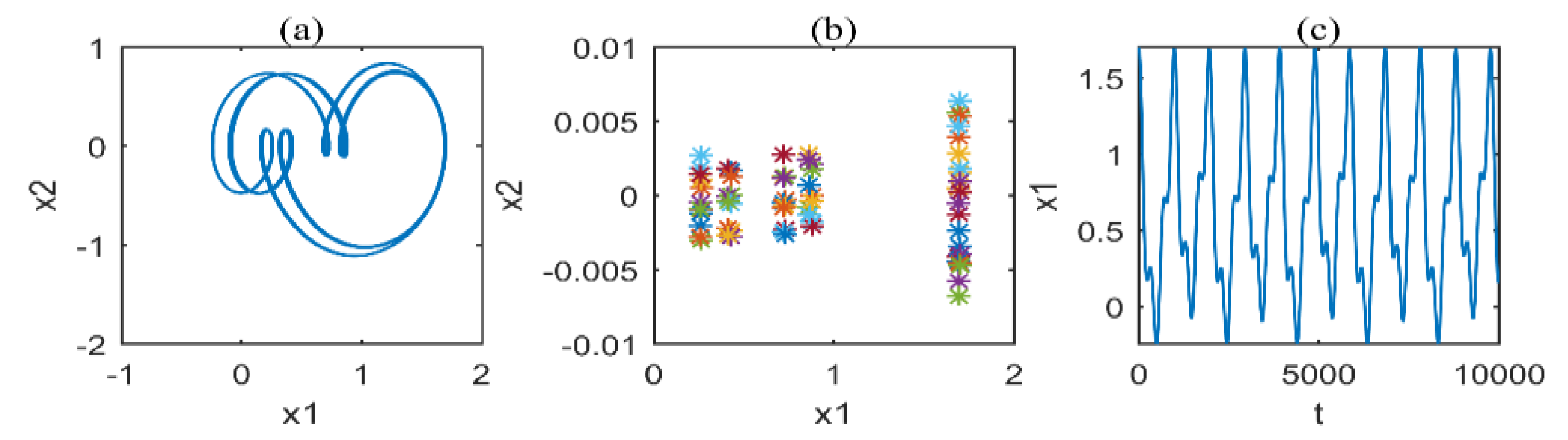
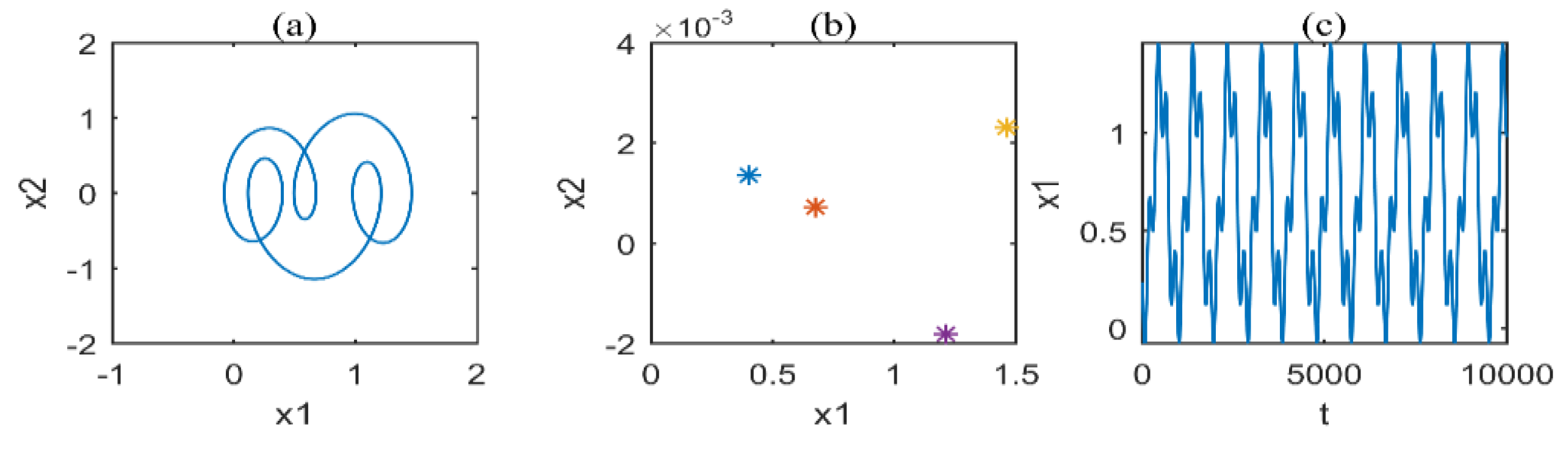
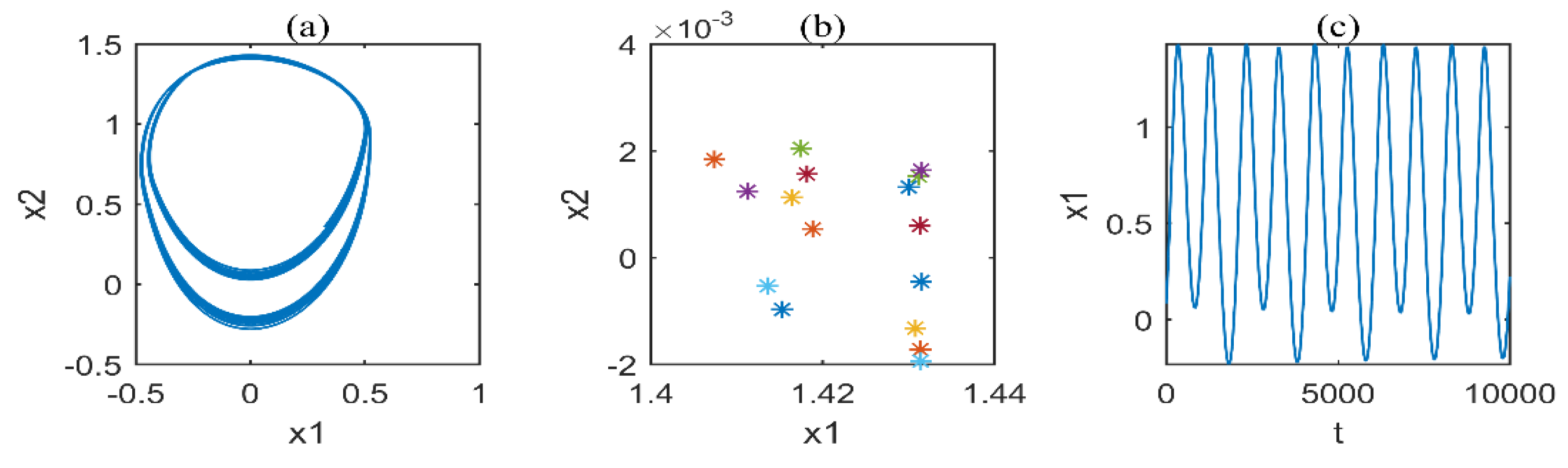
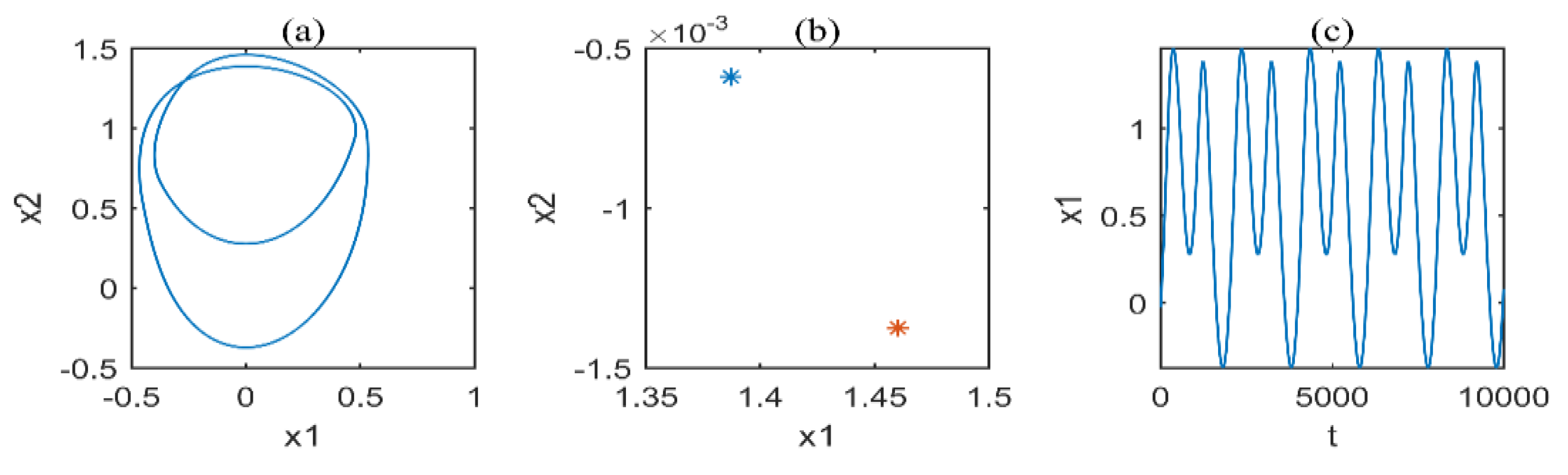
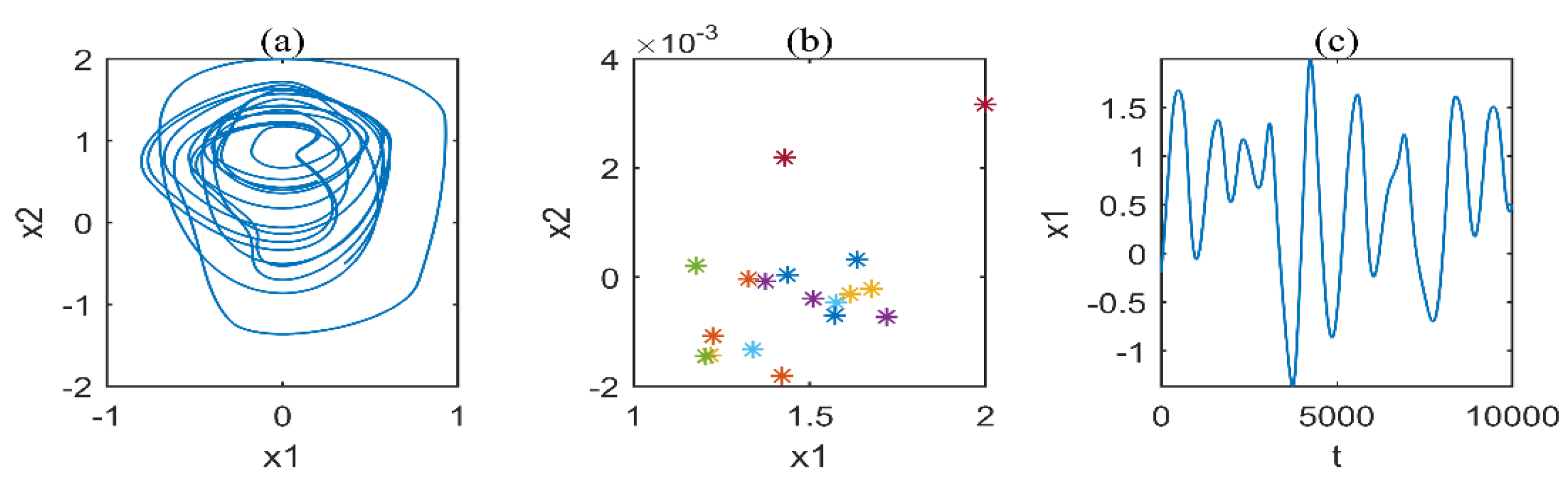
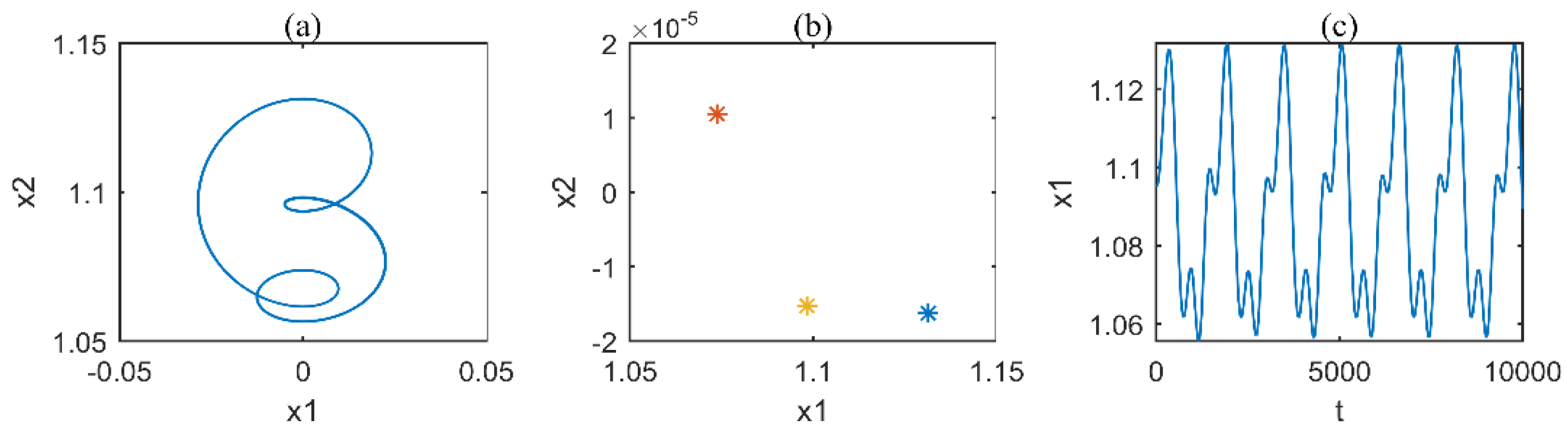
3. Study of the Nonlinear Dynamic Characteristics of Gear System
3.1. Influence of Meshing Frequency
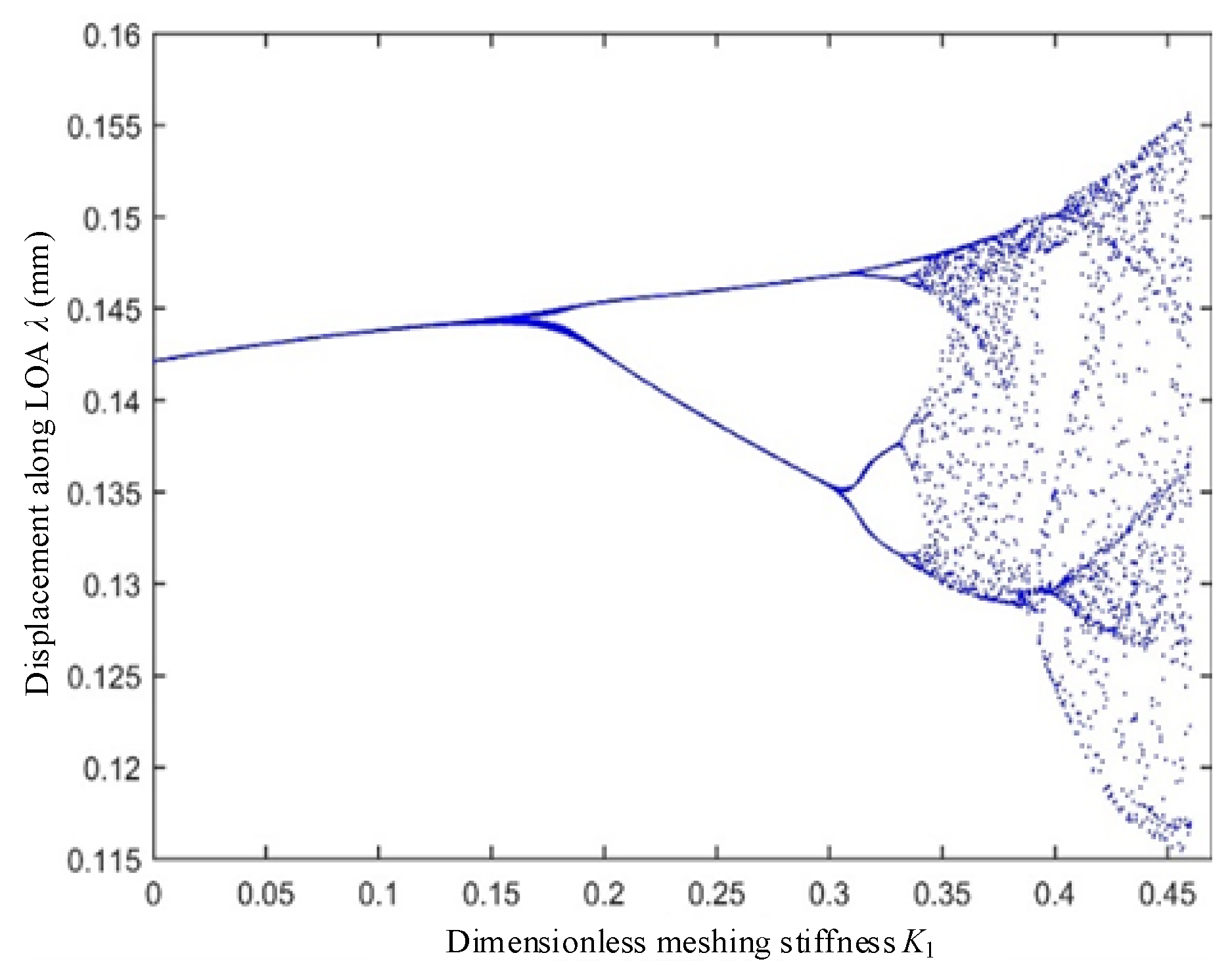
3.2. Influence of Stiffness Excitation
3.2.1. Analysis of Time-Varying Meshing Stiffness
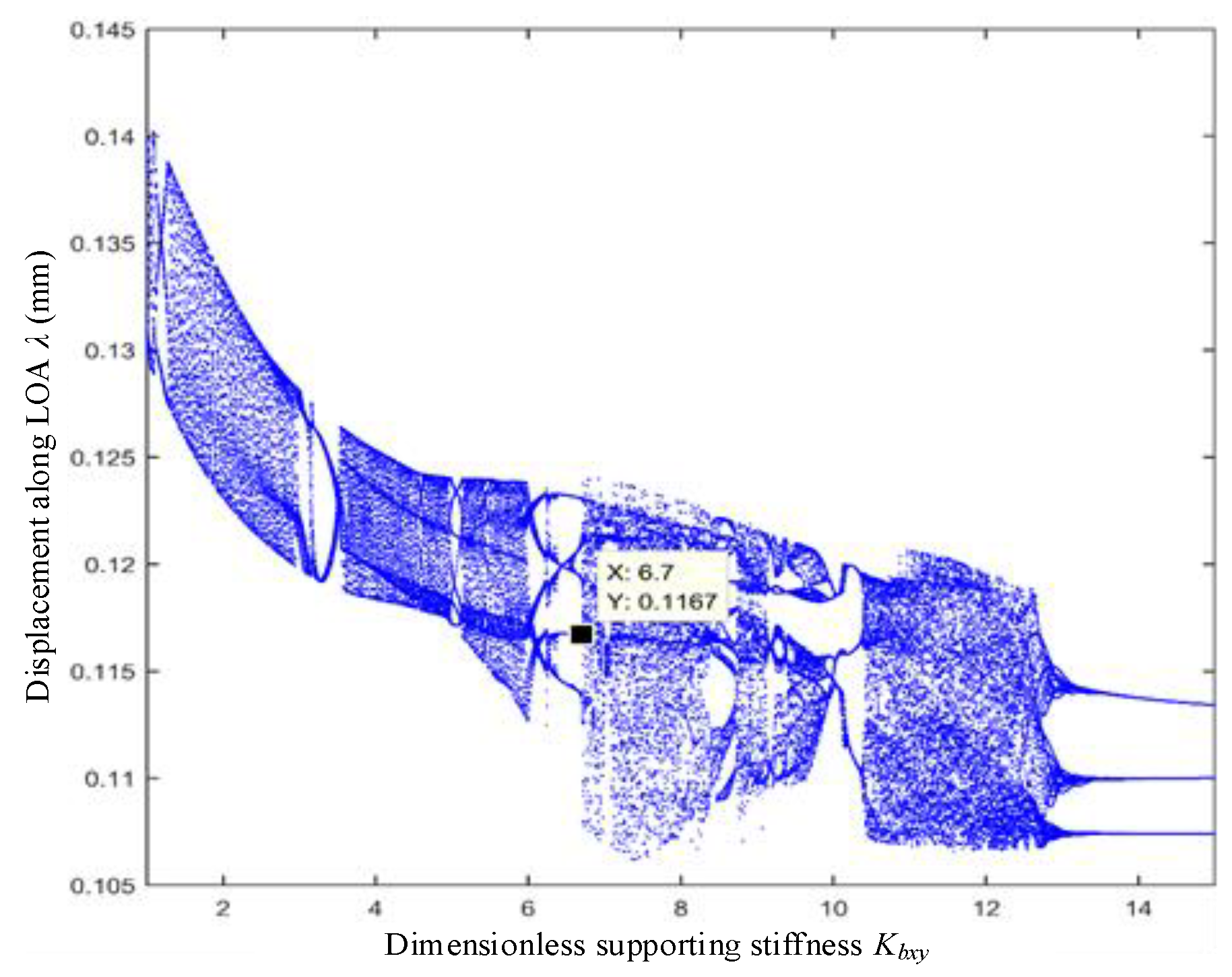
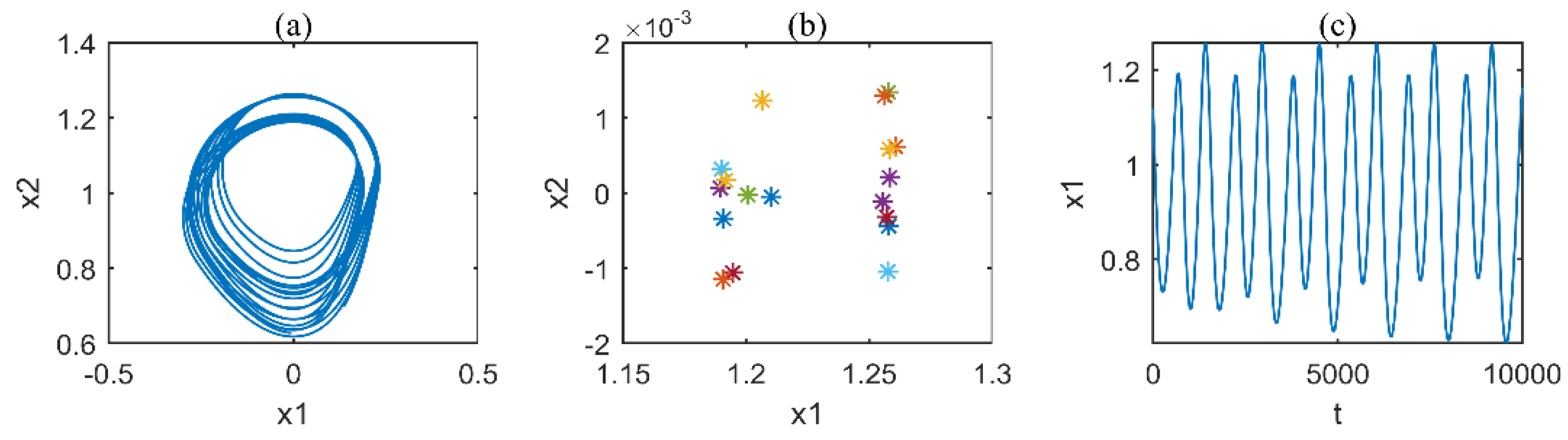
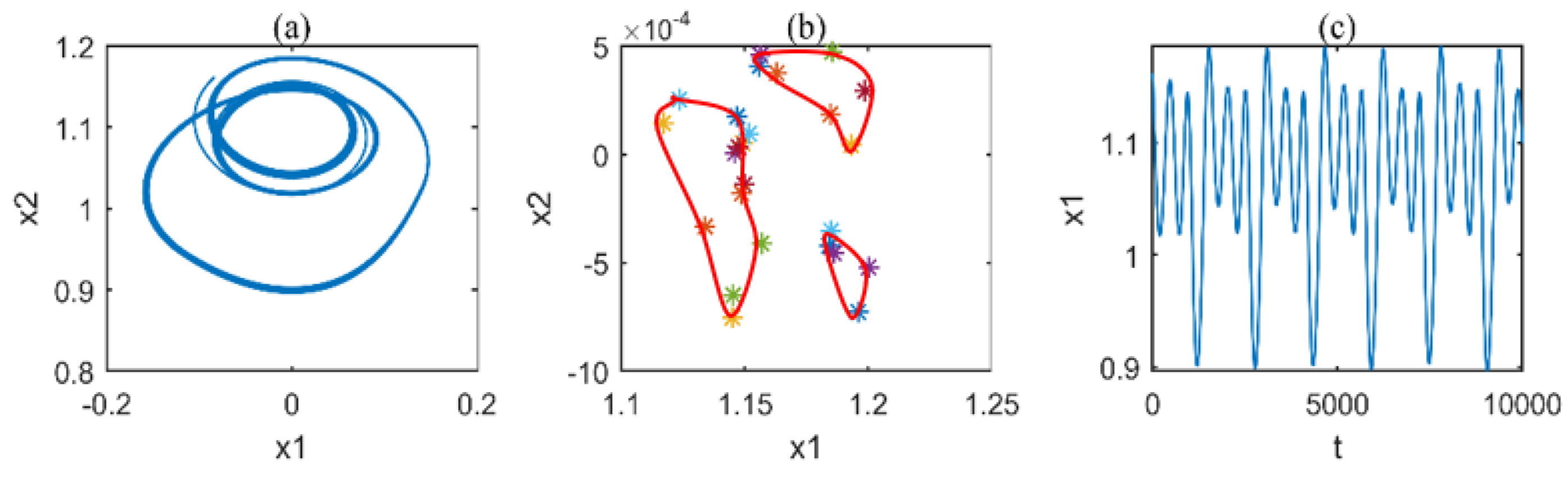
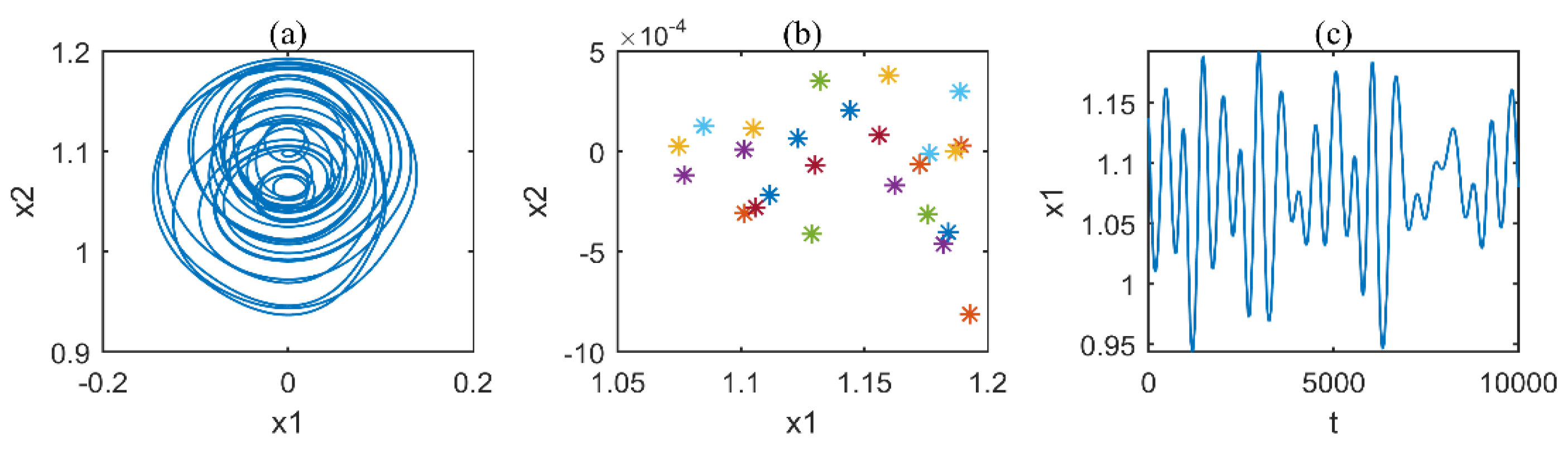
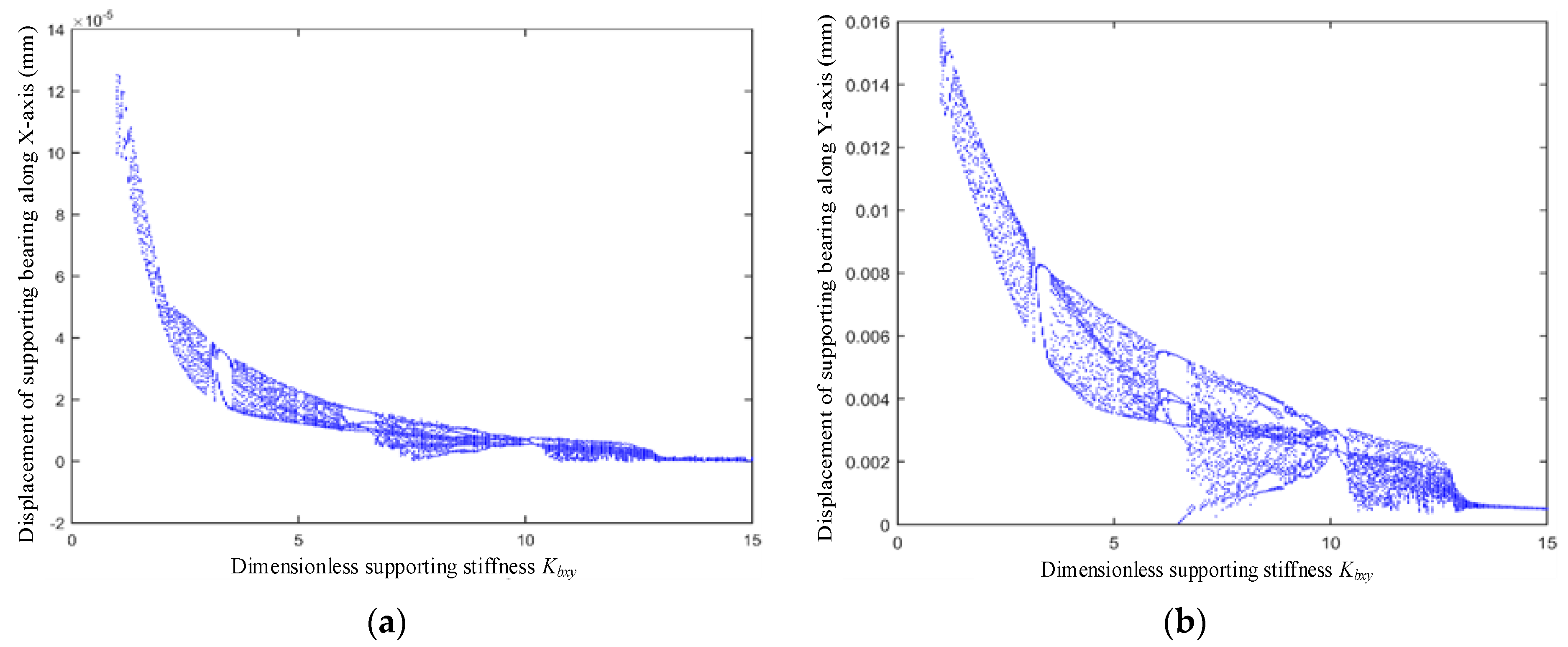
3.2.2. Analysis of Supporting Stiffness
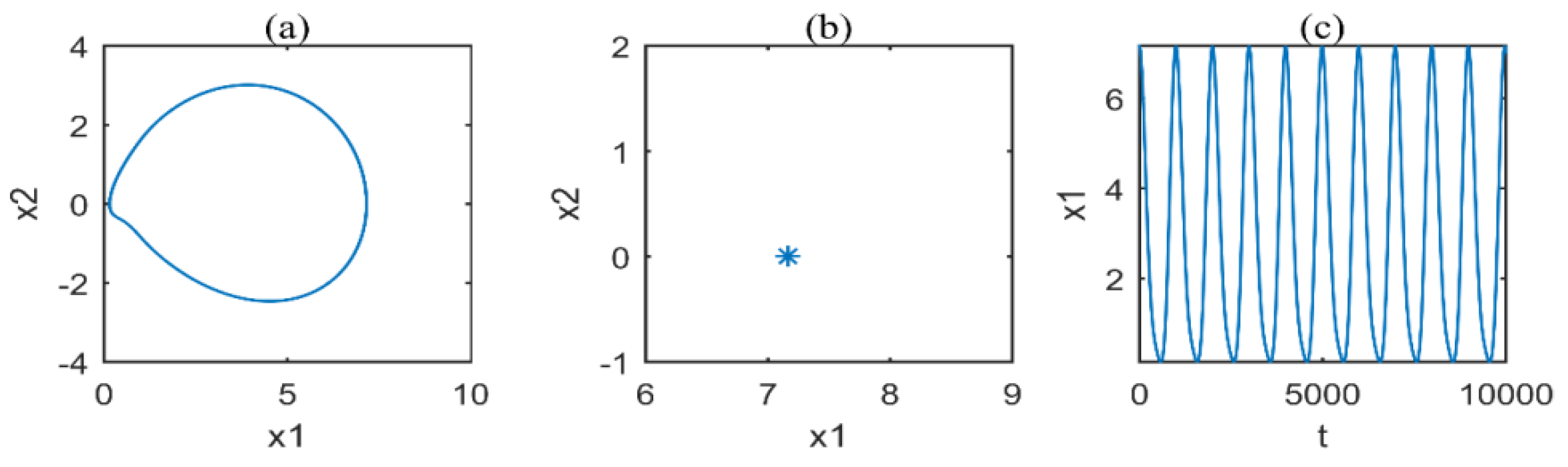
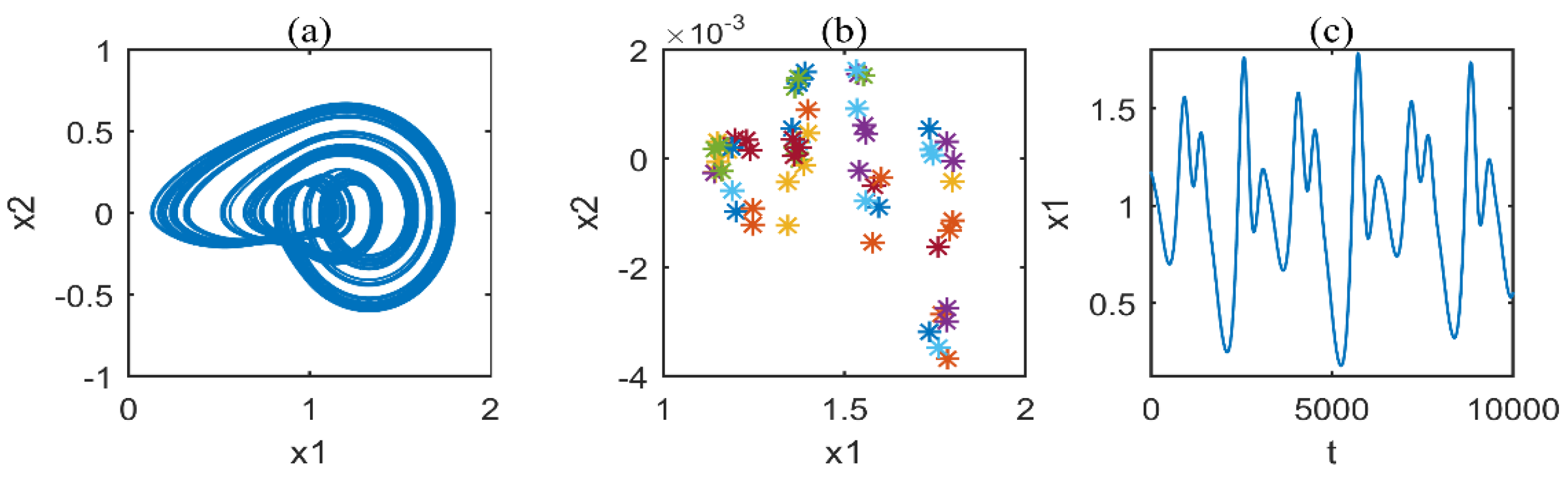
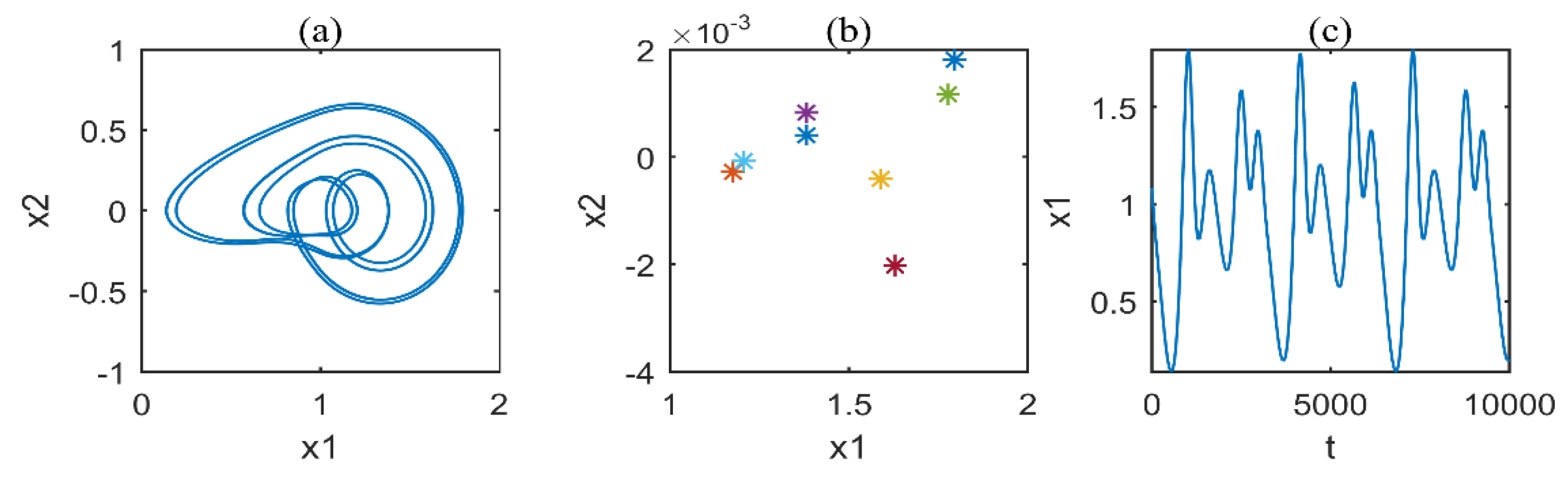
3.3. Influence of Damping
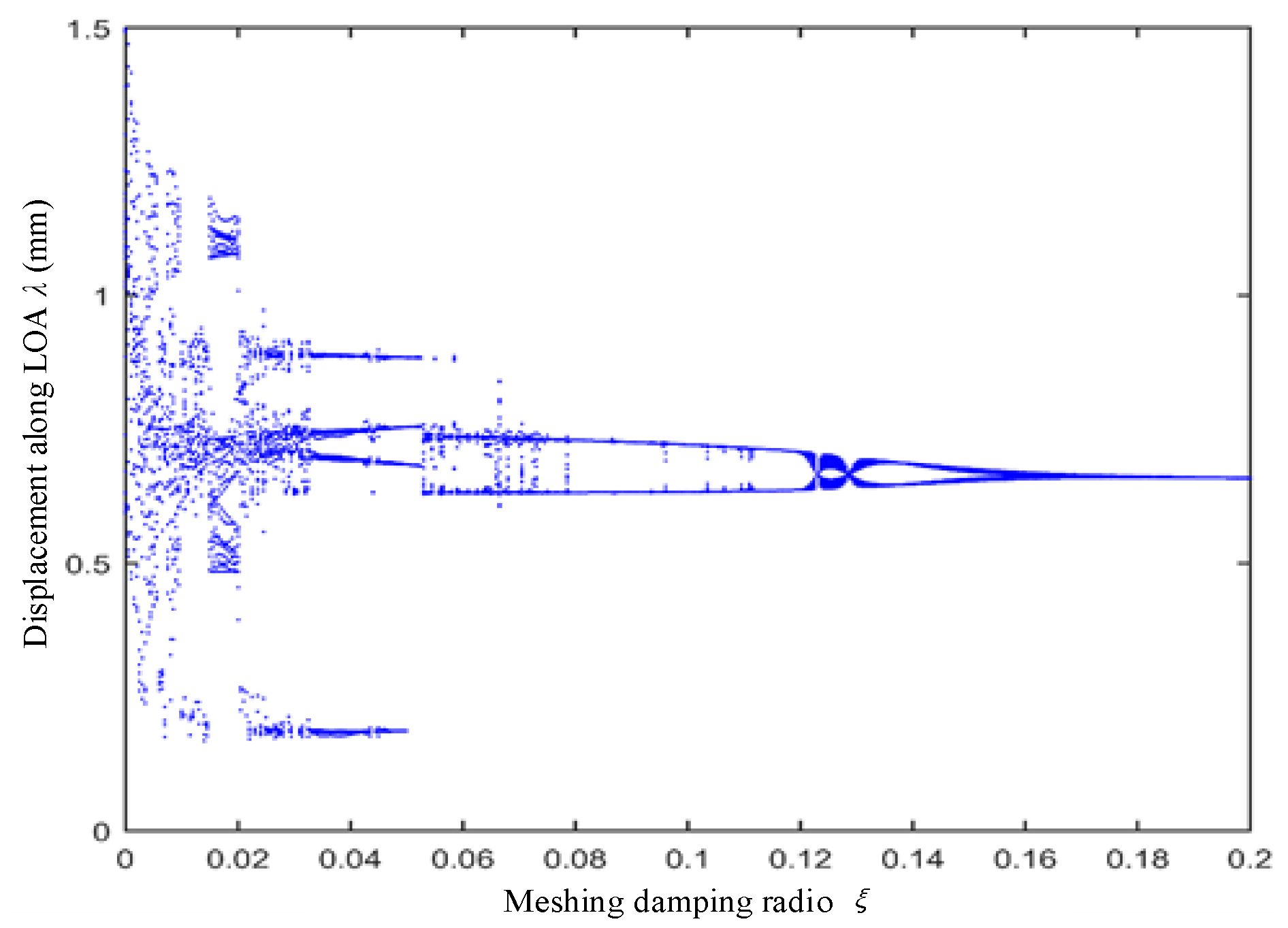
3.3.1. Analysis of Meshing Damping
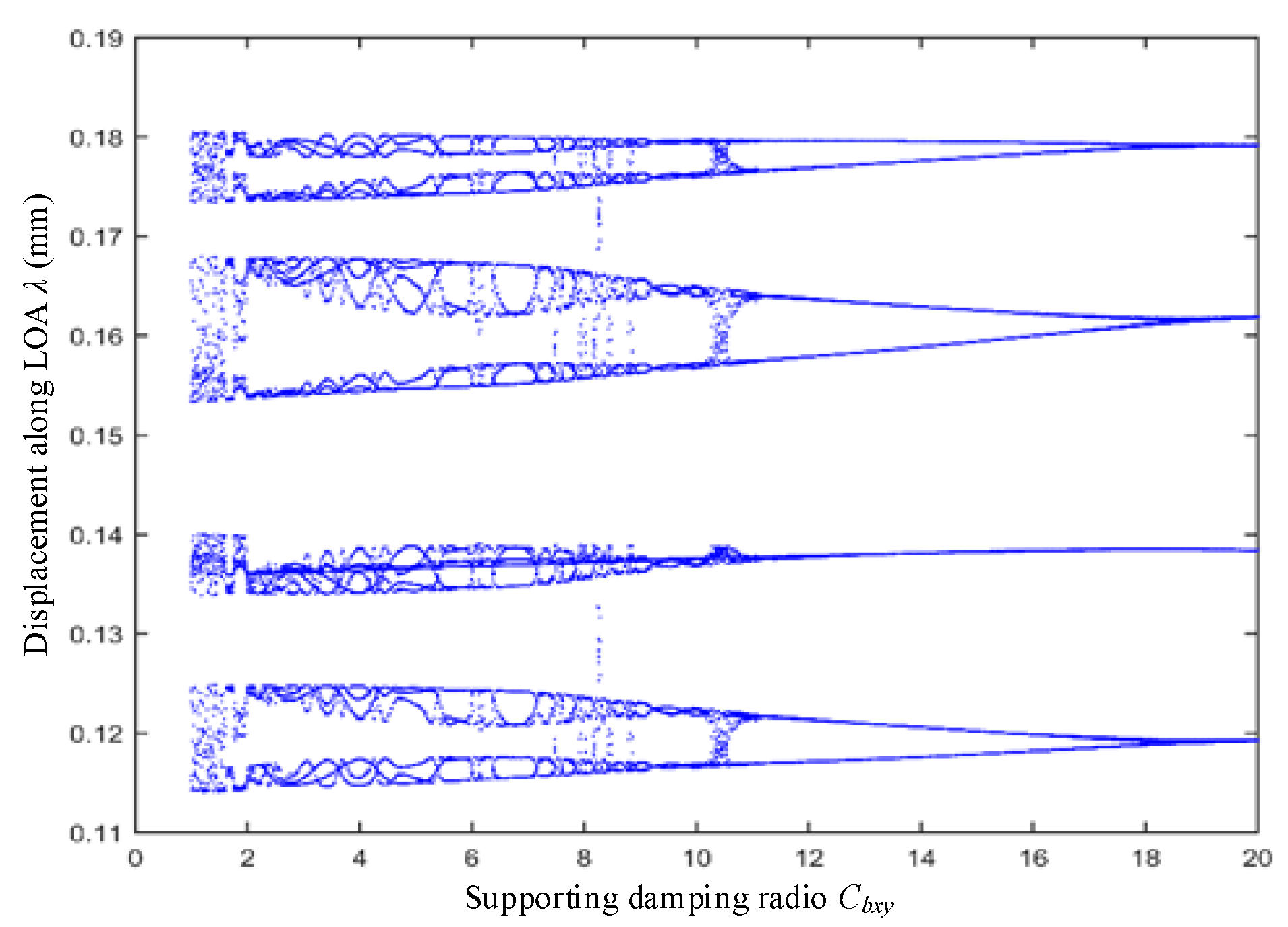
3.3.2. Analysis of Supporting Damping
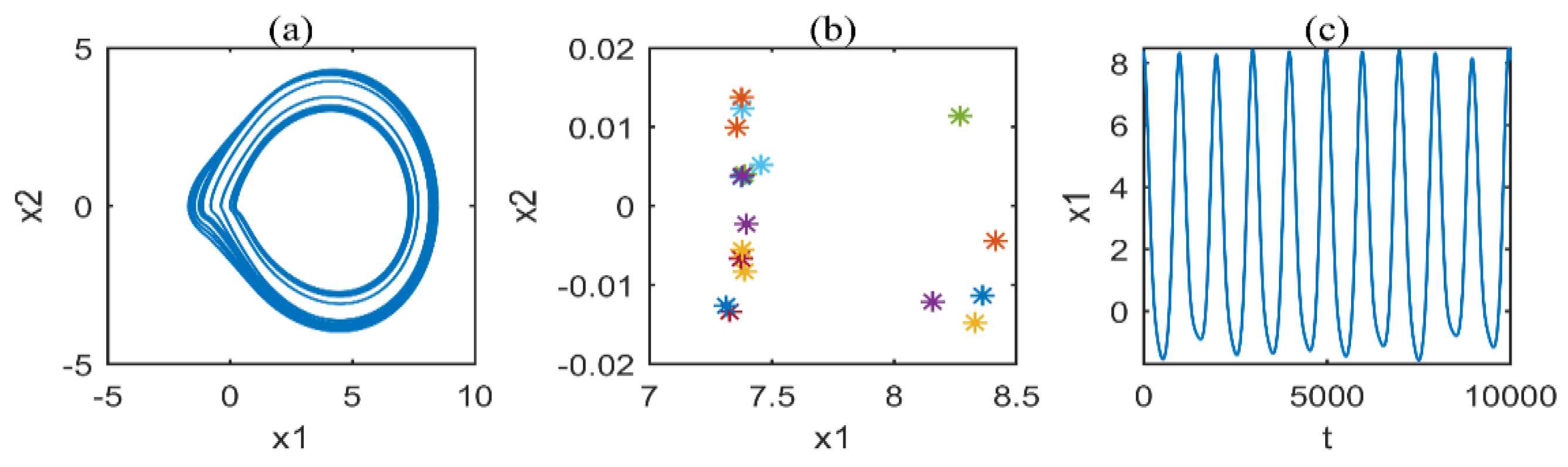
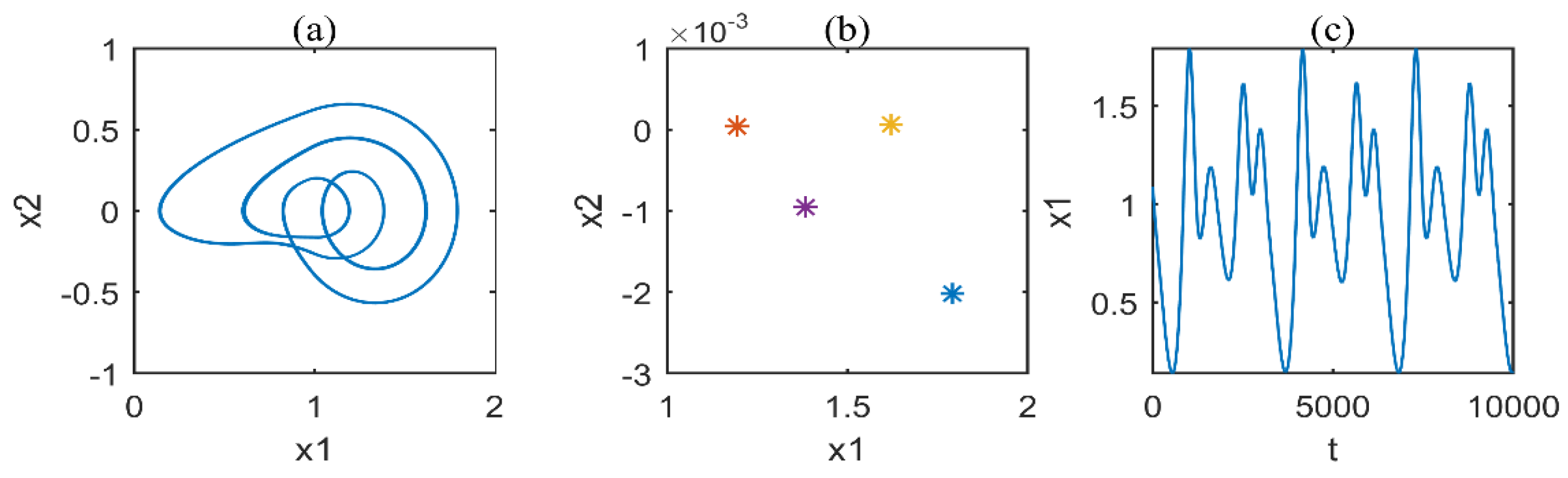
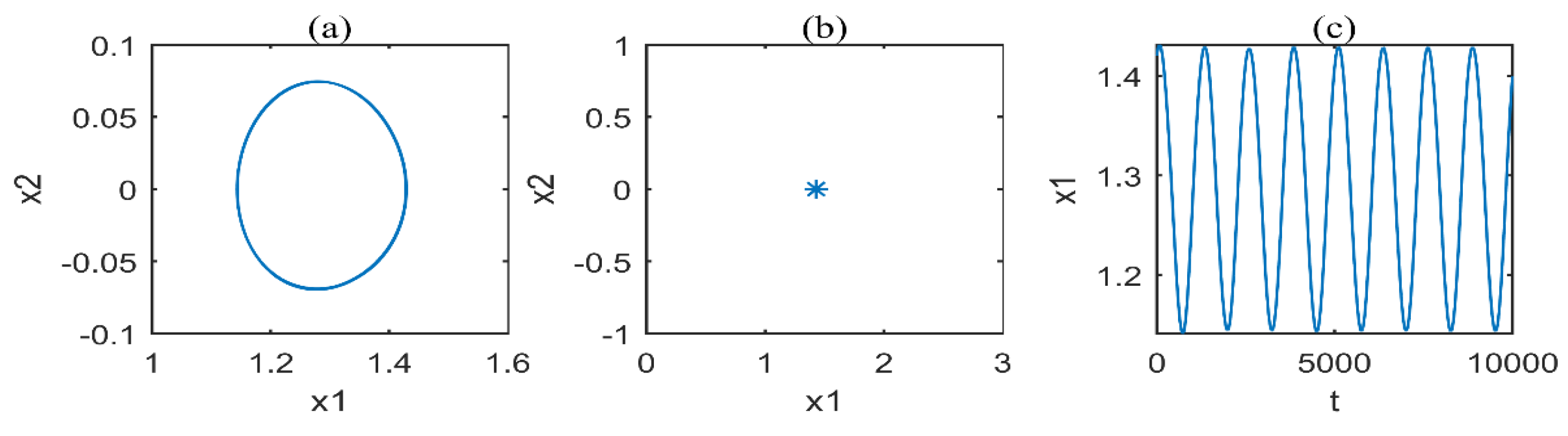
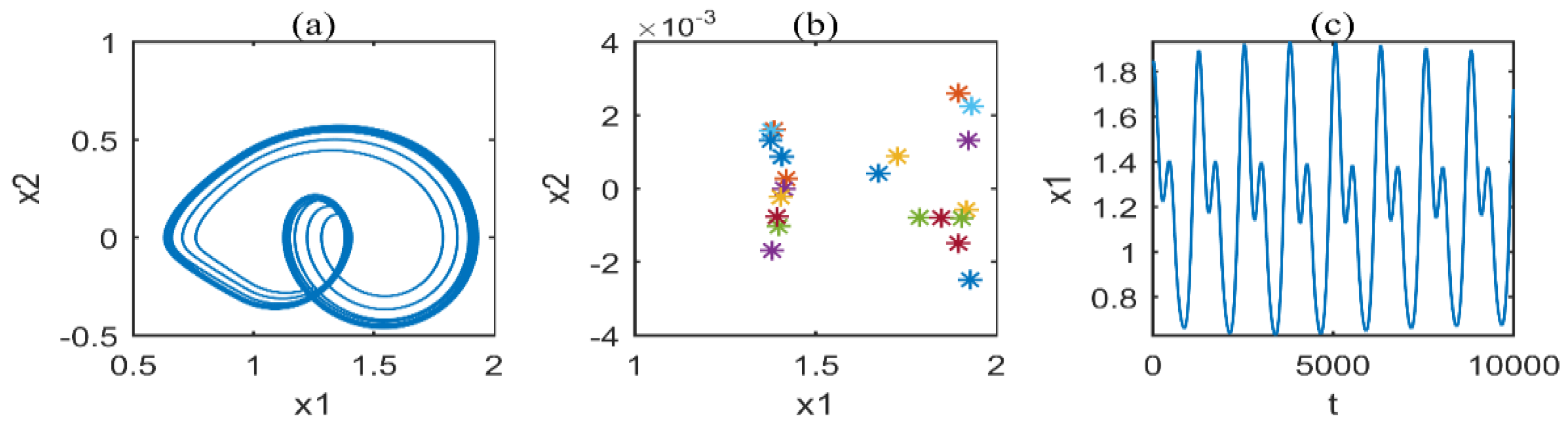
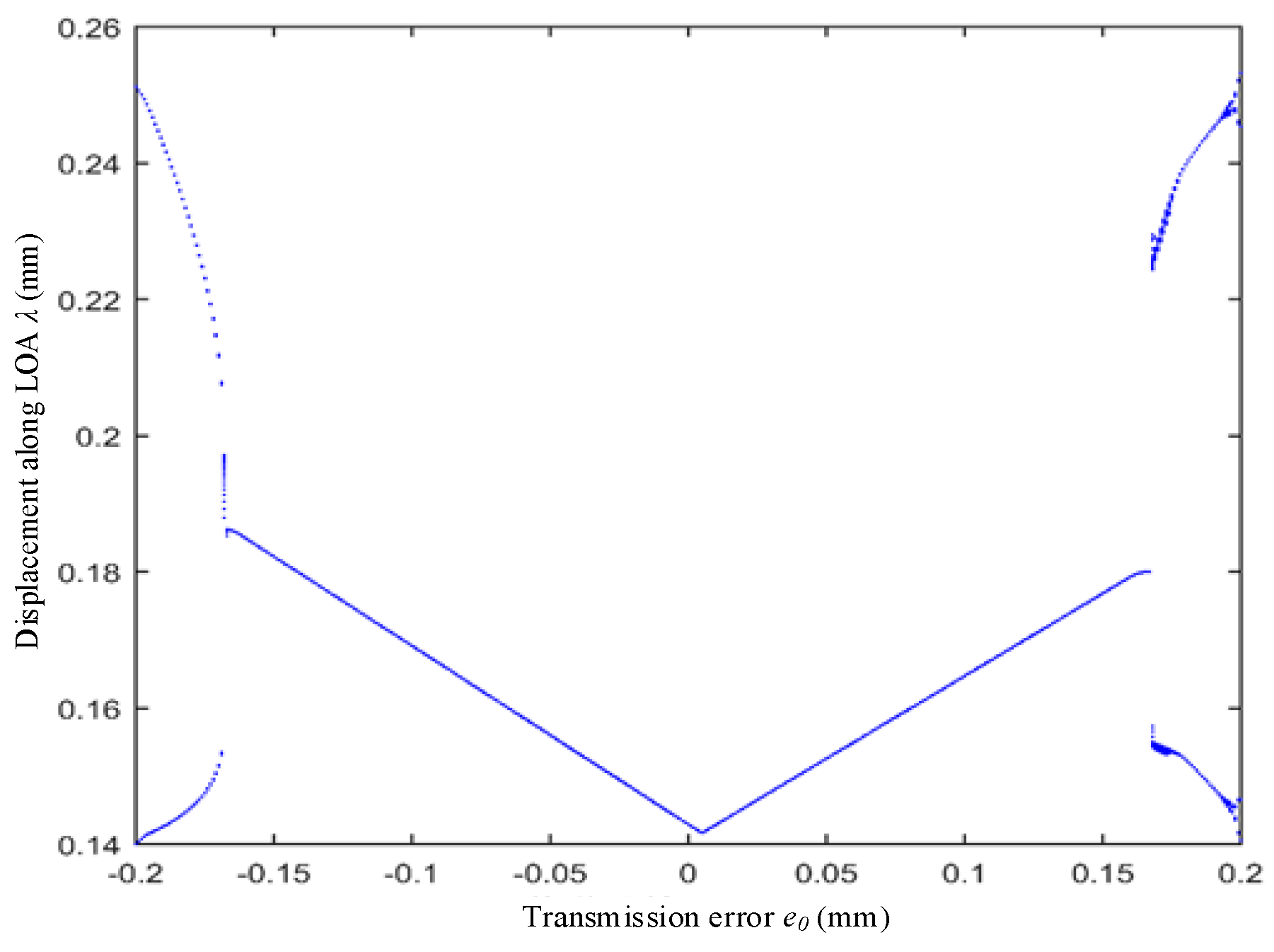
3.4. Influence of Error Excitation
4. Conclusions
- (1)
- The proposed dynamic modeling method of the spur gear system considers a nonuniformly distributed meshing force, dynamic center distance, shaft bending deformation, and time-varying stiffness excitation. This can better reflect the actual dynamic characteristics of a gear system in complicated working situations;
- (2)
- When the meshing frequency is close to n/2 times the system’s natural frequency, resonance will appear. An excessive meshing stiffness amplitude will cause the system to fall into a chaotic motion state, while a large supporting stiffness will improve the system’s stability. Increasing meshing damping and supporting damping can improve system stability and decrease the vibration response. Moreover, the gear system’s dynamic response is sensitive to transmission error when subjected to a light load. In order to evade the bifurcation points and the chaotic motion, the transmission error should be decreased by increasing manufacturing accuracy and assembly accuracy;
- (3)
- Traditionally, a gear system is designed in terms of strength theory, characterized by material selection, force analysis, stiffness verification, and fatigue-strength design. For gear systems that are used in complicated working conditions, design should be considered not in terms of only structural strength but also in terms of motion stability. By evading complicated nonlinear dynamic behaviors, such as bifurcation and chaos, the transmission performance of a gear system can effectively be improved.
Author Contributions
Funding
Conflicts of Interest
References
- Ren, Z.H.; Li, J.L.; Wang, K.; Zhou, S.H. Nonlinear dynamic analysis of a coupled lateral-torsional spur gear with eccentricity. J. Vibroeng. 2016, 18, 4776–4791. [Google Scholar] [CrossRef]
- Han, Q.; Chu, F. Dynamic behaviors of a geared rotor system under time-periodic base angular motions. Mech. Mach. Theory 2014, 78, 1–14. [Google Scholar] [CrossRef]
- Yang, J. Vibration analysis on multi-mesh gear-trains under combined deterministic and random excitations. Mech. Mach. Theory 2013, 59, 20–33. [Google Scholar] [CrossRef]
- Zhang, H.; Wu, S.; Peng, Z. A nonlinear dynamic model for analysis of the combined influences of nonlinear internal excitations on the load sharing behavior of a compound planetary gear set. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2016, 230, 1048–1068. [Google Scholar] [CrossRef]
- Cooley, C.G.; Parker, R.G. A Review of Planetary and Epicyclic Gear Dynamics and Vibrations Research. Appl. Mech. Rev. 2014, 66, 040804. [Google Scholar] [CrossRef]
- Omar, F.K.; Moustafa, K.A.F.; Emam, S. Mathematical modeling of gearbox including defects with experimental verification. J. Vib. Control 2012, 18, 1310–1321. [Google Scholar] [CrossRef]
- Liu, P.F.; Zhu, L.Y.; Gou, X.F.; Shi, J.F.; Jin, G.G. Modeling and analyzing of nonlinear dynamics for spur gear pair with pitch deviation under multi-state meshing. Mech. Mach. Theory 2021, 163, 104378. [Google Scholar] [CrossRef]
- Zhang, C.; Liu, X.; Shi, X.; Ling, X. Research on nonlinear dynamic model of spur gear pair. J. Phys. Conf. Ser. 2021, 1820, 012038. [Google Scholar] [CrossRef]
- He, Z.; Tang, W.; Sun, S. A model for analysis of time-varying mesh stiffness of helical gears with misalignment errors. Trans. Famena 2021, 45, 59–73. [Google Scholar] [CrossRef]
- Yang, X.; Song, C.; Zhu, C.; Liang, C.; Sun, R. Impacts of Misalignments on Mesh Behaviors of Face-Hobbed Hypoid Gear Considering System Deformation. IEEE Access 2019, 7, 79244–79253. [Google Scholar] [CrossRef]
- Shi, Z.; Lim, T.C. Effect of Shaft Misalignment on Hypoid Gear Pair Driven Through a Universal Joint. Int. J. Automot. Technol. 2020, 21, 371–383. [Google Scholar] [CrossRef]
- Zhou, C.; Wang, Q.; Gui, L.; Fan, Z. A numerical method for calculating the misalignments of planetary gears. Proc. Inst. Mech. Eng. Part D-J. Automob. Eng. 2019, 233, 2624–2636. [Google Scholar] [CrossRef]
- Zhang, H.; Wei, G.; Wen, B.; Han, Q.; Hao, H. Meshing characteristics of geared rotor system in integrally geared compressor with unbalance excitation. J. Vib. Control 2019, 25, 26–40. [Google Scholar] [CrossRef]
- Zhou, W.-j.; Wei, X.-s.; Wei, X.-z.; Wang, L.-q. Numerical analysis of a nonlinear double disc rotor-seal system. J. Zhejiang Univ. Sci. A 2014, 15, 39–52. [Google Scholar] [CrossRef]
- Wang, L.; Zhou, W.; Wei, X.; Zhai, L.; Wu, G. A coupling vibration model of multi-stage pump rotor system based on FEM. Mechanika 2016, 22, 31–37. [Google Scholar] [CrossRef]
- Lin, T.; He, Z. Analytical method for coupled transmission error of helical gear system with machining errors, assembly errors and tooth modifications. Mech. Syst. Signal Process. 2017, 91, 167–182. [Google Scholar] [CrossRef]
- Xu, Z.; Deng, H.; Zhang, Y. Piecewise Nonlinear Dynamic Modeling for Gear Transmissions with Rotary Inertia and Backlash. IEEE Access 2019, 7, 176495–176503. [Google Scholar] [CrossRef]
- Brancati, R.; Rocca, E.; Siano, D.; Viscardi, M. Experimental vibro-acoustic analysis of the gear rattle induced by multi-harmonic excitation. Proc. Inst. Mech. Eng. Part D J. Automob. Eng. 2018, 232, 785–796. [Google Scholar] [CrossRef]
- Zhang, R.; Wang, K.; Shi, Y.; Sun, X.; Gu, F.; Wang, T. The Influences of Gradual Wears and Bearing Clearance of Gear Transmission on Dynamic Responses. Energies 2019, 12, 4731. [Google Scholar] [CrossRef]
- Wang, J.; He, G.; Zhang, J.; Zhao, Y.; Yao, Y. Nonlinear dynamics analysis of the spur gear system for railway locomotive. Mech. Syst. Signal Process. 2017, 85, 41–55. [Google Scholar] [CrossRef]
- Xiang, L.; Zhang, Y.; Gao, N.; Hu, A.; Xing, J. Nonlinear Dynamics of a Multistage Gear Transmission System with Multi-Clearance. Int. J. Bifurc. Chaos 2018, 28, 1850034. [Google Scholar] [CrossRef]
- Xiang, L.; Gao, N.; Hu, A. Dynamic analysis of a planetary gear system with multiple nonlinear parameters. J. Comput. Appl. Math. 2018, 327, 325–340. [Google Scholar] [CrossRef]
- Gu, Y.-K.; Li, W.-F.; Zhang, J.; Qiu, G.-Q. Effects of Wear, Backlash, and Bearing Clearance on Dynamic Characteristics of a Spur Gear System. IEEE Access 2019, 7, 117639–117651. [Google Scholar] [CrossRef]
- Geng, Z.; Xiao, K.; Wang, J.; Li, J. Investigation on Nonlinear Dynamic Characteristics of a New Rigid-Flexible Gear Transmission with Wear. J. Vib. Acoust. 2019, 141, 051008. [Google Scholar] [CrossRef]
- Yang, Y.; Li, H.; Dai, Y. Nonlinear vibration characteristics of spur gear system subjected to multiple harmonic excitations. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2019, 233, 6026–6050. [Google Scholar] [CrossRef]
- Huang, K.; Yi, Y.; Xiong, Y.; Cheng, Z.; Chen, H. Nonlinear dynamics analysis of high contact ratio gears system with multiple clearances. J. Braz. Soc. Mech. Sci. Eng. 2020, 42, 98. [Google Scholar] [CrossRef]












| Parameter | Driving Gear | Driven Gear |
|---|---|---|
| Teeth number z | 30 | 36 |
| Mass (g) | 600 | 850 |
| Inertia torque () | 600 | 1200 |
| Modulus (mm) | 3 | |
| Pressure angle () | 20 | |
| Face width (mm) | 12 | |
| Average meshing stiffness k0 (N/mm) | 2.39 × 105 | |
| Tooth side clearance 2b (mm) | 0.1 | |
| Related Works in the Literature Review | Our Work |
|---|---|
| Bending-torsion dynamic model | Bending-torsion-swing dynamic model |
| Uniformly distributed meshing force | Nonuniformly distributed meshing force |
| 6-DOF | 18-DOF |
| Weak coupling of uniformly distributed meshing force, shaft deformation, and dynamic center distance | Strong coupling of nonuniformly distributed meshing force, shaft deformation, and dynamic center distance |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jin, B.; Bian, Y.; Liu, X.; Gao, Z. Dynamic Modeling and Nonlinear Analysis of a Spur Gear System Considering a Nonuniformly Distributed Meshing Force. Appl. Sci. 2022, 12, 12270. https://doi.org/10.3390/app122312270
Jin B, Bian Y, Liu X, Gao Z. Dynamic Modeling and Nonlinear Analysis of a Spur Gear System Considering a Nonuniformly Distributed Meshing Force. Applied Sciences. 2022; 12(23):12270. https://doi.org/10.3390/app122312270
Chicago/Turabian StyleJin, Bohan, Yushu Bian, Xihui Liu, and Zhihui Gao. 2022. "Dynamic Modeling and Nonlinear Analysis of a Spur Gear System Considering a Nonuniformly Distributed Meshing Force" Applied Sciences 12, no. 23: 12270. https://doi.org/10.3390/app122312270
APA StyleJin, B., Bian, Y., Liu, X., & Gao, Z. (2022). Dynamic Modeling and Nonlinear Analysis of a Spur Gear System Considering a Nonuniformly Distributed Meshing Force. Applied Sciences, 12(23), 12270. https://doi.org/10.3390/app122312270





