Analysis and Design of Tunable THz 1-D Leaky-Wave Antennas Based on Nematic Liquid Crystals
Abstract
1. Introduction
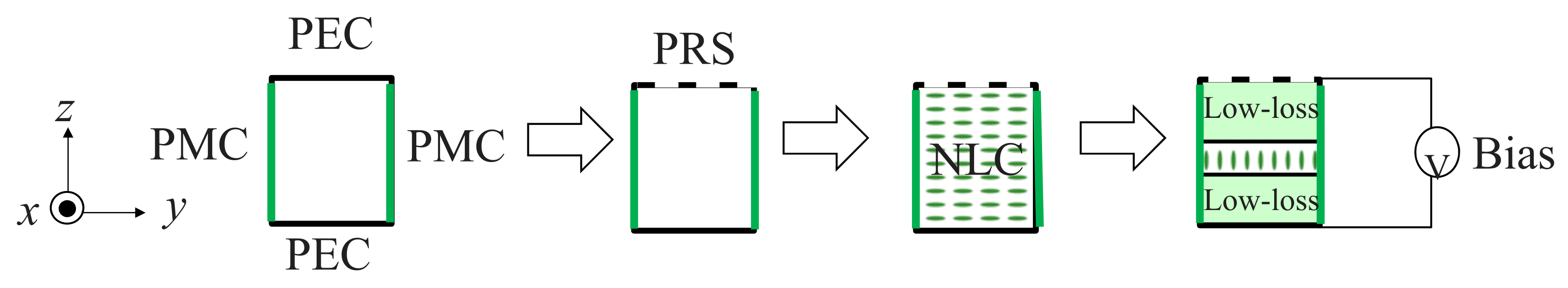
2. Theoretical Analysis
2.1. Closed Waveguide
2.2. Open Leaky Waveguide
2.3. Tunable Leaky Waveguide Filled with NLC
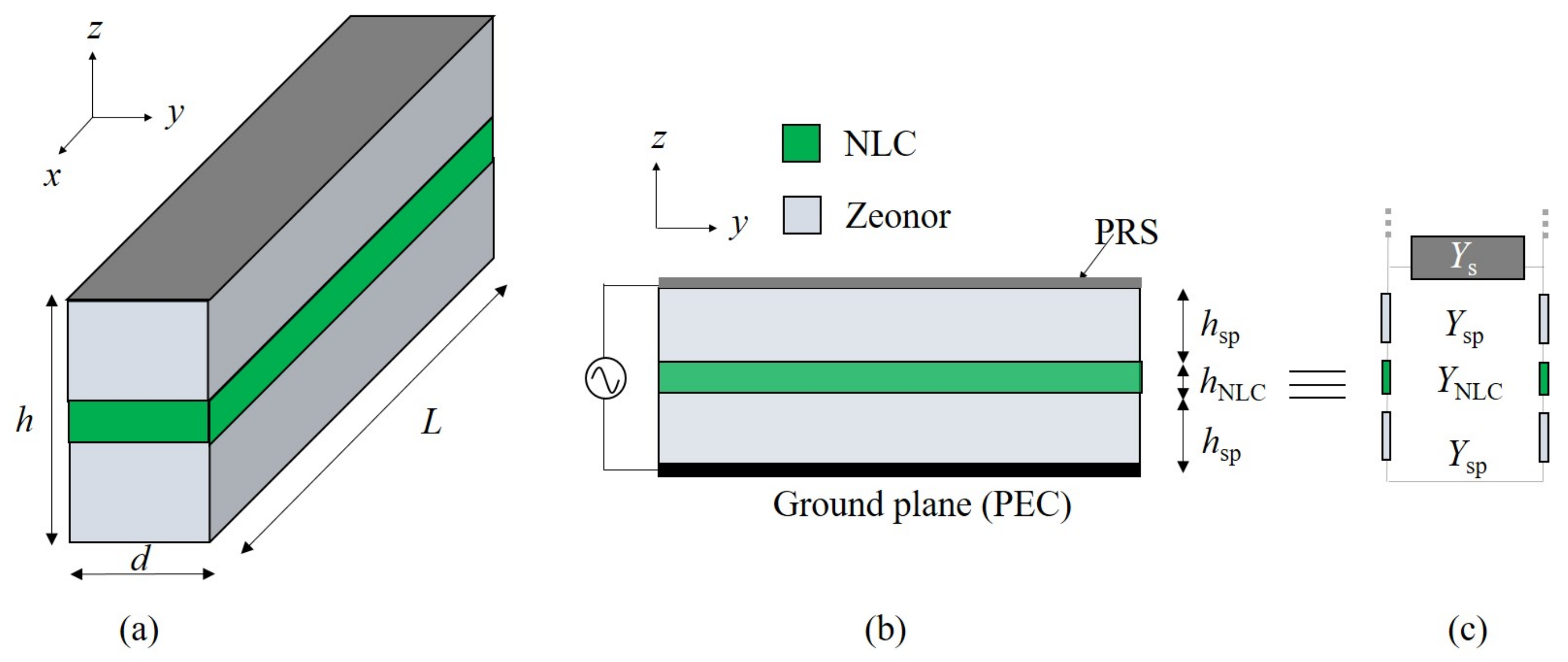
2.4. Tunable Leaky Waveguide Partially Filled with NLC
3. Optimization and Design
3.1. Optimization of the Ideal Structure
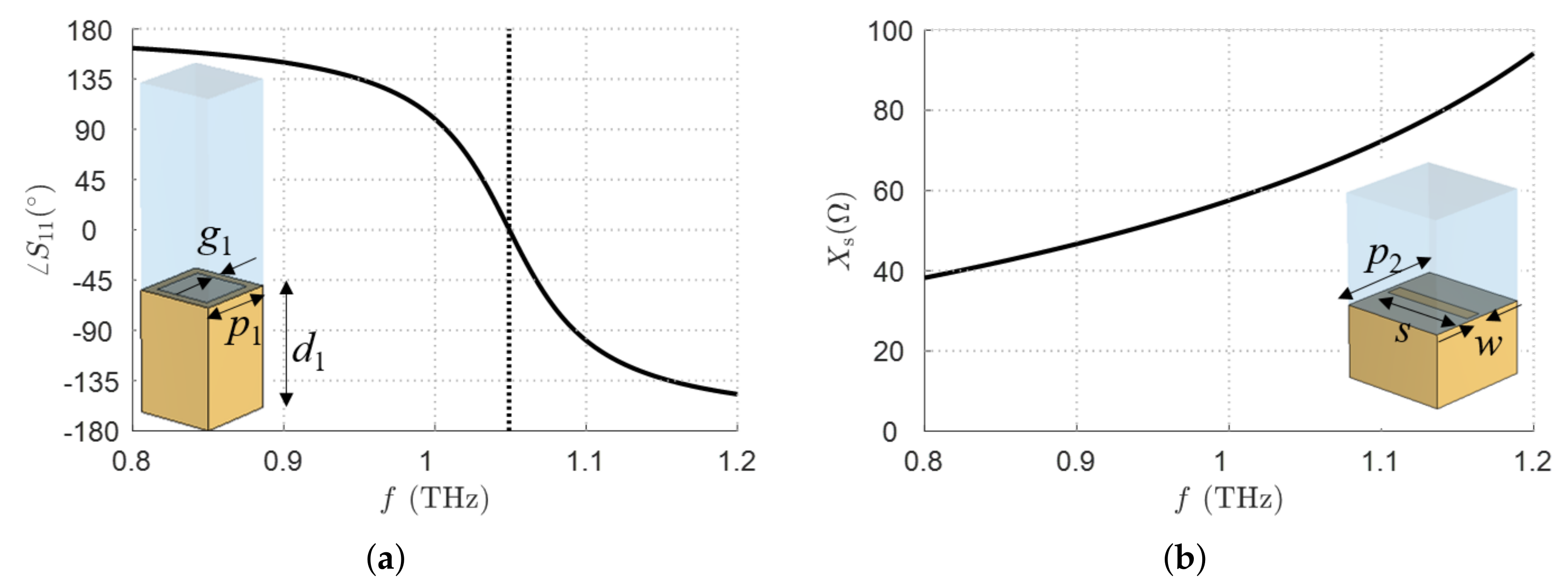
3.2. Design of the AMC Walls
3.3. Design of the PRS
3.4. Liquid-Crystal Switching
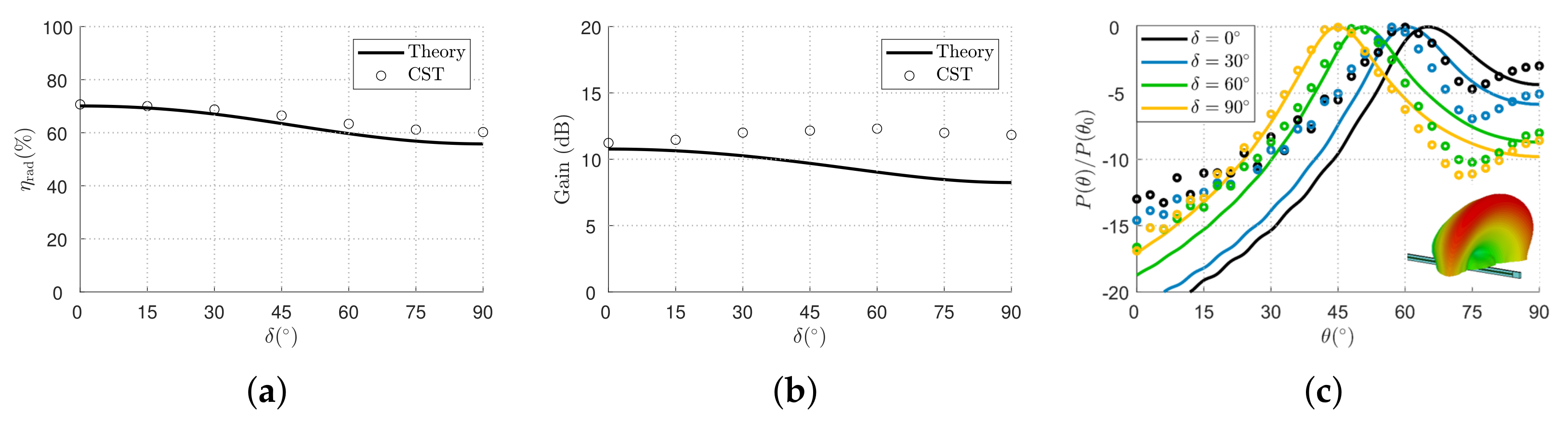
4. Results
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Jackson, D.R.; Caloz, C.; Itoh, T. Leaky-wave antennas. Proc. IEEE 2012, 100, 2194–2206. [Google Scholar] [CrossRef]
- Mittleman, D.M. Perspective: Terahertz science and technology. J. Appl. Phys. 2017, 122, 230901. [Google Scholar] [CrossRef]
- Ghasempour, Y.; Shrestha, R.; Charous, A.; Knightly, E.; Mittleman, D.M. Single-shot link discovery for terahertz wireless networks. Nat. Commun. 2020, 11, 2017. [Google Scholar] [CrossRef]
- Guerboukha, H.; Shrestha, R.; Neronha, J.; Ryan, O.; Hornbuckle, M.; Fang, Z.; Mittleman, D. Efficient leaky-wave antennas at terahertz frequencies generating highly directional beams. Appl. Phys. Lett. 2020, 117, 261103. [Google Scholar] [CrossRef]
- Amarasinghe, Y.; Mendis, R.; Mittleman, D.M. Real-time object tracking using a leaky THz waveguide. Opt. Express 2020, 28, 17997–18005. [Google Scholar] [CrossRef]
- Torabi, Y.; Dadashzadeh, G.; Hadeie, M.; Oraizi, H.; Lalbakhsh, A. A wide-angle scanning sub-terahertz leaky-wave antenna based on a multilayer dielectric image waveguide. Electronics 2021, 10, 2172. [Google Scholar] [CrossRef]
- Sato, K.; Monnai, Y. Two-dimensional terahertz beam steering based on trajectory deflection of leaky-Mode. IEEE Trans. THz Sci. Technol. 2021, 11, 676–683. [Google Scholar] [CrossRef]
- Matsumoto, H.; Watanabe, I.; Kasamatsu, A.; Monnai, Y. Integrated terahertz radar based on leaky-wave coherence tomography. Nat. Electron. 2020, 3, 122–129. [Google Scholar] [CrossRef]
- Karmokar, D.K.; Esselle, K.P.; Hay, S.G. Fixed-frequency beam steering of microstrip leaky-wave antennas using binary switches. IEEE Trans. Antennas Propag. 2016, 64, 2146–2154. [Google Scholar] [CrossRef]
- Oliveri, G.; Werner, D.H.; Massa, A. Reconfigurable electromagnetics through metamaterials—A review. Proc. IEEE 2015, 103, 1034–1056. [Google Scholar] [CrossRef]
- Fuscaldo, W.; Tofani, S.; Burghignoli, P.; Baccarelli, P.; Galli, A. Terahertz Leaky-Wave Antennas Based on Metasurfaces and Tunable Materials. In Metamaterials and Metasurfaces; Canet-Ferrer, J., Ed.; IntechOpen: London, UK, 2018; pp. 93–116. [Google Scholar]
- Zeng, C.; Lu, H.; Mao, D.; Du, Y.; Hua, H.; Zhao, W.; Zhao, J. Graphene-empowered dynamic metasurfaces and metadevices. Opto-Electron. Adv. 2022, 5, 200098-1. [Google Scholar] [CrossRef]
- Clemente, A.; Dussopt, L.; Sauleau, R.; Potier, P.; Pouliguen, P. Wideband 400-element electronically reconfigurable transmitarray in X band. IEEE Trans. Antennas Propag. 2013, 61, 5017–5027. [Google Scholar] [CrossRef]
- Lim, S.; Caloz, C.; Itoh, T. Metamaterial-based electronically controlled transmission-line structure as a novel leaky-wave antenna with tunable radiation angle and beamwidth. IEEE Trans. Microw. Theory Tech. 2005, 53, 161–173. [Google Scholar] [CrossRef]
- Ji, L.Y.; Guo, Y.J.; Qin, P.Y.; Gong, S.X.; Mittra, R. A reconfigurable partially reflective surface (PRS) antenna for beam steering. IEEE Trans. Antennas Propag. 2015, 63, 2387–2395. [Google Scholar] [CrossRef]
- Mavridou, M.; Feresidis, A.P. Dynamically reconfigurable high impedance and frequency selective metasurfaces using piezoelectric actuators. IEEE Trans. Antennas Propag. 2016, 64, 5190–5197. [Google Scholar] [CrossRef]
- Lovat, G.; Burghignoli, P.; Celozzi, S. A tunable ferroelectric antenna for fixed-frequency scanning applications. IEEE Antennas Wireless Propag. Lett. 2006, 5, 353–356. [Google Scholar] [CrossRef]
- Varadan, V.K.; Varadan, V.V.; Jose, K.A.; Kelly, J.F. Electronically steerable leaky wave antenna using a tunable ferroelectric material. Smart Mater. Struct. 1994, 3, 470. [Google Scholar] [CrossRef]
- Ma, S.; Wang, P.Y.; Meng, F.Y.; Fu, J.H.; Wu, Q. Electronically controlled beam steering leaky wave antenna in nematic liquid crystal technology. Int. J. RF Microw. CAD Eng. 2020, 30, e22188. [Google Scholar] [CrossRef]
- Hu, W.; Cahill, R.; Encinar, J.A.; Dickie, R.; Gamble, H.; Fusco, V.; Grant, N. Design and measurement of reconfigurable millimeter wave reflectarray cells with nematic liquid crystal. IEEE Trans. Antennas Propag. 2008, 56, 3112–3117. [Google Scholar] [CrossRef]
- Torabi, E.; Erricolo, D.; Chen, P.Y.; Fuscaldo, W.; Beccherelli, R. Reconfigurable beam-steerable leaky-wave antenna loaded with metamaterial apertures using liquid crystal-based delay lines. Opt. Express 2022, 30, 28966–28983. [Google Scholar] [CrossRef]
- Roig, M.; Maasch, M.; Damm, C.; Jakoby, R. Liquid crystal-based tunable CRLH-transmission line for leaky wave antenna applications at Ka-Band. Int. J. Microw. Wireless Technol. 2014, 6, 325–330. [Google Scholar] [CrossRef]
- Che, B.J.; Jin, T.; Erni, D.; Meng, F.Y.; Lyu, Y.L.; Wu, Q. Electrically controllable composite right/left-handed leaky-wave antenna using liquid crystals in PCB technology. IEEE Trans. Compon. Packag. Manuf. Technol. 2017, 7, 1331–1342. [Google Scholar] [CrossRef]
- Zografopoulos, D.C.; Ferraro, A.; Beccherelli, R. Liquid-Crystal High-Frequency Microwave Technology: Materials and Characterization. Adv. Mater. Technol. 2019, 4, 1800447. [Google Scholar] [CrossRef]
- Fuscaldo, W.; Tofani, S.; Zografopoulos, D.C.; Baccarelli, P.; Burghignoli, P.; Beccherelli, R.; Galli, A. Systematic design of THz leaky-wave antennas based on homogenized metasurfaces. IEEE Trans. Antennas Propag. 2018, 66, 1169–1178. [Google Scholar] [CrossRef]
- Aqlan, B.; Himdi, M.; Vettikalladi, H.; Le-Coq, L. A 300-GHz low-cost high-gain fully metallic Fabry–Perot cavity antenna for 6G terahertz wireless communications. Sci. Rep. 2021, 11, 7703. [Google Scholar] [CrossRef]
- Llombart, N.; Chattopadhyay, G.; Skalare, A.; Mehdi, I. Novel terahertz antenna based on a silicon lens fed by a leaky wave enhanced waveguide. IEEE Trans. Antennas Propag. 2011, 59, 2160–2168. [Google Scholar] [CrossRef]
- Llombart, N.; Lee, C.; Alonso-del Pino, M.; Chattopadhyay, G.; Jung-Kubiak, C.; Jofre, L.; Mehdi, I. Silicon micromachined lens antenna for THz integrated heterodyne arrays. IEEE Trans. THz Sci. Technol. 2013, 3, 515–523. [Google Scholar] [CrossRef]
- Esquius-Morote, M.; Gómez-Díaz, J.S.; Perruisseau-Carrier, J. Sinusoidally modulated graphene leaky-wave antenna for electronic beamscanning at THz. IEEE Trans. THz Sci. Technol. 2014, 4, 116–122. [Google Scholar] [CrossRef]
- Wang, X.C.; Zhao, W.S.; Hu, J.; Yin, W.Y. Reconfigurable terahertz leaky-wave antenna using graphene-based high-impedance surface. IEEE Trans. Nanotechnol. 2015, 14, 62–69. [Google Scholar] [CrossRef]
- Fuscaldo, W.; Burghignoli, P.; Baccarelli, P.; Galli, A. Graphene Fabry–Perot cavity leaky-wave antennas: Plasmonic versus nonplasmonic solutions. IEEE Trans. Antennas Propag. 2017, 65, 1651–1660. [Google Scholar] [CrossRef]
- Zouaghi, W.; Voß, D.; Gorath, M.; Nicoloso, N.; Roskos, H.G. How good would the conductivity of graphene have to be to make single-layer-graphene metamaterials for terahertz frequencies feasible? Carbon 2015, 94, 301–308. [Google Scholar] [CrossRef]
- D’Arco, A.; Mussi, V.; Petrov, S.; Tofani, S.; Petrarca, M.; Beccherelli, R.; Dimitrov, D.; Marinova, V.; Lupi, S.; Zografopoulos, D.C. Fabrication and spectroscopic characterization of graphene transparent electrodes on flexible cyclo-olefin substrates for terahertz electro-optic applications. Nanotechnology 2020, 31, 364006. [Google Scholar] [CrossRef]
- Fuscaldo, W.; Simone, S.D.; Dimitrov, D.; Marinova, V.; Mussi, V.; Beccherelli, R.; Zografopoulos, D.C. Terahertz characterization of graphene conductivity via time-domain reflection spectroscopy on metal-backed dielectric substrates. J. Phys. D Appl. Phys. 2022, 55, 365101. [Google Scholar] [CrossRef]
- Reuter, M.; Vieweg, N.; Fischer, B.M.; Mikulicz, M.; Koch, M.; Garbat, K.; Dabrowski, R. Highly birefringent, low-loss liquid crystals for terahertz applications. APL Mater. 2013, 1, 012107. [Google Scholar] [CrossRef]
- Vasic, B.; Isic, G.; Beccherelli, R.; Zografopoulos, D.C. Tunable beam steering at terahertz frequencies using reconfigurable metasurfaces coupled with liquid crystals. IEEE J. Sel. Top. Quantum Electron. 2020, 26, 1–9. [Google Scholar] [CrossRef]
- Tofani, S.; Fuscaldo, W. Fabry-Perot cavity leaky wave antennas with tunable features for terahertz applications. Condens. Matter 2020, 5, 11. [Google Scholar] [CrossRef]
- Fuscaldo, W.; Tofani, S.; Zografopoulos, D.C.; Baccarelli, P.; Burghignoli, P.; Beccherelli, R.; Galli, A. Tunable Fabry-Perot cavity THz antenna based on leaky-wave propagation in nematic liquid crystals. IEEE Antennas Wireless Propag. Lett. 2017, 16, 2046–2049. [Google Scholar] [CrossRef]
- Tesmer, H.; Razzouk, R.; Polat, E.; Wang, D.; Jakoby, R. Reconfigurable liquid crystal Dielectric image line leaky wave antenna at W-band. IEEE J. Microw. 2022, 2, 480–489. [Google Scholar] [CrossRef]
- Imperato, F.; Burghignoli, P.; Zografopoulos, D.C.; Beccherelli, R.; Galli, A.; Fuscaldo, W. Design of a tunable THz 1-D leaky-wave antenna based on nematic liquid crystals. In Proceedings of the 22th Mediterranean Microwave Symposium (MMS 2022), Pizzo Calabro, Italy, 11–12 May 2015; pp. 1–4. [Google Scholar]
- O’Connor, E.M.; Jackson, D.R.; Long, S.A. Extension of the Hansen-Woodyard condition for endfire leaky-wave antennas. IEEE Antennas Wireless Propag. Lett. 2010, 9, 1201–1204. [Google Scholar] [CrossRef]
- Fuscaldo, W.; Jackson, D.R.; Galli, A. Beamwidth properties of endfire 1-D leaky-wave antennas. IEEE Trans. Antennas Propag. 2017, 65, 6120–6125. [Google Scholar] [CrossRef]
- Beltayib, A.; Sebak, A.R. Analytical design procedure for forward wave couplers in RGW technology based on hybrid PEC/PMC waveguide model. IEEE Access 2019, 7, 119319–119331. [Google Scholar] [CrossRef]
- Marcuvitz, N. On field representations in terms of leaky modes or eigenmodes. IRE Trans. Antennas Propag. 1956, 4, 192–194. [Google Scholar] [CrossRef]
- Mendis, R.; Mittleman, D.M. Comparison of the lowest-order transverse-electric (TE1) and transverse-magnetic (TEM) modes of the parallel-plate waveguide for terahertz pulse applications. Opt. Express 2009, 17, 14839–14850. [Google Scholar] [CrossRef]
- Cliffe, M.J.; Rodak, A.; Graham, D.M.; Jamison, S.P. Generation of longitudinally polarized terahertz pulses with field amplitudes exceeding 2 kV/cm. Appl. Phys. Lett. 2014, 105, 191112. [Google Scholar] [CrossRef]
- Minami, Y.; Kurihara, T.; Yamaguchi, K.; Nakajima, M.; Suemoto, T. Longitudinal terahertz wave generation from an air plasma filament induced by a femtosecond laser. Appl. Phys. Lett. 2013, 102, 151106. [Google Scholar] [CrossRef]
- Fuscaldo, W.; Burghignoli, P.; Galli, A. Genealogy of leaky, surface, and plasmonic modes in partially open waveguides. Phys. Rev. Appl. 2022, 17, 034038. [Google Scholar] [CrossRef]
- Tamir, T.; Oliner, A.A. Guided complex waves. Part 1: Fields at an interface. Proc. IEE 1963, 110, 310–324. [Google Scholar] [CrossRef]
- Tamir, T.; Oliner, A.A. Guided complex waves. Part 2: Relation to radiation patterns. Proc. IEE 1963, 110, 325–334. [Google Scholar] [CrossRef]
- Sorrentino, R. Transverse resonance technique. In Numerical Techniques for Microwave and Millimeter-Wave Passive Structures; Itoh, T., Ed.; John Wiley & Sons: New York, NY, USA, 1989; Chapter 11. [Google Scholar]
- Fuscaldo, W.; Galli, A.; Jackson, D.R. Optimization of the radiating features of 1-D unidirectional leaky-wave antennas. IEEE Trans. Antennas Propag. 2022, 70, 111–125. [Google Scholar] [CrossRef]
- Fuscaldo, W. Rigorous evaluation of losses in uniform leaky-wave antennas. IEEE Trans. Antennas Propag. 2020, 68, 643–655. [Google Scholar] [CrossRef]
- Fuscaldo, W.; Jackson, D.R.; Galli, A. A general and accurate formula for the beamwidth of 1-D leaky-wave antennas. IEEE Trans. Antennas Propag. 2017, 65, 1670–1679. [Google Scholar] [CrossRef]
- Fuscaldo, W.; Galli, A.; Jackson, D.R. Optimization of 1-D unidirectional leaky-wave antennas based on partially reflecting sheets. IEEE Trans. Antennas Propag. 2021, 70, 7853–7868. [Google Scholar] [CrossRef]
- Schühler, M.; Wansch, R.; Hein, M.A. On strongly truncated leaky-wave antennas based on periodically loaded transmission lines. IEEE Trans. Antennas Propag. 2010, 58, 3505–3514. [Google Scholar] [CrossRef]
- Khoo, I.C. Liquid Crystals: Physical Properties and Nonlinear Optical Phenomena; John Wiley & Sons: Hoboken, NJ, USA, 2007; Volume 64. [Google Scholar]
- Zografopoulos, D.C.; Beccherelli, R. Tunable terahertz fishnet metamaterials based on thin nematic liquid crystal layers for fast switching. Sci. Rep. 2015, 5, 13137. [Google Scholar] [CrossRef]
- Zografopoulos, D.C.; Prokopidis, K.P.; Dąbrowski, R.; Beccherelli, R. Time-domain modeling of dispersive and lossy liquid-crystals for terahertz applications. Opt. Mater. Exp. 2014, 4, 449–457. [Google Scholar] [CrossRef]
- Pavone, S.C.; Martini, E.; Caminita, F.; Albani, M.; Maci, S. Surface wave dispersion for a tunable grounded liquid crystal substrate without and with metasurface on top. IEEE Trans. Antennas Propag. 2017, 65, 3540–3548. [Google Scholar] [CrossRef]
- Collin, R.E.; Zucker, F.J. Antenna Theory; McGraw-Hill: New York, NY, USA, 1969. [Google Scholar]
- Galdi, V.; Pinto, I.M. A simple algorithm for accurate location of leaky-wave poles for grounded inhomogeneous dielectric slabs. Microw. Opt. Technol. Lett. 2000, 24, 135–140. [Google Scholar] [CrossRef]
- Lovat, G.; Burghignoli, P.; Jackson, D.R. Fundamental properties and optimization of broadside radiation from uniform leaky-wave antennas. IEEE Trans. Antennas Propag. 2006, 54, 1442–1452. [Google Scholar] [CrossRef]
- Fuscaldo, W.; Burghignoli, P.; Galli, A. The transition between reactive and radiative regimes for leaky modes in planar waveguides based on homogenized partially reflecting surfaces. IEEE Trans. Microw. Theory Tech. 2020, 68, 5259–5269. [Google Scholar] [CrossRef]
- Luukkonen, O.; Simovski, C.; Granet, G.; Goussetis, G.; Lioubtchenko, D.; Raisanen, A.V.; Tretyakov, S.A. Simple and accurate analytical model of planar grids and high-impedance surfaces comprising metal strips or patches. IEEE Trans. Antennas Propag. 2008, 56, 1624–1632. [Google Scholar] [CrossRef]
- Sievenpiper, D.; Zhang, L.; Broas, R.F.; Alexopolous, N.G.; Yablonovitch, E. High-impedance electromagnetic surfaces with a forbidden frequency band. IEEE Trans. Microw. Theory Tech. 1999, 47, 2059–2074. [Google Scholar] [CrossRef]
- Harrington, R.F. Time-Harmonic Electromagnetic Fields; IEEE Press: New York, NY, USA, 2015. [Google Scholar]
- CST Studio Suite. Electromagnetic Field Simulation Software, Dassault Systèmes. Available online: https://www.3ds.com/products-services/simulia/products/cst-studio-suite/ (accessed on 1 June 2022).
- Zeon Corporation. Cyclo-Olefin Polymer: Specialty Plastics. Available online: https://www.zeon.co.jp/en/business/enterprise/resin/cop/ (accessed on 1 June 2022).
- Algorri, J.F.; Morawiak, P.; Bennis, N.; Zografopoulos, D.C.; Urruchi, V.; Rodríguez-Cobo, L.; Jaroszewicz, L.R.; Sánchez-Pena, J.M.; López-Higuera, J.M. Positive-negative tunable liquid crystal lenses based on a microstructured transmission line. Sci. Rep. 2020, 10, 10153. [Google Scholar] [CrossRef]
- Dąbrowski, R.; Kula, P.; Herman, J. High birefringence liquid crystals. Crystals 2013, 3, 443–482. [Google Scholar] [CrossRef]
- Liu, J.; Jackson, D.R.; Long, Y. Substrate integrated waveguide (SIW) leaky-wave antenna with transverse slots. IEEE Trans. Antennas Propag. 2012, 60, 20–29. [Google Scholar] [CrossRef]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fuscaldo, W.; Zografopoulos, D.C.; Imperato, F.; Burghignoli, P.; Beccherelli, R.; Galli, A. Analysis and Design of Tunable THz 1-D Leaky-Wave Antennas Based on Nematic Liquid Crystals. Appl. Sci. 2022, 12, 11770. https://doi.org/10.3390/app122211770
Fuscaldo W, Zografopoulos DC, Imperato F, Burghignoli P, Beccherelli R, Galli A. Analysis and Design of Tunable THz 1-D Leaky-Wave Antennas Based on Nematic Liquid Crystals. Applied Sciences. 2022; 12(22):11770. https://doi.org/10.3390/app122211770
Chicago/Turabian StyleFuscaldo, Walter, Dimitrios C. Zografopoulos, Francesca Imperato, Paolo Burghignoli, Romeo Beccherelli, and Alessandro Galli. 2022. "Analysis and Design of Tunable THz 1-D Leaky-Wave Antennas Based on Nematic Liquid Crystals" Applied Sciences 12, no. 22: 11770. https://doi.org/10.3390/app122211770
APA StyleFuscaldo, W., Zografopoulos, D. C., Imperato, F., Burghignoli, P., Beccherelli, R., & Galli, A. (2022). Analysis and Design of Tunable THz 1-D Leaky-Wave Antennas Based on Nematic Liquid Crystals. Applied Sciences, 12(22), 11770. https://doi.org/10.3390/app122211770







