A Review on Topology Optimization Strategies for Additively Manufactured Continuous Fiber-Reinforced Composite Structures
Abstract
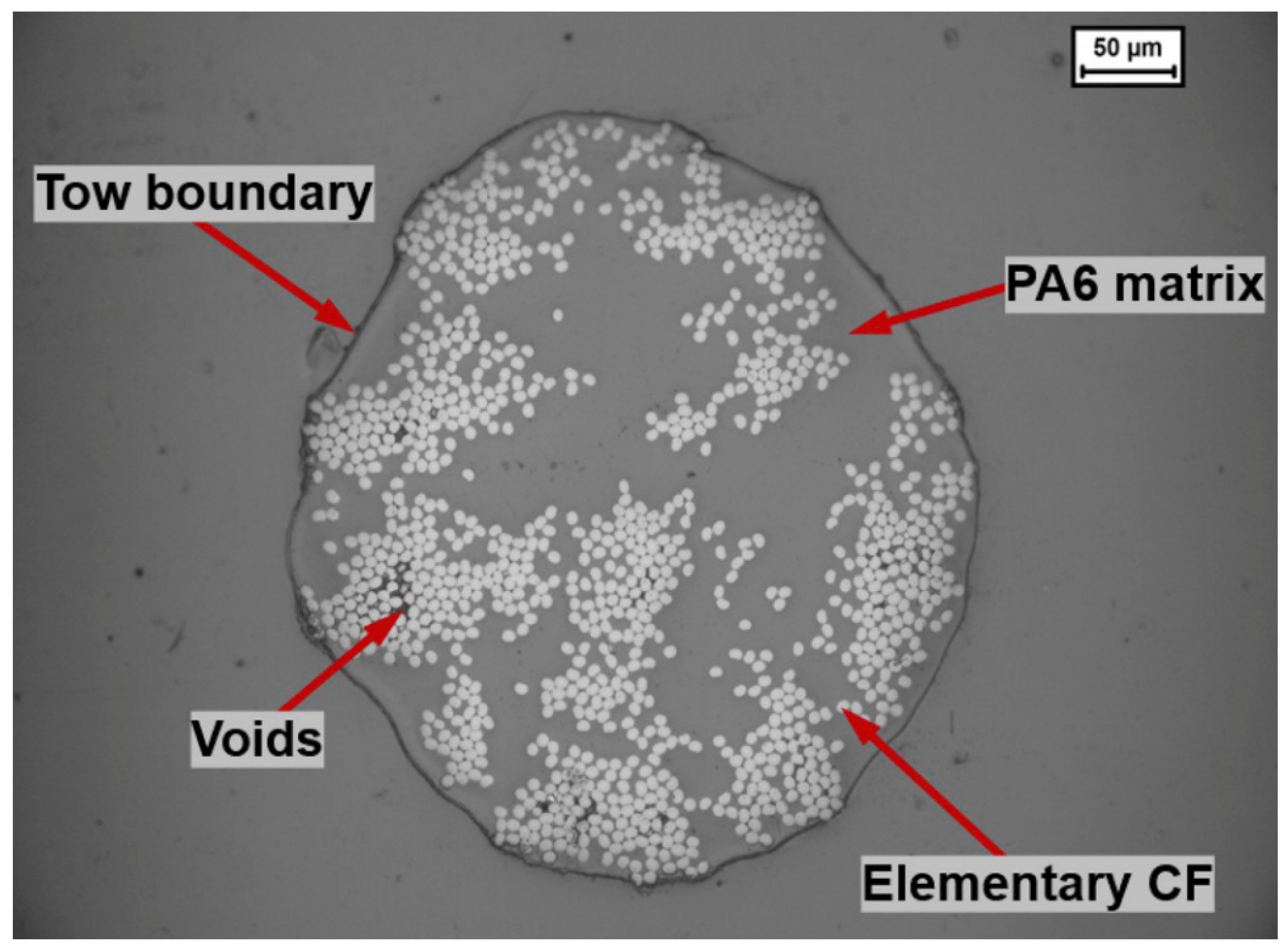
1. Introduction
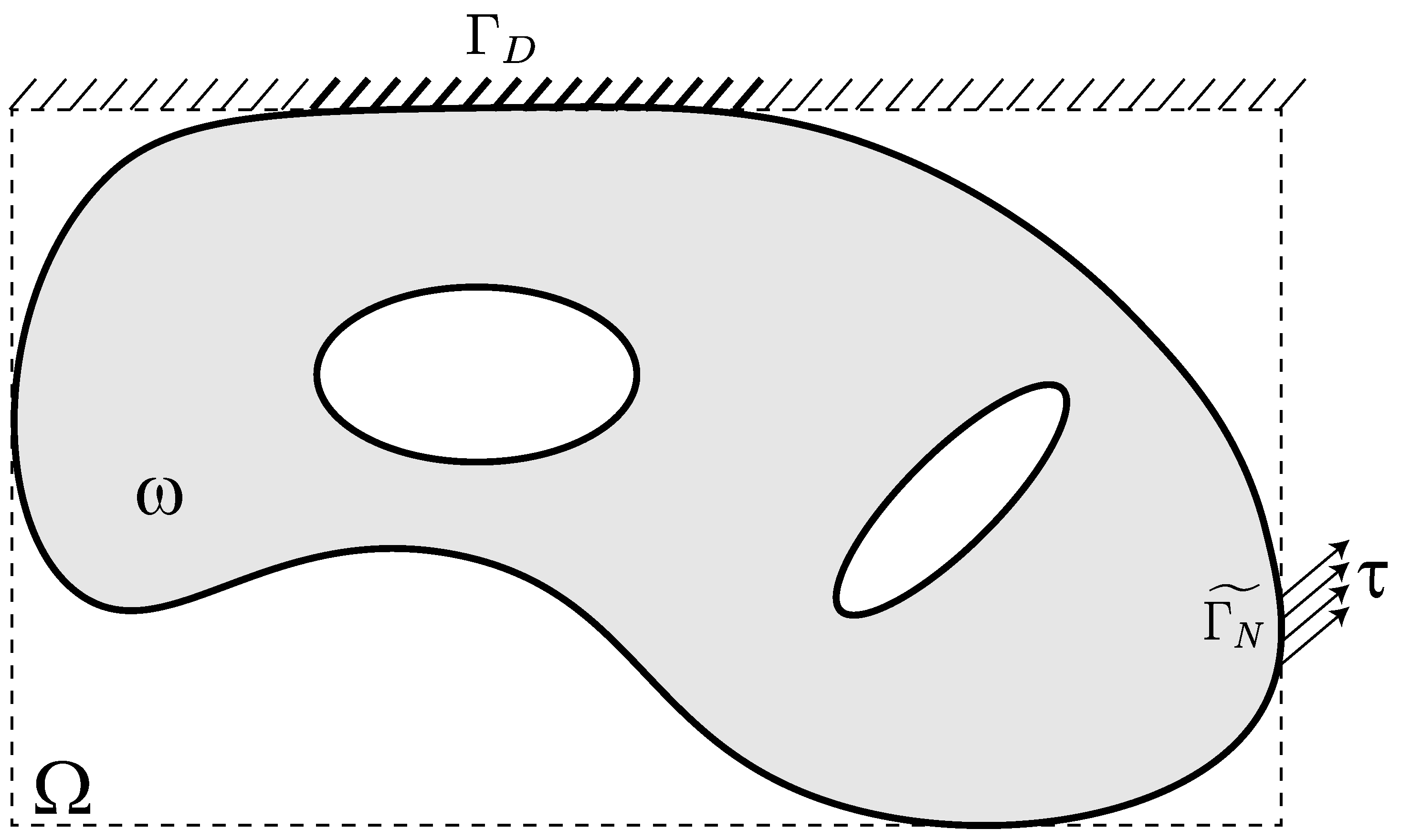
2. Topology Optimization for Continuum Structures
2.1. Shape-Based Topology Optimization
2.2. Density-Based Topology Optimization
2.3. Gradient-Based Update Schemes for Topology Optimization
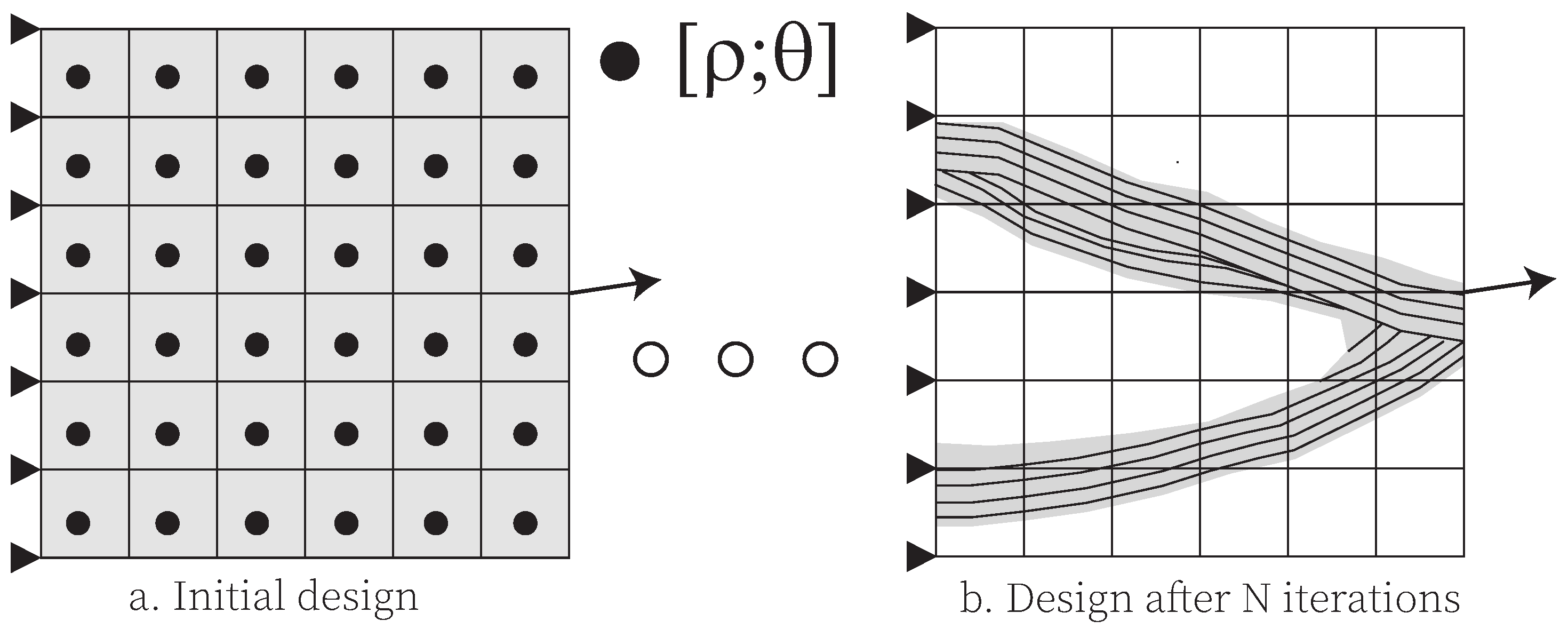
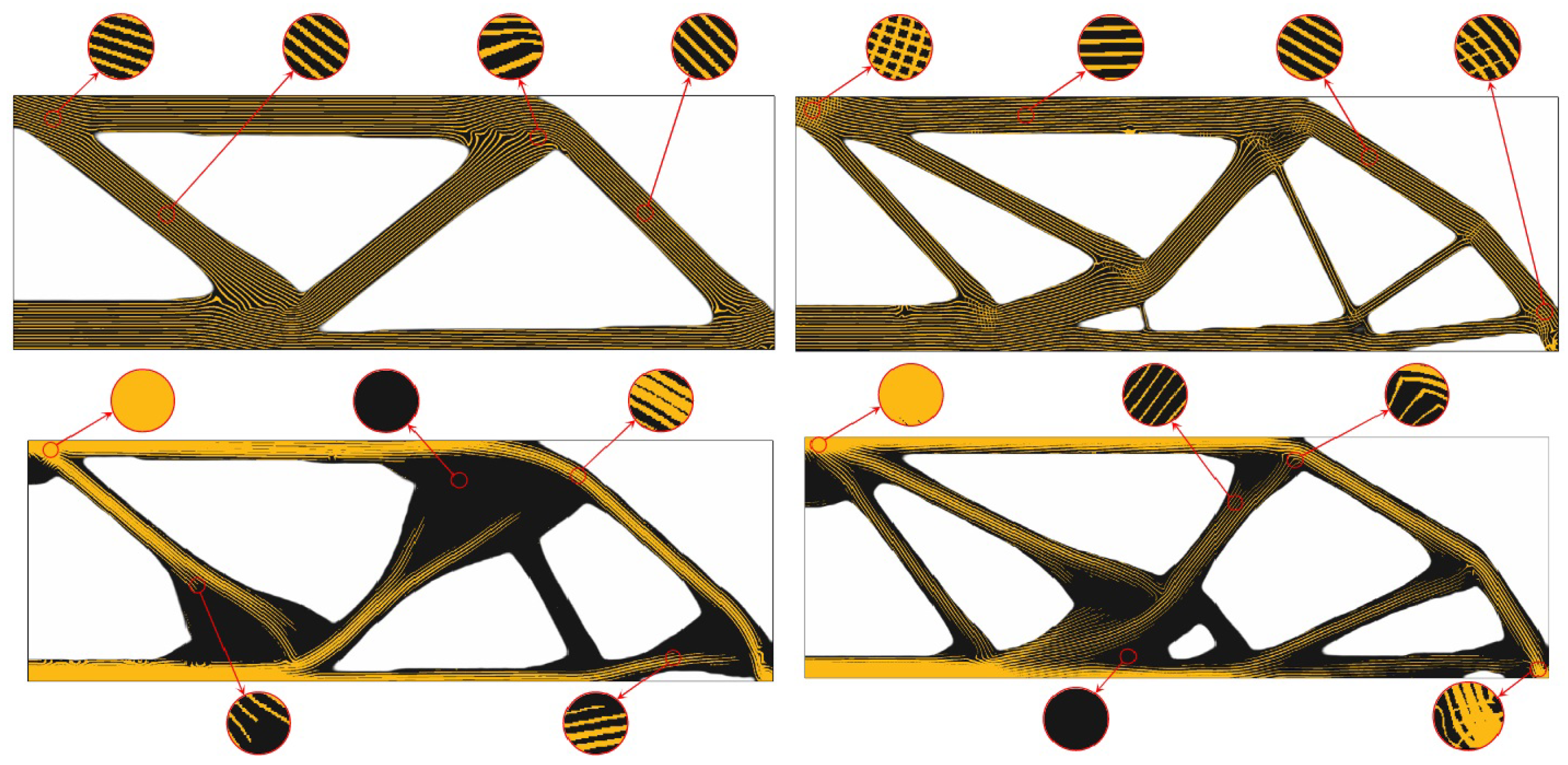
3. Parameterization Schemes for Fiber Orientation
3.1. Continuous Parameterization
3.2. Discrete Parameterization
3.3. Discrete-Continuous Parameterization
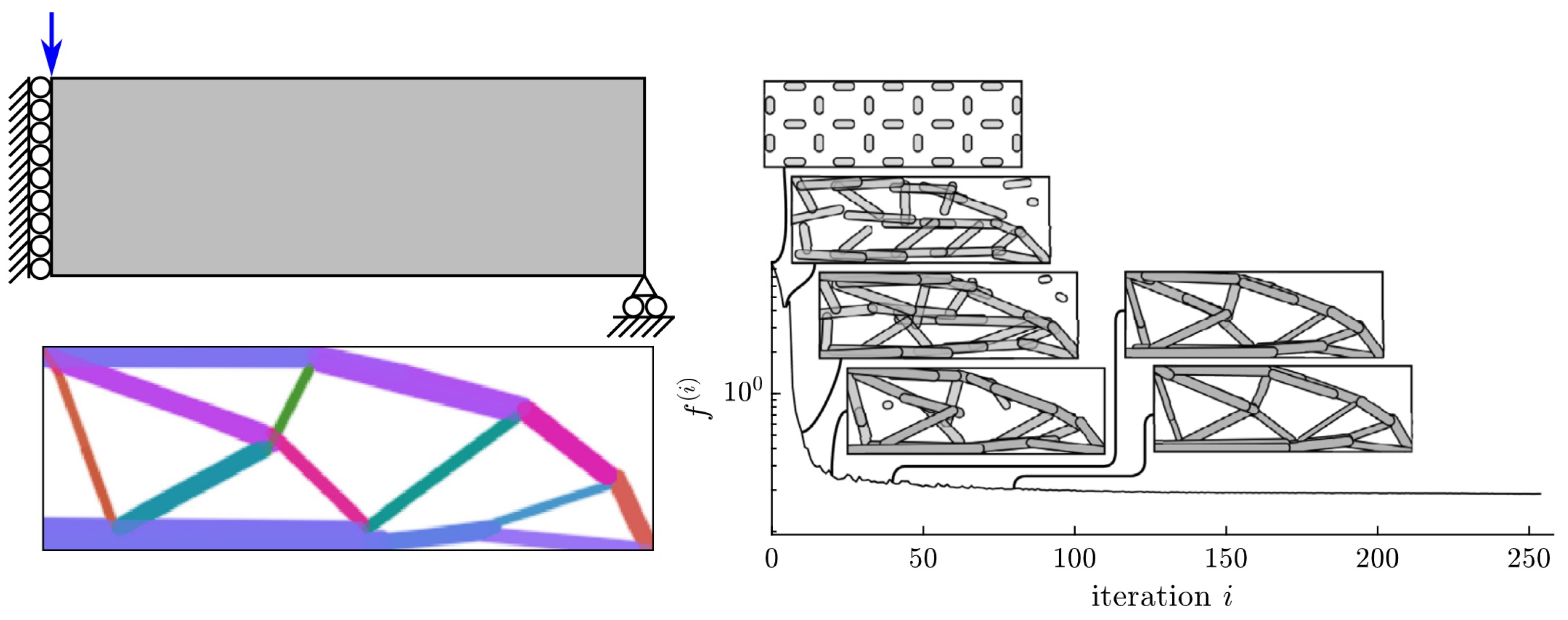
3.4. Feature-Based Parameterization
3.5. Material Parameterization
4. Discussion
5. Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Ye, M.; Gao, L.; Li, H. A design framework for gradually stiffer mechanical metamaterial induced by negative Poisson’s ratio property. Mater. Des. 2020, 192, 108751. [Google Scholar] [CrossRef]
- Luo, Y.; Li, Q.; Liu, S. Topology optimization of shell–infill structures using an erosion-based interface identification method. Comput. Methods Appl. Mech. Eng. 2019, 355, 94–112. [Google Scholar] [CrossRef]
- Kokkinis, D.; Schaffner, M.; Studart, A.R. Multimaterial magnetically assisted 3D printing of composite materials. Nat. Commun. 2015, 6, 1–10. [Google Scholar] [CrossRef] [PubMed]
- Berrocal, L.; Fernández, R.; González, S.; Periñán, A.; Tudela, S.; Vilanova, J.; Rubio, L.; Martín Márquez, J.M.; Guerrero, J.; Lasagni, F. Topology optimization and additive manufacturing for aerospace components. Prog. Addit. Manuf. 2019, 4, 83–95. [Google Scholar] [CrossRef]
- Wu, C.; Gao, Y.; Fang, J.; Lund, E.; Li, Q. Discrete topology optimization of ply orientation for a carbon fiber reinforced plastic (CFRP) laminate vehicle door. Mater. Des. 2017, 128, 9–19. [Google Scholar] [CrossRef]
- Cramer, A.D.; Challis, V.J.; Roberts, A.P. Physically Realizable Three-Dimensional Bone Prosthesis Design with Interpolated Microstructures. J. Biomech. Eng. 2017, 139, 031013. [Google Scholar] [CrossRef]
- Fleck, N.A.; Deshpande, V.S.; Ashby, M.F. Micro-architectured materials: Past, present and future. Proc. R. Soc. A Math. Phys. Eng. Sci. 2010, 466, 2495–2516. [Google Scholar] [CrossRef]
- Maskery, I.; Hussey, A.; Panesar, A.; Aremu, A.; Tuck, C.; Ashcroft, I.; Hague, R. An investigation into reinforced and functionally graded lattice structures. J. Cell. Plast. 2017, 53, 151–165. [Google Scholar] [CrossRef]
- Aremu, A.O.; Brennan-Craddock, J.P.J.; Panesar, A.; Ashcroft, I.A.; Hague, R.J.M.; Wildman, R.D.; Tuck, C. A voxel-based method of constructing and skinning conformal and functionally graded lattice structures suitable for additive manufacturing. Addit. Manuf. 2017, 13, 1–13. [Google Scholar] [CrossRef]
- Cheng, L.; Liang, X.; Belski, E.; Wang, X.; Sietins, J.M.; Ludwick, S.; To, A. Natural Frequency Optimization of Variable-Density Additive Manufactured Lattice Structure: Theory and Experimental Validation. J. Manuf. Sci. Eng. 2018, 140, 105002. [Google Scholar] [CrossRef]
- Parandoush, P.; Lin, D. A review on additive manufacturing of polymer-fiber composites. Compos. Struct. 2017, 182, 36–53. [Google Scholar] [CrossRef]
- Sano, Y.; Matsuzaki, R.; Ueda, M.; Todoroki, A.; Hirano, Y. 3D printing of discontinuous and continuous fibre composites using stereolithography. Addit. Manuf. 2018, 24, 521–527. [Google Scholar] [CrossRef]
- Wang, T.; Li, N.; Link, G.; Jelonnek, J.; Fleischer, J.; Dittus, J.; Kupzik, D. Load-dependent path planning method for 3D printing of continuous fiber reinforced plastics. Compos. Part A Appl. Sci. Manuf. 2021, 140, 106181. [Google Scholar] [CrossRef]
- Mitschang, P.; Blinzler, M.; Wöginger, A. Processing technologies for continuous fibre reinforced thermoplastics with novel polymer blends. Compos. Sci. Technol. 2003, 63, 2099–2110. [Google Scholar] [CrossRef]
- He, Q.; Wang, H.; Fu, K.; Ye, L. 3D printed continuous CF/PA6 composites: Effect of microscopic voids on mechanical performance. Compos. Sci. Technol. 2020, 191, 108077. [Google Scholar] [CrossRef]
- Yang, C.; Tian, X.; Liu, T.; Cao, Y.; Li, D. 3D printing for continuous fiber reinforced thermoplastic composites: Mechanism and performance. Rapid Prototyp. J. 2017, 23, 209–215. [Google Scholar] [CrossRef]
- Tian, X.; Liu, T.; Yang, C.; Wang, Q.; Li, D. Interface and performance of 3D printed continuous carbon fiber reinforced PLA composites. Compos. Part A Appl. Sci. Manuf. 2016, 88, 198–205. [Google Scholar] [CrossRef]
- Kabir, S.F.; Mathur, K.; Seyam, A.F.M. A critical review on 3D printed continuous fiber-reinforced composites: History, mechanism, materials and properties. Compos. Struct. 2020, 232, 111476. [Google Scholar] [CrossRef]
- Ghiasi, H.; Fayazbakhsh, K.; Pasini, D.; Lessard, L. Optimum stacking sequence design of composite materials Part II: Variable stiffness design. Compos. Struct. 2010, 93, 1–13. [Google Scholar] [CrossRef]
- Ghiasi, H.; Pasini, D.; Lessard, L. Optimum stacking sequence design of composite materials Part I: Constant stiffness design. Compos. Struct. 2009, 90, 1–11. [Google Scholar] [CrossRef]
- Sugiyama, K.; Matsuzaki, R.; Malakhov, A.V.; Polilov, A.N.; Ueda, M.; Todoroki, A.; Hirano, Y. 3D printing of optimized composites with variable fiber volume fraction and stiffness using continuous fiber. Compos. Sci. Technol. 2020, 186, 107905. [Google Scholar] [CrossRef]
- Malakhov, A.V.; Polilov, A.N. Design of composite structures reinforced curvilinear fibres using FEM. Compos. Part A Appl. Sci. Manuf. 2016, 87, 23–28. [Google Scholar] [CrossRef]
- Arian Nik, M.; Fayazbakhsh, K.; Pasini, D.; Lessard, L. Surrogate-based multi-objective optimization of a composite laminate with curvilinear fibers. Compos. Struct. 2012, 94, 2306–2313. [Google Scholar] [CrossRef]
- Zhang, J.; Zhang, W.H.; Zhu, J.H. An extended stress-based method for orientation angle optimization of laminated composite structures. Acta Mech. Sin. 2011, 27, 977–985. [Google Scholar] [CrossRef]
- Xu, Y.; Zhu, J.; Wu, Z.; Cao, Y.; Zhao, Y.; Zhang, W. A review on the design of laminated composite structures: Constant and variable stiffness design and topology optimization. Adv. Compos. Hybrid Mater. 2018, 1, 460–477. [Google Scholar] [CrossRef]
- Plocher, J.; Panesar, A. Review on design and structural optimisation in additive manufacturing: Towards next-generation lightweight structures. Mater. Des. 2019, 183, 108164. [Google Scholar] [CrossRef]
- Bendsøe, M.P.; Kikuchi, N. Generating optimal topologies in structural design using a homogenization method. Comput. Methods Appl. Mech. Eng. 1988, 71, 197–224. [Google Scholar] [CrossRef]
- Bendsøe, M.P. Optimal shape design as a material distribution problem. Struct. Optim. 1989, 1, 193–202. [Google Scholar] [CrossRef]
- Rozvany, G.I.N.; Zhou, M.; Birker, T. Generalized shape optimization without homogenization. Struct. Optim. 1992, 4, 250–252. [Google Scholar] [CrossRef]
- Wang, M.Y.; Wang, X.; Guo, D. A level set method for structural topology optimization. Comput. Methods Appl. Mech. Eng. 2003, 192, 227–246. [Google Scholar] [CrossRef]
- Allaire, G.; Jouve, F.; Toader, A.M. Structural optimization using sensitivity analysis and a level-set method. J. Comput. Phys. 2004, 194, 363–393. [Google Scholar] [CrossRef]
- Xie, Y.M.; Steven, G.P. A simple evolutionary procedure for structural optimization. Comput. Struct. 1993, 49, 885–896. [Google Scholar] [CrossRef]
- Bourdin, B.; Chambolle, A. Design-dependent loads in topology optimization. ESAIM: Control Optim. Calc. Var. 2003, 9, 19–48. [Google Scholar] [CrossRef]
- Rozvany, G.I.N. A critical review of established methods of structural topology optimization. Struct. Multidiscip. Optim. 2009, 37, 217–237. [Google Scholar] [CrossRef]
- Van Dijk, N.P.; Maute, K.; Langelaar, M.; Van Keulen, F. Level-set methods for structural topology optimization: A review. Struct. Multidiscip. Optim. 2013, 48, 437–472. [Google Scholar] [CrossRef]
- Deaton, J.D.; Grandhi, R.V. A survey of structural and multidisciplinary continuum topology optimization: Post 2000. Struct. Multidiscip. Optim. 2014, 49, 1–38. [Google Scholar] [CrossRef]
- Liu, J.S.; Parks, G.T.; Clarkson, P.J. Metamorphic Development: A new topology optimization method for continuum structures. Struct. Multidiscip. Optim. 2000, 20, 288–300. [Google Scholar] [CrossRef]
- Liu, C.; Du, Z.; Zhang, W.; Zhu, Y.; Guo, X. Additive Manufacturing-Oriented Design of Graded Lattice Structures Through Explicit Topology Optimization. J. Appl. Mech. 2017, 84, 081008. [Google Scholar] [CrossRef]
- Li, H.; Luo, Z.; Xiao, M.; Gao, L.; Gao, J. A new multiscale topology optimization method for multiphase composite structures of frequency response with level sets. Comput. Methods Appl. Mech. Eng. 2019, 356, 116–144. [Google Scholar] [CrossRef]
- Bendsoe, M.P.; Sigmund, O. Topology Optimization: Theory, Methods, and Applications; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2013. [Google Scholar]
- Nikbakt, S.; Kamarian, S.; Shakeri, M. A review on optimization of composite structures Part I: Laminated composites. Compos. Struct. 2018, 195, 158–185. [Google Scholar] [CrossRef]
- Nikbakht, S.; Kamarian, S.; Shakeri, M. A review on optimization of composite structures Part II: Functionally graded materials. Compos. Struct. 2019, 214, 83–102. [Google Scholar] [CrossRef]
- Sigmund, O.; Maute, K. Topology optimization approaches. Struct. Multidiscip. Optim. 2013, 48, 1031–1055. [Google Scholar] [CrossRef]
- Xia, L.; Xia, Q.; Huang, X.; Xie, Y.M. Bi-directional Evolutionary Structural Optimization on Advanced Structures and Materials: A Comprehensive Review. Arch. Comput. Methods Eng. 2018, 25, 437–478. [Google Scholar] [CrossRef]
- Wein, F.; Dunning, P.D.; Norato, J.A. A review on feature-mapping methods for structural optimization. Struct. Multidiscip. Optim. 2020, 62, 1597–1638. [Google Scholar] [CrossRef]
- Ferreira, I.; Machado, M.; Alves, F.; Torres Marques, A. A review on fibre reinforced composite printing via FFF. Rapid Prototyp. J. 2019, 25, 972–988. [Google Scholar] [CrossRef]
- Sigmund, O.; Petersson, J. Numerical instabilities in topology optimization: A survey on procedures dealing with checkerboards, mesh-dependencies and local minima. Struct. Optim. 1998, 16, 68–75. [Google Scholar] [CrossRef]
- Talischi, C.; Paulino, G.H.; Pereira, A.; Menezes, I.F. PolyTop: A Matlab implementation of a general topology optimization framework using unstructured polygonal finite element meshes. Struct. Multidiscip. Optim. 2012, 45, 329–357. [Google Scholar] [CrossRef]
- Eschenauer, H.A.; Kobelev, V.V.; Schumacher, A. Bubble method for topology and shape optimization of structures. Struct. Optim. 1994, 8, 42–51. [Google Scholar] [CrossRef]
- Sokolowski, J.; Zochowski, A. On the topological derivative in shape optimization. SIAM J. Control Optim. 1999, 37, 1251–1272. [Google Scholar] [CrossRef]
- Novotny, A.A.; Feijóo, R.A.; Taroco, E.; Padra, C. Topological sensitivity analysis. Comput. Methods Appl. Mech. Eng. 2003, 192, 803–829. [Google Scholar] [CrossRef]
- Upadhyay, B.D.; Sonigra, S.S.; Daxini, S.D. Numerical analysis perspective in structural shape optimization: A review post 2000. Adv. Eng. Softw. 2021, 155, 102992. [Google Scholar] [CrossRef]
- Wang, Y.; Wang, Z.; Xia, Z.; Poh, L.H. Structural Design Optimization Using Isogeometric Analysis: A Comprehensive Review. Comput. Model. Eng. Sci. 2020, 117, 455–507. [Google Scholar] [CrossRef]
- Gao, J.; Xiao, M.; Zhang, Y.; Gao, L. A Comprehensive Review of Isogeometric Topology Optimization: Methods, Applications and Prospects. Chin. J. Mech. Eng. 2020, 33, 1–14. [Google Scholar] [CrossRef]
- Sethian, J.A. Level Set Methods and Fast Marching Methods: Evolving Interfaces in Computational Geometry, Fluid Mechanics, Computer Vision, and Materials Science; Cambridge University Press: Cambridge, UK, 1999; Volume 3. [Google Scholar]
- Sethian, J.A. Evolution, implementation, and application of level set and fast marching methods for advancing fronts. J. Comput. Phys. 2001, 169, 503–555. [Google Scholar] [CrossRef]
- Burger, M.; Osher, S.J. A survey on level set methods for inverse problems and optimal design. Eur. J. Appl. Math. 2005, 16, 263–301. [Google Scholar] [CrossRef]
- Sivapuram, R.; Picelli, R.; Xie, Y.M. Topology optimization of binary microstructures involving various non-volume constraints. Comput. Mater. Sci. 2018, 154, 405–425. [Google Scholar] [CrossRef]
- Munk, D.J. A bidirectional evolutionary structural optimization algorithm for mass minimization with multiple structural constraints. Int. J. Numer. Methods Eng. 2019, 118, 93–120. [Google Scholar] [CrossRef]
- Wang, Z.; Sobey, A. A comparative review between Genetic Algorithm use in composite optimisation and the state-of-the-art in evolutionary computation. Compos. Struct. 2020, 233, 111739. [Google Scholar] [CrossRef]
- Sigmund, O. On the usefulness of non-gradient approaches in topology optimization. Struct. Multidiscip. Optim. 2011, 43, 589–596. [Google Scholar] [CrossRef]
- Borrvall, T. Topology optimization of elastic continua using restriction. Arch. Comput. Methods Eng. 2001, 8, 351–385. [Google Scholar] [CrossRef]
- Hasançebi, O.; Çarbas, S.; Saka, M.P. Improving the performance of simulated annealing in structural optimization. Struct. Multidiscip. Optim. 2010, 41, 189–203. [Google Scholar] [CrossRef]
- Reuschel, D.; Mattheck, C. Three-dimensional fibre optimisation with computer aided internal optimisation. Aeronaut. J. 1999, 103, 415–420. [Google Scholar] [CrossRef]
- Voelkl, H.; Wartzack, S. Design for composites: Tailor-made, bio-inspired topology optimization for fiber-reinforced plastics. In Proceedings of the DESIGN 2018 15th International Design Conference, Dubrovnik, Croatia, 21–24 May 2018; pp. 499–510. [Google Scholar] [CrossRef]
- Svanberg, K. The method of moving asymptotes—A new method for structural optimization. Int. J. Numer. Methods Eng. 1987, 24, 359–373. [Google Scholar] [CrossRef]
- Dunning, P.D.; Kim, H.A. Introducing the sequential linear programming level-set method for topology optimization. Struct. Multidiscip. Optim. 2015, 51, 631–643. [Google Scholar] [CrossRef]
- Shen, Y.; Branscomb, D. Orientation optimization in anisotropic materials using gradient descent method. Compos. Struct. 2020, 234, 111680. [Google Scholar] [CrossRef]
- Kim, N.H.; Dong, T.; Weinberg, D.; Dalidd, J. Generalized optimality criteria method for topology optimization. Appl. Sci. 2021, 11, 3175. [Google Scholar] [CrossRef]
- Patnaik, S.N.; Guptill, J.D.; Berke, L. Merits and limitations of optimality criteria method for structural optimization. Int. J. Numer. Methods Eng. 1995, 38, 3087–3120. [Google Scholar] [CrossRef]
- Fuchs, M.; Ali, R.H. A family of homogeneous analysis models for the design of scalable structures. Struct. Optim. 1990, 2, 143–152. [Google Scholar] [CrossRef]
- Fleury, C. CONLIN: An efficient dual optimizer based on convex approximation concepts. Struct. Optim. 1989, 1, 81–89. [Google Scholar] [CrossRef]
- Barthelemy, J.F.M.; Haftka, R.T. Approximation concepts for optimum structural design—A review. Struct. Optim. 1993, 5, 129–144. [Google Scholar] [CrossRef]
- Bruyneel, M.; Fleury, C. Composite structures optimization using sequential convex programming. Adv. Eng. Softw. 2002, 33, 697–711. [Google Scholar] [CrossRef]
- Lindgaard, E.; Lund, E. Optimization formulations for the maximum nonlinear buckling load of composite structures. Struct. Multidiscip. Optim. 2011, 43, 631–646. [Google Scholar] [CrossRef]
- Stegmann, J.; Lund, E. Discrete material optimization of general composite shell structures. Int. J. Numer. Methods Eng. 2005, 62, 2009–2027. [Google Scholar] [CrossRef]
- Zowe, J.; Kocvara, M.; Bendsøe, M.P. Free material optimization via mathematical programming. Math. Program. 1997, 79, 445–466. [Google Scholar] [CrossRef]
- Ben-Tal, A.; Kocvara, M.; Nemirovski, A.; Zowe, J. Free Material Design via Semidefinite Programming: The Multiload Case with Contact Conditions. SIAM J. Optim. 1999, 9, 813–832. [Google Scholar] [CrossRef]
- Nomura, T.; Kawamoto, A.; Kondoh, T.; Dede, E.M.; Lee, J.; Song, Y.; Kikuchi, N. Inverse design of structure and fiber orientation by means of topology optimization with tensor field variables. Compos. Part B: Eng. 2019, 176, 107187. [Google Scholar] [CrossRef]
- Michell, A.G.M. LVIII. The limits of economy of material in frame-structures. Lond. Edinb. Dublin Philos. Mag. J. Sci. 1904, 8, 589–597. [Google Scholar] [CrossRef]
- Pedersen, P. On optimal orientation of orthotropic materials. Struct. Optim. 1989, 1, 101–106. [Google Scholar] [CrossRef]
- Pedersen, P. Bounds on elastic energy in solids of orthotropic materials. Struct. Optim. 1990, 2, 55–63. [Google Scholar] [CrossRef]
- Pedersen, P. On thickness and orientational design with orthotropic materials. Struct. Optim. 1991, 3, 69–78. [Google Scholar] [CrossRef]
- Cheng, H.C.; Kikuchi, N.; Ma, Z.D. An improved approach for determining the optimal orientation of orthotropic material. Struct. Optim. 1994, 8, 101–112. [Google Scholar] [CrossRef]
- Zhou, M.; Rozvany, G.I.N. DCOC: An optimality criteria method for large systems Part I: Theory. Struct. Optim. 1992, 5, 12–25. [Google Scholar] [CrossRef]
- Zhou, M.; Rozvany, G.I. DCOC: An optimality criteria method for large systems Part II: Algorithm. Struct. Optim. 1993, 6, 250–262. [Google Scholar] [CrossRef]
- Suzuki, K.; Kikuchi, N. A homogenization method for shape and topology optimization. Comput. Methods Appl. Mech. Eng. 1991, 93, 291–318. [Google Scholar] [CrossRef]
- Díaz, A.R.; Bendsøe, M.P. Shape optimization of structures for multiple loading conditions using a homogenization method. Struct. Optim. 1992, 4, 17–22. [Google Scholar] [CrossRef]
- Gea, H.C.; Luo, J.H. On the stress-based and strain-based methods for predicting optimal orientation of orthotropic materials. Struct. Multidiscip. Optim. 2004, 26, 229–234. [Google Scholar] [CrossRef]
- Lu, Y.; Tong, L. Concurrent optimization of topologies and fiber orientations for laminated composite structures. Compos. Struct. 2022, 295, 115749. [Google Scholar] [CrossRef]
- Qiu, Z.; Li, Q.; Luo, Y.; Liu, S. Concurrent topology and fiber orientation optimization method for fiber-reinforced composites based on composite additive manufacturing. Comput. Methods Appl. Mech. Eng. 2022, 395, 114962. [Google Scholar] [CrossRef]
- Luo, J.H.; Gea, H.C. Optimal orientation of orthotropic materials using an energy based method. Struct. Optim. 1998, 15, 230–236. [Google Scholar] [CrossRef]
- Luo, J.H.; Gea, H.C. Optimal bead orientation of 3D shell/plate structures. Finite Elem. Anal. Des. 1998, 31, 55–71. [Google Scholar] [CrossRef]
- Yan, X.; Xu, Q.; Hua, H.; Huang, D.; Huang, X. Concurrent topology optimization of structures and orientation of anisotropic materials. Eng. Optim. 2020, 52, 1598–1611. [Google Scholar] [CrossRef]
- Bruyneel, M.; Zein, S. A modified Fast Marching Method for defining fiber placement trajectories over meshes. Comput. Struct. 2013, 125, 45–52. [Google Scholar] [CrossRef]
- Lemaire, E.; Zein, S.; Bruyneel, M. Optimization of composite structures with curved fiber trajectories. Compos. Struct. 2015, 131, 895–904. [Google Scholar] [CrossRef]
- Hao, P.; Liu, C.; Liu, X.; Yuan, X.; Wang, B.; Li, G.; Dong, M.; Chen, L. Isogeometric analysis and design of variable-stiffness aircraft panels with multiple cutouts by level set method. Compos. Struct. 2018, 206, 888–902. [Google Scholar] [CrossRef]
- Brampton, C.J.; Wu, K.C.; Kim, H.A. New optimization method for steered fiber composites using the level set method. Struct. Multidiscip. Optim. 2015, 52, 493–505. [Google Scholar] [CrossRef]
- Papapetrou, V.S.; Patel, C.; Tamijani, A.Y. Stiffness-based optimization framework for the topology and fiber paths of continuous fiber composites. Compos. Part B Eng. 2020, 183, 107681. [Google Scholar] [CrossRef]
- Tian, Y.; Pu, S.; Shi, T.; Xia, Q. A parametric divergence-free vector field method for the optimization of composite structures with curvilinear fibers. Comput. Methods Appl. Mech. Eng. 2021, 373, 113574. [Google Scholar] [CrossRef]
- Riche, R.L.; Haftka, R.T. Optimization of laminate stacking sequence for buckling load maximization by genetic algorithm. AIAA J. 1993, 31, 951–956. [Google Scholar] [CrossRef]
- Nagendra, S.; Jestin, D.; Gürdal, Z.; Haftka, R.T.; Watson, L.T. Improved genetic algorithm for the design of stiffened composite panels. Compos. Struct. 1996, 58, 543–555. [Google Scholar] [CrossRef]
- Liu, B.; Haftka, R.T.; Akgün, M.A.; Todoroki, A. Permutation genetic algorithm for stacking sequence design of composite laminates. Comput. Methods Appl. Mech. Eng. 2000, 186, 357–372. [Google Scholar] [CrossRef]
- Bendsøe, M.P.; Sigmund, O. Material interpolation schemes in topology optimization. Arch. Appl. Mech. 1999, 69, 635–654. [Google Scholar] [CrossRef]
- Yin, L.; Ananthasuresh, G.K. Topology optimization of compliant mechanisms with multiple materials using a peak function material interpolation scheme. Struct. Multidiscip. Optim. 2001, 23, 49–62. [Google Scholar] [CrossRef]
- Bruyneel, M. SFP—A new parameterization based on shape functions for optimal material selectIon: Application to conventional composite plies. Struct. Multidiscip. Optim. 2011, 43, 17–27. [Google Scholar] [CrossRef]
- Gao, T.; Zhang, W.; Duysinx, P. A bi-value coding parameterization scheme for the discrete optimal orientation design of the composite laminate. Int. J. Numer. Methods Eng. 2012, 91, 98–114. [Google Scholar] [CrossRef]
- Sørensen, S.N.; Sørensen, R.; Lund, E. DMTO—A method for discrete material and thickness optimization of laminated composite structures. Struct. Multidiscip. Optim. 2014, 50, 25–47. [Google Scholar] [CrossRef]
- Sørensen, R.; Lund, E. Thickness filters for gradient based multi-material and thickness optimization of laminated composite structures. Struct. Multidiscip. Optim. 2015, 52, 227–250. [Google Scholar] [CrossRef]
- Sjølund, J.; Peeters, D.; Lund, E. A new thickness parameterization for Discrete Material and Thickness Optimization. Struct. Multidiscip. Optim. 2018, 58, 1885–1897. [Google Scholar] [CrossRef]
- Hozić, D.; Thore, C.J.; Cameron, C.; Loukil, M. A new method for simultaneous material and topology optimization of composite laminate structures using Hyperbolic Function Parametrization. Compos. Struct. 2021, 276, 114374. [Google Scholar] [CrossRef]
- Kiyono, C.Y.; Silva, E.C.N.; Reddy, J.N. A novel fiber optimization method based on normal distribution function with continuously varying fiber path. Compos. Struct. 2017, 160, 503–515. [Google Scholar] [CrossRef]
- da Silva, A.L.F.; Salas, R.A.; Silva, E.C.N. Topology optimization of composite hyperelastic material using SPIMFO-method. Meccanica 2021, 56, 417–437. [Google Scholar] [CrossRef]
- Salas, R.A.; Ramírez-Gil, F.J.; Montealegre-Rubio, W.; Silva, E.C.N.; Reddy, J.N. Optimized dynamic design of laminated piezocomposite multi-entry actuators considering fiber orientation. Comput. Methods Appl. Mech. Eng. 2018, 335, 223–254. [Google Scholar] [CrossRef]
- Luo, Y.; Chen, W.; Liu, S.; Li, Q.; Ma, Y. A discrete-continuous parameterization (DCP) for concurrent optimization of structural topologies and continuous material orientations. Compos. Struct. 2020, 236, 111900. [Google Scholar] [CrossRef]
- Nomura, T.; Dede, E.M.; Lee, J.; Yamasaki, S.; Matsumori, T.; Kawamoto, A.; Kikuchi, N. General topology optimization method with continuous and discrete orientation design using isoparametric projection. Int. J. Numer. Methods Eng. 2015, 101, 571–605. [Google Scholar] [CrossRef]
- Xia, Q.; Shi, T. Optimization of composite structures with continuous spatial variation of fiber angle through Shepard interpolation. Compos. Struct. 2017, 182, 273–282. [Google Scholar] [CrossRef]
- Xia, Q.; Shi, T. A cascadic multilevel optimization algorithm for the design of composite structures with curvilinear fiber based on Shepard interpolation. Compos. Struct. 2018, 188, 209–219. [Google Scholar] [CrossRef]
- Ding, H.; Xu, B. A novel discrete–continuous material orientation optimization model for stiffness-based concurrent design of fiber composite. Compos. Struct. 2021, 273, 114288. [Google Scholar] [CrossRef]
- Norato, J.A.; Bell, B.K.; Tortorelli, D.A. A geometry projection method for continuum-based topology optimization with discrete elements. Comput. Methods Appl. Mech. Eng. 2015, 293, 306–327. [Google Scholar] [CrossRef]
- Smith, H.; Norato, J.A. Topology optimization with discrete geometric components made of composite materials. Comput. Methods Appl. Mech. Eng. 2021, 376, 113582. [Google Scholar] [CrossRef]
- Sun, Z.; Song, Z.; Song, J.; Li, H. Structural Optimization of Fiber-Reinforced Material Based on Moving Morphable Components (MMCs). Acta Mech. Solida Sin. 2022, 35, 1–15. [Google Scholar] [CrossRef]
- Udupa, G.; Rao, S.S.; Gangadharan, K.V. Functionally Graded Composite Materials: An Overview. Procedia Mater. Sci. 2014, 5, 1291–1299. [Google Scholar] [CrossRef]
- Fernandez, F.; Compel, W.S.; Lewicki, J.P.; Tortorelli, D.A. Optimal design of fiber reinforced composite structures and their direct ink write fabrication. Comput. Methods Appl. Mech. Eng. 2019, 353, 277–307. [Google Scholar] [CrossRef]
- Jiang, D.; Hoglund, R.; Smith, D.E. Continuous fiber angle topology optimization for polymer composite deposition additive manufacturing applications. Fibers 2019, 7, 14. [Google Scholar] [CrossRef]
- Chandrasekhar, A.; Kumar, T.; Suresh, K. Build optimization of fiber-reinforced additively manufactured components. Struct. Multidiscip. Optim. 2020, 61, 77–90. [Google Scholar] [CrossRef]
- Lee, J.; Kim, D.; Nomura, T.; Dede, E.M.; Yoo, J. Topology optimization for continuous and discrete orientation design of functionally graded fiber-reinforced composite structures. Compos. Struct. 2018, 201, 217–233. [Google Scholar] [CrossRef]
- Desai, A.; Mogra, M.; Sridhara, S.; Kumar, K.; Sesha, G.; Ananthasuresh, G. Topological-derivative-based design of stiff fiber-reinforced structures with optimally oriented continuous fibers. Struct. Multidiscip. Optim. 2021, 63, 703–720. [Google Scholar] [CrossRef]
- Wu, J.; Sigmund, O.; Groen, J.P. Topology optimization of multi-scale structures: A review. Struct. Multidiscip. Optim. 2021, 63, 1455–1480. [Google Scholar] [CrossRef]
- Kim, D.; Lee, J.; Nomura, T.; Dede, E.M.; Yoo, J.; Min, S. Topology optimization of functionally graded anisotropic composite structures using homogenization design method. Comput. Methods Appl. Mech. Eng. 2020, 369, 113220. [Google Scholar] [CrossRef]
- Groen, J.P.; Sigmund, O. Homogenization-based topology optimization for high-resolution manufacturable microstructures. Int. J. Numer. Methods Eng. 2018, 113, 1148–1163. [Google Scholar] [CrossRef]
- Jung, T.; Lee, J.; Nomura, T.; Dede, E.M. Inverse design of three-dimensional fiber reinforced composites with spatially-varying fiber size and orientation using multiscale topology optimization. Compos. Struct. 2022, 279, 114768. [Google Scholar] [CrossRef]
- Boddeti, N.; Tang, Y.; Maute, K.; Rosen, D.W.; Dunn, M.L. Optimal design and manufacture of variable stiffness laminated continuous fiber reinforced composites. Sci. Rep. 2020, 10, 1–15. [Google Scholar] [CrossRef]
- Hvejsel, C.F.; Lund, E. Material interpolation schemes for unified topology and multi-material optimization. Struct. Multidiscip. Optim. 2011, 43, 811–825. [Google Scholar] [CrossRef]
- Kennedy, G.J.; Martins, J.R. A laminate parameterization technique for discrete ply-angle problems with manufacturing constraints. Struct. Multidiscip. Optim. 2013, 48, 379–393. [Google Scholar] [CrossRef]
- Lund, E. Discrete Material and Thickness Optimization of laminated composite structures including failure criteria. Struct. Multidiscip. Optim. 2018, 57, 2357–2375. [Google Scholar] [CrossRef]
- Gandhi, Y.; Pirondi, A.; Collini, L. Optimal design of shape memory alloy composite under deflection constraint. Materials 2019, 12, 1733. [Google Scholar] [CrossRef]
- Tsai, S.W.; Pagano, N.J. Invariant Properties of Composite Materials; Defense Technical Information Center: Fort Belvoir, VA, USA, 1968; pp. 233–253. [Google Scholar]
- Albazzan, M.A.; Harik, R.; Tatting, B.F.; Gürdal, Z. Efficient design optimization of nonconventional laminated composites using lamination parameters: A state of the art. Compos. Struct. 2019, 209, 362–374. [Google Scholar] [CrossRef]
- Bohrer, R.Z.G.; Kim, I.Y. Concurrent topology and stacking sequence optimization of composite laminate plates using lamination parameters. Compos. Struct. 2021, 276, 114556. [Google Scholar] [CrossRef]
- Demir, E.; Yousefi-Louyeh, P.; Yildiz, M. Design of variable stiffness composite structures using lamination parameters with fiber steering constraint. Compos. Part B Eng. 2019, 165, 733–746. [Google Scholar] [CrossRef]
- Peeters, D.; Hong, Z.; Abdalla, M. A compliance approximation method applied to variable stiffness composite optimisation. Struct. Multidiscip. Optim. 2018, 58, 1981–2001. [Google Scholar] [CrossRef]
- Peeters, D.; van Baalen, D.; Abdallah, M. Combining topology and lamination parameter optimisation. Struct. Multidiscip. Optim. 2015, 52, 105–120. [Google Scholar] [CrossRef]
- Chen, Y.; Ye, L. Topological design for 3D-printing of carbon fibre reinforced composite structural parts. Compos. Sci. Technol. 2021, 204, 108644. [Google Scholar] [CrossRef]
- Liu, J.; Yu, H. Concurrent deposition path planning and structural topology optimization for additive manufacturing. Rapid Prototyp. J. 2017, 23, 930–942. [Google Scholar] [CrossRef]
- Schmidt, M.P.; Couret, L.; Gout, C.; Pedersen, C.B. Structural topology optimization with smoothly varying fiber orientations. Struct. Multidiscip. Optim. 2020, 62, 3105–3126. [Google Scholar] [CrossRef]
- Kubalak, J.R.; Wicks, A.L.; Williams, C.B. Investigation of Parameter Spaces for Topology Optimization with Three-Dimensional Orientation Fields for Multi-Axis Additive Manufacturing. J. Mech. Des. 2020, 143, 051701. [Google Scholar] [CrossRef]
- Fedulov, B.; Fedorenko, A.; Khaziev, A.; Antonov, F. Optimization of parts manufactured using continuous fiber three-dimensional printing technology. Compos. Part B Eng. 2021, 227, 109406. [Google Scholar] [CrossRef]
- Lyu, N.; Saitou, K. Topology Optimization of Multicomponent Beam Structure via Decomposition-Based Assembly Synthesis. J. Mech. Des. 2005, 127, 170–183. [Google Scholar] [CrossRef]
- Zhou, Y.; Saitou, K. Gradient-based multi-component topology optimization for stamped sheet metal assemblies (MTO-S). Struct. Multidiscip. Optim. 2018, 58, 83–94. [Google Scholar] [CrossRef]
- Zhou, Y.; Nomura, T.; Saitou, K. Multi-component topology and material orientation design of composite structures (MTO-C). Comput. Methods Appl. Mech. Eng. 2018, 342, 438–457. [Google Scholar] [CrossRef]


| Parameterization | Design Freedom | Advantages | Applicability | Drawbacks |
|---|---|---|---|---|
| Continuous | Fully relaxed material orientation space. | Spatially varying fiber path both in 2D and 3D. | Adopted scheme for CF4 part design and verification. | Initial design dependency, significant variation in fiber angles, and ambiguity result in poor local minima. |
| Discrete | Most restrictive material orientation space. | Most effective gradient-based method for discrete settings 1, e.g., multi-phase TO. | Numerous studies on designing multi-layered composite laminates. | Several design variables, fiber convergence, and material discontinuity lead to ambiguous design. |
| Discrete-continuous | Continuous orientations are penalized for attaining assigned discrete directions. | General framework for both continuous and discrete settings. | Promising framework to withstand various FRC manufacturing units. | Only a few works are available, and an efficient optimization formulation is needed to tackle the general setting. |
| Feature-based | Most restrictive material distribution space 2. | Least number of optimization variables, easy-to-control feature size, and ready-to-manufacture design. | Favorable for large-scale application and industrial manufacturing units due to its simple topology. | Topology is restricted 3 to feature shape, thus limiting CF4 capabilities |
| Material | Completely relaxed in material distribution and orientation space | Allows spatially varying fiber path and volume fraction, complex loading, integration of failure criteria, damage model, etc. | Fully exploit the capabilities of CF4 with a design that can follow the response of the actual part. | Validation of numerical framework is difficult because the attained topology is complex |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gandhi, Y.; Minak, G. A Review on Topology Optimization Strategies for Additively Manufactured Continuous Fiber-Reinforced Composite Structures. Appl. Sci. 2022, 12, 11211. https://doi.org/10.3390/app122111211
Gandhi Y, Minak G. A Review on Topology Optimization Strategies for Additively Manufactured Continuous Fiber-Reinforced Composite Structures. Applied Sciences. 2022; 12(21):11211. https://doi.org/10.3390/app122111211
Chicago/Turabian StyleGandhi, Yogesh, and Giangiacomo Minak. 2022. "A Review on Topology Optimization Strategies for Additively Manufactured Continuous Fiber-Reinforced Composite Structures" Applied Sciences 12, no. 21: 11211. https://doi.org/10.3390/app122111211
APA StyleGandhi, Y., & Minak, G. (2022). A Review on Topology Optimization Strategies for Additively Manufactured Continuous Fiber-Reinforced Composite Structures. Applied Sciences, 12(21), 11211. https://doi.org/10.3390/app122111211







