Energy Consumption Analysis of a Rolling Mechanism Based on a Five-Bow-Shaped-Bar Linkage
Abstract
1. Introduction
2. Trajectory Planning of the Mechanism’s CoM
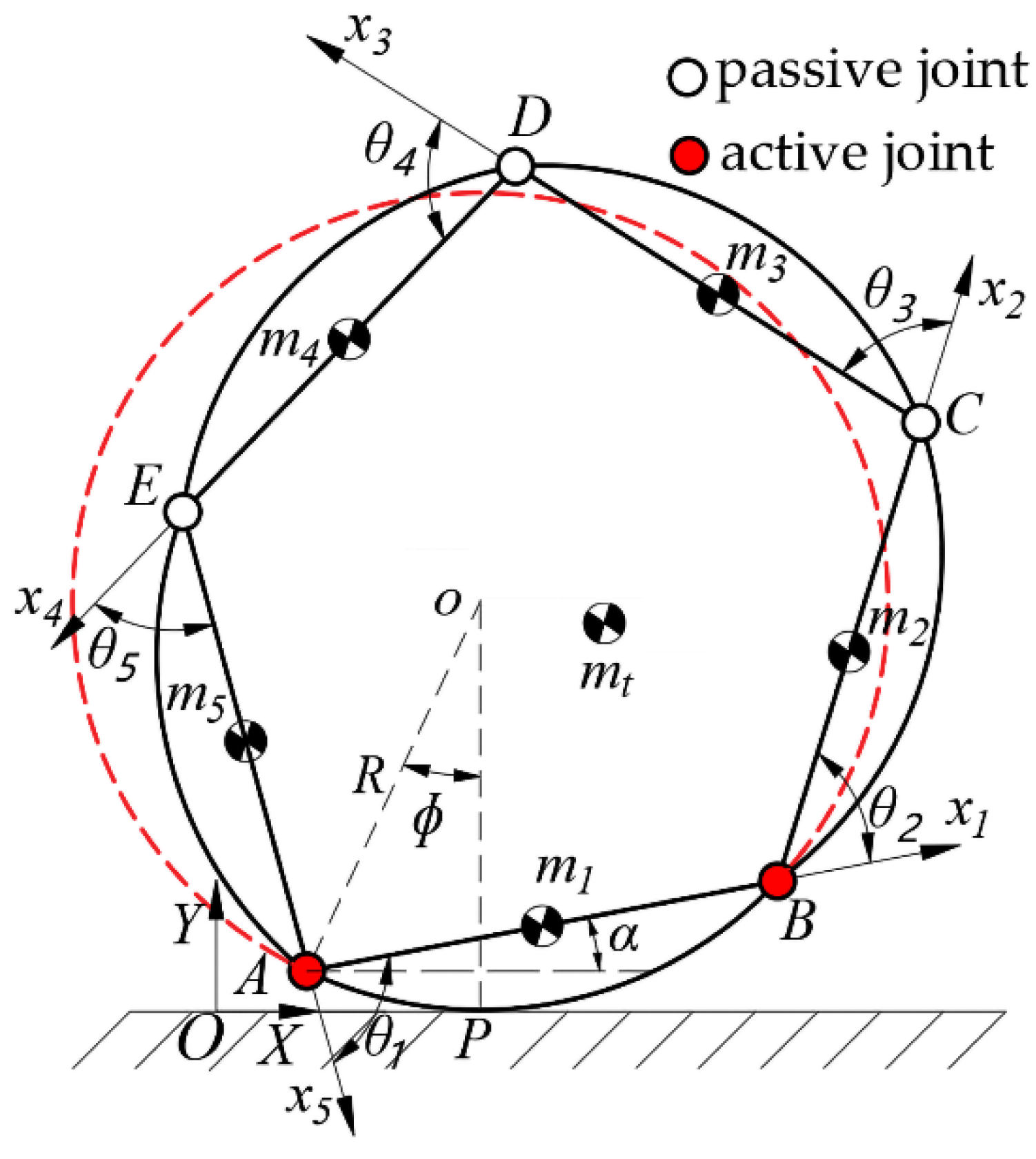
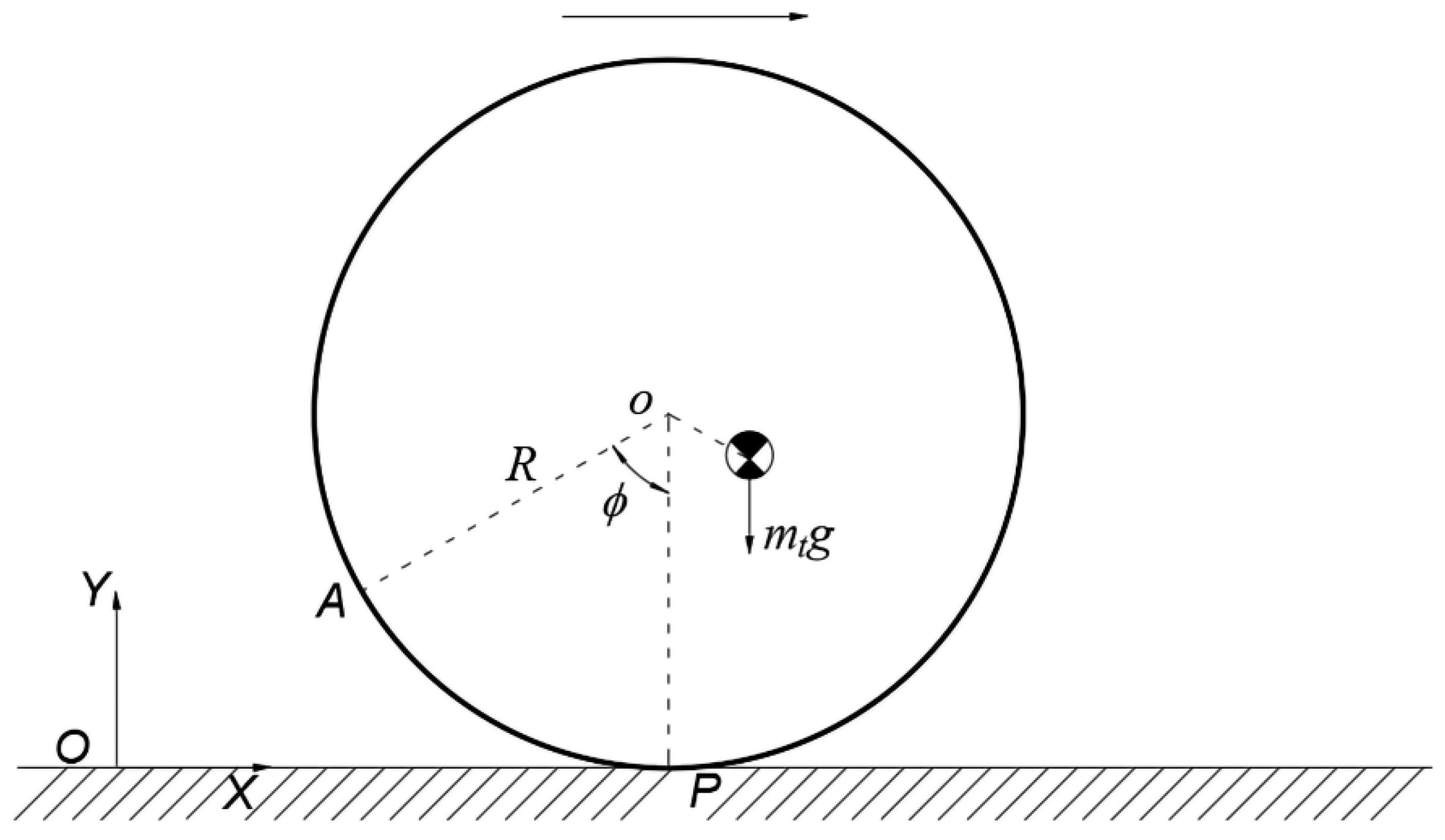
2.1. Mechanical Structure of the Rolling Mechanism
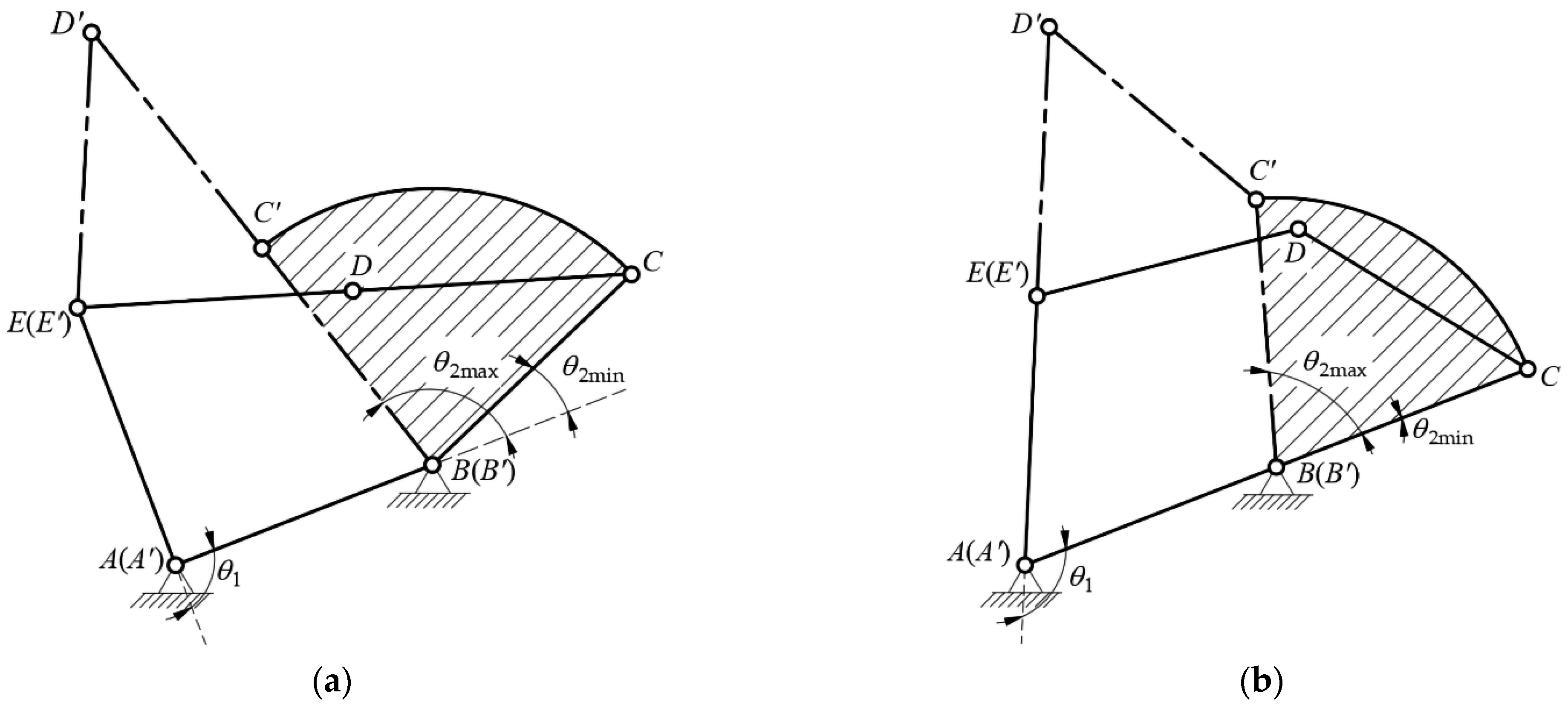
2.2. Kinematics Model
- (1)
- .
- (2)
- .
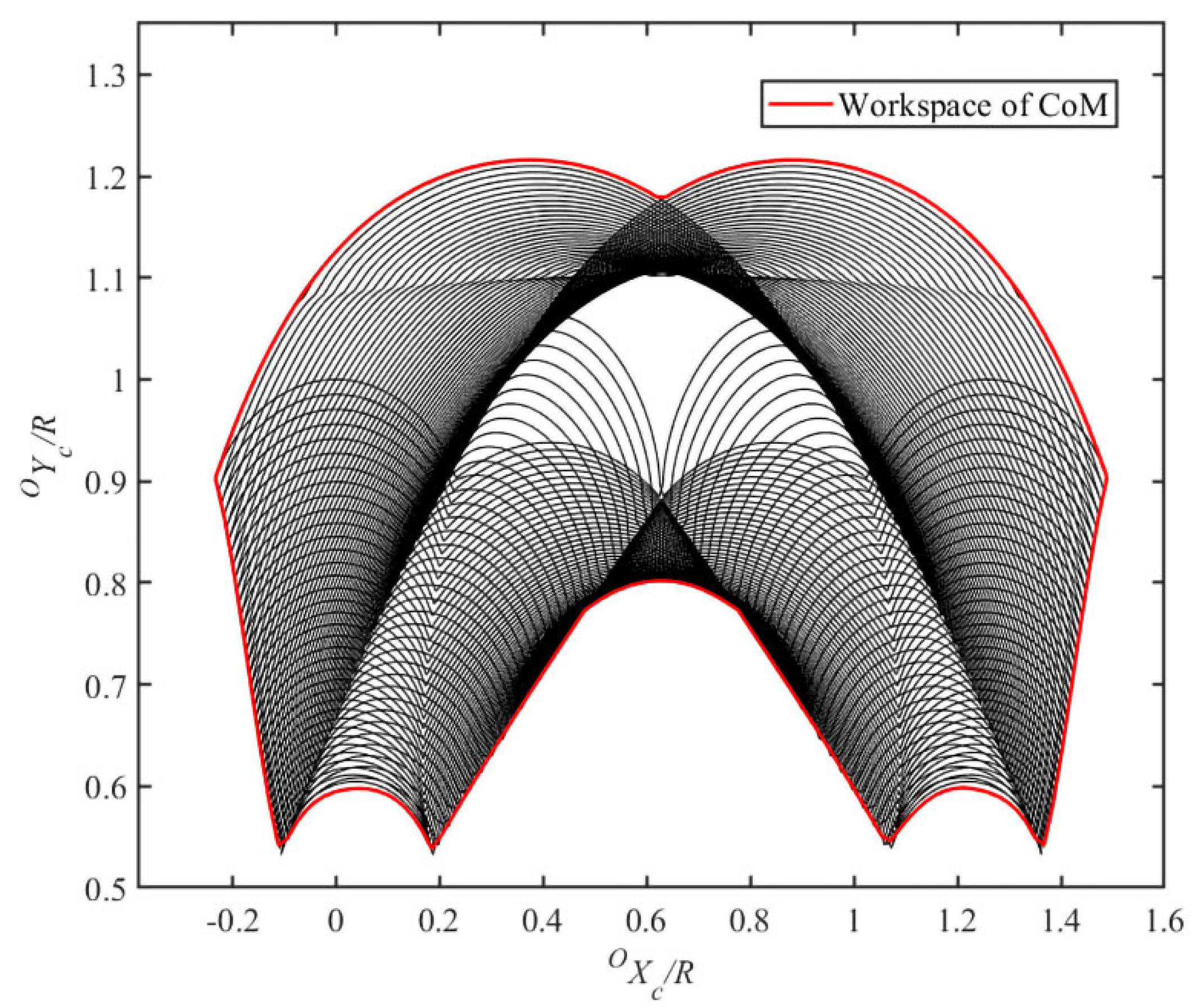
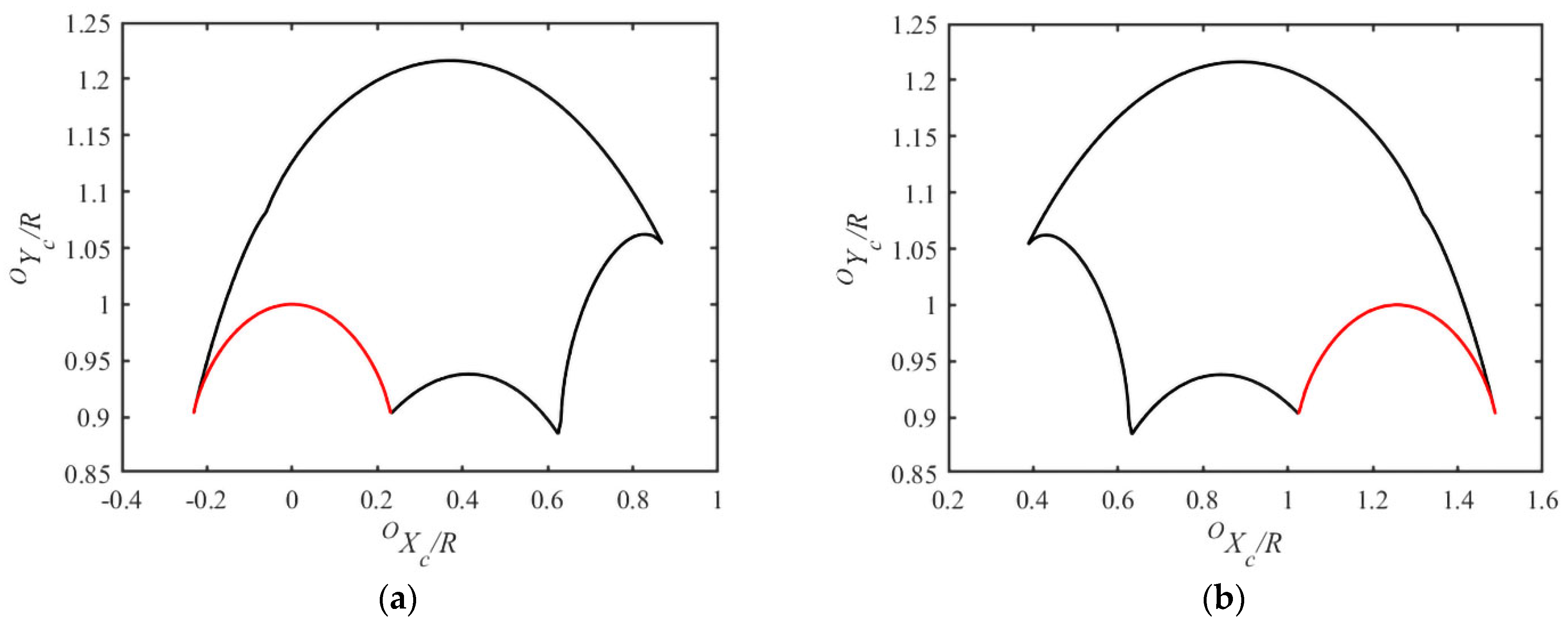
2.3. Trajectory Planning of the CoM
3. Dynamics and Energy Consumption Analysis
3.1. Dynamics Model
3.2. System Rolling Angle Acceleration Planning
3.3. Energy Consumption Analysis
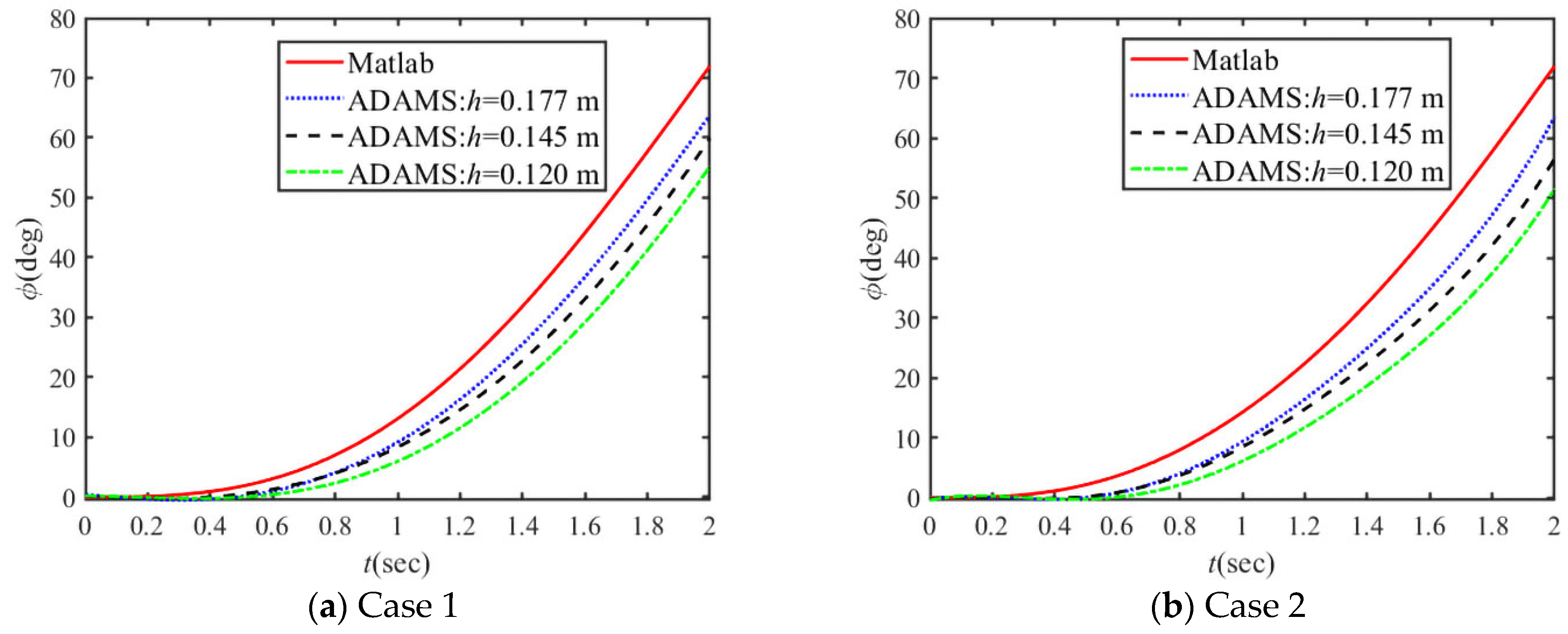
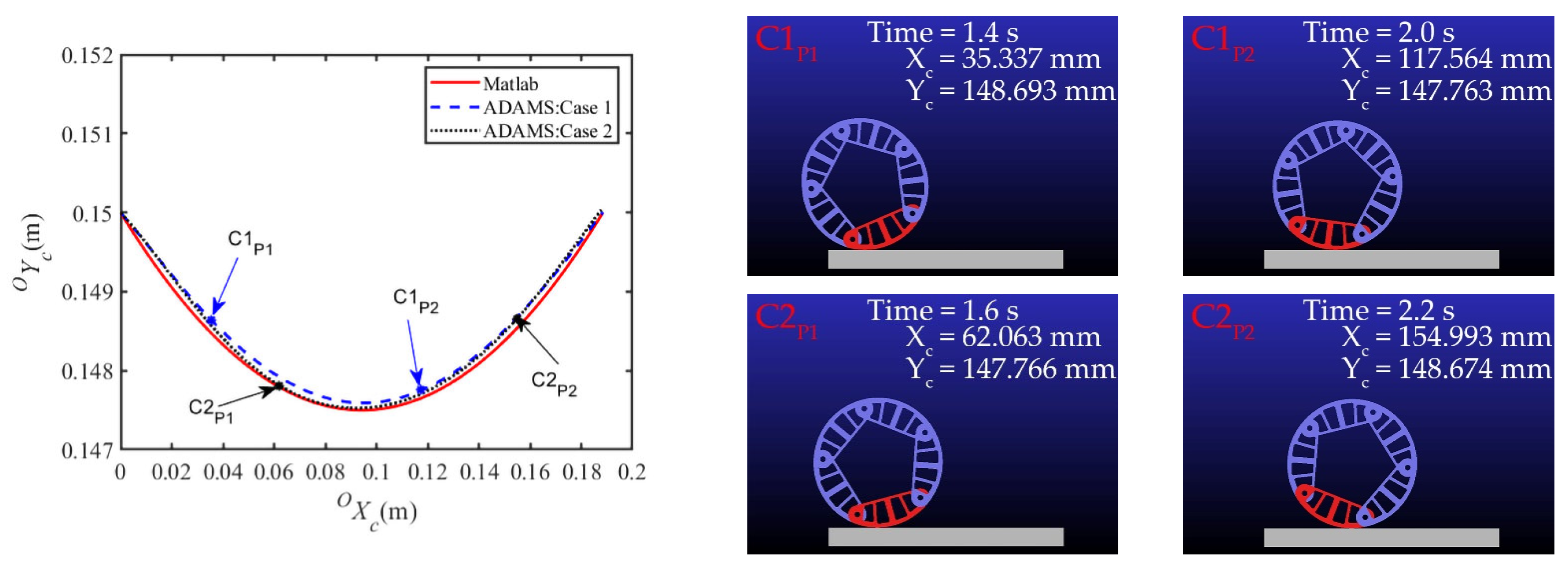
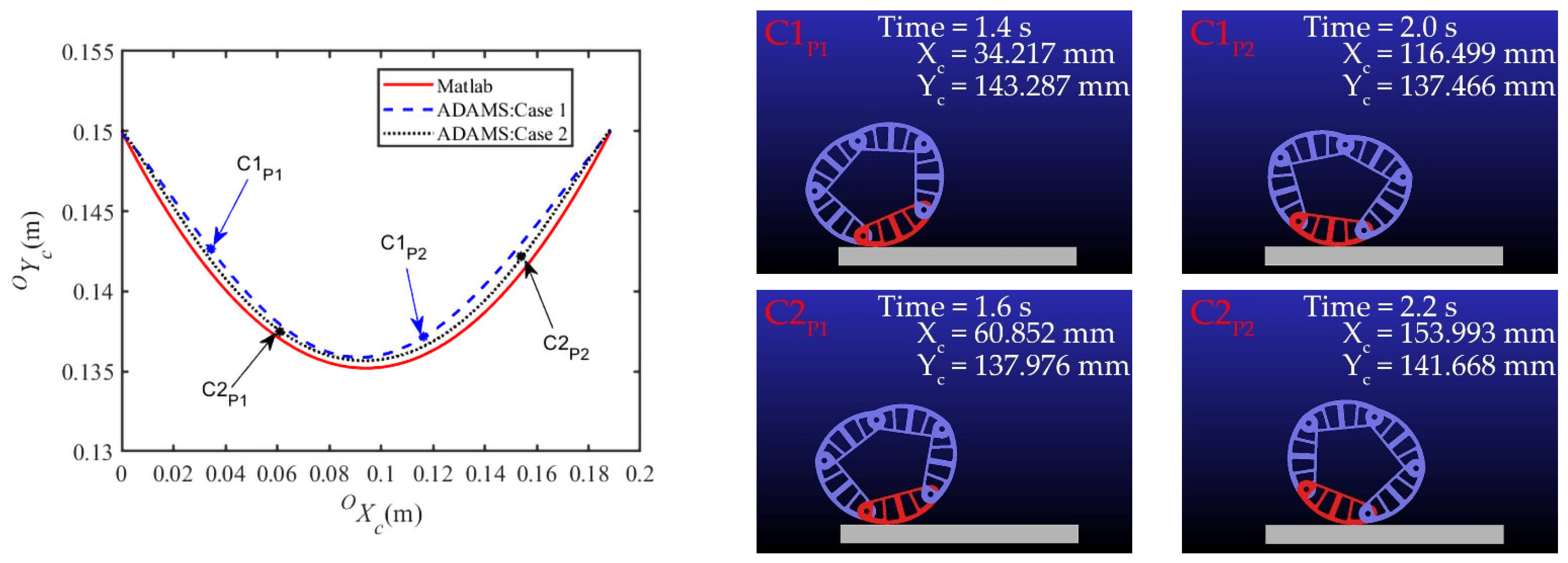
4. Virtual Prototype Simulation
5. Conclusions
- The workspace of the closed five-bow-shaped-bar linkage’s CoM with the rolling angle ranging from 0 to 2/5 is symmetric about = 0.63R.
- By high-order differentiable composite polynomial functions to plan the CoM trajectory, the mechanism obtains smooth joint trajectories.
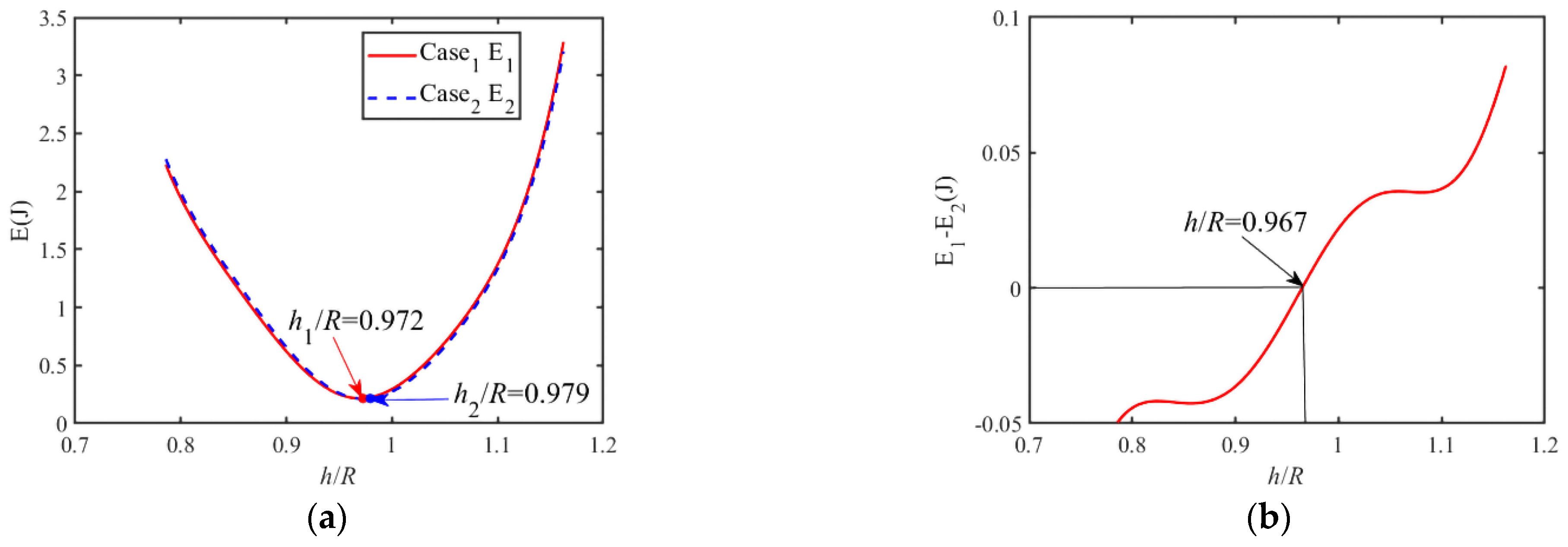
- The energy consumption decreases and then increases with the height h of the via point increasing in the schemes of sinusoid acceleration and modified trapezoidal curve acceleration, where the range of h is from 0.801R to 1.183R.
- The energy consumption of the sinusoid acceleration scheme is lowest when the height h of the via point is 0.972R, and the modified trapezoidal curve acceleration scheme is lowest when the height h of the via point is 0.979R.
- The energy consumption of the sinusoid acceleration scheme is lower when the height of the via point is less than 0.967R, and the modified trapezoidal curve acceleration scheme is lower when the height of the via point is higher than 0.967R.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Liu, R.; Yao, Y.; Li, Y. Design and analysis of a deployable tetrahedron-based mobile robot constructed by Sarrus linkages. Mech. Mach. Theory 2020, 152, 103964. [Google Scholar] [CrossRef]
- Guffanti, D.; Brunete, A.; Hernando, M. Development and validation of a ROS-based mobile robotic platform for human gait analysis applications. Robot. Auton. Syst. 2021, 145, 103869. [Google Scholar] [CrossRef]
- Wang, W.; Wang, K.; Zhang, H. Crawling gait realization of the mini-modular climbing caterpillar robot. Prog. Nat. Sci. 2009, 19, 1821–1829. [Google Scholar] [CrossRef]
- Bruzzone, L.; Quaglia, G. Review article: Locomotion systems for ground mobile robots in unstructured environments. Mech. Sci. 2012, 3, 49–62. [Google Scholar] [CrossRef]
- Paskarbeit, J.; Beyer, S.; Engel, M.; Gucze, A.; Schröder, J.; Schneider, A. Ourobot—A sensorized closed-kinematic-chain robot for shape-adaptive rolling in rough terrain. Robot. Auton. Syst. 2021, 140, 103715. [Google Scholar] [CrossRef]
- Wang, J.; Fei, Y.; Liu, Z. Locomotion modeling of a triangular closed-chain soft rolling robot. Mechatronics 2019, 57, 150–163. [Google Scholar] [CrossRef]
- Wei, X.; Tian, Y.; Wen, S. Design and locomotion analysis of a novel modular rolling robot. Mech. Mach. Theory 2019, 133, 23–43. [Google Scholar] [CrossRef]
- Wang, Y.; Wu, C.; Yu, L.; Mei, Y. Trajectory planning of a rolling robot of closed five-bow-shaped-bar linkage. Robot. Comput.-Integr. Manuf. 2018, 53, 81–92. [Google Scholar] [CrossRef]
- Wang, Y.; Wu, C.; Yu, L.; Mei, Y. Dynamics of a rolling robot of closed five-arc-shaped-bar linkage. Mech. Mach. Theory 2018, 121, 75–91. [Google Scholar] [CrossRef]
- Yu, L.; Mei, Y.; Wang, Y.; Wu, C. Research on Slope Climbing Capacity of a Close Chain Five-Bow-Shaped-Bar Linkage. In Mechanism and Machine Science; Springer: Singapore, 2016; pp. 1365–1376. [Google Scholar]
- LaValle, S.M. Planning Algorithms; Cambridge University Press: Cambridge, UK, 2006. [Google Scholar]
- Wang, H.; Wang, H.; Huang, J.; Quan, L. Smooth point-to-point trajectory planning for industrial robots with kinematical constraints based on high-order polynomial curve. Mech. Mach. Theory 2019, 139, 284–293. [Google Scholar] [CrossRef]
- Li, X.; Gao, X.; Zhang, W.; Hao, L. Smooth and collision-free trajectory generation in cluttered environments using cubic B-spline form. Mech. Mach. Theory 2022, 169, 104606. [Google Scholar] [CrossRef]
- Zhou, X.; Wang, X.; Xie, Z.; Gu, X. Online obstacle avoidance path planning and application for arc welding robot. Robot. Comput.-Integr. Manuf. 2022, 78, 102413. [Google Scholar] [CrossRef]
- Rybus, T.; Wojtunik, M.; Basmadji, F.L. Optimal collision-free path planning of a free-floating space robot using spline-based trajectories. Acta Astronaut. 2022, 190, 395–408. [Google Scholar] [CrossRef]
- Shi, J.; Sun, D.; Qin, D.; Hu, M.; Kan, Y.; Ma, K.; Chen, R. Planning the trajectory of an autonomous wheel loader and tracking its trajectory via adaptive model predictive control. Robot. Auton. Syst. 2020, 131, 103570. [Google Scholar] [CrossRef]
- Garg, A.; Aggarwal, P.; Aggarwal, Y.; Belarbi, M.O.; Chalak, H.D.; Tounsi, A.; Gulia, R. Machine learning models for predicting the compressive strength of concrete containing nano silica. Comput. Concr. 2022, 30, 33–42. [Google Scholar]
- Garg, A.; Belarbi, M.O.; Tounsi, A.; Li, L.; Singh, A.; Mukhopadhyay, T. Predicting elemental stiffness matrix of FG nanoplates using Gaussian Process Regression based surrogate model in framework of layerwise model. Eng. Anal. Bound. Elem. 2022, 143, 779–795. [Google Scholar] [CrossRef]
- Chen, G.; Lu, Y.; Yang, X.; Hu, H. Reinforcement learning control for the swimming motions of a beaver-like, single-legged robot based on biological inspiration. Robot. Auton. Syst. 2022, 154, 104116. [Google Scholar] [CrossRef]
- Yin, S.; Ji, W.; Wang, L. A machine learning based energy efficient trajectory planning approach for industrial robots. Procedia CIRP 2019, 81, 429–434. [Google Scholar] [CrossRef]
- Ji, Q.; Fu, S.; Tan, K.; Muralidharan, S.T.; Lagrelius, K.; Danelia, D.; Andrikopoulos, G.; Wang, X.V.; Wang, L.; Feng, L. Synthesizing the optimal gait of a quadruped robot with soft actuators using deep reinforcement learning. Robot. Comput.-Integr. Manuf. 2022, 78, 102382. [Google Scholar] [CrossRef]
- Mohamed, K.; Elgamal, H.; Elsharkawy, A. Dynamic analysis with optimum trajectory planning of multiple degree-of-freedom surgical micro-robot. Alex. Eng. J. 2018, 57, 4103–4112. [Google Scholar] [CrossRef]
- Liu, H.; Yao, Y.; Wang, J.; Qin, Y.; Li, T. A control architecture to coordinate energy management with trajectory tracking control for fuel cell/battery hybrid unmanned aerial vehicles. Int. J. Hydrog. Energy 2022, 47, 15236–15253. [Google Scholar] [CrossRef]
- Guo, L.; Zhang, X.; Zou, Y.; Han, L.; Du, G.; Guo, N.; Xiang, C. Co-optimization strategy of unmanned hybrid electric tracked vehicle combining eco-driving and simultaneous energy management. Energy 2022, 246, 123309. [Google Scholar] [CrossRef]
- Jin, Q.B.; Huang, J. The solar-tracking optimal trajectory planning research based on minimum energy consumption in SSPS. Aerosp. Sci. Technol. 2018, 76, 272–279. [Google Scholar] [CrossRef]
- Bo, B.; Zhang, S.; Liu, W.; Liu, L.; Shi, Y. Simulation of workspace and trajectory of a weeding mechanism. Alex. Eng. J. 2022, 61, 1133–1143. [Google Scholar] [CrossRef]
- Li, H.; Qi, C.; Gao, F.; Chen, X.; Zhao, Y.; Chen, Z. Mechanism design and workspace analysis of a hexapod robot. Mech. Mach. Theory 2022, 174, 104917. [Google Scholar] [CrossRef]
- Schreiber, L.T.; Gosselin, C. Kinematically redundant planar parallel mechanisms: Kinematics, workspace and trajectory planning. Mech. Mach. Theory 2018, 119, 91–105. [Google Scholar] [CrossRef]
- Dion-Gauvin, P.; Gosselin, C. Beyond-the-static-workspace point-to-point trajectory planning of a 6-DoF cable-suspended mechanism using oscillating SLERP. Mech. Mach. Theory 2022, 174, 104894. [Google Scholar] [CrossRef]
- Sharma, S.K.; Duvedi, R.K.; Bedi, S.; Mann, S. A multipoint tool positioning method for five-axis machining in the region of two intersecting tensor product Bézier surfaces. Int. J. Mach. Tools Manuf. 2019, 142, 42–53. [Google Scholar] [CrossRef]
- Sun, S.; Altintas, Y. A G3 continuous tool path smoothing method for 5-axis CNC machining. CIRP J. Manuf. Sci. Technol. 2021, 32, 529–549. [Google Scholar] [CrossRef]
- Fan, W.; Ji, J.; Wu, P.; Wu, D.; Chen, H. Modeling and simulation of trajectory smoothing and feedrate scheduling for vibration-damping CNC machining. Simul. Model. Pract. Theory 2020, 99, 102028. [Google Scholar] [CrossRef]
- Hu, G.; Wu, J.; Li, H.; Hu, X. Shape optimization of generalized developable H-Bézier surfaces using adaptive cuckoo search algorithm. Adv. Eng. Softw. 2020, 149, 102889. [Google Scholar] [CrossRef]
- Zhu, X.; Ji, Y.; Zhu, C.; Hu, P.; Ma, Z.D. Isogeometric analysis for trimmed CAD surfaces using multi-sided toric surface patches. Comput. Aided Geom. Des. 2020, 79, 101847. [Google Scholar] [CrossRef]
- Deng, X.; Li, R.; Zhao, L.; Wang, K.; Gui, X. Multi-obstacle path planning and optimization for mobile robot. Expert Syst. Appl. 2021, 183, 115445. [Google Scholar] [CrossRef]
- Duraklı, Z.; Nabiyev, V. A new approach based on Bezier curves to solve path planning problems for mobile robots. J. Comput. Sci. 2022, 58, 101540. [Google Scholar] [CrossRef]
- Song, B.; Wang, Z.; Zou, L. An improved PSO algorithm for smooth path planning of mobile robots using continuous high-degree Bezier curve. Appl. Soft Comput. 2021, 100, 106960. [Google Scholar] [CrossRef]
- Chen, L.; Qin, D.; Xu, X.; Cai, Y.; Xie, J. A path and velocity planning method for lane changing collision avoidance of intelligent vehicle based on cubic 3-D Bezier curve. Adv. Eng. Softw. 2019, 132, 65–73. [Google Scholar] [CrossRef]
- Xu, L.; Cao, M.; Song, B. A new approach to smooth path planning of mobile robot based on quartic Bezier transition curve and improved PSO algorithm. Neurocomputing 2022, 473, 98–106. [Google Scholar] [CrossRef]
- Dinçer, Ü.; Çevik, M. Improved trajectory planning of an industrial parallel mechanism by a composite polynomial consisting of Bézier curves and cubic polynomials. Mech. Mach. Theory 2019, 132, 248–263. [Google Scholar] [CrossRef]
- Rocha, C.R.; Tonetto, C.P.; Dias, A. A comparison between the Denavit–Hartenberg and the screw-based methods used in kinematic modeling of robot manipulators. Robot. Comput.-Integr. Manuf. 2011, 27, 723–728. [Google Scholar] [CrossRef]
- Škrjanc, I.; Klančar, G. Optimal cooperative collision avoidance between multiple robots based on Bernstein–Bézier curves. Robot. Auton. Syst. 2010, 58, 1–9. [Google Scholar] [CrossRef]
- Ostrowski, J. Reduced equations for nonholonomic mechanical systems with dissipative forces. Rep. Math. Phys. 1998, 42, 185–209. [Google Scholar] [CrossRef]








| Point | Explanation | ||
|---|---|---|---|
| 1 | 0 | R | start |
| 2 | πR/5 | via | |
| 3 | 2πR/5 | R | end |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yu, L.; Zhang, Y.; Feng, N.; Zhou, T.; Xiong, X.; Wang, Y. Energy Consumption Analysis of a Rolling Mechanism Based on a Five-Bow-Shaped-Bar Linkage. Appl. Sci. 2022, 12, 11164. https://doi.org/10.3390/app122111164
Yu L, Zhang Y, Feng N, Zhou T, Xiong X, Wang Y. Energy Consumption Analysis of a Rolling Mechanism Based on a Five-Bow-Shaped-Bar Linkage. Applied Sciences. 2022; 12(21):11164. https://doi.org/10.3390/app122111164
Chicago/Turabian StyleYu, Lianqing, Yong Zhang, Na Feng, Tiandu Zhou, Xiaoshuang Xiong, and Yujin Wang. 2022. "Energy Consumption Analysis of a Rolling Mechanism Based on a Five-Bow-Shaped-Bar Linkage" Applied Sciences 12, no. 21: 11164. https://doi.org/10.3390/app122111164
APA StyleYu, L., Zhang, Y., Feng, N., Zhou, T., Xiong, X., & Wang, Y. (2022). Energy Consumption Analysis of a Rolling Mechanism Based on a Five-Bow-Shaped-Bar Linkage. Applied Sciences, 12(21), 11164. https://doi.org/10.3390/app122111164






