A Study of the Effect of a Kinematic Pair Containing Clearance on the Dynamic Characteristics of a Tool-Changing Robot
Abstract
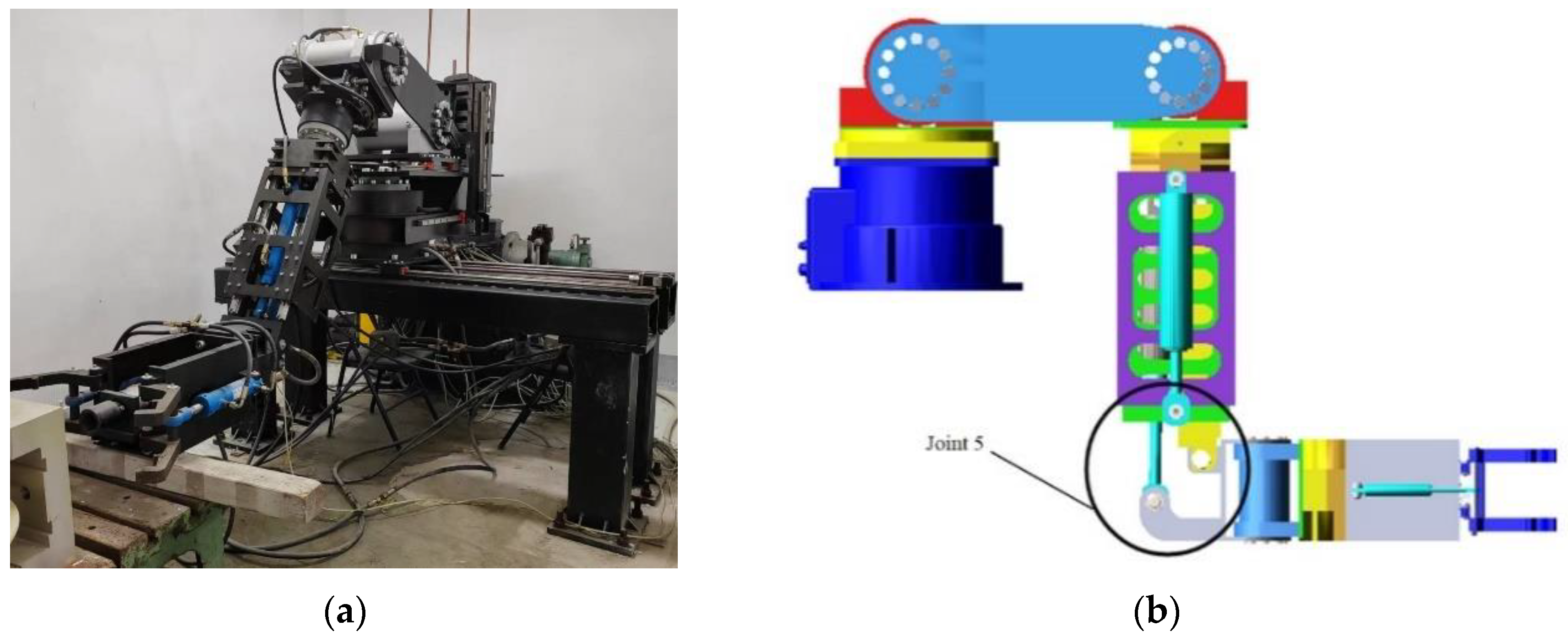
1. Introduction
2. Models about Joint with Clearance
2.1. Model of Kinematic Pairs with Clearance
2.2. Contact Force Model
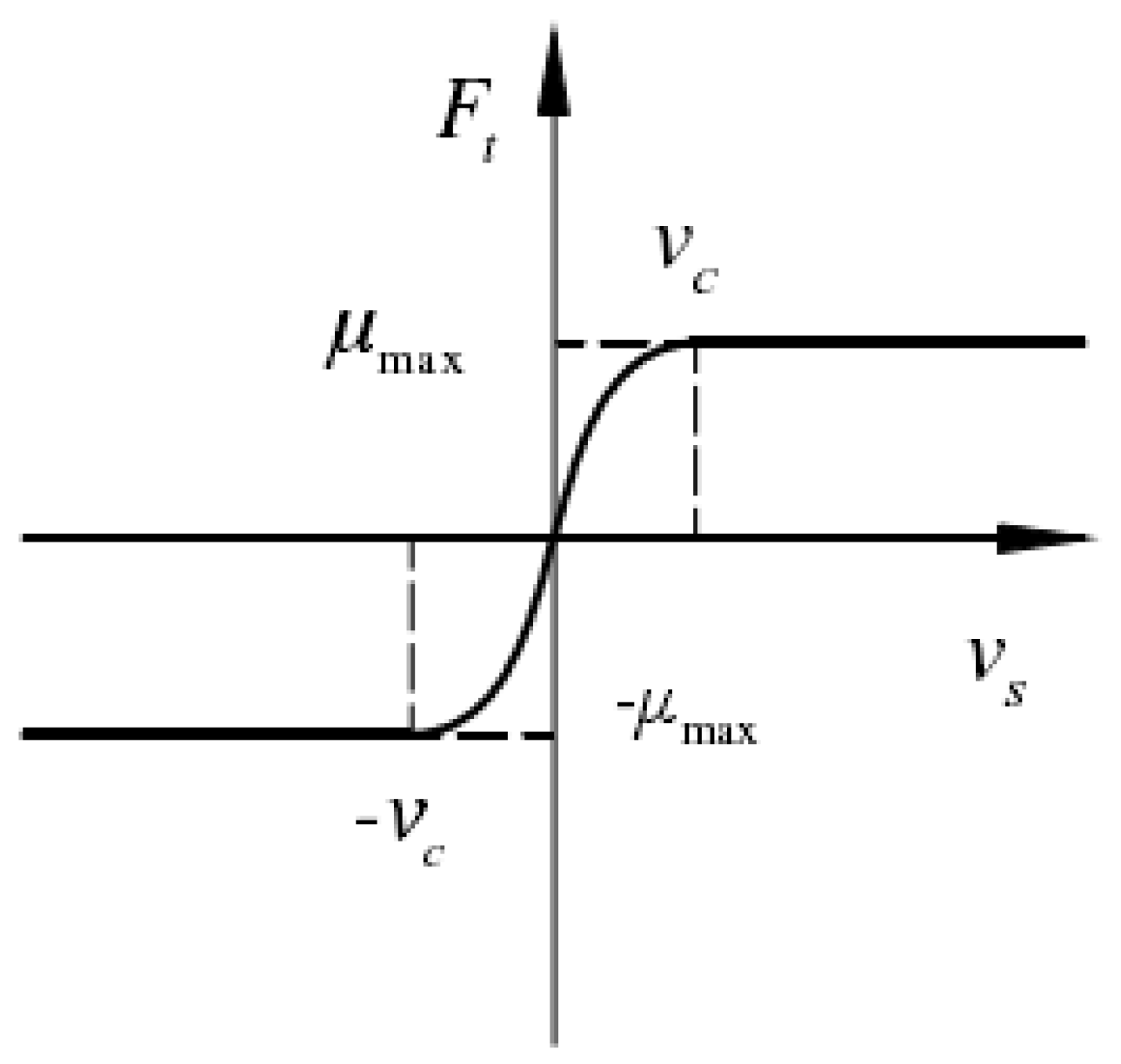
2.3. Friction Model
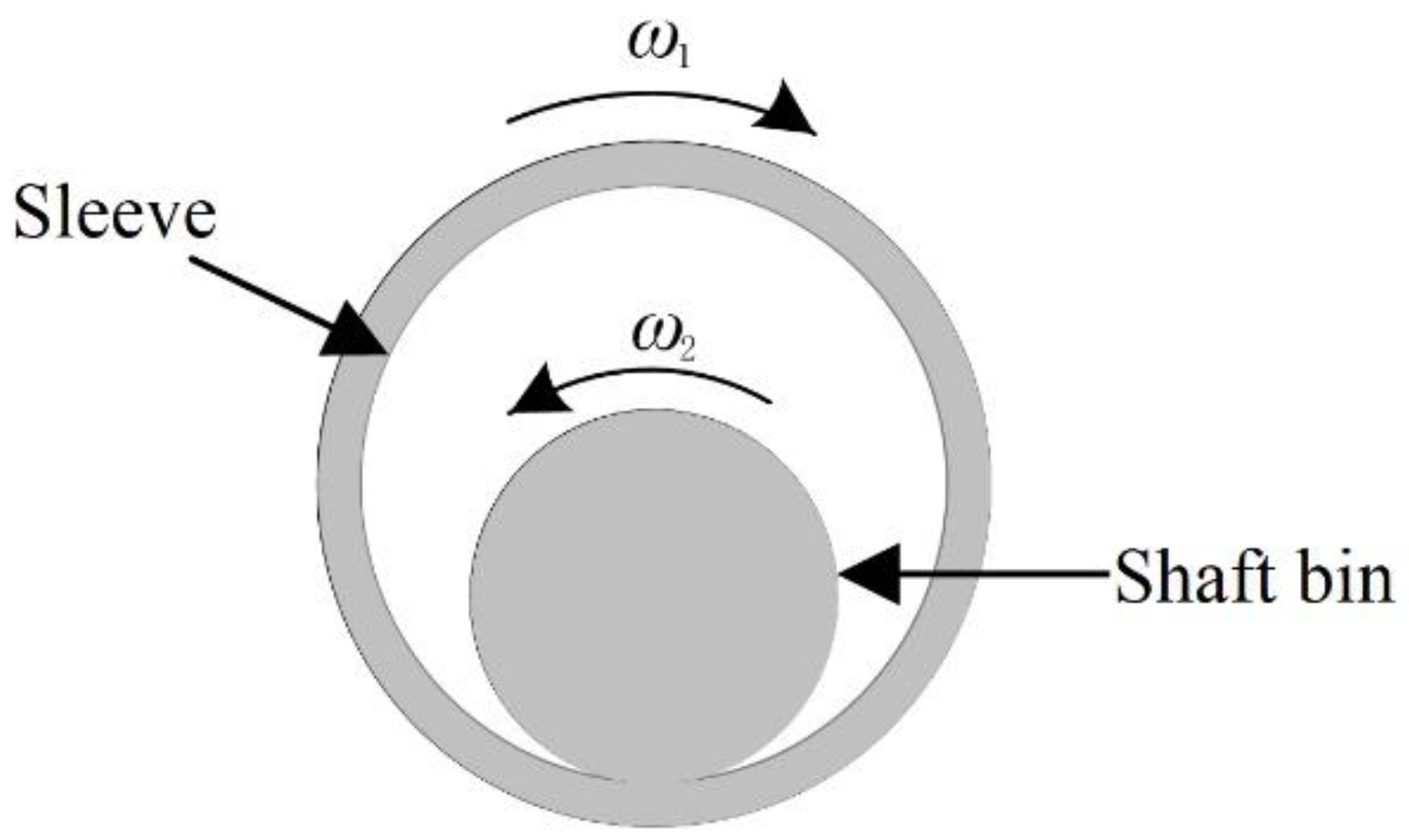
3. Wear Characteristics of Rotating Pairs with Clearance
3.1. Archard Wear Model
3.2. Determination of Wear Calculation Parameters
4. Dynamic Characteristics of Mechanism with Clearance
4.1. Determination of Wear Calculation Parameters
4.1.1. Effect of Clearance Size on the Dynamic Characteristics of Mechanisms with Clearance
4.1.2. Effect of Rotational Speed on the Dynamic Characteristics of Mechanisms with Clearance
4.1.3. Effect of Friction on the Dynamic Characteristics of Mechanisms with Clearance
4.2. Wear Analysis of Clearance Joints
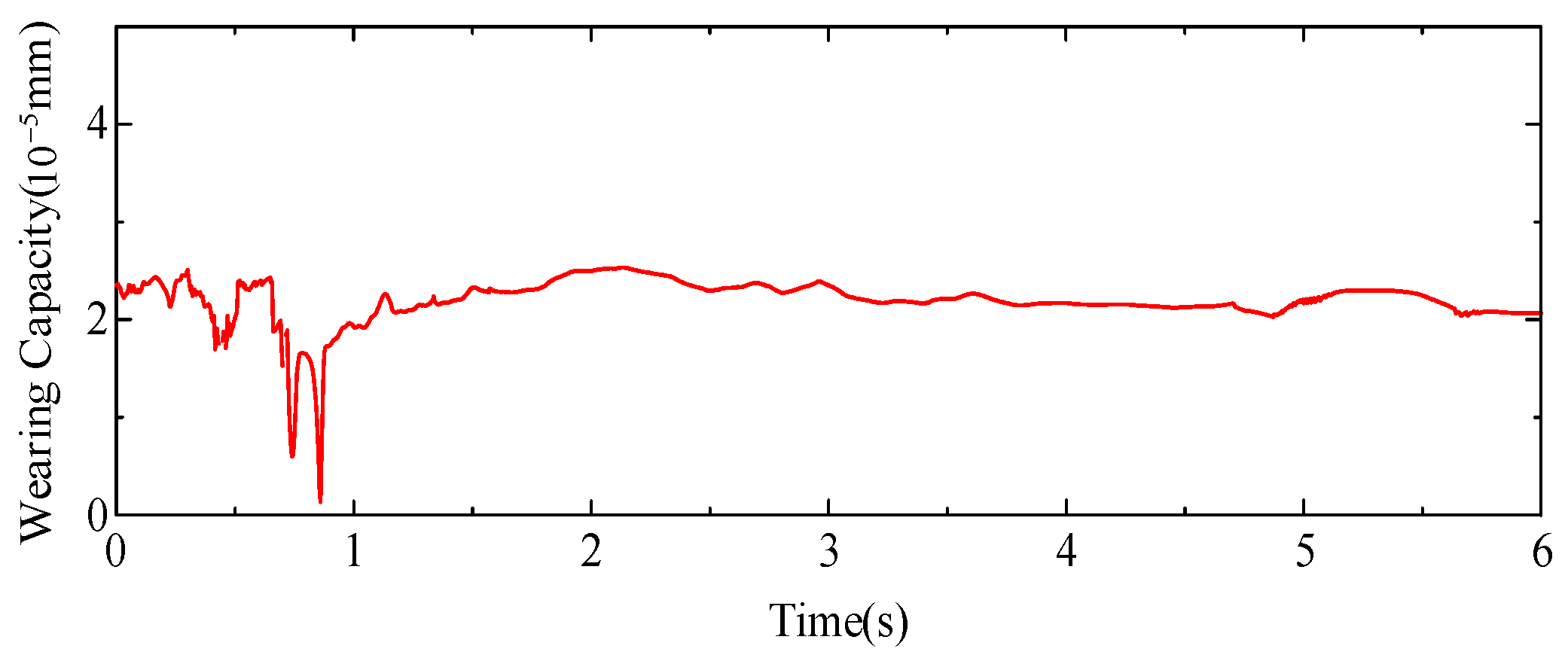
4.2.1. Wear Prediction for Rotating Joint with Clearance
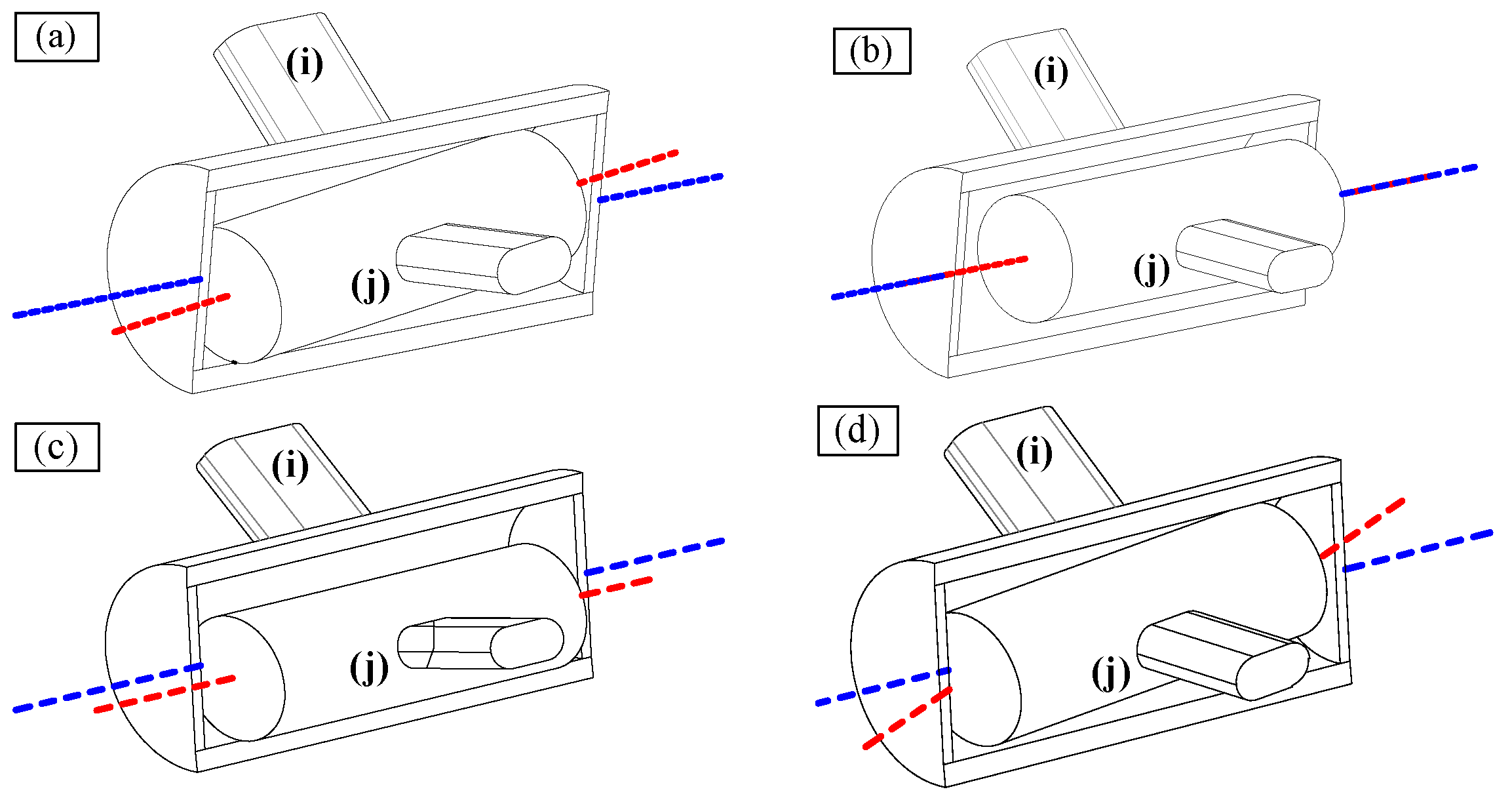
4.2.2. Rearrangement of Rotating Joint Sleeve with Clearance
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Xiang, W.; Yan, S. Dynamic analysis of space robot manipulator considering clearance joint and parameter uncertainty: Modeling, analysis and quantification. Acta Astronaut. 2020, 169, 158–169. [Google Scholar] [CrossRef]
- Liu, C.S.; Chen, B. The oblique impact dynamic study for a flexible beam undergoing large overall motion. Acta Mech. Sin. 2000, 32, 457–465. [Google Scholar]
- Masumi, O.; Takeda, Y. Design of target trajectories for the detection of joint clearances in parallel robot based on the actuation torque measurement. Mech. Mach. Theory 2021, 155, 104081. [Google Scholar]
- Cao, D.Q.; Chu, S.M.; Li, Z.F. Study on the non-smooth mechanical models and dynamics for space deployable mechanism. Chin. J. Theor. Mech. 2013, 45, 3–14. [Google Scholar]
- Chen, X.; Yang, W. Dynamic modeling and analysis of spatial parallel mechanism with revolute joints considering radial and axial clearances. Nonlinear Dyn. 2021, 106, 1929–1953. [Google Scholar] [CrossRef]
- Ting, K.L.; Hsu, K.L.; Yu, Z. Clearance-induced output position uncertainty of planar linkages with revolute and prismatic joints. Mech. Mach. Theory 2016, 111, 66–75. [Google Scholar] [CrossRef]
- Lara-Molina, F.A.; Dumur, D. A fuzzy approach for the kinematic reliability assessment of robotic manipulators. Robotica 2021, 39, 2095–2109. [Google Scholar] [CrossRef]
- Dubowsky, S.; Freudenstein, F. Dynamic analysis of mechanical systems with clearances, Part 1: Formulation of dynamic model. J. Eng. Ind. 1971, 93, 305–309. [Google Scholar] [CrossRef]
- Jiang, S.; Wang, T.; Xiao, L. Experiment research and dynamic behavior analysis of multi-link mechanism with wearing clearance joint. Nonlinear Dyn. 2022, 109, 1325–1340. [Google Scholar] [CrossRef]
- Birhanu, F.F.; Chen, Z.B.; Ma, W.S. Modeling and simulation satellite solar panel development and locking. Inf. Tech. J. 2010, 9, 600–604. [Google Scholar]
- Chen, S.; Cheng, G.; Pang, Y. Dynamic analysis and trajectory tracking control for a parallel manipulator with joint friction. Appl. Sci. 2022, 12, 6682. [Google Scholar] [CrossRef]
- Le Chau, N.; Dang, M.P.; Prakash, C.; Buddhi, D.; Dao, T.H. Structural optimization of a rotary joint by hybrid method of fem, neural-fuzzy and water cycle–moth flame algorithm for robotics and automation manufacturing. Robotics and Autonomous Systems. Robot. Auton. Syst. 2022, 156, 104199. [Google Scholar] [CrossRef]
- Yang, B.; Yang, W.; Zhang, G.; Ran, Y. Precision analysis of series-parallel machine tool based on hierarchy motion function units. Mech. Mach. Theory 2022, 172, 104834. [Google Scholar] [CrossRef]
- Earles, S.W.E.; Wu, C.L.S. Motion analysis of a rigid-link mechanism with clearance at a bearing using Lagrangian mechanism and digital computation. Mechanisms 1973, 1, 83–89. [Google Scholar]
- Flores, P.; Lankarani, H.M. Dynamic response of multibody systems with multiple clearance joints. J. Comput. Nonlin. Dyn. 2011, 7, 636–647. [Google Scholar]
- Muvengei, M.; Kihiu, J.; Ikua, B. Effects of clearance size on the dynamic response of planar multi-body systems with differently located frictionless revolute clearance joints. J. Energ. Tech. Policy 2012, 2, 7–19. [Google Scholar]
- Varedi, S.M.; Danili, H.M.; Dardel, M. Optimal dynamic design of a planar slider-crank mechanism with a joint clearance. Mech. Mach. Theory 2015, 86, 191–200. [Google Scholar] [CrossRef]
- Shi, B.; Jin, Y. Dynamic simulation and modeling of revolute clearance joint for virtual prototyping. J. Mech. Eng. 2009, 45, 299–303. [Google Scholar] [CrossRef]
- Zhao, Y.; Bai, Z.F.; Wang, X.G. Dynamics analysis of two-axis-position mechanism of satellite antennas with joint clearance. J. Astronaut. 2010, 31, 1533–1539. [Google Scholar]
- Efremenkov, E.A.; Martyushev, N.V.; Skeeba, V.Y.; Grechneva, M.V.; Olisov, A.V.; Ens, A.D. Research on the possibility of lowering the manufacturing accuracy of cycloid transmission wheels with intermediate rolling elements and a free cage. Appl. Sci. 2022, 12, 5. [Google Scholar] [CrossRef]
- Hao, X.Q.; Chen, J.Y. Effects of different materials in joints on dynamic characteristics of a mechanism with clearance. J. Vib. Shock. 2012, 31, 19–21. [Google Scholar]
- Xu, L.X.; Yang, Y.H.; Li, Y.G. Modeling and analysis of planar multibody systems containing deep groove ball bearing with clearance. Mech. Mach. Theory 2002, 56, 69–88. [Google Scholar] [CrossRef]
- Fateh, M.M.; Khorashadizadeh, S. Robust control of electrically driven robots by adaptive fuzzy estimation of uncertainty. Nonlinear Dyn. 2012, 69, 1465–1477. [Google Scholar] [CrossRef]
- Yuan, X.; Meng, Q.; Xie, F.; Liu, X.-J.; Wang, J. Position error modeling and accuracy evaluation of n-dof translational parallel manipulators that can be transformed into n four-bar mechanisms based on motion/force transmissibility. Mech. Mach. Theory 2022, 176, 105012. [Google Scholar] [CrossRef]
- Pereira, M.S.; Nikravesh, P. Impact dynamics of multibody systems with frictional contact using joint coordinates and canonical equations of motion. Nonlinear Dyn. 1996, 9, 53–71. [Google Scholar] [CrossRef]
- Guo, A.; Batzer, S. Substructures analysis of a flexible system contact-impact event. Trans. ASME 2004, 126, 126–131. [Google Scholar] [CrossRef]
- Yu, L.X.; Liu, C.S. Dynamic simulation and kinetic description of revolute joint with spatial clearance. Acta Scicentiarum Nat. Univ. Pekinesis. 2005, 41, 679–686. [Google Scholar]
- Zhao, Y.; Bai, Z.F. Dynamics analysis of space robot manipulator with joint clearance. Acta Astronaut. 2011, 68, 1147–1155. [Google Scholar] [CrossRef]
- Su, Y.W.; Chen, W.; Zhu, A. Numerical analysis of wear coupling with dynamics behavior of machine. Tribology 2009, 29, 50–54. [Google Scholar]
- Zhao, Q.; Guo, J.; Hong, J. Time-dependent system kinematic reliability analysis for planar parallel manipulators. Mech. Mach. Theory 2020, 152, 103939. [Google Scholar] [CrossRef]
- Zhang, Y.J.; Zhao, N.; Lu, J. Finite element modal analysis of reciprocating compressor crankshaft based on Ansys workbench. Appl. Mech. Mater. 2014, 488–489, 1208–1210. [Google Scholar] [CrossRef]
- Freidovich, L.; Robertsson, A. Lugre-model-based friction compensation. IEEE T. Contr. Syst. T. 2010, 18, 194–200. [Google Scholar] [CrossRef]
- Liu, L.L.; Liu, H.Z.; Wu, Z.Y. Research progress of friction model in mechanical system. Adv. Mech. 2008, 38, 201–213. [Google Scholar]
- Zhou, Z.; Zheng, X.; Wang, Q.; Chen, Z.; Sun, Y.; Liang, B. Modeling and simulation of point contact multibody system dynamics based on the 2d Lugre friction model. Mech. Mach. Theory 2021, 158, 104244. [Google Scholar] [CrossRef]
- Popov, V.L. Generalized archard law of wear based on rabinowicz criterion of wear particle formation. Facta Univ. Ser. Mech. Eng. 2019, 17, 39–45. [Google Scholar] [CrossRef]
- Hanief, M.; Charoo, M.S. Archard’s wear law revisited to measure accurate wear coefficient considering actual sliding velocity. Mater. Today Proc. 2021, 47, 5598–5600. [Google Scholar] [CrossRef]
- Lankarani, H.M.; Nikravesh, P.E. A contact force model with hysteresis damping for impact analysis of multibody systems. J. Mech. Design 1990, 112, 369–376. [Google Scholar] [CrossRef]
- Zhang, J.; Fang, M.Y.; Zhao, L.; Zhao, Q.L.; Liang, X.; He, G.P. A continuous contact force model for the impact analysis of hard and soft materials. Mech. Mach. Theor. 2022, 177, 105065. [Google Scholar] [CrossRef]
- Flores, P.; Ambrósio, J.; Claro, J.C.P.; Lankarani, H.M.; Koshy, C.S. A study on dynamics of mechanical systems including joints with clearance and lubrication. Mech. Mach. Theor. 2006, 41, 247–261. [Google Scholar] [CrossRef]
- Li, Y.Y.; Yang, Y.; Li, M.; Liu, Y.F.; Huang, Y.F. Dynamics analysis and wear prediction of rigid-flexible coupling deployable solar array system with clearance joints considering solid lubrication. Mech. Syst. Signal. Proc. 2022, 162, 108059. [Google Scholar] [CrossRef]
- Lai, X.; He, H.; Lai, Q.; Wang, C.; Yang, J.; Zhang, Y.; Fang, H.; Liao, S. Computational Prediction and Experimental Validation of Revolute Joint Clearance Wear in the Low-velocity Planar Mechanism. Mech. Syst. Signal Process. 2017, 85, 963–976. [Google Scholar] [CrossRef]
- Archard, J.F. Contact and Rubbing of Flat Surfaces. J. Appl. Phys. 1953, 24, 981–988. [Google Scholar] [CrossRef]
- Aghababaei, R.; Zhao, K. Micromechanics of material detachment during adhesive wear: A numerical assessment of Archard’s wear model. Wear 2021, 476, 203739. [Google Scholar] [CrossRef]

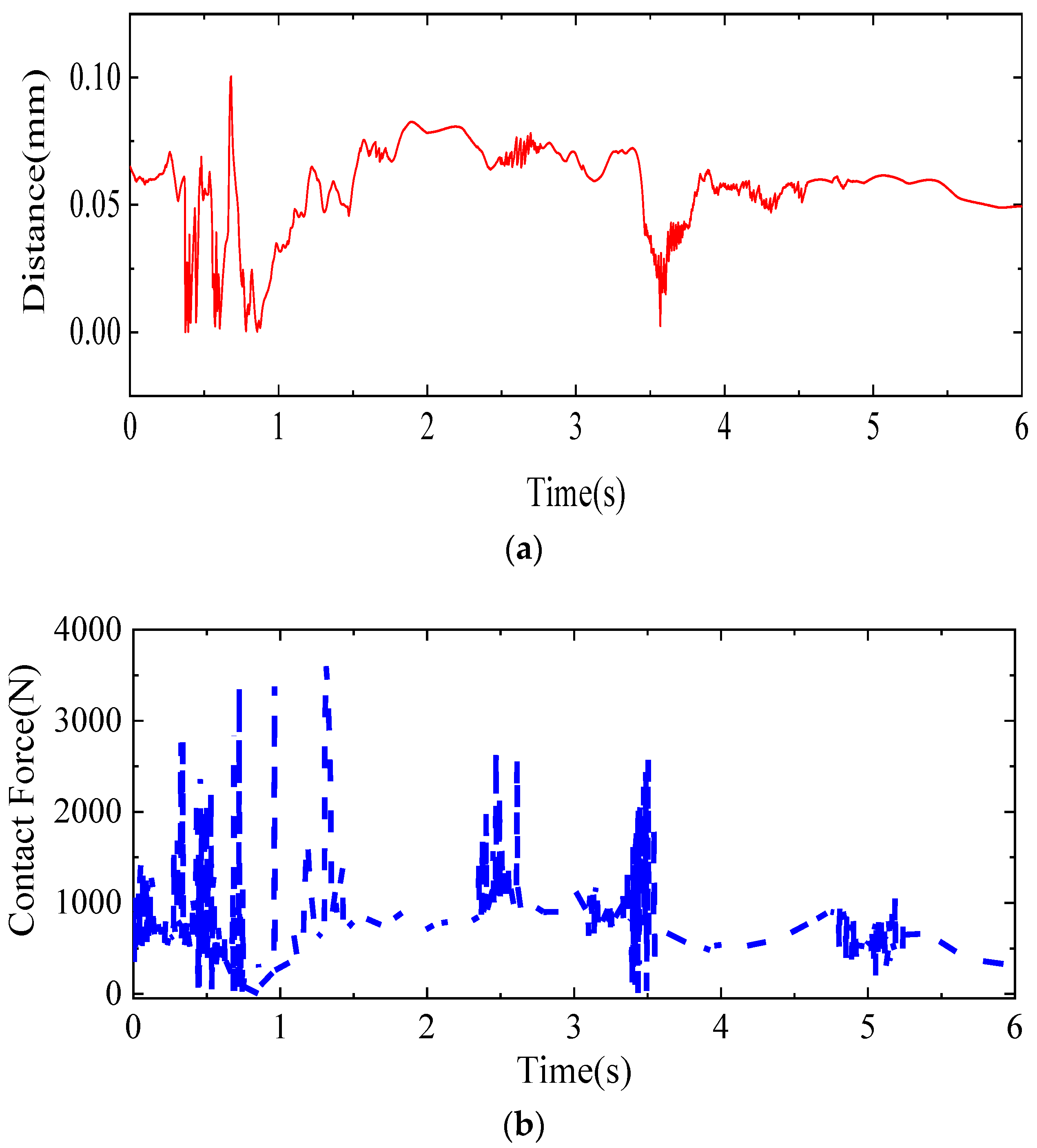
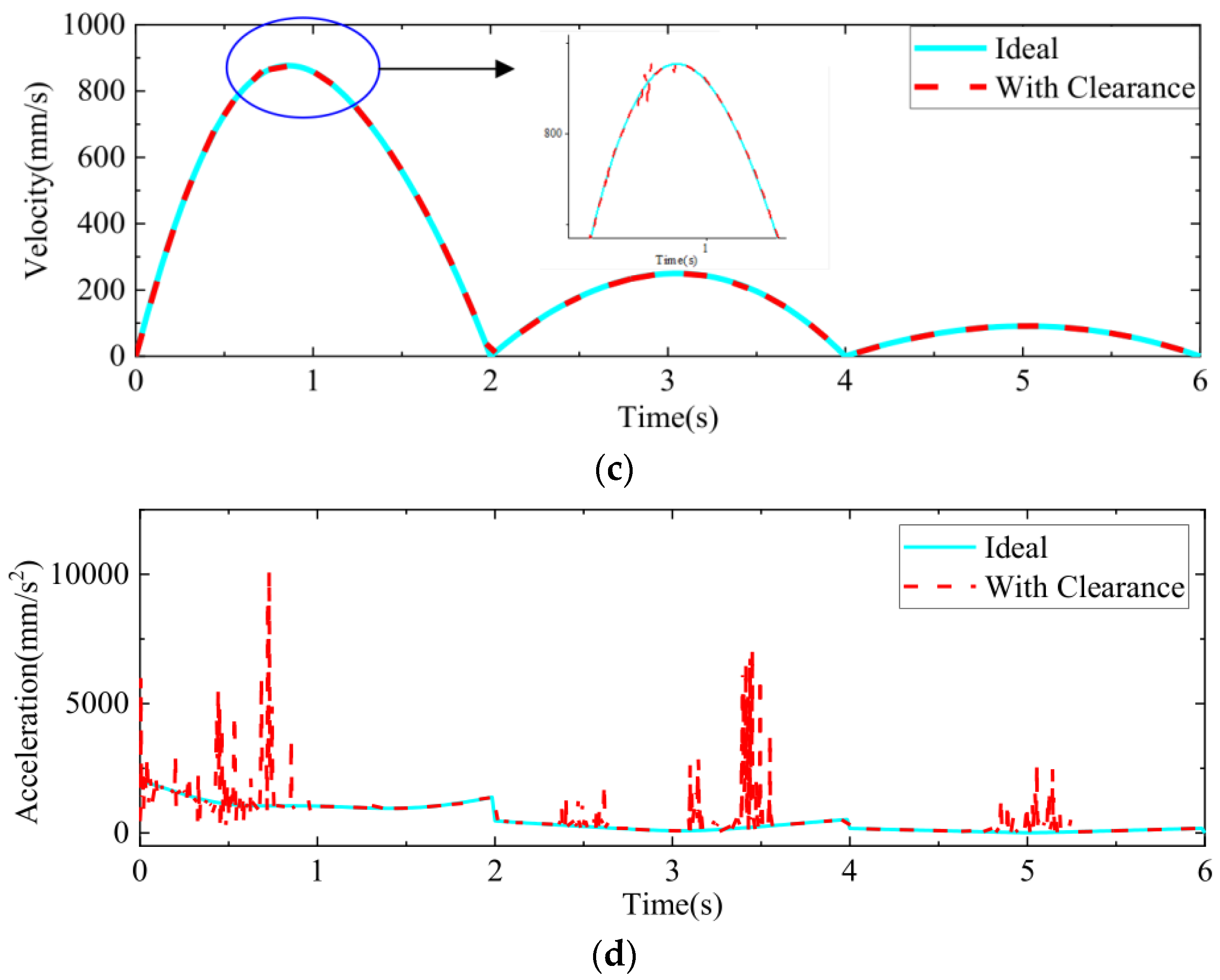




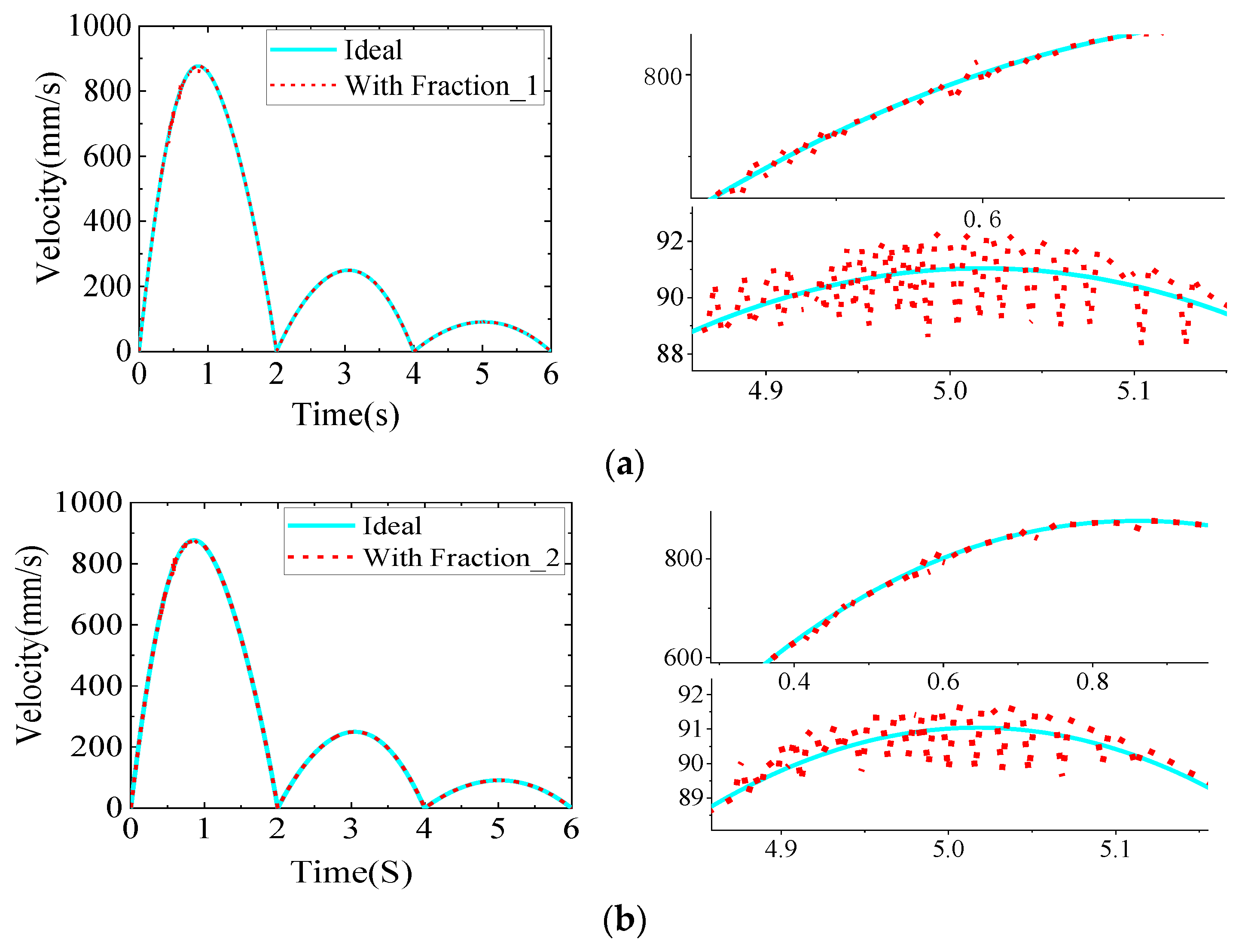

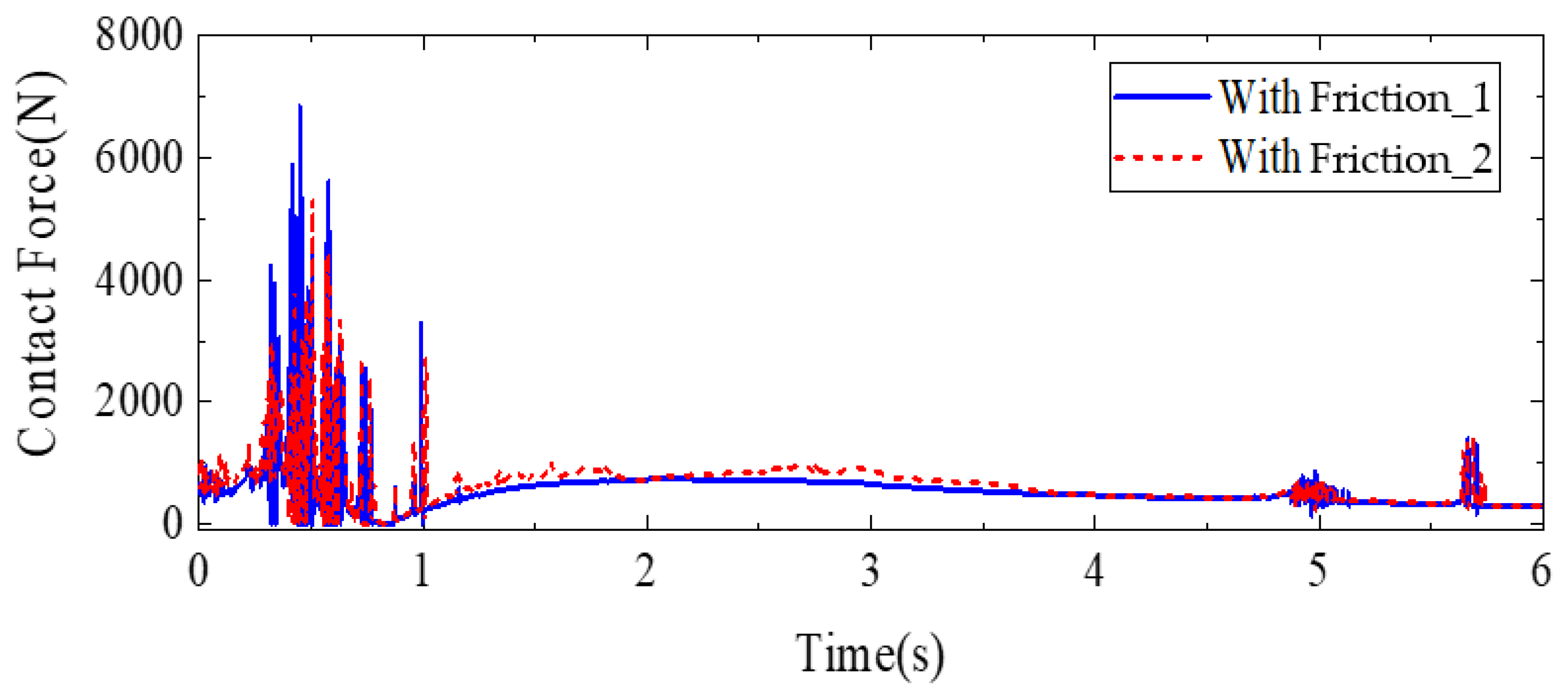

| Parameters | Pin (Radius: 25 mm) | Sleeve (Radius: 25.05 mm) |
|---|---|---|
| R | 12.512 | |
| K | 2.487E + 6 | |
| D | ||
| Elastic Modulus | 2.01E + 5 | 2.06E + 5 |
| Poisson ratio | 0.3 | |
| Velocity Number | Joint Driving Function |
|---|---|
| Velocity_1 | step(time, 0, 0, 0.5, 90 d) + step(time, 0.5, 0, 1, 30 d) + step(time, 1, 0, 1.5, 10 d) |
| Velocity_2 | step(time, 0, 0, 1, 90 d) + step(time, 1, 0, 2, 30 d) + step(time, 2, 0, 3, 10 d) |
| Velocity_3 | step(time, 0, 0, 2, 90 d) + step(time, 2, 0, 4, 30 d) + step(time, 4, 0, 6, 10 d) |
| Velocity_4 | step(time, 0, 0, 3, 90 d) + step(time, 3, 0, 6, 30 d) + step(time, 6, 0, 8, 10 d) |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Qian, W.; Song, S.; Zhao, J.; Hou, J.; Wang, L.; Yin, X. A Study of the Effect of a Kinematic Pair Containing Clearance on the Dynamic Characteristics of a Tool-Changing Robot. Appl. Sci. 2022, 12, 11041. https://doi.org/10.3390/app122111041
Qian W, Song S, Zhao J, Hou J, Wang L, Yin X. A Study of the Effect of a Kinematic Pair Containing Clearance on the Dynamic Characteristics of a Tool-Changing Robot. Applied Sciences. 2022; 12(21):11041. https://doi.org/10.3390/app122111041
Chicago/Turabian StyleQian, Wenxue, Shuai Song, Jianhang Zhao, Jinqiang Hou, Lei Wang, and Xiaowei Yin. 2022. "A Study of the Effect of a Kinematic Pair Containing Clearance on the Dynamic Characteristics of a Tool-Changing Robot" Applied Sciences 12, no. 21: 11041. https://doi.org/10.3390/app122111041
APA StyleQian, W., Song, S., Zhao, J., Hou, J., Wang, L., & Yin, X. (2022). A Study of the Effect of a Kinematic Pair Containing Clearance on the Dynamic Characteristics of a Tool-Changing Robot. Applied Sciences, 12(21), 11041. https://doi.org/10.3390/app122111041






