All-Optical Method of Determining Laser Power from the Photomechanical Effect
Abstract
1. Introduction
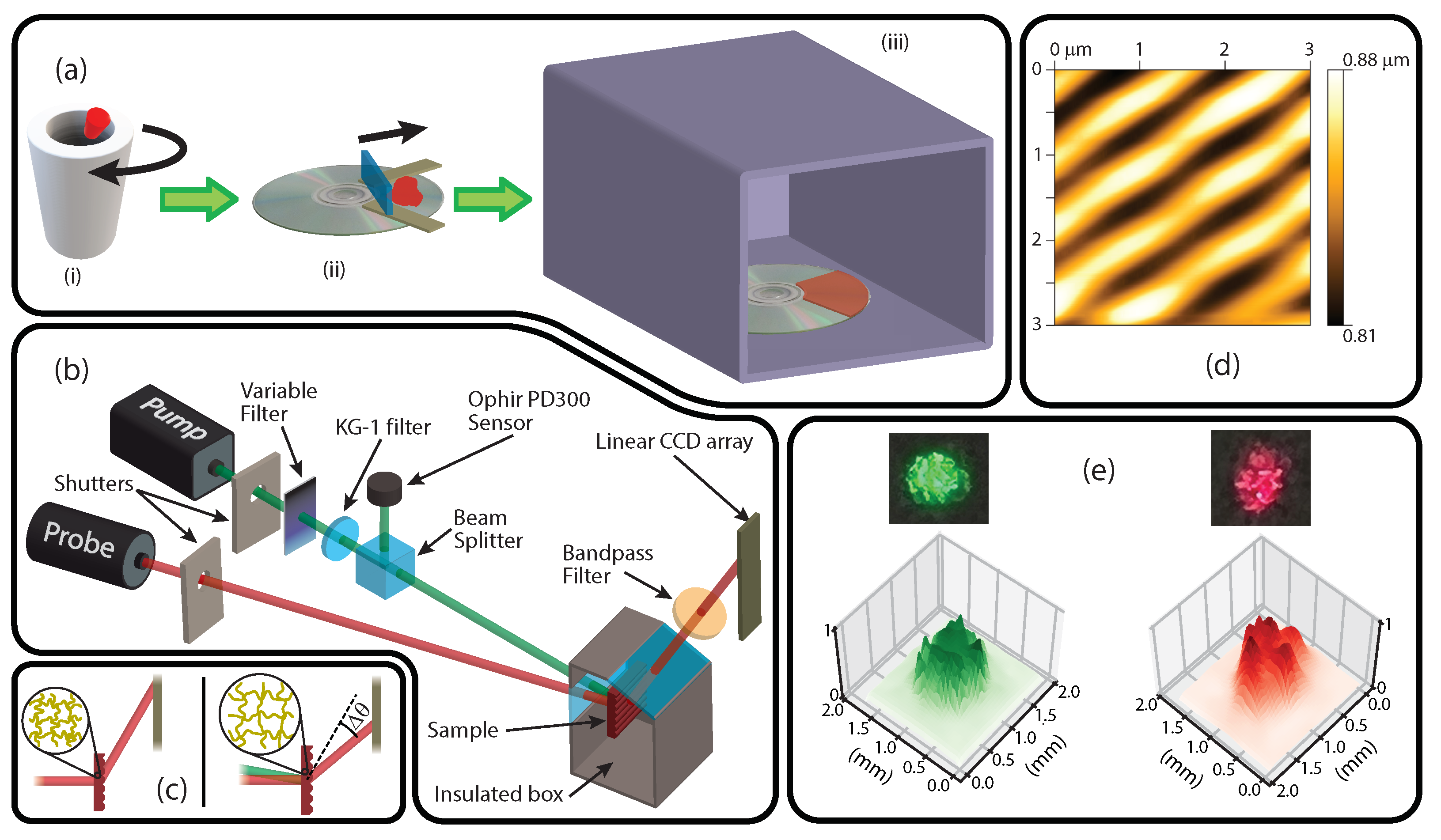
2. Materials and Methods
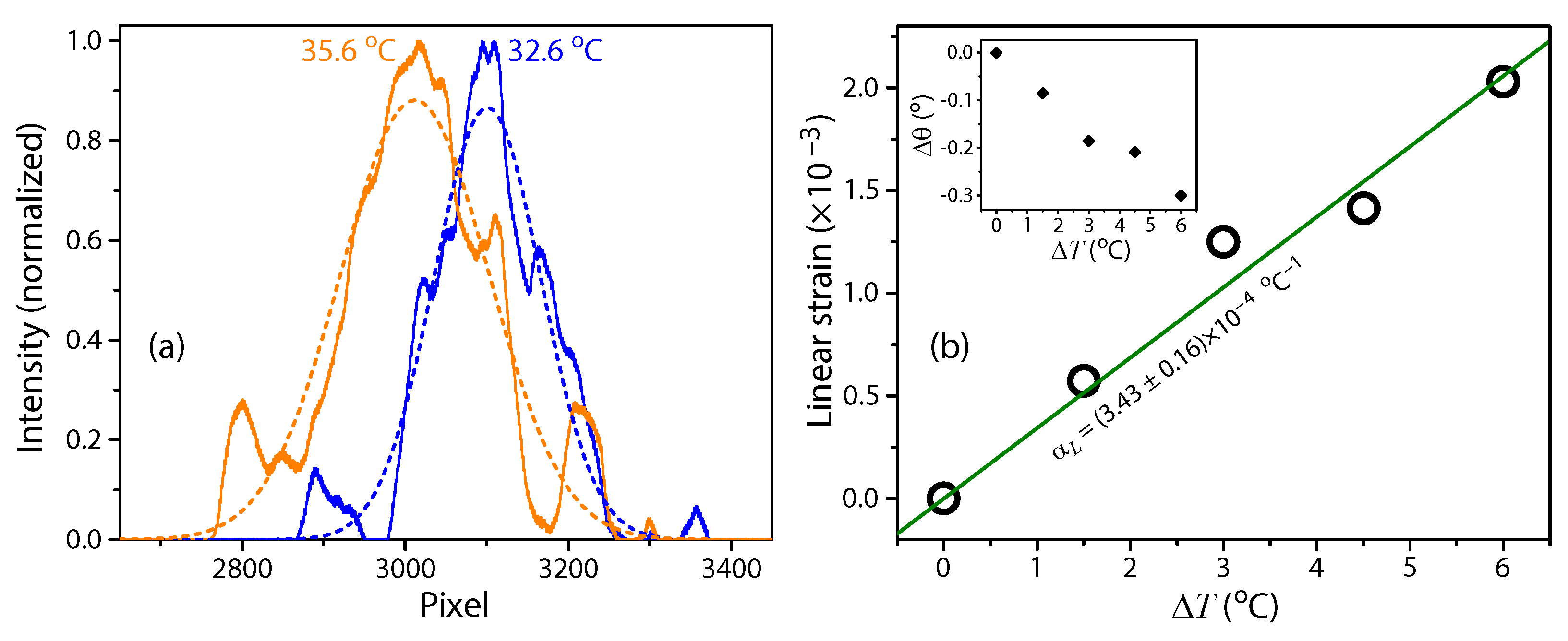
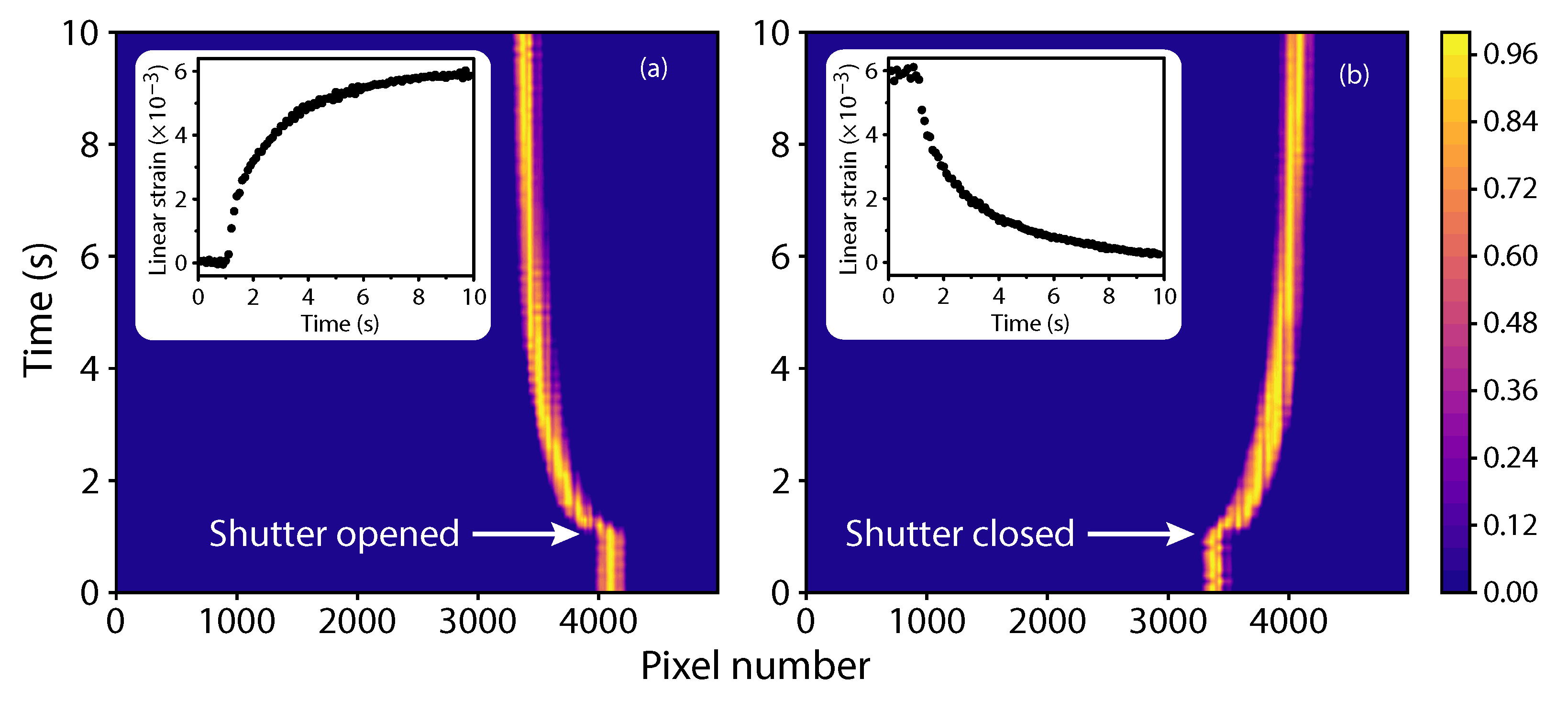
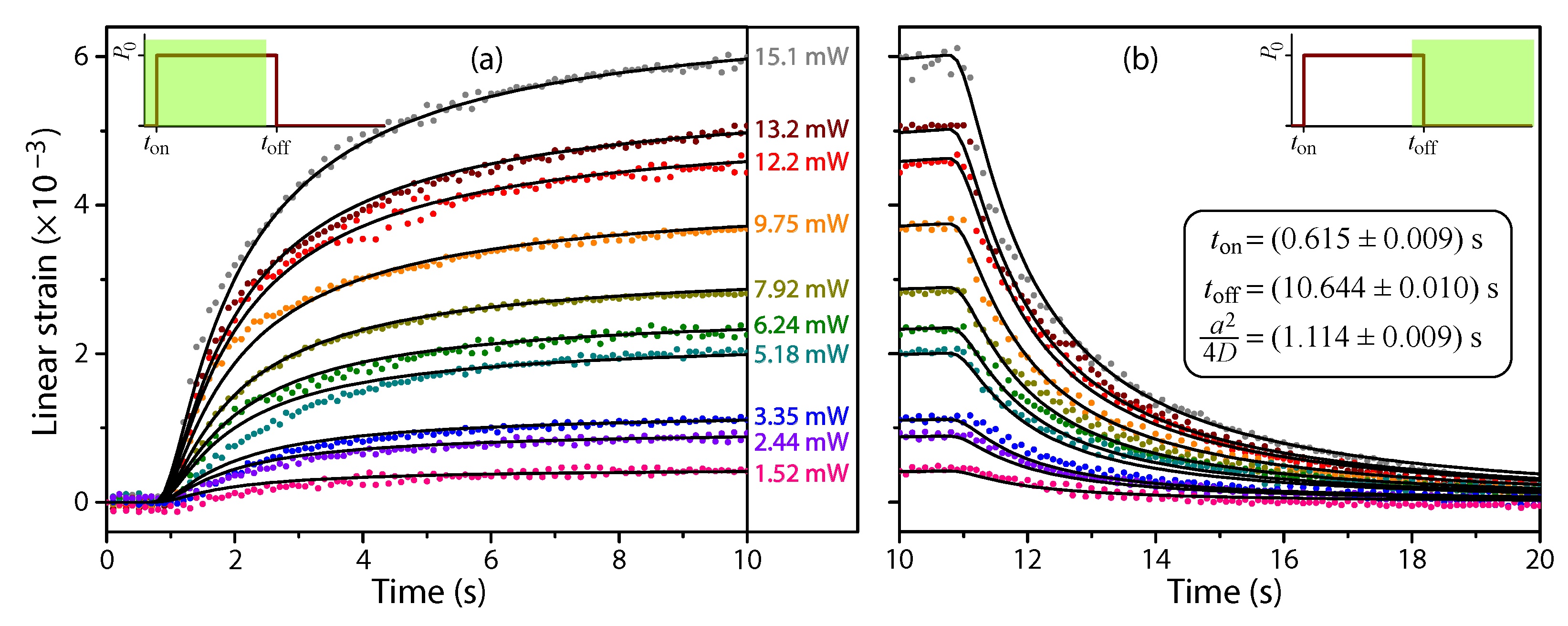
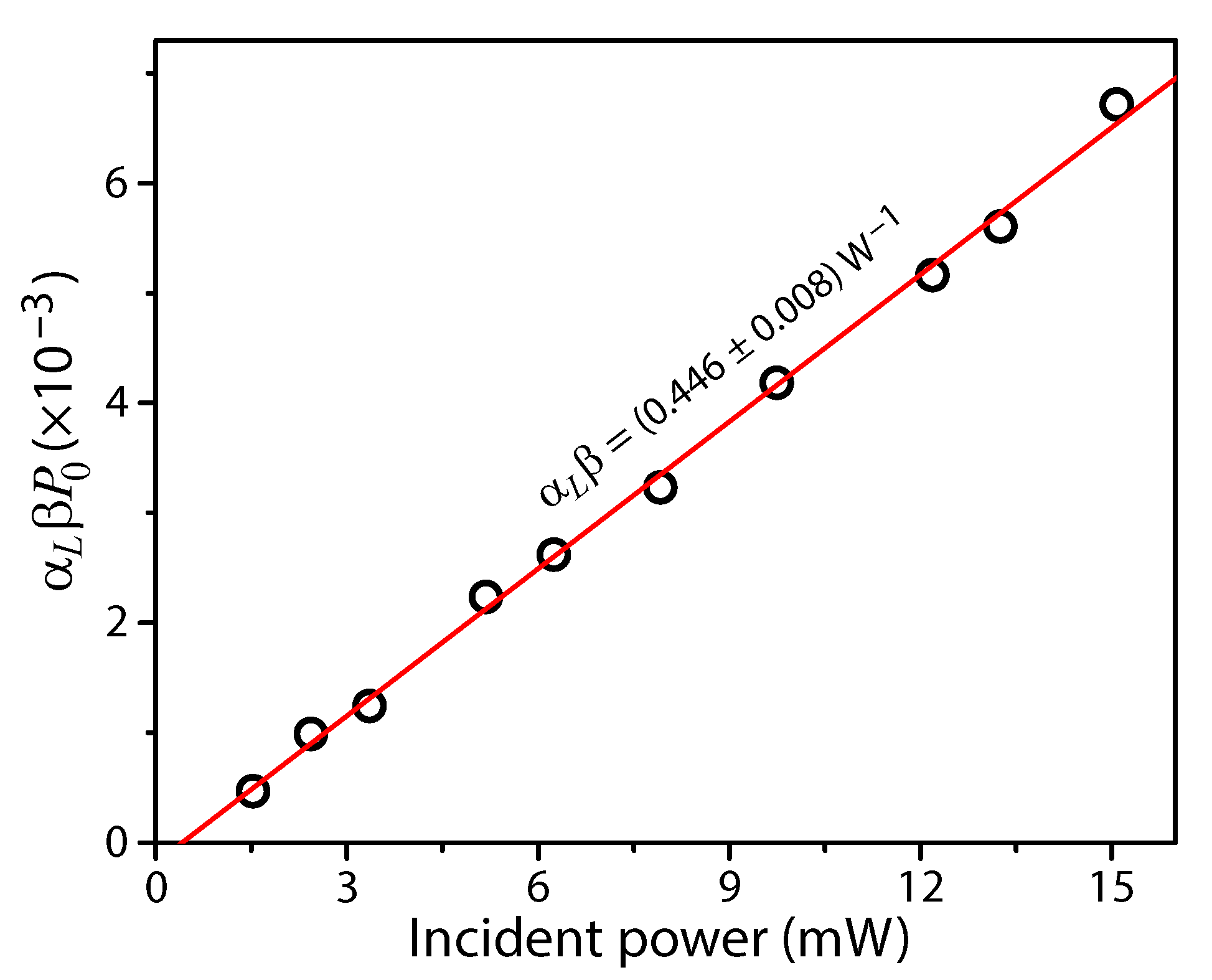
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Sample Availability
Abbreviations
| CCD | Charge coupled device |
| DVD | Digital video disc |
| PDMS | Polydimethylsiloxane |
| CW | Continuous wave |
| AFM | Atomic force microscope |
References
- Putley, E. Chapter 6 The Pyroelectric Detector. In Infrared Detectors; Willardson, R., Beer, A.C., Eds.; Semiconductors and Semimetals; Elsevier: Amsterdam, The Netherlands, 1970; Volume 5, pp. 259–285. [Google Scholar] [CrossRef]
- Lachambre, J.L. A Pyroelectric Energy Meter. Rev. Sci. Instrum. 1971, 42, 74–77. [Google Scholar] [CrossRef]
- Touayar, O.; Sifi, N.; Ktari, T.; Bastié, J. Experimental evaluation of a pyroelectric detector linearity used for pulsed laser energy absolute measurement. Sens. Actuator A Phys. 2005, 120, 482–489. [Google Scholar] [CrossRef]
- Sakurai, K.; Mitsuhashi, Y.; Honda, T. A Laser Microcalorimeter. IEEE Trans. Instrum. Meas. 1967, 16, 212–219. [Google Scholar] [CrossRef]
- Gunn, S.R. Calorimetric measurements of laser energy and power. J. Phys. E Sci. Instr. 1973, 6, 105–114. [Google Scholar] [CrossRef]
- Kodato, S.; Naito, Y.; Kuroda, K.; Kodama, S. A high-accuracy quick-response optical power sensor with μc-Ge:H thin film. Sens. Actuator A Phys. 1991, 28, 63–68. [Google Scholar]
- Kuroda, K.; Kodato, S. Quick response, high accuracy standard optical power meter. In Proceedings of the IECON ’91: 1991 International Conference on Industrial Electronics, Control and Instrumentation, Kobe, Japan, 28 October–1 November 1991; Volume 3, pp. 2433–2438. [Google Scholar]
- Schiel, E.; Bolmarcich, J. Absolute measurement of GaAs diodes radiation using solar cells. Proc. IEEE 1963, 51, 1780–1781. [Google Scholar] [CrossRef]
- Deb, S.; Mukherjee, M. Measurement of radiant power output with a solar cell. IEEE J. Quantum Electron. 1965, 1, 219–220. [Google Scholar] [CrossRef]
- Schinke, D.P.; Smith, R.G.; Hartman, A.R. Photodetectors. In Semiconductor Devices for Optical Communication; Kressel, H., Ed.; Springer: Berlin/Heidelberg, Germany, 1982; pp. 63–87. [Google Scholar] [CrossRef]
- Thorlabs, Inc. Product Specification Sheet: FDS010 Photodiode. 2010. Available online: https://datasheetspdf.com/pdf-file/1310902/Thorlabs/FDS010/1 (accessed on 12 October 2022).
- Thorlabs, Inc. FGA10 InGaAs Photodiode. 1997. Available online: https://datasheetspdf.com/pdf-file/1310910/Thorlabs/FGA10/1 (accessed on 12 October 2022).
- White, T.J. Photomechanical Materials, Composites, and Systems: Wireless Transduction of Light into Work; John Wiley & Sons: Hoboken, NJ, USA, 2017. [Google Scholar]
- Sekkat, Z. Enhancement of molecular mobility in solid polymers by light: Fundamentals and applications. Appl. Phys. B Lasers Opt. 2022, 128, 19. [Google Scholar] [CrossRef]
- Uchino, K.; Aizawa, M. Photostrictive Actuator Using PLZT Ceramics. Jpn. J. Appl. Phys. 1985, 24, 139–141. [Google Scholar] [CrossRef]
- Uchino, K. Photostrictive Actuators Based on Piezoelectrics. In Advanced Piezoelectric Materials—Science and Technology, 2nd ed.; Elsevier: Amsterdam, The Netherlands, 2017; pp. 755–785. [Google Scholar]
- Warner, M.; Terentjev, E.M. Liquid Crystal Elastomers; Oxford University Press: Oxford, UK, 2005. [Google Scholar]
- Saed, M.O.; Torbati, A.H.; Nair, D.P.; Yakacki, C.M. Synthesis of Programmable Main-chain Liquid-crystalline Elastomers Using a Two-stage Thiol-acrylate Reaction. J. Vis. Exp. 2016, 107, e53546. [Google Scholar] [CrossRef]
- Marshall, J.E.; Terentjev, E.M. Photo-sensitivity of dye-doped liquid crystal elastomers. Soft Mater. 2013, 9, 8547–8551. [Google Scholar] [CrossRef]
- Garcia-Amorós, J.; Martínez, M.; Finkelmann, H.; Velasco, D. Photoactuation and thermal isomerisation mechanism of cyanoazobenzene-based liquid crystal elastomers. Phys. Chem. Chem. Phys. 2014, 16, 8448–8454. [Google Scholar] [CrossRef]
- Lahikainen, M.; Kuntze, K.; Zeng, H.; Helantera, S.; Hecht, S.; Priimagi, A. Tunable Photomechanics in Diarylethene-Driven Liquid Crystal Network Actuators. ACS Appl. Mater. Interfaces 2020, 12, 47939–47947. [Google Scholar] [CrossRef] [PubMed]
- Sun, J.; Wang, F.; Zhang, H.; Liu, K. Azobenzene-Based Photomechanical Biomaterials. Adv. NanoBiomed Res. 2021, 1, 2100020. [Google Scholar] [CrossRef]
- Wei, Z.; Bai, R. Temperature-modulated photomechanical actuation of photoactive liquid crystal elastomers. Extrem. Mech. Lett. 2022, 51, 101614. [Google Scholar] [CrossRef]
- Corbett, D.; Warner, M. Linear and nonlinear photoinduced deformations of cantilevers. Phys. Rev. Lett. 2007, 99, 174302. [Google Scholar] [CrossRef]
- Corbett, D.; Warner, M. Changing liquid crystal elastomer ordering with light—A route to opto-mechanically responsive materials. Liq. Cryst. 2009, 36, 1263–1280. [Google Scholar] [CrossRef]
- Ube, T.; Ikeda, T. Cross-Linked Liquid-Crystalline Polymers as Photomobile Materials. In Mechanically Responsive Materials for Soft Robotics; Wiley-VCH Verlag GmbH & Co. KGaA: Weinheim, Germany, 2020; pp. 209–232. [Google Scholar]
- Grabowski, P.; Haberko, J.; Wasylczyk, P. Photo-Mechanical Response Dynamics of Liquid Crystal Elastomer Linear Actuators. Materials 2020, 13, 2933. [Google Scholar] [CrossRef]
- Bai, R.; Bhattacharya, K. Photomechanical coupling in photoactive nematic elastomers. J. Mech. Phys. Solids 2020, 144, 104115. [Google Scholar] [CrossRef]
- Wang, D.H.; Lee, K.M.; Yu, Z.; Koerner, H.; Vaia, R.A.; White, T.J.; Tan, L.S. Photomechanical Response of Glassy Azobenzene Polyimide Networks. Macromolecules 2011, 44, 3840–3846. [Google Scholar] [CrossRef]
- He, L.H. Deformation of amorphous azobenzene-containing polymer films induced by polarized light. Acta Mech. Sin. 2012, 28, 1203–1208. [Google Scholar] [CrossRef]
- Wang, D.H.; Lee, K.M.; Koerner, H.; Yu, Z.; Vaia, R.A.; White, T.J.; Tan, L.S. Flexural-Torsional Photomechanical Responses in Azobenzene-Containing Crosslinked Polyimides. Macromol. Mater. Eng. 2012, 297, 1167–1174. [Google Scholar] [CrossRef]
- Hosono, N.; Yoshikawa, M.; Furukawa, H.; Totani, K.; Yamada, K.; Watanabe, T.; Horie, K. Photoinduced Deformation of Rigid Azobenzene-Containing Polymer Networks. Macromolecules 2013, 46, 1017–1026. [Google Scholar] [CrossRef]
- Wang, D.H.; Wie, J.J.; Lee, K.M.; White, T.J.; Tan, L.S. Impact of Backbone Rigidity on the Photomechanical Response of Glassy, Azobenzene-Functionalized Polyimides. Macromolecules 2014, 47, 659–667. [Google Scholar] [CrossRef]
- Wen, H.; Zhang, W.; Weng, Y.; Hu, Z. Photomechanical bending of linear azobenzene polymer. RSC Adv. 2014, 4, 11776–11781. [Google Scholar] [CrossRef]
- Toccafondi, C.; Occhi, L.; Cavalleri, O.; Penco, A.; Castagna, R.; Bianco, A.; Bertarelli, C.; Comoretto, D.; Canepa, M. Photochromic and photomechanical responses of an amorphous diarylethene-based polymer: A spectroscopic ellipsometry investigation of ultrathin films. J. Mater. Chem. C 2014, 2, 4692–4698. [Google Scholar] [CrossRef]
- Kondo, M. Photomechanical materials driven by photoisomerization or photodimerization. Polym. J. 2020, 52, 1027–1034. [Google Scholar] [CrossRef]
- Loomis, J.; King, B.; Burkhead, T.; Xu, P.; Bessler, N.; Terentjev, E.; Panchapakesan, B. Graphene-nanoplatelet-based photomechanical actuators. Nanotechnology 2012, 23, 045501. [Google Scholar] [CrossRef]
- Ansari, S.; Rahima, C.; Muralidharan, M.N. Photomechanical Characteristics of Thermally Reduced Graphene Oxide-Polydimethylsiloxane Nanocomposites. Polym. Plast. Technol. Eng. 2013, 52, 1604–1610. [Google Scholar] [CrossRef]
- Sánchez-Arévalo, F.M.; Garnica-Palafox, I.M.; Jagdale, P.; Hernández-Cordero, J.; Rodil, S.E.; Okonkwo, A.O.; Hernandez, F.C.R.; Tagliaferro, A. Photomechanical response of composites based on PDMS and carbon soot nanoparticles under IR laser irradiation. Opt. Mater. Express 2015, 5, 1792–1805. [Google Scholar] [CrossRef]
- Hiremath, S.; Kulkarni, S.M. Photomechanical actuation of polydimethylsiloxane/carbon black nanocomposite. Micro Nano Lett. 2020, 15, 437–440. [Google Scholar] [CrossRef]
- López-Lugo, J.D.; Pimentel-Domínguez, R.; Benítez-Martínez, J.A.; Hernández-Cordero, J.; Vélez-Cordero, J.R.; Sánchez-Arévalo, F.M. Photomechanical Polymer Nanocomposites for Drug Delivery Devices. Molecules 2021, 26, 5376. [Google Scholar] [CrossRef]
- Ghorbanishiadeh, Z.; Zhou, B.; Sheibani Karkhaneh, M.; Oehler, R.; Kuzyk, M.G. Photothermal and Reorientational Contributions to the Photomechanical Response of DR1 Azo Dye-Doped PMMA Fibers. Appl. Sci. 2021, 12, 315. [Google Scholar] [CrossRef]
- Dawson, N.J.; Kuzyk, M.G.; Neal, J.; Luchette, P.; Palffy-Muhoray, P. Experimental Studies of the Mechanisms of Photomechanical Effects in a Nematic Liquid Crystal Elastomer. J. Opt. Soc. Am. B 2011, 28, 1916–1921. [Google Scholar] [CrossRef]
- Dawson, N.J.; Kuzyk, M.G.; Neal, J.; Luchette, P.; Palffy-Muhoray, P. Modeling the mechanisms of the photomechanical response of a nematic liquid crystal elastomer. J. Opt. Soc. Am. B 2011, 28, 2134–2141. [Google Scholar] [CrossRef]
- Wu, Z.; Cheng, P.; Zhao, W.; Fang, J.; Xu, T.; Chen, D. Allyl sulfide-based visible light-induced dynamically reshaped liquid crystalline elastomer/SWCNT nanocomposites capable of multimode NIR photomechanical actuations. New J. Chem. 2020, 44, 10902–10910. [Google Scholar] [CrossRef]
- Dannis, M.L. Thermal expansion measurements and transition temperatures, first and second order. J. Appl. Polym. Sci. 1959, 1, 121–126. [Google Scholar] [CrossRef]
- Drebushchak, V.A. Thermal expansion of solids: Review on theories. J. Therm. Anal. Calorim. 2020, 142, 1097–1113. [Google Scholar] [CrossRef]
- Ye, X.; Kuzyk, M.G. Photomechanical response of disperse red 1 azobenzene dye-doped PMMA polymer fiber. Opt. Commun. 2014, 312, 210–215. [Google Scholar] [CrossRef]
- Bhuyan, A. Mechanism of the Photomechanical Response in Composite Polymeric Materials. Ph.D. Thesis, Washington State University, Pullman, WA, USA, 2020. [Google Scholar]
- Hu, J.; Wang, W.; Yu, H. Endowing Soft Photo-Actuators with Intelligence. Adv. Intell. Syst. 2019, 1, 1900050. [Google Scholar] [CrossRef]
- Kuzyk, M.G.; Dawson, N.J. Photomechanical materials and applications: A tutorial. Adv. Opt. Photonics 2020, 12, 847–1011. [Google Scholar] [CrossRef]
- Zhuo, Z.; Lin, J.; Li, J.; Wu, S.; Hu, W.; Gong, J. Research progress of mechanically flexible molecular crystals: From bending mechanisms to applications. Chem. Eng. J. 2022, 450, 138333. [Google Scholar] [CrossRef]
- Welker, D.J.; Kuzyk, M.G. Optical and Mechanical Multistability in a Dye-Doped Polymer Fiber Fabry-Perot Waveguide. Appl. Phys. Lett. 1995, 66, 2792–2794. [Google Scholar] [CrossRef]
- Welker, D.J.; Kuzyk, M.G. All-optical switching in a dye-doped polymer fiber Fabry-Perot waveguide. Appl. Phys. Lett. 1996, 69, 1835–1836. [Google Scholar] [CrossRef]
- Welker, D.J.; Kuzyk, M.G. Suppressing Vibrations in a Sheet with a Fabry-Perot Photomechanical Device. Opt. Lett. 1997, 22, 417–418. [Google Scholar] [CrossRef]
- Kuzyk, M.G.; Garvey, D.W.; Vigil, S.R.; Welker, D.J. All-optical devices in polymer optical fiber. Chem. Phys. 1999, 245, 533–544. [Google Scholar] [CrossRef]
- Bian, S.; Robinson, D.; Kuzyk, M.G. Optically activated cantilever using photomechanical effects in dye-doped polymer fibers. J. Opt. Soc. Am. B 2006, 23, 697–708. [Google Scholar] [CrossRef]
- Wang, E.; Desai, M.S.; Lee, S.W. Light-Controlled Graphene-Elastin Composite Hydrogel Actuators. Nano Lett. 2013, 13, 2826–2830. [Google Scholar] [CrossRef]
- Zhao, J.; Xu, P.; Yu, Y.; Li, K. Controllable vibration of liquid crystal elastomer beams under periodic illumination. Int. J. Mech. Sci. 2020, 170, 105366. [Google Scholar] [CrossRef]
- Liu, H.; Niu, D.; Jiang, W.; Zhao, T.; Lei, B.; Yin, L.; Shi, Y.; Chen, B.; Lu, B. Illumination-oriented and thickness-dependent photomechanical bilayer actuators realized by graphene-nanoplatelets. Sensor. Actuat. A Phys. 2016, 239, 45–53. [Google Scholar] [CrossRef]
- Niu, D.; Jiang, W.; Liu, H.; Zhao, T.; Lei, B.; Li, Y.; Yin, L.; Shi, Y.; Chen, B.; Lu, B. Reversible Bending Behaviors of Photomechanical Soft Actuators Based on Graphene Nanocomposites. Sci. Rep. 2016, 6, 27366. [Google Scholar] [CrossRef]
- Zhou, Y.; Hauser, A.W.; Bende, N.P.; Kuzyk, M.G.; Hayward, R.C. Waveguiding Microactuators Based on a Photothermally Responsive Nanocomposite Hydrogel. Adv. Funct. Mater. 2016, 26, 5447–5452. [Google Scholar] [CrossRef]
- Leeladhar; Raturi, P.; Kumar, A.; Singh, J.P. Graphene-polydimethylsiloxane/chromium bilayer-based flexible, reversible, and large bendable photomechanical actuators. Smart Mater. Struct. 2017, 26, 095030. [Google Scholar] [CrossRef]
- Leeladhar; Singh, J.P. Human skin-inspired multiresponsive actuators based on graphene oxide/polydimethylsiloxane bilayer film: Bi-directional transformation of semi-tube into plane sheet/tube under different stimuli. Smart Mater. Struct. 2020, 29, 75022. [Google Scholar] [CrossRef]
- Wang, J.; Huang, S.; Zhang, Y.; Liu, J.; Yu, M.; Yu, H. Hydrogen Bond Enhances Photomechanical Swing of Liquid-Crystalline Polymer Bilayer Films. ACS Appl. Mater. Interfaces 2021, 13, 6585–6596. [Google Scholar] [CrossRef]
- Satapathy, P.; Adiga, R.; Kumar, M.; Hegde, G.; Prasad, S.K. Porous nanocarbon particles drive large magnitude and fast photomechanical actuators. J. Nanostruct. Chem. 2022, 12, 235–248. [Google Scholar] [CrossRef]
- Kim, T.; Zhu, L.; Al-Kaysi, R.O.; Bardeen, C.J. Organic Photomechanical Materials. ChemPhysChem 2014, 15, 400–414. [Google Scholar] [CrossRef]
- Zhou, B.; Yan, D. Recent advances of dynamic molecular crystals with light-triggered macro-movements. Appl. Phys. Rev. 2021, 8, 041310. [Google Scholar] [CrossRef]
- Camacho-Lopez, M.; Finkelmann, H.; Palffy-Muhoray, P.; Shelley, M. Fast liquid-crystal elastomer swims into the dark. Nat. Mater. 2004, 3, 307–310. [Google Scholar] [CrossRef]
- Ma, S.; Li, X.; Huang, S.; Hu, J.; Yu, H. A Light-Activated Polymer Composite Enables On-Demand Photocontrolled Motion: Transportation at the Liquid/Air Interface. Angew. Chem. (Int. Ed.) 2019, 58, 2655–2659. [Google Scholar] [CrossRef]
- Song, T.; Lei, H.; Clancy, A.J.; Ma, S.; Yu, H.; Zhang, L. Supramolecular hydrogen bond enables Kapton nanofibers to reinforce liquid-crystalline polymers for light-fueled flight. Nano Energy 2021, 87, 106207. [Google Scholar] [CrossRef]
- Uchino, K. Ceramic Actuators: Principles and Applications. MRS Bull. April 1993, 29, 42. [Google Scholar] [CrossRef]
- Mu, J.; Hou, C.; Wang, H.; Li, Y.; Zhang, Q.; Zhu, M. Origami-inspired active graphene-based paper for programmable instant self-folding walking devices. Sci. Adv. 2015, 1, e1500533. [Google Scholar] [CrossRef] [PubMed]
- Yu, L.; Yu, H. Light-powered tumbler movement of graphene oxide/polymer nanocomposites. ACS Appl. Mater. Interfaces 2015, 7, 3834–3839. [Google Scholar] [CrossRef]
- Gelebart, A.H.; Jan Mulder, D.; Varga, M.; Konya, A.; Vantomme, G.; Meijer, E.W.; Selinger, R.L.B.; Broer, D.J. Making waves in a photoactive polymer film. Nature 2017, 546, 632–636. [Google Scholar] [CrossRef] [PubMed]
- Norikane, Y.; Saito, K. Crawling Motion of Crystals on Solid Surfaces by Photo-induced Reversible Crystal-to-Melt Phase Transition. In Mechanically Responsive Materials for Soft Robotics; Wiley-VCH Verlag GmbH & Co. KGaA: Weinheim, Germany, 2020; pp. 83–103. [Google Scholar]
- Dradrach, K.; Rogóż, M.; Grabowski, P.; Xuan, C.; Wȩgłowski, R.; Konieczkowska, J.; Schab-Balcerzak, E.; Piecek, W.; Wasylczyk, P. Traveling Wave Rotary Micromotor Based on a Photomechanical Response in Liquid Crystal Polymer Networks. ACS Appl. Mater. Interfaces 2020, 12, 8681–8686. [Google Scholar] [CrossRef] [PubMed]
- Uǧur, G.; Chang, J.; Xiang, S.; Lin, L.; Lu, J. A Near-Infrared Mechano Responsive Polymer System. Adv. Mater. 2012, 24, 2685–2690. [Google Scholar] [CrossRef] [PubMed]
- Wie, J.J.; Wang, D.H.; Tondiglia, V.P.; Tabiryan, N.V.; Vergara-Toloza, R.O.; Tan, L.S.; White, T.J. Photopiezoelectric Composites of Azobenzene-Functionalized Polyimides and Polyvinylidene Fluoride. Macromol. Rapid Commun. 2014, 35, 2050–2056. [Google Scholar] [CrossRef] [PubMed]
- Tang, R.; Liu, Z.; Xu, D.; Liu, J.; Yu, L.; Yu, H. Optical Pendulum Generator Based on Photomechanical Liquid-Crystalline Actuators. ACS Appl. Mater. Interfaces 2015, 7, 8393–8397. [Google Scholar] [CrossRef] [PubMed]
- Wei, W.; Gao, J.; Yang, J.; Wei, J.; Guo, J. A NIR light-triggered pyroelectric-dominated generator based on a liquid crystal elastomer composite actuator for photoelectric conversion and self-powered sensing. RSC Adv. 2018, 8, 40856–40865. [Google Scholar] [CrossRef]
- Xiong, Y.; Zhang, L.; Weis, P.; Naumov, P.; Wu, S. A solar actuator based on hydrogen-bonded azopolymers for electricity generation. J. Mater. Chem. A 2018, 6, 3361–3366. [Google Scholar] [CrossRef]
- Zhao, D.; Liu, Y. Photomechanical vibration energy harvesting based on liquid crystal elastomer cantilever. Smart Mater. Struct. 2019, 28, 075017. [Google Scholar] [CrossRef]
- Mejia, J.C.; Aizawa, M.; Hisano, K.; Matsumoto, K.; Hashimoto, S.; Kubo, S.; Shishido, A. Surface Topographical Control of a Liquid Crystal Microlens Array Embedded in a Polymer Network. Appl. Sci. 2022, 12, 7901. [Google Scholar] [CrossRef]
- Wani, O.M.; Zeng, H.; Priimagi, A. A light-driven artificial flytrap. Nat. Commun. 2017, 8, 15546. [Google Scholar] [CrossRef] [PubMed]
- Hu, J.; Li, X.; Ni, Y.; Ma, S.; Yu, H. A programmable and biomimetic photo-actuator: A composite of a photo-liquefiable azobenzene derivative and commercial plastic film. J. Mater. Chem. C 2018, 6, 10815–10821. [Google Scholar] [CrossRef]
- Li, X.; Ma, S.; Hu, J.; Ni, Y.; Lin, Z.; Yu, H. Photo-activated bimorph composites of Kapton and liquid-crystalline polymer towards biomimetic circadian rhythms of Albizia julibrissin leaves. J. Mater. Chem. C 2019, 7, 622–629. [Google Scholar] [CrossRef]
- Luo, P.; Xiang, S.; Li, C.; Zhu, M. Photomechanical polymer hydrogels based on molecular photoswitches. J. Polym. Sci. 2021, 59, 2246–2264. [Google Scholar] [CrossRef]
- Zhu, C.; Qin, L.; Lu, Y.; Sun, J.; Yu, Y. Photomechanical Liquid Crystal Polymers and Bioinspired Soft Actuators. In Mechanically Responsive Materials for Soft Robotics; John Wiley & Sons, Ltd.: Hoboken, NJ, USA, 2020; Chapter 9; pp. 233–256. [Google Scholar] [CrossRef]
- Bell, A.G. On the Production and Reproduction of Sound by Light. Proc. Am. Assoc. Adv. Sci. 1881, 29, 115–136. [Google Scholar] [CrossRef]
- Dawson, N.J.; Kuzyk, M.G.; Neal, J.; Luchette, P.; Palffy-Muhoray, P. Cascading of liquid crystal elastomer photomechanical optical devices. Opt. Commun. 2011, 284, 991–993. [Google Scholar] [CrossRef]
- Dawson, N.J.; Kuzyk, M.G.; Neal, J.; Luchette, P.; Palffy-Muhoray, P. Integration of liquid crystal elastomer photomechanical optical devices. In Proceedings of the Liquid Crystals XVI, San Diego, CA, USA, 12–16 August 2012; Volume 8475, pp. 41–48. [Google Scholar] [CrossRef]
- Kuenstler, A.S.; Kim, H.; Hayward, R.C. Liquid Crystal Elastomer Waveguide Actuators. Adv. Mater. 2019, 31, 1901216. [Google Scholar] [CrossRef]
- Zhao, D.; Liu, Y. Programmable liquid crystal elastomer matrix through photomechanical responses. J. Appl. Polym. Sci. 2020, 137, 48997. [Google Scholar] [CrossRef]
- Niu, D.; Jiang, W.; Li, D.; Ye, G.; Luo, F.; Liu, H. Reconfigurable shape-morphing flexible surfaces realized by individually addressable photoactuator arrays. Smart Mater. Struct. 2021, 30, 125032. [Google Scholar] [CrossRef]
- Fang, L.; Zhang, H.; Li, Z.; Zhang, Y.; Zhang, Y.; Zhang, H. Synthesis of Reactive Azobenzene Main-Chain Liquid Crystalline Polymers via Michael Addition Polymerization and Photomechanical Effects of Their Supramolecular Hydrogen-Bonded Fibers. Macromolecules 2013, 46, 7650–7660. [Google Scholar] [CrossRef]
- Wie, J.J.; Wang, D.H.; Lee, K.M.; White, T.J.; Tan, L.S. The contribution of hydrogen bonding to the photomechanical response of azobenzene-functionalized polyamides. J. Mater. Chem. C 2018, 6, 5964–5974. [Google Scholar] [CrossRef]
- Min Lee, K.; Lynch, B.M.; Luchette, P.; White, T.J. Photomechanical effects in liquid crystal polymer networks prepared with m-fluoroazobenzene. J. Polym. Sci. Part A Polym. Chem. 2014, 52, 876–882. [Google Scholar] [CrossRef]
- Kim, J.H.; Jang, K.L.; Ahn, K.; Yoon, T.; Lee, T.I.; Kim, T.S. Thermal expansion behavior of thin films expanding freely on water surface. Sci. Rep. 2019, 9, 7071. [Google Scholar] [CrossRef] [PubMed]
- Zou, C.; Lin, L.Y. Grating-patterned Perovskite Light Emitting Diodes for Enhanced Performance. In Proceedings of the CLEO. Optica Publishing Group, San Jose, CA, USA, 5–10 May 2019; p. JTu2A.113. [Google Scholar] [CrossRef]
- Li, G.; Qin, J.; Wang, Y.; Wu, Y.; Li, M.; Chen, J. Microscale gratings patterned in perovskite films to promote the electron-optical conversion efficiency of perovskite light-emitting diodes. Appl. Opt. 2022, 61, 35–40. [Google Scholar] [CrossRef] [PubMed]
- Toshiba. Data Sheet: TCD1501C. 1997. Available online: https://pdf1.alldatasheet.com/datasheet-pdf/view/32203/TOSHIBA/TCD1501C.html (accessed on 3 October 2022).
- The Dow Chemical Company. Technical Data Sheet: SYLGARD 184 Silicone Elastomer. 2017. Available online: https://pdf1.alldatasheet.com/datasheet-pdf/view/1425043/ETC/SYLGARD-184.html (accessed on 3 October 2022).
- Anderson, B.R.; Hung, S.T.; Kuzyk, M.G. Imaging studies of temperature dependent photodegradation and self-healing in disperse orange 11 dye-doped polymers. J. Chem. Phys. 2016, 145, 024901. [Google Scholar] [CrossRef] [PubMed]
- Stubbs, N.; Bridgewater, M.; Stubbs, M.; Kabir, A.; Crescimanno, M.; Kuzyk, M.G.; Dawson, N.J. Polylactic acid promotes healing of photodegraded disperse orange 11 molecules. Opt. Mater. 2018, 76, 11–15. [Google Scholar] [CrossRef]
- Christianson, N.D.; Lu, Y.; Dawson, N.J. Recovery of photodegraded rhodamine 6g in ester-containing polymer matrices. Photochem. Photobiol. Sci. 2019, 18, 2865–2874. [Google Scholar] [CrossRef]
- Anderson, B.R.; Kuzyk, M.G. Imaging studies of photodegradation and self-healing in anthraquinone derivative dye-doped PMMA. Phys. Chem. Chem. Phys. 2020, 22, 28154–28164. [Google Scholar] [CrossRef] [PubMed]
- Lu, Y.; Lowther, Z.; Christianson, N.D.; Li, Z.; Baer, E.; Kuzyk, M.G.; Dawson, N.J. Demonstration of a self-healing all-polymer distributed Bragg reflector laser. Appl. Phys. Lett. 2020, 116, 103301. [Google Scholar] [CrossRef]
- Park, C.S.; Joo, K.I.; Kang, S.W.; Kim, H.R. A PDMS-Coated Optical Fiber Bragg Grating Sensor for Enhancing Temperature Sensitivity. J. Opt. Soc. Korea 2011, 15, 329–334. [Google Scholar] [CrossRef]
- Schift, H.; Heyderman, L.J.; Maur, M.A.d.; Gobrecht, J. Pattern formation in hot embossing of thin polymer films. Nanotechnology 2001, 12, 173–177. [Google Scholar] [CrossRef]
- Ponelyte, S.; Prosycevas, I.; Guobiene, A.; Balciunas, R.; Puiso, J. Formation of MEMS nanocomposit layers and investigation of their mechanical properties. Mechanika 2009, 2, 77–82. [Google Scholar]
- Song, Z.; Qian, M.; Zhang, H.; Wang, T.; Ding, G.; Liu, J. The fabrication and molecular alignment of poly(ethylene oxide) grating film based on hot embossing technology. New J. Chem. 2019, 43, 16604–16611. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sun, F.; Shasho, R.T.; Crescimanno, M.; Dawson, N.J. All-Optical Method of Determining Laser Power from the Photomechanical Effect. Appl. Sci. 2022, 12, 10708. https://doi.org/10.3390/app122110708
Sun F, Shasho RT, Crescimanno M, Dawson NJ. All-Optical Method of Determining Laser Power from the Photomechanical Effect. Applied Sciences. 2022; 12(21):10708. https://doi.org/10.3390/app122110708
Chicago/Turabian StyleSun, Frank, Rocco T. Shasho, Michael Crescimanno, and Nathan J. Dawson. 2022. "All-Optical Method of Determining Laser Power from the Photomechanical Effect" Applied Sciences 12, no. 21: 10708. https://doi.org/10.3390/app122110708
APA StyleSun, F., Shasho, R. T., Crescimanno, M., & Dawson, N. J. (2022). All-Optical Method of Determining Laser Power from the Photomechanical Effect. Applied Sciences, 12(21), 10708. https://doi.org/10.3390/app122110708







