Optimizing Transportation between Sea Ports and Regions by Road Transport and Rail and Inland Waterway Transport Means Including “Last Mile” Solutions
Abstract
1. Introduction
- -
- How much does competition between transport modes that include increasing or decreasing energy demands and costs influence the selection of optimal transport chains?
- -
- What are the advantages of improving transportation processes during cargo delivery?
2. Analysis of Opportunities and Literature Sources for Optimization of Freight Transport and Energy Saving in Transport Routes
3. Theoretical Basis for the Energy Saving by Cargo Transportation Modes and Its Combinations, Methods
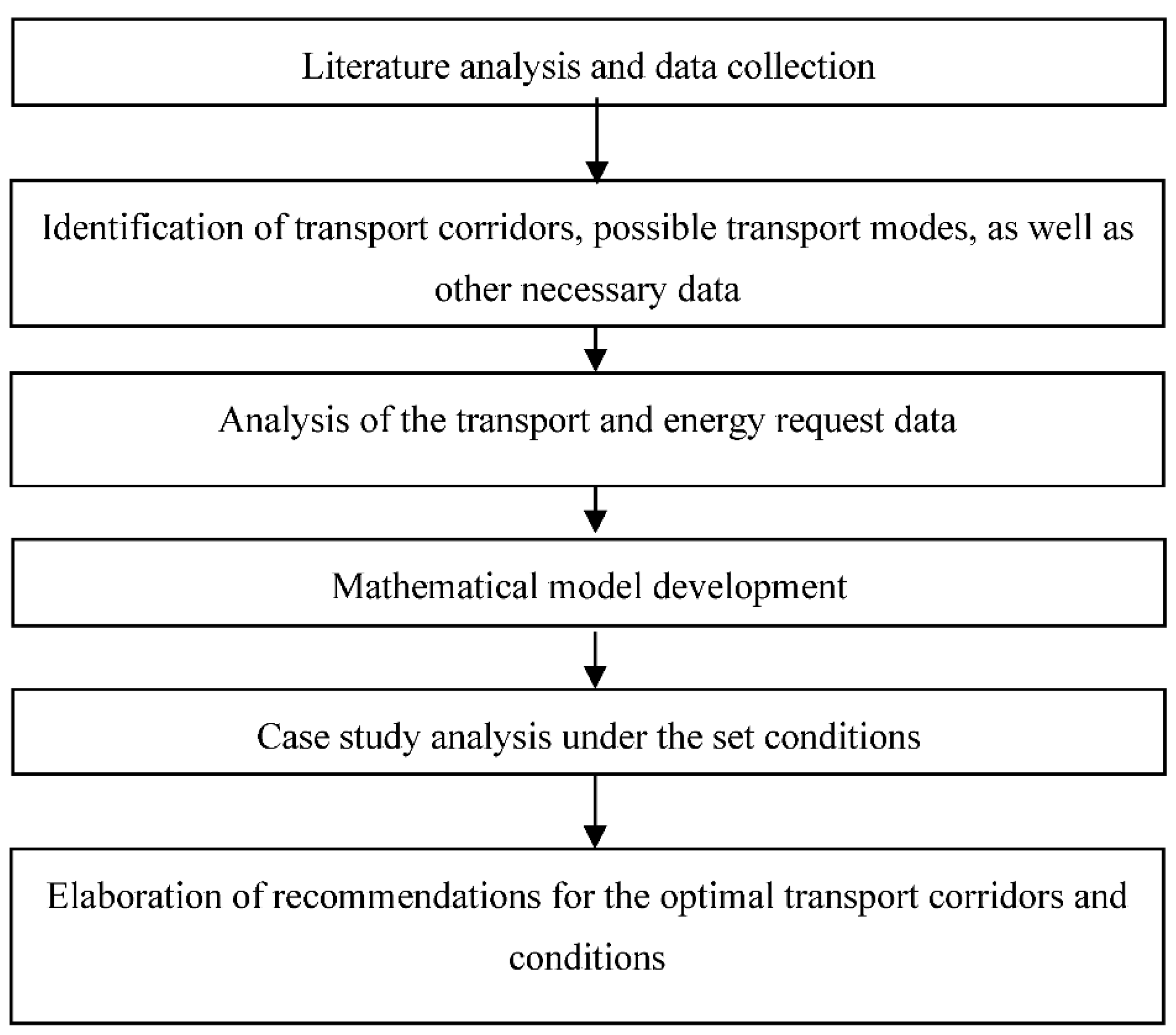
3.1. Research Methodology
- Collection and analysis of the data mentioned above.
- Planning possible distances between regions, ports, and main reloading points (intermodal terminals).
- Calculation of the cargo reloading time and costs in cargo reloading points, based on collected data.
- Calculation of particular transportation parameters, such as time costs and fuel consumption.
- Calculations of transport modes energy comparative index for the transport corridors.
- Drawing the conclusions and recommendations for the specific conditions.
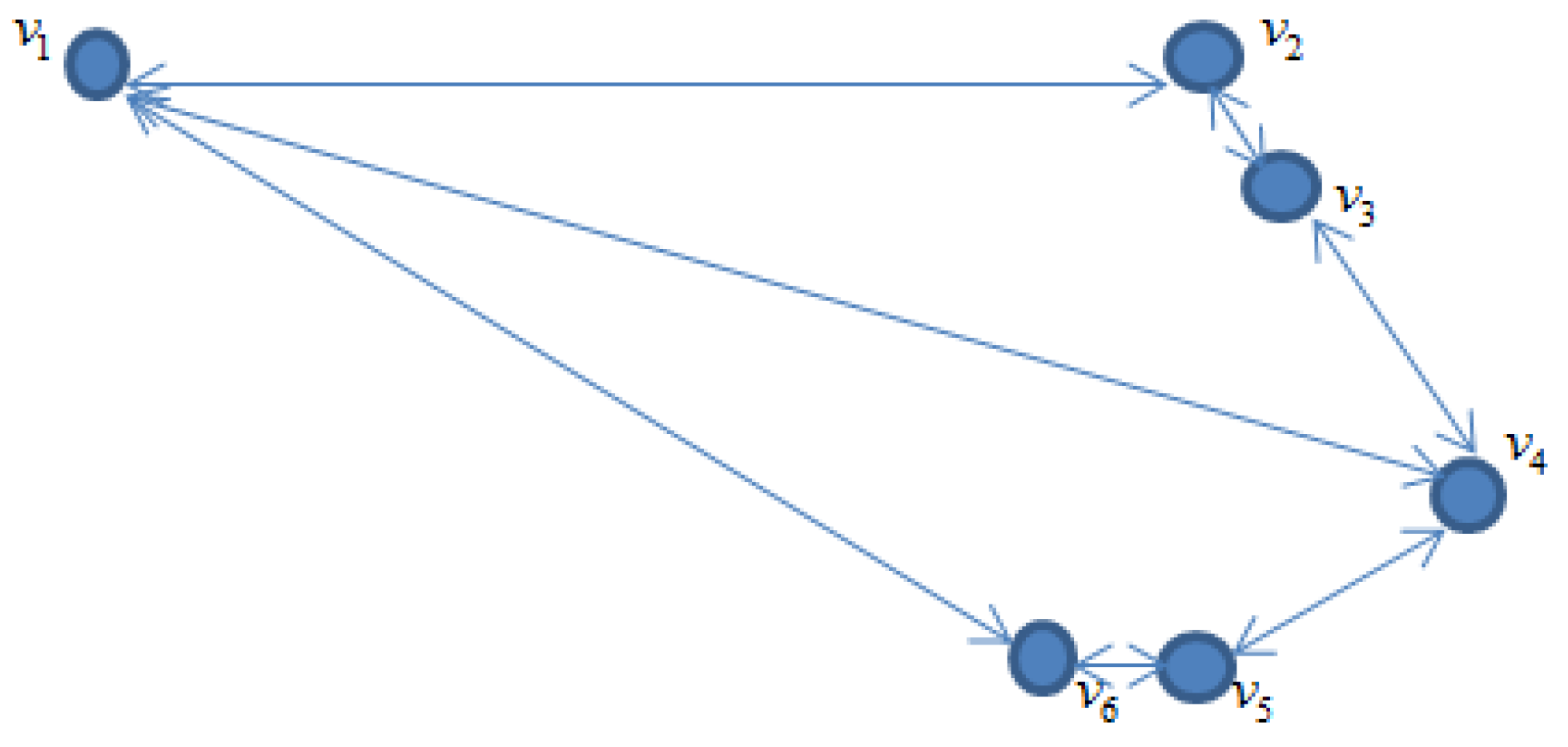
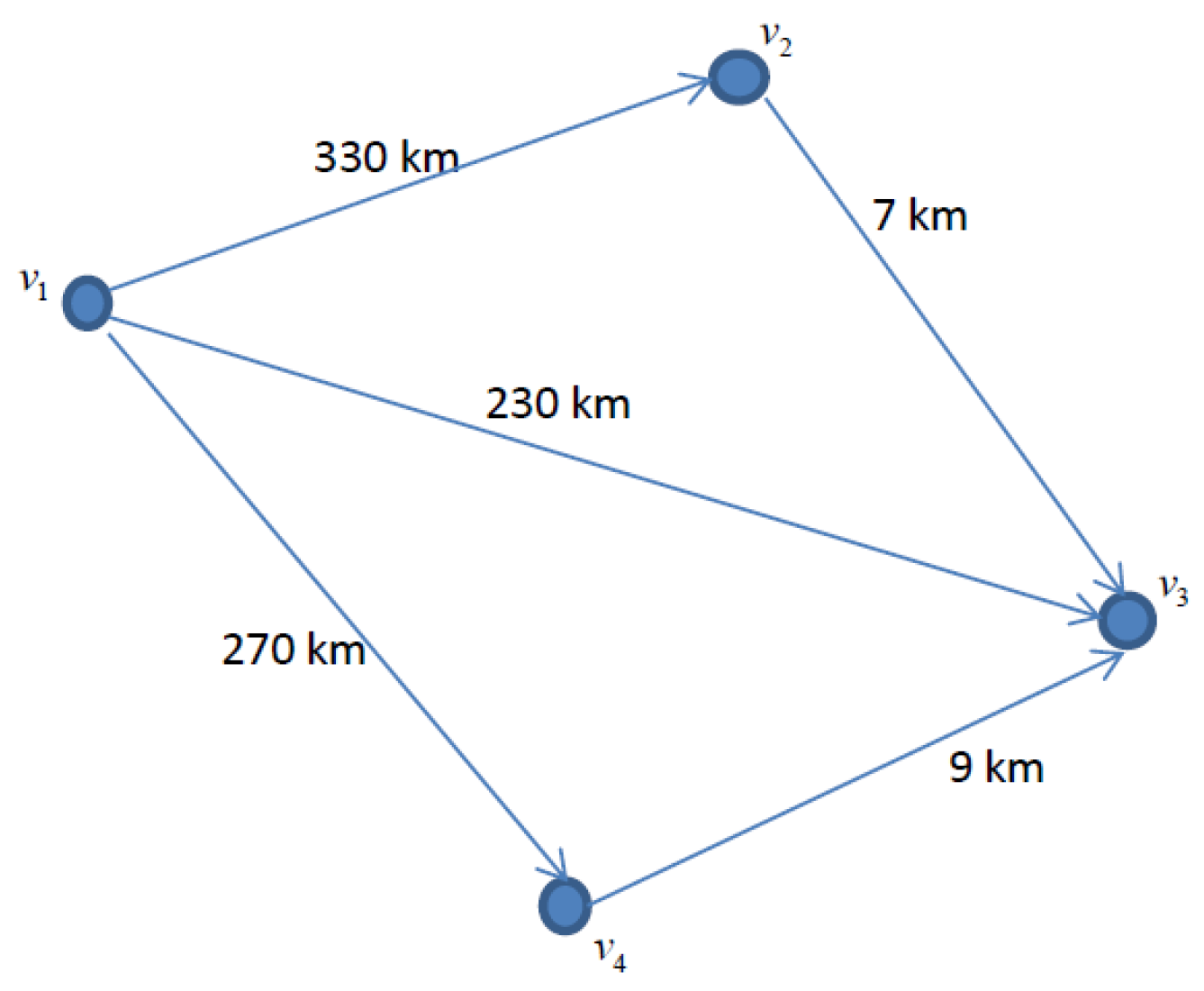
3.2. Mathematical Model
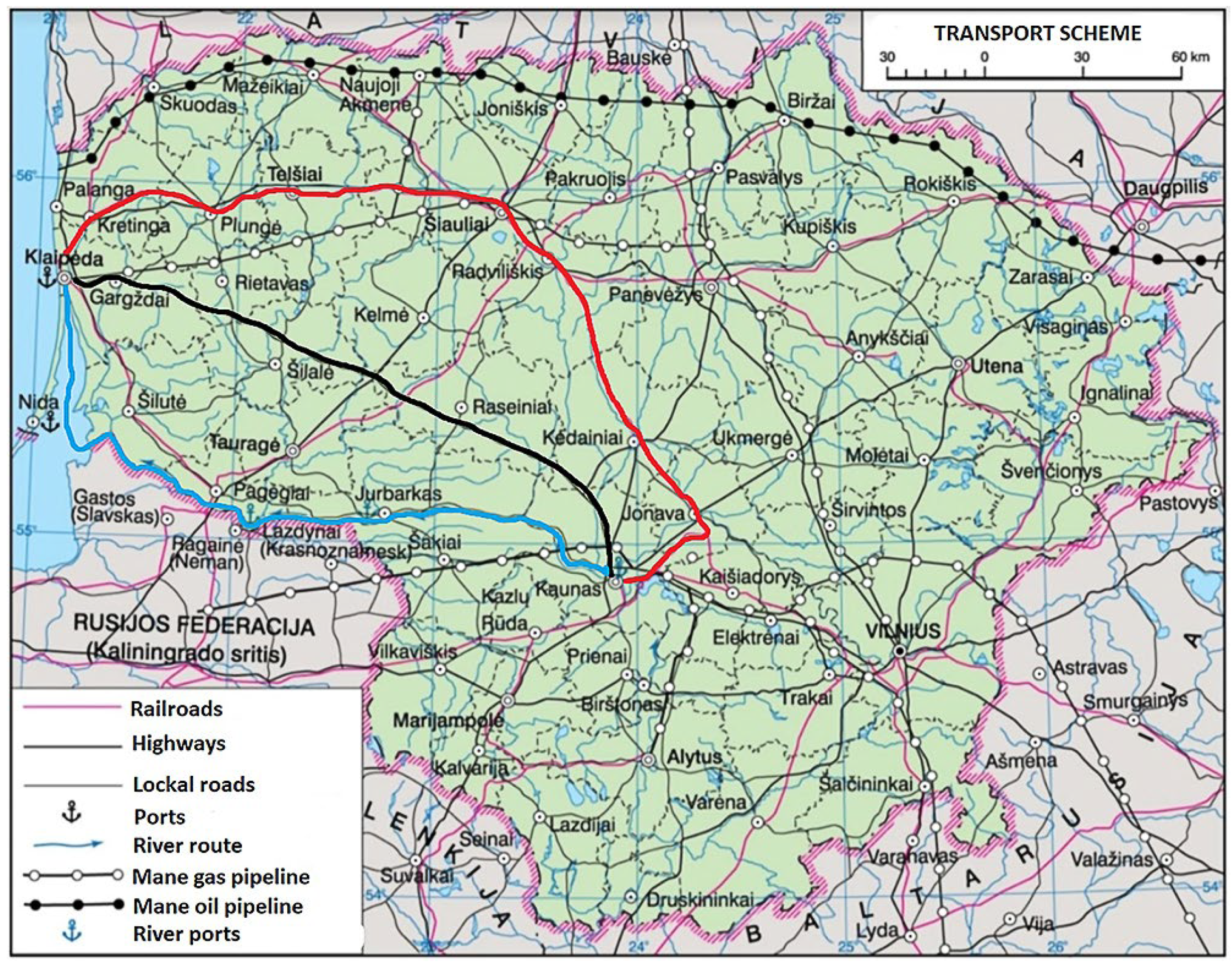
4. Case Study on the Different Transport Modes between Ports and Consignee Location Evaluation (Lithuania Case)
5. Discussions
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ritchie, H.; Roser, M.; Rosado, P. Energy. 2020. Available online: https://ourworldindata.org/energy (accessed on 15 May 2022).
- U.S. Department of Energy Washington. Global Transportation Energy Consumption: Examination of Scenarios to 2040 Using ITEDD; U.S. Department of Energy Washington: Washington, DC, USA, 2017; 38p.
- Kwon, M.; Lee, J.; Hong, Y.S. Product-service system business modelling methodology using morphological analysis. Sustainability 2020, 11, 1376. [Google Scholar] [CrossRef]
- Szeto, W.Y.; Lo, H.K. Transportation Network Improvement and Tolling Strategies: The Issue of Intergeneration Equity. Transp. Res. Part A Policy Pract. 2006, 40, 227–243. [Google Scholar] [CrossRef]
- Jarašūnienė, A.; Čižiūnienė, K.; Petraška, A. Research on rail and maritime transport interoperability in the area of information systems: The case of Lithuania. Transport 2019, 34, 467–475. [Google Scholar] [CrossRef]
- Zhou, W.; You, X.; Fan, W. A mixed integer linear programming method for simultaneous multi-periodic train timetabling and routing on a high-speed rail network. Sustainability 2020, 12, 1131. [Google Scholar] [CrossRef]
- Saakian, I.; Savchuk, V. Railway transportation: Problems and solutions. Probl. Econ. Transit. 2013, 56, 73–95. [Google Scholar] [CrossRef]
- Mańkowska, M. The competitiveness of cross-border transportation networks: A case study of the Szczecin–Berlin inland waterway. Sci. J. Zesz. Nauk. Marit. Univ. Szczec. 2019, 58, 93–104. [Google Scholar] [CrossRef]
- Valkhof, H.H.; Hoogeveen, T.; Dallinga, R.P.; Toxopeus, L.S. A Tug and Barge System for Sea and River Service. SNAME Annu. Meet. 2000, 24. Available online: https://www.researchgate.net/publication/257817071_A_Tug_and_barge_system_for_sea_and_river_service (accessed on 15 May 2022).
- Müller, J.; Straub, M.; Richter, G.; Rudloff, C. Integration of Different Mobility Behaviors and Intermodal Trips in MATSim. Sustainability 2022, 14, 428. [Google Scholar] [CrossRef]
- Bouman, E.A.; Lindstad, E.; Rialland, A.I.; Strømman, A.H. State-of-the-art technologies, measures, and potential for reducing GHG emissions from shipping–A review. Transp. Res. Part D Transp. Environ. 2017, 52, 408–421. [Google Scholar] [CrossRef]
- Ali, N.; Nakayama, S.; Yamaguchi, H. Analysis of Bus Fare Structure to Observe Modal Shift, Operator Profit, and Land-Use Choices through Combined Unified Transport Model. Sustainability 2022, 14, 139. [Google Scholar] [CrossRef]
- Smaoui, H.; Zouhri, L.; Kaidi, S. Identification of Hydrodynamic Dispersion Tensor by Optimization Algorithm Based LBM/CMA-ES Combination. Water 2022, 14, 125. [Google Scholar] [CrossRef]
- Di Vaio, A.; Varriale, L. Management innovation for environmental sustainability in seaports: Managerial accounting instruments and training for competitive green ports beyond the regulations. Sustainability 2018, 10, 783. [Google Scholar] [CrossRef]
- Hale, J.; Long, S.A. Time Series Sustainability Assessment of a Partial Energy Portfolio Transition. Energies 2021, 14, 141. [Google Scholar] [CrossRef]
- Paulauskas, V.; Filina-Dawidowicz, L.; Paulauskas, D. The Method to Decrease Emissions from Ships in Port Areas. Sustainability 2020, 12, 4374. [Google Scholar] [CrossRef]
- Colette, A.; Granier, C.Ø.; Hodnebrog, Ø.H.; Jakobs, H.; Maurizi, A.; Nyiri, A.; Bessagnet, B.; D’Angiola, A.; D’Isidoro, M.; Gauss, M. Air quality trends in Europe over the past decade: A first multi-model assessment. Atmos. Chem. Phys. 2011, 11, 11657–11678. [Google Scholar] [CrossRef]
- Semenov, I.N.; Filina-Dawidowicz, L. Topology-based Approach to the Modernization of Transport and Logistics Systems with Hybrid Architecture. Part 1 Proof—Concept Study. Arch. Transp. 2019, 43, 105–124. [Google Scholar]
- Ignatavicius, G.; Toleikiene, M. Optimization of the conservation of rare and vulnerable plant species in the perspective of climate change in Lithuanian (nature) reserves. Arch. Environ. Prot. 2017, 43, 61–73. [Google Scholar] [CrossRef][Green Version]
- Paulauskas, V. Ships Entering the Ports; N.I.M.S Publish House: Riga, Latvia, 2013; 240p, ISBN 9984-679-71-3. [Google Scholar]
- Omarov, M.; Ismail, S.; Rani, W.N.M.W.M.; Durdyev, S. Estimation of Traffic Delay Due to U-Turns at Uncontrolled Medians: Case Study in Phnom Penh, Cambodia. Sustainability 2022, 14, 118. [Google Scholar] [CrossRef]
- Penmetsa, P.; Ghosh, I.; Chandra, S. Evaluation of performance measures for two-lane intercity highways under mixed traffic conditions. J. Transp. Eng. 2015, 141, 04015021. [Google Scholar] [CrossRef]
- Romanovaa, A.; Vygnanova, A.; Vygnanovaa, M.; Sokolovaa, E.; Eiduks, J. Problems of the formation of a single transport space on sections of international transport corridors. ICTE Transp. Logistics. 2019, 149, 537–541. [Google Scholar] [CrossRef]
- Belen, M.; Emilio Ortega, E.; Cuevas-Wizner, R.; Ledda, A.; Andrea De Montis, A. Assessing road network resilience: An accessibility comparative analysis. Transp. Res. Part D 2021, 95, 102851. [Google Scholar] [CrossRef]
- Li, Y.; Chen, Y. Driver Vision Based Perception-Response Time Prediction and Assistance Model on Mountain Highway Curve. Int. J. Environ. Res. Public Health 2017, 14, 31. [Google Scholar] [CrossRef]
- Hu, Q.; Gu, W.; Wang, S. Optimal subsidy scheme design for promoting intermodal freight transport. Transp. Res. Part E 2021, 157, 102561. [Google Scholar] [CrossRef]
- Lithuania Transport Map. 2022. Available online: https://www.vle.lt/straipsnis/lietuvos-transportas/ (accessed on 15 May 2022).
- Jezierski, W.; Sadowska, B.; Pawłowski, K. Impact of Changes in the Required Thermal Insulation of Building Envelope on Energy Demand, Heating Costs, Emissions, and Temperature in Buildings. Energies 2021, 14, 56. [Google Scholar] [CrossRef]
- Šakalys, R.; Batarlienė, N. Research on intermodal terminal interaction in international transport corridors. Procedia Eng. 2017, 187, 281–288. [Google Scholar] [CrossRef]
- European Inland Waterways Map. Available online: https://unece.org/DAM/trans/main/sc3/AGN_map_2018.pdf (accessed on 15 May 2022).
- Ypsilantis, P.; Zuidwijk, R. Collaborative fleet deployment and routing for sustainable transport. Sustainablitiy 2019, 11, 5666. [Google Scholar] [CrossRef]
- Kotug Forms Inland Shipping Division Based on Electric Pusher Tugs. 2021. Available online: https://www.offshore-energy.biz/kotug-forms-inland-shipping-division-based-on-electric-pusher-tugs/ (accessed on 15 May 2022).
- Łebkowski, A. Reduction of fuel consumption and pollution emissions in inland water transport by application of hybrid powertrain. Energies 2018, 11, 1981. [Google Scholar] [CrossRef]
- Hendrickx, C.; Breemersch, T. The Effect of Climate Change on Inland Waterway Transport. Procedia—Soc. Behav. Sci. 2012, 48, 1837–1847. [Google Scholar] [CrossRef]
- Translate File: Freight Transport in the EU-28 Modal Split of Inland Transport Modes (% of Total Tonne-Kilometres). 2017. Available online: https://ec.europa.eu/eurostat/statistics-explained/index.php?title=File:Freight_transport_in_the_EU-28_modal_split_of_inland_transport_modes_(%25_of_total_tonne-kilometres).png&oldid=336869 (accessed on 15 May 2022).
- Wiegmans, B.; Konings, R. Intermodal Inland Waterway Transport: Modelling Conditions Influencing Its Cost Competitiveness. Asian J. Shipp. Logist. 2015, 31, 273–294. [Google Scholar] [CrossRef]
- Kurtulus, E.; Cetin, I.B. Assessing the environmental benefits of dry port usage: A case of inland container transport in Turkey. Sustainability 2019, 11, 6793. [Google Scholar] [CrossRef]
- Inland Waterways Freight Transport by Type of Vessel. 2018. Available online: https://ec.europa.eu/eurostat/statistics-explained (accessed on 10 March 2022).
- Mako, P.; Dávid, A.; Böhm, P.; Savu, S. Sustainable transport in the Danube region. Sustainability 2021, 13, 6797. [Google Scholar] [CrossRef]
- Morales-Fusco, P.; Grau, M.; Saurí, S. Effects of RoPax shipping line strategies on freight price and transporter‘s choice. Policy implications for promoting MoS. Transp. Policy 2018, 67, 67–76. [Google Scholar] [CrossRef]
- Matuliauskas, K. Grafų Teorija; Vilnius University Publish House: Vilnius, Lithuania, 2010; 21p. [Google Scholar]
- Andreae, T. On independent cycles and edges in graphs. Discrete Math. 1996, 149, 291–297. [Google Scholar] [CrossRef][Green Version]
- Bondy, J.A.; Chvatal, V. A method in graph theory. Discret. Math. 1976, 15, 111–135. [Google Scholar] [CrossRef]
- Curtis, A.R.; Izurieta, C.; Joeris, B.; Lundberg, S.; McConnell, R.M. An Implicit Representation of Chordal Comparability Graphs in Linear Time. The 32nd International Conference on Graph-2006. Theoretic Concepts in Computer Science, LNCS. 2010, Volume 4271, pp. 168–178. Available online: https://www.sciencedirect.com/science/article/pii/S0166218X1000017X (accessed on 15 May 2022). [CrossRef]
- Fujita, S. Recent results on disjoint cycles in graphs. Electron. Notes Discret. Math. 2005, 22, 409–412. [Google Scholar] [CrossRef]
- Sadeghian, P.; Hakansson, J.; Zhao, X. Review and evaluation of methods in transport mode detection based on GPS tracking data. Traffic Transp. Eng. 2021, 167–182. [Google Scholar] [CrossRef]
- Mouschoutzi, M.; Ponis, S.T. A comprehensive literature review on spare parts logistics management in the maritime industry. Shipp. Logist. 2022, 38, 71–83. [Google Scholar] [CrossRef]
- Gao, S.; Liu, N. Improving the Resilience of Port–Hinterland Container Logistics Transportation Systems: A Bi-Level Programming Approach. Sustainability 2022, 14, 180. [Google Scholar] [CrossRef]
- Martins, V.; Correia, C.; Cunha-Lopes, I.; Faria, T.; Diapouli, E.; Manousakas, M.I.; Eleftheriadis, K.; Almeida, S.M. Chemical characterisation of particulate matter in urban transport modes. Environ. Sci. 2021, 100, 51–61. [Google Scholar] [CrossRef]
- Eyring, V.; Köhler, H.W.; Lauer, A.; Lemper, B. Emissions from international shipping. Impact of future technologies on scenarios until 2050. J. Geophys. Res. 2005, 110, D17306. [Google Scholar] [CrossRef]
- Heinrich, L.; Koschinsky, A.; Markus, T.; Singh, P. Quantifying the fuel consumption, greenhouse gas emissions and air pollution of a potential commercial manganese nodule mining operation. Marit. Policy 2020, 114, 103678. [Google Scholar] [CrossRef]
- Czermański, E.; Pawłowska, B.; Oniszczuk-Jastrząbek, A.; Cirella, G.T. Decarbonization of maritime transport: Analysis of external costs. Front. Energy Res. 2020, 8, 28. [Google Scholar] [CrossRef]
- Behdani, B.; Fan, Y.; Wiegmans, B.; Zuidwijk, R. Multimodal Schedule Design for Synchromodal Freight Transport Systems. Eur. J. Transp. Infrastruct. Res. 2016, 16, 424–444. [Google Scholar] [CrossRef]
- Iheonu, N.O.; Inyama, S.C. On the Optimization of Transportation Problem. Br. J. Math. Comput. Sci. 2016, 13, 1–11. [Google Scholar] [CrossRef]
- Castaneda, J.; Ghorbani, E.; Ammouriova, M.; Panadero, J.; Juan, A.A. Optimizing Transport Logistics under Uncertainty with Simheuristics: Concepts, Review and Trends. Logistics 2022, 6, 42. [Google Scholar] [CrossRef]
- Congli, H.; Yixiang, Y. Optimization on Combination of Transport Routes and Modes on Dynamic Programming for a Container Multimodal Transport System. Procedia Eng. 2016, 137, 382–390. [Google Scholar] [CrossRef]

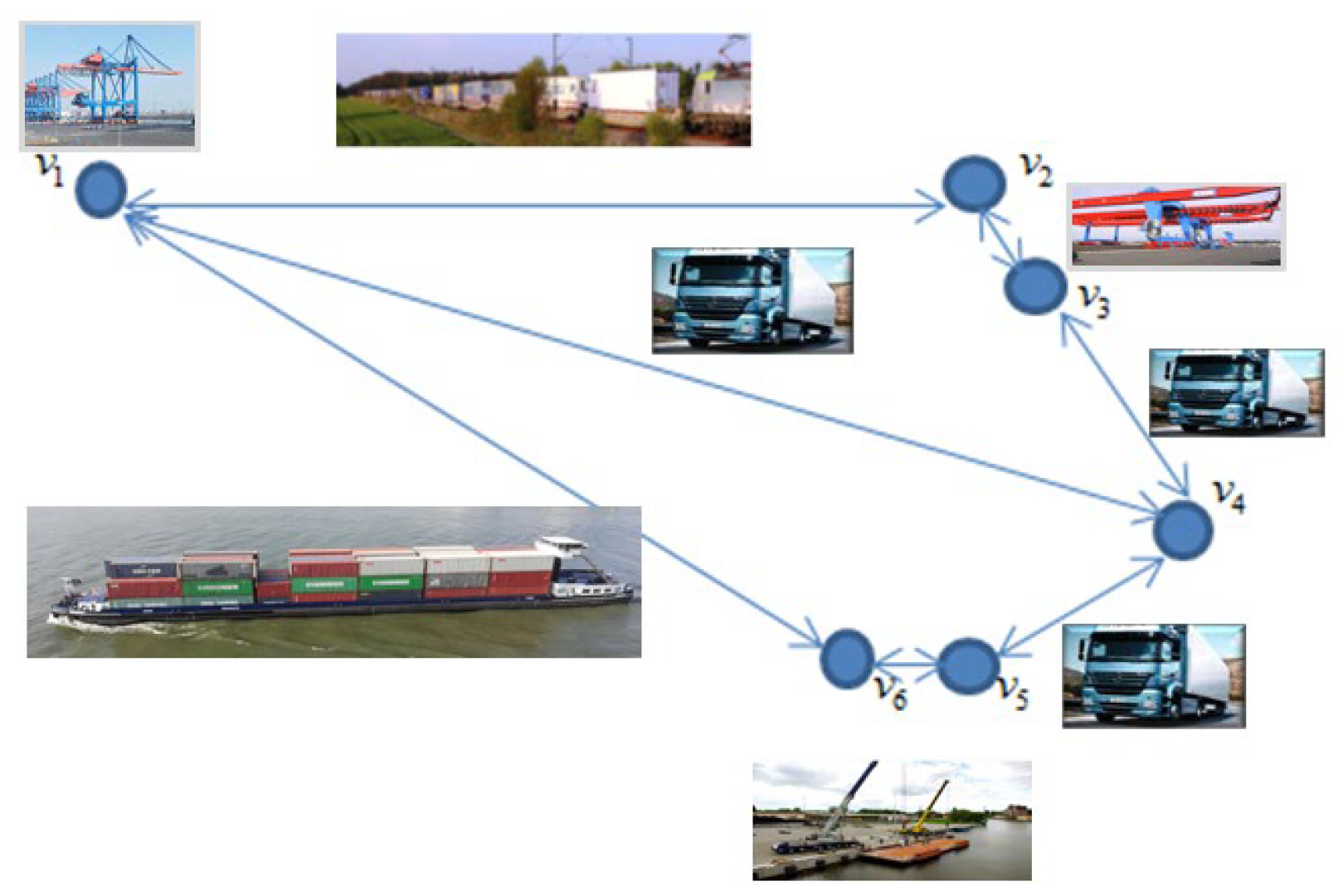
| Trends | Consequences |
|---|---|
| Increasing competition between transport modes | More efficient transport systems |
| Globalization | More investments in modern transport systems |
| More effective transport systems | Faster logistics |
| Increasing energy costs | Energy savings |
| Increasing environmental demands | New fuels, removable energy, automation |
| Transport Means | Transportation Costs (Factors) | Transportation Time (Factors) | Transportation Energy Demand (Factors) | Environmental Factors | Complex Factors | Remarks |
|---|---|---|---|---|---|---|
| Road | [4,7,12,21,22,23,24,25,29,31,40] | [4,7,21,22,23,24,25,28,29,31,34,40] | [13] | [17,18] | [3] | The factors of road transport are well researched, but there are limited comprehensive studies. |
| Rail | [5,6,29,40] | [5,25,40] | [13] | [17,18] | [3] | The railway transport part of the factor is investigated, but in most cases, it does not include complex investigations. |
| Inland water ways | [8,31,36,37,39,40] | [8,20,31,37,39,40] | [9,39] | [11,14,16,17,19,34] | Inland waterway transport lacks in-depth comprehensive research. | |
| Intermodal | [26,29] | [26,29] | [28] | [18] | Research on intermodal transport mainly covers road and rail and maritime transport, but excludes inland waterway transport. | |
| Logistics | [10,18] | [18] | [19] | Logistics research lacks the assessment of complex transportation factors that include all modes of transport. | ||
| Complex transport | [18] | [18] | [3] | [18,19] | [3] | Limited studies have been carried out on the comprehensive assessment methods of all factors |
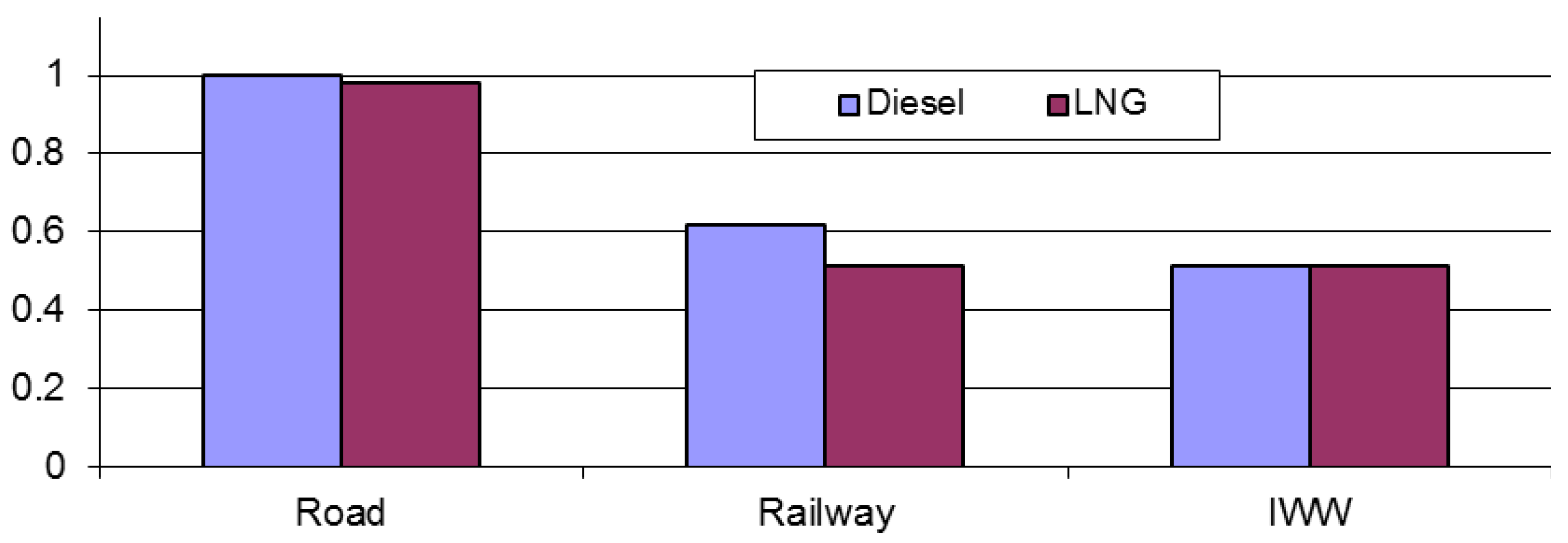
| Transport Mode | Number of Real Data (Cases) | Dispersion | ||
|---|---|---|---|---|
| Road | 53 | 1.0 | 0.034 | 0.18 |
| Railway | 22 | 0.65 | 0.021 | 0.14 |
| Inland waterway | 7 | 0.53 | 0.012 | 0.11 |
| Factors | Existing Methods | Developed Method in This Paper | Future Research Prospects |
|---|---|---|---|
| Transportation time, costs and energy demand factors | Exist methods evaluate costs and time transportation; problematic to evaluate energy demand | It is possible evaluate optimal costs, transportation time, energy demand and additional factors | It is important make research works that more accurate receive factors weight coefficients |
| Environmental factors | It is possible to evaluate environmental impact factors and existing limitations and provide complex evaluation together with costs, time and energy demand evaluation | Method could be adopted evaluate economics and environmental factors | In future need research works more accurate evaluate economics and environmental factors in case of non-standard transportation processes |
| Complex evaluation of the main factors | Problematic, because in case of many factors not clear systems | Possible, but need clear evaluate weight of the factors | Finding weight of the factors could be main research tasks in future adopted to concrete conditions |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Paulauskas, V.; Henesey, L.; Plačiene, B.; Jonkus, M.; Paulauskas, D.; Barzdžiukas, R.; Kaulitzky, A.; Simutis, M. Optimizing Transportation between Sea Ports and Regions by Road Transport and Rail and Inland Waterway Transport Means Including “Last Mile” Solutions. Appl. Sci. 2022, 12, 10652. https://doi.org/10.3390/app122010652
Paulauskas V, Henesey L, Plačiene B, Jonkus M, Paulauskas D, Barzdžiukas R, Kaulitzky A, Simutis M. Optimizing Transportation between Sea Ports and Regions by Road Transport and Rail and Inland Waterway Transport Means Including “Last Mile” Solutions. Applied Sciences. 2022; 12(20):10652. https://doi.org/10.3390/app122010652
Chicago/Turabian StylePaulauskas, Vytautas, Lawrence Henesey, Birute Plačiene, Martynas Jonkus, Donatas Paulauskas, Raimondas Barzdžiukas, Artur Kaulitzky, and Martynas Simutis. 2022. "Optimizing Transportation between Sea Ports and Regions by Road Transport and Rail and Inland Waterway Transport Means Including “Last Mile” Solutions" Applied Sciences 12, no. 20: 10652. https://doi.org/10.3390/app122010652
APA StylePaulauskas, V., Henesey, L., Plačiene, B., Jonkus, M., Paulauskas, D., Barzdžiukas, R., Kaulitzky, A., & Simutis, M. (2022). Optimizing Transportation between Sea Ports and Regions by Road Transport and Rail and Inland Waterway Transport Means Including “Last Mile” Solutions. Applied Sciences, 12(20), 10652. https://doi.org/10.3390/app122010652









