Linear and Nonlinear Reduced Order Models for Sloshing for Aeroelastic Stability and Response Predictions
Abstract
:1. Introduction
2. Flexible Aircraft with Sloshing Integrated Modeling
3. Case Studies
4. Aeroelastic Stability and Response Analyses
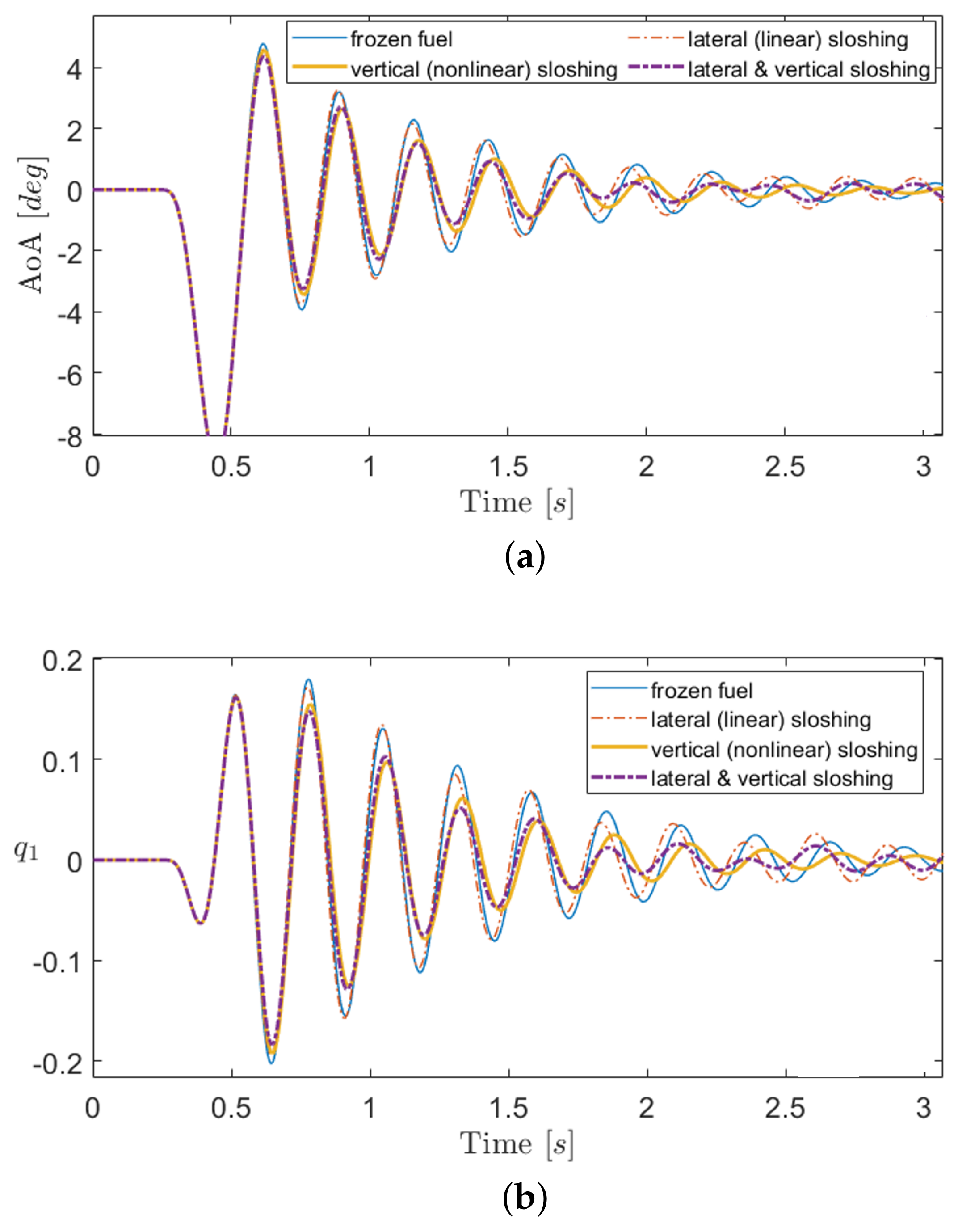
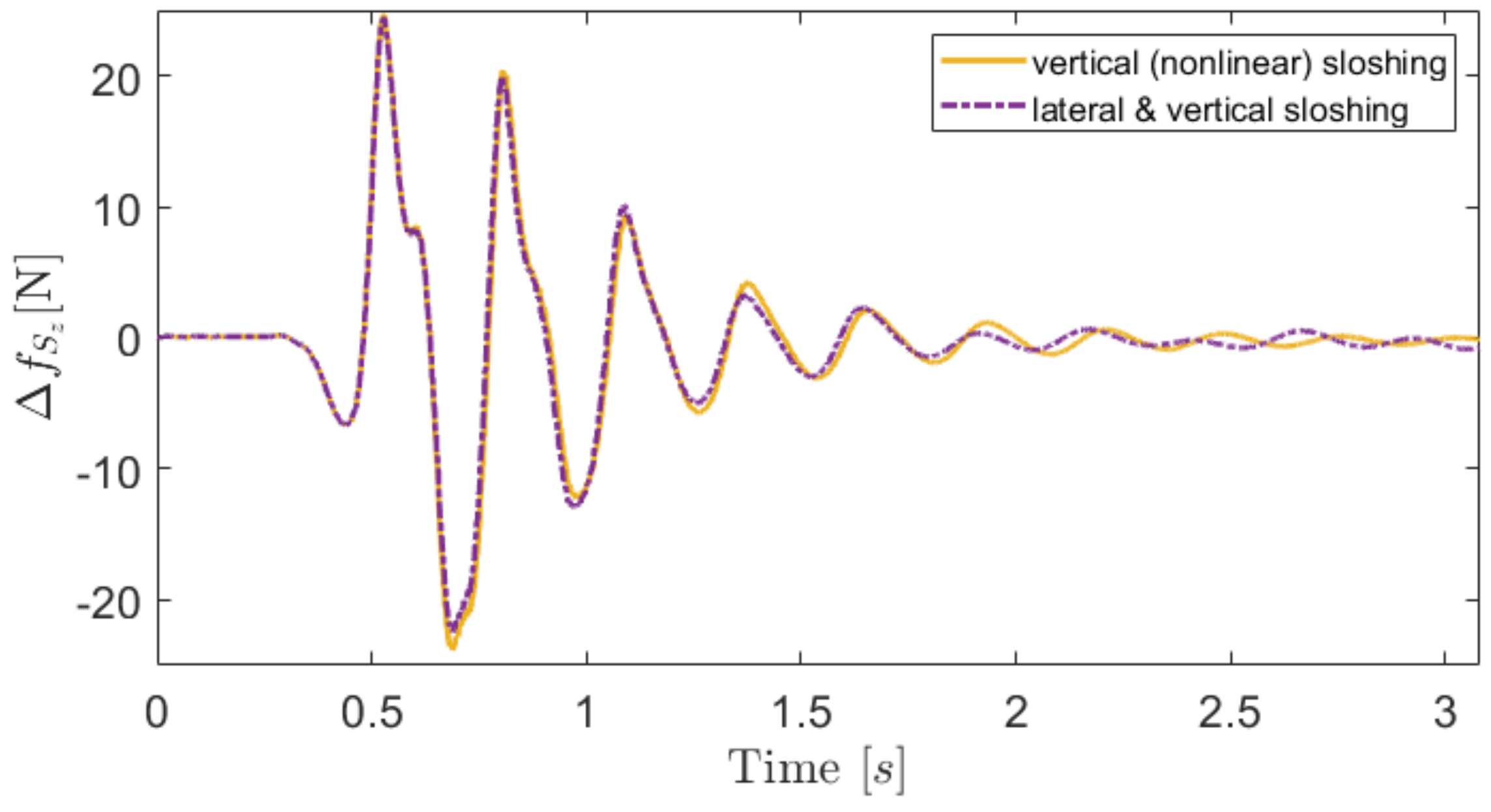
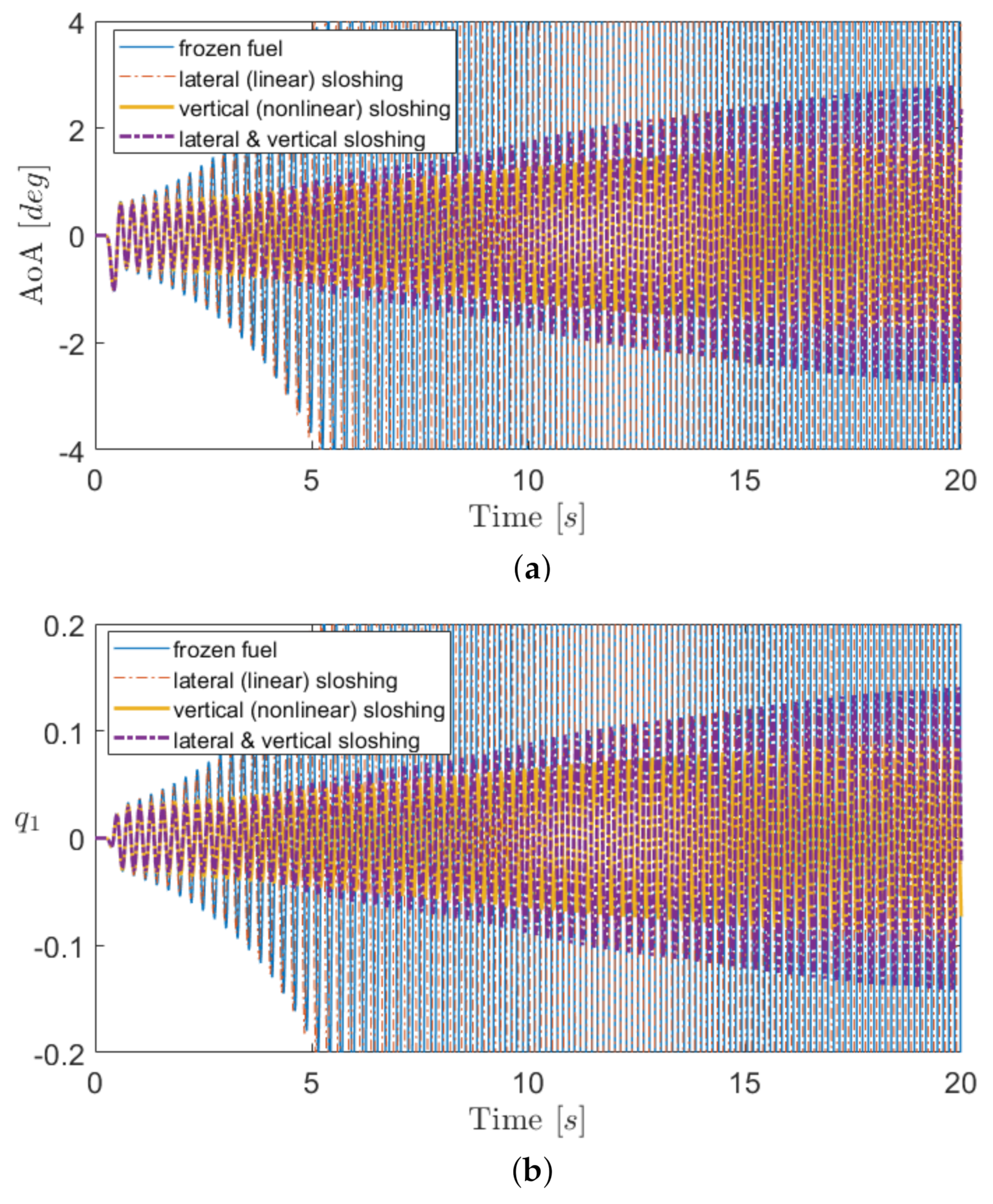
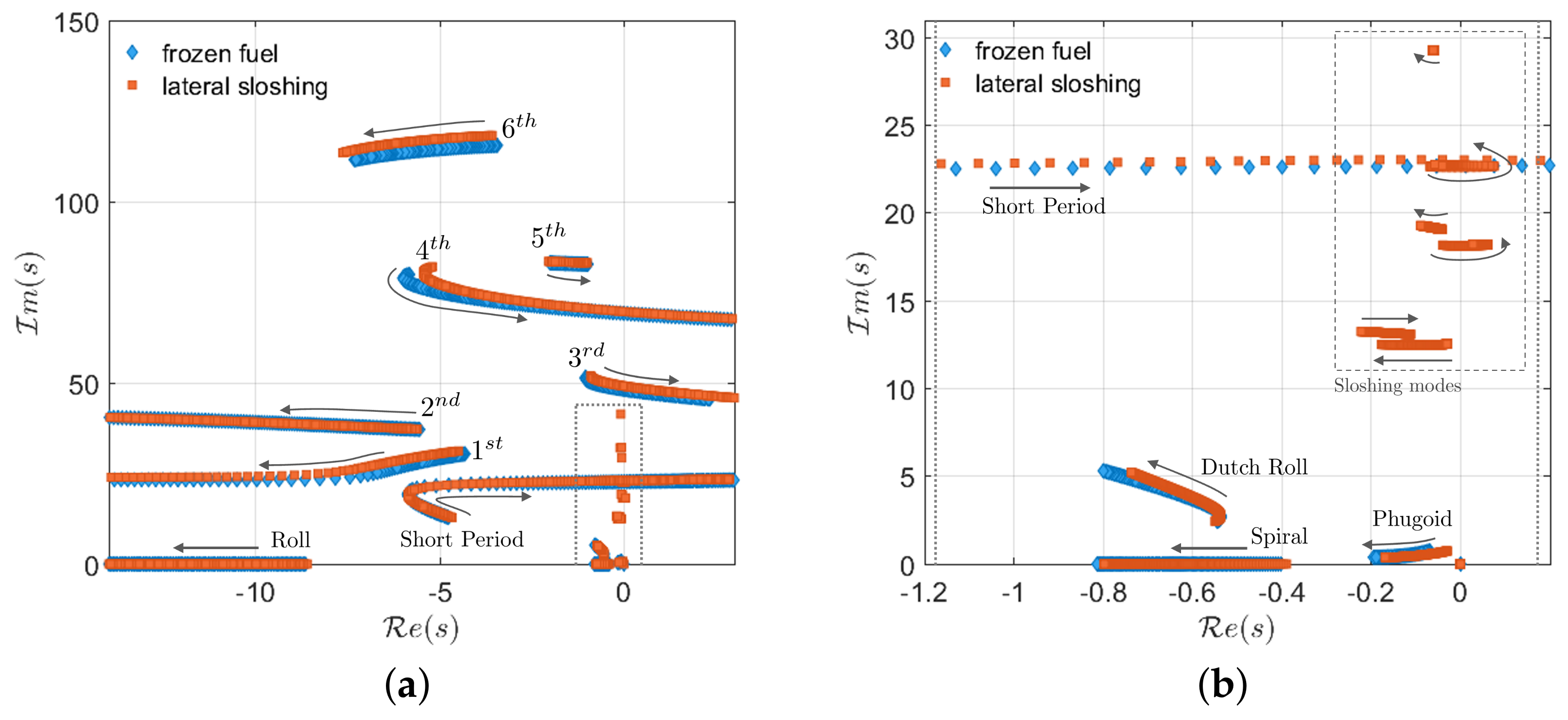
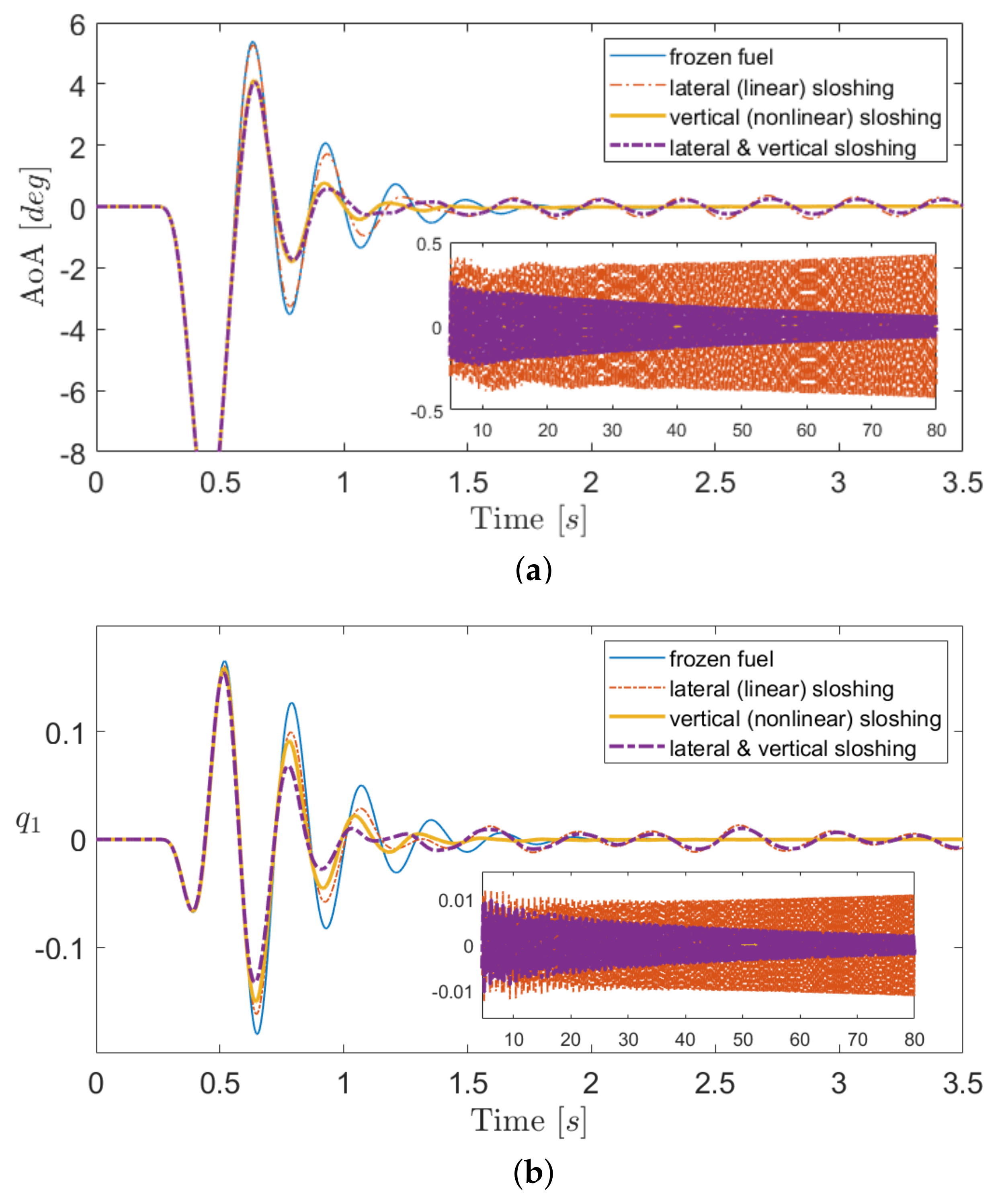
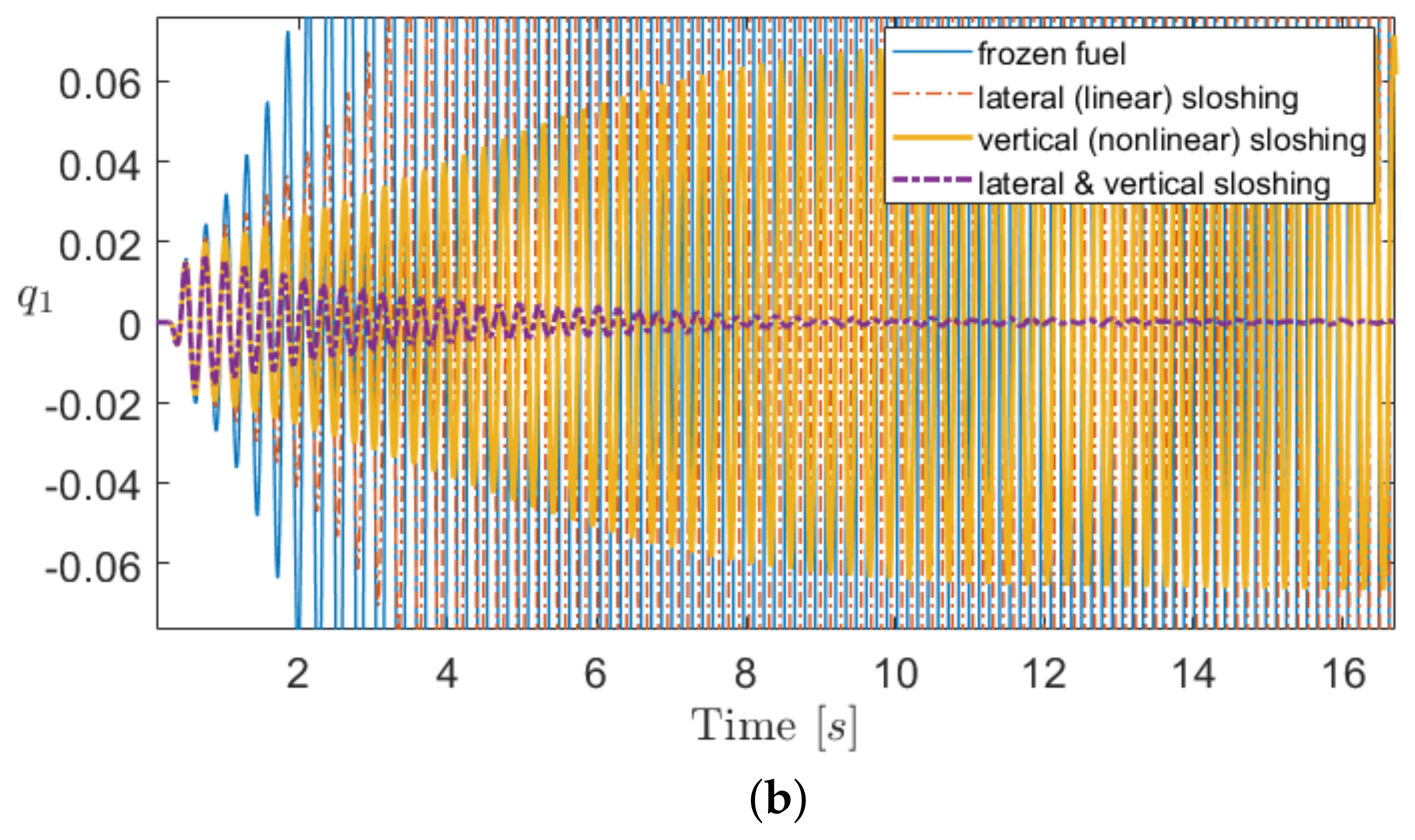
4.1. Test Case 1
4.2. Test Case 2
5. Concluding Remarks
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Acknowledgments
Conflicts of Interest
References
- Gambioli, F.; Chamos, A.; Jones, S.; Guthrie, P.; Webb, J.; Levenhagen, J.; Behruzi, P.; Mastroddi, F.; Malan, A.; Longshaw, S.; et al. Sloshing Wing Dynamics-Project Overview Sloshing Wing Dynamics—Project Overview. In Proceedings of the 8th Transport Research Arena TRA 2020, Helsinki, Finland, 27–30 April 2020. [Google Scholar]
- Firouz-Abadi, R.D.; Zarifian, P.; Haddadpour, H. Effect of Fuel Sloshing in the External Tank on the Flutter of Subsonic Wings. J. Aerosp. Eng. 2014, 27, 04014021. [Google Scholar] [CrossRef]
- Farhat, C.; Chiu, E.K.y.; Amsallem, D.; Schotté, J.S.; Ohayon, R. Modeling of Fuel Sloshing and its Physical Effects on Flutter. AIAA J. 2013, 51, 2252–2265. [Google Scholar] [CrossRef]
- Colella, M.; Saltari, F.; Pizzoli, M.; Mastroddi, F. Sloshing reduced-order models for aeroelastic analyses of innovative aircraft configurations. Aerosp. Sci. Technol. 2021, 118, 107075. [Google Scholar] [CrossRef]
- Pizzoli, M. Investigation of Sloshing Effects on Flexible Aircraft Stability and Response. Aerotec. Missili Spaz. 2020, 99, 297–308. [Google Scholar] [CrossRef]
- Abramson, H.N. The Dynamic Behaviour of Liquids in Moving Containers with Applications to Space Vehicle Technology; The National Aeronautics and Space Administration: Washington, DC, USA, 1966; p. 464. [Google Scholar]
- Ibrahim, R. Liquid Sloshing Dynamics: Theory and Applications; EngineeringPro Collection; Cambridge University Press: Cambridge, UK, 2005. [Google Scholar]
- Graham, E.; Rodriquez, A.M. The Characteristics of Fuel Motion Which Affect Airplane Dynamics; Technical Report; Douglas Aircraft Co. Inc., Defense Technical Information Center: Los Angeles, CA, USA, 1951. [Google Scholar]
- Benjamin, T.B.; Ursell, F.J.; Taylor, G.I. The stability of the plane free surface of a liquid in vertical periodic motion. Proc. R. Soc. London. Ser. A Math. Phys. Sci. 1954, 225, 505–515. [Google Scholar]
- Wright, M.D.; Gambioli, F.; Malan, A.G. CFD Based Non-Dimensional Characterization of Energy Dissipation Due to Verticle Slosh. Appl. Sci. 2021, 11, 33. [Google Scholar] [CrossRef]
- Calderon-Sanchez, J.; Martinez-Carrascal, J.; Gonzalez-Gutierrez, L.M.; Colagrossi, A. A global analysis of a coupled violent vertical sloshing problem using an SPH methodology. Eng. Appl. Comput. Fluid Mech. 2021, 15, 865–888. [Google Scholar] [CrossRef]
- Marrone, S.; Colagrossi, A.; Gambioli, F.; González-Gutiérrez, L. Numerical study on the dissipation mechanisms in sloshing flows induced by violent and high-frequency accelerations. I. Theoretical formulation and numerical investigation. Phys. Rev. Fluids 2021, 6, 114801. [Google Scholar] [CrossRef]
- Marrone, S.; Colagrossi, A.; Calderon-Sanchez, J.; Martinez-Carrascal, J. Numerical study on the dissipation mechanisms in sloshing flows induced by violent and high-frequency accelerations. II. Comparison against experimental data. Phys. Rev. Fluids 2021, 6, 114802. [Google Scholar] [CrossRef]
- Titurus, B.; Cooper, J.E.; Saltari, F.; Mastroddi, F.; Gambioli, F. Analysis of a Sloshing Beam Experiment. In Proceedings of the International Forum on Aeroelasticity and Structural Dynamics, Savannah, GA, USA, 9–13 June 2019; Volume 139. [Google Scholar]
- Wright, M.; Gambioli, F.; Malan, A.G. A Non-dimensional Characterization of Structural Vibration Induced Vertical Slosh. In Proceedings of the 31st International Ocean and Polar Engineering Conference, Rhodes, Greece, 20–25 June 2021. [Google Scholar]
- Saltari, F.; Pizzoli, M.; Coppotelli, G.; Gambioli, F.; Cooper, J.E.; Mastroddi, F. Experimental characterisation of sloshing tank dissipative behaviour in vertical harmonic excitation. J. Fluids Struct. 2022, 109, 103478. [Google Scholar] [CrossRef]
- Constantin, L.; De Courcy, J.; Titurus, B.; Rendall, T.; Cooper, J. Sloshing induced damping across Froude numbers in a harmonically vertically excited system. J. Sound Vib. 2021, 510, 116302. [Google Scholar] [CrossRef]
- Liu, W.; Longshaw, S.M.; Skillen, A.; Emerson, D.R.; Valente, C.; Gambioli, F. A High-performance Open-source Solution for Multiphase Fluid-Structure Interaction. Int. J. Offshore Polar Eng. 2022, 32, 1–7. [Google Scholar] [CrossRef]
- Longshaw, S.M.; Liu, W.; Skillen, A.; Jones, B.W.; Malan, A.G.; Michel, J.; Marrone, S.; Gambioli, F. A Coupled FSI Framework Using the Multiscale Universal Interface. In Proceedings of the International Forum on Aeroelasticity and Structural Dynamics, Madrid, Spain, 13–17 June 2022. [Google Scholar]
- Malan, A.G.; Jones, B.W.S.; Malan, L.C.; Wright, M. Accurate Prediction of Violent Slosh Loads via a Weakly Compressible VoF Formulation. In Proceedings of the 31st International Ocean and Polar Engineering Conference, Rhodes, Greece, 20–25 June 2021. [Google Scholar]
- Jones, B.W.A.; Wright, M.D.; Malan, A.G.; Farao, J.; Gambioli, F.; Longshaw, S. A High Fidelity Fluid-Structure-Interaction Model of the Airbus Protospace Slosh Damping Experiment. In Proceedings of the International Forum on Aeroelasticity and Structural Dynamics 2022, Madrid, Spain, 13–17 June 2022. [Google Scholar]
- Hall, J.; Rendall, T.; Allen, C.B. A Two-Dimensional Computational Model of Fuel Sloshing Effects on Aeroelastic Behaviour. In Proceedings of the 31st AIAA Applied Aerodynamics Conference, San Diego, CA, USA, 24–27 June 2013. [Google Scholar]
- Courcy, J.J.D.; Constantin, L.; Titurus, B.; Rendall, T.; Cooper, J.E. Gust Loads Alleviation Using Sloshing Fuel. In Proceedings of the AIAA Scitech 2021 Forum, Online, 11–15 & 19–21 January 2021. [Google Scholar]
- Pizzoli, M.; Saltari, F.; Mastroddi, F.; Martinez-Carrascal, J.; González-Gutiérrez, L.M. Nonlinear reduced-order model for vertical sloshing by employing neural networks. Nonlinear Dyn. 2021, 107, 1469–1478. [Google Scholar] [CrossRef]
- Saltari, F.; Pizzoli, M.; Gambioli, F.; Jetzschmann, C.; Mastroddi, F. Sloshing reduced-order model based on neural networks for aeroelastic analyses. Aerosp. Sci. Technol. 2022, 127, 107708. [Google Scholar] [CrossRef]
- Haykin, S.O. Neural Networks and Learning Machines, 3rd ed.; Pearson: London, UK, 2009. [Google Scholar]
- Saltari, F.; Riso, C.; Matteis, G.D.; Mastroddi, F. Finite-Element-Based Modeling for Flight Dynamics and Aeroelasticity of Flexible Aircraft. J. Aircr. 2017, 54, 2350–2366. [Google Scholar] [CrossRef]
- Albano, E.; Rodden, W.P. MSC/NASTRAN Aeroelastic Analysis’ User’s Guide; MSC Software: Newport Beach, CA, USA, 1994. [Google Scholar]
- Morino, L.; Mastroddi, F.; Troia, R.D.; Ghiringhelli, G.L.; Mantegazza, P. Matrix fraction approach for finite-state aerodynamic modeling. AIAA J. 1995, 33, 703–711. [Google Scholar] [CrossRef]
- Burnett, E.; Atkinson, C.; Beranek, J.; Sibbitt, B.; Holm-Hansen, B.; Nicolai, L. NDOF Simulation Model for Flight Control Development with Flight Test Correlation. In Proceedings of the AIAA Modeling and Simulation Technologies Conference, Toronto, ON, Canada, 2–5 August 2010; pp. 2010–7780. [Google Scholar]
- Schmidt, D. Stability augmentation and active flutter suppression of a flexible flying-wing drone. J. Guid. Control. Dyn. 2016, 39, 409–422. [Google Scholar] [CrossRef]
- Saltari, F.; Traini, A.; Gambioli, F.; Mastroddi, F. A linearized reduced-order model approach for sloshing to be used for aerospace design. Aerosp. Sci. Technol. 2021, 108, 106369. [Google Scholar] [CrossRef]
- Pizzoli, M.; Saltari, F.; Coppotelli, G.; Mastroddi, F. Experimental Validation of Neural-Network-Based Nonlinear Reduced-Order Model for Vertical Sloshing. In Proceedings of the AIAA Scitech 2022 Forum, San Diego, CA, USA & Virtual, 3–7 January 2022. [Google Scholar]


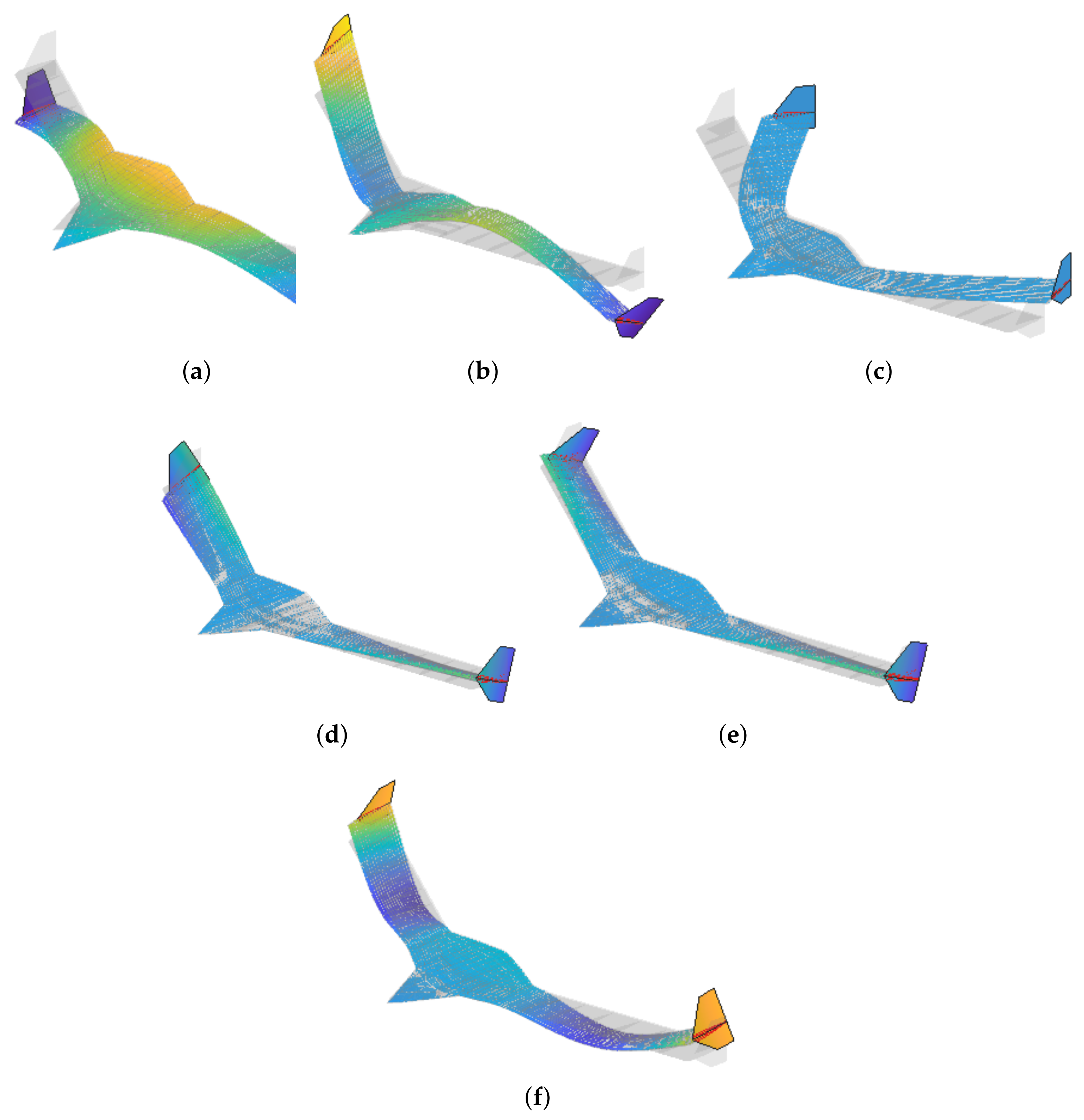






| Parameter | Value (m) |
|---|---|
| side in direction x | |
| side in direction y | |
| h (height) | |
| (filling level ) |
| Mode Number | Direction | Frequency (rad/s) |
|---|---|---|
| 1,2 | x, y | |
| 3,4 | x, y | |
| 5,6 | x, y |
| Parameter | Value (m) |
|---|---|
| side in direction x | |
| side in direction y | |
| h (height) | |
| (filling level ) |
| Mode Number | Direction | Frequency (rad/s) |
|---|---|---|
| 1 | x | |
| 2 | y | |
| 3 | x | |
| 4 | y | |
| 5 | x | |
| 6 | y |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pizzoli, M.; Saltari, F.; Mastroddi, F. Linear and Nonlinear Reduced Order Models for Sloshing for Aeroelastic Stability and Response Predictions. Appl. Sci. 2022, 12, 8762. https://doi.org/10.3390/app12178762
Pizzoli M, Saltari F, Mastroddi F. Linear and Nonlinear Reduced Order Models for Sloshing for Aeroelastic Stability and Response Predictions. Applied Sciences. 2022; 12(17):8762. https://doi.org/10.3390/app12178762
Chicago/Turabian StylePizzoli, Marco, Francesco Saltari, and Franco Mastroddi. 2022. "Linear and Nonlinear Reduced Order Models for Sloshing for Aeroelastic Stability and Response Predictions" Applied Sciences 12, no. 17: 8762. https://doi.org/10.3390/app12178762
APA StylePizzoli, M., Saltari, F., & Mastroddi, F. (2022). Linear and Nonlinear Reduced Order Models for Sloshing for Aeroelastic Stability and Response Predictions. Applied Sciences, 12(17), 8762. https://doi.org/10.3390/app12178762





