3.1. Verification of Numerical Results
In the author’s previous study, a fixed offshore structure was analyzed using the developed program to verify natural periods, dynamic responses, and bending stresses with commercial programs [
39]. The dynamic response of the floating body was also verified through regular wave analysis and free-decay tests in the previous study [
19].
In general, the motion characteristics of a floating body can be evaluated by the Response Amplitude Operator (RAO), which expresses the relationship between the wave amplitude and the body motion according to the period of the incident wave.
In this section, the RAO of the floating body for linear and body-nonlinear analysis was obtained through the time-domain simulations under irregular wave conditions, and the validity of the developed program was verified by comparing the RAO with previous studies.
The RAO was calculated using cross- and auto-spectral densities [
40]. The frequency interval between regular waves was set randomly to prevent repeatability of the generated wave.
where
and
are the cross- and auto-spectral densities, respectively. In this process, the input signal
represents a time history of a random wave, and the output signal
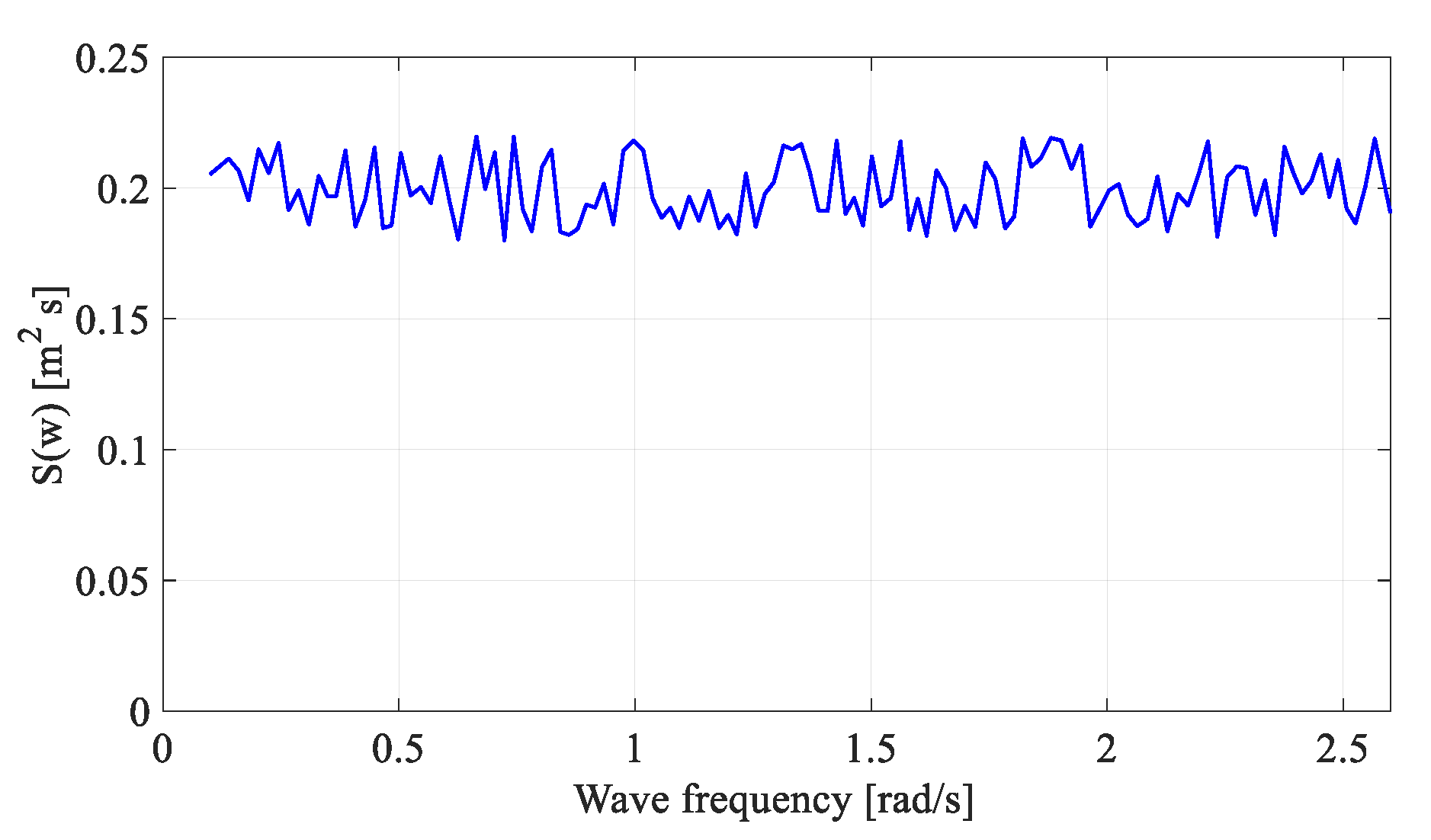
represents system responses, such as the heave or pitch motion of the float. The wave was generated using the white noise spectrum shown in
Figure 2 to calculate the RAO in the assigned frequency range.
3.1.1. Heave RAO of a Spherical Float
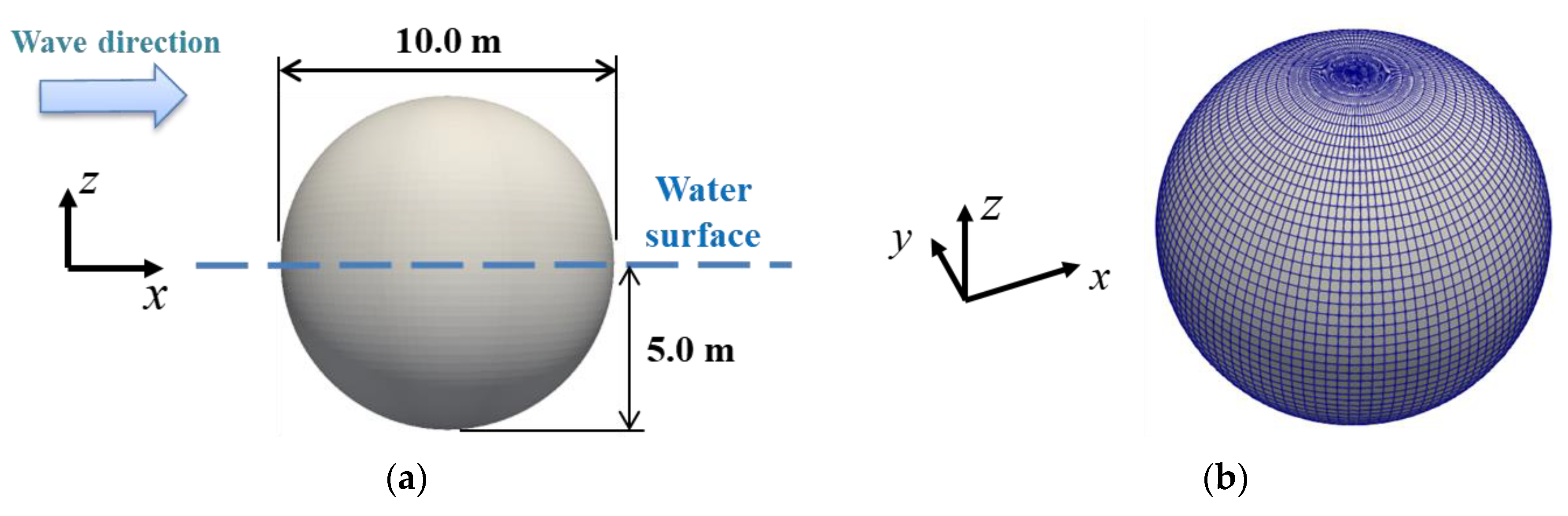
Figure 3 depicts a spherical float with a diameter of 10 m and a draft of 5 m to verify the RAO calculation. The mass of the float was 261,800 kg. The water depth was infinite, and the density of the water was 1000 kg/m
3. The float was modeled with 7200 quadrilateral elements. The float has one degree of freedom, with the motion constrained except for vertical (heave) motion. Frequency-domain hydrodynamic parameters (added mass, radiation-damping coefficient, and diffraction force) were obtained using WAMIT. Among the external forces acting on the float, the diffraction and radiation forces were applied as linear forces.
A time history of wave elevation was generated using the white noise spectrum shown in
Figure 2. The frequency ranged from 0.1 rad/s to 4.0 rad/s, and irregular waves were generated with 196 regular component waves. A simulation result of 3600 s after a 100 s ramping period was used to remove the initial transient motions.
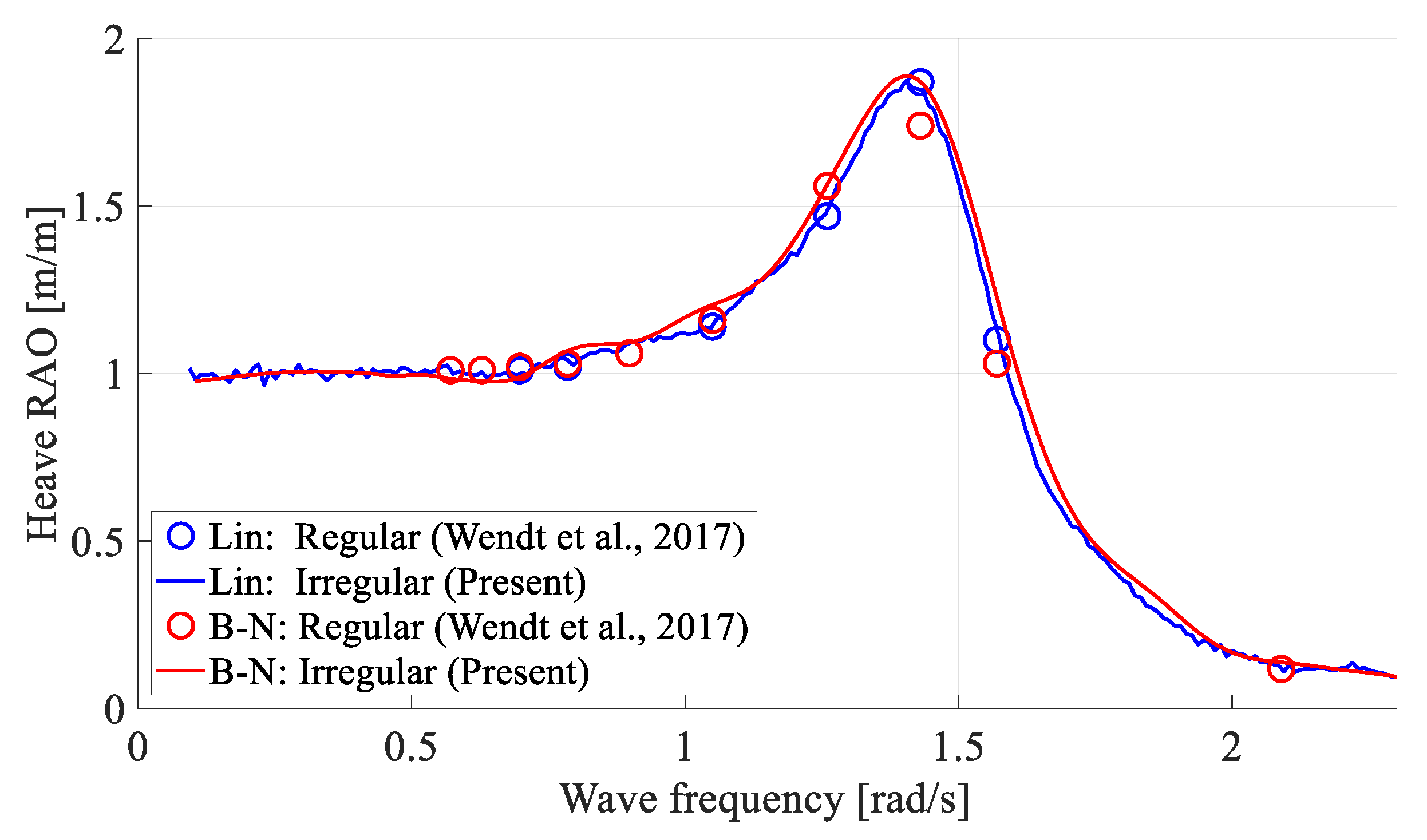
Figure 4 compares the heave RAOs of the half-submerged sphere with the results of a previous study [
41]. While [
41] obtained RAOs in regular waves of respective frequencies, the present method calculated the RAOs at all frequencies from one time-domain simulation using cross- and auto-spectral densities. The two independent results agreed well across all frequency ranges. In general, the results of the body-nonlinear analysis were similar to those of linear analysis over the entire frequency range for the given example.
3.1.2. Pitch RAO of a Spherical Float Constrained to a Hinge Point
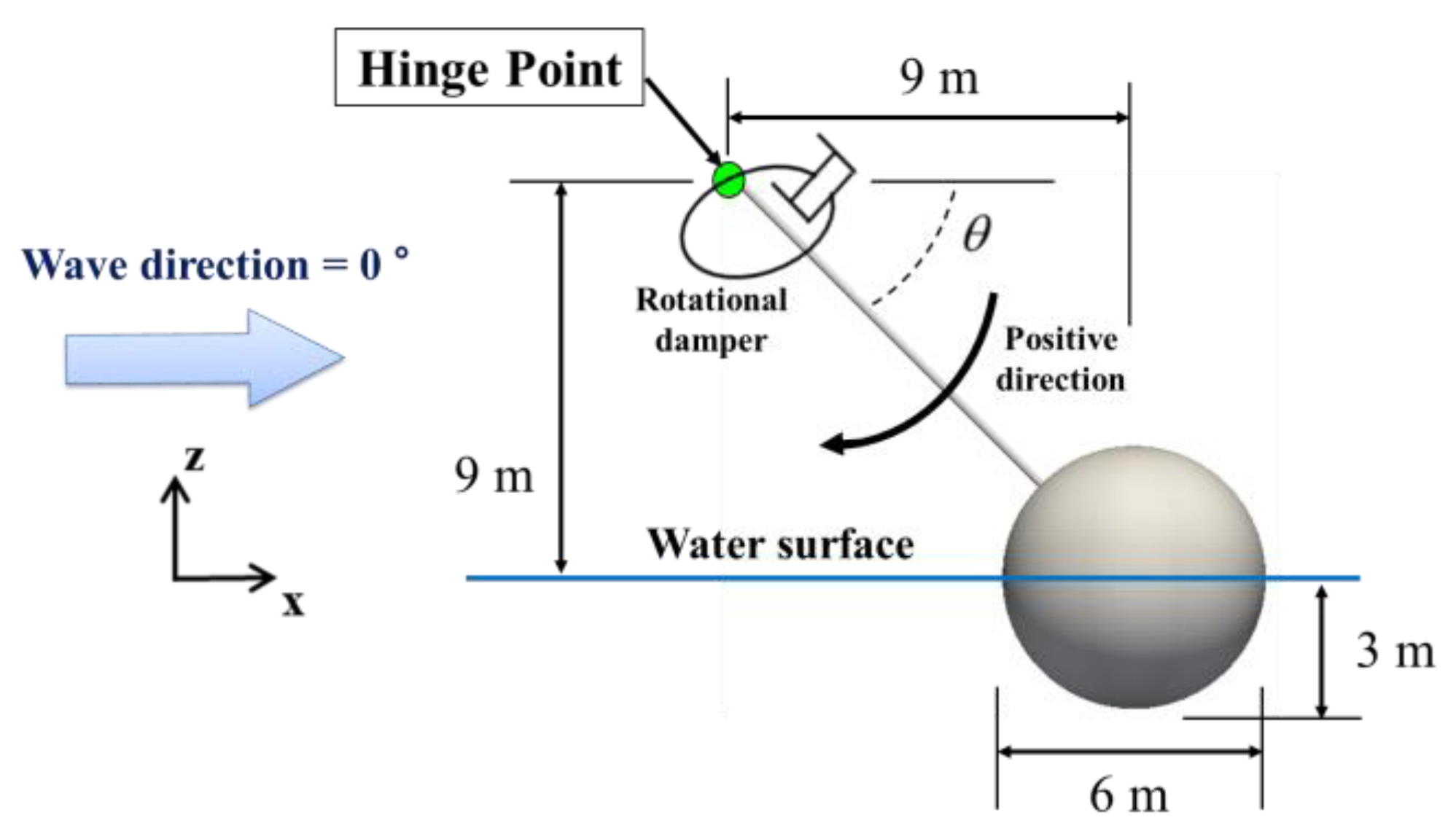
Figure 5 depicts a spherical float constrained to a hinge point with a massless rigid arm. The diameter and draft of the float were 6 m and 5 m, respectively. The mass of the float was 57,962 kg. The water depth was 20 m, and the density of the water was 1025 kg/m
3. The float was modeled with 7200 quadrilateral elements. The float was constrained in motion except for the rotational motion in the
y-axis by a hinge joint. A linear rotational damper was applied to the hinge point to describe the PTO energy extraction (damping) system. The moment acting on the hinge point (
) can be expressed as
where
is the angular velocity of the float, and
is the rotational PTO damping coefficient of a rotational damper.
= 3.52
10
6 N·m/(rad/s) was applied for comparison. Frequency-domain hydrodynamic parameters were also obtained using WAMIT. The diffraction and radiation forces were applied as linear forces among the external forces.
For this example, a time history of the incident random wave was generated using the white noise spectrum of frequency range from 0.1 rad/s to 2.6 rad/s with 126 regular component waves. A simulation result of 3600 s after a 100 s ramping period was used for the subsequent analysis.
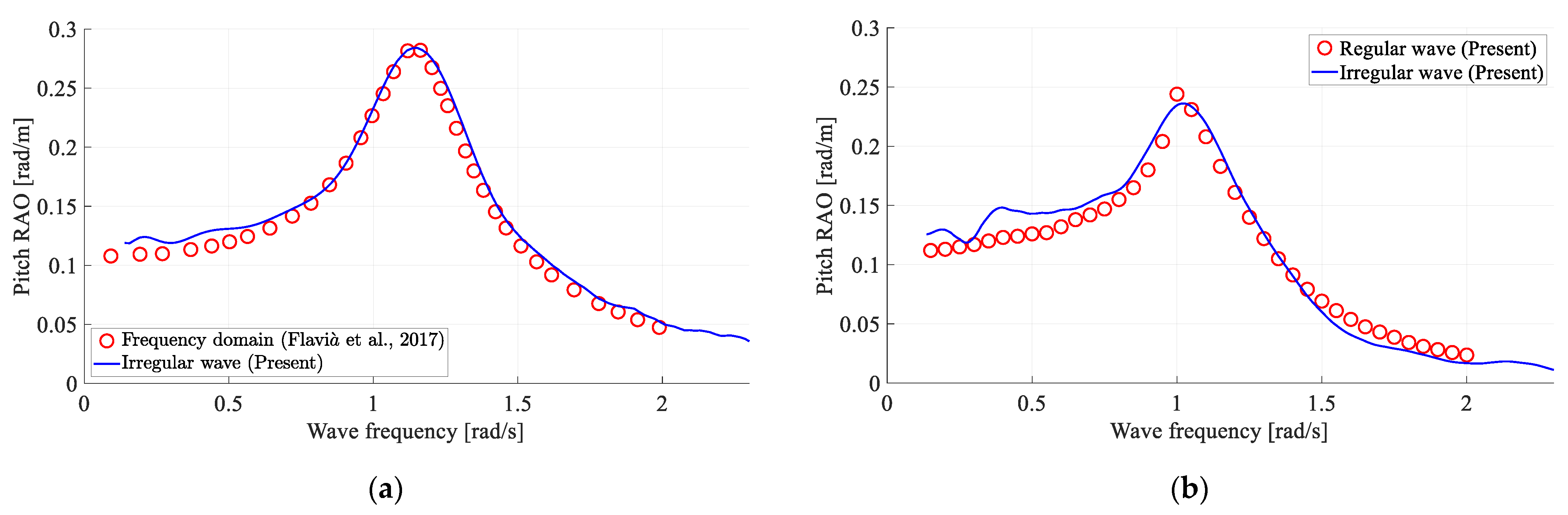
The linear-analysis results are first presented in
Figure 6a to compare the pitch RAO of the float with the previous study [
42], which generally shows good agreement.
Figure 6b presents the pitch RAOs obtained by authors through the body-nonlinear analysis under regular and irregular wave conditions. Both results agree well except for some minor differences in the low-frequency region. When comparing
Figure 6a,b, the magnitude of the pitch RAO became smaller when the body-nonlinear analysis was performed. This trend was different from the case of
Figure 4 (only heave-allowed sphere) without a rotating arm. When the floating body rotates around the hinge point, the floating body moves up and down but also in the horizontal direction, i.e., the instantaneous x-position of the body also changes. This caused the increased difference between the linear and body-nonlinear results of
Figure 6b compared to
Figure 4. This example illustrates that the body-nonlinear analysis can estimate the performance of the hinged-buoy system more accurately than the linear analysis.
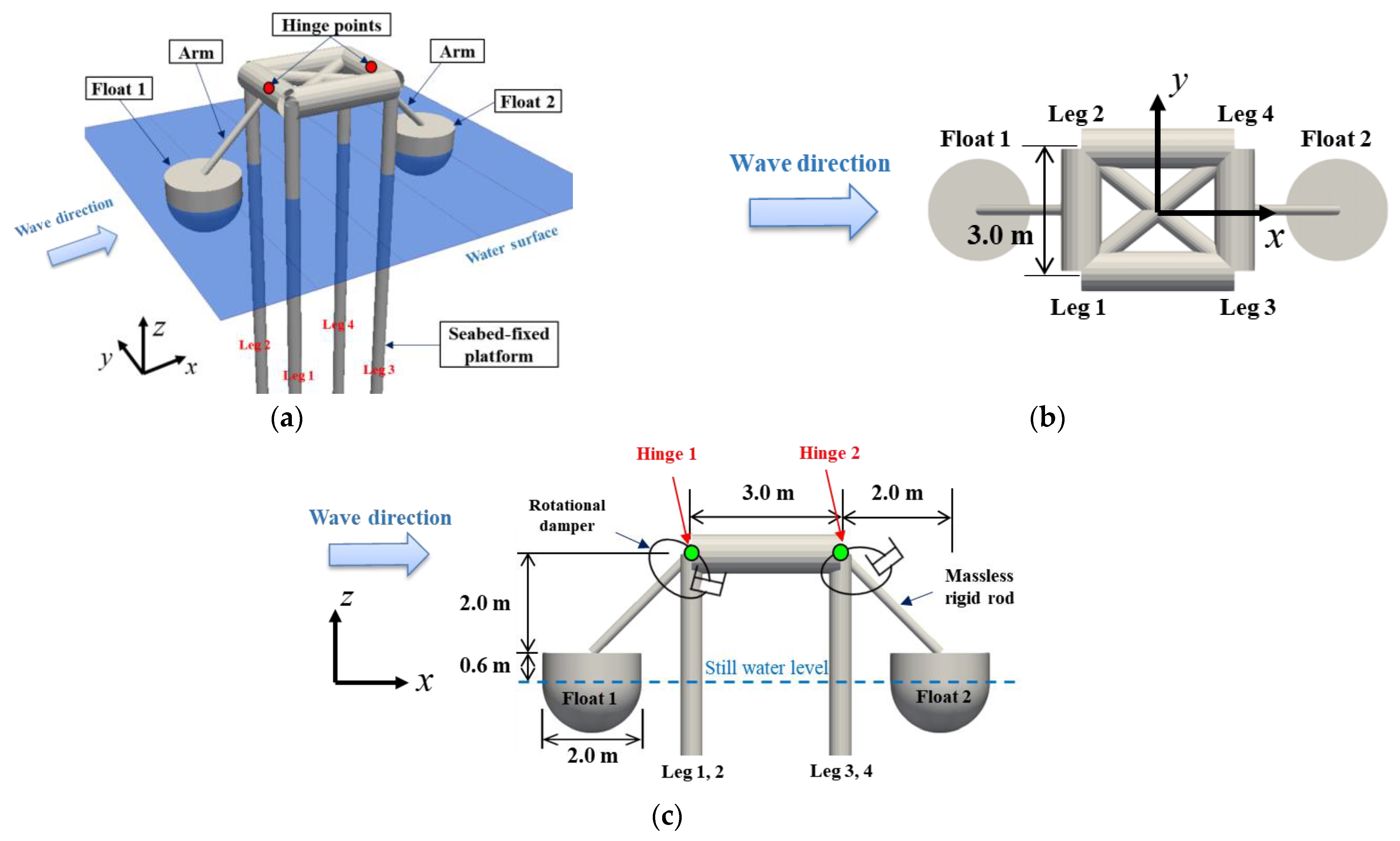
3.2. Simulation Results and Analysis of the Proposed System
In this study, the floating body is composed of a hemispherical shape (diameter = 2 m and draft = 1 m below the still water level) with the upper part as a vertical circular cylinder of diameter = 2 m, and height = 0.6 m. The mass of each float was 2147 kg. Each float consists of 8520 quadrilateral elements for hydrodynamic computation.
Figure 7 describes the float.
Before analyzing the coupled effects with the foundation structure, the pitch RAO difference between the spherical float and the present body (hemispherical float with vertical circular cylinder on top) was analyzed. The single spherical float has the same mass as the present body and consists of the same number of quadrilateral elements. The water depth was 50 m, and the applied seawater density was 1025 kg/m
3. Each float was fixed to a massless rigid rod, the other end of which was hinge-connected to the foundation structure. This connection allowed rotational movement with respect to the joint at the foundation structure as the center of rotation. The rotational damping proportional to the rotational velocity was applied to the hinge point to describe the PTO system, as shown in Equation (23). This PTO moment resists the rotational motion. The extraction coefficient of 100 kN·m/(rad/s) was applied through a parametric study for a regular wave [
19].
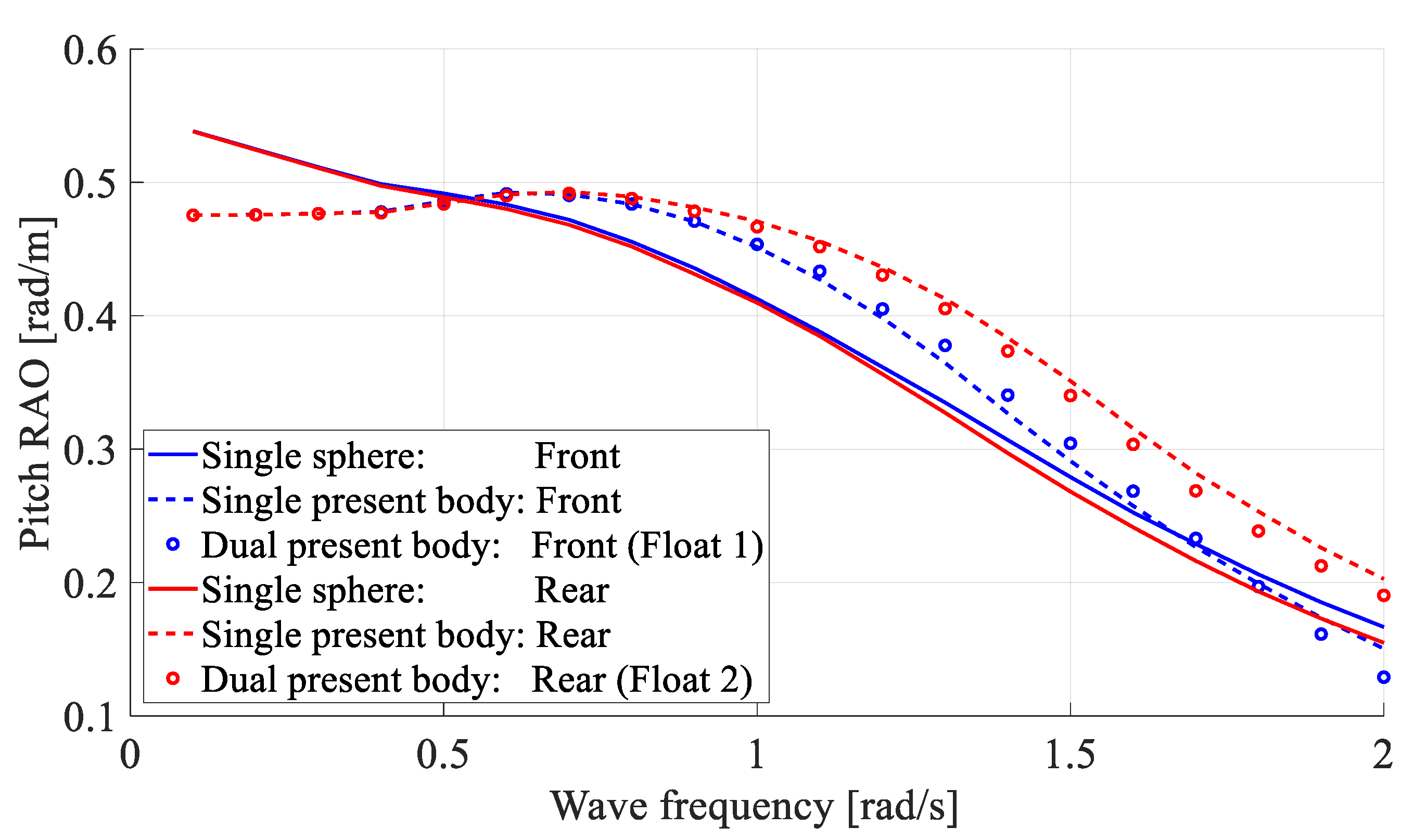
Figure 8 and
Figure 9 compare their pitch RAOs, showing the body-nonlinear results. The pitch RAOs of the present body were larger than those of the sphere when the wave frequency was larger than 0.5 rad/s, i.e., in practical sea states, and the present body was superior to the sphere. In this regard, the present body shape was chosen for the subsequent section.
In addition, the pitch RAOs of the dual present bodies were calculated and compared.
Figure 8 shows the set-up of single and dual floating bodies. The rotational displacement of the floating body was affected by the propagation direction of the incident wave. When the incident wave propagated in the x-direction parallel to the arm, the rotational displacement of the floating body became a maximum [
19], which was assumed in this paper. The weather-side buoy was called the “front”, and the lee-side buoy was called the “rear”. In the case of dual buoys, the full hydrodynamic interactions of the two floating bodies were included.
Figure 9 shows the pitch RAOs of various cases. The pitch RAOs of the front and rear tend to be similar, but the rear becomes slightly larger when the wave frequency is larger than 0.7 rad/s. The differences between the single and dual were small, suggesting that the two-body interaction effects were insignificant in this example. For multiple WECs, more detailed research on the optimal arrangement needs to be investigated for maximum energy extraction efficiency [
43].
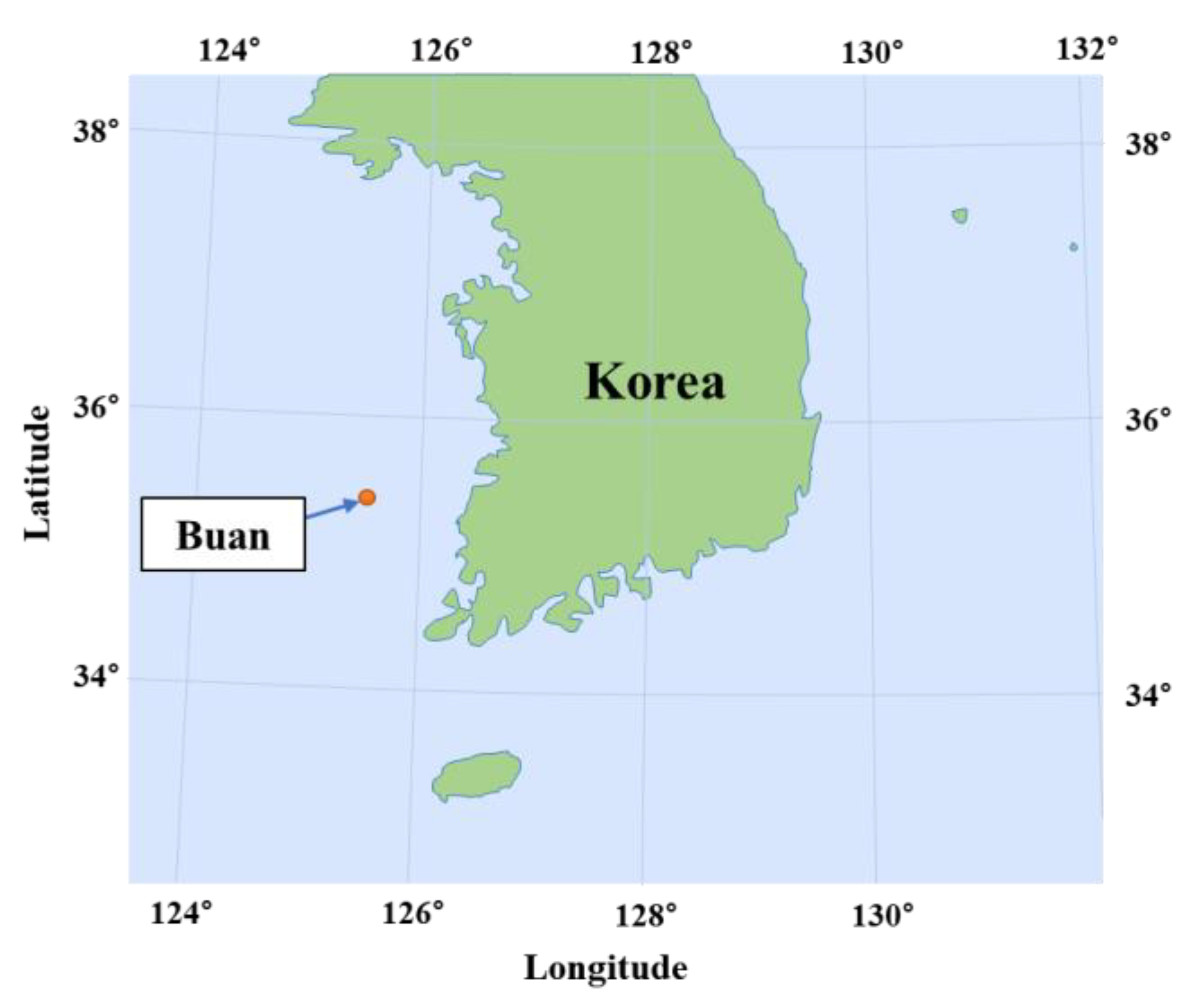
In the remainder of this study, the fully coupled dynamic analysis of a bottom-fixed elastic foundation platform with WECs attached was performed. For the real sea conditions, Buan, one of the nearshore regions of Korea, was selected by referring to previous studies [
19,
25].
Figure 10 shows the location of the wave buoy installed in the Buan site for wave data measurement. The five-year average winter wave data were collected by the Korea Meteorological Administration (KMA) from November to February, from 2017 to 2021 [
44].
Table 1 lists the average significant wave height, average peak period, and percentage of overall availability. The direction of all incident waves was the same to estimate the potential maximum power production. Based on the actual sea data, a representative significant wave height of 1.28 m and a peak period of 5.46 s (=1.15 rad/s) were selected. The water depth was 50 m, and the applied seawater density was 1025 kg/m
3.
A PM spectrum was applied to generate a time history of the incident wave. The wave direction was fixed in the
x-axis direction, and 130 regular component waves were superposed. The frequency range was 0.7 rad/s to 3.4 rad/s, excluding values less than 1% of the maximum spectrum value under the corresponding environmental condition. A simulation result of 3600 s after a 100-s ramping period was used for further analysis.
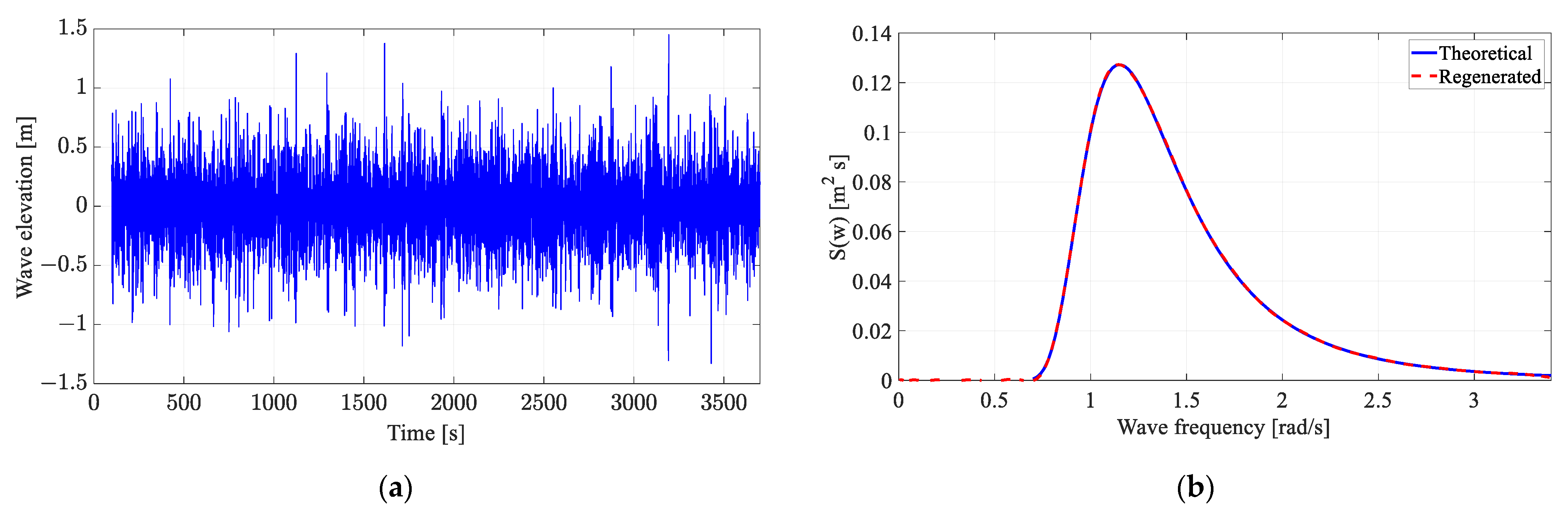
Figure 11a shows the generated incident wave time series applied in this study. For validation, the theoretical PM spectrum and the regenerated spectrum obtained using the fast Fourier transform (FFT) with smoothing from the time series were compared (
Figure 11b).
Figure 12 shows the bottom-fixed foundation structure with two WECs, and
Table 2 lists the detailed structural properties of the platform. The platform consisted of 122 nodal points and 124 three-dimensional beam elements, and the deck was supported by four legs. The nodal points at the bottom are fixed to the seabed. The structural elements were all slender, and the Morison equation could be used. The inertia and drag coefficients of each leg were 2.0 and 1.0, respectively, which is typical for such slender cylindrical elements. The deck weight was 980 kN, and it acted equally on each leg. Two floating buoys in
Figure 7 were connected to the foundation structure, as described in the previous sections.
The rotational damping proportional to the rotational velocity of the float was applied to the center of rotation as the PTO system. The optimal extraction coefficient was obtained as 100 kN·m/(rad/s) through a parametric study for the regular wave [
19]. The wave power generated by the PTO system was proportional to the square of the rotational velocity of the float, as shown in the following equation.
The distance between hinge points was set to 3 m. This distance does not affect the pitch RAO of the float significantly when the platform is fixed [
19]. The frequency-dependent hydrodynamic coefficients considering the interaction between the two floats were obtained using multi-body WAMIT. The interactive hydrodynamic coefficients are the same because the two floats have the same shape and size.
The natural frequency of the platform was obtained by modal analysis, and the total number of vibration modes was 708.
Table 3 lists the natural frequencies up to the fifth mode. In this study, all vibration modes were considered, and the equation of motion of the platform was expressed using 708 SDOFs.
The results without WECs were first compared to identify the dynamic response characteristics of the platform.
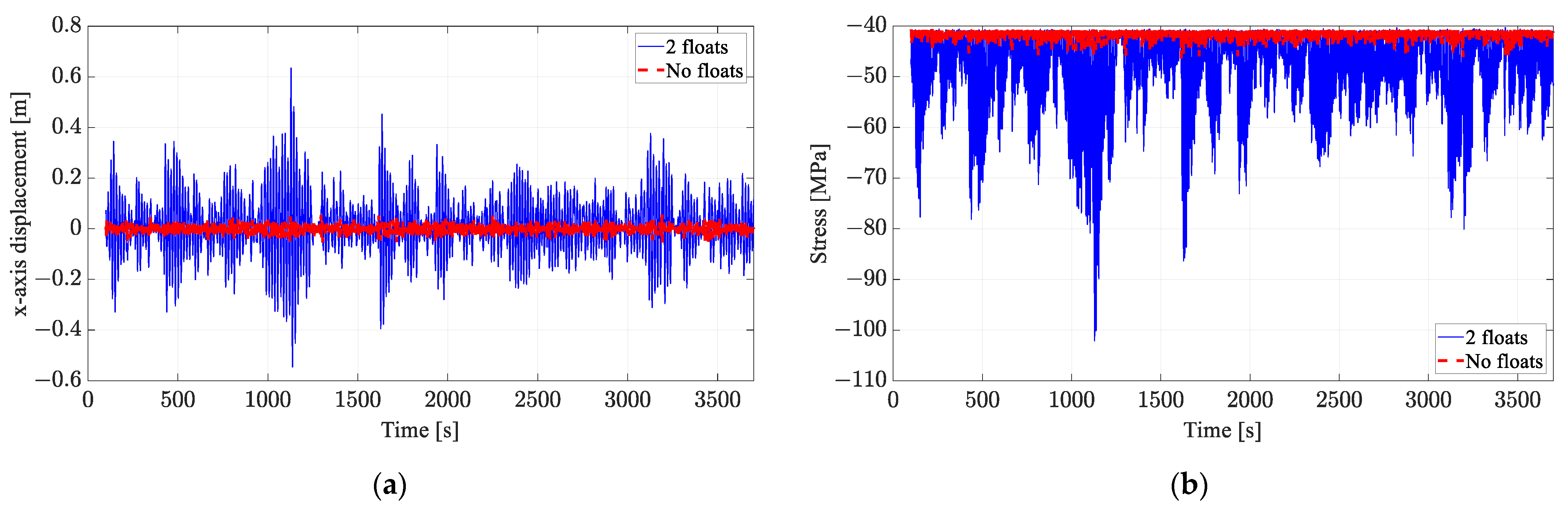
Figure 13 shows the time histories of
x-axis displacement at the top of Leg 1 and stress at the bottom of Leg 1. The stress shown here represents the sum of axial stress and bending stress acting on each element of the structure. According to the figure, the displacement and stress increased significantly due to the influence of the floats. The response of the platform was affected by the horizontal and vertical constraint forces from the floats. The negative (compressive) stress was caused by external forces from the floats and the weight of the platform. The former particularly plays a significant role in the increase in dynamic stress. As the displacement increases, the bending stress of each element also increases, resulting in a similar trend to the time series of displacement and stress. The results underscore that this kind of fully coupled dynamic analysis is essential for a reliable estimation of the fatigue life of the system.
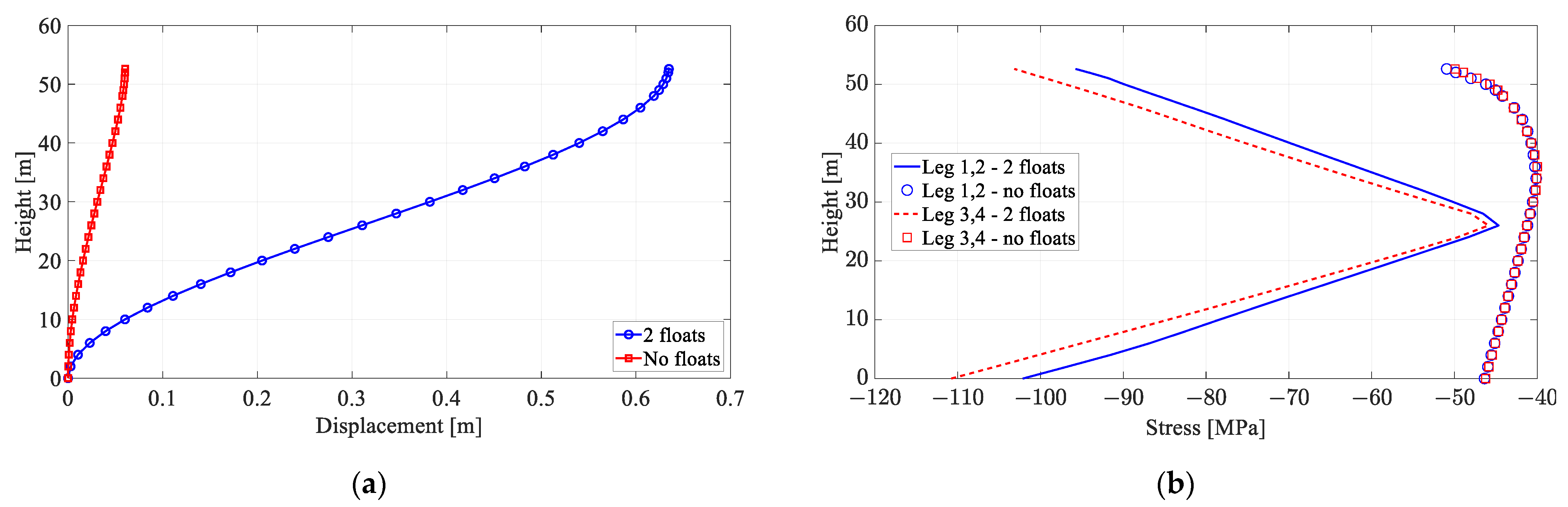
Figure 14 shows the distribution of the maximum absolute value of the displacement and stress of each element from the seabed to the deck. The displacement at the top had the same value in all legs because the stiffness of the deck was considerable. In addition, the stresses on legs 1 and 2 (front legs) are the same because the structure/loading is
x-axis-symmetric. Similarly, the stresses on legs 3 and 4 (rear legs) were the same. The displacements were zero at the sea bottom because the structure was fixed there and increased monotonically toward the top, as shown in
Figure 14a. The top displacement was increased significantly (~10 times) with the two floats compared to the no-float case. The maximum stresses occurred at the top and the bottom of the foundation structure. The significantly increased stress at the top was attributed to the dynamic coupling with the WECs, as shown in
Figure 14b. The magnitude of the stress was the smallest in the middle of the leg because of the smallest degree of bending. The rear legs had a maximum stress approximately 7% greater than the front legs because of the differences in interaction forces by front and rear floats.
Table 4 lists the statistics of the dynamic responses and stresses of the platform. Owing to the influence of WECs, the positive maximum x-displacement at the top of the structure was larger than the negative maximum. This means that the structure tends to move more in the incident wave direction. In this study, simulation results were analyzed using Root Mean Square (RMS), one of the statistical parameters as a measure of dynamic fluctuation. The RMS values of the stress were approximately 20% increased because of the influence of the floats. On the other hand, the maximum value differed by approximately 2.2 times. Hence, fully coupled analysis is essential for the reliable design of the structure.
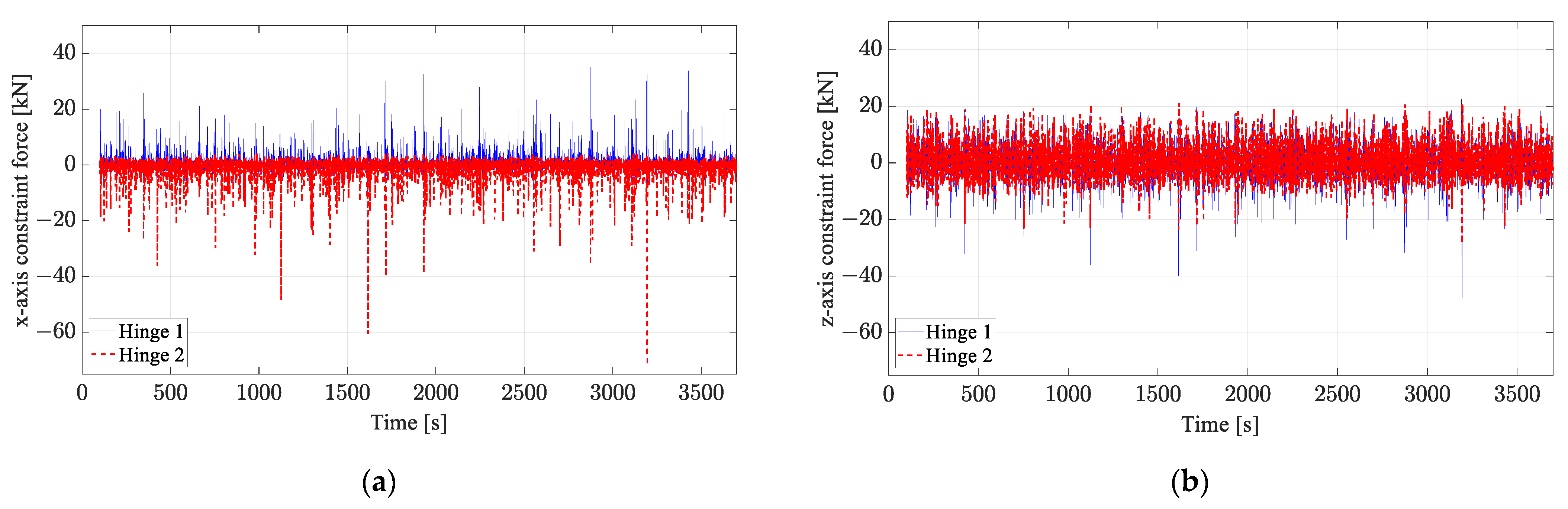
Figure 15 and
Table 5 show the time histories of the constraint forces acting on the hinge points and their statistics considering the influence of the floats. As shown in
Figure 15a, the
x-axis constraint force at Hinge 1 (front) has large positive values, and that at Hinge 2 (rear) has similar negative values. This is because the movement of each float is constrained by the hinge point, so the constraint force acts in the opposite direction to the movement of the float to satisfy the constraint condition. The
z-axis constraint forces acting on the two hinge points are similar in magnitude, as shown in
Figure 15b. The RMS value of the constraint force is larger in the
z-axis, but the maximum value occurred on the
x-axis.
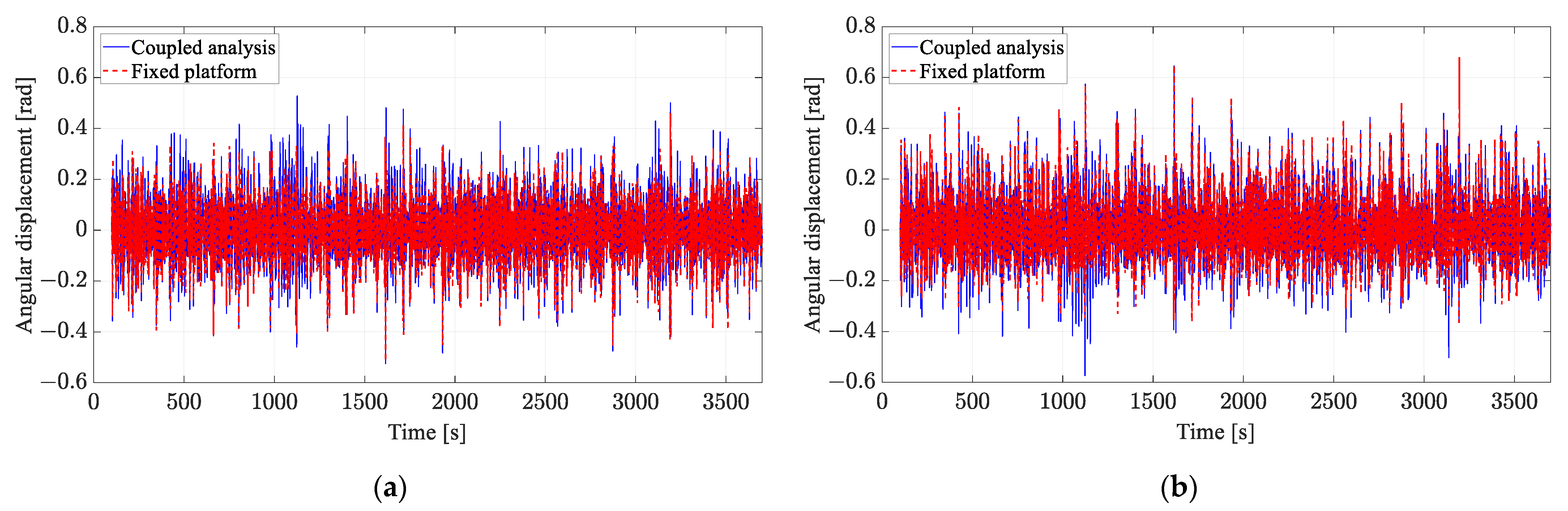
Figure 16 and
Table 6 show the differences in the float rotational motions with or without considering platform elastic responses, i.e., elastic platform vs. fixed platform. The rotational motions of both floats are slightly increased after including the elastic response of the platform. Because the foundation structure is not as flexible, their differences are expected to be small, as in
Figure 16 and
Table 6.
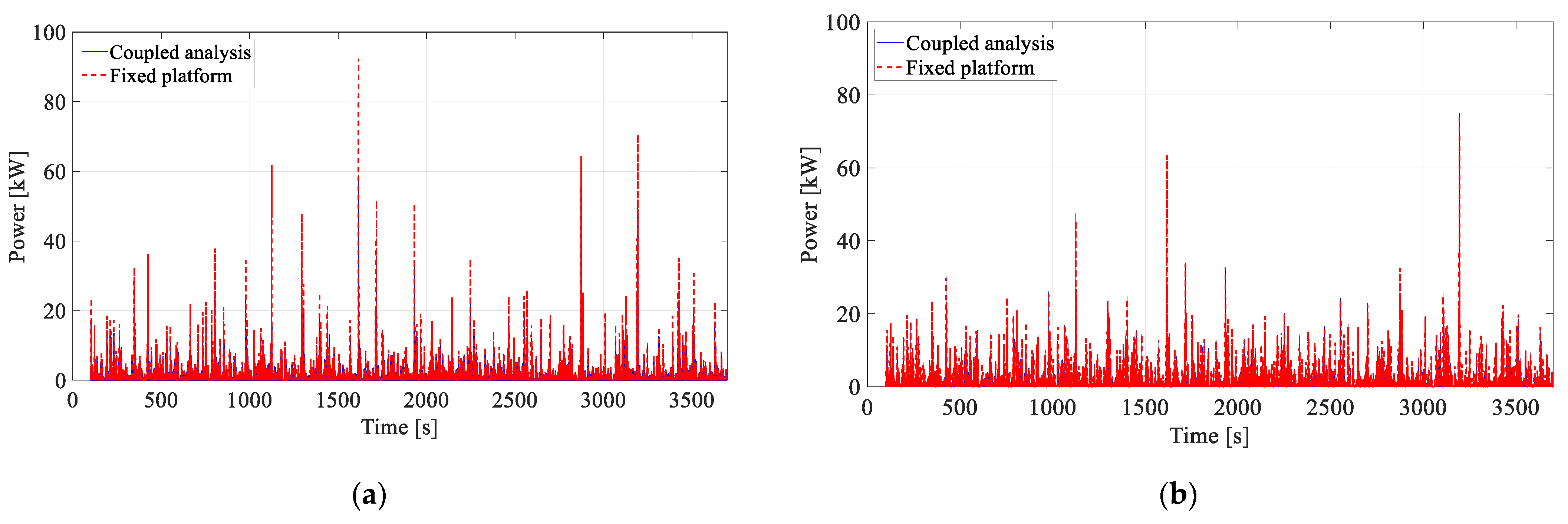
Figure 17 and
Table 7 show the time histories of the produced wave power and their statistics for both fixed and elastic foundation structures. Similar to the previous results, the RMS values of the produced power from Floats 1 and 2 are affected slightly by the elastic responses of the platform. Interestingly, the average power production of Float 2 (rear) was slightly larger than that of Float 1 (front) because of two-body hydrodynamic interactions (see
Figure 9) and other coupled effects. The power fluctuations (standard deviation) were reduced slightly, which is beneficial, by considering coupled effects with the platform elasticity.