Improved Interaction Formula for the Plastic Resistance of I- and H-Sections under a Combination of Bending Moments M_y,Ed, M_z,Ed, and Bimoment B_Ed

Abstract

The resistances of HVH sections (H—horizontal upper flange, V—vertical web, H—horizontal bottom flange) were investigated by the authors in the frame of the large parametrical studies focused on the complete HVH-section categories: channels, Z-sections, I-sections, and H-sections. The three different variants of the approximate formulae for the calculation of the elastic and plastic resistances of I-shaped sections loaded by several internal forces were previously published by the authors. In this paper, the improved approximate formulae are presented for the plastic resistance of I- and H-sections loaded by bending moments M_y,Ed, M_z,Ed, and bimoment B_Ed. It is shown in the graphical form that the proposed approximate formulae give almost identical values of relative plastic resistances of I-shaped sections to the exact solution, in which Pattern Search Algorithm, offered by MATLAB, is used. It is proven that the proposed approximate formulae are better then previous authors’ formulae, which are themselves better than the older Greek proposal and the newer German proposals. The approximate formulae may be used in the CEN Technical Specification (TS) or in Non Contradictory Complementary Information (NCCI) for supporting safe and economical design according to metal (steel and aluminum) Eurocodes.

1. Introduction

The current metal Eurocodes [1,2] do not contain rules for the calculation of the plastic resistance of members loaded by a combination of bending moment(s) and bimoment. Eurocode [1] contains only a conservative linear-interaction formula (Equation (6.2) in [1]). Equation (6.2) is valid for class 1, class 2, or class 3 cross sections subjected to the combination of N_Ed, M_y,Ed, and M_z,Ed. According to the Eurocode [1]: “The plastic resistance of cross sections should be verified by finding a stress distribution which is in equilibrium with the internal forces and moments without exceeding the yield strength. This stress distribution should be compatible with the associated plastic deformations” (cl. 6.2.1(6) in [1]). Similar clauses are missing in [2].

Both drafts of the new generation of Eurocodes [3,4] contain the equations for the calculation of the design bending moment reduced by the bimoment. In cl. 8.2.7(7) [3], the authors state: “For Class 1 or Class 2 symmetric I-cross-sections in bending about the major axis, the design plastic bending moment resistance M_c,B,Rd reduced by the bimoment B_Ed may be calculated from Formula (8.29)”. Formula (8.29) is the second Formula (3) in this paper, and the history of its development is described below. The draft of the Eurocode [4] applies a similar formula (see formula (8.38) in cl. 8.2.7(6) [4]). Both drafts of the Eurocodes [3,4] contain the following the sentence: “For determining the plastic moment resistance of a cross-section due to bending and torsion, only torsion effects B_Ed should be derived from elastic analysis” (see 8.2.7(6) in [3] or 8.2.7(5) in [4]).

The need for an interaction formula for the calculation of the plastic resistance of the I-shaped section was solved by the authors in [5], in which three variants of the formula were presented, taking into account the influence of three internal forces: two bending moments, M_y,Ed and M_z,Ed, and a bimoment, B_Ed. In this paper, variant No.4 is developed, which is more exact than the previous variants, No.1, No.2, and No.3 published in [5].

In Table 1 in [5], the authors present three approximate formulae variants. Table 1 in [5] shows that variant No. 3 is the best. The purpose of this paper is to present variant No. 4, which is better than variant No. 3. Variant No. 4 is defined by the formulae in Table 1, below, and by the equations in (37). The approximate results agree well with the exact results obtained by linear programming (Figure 1 and Figure 2). Figure 1 and Figure 2 show also comparisons with the older results obtained by Vayas (Equations (9) and (21c) in [6]), and to the brand new results obtained by Ludwig (Equation (29) in [7]). We can see that the results of the proposed variant No. 4 fit the exact curves well (Figure 1 and Figure 2). The proposals of Vayas and Ludwig substantially differ within some intervals of the exact results.

It is worth mentioning that Ludwig informed us that he used paper [8]. He forgot to include [8] in the list of references in [7]. Moreover, Ludwig mistakenly referred to the formula in Table 6 [7] as Mirambell’s formula and referred to [26] ([9] here). The formula in this form is not found in [26]. In fact, it is Baláž’s formula, taken by Ludwig from [8], and not from [26]. Baláž’s formula was accepted for the last draft of prEN 1993-1-1 [2], where it replaced Mirambell’s original, incorrect formula (see Figure 3b in [8]). The exact solution of the plastic resistance of the section under a combination of several internal forces can be obtained by (a) dividing a section into three elements (upper flange, bottom flange, and web) and (b) using linear programming (Osterrieder [10]). Method (a) was applied by Yang, Chern, and Fan [11], Kindmann and Frickel [12], and Rubin [13] by taking into account up to eight internal forces [14]. The programs developed by DLUBAL [15] and Aguero [16] are based on the simplex method.

1.1. Linear-Interaction Formula

The conservative approach for all cross-section classes is represented by formula (6.2) in EN 1993-1-1:2005 [1]. It is the linear summation of the utilization ratios of all the components. For Classes 1 and 2, Formula (6.2) [1] takes, without the influence of the normal force N_Ed, the following form, if the bimoment B_Ed is added:

$$\frac{M_{y,Ed}}{M_{pl,y,Rd}}+\frac{M_{z,Ed}}{M_{pl,z,Rd}}+\frac{B_{Ed}}{B_{pl,Rd}}\le 1$$

(1)

1.2. Proposals for Interaction Formula Containing My,Ed and BEd

Streľbickaja derived such a formula for channel and I-shaped sections. The formula for I-shaped sections is as follows:

$${\left(\frac{M_{y,Ed}-M_{w,pl,y,Rd}}{M_{pl,y,Rd}-M_{w,pl,y,Rd}}\right)}^2+\frac{B_{Ed}}{B_{pl,Rd}}\le 1$$

(2)

Formula (2) was simplified by Baláž in the form (3) and was applied in [17,18,19,20,21].

$$\begin{gathered} {\left(\frac{M_{y,Ed}}{M_{pl,y,Rd}}\right)}^2+\frac{B_{Ed}}{B_{pl,Rd}}\le 1; \\ {M}_{c,B,Rd}=\sqrt{1-\frac{B_{Ed}}{B_{pl,Rd}}}{M}_{pl,Rd} \end{gathered}$$

(3)

The first proposal (4) for the previous prEN 1993-1-1 draft was made by Mirambell, Bordallo, and Real [9], where relevant hypotheses are described in detail. In the derivation of Formula (4), the analogy was utilized between the stress distribution in a rectangle section due to normal force combined with bending moment and the stress distribution in the flange of an I-section due to the bending moment and bimoment in the plastic state. Formula (4) was the first approach to solve the bending–warping torsion interaction within the framework of prEN1993-1-1 [2]. This approach was proposed because it was simple and easy to use and because it also employed a formulation already implemented into EN 1993-1-1 [1] to consider the shear–torsion interaction.

$$M_{c,B,Rd}=\sqrt{1-\frac{{\sigma }_{w,Ed,\max }}{\frac{1.25f_y}{{\gamma }_{M0}}}}{M}_{pl,y,Rd}$$

(4)

Working group EN 1993-1-1 finally accepted Formula (3), proposed by Baláž in the Formula (3).

In this paper, a generalization of Equation (3) also considers M_z,Ed, which needs to be taken into account when analyzing the behavior of unrestrained beams sensitive to lateral torsional buckling (Agüero et al. [22]).

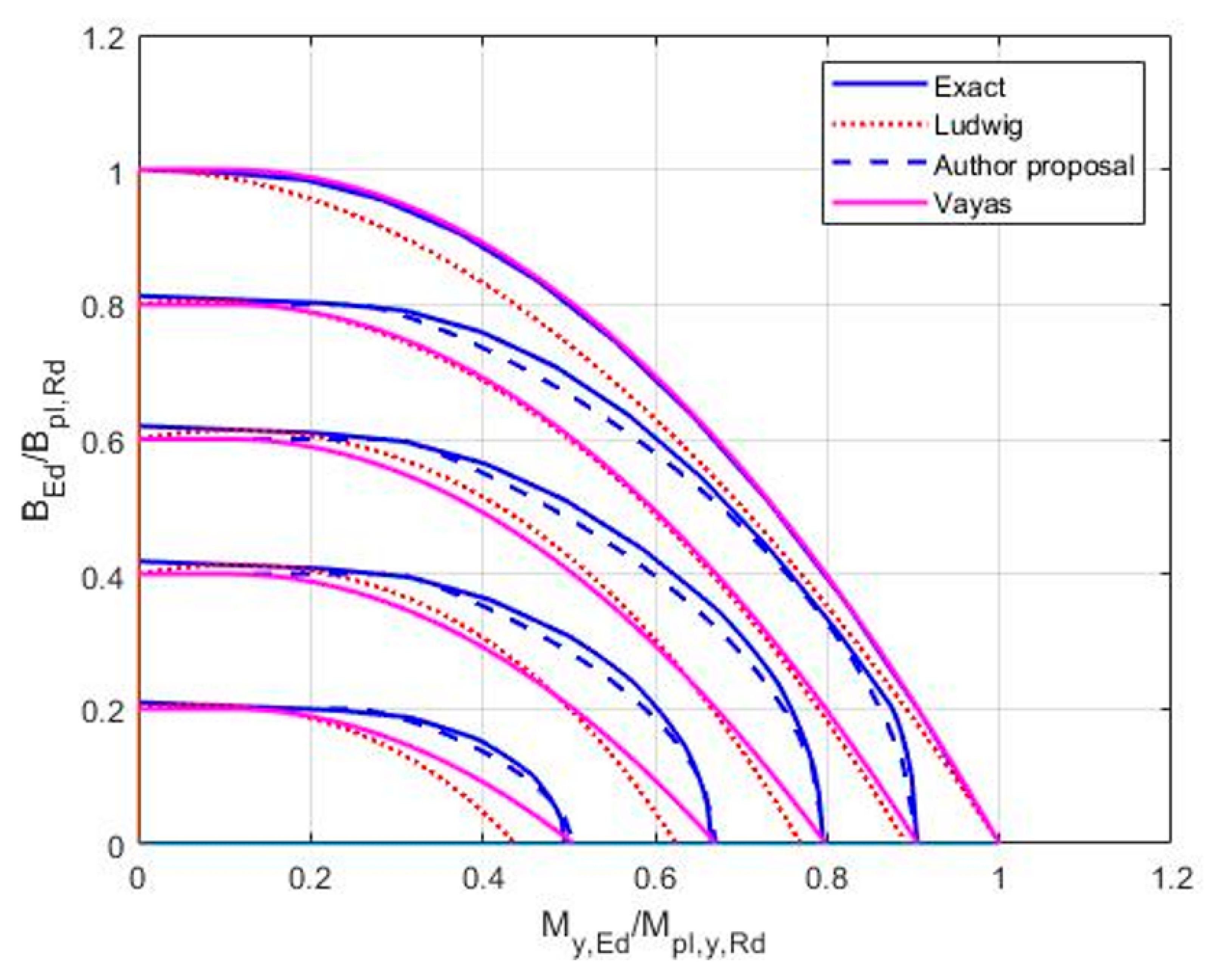
1.3. Calculation of Factor ξ

In the ξ factor by which internal forces must be multiplied to reach the plastic resistance, the lower-bound theorem can be used.

This lower-bound procedure was applied in two methods: (i) Method A divides the section into elements whose stresses are unknown; (ii) Method B divides the section into three parts (flanges and web). In each part, the unknowns are the axial force and the bending moment.

1.3.1. Method A (According to Osterrieder et al.)

Step 1. Dividing section into elements.

Step 2. Considering linear constraints by Equations (5)–(9):

Limitation of normal stresses:

$$-f_y\le {\sigma }_i\le f_y$$

(5)

Equilibrium equations:

$${\displaystyle \sum _{i=1}^n{\sigma }_i{A}_i}=\xi N_{Ed}$$

(6)

$${\displaystyle \sum _{i=1}^n-{\sigma }_i{A}_i}{y}_i=\xi M_{z,Ed}$$

(7)

$${\displaystyle \sum _{i=1}^n{\sigma }_i{A}_i}{\omega }_i={\displaystyle \sum _{i=1}^n{\sigma }_i{A}_i}{y}_i{z}_i=\xi B_{Ed}$$

(8)

$${\displaystyle \sum _{i=1}^n{\sigma }_i{A}_i}{z}_i=\xi M_{y,Ed}$$

(9)

Step 3. Calculation of maximum ξ:

$$\max \left(\xi \right)$$

(10)

The problem can be solved by linear programming.

1.3.2. Method B (According to Rubin and Kindmann et al.)

Method B is according to Rubin [13] and Kindmann et al. [23,24,25]), the computer programs in [15,16,25] give similar results.

The I-section consists of three parts: top flange–index Tfl, bottom flange–index Bfl and web–index w. In Method B, the following are calculated: factor ξ to accomplish plastic resistance, axial forces N {$N_{Tfl,Ed}$; $N_{Bfl,Ed}$; $N_{w,Ed}$}, and bending moments M in each part {$M_{Tfl,z,Ed}$; $M_{Bfl,z,Ed}$; $M_{w,y,Ed}$}.

Step 1. Dividing section into three parts.

Calculation of axial and bending resistances of all the parts:

$$\begin{array}{l}{N}_{fl,pl,Rd}=N_{Tfl,pl,Rd}=N_{Bfl,pl,Rd}=b\cdot t_f\cdot f_y;N_{wpl,Rd}=h_w\cdot t_w\cdot f_y\\ M_{fl,pl,z,Rd}=M_{Tfl,pl,z,Rd}=M_{Bfl,pl,z,Rd}=\frac{b^2·t_f·f_y}{4};M_{w,pl,y,Rd}=\frac{h_w^2·t_w·f_y}{4}\end{array}$$

(11)

Step 2. Considering constraints:

Plastic resistances of the top flange in the nonlinear-interaction formula:

$${\left(\frac{N_{Tfl,Ed}}{N_{Tfl,pl,Rd}}\right)}^2+\left|\frac{M_{Tfl,z,Ed}}{M_{Tfl,pl,z,Rd}}\right|\le 1$$

(12)

Plastic resistances of the bottom flange in the nonlinear-interaction formula:

$${\left(\frac{N_{Bfl,Ed}}{N_{Bfl,pl,Rd}}\right)}^2+\left|\frac{M_{Bfl,z,Ed}}{M_{Bfl,pl,z,Rd}}\right|\le 1$$

(13)

Plastic resistances of the web in the nonlinear-interaction formula:

$${\left(\frac{N_{w,Ed}}{N_{w,pl,Rd}}\right)}^2+\left|\frac{M_{w,y,Ed}}{M_{w,pl,y,Rd}}\right|\le 1$$

(14)

Equilibrium equations:

$$\begin{gathered} N_{Tfl,Ed}+N_{Bfl,Ed}+N_{w,Ed}=\xi N_{Ed} \\ {M}_{Tfl,z,Ed}+M_{Bfl,z,Ed}=\xi M_{zEd} \\ \frac{h-t_f}{2}\left(M_{Tfl,z,Ed}-M_{Bfl,z,Ed}\right)=\xi B_{Ed} \\ {M}_{w,y,Ed}+\frac{h-t_f}{2}\left(N_{Bfl,Ed}-N_{Tfl,Ed}\right)=\xi M_{yEd} \end{gathered}$$

(15)

Step 3. Calculation of maximum ξ:

$$\max \left(\xi \right)$$

(16)

1.4. Research Significance

The steps of Method A and Method B are described in detail. They make it possible to obtain the exact interaction formulae.

Three interaction formulae for the calculation of the plastic resistance of cross sections under the bending moment about the strong axis, M_y,Ed, the bending moment about the weak axis, M_z,Ed, and the bimoment, B_Ed, are compared for the beams with the I- and H-sections. The proposal is presented in Section 3.

2. Calculation of Exact Interaction Curves

Method A is applied to obtain the interaction curves with parameter M_z,Ed

Step 1. Calculation of M_pl,z,Rd for the given N_Ed = 0.0, M_y,Ed = 0.0 and B_Ed = 0.0.

Considering constraints by Equations (17)–(20):

$$-f_y\le {\sigma }_i\le f_y$$

(17)

$${\displaystyle \sum _{i=1}^n{\sigma }_i{A}_i}=N_{Ed}=0.0$$

(18)

$${\displaystyle \sum _{i=1}^n{\sigma }_i{A}_i}{z}_i=M_{y,Ed}=0.0$$

(19)

$${\displaystyle \sum _{i=1}^n{\sigma }_i{A}_i}{y}_i{z}_i=B_{Ed}=0.0$$

(20)

Calculation of maximum M_z,Ed:

$$M_{pl,z,Rd}=\max \left(M_{z,Ed}\right)=\max \left({\displaystyle \sum _{i=1}^n{\sigma }_i{A}_i}{y}_i\right)$$

(21)

Step 2. Calculation of B_Ed,γ to achieve plastic resistance for the given N_Ed = 0.0, M_y,Ed = 0.0, M_z,Ed = γ·M_pl,z,Rd; γ [0,1]

Constraints Equations (22)–(25):

$$-f_y\le {\sigma }_i\le f_y$$

(22)

$${\displaystyle \sum _{i=1}^n{\sigma }_i{A}_i}=N_{Ed}=0.0$$

(23)

$${\displaystyle \sum _{i=1}^n{\sigma }_i{A}_i}{z}_i=M_{y,Ed}=0.0$$

(24)

$${\displaystyle \sum _{i=1}^n{\sigma }_i{A}_i}{y}_i=M_{z,Ed}=\gamma ·M_{pl,z,Rd}$$

(25)

Calculation of maximum B_pl,Rd,γ:

$$B_{pl,Rd,\gamma }\left(M_{z,Ed}\right)=\max \left({\displaystyle \sum _{i=1}^n{\sigma }_i{A}_i}{y}_i{z}_i\right)$$

(26)

Step 3. Calculation of M_y,Ed,γ,η to achieve the plastic resistance for the given N_Ed = 0.0, B_Ed = ηB_pl,Rd,γ, M_z,Ed = γ·M_pl,z,Rd; γ [0,1] and η [0,1].

Considering constraints by Equations (27)–(30):

$$-f_y\le {\sigma }_i\le f_y$$

(27)

$${\displaystyle \sum _{i=1}^n{\sigma }_i{A}_i}=N_{Ed}=0.0$$

(28)

$${\displaystyle \sum _{i=1}^n{\sigma }_i{A}_i}{y}_i=M_{z,Ed}=\gamma M_{pl,z,Rd}$$

(29)

$${\displaystyle \sum _{i=1}^n{\sigma }_i{A}_i}{y}_i{z}_i=B_{Ed}=\eta B_{pl,Rd,\gamma }$$

(30)

Calculation of maximum M_yEdγ,η:

$$M_{y,Ed,\gamma ,\eta }\left(B_{Ed};M_{z,Ed}\right)=\max \left({\displaystyle \sum _{i=1}^n{\sigma }_i{A}_i}{z}_i\right)$$

(31)

Application of Method B:

Dividing the section into three parts (top flange, bottom flange, and web):

The axial force that leads to the plastic behavior of the top flange:

$$N_{Tfl,Ed}=N_{fl,pl,Rd}\sqrt{1-\left|\frac{M_{z,Ed}}{2M_{fl,pl,z,Rd}}+\frac{B_{Ed}}{\left(h-t_f\right)M_{fl,pl,z,Rd}}\right|}$$

(32)

The axial force that leads to the plastic behavior of the bottom flange:

$$N_{Bfl,Ed}=N_{fl,pl,Rd}\sqrt{1-\left|\frac{M_{z,Ed}}{2M_{fl,pl,z,Rd}}-\frac{B_{Ed}}{\left(h-t_f\right)M_{fl,pl,z,Rd}}\right|}$$

(33)

If the axial force equals zero (N_Ed = 0), the axial force at the web will be as follows:

$$N_{w,Ed}=N_{Tfl,Ed}-N_{Bfl,Ed}$$

(34)

The bending moment that leads to the plastic behavior at the web:

$$M_{w,y,Ed}=M_{w,pl,y,Rd}\left(1-{\left(\frac{N_{w,Ed}}{N_{w,pl,Rd}}\right)}^2\right)$$

(35)

The resultant bending moment M_y,Ed is then:

$$M_{y,Ed}=M_{w,y,Ed}+\left(N_{Tfl,Ed}+N_{Bfl,Ed}\right)\frac{\left(h-t_f\right)}{2}$$

(36)

3. Proposal for Improved Interaction Formulae (Variant No. 4)

The original formulae of variants Nos. 1, 2, and 3, presented in Table 1 [1], are improved by variant No. 4. In variant No. 4, the definitions of the parameters α, β, and δ are different. The improved formulae of variant No. 4 with the changed parameters (Equations in (37)) provide results that agree well with the exact results obtained by linear programming (Figure 1 and Figure 2).

Table 1. Improved interaction formulae with changed parameters α, β, and δ (variant No. 4).

$0\le \frac{M_{y,Ed}}{M_{pl,y,Rd}}<\delta$	$\delta \le \frac{M_{y,Ed}}{M_{pl,y,Rd}}\le 1.0$
$\frac{\frac{B_{Ed}}{B_{pl,Rd}}}{1-\frac{M_{z,Ed}}{M_{pl,z,Rd}}}\le 1.0$	${\left(\frac{\frac{M_{y,Ed}}{M_{pl,y,Rd}}-\delta }{\left(\sqrt{1-\frac{M_{z,Ed}}{M_{pl,z,Rd}}}\left(1-\frac{M_{w,pl,y,Rd}}{M_{pl,y,Rd}}\right)+\frac{M_{w,pl,y,Rd}}{M_{pl,y,Rd}}\right)-\delta }\right)}^{\alpha }+{\left(\frac{\frac{B_{Ed}}{B_{pl,Rd}}}{1-\frac{M_{z,Ed}}{M_{pl,z,Rd}}}\right)}^{\beta }\le 1$

Table 1. Improved interaction formulae with changed parameters α, β, and δ (variant No. 4).

$0\le \frac{M_{y,Ed}}{M_{pl,y,Rd}}<\delta$	$\delta \le \frac{M_{y,Ed}}{M_{pl,y,Rd}}\le 1.0$
$\frac{\frac{B_{Ed}}{B_{pl,Rd}}}{1-\frac{M_{z,Ed}}{M_{pl,z,Rd}}}\le 1.0$	${\left(\frac{\frac{M_{y,Ed}}{M_{pl,y,Rd}}-\delta }{\left(\sqrt{1-\frac{M_{z,Ed}}{M_{pl,z,Rd}}}\left(1-\frac{M_{w,pl,y,Rd}}{M_{pl,y,Rd}}\right)+\frac{M_{w,pl,y,Rd}}{M_{pl,y,Rd}}\right)-\delta }\right)}^{\alpha }+{\left(\frac{\frac{B_{Ed}}{B_{pl,Rd}}}{1-\frac{M_{z,Ed}}{M_{pl,z,Rd}}}\right)}^{\beta }\le 1$

where

$$\begin{array}{l}\left\{\begin{array}{c}\alpha \\ \beta \\ \delta \end{array}\right\}=\left\{\begin{array}{c}2\\ 1\\ \frac{M_{w,pl,y,Rd}}{M_{pl,y,Rd}}\end{array}\right\}+\left\{\begin{array}{c}\alpha *-2\\ \beta *-1\\ \delta *-\frac{M_{w,pl,y,Rd}}{M_{pl,y,Rd}}\end{array}\right\}\cos \left({\left|\frac{\frac{M_{z,Ed}}{M_{pl,z,Rd}}-0.5}{0.5}\right|}^{1.5}\frac{\pi }{2}\right)\\ \left\{\begin{array}{c}\alpha *\\ \beta *\end{array}\right\}=\left\{\begin{array}{c}1.1\\ 2\end{array}\right\}+\left\{\begin{array}{c}1\\ 1\end{array}\right\}4\left(\frac{M_{w,pl,y,Rd}}{M_{pl,y,Rd}}-0.1\right){\left(\cos \left(\left|\frac{\frac{M_{z,Ed}}{M_{pl,z,Rd}}-0.5}{0.5}\right|\frac{\pi }{2}\right)\right)}^4\\ \delta *=0.2+\frac{M_{w,pl,y,Rd}}{M_{pl,y,Rd}}\end{array}$$

(37)

4. Conclusions

The plastic resistance of the I- and H-sections under a combination of bending moments, M_y,Ed, M_z,Ed, and bimoment B_Ed, was investigated. The paper suggested an improvement to a previous proposal, published by the authors in [5], in which the three variants, No.1, No.2, and No.3, of approximate-interaction formulae were investigated. The proposed formulae may be called variant No.4. It was shown in graphical form that the proposed variant No.4 of the approximate Formula (37) gives almost identical values of the relative plastic resistances of I-shaped sections to the exact results obtained by linear programming (Figure 1 and Figure 2).

The comparisons of the results of the new approximate formulae with the results obtained by Vayas [6] and Ludwig [7] show that they agree better with the exact theoretical results, which were presented in the form of diagrams (Figure 1 and Figure 2).

The proposed Formulae (37) are rather complex and long. Therefore, they can hardly be accepted directly for the new generation of the metal Eurocode prEN 1993-1-1 design of steel structures [2] or the prEN 1999-1-1 design of aluminum structures [4]. However, they may be given in the CEN Technical Specification, and they may be part of the national Non Contradictory Complementary Information. The reason for this is that the approximate formulae and exact results of the submitted theory have useful practical applications. They enable the safe and economical design of steel and aluminum thin-walled structures. The exploitation of the plastic-section resistance reserves helps to save the structural material.