Effect of Fuel Sloshing on the Damping of a Scaled Wing Model—Experimental Testing and Numerical Simulations
Abstract
:1. Introduction
2. Methodology
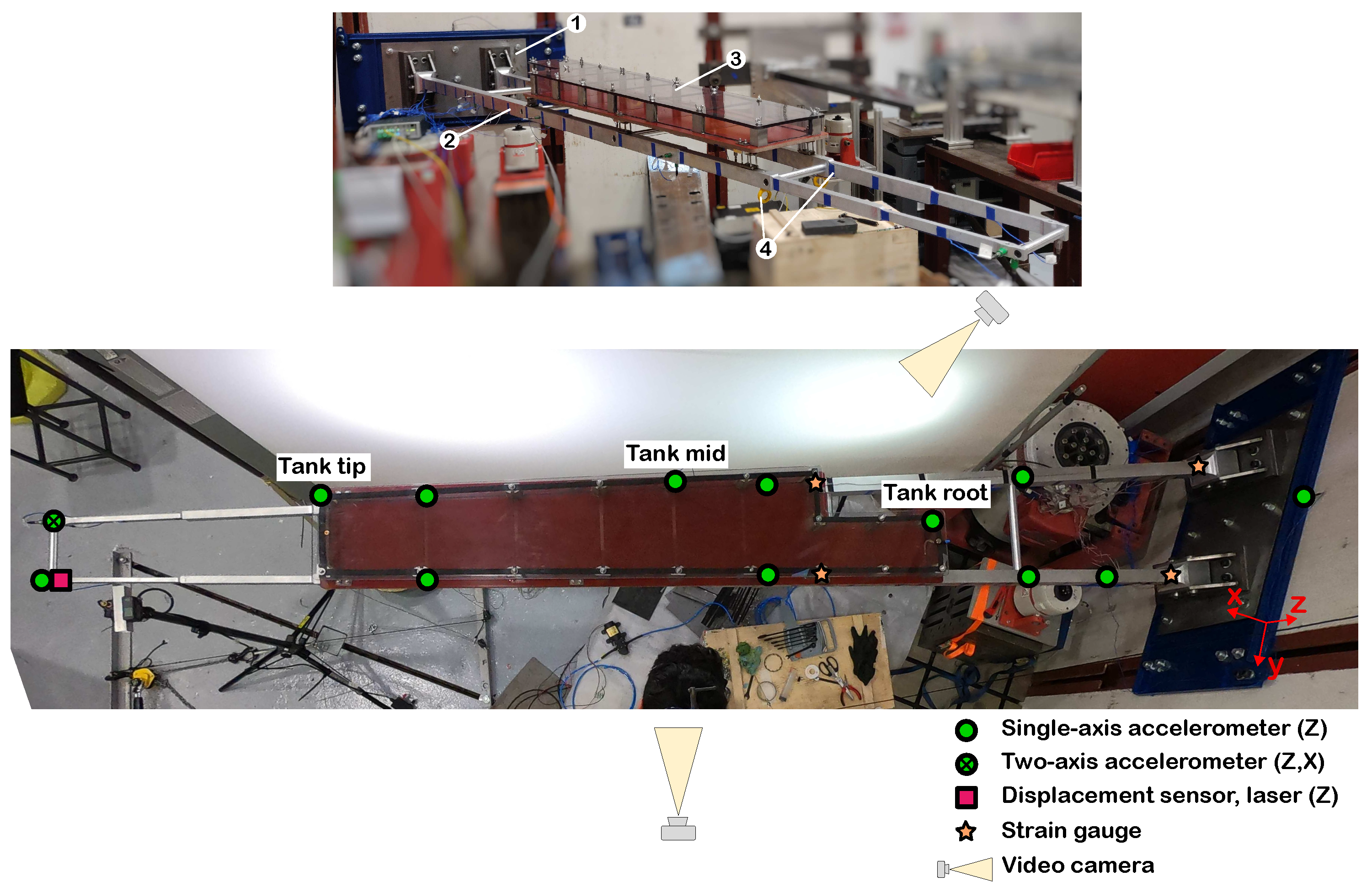
2.1. Experimental Methodology
- Ground vibration tests with and without tank attached, part of which was presented in [13],
- Step release tests without liquid (dry tests), with and without additional mass to account for the “frozen” liquid,
- Step release tests with tap water as the working liquid, with and without added dye
- Studies with and without the baffles, with the baffles of different solidity ratios,
- Studies with varying filling levels.
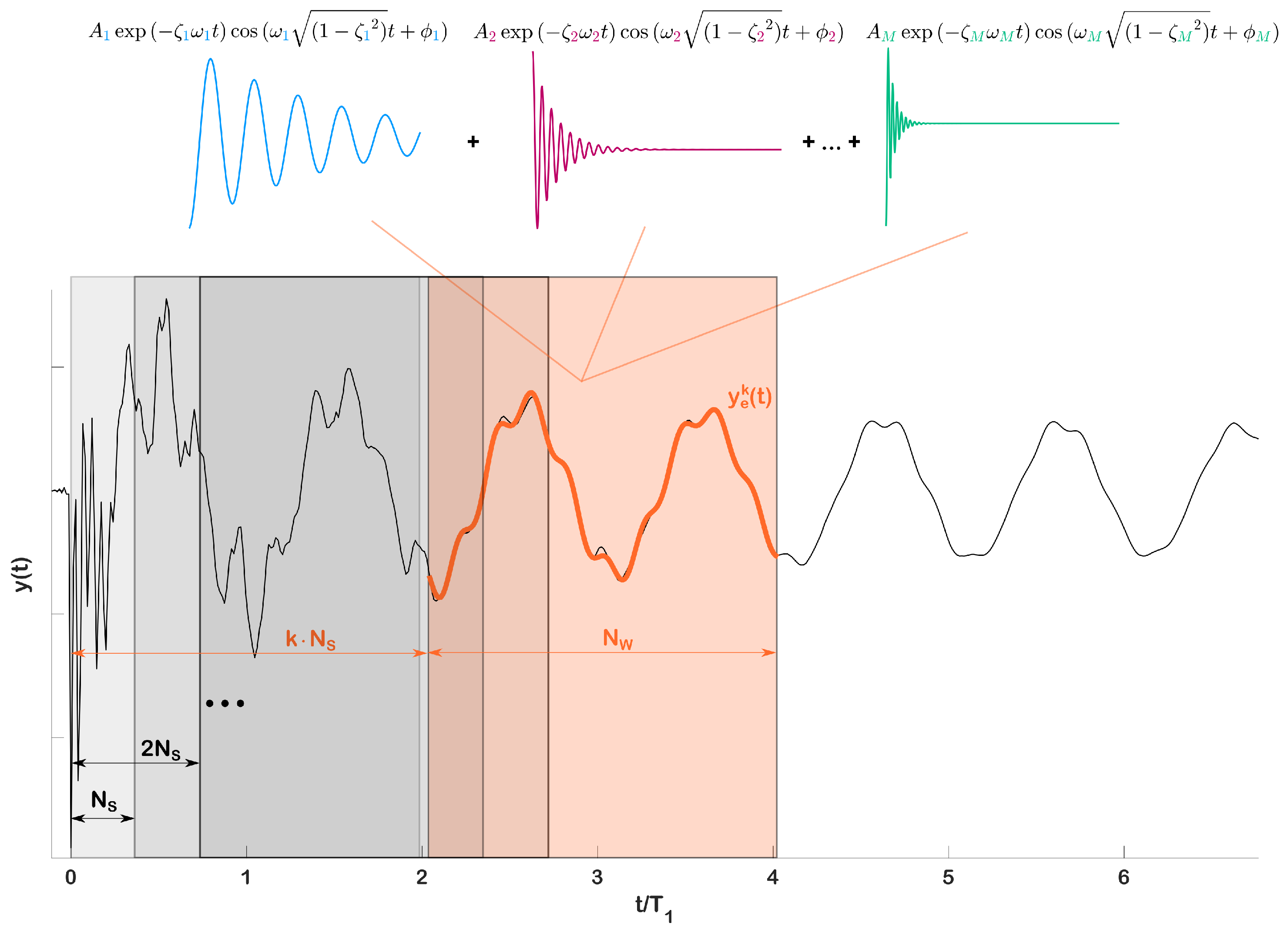
2.1.1. Nonlinear System Identification
- The amplitude of each component is limited at .
- The frequency of each component is limited in the interval , where is the linear estimate of the frequency component and x is the frequency deviation allowed for.
- If the damping ratio of one component exceeds a threshold value (set here at 0.9, or of critical damping), the respective component is eliminated from the signal estimate for all subsequent bins.
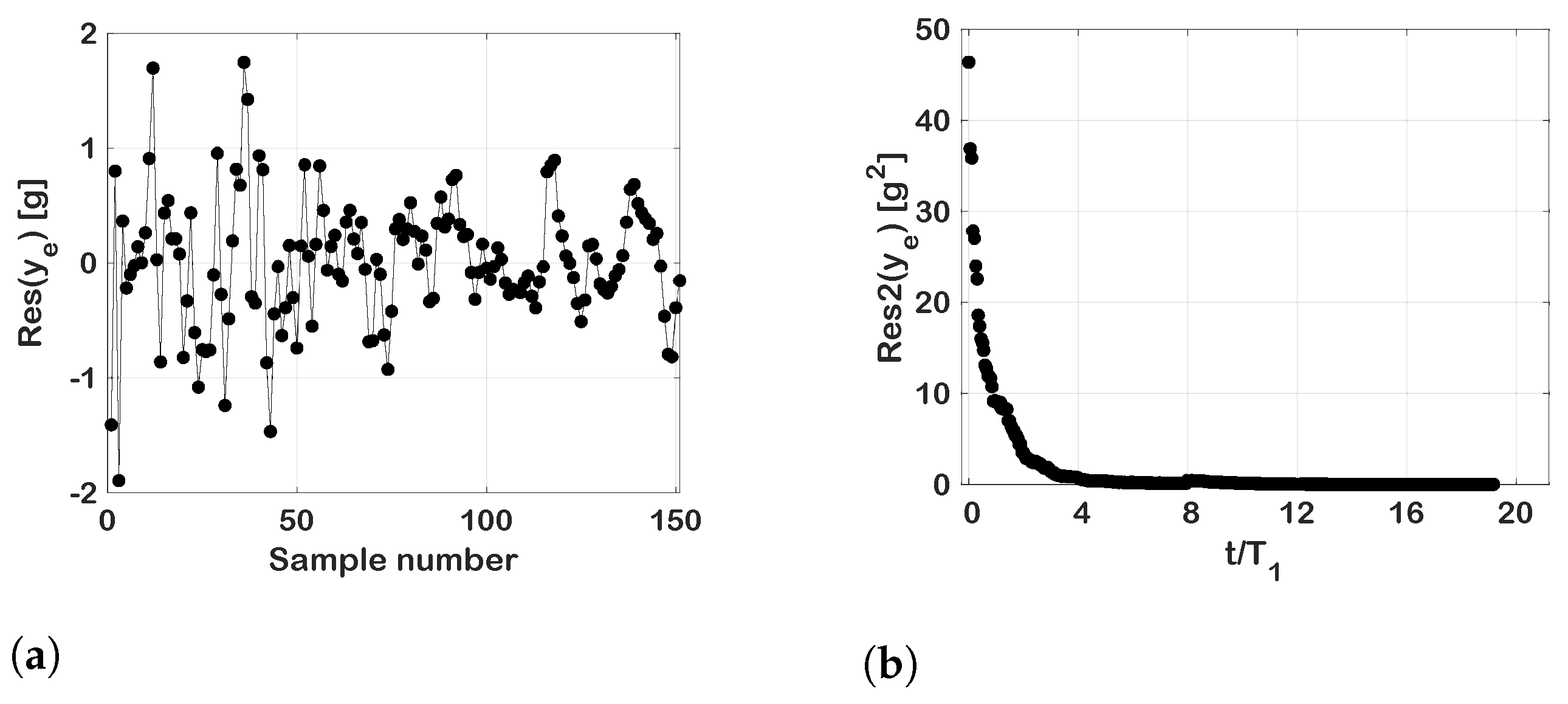
2.1.2. Example of Miniwot Data Curve Fitting
2.2. Numerical Methodology
2.2.1. Structural Model
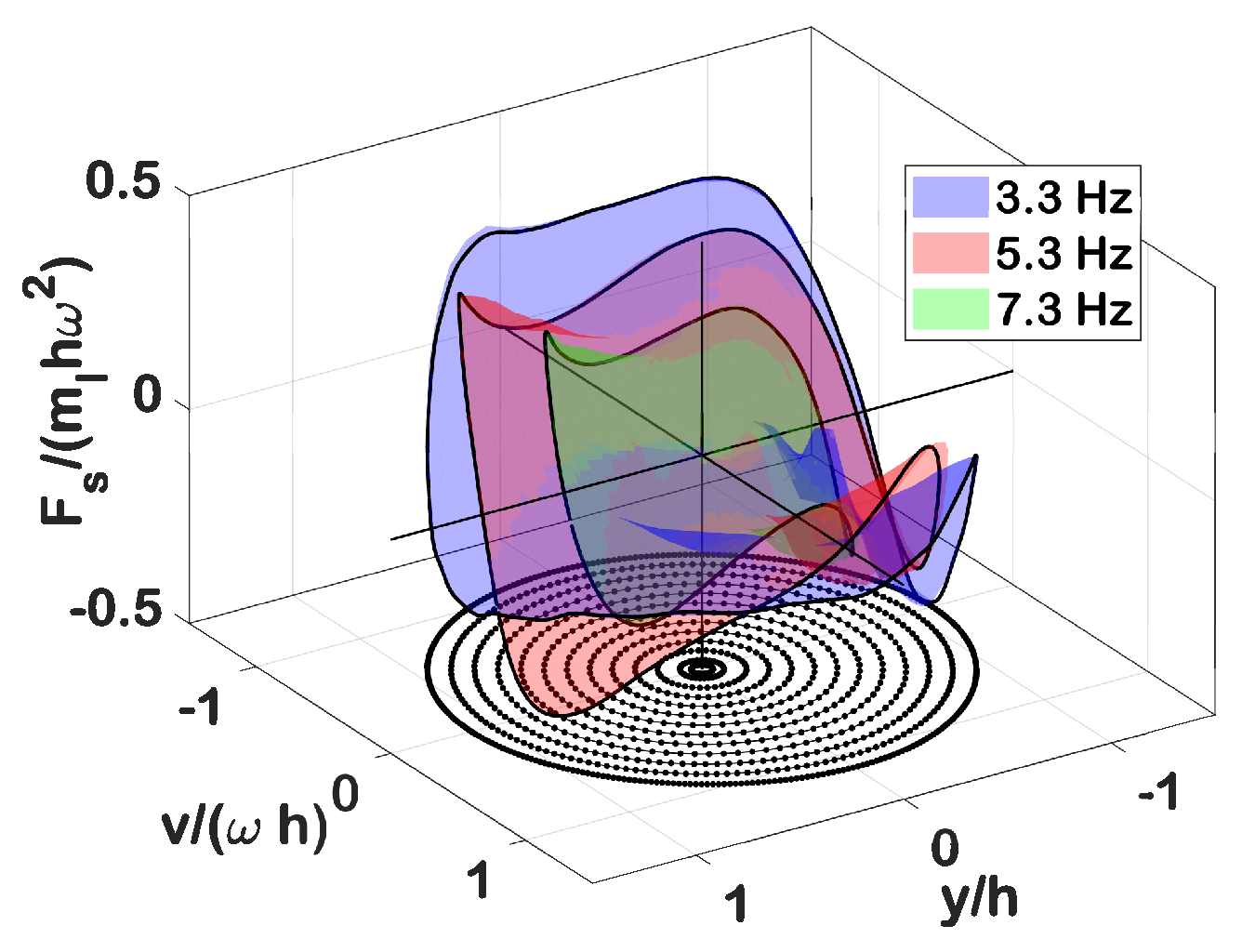
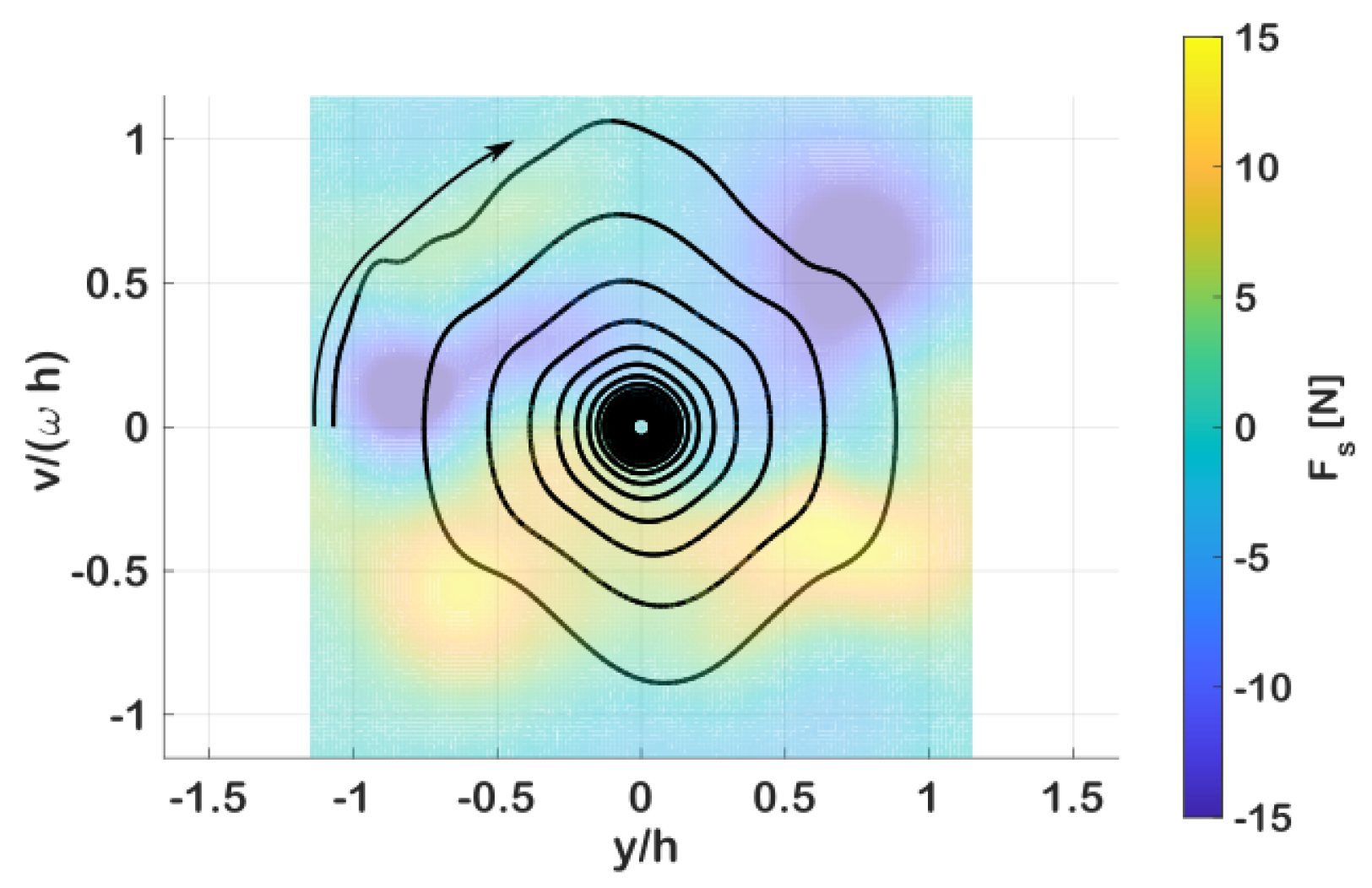
2.2.2. Quasi-Steady Model of the Transversal Sloshing Force
- Scaling between the experimental and numerical (MiniWoT) tank dimensions.
- Producing a continuous and smooth sloshing force for numerical analysis, which can not be directly extracted from the experimental data. A result of the finite sampling frequency producing discrete data points in each cycle, plus discrete amplitudes and frequencies of the harmonic cycles considered.
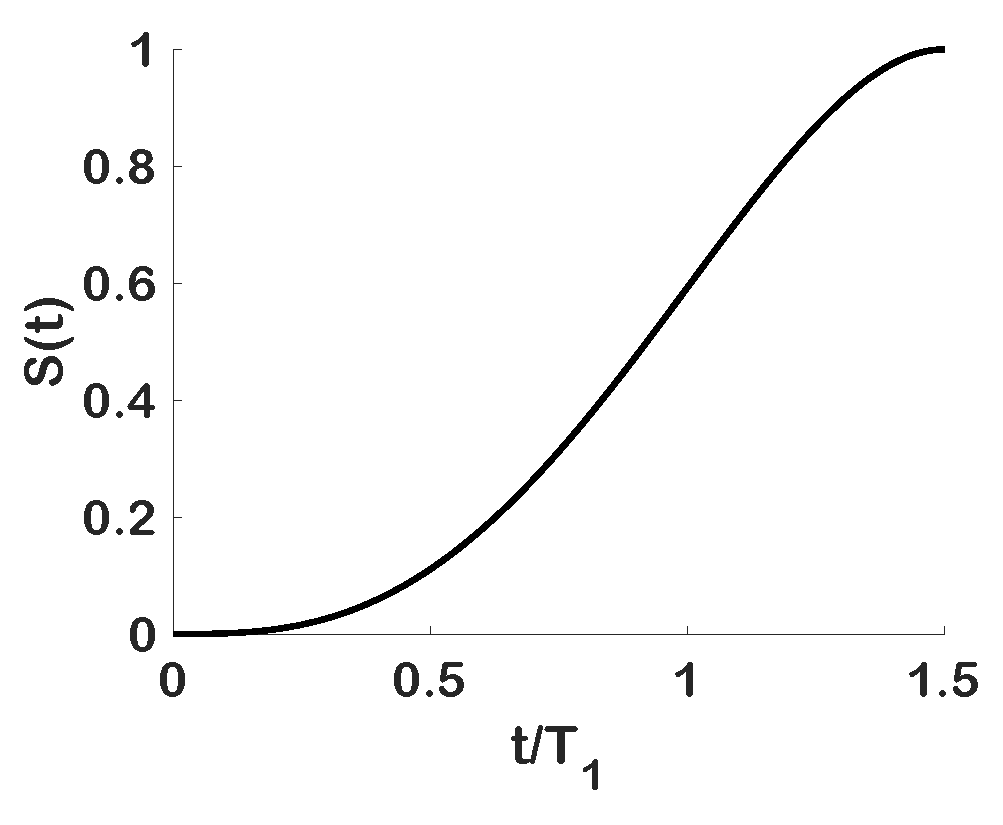
- Identified forces assume the fluid has reached a quasi-steady state under the excitation and, therefore, can not account for sharp transients (i.e., free-release of the structure). A mechanism must be introduced to account for the initial energy input into the fluid to excite the identified motions within the data-set.
3. Results
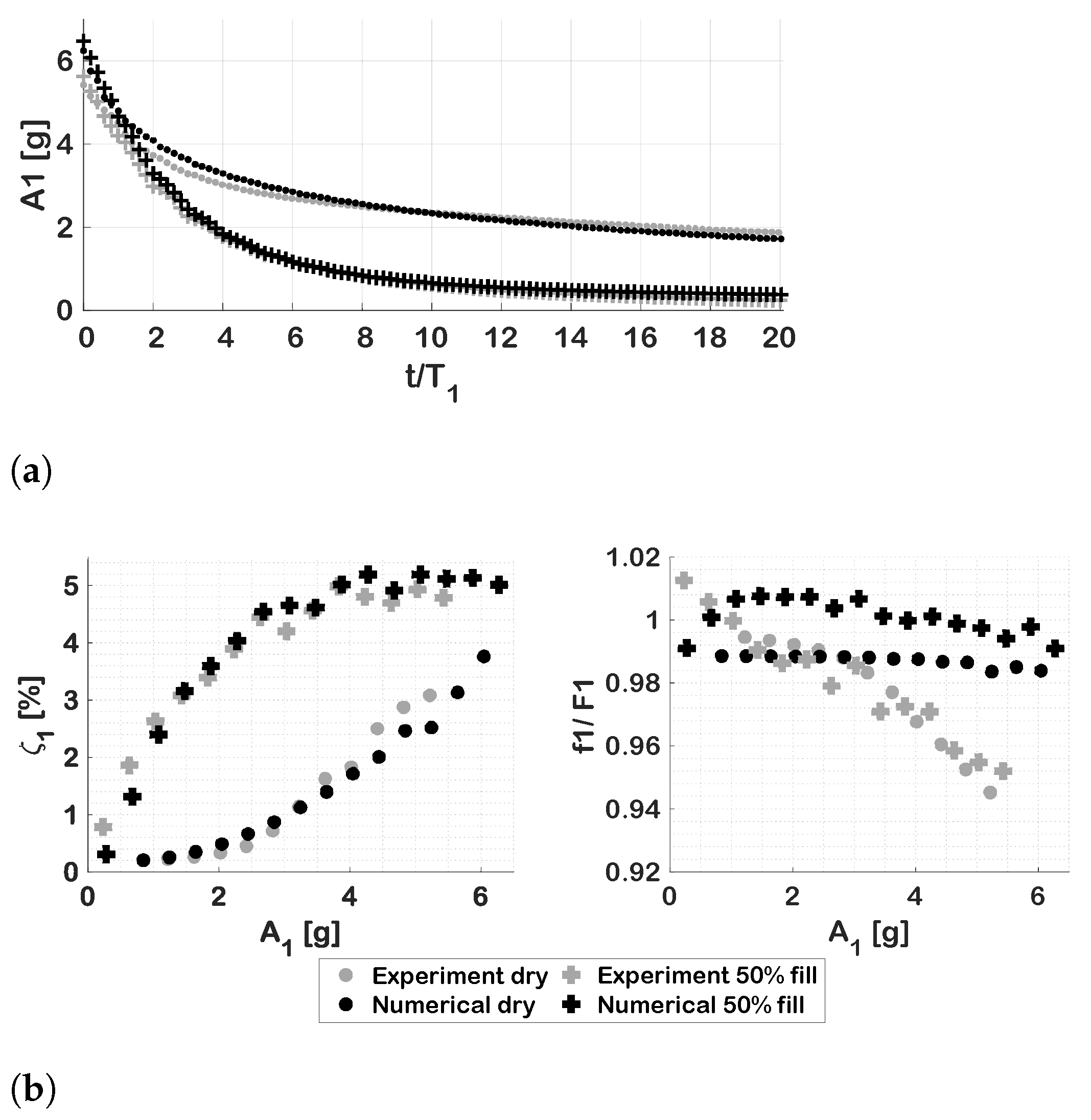
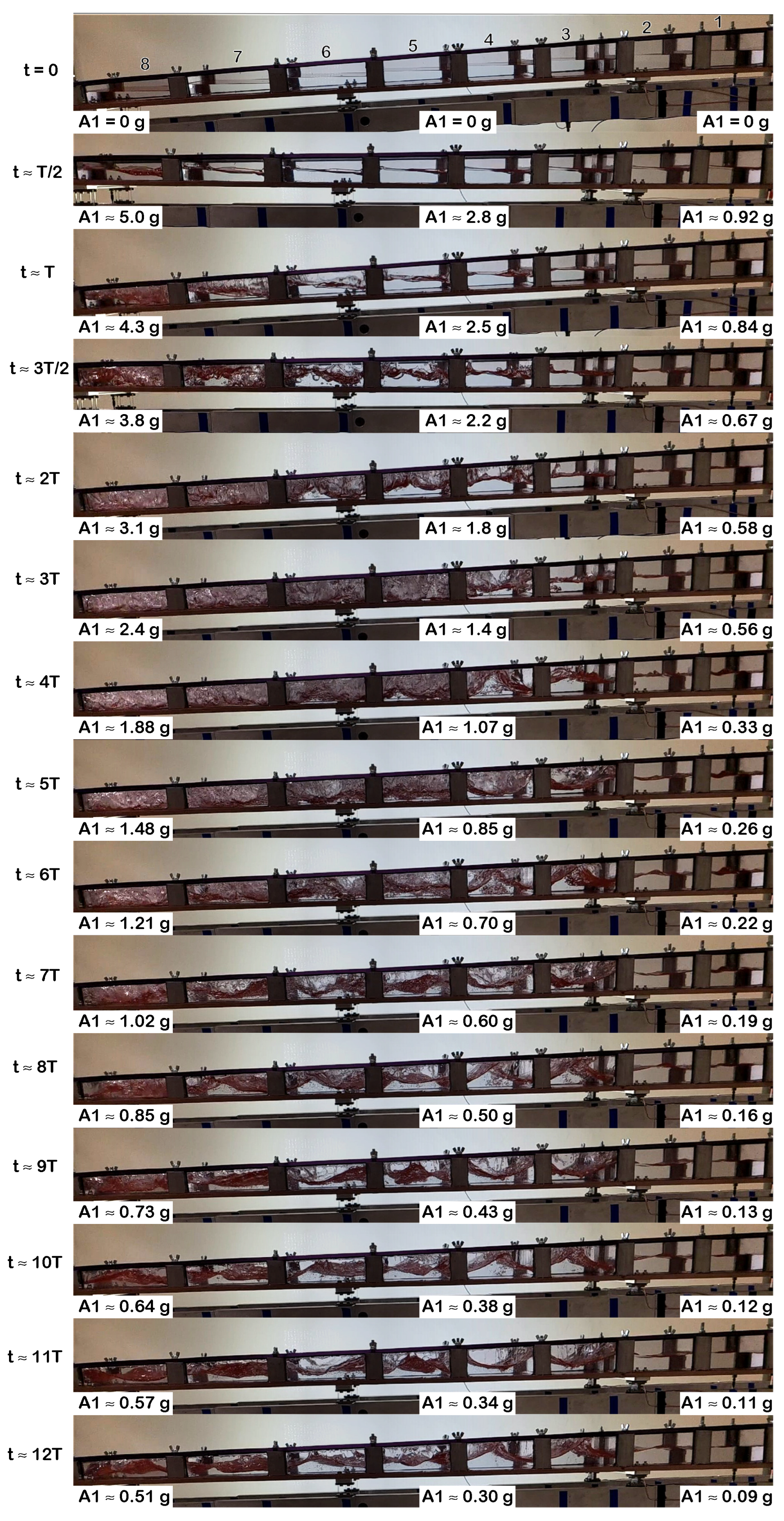
3.1. Large Amplitude Vibrations of the Dry System
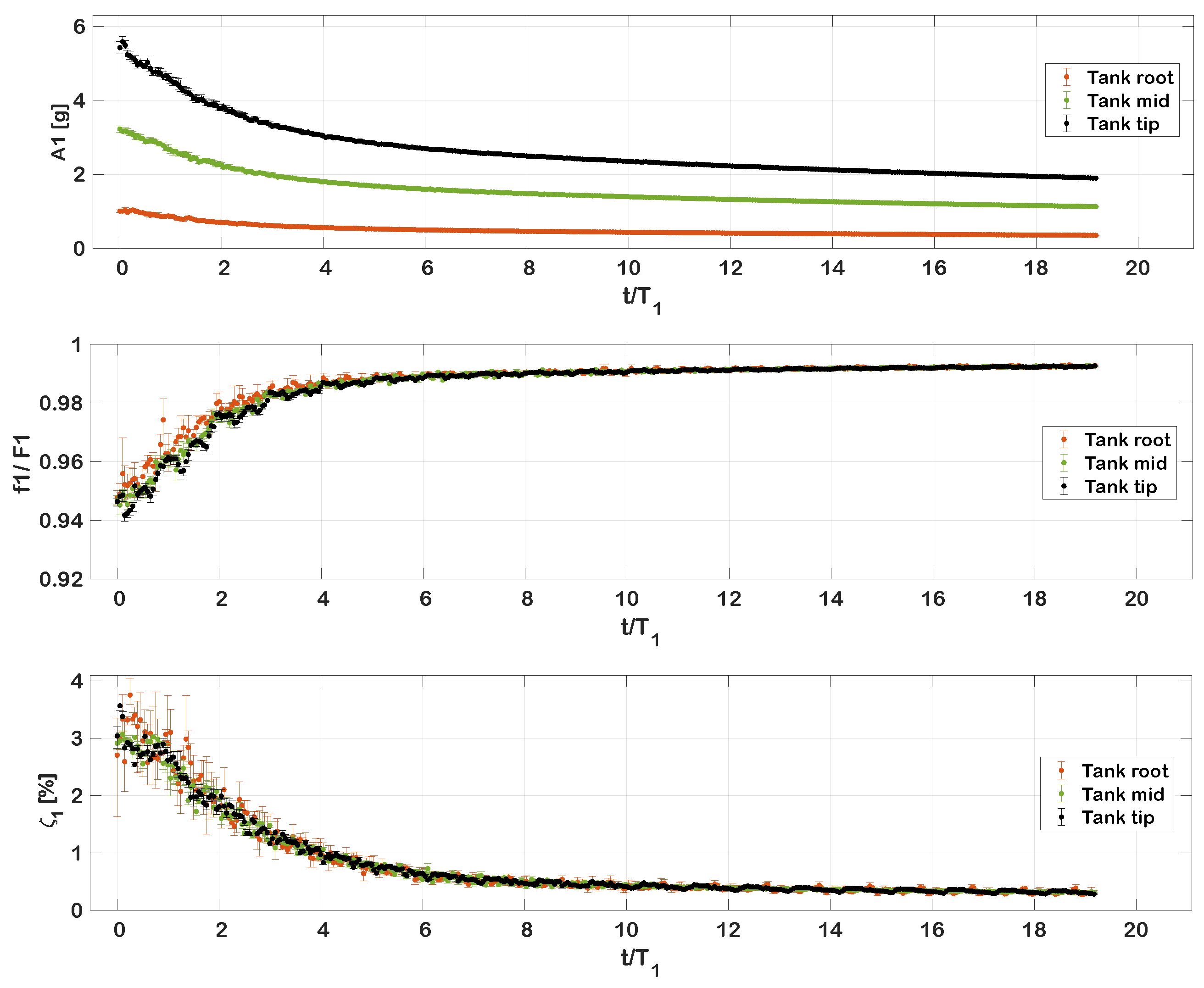
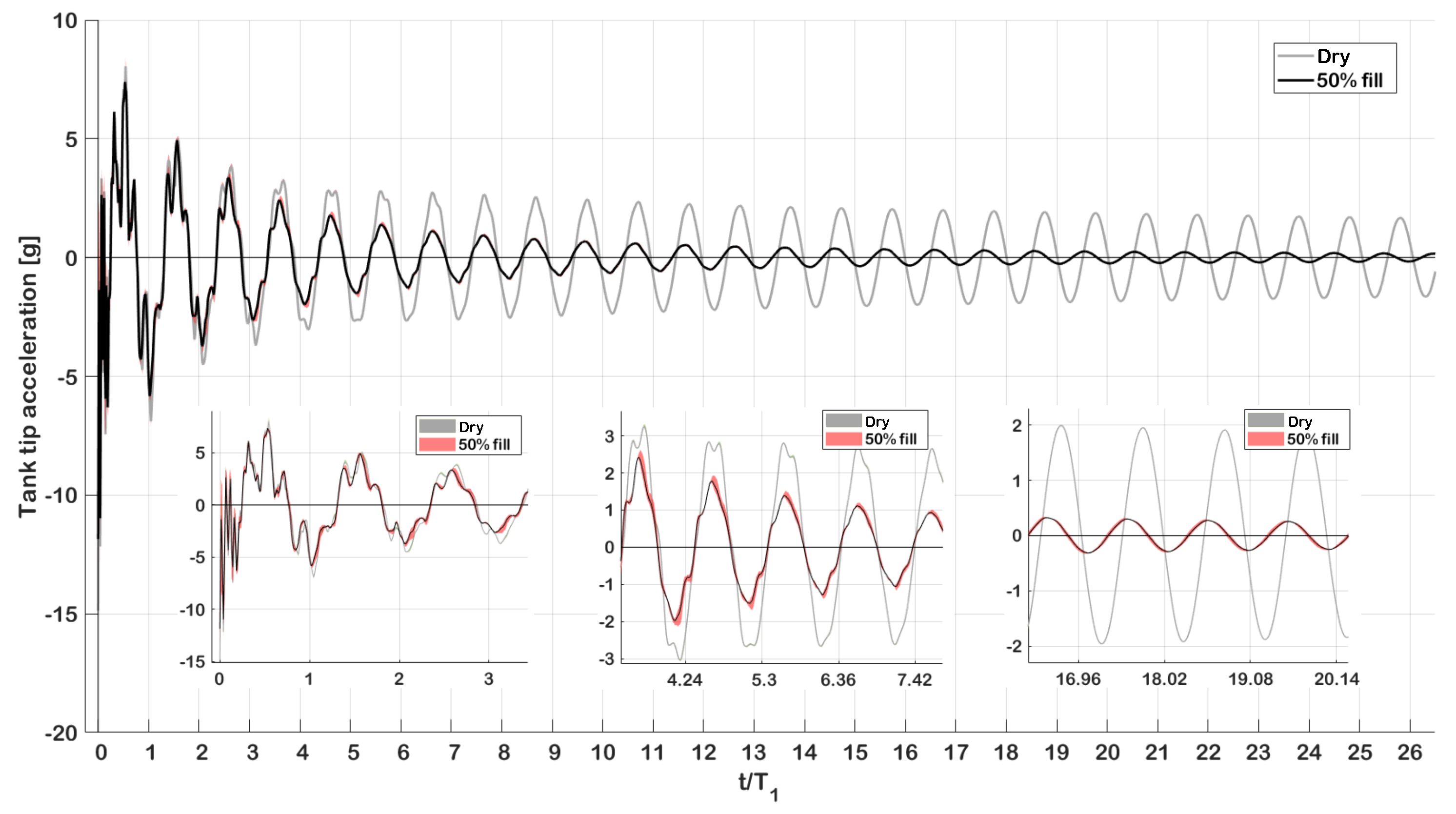
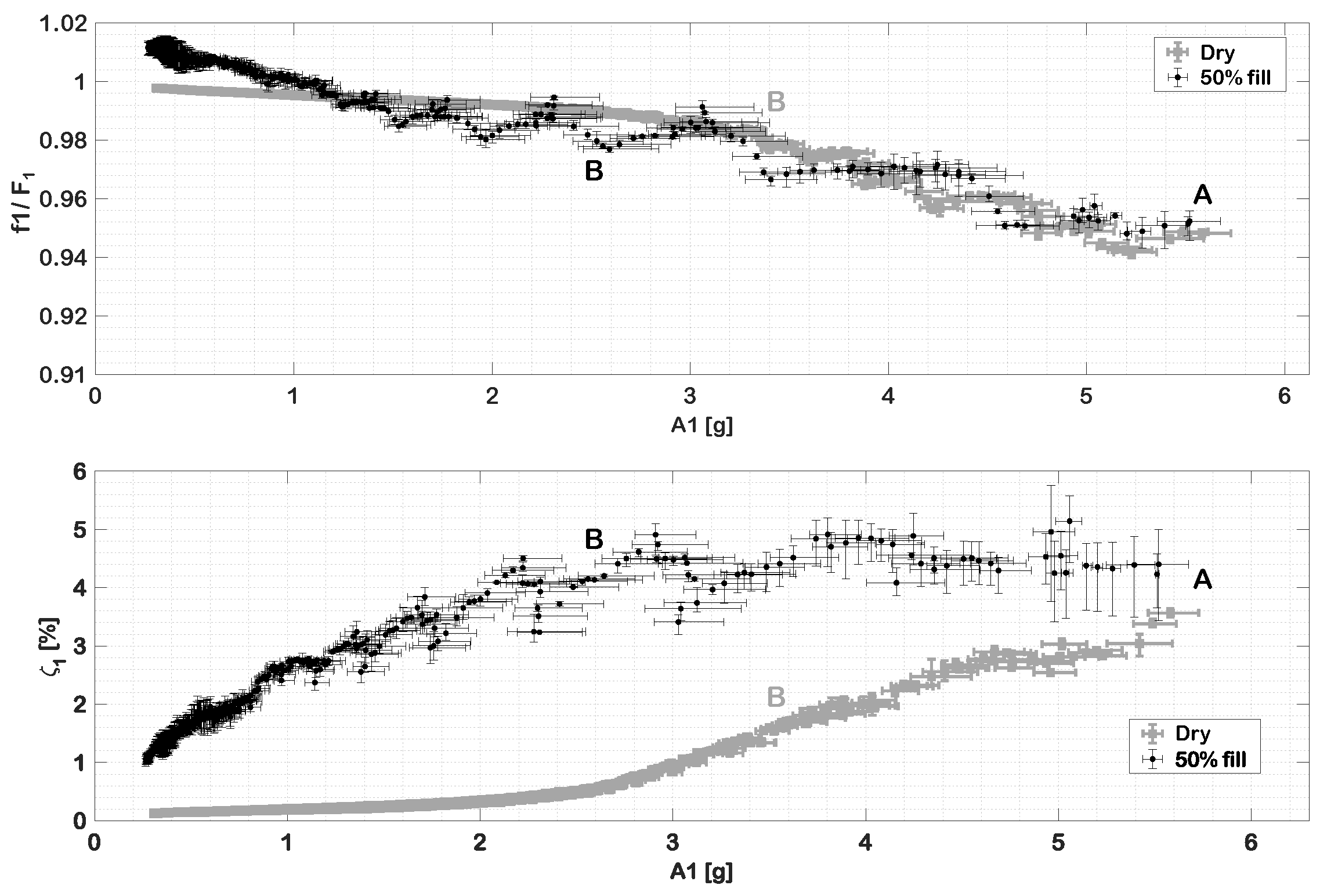
3.2. Large Amplitude Vibrations of the Wet System
3.3. Numerical Results
4. Discussion
- The spanwise location of the fuel influences the vertical sloshing regimes. Previous single degree-of-freedom studies indicate substantial sloshing-induced damping effects that depend on the sloshing regime [5,7]. There is thus an indication that depending on the available amounts of fuel, the net damping following the excitation similar to the step release (such as a discrete gust) may be increased by moving the liquid to the specific spanwise positions, taking into account the filling level as well. Supplementary investigations are planned in order to further assess such effects.
- Liquid sloshing patterns dominated by different sloshing modes are prominent at different spanwise locations and persistent even at the lowest amplitudes of excitation. Such sloshing modes are highly dependent on the geometry of the tank and filling level, and they have previously been observed to add substantial additional damping at lower amplitudes by promoting liquid impacts with the top of the tank. Therefore, there exists the possibility that the geometry of the fuel tank could be tailored to promote such sloshing patterns and, hence, achieve an increased sloshing-induced damping.
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| f | Frequency of the nonlinear signal component |
| y | Original signal, Displacement |
| Fitted function | |
| Fitted function in time bin k | |
| A | Amplitude of the nonlinear signal component |
| F | Natural frequency of vibration |
| M | Number of frequency components in the NLS analysis |
| Bin sliding size in the NLS analysis | |
| Time bin size in the NLS analysis | |
| T | Natural period of vibration |
| Damping ratio of the nonlinear signal component | |
| Phase of the nonlinear signal component, compact basis function | |
| Vector of modal displacements | |
| Loading force | |
| Sloshing force | |
| Total force | |
| , | Liquid and structural mass |
| Non-dimensional quantity | |
| Vector | |
| Modal stiffness matrix | |
| Modal damping matrix | |
| Characteristic frequency | |
| v | Velocity |
| h | Height of fuel tank |
| , | Nonlinear damping parameters |
References
- EASA. Certification Specifications and Acceptable Means of Compliance for Large Aeroplanes (CS-25) Amendment 27; Technical Report AMC 25.341 Gust and Continuous Turbulence Design Criteria, Section 6—Aeroplane Modelling Considerations; European Union Safety Aviation Agency (EASA): Cologne, Germany, 24 November 2021.
- Stodieck, O.; Cooper, J.E.; Weaver, P.M.; Kealy, P. Aeroelastic Tailoring of a Representative Wing Box Using Tow-Steered Composites. AIAA J. 2017, 55, 1425–1439. [Google Scholar] [CrossRef] [Green Version]
- Castrichini, A.; Siddaramaiah, V.H.; Calderon, D.; Cooper, J.; Wilson, T.; Lemmens, Y. Preliminary investigation of use of flexible folding wing tips for static and dynamic load alleviation. Aeronaut. J. 2017, 121, 73–94. [Google Scholar] [CrossRef] [Green Version]
- Constantin, L.; De Courcy, J.J.; Titurus, B.; Rendall, T.; Cooper, J.E.; Gambioli, F. Initial Experimental Analysis of Damping due to Liquid Sloshing in a Scaled Aircraft Wing Model. In Proceedings of the International Forum on Aeroelasticity and Structural Dynamics IFASD 2022, Madrid, Spain, 13–17 June 2022. [Google Scholar]
- Constantin, L.; De Courcy, J.; Titurus, B.; Rendall, T.; Cooper, J. Analysis of damping from vertical sloshing in a SDOF system. Mech. Syst. Signal Process. 2021, 152, 107452. [Google Scholar] [CrossRef]
- Martinez-Carrascal, J.; González-Gutiérrez, L. Experimental study of the liquid damping effects on a SDOF vertical sloshing tank. J. Fluids Struct. 2021, 100, 103172. [Google Scholar] [CrossRef]
- Constantin, L.; De Courcy, J.; Titurus, B.; Rendall, T.; Cooper, J. Sloshing induced damping across Froude numbers in a harmonically vertically excited system. J. Sound Vib. 2021, 510, 116302. [Google Scholar] [CrossRef]
- Saltari, F.; Pizzoli, M.; Coppotelli, G.; Gambioli, F.; Cooper, J.E.; Mastroddi, F. Experimental characterisation of sloshing tank dissipative behaviour in vertical harmonic excitation. J. Fluids Struct. 2022, 109, 103478. [Google Scholar] [CrossRef]
- Calderon-Sanchez, J.; Martinez-Carrascal, J.; Gonzalez-Gutierrez, L.M.; Colagrossi, A. A global analysis of a coupled violent vertical sloshing problem using an SPH methodology. Eng. Appl. Comput. Fluid Mech. 2021, 15, 865–888. [Google Scholar] [CrossRef]
- Wright, M.D.; Gambioli, F.; Malan, A.G. CFD Based Non-Dimensional Characterization of Energy Dissipation Due to Verticle Slosh. Appl. Sci. 2021, 11, 10401. [Google Scholar] [CrossRef]
- Saltari, F.; Pizzoli, M.; Gambioli, F.; Jetzschmann, C.; Mastroddi, F. Sloshing reduced-order model based on neural networks for aeroelastic analyses. Aerosp. Sci. Technol. 2022, 127, 107708. [Google Scholar] [CrossRef]
- Titurus, B.; Cooper, J.E.; Saltari, F.; Mastroddi, F.; Gambioli, F. Analysis of a Sloshing Beam Experiment. In Proceedings of the International Forum on Aeroelasticity and Structural Dynamics, Savannah, GA, USA, 9–13 June 2019. [Google Scholar]
- Constantin, L.; De Courcy, J.J.; Titurus, B.; Rendall, T.; Cooper, J.E. Design and GVT of a dynamically scaled wing structure for fuel sloshing investigations. In Proceedings of the AIAA SCITECH 2022 Forum, San Diego, CA, USA, 3–7 January 2022. [Google Scholar] [CrossRef]
- Billings, S.A. Nonlinear System Identification: NARMAX Methods in the Time, Frequency, and Spatio-Temporal Domains; John Wiley & Sons: Hoboken, NJ, USA, 2013. [Google Scholar]
- Billings, S.A.; Voon, W.S.F. Piecewise linear identification of non-linear systems. Int. J. Control 1987, 46, 215–235. [Google Scholar] [CrossRef]
- MSC Software. NASA STRuctrual ANalysis (NASTRAN) 2018. Available online: https://www.mscsoftware.com/product/msc-nastran (accessed on 1 August 2022).
- MSC Software. MSC Patran 2018. Available online: https://www.mscsoftware.com/product/patran (accessed on 1 August 2022).
- Cveticanin, L. Oscillators with nonlinear elastic and damping forces. Comput. Math. Appl. 2011, 62, 1745–1757. [Google Scholar] [CrossRef] [Green Version]
- Cveticanin, L.; Kalamiyazdi, M.; Askari, H.; Saadatnia, Z. Vibration of a two-mass system with non-integer order nonlinear connection. Mech. Res. Commun. 2012, 43, 22–28. [Google Scholar] [CrossRef]
- Shampine, L.F.; Reichelt, M.W. The matlab ode suite. SIAM J. Sci. Comput. 1997, 18, 1–22. [Google Scholar] [CrossRef] [Green Version]
- Dodge, F. The New “Dynamic Behavior of Liquids in Moving Containers”; Technical Report; Southwest Research Institute: San Antonio, TX, USA, 2000. [Google Scholar]
- Farid, M.; Gendelman, O. Response regimes in equivalent mechanical model of moderately nonlinear liquid sloshing. Nonlinear Dyn. 2018, 92, 1517–1538. [Google Scholar] [CrossRef] [Green Version]
- Pizzoli, M.; Saltari, F.; Mastroddi, F.; Martinez-Carrascal, J.; González-Gutiérrez, L.M. Nonlinear reduced-order model for vertical sloshing by employing neural networks. Nonlinear Dyn. 2022, 107, 1469–1478. [Google Scholar] [CrossRef]
- Pizzoli, M.; Saltari, F.; Coppotelli, G.; Mastroddi, F. Experimental Validation of Neural-Network-Based Nonlinear Reduced-Order Model for Vertical Sloshing. In Proceedings of the AIAA Scitech 2022 Forum, San Diego, CA, USA, 3–7 January 2022; p. 1186. [Google Scholar]
- Wendland, H. Scattered Data Approximation; Cambridge University Press: Cambridge, UK, 2004; Volume 17. [Google Scholar]
- Saltari, F.; De Courcy, J.; Pizzoli, M.; Constantin, L.; Mastroddi, F.; Coppotelli, G.; Titurus, B.; Rendall, T.; Cooper, J.; Gambioli, F. Data driven and model-based vertical sloshing reduced order models for aeroelastic analysis. In Proceedings of the International Forum on Aeroelasticity and Structural Dynamics, Madrid, Spain, 13–17 June 2022. [Google Scholar]
- Malatkar, P.; Nayfeh, A.H. On the Transfer of Energy between Widely Spaced Modes in Structures. Nonlinear Dyn. 2003, 31, 225–242. [Google Scholar] [CrossRef]
- Ibrahim, R.A. Liquid Sloshing Dynamics: Theory and Applications; Cambridge University Press: Cambridge, UK, 2005. [Google Scholar]

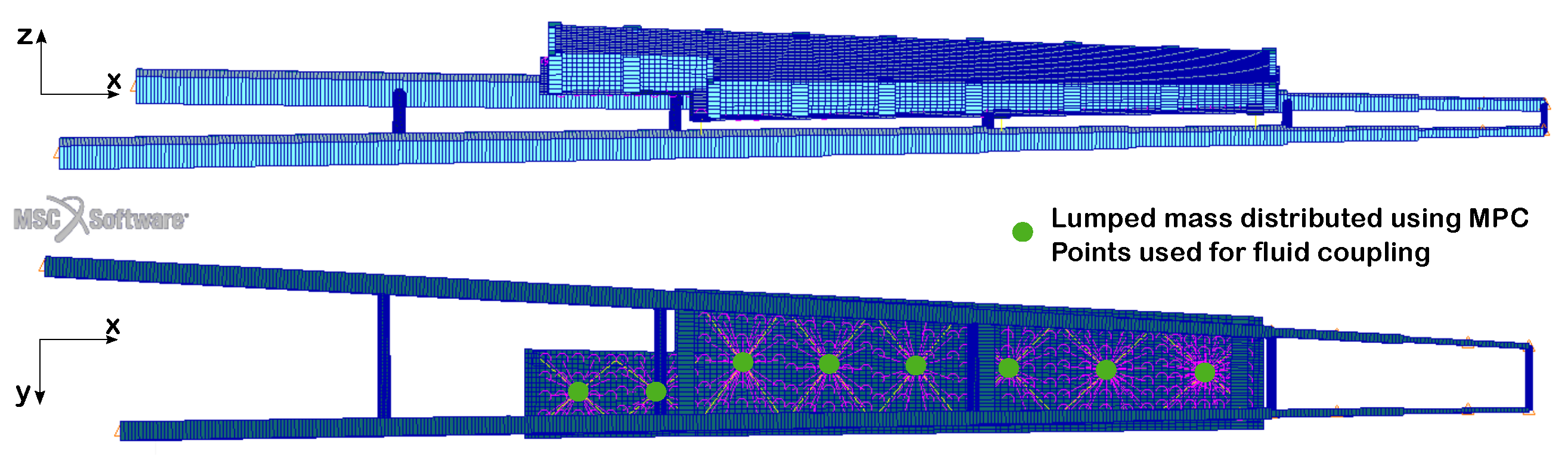




| Element Type | Nastran Element | Number of Elements |
|---|---|---|
| Plate | CQUAD4 | 10,147 |
| Beam | CBEAM | 1200 |
| Spring | CBUSH | 17 |
| Spring | CELAS1 | 6 |
| Mass | CONM2 | 55 |
| MPC | RBE3 | 22 |
| Number of nodes | 10,903 |
| Parameter | |||||
|---|---|---|---|---|---|
| Value | 4.71 | 0.70 | 1.29 | 2.64 | 3.63 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Constantin, L.; De Courcy, J.J.; Titurus, B.; Rendall, T.C.S.; Cooper, J.E.; Gambioli, F. Effect of Fuel Sloshing on the Damping of a Scaled Wing Model—Experimental Testing and Numerical Simulations. Appl. Sci. 2022, 12, 7860. https://doi.org/10.3390/app12157860
Constantin L, De Courcy JJ, Titurus B, Rendall TCS, Cooper JE, Gambioli F. Effect of Fuel Sloshing on the Damping of a Scaled Wing Model—Experimental Testing and Numerical Simulations. Applied Sciences. 2022; 12(15):7860. https://doi.org/10.3390/app12157860
Chicago/Turabian StyleConstantin, Lucian, Joe J. De Courcy, Branislav Titurus, Thomas C. S. Rendall, Jonathan E. Cooper, and Francesco Gambioli. 2022. "Effect of Fuel Sloshing on the Damping of a Scaled Wing Model—Experimental Testing and Numerical Simulations" Applied Sciences 12, no. 15: 7860. https://doi.org/10.3390/app12157860
APA StyleConstantin, L., De Courcy, J. J., Titurus, B., Rendall, T. C. S., Cooper, J. E., & Gambioli, F. (2022). Effect of Fuel Sloshing on the Damping of a Scaled Wing Model—Experimental Testing and Numerical Simulations. Applied Sciences, 12(15), 7860. https://doi.org/10.3390/app12157860






