Analysis and Application of Lining Resistance to Water Pressure in Tunnel through Karst Cave
Abstract
:1. Introduction
2. Failure Mode of Grouting in Tunnels Crossing High-Pressure Karst Caves
2.1. Failure Characteristics of Grouting in Tunnel Crossing the High-Pressure Karst Cave
2.1.1. Establishment of the Numerical Calculation Model
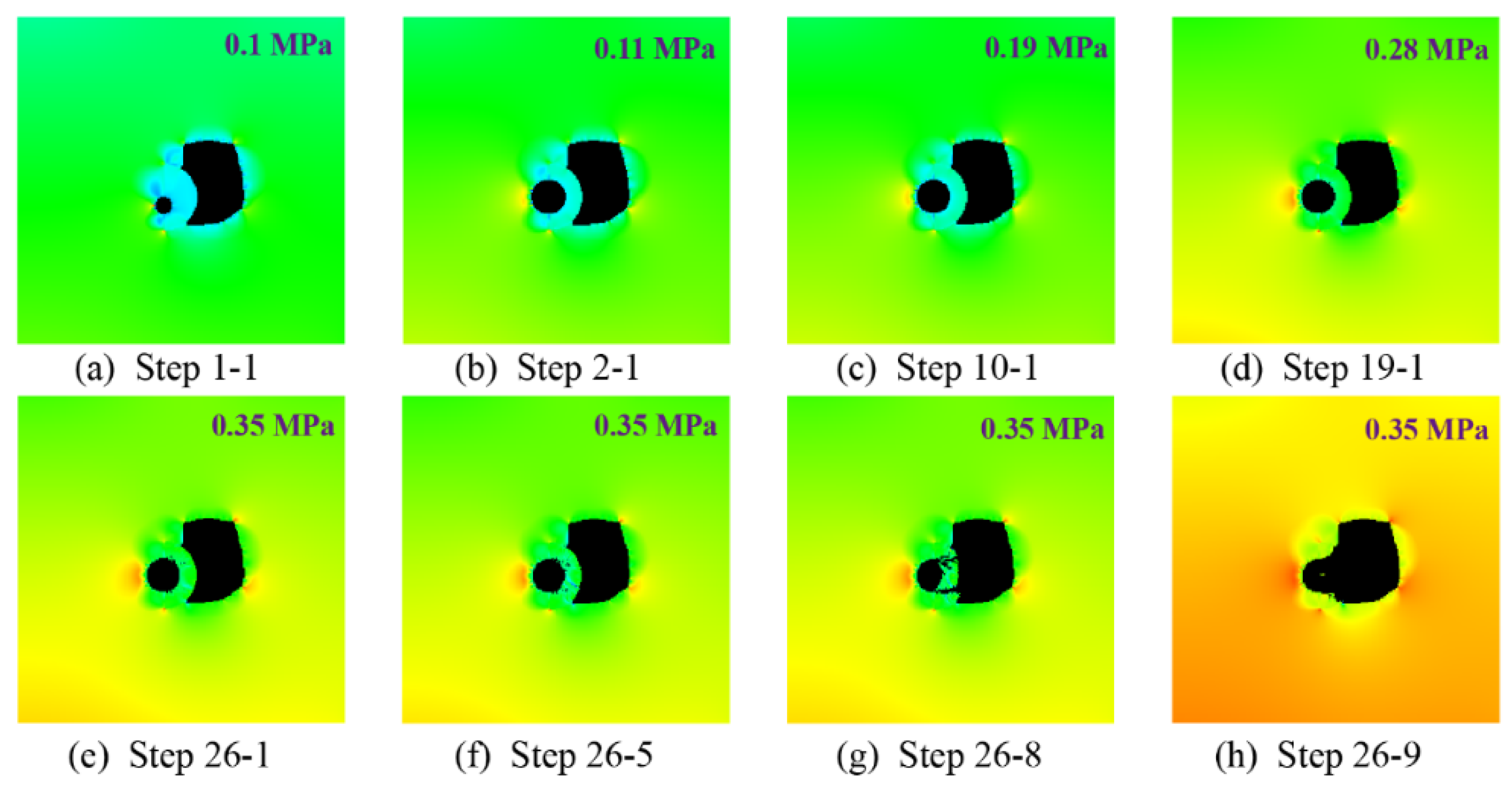
2.1.2. Results of Numerical Calculations
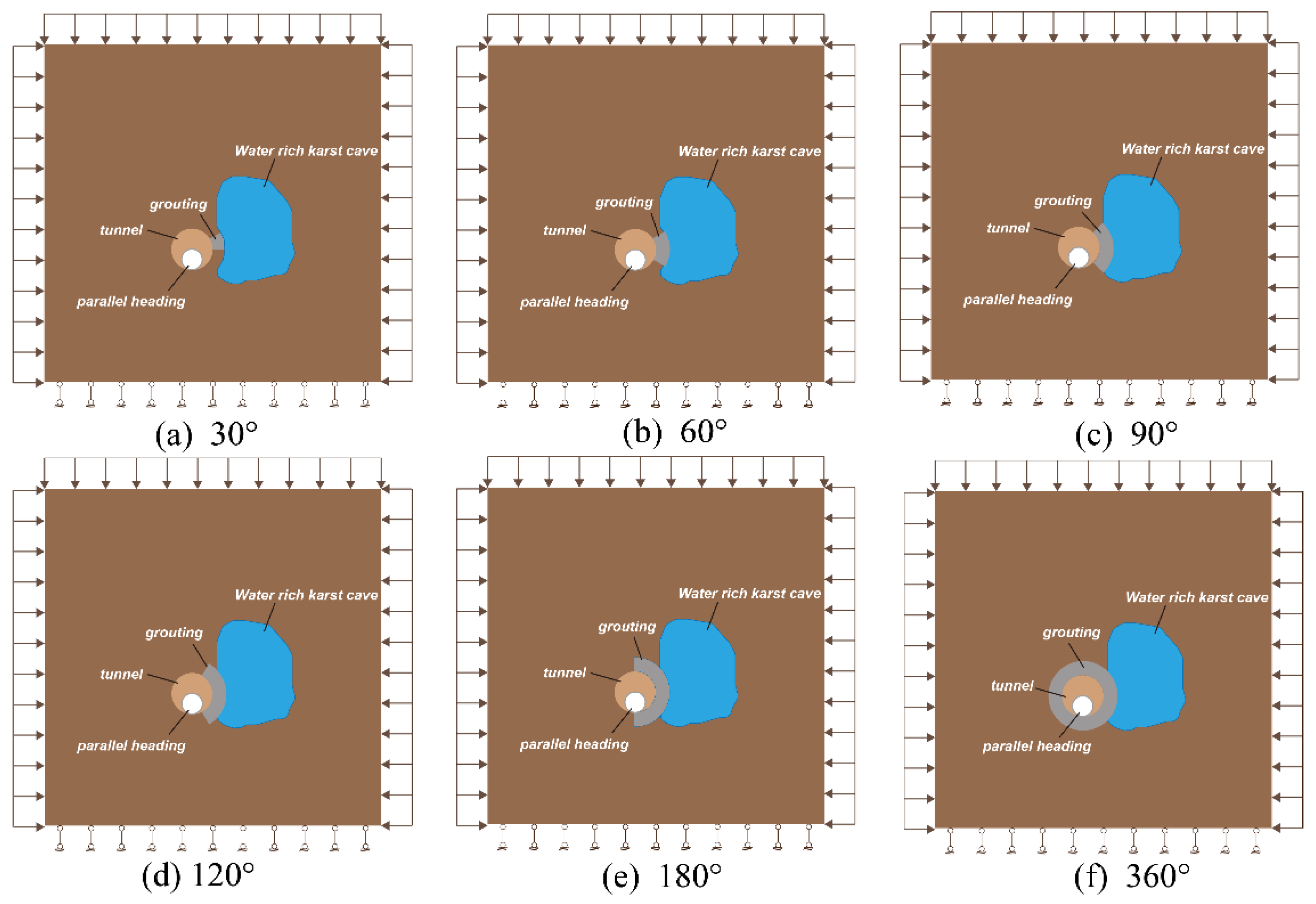
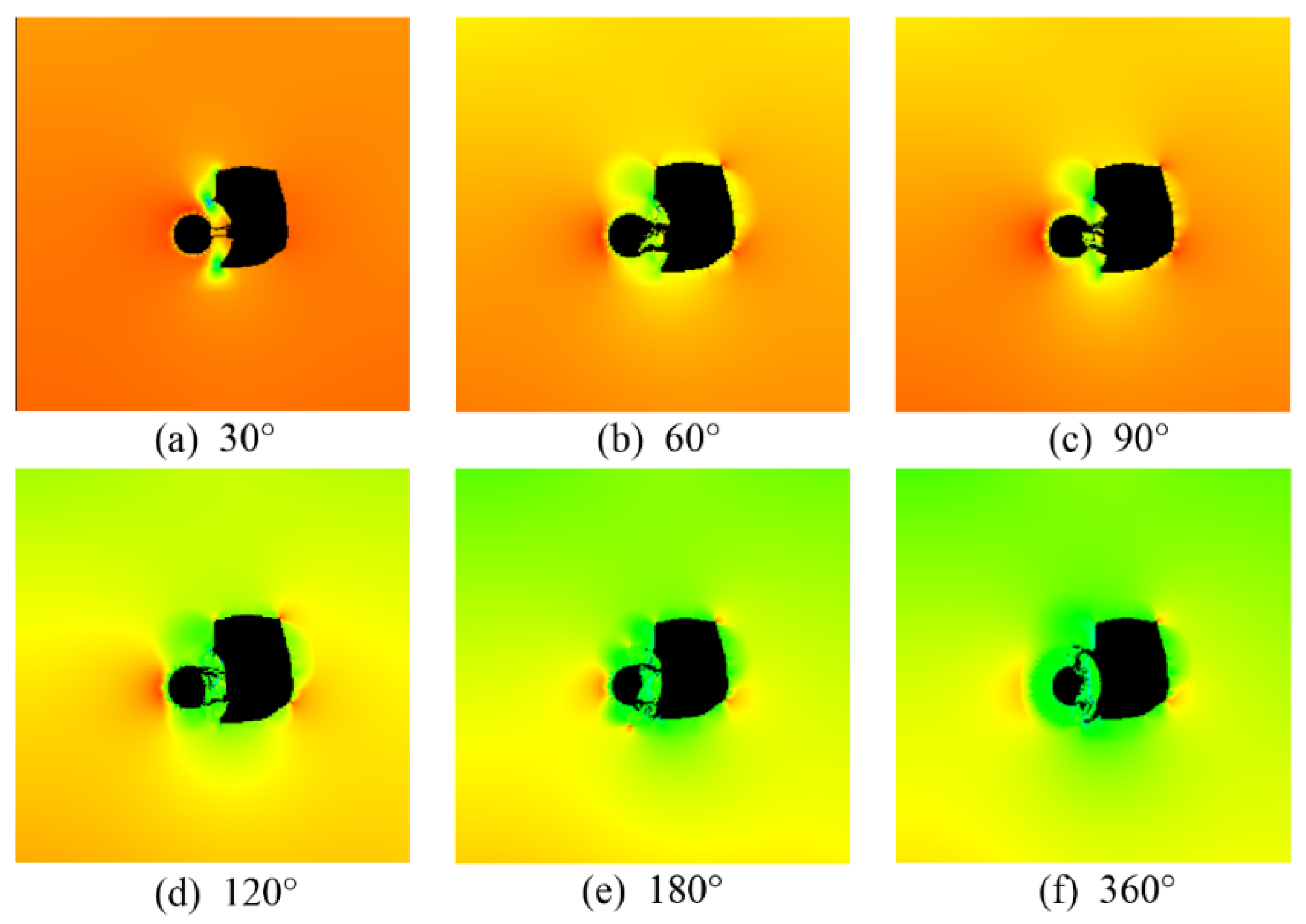
2.2. Influence of Cavity Location on the Effect of Grouting
2.2.1. Establishment of Numerical Calculation Models
2.2.2. Results of Numerical Calculations
2.3. Influence of Grouting Range on the Effect of Grouting
2.3.1. Establishment of Numerical Calculation Models
2.3.2. Results of Numerical Calculations
3. Analysis of the Safety Thickness of Composite Surrounding Rock in Tunnels Crossing High-Pressure Karst Caves
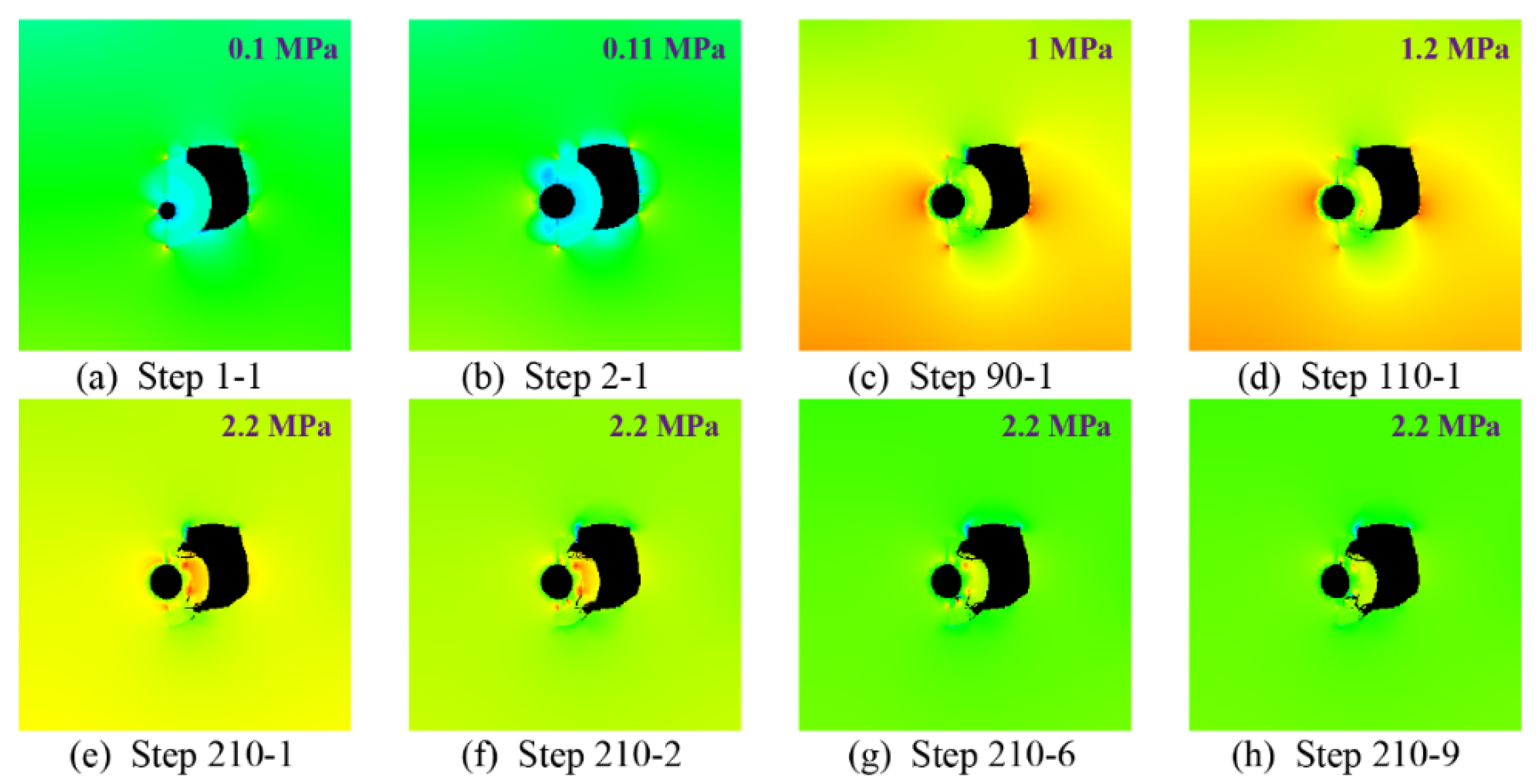
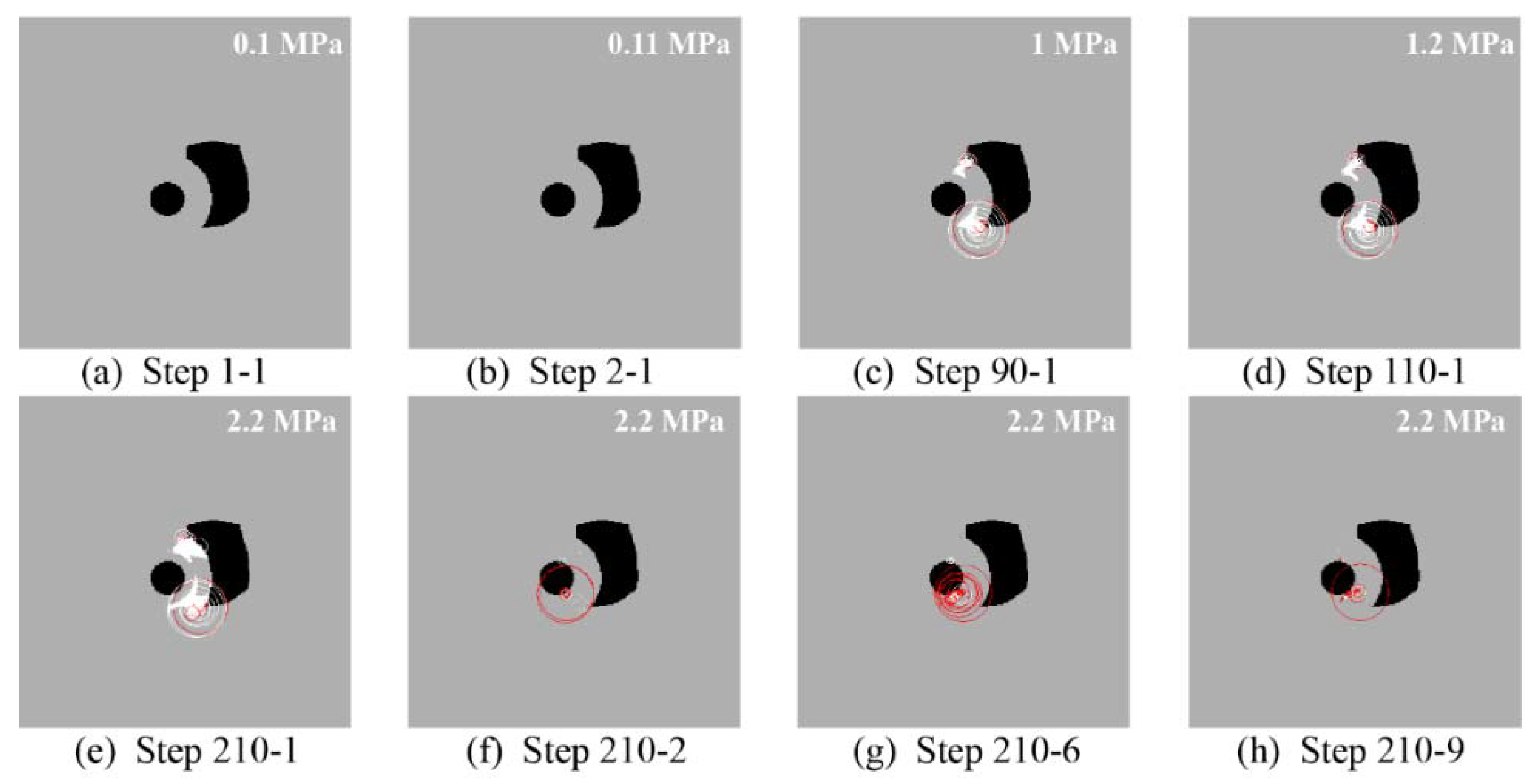
3.1. Failure Characteristics of Surrounding Rock with Grouting in Tunnel Crossing the High-Pressure Karst Cave
3.1.1. Establishment of the Numerical Calculation Model
3.1.2. Results of Numerical Calculations
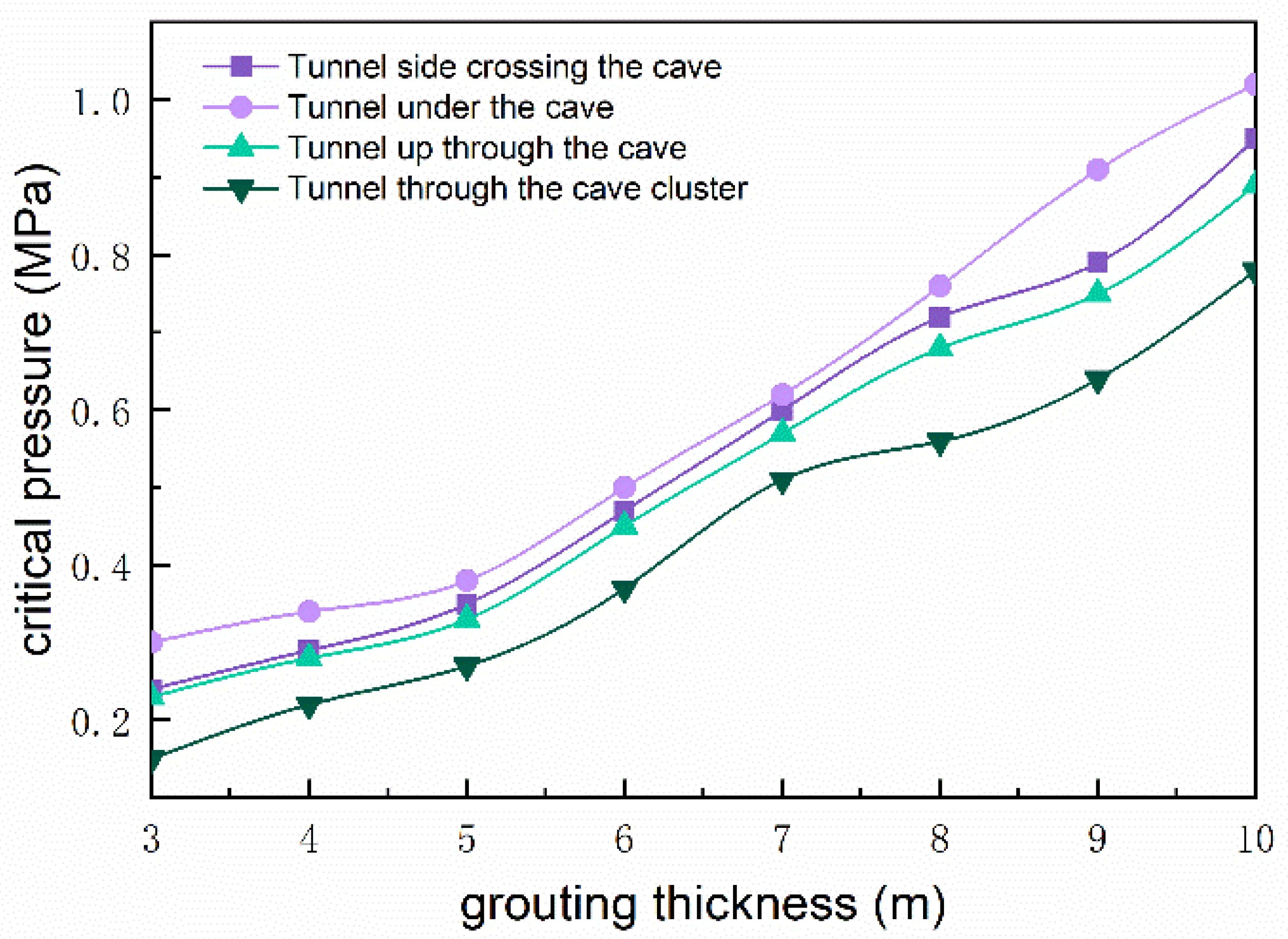
3.2. Analysis of Tunnel Safety Thickness for Different Calculation Cases
3.2.1. Analysis of Safety Thickness of the Surrounding Rock
3.2.2. Analysis of Safety Thickness of the Composite Surrounding Rock
3.2.3. Analysis of Safety Thickness of the Composite Structure
4. Engineering Application
4.1. Project Overview
4.2. Construction Plan for Excavation Expansion
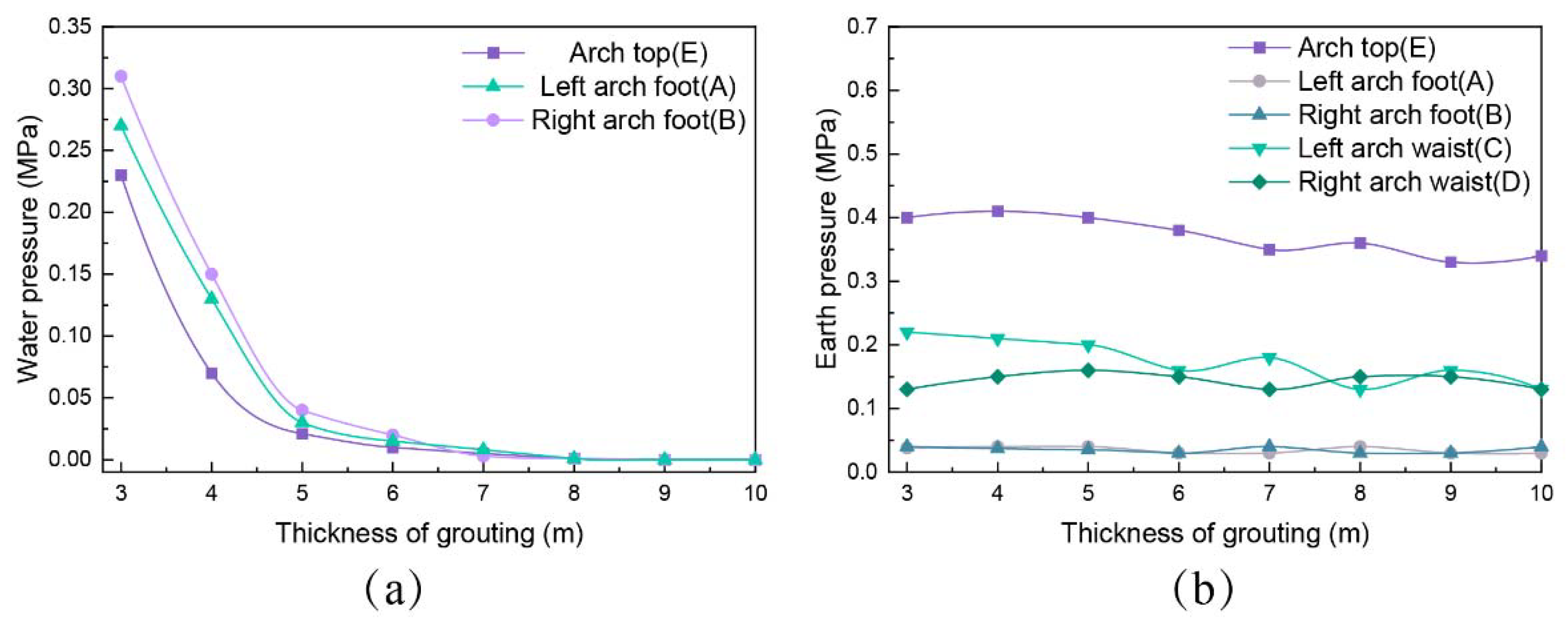
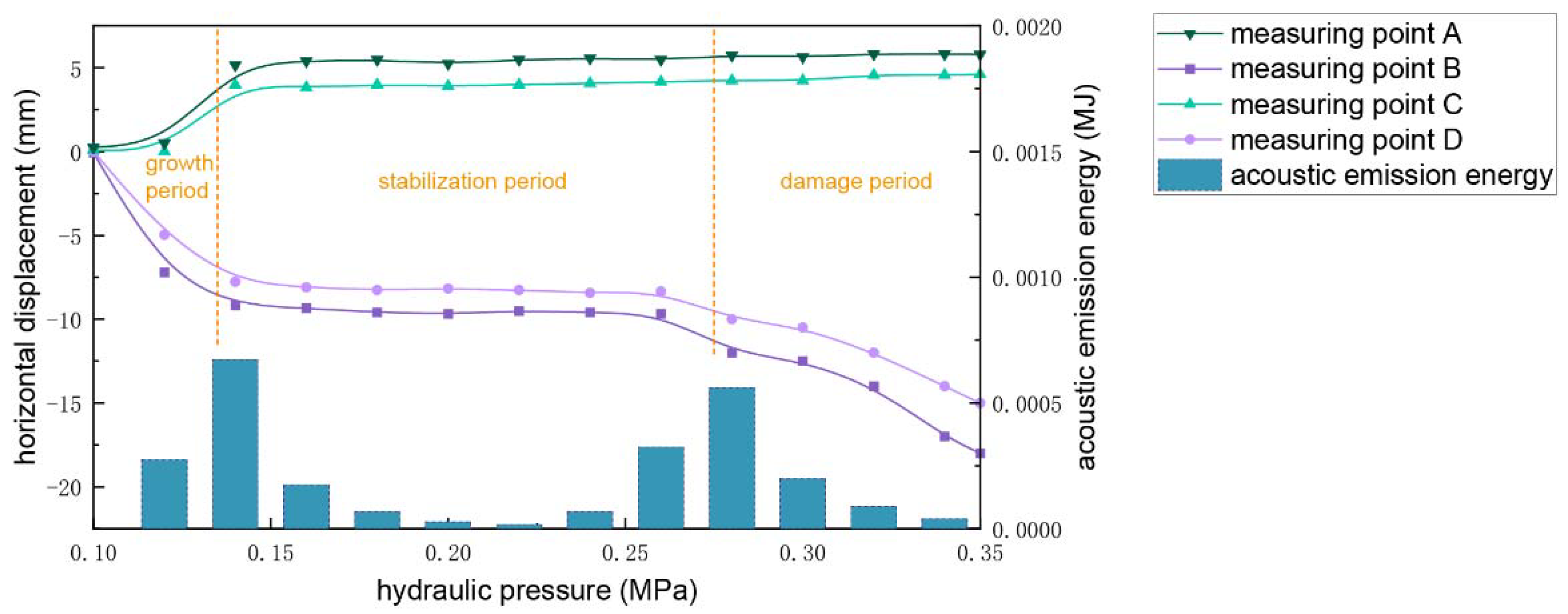
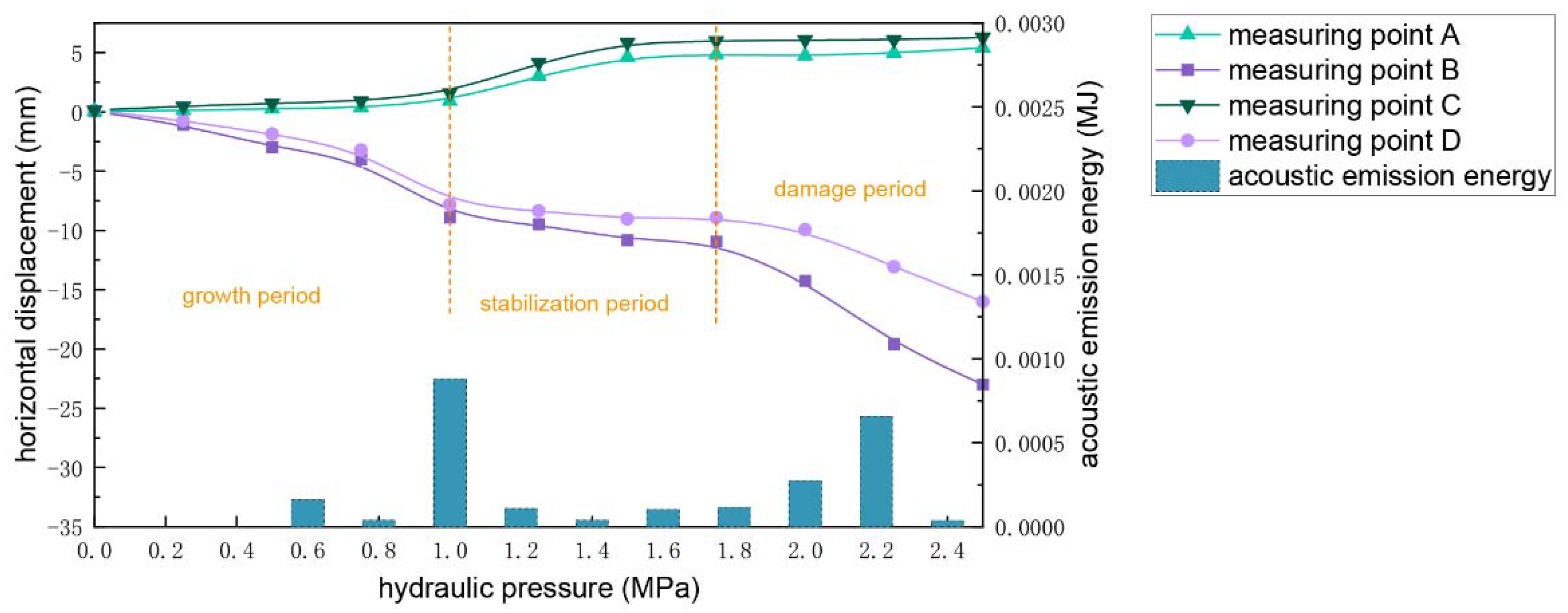
4.3. Field Test Verification
5. Discussion
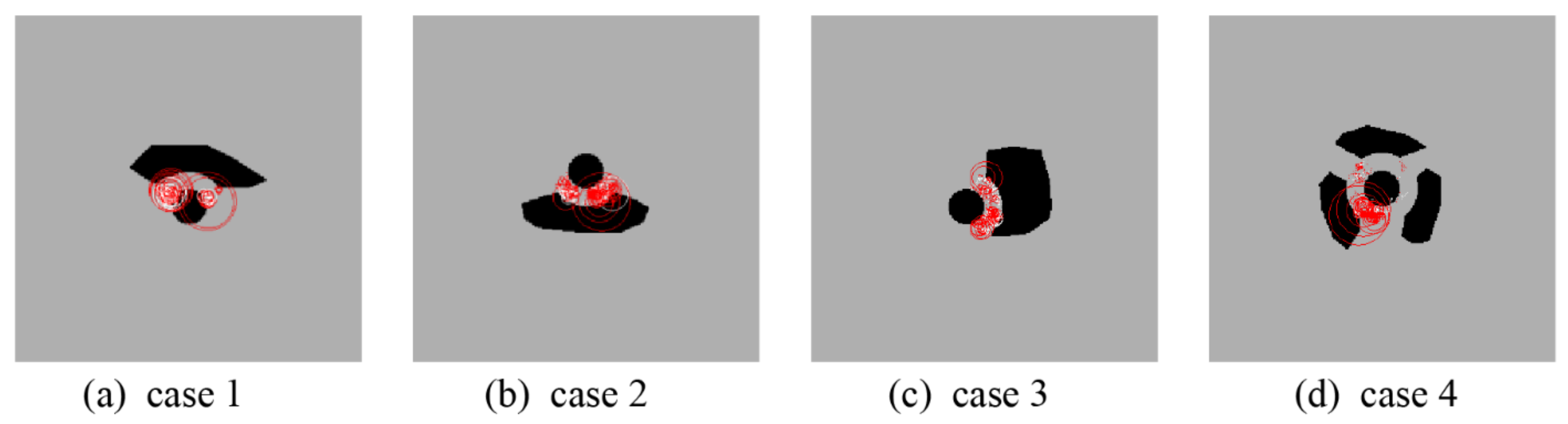
5.1. Analysis of Damage Characteristics
5.2. Verification of Model Reliability
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Pan, X.D.; Fang, Y.C.; Lai, Y.; Sun, H.L.; Cai, Y.Q.; Shi, L.; Geng, X.Y. Three-dimensional numerical modeling of water distribution tunnels in karst area. Arab. J. Geosci. 2020, 13, 1242. [Google Scholar] [CrossRef]
- Liu, Y.; Feng, Y.; Xu, M.; Zhang, Y.; Long, H.; Zhu, H. Effect of an incremental change in external water pressure on tunnel lining: A case study from the Tongxi karst tunnel. Nat. Hazards 2019, 98, 343–377. [Google Scholar] [CrossRef]
- Yang, J.; Zhang, C.; Fu, J.; Wang, S.; Ou, X.; Xie, Y. Pre-grouting reinforcement of underwater karst area for shield tunneling passing through Xiangjiang River in Changsha, China. Tunn. Undergr. Space Technol. 2020, 100, 103380. [Google Scholar] [CrossRef]
- Zhao, H.; Zhang, F.; Yao, X. Hydromechanical Simulation of Tunnel Excavation in Rock Considering a Nearby Karst Cave. Math. Probl. Eng. 2021, 2021, 7875725. [Google Scholar] [CrossRef]
- Hasanipanah, M.; Jamei, M.; Mohammed, A.S.; Amar, M.N.; Hocine, O.; Khedher, K.M. Intelligent prediction of rock mass deformation modulus through three optimized cascaded forward neural network models. Earth Sci. Inform. 2022, 1–11. [Google Scholar] [CrossRef]
- Chen, H.; Sha, C. Stability Analysis of Surrounding Rock and Treatment Structures in Superlarge Karst Cave of Naqiu Tunnel. Adv. Civ. Eng. 2018, 2018, 4842308. [Google Scholar] [CrossRef]
- Tian, Q.; Zhang, J.; Zhang, Y. Similar simulation experiment of expressway tunnel in karst. Constr. Build. Mater. 2018, 176, 1–13. [Google Scholar] [CrossRef]
- Xie, Y.; Yang, J.; Zhang, C.; Fu, J. Structures and Settlement Control of Yujingshan High-Speed Railway Tunnel Crossing Massive Rockfill in a Giant Karst Cave. Transp. Res. Rec. 2022, 2676, 589–602. [Google Scholar] [CrossRef]
- Shi, Z.; Wang, Q.; Pang, C.; Yuan, Y.; Wang, F.; Song, H.; Liu, J.; Zhang, Z.; Sun, R.; Liu, Y. Establishment and Application of the Spatial Decomposition Method (SDM) for Tunnels Passing Through Large Karst Caves. Appl. Sci. 2020, 10, 7204. [Google Scholar] [CrossRef]
- Lv, J.; Li, X.; He, Y.; Fu, H.; Yin, Y. Analytical analyses of the effect of filled karst cavern on tunnel lining structure under complex geological conditions. AIP Adv. 2019, 9, 035148. [Google Scholar] [CrossRef] [Green Version]
- Guo, J.; Ren, L.; Wang, G. Stability analysis of rock pillar between tunnel and large-scale concealed cave on top of tunnel. In Proceedings of the 2nd International Conference on Civil Engineering and Transportation (ICCET 2012), Guilin, China, 27–28 October 2012; pp. 1325–1328. [Google Scholar]
- Guo, J.; Ren, L.; Liu, X. Study on Safe Thickness of Comparatively Intact Rock ahead of Karst Tunnel Face. In Proceedings of the International Conference on Civil Engineering and Transportation (ICCET 2011), Jinan, China, 14–16 October 2011; pp. 2456–2459. [Google Scholar]
- Wu, W.; Liu, X.; Guo, J.; Sun, F.; Huang, X.; Zhu, Z. Upper limit analysis of stability of the water-resistant rock mass of a Karst tunnel face considering the seepage force. Bull. Eng. Geol. Environ. 2021, 80, 5813–5830. [Google Scholar] [CrossRef]
- Li, L.P.; Xiong, Y.F.; Wang, J.; Gao, X.C.; Wang, K.; Sun, H.C.; Fang, Z.D. Comprehensive Influence Analysis of Multiple Parameters on the Safety Thickness against Water Inrush in Shield Tunnel. Int. J. Geomech. 2020, 20, 04020226. [Google Scholar] [CrossRef]
- Li, J.; Chen, Q.; Huang, X.; Zou, G.; Deng, J. Pretreatment for Tunnel Karst Cave during Excavation: A Case Study of Guangxi, China. Adv. Civ. Eng. 2021, 2021, 9013815. [Google Scholar] [CrossRef]
- Zhang, Q.; Huang, B.; He, M.; Guo, S.; Tinivella, U. A Numerical Investigation on the Hydraulic Fracturing Effect of Water Inrush during Tunnel Excavation. Geofluids 2020, 2020, 6196327. [Google Scholar] [CrossRef]
- Hu, J.; Xu, N. Numerical analysis of failure mechanism of tunnel under different confining pressure. In Proceedings of the 1st International Symposium on Mine Safety Science and Engineering (ISMSSE), Beijing, China, 26–29 October 2011. [Google Scholar]
- Wang, S.Y.; Sloan, S.W.; Tang, C.A.; Zhu, W.C. Numerical simulation of the failure mechanism of circular tunnels in transversely isotropic rock masses. Tunn. Undergr. Space Technol. 2012, 32, 231–244. [Google Scholar] [CrossRef]
- Tan, Z.H.; Tang, C.A.; Cao, W.A.; Yang, T.H. Influence of Karst cave’s position on wall rock long-term stability of tunnel. In Proceedings of the 5th International Conference on Nonlinear Mechanics (ICNM-V), Shanghai, China, 11–14 June 2007; pp. 493–497. [Google Scholar]
- Filipponi, M.; Jeannin, P.Y.; Tacher, L. Evidence of inception horizons in karst conduit networks. Geomorphology 2009, 106, 86–99. [Google Scholar] [CrossRef]










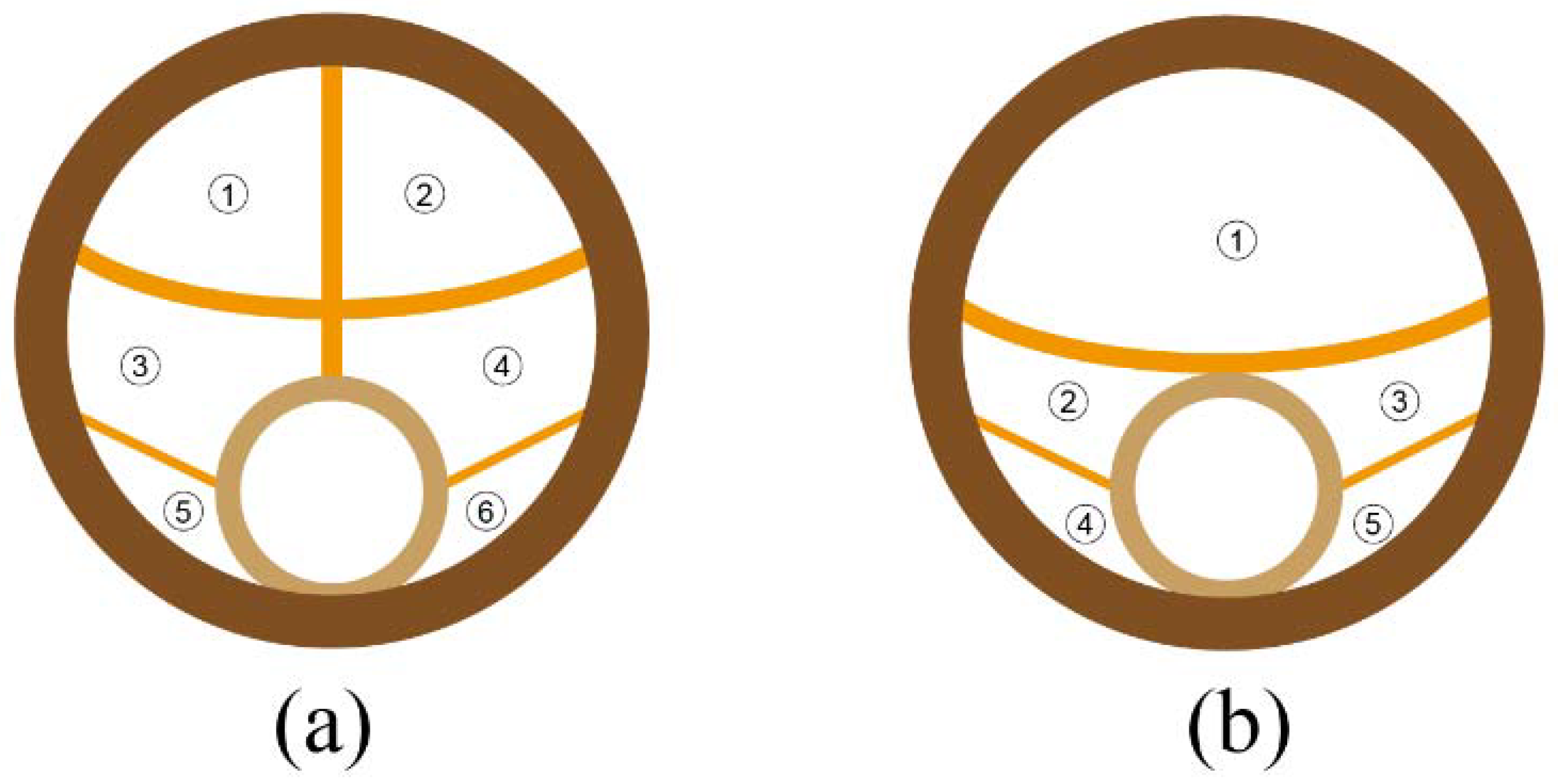
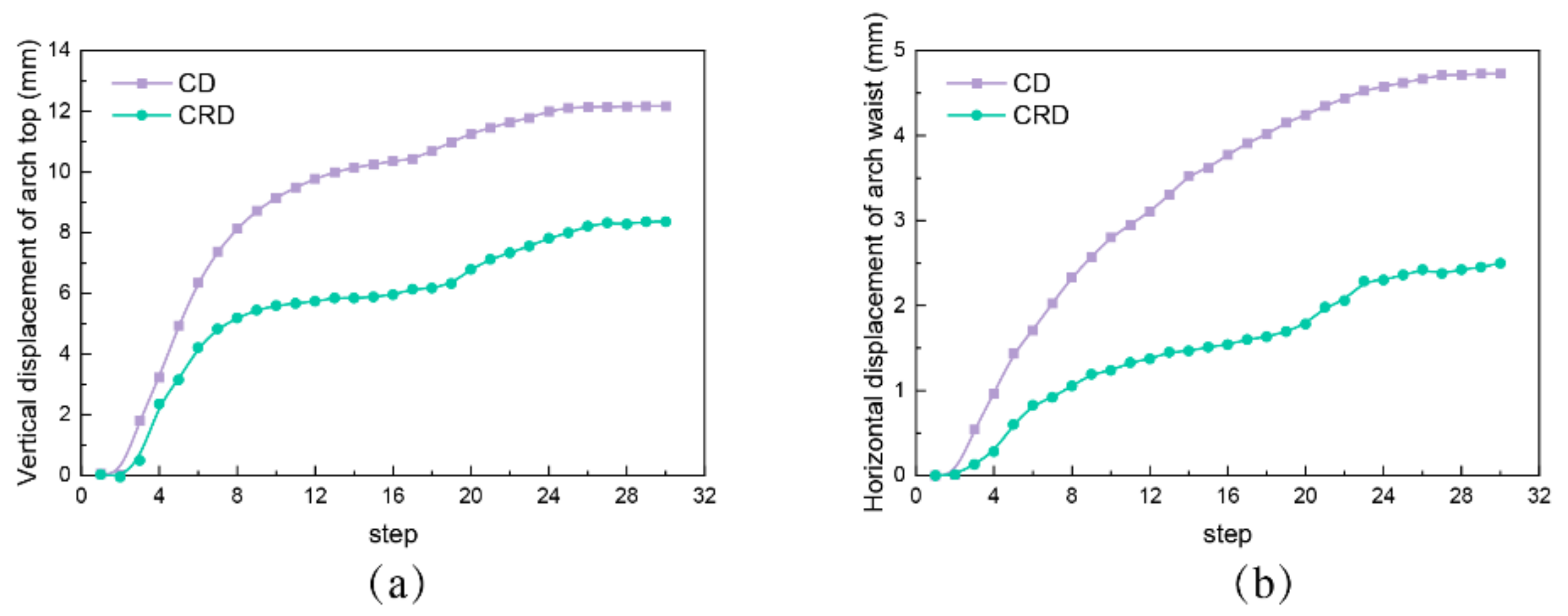
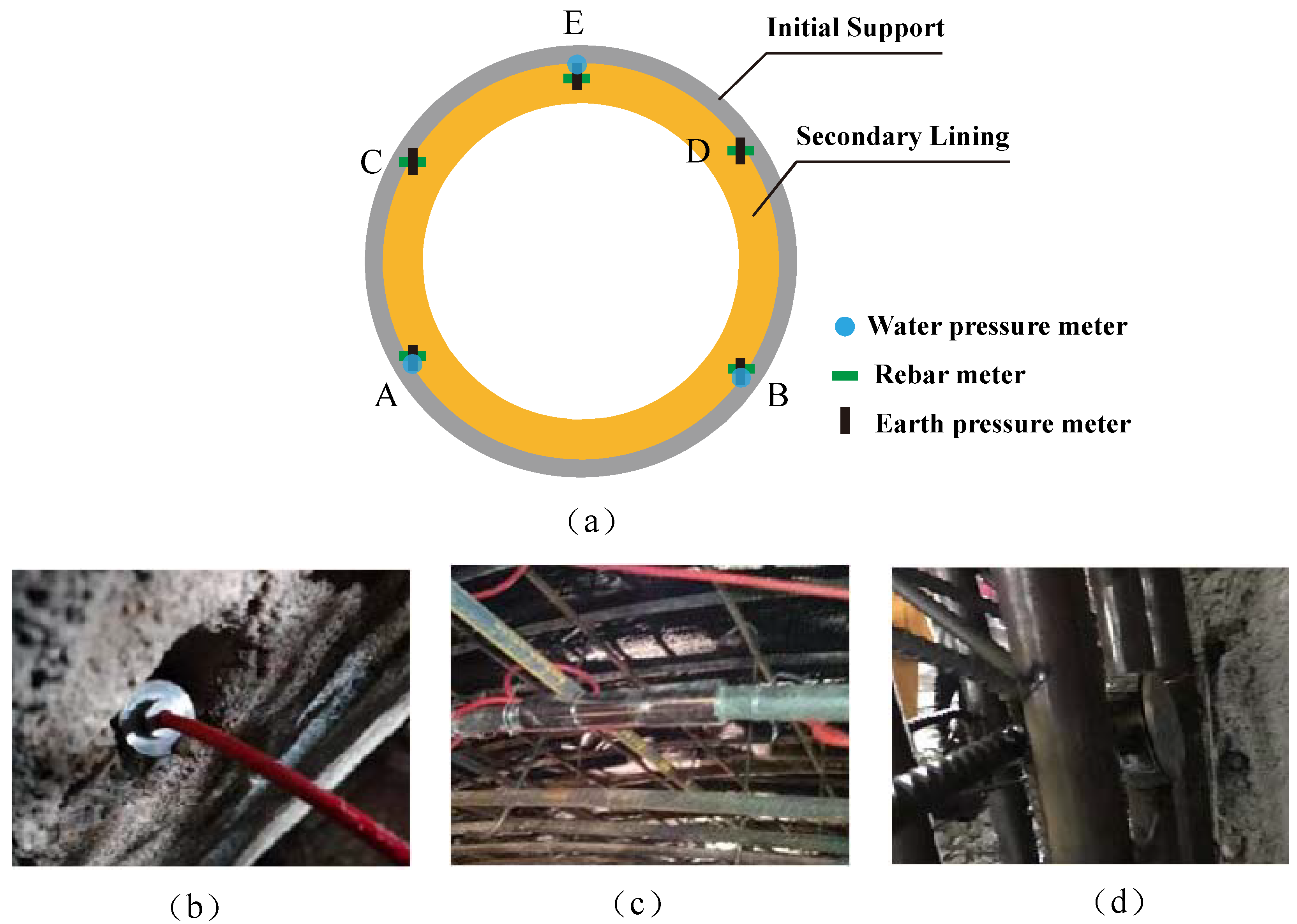
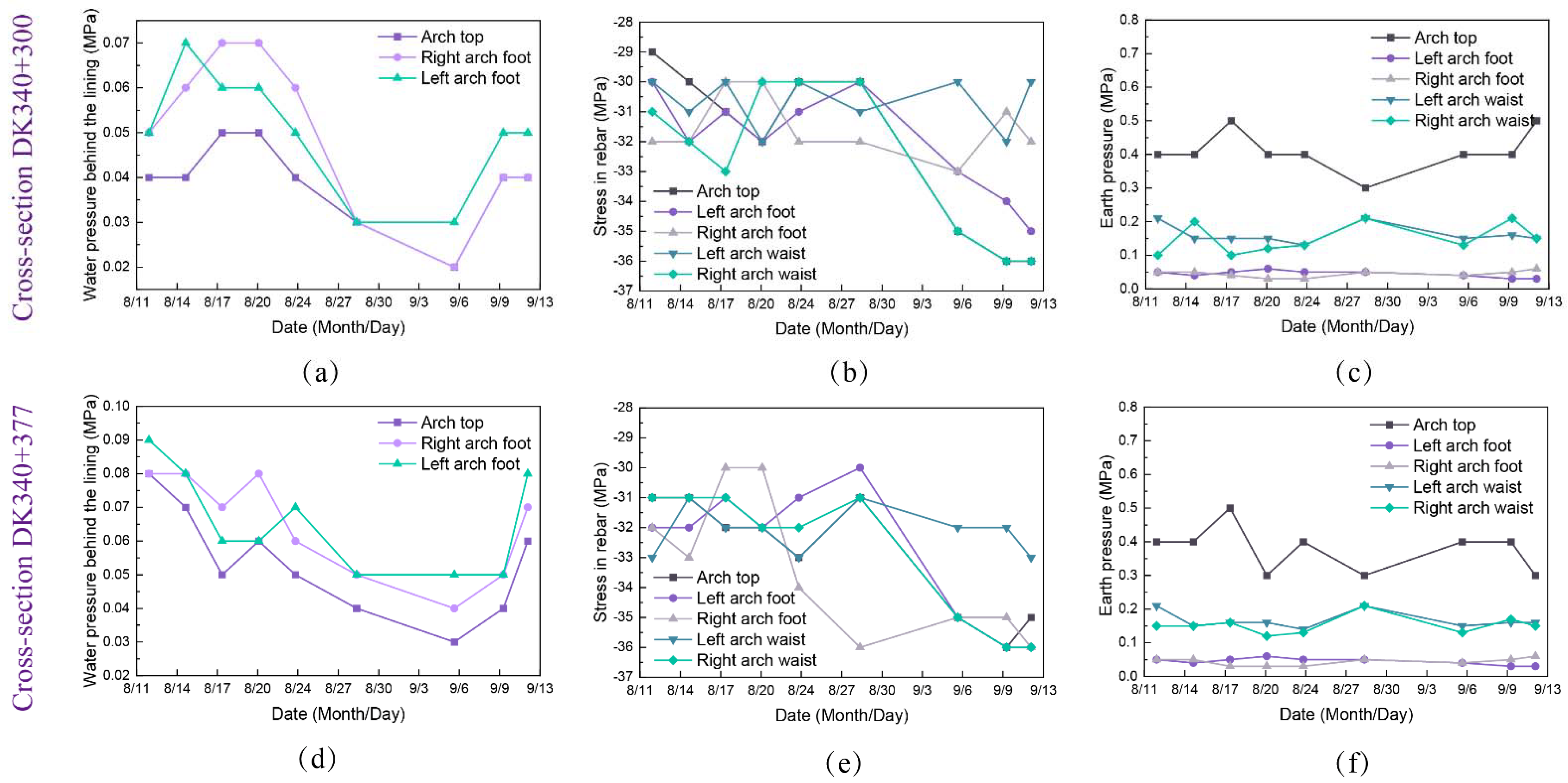

| Materials | Elastic Modulus (GPa) | Poisson’s Ratio | Angle of Internal Friction (°) | Permeability Coefficient (m/Day) | Cohesion (MPa) | Gravity (kN/m3) |
|---|---|---|---|---|---|---|
| Rock | 0.48 | 0.3 | 35 | 0.044 | 0.05 | 20 |
| Grouting | 2.0 | 0.35 | 35 | 0.00443 | 0.065 | 24 |
| Thickness of Secondary Lining (m) | 0 | 0.4 | 0.8 | 1.0 | 1.2 |
|---|---|---|---|---|---|
| Critical water pressure (MPa) | 0.68 | 1.51 | 2.30 | 3.56 | 4.44 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Huang, M.; Li, J.; Yang, Z.; Zhang, Z.; Song, Y. Analysis and Application of Lining Resistance to Water Pressure in Tunnel through Karst Cave. Appl. Sci. 2022, 12, 7605. https://doi.org/10.3390/app12157605
Huang M, Li J, Yang Z, Zhang Z, Song Y. Analysis and Application of Lining Resistance to Water Pressure in Tunnel through Karst Cave. Applied Sciences. 2022; 12(15):7605. https://doi.org/10.3390/app12157605
Chicago/Turabian StyleHuang, Mingli, Jiacheng Li, Ze Yang, Zhien Zhang, and Yuan Song. 2022. "Analysis and Application of Lining Resistance to Water Pressure in Tunnel through Karst Cave" Applied Sciences 12, no. 15: 7605. https://doi.org/10.3390/app12157605
APA StyleHuang, M., Li, J., Yang, Z., Zhang, Z., & Song, Y. (2022). Analysis and Application of Lining Resistance to Water Pressure in Tunnel through Karst Cave. Applied Sciences, 12(15), 7605. https://doi.org/10.3390/app12157605






