Identification of Areas of Anomalous Tremor of the Earth’s Surface on the Japanese Islands According to GPS Data
Abstract
:1. Introduction
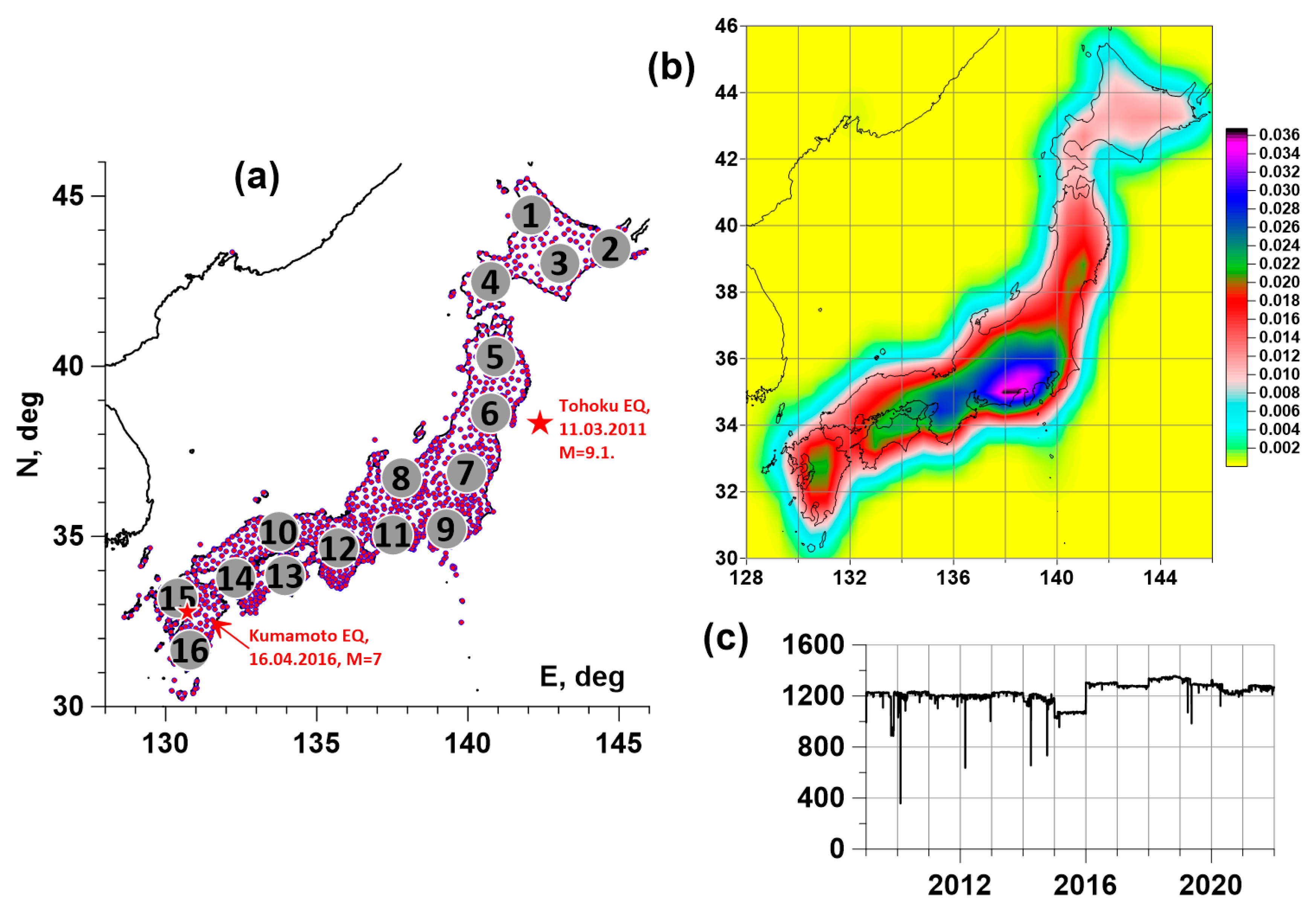
2. Materials and Methods
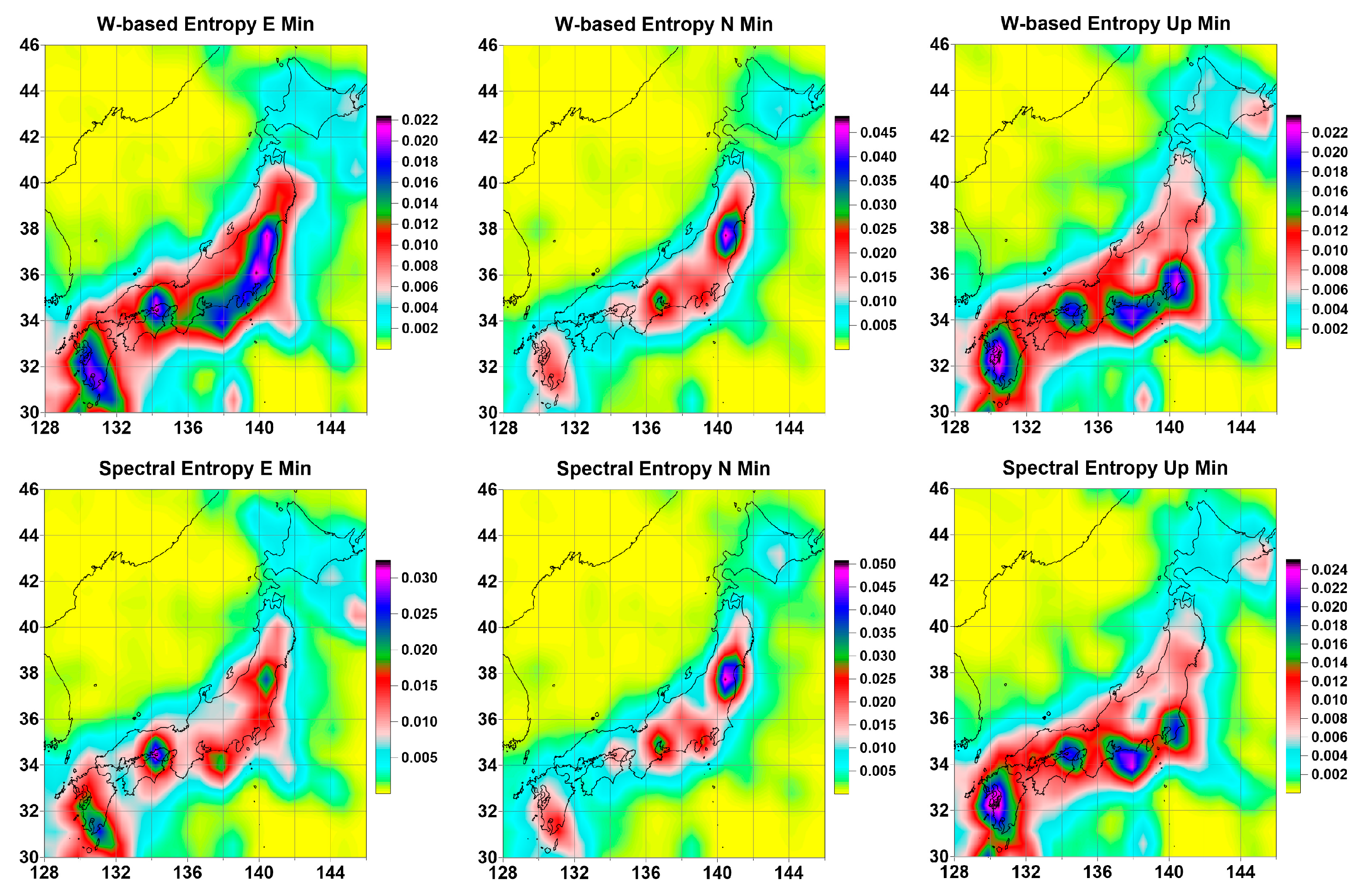
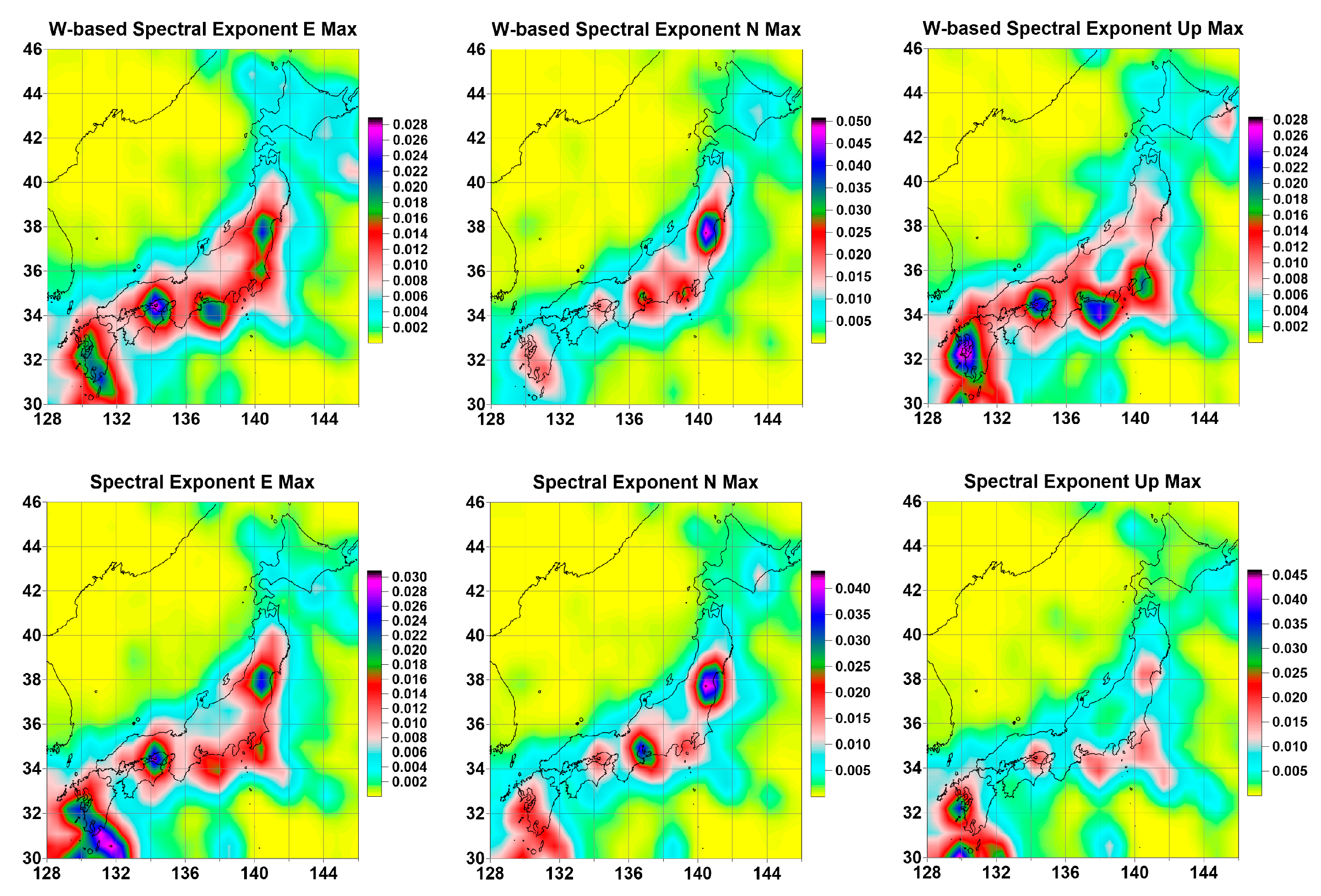
2.1. Minimum Normalized Wavelet-Based Entropy and Wavelet-Based Spectral Index
2.2. Spectral Normalized Entropy and “Usual” Spectral Index
2.3. Probability Densities of Extreme Values
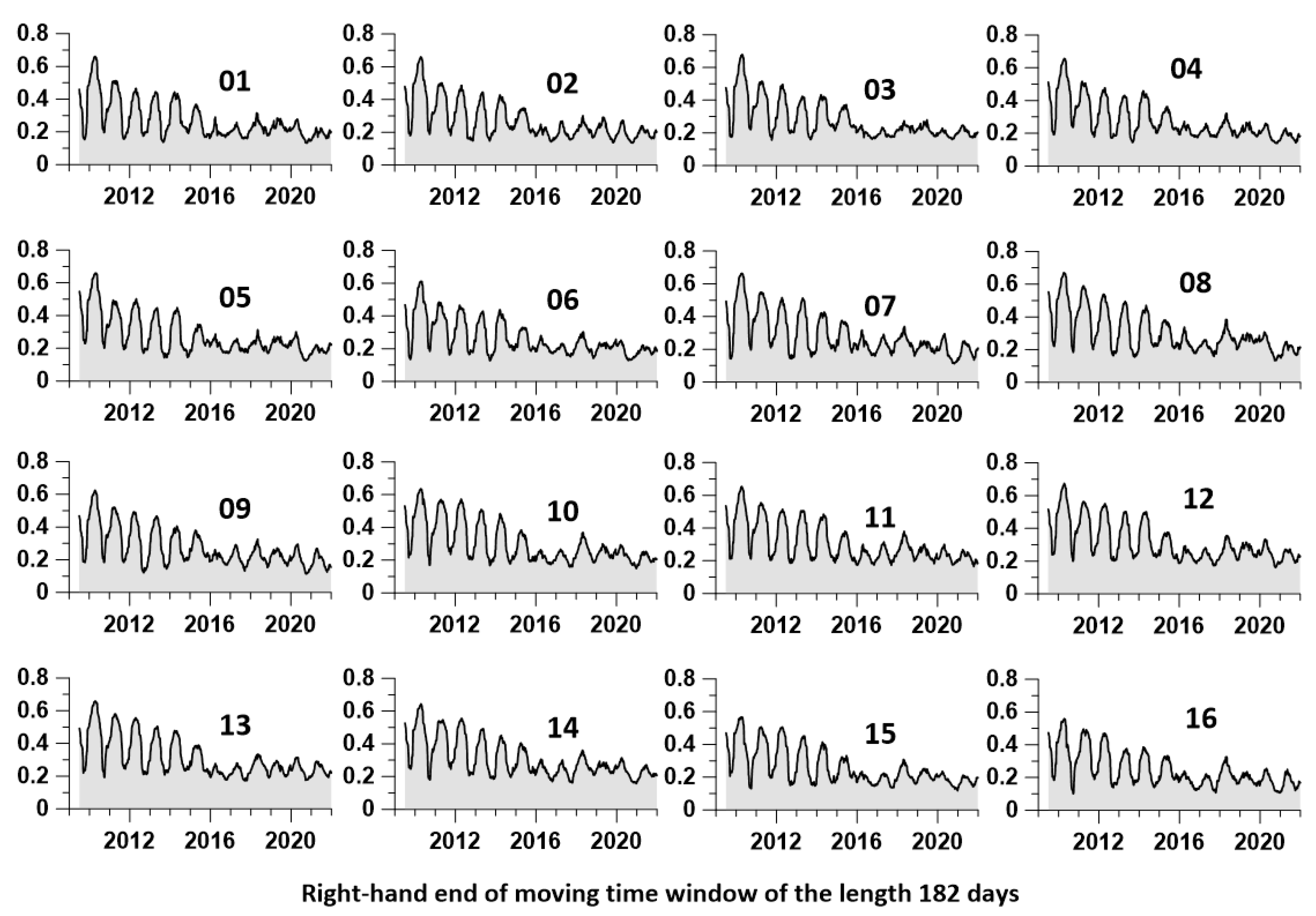
3. Trajectory of Extreme Mean Probability Density Maxima
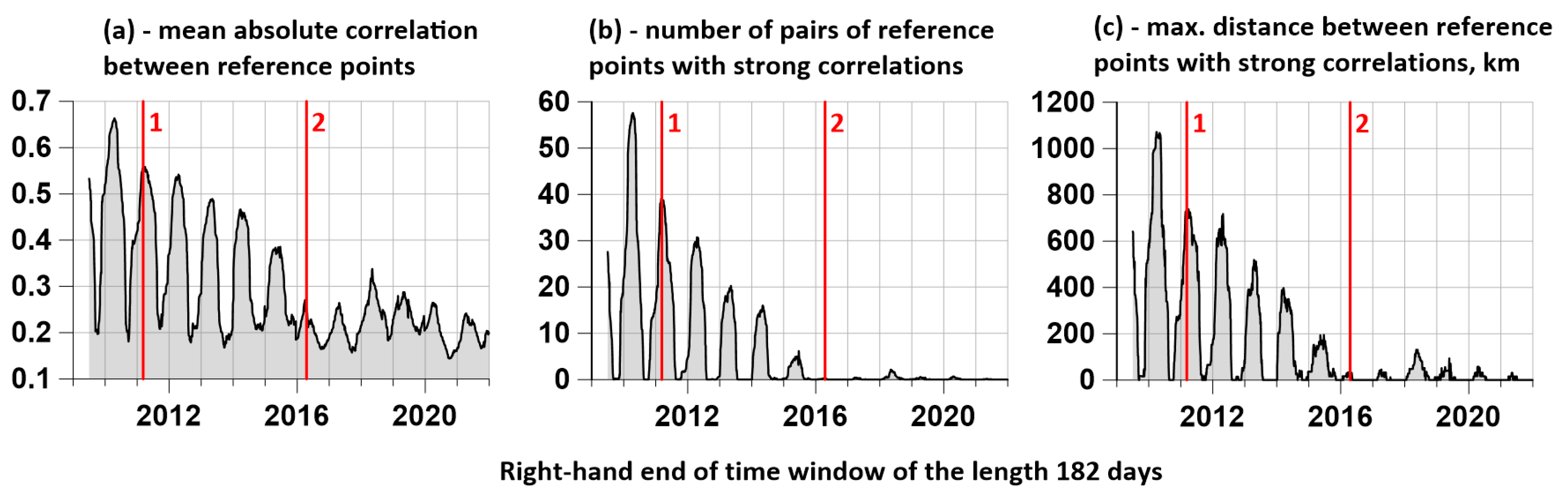
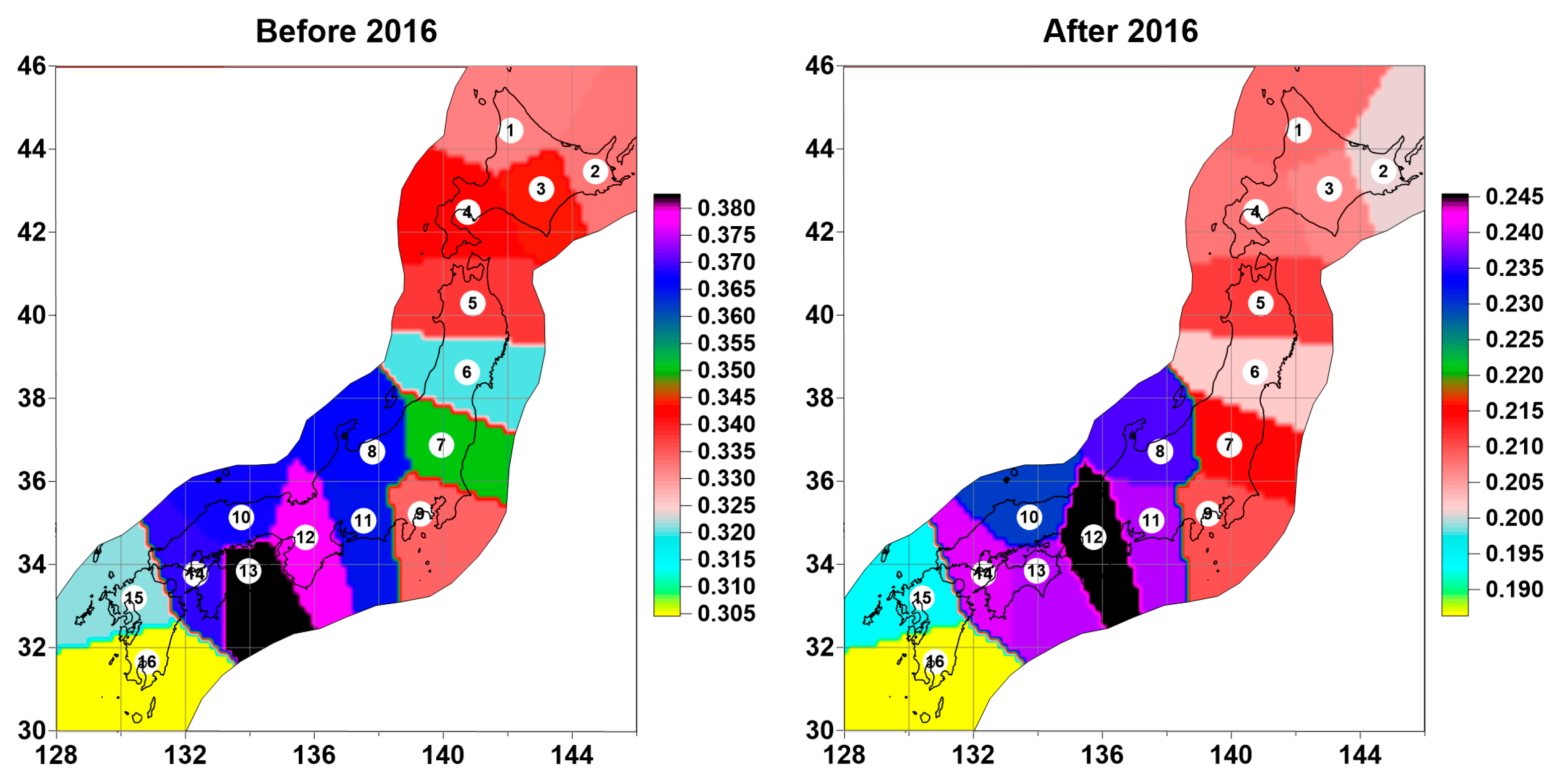
4. Spatial Correlations of Tremor Properties
5. Discussion
Funding
Data Availability Statement
Conflicts of Interest
References
- Langbein, J.; Johnson, H. Correlated errors in geodetic time series, implications for time-dependent deformation. J. Geophys. Res. 1997, 102, 591–603. [Google Scholar] [CrossRef]
- Zhang, J.; Bock, Y.; Johnson, H.; Fang, P.; Williams, S.; Genrich, J.; Wdowinski, S.; Behr, J. Southern California permanent GPS geodetic array: Error analysis of daily position estimates and site velocities. J. Geophys. Res. 1997, 102, 18035–18055. [Google Scholar] [CrossRef]
- Mao, A.; Harrison, C.G.A.; Dixon, T.H. Noise in GPS coordinate time series. J. Geophys. Res. 1999, 104, 2797–2816. [Google Scholar] [CrossRef] [Green Version]
- Williams, S.D.P.; Bock, Y.; Fang, P.; Jamason, P.; Nikolaidis, R.M.; Prawirodirdjo, L.; Miller, M.; Johnson, D.J. Error analysis of continuous GPS time series. J. Geophys. Res. 2004, 109, B03412. [Google Scholar] [CrossRef] [Green Version]
- Bos, M.S.; Bastos, L.; Fernandes, R.M.S. The influence of seasonal signals on the estimation of the tectonic motion in short continuous GPS time-series. J. Geodyn. 2010, 49, 205–209. [Google Scholar] [CrossRef] [Green Version]
- Wang, W.; Zhao, B.; Wang, Q.; Yang, S. Noise analysis of continuous GPS coordinate time series for CMONOC. Adv. Space Res. 2012, 49, 943–956. [Google Scholar] [CrossRef]
- Caporali, A. Average strain rate in the Italian crust inferred from a permanent GPS network—I. Statistical analysis of the time-series of permanent GPS stations. Geophys. J. Int. 2003, 155, 241–253. [Google Scholar] [CrossRef]
- Li, J.; Miyashita, K.; Kato, T.; Miyazaki, S. GPS time series modeling by autoregressive moving average method, Application to the crustal deformation in central Japan. Earth Planets Space 2000, 52, 155–162. [Google Scholar] [CrossRef] [Green Version]
- Beavan, J. Noise properties of continuous GPS data from concrete pillar geodetic monuments in New Zealand and comparison with data from U.S. deep drilled braced monuments. J. Geophys. Res. 2005, 110, B08410. [Google Scholar] [CrossRef]
- Langbein, J. Noise in GPS displacement measurements from Southern California and Southern Nevada. J. Geophys. Res. 2008, 113, B05405. [Google Scholar] [CrossRef]
- Blewitt, G.; Lavallee, D. Effects of annual signal on geodetic velocity. J. Geophys. Res. 2002, 107, 2145. [Google Scholar] [CrossRef] [Green Version]
- Bos, M.S.; Fernandes, R.M.S.; Williams, S.D.P.; Bastos, L. Fast error analysis of continuous GPS observations. J. Geod. 2008, 82, 157–166. [Google Scholar] [CrossRef] [Green Version]
- Teferle, F.N.; Williams, S.D.P.; Kierulf, H.P.; Bingley, R.M.; Plag, H.P. A continuous GPS coordinate time series analysis strategy for high-accuracy vertical land movements. Phys. Chem. Earth Parts A/B/C 2008, 33, 205–216. [Google Scholar] [CrossRef] [Green Version]
- Chen, Q.; van Dam, T.; Sneeuw, N.; Collilieux, X.; Weigelt, M.; Rebischung, P. Singular spectrum analysis for modeling seasonal signals from GPS time series. J. Geodyn. 2013, 72, 25–35. [Google Scholar] [CrossRef]
- Bock, Y.; Melgar, D.; Crowell, B.W. Real-Time Strong-Motion Broadband Displacements from Collocated GPS and Accelerometers. Bull. Seismol. Soc. Am. 2011, 101, 2904–2925. [Google Scholar] [CrossRef]
- Hackl, M.; Malservisi, R.; Hugentobler, U.; Jiang, Y. Velocity covariance in the presence of anisotropic time correlated noise and transient events in GPS time series. J. Geodyn. 2013, 72, 36–45. [Google Scholar] [CrossRef]
- Goudarzi, M.A.; Cocard, M.; Santerre, R.; Woldai, T. GPS interactive time series analysis software. GPS Solut. 2013, 17, 595–603. [Google Scholar] [CrossRef]
- Khelif, S.; Kahlouche, S.; Belbachir, M.F. Analysis of position time series of GPS-DORIS co-located stations. Int. J. Appl. Earth Observ. Geoinf. 2013, 20, 67–76. [Google Scholar] [CrossRef]
- Lyubushin, A. Global coherence of GPS-measured high-frequency surface tremor motions. GPS Solut. 2018, 22, 116. [Google Scholar] [CrossRef]
- Lyubushin, A. Field of coherence of GPS-measured earth tremors. GPS Solut. 2019, 23, 120. [Google Scholar] [CrossRef]
- Filatov, D.M.; Lyubushin, A.A. Fractal analysis of GPS time series for early detection of disastrous seismic events. Phys. A 2017, 469, 718–730. [Google Scholar] [CrossRef]
- Filatov, D.M.; Lyubushin, A.A. Precursory Analysis of GPS Time Series for Seismic Hazard Assessment. Pure Appl. Geophys. 2019, 177, 509–530. [Google Scholar] [CrossRef]
- Filatov, D.M.; Lyubushin, A.A. Stochastic dynamical systems always undergo trending mechanisms of transition to criticality. Phys. A 2019, 527, 121309. [Google Scholar] [CrossRef]
- Nevada Geodetic Laboratory. Available online: http://geodesy.unr.edu/NGLStationPages/RapidStationList (accessed on 1 July 2022).
- Blewitt, G.; Hammond, W.C.; Kreemer, C. Harnessing the GPS data explosion for interdisciplinary science. Eos 2018, 99, 485. [Google Scholar] [CrossRef]
- Duda, R.O.; Hart, P.E.; Stork, D.G. Pattern Classification; Wiley: Hoboken, NJ, USA, 2000. [Google Scholar]
- Mallat, S. A Wavelet Tour of Signal Processing, 2nd ed.; Academic Press: Cambridge, MA, USA, 1999. [Google Scholar]
- Marple, S.L., Jr. Digital Spectral Analysis with Applications; Prentice-Hall, Inc.: Englewood Cliffs, NJ, USA, 1987. [Google Scholar]
- Jolliffe, I.T. Principal Component Analysis; Springer: Berlin/Heidelberg, Germany, 1986. [Google Scholar]



| 1 | 0.8872 | 0.9315 | 0.9668 | 0.8746 | 0.9269 | 0.9884 | 0.8753 | 0.9336 | 0.9387 | 0.9049 | 0.8469 | |
| 0.8872 | 1 | 0.7922 | 0.9052 | 0.9832 | 0.8161 | 0.8799 | 0.9903 | 0.8129 | 0.8215 | 0.941 | 0.7044 | |
| 0.9315 | 0.7922 | 1 | 0.9129 | 0.7887 | 0.9764 | 0.9193 | 0.7826 | 0.9879 | 0.8994 | 0.8541 | 0.8948 | |
| 0.9668 | 0.9052 | 0.9129 | 1 | 0.9141 | 0.9308 | 0.9625 | 0.8975 | 0.9253 | 0.9198 | 0.9045 | 0.8154 | |
| 0.8746 | 0.9832 | 0.7887 | 0.9141 | 1 | 0.8227 | 0.8644 | 0.9851 | 0.815 | 0.8102 | 0.9372 | 0.6936 | |
| 0.9269 | 0.8161 | 0.9764 | 0.9308 | 0.8227 | 1 | 0.9074 | 0.8041 | 0.9894 | 0.862 | 0.8402 | 0.8261 | |
| 0.9884 | 0.8799 | 0.9193 | 0.9625 | 0.8644 | 0.9074 | 1 | 0.8701 | 0.9127 | 0.9486 | 0.8988 | 0.8565 | |
| 0.8753 | 0.9903 | 0.7826 | 0.8975 | 0.9851 | 0.8041 | 0.8701 | 1 | 0.803 | 0.8236 | 0.9477 | 0.7055 | |
| 0.9336 | 0.8129 | 0.9879 | 0.9253 | 0.815 | 0.9894 | 0.9127 | 0.803 | 1 | 0.8787 | 0.8524 | 0.85 | |
| 0.9387 | 0.8215 | 0.8994 | 0.9198 | 0.8102 | 0.862 | 0.9486 | 0.8236 | 0.8787 | 1 | 0.905 | 0.9326 | |
| 0.9049 | 0.941 | 0.8541 | 0.9045 | 0.9372 | 0.8402 | 0.8988 | 0.9477 | 0.8524 | 0.905 | 1 | 0.8453 | |
| 0.8469 | 0.7044 | 0.8948 | 0.8154 | 0.6936 | 0.8261 | 0.8565 | 0.7055 | 0.85 | 0.9326 | 0.8453 | 1 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lyubushin, A. Identification of Areas of Anomalous Tremor of the Earth’s Surface on the Japanese Islands According to GPS Data. Appl. Sci. 2022, 12, 7297. https://doi.org/10.3390/app12147297
Lyubushin A. Identification of Areas of Anomalous Tremor of the Earth’s Surface on the Japanese Islands According to GPS Data. Applied Sciences. 2022; 12(14):7297. https://doi.org/10.3390/app12147297
Chicago/Turabian StyleLyubushin, Alexey. 2022. "Identification of Areas of Anomalous Tremor of the Earth’s Surface on the Japanese Islands According to GPS Data" Applied Sciences 12, no. 14: 7297. https://doi.org/10.3390/app12147297
APA StyleLyubushin, A. (2022). Identification of Areas of Anomalous Tremor of the Earth’s Surface on the Japanese Islands According to GPS Data. Applied Sciences, 12(14), 7297. https://doi.org/10.3390/app12147297






