Performance of Optimum Tuned PID Controller with Different Feedback Strategies on Active-Controlled Structures
Abstract
:1. Introduction
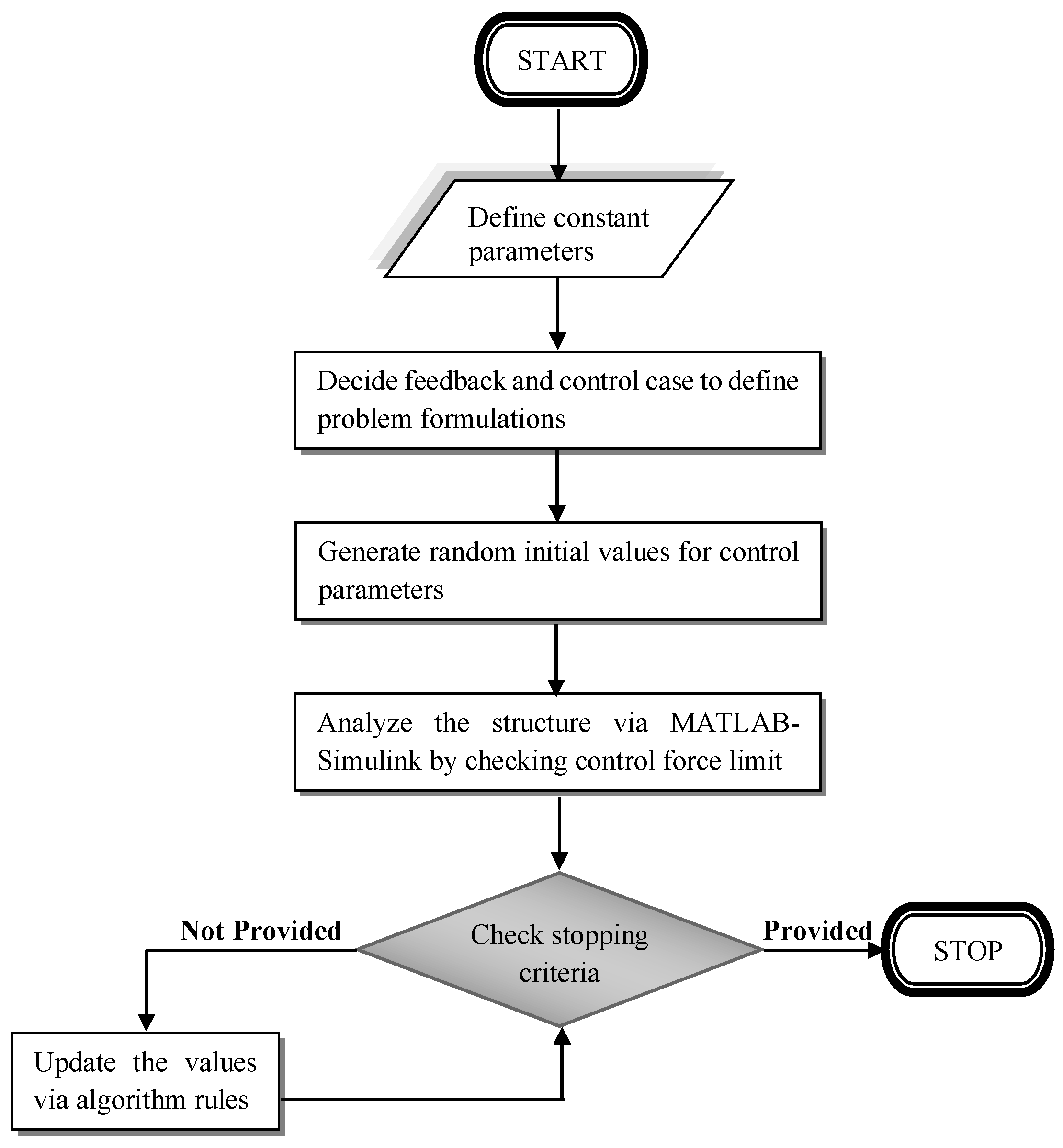
2. The Proposed Methodology
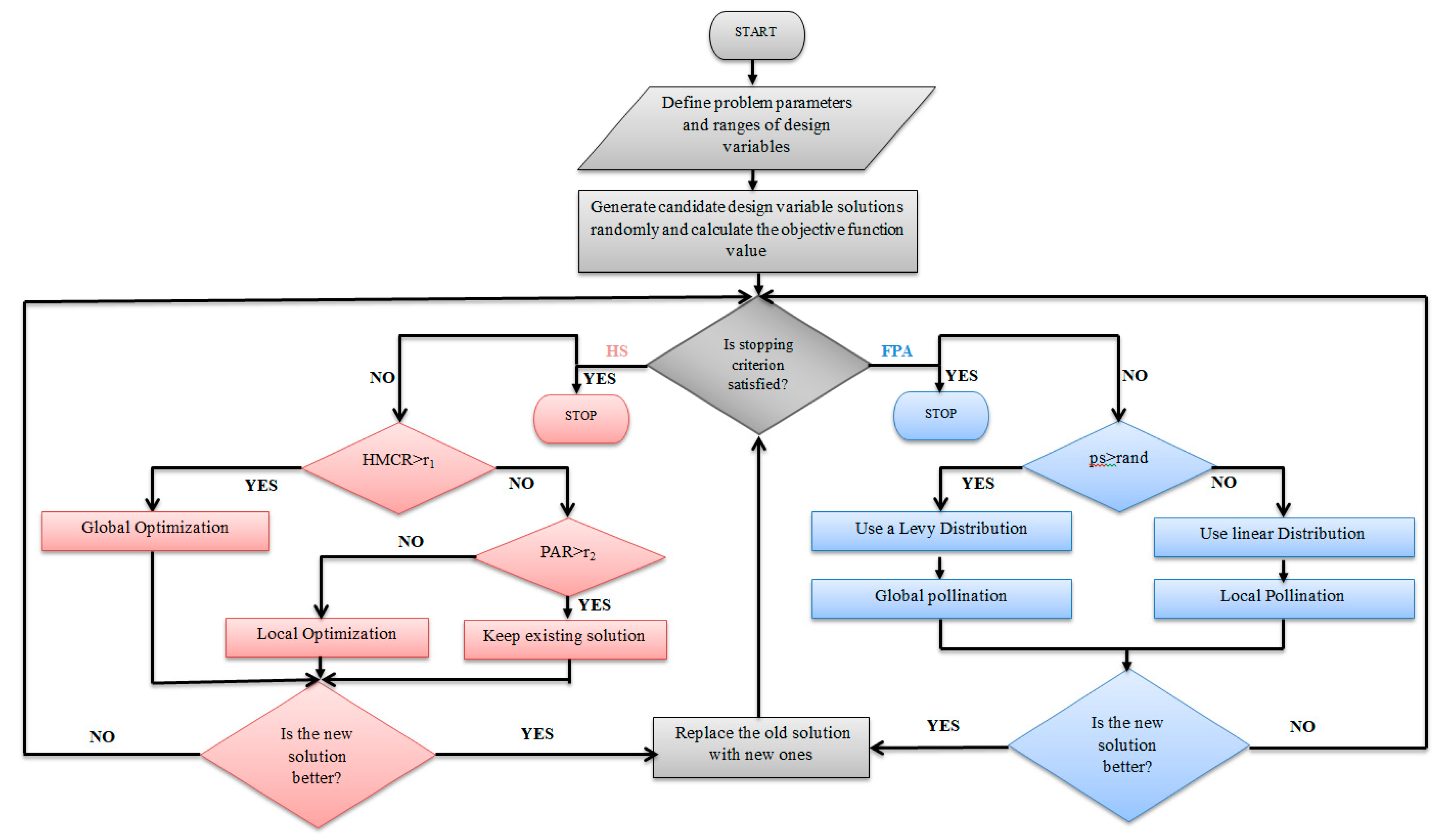
2.1. Harmony Search Algorithm
- Playing notes of any popular musical performance in their memory;
- Playing new notes similar to the notes of a known musical performance;
- Playing completely different notes.
2.2. Flower Pollination Algorithm
2.3. PID (Proportional–Integral–Derivative) Controllers
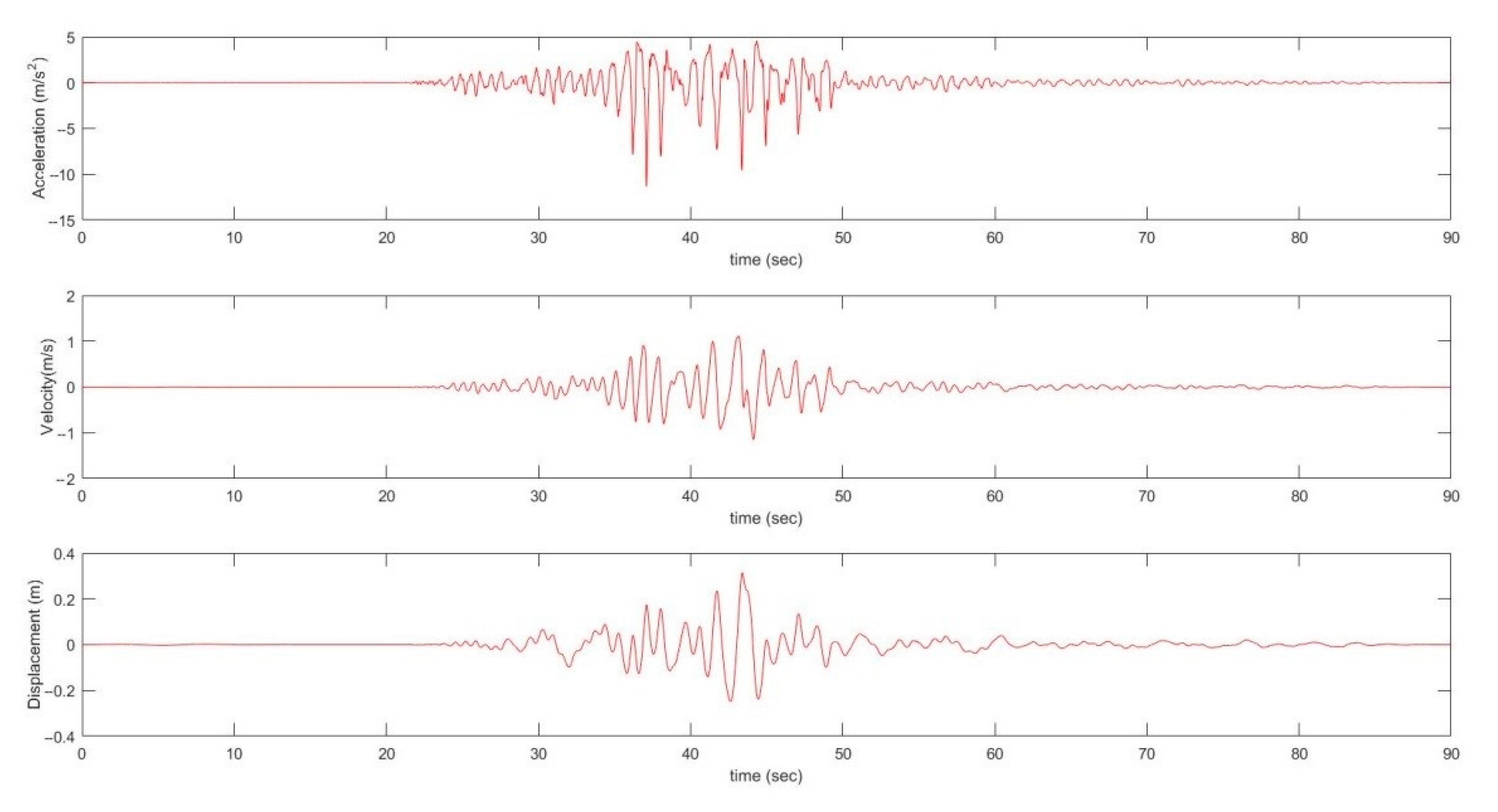
2.4. Near-Fault Ground Motions
2.5. The Equation of Motion of Active-Controlled Structures
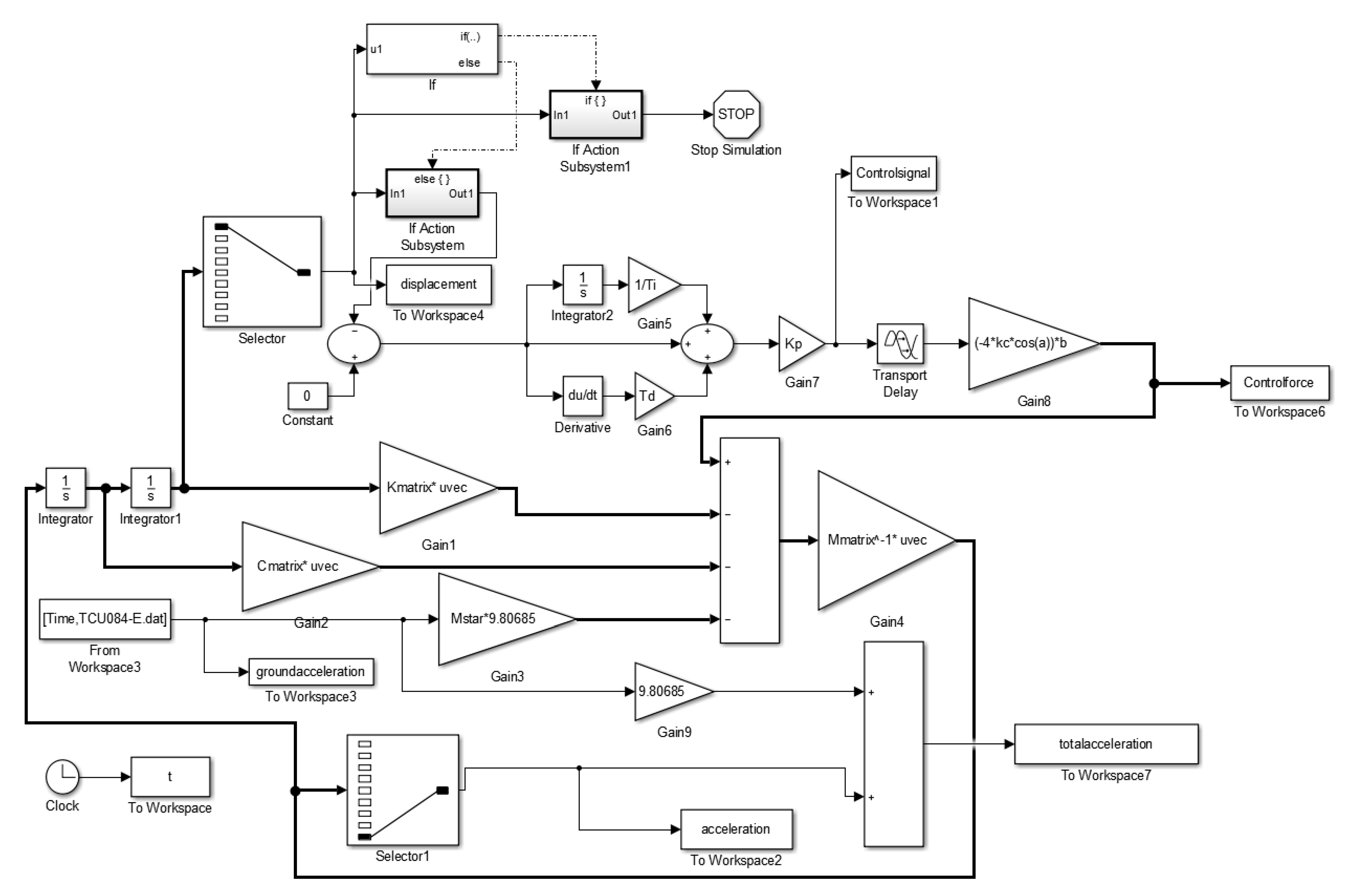
2.6. The Dynamic Analysis of the Controlled Structures
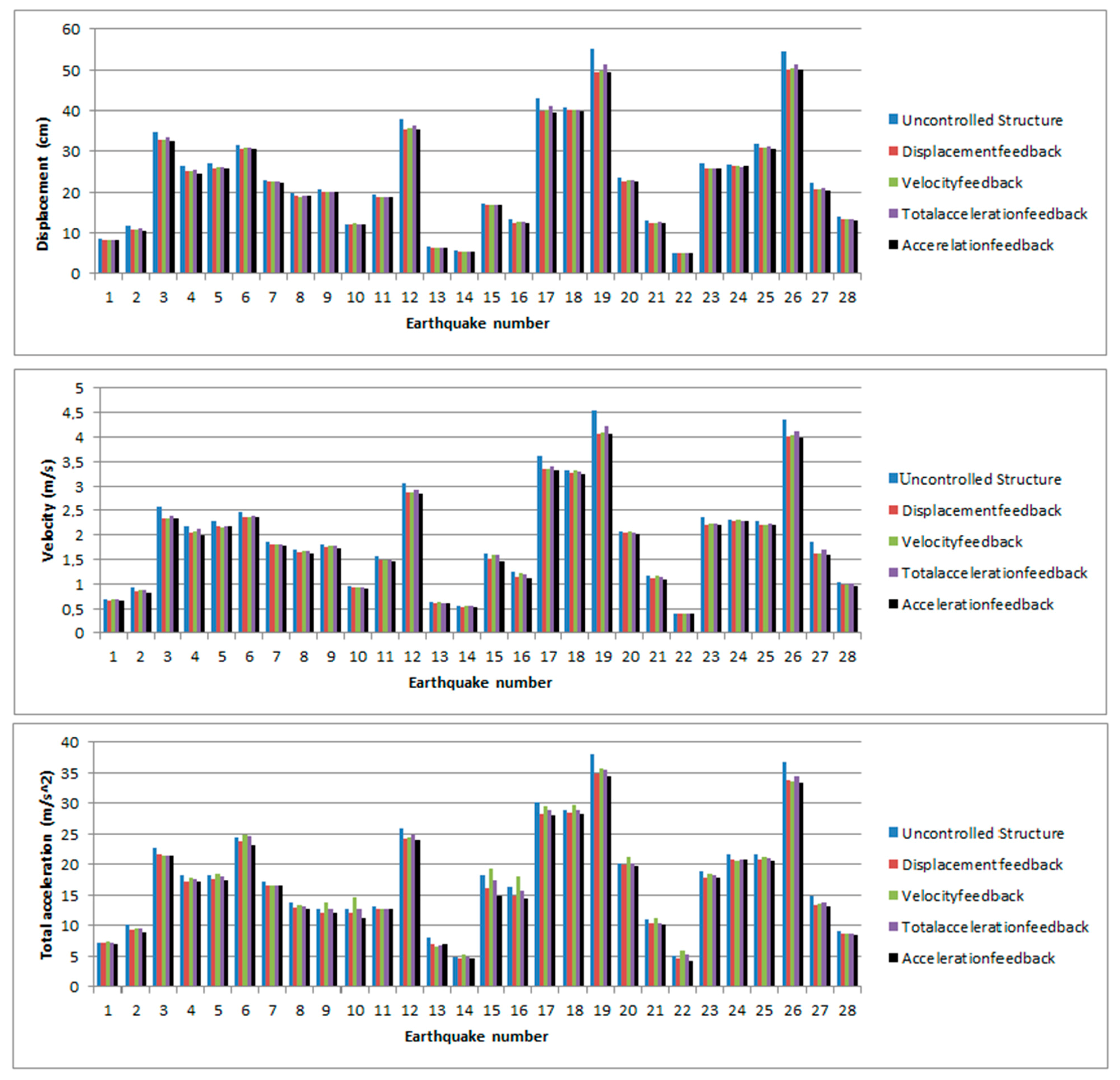
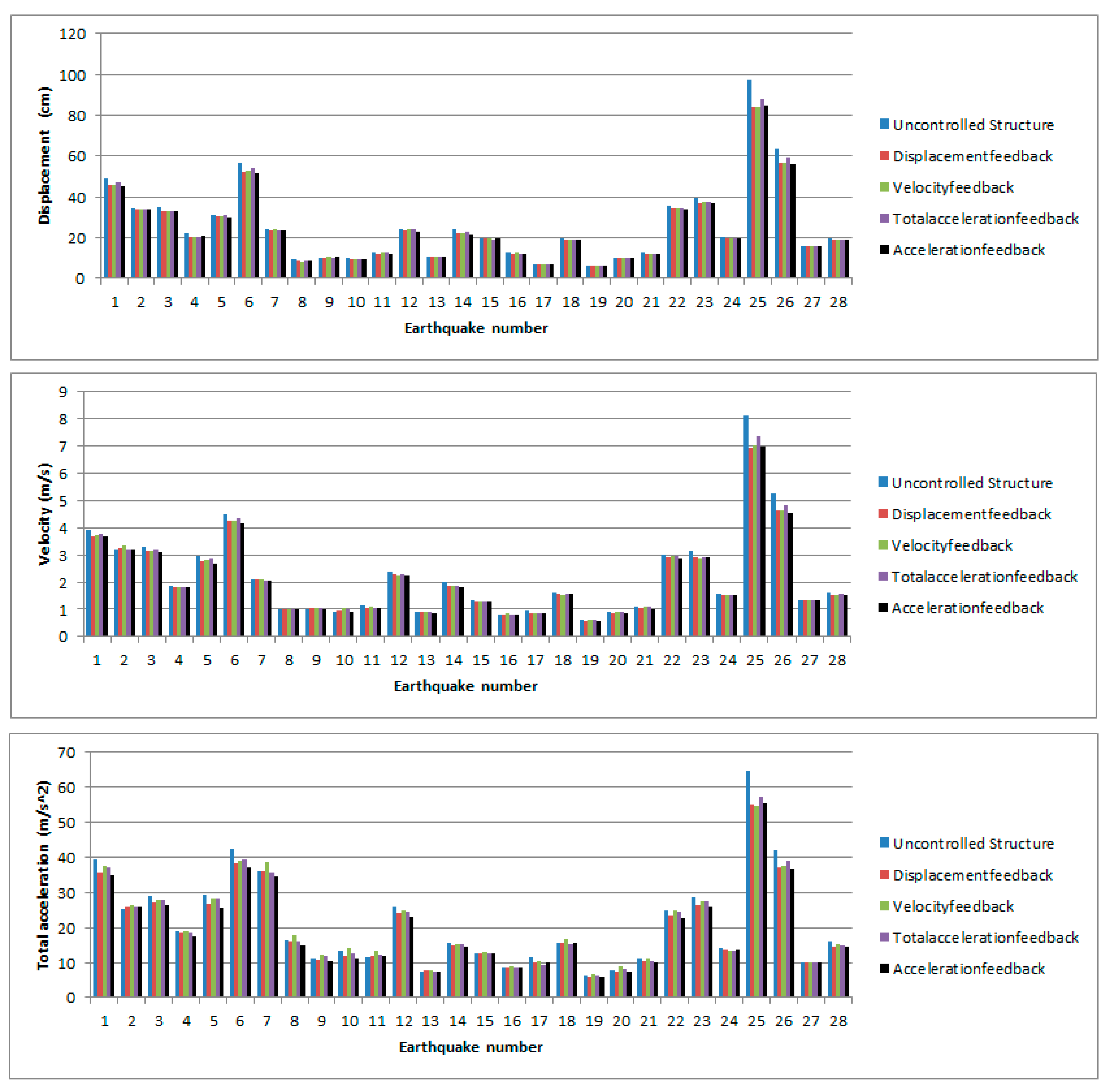
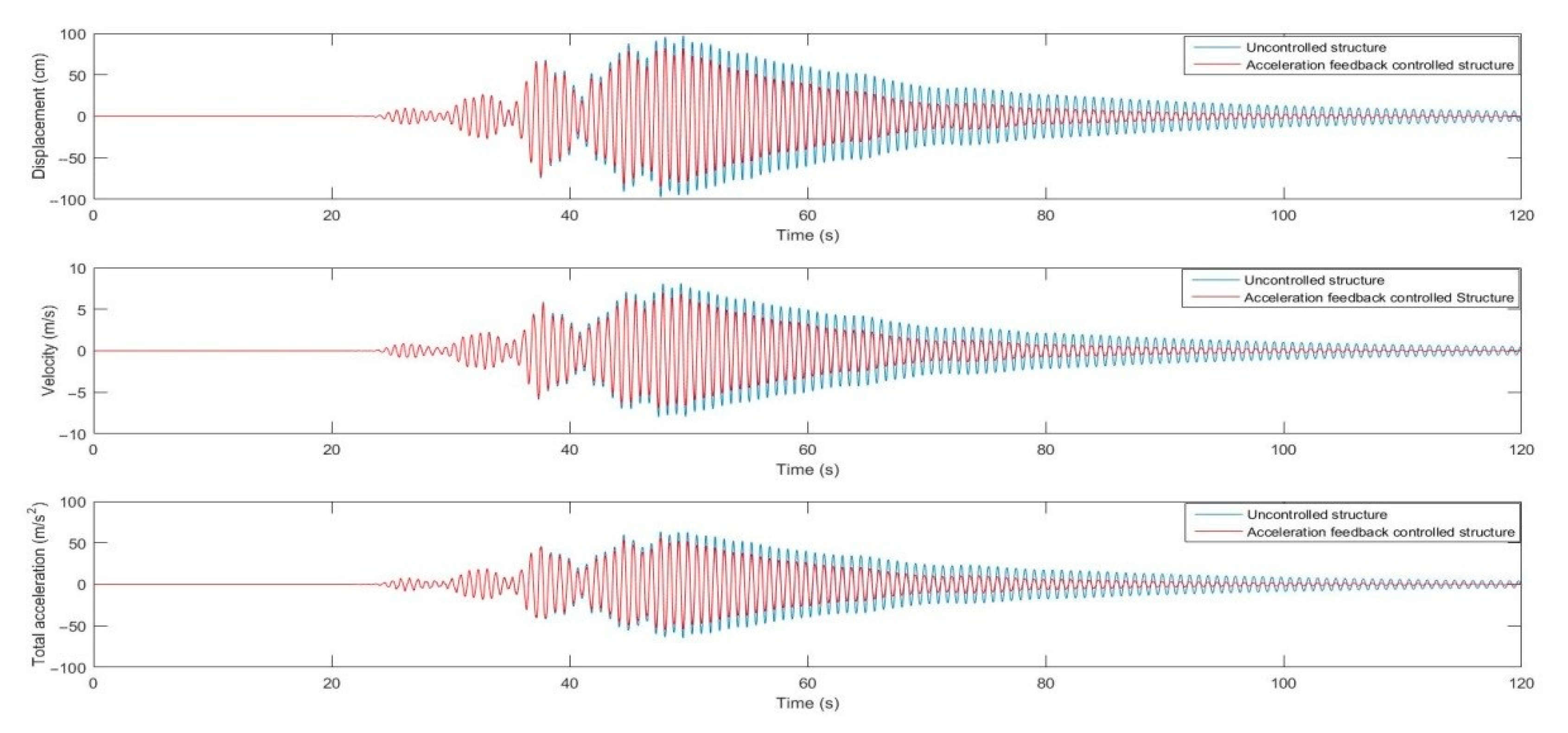
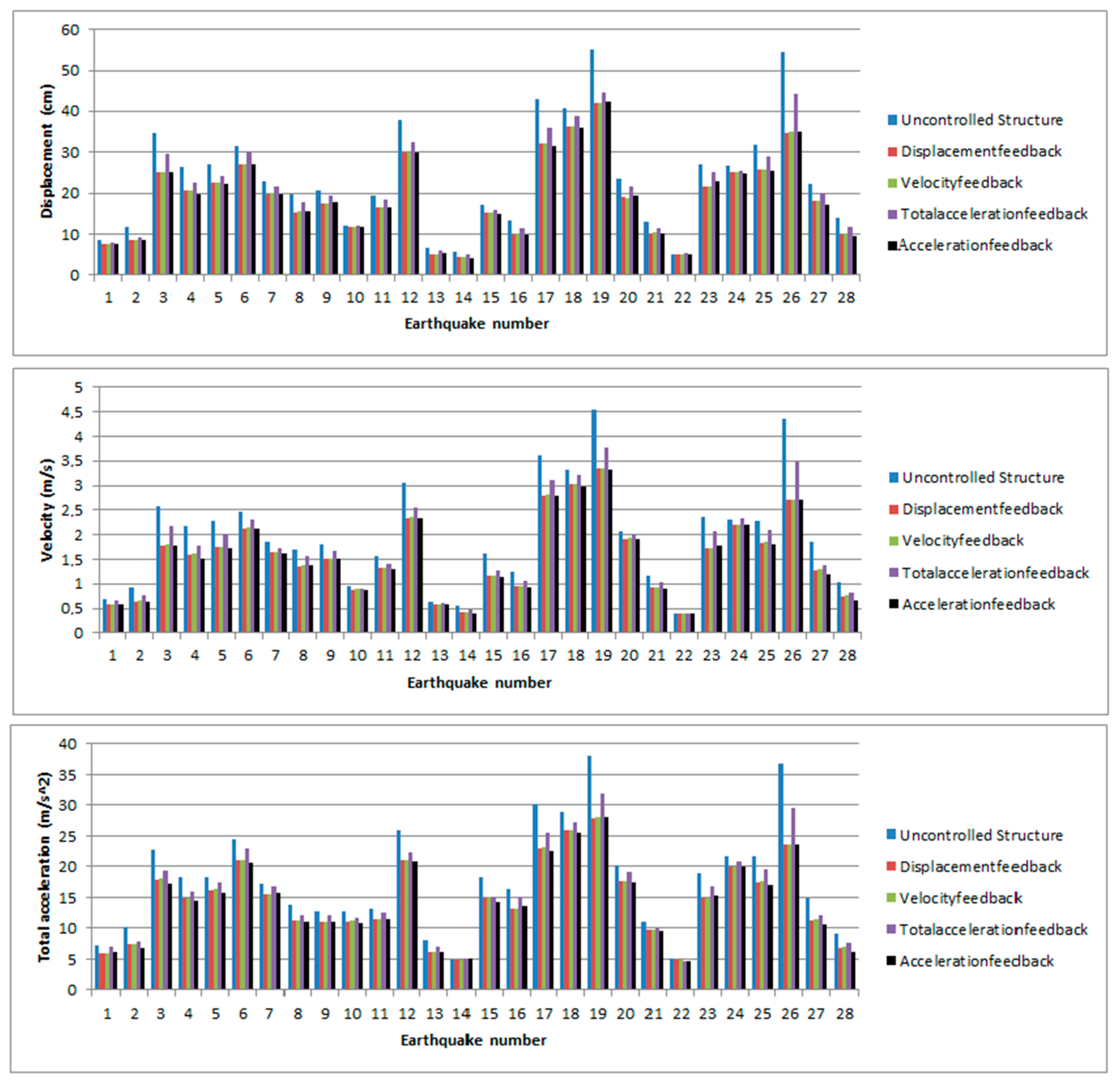
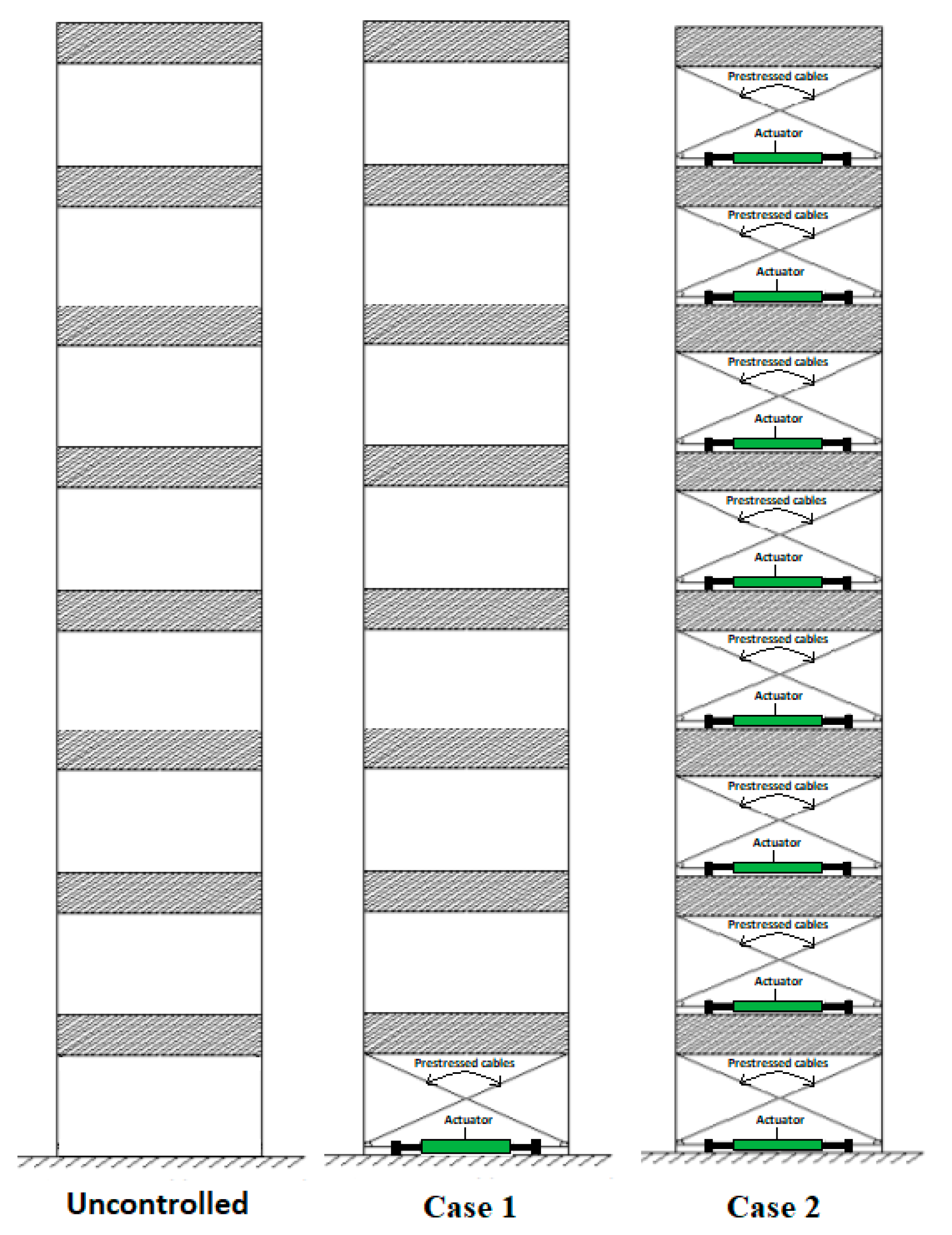
3. Numerical Examples
4. Conclusions
- (1)
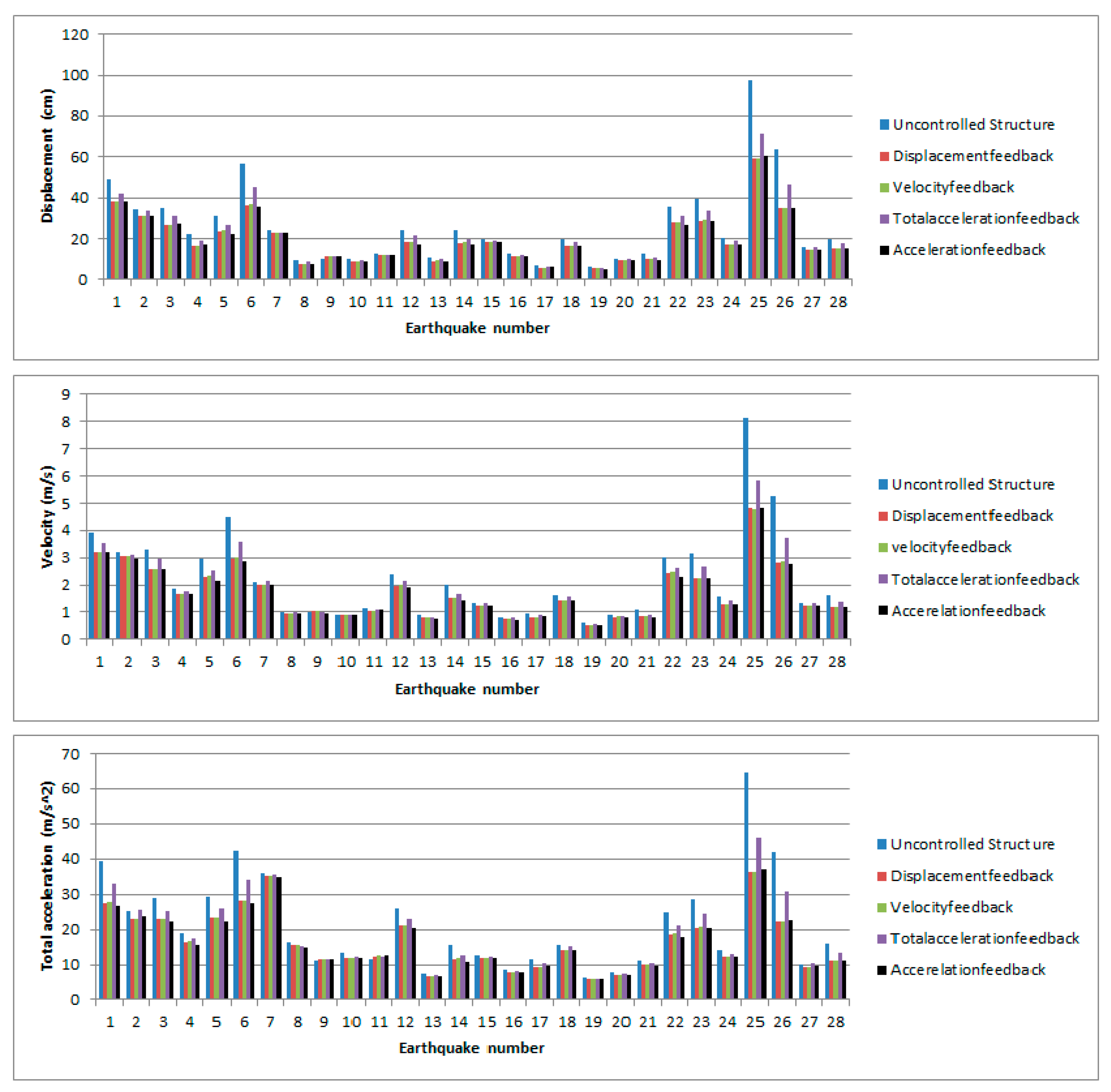
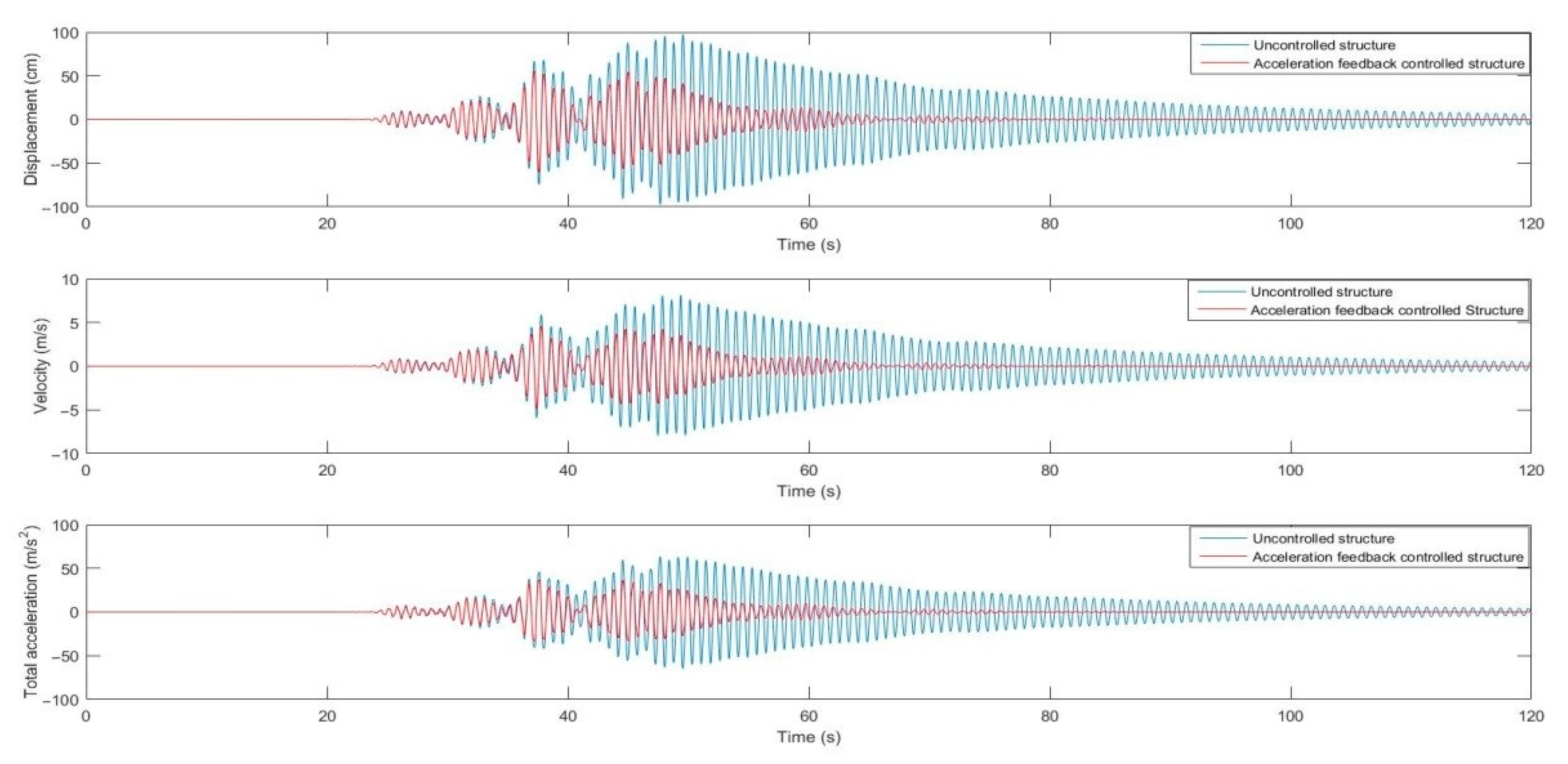
- The structural responses such as maximum displacement (from 97.53 to 60.39 cm), maximum velocity (from 8.14 to 4.85 m/s), and maximum total acceleration (from 64.72 to 37.02 m/s2) on the first (ground) floor are reduced under seismic excitations using the active control system, respectively.
- (2)
- The properties of the structure and the ground motions play an important role in the performances of the feedback strategies. Acceleration feedback (more successful in reducing structural reactions of at least 30 out of 56 ground motions) is generally the most appropriate type of feedback for numerical examples and earthquake records.
- (3)
- HS and FPA are an important solution method to determine the optimum PID parameters for different feedback strategies.
- (4)
- The position of the control system on each floor is more advantageous to keep the control force limit (3050 for Case 1 and 338 KN for Case 2) at the appropriate value in terms of applicability.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A


References
- Zuk, W. Kinetic structures. Civil Eng. 1968, 39, 62–64. [Google Scholar]
- Yang, J.N.; Samali, B. Control of tall buildings in along-wind motion. J. Struct. Eng. 1983, 109, 50–68. [Google Scholar] [CrossRef]
- Abdel-Rohman, M.; Leipholz, H.H. Active control of tall buildings. J. Struct. Eng. 1983, 109, 628–645. [Google Scholar] [CrossRef]
- Samali, B.; Yang, J.N.; Liu, S.C. Active control of seismic-excited buildings. J. Struct. Eng. 1985, 111, 2165–2180. [Google Scholar] [CrossRef]
- Chung, L.L.; Lin, R.C.; Soong, T.T.; Reinhorn, A.M. Experimental study of active control for MDOF seismic structures. J. Eng. Mech. 1989, 115, 1609–1627. [Google Scholar] [CrossRef]
- Chung, L.; Wu, L.; Jin, T. Acceleration feedback control of seismic structures. Eng. Struct. 1998, 20, 62–74. [Google Scholar] [CrossRef]
- Nigdeli, S.M. Effect of feedback on PID controlled active structures under earthquake excitations. Earthq. Struct. 2014, 6, 217–235. [Google Scholar] [CrossRef]
- Ulusoy, S.; Bekdas, G.; Nigdeli, S.M. Active structural control via metaheuristic algorithms considering soil-structure interaction. Struct. Eng. Mech. 2020, 75, 175–191. [Google Scholar]
- Ulusoy, S.; Nigdeli, S.M.; Bekdaş, G. Novel metaheuristic-based tuning of PID controllers for seismic structures and verification of robustness. J. Build. Eng. 2020, 33, 101647. [Google Scholar] [CrossRef]
- Arfiadi, Y.; Hadi, M.N.S. Passive and active control of three-dimensional buildings. Earthq. Eng. Struct. Dynam. 2000, 29, 377–396. [Google Scholar] [CrossRef]
- Bakioglu, M.; Aldemir, U. A new numerical algorithm for sub-optimal control of earthquake excited linear structures. Int. J. Numer. Methods Eng. 2001, 50, 2601–2616. [Google Scholar] [CrossRef]
- Alli, H.; Yakut, O. Fuzzy sliding-mode control of structures. Eng. Struct. 2005, 27, 277–284. [Google Scholar] [CrossRef]
- Nigdeli, S.M.; Boduroglu, B.H. Active tendon control of torsionally irregular structures under near-fault ground motion excitation. Comput. Aid. Civil Infrastruct. Eng. 2013, 28, 718–736. [Google Scholar] [CrossRef]
- Jiang, X.; Adeli, H. Neuro-genetic algorithm for non-linear active control of structures. Int. J. Numer. Methods Eng. 2008, 75, 770–786. [Google Scholar] [CrossRef]
- Yakut, O.; Alli, H. Neural based sliding-mode control with moving sliding surface for the seismic isolation of structures. J. Vib. Control. 2011, 17, 2103–2116. [Google Scholar] [CrossRef]
- Guclu, R. Sliding mode and PID control of a structural system against earthquake. Math. Comput. Model. 2006, 44, 210–217. [Google Scholar] [CrossRef]
- Ghaffarzadeh, H.; Younespour, A. Active tendons control of structures using block pulse functions. Struct. Control. Health Monit. 2014, 21, 1453–1464. [Google Scholar] [CrossRef]
- Kayabekir, A.E.; Bekdaş, G.; Nigdeli, S.M.; Geem, Z.W. Optimum design of PID controlled active tuned mass damper via modified harmony search. Appl. Sci. 2020, 10, 2976. [Google Scholar] [CrossRef]
- Geem, Z.W.; Kim, J.H.; Loganathan, G.V. A new heuristic optimization algorithm: Harmony search. Simulation 2001, 76, 60–68. [Google Scholar] [CrossRef]
- Yang, X.S. Flower pollination algorithm for global optimization. In Proceedings of the International Conference on Unconventional Computing and Natural Computation, Orleans, France, 3–7 September 2012; Springer: Berlin/Heidelberg, Germany, 2012; pp. 240–249. [Google Scholar]
- MathWorks Inc. MATLAB R2015b; MathWorks Inc.: Natick, MA, USA, 2015. [Google Scholar]
- Federal Emergency Management Agency. FEMA P-695. In Quantification of Building Seismic Performance Factors; Federal Emergency Management Agency: Washington, DC, USA, 2009. [Google Scholar]
- Chopra, A.K.; Chintanapakdee, C. Comparing response of SDF systems to near-fault and far-fault earthquake motions in the context of spectral regions. Earthq. Eng. Struct. Dyn. 2001, 30, 1769–1789. [Google Scholar] [CrossRef]
- Pacific Earthquake Engineering Research Center (PEER NGA DATABASE). Available online: http://peer.berkeley.edu/nga (accessed on 20 May 2020).
- Pnevmatikos, N.G.; Gantes, C.J. Control strategy for mitigating the response of structures subjected to earthquake Actions. Eng. Struct. 2010, 32, 3616–3628. [Google Scholar] [CrossRef]
| Number. | Name | Record | Year | Component 1 | Component 2 | Magnitude |
|---|---|---|---|---|---|---|
| 1–2 | Northridge-01 | LA—Sepulveda VA | 1994 | 0637-270 | 0637-360 | 6.7 |
| 3–4 | Loma Prieta | Bran | 1989 | BRN000 | BRN090 | 6.9 |
| 5–6 | Loma Prieta | Corralitos | 1989 | CLS000 | CLS090 | 6.9 |
| 7–8 | Cape Mendocino | Cape Mendocino | 1992 | CPM000 | CPM090 | 7.0 |
| 9–10 | Gazli, USSR | Karakyr | 1976 | GAZ000 | GAZ090 | 6.8 |
| 11–12 | Imperial Valley-06 | Bonds Corner | 1979 | H-BCR140 | H-BCR230 | 6.5 |
| 13–14 | Imperial Valley-06 | Chıhuahua | 1979 | H-CHI012 | H-CHI282 | 6.5 |
| 15–16 | Denali, Alaska | TAPS Pump Sta. #10 | 2002 | PS10047 | PS10317 | 7.9 |
| 17–18 | Nahanni, Canada | Site 1 | 1985 | S1010 | S1280 | 6.8 |
| 19–20 | Nahanni, Canada | Site 2 | 1985 | S2240 | S2330 | 6.8 |
| 21–22 | Northridge-01 | Northridge—Saticoy | 1994 | STC090 | STC180 | 6.7 |
| 23–24 | Chi-Chi, Taiwan | TCU067 | 1999 | TCU067-E | TCU084-N | 7.6 |
| 25–26 | Chi-Chi, Taiwan | TCU084 | 1999 | TCU084-E | TCU084-N | 7.6 |
| 27–28 | Kocaeli, Turkey | Yarimca | 1999 | YPT060 | YPT330 | 7.5 |
| Number | Name | Record | Year | Component 1 | Component 2 | Magnitude |
|---|---|---|---|---|---|---|
| 1–2 | Irpinia, Italy-01 | Sturno | 1980 | A-STU000 | A-STU270 | 6.9 |
| 3–4 | Superstition Hills-02 | Parachute Test Site | 1987 | B-PTS225 | B-PTS315 | 6.5 |
| 5–6 | Duzce, Turkey | Duzce | 1999 | DZC180 | DZC270 | 7.1 |
| 7–8 | Erzican, Turkey | Erzican | 1992 | ERZ-EW | ERZ-NS | 6.7 |
| 9–10 | Imperial Valley-06 | El Centro Array #6 | 1979 | H-E06140 | H-E06230 | 6.5 |
| 11–12 | Imperial Valley-06 | El Centro Array #7 | 1979 | H-E06140 | H-E07230 | 6.5 |
| 13–14 | Kocaeli, Turkey | Izmit | 1999 | IZT090 | IZT180 | 7.5 |
| 15–16 | Landers | Lucerne | 1992 | LCN260 | LCN345 | 7.3 |
| 17–18 | Cape Mendocino | Petrolia | 1992 | PET000 | PET090 | 7.0 |
| 19–20 | Northridge-01 | 01 Rinaldi Receiving Sta | 1994 | RRS228 | RRS318 | 6.7 |
| 21–22 | Loma Prieta | Saratoga—Aloha | 1989 | STG000 | STG090 | 6.9 |
| 23–24 | Northridge-01 | 01 Sylmar—Olive View | 1994 | SYL090 | SYL360 | 6.7 |
| 25–26 | Chi-Chi, Taiwan | TCU065 | 1999 | TCU065-E | TCU065-N | 7.6 |
| 27–28 | Chi-Chi, Taiwan | TCU102 | 1999 | TCU102-E | TCU102-N | 7.6 |
| Story | Mi (t) | Ki (KN/m) | Ci (KNs/m) |
|---|---|---|---|
| 1–8 | 346.6 | 680,000 | 734 |
| Case 1 | Case 2 | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Feedback | Kp | Td | Ti | xr | xtop | Kp | Td | Ti | xr | xtop | |
| Displacement | HS | −4.5516 | 0.3865 | −2.4710 | 0.1562 | 0.8384 | −0.0738 | 4.8797 | −3.8628 | 0.1161 | 0.5930 |
| FPA | −3.4661 | 0.5286 | −3.4663 | 0.1562 | 0.8360 | −0.2285 | 1.5507 | 1.3225 | 0.1165 | 0.5948 | |
| Velocity | HS | −1.7584 | −0.0453 | −2.0845 | 1.2073 | 7.0157 | −0.3618 | −0.0103 | 5.000 | 0.7644 | 4.8030 |
| FPA | −1.6276 | −0.0502 | 1.4383 | 1.2061 | 7.1166 | −0.3620 | −0.0107 | 5.000 | 0.7640 | 4.8025 | |
| Acceleration | HS | −0.0408 | −0.0033 | 0.0232 | 11.706 | 55.678 | −0.0146 | 0.0047 | 0.0432 | 8.7490 | 34.218 |
| FPA | −0.0880 | −0.0014 | 0.0549 | 11.729 | 56.481 | −0.0148 | 0.0045 | 0.0433 | 8.7342 | 34.142 | |
| Total acceleration | HS | 0.0575 | −0.0025 | −0.0551 | 16.500 | 57.409 | −0.0075 | 0.0112 | 0.0489 | 15.450 | 46.829 |
| FPA | 0.0734 | −0.0018 | −3.4079 | 16.781 | 63.912 | 0.0124 | 0.0111 | −1.9245 | 16.383 | 63.400 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ulusoy, S.; Bekdaş, G.; Nigdeli, S.M.; Kim, S.; Geem, Z.W. Performance of Optimum Tuned PID Controller with Different Feedback Strategies on Active-Controlled Structures. Appl. Sci. 2021, 11, 1682. https://doi.org/10.3390/app11041682
Ulusoy S, Bekdaş G, Nigdeli SM, Kim S, Geem ZW. Performance of Optimum Tuned PID Controller with Different Feedback Strategies on Active-Controlled Structures. Applied Sciences. 2021; 11(4):1682. https://doi.org/10.3390/app11041682
Chicago/Turabian StyleUlusoy, Serdar, Gebrail Bekdaş, Sinan Melih Nigdeli, Sanghun Kim, and Zong Woo Geem. 2021. "Performance of Optimum Tuned PID Controller with Different Feedback Strategies on Active-Controlled Structures" Applied Sciences 11, no. 4: 1682. https://doi.org/10.3390/app11041682
APA StyleUlusoy, S., Bekdaş, G., Nigdeli, S. M., Kim, S., & Geem, Z. W. (2021). Performance of Optimum Tuned PID Controller with Different Feedback Strategies on Active-Controlled Structures. Applied Sciences, 11(4), 1682. https://doi.org/10.3390/app11041682










