Prediction of Effective Thermal Conductivities of Four-Directional Carbon/Carbon Composites by Unit Cells with Different Sizes
Abstract
1. Introduction
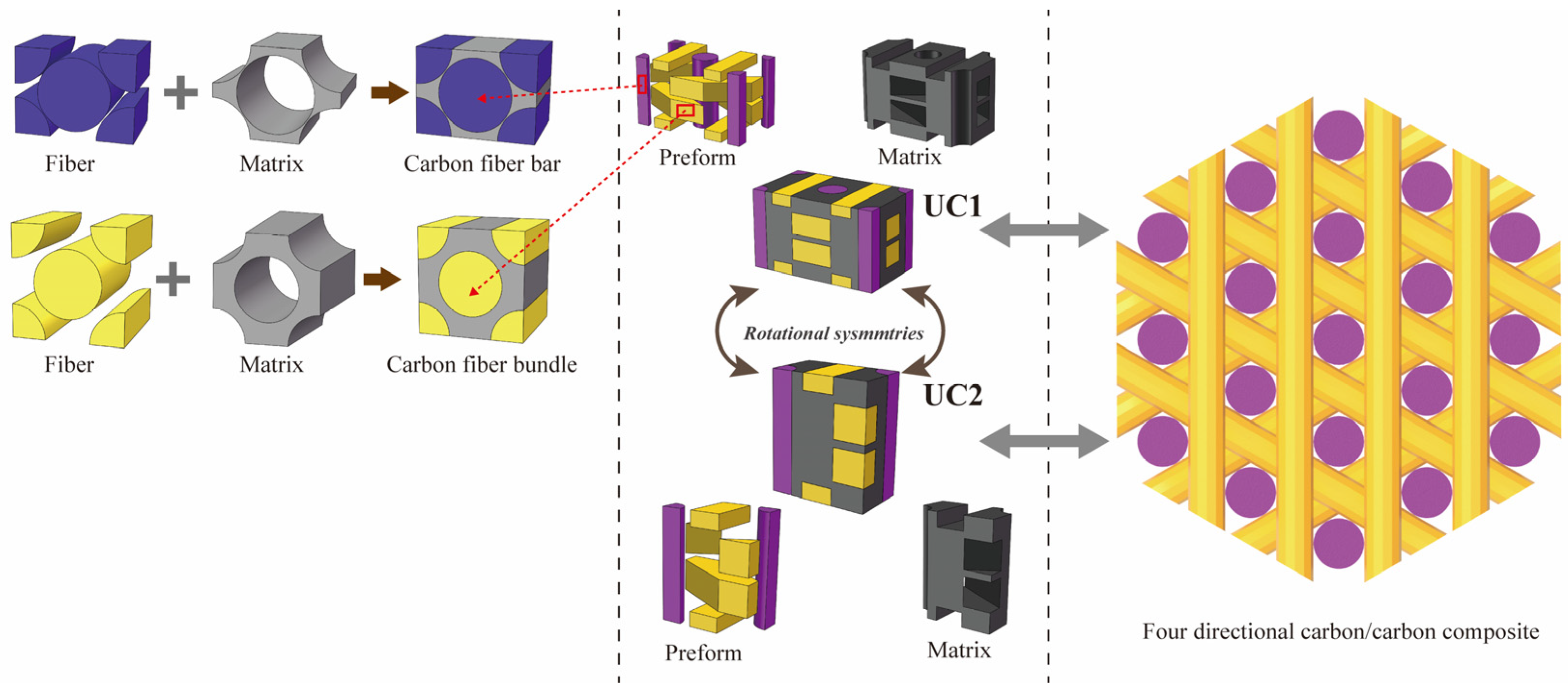
2. Derivation of Boundary Conditions of 4D C/C Composite
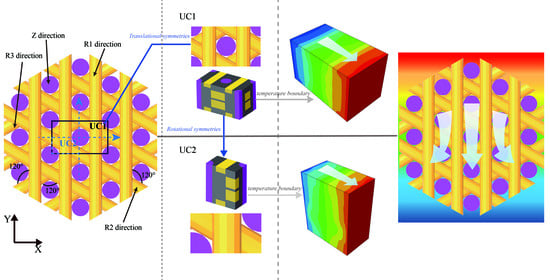
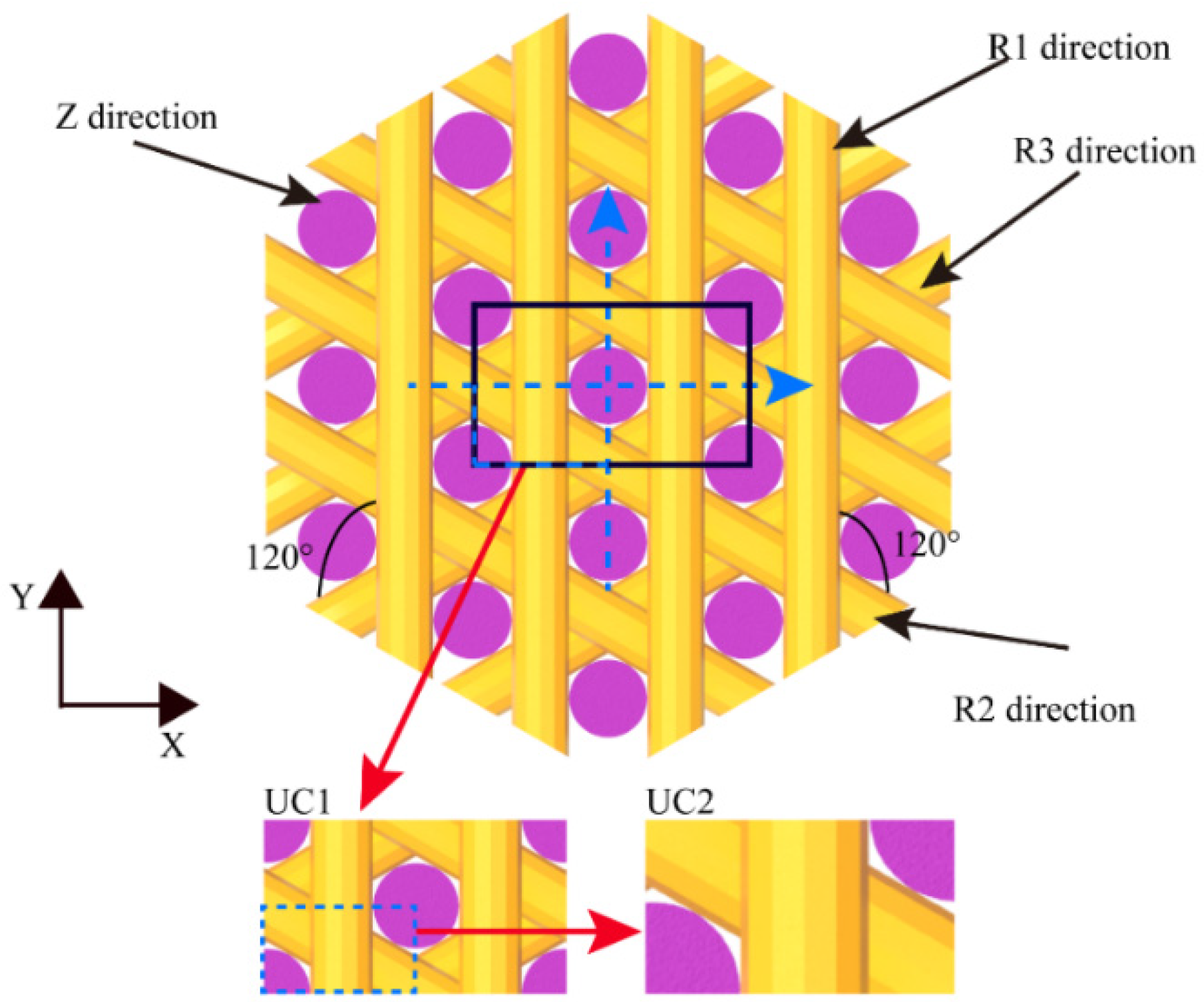
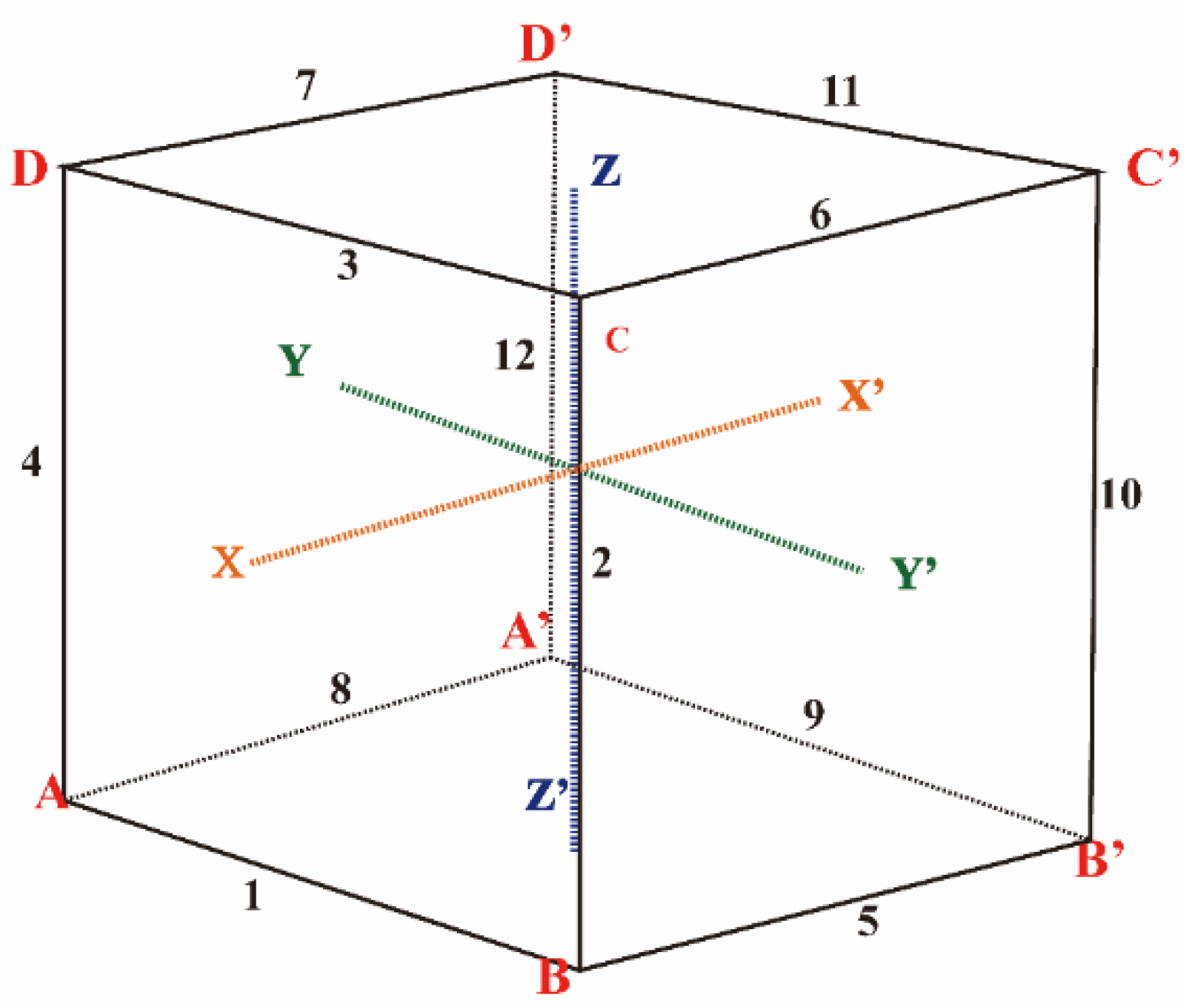
2.1. The Temperature and Heat Flux of Unit Cells Formed by 180° Rotational Symmetry
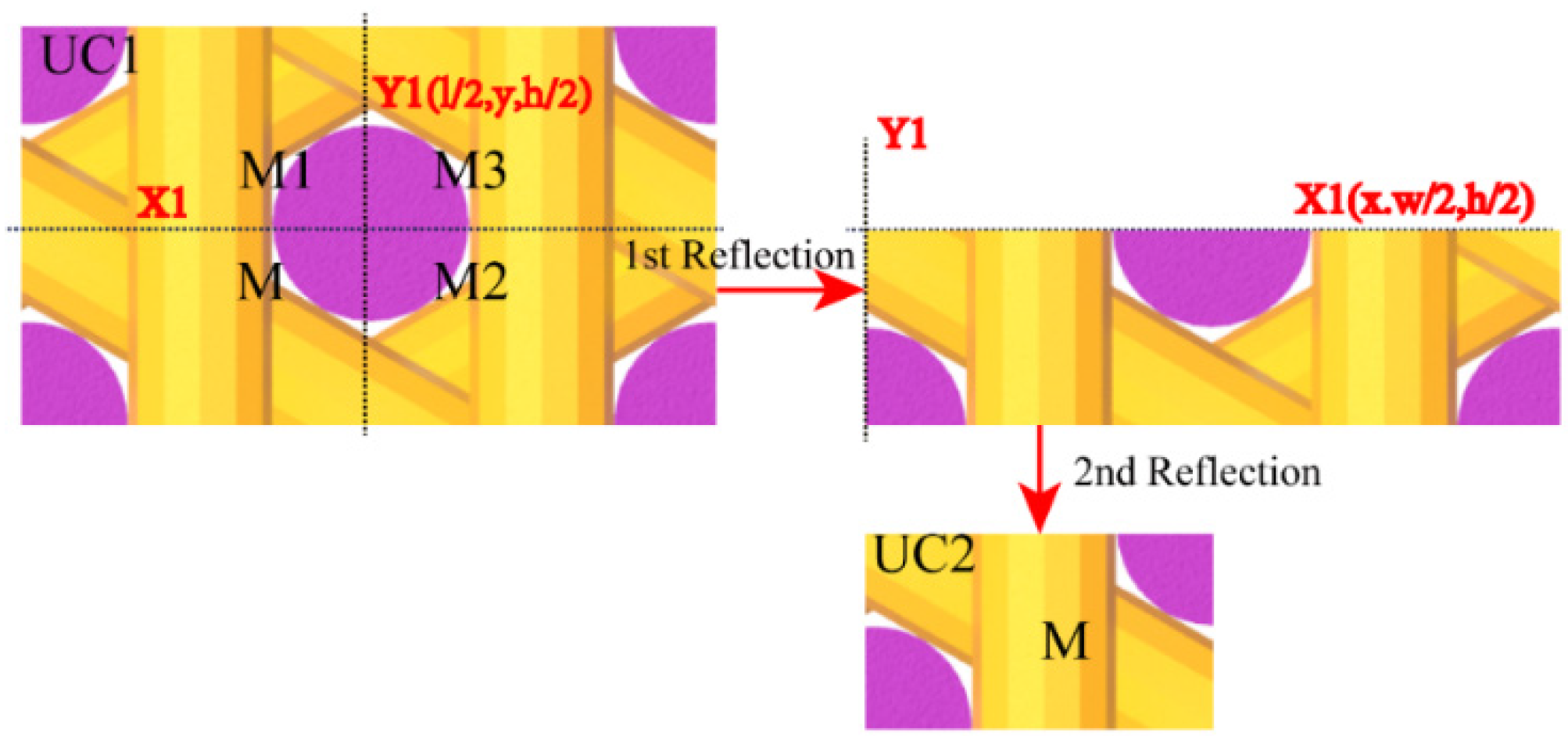
2.2. The Temperature Boundary Conditions of UC1
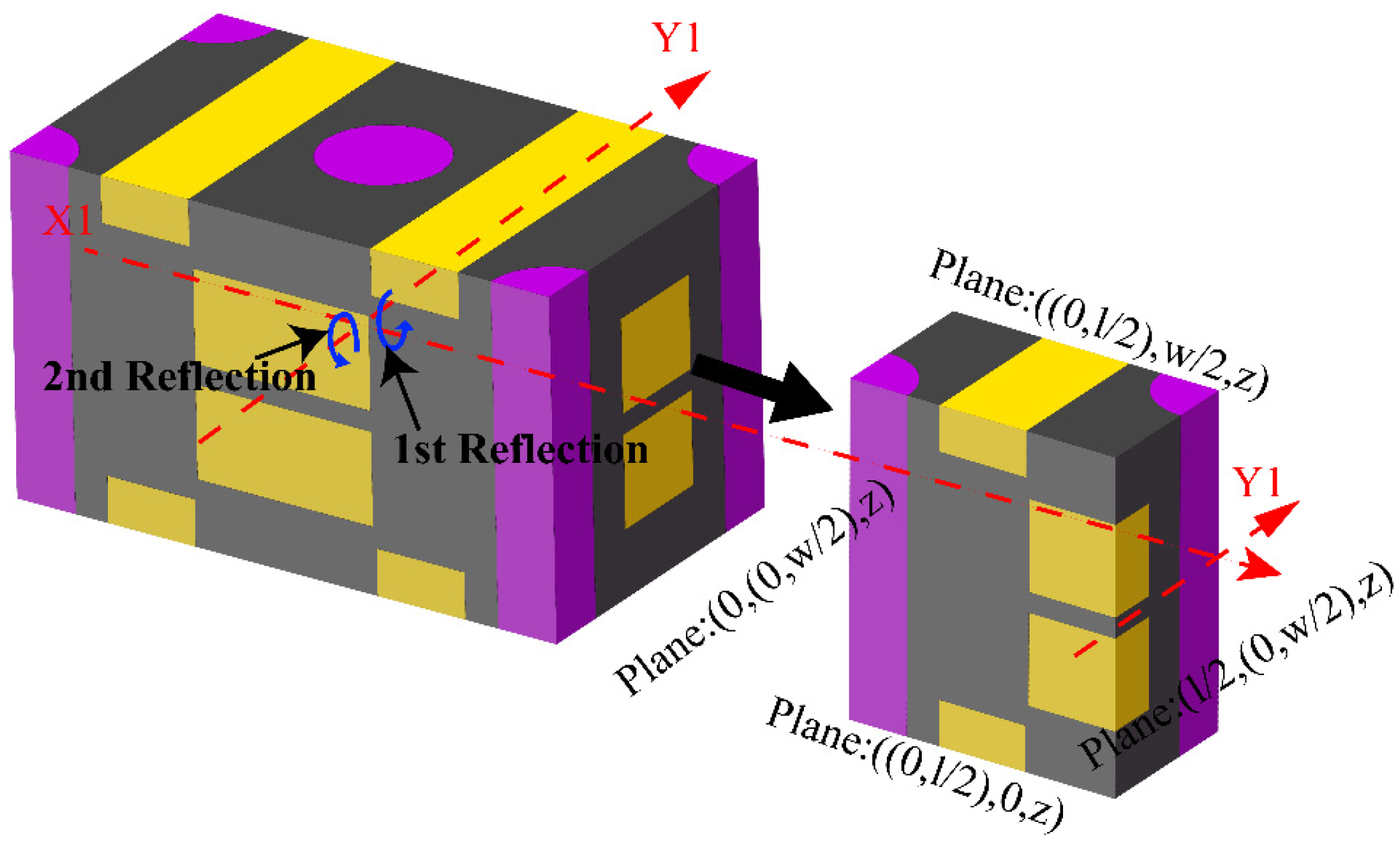
2.3. The Temperature Boundary Conditions of UC2
2.3.1. Boundary Conditions for Calculating of UC2
Boundary Conditions of and
Boundary Conditions of and
2.3.2. Boundary Conditions for Calculating of UC2
2.3.3. Boundary Conditions for Calculating of UC2
3. Finite Element Analyses
3.1. Governing Equation of the Thermal Conduction
3.2. Material Properties
3.3. Thermal Conductivities of Carbon Fiber Rods and Carbon Fiber Bundles
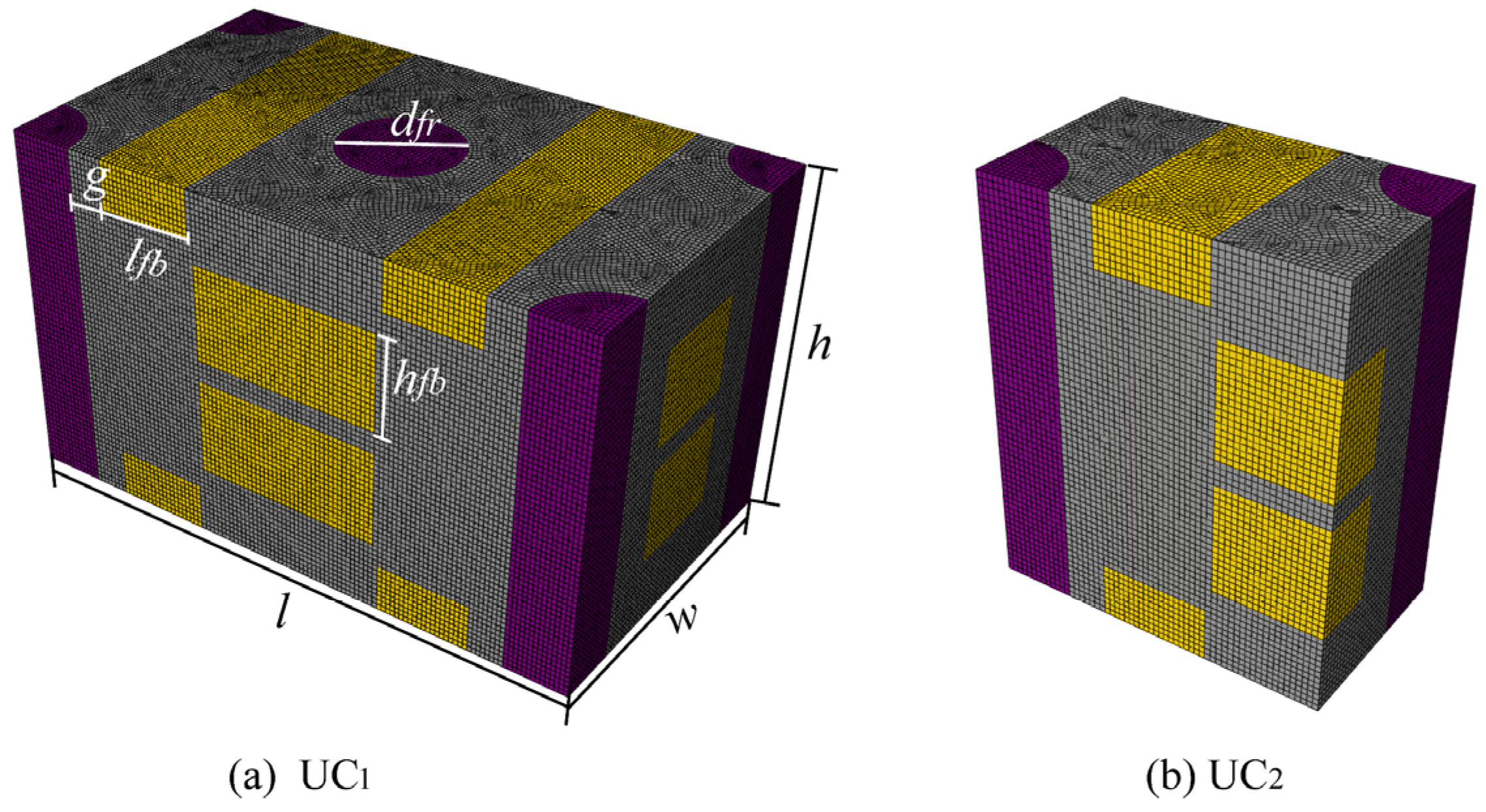
3.4. Domain Discretization of UC1 and UC2
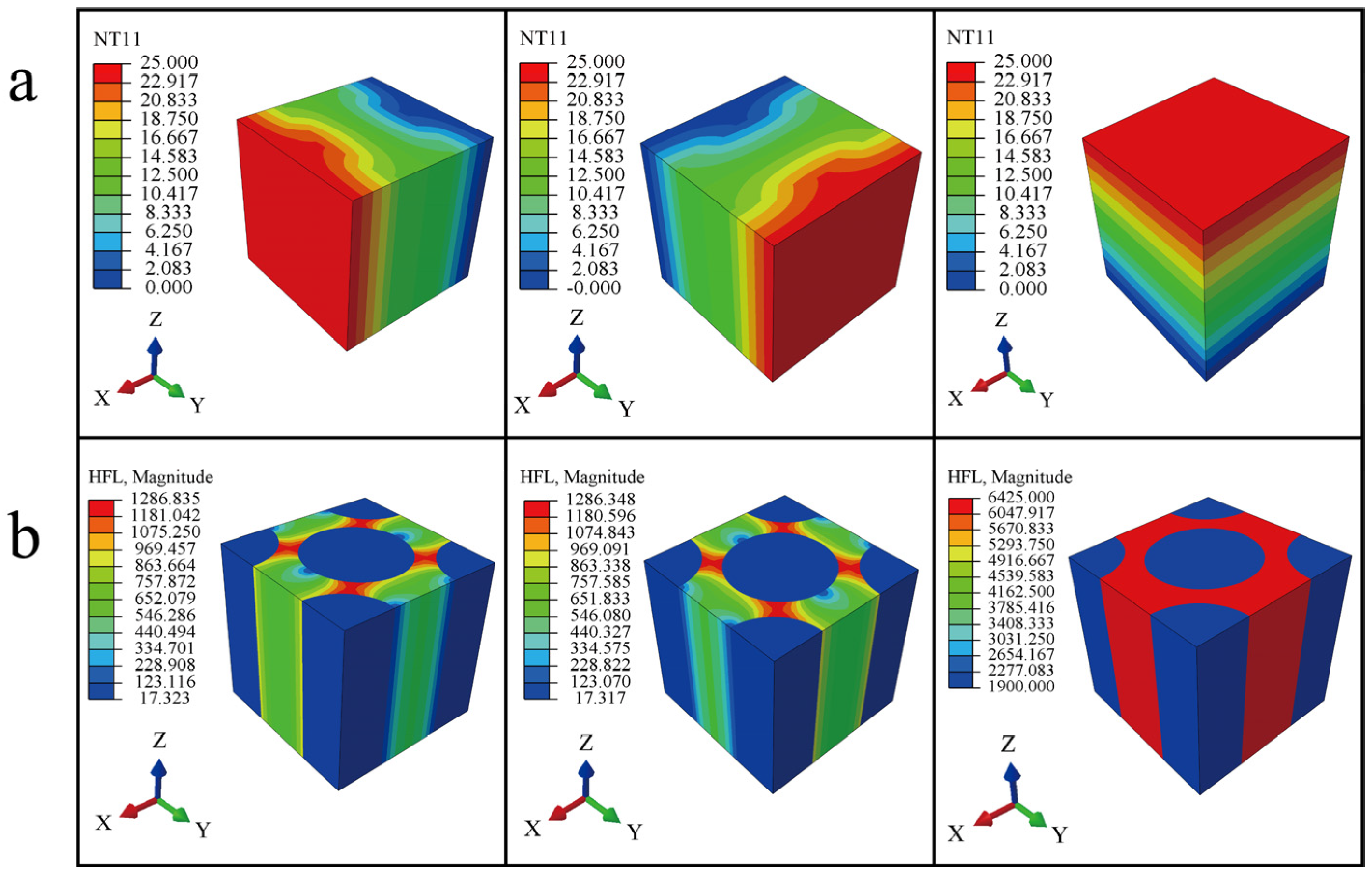
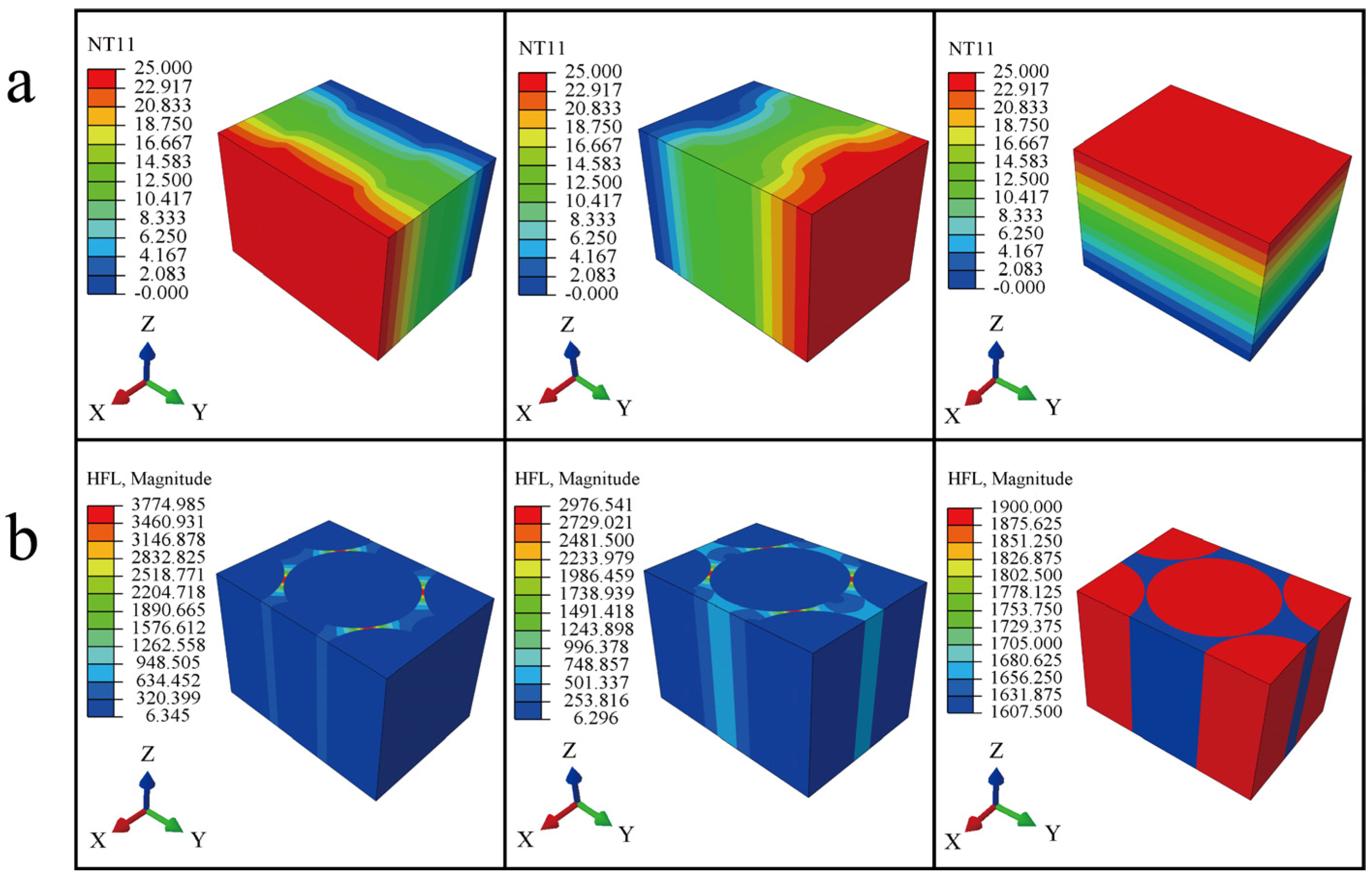
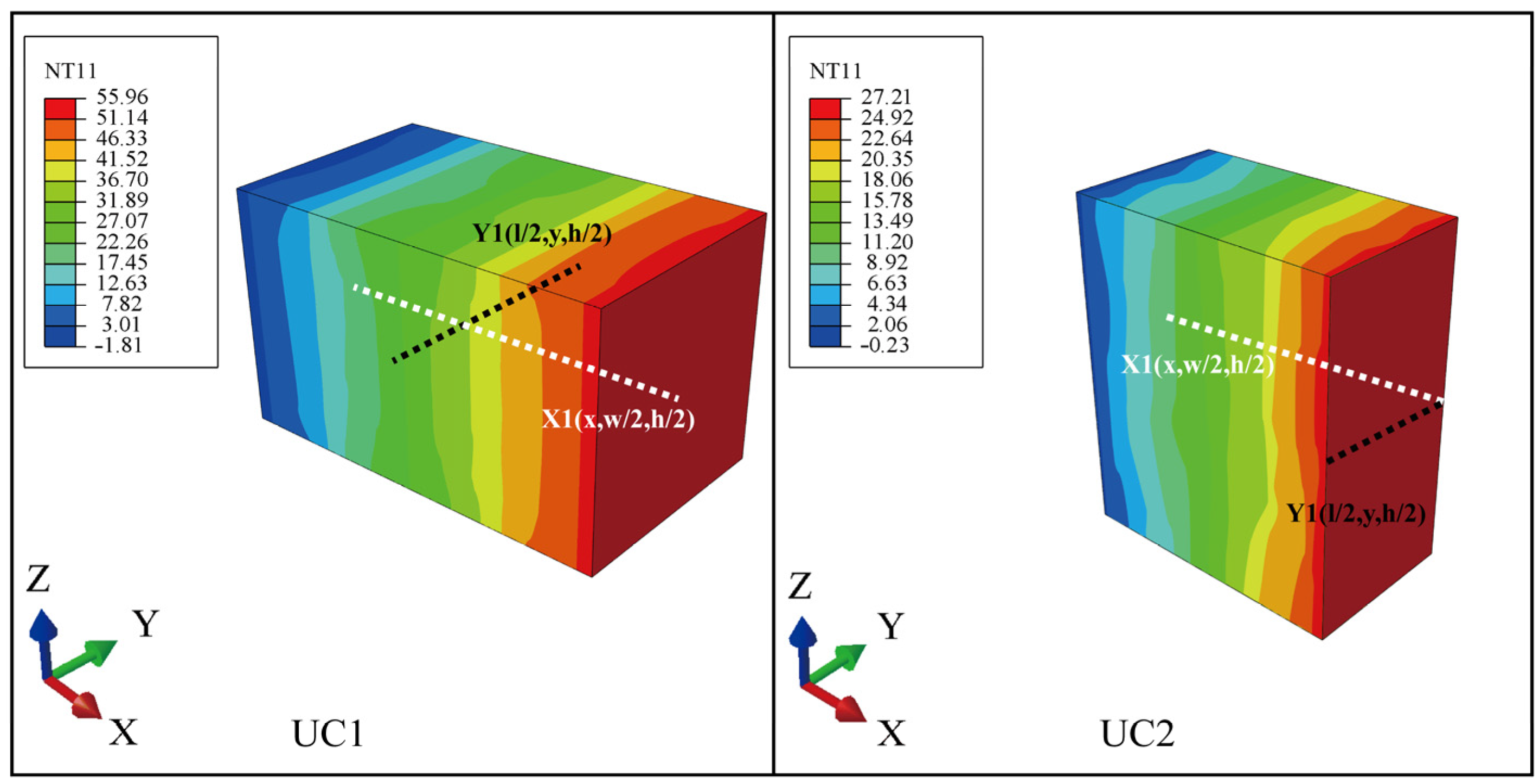
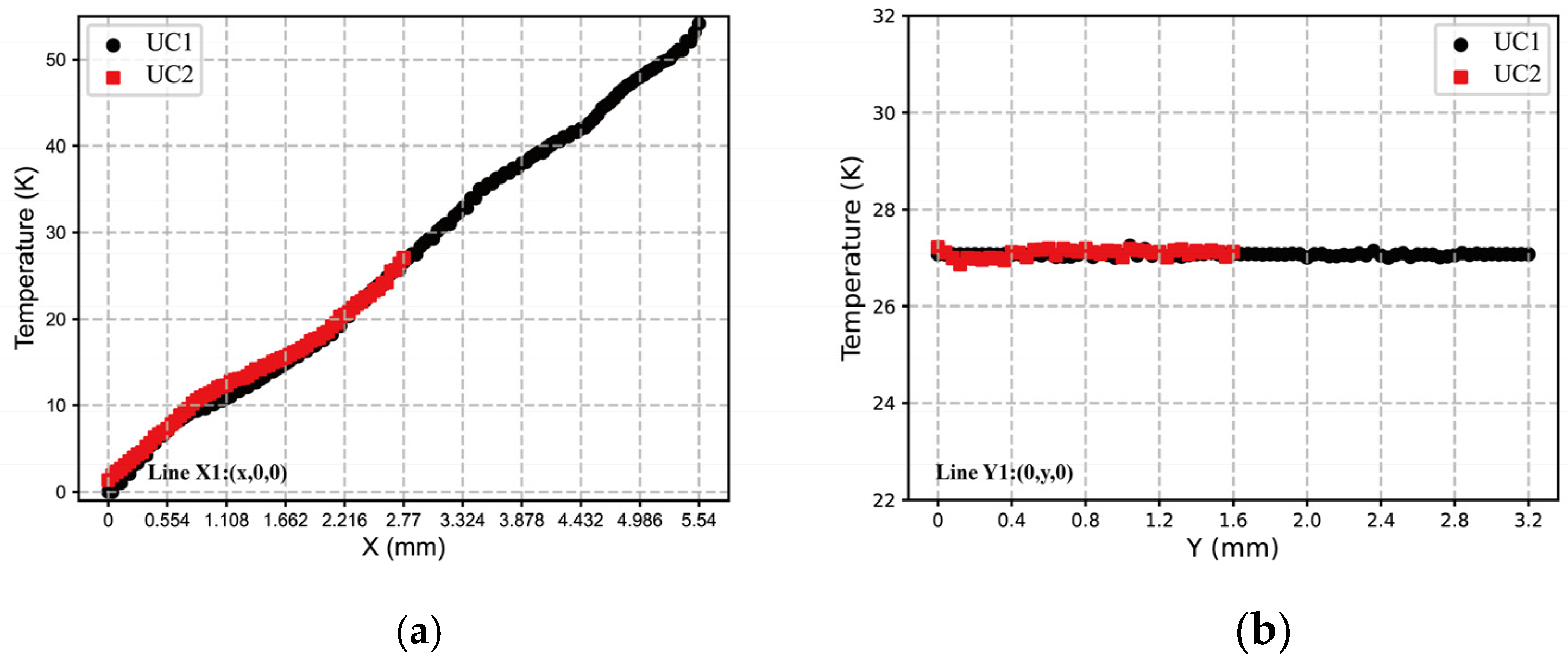
4. Results and Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Hui, W.H.; Bao, F.T.; Wei, X.G.; Liu, Y. Analysis of Ablative Performance of C/C Composite Throat Containing Defects Based on X-ray 3D Reconstruction in a Solid Rocket Motor. Int. J. Turbo. Jet-Engines 2015, 32, 351–359. [Google Scholar] [CrossRef]
- Ozcan, S.; Tezcan, J.; Filip, P. Microstructure and elastic properties of individual components of C/C composites. Carbon 2009, 47, 3403–3414. [Google Scholar] [CrossRef]
- Zaman, W.; Li, K.-Z.; Li, W.; Zaman, H.; Ali, K. Flexural strength and thermal expansion of 4D carbon/carbon composites after flexural fatigue loading. N. Carbon Mater. 2014, 29, 169–175. [Google Scholar] [CrossRef]
- Li, B.-L.; Guo, J.-G.; Xun, B.; Xu, H.-T.; Dong, Z.-J.; Li, X.-K. Preparation, microstructure and properties of three-dimensional carbon/carbon composites with high thermal conductivity. N. Carbon Mater. 2020, 35, 567–575. [Google Scholar] [CrossRef]
- Mierzwiczak, M.; Kolodziej, J.A. The inverse determination of volume fraction of fibres in reinforced composite with imperfect thermal contact between constituents. J. Theor. Appl. Mech. 2011, 49, 987–1001. [Google Scholar]
- Franco, A. An apparatus for the routine measurement of thermal conductivity of materials for building application based on a transient hot-wire method. Appl. Therm. Eng. 2007, 27, 2495–2504. [Google Scholar] [CrossRef]
- Gustafsson, S.E. Transient plane source techniques for thermal-conductivity and thermal-diffusivity measurements of solid materials. Rev. Sci. Instrum. 1991, 62, 797–804. [Google Scholar] [CrossRef]
- Jiang, C.P.; Chen, F.L.; Yan, P.; Song, F. A four-phase confocal elliptical cylinder model for predicting the effective thermal conductivity of coated fibre composites. Philos. Mag. 2010, 90, 3601–3615. [Google Scholar] [CrossRef][Green Version]
- Sihn, S.; Roy, A.K. Micromechanical analysis for transverse thermal conductivity of composites. J. Compos. Mater. 2011, 45, 1245–1255. [Google Scholar] [CrossRef]
- Mierzwiczak, M.; Kolodziej, J.A. The inverse determination of the volume fraction of fibers in a unidirectionally reinforced composite for a given effective thermal conductivity. J. Mech. Mater. Struct. 2012, 7, 229–238. [Google Scholar] [CrossRef][Green Version]
- Mierzwiczak, M.; Kolodziej, J.A. The inverse determination of the thermal contact resistance components of unidirectionally reinforced composite. Inverse Probl. Sci. Eng. 2013, 21, 283–297. [Google Scholar] [CrossRef]
- Asif, M.; Tariq, A.; Singh, K.M. Estimation of thermal contact conductance using transient approach with inverse heat conduction problem. Heat Mass Transf. 2019, 55, 3243–3264. [Google Scholar] [CrossRef]
- Marcos-Gomez, D.; Ching-Lloyd, J.; Elizalde, M.R.; Clegg, W.J.; Molina-Aldareguia, J.M. Predicting the thermal conductivity of composite materials with imperfect interfaces. Compos. Sci. Technol. 2010, 70, 2276–2283. [Google Scholar] [CrossRef]
- Li, X.; Xing, L.X.; Zheng, K.L.; Wei, P.; Du, L.F.; Shen, M.W.; Shi, X.Y. Formation of Gold Nanostar-Coated Hollow Mesoporous Silica for Tumor Multimodality Imaging and Photothermal Therapy. ACS Appl. Mater. Interfaces 2017, 9, 5817–5827. [Google Scholar] [CrossRef] [PubMed]
- Gou, J.J.; Dai, Y.J.; Li, S.G.; Tao, W.Q. Numerical study of effective thermal conductivities of plain woven composites by unit cells of different sizes. Int. J. Heat Mass Transf. 2015, 91, 829–840. [Google Scholar] [CrossRef]
- Jiang, L.L.; Xu, G.D.; Cheng, S.; Lu, X.M.; Zeng, T. Predicting the thermal conductivity and temperature distribution in 3D braided composites. Compos. Struct. 2014, 108, 578–583. [Google Scholar] [CrossRef]
- Dong, K.; Zhang, J.J.; Cao, M.; Wang, M.L.; Gu, B.H.; Sun, B.Z. A mesoscale study of thermal expansion behaviors of epoxy resin and carbon fiber/epoxy unidirectional composites based on periodic temperature and displacement boundary conditions. Polym. Test 2016, 55, 44–60. [Google Scholar] [CrossRef]
- Ning, Q.G.; Chou, T.W. Closed-form solutions of the inpalne effective thermal-conductivities of woven-fabric composites. Compos. Sci. Technol. 1995, 55, 41–48. [Google Scholar] [CrossRef]
- Ning, Q.G.; Chou, T.W. A general analytical model for predicting the transverse effective thermal conductivities of woven fabric composites. Compos. Part A Appl. Sci. Manuf. 1998, 29, 315–322. [Google Scholar] [CrossRef]
- Tu, Z.C.; Mao, J.K.; Han, X.S.; He, Z.Z. Prediction Model for the Anisotropic Thermal Conductivity of a 2.5-D Braided Ceramic Matrix Composite with Thin-Wall Structure. Appl. Sci. Basel 2019, 9, 875. [Google Scholar] [CrossRef]
- Gou, J.J.; Fang, W.Z.; Dai, Y.J.; Li, S.G.; Tao, W.Q. Multi-size unit cells to predict effective thermal conductivities of 3D four-directional braided composites. Compos. Struct. 2017, 163, 152–167. [Google Scholar] [CrossRef]
- Liu, Y.; Qu, Z.G.; Guo, J.; Zhao, X.M. Numerical study on effective thermal conductivities of plain woven C/SiC composites with considering pores in interlaced woven yarns. Int. J. Heat Mass Transf. 2019, 140, 410–419. [Google Scholar] [CrossRef]
- Ming, X.L.; Chen, H.T.; Wang, D.H. Optimization of Processing Parameters to Increase Thermal Conductivity of Rice Straw Fiber Film. Appl. Sci. Basel 2019, 9, 4645. [Google Scholar] [CrossRef]
- Sun, Z.; Shan, Z.D.; Shao, T.M.; Zhang, Q. Numerical analysis of out-of-plane thermal conductivity of C/C composites by flexible oriented 3D weaving process considering voids and fiber volume fractions. J. Mater. Res. 2020, 35, 1888–1897. [Google Scholar] [CrossRef]
- Gou, J.J.; Ren, X.J.; Fang, W.Z.; Li, S.G.; Tao, W.Q. Two small unit cell models for prediction of thermal properties of 8-harness satin woven pierced composites. Compos. Part B Eng. 2018, 135, 218–231. [Google Scholar] [CrossRef]
- Li, H.Z.; Li, S.G.; Wang, Y.C. Prediction of effective thermal conductivities of woven fabric composites using unit cells at multiple length scales. J. Mater. Res. 2011, 26, 384–394. [Google Scholar] [CrossRef]
- Wei, K.L.; Li, J.; Shi, H.B.; Tang, M. Two-Scale Prediction of Effective Thermal Conductivity of 3D Braided C/C Composites Considering Void Defects by Asymptotic Homogenization Method. Appl. Compos. Mater. 2019, 26, 1367–1387. [Google Scholar] [CrossRef]
- Alghamdi, A.; Alharthi, H.; Alamoudi, A.; Alharthi, A.; Kensara, A.; Taylor, S. Effect of Needling Parameters and Manufacturing Porosities on the Effective Thermal Conductivity of a 3D Carbon-Carbon Composite. Materials 2019, 12, 13. [Google Scholar] [CrossRef] [PubMed]
- Lee, J.; Lee, H.I.; Paik, J.G. Thermal conductivities of a needle-punched carbon/carbon composite with unbalanced structures. Carbon Lett. 2020, 9. [Google Scholar] [CrossRef]
- Penide-Fernandez, R.; Sansoz, F. Anisotropic thermal conductivity under compression in two-dimensional woven ceramic fibers for flexible thermal protection systems. Int. J. Heat Mass Transf. 2019, 145, 11. [Google Scholar] [CrossRef]
- Islam, M.R.; Pramila, A. Thermal conductivity of fiber reinforced composites by the FEM. J. Compos. Mater. 1999, 33, 1699–1715. [Google Scholar] [CrossRef]
- Li, S. General unit cells for micromechanical analyses of unidirectional composites. Composites Part A Appl. Sci. Manuf. 2001, 32, 815–826. [Google Scholar] [CrossRef]
- Li, S.; Reid, S.R. On the symmetry conditions for laminated fiber-reinforced composite structures. Int. J. Solids Struct. 1992, 29, 2867–2880. [Google Scholar] [CrossRef]
- Zhu, X.; Han, L.; Yang, F.; Jiang, J.; Jia, X.L. Lightweight mesoporous carbon fibers with interconnected graphitic walls for supports of form-stable phase change materials with enhanced thermal conductivity. Sol. Energy Mater. Sol. Cells 2020, 208, 11. [Google Scholar] [CrossRef]
- Tanov, R.; Tabiei, A. Computationally efficient micromechanical models for woven fabric composite elastic moduli. J. Appl. Mech. Trans. ASME 2001, 68, 553–560. [Google Scholar] [CrossRef]
- Lu, J.; Hao, K.; Liu, L.; Li, H.; Li, K.; Qu, J.; Yan, X. Ablation resistance of SiC-HfC-ZrC multiphase modified carbon/carbon composites. Corros. Sci. 2016, 103, 1–9. [Google Scholar] [CrossRef]
- Li, X.; Xiong, Z.G.; Xu, X.Y.; Luo, Y.; Peng, C.; Shen, M.W.; Shi, X.Y. Tc-99m-Labeled Multifunctional Low-Generation Dendrimer-Entrapped Gold Nanoparticles for Targeted SPECT/CT Dual-Mode Imaging of Tumors. ACS Appl. Mater. Interfaces 2016, 8, 19883–19891. [Google Scholar] [CrossRef] [PubMed]
- Li, S.; Zhou, C.; Yu, H.; Li, L. Formulation of a unit cell of a reduced size for plain weave textile composites. Comput. Mater. Sci. 2011, 50, 1770–1780. [Google Scholar] [CrossRef]
- Xia, Z.H.; Zhang, Y.F.; Ellyin, F. A unified periodical boundary conditions for representative volume elements of composites and applications. Int. J. Solids Struct. 2003, 40, 1907–1921. [Google Scholar] [CrossRef]
- Gou, J.-J.; Zhang, H.; Dai, Y.-J.; Li, S.; Tao, W.-Q. Numerical prediction of effective thermal conductivities of 3D four-directional braided composites. Compos. Struct. 2015, 125, 499–508. [Google Scholar] [CrossRef]
- Li, X.; Lu, S.Y.; Xiong, Z.G.; Hu, Y.; Ma, D.; Lou, W.Q.; Peng, C.; Shen, M.W.; Shi, X.Y. Light-Addressable Nanoclusters of Ultrasmall Iron Oxide Nanoparticles for Enhanced and Dynamic Magnetic Resonance Imaging of Arthritis. Adv. Sci. 2019, 6, 9. [Google Scholar] [CrossRef] [PubMed]
- Grujicic, M.; Zhao, C.L.; Dusel, E.C.; Morgan, D.R.; Miller, R.S.; Beasley, D.E. Computational analysis of the thermal conductivity of the carbon-carbon composite materials. J. Mater. Sci. 2006, 41, 8244–8256. [Google Scholar] [CrossRef]
- Dong, K.; Zhang, J.; Jin, L.; Gu, B.; Sun, B. Multi-scale finite element analyses on the thermal conductive behaviors of 3D braided composites. Compos. Struct. 2016, 143, 9–22. [Google Scholar] [CrossRef]
- Charles, J.; Wilson, D. A model of passive thermal nondestructive evaluation of composite laminates. Polym. Compos. 1981, 2, 105–111. [Google Scholar] [CrossRef]
- Pilling, M.; Yates, B.; Black, M.; Tattersall, P. The thermal conductivity of carbon fibre-reinforced composites. J. Mater. Sci. 1979, 14, 1326–1338. [Google Scholar] [CrossRef]
- Zhou, L.C.; Sun, X.H.; Chen, M.W.; Zhu, Y.B.; Wu, H.G. Multiscale modeling and theoretical prediction for the thermal conductivity of porous plain-woven carbonized silica/phenolic composites. Compos. Struct. 2019, 215, 278–288. [Google Scholar] [CrossRef]





| Material/Heat Treating Temperature | Thermal Conductivity/(W∙m−1∙K−1) | ||
|---|---|---|---|
| Transverse | Circumferential | Longitudinal | |
| T300 fiber/PAN 2673 K | 0.76 | 0.76 | 76 |
| Resin based Matrix 2673 K | 64.3 | 64.3 | 64.3 |
| Pitch based Matrix 2673 K | 0.64 | 64.3 | 257 |
| Disordered Graphite | 110 | 110 | 110 |
| Model | Fiber Bundle/(W∙m−1∙K−1) | Fiber Rod/(W∙m−1∙K−1) | ||
|---|---|---|---|---|
| Longitudinal Thermal Conductivity | Transverse Thermal Conductivity | Longitudinal Thermal Conductivity | Transverse Thermal Conductivity | |
| Finite element analysis | 149.24 | 11.33 | 73.74 | 3.89 |
| Parallel Model | 153.82 | 73.66 | ||
| Series Model | 1.32 | 0.95 | ||
| Charles model | 0.29 | 0.12 | ||
| Pilling Model | 13.08 | 3.88 | ||
| Maxwell Model | 18.14 | 73.74 | 7.71 | |
| Diameter of the Fiber Rods | The Size of Unit Cell | |
|---|---|---|
| The Number of Elements of UC1 | The Number of Elements of UC2 | |
| 1.2 mm | 734,448 | 184,276 |
| 1.4 mm | 841,896 | 211,464 |
| 1.6 mm | 971,520 | 243,672 |
| 1.8 mm | 1,112,496 | 273,240 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xu, C.; Sun, Z.; Shao, G. Prediction of Effective Thermal Conductivities of Four-Directional Carbon/Carbon Composites by Unit Cells with Different Sizes. Appl. Sci. 2021, 11, 1171. https://doi.org/10.3390/app11031171
Xu C, Sun Z, Shao G. Prediction of Effective Thermal Conductivities of Four-Directional Carbon/Carbon Composites by Unit Cells with Different Sizes. Applied Sciences. 2021; 11(3):1171. https://doi.org/10.3390/app11031171
Chicago/Turabian StyleXu, Chang, Zhihong Sun, and Guowei Shao. 2021. "Prediction of Effective Thermal Conductivities of Four-Directional Carbon/Carbon Composites by Unit Cells with Different Sizes" Applied Sciences 11, no. 3: 1171. https://doi.org/10.3390/app11031171
APA StyleXu, C., Sun, Z., & Shao, G. (2021). Prediction of Effective Thermal Conductivities of Four-Directional Carbon/Carbon Composites by Unit Cells with Different Sizes. Applied Sciences, 11(3), 1171. https://doi.org/10.3390/app11031171