Magnetically-Induced Pressure Generation in Magnetorheological Fluids under the Influence of Magnetic Fields
Abstract
1. Introduction
2. Materials and Methods
3. Results
3.1. Simulation Results
3.2. Experiment Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| AWG | American Wire Gauge |
| DC | Direct Current |
| FEMM | Finite Element Method Magnetics |
| MCU | Microcontroller Unit |
| MRFs | Magnetorheological Fluids |
| PVC | Polyvinyl Chloride |
References
- Utami, D.; Ubaidillah; Mazlan, S.A.; Imaduddin, F.; Nordin, N.A.; Bahiuddin, I.; Abdul Aziz, S.A.; Mohamad, N.; Choi, S.-B. Material Characterization of a Magnetorheological Fluid Subjected to Long-Term Operation in Damper. Materials 2018, 11, 2195. [Google Scholar] [CrossRef]
- Imaduddin, F.; Mazlan, S.; Zamzuri, H. A design and modelling review of rotary magnetorheological damper. Mater. Des. 2013, 51, 575–591. [Google Scholar] [CrossRef]
- Bahiuddin, I.; Mazlan, S.A.; Shapiai, M.I.; Choi, S.-B.; Imaduddin, F.; Rahman, M.A.A.; Ariff, M.H.M. A new constitutive model of a magneto-rheological fluid actuator using an extreme learning machine method. Sens. Actuators A Phys. 2018, 281, 209–221. [Google Scholar] [CrossRef]
- Ubaidillah; Hudha, K.; Akmar, F.; Kadir, A. Modelling, characterisation and force tracking control of a magnetorheological damper under harmonic excitation. Int. J. Model. Identif. Control 2011, 13, 9–21. [Google Scholar] [CrossRef]
- Ubaidillah; Lenggana, B.; Son, L.; Imaduddin, F.; Widodo, P.; Harjana, H.; Doewes, R. A new magnetorheological fluids damper for unmanned aerial vehicles. J. Adv. Res. Fluid Mech. Therm. 2020, 73, 35–45. [Google Scholar] [CrossRef]
- Imaduddin, F.; Mazlan, S.A.; Ubaidillah; Idris, M.H.; Bahiuddin, I. Characterization and modeling of a new magnetorheological damper with meandering type valve using neuro-fuzzy. J. King Saud Univ.-Sci. 2017, 29, 468–477. [Google Scholar] [CrossRef]
- Gadekar, P.; Kanthale, V.S.; Khaire, N.D. Magnetorheological Fluid and its Applications. Int. J. Curr. Eng. Technol. 2017, 7, 32–37. [Google Scholar]
- Idris, M.H.; Imaduddin, F.; Ubaidillah; Mazlan, S.A.; Choi, S.-B. A concentric design of a bypass magnetorheological fluid damper with a serpentine flux valve. Actuators 2020, 9, 16. [Google Scholar] [CrossRef]
- Milecki, A.; Hauke, M. Application of magnetorheological fluid in industrial shock absorbers. Mech. Syst. Signal Process. 2012, 28, 528–541. [Google Scholar] [CrossRef]
- Hu, G.; Wu, L.; Li, L. Torque characteristics analysis of a magnetorheological brake with double brake disc. Actuators 2021, 10, 23. [Google Scholar] [CrossRef]
- Nya’Ubit, I.R.; Priyandoko, G.; Imaduddin, F.; Adiputra, D.; Ubaidillah. Torque characterization of t-shaped magnetorheological brake featuring serpentine magnetic flux. J. Adv. Res. Fluid Mech. Therm. 2020, 78, 85–97. [Google Scholar]
- Latha, H.; Pantangi, U.S.; Seetharamaiah, N. Design and manufacturing aspects of magneto-rheological fluid (mrf) clutch. Mater. Today Proc. 2017, 4, 1525–1534. [Google Scholar] [CrossRef]
- Jamalpour, R.; Nekooei, M.; Moghadam, A. Seismic response reduction of steel mrf using sma equipped innovated low-damage column foundation connection. Civ. Eng. J. 2017, 3, 1–14. [Google Scholar] [CrossRef]
- Dyke, S.J.; Spencer, B.F.; Sain, M.K.; Carlson, J.D. Seismic Response Reduction Using Magnetorheological Dampers. IFAC Proc. Vol. 1996, 29, 5530–5535. [Google Scholar] [CrossRef]
- Satria, R.R.; Ubaidillah, U.; Imaduddin, F. Analytical approach of a pure flow mode serpentine path rotary magnetorheological damper. Actuators 2020, 9, 56. [Google Scholar] [CrossRef]
- Abd Fatah, A.Y.; Mazlan, S.; Koga, T.; Zamzuri, H.; Imaduddin, F. Design of magnetorheological valve using serpentine flux path method. Int. J. Appl. Electromagn. Mech. 2016, 50, 29–44. [Google Scholar] [CrossRef]
- Sarkar, C.; Hirani, H.; Sasane, A. Magnetorheological smart automotive engine mount. Int. J. Curr. Eng. Technol. 2015, 5, 419–428. [Google Scholar]
- Zeinali, M.; Mazlan, S.A.; Choi, S.-B.; Imaduddin, F.; Hamdan, L.H. Influence of piston and magnetic coils on the field-dependent damping performance of a mixed-mode magnetorheological damper. Smart Mater. Struct. 2016, 25, 055010. [Google Scholar] [CrossRef]
- Sapinski, B. Simulation of an MR squeeze-mode damper for an automotive engine mount. In Proceedings of the 17th International Carpathian Control Conference (ICCC), IEEExplore, High Tatras, Slovakia, 29 May–1 June 2016; pp. 641–644. [Google Scholar]
- Yildirim, G.; Genc, S. Experimental study on heat transfer of the magnetorheological fluids. Smart Mater. Struct. 2013, 22, 085001. [Google Scholar] [CrossRef]
- Adiputra, D.; Rahman, M.A.A.; Bahiuddin, I.; Ubaidillah; Imaduddin, F.; Nazmi, N. Sensor number optimization using neural network for ankle foot orthosis equipped with magnetorheological brake. Open Eng. 2021, 11, 91–101. [Google Scholar] [CrossRef]
- Bhavsar, P.; Unune, D.R. Magnetorheological Polishing Tool for Nano-Finishing of Biomaterials. In Proceedings of the 10th International Conference on Precesion, Meso, Micro and Nano Engineering, Chennai, India, 7–9 December 2017; pp. 6–10. [Google Scholar]
- Purnomo, E.D.; Ubaidillah; Imaduddin, F.; Yahya, I.; Mazlan, S. Preliminary experimental evaluation of a novel loudspeaker featuring magnetorheological fluid surround absorber. Indones. J. Electr. Eng. Comput. 2020, 17, 922–928. [Google Scholar] [CrossRef]
- Dyana Kumara, K.A.; Ubaidillah, U.; Priyandoko, I.Y.G.; Wibowo, W. Magnetostatic Simulation in a Novel Magnetorheological Elastomer Based Loudspeaker Surround. In Proceedings of the 2019 6th International Conference on Electric Vehicular Technology (ICEVT), Bali, Indonesia, 18–21 November 2019; pp. 295–299. [Google Scholar]
- Ahamed, R.; Choi, S.-B.; Ferdaus, M.M. A state of art on magneto-rheological materials and their potential applications. J. Intell. Mater. Syst. Struct. 2018, 29, 2051–2095. [Google Scholar] [CrossRef]
- Rahman, M.; Chao, O.; Julai, S.; Ferdaus, M.M.; Ahamed, R. A review of advances in magnetorheological dampers: Their design optimization and applications. J. Zhejiang Univ. Sci. A 2017, 18, 991–1010. [Google Scholar] [CrossRef]
- de Vicente, J.; Klingenberg, D.J.; Hidalgo-Alvarez, R. Magnetorheological fluids: A review. Soft Matter 2011, 7, 3701–3710. [Google Scholar] [CrossRef]
- Ostadfar, A. Chapter 1—Fluid Mechanics and Biofluids Principles. In Biofluid Mechanics; Ostadfar, A., Ed.; Academic Press: London, UK, 2016; pp. 1–60. [Google Scholar]
- Vijayaraghavan, G.K.; Sundaravalli, S. Pressure and Pressure Measuring Devices. In Fluid Mechanics and Machinery; Lakshmi Publications: Chennai, India, 2018; ISBN 978-93-831030-8-9. [Google Scholar]
- Ubaidillah; Wirawan, J.W.; Lenggana, B.W.; Purnomo, E.D.; Widyarso, W.; Mazlan, S.A. Design and Performance Analysis of Magnetorheological Valve for Upside-Down Damper. J. Adv. Res. Fluid Mech. Therm. 2019, 63, 164–173. [Google Scholar]
- Yatchev, I.; Gueorgiev, V.; Ivanov, R.; Hinov, K. Simulation of the dynamic behaviour of a permanent magnet linear actuator. Facta Univ.-Ser. Electron. Energy 2010, 23, 37–43. [Google Scholar] [CrossRef][Green Version]
- Białek, M.; Nowak, P.; Lindner, T.; Wyrwał, D. FEMM Examination of the Gripper with Magnetorheological Cushion. In Proceedings of the 2020 International Conference Mechatronic Systems and Materials (MSM), Bialystok, Poland, 1–3 July 2020; pp. 1–5. [Google Scholar]
- Ubaidillah; Lenggana, B.W. Finite Element Magnetic Method for Magnetorheological Based Actuators. In Finite Element Methods and Their Applications; IntechOpen: London, UK, 2020; pp. 1–18. [Google Scholar] [CrossRef]
- Freescale Semiconductors Inc. Technical Data Sheet: MPXV5010DP, 0 to 10 kPa, Differential, Gauge, and Absolute, Integrated, Pressure Sensors; Freescale Semiconductors Inc.: Austin, TX, USA, 2012. [Google Scholar]
- Volpato, O. Thermal Modeling of Heated Injectors; SAE Technical Papers 2011-36-0087; SAE: Warrendale, PA, USA, 2011. [Google Scholar] [CrossRef]
- Arafat, M.M.; Dinan, B.; Akbar, S.A.; Haseeb, A.S.M.A. Gas sensors based on one dimensional nanostructured metal-oxides: A review. Sensors 2012, 12, 7207–7258. [Google Scholar] [CrossRef]

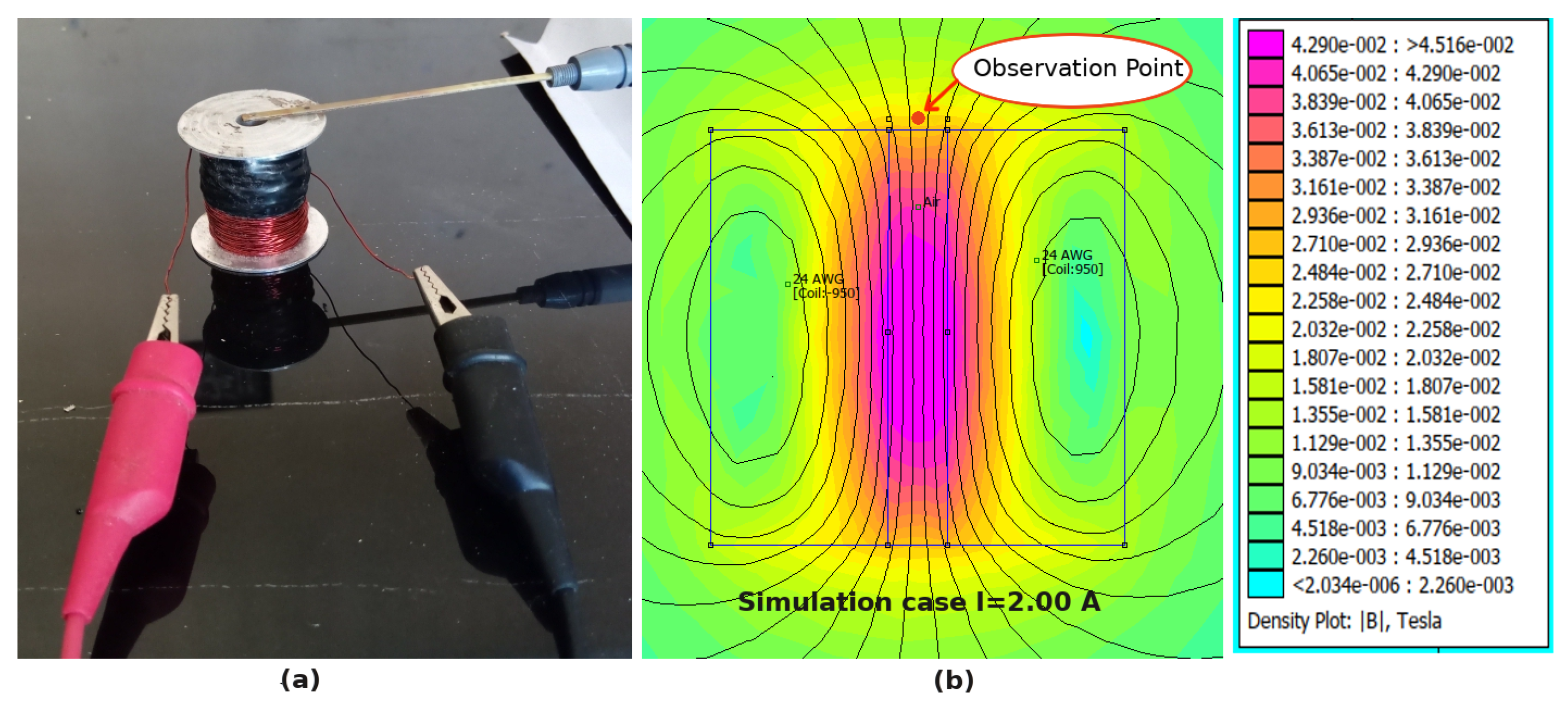
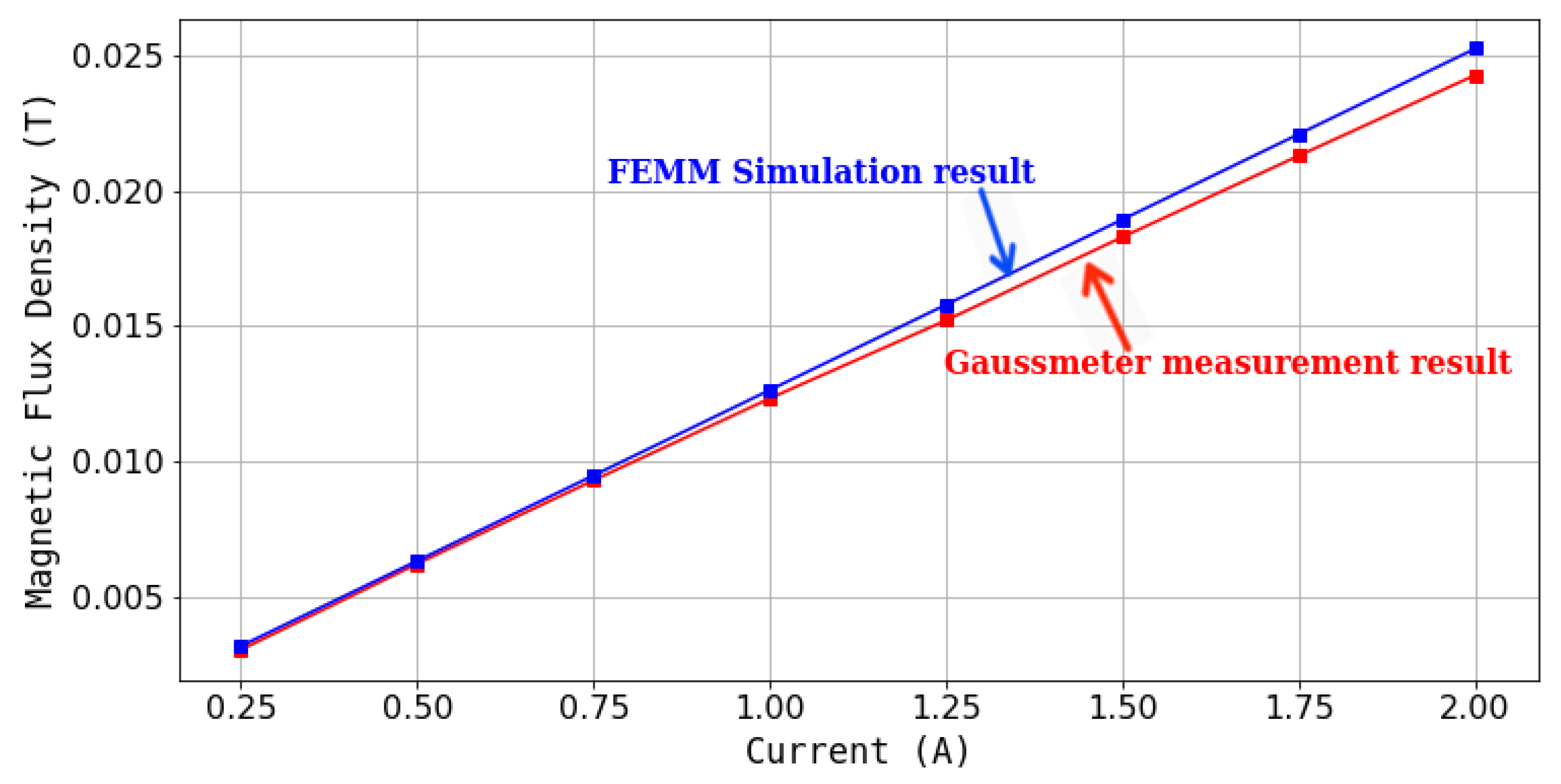

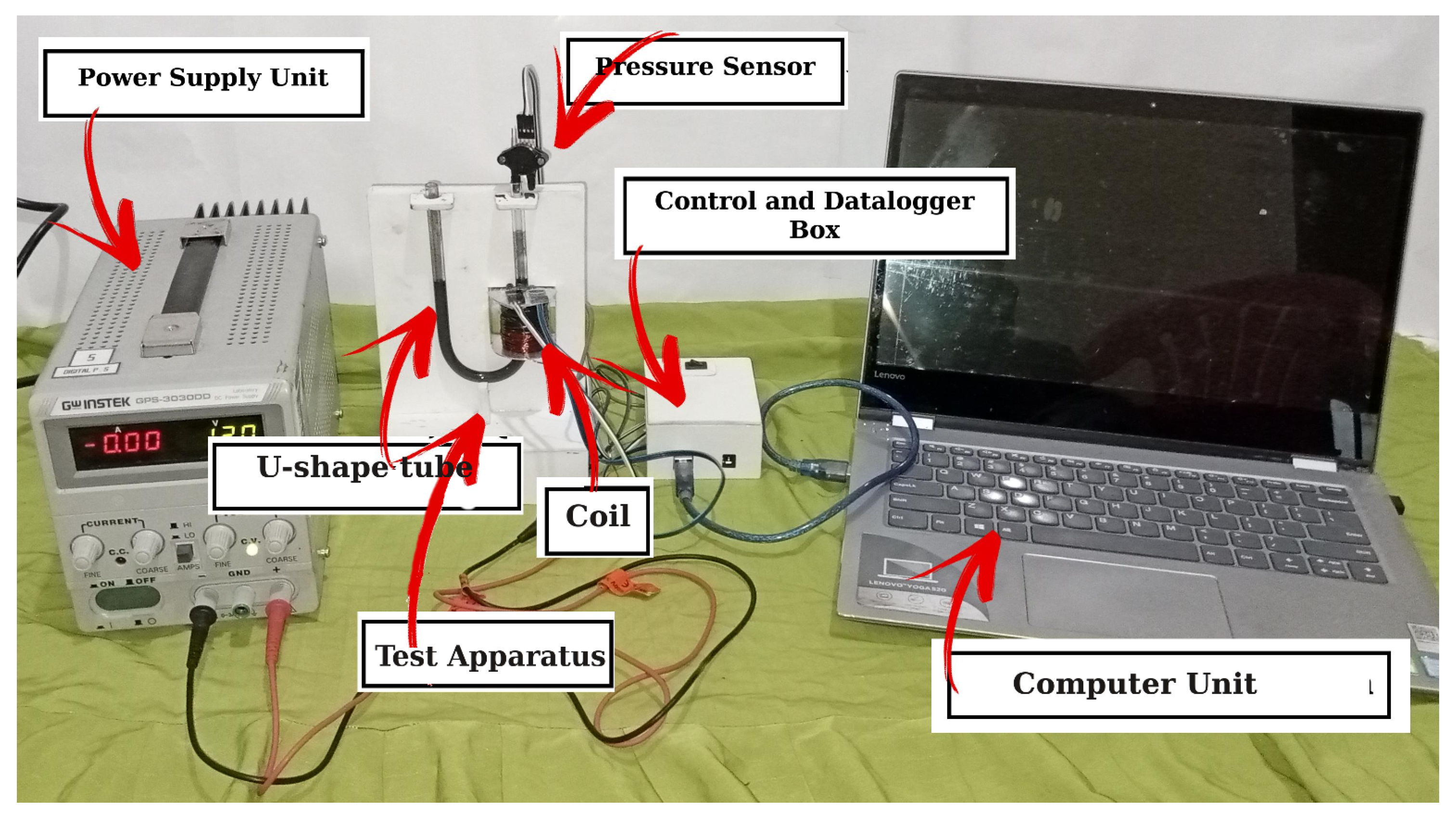
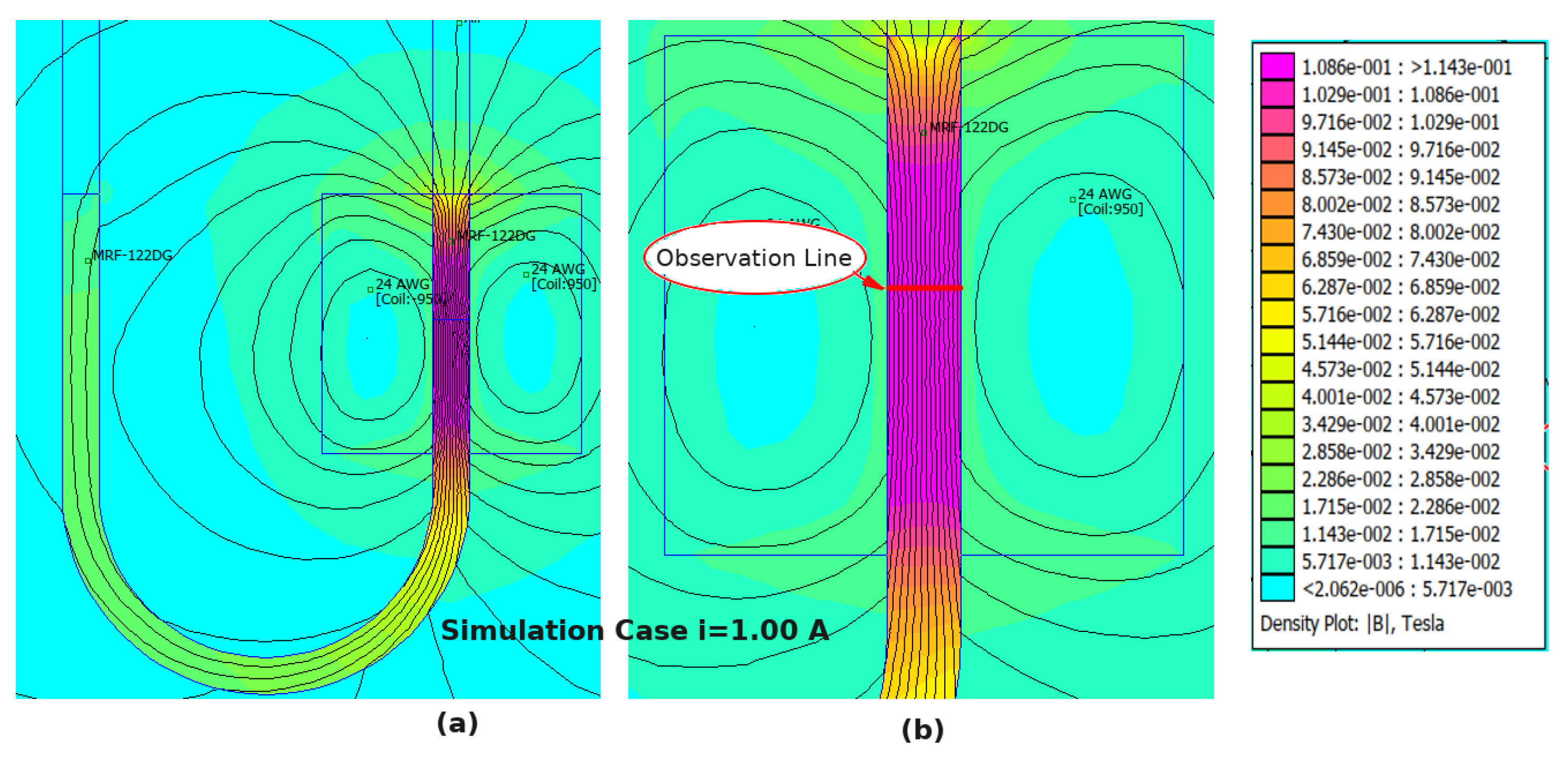
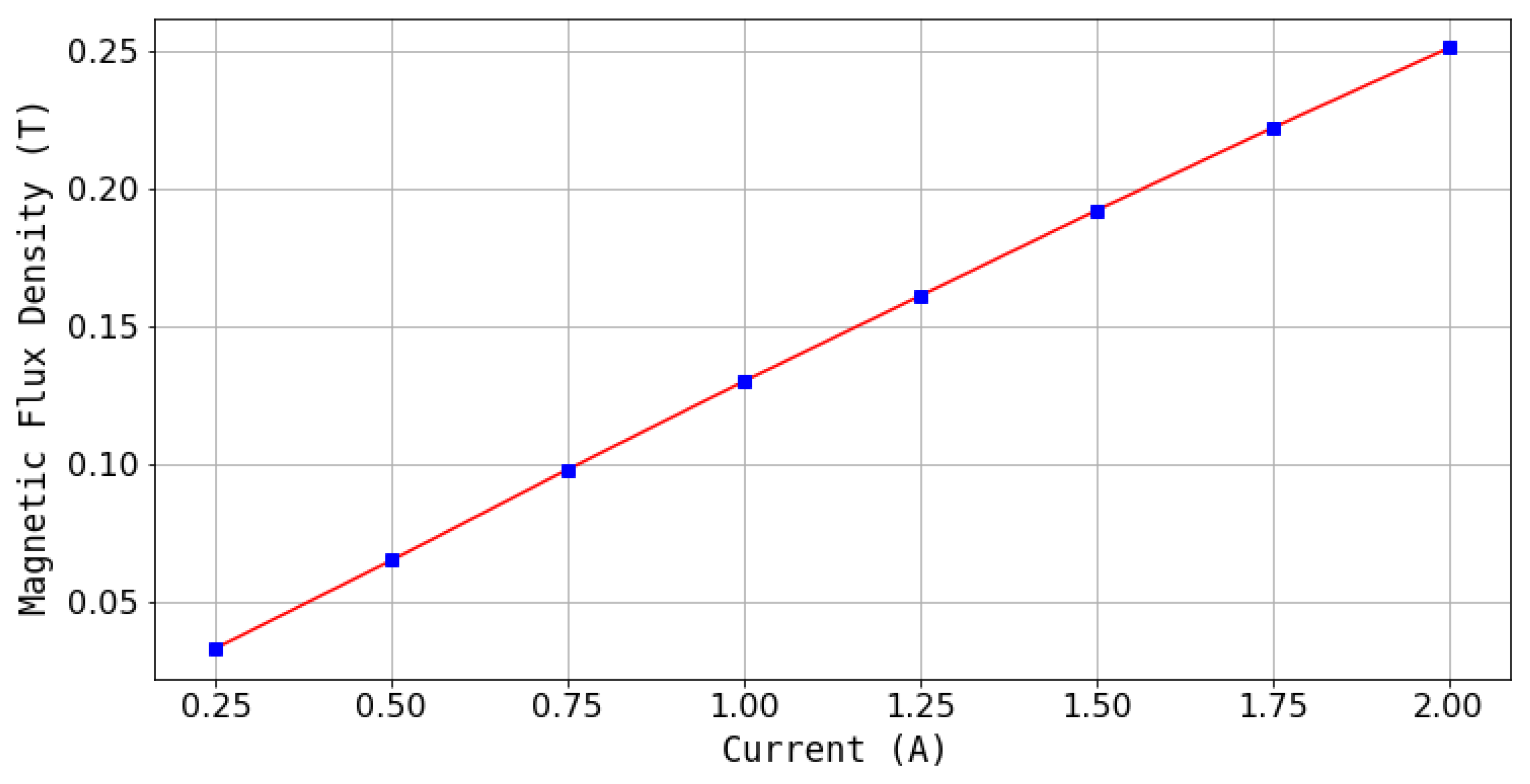

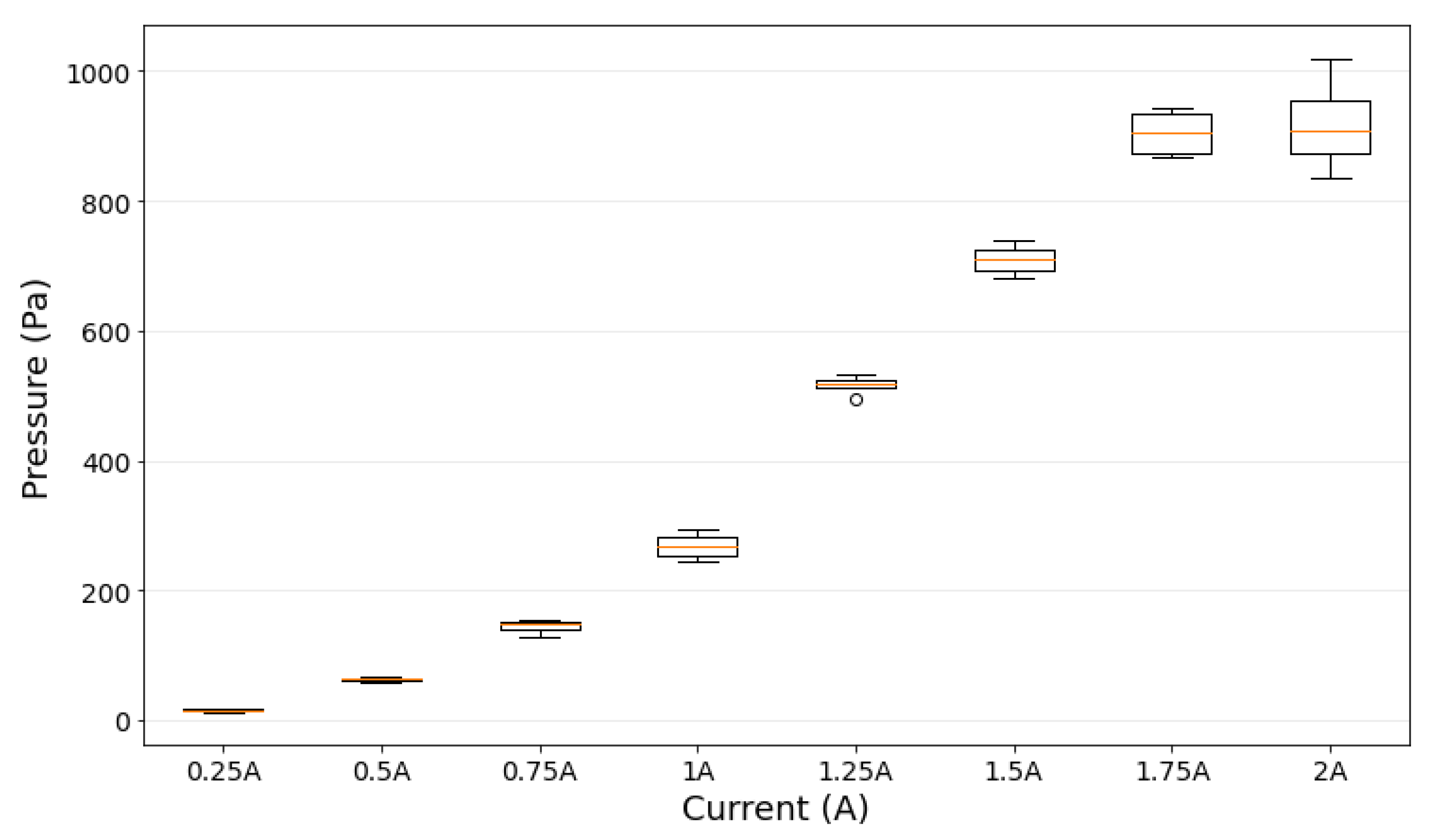

| Current Input (A) | 0.25 | 0.50 | 0.75 | 1.00 | 1.25 | 1.50 | 1.75 | 2.00 |
|---|---|---|---|---|---|---|---|---|
| ∆Pmin (Pa) | 13.06 | 58.98 | 129.17 | 244.49 | 496.04 | 679.79 | 867.36 | 835.50 |
| ∆Pmax (Pa) | 18.39 | 65.91 | 155.64 | 292.53 | 533.77 | 739.02 | 942.50 | 1020.01 |
| ∆Pavg (Pa) | 15.53 | 62.80 | 144.77 | 267.80 | 517.16 | 709.20 | 904.64 | 918.55 |
| ∆Pmedian (Pa) | 15.34 | 63.16 | 147.14 | 267.09 | 519.42 | 708.99 | 904.34 | 909.34 |
| Std Deviation | 2.68 | 3.12 | 11.52 | 21.29 | 15.63 | 25.85 | 38.37 | 78.84 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Widodo, P.J.; Budiana, E.P.; Ubaidillah, U.; Imaduddin, F. Magnetically-Induced Pressure Generation in Magnetorheological Fluids under the Influence of Magnetic Fields. Appl. Sci. 2021, 11, 9807. https://doi.org/10.3390/app11219807
Widodo PJ, Budiana EP, Ubaidillah U, Imaduddin F. Magnetically-Induced Pressure Generation in Magnetorheological Fluids under the Influence of Magnetic Fields. Applied Sciences. 2021; 11(21):9807. https://doi.org/10.3390/app11219807
Chicago/Turabian StyleWidodo, Purwadi Joko, Eko Prasetya Budiana, Ubaidillah Ubaidillah, and Fitrian Imaduddin. 2021. "Magnetically-Induced Pressure Generation in Magnetorheological Fluids under the Influence of Magnetic Fields" Applied Sciences 11, no. 21: 9807. https://doi.org/10.3390/app11219807
APA StyleWidodo, P. J., Budiana, E. P., Ubaidillah, U., & Imaduddin, F. (2021). Magnetically-Induced Pressure Generation in Magnetorheological Fluids under the Influence of Magnetic Fields. Applied Sciences, 11(21), 9807. https://doi.org/10.3390/app11219807







